Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation
Abstract
:1. Introduction
2. Theoretical Background
2.1. Magnetorheological Fluid
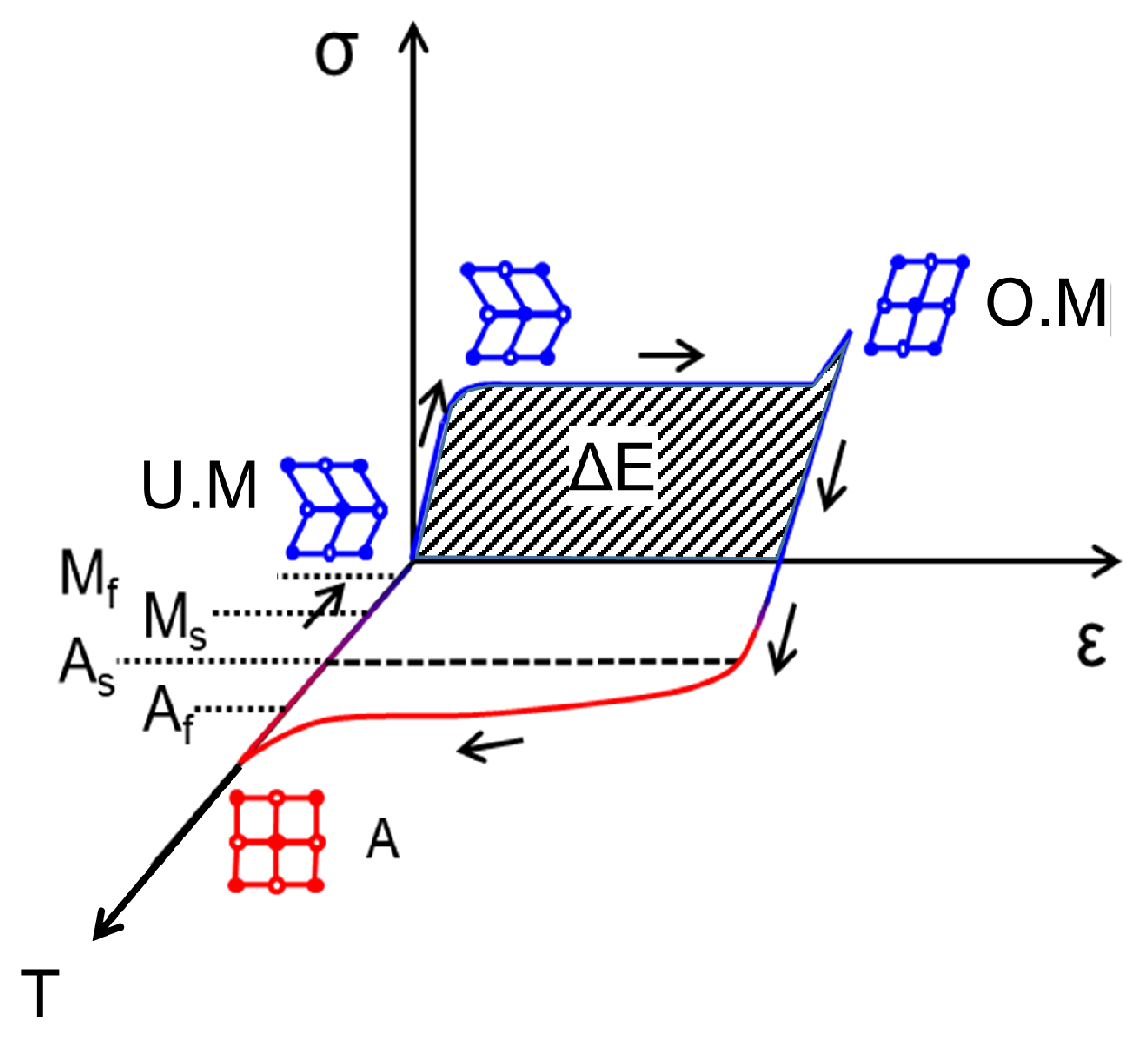
2.2. Shape Memory Alloy (SMA)
3. Design of the Hybrid Damper
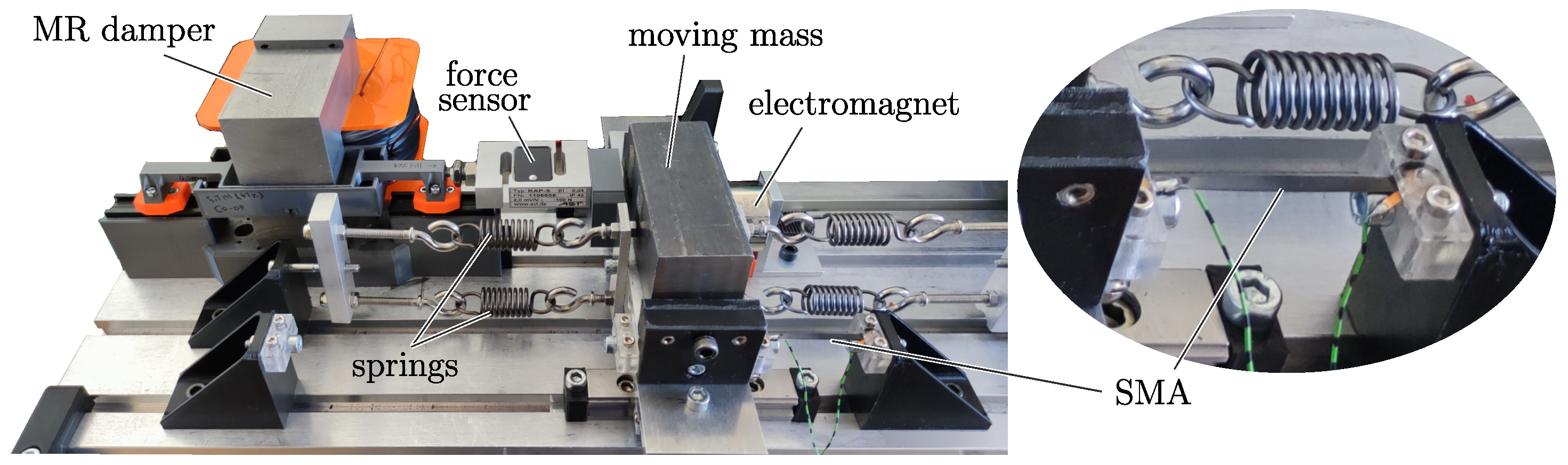
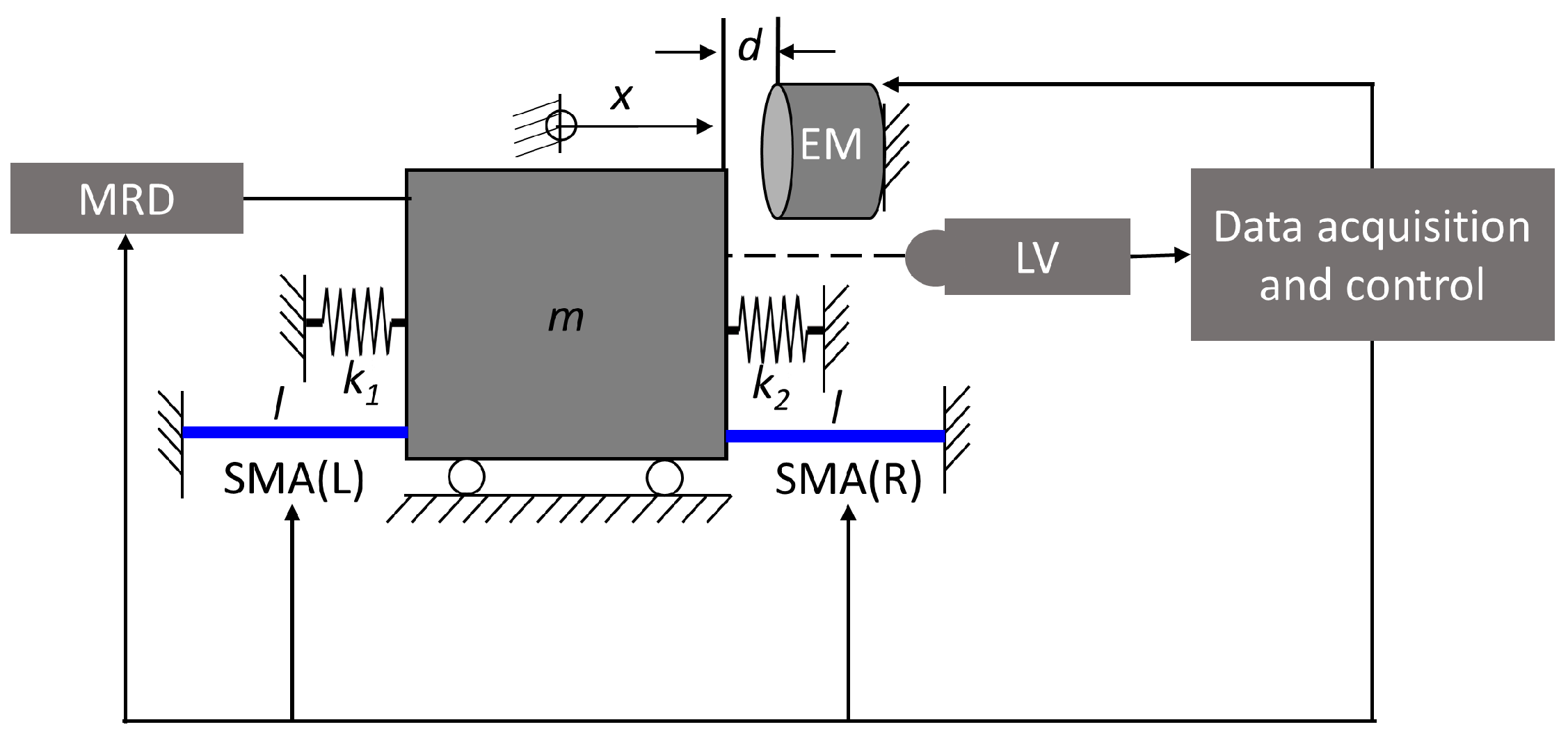
3.1. Experimental Setup Configuration
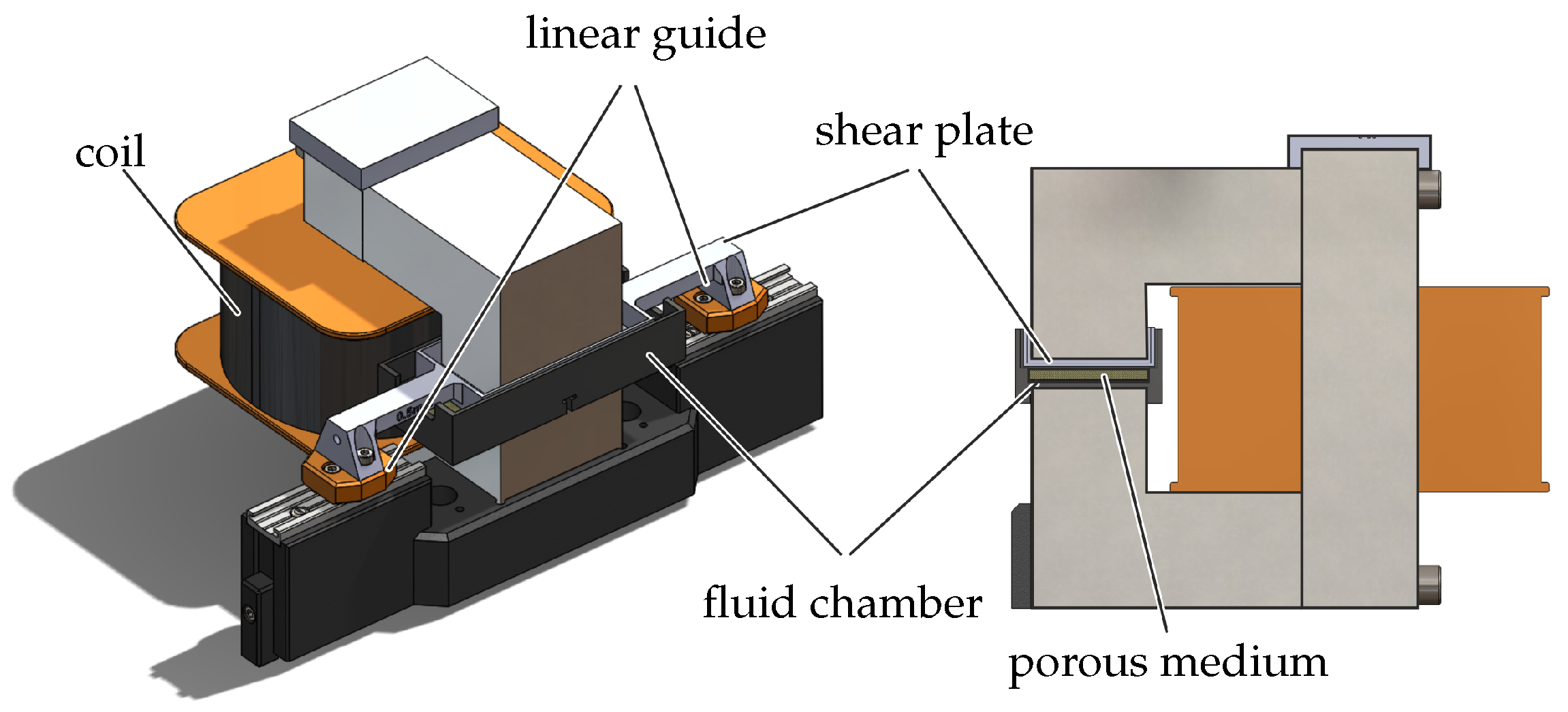
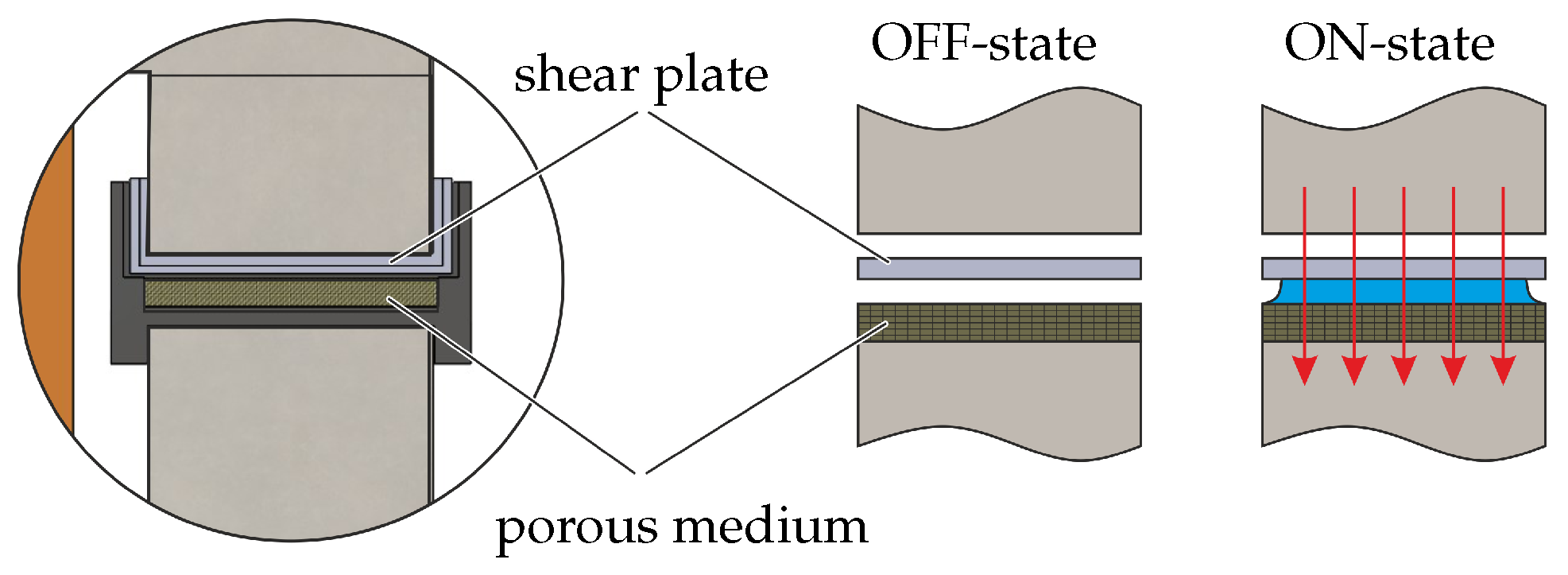
3.2. Magnetorheological Fluid-Based Damper
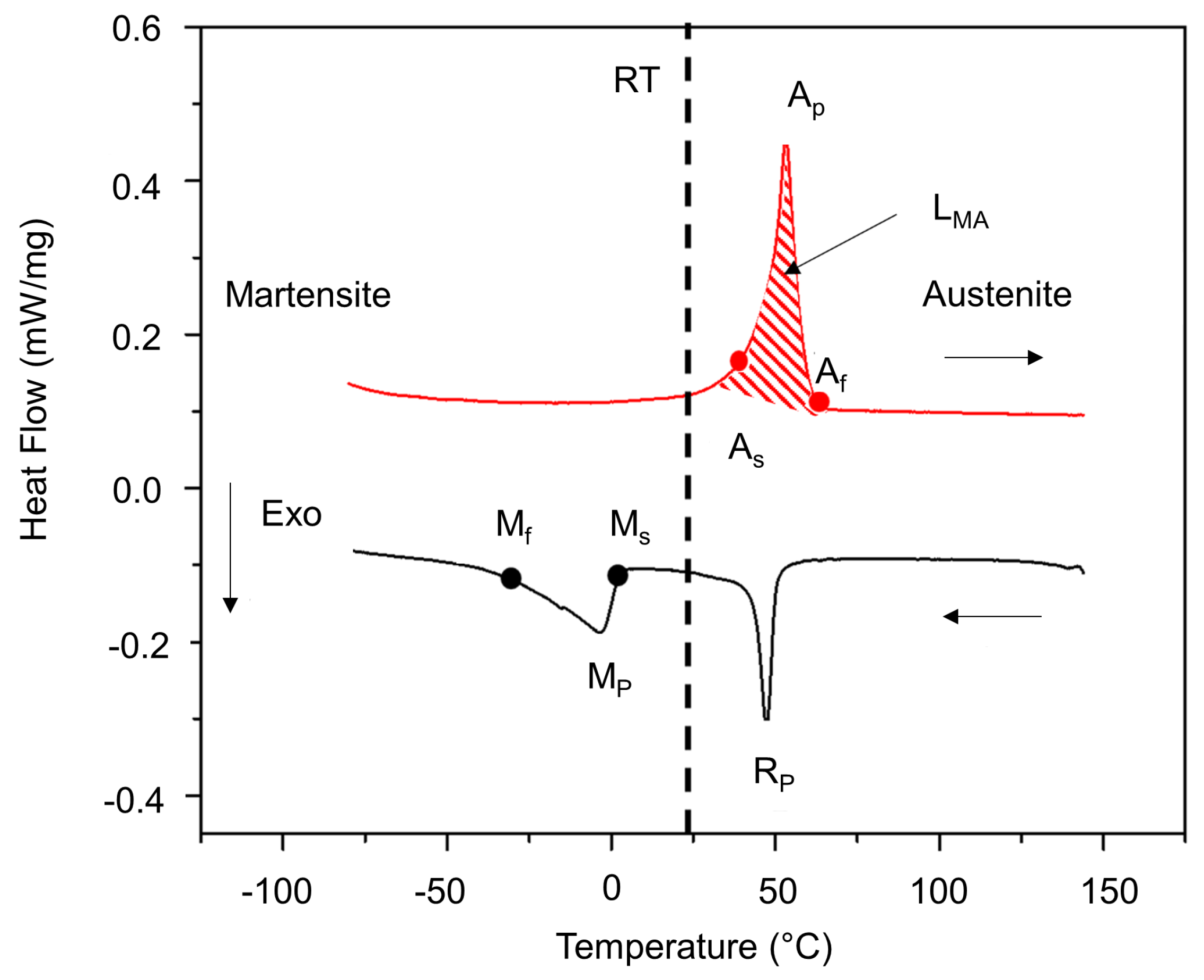
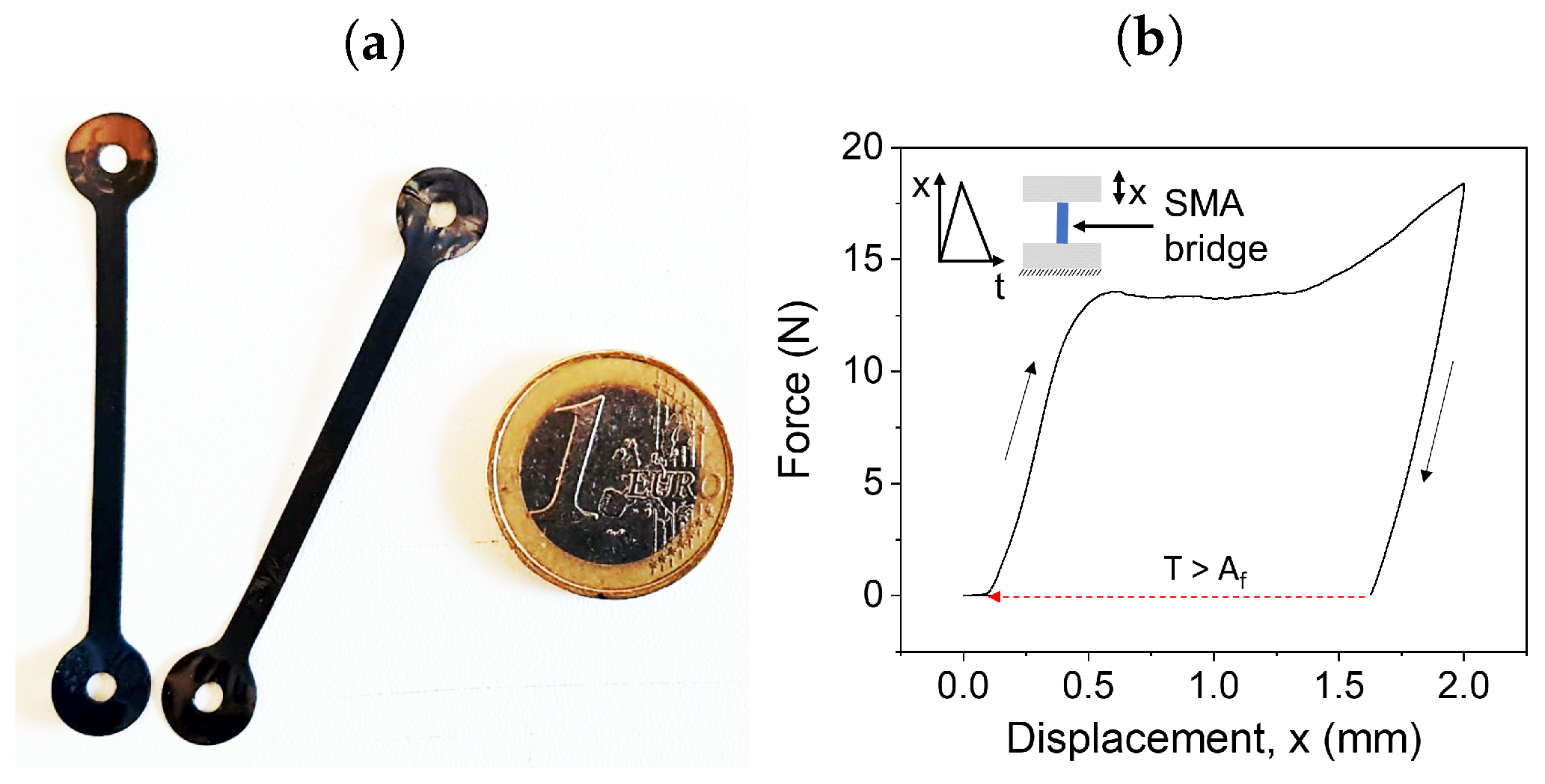
3.3. Shape Memory Alloy
4. Experiments and Measurement Results
4.1. Experimental Procedure
4.2. Identification of Best Damper Configuration
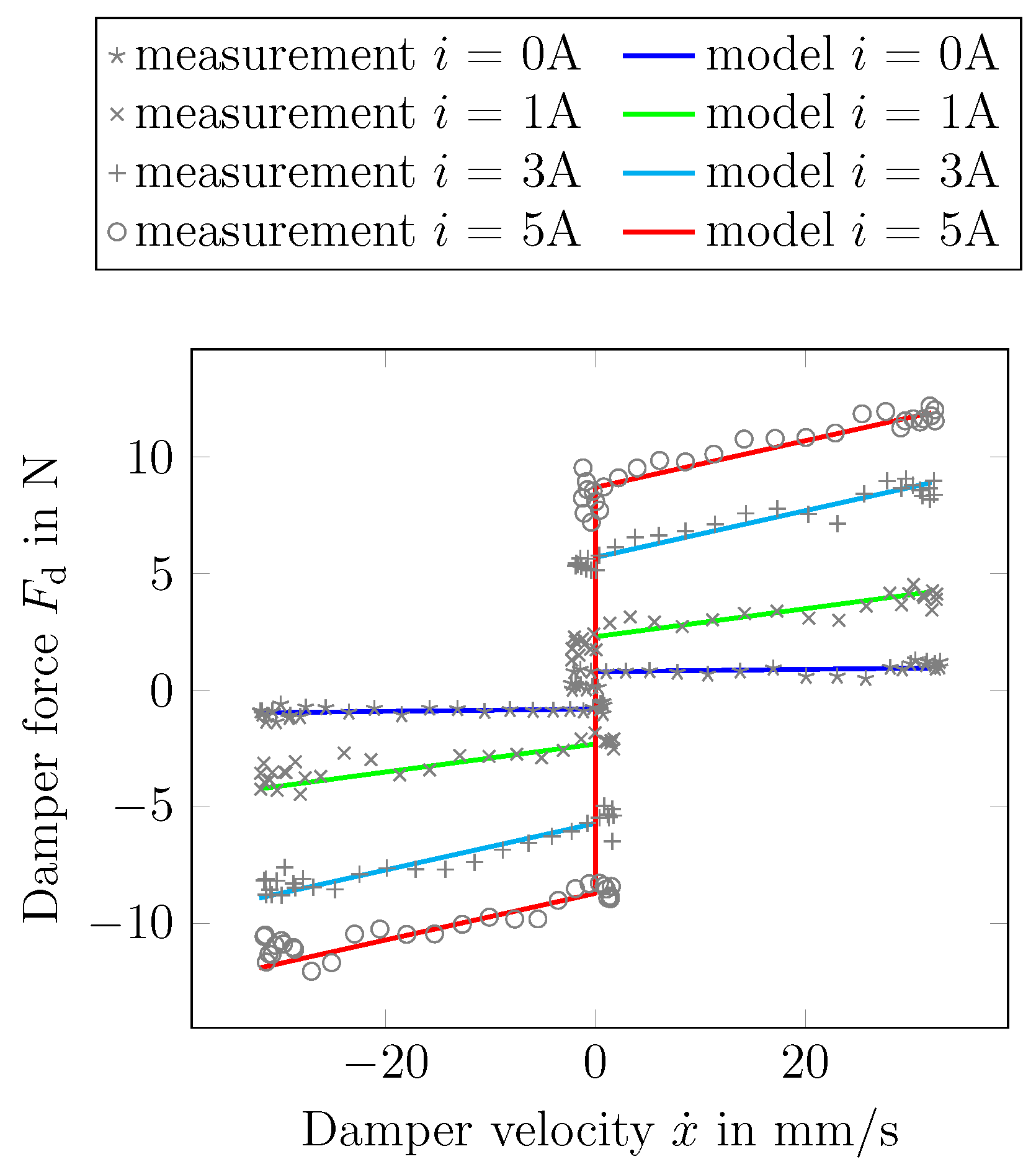
4.2.1. Magnetorheological Damper
4.2.2. SMA Damper
- Heating Pulse:
- SMA Position:
- Identification of Best Operating Conditions:
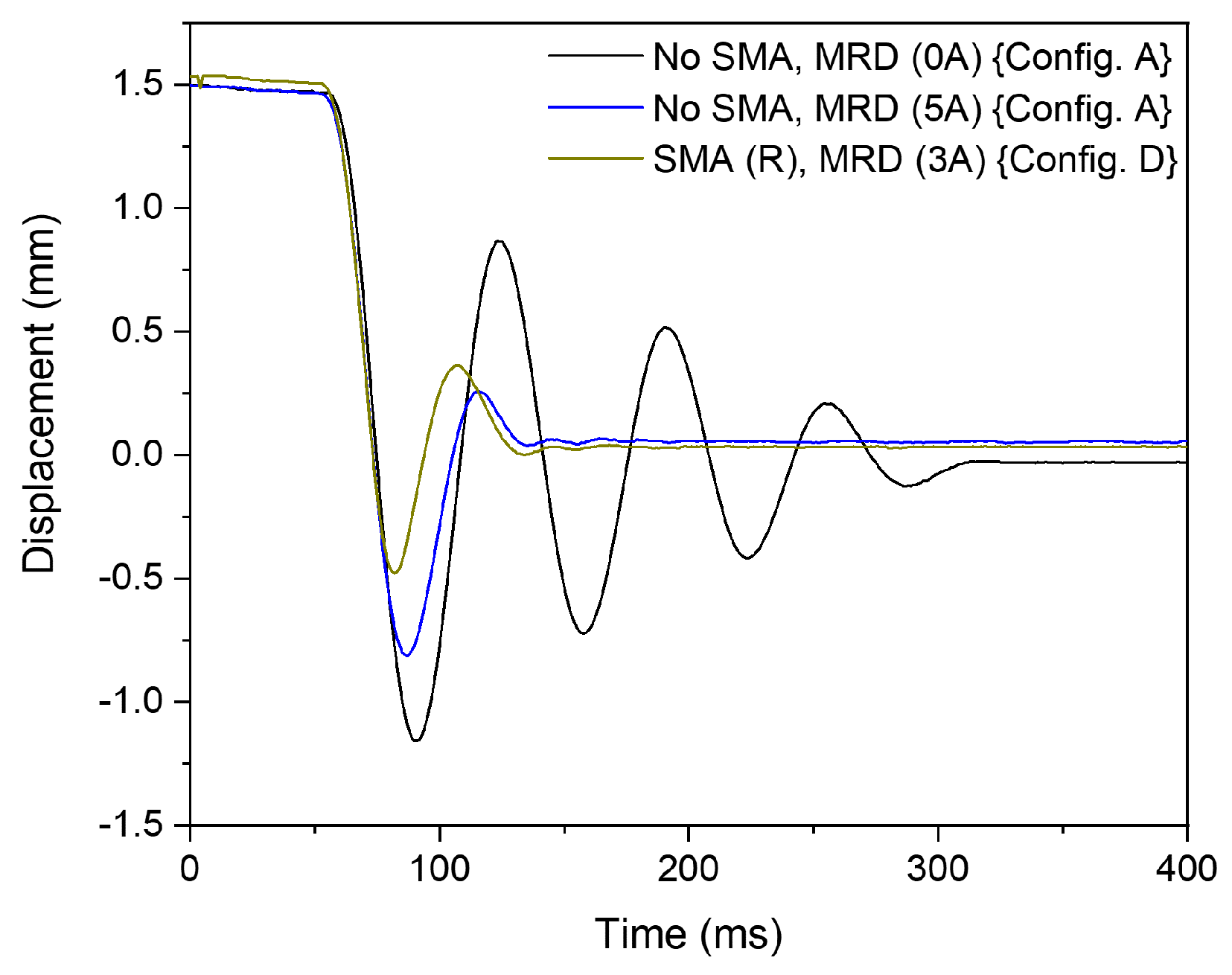
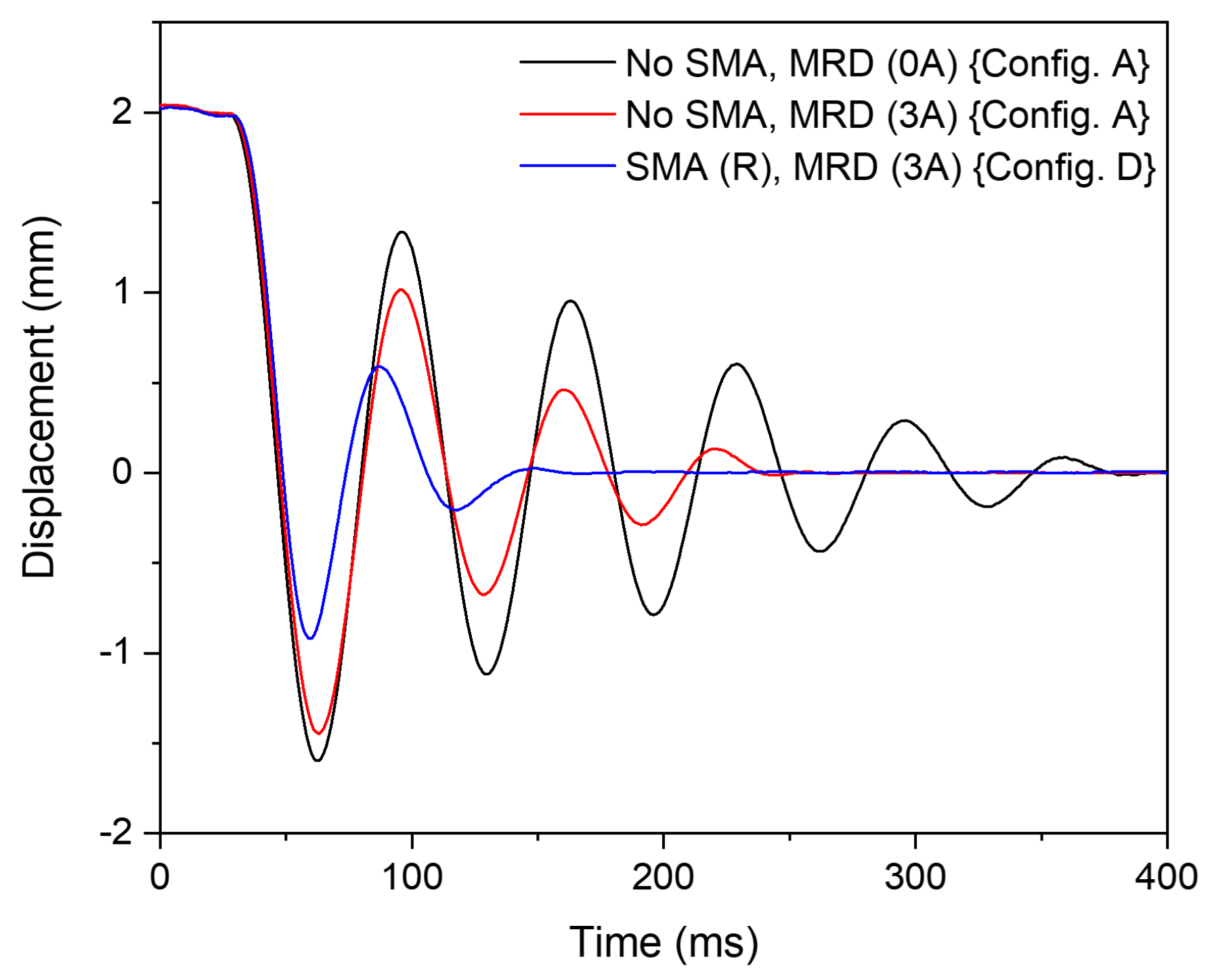
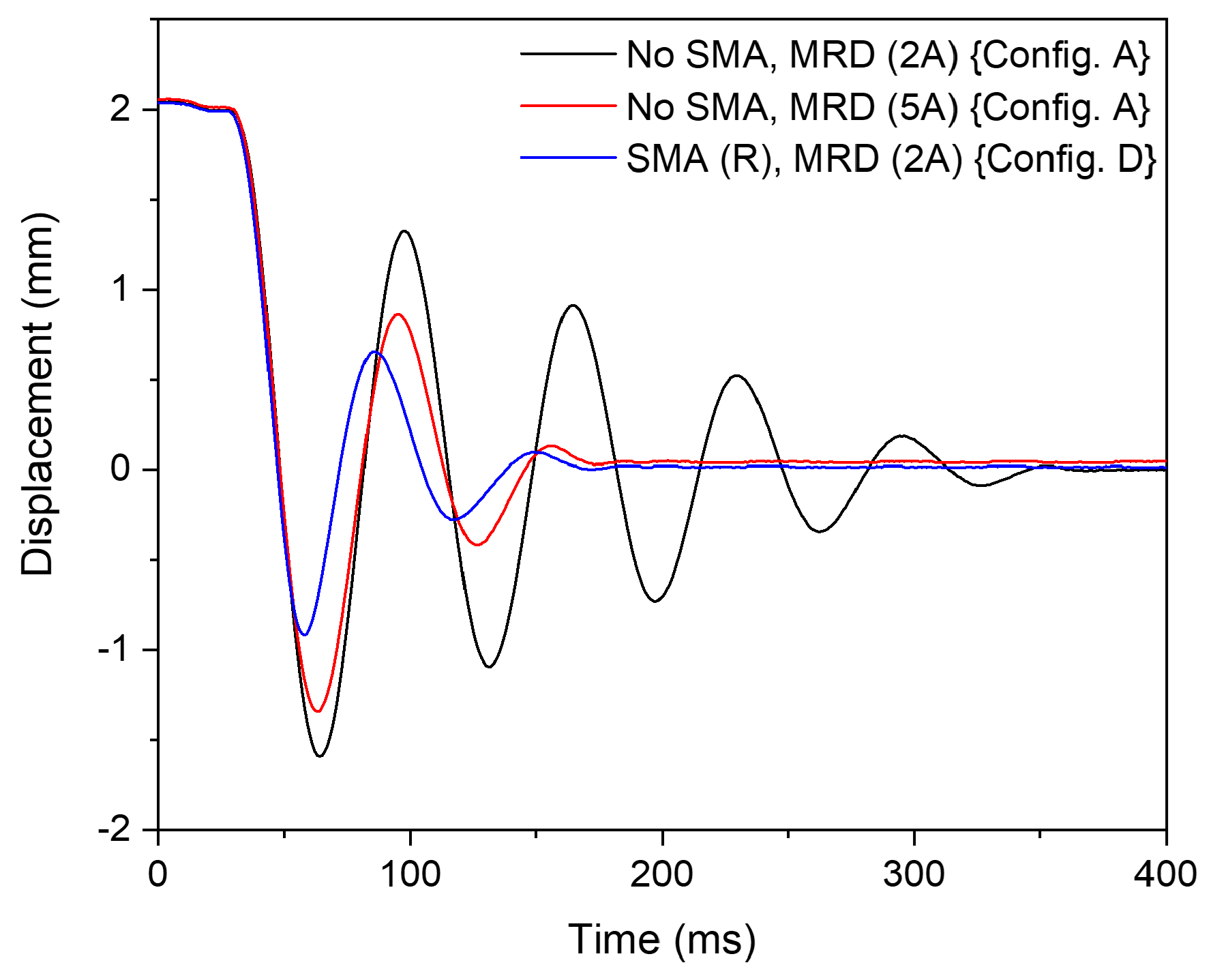
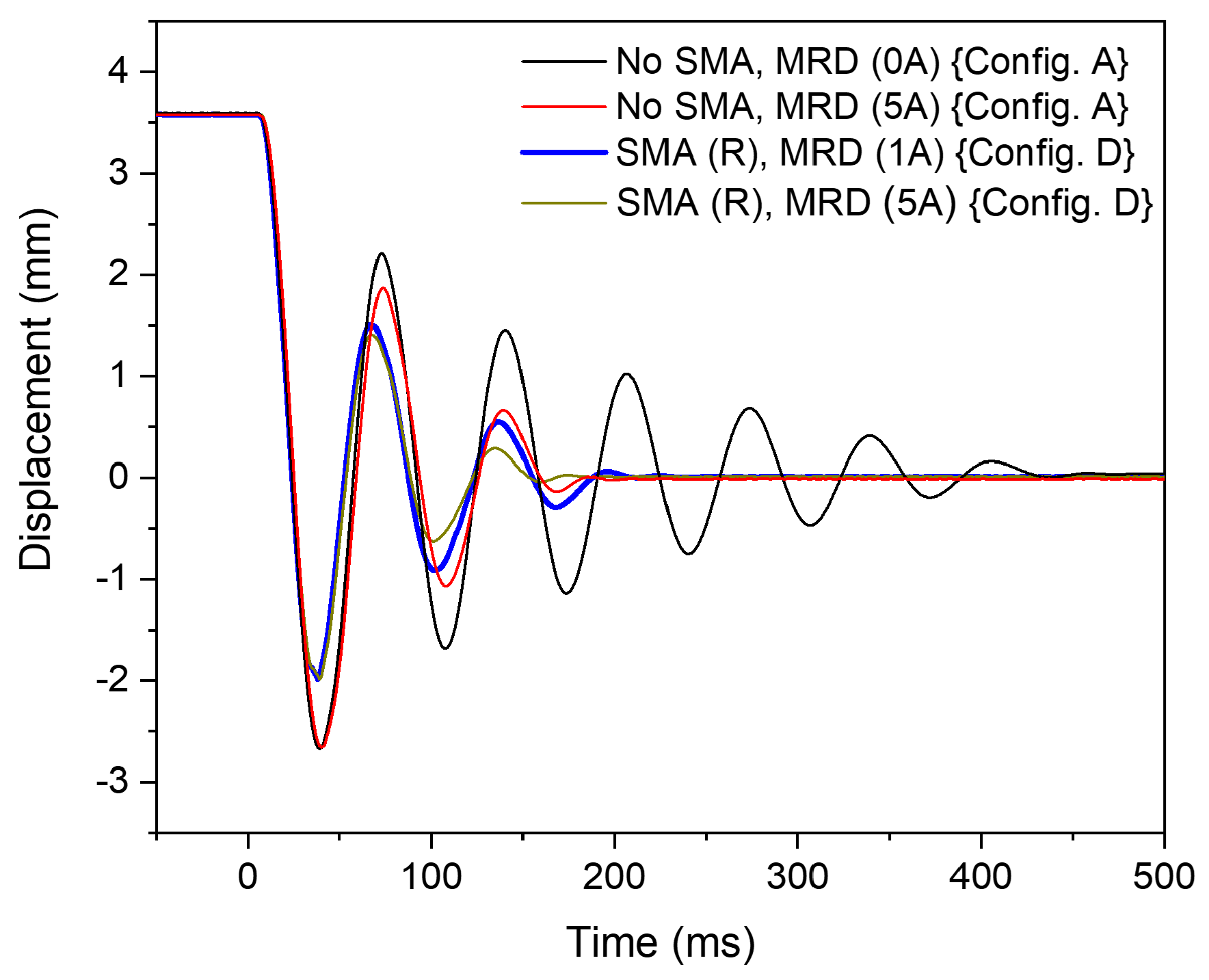
4.3. Hybrid Damper Configuration
4.4. Trend in Damping Performance with Shock Load of 2 mm
4.5. The Extent of Downscaling
4.6. Summary
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dixon, J.C. The Shock Absorber Handbook; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Tu, F.; Yang, Q.; He, C.; Wang, L. Experimental study and design on automobile suspension made of magneto-rheological damper. Energy Proc. 2012, 16, 417–425. [Google Scholar] [CrossRef]
- Kurazume, R.; Hirose, S. Development of image stabilization system for remote operation of walking robots. In Proceedings of the 2000 ICRA, Millennium Conference, IEEE International Conference on Robotics and Automation, Symposia Proceedings (Cat. No. 00CH37065), San Francisco, CA, USA, 24–28 April 2000; Volume 2, pp. 1856–1861. [Google Scholar]
- Kazi, A.; Honold, M.; Rimkus, W.; Lokner, T.; Baeuml, M.; Koepfer, M. SMA actuator for optical image stabilization. In Proceedings of the ACTUATOR 2018, 16th International Conference on New Actuators, Bremen, Germany, 25–27 June 2018; pp. 1–4. [Google Scholar]
- Hamaguchi, M. Damping and Transfer Control System with Parallel Linkage Mechanism-Based Active Vibration Reducer for Omnidirectional Wheeled Robots. IEEE/ASME Trans. Mechatron. 2018, 23, 2424–2435. [Google Scholar] [CrossRef]
- Foshage, G.K.; Davis, T.; Sullivan, J.M.; Hoffman, T.; Das, A. Hybrid active/passive actuator for spacecraft vibration isolation and suppression. In Proceedings of the Actuator Technology and Applications, International Society for Optics and Photonics, Denver, CO, USA, 4–9 August 1996; Volume 2865, pp. 104–122. [Google Scholar]
- Suda, Y.; Nakadai, S.; Nakano, K. Hybrid suspension system with skyhook control and energy regeneration (development of self-powered active suspension). Veh. Syst. Dyn. 1998, 29, 619–634. [Google Scholar] [CrossRef]
- Martins, I.; Esteves, M.; Da Silva, F.P.; Verdelho, P. Electromagnetic hybrid active-passive vehicle suspension system. In Proceedings of the 1999 IEEE 49th Vehicular Technology Conference (Cat. No. 99CH36363), Houston, TX, USA, 16–20 May 1999; Volume 3, pp. 2273–2277. [Google Scholar]
- Ebrahimi, B. Development of Hybrid Electromagnetic Dampers for Vehicle Suspension Systems. Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 2019. [Google Scholar]
- Olabi, A.G.; Grunwald, A. Design and application of magneto-rheological fluid. Mater. Des. 2007, 28, 2658–2664. [Google Scholar] [CrossRef]
- Jacob, K.; Sessner, V.; Miyazaki, S.; Kohl, M. Active Vibration Damping and Control using SMA Foil-based Devices. In Proceedings of the ACTUATOR, International Conference and Exhibition on New Actuator Systems and Applications 2021, Online, 17–19 February 2021; pp. 1–4. [Google Scholar]
- Jacob, K.; Ahmadi, S.; Wendler, F.; Miyazaki, S.; Gueltig, M.; Kohl, M. Shape Memory Foil-Based Active Micro Damping for Portable Applications. In Proceedings of the 2019 20th International Conference on Solid-State Sensors, Actuators and Microsystems & Eurosensors XXXIII (TRANSDUCERS & EUROSENSORS XXXIII), Berlin, Germany, 23–27 June 2019; pp. 590–593. [Google Scholar]
- Zareie, S.; Alam, M.S.; Seethaler, R.J.; Zabihollah, A. Stability control of a novel frame integrated with an SMA-MRF control system for marine structural applications based on the frequency analysis. Appl. Ocean Res. 2020, 97, 102091. [Google Scholar] [CrossRef]
- Zareie, S.; Hamidia, M.; Zabihollah, A.; Ahmad, R.; Dolatshahi, K.M. Design, validation, and application of a hybrid shape memory alloy-magnetorheological fluid-based core bracing system under tension and compression. Structures 2022, 35, 1151–1161. [Google Scholar] [CrossRef]
- Arus MR Tech Pvt Ltd. AMT-SMARTEC. 2021. Available online: https://arusmrtech.com/product/amt-smartec/ (accessed on 17 June 2022).
- Janocha, H. (Ed.) Adaptronics and Smart Structures, 2nd ed.; Springer: Berlin, Germany, 2007. [Google Scholar]


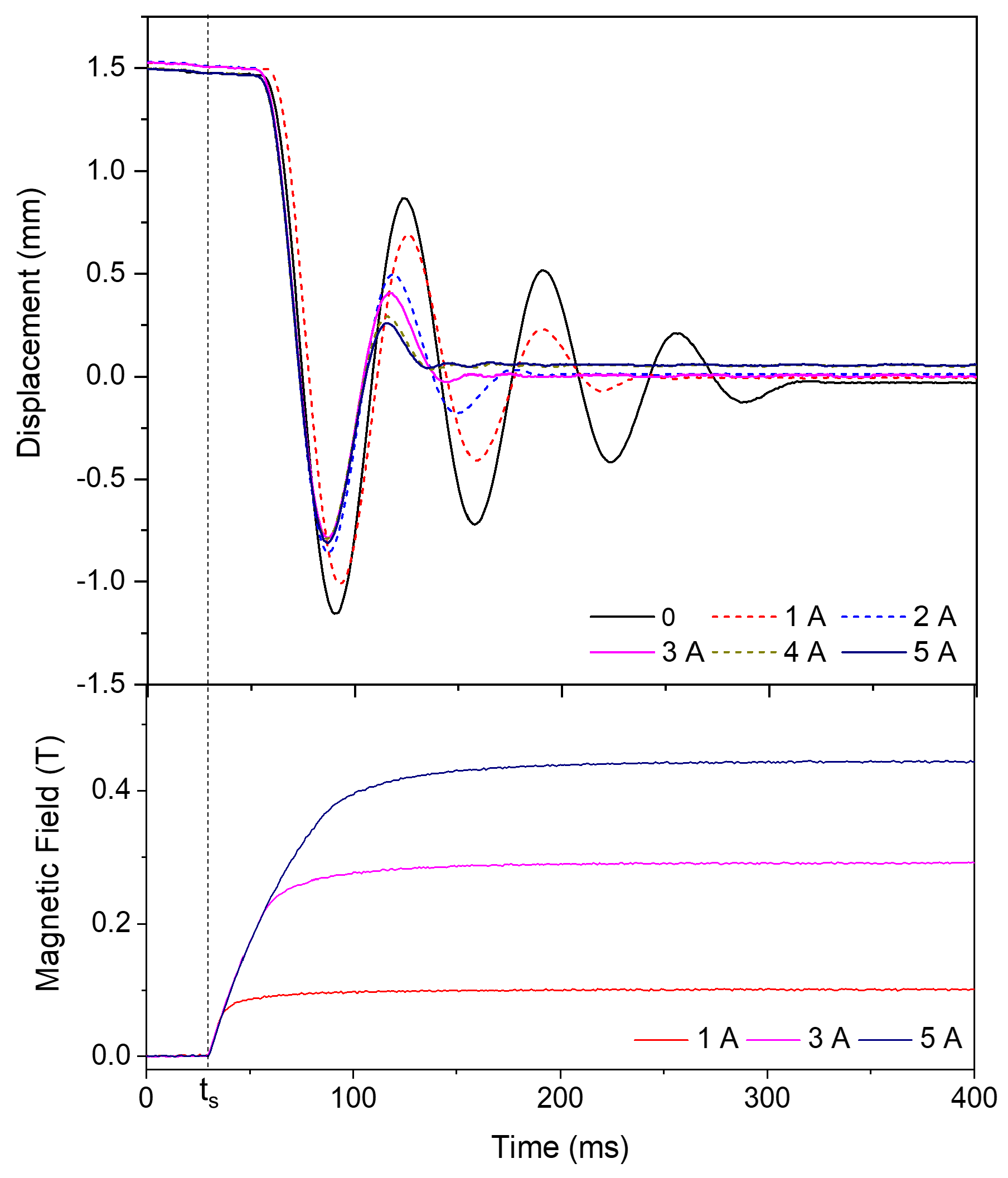
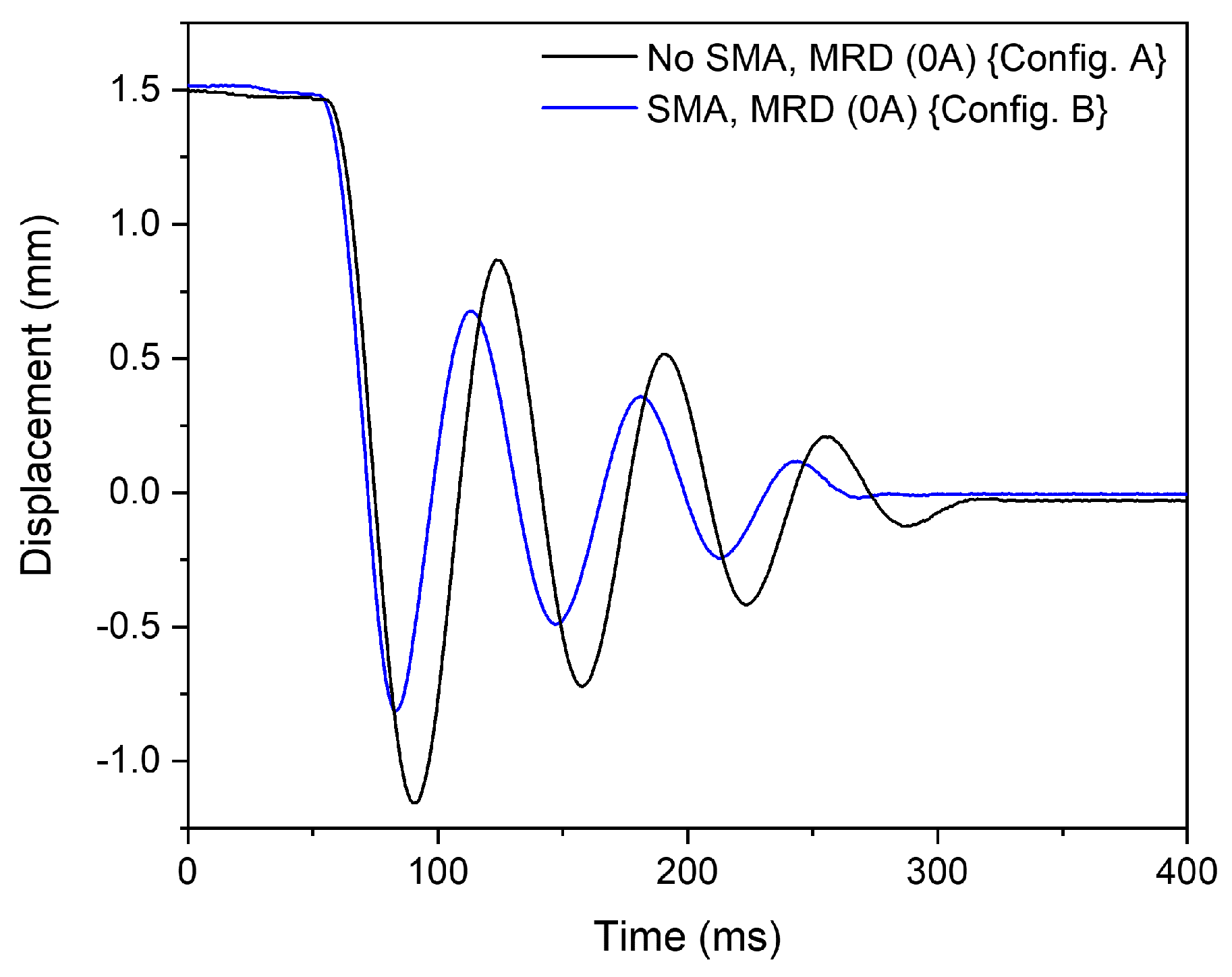
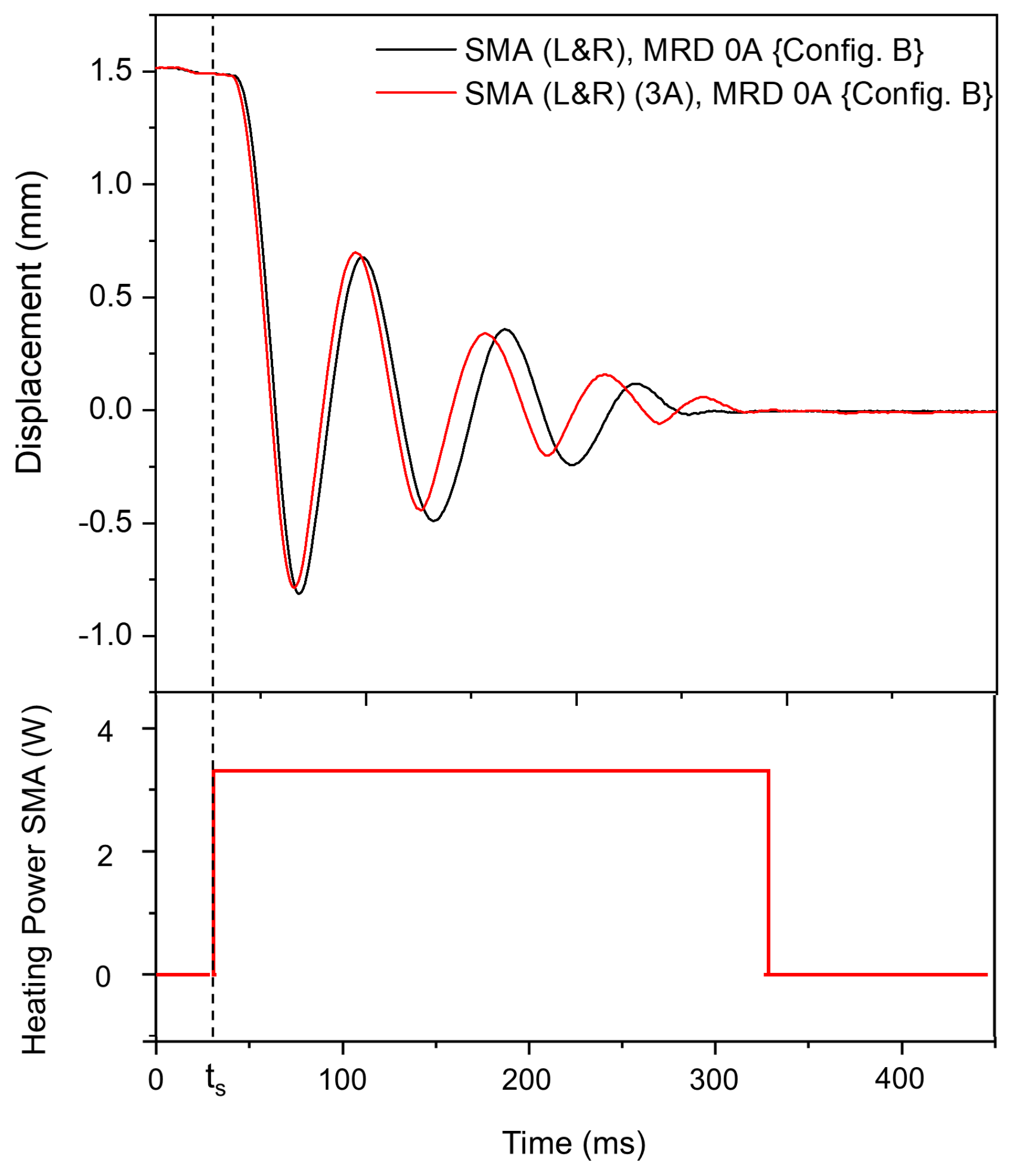
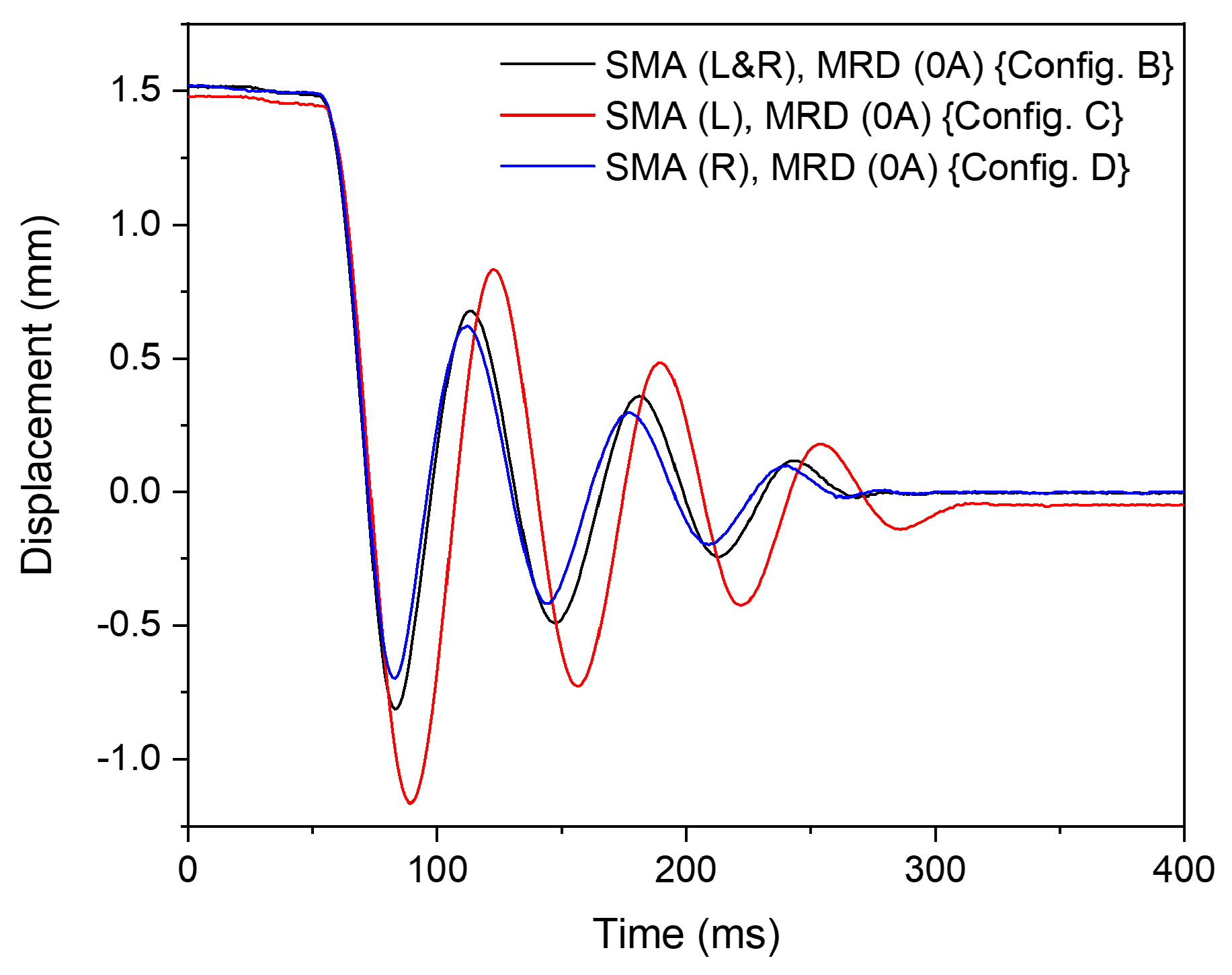
| Physical Property | Value | Unit |
|---|---|---|
| Dynamic viscosity | 0.37 | Pa.s |
| Density | 2900 | kg/m3 |
| Operating temperature T | −20–150 | °C |
| Yield stress at 140 kA/m | 69 | kPa |
| Physical Property | Value | Unit |
|---|---|---|
| MR Damper | ||
| Shear mode | ||
| - effective plate length | 50 | mm |
| - effective plate width | 30 | mm |
| - gap height | 0.5 | mm |
| - operating area | 1500 | mm |
| Electromagnet | ||
| - length | 100 | mm |
| - width | 50 | mm |
| - height | 113.6 | mm |
| MR damper size | ||
| - total length | 221 | mm |
| - total width | 149 | mm |
| - total height | 113.6 | mm |
| Shape Memory Alloy | ||
| - Length | 40 | mm |
| - Width | 3 | mm |
| - Thickness | 30 | µm |
| Vibratory System | ||
| Moving mass, m | 2.53 | kg |
| Total stiffness, k | 25 | kN/m |
| Natural frequency, | 15.82 | Hz |
| Natural period, | 63.2 | ms |
| d | |||
|---|---|---|---|
| 1.5 mm | 0.8 mm | 3 A | 64 % |
| 2.0 mm | 1.0 mm | 2 A | 84 % |
| 3.6 mm | 2.0 mm | 1 A | 96 % |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jacob, K.; Tan, A.S.; Sattel, T.; Kohl, M. Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation. Actuators 2022, 11, 280. https://doi.org/10.3390/act11100280
Jacob K, Tan AS, Sattel T, Kohl M. Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation. Actuators. 2022; 11(10):280. https://doi.org/10.3390/act11100280
Chicago/Turabian StyleJacob, Kiran, Aditya Suryadi Tan, Thomas Sattel, and Manfred Kohl. 2022. "Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation" Actuators 11, no. 10: 280. https://doi.org/10.3390/act11100280
APA StyleJacob, K., Tan, A. S., Sattel, T., & Kohl, M. (2022). Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation. Actuators, 11(10), 280. https://doi.org/10.3390/act11100280







