The Novel Singular-Perturbation-Based Adaptive Control with σ-Modification for Cable Driven System
Abstract
1. Introduction
- (1)
- Based on the singular perturbation theory, an adaptive control method with -modified adaptive law is proposed.
- (2)
- The stability of the flexible system is proved theoretically, and sufficient conditions for system stability are given.
- (3)
- This method is the first time to be used for the control of a flexible system with high-frequency motion.
- (4)
- The effectiveness of the proposed method is validated by experiments.
2. Analysis of Flexible System Kinematics and Dynamics
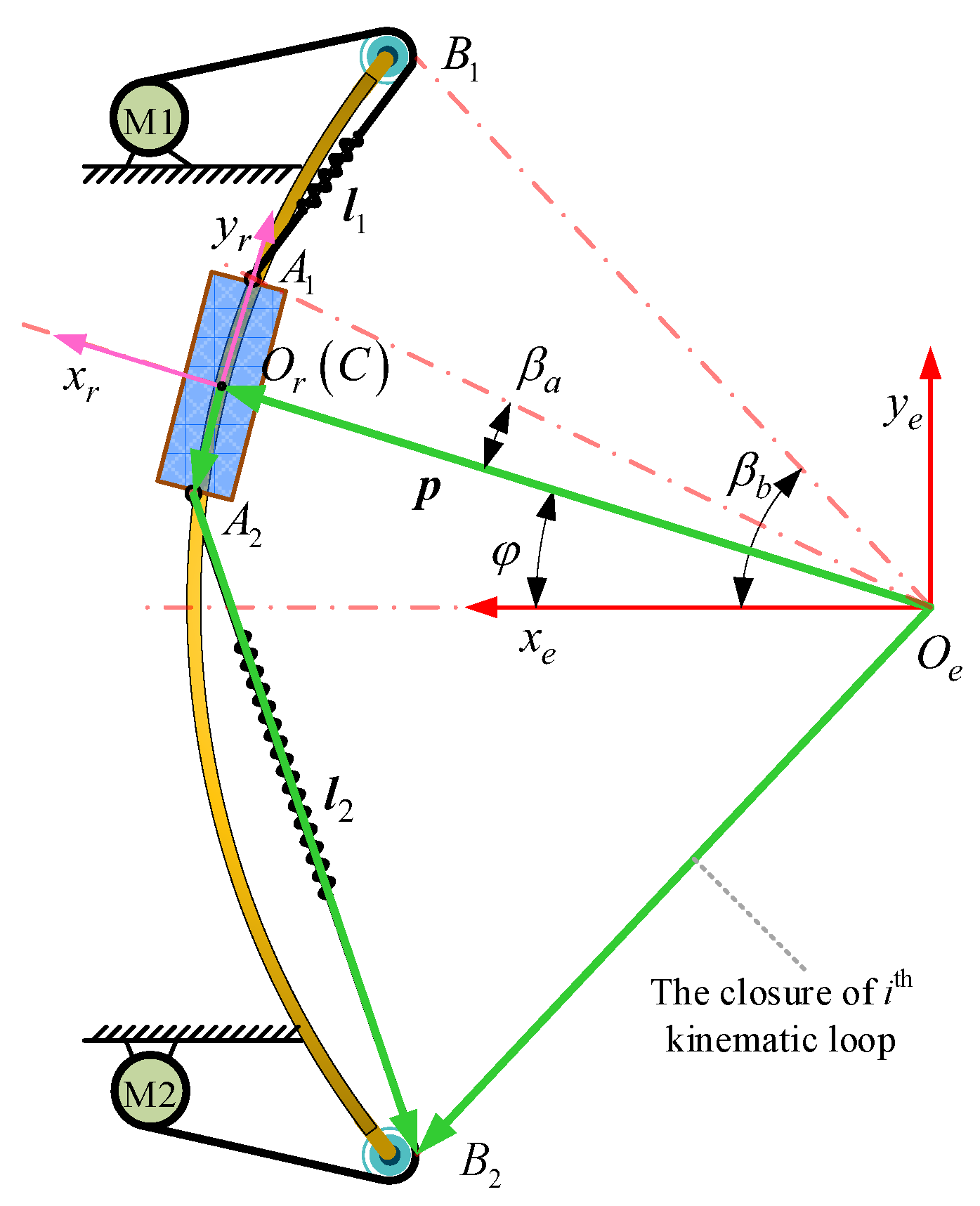
2.1. System Architecture
2.2. Analysis of System Kinematics
2.3. Analysis of System Dynamics
2.3.1. Dynamics Model
2.3.2. Dynamics Model Analysis
3. Composite Control Scheme
4. Stability Analysis
4.1. Singular Perturbation Standardization
4.2. Boundary Layer and Stability Analysis
4.3. Reduced-Order System and Stability Analysis
4.4. Stability Analysis of the Flexible System
5. Experimental Validation
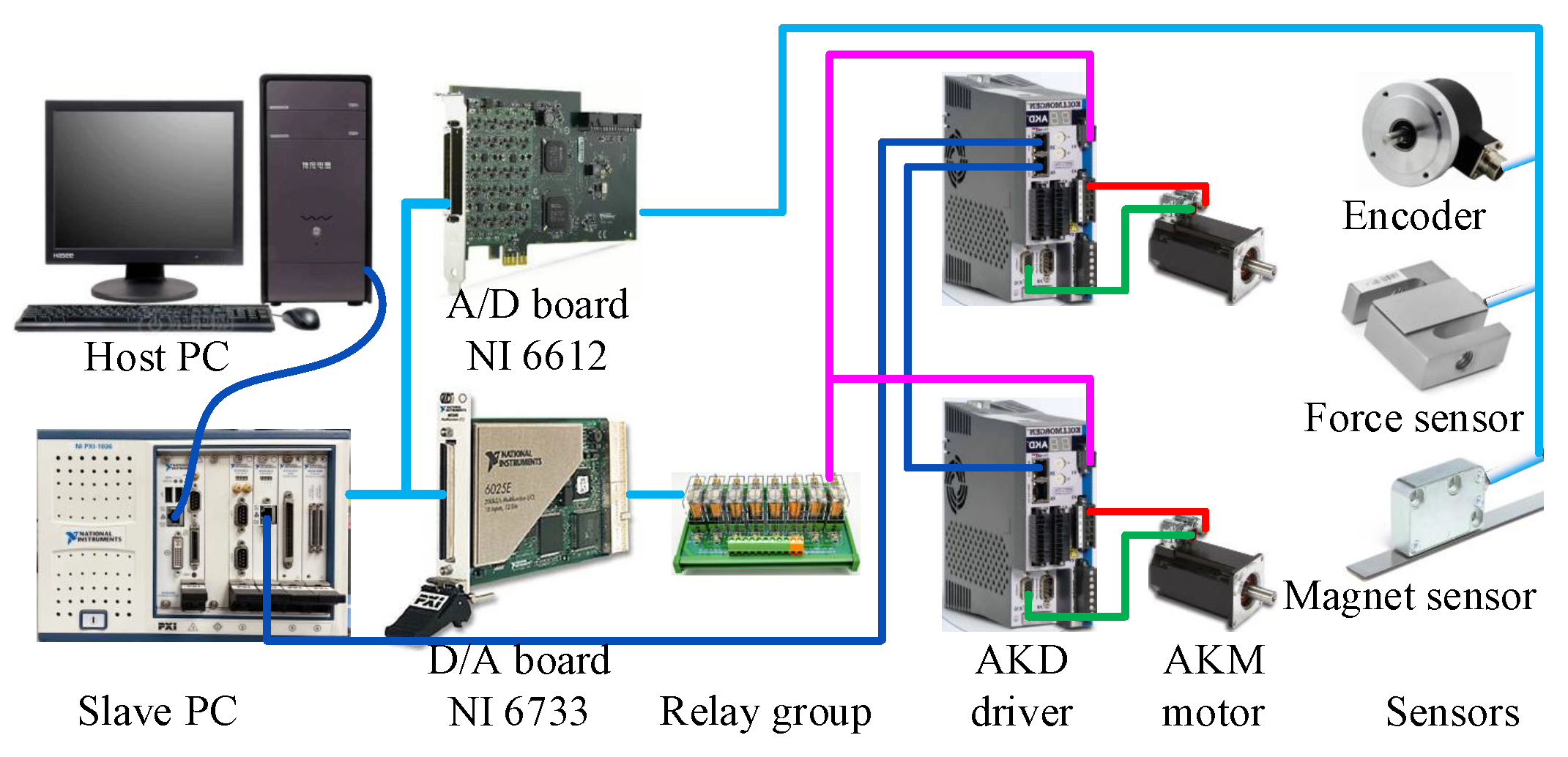
5.1. Experimental Setup
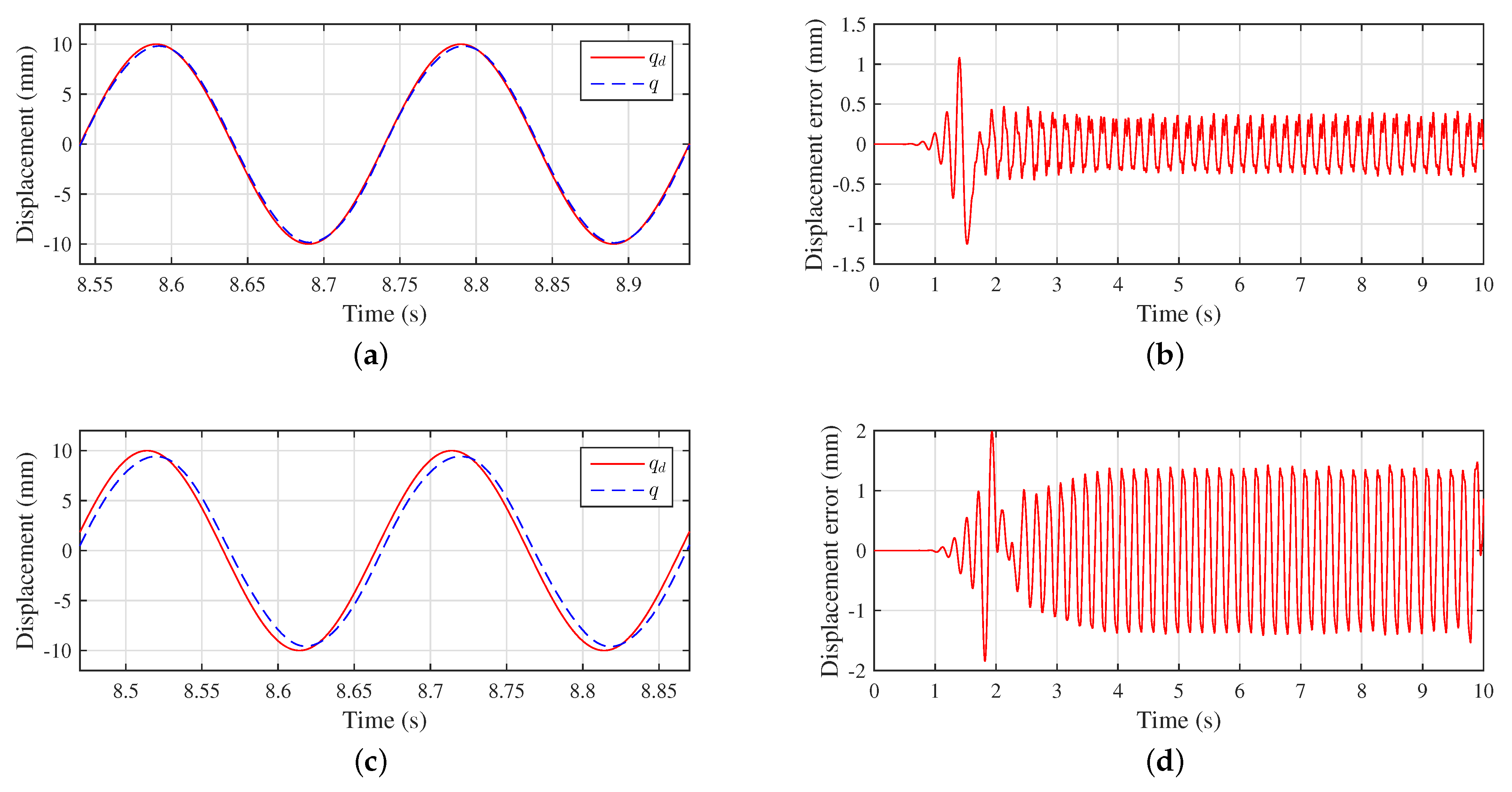
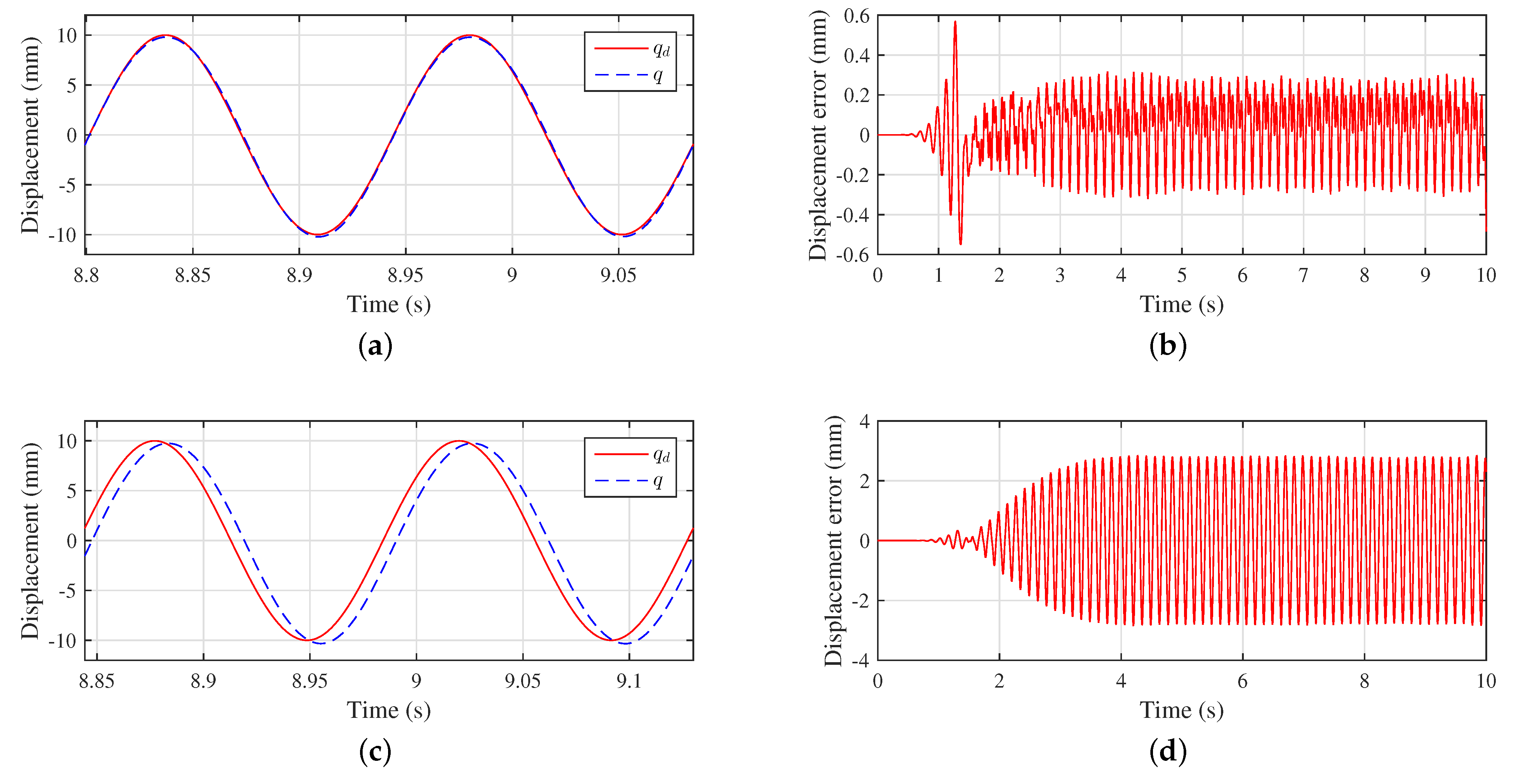
5.2. Experimental Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| -CAC | composite adaptive control with -modification |
| TAC | traditional adaptive control |
Appendix A
Appendix B
- Corollary 1
- Corollary 2
- Corollary 3
References
- Shiang, W.J.; Cannon, D.; Gorman, J. Dynamic analysis of the cable array robotic crane. In Proceedings of the 1999 IEEE International Conference on Robotics and Automation, Detroit, MI, USA, 10–15 May 1999; pp. 2495–2500. [Google Scholar] [CrossRef]
- Vrabel, R. Design of the state feedback-based feed-forward controller asymptotically stabilizing the double-pendulum-type overhead cranes with time-varying hoisting rope length. Int. J. Nonlinear Sci. Numer. Simul. 2020, 1–12. [Google Scholar] [CrossRef]
- Nan, R.; Li, D.; Jin, C.; Wang, Q.; Zhu, L.; Zhu, W.; Zhang, H.; Yue, Y.; Qian, L. The five-hundred-meter aperture spherical radio telescope (FAST) project. Int. J. Mod. Phys. D 2011, 20, 989–1024. [Google Scholar] [CrossRef]
- Pott, A.; Mütherich, H.; Kraus, W.; Schmidt, V.; Miermeister, P.; Verl, A. IPAnema: A family of Cable-Driven Parallel Robots for Industrial Applications. In Cable-Driven Parallel Robots, 1st ed.; Bruckmann, T., Pott, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; Volume 12, pp. 119–134. [Google Scholar]
- Wang, Y.; Song, C.; Zheng, T.; Lau, D.; Yang, K.; Yang, G. Cable Routing Design and Performance Evaluation for Multi-link Cable-Driven Robots with Minimal Number of Actuating Cables. IEEE Access 2019, 7, 135790–135800. [Google Scholar] [CrossRef]
- Lachele, P.M.; Boss, R. The cablerobot simulator large scale motion platform based on cable robot technology. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 3024–3029. [Google Scholar] [CrossRef]
- Tadokoro, S.; Murao, Y.; Hiller, M.; Murata, R.; Matsushima, T. A motion base with 6-DOF by parallel cable drive architecture. IEEE-ASME Trans. Mechatron. 2002, 7, 115–123. [Google Scholar] [CrossRef]
- Lee, T.; Kim, I.; Baek, Y.S. Design of a 2DoF Ankle Exoskeleton with a Polycentric Structure and a Bi-Directional Tendon-Driven Actuator Controlled Using a PID Neural Network. Actuators 2021, 10, 9. [Google Scholar] [CrossRef]
- Xiao, Y.; Lin, Q.; Zheng, Y.; Liang, B. Model Aerodynamic Tests with a Wire-driven Parallel Suspension System in Low-speed Wind Tunnel. Chin. J. Aeronaut. 2010, 23, 393–400. [Google Scholar] [CrossRef]
- Qian, S.; Zi, B.; Wang, D.; Li, Y. Development of Modular Cable-Driven Parallel Robotic Systems. IEEE Access 2018, 7, 5541–5553. [Google Scholar] [CrossRef]
- Xiong, H.; Diao, X. Cable tension control of cable-driven parallel manipulators with position-controlling actuators. In Proceedings of the 2017 IEEE International Conference on Robotics and Biomimetics (ROBIO), Macau, China, 5–8 December 2017; pp. 1763–1768. [Google Scholar] [CrossRef]
- Caverly, R.J.; Forbes, J.R. Flexible Cable-Driven Parallel Manipulator Control: Maintaining Positive Cable Tensions. IEEE Trans. Control Syst. Technol. 2018, 26, 1874–1883. [Google Scholar] [CrossRef]
- Oh, S.R.; Agrawal, S.K. Cable suspended planar robots with redundant cables: Controllers with positive tensions. IEEE Trans. Robot. 2005, 21, 457–465. [Google Scholar] [CrossRef]
- Shang, W.; Cong, S. Nonlinear computed torque control for a high-speed planar parallel manipulator. Mechatronics 2009, 19, 987–992. [Google Scholar] [CrossRef]
- Shang, W.; Zhang, B.; Fei, Z.; Shuang, C. Synchronization Control in the Cable Space for Cable-Driven Parallel Robots. IEEE Trans. Ind. Electron. 2019, 66, 4544–4554. [Google Scholar] [CrossRef]
- Najafi, F.; Bakhshizadeh, M. Development a fuzzy PID controller for a parallel cable robot with flexible cables. In Proceedings of the 2016 4th International Conference on Robotics and Mechatronics (ICROM), Tehran, Iran, 26–28 October 2016; pp. 90–97. [Google Scholar] [CrossRef]
- Jia, H.; Shang, W.; Xie, F.; Zhang, B.; Cong, S. Second-Order Sliding-Mode-Based Synchronization Control of Cable-Driven Parallel Robots. IEEE-ASME Trans. Mechatron. 2019, 25, 383–394. [Google Scholar] [CrossRef]
- Li, B.; Yan, L.; Zhang, L.; Gerada, C. Adaptive Robust Control of the Cable Driven System for Position Tracking. In Proceedings of the 15th IEEE Conference on Industrial Electronics and Applications (ICIEA), Kristiansand, Norway, 9–13 November 2020; pp. 253–257. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, F.; Zhu, K.; Chen, B.; Wu, H. Practical Adaptive Integral Terminal Sliding Mode Control for Cable-Driven Manipulators. IEEE Access 2020, 6, 78575–78586. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, F.; Chen, J.; Chen, B. Continuous Nonsingular Fast Terminal Sliding Mode Control of Cable-Driven Manipulators with Super-Twisting Algorithm. IEEE Access 2018, 6, 49626–49636. [Google Scholar] [CrossRef]
- El-Ghazaly, G.; Gouttefarde, M.; Creuze, V. Adaptive Terminal Sliding Mode Control of a Redundantly-Actuated Cable-Driven Parallel Manipulator: CoGiRo. In Cable-Driven Parallel Robots, 1st ed.; Bruckmann, T., Pott, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; Volume 32, pp. 179–200. [Google Scholar]
- Kino, H.; Yahiro, T.; Takemura, F.; Morizono, T. Robust PD Control Using Adaptive Compensation for Completely Restrained Parallel-Wire Driven Robots: Translational Systems Using the Minimum Number of Wires Under Zero-Gravity Condition. IEEE Trans. Robot. 2007, 23, 803–812. [Google Scholar] [CrossRef]
- Jabbari Asl, H.; Yoon, J. Robust trajectory tracking control of cable-driven parallel robots. Nonlinear Dyn. 2017, 89, 2769–2784. [Google Scholar] [CrossRef]
- Alp, A.B.; Alp, S.K. Cable suspended robots: Feedback controllers with positive inputs. In Proceedings of the 2002 American Control Conference, Anchorage, AK, USA, 8–10 May 2002; pp. 815–820. [Google Scholar] [CrossRef]
- Shang, W.; Xie, F.; Zhang, H.; Cong, S.; Li, Z. Adaptive Cross-Coupled Control of Cable-Driven Parallel Robots with Model Uncertainties. IEEE Robot. Autom. Lett. 2020, 5, 4110–4117. [Google Scholar] [CrossRef]
- Lamaury, J.; Gouttefarde, M.; Chemori, A.; Herve, P.-E. Dual-space adaptive control of redundantly actuated cable-driven parallel robots. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013; pp. 4879–4886. [Google Scholar] [CrossRef]
- Caverly, R.J.; Forbes, J.R. Dynamic Modeling and Noncollocated Control of a Flexible Planar Cable-Driven Manipulator. IEEE Trans. Robot. 2014, 30, 1386–1397. [Google Scholar] [CrossRef]
- Khosravi, M.A.; Taghirad, H.D. Robust PID control of fully-constrained cable driven parallel robots. Mechatronics 2013, 25, 87–97. [Google Scholar] [CrossRef]
- Khosravi, M.A.; Taghirad, H.D. Experimental performance of robust PID controller on a planar cable robot. In Cable-Driven Parallel Robots, 1st ed.; Bruckmann, T., Pott, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; Volume 12, pp. 337–352. [Google Scholar]
- Babaghasabha, R.; Khosravi, M.A.; Taghirad, H.D. Adaptive robust control of fully-constrained cable driven parallel robots. Mechatronics 2015, 25, 27–36. [Google Scholar] [CrossRef]
- Khosravi, M.A.; Taghirad, H.D. Dynamic modeling and control of parallel robots with elastic cables: Singular perturbation approach. IEEE Trans. Robot. 2014, 30, 694–704. [Google Scholar] [CrossRef]
- Vafaei, A.; Khosravi, M.A.; Taghirad, H.D. Modeling and Control of Cable Driven Parallel Manipulators with Elastic Cables: Singular Perturbation Theory. In Proceedings of the 4th International Intelligent Robotics and Applications, Aachen, Germany, 6–8 December 2011; pp. 455–464. [Google Scholar]





| Symbol | Specification | σ-CAC | TAC |
|---|---|---|---|
| Initial Value of | 20 kg | 20 kg | |
| Initial Value of | 10 N/m/s | 10 N/m/s | |
| Initial Value of | 100 N | 100 N | |
| Initial Value of | 200 N | 200 N | |
| Gain of | 0.2 | 0.2 | |
| Gain of | 1 | 1 | |
| Gain of | 0.4 | 0.4 | |
| Gain of | 0.2 | 0.2 | |
| Feedback gain | 15 | 15 | |
| Feedback gain | 100 | 100 | |
| Coefficient | 10 | – | |
| Coefficient | 10 | – | |
| Constant positive value | 0.1 | – |
| Frequency (Hz) | -CAC | TAC | ||
|---|---|---|---|---|
| Magnitude | Phase (deg) | Magnitude | Phase (deg) | |
| 2 | 3.65% | −2.4 | −0.72% | 3.6 |
| 3 | 0.39% | −3.24 | −1.38% | −4.32 |
| 4 | −2.09% | −1.44 | 1.09% | −6.91 |
| 5 | −1.39% | −2.16 | −6.54% | −9.1 |
| 6 | −0.99% | 0.864 | −4.64% | −12.96 |
| 7 | −1.17% | −1.8 | −2.55% | −16.13 |
| 8 | −2.58% | −5.78 | - | - |
| 9 | −2.01% | −9.2 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, B.; Yan, L.; Gerada, C. The Novel Singular-Perturbation-Based Adaptive Control with σ-Modification for Cable Driven System. Actuators 2021, 10, 45. https://doi.org/10.3390/act10030045
Li B, Yan L, Gerada C. The Novel Singular-Perturbation-Based Adaptive Control with σ-Modification for Cable Driven System. Actuators. 2021; 10(3):45. https://doi.org/10.3390/act10030045
Chicago/Turabian StyleLi, Bin, Liang Yan, and Chris Gerada. 2021. "The Novel Singular-Perturbation-Based Adaptive Control with σ-Modification for Cable Driven System" Actuators 10, no. 3: 45. https://doi.org/10.3390/act10030045
APA StyleLi, B., Yan, L., & Gerada, C. (2021). The Novel Singular-Perturbation-Based Adaptive Control with σ-Modification for Cable Driven System. Actuators, 10(3), 45. https://doi.org/10.3390/act10030045





