Design of a Maglev Inertial Actuator with High Mass Power Ratio for Lateral Vibration Control of Propulsion Shafting
Abstract
:1. Introduction
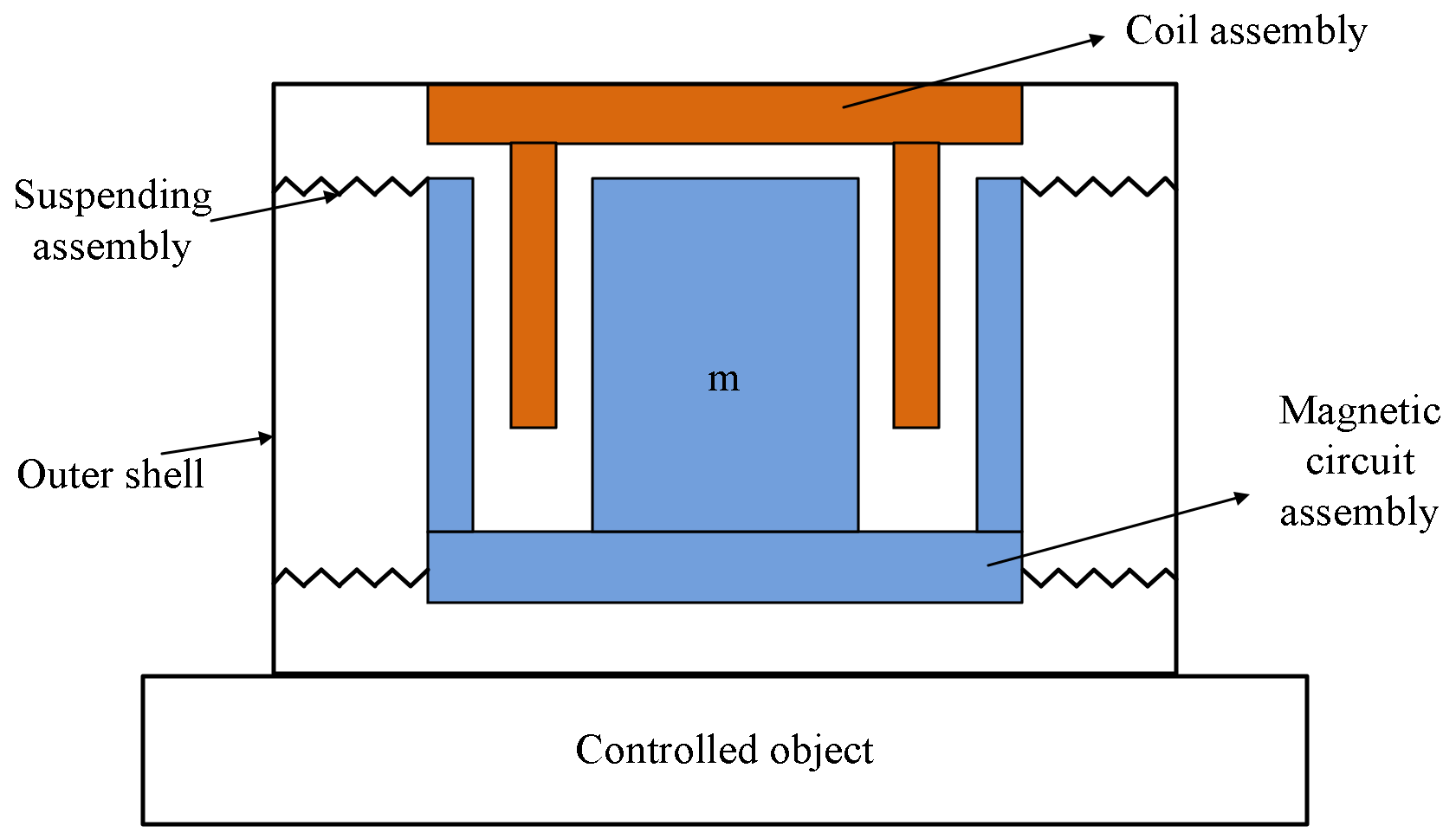
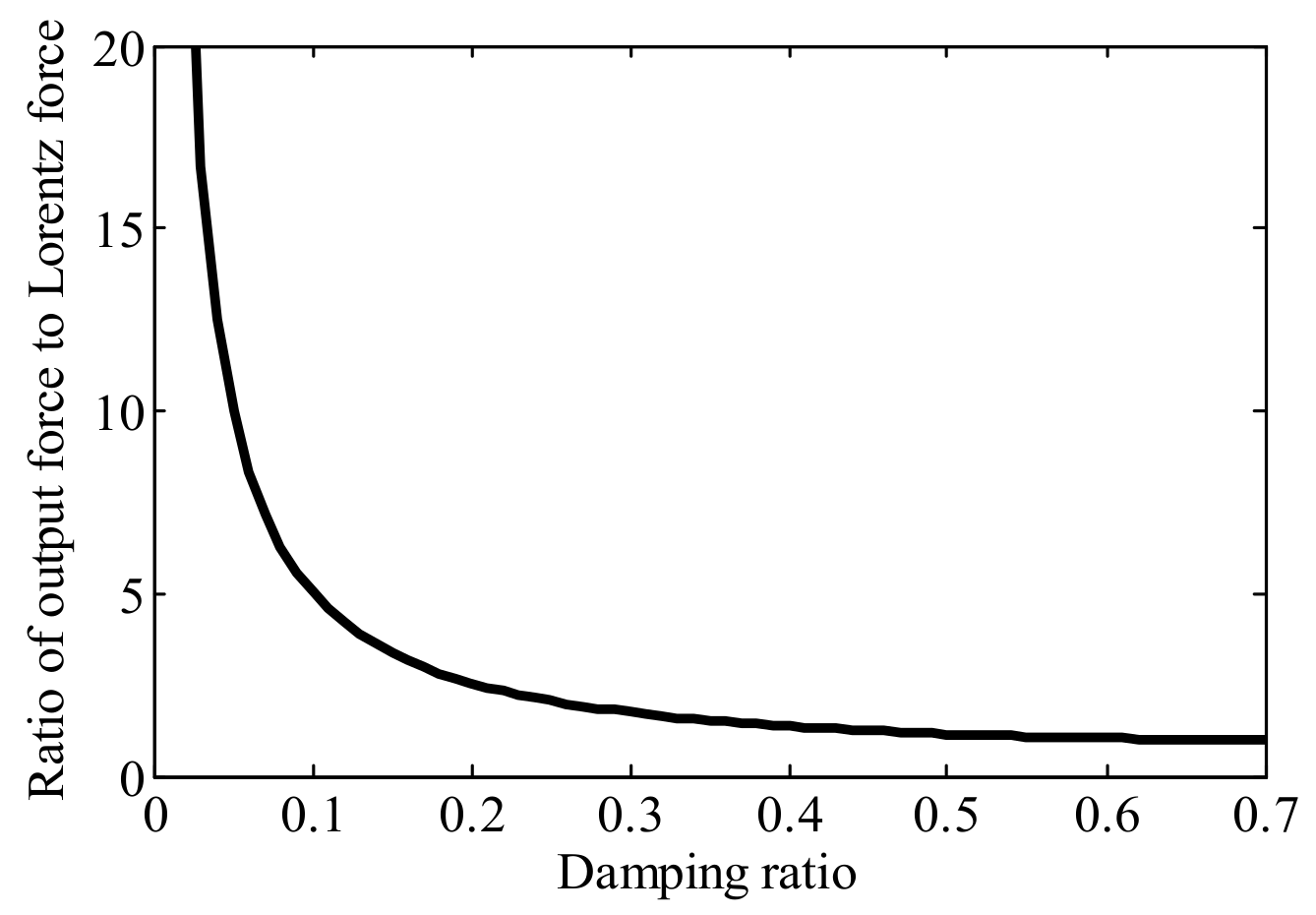
2. The Working Principle of a Maglev Inertial Actuator
3. Design of The Proposed Maglev Inertial Actuator
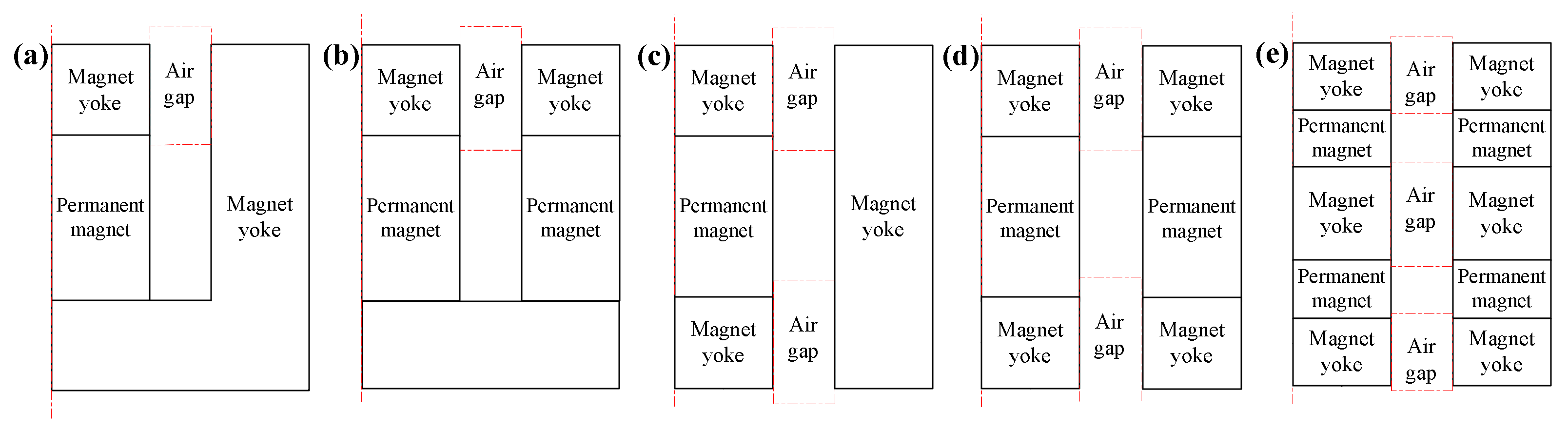
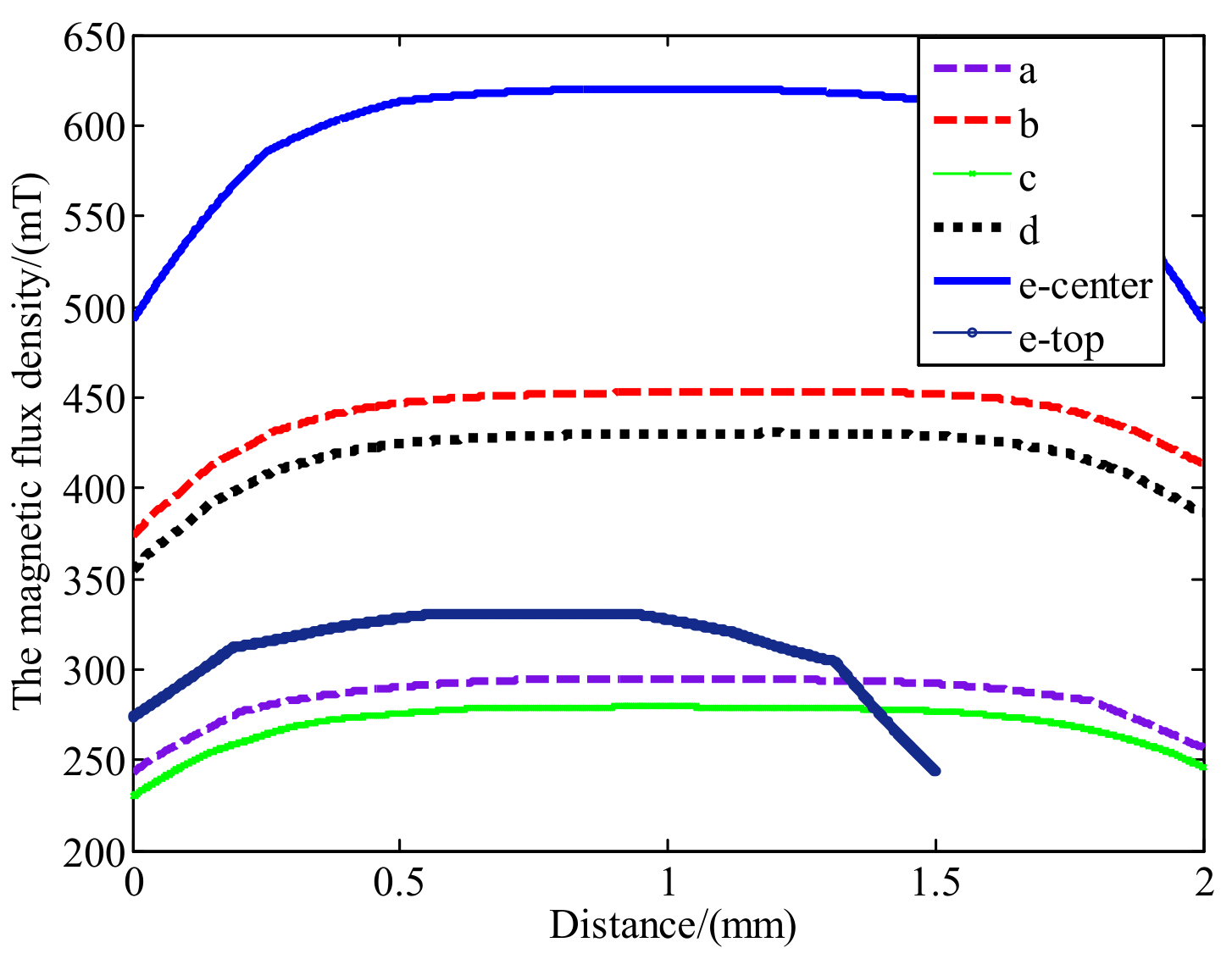
3.1. Design and Scheme Optimization of Magnetic Circuit Assembly
3.2. Design and Scheme Optimization of the Suspending Assembly
4. Results and Discussion
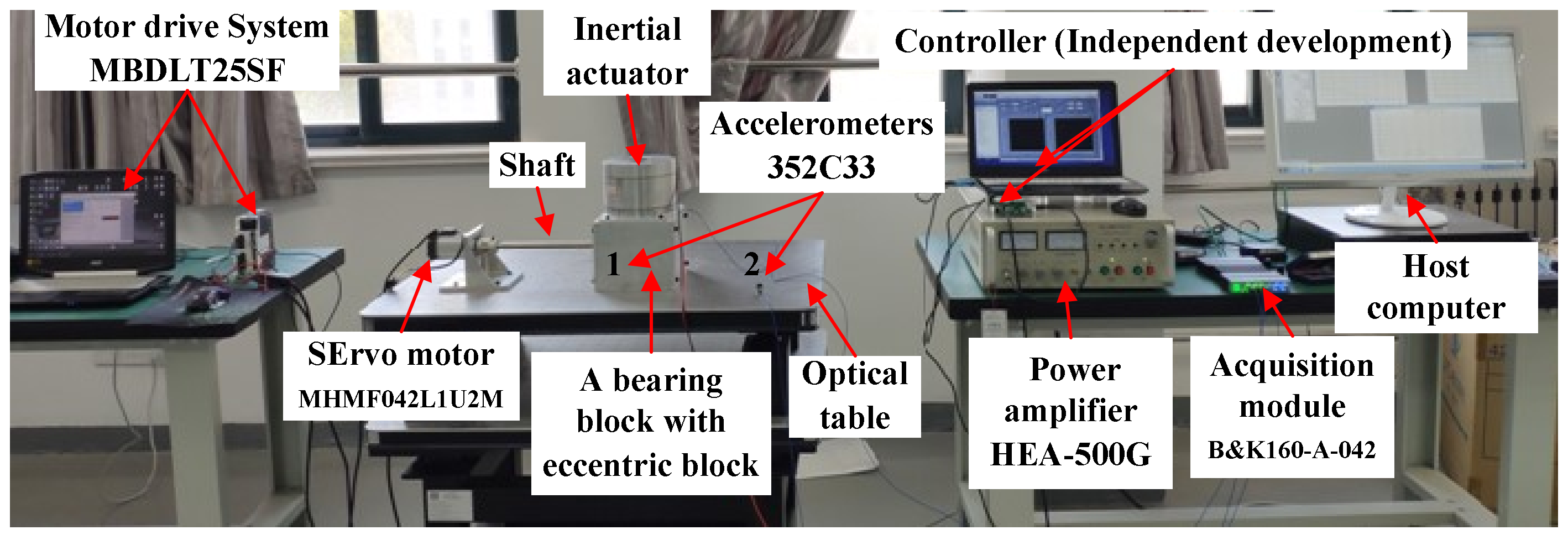
4.1. Results of Prototype Experiments
4.2. Results of Active Control of Lateral Vibration of Propulsion Shafting
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lin, C.G.; Zou, M.S.; Sima, C. Friction-induced vibration and noise of marine stern tube bearings considering perturbations of the stochastic rough surface. Tribol. Int. 2019, 131, 661–671. [Google Scholar] [CrossRef]
- Lucke, K.; Martin, S.B.; Racca, R. Evaluating the predictive strength of underwater noise exposure criteria for marine mammals. J. Acoust. Soc. Am. 2020, 147, 3985–3991. [Google Scholar] [CrossRef] [PubMed]
- Xie, X.; Ren, M.; Zhu, Y.; Zhang, Z. Simulation and experiment on lateral vibration transmission control of a shafting system with active stern support. Int. J. Mech. Sci. 2019, 170, 105363. [Google Scholar] [CrossRef]
- Fereidoun, A.; Hassan, R.; Majid, A.A. Adaptive control of rotationally non-linear asymmetric structures under seismic loads. Struct. Eng. Mech. 2018, 65, 721–730. [Google Scholar] [CrossRef]
- Serdar, U.; Gebrail, B.; Sinan, M.N. Active structural control via metaheuristic algorithms considering soil-structure interaction. Struct. Eng. Mech. 2020, 75, 175–191. [Google Scholar] [CrossRef]
- Lu, Y.-F.; Yue, H.-H.; Deng, Z.-Q.; Tzou, H.-S. Adaptive shape control for thermal deformation of membrane mirror with in-plane PVDF actuators. Chin. J. Mech. Eng. 2018, 31, 9. [Google Scholar] [CrossRef] [Green Version]
- Su, Z.Q.; Zhou, M.; Han, F.F.; Zhu, Y.W.; Song, D.L.; Guo, T.T. Attitude control of underwater glider combined reinforcement learning with active disturbance rejection control. J. Mar. Sci. Tech. 2019, 24, 686–704. [Google Scholar] [CrossRef]
- Wang, R.; Li, X.; Liu, Y.; Ahemd, Q.; Yang, Y.; Feng, C.; Ma, X. Variable sampling rate based active disturbance control for a marine diesel engine. Electronics 2019, 8, 370. [Google Scholar] [CrossRef] [Green Version]
- Kitamura, E.; Nabae, H.; Endo, G.; Suzumori, K. Self-excitation pneumatic soft actuator inspired by vocal cords. Sensor Actuator A Phys. 2021, 331, 112816. [Google Scholar] [CrossRef]
- Nahian, S.A.; Dinh, T.Q.; Dao, H.V.; Ahn, K.K. An unknown input observer-EFIR combined estimator for electro-hydraulic actuator in sensor fault tolerant control application. IEEE/ASME Trans. Mechatron. 2020, 5, 2208–2219. [Google Scholar] [CrossRef]
- Kim, M.; Kim, H.; Gweon, D. Design and optimization of voice coil actuator for six degree of freedom active vibration isolation system using Halbach magnet array. Rev. Sci. Instrum. 2012, 83, 105–117. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.; Yang, B.T.; Li, H.; Meng, G. Dynamic modeling and adaptive vibration control study for giant magnetostrictive actuators. Sensor Actuator A Phys. 2013, 190, 96–105. [Google Scholar] [CrossRef]
- Csencsics, E.; Thier, M.; Hainisch, R.; Schitter, G. System and control design of a voice coil actuated mechanically decoupling two-body vibration isolation system. IEEE/ASME Trans. Mechatron. 2017, 23, 321–330. [Google Scholar] [CrossRef]
- Hong, J.; Wang, S.; Sun, Y.; Cao, H. A method of modal parameter estimation of the motor based on electromagnetic vibration exciter. IEEE Trans. Ind. Appl. 2020, 56, 2636–2643. [Google Scholar] [CrossRef]
- Wang, T.; Liu, Y.; Xu, Q.-N.; Han, D. Novel structure for waveform control of twin rotary flowrate valve controlled vibration exciter. IEEE/ASME Trans. Mechatron. 2021, 26, 1183–1188. [Google Scholar] [CrossRef]
- Grodsinsky, C.M.; Whorton, M.S. Survey of active vibration isolation systems for microgravity applications. J. Spacecr. Rockets 2000, 37, 586–596. [Google Scholar] [CrossRef] [Green Version]
- Kim, M.H.; Kim, H.Y.; Kim, H.C.; Ahn, D.; Gweon, D.G. Design and control of a 6-DOF active vibration isolation system using a Halbach magnet array. IEEE/ASME Trans. Mechatron. 2016, 21, 2185–2196. [Google Scholar] [CrossRef]
- Kato, H.; Mashino, M.; Ishida, M.; Hasegawa, S.; Oshinoya, Y. Active seat suspension for ultra-compact electric vehicle: Fundamental consideration on ride comfort evaluation using myoelectric potential. J. Jpn. Soc. Appl. Electromagn. Mech. 2015, 23, 74–79. [Google Scholar] [CrossRef] [Green Version]
- Foo, E.; Goodall, R. Active suspension control of flexible-bodied railway vehicles using electro-hydraulic and electro-magnetic actuators. Control Eng. Pract. 2000, 8, 507–518. [Google Scholar] [CrossRef]
- Caresta, M. Active control of sound radiated by a submarine in bending vibration. J. Sound Vib. 2011, 330, 615–624. [Google Scholar] [CrossRef]
- Janzen, P.C.; Keas, P.J. Implementation of an active vibration damping system for the SOFIA telescope assembly. In Proceedings of SPIE Ground-Based and Airborne Telescopes V; SPIE: Bellingham, WA, USA, 2014; Volume 91452, p. 91452N. [Google Scholar] [CrossRef]


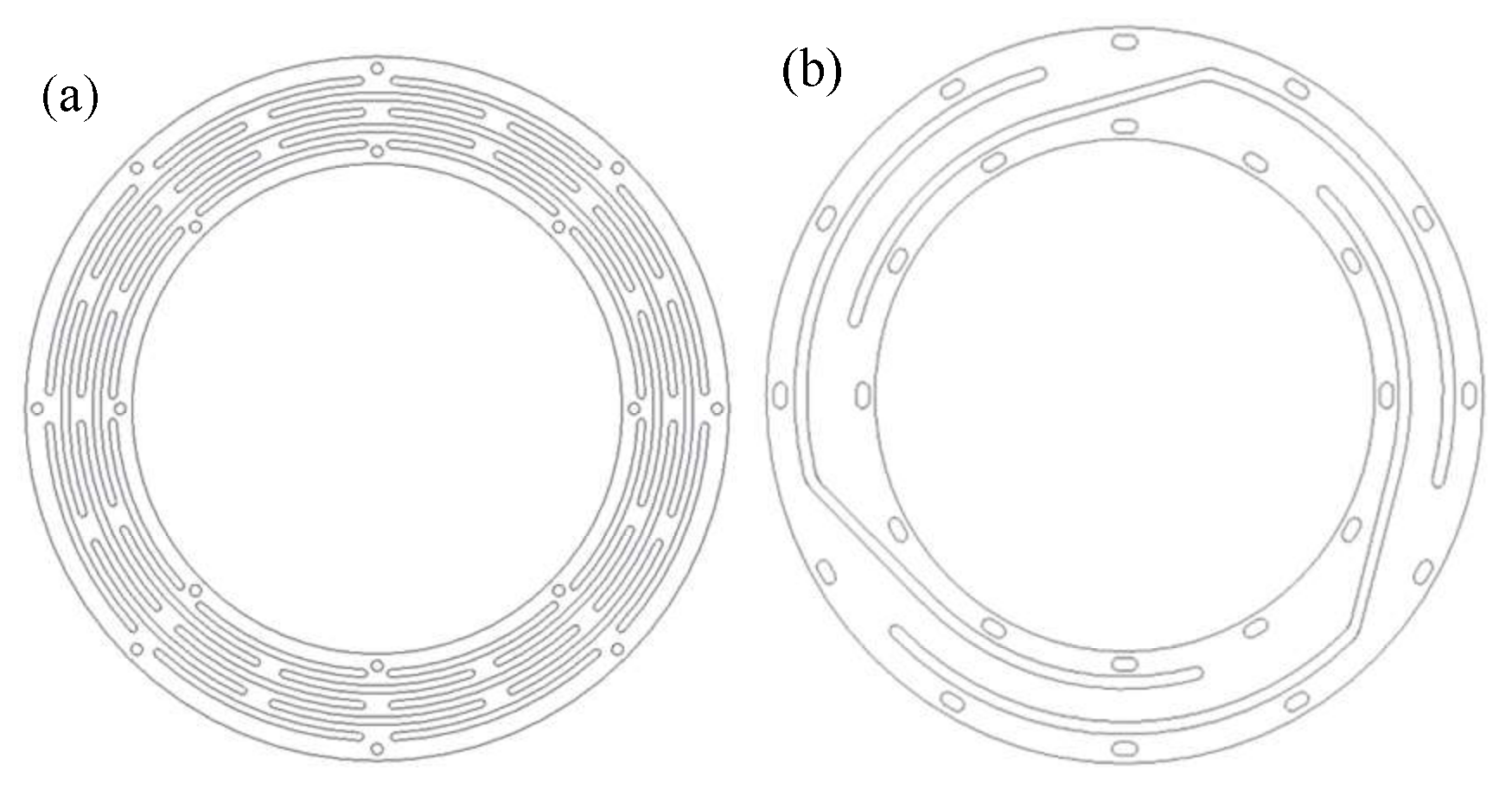
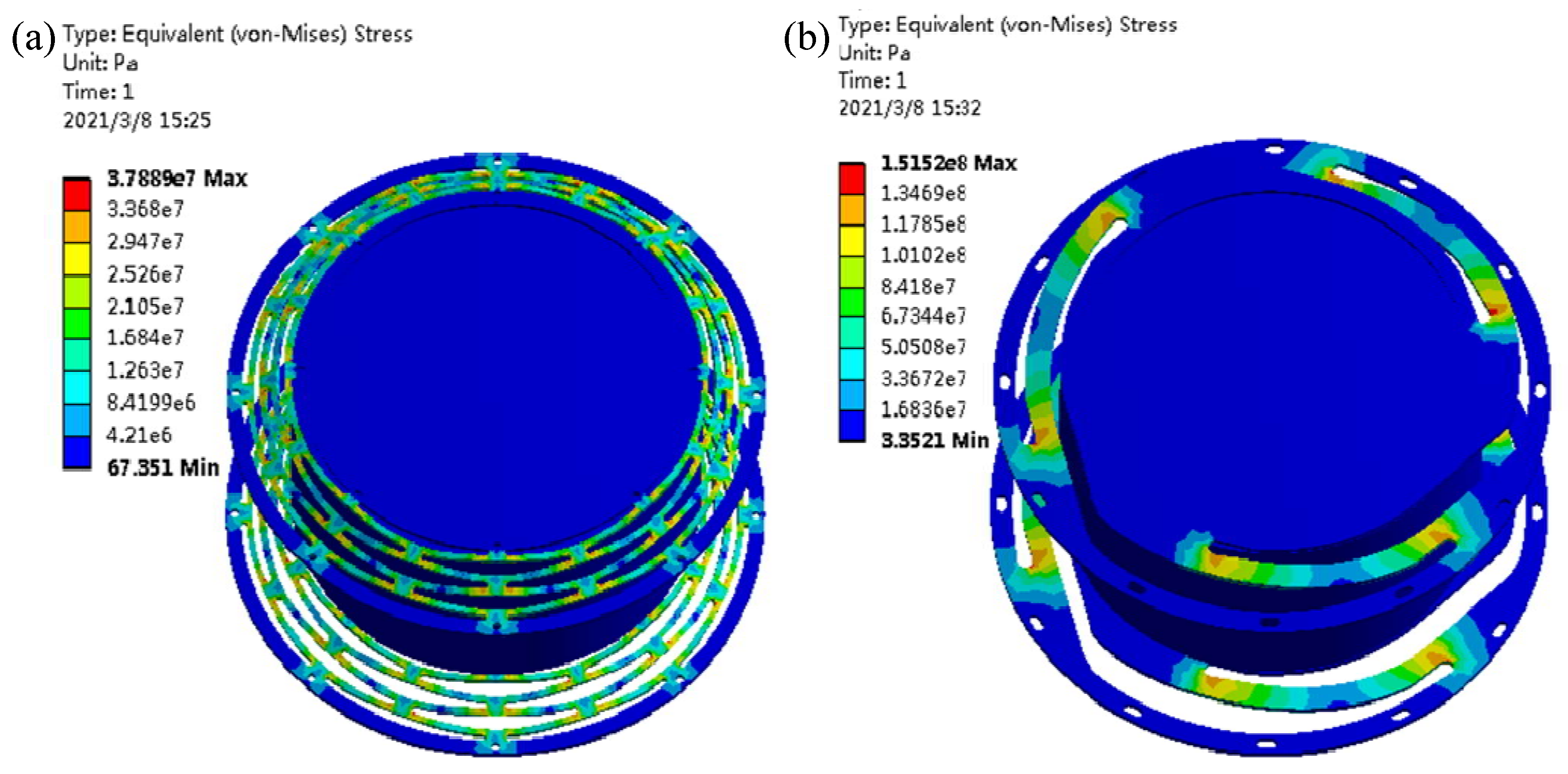

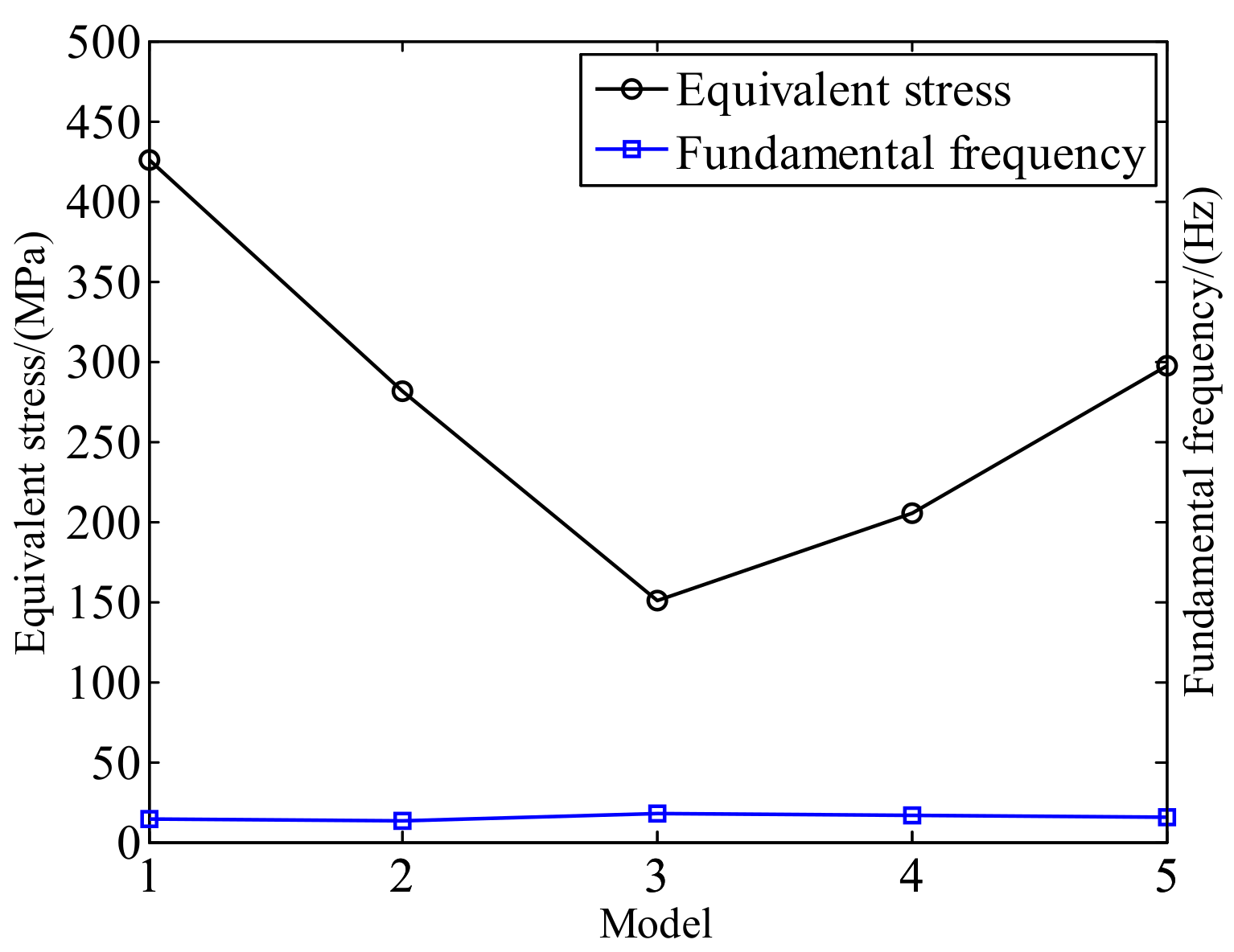


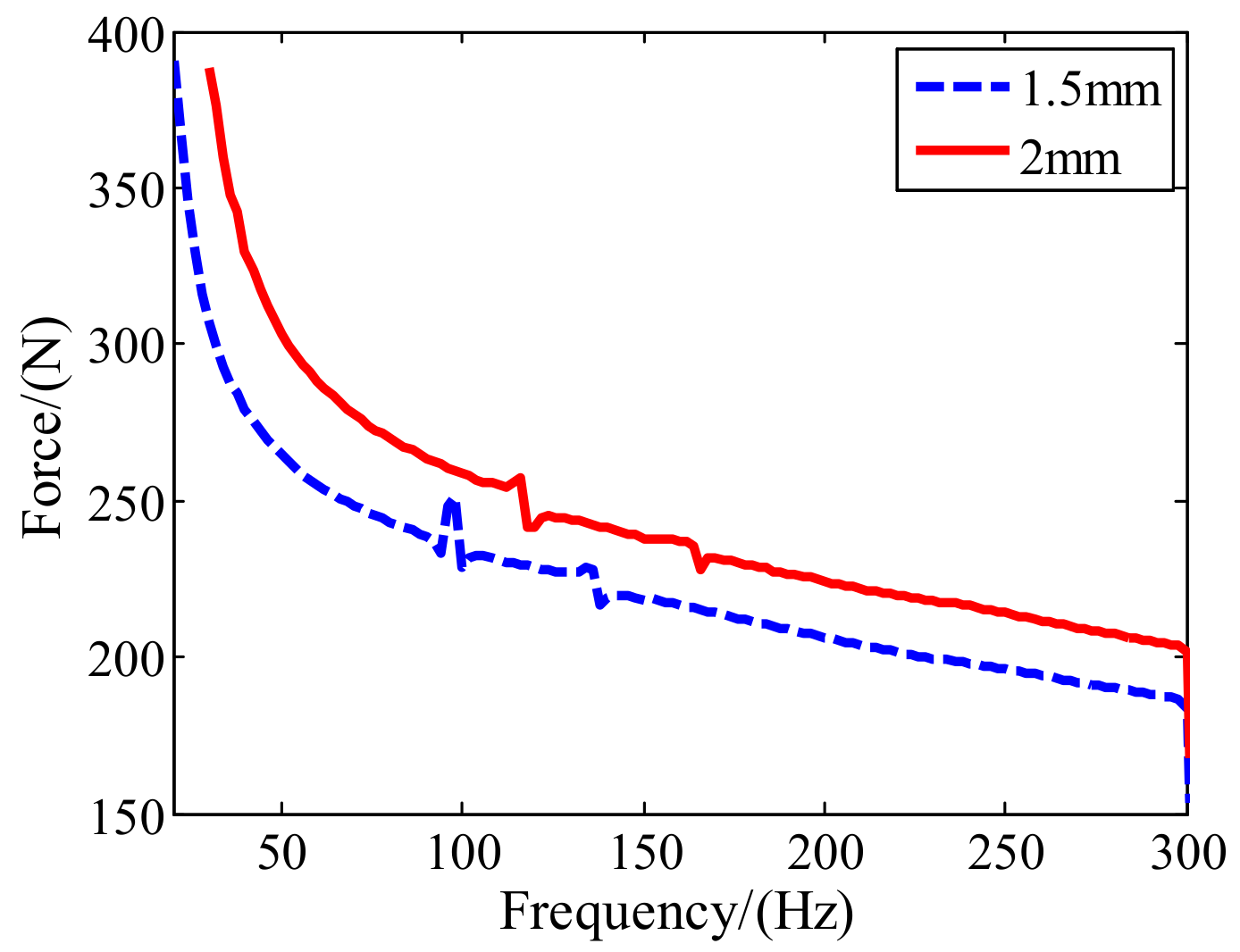


| Scheme | Fundamental Frequency | Equivalent Stress |
|---|---|---|
| a | 58.022 Hz | 37.9 MPa |
| b | 15.409 Hz | 297 MPa |
| Materials | Electrical Conductivity (S/m) | Magnetic Conductivity (H/m) | Thermal Conductivity (W/mK) | Density (g/cm3) | Specific Heat J/(kg·°C) |
|---|---|---|---|---|---|
| Aluminium alloy frame 6061 | 3.5 × 107 | 4π × 10−7 | 150 | 2.7 | 0.88 × 103 |
| Magnet yokeDT4 | 9.93 × 106 | 4000 × 4π × 10−7 | 46.5 | 7.8 | 0.46 × 103 |
| Leaf spring 65Mn | 9.93 × 106 | 129 × 4π × 10−7 | 46.5 | 7.8 | 0.46 × 103 |
| Permanent magnet N35SH | 7 × 105 | 1.05 × 4π × 10−7 | 89.55 | 7.8 | 0.50 × 103 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Q.; Liu, Z.; An, F.; Liu, B. Design of a Maglev Inertial Actuator with High Mass Power Ratio for Lateral Vibration Control of Propulsion Shafting. Actuators 2021, 10, 315. https://doi.org/10.3390/act10120315
Wu Q, Liu Z, An F, Liu B. Design of a Maglev Inertial Actuator with High Mass Power Ratio for Lateral Vibration Control of Propulsion Shafting. Actuators. 2021; 10(12):315. https://doi.org/10.3390/act10120315
Chicago/Turabian StyleWu, Qianqian, Zhihui Liu, Fengyan An, and Bilong Liu. 2021. "Design of a Maglev Inertial Actuator with High Mass Power Ratio for Lateral Vibration Control of Propulsion Shafting" Actuators 10, no. 12: 315. https://doi.org/10.3390/act10120315
APA StyleWu, Q., Liu, Z., An, F., & Liu, B. (2021). Design of a Maglev Inertial Actuator with High Mass Power Ratio for Lateral Vibration Control of Propulsion Shafting. Actuators, 10(12), 315. https://doi.org/10.3390/act10120315





