The Trade Impact of EU Tariff Margins: An Empirical Assessment
Abstract
:1. Introduction
2. A Brief Literature Review
3. Structural Gravity Model
3.1. The Gravity Equation
3.2. Econometric Approach
- -
- We could not accept the null hypothesis of homoskedasticity, and the PPML estimator is generally well behaved even when the conditional variance is far from being proportional to the conditional mean;
- -
- The fact that the dependent variable has a large proportion of zeros does not affect the performance of the estimator;
- -
- Estimation of gravity with PPML including exporter and product (HS6 digit), that enables to control for any other observable and unobservable characteristics that vary over exporters and sectors, respectively, is consistent with the ‘structural approach’ to gravity analyses.
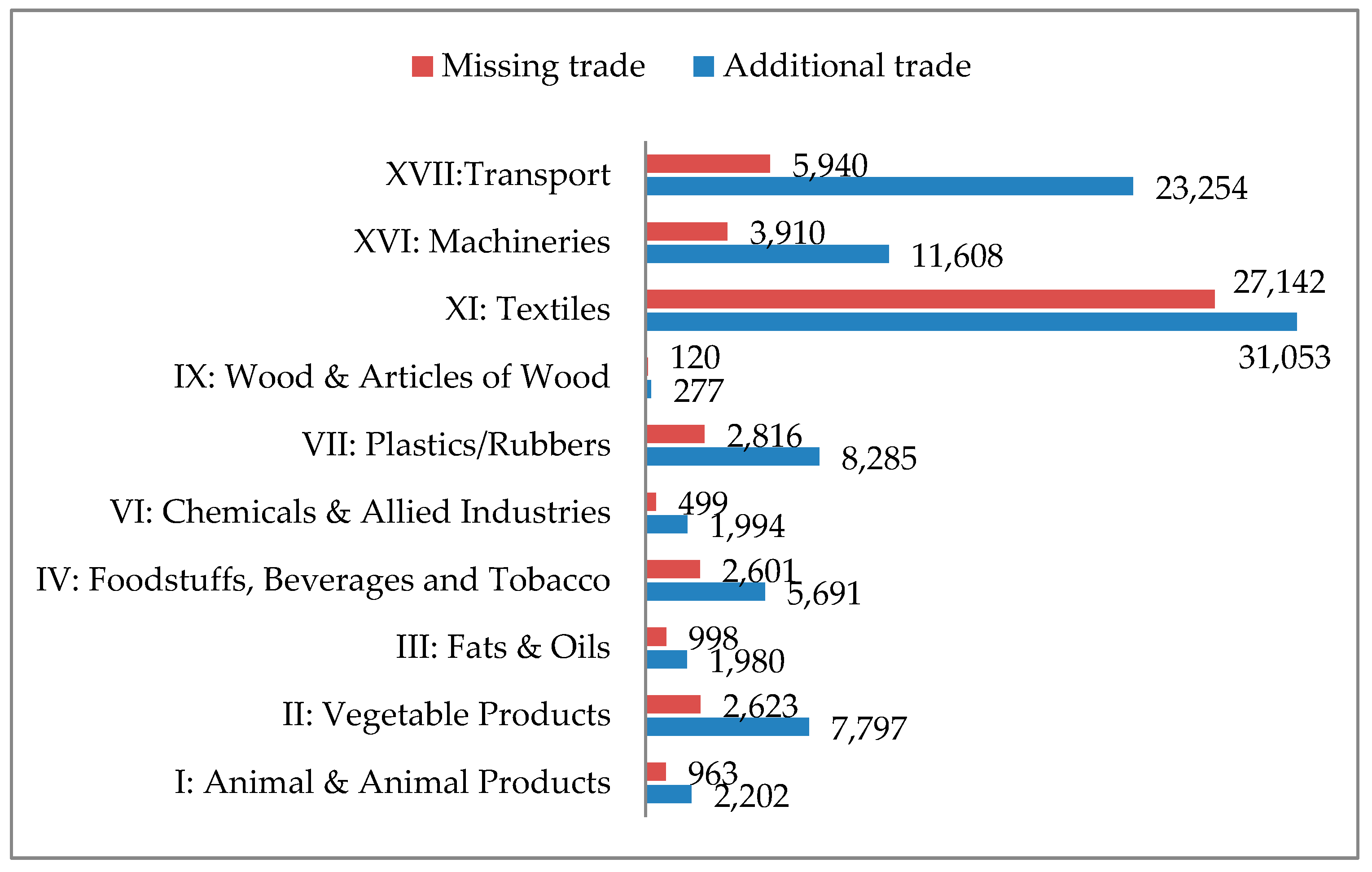
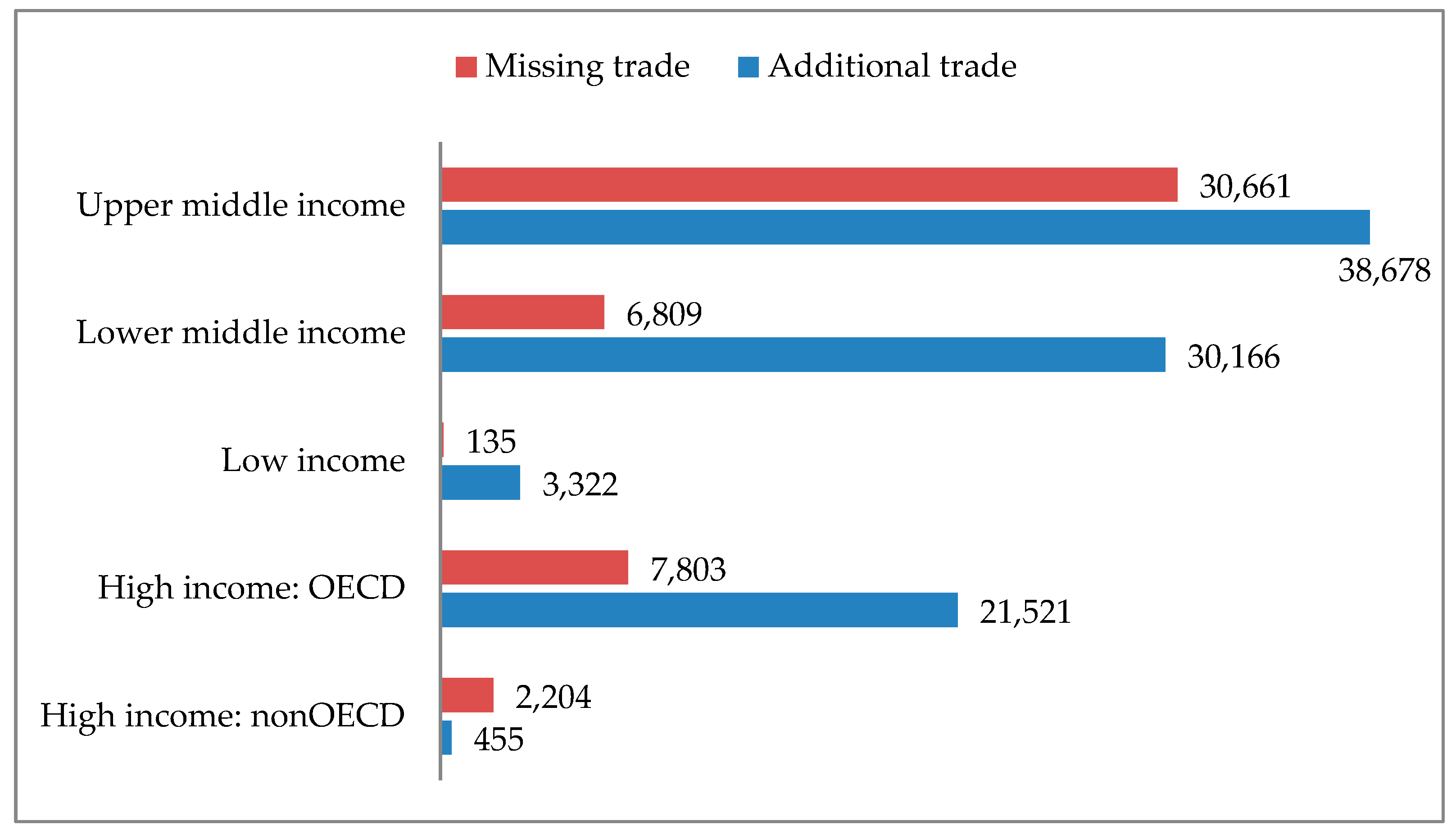
- We remove the preferential policies setting all tariffs equal to “zero” and estimate the counterfactual EU imports. The difference between the counterfactual (i.e., free-trade) and predicted flows represents the trade decrease resulting from the protectionist impact of EU tariffs. When all tariffs are removed, the numerator (i.e., the reference tariff T) decreases faster than the denominator (i.e., the bilateral tariff ti). This increases to 1 the values of the extra-EU countries facing negative margins (i.e., ). Accordingly, their export to the EU will increase at the expense of intra-EU trade flows and exports originating from extra-EU countries facing positive margins (i.e., ). The reduction in exports suffered by the preferred countries corresponds to the value of preference erosion in the case of unilateral liberalization by the EU. However, it is worth emphasizing that preference erosion is ubiquitous: Even the change of bilateral tariff will affect the CES aggregator modifying the margins of each exporter;
- We remove the preferential policies setting all bilateral tariffs equal to the MFN rate and estimate the counterfactual EU imports. In this case, the difference between the counterfactual (i.e., non-discriminatory protection) and predicted flows represents trade flows that either would or would not take place without preferences. The former represents the trade diversion (Viner 1950). As far as the latter is concerned, unlike Cipollina et al. (2017), the CES reference tariff takes into account extra- as well as intra-EU trade. Hence, the trade flows generated by the EU trade preferences include both trade creation and trade diversion.
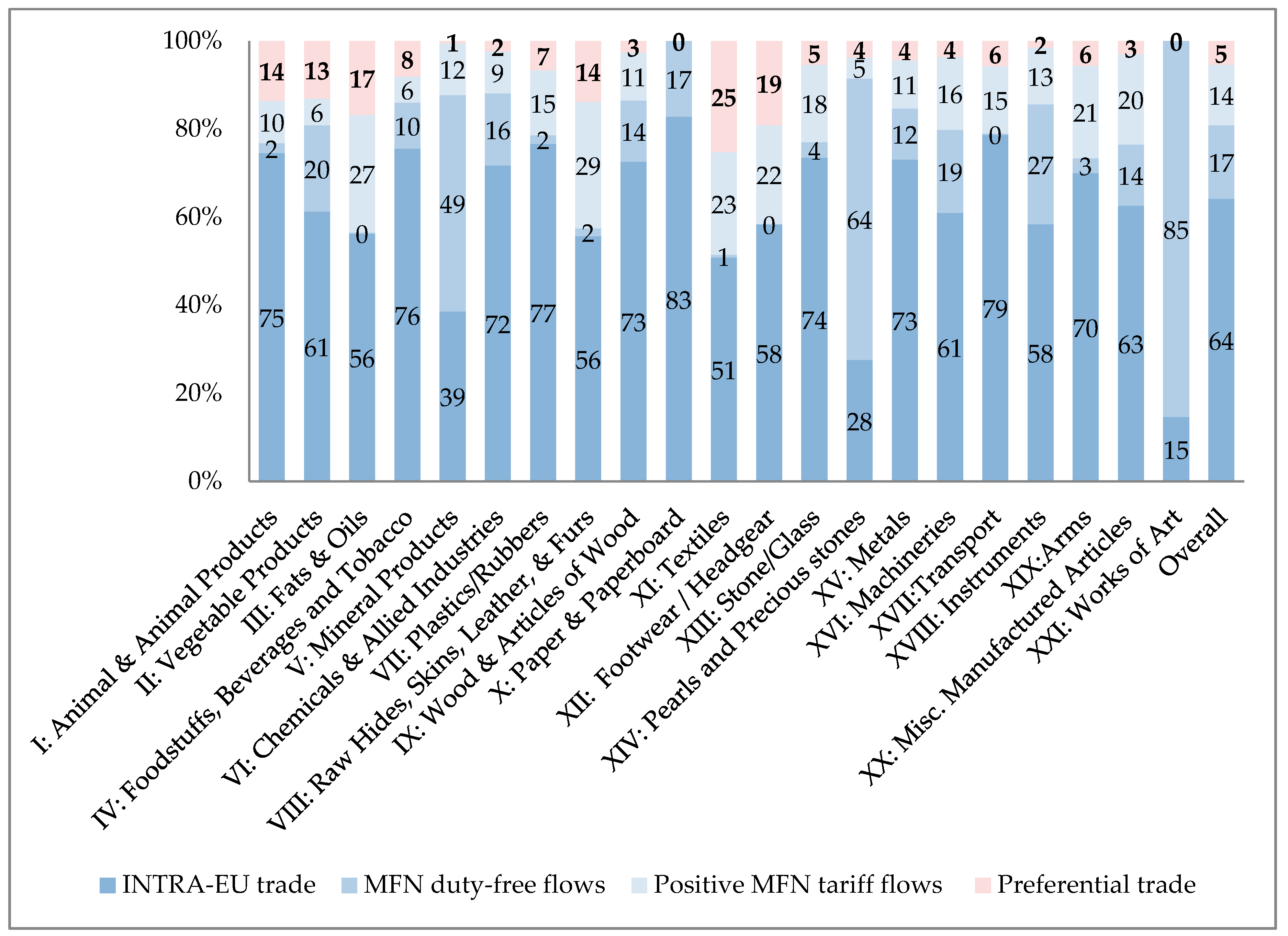
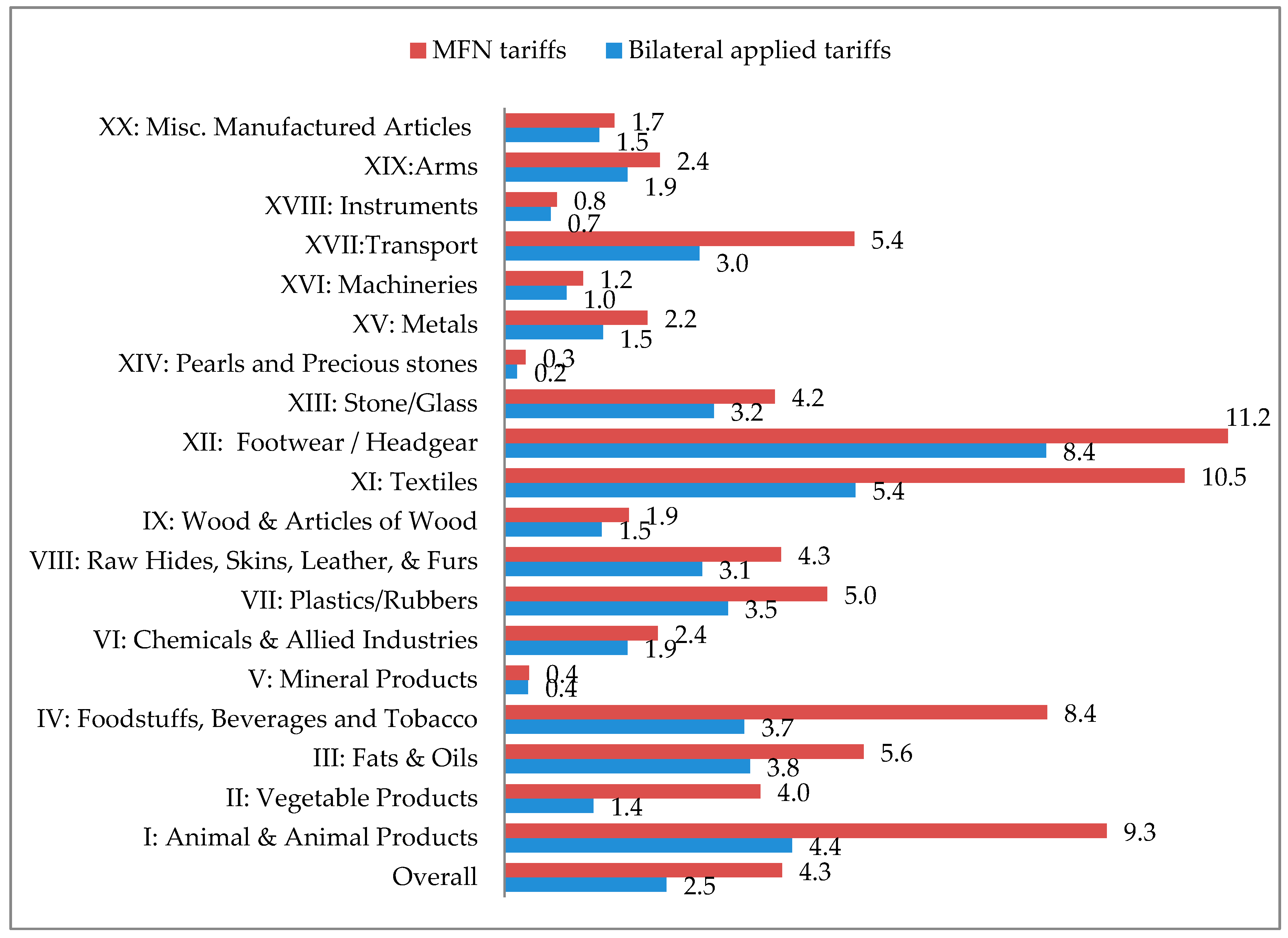
4. Data Sources
5. The Results
5.1. Elasticities of Substitutions by Sections
5.2. Trade Effect
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Anderson, James E. 1979. A Theoretical Foundation for the Gravity Equation. The American Economic Review 69: 106–16. Available online: http://www.jstor.org/stable/1802501 (accessed on 10 September 2018).
- Anderson, James E., and Eric Van Wincoop. 2003. Gravity with Gravitas: A Solution to the Border Puzzle. The American Economic Review 93: 170–92. [Google Scholar] [CrossRef]
- Baier, Scott L., and Jeffrey H. Bergstrand. 2001. The growth of world trade: tariffs, transport costs, and income similarity. Journal of International Economics 53: 1–27. [Google Scholar] [CrossRef]
- Bergstrand, Jeffrey H., Mario Larch, and Yoto V. Yotov. 2015. Economic Integration Agreements, Border Effects, and Distance Elasticities in the Gravity Equation. European Economic Review 78: 307–27. [Google Scholar] [CrossRef]
- Caliendo, Lorenzo, and Fernando Parro. 2015. Estimates of the trade and welfare effects of Nafta. Review of Economic Studies 82: 1–44. [Google Scholar] [CrossRef]
- Cipollina, Maria, David Laborde Debucquet, and Luca Salvatici. 2017. The tide that does not raise all boats: An assessment of EU preferential trade policies. Review of World Economics 153: 199–31. [Google Scholar] [CrossRef]
- Corbo, Vesna, and Chiara Osbat. 2013. Trade Adjustment in the European Union. A Structural Estimation Approach, European Central Bank Working Paper Series, no. 1535; Frankfurt: ECB Working Paper. [Google Scholar]
- Dai, Mian, Yoto V. Yotov, and Thomas Zylkin. 2014. On the Trade-Diversion Effects of Free Trade Agreements. Economic Letters 122: 321–25. [Google Scholar] [CrossRef]
- De Melo, Jaime, and Alessandro Nicita, eds. 2018. Non-Tariff Measures: Economic Assessment and Policy Options for Development. Geneva: United Nations Conference on Trade and Development. [Google Scholar]
- European Commission. 2016. Strategic Plan 2016-2020, DG Trade. Available online: https://trade.ec.europa.eu/doclib/docs/2016/august/tradoc_154919.pdf (accessed on 1 June 2019).
- Fally, Thibault. 2015. Structural Gravity and Fixed Effects. Journal of International Economics 97: 76–85. [Google Scholar] [CrossRef]
- Feenstra, Robert C., Philip Luck, Maurice Obstfeld, and Katheryn N. Russ. 2018. In Search of the Armington Elasticity. The Review of Economics and Statistics 100: 135–50. [Google Scholar] [CrossRef]
- Head, Keith, and Thierry Mayer. 2014. Gravity equations: Workhorse, toolkit, and cookbook. In Handbook of International Economics. Amsterdam: Elsevier, vol. 4, pp. 131–95. [Google Scholar]
- Heckman, James J. 1979. Sample selection bias as a specification error. Econometrica 47: 153–61. [Google Scholar] [CrossRef]
- Heid, Benedikt, Mario Larch, and Yoto Yotov. 2017. Estimating the Effects of Non-discriminatory Trade Policies within Structural Gravity Models. CESifo Working Paper no. 6735. München: CESifo Working Paper Series. [Google Scholar]
- Helpman, Elhanan, Marc Melitz, and Yona Rubinstein. 2008. Estimating trade flows: Trading partners and trading volumes. Quarterly Journal of Economics 123: 441–87. [Google Scholar] [CrossRef]
- Imbs, Jean, and Méjean Isabelle. 2009. Elasticity Optimism. International Monetary Fund Working Paper Series, 09/279; Washington: IMF. [Google Scholar]
- Lai, Huiwen, and Susan Chun Zhu. 2004. The Determinants of Bilateral Trade. Canadian Journal of Economics 37: 459–83. [Google Scholar] [CrossRef]
- Low, Patrick, Roberta Piermartini, and Jürgen Richtering. 2009. Multilateral solutions to the erosion of nonreciprocal preferences in nonagricultural market access. In Trade Preference Erosion: Measurement and Policy Response. Edited by Bernard Hoekman, Will Martin and Carlos A. Primo Braga. Washington: World Bank, pp. 219–67. [Google Scholar]
- Ornelas, Emanuel, and Marcos Ritel. 2018. The Not-So-Generalized Effects of the Generalized System of Preferences. CEP Discussion Paper No 1578. London: Centre for Economic Policy Research. [Google Scholar]
- Martin, Will, and Cong S. Pham. 2016. Estimating the Gravity Model When Zero Trade Flows are Frequent and Economically Determined. World Bank Policy Research Working Paper No. 7308. Washington: World Bank. [Google Scholar]
- Romalis, John. 2007. Nafta’s and cusfta’s impact on international trade. Review of Economics and Statistics 89: 416–35. [Google Scholar] [CrossRef]
- Silva, JMC Santos, and Silvana Tenreyro. 2006. The log of gravity. The Review of Economics and Statistics 88: 641–58. [Google Scholar] [CrossRef]
- Scoppola, Margherita, Valentina Raimondi, and Alessandro Olper. 2018. The impact of EU trade preferences on the extensive and intensive margins of agricultural and food products. In Agricultural Economics. Milwaukee: International Association of Agricultural Economists, vol. 49, pp. 251–63. [Google Scholar]
- Tinbergen, Jan J. 1962. Shaping the World Economy: Suggestions for an International Economic Policy. The Twentieth New York: Century Fund. [Google Scholar]
- Viner, Jacob. 1950. The Customs Union Issue. New York: Carnegie Endowment for International Peace. [Google Scholar]
- Yotov, Yoto V. 2012. A Simple Solution to the Distance Puzzle in International Trade. Economics Letters 117: 794–98. [Google Scholar] [CrossRef]
- Yotov, Yoto V., Roberta Piermartini, José-Antonio Monteiro, and Mario Larch. 2016. An Advanced Guide to Trade Policy Analysis: The Structural Gravity Model. Geneva: World Trade Organization. [Google Scholar]
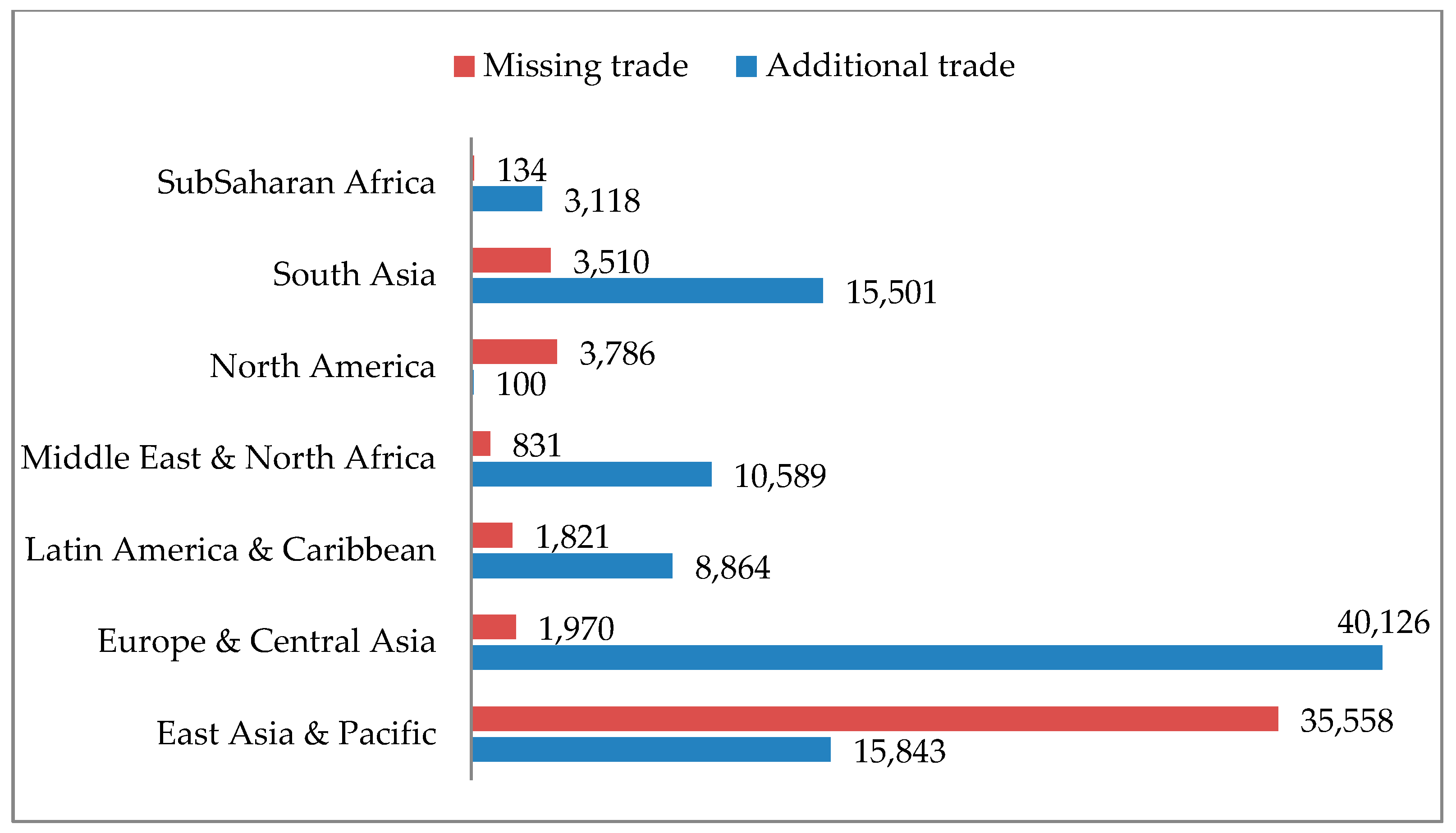
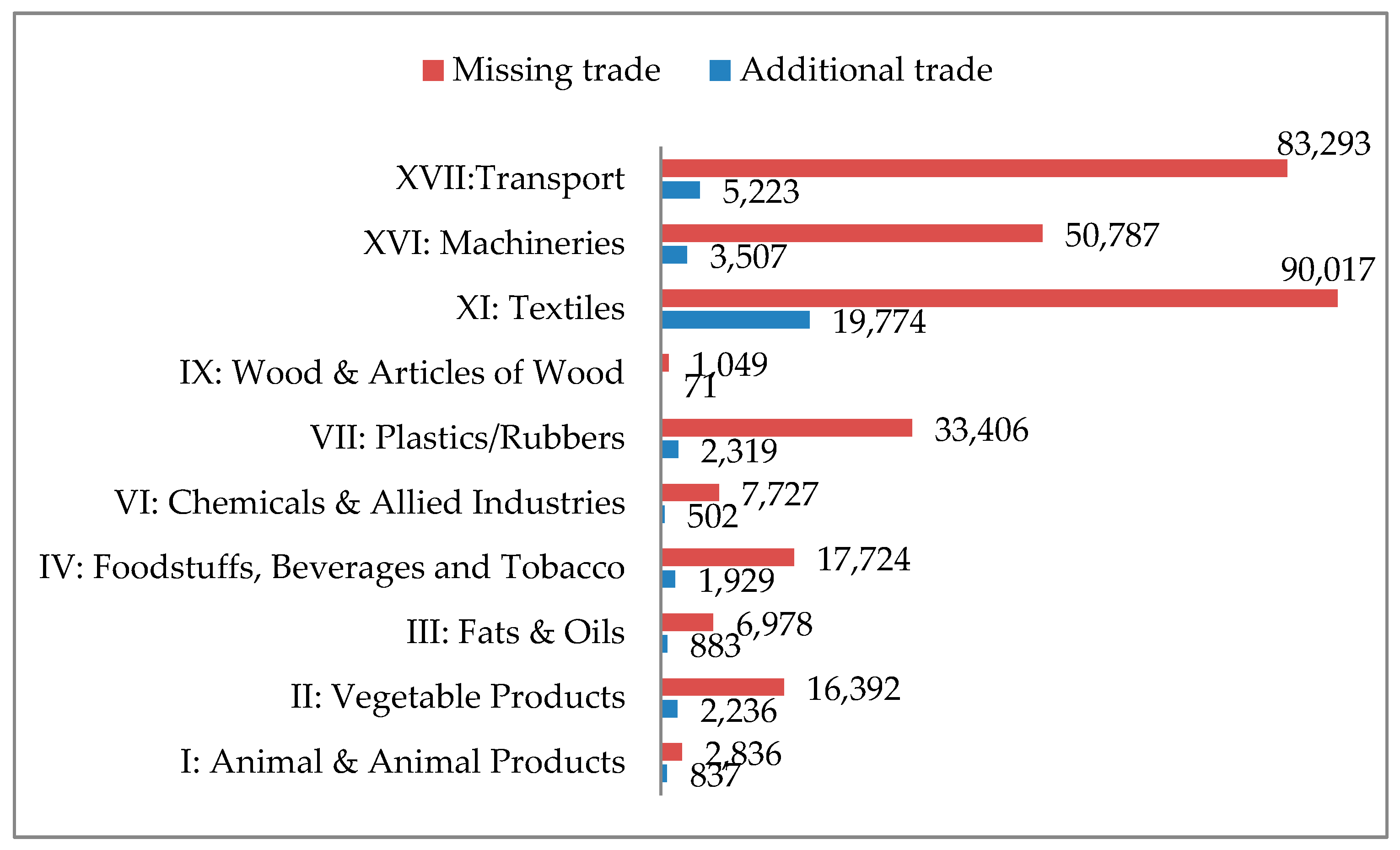
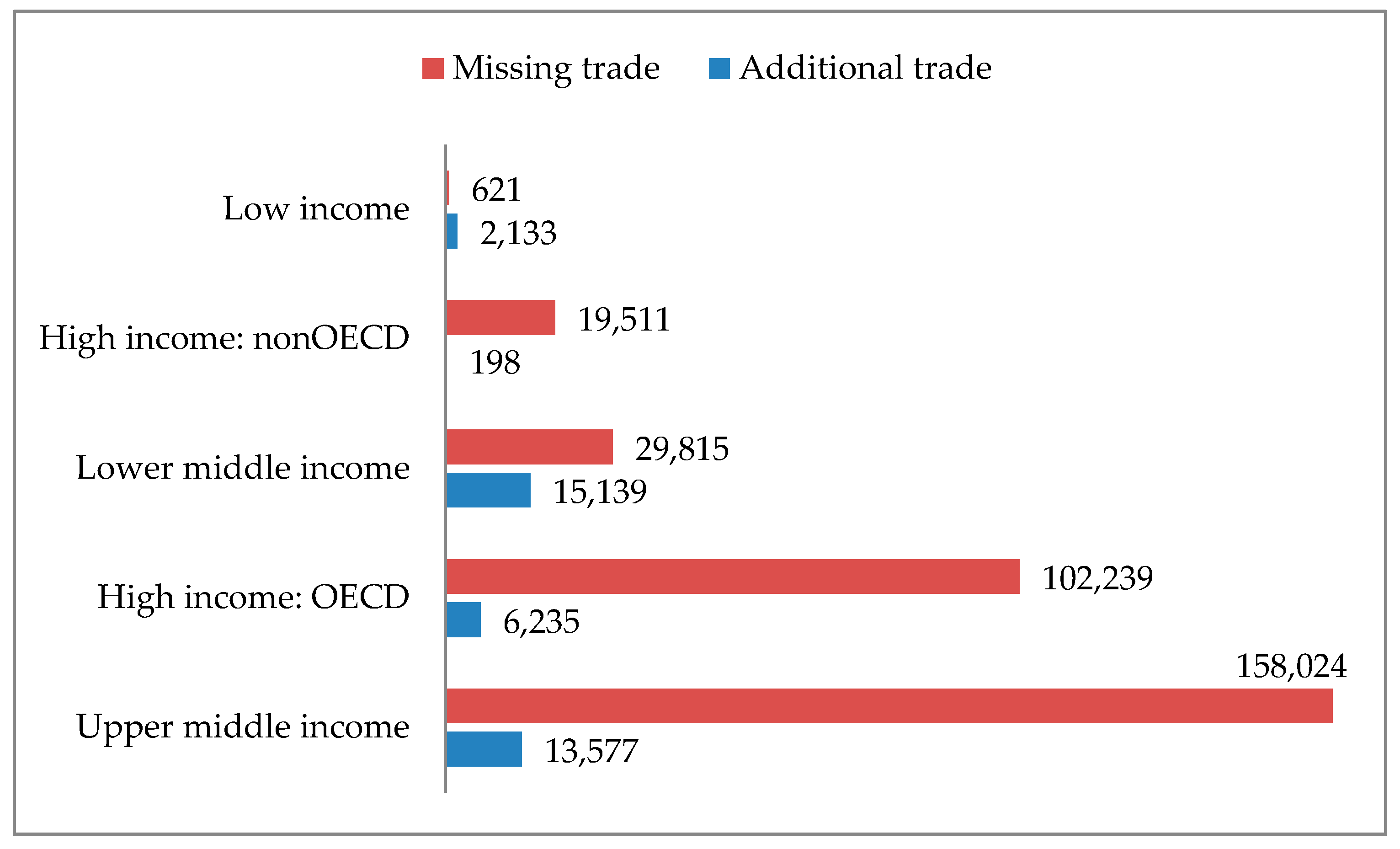
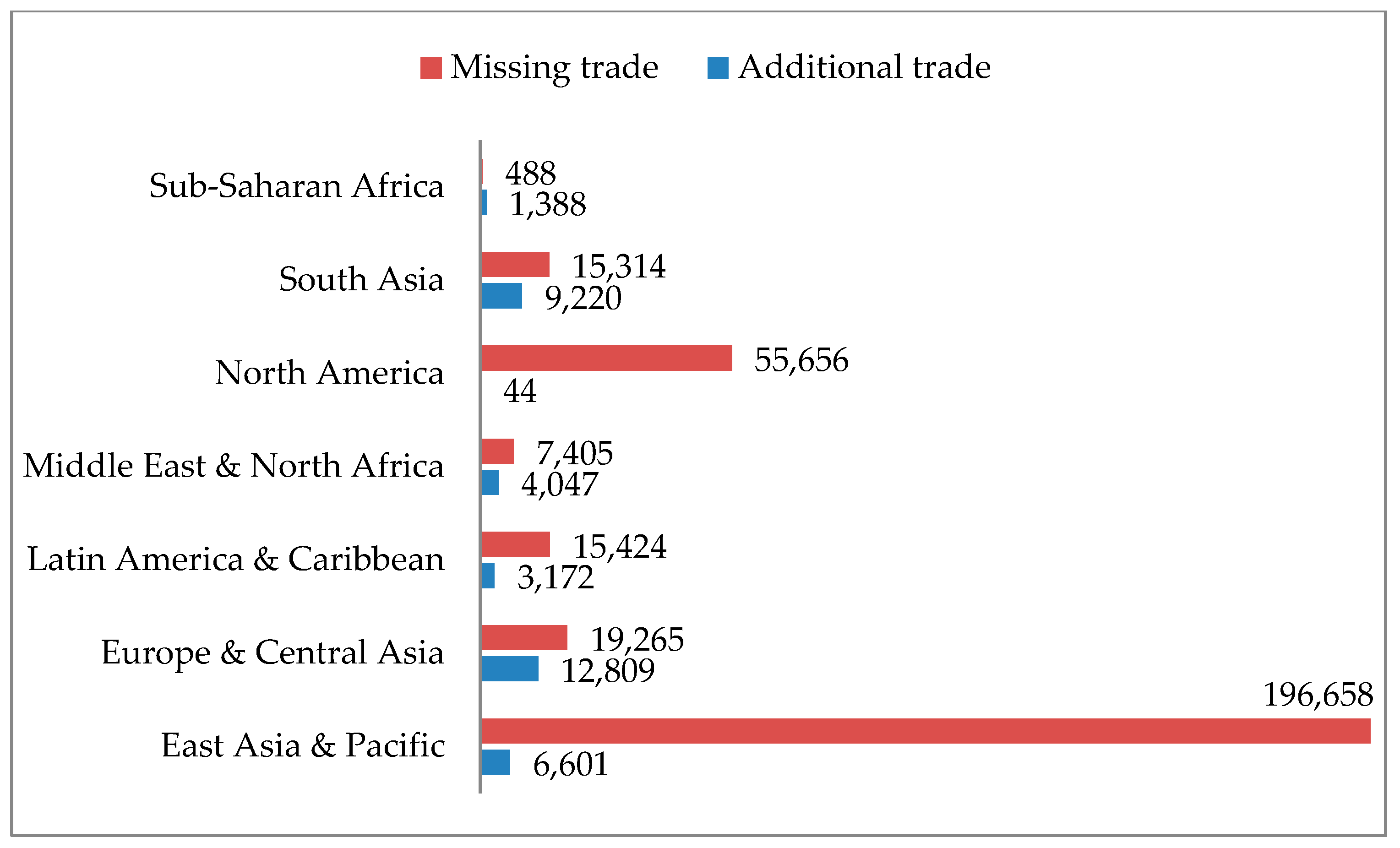
| Sections | Sigma () | N. of Obs. |
|---|---|---|
| I: Animal and Animal Products | 5.2 *** | 4.5798 |
| II: Vegetable Products | 19.5 *** | 45.545 |
| III: Fats and Oils | 20.2 *** | 7.024 |
| IV: Foodstuffs, Beverages, and Tobacco | 9.4 *** | 28.676 |
| VI: Chemicals and Allied Industries | 3.3 ** | 152.831 |
| VII: Plastics/Rubbers | 14.3 *** | 39.659 |
| VIII: Raw Hides, Skins, Leather, and Furs | −0.9 | 12.471 |
| IX: Wood and Articles of Wood | 7.3 ** | 19.865 |
| XI: Textiles | 12.8 *** | 160.859 |
| XII: Footwear/Headgear | 1.4 | 9.001 |
| XIII: Stone/Glass | −0.8 | 24.829 |
| XV: Metals | 2.5 | 105.729 |
| XVI: Machineries | 14.1 *** | 151.063 |
| XVII: Transport | 14.8 *** | 26.398 |
| XVIII: Instruments | 2.6 | 36.007 |
| XX: Misc. Manufactured Articles | 5.54 | 23.304 |
| Sections | Preferential Trade Flows | MFN Flows | ||||
|---|---|---|---|---|---|---|
| Margin > 1 | Margin = 1 | Margin < 1 | Margin > 1 | Margin = 1 | Margin < 1 | |
| Overall | 189,929 | 468 | 17,926 | 11,108 | 340,979 | 477,131 |
| (18) | (0) | (2) | (1) | (33) | (46) | |
| I: Animal and Animal Products | 6423 | 7 | 2725 | 44 | 1451 | 6515 |
| (37) | (0) | (16) | (0) | (8) | (38) | |
| II: Vegetable Products | 12,118 | 402 | 4659 | 603 | 24,783 | 8099 |
| (24) | (1) | (9) | (1) | (49) | (16) | |
| III: Fats and Oils | 3712 | 0 | 261 | 0 | 75 | 6281 |
| (36) | (0) | (3) | (1) | (61) | ||
| IV: Foodstuffs, Beverages, and Tobacco | 9458 | 59 | 1301 | 560 | 13,217 | 7927 |
| (29) | (0) | (4) | (2) | (41) | (24) | |
| VI: Chemicals and Allied Industries | 13,091 | 0 | 568 | 4482 | 82,313 | 61,502 |
| (8) | (0) | (3) | (51) | (38) | ||
| VII: Plastics/Rubbers | 16,390 | 0 | 737 | 342 | 4313 | 37,748 |
| (28) | (1) | (1) | (7) | (63) | ||
| IX: Wood and Articles of Wood | 954 | 0 | 177 | 195 | 5765 | 4878 |
| (8) | (1) | (2) | (48) | (41) | ||
| XI: Textiles | 49,836 | 0 | 7345 | 38 | 1374 | 52,759 |
| (45) | (7) | (0) | (1) | (47) | ||
| XVI: Machineries | 42,094 | 0 | 21 | 4842 | 205,692 | 195,021 |
| (9) | (0) | (1) | (46) | (44) | ||
| XVII: Transport | 35,853 | 0 | 132 | 2 | 1994 | 96,400 |
| (27) | (0) | (0) | (1) | (72) | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cipollina, M.; Salvatici, L. The Trade Impact of EU Tariff Margins: An Empirical Assessment. Soc. Sci. 2019, 8, 261. https://doi.org/10.3390/socsci8090261
Cipollina M, Salvatici L. The Trade Impact of EU Tariff Margins: An Empirical Assessment. Social Sciences. 2019; 8(9):261. https://doi.org/10.3390/socsci8090261
Chicago/Turabian StyleCipollina, Maria, and Luca Salvatici. 2019. "The Trade Impact of EU Tariff Margins: An Empirical Assessment" Social Sciences 8, no. 9: 261. https://doi.org/10.3390/socsci8090261
APA StyleCipollina, M., & Salvatici, L. (2019). The Trade Impact of EU Tariff Margins: An Empirical Assessment. Social Sciences, 8(9), 261. https://doi.org/10.3390/socsci8090261





