“Playing” with Color: How Similar Is the “Geometry” of Color Harmony in the CIELAB Color Space across Countries?
Abstract
1. Introduction
- (1)
- The projections of color choices into the CIELCh color circle;
- (2)
- The frequency and predominance of colors with specific attributes (hue angle (h°), chroma (C), and lightness (L*);
- (3)
- The “geometric” properties of the color triads plotted in the CIELAB color space.
2. Methods
2.1. Participants
2.2. Experimental Stimuli
2.3. Procedure
3. Data Analysis
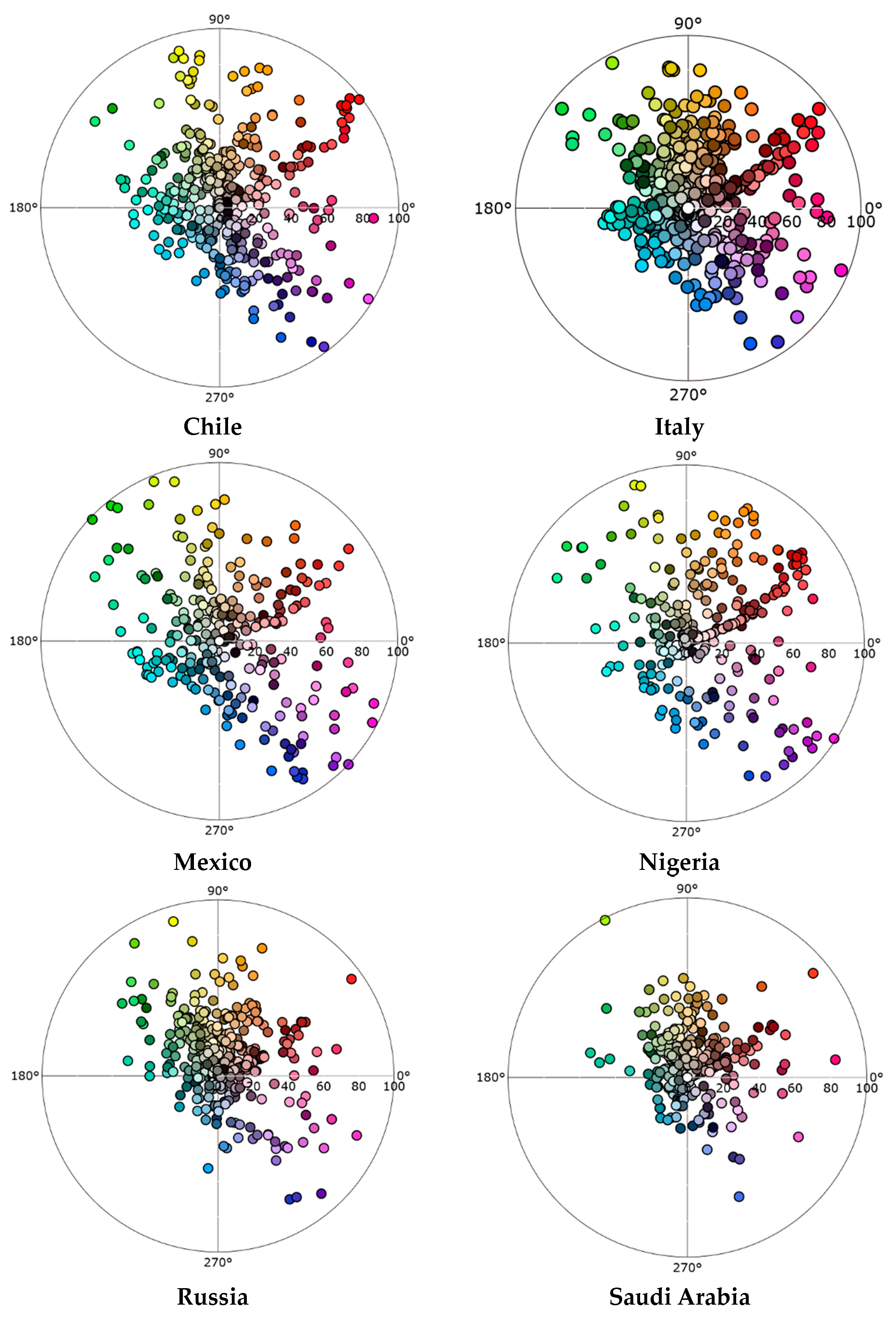
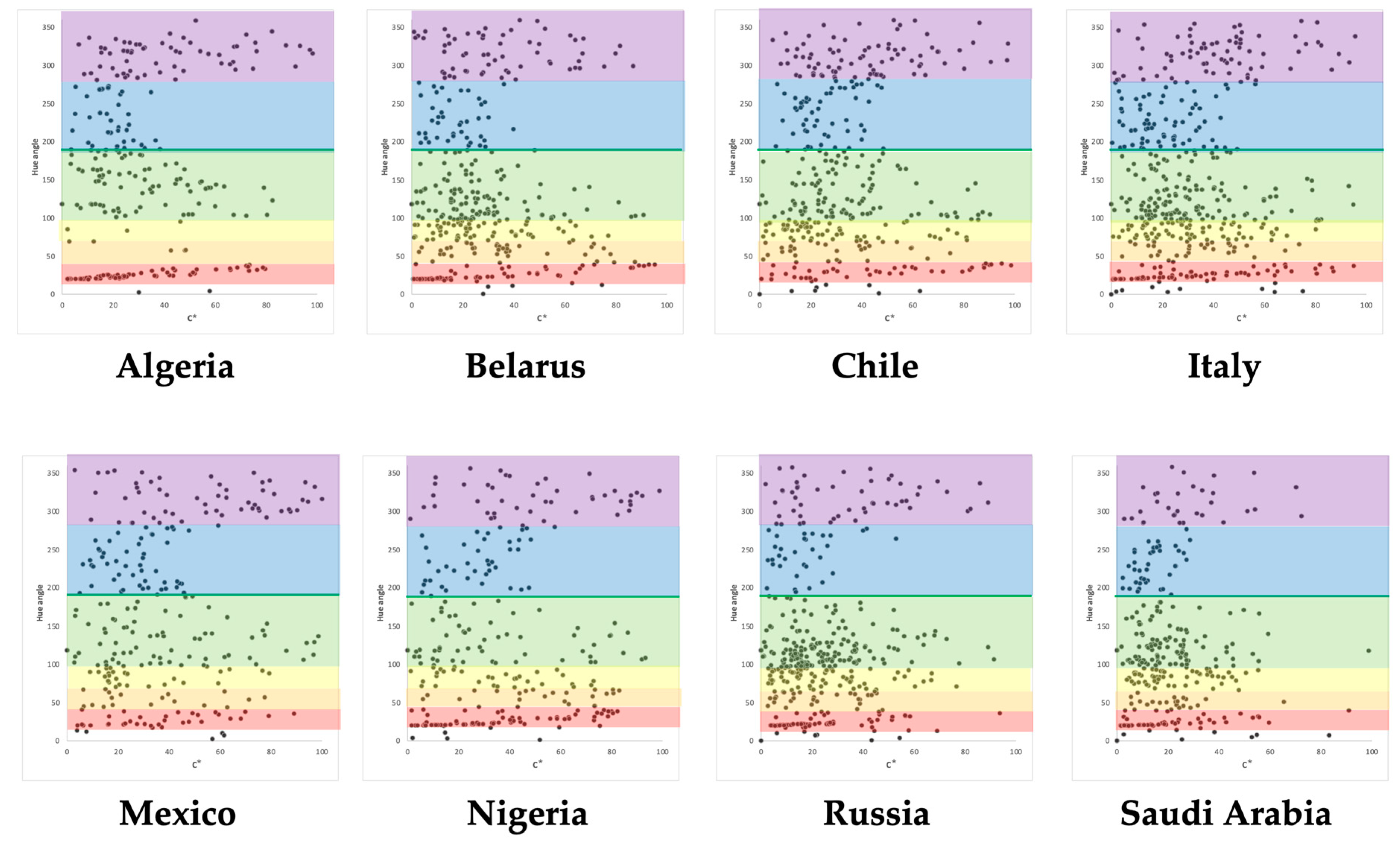
3.1. Projection of Color Choices into the CIELCh Color Circle
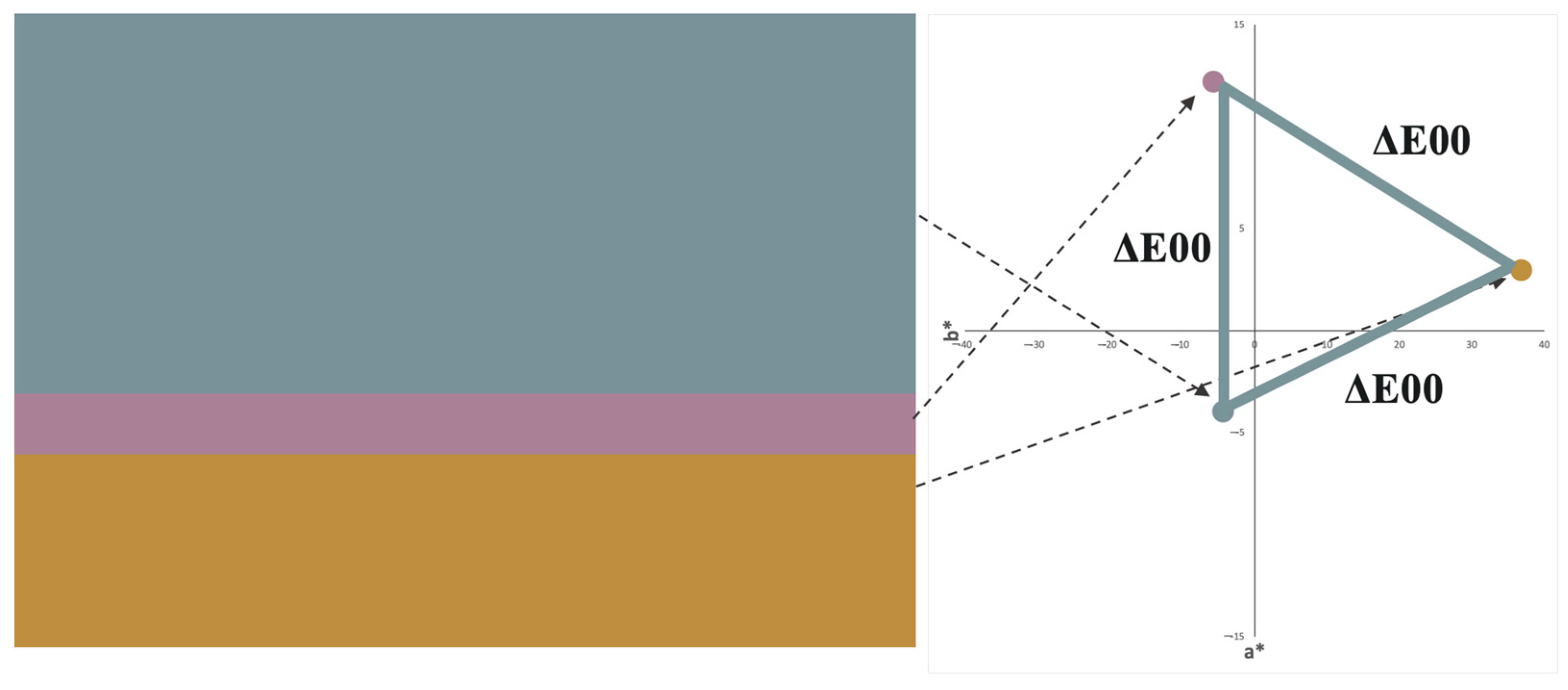
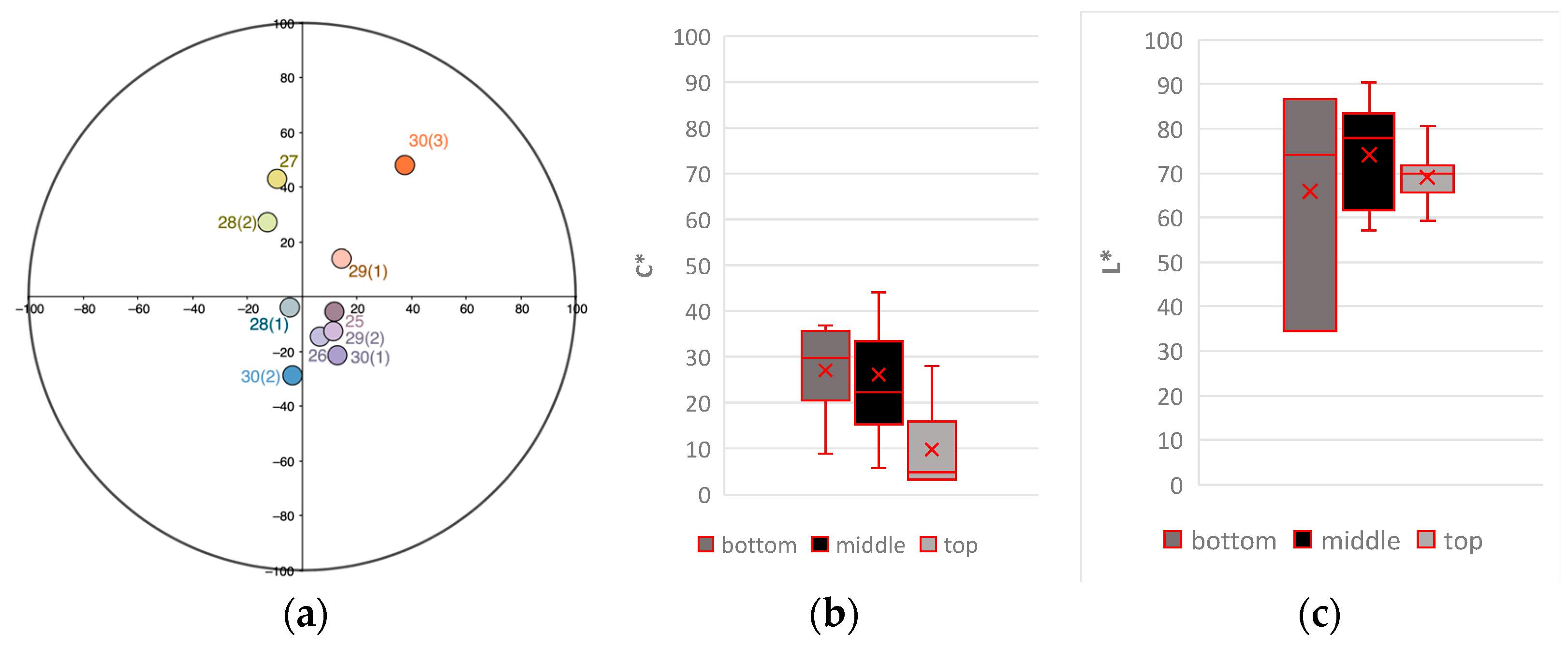
3.2. “Geometric” Properties of the Color Triads Plotted in CIELAB
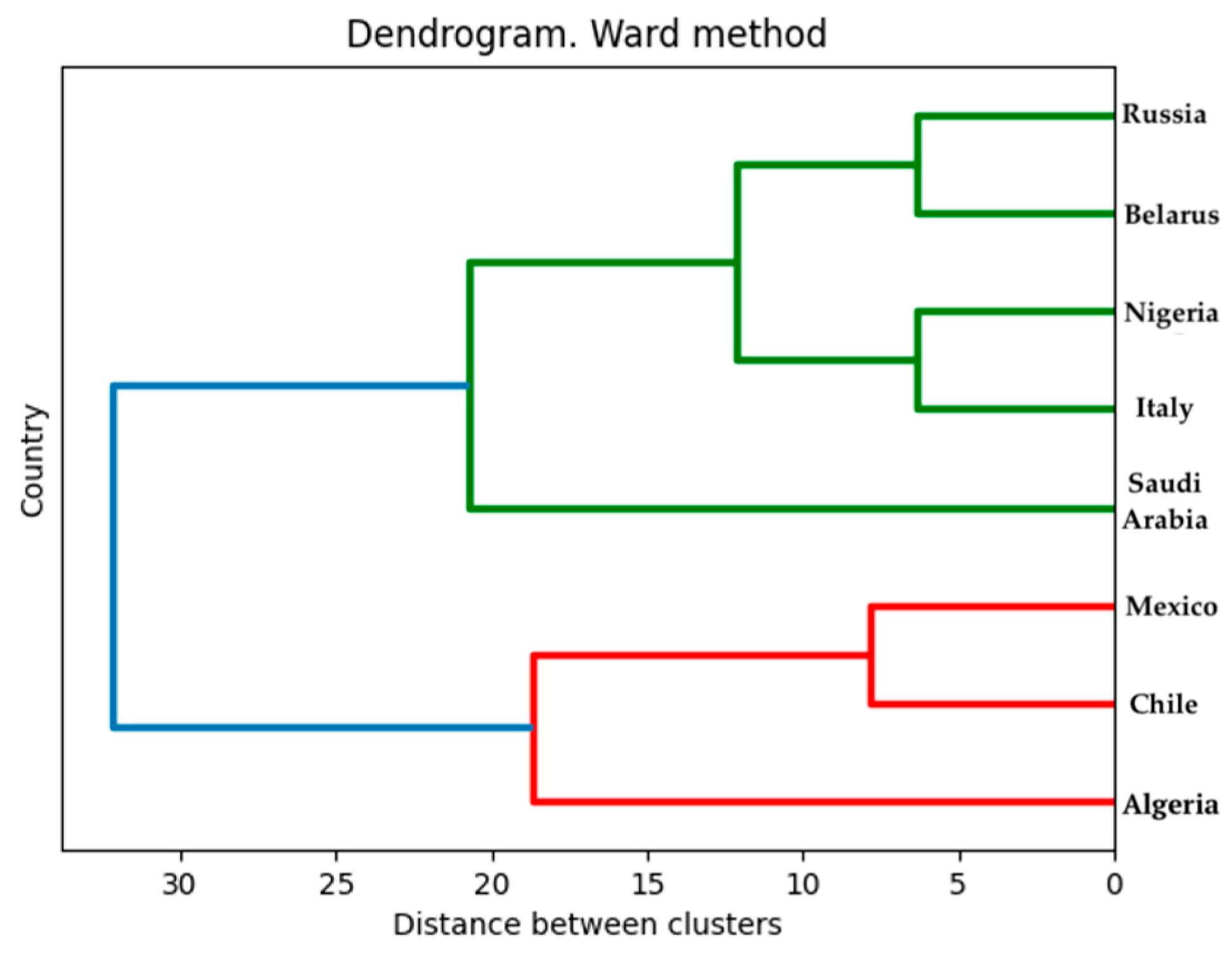
3.3. Cluster Analysis of the Data
4. Results
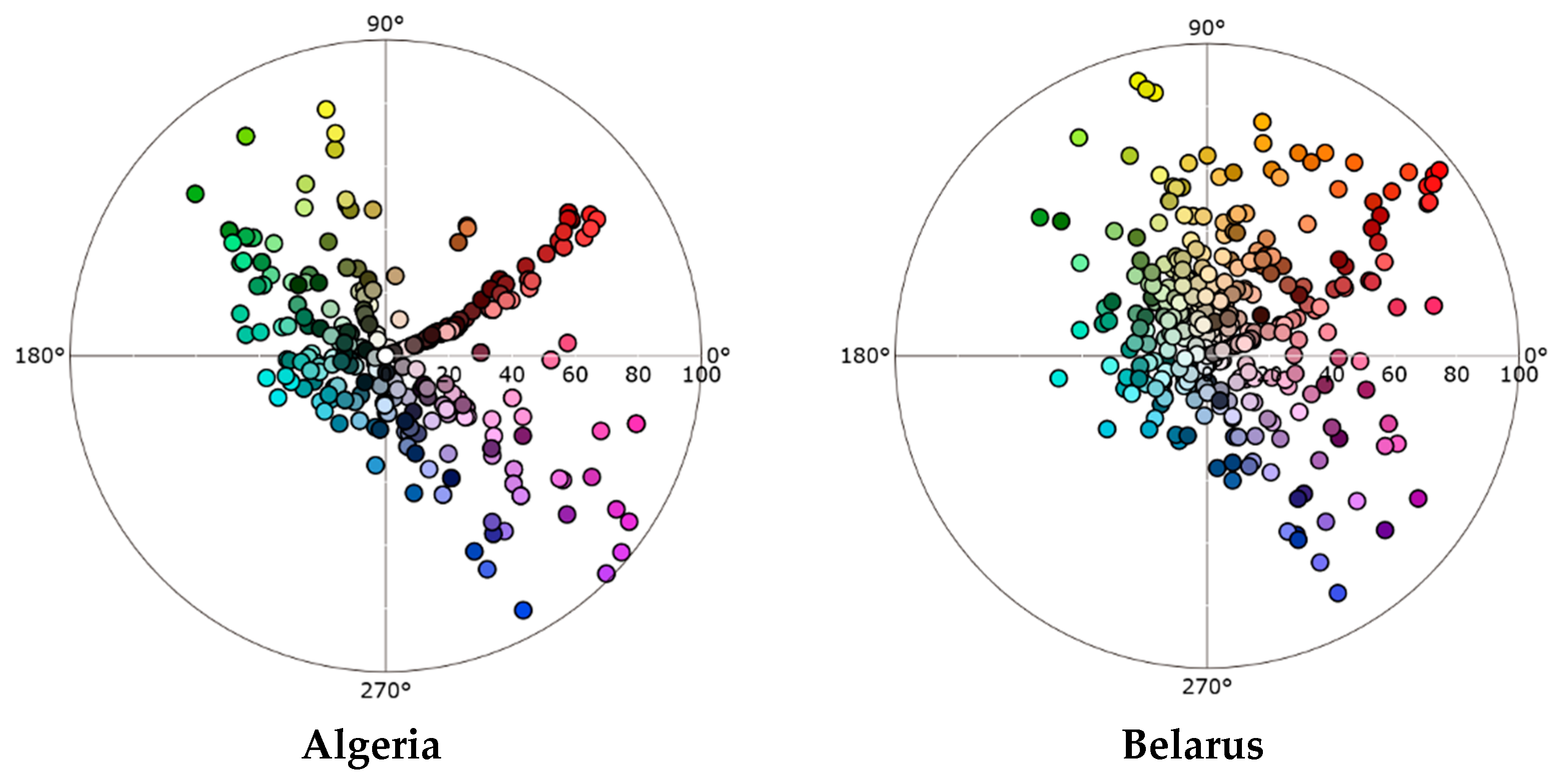
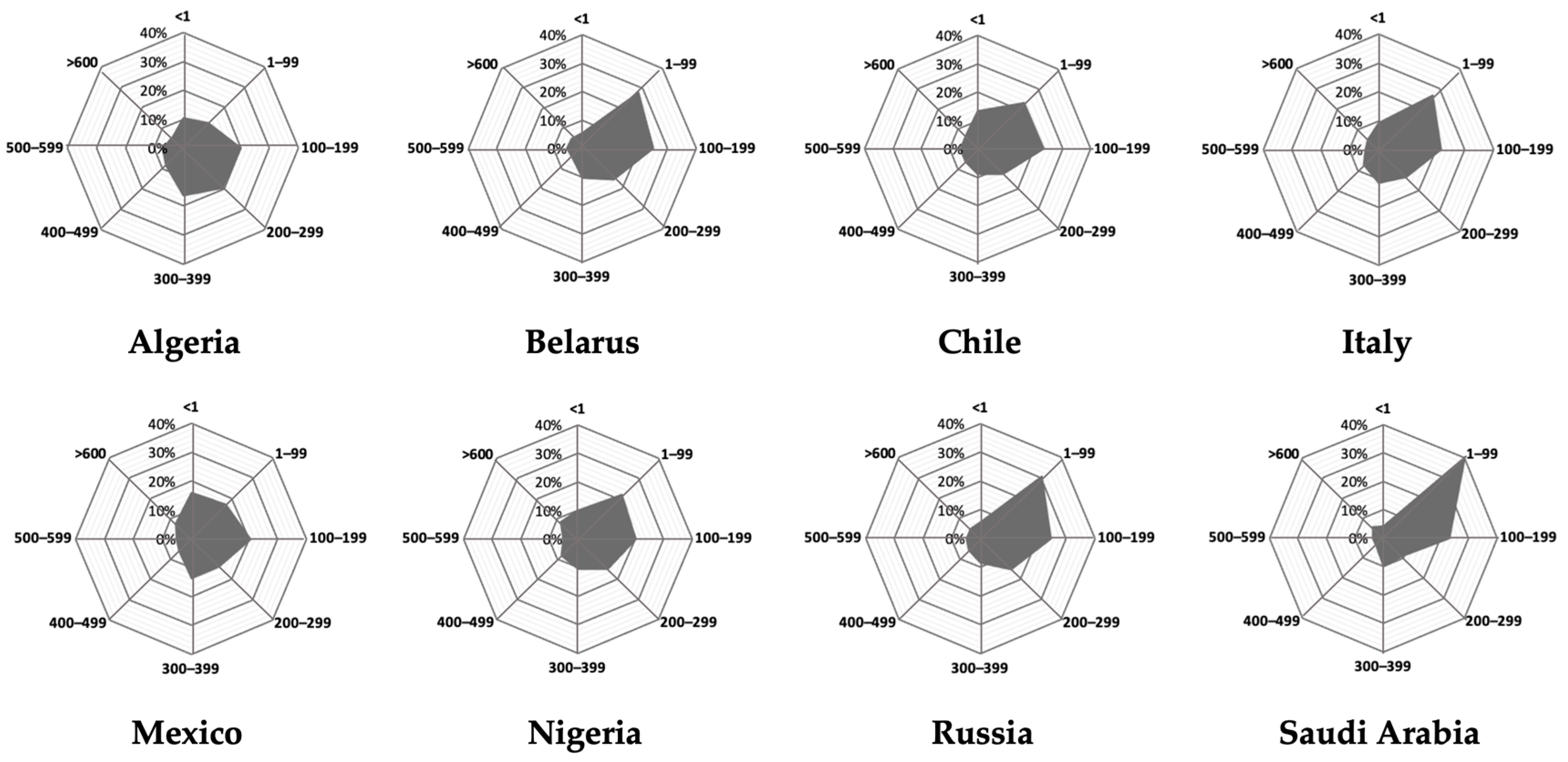
4.1. Projection of Color Choices into the CIELCh Color Circle
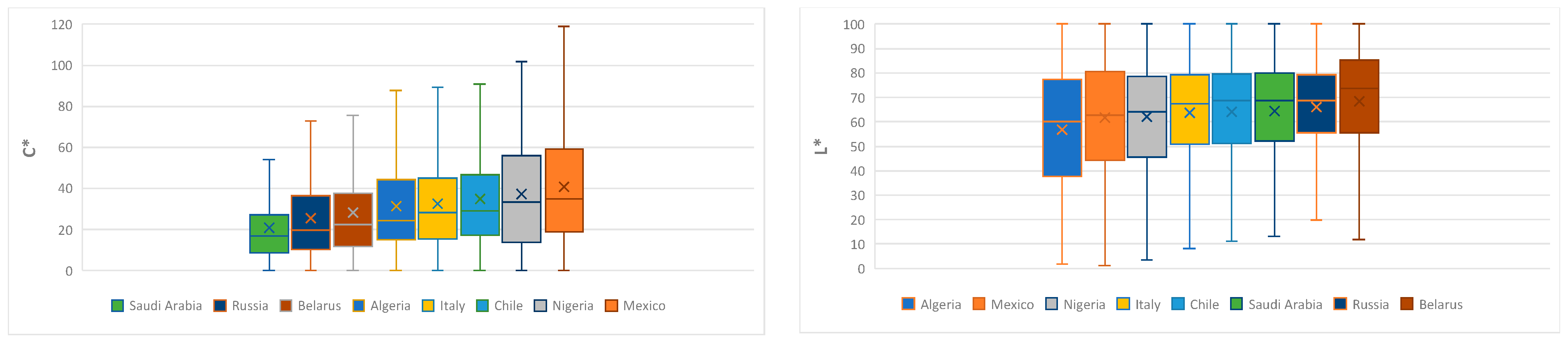
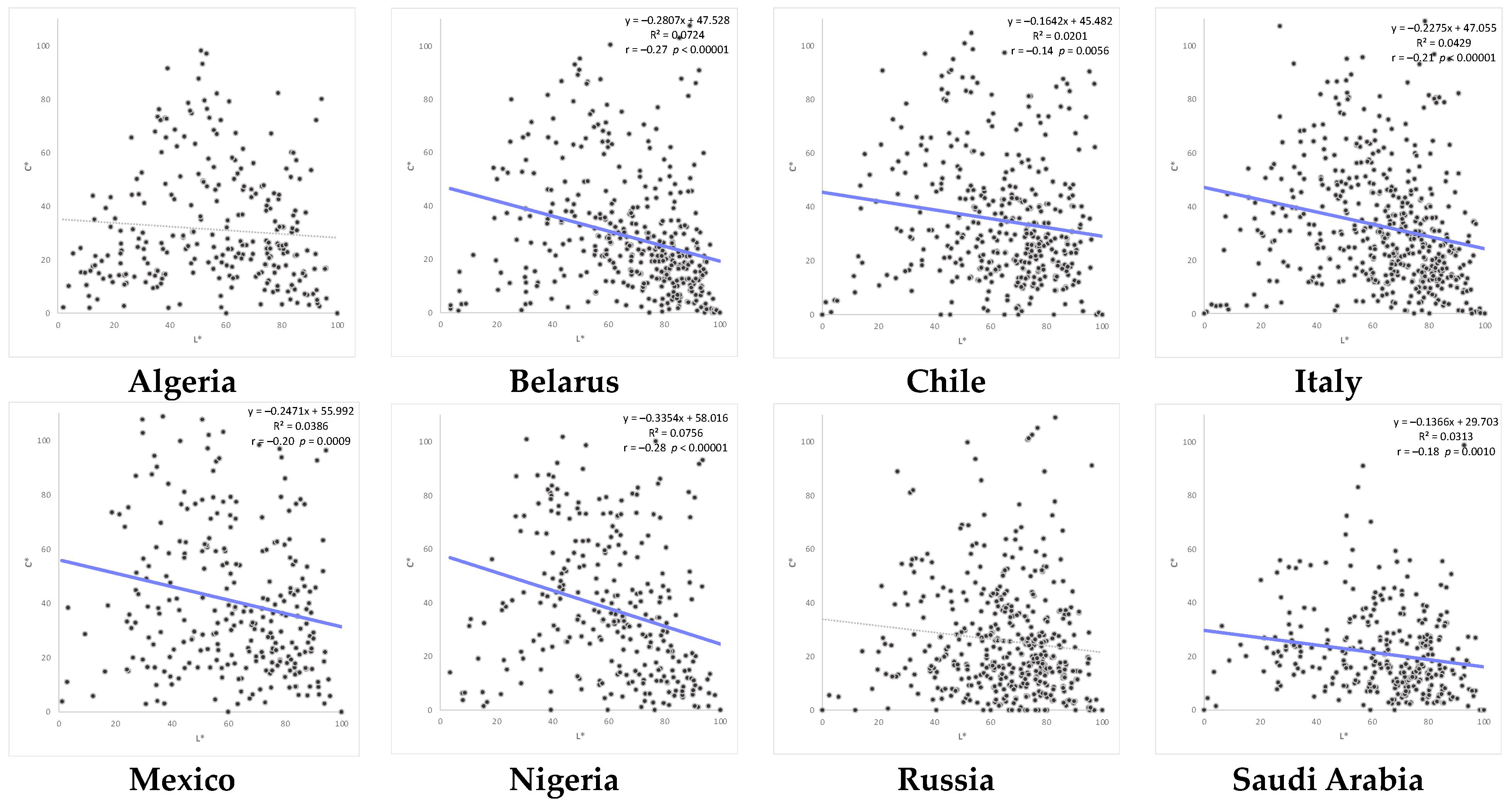
4.2. Chroma–Lightness Patterns
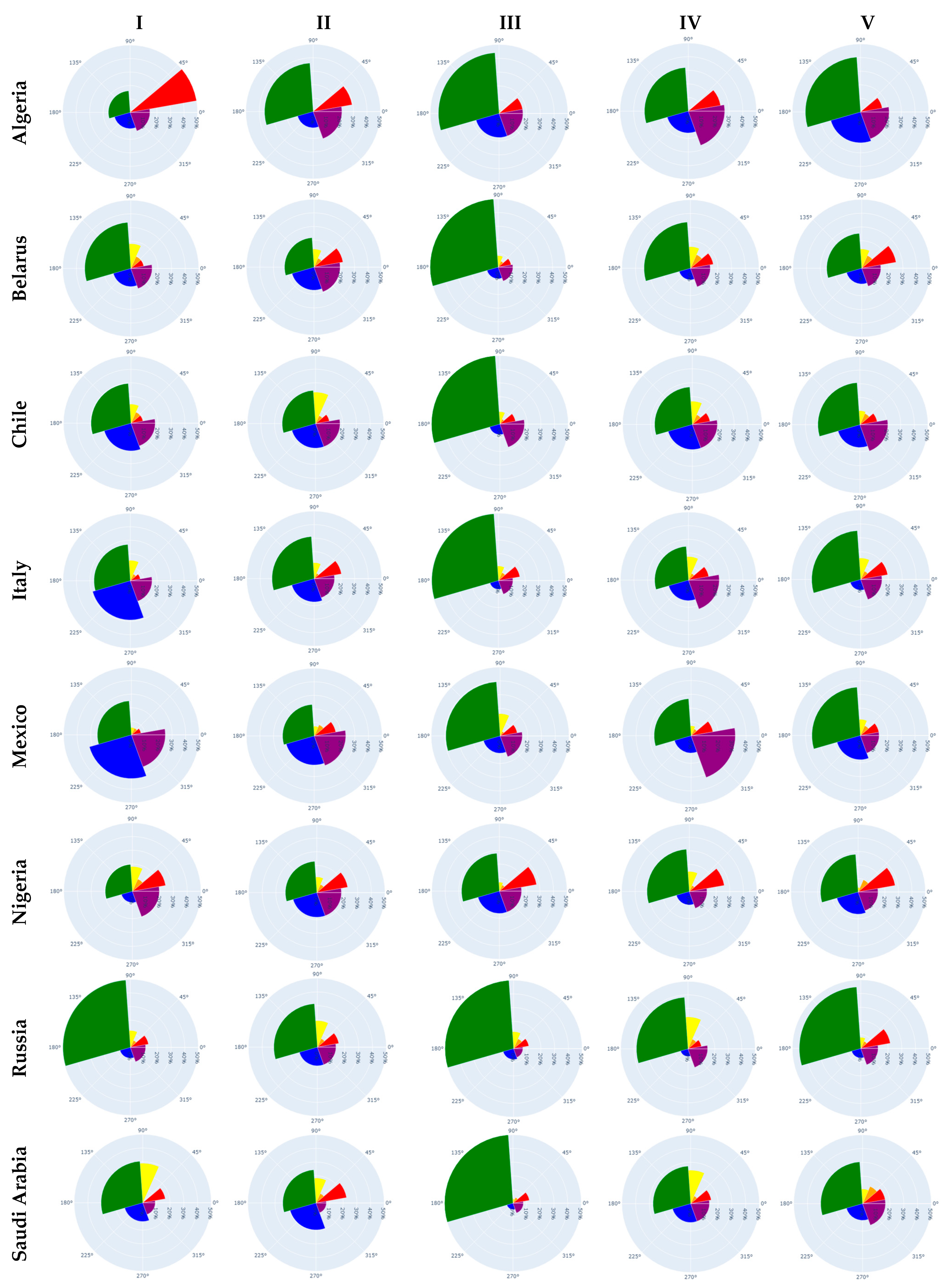
4.3. Hue Patterns
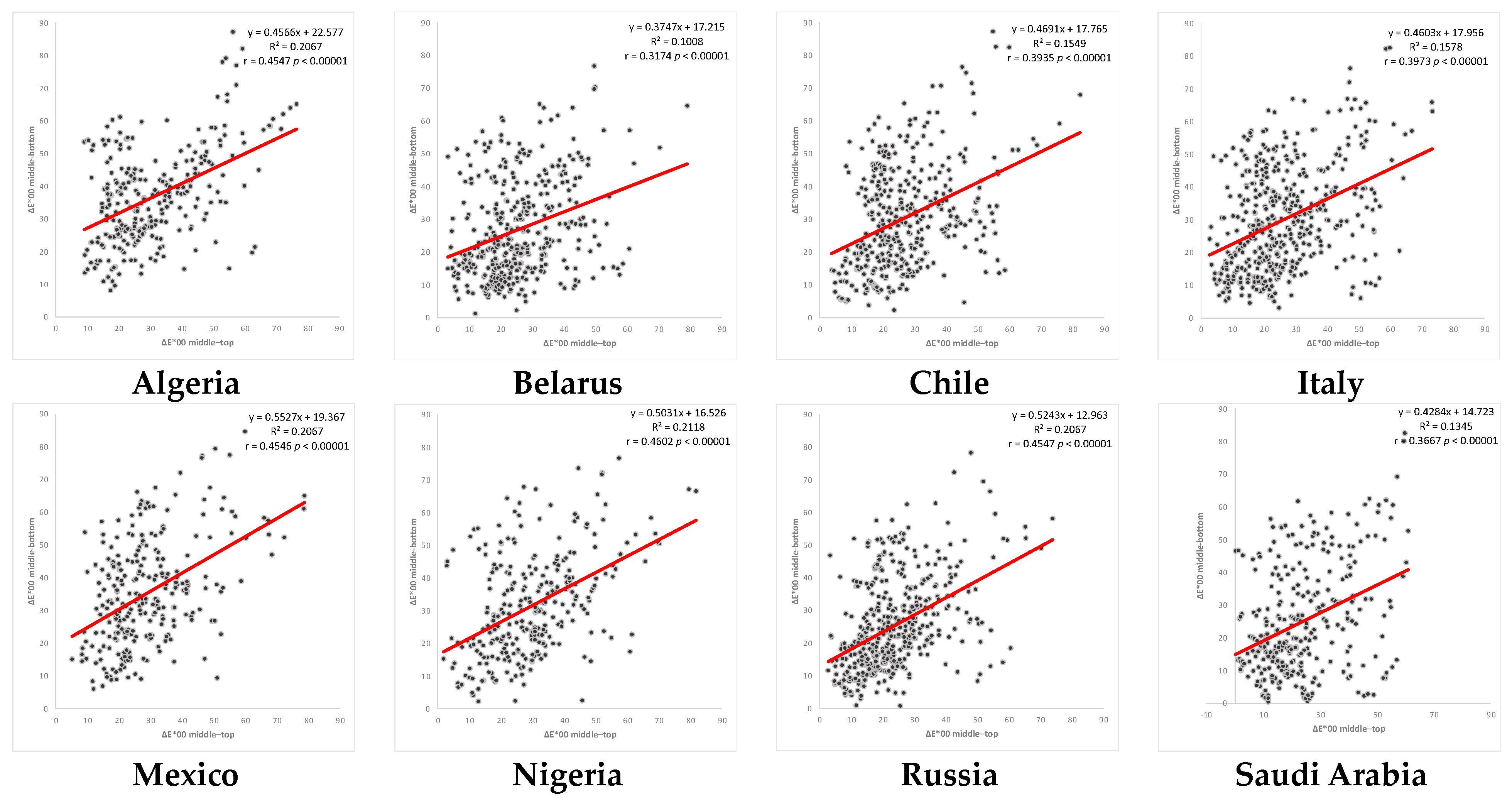
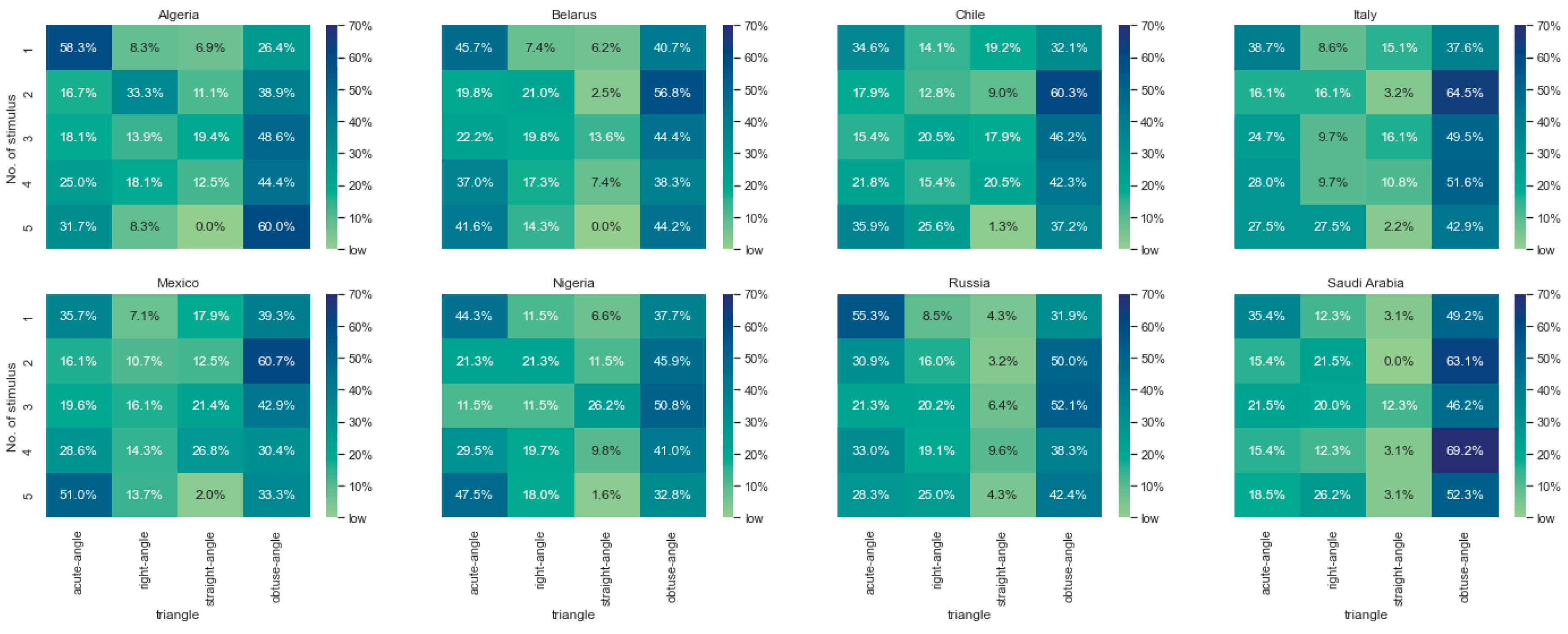
4.4. “Geometric” Properties of the Color Triads Plotted in CIELAB
4.5. Cluster Analysis of the Data
4.6. The “Geometry” of Matyushin’s Color Triads
4.7. Satisfaction with the Choice Made
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ball, Philip, and Mario Ruben. 2004. Color theory in science and art: Ostwald and the Bauhaus. Angewandte Chemie 43: 4842–46. [Google Scholar] [CrossRef] [PubMed]
- Beretta, Giordano B., and Nathan Moroney. 2012. Harmonious colors: From alchemy to science. Proceedings of the SPIE 8292: 82920I. [Google Scholar] [CrossRef]
- Braun, Doris I., and Katja Doerschner. 2019. Kandinsky or me? How free is the eye of the beholder in abstract art? i-Perception 10: 1–29. [Google Scholar] [CrossRef] [PubMed]
- Burchett, Kenneth E. 2002. Color harmony. Color Research and Application 27: 28–31. [Google Scholar] [CrossRef]
- Carter, Ellen C., János D. Schanda, Robert Hirschler, Sophie Jost, Ming R. Luo, Manuel Melgosa, Yoshi Ohno, Michael R. Pointer, Daniel C. Rich, Françoise Viénot, and et al. 2018. CIE 15:2018. Colorimetry, 4th ed. Vienna: International Commission on Illumination, CIE. [Google Scholar] [CrossRef]
- Conway, Bevil R. 2012. Color consilience: Color through the lens of art practice, history, philosophy, and neuroscience. Annals of the New York Academy of Sciences 1251: 77–94. [Google Scholar] [CrossRef]
- Cramér, Harald. 1946. Mathematical Methods of Statistics. Princeton: Princeton University Press. ISBN 0-691-08004-6. [Google Scholar]
- Die kleine Farborgel. 2023. Wilhelm Ostwald Park Museum. Available online: https://www.wilhelm-ostwald-park.de/die-kleine-farborgel (accessed on 22 October 2023).
- Griber, Yulia A. 2021. A livable colour. Project Baikal 18: 82–87. [Google Scholar] [CrossRef]
- Griber, Yulia A. 2022a. Color from within: A new vector in the studies of urban coloristics. Project Baikal 19: 144–49. Available online: https://projectbaikal.com/index.php/pb/article/view/2089 (accessed on 22 October 2023).
- Griber, Yulia A. 2022b. The “geometry” of Matyushin’s color triads: Mapping color combinations from the Reference Book of Color in CIELAB. Arts 11: 125. [Google Scholar] [CrossRef]
- Griber, Yulia A. 2023. Ekologicheskaya motivaciya cveta v gorodskoj koloristike [Ecological motivation of color in urban coloristics]. Project Baikal 4: 38–43. (In Russian). [Google Scholar]
- Griber, Yulia A., and Tatiana A. Samoilova. 2022. Impact of professional art education on harmonious colour combinations. Perspektivy nauki i obrazovania [Perspectives of Science and Education] 59: 89–105. Available online: https://pnojournal.wordpress.com/2022/11/07/griber-2/ (accessed on 22 October 2023). (In Russian).
- Griber, Yulia A., Dimitris Mylonas, and Galina V. Paramei. 2018. Objects as culture-specific referents of color terms in Russian. Color Research and Application 43: 958–75. [Google Scholar] [CrossRef]
- Hård, Anders, and Lars Sivik. 2001. A theory of colors in combination—A descriptive model related to the NCS color-order system. Color Research and Application 26: 4–28. [Google Scholar] [CrossRef]
- Hartigan, John A., and Manchek A. Wong. 1979. Algorithm as 136: A k-means clustering algorithm. Journal of the Royal Statistical Society. Series C (Applied Statistics) 28: 100–8. [Google Scholar] [CrossRef]
- Hu, Guosheng, Zhigeng Pan, Mingmin Zhang, De Chen, Wenzhen Yang, and Jian Chen. 2014. An interactive method for generating harmonious color schemes. Color Research and Application 39: 70–78. [Google Scholar] [CrossRef]
- Itten, Johannes. 1961. The Art of Color: The Subjective Experience and Objective Rationale of Color. New York: Reinhold Publishing Corporation. [Google Scholar]
- Jonauskaite, Domicele, Ahmad Abu-Akel, Nele Dael, Daniel Oberfeld, Ahmed M. Abdel-Khalek, Abdulrahman S. Al-Rasheed, Jean-Philippe Antonietti, Victoria Bogushevskaya, Amer Chamseddine, Eka Chkonia, and et al. 2020. Universal patterns in color-emotion associations are further shaped by linguistic and geographic proximity. Psychological Science 31: 1245–60. [Google Scholar] [CrossRef]
- Josserand, Mathilde, Emma Meeussen, Asifa Majid, and Dan Dediu. 2021. Environment and culture shape both the colour lexicon and the genetics of colour perception. Scientific Reports 11: 19095. [Google Scholar] [CrossRef]
- Judd, Deane, and Gunter Wyszecki. 1975. Color in Business Science, and Industry. Hoboken: John Wiley and Sons. [Google Scholar]
- Kirchner, Eric. 2023. How Itten’s color diagram fails to illustrate color mixing of paints. Optics Express 31: 25191–206. [Google Scholar] [CrossRef]
- Kirchner, Eric, and Phil Koken. 2022. The art of painting rainbows—Between color science and painter’s practice. Color Research and Application 47: 1372–91. [Google Scholar] [CrossRef]
- Koenderink, Jan, van Andrea Doorn, and Vebjørn Ekroll. 2016. Color picking: The initial 20s. ACM Transactions on Applied Perception 13: 1–26. [Google Scholar] [CrossRef]
- Lara-Alvarez, Carlos, and Tania Reyes. 2019. A geometric approach to harmonic color palette design. Color Research and Application 44: 106–14. [Google Scholar] [CrossRef]
- Lenclos, Jean-Philippe, and Dominique Lenclos. 2004. Colors of the World: The Geography of Color. New York: Norton. [Google Scholar]
- Li, Ke-Run, Ya-Qian Yang, and Zhi-Qiang Zheng. 2020. Research on color harmony of building façades. Color Research and Application 45: 105–19. [Google Scholar] [CrossRef]
- Lin, Helen, Ming R. Luo, Lindsay MacDonald, and Arthur W. S. Tarrant. 2001. A cross-cultural colour-naming study. Part III—A colour-naming model. Color Research and Application 26: 270–77. [Google Scholar] [CrossRef]
- Luo, Ming R. 2015. CIEDE2000, history, use, and performance. In Encyclopedia of Color Science and Technology. Edited by Ronnier Luo. Berlin/Heidelberg: Springer. [Google Scholar] [CrossRef]
- Matyushin, Mikhail. 2007. Spravochnik po tsvetu: Zakonomernost’ izmenyayemosti tsvetovykh sochetany [A Reference Book of Color: The Laws Governing Variability of Color Combinations]. Moscow: D. Aronov. First published 1932. Available online: https://monoskop.org/images/a/ac/Matiushin_Mikhail_Spravochnik_po_tsvetu_2007.pdf (accessed on 22 October 2023). (In Russian)
- Minah, Galen. 2008. Colour as idea: The conceptual basis for using colour in architecture and urban design. Colour: Design & Creativity 2: 1–9. Available online: https://www.aic-color.org/resources/Documents/jaic_v2_02.pdf (accessed on 22 October 2023).
- Nemcsics, Antal. 2007. Experimental determination of laws of color harmony. I. Harmony content of different scales with similar hue. Color Research and Application 32: 477–88. [Google Scholar] [CrossRef]
- O’Connor, Zena. 2010. Colour harmony revisited. Color Research and Application 35: 267–73. [Google Scholar] [CrossRef]
- Ostwald, Wilhelm. 2013. Lebenslinien: Eine Selbstbiographie. Berlin: Zenodot Verlagsgesellschaft. [Google Scholar]
- Ou, Li-Chen, Yinqiu Yuan, Tetsuya Sato, Wen-Yuan Lee, Ferenc Szabó, Suchitra Sueeprasan, and Rafael Huertas. 2018. Universal models of colour emotion and colour harmony. Color Research and Application 43: 736–48. [Google Scholar] [CrossRef]
- Palmer, Stephen E., and Karen B. Schloss. 2010. An ecological valence theory of human color preference. Proceedings of the National Academy of Sciences of the United States of America 107: 8877–82. [Google Scholar] [CrossRef] [PubMed]
- Paramei, Galina V., and Yulia A. Griber. 2019. The influence of professional color competence on color lexicon and naming pattern. European Proceedings of Social and Behavioural Sciences 73: 647–63. [Google Scholar] [CrossRef]
- Rüegg, Arthur, ed. 2006. Le Corbusier—Polychromie architecturale: Le Corbusier Farbenklaviaturen von 1931 und 1950 = Le Corbusier’s Color Keyboards from 1931 and 1959 = Les claviers de couleurs de Le Corbusier de 1931 et de 1959, 2nd ed. Basel: Birkhäuser Architecture. [Google Scholar]
- Schloss, Karen B., and Isobel A. Heck. 2017. Seasonal changes in color preferences are linked to variations in environmental colors: A longitudinal study of fall. i-Perception 8: 2041669517742177. [Google Scholar] [CrossRef]
- Schloss, Karen B., and Stephen E. Palmer. 2011. Aesthetic response to color combinations: Preference, harmony, and similarity. Attention, Perception & Psychophysics 73: 551–71. [Google Scholar] [CrossRef]
- Schwarz, Andreas. 1999. Die Lehren von der Farbenharmonie: Eine Enzyklopädie zur Geschichte und Theorie der Farbenharmonielehren. Northeim-Sudheim: Hans Hansen-Schmidt. [Google Scholar]
- Schwarz, Andreas. 2018. Farbtheorie im Kunstunterricht. Eine qualitative empirische Wirkungsforschung zum Umgang mit Farbe. Oberhausen: Athena Verlag. [Google Scholar]
- Semper, Gottfried. 1834. Vorläufige Bemerkungen über bemalte Architektur und Plastik bei den Alten. Altona: J. F. Hammerich. [Google Scholar]
- Serra, Juan, Banu Manav, and Yacine Gouaich. 2021. Assessing architectural color preference after Le Corbusier’s 1931 Salubra keyboards: A cross cultural analysis. Collection of Frontiers of Architectural Research 10: 502–15. [Google Scholar] [CrossRef]
- Serra, Juan, Jorge Llopis, Ana Torres, and Manuel Diego Giménez. 2016. Color combination criteria in Le Corbusier’s Purist architecture based on Salubra claviers from 1931. Color Research and Application 41: 85–100. [Google Scholar] [CrossRef]
- Sharma, Gaurav, Wencheng Wu, and Edul N. Dalal. 2005. The CIEDE2000 color-difference formula: Implementation notes, supplementary test data, and mathematical observations. Color Research and Application 30: 21–30. [Google Scholar] [CrossRef]
- Szabó, Ferenc, Péter Bodrogi, and János Schanda. 2010. Experimental modeling of colour harmony. Color Research and Application 35: 34–49. [Google Scholar] [CrossRef]
- Tager, Allen, Eric Kirchner, and Elena Fedorovskaya. 2021. Computational evidence of first extensive usage of violet in the 1860s. Color Research and Application 46: 961–77. [Google Scholar] [CrossRef]
- Tillberg, Margareta. 2003. Coloured Universe and the Russian Avant-Garde. Matiushin on Colour Vision in Stalin’s Russia, 1932. Stockholm: Stockholm University. [Google Scholar]
- Wang, Shuang, Jingyu Liu, Jian Jiang, Yujian Jiang, and Jing Lan. 2022. Attribute analysis and modeling of color harmony based on multi-color feature extraction in real-life scenes. Frontiers in Psychology 13: 945951. [Google Scholar] [CrossRef] [PubMed]
- Ward, Joe H., Jr. 1963. Hierarchical grouping to optimize an objective function. Journal of the American Statistical Association 58: 236–44. [Google Scholar] [CrossRef]
- Westland, Stephen, Kevin Laycock, Vien Cheung, Phil Henry, and Forough Mahyar. 2007. Colour harmony. Colour: Design & Creativity 1: 1–15. [Google Scholar]
- Zhadova, Larisa. 2007. Cvetovaja sistema Mikhaila Matyushina [Matyushin’s color system]. In Spravochnik po tsvetu: Zakonomernost’ izmenyayemosti tsvetovykh sochetany [A Reference Book of Color: The Laws Governing Variability of Color Combinations]. Moscow: D. Aronov, pp. 5–12. Available online: https://monoskop.org/images/a/ac/Matiushin_Mikhail_Spravochnik_po_tsvetu_2007.pdf (accessed on 22 October 2023). (In Russian)
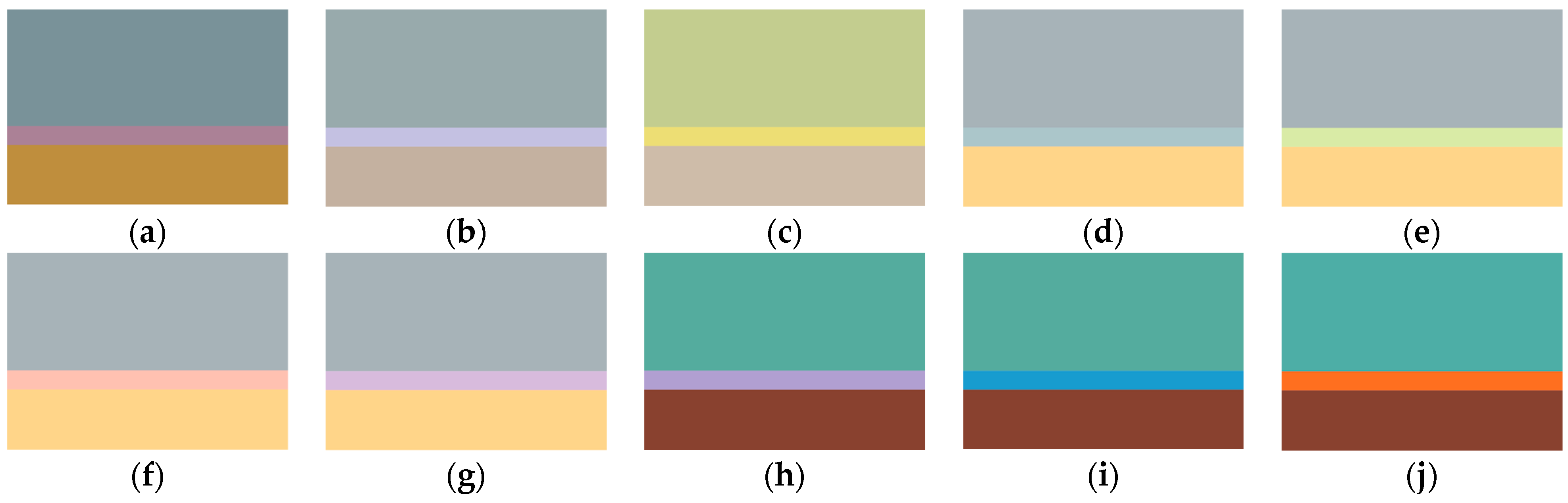
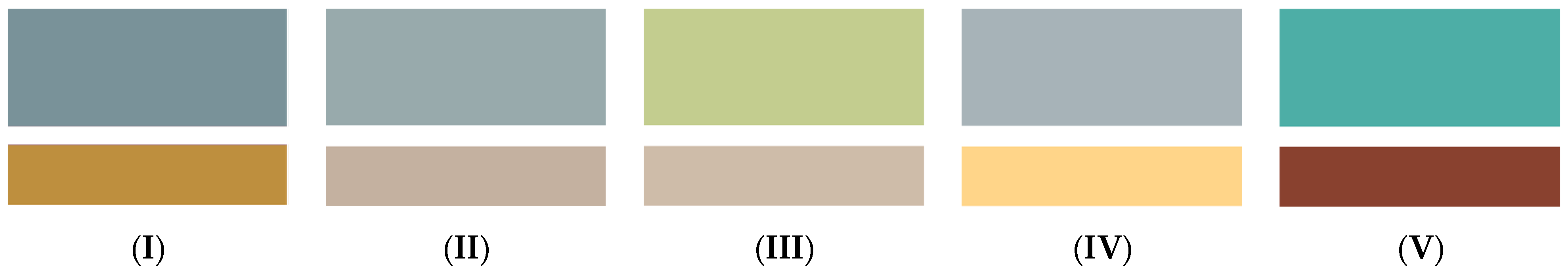
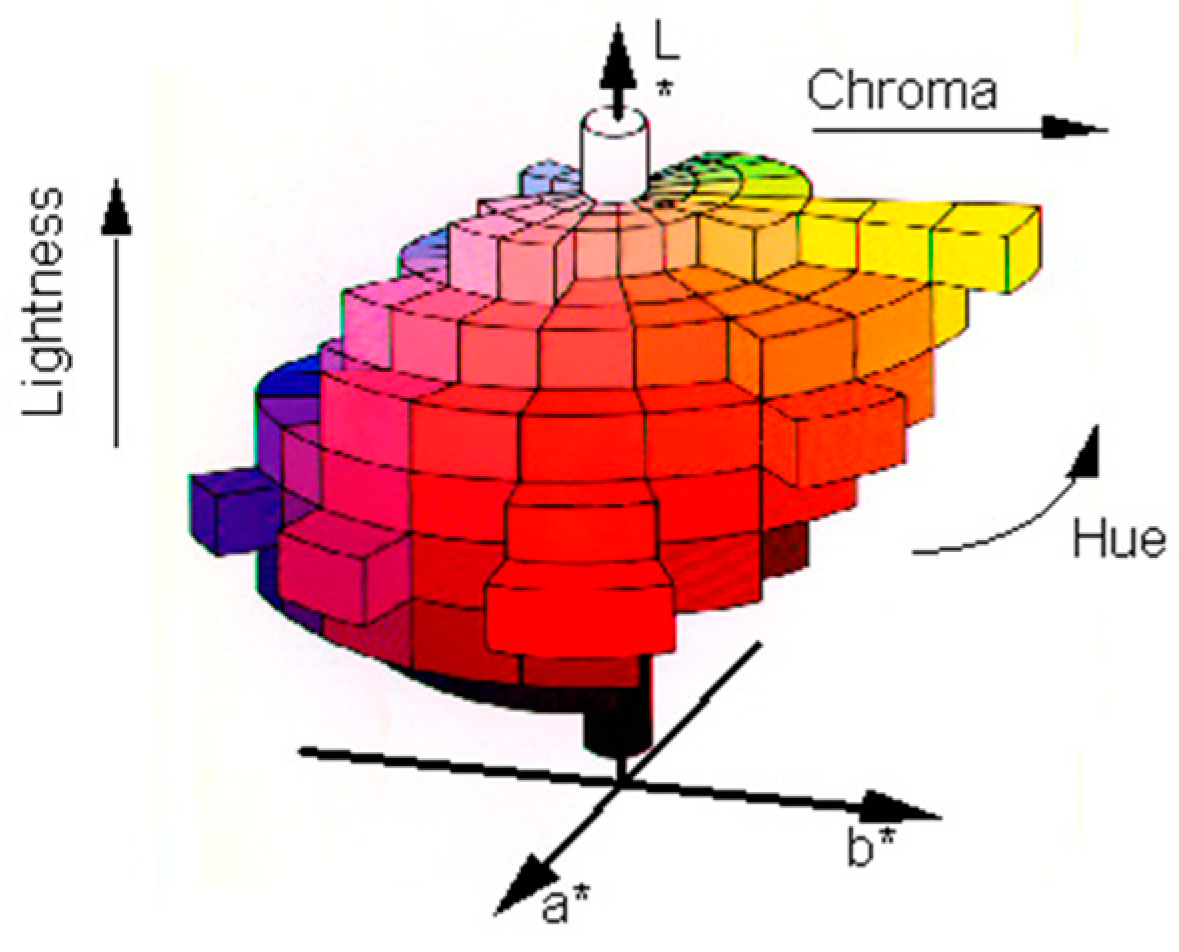



| Color | Chart | Bottom | L* | a* | b* | Top | L* | a* | b* |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 25 | 60.79 | 2.96 | 36.80 | 59.23 | −3.96 | −4.36 | ||
| 2 | 26 | 72.09 | 1.89 | 8.72 | 68.15 | −3.12 | −2.24 | ||
| 3 | 27 | 76.34 | 1.00 | 10.01 | 80.42 | −10.72 | 25.81 | ||
| 4 | 28, 29 | 86.78 | 3.41 | 35.53 | 71.78 | −1.67 | −2.89 | ||
| 5 | 30 | 34.47 | 17.09 | 16.92 | 65.77 | −15.90 | 1.26 |
| Color Category | Hue Angle |
|---|---|
| Red | 10 ≤ h° < 40 |
| Orange | 40 ≤ h° < 66 |
| Yellow | 66 ≤ h° < 94 |
| Green | 94 ≤ h° < 196 |
| Blue | 196 ≤ h° < 290 |
| Purple | 290 ≤ h° < 10 |
| Country | Degrees of Freedom | Cramér’s V |
|---|---|---|
| Algeria | 3 | 0.233 |
| Belarus | 3 | 0.145 |
| Chile | 3 | 0.155 |
| Italy | 3 | 0.165 |
| Mexico | 3 | 0.169 |
| Nigeria | 3 | 0.185 |
| Russia | 3 | 0.133 |
| Saudi Arabia | 3 | 0.141 |
| Total | 3 | 0.150 |
| Stimulus | Chart | Colour | L* | a* | b* | Category |
|---|---|---|---|---|---|---|
| 1 | 25 | 57.02 | 12.21 | −5.69 | Purple | |
| 2 | 26 | 78.36 | 6.56 | −14.69 | Purple | |
| 3 | 27 | 87.06 | −9.31 | 43.13 | Green | |
| 4 | 28(1) | 78.15 | −4.32 | −3.75 | Blue | |
| 4 | 28(2) | 90.35 | −12.74 | 27.16 | Green | |
| 4 | 29(1) | 77.74 | 11.54 | −12.83 | Orange | |
| 4 | 29(2) | 82.13 | 14.59 | 13.91 | Purple | |
| 5 | 30(1) | 67.31 | 12.56 | −21.20 | Purple | |
| 5 | 30(2) | 61.57 | −3.58 | −28.69 | Blue | |
| 5 | 30(3) | 61.42 | 37.41 | 48.36 | Orange |
| Stimulus | Chart | Triangle Type | t–b | m–t | m–b | Area |
|---|---|---|---|---|---|---|
| 1 | 25 | right-angled | 25.380 | 20.034 | 30.853 | 253.15 |
| 2 | 26 | right-angled | 11.847 | 16.103 | 19.770 | 95.36 |
| 3 | 27 | obtuse-angled | 13.820 | 8.775 | 17.974 | 59.05 |
| 4 | 28(1) | right-angled | 25.564 | 5.722 | 25.257 | 72.14 |
| 4 | 28(2) | acute-angled | 25.564 | 24.626 | 13.520 | 162.97 |
| 4 | 29(1) | acute-angled | 25.564 | 22.306 | 17.437 | 190.92 |
| 4 | 29(2) | obtuse-angled | 25.564 | 16.294 | 34.551 | 196.62 |
| 5 | 30(1) | acute-angled | 46.659 | 27.430 | 41.104 | 559.84 |
| 5 | 30(2) | right-angled | 46.659 | 22.032 | 42.719 | 469.41 |
| 5 | 30(3) | acute-angled | 46.659 | 39.382 | 29.705 | 581.39 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Griber, Y.A.; Samoilova, T.; Al-Rasheed, A.S.; Bogushevskaya, V.; Cordero-Jahr, E.; Delov, A.; Gouaich, Y.; Manteith, J.; Mefoh, P.; Odetti, J.V.; et al. “Playing” with Color: How Similar Is the “Geometry” of Color Harmony in the CIELAB Color Space across Countries? Arts 2024, 13, 53. https://doi.org/10.3390/arts13020053
Griber YA, Samoilova T, Al-Rasheed AS, Bogushevskaya V, Cordero-Jahr E, Delov A, Gouaich Y, Manteith J, Mefoh P, Odetti JV, et al. “Playing” with Color: How Similar Is the “Geometry” of Color Harmony in the CIELAB Color Space across Countries? Arts. 2024; 13(2):53. https://doi.org/10.3390/arts13020053
Chicago/Turabian StyleGriber, Yulia A., Tatyana Samoilova, Abdulrahman S. Al-Rasheed, Victoria Bogushevskaya, Elisa Cordero-Jahr, Alexey Delov, Yacine Gouaich, James Manteith, Philip Mefoh, Jimena Vanina Odetti, and et al. 2024. "“Playing” with Color: How Similar Is the “Geometry” of Color Harmony in the CIELAB Color Space across Countries?" Arts 13, no. 2: 53. https://doi.org/10.3390/arts13020053
APA StyleGriber, Y. A., Samoilova, T., Al-Rasheed, A. S., Bogushevskaya, V., Cordero-Jahr, E., Delov, A., Gouaich, Y., Manteith, J., Mefoh, P., Odetti, J. V., Politi, G., & Sivova, T. (2024). “Playing” with Color: How Similar Is the “Geometry” of Color Harmony in the CIELAB Color Space across Countries? Arts, 13(2), 53. https://doi.org/10.3390/arts13020053







