Multiscale Seismic Vulnerability Assessment and Retrofit of Existing Masonry Buildings
Abstract
1. Introduction
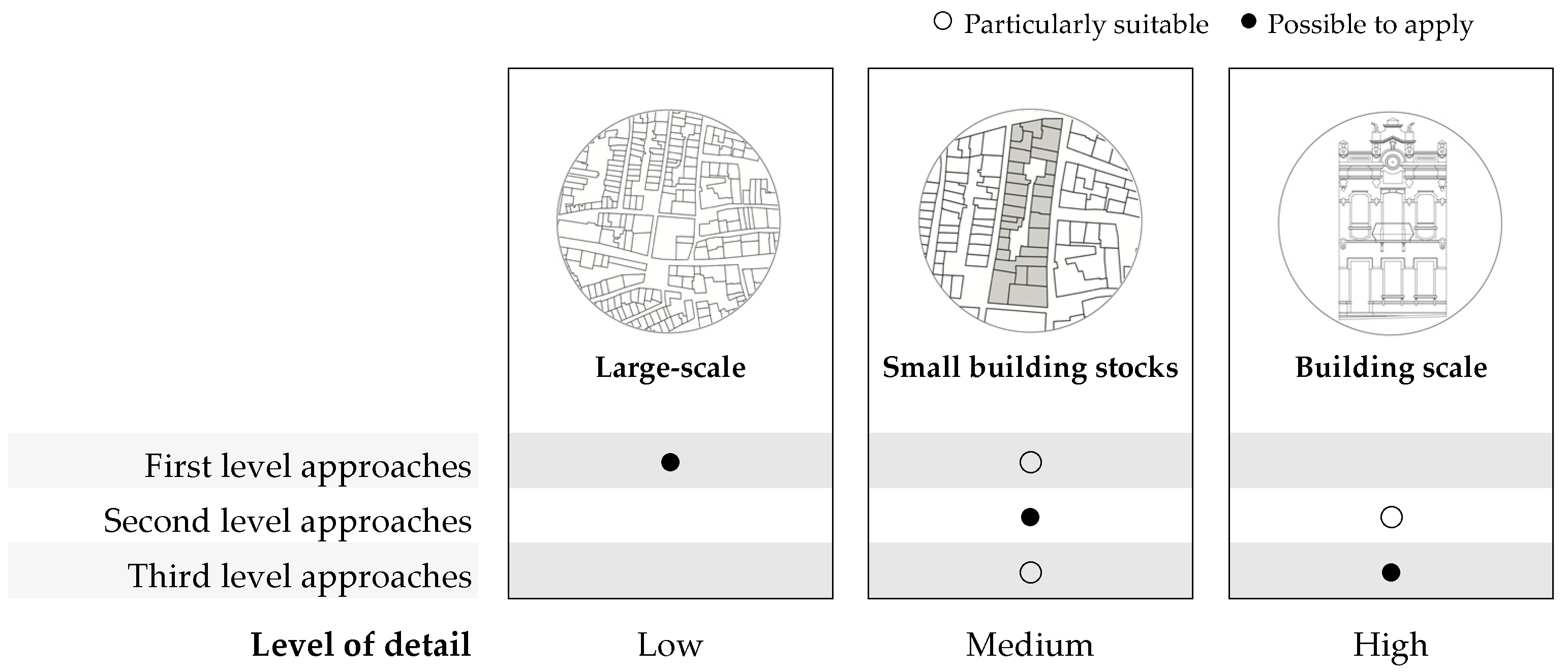
2. Seismic Vulnerability Assessment Approaches at Different Scales
3. Large-Scale Seismic Vulnerability Assessment Methods
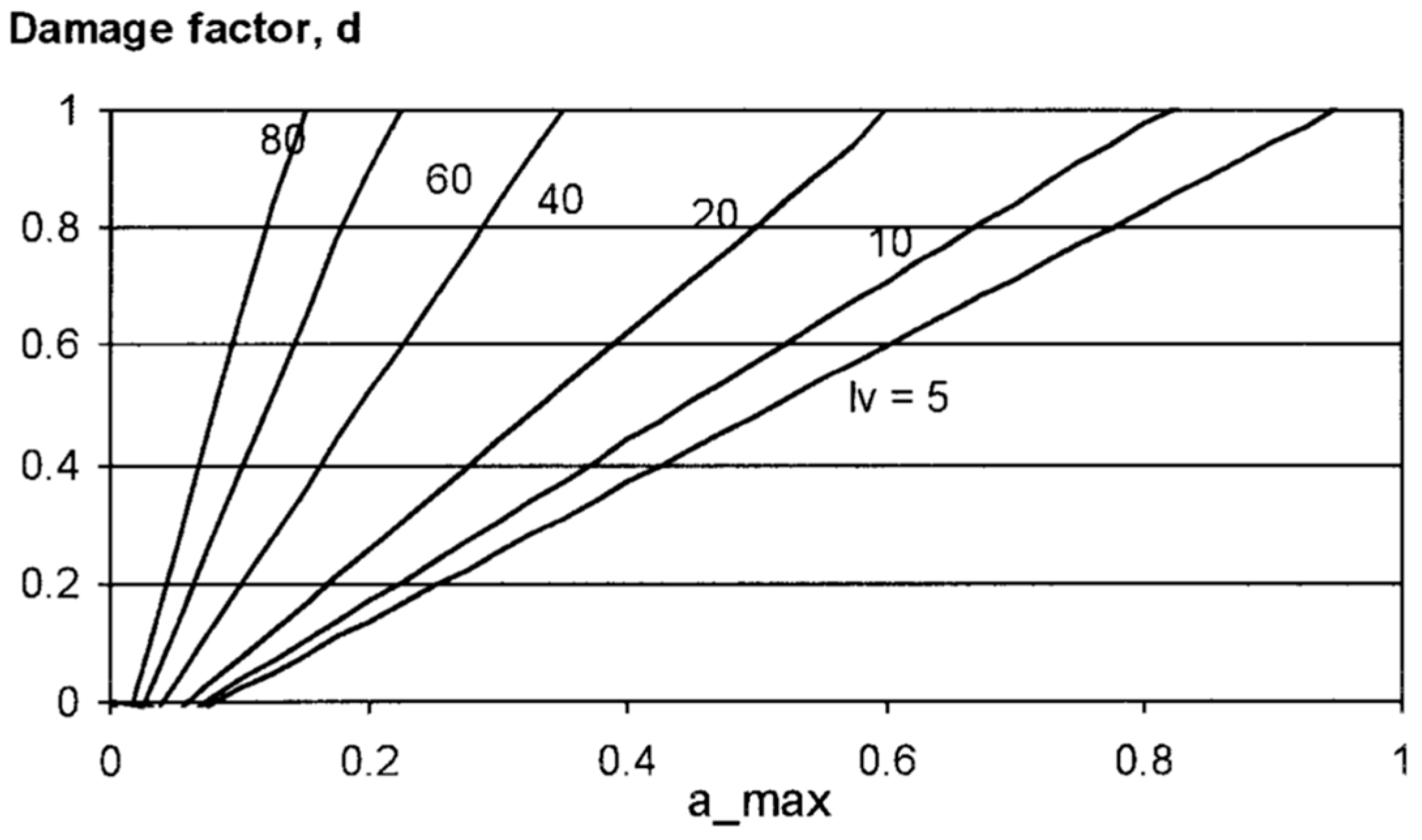
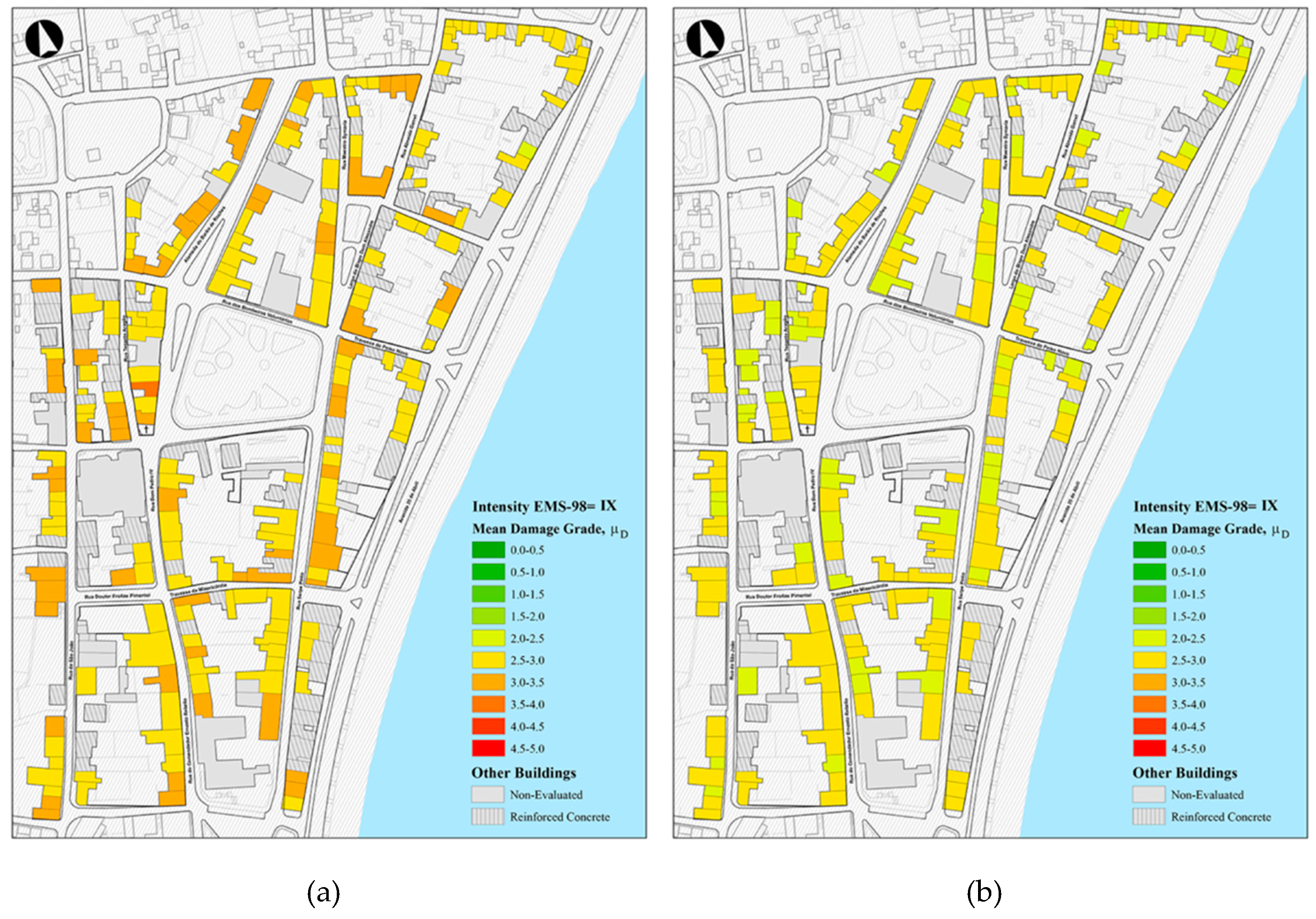
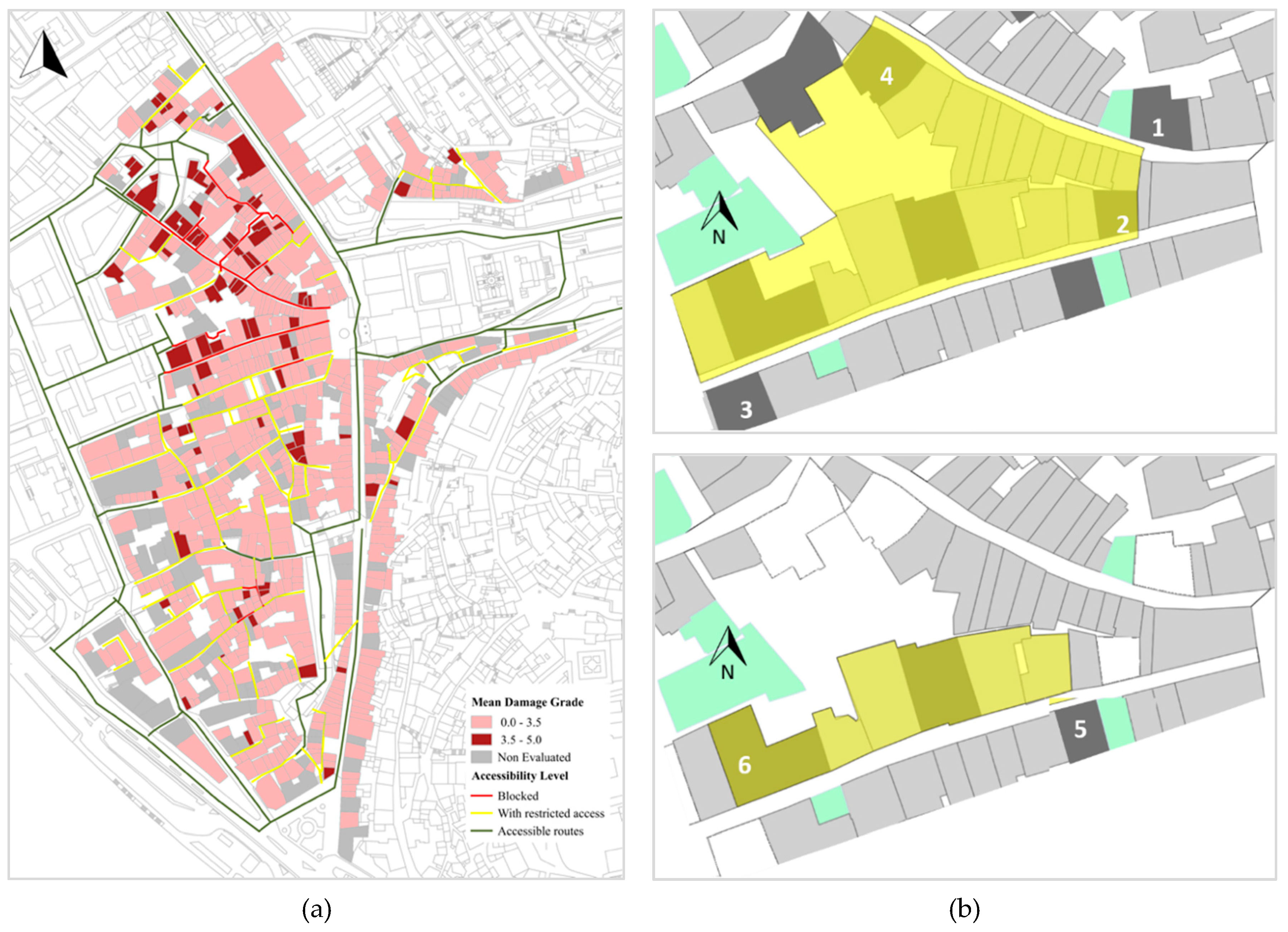
3.1. Typological Methods
3.2. Indirect Methods
3.3. Conventional Methods
3.4. Hybrid Methods
4. Seismic Vulnerability Assessment at Building Scale
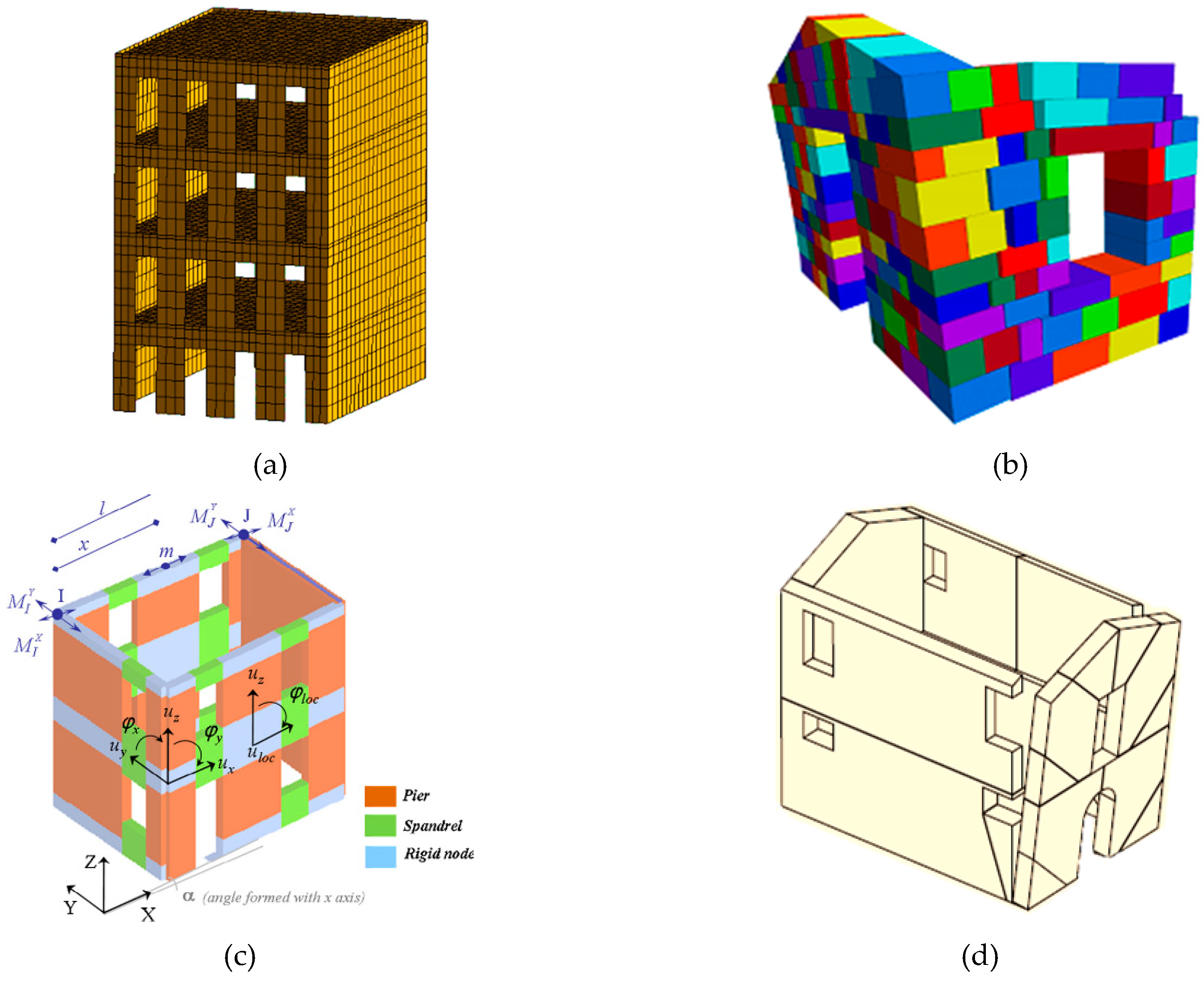
4.1. Numerical Modelling Approaches at Material Level
4.2. Numerical Modelling Methods
4.3. Types of Analysis
4.4. Assessment Criteria
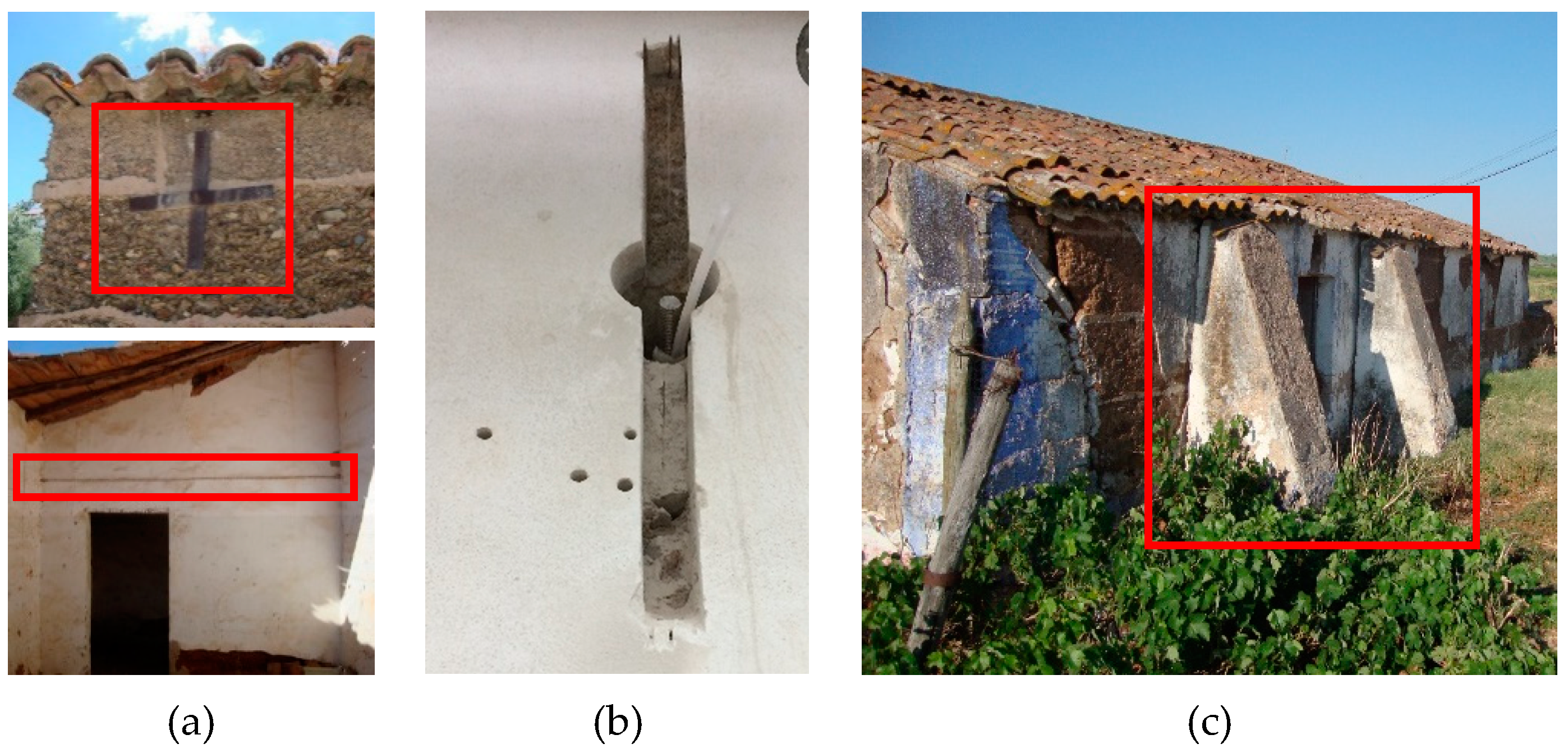
5. Seismic Retrofitting Techniques for Masonry Buildings
5.1. Traditional Solutions
5.2. Innovative Solutions
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Whitman, R.V.; Reed, J.W.; Hong, S.-T. Earthquake Damage Probability Matrices. In Proceedings of the Fifth World Conference Earthquakes Engineering, Rome, Italy, 25–29 June 1973. [Google Scholar]
- Calvi, G.M.; Pinho, R.; Magenes, G.; Bommer, J.J.; Crowley, H. Development of seismic vulnerability assessment methodologies over the past 30 years. ISET J. Earthq. Technol. 2006, 43, 75–104. [Google Scholar]
- Borzi, B.; Faravelli, M.; Polli, D.A. Central Italy sequence: Simulated damage scenario for the main 2016 shocks. Bull. Earthq. Eng. 2018. [Google Scholar] [CrossRef]
- Braga, F.; Dolce, M.; Liberatore, D. A statistical study on damaged buildings and an ensuing review of the MSK-76 scale. In Proceedings of the 7th European Conference on Earthquake Engineering, Athens, Greece, 20–25 September 1982; pp. 431–450. [Google Scholar]
- Medvedev, S.W.; Sponheuer, W.; Karnik, V. Seismic intensity scale version MSK 1964. In Proceedings of the First Meeting, Working Group on Seismicity and Seismo-Tectonics, Tbilissi, Georgia, 8–12 June 1965. [Google Scholar]
- Ferreira, T.M.; Maio, R.; Costa, A.A.; Vicente, R. Seismic vulnerability assessment of stone masonry façade walls: Calibration using fragility-based results and observed damage. Soil Dyn. Earthq. Eng. 2017, 103, 21–37. [Google Scholar] [CrossRef]
- Di Pasquale, G.; Orsini, G.; Pugliese, A.; Romeo, R. Damage scenario from future earthquakes. In Proceedings of the 11th European Conference on Earthquake Engineering, Paris la Défense, France, 6–11 September 1998. [Google Scholar]
- Sieberg, A. Geologie der Erdbeben. Handb. Geophys. 1930, 2, 552–555. [Google Scholar]
- Dolce, M.; Masi, A.; Marino, M.; Vona, M. Earthquake damage scenarios of the building stock of Potenza (Southern Italy) including site effects. Bull. Earthq. Eng. 2003, 1, 115–140. [Google Scholar] [CrossRef]
- Grünthal, G. European Macroseismic Scale 1998 (EMS-98); Cahiers du Centre Européen de Géodynamique et Séismologie: Luxembourg, 1998; Volume 15. [Google Scholar]
- Lagomarsino, S.; Giovinazzi, S. Macroseismic and mechanical models for the vulnerability and damage assessment of current buildings. Bull. Earthq. Eng. 2006, 4, 415–443. [Google Scholar] [CrossRef]
- Benedetti, D.; Petrini, V. Sulla vulnerabilita sismica di edifici in muratura: Proposta su un metodo di valutazione. L’industria delle Costr. 1984, 149, 66–74. [Google Scholar]
- GNDT-SSN. Scheda di Esposizione e Vulnerabilità e di Rilevamento Danni di Primo e Secondo Livello (Murata e Cemento Armato); GNDT-SSN: Rome, Italy, 1994. (In Italian) [Google Scholar]
- Faccioli, E.; Pessina, V.; Calvi, G.M.; Borzi, B. A study on damage scenarios for residential buildings in Catania city. J. Seismol. 1999, 3, 327–343. [Google Scholar] [CrossRef]
- ATC-21 Rapid Visual Screening of Buildings for Potential Seismic Hazards; Federal Emergency Management Agency: Washington, DC, USA, 1988.
- Bernardini, A. La Vulnerabilità Degli Edifici: Valutazione a Scala Nazionale Della Vulnerabilità Sismica Degli Edifici Ordinari; CNR-Gruppo Nazionale per la Difesa dai Terremoti: Roma, Italy, 2000. [Google Scholar]
- Petrini, V. Evaluation of risk levels. In Seismic Hazard and First Evaluation of Risk in Tuscany, CNR—Regione Toscana Technical Report; Petrini, V., Ed.; Kluwer Academic Publishers: Milano, Italy, 1999. (In Italian) [Google Scholar]
- Vicente, R.; D’Ayala, D.; Ferreira, T.M.; Varum, H.; Costa, A.; da Silva, J.A.R.M.; Lagomarsino, S. Seismic Vulnerability and Risk Assessment of Historic Masonry Buildings. In Structural Rehabilitation of Old Buildings; Costa, A., Guedes, J.M., Varum, H., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 307–348. ISBN 978-3-642-39686-1. [Google Scholar]
- ATC-13. Earthquake Damage Estimation Data for California; Report ATC-13; Applied Technology Council: Redwood City, CA, USA, 1985. [Google Scholar]
- Wood, H.O.; Neumann, F. Modified Mercalli intensity scale of 1931. Bull. Seismol. Soc. Am. 1931, 21, 277–283. [Google Scholar]
- Barbat, A.H.; Carreño, M.L.; Pujades, L.G.; Lantada, N.; Cardona, O.D.; Marulanda, M.C. Seismic vulnerability and risk evaluation methods for urban areas. A review with application to a pilot area. Struct. Infrastruct. Eng. 2010, 6, 17–38. [Google Scholar] [CrossRef]
- Eleftheriadou, A.K.; Karabinis, A.I. Development of damage probability matrices based on Greek earthquake damage data. Earthq. Eng. Eng. Vib. 2011, 10, 129–141. [Google Scholar] [CrossRef]
- Fäh, D.; Kind, F.; Lang, K.; Giardini, D. Earthquake scenarios for the city of Basel. Soil Dyn. Earthq. Eng. 2001, 21, 405–413. [Google Scholar] [CrossRef]
- Cardona, O.D.; Yamín, L.E. Seismic Microzonation and Estimation of Earthquake Loss Scenarios: Integrated Risk Mitigation Project of Bogota’, Colombia. Earthq. Spectra 1997, 13, 795–814. [Google Scholar] [CrossRef]
- McCormack, T.C.; Rad, F.N. An Earthquake Loss Estimation Methodology for Buildings Based on ATC-13 and ATC-21. Earthq. Spectra 1997, 13, 605–621. [Google Scholar] [CrossRef]
- Maio, R.; Ferreira, T.M.; Vicente, R.; Estêvão, J. Seismic vulnerability assessment of historical urban centres: Case study of the old city centre of Faro, Portugal. J. Risk Res. 2016, 19, 551–580. [Google Scholar] [CrossRef]
- Athmani, A.E.; Ferreira, T.M.; Vicente, R. Seismic risk assessment of the historical urban areas of Annaba city, Algeria. Int. J. Archit. Herit. 2018, 12. [Google Scholar] [CrossRef]
- Lamego, P.; Lourenço, P.B.; Sousa, M.L.; Marques, R. Seismic vulnerability and risk analysis of the old building stock at urban scale: Application to a neighbourhood in Lisbon. Bull. Earthq. Eng. 2017, 15, 2901–2937. [Google Scholar] [CrossRef]
- Maio, R.; Vicente, R.; Formisano, A.; Varum, H. Seismic vulnerability of building aggregates through hybrid and indirect assessment techniques. Bull. Earthq. Eng. 2015, 13, 2995–3014. [Google Scholar] [CrossRef]
- Ferreira, T.M.; Maio, R.; Vicente, R. Analysis of the impact of large scale seismic retrofitting strategies through the application of a vulnerability-based approach on traditional masonry buildings. Earthq. Eng. Eng. Vib. 2017, 16, 329–348. [Google Scholar] [CrossRef]
- Aguado, J.L.P.; Ferreira, T.M.; Lourenço, P.B. The Use of a Large-Scale Seismic Vulnerability Assessment Approach for Masonry Façade Walls as an Effective Tool for Evaluating, Managing and Mitigating Seismic Risk in Historical Centers. Int. J. Archit. Herit. 2018, 12, 1259–1275. [Google Scholar] [CrossRef]
- Lourenço, P.B. Computational Strategies for Masonry Structures. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1996. [Google Scholar]
- Mendes, N.; Lourenço, P.B. Seismic performance of ancient masonry buildings: A sensitivity analysis. In Proceedings of the ECCOMAS Thematic Conference—COMPDYN 2013: 4th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Kos Island, Greece, 12–14 June 2013; pp. 1624–1638. [Google Scholar]
- Kurdo, F.A.; Lee, S.C.; Martin, G. Simulating masonry wall behaviour using a simplified micro-model approach. Eng. Struct. 2017, 151, 349–365. [Google Scholar] [CrossRef]
- Andreotti, G.; Graziotti, F.; Magenes, G. Detailed micro-modelling of the direct shear tests of brick masonry specimens: The role of dilatancy. Eng. Struct. 2018, 168, 929–949. [Google Scholar] [CrossRef]
- DIANA—Finite Element Analysis. DIsplacement Method ANAlyzer; DIANA FEA BV: Delft, The Netherlands, 2019.
- ANSYS. Academic Research Mechanical; ANSYS, Inc.: Pennsylvania, PA, USA, 2019. [Google Scholar]
- Abaqus Unified FEA; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2019.
- Lourenço, P.B.; Mendes, N.; Ramos, L.F.; Oliveira, D.V. Analysis of masonry structures without box behavior. Int. J. Archit. Herit. 2011, 5, 369–382. [Google Scholar] [CrossRef]
- Mendes, N.; Lourenço, P.B. Seismic Assessment of Masonry “Gaioleiro” Buildings in Lisbon, Portugal. J. Earthq. Eng. 2010, 14, 80–101. [Google Scholar] [CrossRef][Green Version]
- Betti, M.; Galano, L.; Vignoli, A. Time-History Seismic Analysis of Masonry Buildings: A Comparison between Two Non-Linear Modelling Approaches. Buildings 2015, 5, 597–621. [Google Scholar] [CrossRef]
- Janaraj, T.; Dhanasekar, M. Finite element analysis of the in-plane shear behaviour of masonry panels confined with reinforced grouted cores. Constr. Build. Mater. 2014, 65, 495–506. [Google Scholar] [CrossRef]
- Ferreira, T.M.; Costa, A.A.; Costa, A. Analysis of the Out-Of-Plane Seismic Behavior of Unreinforced Masonry: A Literature Review. Int. J. Archit. Herit. 2015, 9, 949–972. [Google Scholar] [CrossRef]
- Lemos, J.V. Discrete element modeling of masonry structures. Int. J. Archit. Herit. 2007, 1, 190–213. [Google Scholar] [CrossRef]
- Smoljanović, H.; Živaljić, N.; Nikolić, Z. A combined finite-discrete element analysis of dry stone masonry structures. Eng. Struct. 2013, 52, 89–100. [Google Scholar] [CrossRef]
- UDEC—Distinct Element Modeling of Jointed and Blocky Material in 2D; ITASCA Consulting Group, Inc.: Minneapolis, MN, USA, 2019.
- 3DEC—Distinct Element Modeling of Jointed and Blocky Material in 3D; ITASCA Consulting Group, Inc.: Minneapolis, MN, USA, 2019.
- DEMpack; Center for Numerical Methods in Engineering: Barcelona, Spain, 2019.
- Mendes, N.; Zanotti, S.; Lemos, J.V. Seismic performance of historical buildings based on Discrete Element Method: An adobe church. J. Earthq. Eng. 2018. [Google Scholar] [CrossRef]
- Lemos, J.V.; Campos-Costa, A. Simulation of Shake Table Tests on Out-of-Plane Masonry Buildings. Part (V): Discrete Element Approach. Int. J. Archit. Herit. 2017, 11, 117–124. [Google Scholar] [CrossRef]
- De Felice, G. Out-of-plane seismic capacity of masonry depending on wall section morphology. Int. J. Archit. Herit. 2011, 5, 466–482. [Google Scholar] [CrossRef]
- Tomaževič, M. The Computer Program POR; Report ZRMK; Institute for Testing and Research in Materials and Structures: Ljubljana, Slovenia, 1978. [Google Scholar]
- 3Muri Software; S.T.A. DATA: Torino, Italy, 2019.
- PRO_SAM, Plugin of the PRO_SAP, ANDIL; Associazione Nazionale Degli Industriali dei Laterizi: Roma, Italy, 2019.
- 3DMacro; Gruppo Sismica s.r.l.: Catania, Italy, 2019.
- SAP200. Structural Analysis Program2000; Computers and Structures, Inc.: Walnut Creek, CA, USA, 2019. [Google Scholar]
- Petrovčič, S.; Kilar, V. Seismic failure mode interaction for the equivalent frame modelling of unreinforced masonry structures. Eng. Struct. 2013, 54, 9–22. [Google Scholar] [CrossRef]
- Lagomarsino, S.; Penna, A.; Galasco, A.; Cattari, S. TREMURI program: An equivalent frame model for the nonlinear seismic analysis of masonry buildings. Eng. Struct. 2013, 56, 1787–1799. [Google Scholar] [CrossRef]
- Magenes, G.; Fontana, A.D. Simplified non-linear seismic analysis of masonry buildings. In Proceedings of the 5th International Masonry Conference, London, UK, 12–14 October 1998; British Masonry Society: London, UK, 1998; Volume 1, pp. 190–195, ISSN 0950-9615. [Google Scholar]
- Penna, A.; Lagomarsino, S.; Galasco, A. A nonlinear macroelement model for the seismic analysis of masonry buildings. Earthq. Eng. Struct. Dyn. 2014, 43, 159–179. [Google Scholar] [CrossRef]
- Mendes, N.; Lourenço, P.B. Sensitivity analysis of the seismic performance of existing masonry buildings. Eng. Struct. 2014, 80, 137–146. [Google Scholar] [CrossRef]
- Galaso, A.; Lagomarsino, S.; Penna, A. TREMURI Program: Seismic Analysis of 3D Masonry Buildings; Software; University of Genoa: Genoa, Italy, 2002. [Google Scholar]
- Orduña, A. Seismic Assessment of Ancient Masonry Structures by Rigid Blocks Limit Analysis. Ph.D. Thesis, University of Minho, Braga, Portugal, 2003. [Google Scholar]
- Mendes, N.; Costa, A.A.; Lourenço, P.B.; Bento, R.; Beyer, K.; De Felice, G.; Gams, M.; Griffith, M.; Ingham, J.; Lagomarsino, S.; et al. Methods and approaches for blind test predictions of out-of-plane behavior of masonry walls: A numerical comparative study. Int. J. Archit. Herit. 2007, 11, 59–71. [Google Scholar] [CrossRef]
- Gilbert, M.; Casapulla, C.; Ahmed, H.M. Limit analysis of masonry block structures with non-associative frictional joints using linear programming. Comput. Struct. 2006, 84, 873–887. [Google Scholar] [CrossRef]
- Orduña, A.; Lourenço, P.B. Three-dimensional limit analysis of rigid blocks assemblages. Part I: Torsion failure on frictional interfaces and limit analysis formulation. Int. J. Solids Struct. 2005, 42, 5140–5160. [Google Scholar] [CrossRef]
- Mendes, N. Masonry Macro-block Analysis. In Encyclopedia of Earthquake Engineering; Ioannis, M.B., Kougioumtzoglou, A., Patelli, E., Au, I.S., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 978-3-642-36197-5. [Google Scholar]
- Fajfar, P. A nonlinear analysis method for performance-based seismic design. Earthq. Spectra 2000, 16, 573–592. [Google Scholar] [CrossRef]
- FEMA 440. Improvement of Nonlinear Static Seismic Analysis Procedures: FEMA 440; Applied Technology Council (ATC-55 Project) for the Federal Emergency Management Agency (FEMA): Washington, DC, USA, 2005.
- ASCE. Seismic Evaluation and Retrofit of Existing Buildings: ASCE/SEI 41-13; Virginia American Society of Civil Engineers: Reston, VA, USA, 2013. [Google Scholar]
- Azizi-Bondarabadi, H.; Mendes, N.; Lourenço, P.B. Higher Mode Effects in Pushover Analysis of Irregular Masonry Buildings. J. Earthq. Eng. 2019, in press. [Google Scholar] [CrossRef]
- Chácara, C.; Mendes, N.; Lourenço, P.B. Simulation of Shake Table Tests on Out-of-Plane Masonry Buildings. Part (IV): Macro and Micro FEM Based Approaches. Int. J. Archit. Herit. 2017, 11, 103–116. [Google Scholar] [CrossRef]
- Housner, G.W. The behavior of inverted pendulum structures during earthquakes. Bull. Seismol. Soc. Am. 1963, 53, 403–417. [Google Scholar]
- Sorrentino, L.; D’Ayala, D.; De Felice, G.; Griffith, M.C.; Lagomarsino, S.; Magenes, G. Review of Out-of-Plane Seismic Assessment Techniques Applied to Existing Masonry Buildings. Int. J. Archit. Herit. 2017, 11, 2–21. [Google Scholar] [CrossRef]
- Ortega, J.; Vasconcelos, G.; Rodrigues, H.; Correia, M.; Lourenço, P.B. Traditional earthquake resistant techniques for vernacular architecture and local seismic cultures: A literature review. J. Cult. Herit. 2017, 27, 181–196. [Google Scholar] [CrossRef]
- Moreira, S.; Ramos, L.F.; Oliveira, D.V.; Lourenço, P.B. Design parameters for seismically retrofitted masonry-to-timber connections: Injection anchors. Int. J. Archit. Herit. 2016, 10, 217–234. [Google Scholar] [CrossRef]
- Vintzileou, E. Effect of timber ties on the behavior of historic masonry. J. Struct. Eng. 2008, 134, 961–972. [Google Scholar] [CrossRef]
- Michiels, T.L. Seismic retrofitting techniques for historic adobe buildings. Int. J. Archit. Herit. 2015, 9, 1059–1068. [Google Scholar] [CrossRef]
- Moreira, S.; Ramos, L.F.; Oliveira, D.V.; Lourenço, P.B. Experimental behavior of masonry wall-to-timber elements connections strengthened with injection anchors. Eng. Struct. 2014, 81, 98–109. [Google Scholar] [CrossRef]
- Paganoni, S.; D’Ayala, D. Testing and design procedure for corner connections of masonry heritage buildings strengthened by metallic grouted anchors. Eng. Struct. 2014, 70, 278–293. [Google Scholar] [CrossRef]
- Senaldi, I.; Magenes, G.; Penna, A.; Galasco, A.; Rota, M. The effect of stiffened floor and roof diaphragms on the experimental seismic response of a full-scale unreinforced stone masonry building. J. Earthq. Eng. 2014, 18, 407–443. [Google Scholar] [CrossRef]
- Binda, L. The difficult choice of materials used for the repair of brick and stone masonry walls. In Proceedings of the 1st International Conference on Restoration of Heritage Masonry Structures, Cairo, Egypt, 24–27 April 2006. [Google Scholar]
- Oliveira, D.V.; Silva, R.A.; Garbin, E.; Lourenço, P.B. Strengthening of three-leaf stone masonry walls: An experimental research. Mater. Struct. 2012, 45, 1259–1276. [Google Scholar] [CrossRef]
- Hurd, J. Observing and applying ancient repair techniques to pisé and adobe in seismic regions of Central Asia and Trans-Himalaya. In Proceedings of the Getty Seismic Adobe Project 2006 Colloquium, Getty Conservation Institute, Los Angeles, CA, USA, 11–13 April 2006; pp. 101–108. [Google Scholar]
- Valluzzi, M.R.; Binda, L.; Modena, C. Mechanical behaviour of historic masonry structures strengthened by bed joints structural repointing. Constr. Build. Mater. 2005, 19, 63–73. [Google Scholar] [CrossRef]
- Karakostas, C.; Lekidis, V.; Makarios, T.; Salonikios, T.; Sous, I.; Demosthenous, M. Seismic response of structures and infrastructure facilities during the Lefkada, Greece earthquake of 14/8/2003. Eng. Struct. 2005, 27, 213–227. [Google Scholar] [CrossRef]
- Toumbakari, E. Lime-Pozzolan-Cement Grouts and their Structural Effects on Composite Masonry Walls. Ph.D. Thesis, Catholic University of Leuven, Leuven, Belgium, 2002. [Google Scholar]
- Mazzon, N.; Valluzzi, M.R.; Aoki, T.; Garbin, E.; De Canio, G.; Ranieri, N.; Modena, C. Shaking table tests on two multi-leaf stone masonry buildings. In Proceedings of the 11th Canadian Masonry Symposium, Toronto, ON, Canada, 31 May–3 June 2009. [Google Scholar]
- Illampas, R.; Silva, R.A.; Charmpis, D.C.; Lourenço, P.B.; Ioannou, I. Validation of the repair effectiveness of clay-based grout injections by lateral load testing of an adobe model building. Constr. Build. Mater. 2017, 153, 174–184. [Google Scholar] [CrossRef]
- Papanicolaou, C.G.; Triantafillou, T.C.; Papathanasiou, M.; Kyriakos, K. Textile Reinforced Mortar (TRM) versus FRP as Strengthening Material of URM Walls: Out-of-plane Cyclic Loading. Mater. Struct. 2008, 41, 143–157. [Google Scholar] [CrossRef]
- Valluzzi, M.R.; Modena, C.; De Felice, G. Current Practice and Open Issues in Strengthening Historical Buildings with Composites. Mater. Struct. 2014, 47, 1971–1985. [Google Scholar] [CrossRef]
- De Felice, G.; De Santis, S.; Garmendia, L.; Ghiassi, B.; Larrinaga, P.; Lourenço, P.B.; Oliveira, D.V.; Paolacci, F.; Papanicolaou, C.G. Mortar-based Systems for Externally Bonded Strengthening of Masonry. Mater. Struct. 2014, 47, 2021–2037. [Google Scholar] [CrossRef]
- Ascione, L.; De Felice, G.; De Santis, S.A. Qualification Method for Externally Bonded Fiber Reinforced Cementitious Matrix (FRCM) Strengthening Systems. Compos. Part B Eng. 2015, 78, 497–506. [Google Scholar] [CrossRef]
- ACI 440.7R-10: Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Unreinforced Masonry Structures; American Concrete Institute: Farmington Hills, MI, USA, 2010.
- CNR-DT200 R1/2012: Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Structures; Italian Council of Research (CNR): Rome, Italy, 2012; pp. 1–167.
- ACI 549.4R13: Guide to Design and Construction of Externally Bonded Fabric-Reinforced Cementitious Matrix (FRCM) Systems for Repair and Strengthening Concrete and Masonry Structures; American Concrete Institute (ACI): Farmington Hills, MI, USA, 2013; ISBN 9780870318528.
- Bailey, J.S.; Allen, E.W. Seismic isolation retrofitting: Salt Lake City and county building. APT Bull. J. Preserv. Technol. 1988, 20, 33–44. [Google Scholar] [CrossRef]
- Castellano, M.G.; Tosti, G.; Bolletti, G.P.; Tosti, M. Fluid Spring Dampers for Seismic Protection of the Cathedral of Siena; Protection of Historical Buildings—PROHITECH: Rome, Italy, 2009; pp. 669–674. [Google Scholar]
- Maio, R.; Ferreira, T.M.; Vicente, R. A critical discussion on the earthquake risk mitigation of urban cultural heritage assets. Int. J. Disaster Risk Reduct. 2018, 27, 239–247. [Google Scholar] [CrossRef]


| Damage Grade | CDF (%) | Intensity | |||||
|---|---|---|---|---|---|---|---|
| V | VI | VII | VIII | IX | X | ||
| 1 | 5 | 8 | 35 | 20 | 20 | 5 | 0 |
| 2 | 20 | 0 | 8 | 37 | 37 | 15 | 7 |
| 3 | 55 | 0 | 0 | 35 | 35 | 37 | 15 |
| 4 | 90 | 0 | 0 | 8 | 8 | 35 | 37 |
| 5 | 100 | 0 | 0 | 0 | 0 | 8 | 41 |
| MDF | 0.4 | 3 | 14 | 35 | 63 | 84 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferreira, T.M.; Mendes, N.; Silva, R. Multiscale Seismic Vulnerability Assessment and Retrofit of Existing Masonry Buildings. Buildings 2019, 9, 91. https://doi.org/10.3390/buildings9040091
Ferreira TM, Mendes N, Silva R. Multiscale Seismic Vulnerability Assessment and Retrofit of Existing Masonry Buildings. Buildings. 2019; 9(4):91. https://doi.org/10.3390/buildings9040091
Chicago/Turabian StyleFerreira, Tiago Miguel, Nuno Mendes, and Rui Silva. 2019. "Multiscale Seismic Vulnerability Assessment and Retrofit of Existing Masonry Buildings" Buildings 9, no. 4: 91. https://doi.org/10.3390/buildings9040091
APA StyleFerreira, T. M., Mendes, N., & Silva, R. (2019). Multiscale Seismic Vulnerability Assessment and Retrofit of Existing Masonry Buildings. Buildings, 9(4), 91. https://doi.org/10.3390/buildings9040091







