Dynamic Responses of Single-Layer Reticulated Shells under Oblique Impact Loading
Abstract
1. Introduction
2. Experimental Verification
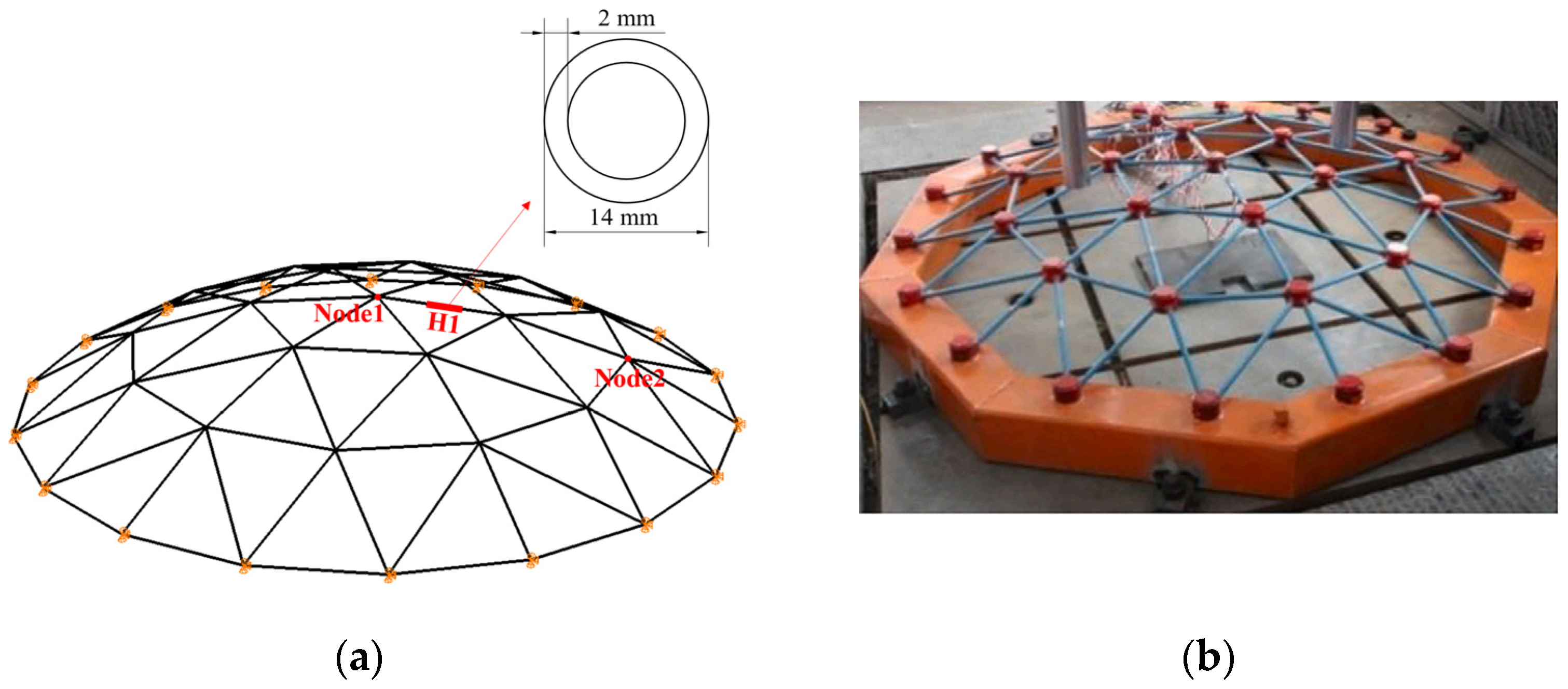
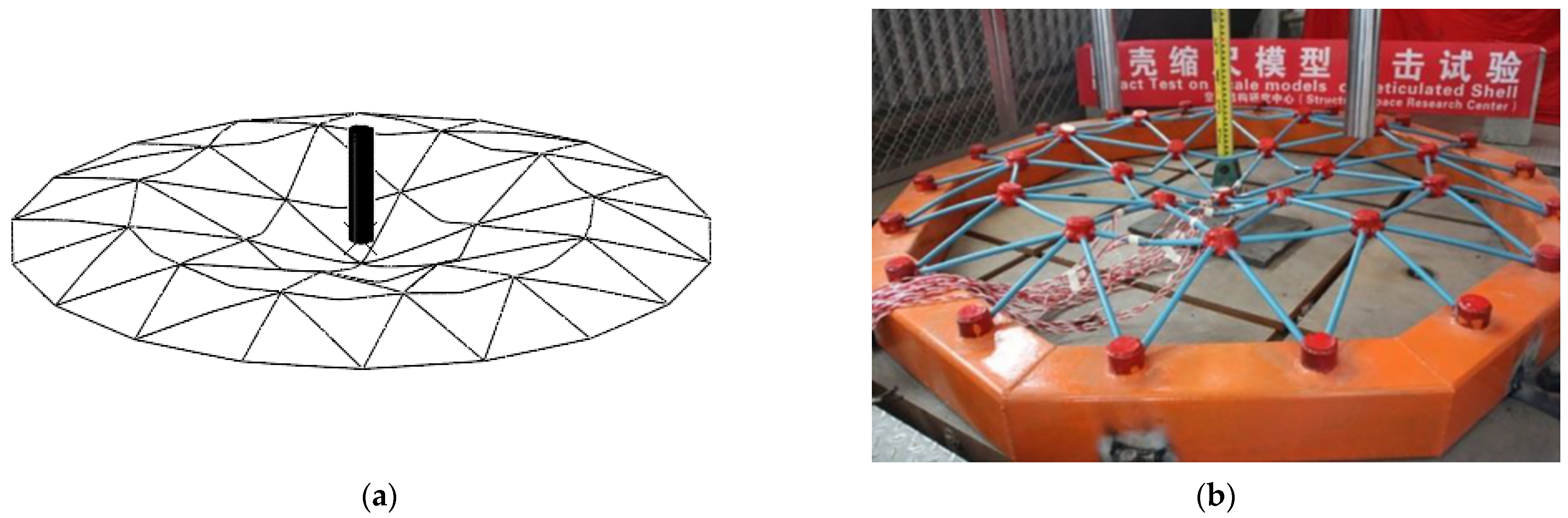
2.1. Finite Element Modelling
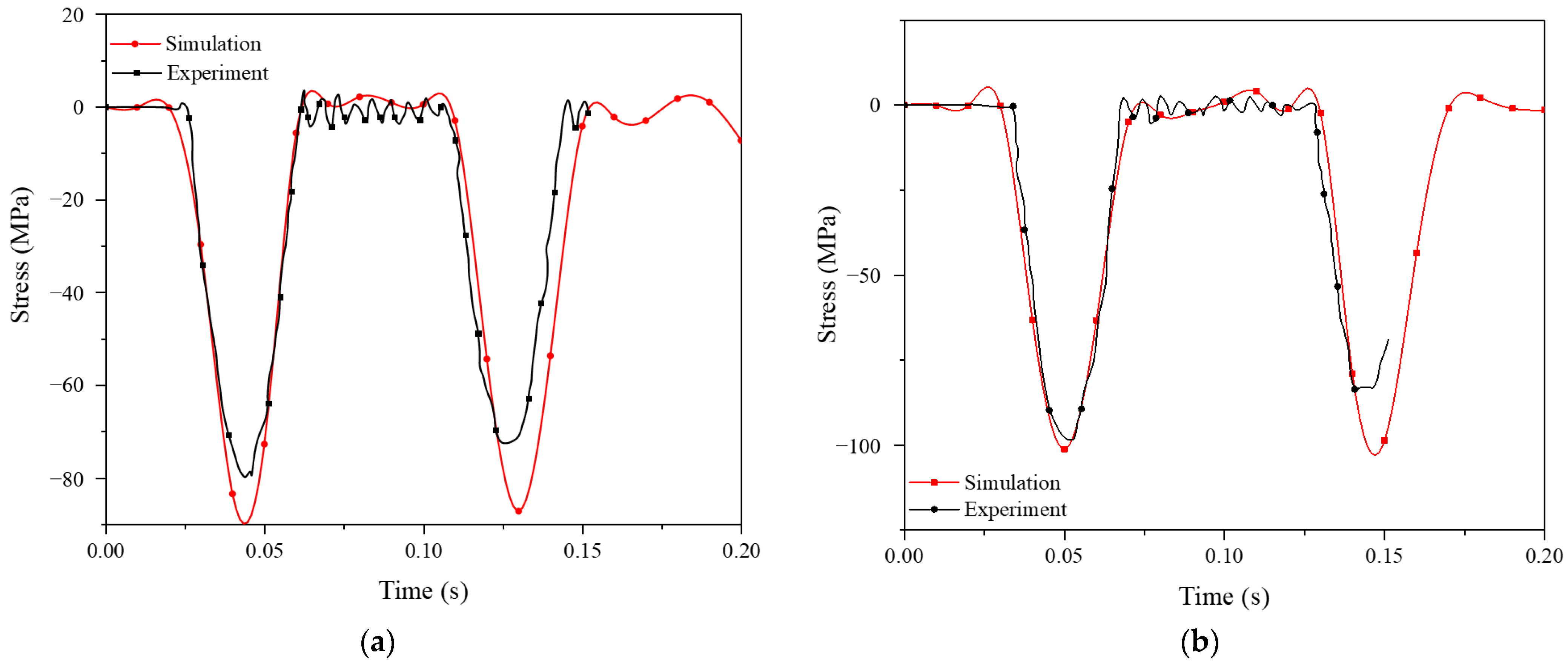
2.2. Verification of the Numerical Simulation Method
3. Impact Response of Reticulated Shell Structures under Oblique Impact Load
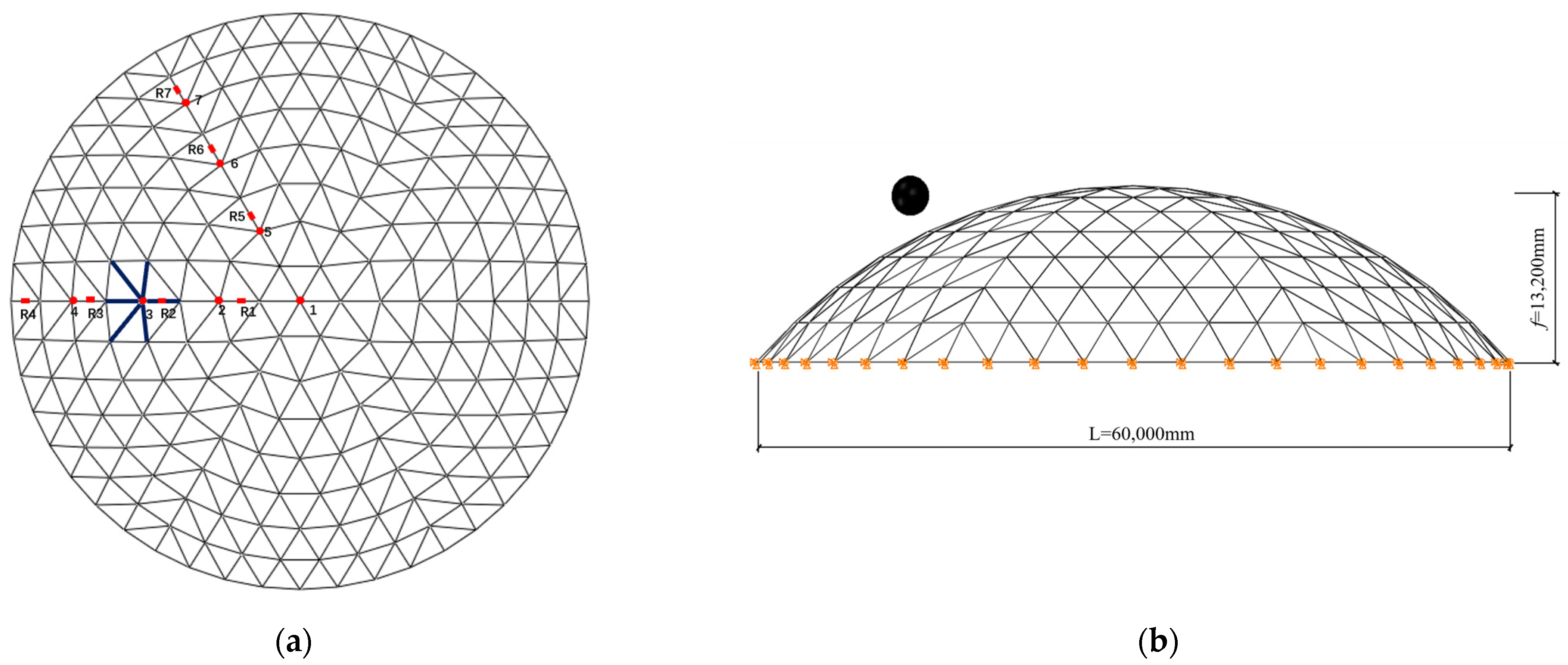
3.1. Analytical Model
3.2. Failure Mode
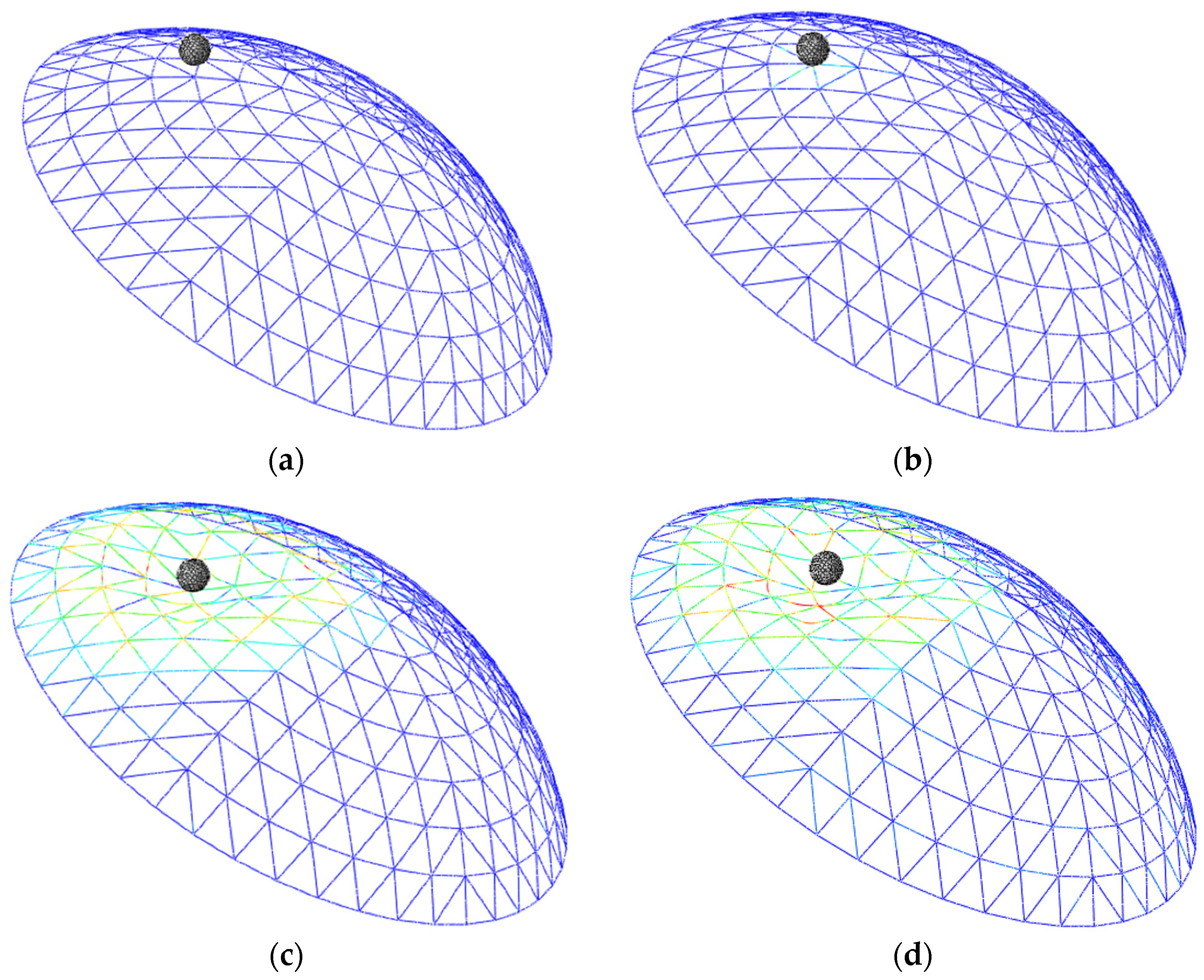
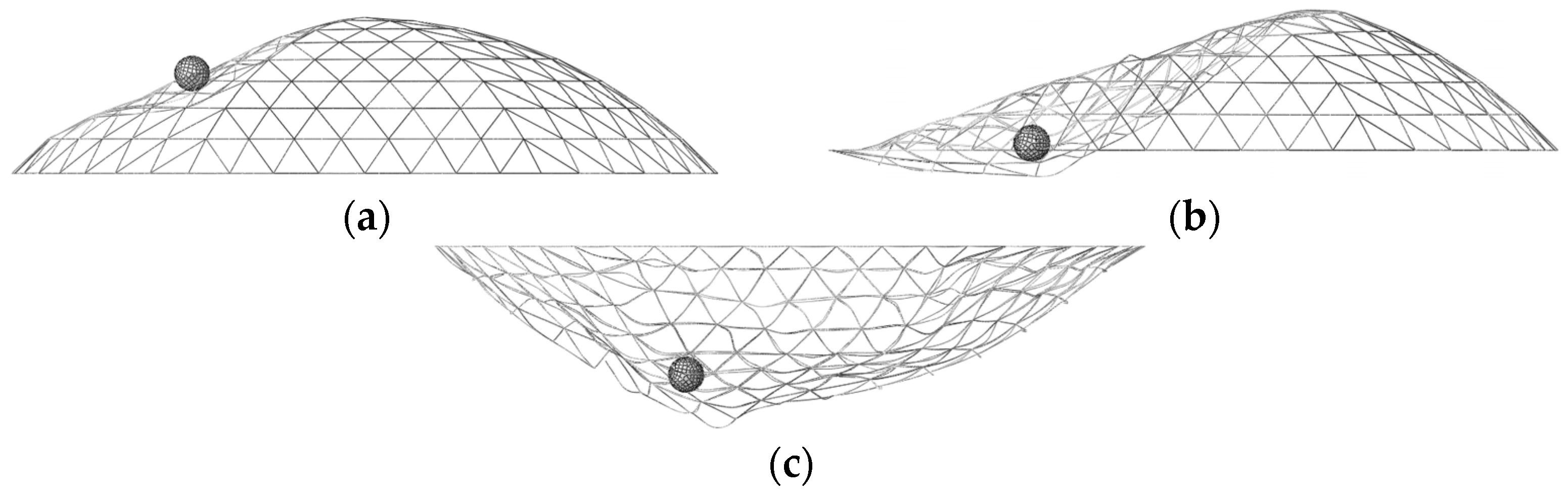
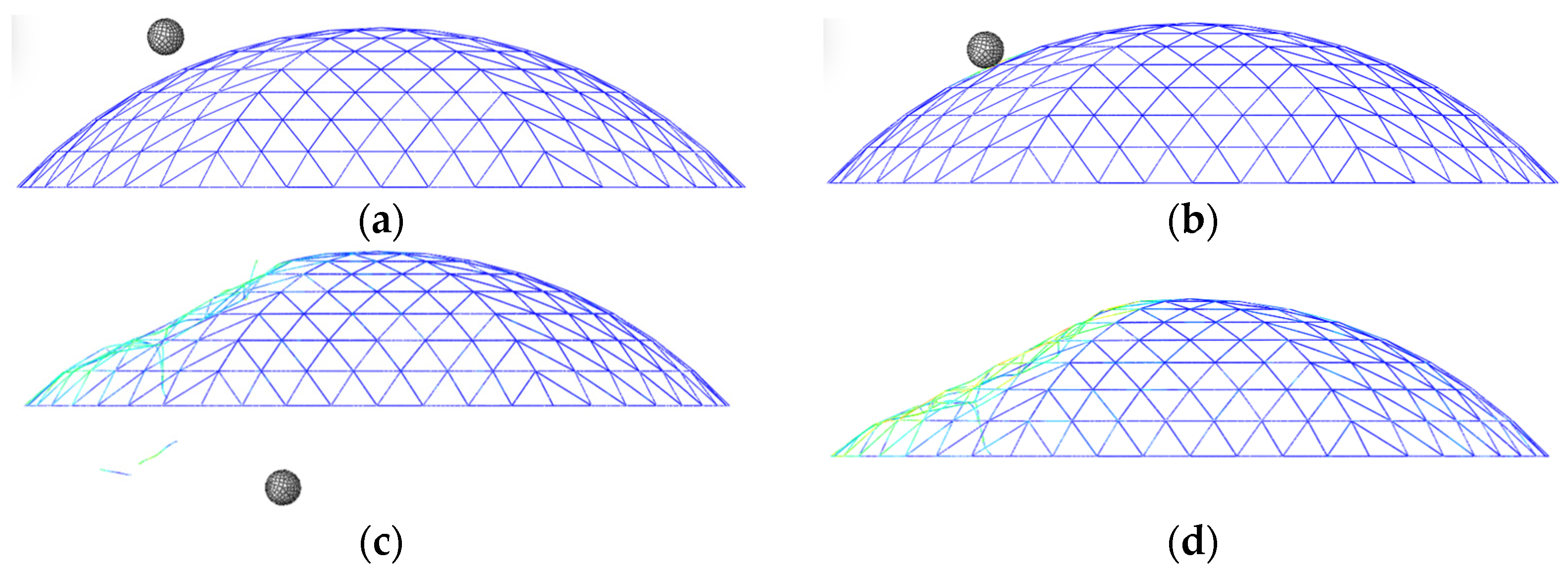
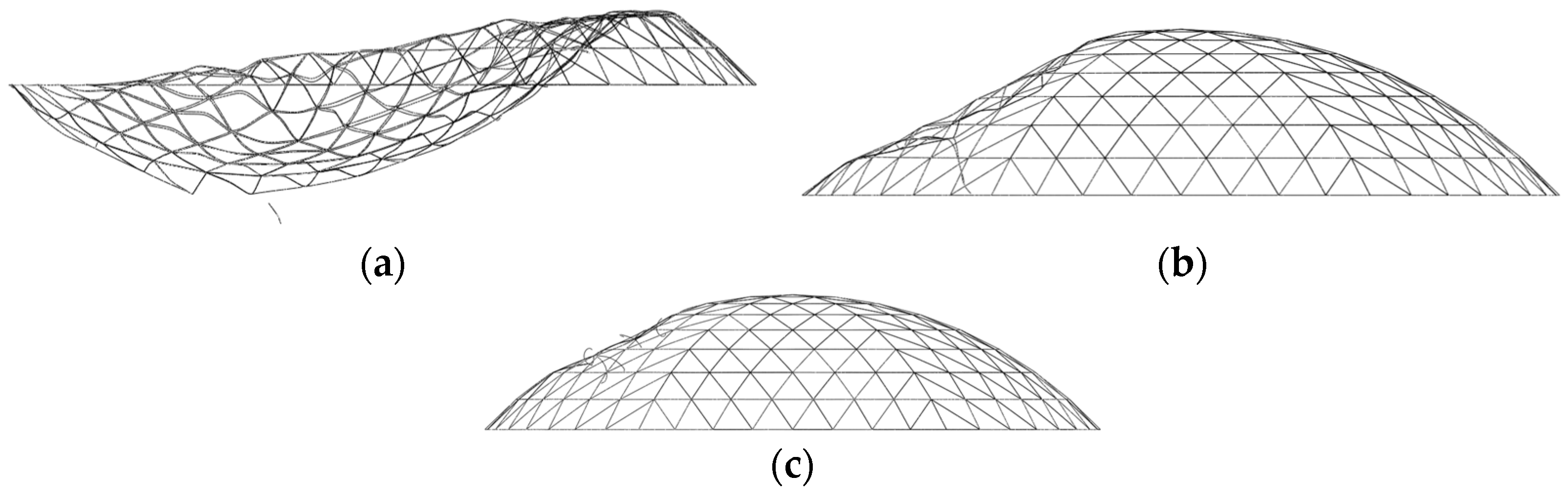
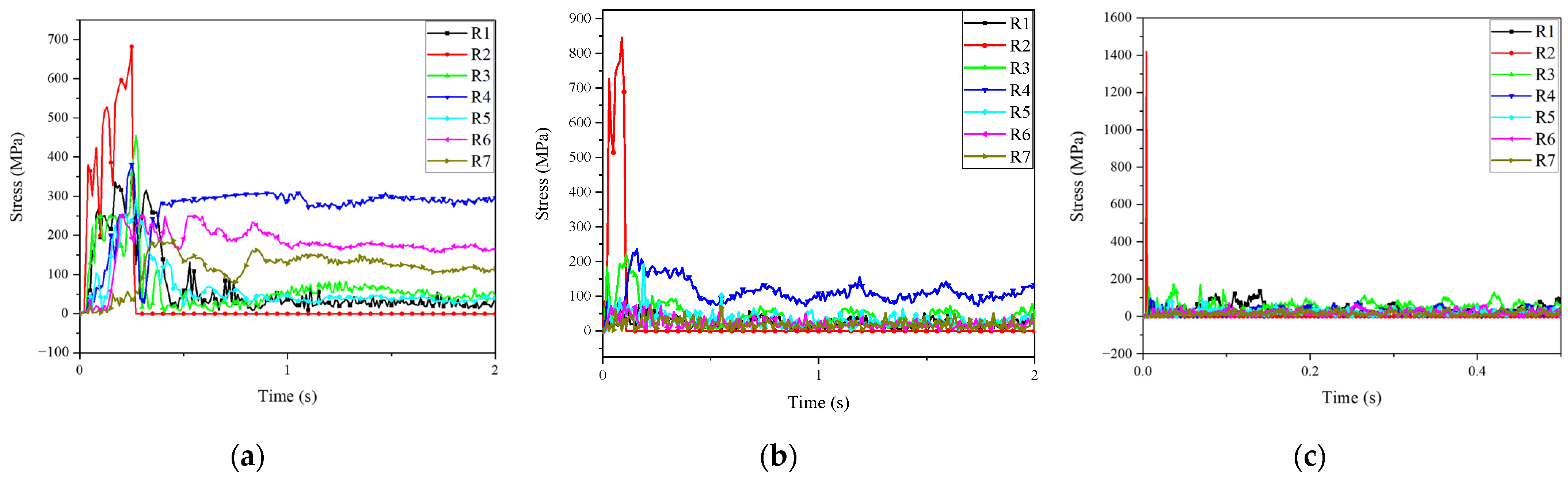
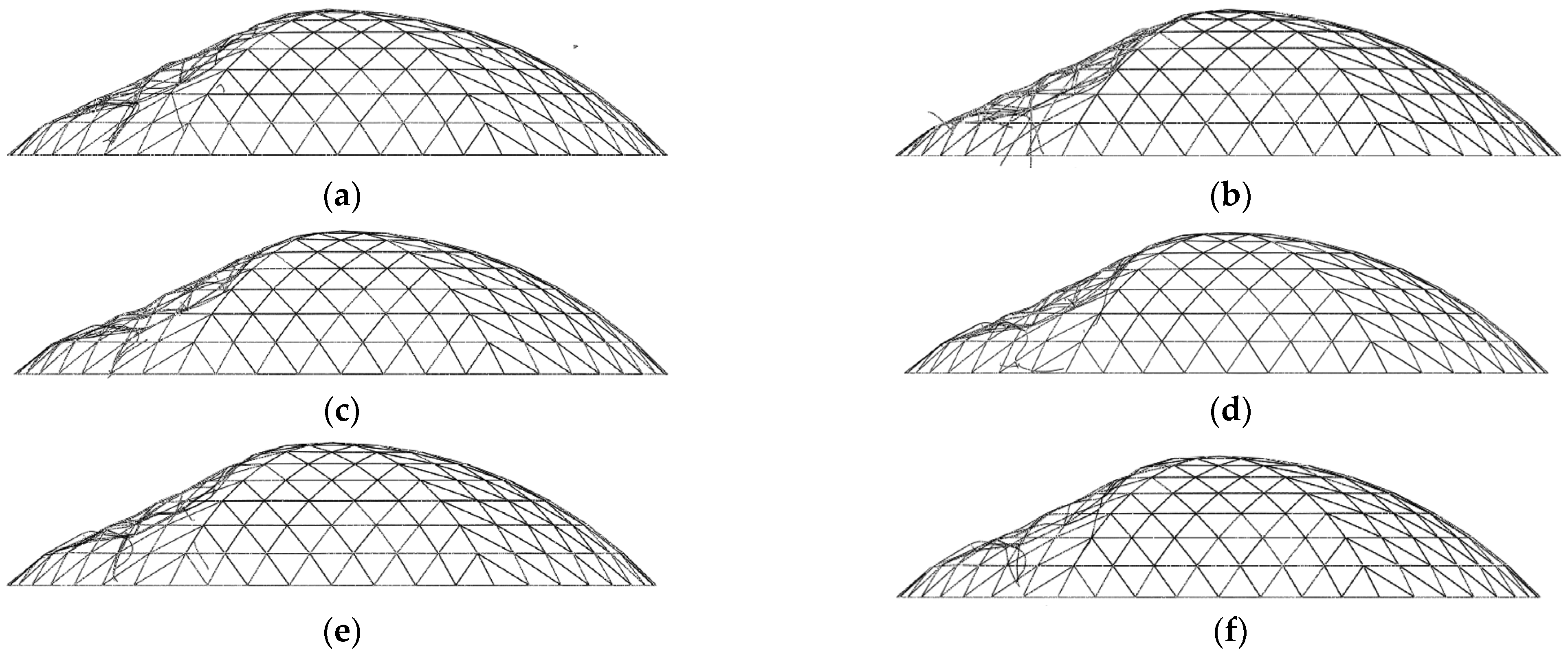
3.2.1. Unpenetrated Failure
3.2.2. Penetrated Failure
4. Comparative Analysis of the Dynamic Response of Reticulated Shell Structure at Different Impact Angles
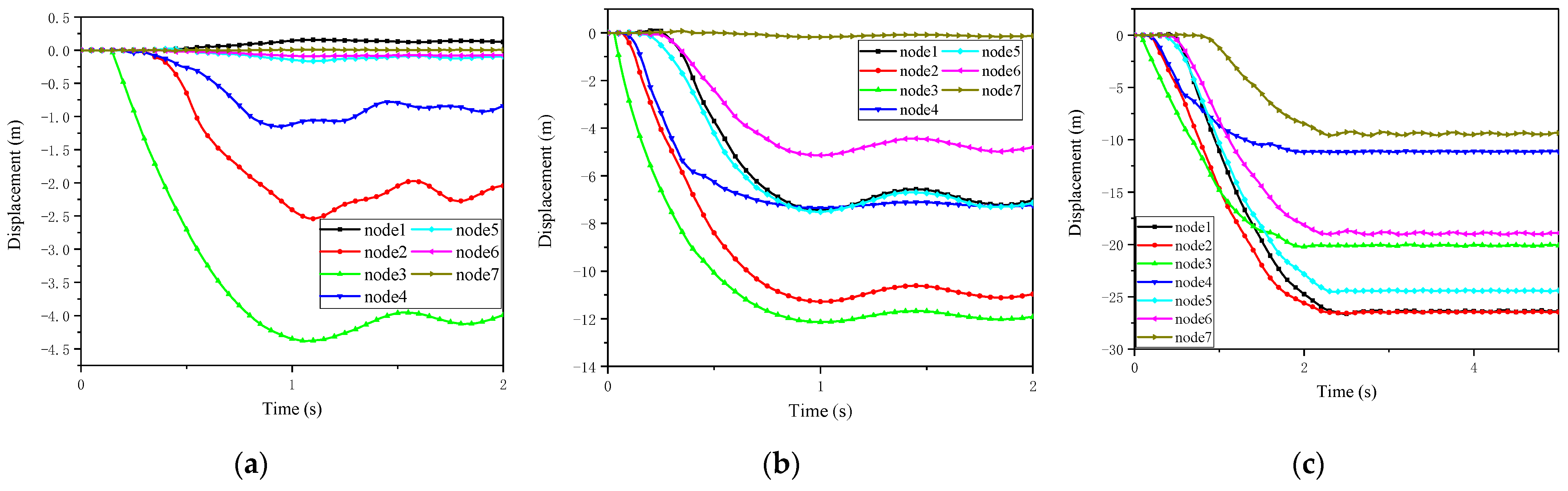
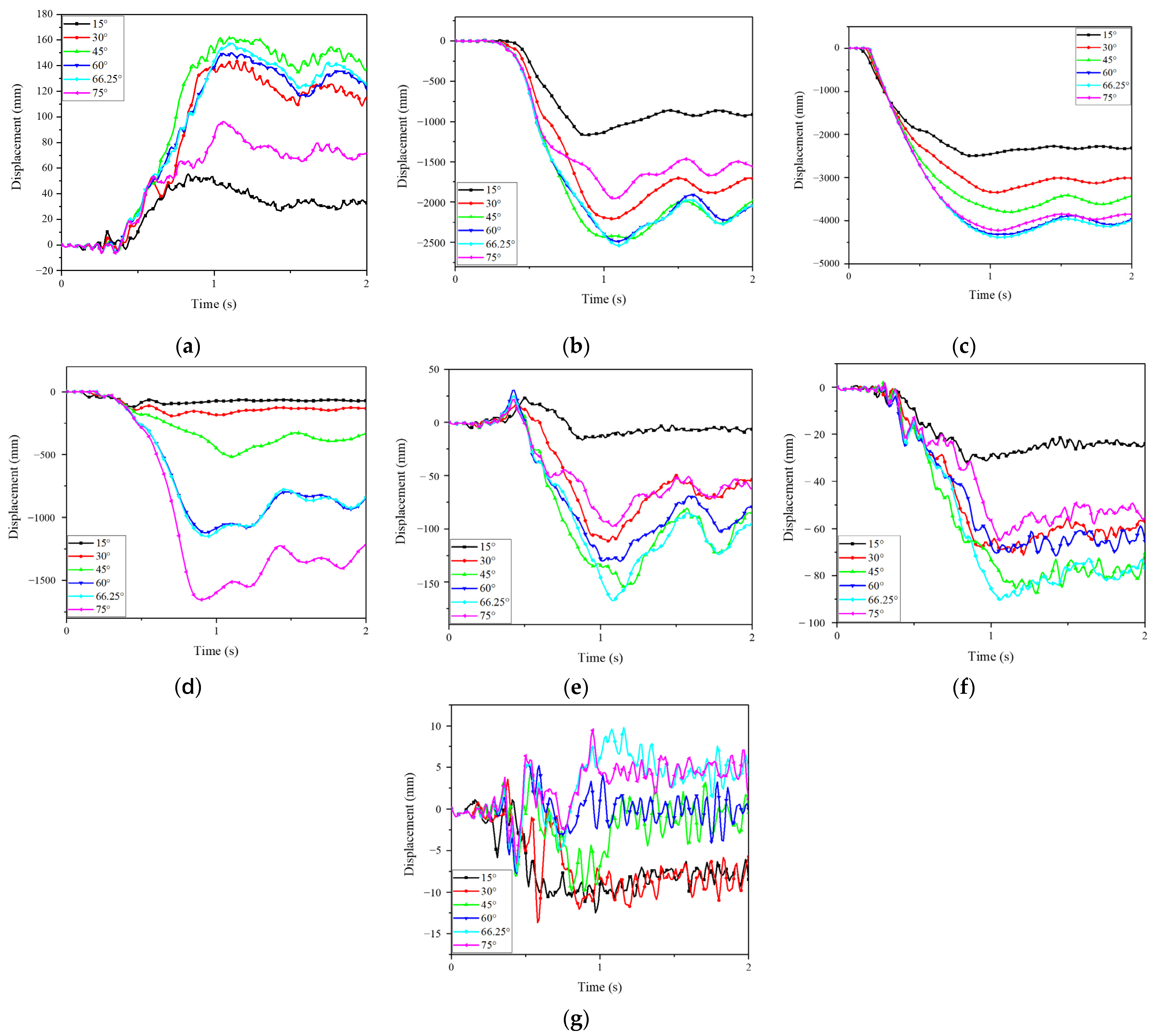
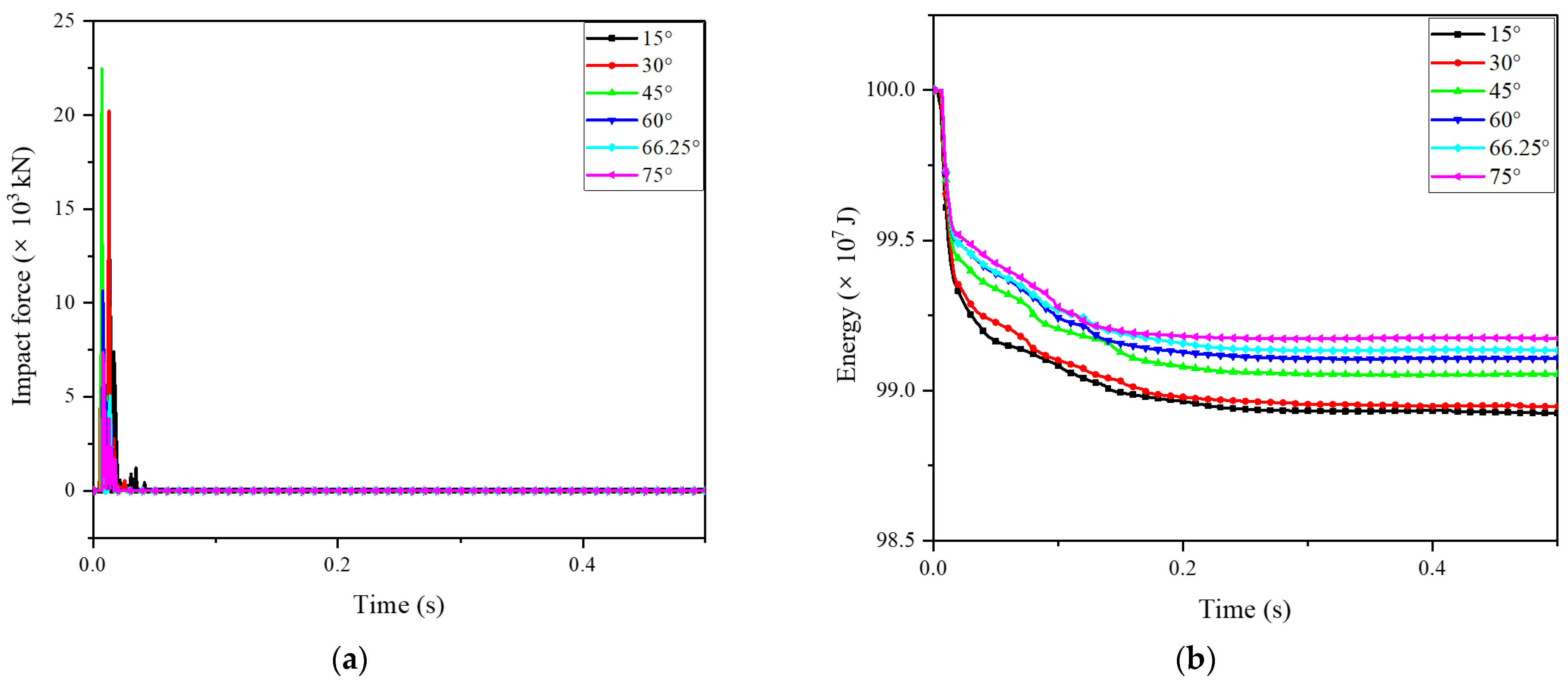
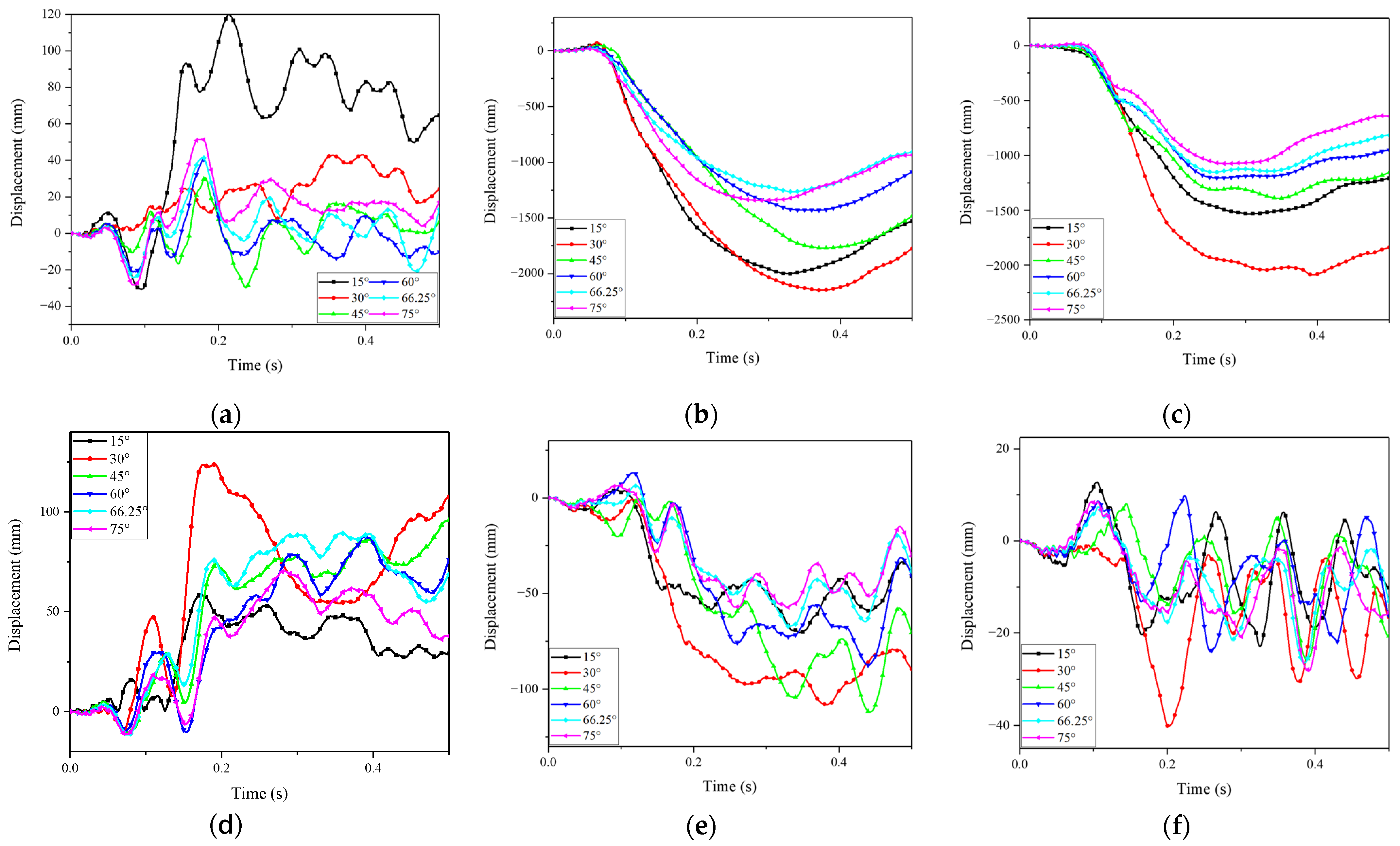
4.1. Influence of Oblique Impact Angle on Impact Response of Reticulated Shell Structure Experiencing Unpenetrated Failure
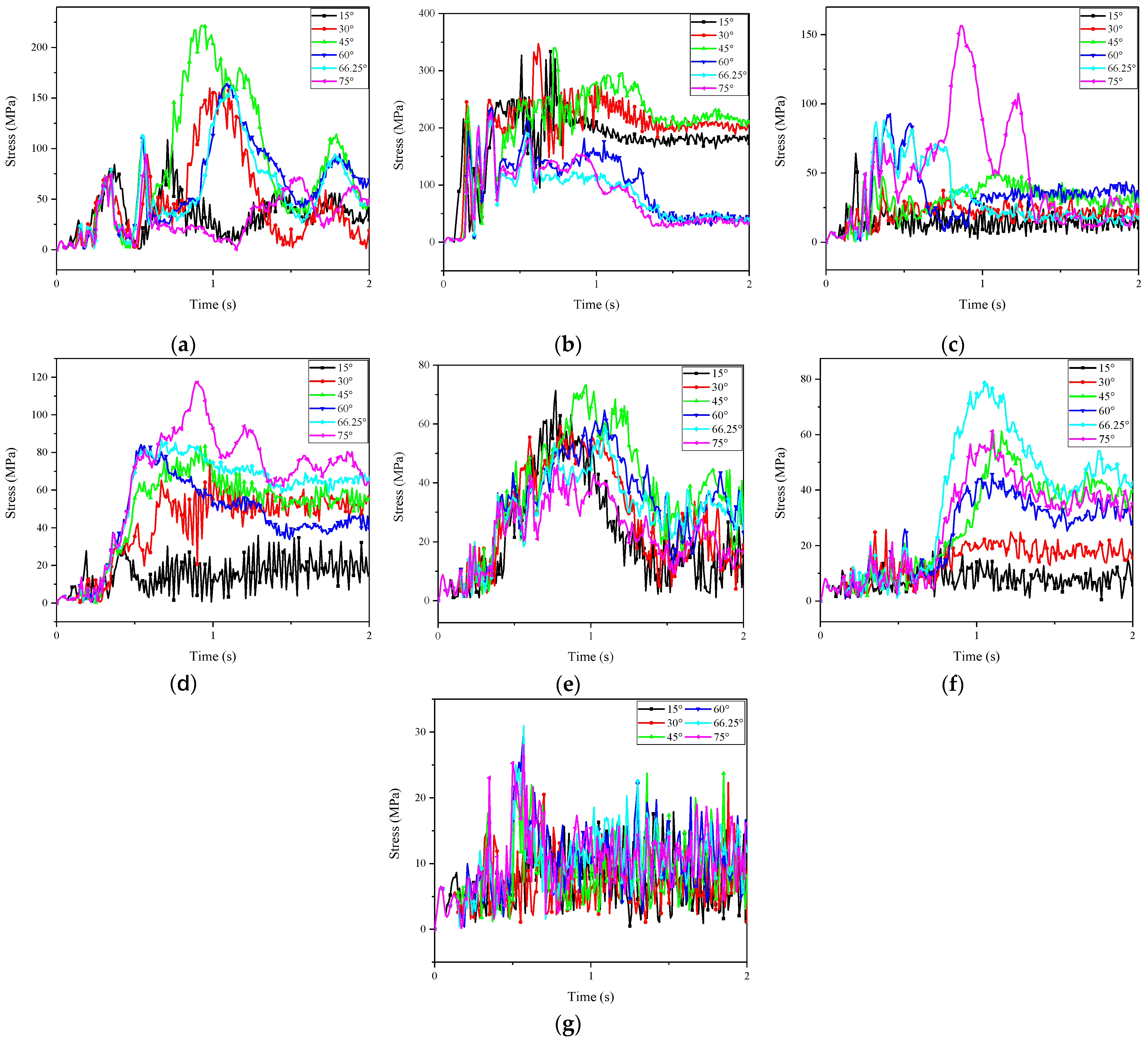
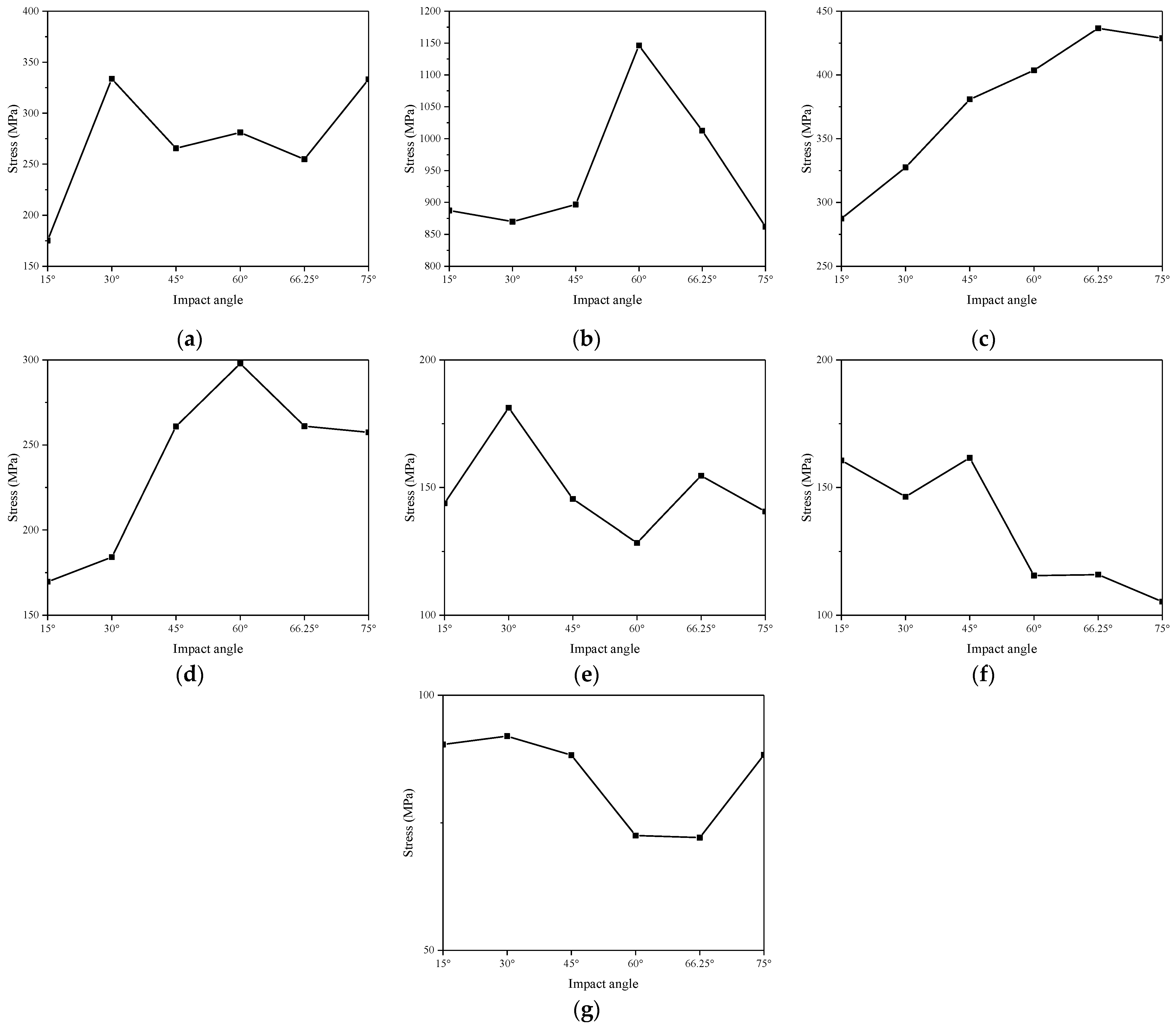
4.2. Influence of Oblique Impact Angle on Impact Response of Reticulated Shell Structure Experiencing Penetrated Failure
5. Conclusions
- The failure modes of the reticulated shell structure under oblique impact loads were divided into two types: unpenetrated and penetrated failure modes. In the case of unpenetrated failure, most of the impact energy was absorbed by the reticulated shell structure; the structures always exhibited a local bending response for the impactor with a small initial kinetic energy and an overall bending/shear response for the impactor with a large one. In the case of penetrated failure, only a small part of the impact energy was absorbed by the reticulated shell structure; the structures exhibited an overall bending/shear response or local penetration, depending on the initial kinetic energy of the impactor.
- For the reticulated shell structure experiencing unpenetrated failure mode, the oblique impact angle had a quite limited effect on the impact duration and maximum impact force, but had an important effect on the energy dissipation capacity of the structures, especially for the oblique impact angle less than 60°. When the impact angle exceeded 60°, the energy change rates of the structure remained consistently above 90%, and the influence of the oblique impact angle on the energy change rate was limited.
- For the reticulated shell structure experiencing penetrated failure mode, the oblique impact angle had a slight effect on the energy change rate of the structure. However, it played an important part in the maximum impact force of the structures. Compared to other angles, the structure exhibits the greatest impact force at an oblique impact angle of 45°. It was noteworthy that the impact angle had a relatively limited influence on the response mode of the structures experiencing both unpenetrated and penetrated failure modes.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cowper, G.R.; Symonds, P.S. Strain-harding and strain-rate effects in the impact loading of cantilever beams. Small Bus. Econ. 1957, 31, 235–263. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperature. In Proceedings of the 7th International Symposium on Ballistics, Hague, The Netherlands, 19–21 April 1983. [Google Scholar]
- Paul, S.K. Predicting the flow behavior of metals under different strain rate and temperature through phenomenological modeling. Comput. Mater. Sci. 2012, 65, 91–99. [Google Scholar] [CrossRef]
- Al-Thairy, H.; Wang, Y.C. A numerical study of the behaviour and failure modes of axially compressed steel columns subjected to transverse impact. Int. J. Impact Eng. 2011, 39, 732–744. [Google Scholar] [CrossRef]
- Liang, C.; Wang, C.J.; Nguyen, V.B.; English, M.; Mynors, D. Experimental and numerical study on crashworthiness of cold-formed dimpled steel columns. Thin Walled Struct. 2017, 112, 83–91. [Google Scholar] [CrossRef]
- Liang, C.; Wang, C.J.; English, M.; Mynors, D. Behaviour of cold-formed dimpled columns under lateral impact. Eng. Struct. 2018, 163, 167–176. [Google Scholar] [CrossRef]
- Li, W.; Gu, Y.Z.; Han, L.H.; Zhao, X.L. Behaviour of grout-filled double-skin steel tubular T-joint subjected to low-velocity impact. Thin Walled Struct. 2019, 144, 106270. [Google Scholar] [CrossRef]
- Xiang, S.; He, Y.J.; Zhou, X.H. Behaviour and failure modes of steel parking structure column under transverse impact. Thin Walled Struct. 2021, 167, 108163. [Google Scholar] [CrossRef]
- Bambach, M.R.; Jama, H.; Zhao, X.L.; Grzebieta, R.H. Hollow and concrete filled steel hollow sections under transverse impact load. Eng. Struct. 2008, 30, 2859–2870. [Google Scholar] [CrossRef]
- Wang, Y.; Qian, X.D.; Liw, J.Y.R.; Zhang, M.H. Experimental behavior of cement filled pipe-in-pipe composite structures under transverse impact. Int. J. Impact Eng. 2014, 72, 1–16. [Google Scholar] [CrossRef]
- Goswami, A.; Adhikary, S.D.; Li, B. Predicting the punching shear failure of concrete slabs under low velocity impact loading. Eng. Struct. 2019, 184, 37–51. [Google Scholar] [CrossRef]
- Lee, K.K.; Shin, J.W.; Kim, K.S.; Varma, A. Local responses of steel-plate composite walls subjected to impact loads: Intermediate scale tests. Eng. Struct. 2020, 206, 110131. [Google Scholar] [CrossRef]
- Li, H.W.; Chen, W.S.; Huang, Z.J.; Hao, H.; Ngo, T.T.; Pham, T.M.; Yeoh, K.J. Dynamic response of monolithic and precast concrete joint with wet connections under impact loads. Eng. Struct. 2022, 250, 113434. [Google Scholar] [CrossRef]
- Li, L.; Wang, H.W.; Wu, J.; Du, X.L.; Zhang, X.Y.; Yao, Y.L. Experimental and numerical investigation on impact dynamic performance of steel fiber reinforced concrete beams at elevated temperatures. J. Build. Eng. 2022, 47, 103841. [Google Scholar] [CrossRef]
- Slimane, S.A.; Slimane, A.S.; Guelailia, A.; Boudjemai, A.; Kebdani, S.; Smahat, A.; Mouloud, D. Hypervelocity impact on honeycomb structure reinforced with bi-layer ceramic/aluminum factsheets used for spacecraft shielding. Mech. Adv. Mater. Struct. 2022, 29, 4487–4505. [Google Scholar] [CrossRef]
- Shen, Y.; Lin, L.; Tang, P.; Xu, T. Impact Dynamic Response of Spherical Net Shells: Finite Element-Based Computational Analysis Investigating the Influence of Dynamic Constitutive Models. Buildings 2023, 13, 1849. [Google Scholar] [CrossRef]
- Yang, Q.F.; Zhang, R. Analysis of lateral impact resistance performance of LS-DYNA round steel pipes. Low Temp. Archit. Technol. 2014, 36, 38–41. [Google Scholar] [CrossRef]
- Gupta, N.K.; Venkatesh. Experimental and numerical studies of dynamic axial compression of thin walled spherical shells. Int. J. Impact Eng. 2004, 30, 1225–1240. [Google Scholar] [CrossRef]
- Gupta, N.K.; Mohamed Sheriff, N.R.; Velmurugan. Experimental and numerical investigations into collapse behaviour of thin spherical shells under drop hammer impact. Int. J. Solids Struct. 2007, 44, 3136–3155. [Google Scholar] [CrossRef]
- Gupta, N.K.; Mohamed Sheriff, N.R.; Velmurugan. Analysis of collapse behaviour of combined geometry metallic shells under axial impact. Int. J. Impact Eng. 2008, 35, 731–741. [Google Scholar] [CrossRef]
- Gupta, N.K.; Mohamed Sheriff, N.R.; Velmurugan. Experimental and theoretical studies on buckling of thin spherical shells under axial loads. Int. J. Mech. Sci. 2008, 50, 422–432. [Google Scholar] [CrossRef]
- Fan, F.; Wang, D.Z.; Zhi, X.D.; Shen, S.Z. Failure modes for single-layer reticulated domes under impact loads. Trans. Tianjin Univ. 2008, 14, 545–550. [Google Scholar] [CrossRef]
- Wang, D.; Zhi, X.; Fan, F.; Shen, S. Failure process and energy transmission for single-layer reticulated domes under impact loads. Trans. Tianjin Univ. 2008, 14, 551–557. [Google Scholar] [CrossRef]
- Fan, F.; Wang, F.D.; Zhi, X.D.; Shen, S.Z. Failure modes of reticulated domes subjected to impact and the judgment. Thin Walled Struct. 2010, 48, 143–149. [Google Scholar] [CrossRef]
- Zhi, X.D.; Fan, F.; Shen, S.Z. Elasto-plastic instability of single-layer reticulated shells under dynamic actions. Thin Walled Struct. 2010, 48, 837–845. [Google Scholar] [CrossRef]
- Zhi, X.; Wang, D.; Fan, F. Cost-effectiveness of protective measures for single-layer reticulated domes subject to impact loading. Int. J. Steel Struct. 2012, 12, 311–319. [Google Scholar] [CrossRef]
- Zhai, X.M.; Wang, Y.H.; Huang, M. Performance and protection approach of single-layer reticulated dome subjected to blast loading. Thin Walled Struct. 2013, 73, 57–67. [Google Scholar] [CrossRef]
- Wang, D.Z.; Fan, F.; Zhi, X.D.; Dai, J.W. Failure modes and characteristics of dynamic response for reticulated shells under impact. Eng. Mech. 2014, 31, 180–189. [Google Scholar] [CrossRef]
- Wang, D.Z.; Zhi, X.D.; Fan, F.; Lin, L. The energy-based failure mechanism of reticulated domes subjected to impact. Thin Walled Struct. 2017, 119, 356–370. [Google Scholar] [CrossRef]
- Ma, J.L.; Fan, F.; Wu, C.Q.; Zhi, X.D. Counter-intuitive collapse of single-layer reticulated domes subject to interior blast loading. Thin Walled Struct. 2015, 96, 130–138. [Google Scholar] [CrossRef]
- Ma, X.; Wang, X. Dynamic stability of single-layer reticulated shell structures subjected to impact loads. J. Vib. Shock 2015, 34, 119–124. [Google Scholar] [CrossRef]
- Hu, J.X.; Lu, G.Y.; Yang, H.W.; Yu, T.X.; Xu, J. Dynamic response of internally nested hemispherical shell system to impact loading. Thin Walled Struct. 2017, 120, 29–37. [Google Scholar] [CrossRef]
- Su, Q.Q.; Zhai, X.M. Dynamic response of single-layer reticulated shell with explosion-protection wall under blast loading. Thin Walled Struct. 2018, 127, 389–401. [Google Scholar] [CrossRef]
- Zhi, X.D.; Qi, S.B.; Fan, F.; Zhai, X.M. Experimental and numerical investigations of a single-layer reticulated dome subjected to external blast loading. Eng. Struct. 2018, 176, 103–114. [Google Scholar] [CrossRef]
- Ma, X.; Wang, X.; Bao, C.; Lu, H.; Yang, W. Dynamic Response Analysis and Model Test Research on K6 Single-Layer Spherical Reticulated Shells Subjected to Impact Load. Int. J. Steel Struct. 2019, 19, 1446–1453. [Google Scholar] [CrossRef]
- Nazari, E.; Shekastehband, B. Failure behavior of double-layer-domes subjected to impact. Int. J. Struct. Stab. Dyn. 2021, 21, 2150015. [Google Scholar] [CrossRef]
- Wu, C.; Yang, Y.; Gou, B.; Wu, J. Research on a multi-point impact test of single-layer spherical reticulated shell. J. Constr. Steel Res. 2021, 186, 106897. [Google Scholar] [CrossRef]
- Deepshikha, N.; Keita, I.; Yuki, T.; Ben, S. Higher mode effects of multistorey substructures on the seismic response of double-layered steel grid shell domes. Eng. Struct. 2021, 243, 112677. [Google Scholar] [CrossRef]
- Pilarska, D.; Maleska, T. Numerical analysis of steel geodesic dome under seismic excitations. Materials 2021, 14, 4493. [Google Scholar] [CrossRef]
- Xu, T.L.; Lin, L.; Zhi, X.D.; Fan, F. Experimental study on the impact resistance of single-layer spherical reticulated shell roof panels. Structures 2022, 45, 23–38. [Google Scholar] [CrossRef]
- Xu, J.H.; Zhang, Y.Y.; Zhao, Q.C.; Zhang, L.L. Experiment and simulation analysis on dynamic response of plane cable-membrane structure under impact load. Thin Walled Struct. 2022, 171, 108814. [Google Scholar] [CrossRef]
- Rossot, D.; Machado, R.D.; Barbieri, N.; de Lima, K.F. Experimental and Numerical Study of a Geodesic Dome Under Static and Dynamic Loads and the Influence of Nodal Connections. Exp. Tech. 2022, 46, 823–834. [Google Scholar] [CrossRef]
- Gou, B.L.; Wang, X.L.; Wu, C. Experimental and numerical study on the behavior of single-layer spherical reticulated shells under the combined action of temperature and impact load. Thin Walled Struct. 2023, 182, 110333. [Google Scholar] [CrossRef]
- Wu, C.; Yang, Y.P.; Wang, R.H. Performance of Reticulated Shells under Different Impact Angles. J. Vib. Control 2021, 41, 7–12. [Google Scholar] [CrossRef]
- Zhang, Y.H.; Chen, C.H.; Zhu, X. Ballistic performance of Q235 steel plate subjected to impact by middle and high velocity projectiles. Ship Sci. Technol. 2017, 39, 52–54. [Google Scholar] [CrossRef]






| Material | Q235B |
|---|---|
| Density (ρ)/kg/m3 | 7850 |
| Yield strength (σy)/MPa | 235 |
| Young’s modulus (E)/GPa | 206 |
| Poisson’s ratio (ν) | 0.3 |
| Drop Hammer Heights (mm) | Experimental Results [40] (MPa) | Numerical Results (MPa) | Differences |
|---|---|---|---|
| 8 | −82.94 | −87.10 | 5.0% |
| 10 | −96.70 | −101.21 | 4.7% |
| Comparison Index | Node 1 (m) | Node 2 (m) |
|---|---|---|
| Experiment [40] | −0.231 | −0.025 |
| Simulation | −0.253 | −0.027 |
| Error | 9.52% | 8.00% |
| Impact Parameter | Scope |
|---|---|
| Mass (×103 kg) | 0.5, 1, 5, 10, 20, 50, 100 |
| Velocity (m/s) | 5, 10, 50, 100, 120, 150, 200, 500 |
| Impact Angle (°) | Impact Duration (s) | Maximum Impact Force (kN) | Energy Transfer Rate (%) |
|---|---|---|---|
| 15 | 1.29 | 323.63 | 56.9 |
| 30 | 1.23 | 325.78 | 77.8 |
| 45 | 1.31 | 333.79 | 91.8 |
| 60 | 1.29 | 293.35 | 95.8 |
| 66.25 | 1.27 | 300.99 | 96.06 |
| 75 | 1.21 | 291.74 | 96.04 |
| CoV | 0.028 | 0.054 | 0.168 |
| Impact Angle (°) | Impact Duration (s) | Maximum Impact Force (×103 kN) | Energy Transfer Rate (%) |
|---|---|---|---|
| 15 | 0.015 | 12.28 | 1.07 |
| 30 | 0.017 | 20.22 | 1.05 |
| 45 | 0.012 | 22.48 | 0.95 |
| 60 | 0.008 | 10.64 | 0.90 |
| 66.25 | 0.010 | 5.05 | 0.87 |
| 75 | 0.010 | 7.37 | 0.83 |
| CoV | 0.259 | 0.289 | 0.094 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Chen, G.; Lu, H.; Ke, L.; Wang, H.; Jian, B. Dynamic Responses of Single-Layer Reticulated Shells under Oblique Impact Loading. Buildings 2024, 14, 633. https://doi.org/10.3390/buildings14030633
Li P, Chen G, Lu H, Ke L, Wang H, Jian B. Dynamic Responses of Single-Layer Reticulated Shells under Oblique Impact Loading. Buildings. 2024; 14(3):633. https://doi.org/10.3390/buildings14030633
Chicago/Turabian StyleLi, Pengcheng, Guohan Chen, Hongxin Lu, Lu Ke, Hao Wang, and Bin Jian. 2024. "Dynamic Responses of Single-Layer Reticulated Shells under Oblique Impact Loading" Buildings 14, no. 3: 633. https://doi.org/10.3390/buildings14030633
APA StyleLi, P., Chen, G., Lu, H., Ke, L., Wang, H., & Jian, B. (2024). Dynamic Responses of Single-Layer Reticulated Shells under Oblique Impact Loading. Buildings, 14(3), 633. https://doi.org/10.3390/buildings14030633







