Numerical Simulation of Severe Damage to a Historical Masonry Building by Soil Settlement
Abstract
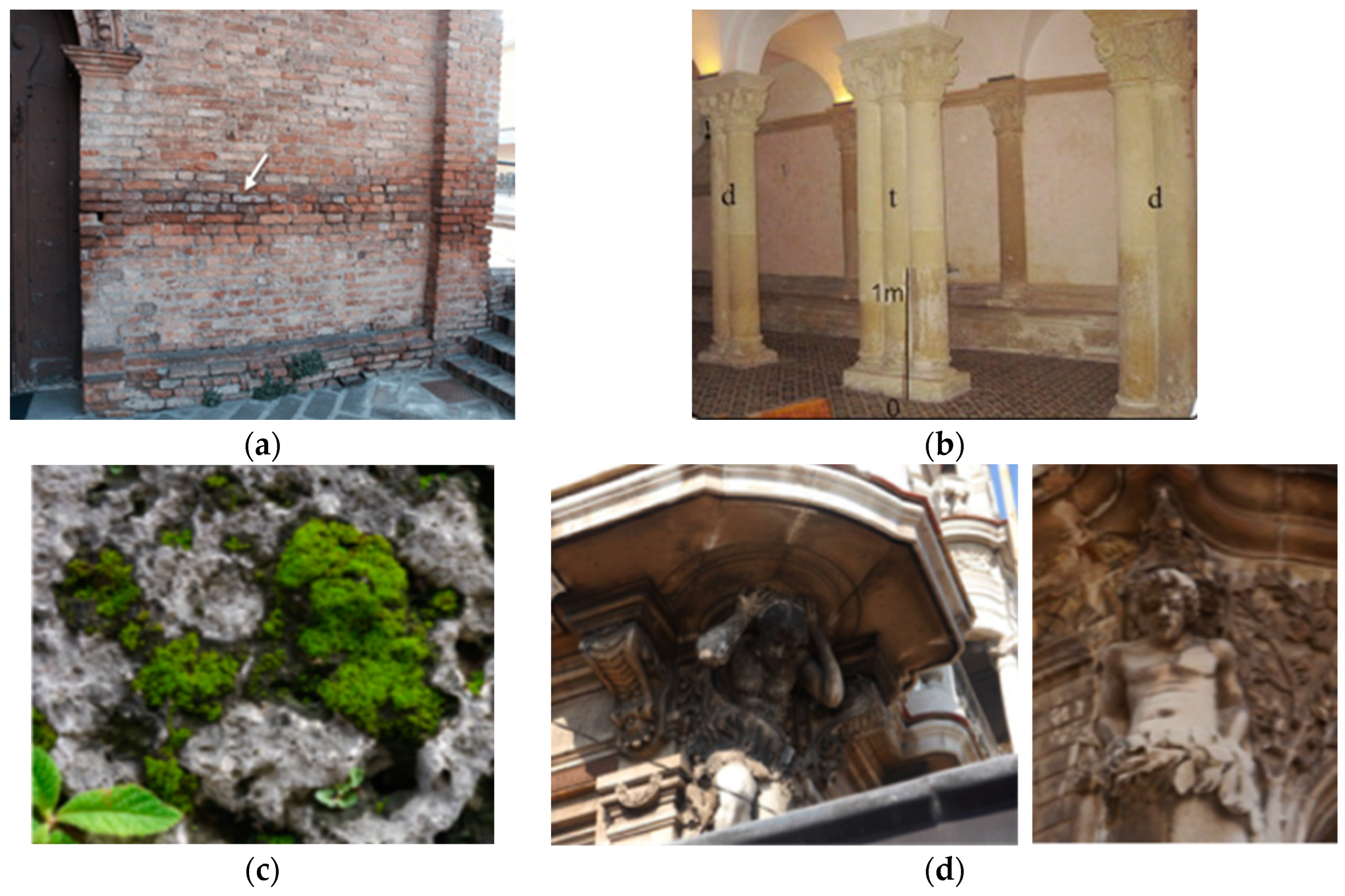
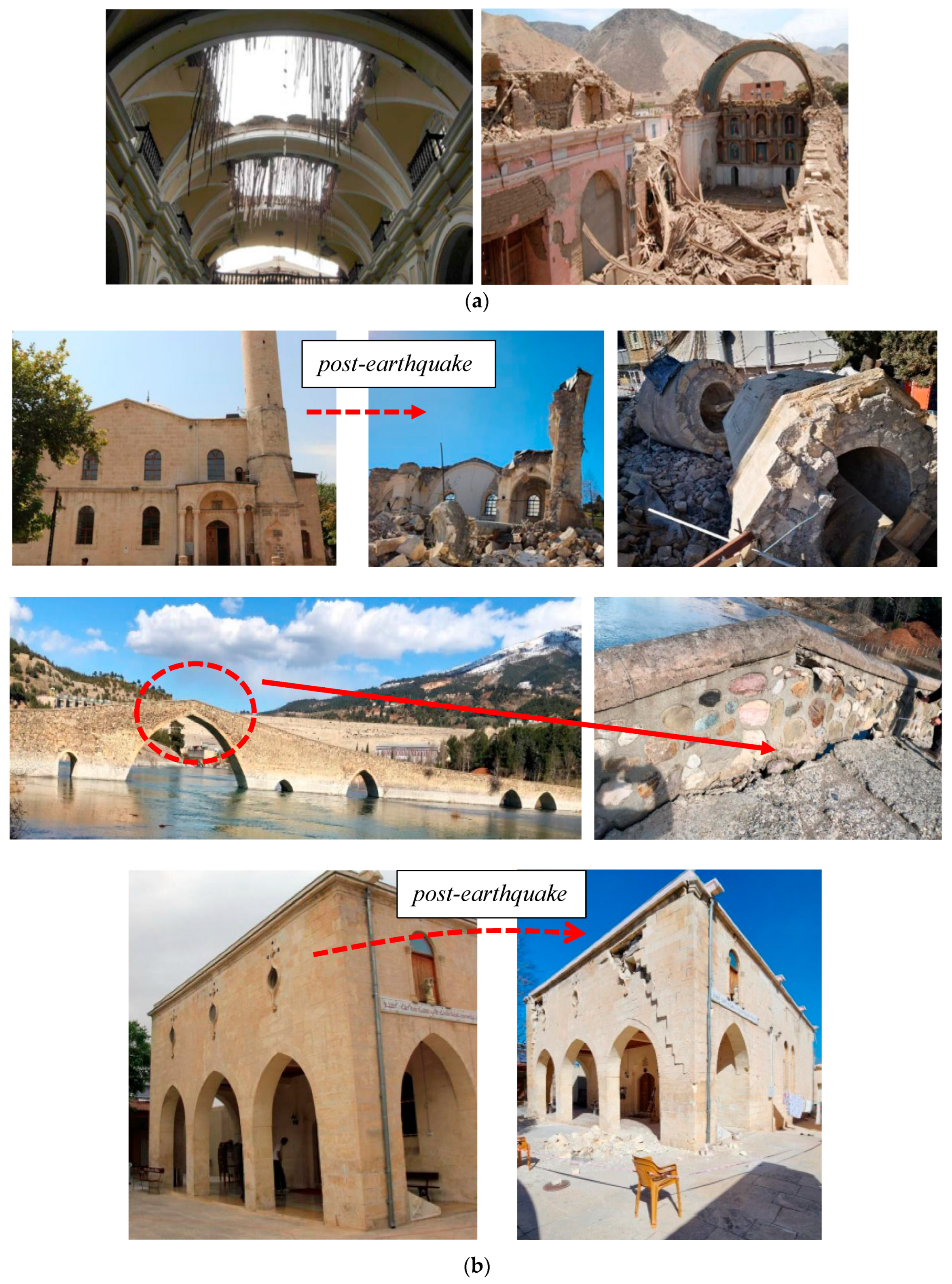
1. Introduction
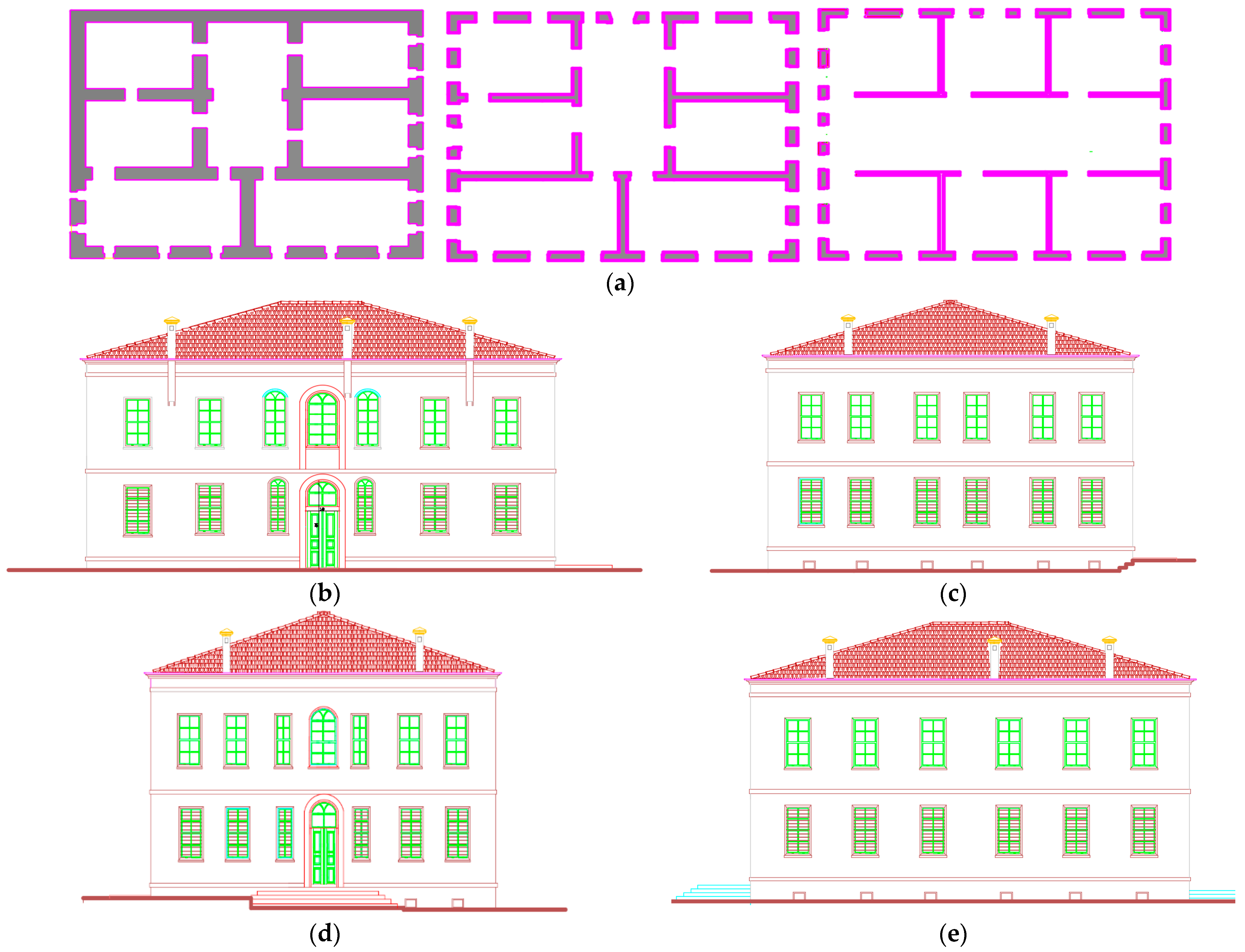
2. Historical Masonry Building
3. Finite Element Model
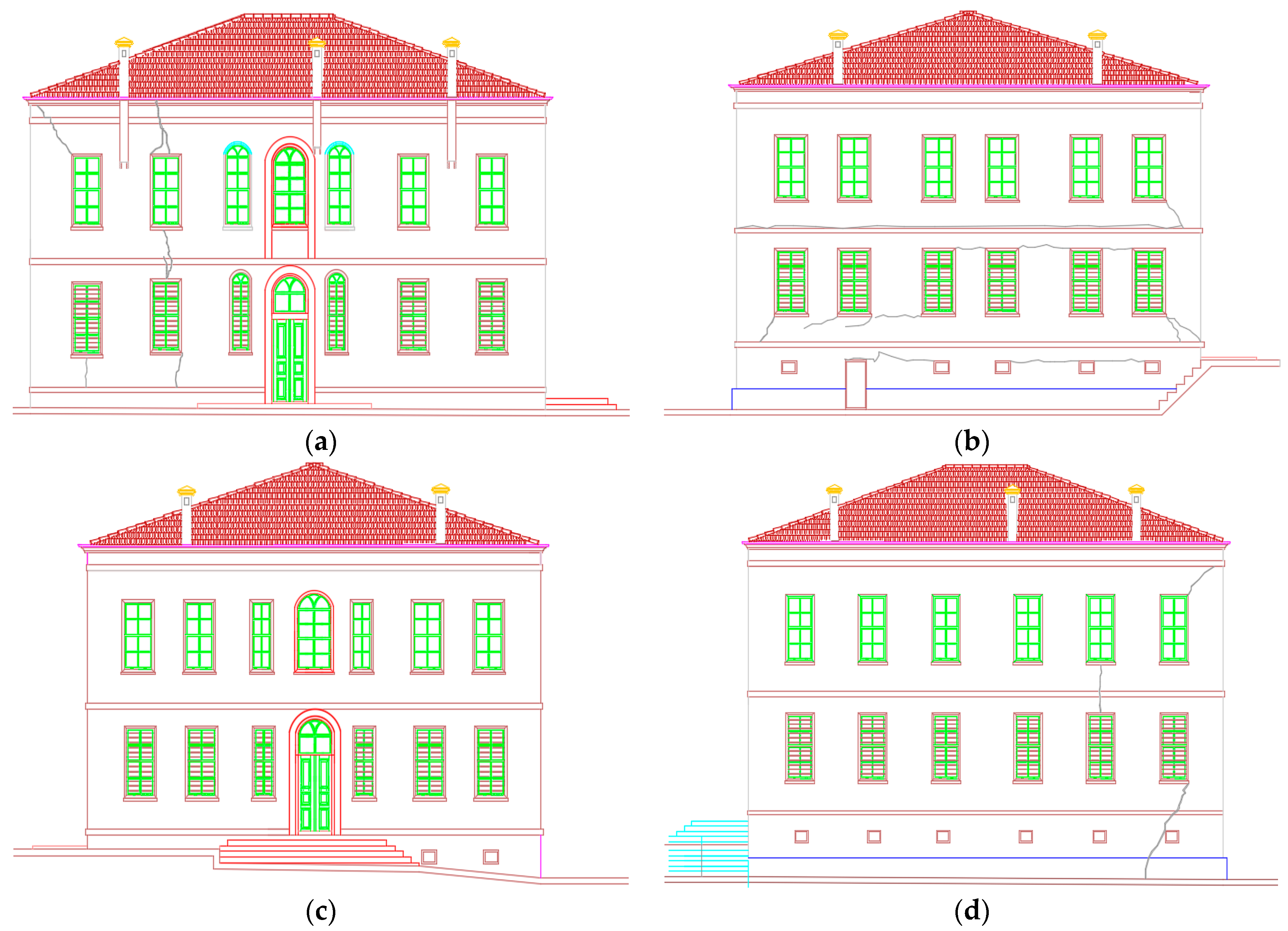
4. Numerical Evaluation
5. Conclusions
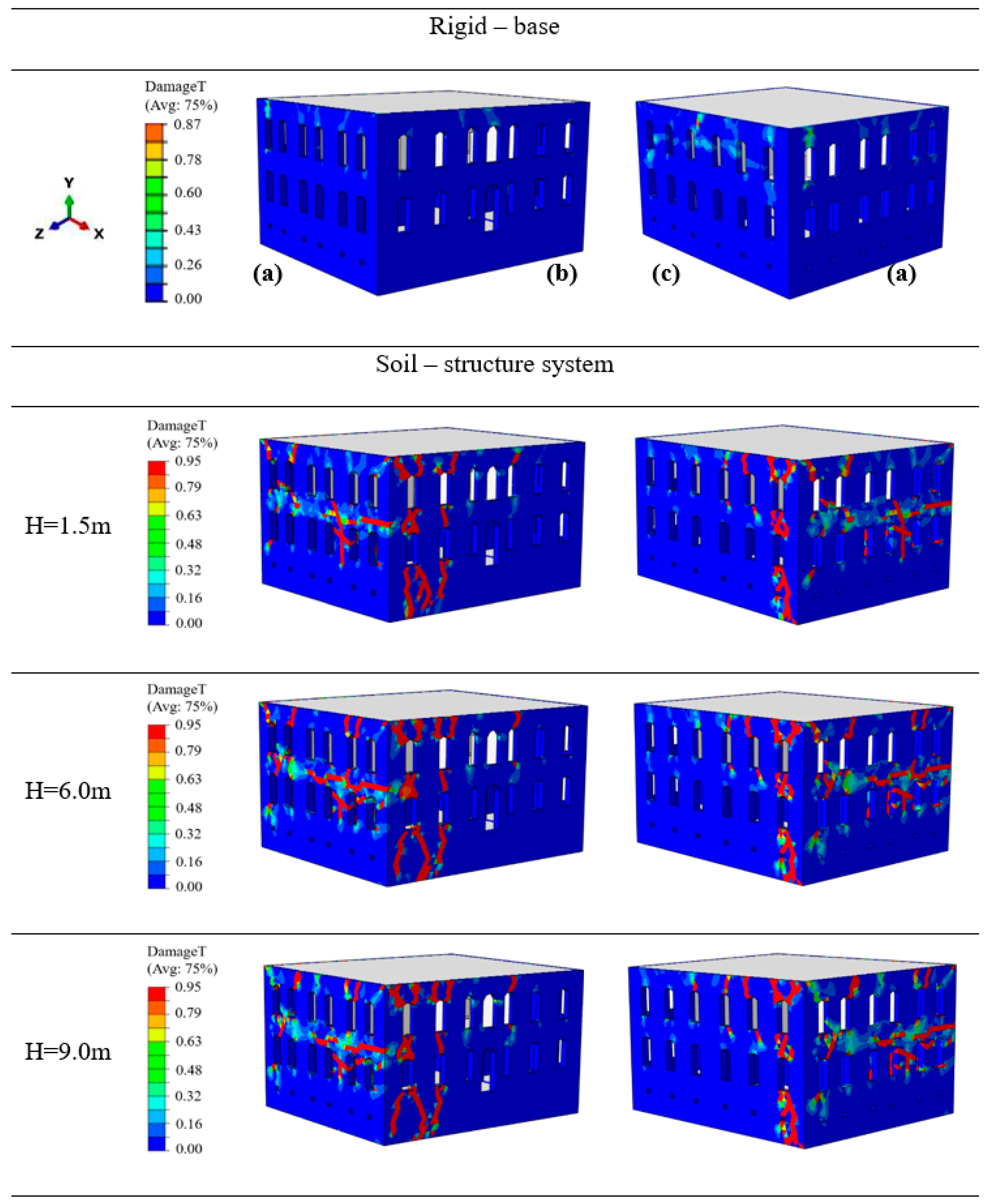
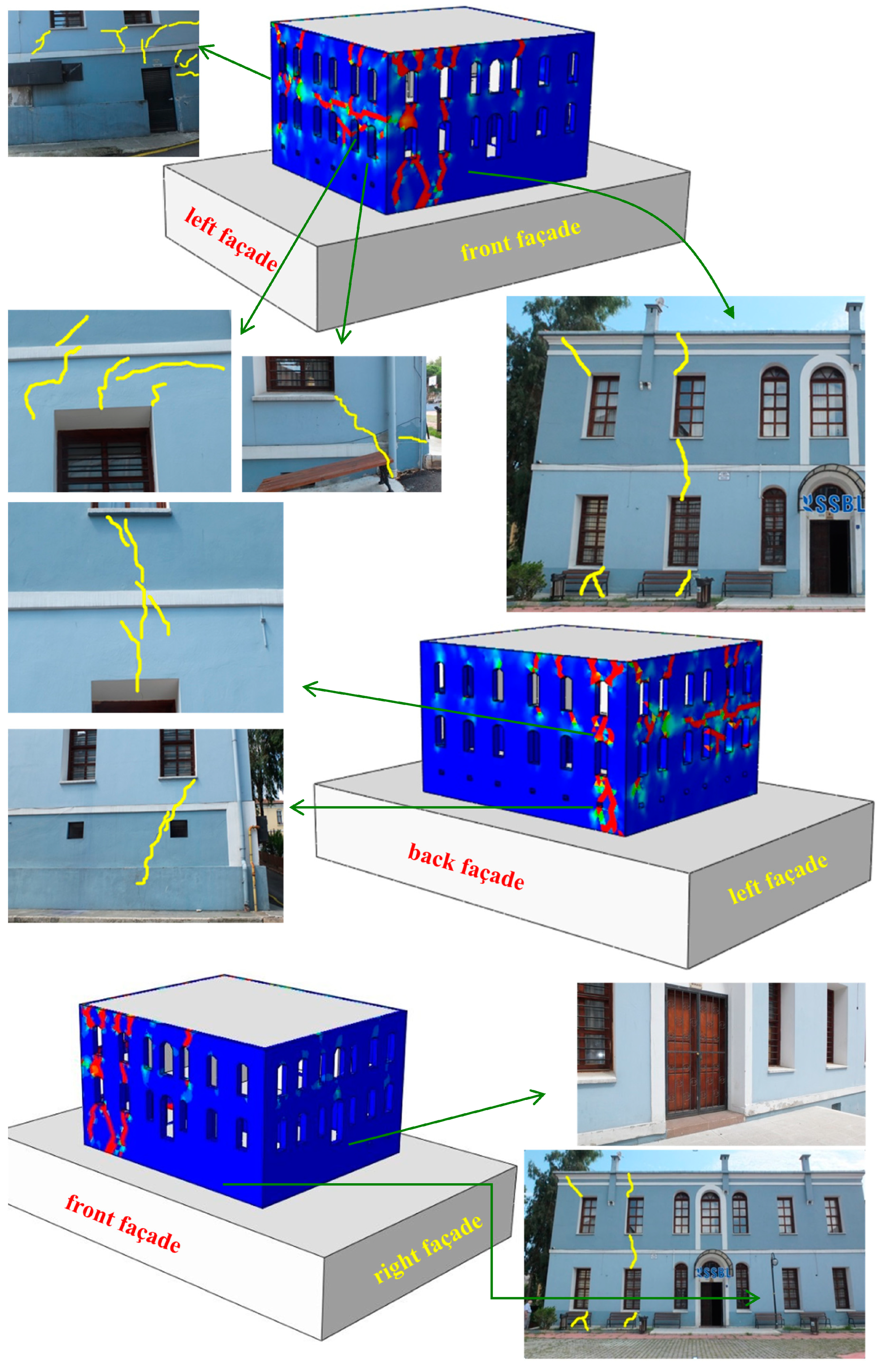
- The direct soil–structure interaction approach provided an effective representation of existing damage to the building due to the soil settlement. But the rigid-base condition lagged the soil–structure interaction approach in the representation of existing damage to the building.
- The soil depth was selected as 1.5 m, 6 m, and 9 m. The maximum displacement values increased with the increasing soil depth. The maximum vertical and horizontal displacement values were obtained as 22 mm and 85 mm. These caused the diagonal and incline tension cracks, which are similar to existing damage propagation on the building.
- The highest damage level was obtained as 4.77% for the soil depth of 6 m. The crack propagation was almost the same for the 6 m and 9 m soil depths. This indicated that the soil depth of 6 m is acceptable to take into consideration the soil–structure interaction. For the effective soil depth, the sum of rigid basement and soil depth can be selected to be equal to total height of the building participating in free vibration.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Giaccone, D.; Santamaria, U.; Corradi, M. An experimental study on the effect of water on historic brickwork masonry. Haritage 2020, 3, 29–46. [Google Scholar] [CrossRef]
- Franzoni, E. Rising damp removal from historical masonries: A still open challenge. Constr. Build. Mater. 2014, 54, 123–136. [Google Scholar] [CrossRef]
- D’Agostino, D. Moisture dynamics in an historical masonry structure: The Cathedral of Lecce (South Italy). Build. Environ. 2013, 63, 122–133. [Google Scholar] [CrossRef]
- Döndüren, M.S.; Şişik, Ö.; Demiröz, A. Types of damage in historical buildings. Selçuk Univ. J. Soc. Tech. Res. 2017, 13, 45–58. (In English) [Google Scholar]
- Erkal, A.; D’Ayala, D.; Stephenson, V. Evaluation of environmental impact on historical stone masonry through on-site monitoring appraisal. Q. J. Eng. Geol. Hydrogeol. 2013, 46, 449–458. [Google Scholar] [CrossRef]
- Hernández, A. Impact of environmental pollution in the historical buildings of Havana, Cuba. Effect of future climate change. Rev. Ing. Constr. 2018, 33, 219–228. [Google Scholar] [CrossRef]
- Gaylarde, C.C. Influence of environment on microbial colonization of historic stone buildings with emphasis on cyanobacteria. Heritage 2020, 3, 1469–1483. [Google Scholar] [CrossRef]
- Cancino, C.; Farneth, S.; Garnier, P.; Vargas Neumann, J.; Webster, F. Damage Assessment of Historic Earthen Buildings after the August 15, 2007 Pisco, Peru Earthquake; The Getty Conservation Institute: Los Angeles, CA, USA, 2009. [Google Scholar]
- FHB. English Heritage: Flooding and Historic Buildings; English Heritage Publishing: Swindon, UK, 2015. [Google Scholar]
- Doğangün, A.; Acar, R.; Livaoğlu, R.; Tuluk, Ö.İ. Performance of masonry minarets against earthquakes and winds in Turkey. In Proceedings of the 1st International Conference on Restoration of Heritage Masonry Structures, Cairo, Egypt, 24–27 April 2006. [Google Scholar]
- Brandonisio, G.; Lucibello, G.; Mele, E.; De Luca, A. Damage and performance evaluation of masonry churches in the 2009 L’Aquila earthquake. Eng. Fail. Anal. 2013, 34, 693–714. [Google Scholar] [CrossRef]
- Indirli, M.; SKouris, L.A.; Formisano, A.; Borg, R.P.; Mazzolani, F.M. Seismic damage assessment of unreinforced masonry structures after The Abruzzo 2009 earthquake: The case study of the historical centers of L’Aquila and Castelvecchio Subequo. Int. J. Archit. Herit. 2013, 7, 536–578. [Google Scholar] [CrossRef]
- Ciocci, M.P.; Sharma, S.; Lourenço, P.B. Engineering simulations of a super-complex cultural heritage building: Ica Cathedral in Peru. Meccanica 2018, 53, 1931–1958. [Google Scholar] [CrossRef]
- Mosoarca, M.; Keller, A.L.; Bocan, C. Failure analysis of church towers and roof structures due to high wind velocities. Eng. Fail. Anal. 2019, 100, 76–87. [Google Scholar] [CrossRef]
- Penna, A.; Calderini, C.; Sorrentino, L.; Carocci, C.F.; Cescatti, E.; Sisti, R.; Prota, A. Damage to churches in the 2016 central Italy earthquakes. Bull. Earthq. Eng. 2019, 17, 5763–5790. [Google Scholar] [CrossRef]
- Al-Taie, E.; Al-Ansari, N.; Knutsson, S. Estimation of settlement under shallow foundation for different regions in Iraq using safe software. Engineering 2015, 7, 379–386. [Google Scholar] [CrossRef]
- Abaqus, 6.14; Dassault Systémes Simulia Corp: Providence, RI, USA, 2014.
- Li, M.; Lu, X.; Lu, X.; Ye, L. Influence of soil structure interaction on seismic collapse resistance of super-tall buildings. J. Rock Mech. Geotech. Eng. 2014, 6, 477–485. [Google Scholar] [CrossRef]
- GMERHS. Guide to the Management of Earthquake Risks of Historical Structures; General Directorate for Foundations: Ankara, Turkey, 2017.
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. A plastic-damage concrete model for earthquake analysis of dams. Earthq. Eng. Struct. Dyn. 1998, 27, 937–956. [Google Scholar] [CrossRef]
- Tiberti, S.; Acito, M.; Milani, G. Comprehensive FE numerical insight into Finale Emilia Castle behavior under 2012 Emilia Romagna seismic sequence: Damage causes and seismic vulnerability mitigation hypothesis. Eng. Struct. 2016, 117, 397–421. [Google Scholar] [CrossRef]
- Kujawa, M.; Lubowiecka, I.; Szymczak, C. Finite element modelling of a historic church structure in the context of a masonry damage analysis. Eng. Fail. Anal. 2020, 107, 104233. [Google Scholar] [CrossRef]
- Wolf, J. Dynamic Soil-Structure Interaction; Prentice Hall: Englewood Clif, NJ, USA, 1985. [Google Scholar]
- Gazetas, G. Formulas and charts for impedances of surface and embedded foundations. J. Geotech. Eng. 1991, 117, 1363–1381. [Google Scholar] [CrossRef]
- Mylonakis, G.; Nikolaou, S.; Gazetas, G. Footings under seismic loading: Analysis and design issues with emphasis on bridge foundations. Soil Dyn. Earthq. Eng. 2006, 26, 824–853. [Google Scholar] [CrossRef]
- NEHRP. Soil-Structure Interaction for Building Structures; NIST GCR 12-917-21; U. S. Department of Commerce National Institute of Standards and Technology: Gaithersburg, MD, USA, 2012.
- Milani, G.; Valente, M. Failure analysis of seven masonry churches severely damaged during the 2012 Emilia-Romagna (Italy) earthquake: Non-linear dynamic analyses vs conventional static approaches. Eng. Fail. Anal. 2015, 54, 13–56. [Google Scholar] [CrossRef]
- Valente, M.; Milani, G. Non-linear dynamic and static analyses on eight historical masonry towers in the North-East of Italy. Eng. Struct. 2016, 114, 241–270. [Google Scholar] [CrossRef]
- Llopis-Pulido, V.; Durá, A.A.; Fenollosa, E.; Martínez, A. Analysis of the structural behavior of the historical constructions: Seismic evaluation of the Cathedral of Valencia (Spain). Int. J. Archit. Herit. 2019, 13, 205–214. [Google Scholar] [CrossRef]
- Lazizi, A.; Tahghighi, H. Seismic Response Evaluation of Kashan Historical Bazaar Structure Including Soil-Structure Interaction. J. Seismol. Earthq. Eng. 2019, 21, 77–93. [Google Scholar] [CrossRef]
- Anastasios, D.; Verstrynge, E.; Szekér, P.; Heirman, G.; Bejarano-Urrego, E.; Giardina, G.; Van Balen, K. Numerical modeling of a church nave wall subjected to differential settlements: Soil-structure interaction, time-dependence and sensitivity analysis. Int. J. Archit. Herit. 2020, 14, 1221–1238. [Google Scholar] [CrossRef]
- Altıok, T.Y.; Demir, A. Collapse mechanism estimation of a historical masonry minaret considered soil-structure interaction. Earthq. Struct. 2021, 21, 161–172. [Google Scholar] [CrossRef]
- Longo, M.; Sousamli, M.; Korswagen, P.A.; Van Staalduinen, P.; Rots, J.G. Sub-structure-based ‘three-tiered’ finite element approach to soil-masonry-wall interaction for light seismic motion. Eng. Struct. 2021, 245, 112847. [Google Scholar] [CrossRef]
- Brunelli, A.; De Silva, F.; Piro, A.; Parisi, F.; Sica, S.; Silvestri, F.; Cattari, S. Numerical simulation of the seismic response and soil–structure interaction for a monitored masonry school building damaged by the 2016 Central Italy earthquake. Bull. Earthq. Eng. 2021, 19, 181–1211. [Google Scholar] [CrossRef]
- Mallardo, V.; Malvezzi, R.; Milani, E.; Milani, G. Seismic vulnerability of historical masonry buildings: A case study in Ferrara. Eng. Struct. 2008, 30, 2223–2241. [Google Scholar] [CrossRef]
- Casalegno, C.; Cecchi, A.; Reccia, E.; Russo, S. Heterogeneous and contınuous models: Comparatıve analysıs of masonry wall subjected to dıfferentıal settlements. Compos. Mech. Comput. Appl. Int. J. 2013, 4, 187–207. [Google Scholar] [CrossRef]
- Shehu, R. Preliminary assessment of the seismic vulnerability of three inclined bell-towers in ferrara, Italy. Int. J. Archit. Herit. 2020, 16, 485–517. [Google Scholar] [CrossRef]
- Ou, W.; Chen, X.; Chan, A.; Cheng, Y.; Wang, H. Fdem simulation on the failure behavior of historic masonry heritages subjected to differential settlement. Buildings 2022, 12, 1592. [Google Scholar] [CrossRef]
- Angjeliu, G.; Bruggi, M.; Taliercio, A. Implementation of an elastic no-tension material model in a sequentially linear analysis framework. Finite Elem. I Anal. Des. 2023, 216, 103891. [Google Scholar] [CrossRef]
- Altıok, T.Y.; Demir, A. Seismic damage assessment of a historical masonry minaret considering soil-structure interaction. J. Struct. Eng. Appl. Mech. 2021, 4, 196–212. [Google Scholar] [CrossRef]
- Hökelekli, E.; Al-Helwani, A. Effect of soil properties on the seismic damage assessment of historical masonry minaret-soil interaction systems. Struct. Des. Tall Spec. Build. 2019, 29, e1694. [Google Scholar] [CrossRef]
- Kramer, S. Geotechnical Earthquake Engineering; Prentice Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]






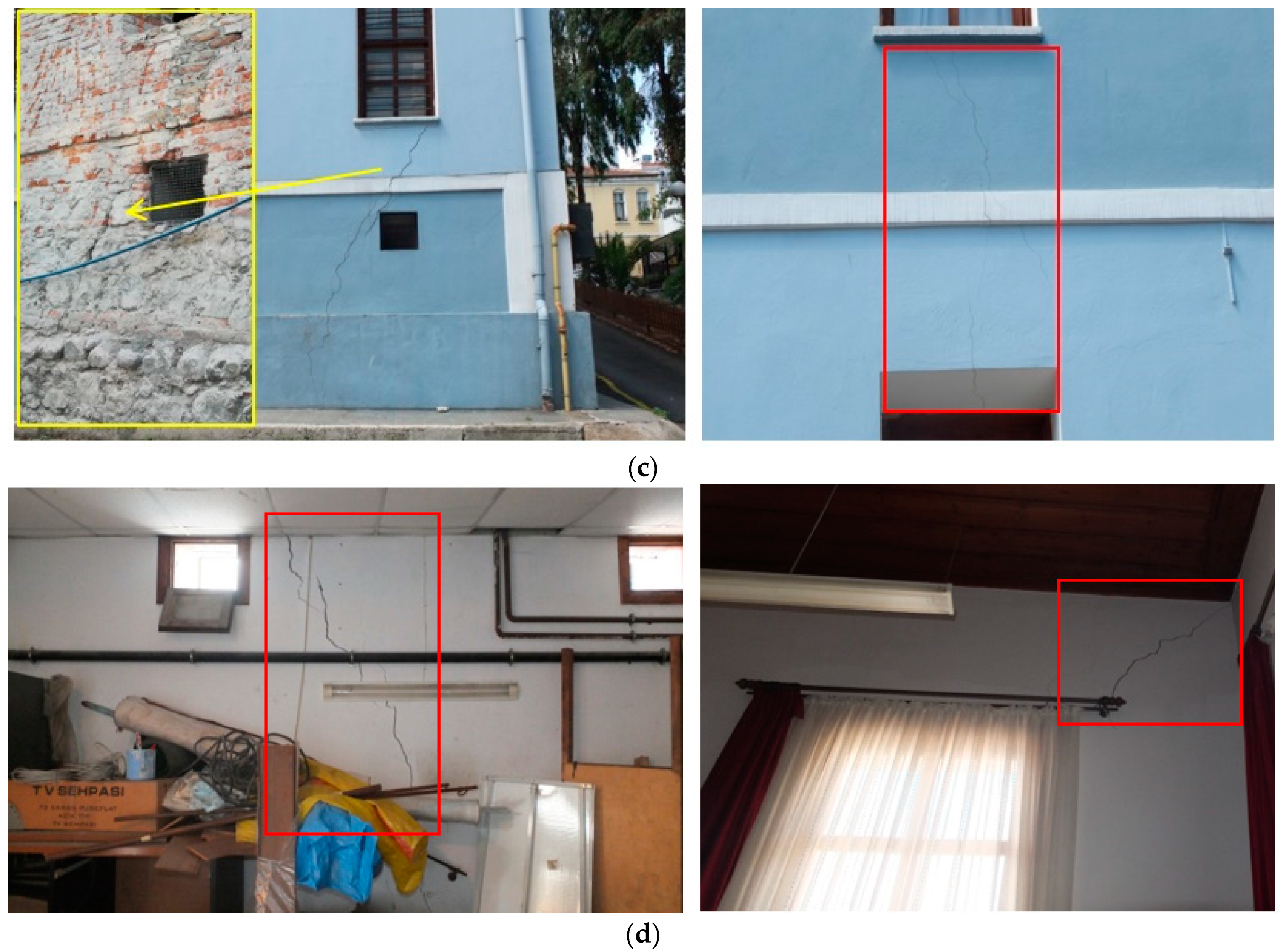

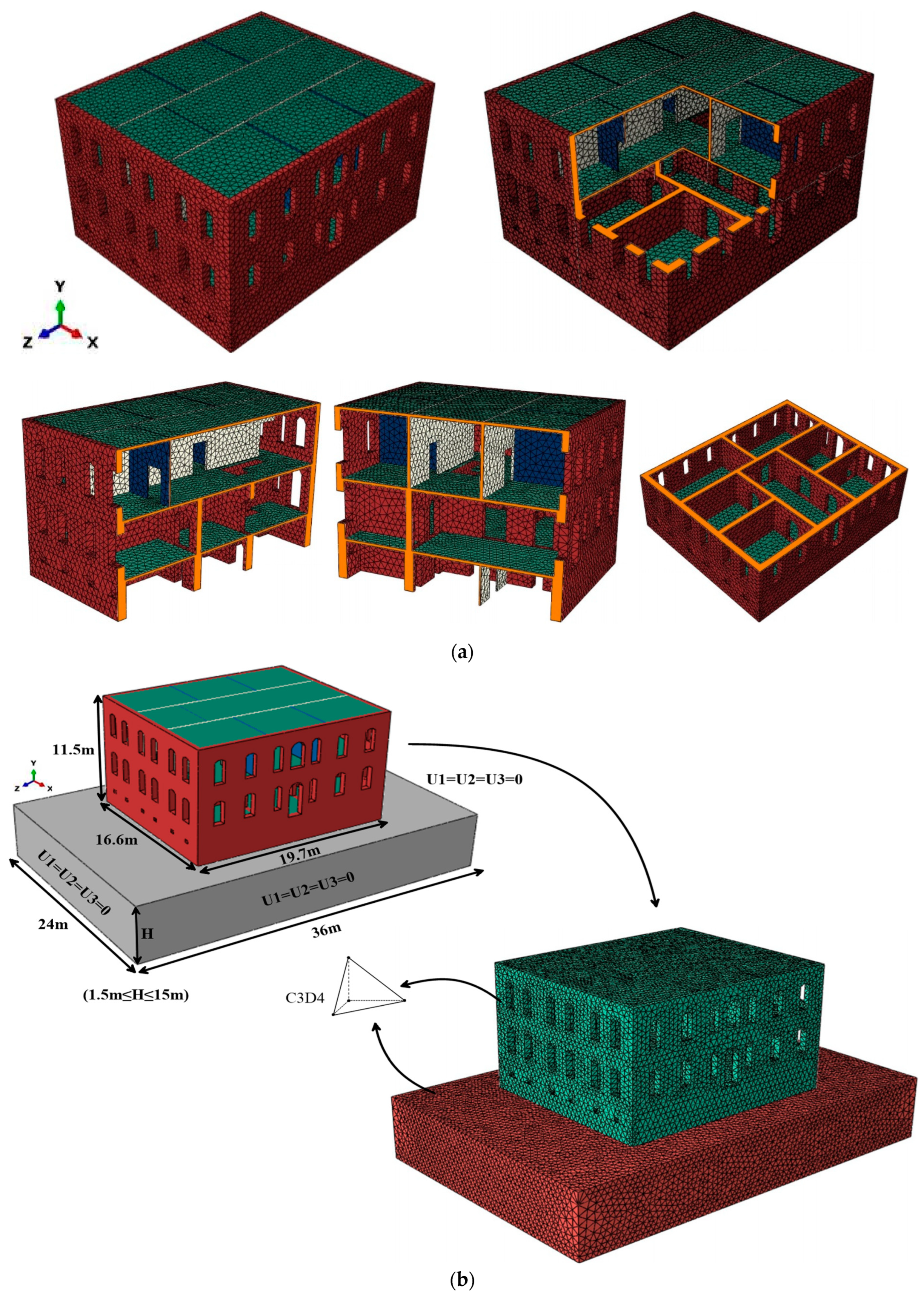

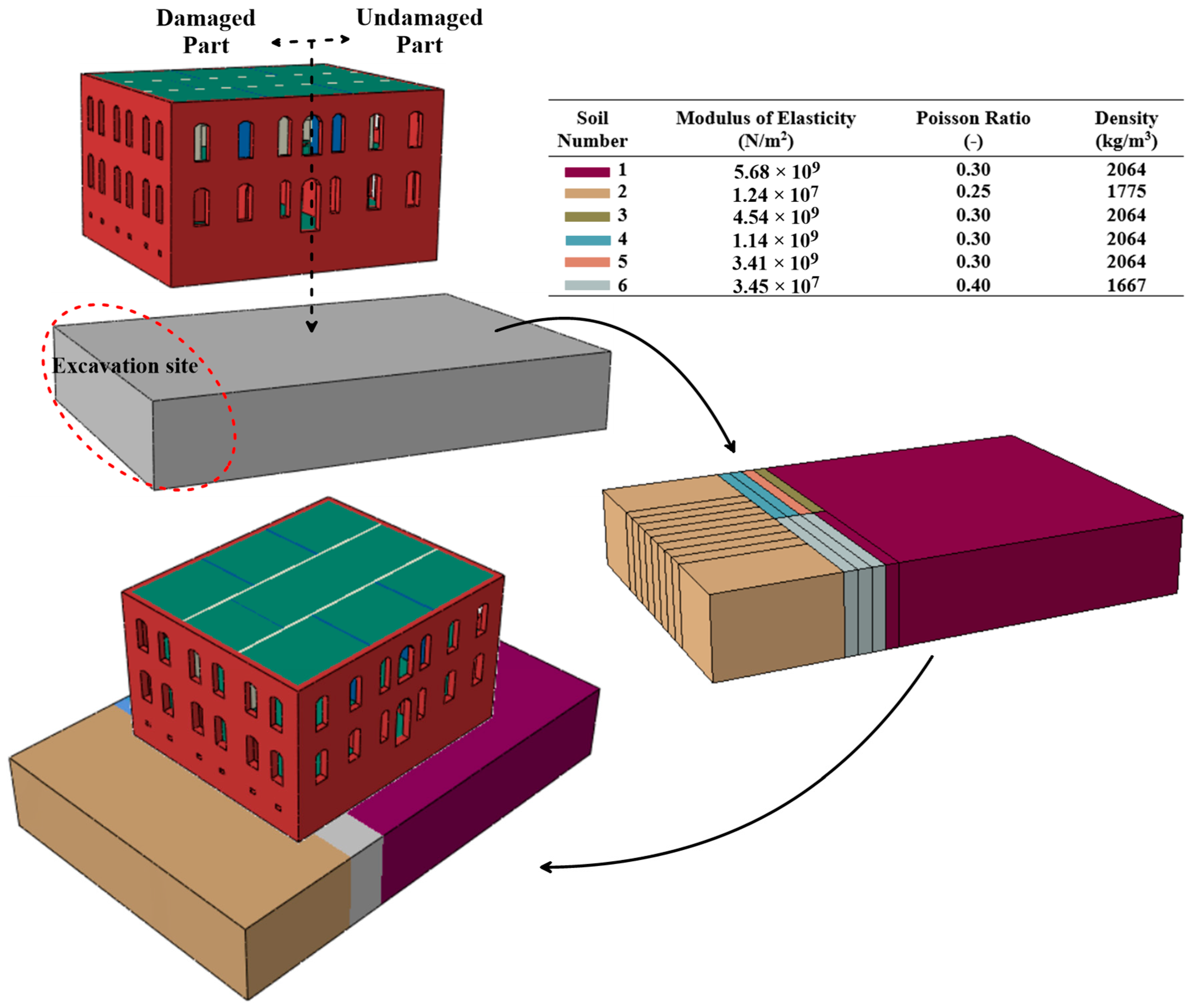

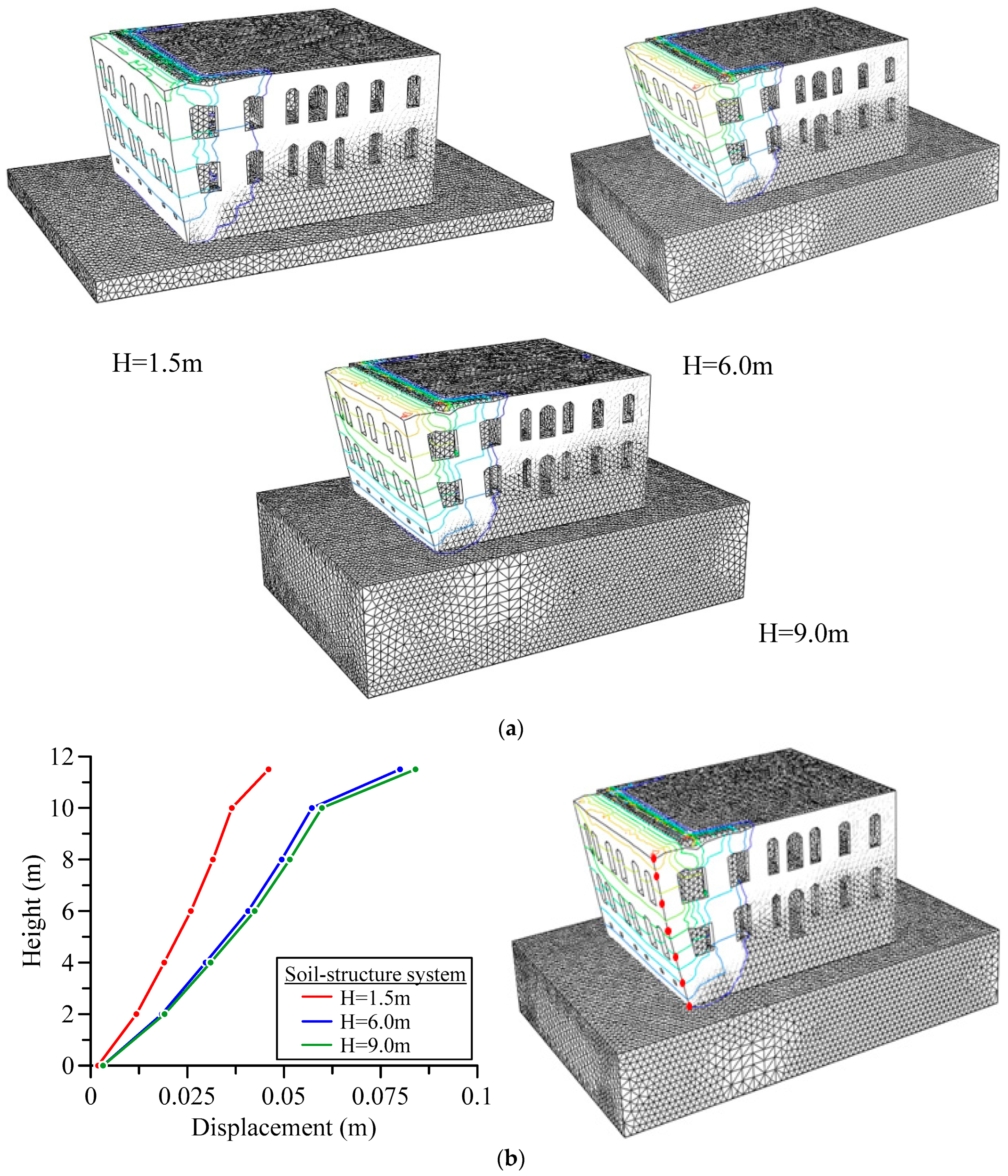
| Element | Elasticity Modulus (N/m2) | Poisson Ratio (-) | Density (kg/m3) |
|---|---|---|---|
| Masonry | 1.8 × 109 | 0.2 | 1800 |
| Dilation Angle | Eccentricity | fb0/fc0 | Kc | Viscosity Parameter |
|---|---|---|---|---|
| 10 | 0.1 | 1.16 | 0.666 | 0.002 |
| Compression | Tension | Tensile Damage Parameters | |||
|---|---|---|---|---|---|
| σ (MPa) | εpl | σ (MPa) | εpl | dt | |
| 1.22 | 0 | 0.04 | 0 | 0 | 0 |
| 0.95 | 0.005 | 0.0005 | 0.003 | 0.95 | 0.003 |
| 0.95 | 0.001 | 0.0005 | 0.100 | ||
| 0.8 | 0.100 | - | - | ||
| System | Maximum Displacement (m) | |
|---|---|---|
| U1 | U3 | |
| Rigid-base | 0.00079 | 0.0034 |
| Diff. (%) | 94.73 | 93.06 |
| Soil–structure system/H = 1.5 m | 0.015 | 0.049 |
| Diff. (%) | 21.05 | 40.24 |
| Soil–structure system/H = 6.0 m | 0.019 | 0.082 |
| Diff. (%) | 13.64 | 3.53 |
| Soil–structure system/H = 9.0 m | 0.022 | 0.085 |
| System | Number of Damaged Elements | * Percentage (%) | |
|---|---|---|---|
| Rigid-base | 0.0 | 0.00 | |
| Soil–structure interaction | H = 1.5 m | 1809 | 4.18 |
| H = 6.0 m | 2067 | 4.77 | |
| H = 9.0 m | 1941 | 4.48 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ertürk Atmaca, E.; Genç, A.F.; Altunişik, A.C.; Günaydin, M.; Sevim, B. Numerical Simulation of Severe Damage to a Historical Masonry Building by Soil Settlement. Buildings 2023, 13, 1973. https://doi.org/10.3390/buildings13081973
Ertürk Atmaca E, Genç AF, Altunişik AC, Günaydin M, Sevim B. Numerical Simulation of Severe Damage to a Historical Masonry Building by Soil Settlement. Buildings. 2023; 13(8):1973. https://doi.org/10.3390/buildings13081973
Chicago/Turabian StyleErtürk Atmaca, Esin, Ali Fuat Genç, Ahmet Can Altunişik, Murat Günaydin, and Barış Sevim. 2023. "Numerical Simulation of Severe Damage to a Historical Masonry Building by Soil Settlement" Buildings 13, no. 8: 1973. https://doi.org/10.3390/buildings13081973
APA StyleErtürk Atmaca, E., Genç, A. F., Altunişik, A. C., Günaydin, M., & Sevim, B. (2023). Numerical Simulation of Severe Damage to a Historical Masonry Building by Soil Settlement. Buildings, 13(8), 1973. https://doi.org/10.3390/buildings13081973






