Bridging Law Application to Fracture of Fiber Concrete Containing Oil Shale Ash
Abstract
1. Introduction
2. Experimental Work
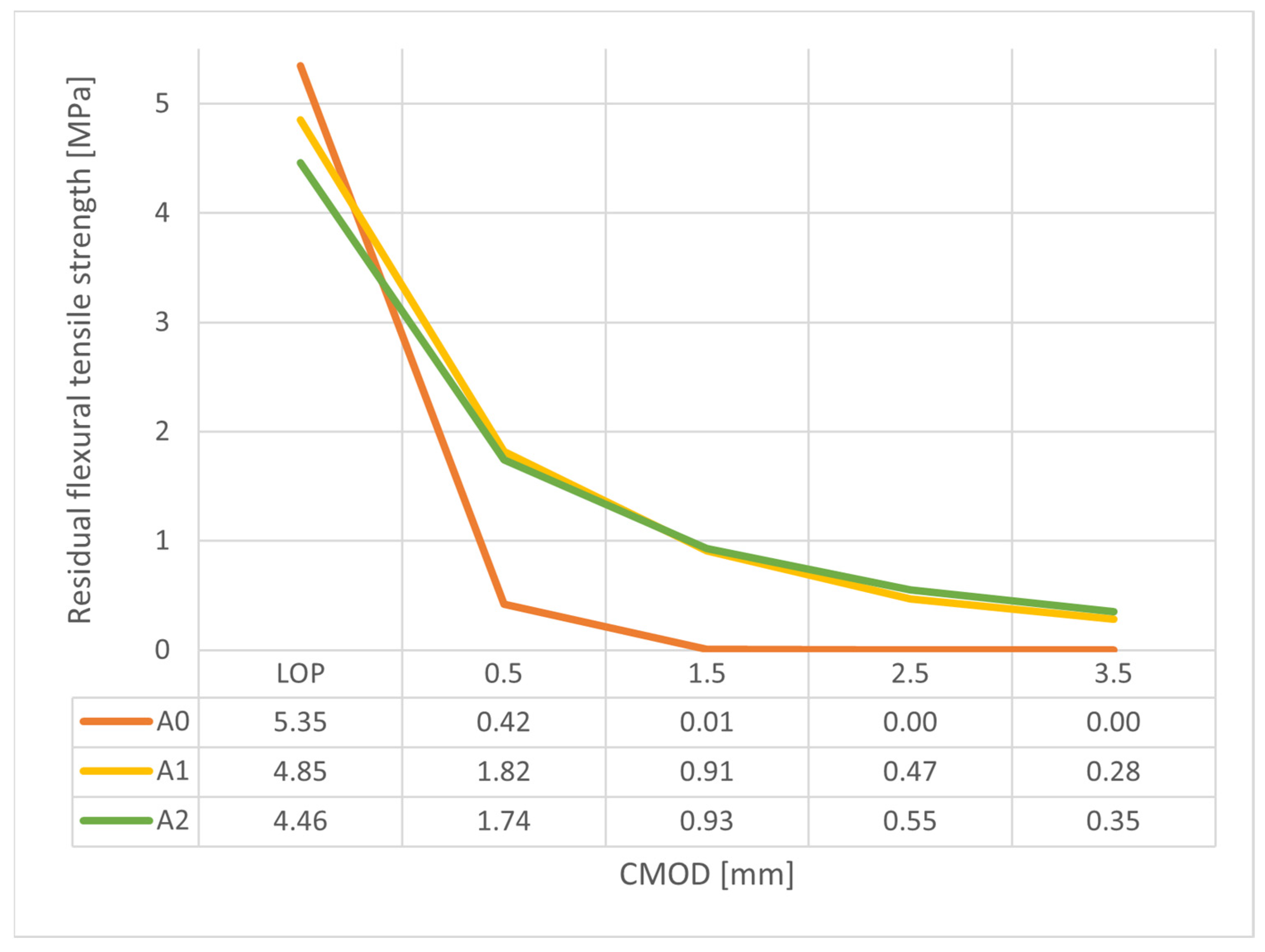
2.1. Mix Design with Composite Basalt Fibers for Three-Point Bending Test Experiments
2.2. Fabrication of Basalt Fiber Concrete with OSA for Four-Point Bending Test Experiments
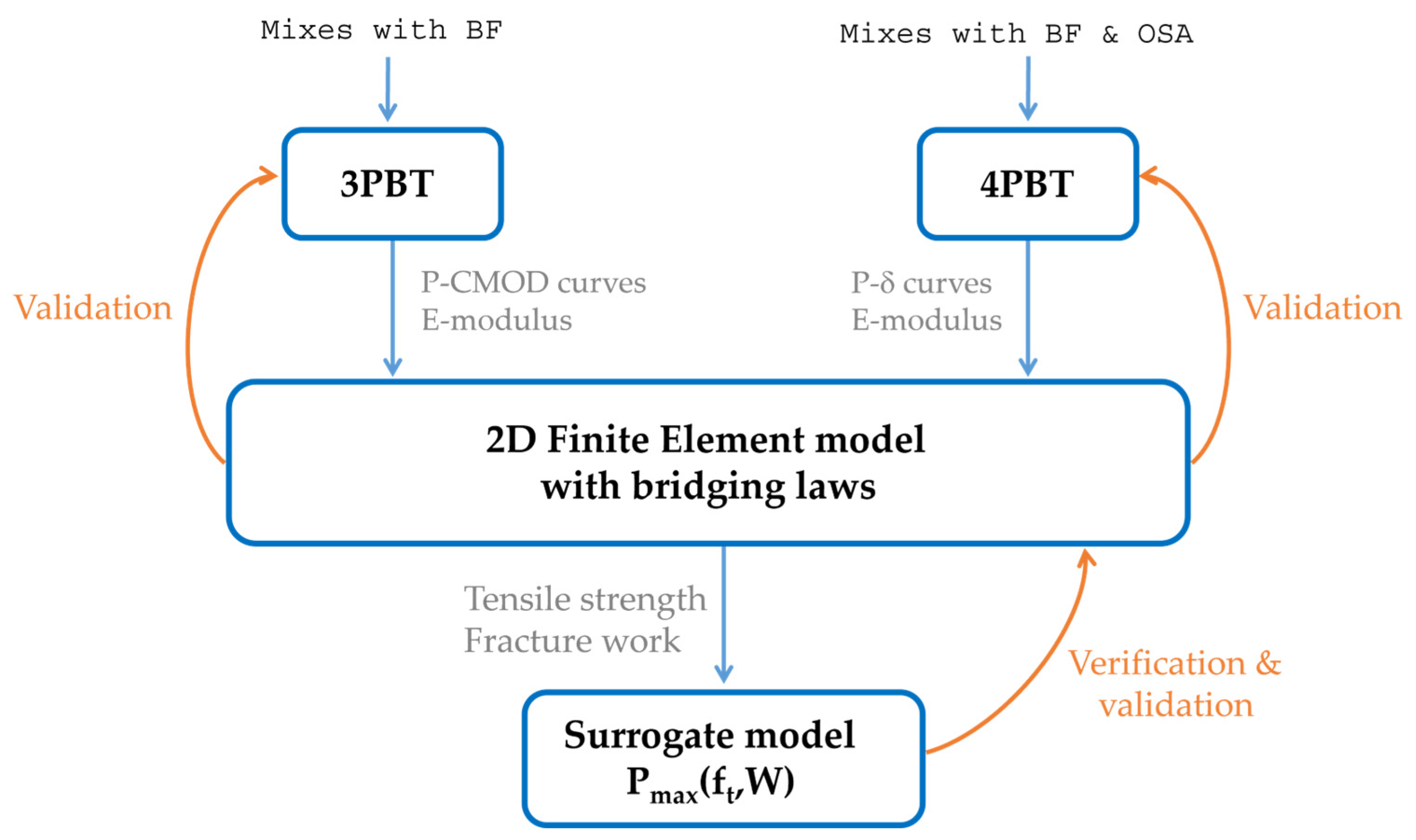
3. Modeling Methodology of Crack Propagation and Related Parameters
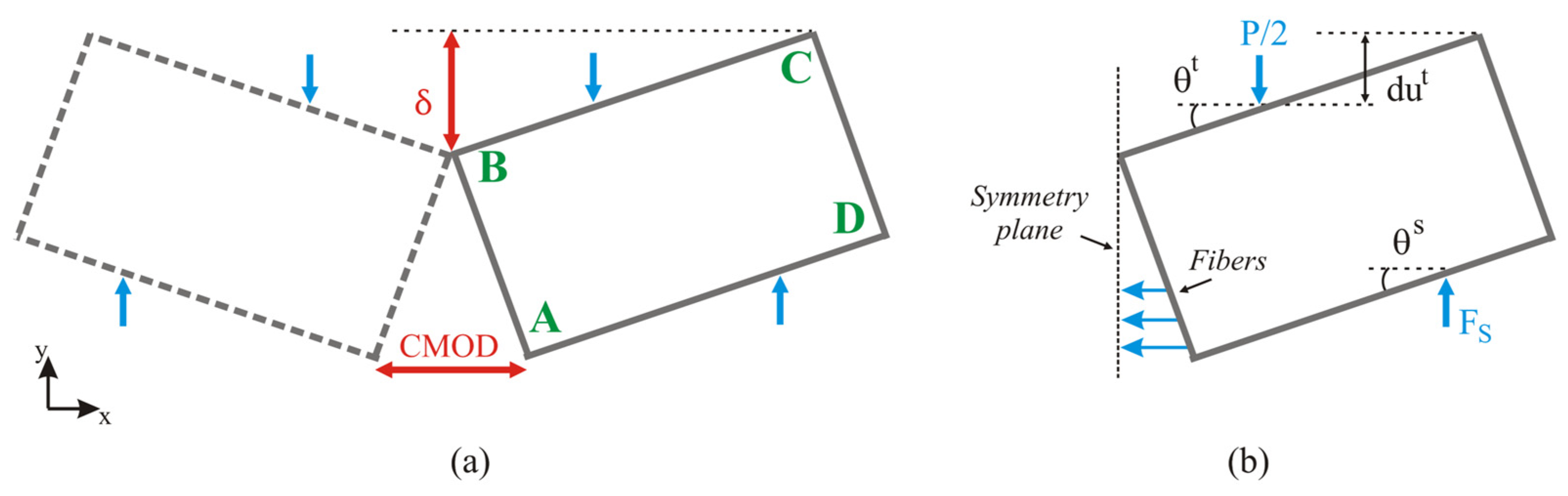
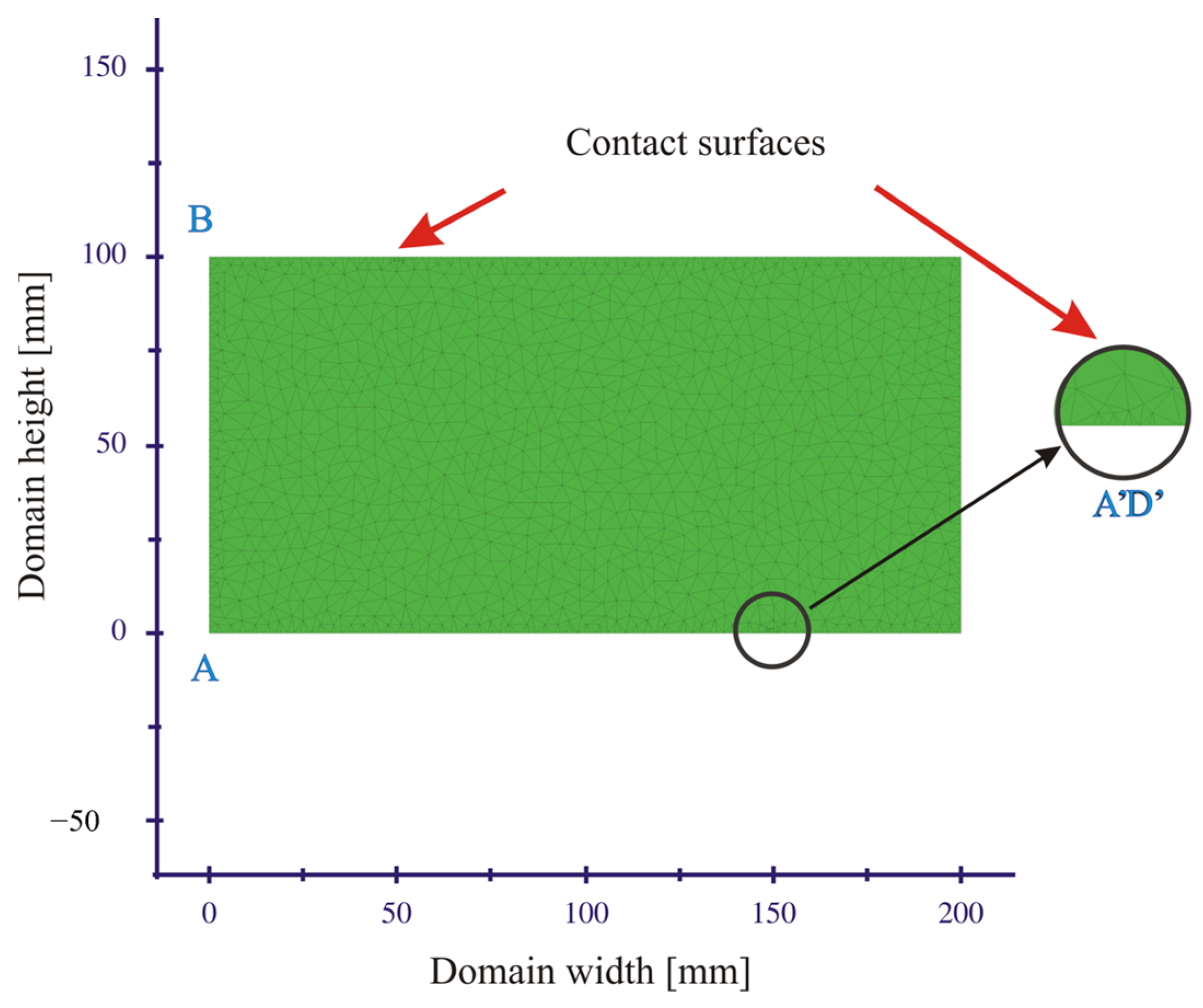
3.1. Finite Element Model
- The constant displacement dus = 0 and the rotation angle θs = 0 is defined for the support (lower) roller. The roller of the test machine (upper) is loaded with the displacement dut, but the angle θt = 0 (see Figure 4).
- The constant displacement dus = 0 is defined for the support roller; it is loaded with the rotation angle dθs. For the roller of the test machine, the displacement is constant dut = 0, and the angle is zero.
- The support roller has the constant displacement dus = 0 and the rotation angle θs = 0, while the roller of the test machine is loaded with the rotation angle dθt, and the displacement is constant dut = 0.
- The support roller has the constant displacement dus = 0, but it is loaded with the rotation angle dθs, while the roller of the test machine is loaded with the displacement dut, but θt = 0.
- The support roller has a constant displacement dus = 0, and the rotation angle is zero. The roller of the test machine is loaded with both the displacement dut and the angle of rotation dθt.
- Both rollers are loaded with the angle of rotation, but the displacements of both rollers are constant.
3.2. Analyzed Bridging Law Functions
3.2.1. Bilinear Bridging Law Function
3.2.2. Nonlinear Bridging Law Function
3.3. Surrogate Modeling
4. Numerical Examples
4.1. Three-Point Bending Tests
4.2. Four-Point Bending Tests
4.3. Surrogate Model to Predict Maximum Load in 4PBT
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
- (1)
- Data input/output and memory management;
- (2)
- Two-dimensional mesh generator and 3D mesh automatic generator by mesh extrude method;
- (3)
- Common sparse FEM matrix generator by energy minimum and Galerkin methods;
- (4)
- Solver of linear real/complex equation system, direct, and Krylov space methods;
- (5)
- Plastic flow modulus, dynamic, Prager, and Armstrong–Frederich types of plasticity;
- (6)
- Linear-elastic, thermal-elastic and plastic/triangular, tetrahedral, and beam elements;
- (7)
- The procedural script interpretation modulus gives access to all numerical modules mentioned, used for boundary conditions and extra physical feature coding.
References
- UN Environment; Scrivener, K.L.; John, V.M.; Gartner, E.M. Eco-efficient cements: Potential economically viable solutions for a low-CO2 cement-based materials industry. Cem. Concr. Res. 2018, 114, 2–26. [Google Scholar] [CrossRef]
- Low-Carbon Concrete and Construction: A Review of Green Public Procurement Programmes. Available online: https://www.iea.org/reports/cement (accessed on 5 May 2023).
- Uibu, M.; Somelar, P.; Raado, L.-M.; Irha, N.; Hain, T.; Koroljova, A.; Kuusik, R. Oil shale ash based backfilling concrete—Strength development, mineral transformations and leachability. Constr. Build. Mater. 2016, 102, 620–630. [Google Scholar] [CrossRef]
- Hemalatha, T.; Ramaswamy, A. A review on fly ash characteristics—Towards promoting high volume utilization in developing sustainable concrete. J. Clean. Prod. 2017, 147, 546–559. [Google Scholar] [CrossRef]
- Irha, N.; Uibu, M.; Jefimova, J.; Raado, L.-M.; Hain, T.; Kuusik, R. Leaching behaviour of Estonian oil shale ash-based construction mortars. Oil Shale 2014, 31, 394–411. [Google Scholar] [CrossRef]
- Othman, H.; Sabrah, T.; Marzouk, H. Conceptual design of ultra-high performance fiber reinforced concrete nuclear waste container. Nucl. Eng. Technol. 2018, 51, 588–599. [Google Scholar] [CrossRef]
- Carvalho, M.R.; Barros, J.; Zhang, Y.; Dias-Da-Costa, D. A computational model for simulation of steel fibre reinforced concrete with explicit fibres and cracks. Comput. Methods Appl. Mech. Eng. 2020, 363, 112879. [Google Scholar] [CrossRef]
- Dhand, V.; Mittal, G.; Rhee, Y.K.; Park, S.J.; Hui, D. A short review on basalt fibre reinforced polymer composites. Composites Part B Eng. 2015, 73, 166–180. [Google Scholar] [CrossRef]
- Alnahhal, W.; Aljidda, O. Flexural behavior of basalt fiber reinforced concrete beams with recycled concrete coarse aggregates. Constr. Build. Mater. 2018, 169, 165–178. [Google Scholar] [CrossRef]
- Novakova, I.; Thorhallsson, E.R.; Wallevik, O.H. Influence of environmentally friendly basalt fibres on early-age strength development. In Proceedings of the Fib Symposium Kraków, Krakow, Poland, 27–29 May 2019; pp. 266–273. [Google Scholar]
- Bheel, N. Basalt fibre-reinforced concrete: Review of fresh and mechanical properties. J. Build. Pathol. Rehabilitation 2021, 6, 12. [Google Scholar] [CrossRef]
- Monaldo, E.; Nerilli, F.; Vairo, G. Basalt-based fiber-reinforced materials and structural applications in civil engineering. Compos. Struct. 2019, 214, 246–263. [Google Scholar] [CrossRef]
- George, E.H.; Bhuvaneshwari, B.; Palani, G.S.; Sakaria, P.E.; Iyer, N.R. Effect of Basalt Fibre on Mechanical Properties of Concrete Containing Fly Ash and Metakaolin. Int. J. Innov. Res. Sci. Eng. Technol. 2014, 3, 444–451. [Google Scholar]
- Jia, Z.-M.; Zhou, X.-P. Field-enriched finite element method for simulating complex cracks in brittle solids. Eng. Fract. Mech. 2022, 268, 108504. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L.; Zhu, J.Z. The Finite Element Method: Its Basis and Fundamentals, 7th ed.; Butterworth Heinemann: Oxford, UK, 2013. [Google Scholar] [CrossRef]
- Quek, S.S.; Liu, G.R. The Finite Element Method: A Practical Course; Butterworth-Heinemann: Oxford, UK, 2003. [Google Scholar]
- Khormani, M.; Jaari, V.R.K.; Aghayan, I.; Ghaderi, S.H.; Ahmadyfard, A. Compressive strength determination of concrete specimens using X-ray computed tomography and finite element method. Constr. Build. Mater. 2020, 256, 119427. [Google Scholar] [CrossRef]
- Le, L.A.; Nguyen, G.D.; Bui, H.H.; Sheikh, A.H.; Kotousov, A. Incorporation of micro-cracking and fibre bridging mechanisms in constitutive modelling of fibre reinforced concrete. J. Mech. Phys. Solids 2019, 133, 103732. [Google Scholar] [CrossRef]
- Gu, Y.; Zhang, C. Novel special crack-tip elements for interface crack analysis by an efficient boundary element method. Eng. Fract. Mech. 2020, 239, 107302. [Google Scholar] [CrossRef]
- Li, C.; Niu, Z.; Hu, Z.; Hu, B.; Cheng, C. Effectiveness of the stress solutions in notch/crack tip regions by using extended boundary element method. Eng. Anal. Bound. Elements 2019, 108, 1–13. [Google Scholar] [CrossRef]
- Jiang, S.; Gu, Y.; Fan, C.-M.; Qu, W. Fracture mechanics analysis of bimaterial interface cracks using the generalized finite difference method. Theor. Appl. Fract. Mech. 2021, 113, 102942. [Google Scholar] [CrossRef]
- Yan, C.; Zheng, Y.; Wang, G. A 2D adaptive finite-discrete element method for simulating fracture and fragmentation in geomaterials. Int. J. Rock Mech. Min. Sci. 2023, 169, 105439. [Google Scholar] [CrossRef]
- Ren, H.; Song, S.; Ning, J. Damage evolution of concrete under tensile load using discrete element modeling. Theor. Appl. Fract. Mech. 2022, 122, 103622. [Google Scholar] [CrossRef]
- Planas, J.; Elices, M.; Guinea, G.; Gómez, F.; Cendón, D.; Arbilla, I. Generalizations and specializations of cohesive crack models. Eng. Fract. Mech. 2003, 70, 1759–1776. [Google Scholar] [CrossRef]
- Elices, M.; Rocco, C.; Roselló, C. Cohesive crack modelling of a simple concrete: Experimental and numerical results. Eng. Fract. Mech. 2009, 76, 1398–1410. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Planas, J. Fracture and Size Effect in Concrete and Other Quasibrittle Materials; Taylor & Francis: New York, NY, USA, 1998; 640p. [Google Scholar]
- Ambati, M.; Gerasimov, T.; De Lorenzis, L. A review on phase-field models of brittle fracture and a new fast hybrid formulation. Comput. Mech. 2014, 55, 383–405. [Google Scholar] [CrossRef]
- Lammen, H.; Conti, S.; Mosler, J. A finite deformation phase field model suitable for cohesive fracture. J. Mech. Phys. Solids 2023, 178, 105349. [Google Scholar] [CrossRef]
- Lateef, H.A.; Laftah, R.M.; Jasim, N.A. Investigation of crack propagation in plain concrete using Phase-field model. Mater. Today Proc. 2022, 57, 375–382. [Google Scholar] [CrossRef]
- Feng, D.-C.; Wu, J.-Y. Phase-field regularized cohesive zone model (CZM) and size effect of concrete. Eng. Fract. Mech. 2018, 197, 66–79. [Google Scholar] [CrossRef]
- Li, H.; Huang, Y.; Yang, Z.; Yu, K.; Li, Q. 3D meso-scale fracture modelling of concrete with random aggregates using a phase-field regularized cohesive zone model. Int. J. Solids Struct. 2022, 256, 111960. [Google Scholar] [CrossRef]
- Niu, Y.; Wei, J.; Jiao, C. Multi-scale fiber bridging constitutive law based on meso-mechanics of ultra high-performance concrete under cyclic loading. Constr. Build. Mater. 2022, 354, 129065. [Google Scholar] [CrossRef]
- Qiu, J.; Yang, E.-H. A micromechanics-based fatigue dependent fiber-bridging constitutive model. Cem. Concr. Res. 2016, 90, 117–126. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modéer, M.; Petersson, P.-E. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements. Cem. Concr. Res. 1976, 6, 773–781. [Google Scholar] [CrossRef]
- Guinea, G.V.; Planas, J.; Elices, M. A general bilinear fit for the softening curve of concrete. Mater. Struct. 1994, 27, 99–105. [Google Scholar] [CrossRef]
- Fathy, A.M.; Sanz, B.; Sancho, J.M.; Planas, J. Determination of the bilinear stress-crack opening curve for normal- and high-strength concrete. Fatigue Fract. Eng. Mater. Struct. 2008, 31, 539–548. [Google Scholar] [CrossRef]
- Cornelissen, H.A.W.; Hordijk, D.; Reinhardt, H. Experimental determination of crack softening characteristics of normalweight and lightweight concrete. HERON 1986, 31, 45–56. [Google Scholar]
- Karihaloo, B.L. Fracture Mechanics and Structural Concrete, Concrete Design and Construction Series; Longman Scientific & Technical: London, UK, 1995. [Google Scholar]
- Lukasenoks, A.; Macanovskis, A.; Krasņikovs, A.; Lapsa, V. Composite fiber pull-out in concretes with various strengths. In Proceedings of the 15th International Sci-entific Conference on Engineering for Rural Development, Jelgava, Latvia, 25 May 2016; pp. 1417–1423. [Google Scholar]
- Macanovskis, A.; Lukasenoks, A.; Krasnikovs, A.; Stonys, R.; Lusis, V. Composite fibers in concretes with various strengths. ACI Mater. J. 2018, 115, 647–652. [Google Scholar] [CrossRef]
- Lapsa, V.; Krasnikovs, A. Composite Material Fiber and its Production Process. Latvian Patent LV15339, 20 March 2021. [Google Scholar]
- MiniBars. Available online: https://reforcetech.com/technology/minibars/ (accessed on 11 July 2023).
- Ramesh, B.; Eswari, S. Mechanical behaviour of basalt fibre reinforced concrete: An experimental study. Mater. Today Proc. 2021, 43, 2317–2322. [Google Scholar] [CrossRef]
- Chen, X.-F.; Kou, S.-C.; Xing, F. Mechanical and durable properties of chopped basalt fiber reinforced recycled aggregate concrete and the mathematical modeling. Constr. Build. Mater. 2021, 298, 123901. [Google Scholar] [CrossRef]
- EN 14651:2005+A1:2007; Test Method for Metallic Fibre Concrete-Measuring the Flexural Tensile Strength (Limit of Proportionality (LOP), Residual). Available online: https://www.en-standard.eu/bs-en-14651-2005-a1-2007-test-method-for-metallic-fibre-concrete-measuring-the-flexural-tensile-strength-limit-of-proportionality-lop-residual/ (accessed on 5 May 2023).
- Turbobuild Integral. Available online: https://www.deutsche-basalt-faser.de/en/products/turbobuild-integral/ (accessed on 5 May 2023).
- EN 12390-5:2009; Testing Hardened Concrete. Flexural Strength of Test Specimens. Available online: https://www.en-standard.eu/bs-en-12390-5-2019-testing-hardened-concrete-flexural-strength-of-test-specimens/ (accessed on 5 May 2023).
- Lindhagen, J.; Jekabsons, N.; Berglund, L. Application of bridging-law concepts to short-fibre composites 4. FEM analysis of notched tensile specimens. Compos. Sci. Technol. 2000, 60, 2895–2901. [Google Scholar] [CrossRef]
- Li, H.; Yang, Z.-J.; Li, B.-B.; Wu, J.-Y. A phase-field regularized cohesive zone model for quasi-brittle materials with spatially varying fracture properties. Eng. Fract. Mech. 2021, 256, 107977. [Google Scholar] [CrossRef]
- Mujika, F.; Arrese, A.; Adarraga, I.; Oses, U. New correction terms concerning three-point and four-point bending tests. Polym. Test. 2016, 55, 25–37. [Google Scholar] [CrossRef]
- Herrmann, H.J.; Roux, S. Statistical Models for the Fracture of Disordered Media; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Zhang, C.; Shi, F.; Cao, P.; Liu, K. The fracture toughness analysis on the basalt fiber reinforced asphalt concrete with prenotched three-point bending beam test. Case Stud. Constr. Mater. 2022, 16, e01079. [Google Scholar] [CrossRef]
- Petersson, P.E. Crack Growth and Development of Fracture Zone in Plain Concrete and Similar Materials. TVBM-1006 ed. Ph.D. Thesis, Division of Building Materials, Lund Institute of Technology, Lund, Sweden, 1981. [Google Scholar]
- Reinhardt, H.W.; Cornelissen, H.A.W.; Hordijk, D.A. Tensile tests and failure analysis of concrete. J. Struct. Eng. ASCE 1986, 112, 2462–2477. [Google Scholar] [CrossRef]
- Cui, X.; Liu, G.; Li, Z. A high-order edge-based smoothed finite element (ES-FEM) method with four-node triangular element for solid mechanics problems. Eng. Anal. Bound. Elements 2023, 151, 490–502. [Google Scholar] [CrossRef]
- Auzins, J. High order orthogonal designs of experiments for metamodeling, identification and optimization of mechanical systems. In Proceedings of the 11th World Congress on Computational Mechanics, 5th European Conference on Computational Mechanics, and 6th European Conference on Computational Fluid Dynamics, Barcelona, Spain, 20–25 July 2014; pp. 3190–3201. [Google Scholar]
- Jekabsons, J. Mechanics of Composites with Fiber Bundle Meso-Structure. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2002. [Google Scholar]
- Shewchuk, J.R. Triangle: Engineering a 2D Quality Mesh Generator and Delaunay Triangulator; Carnegie Mellon University: Pittsburgh, PA, USA, 2005. [Google Scholar]



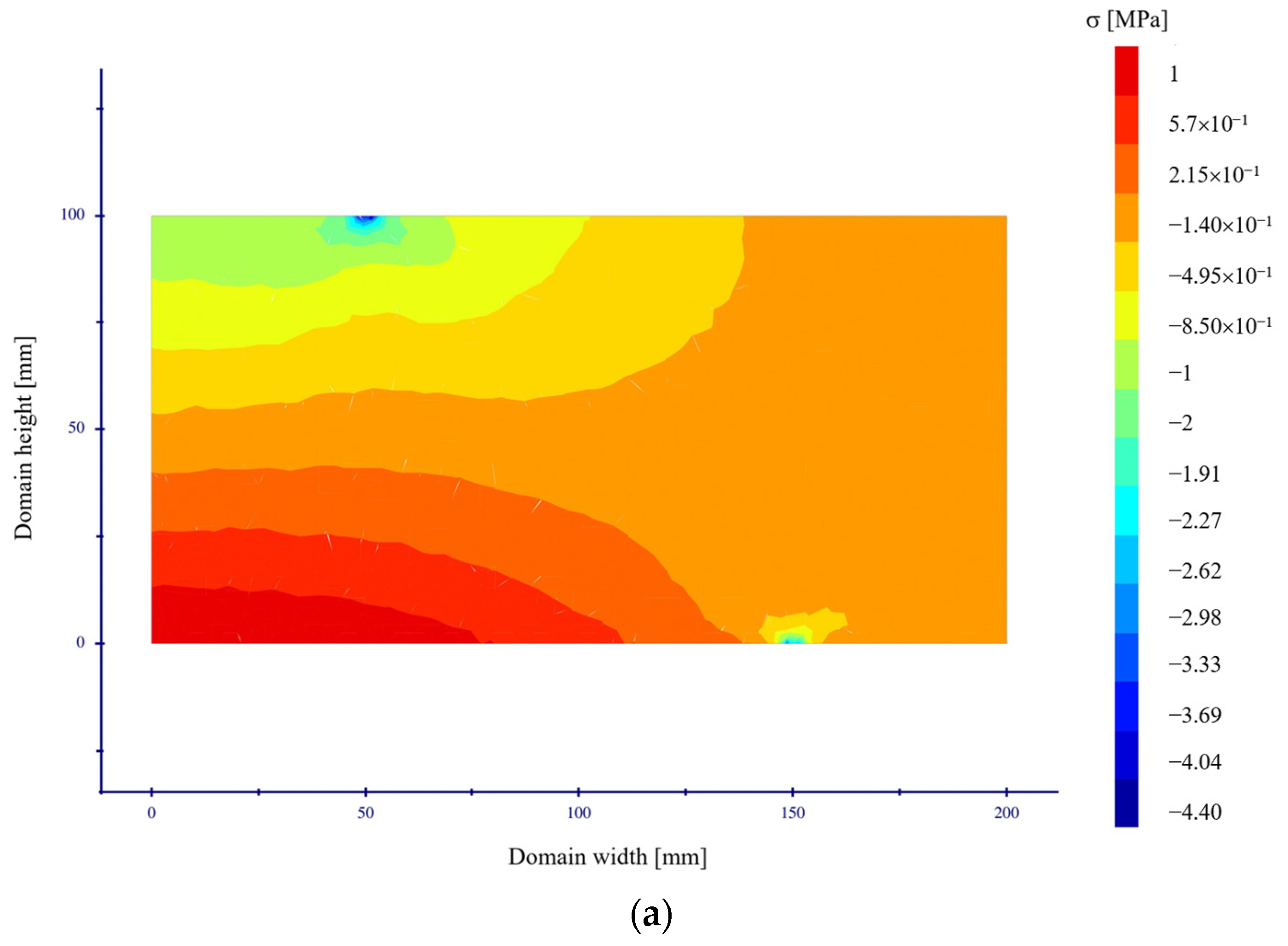
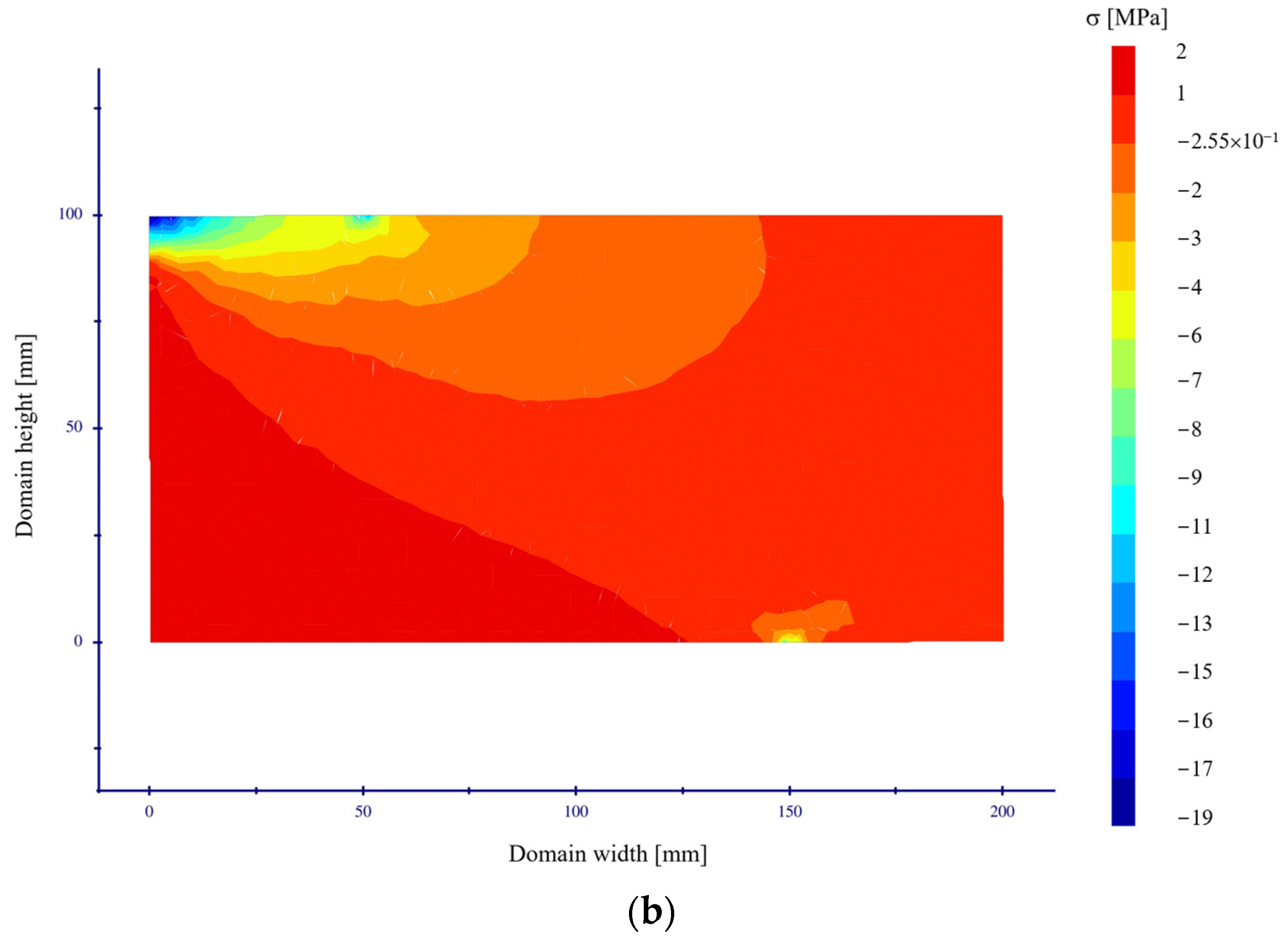

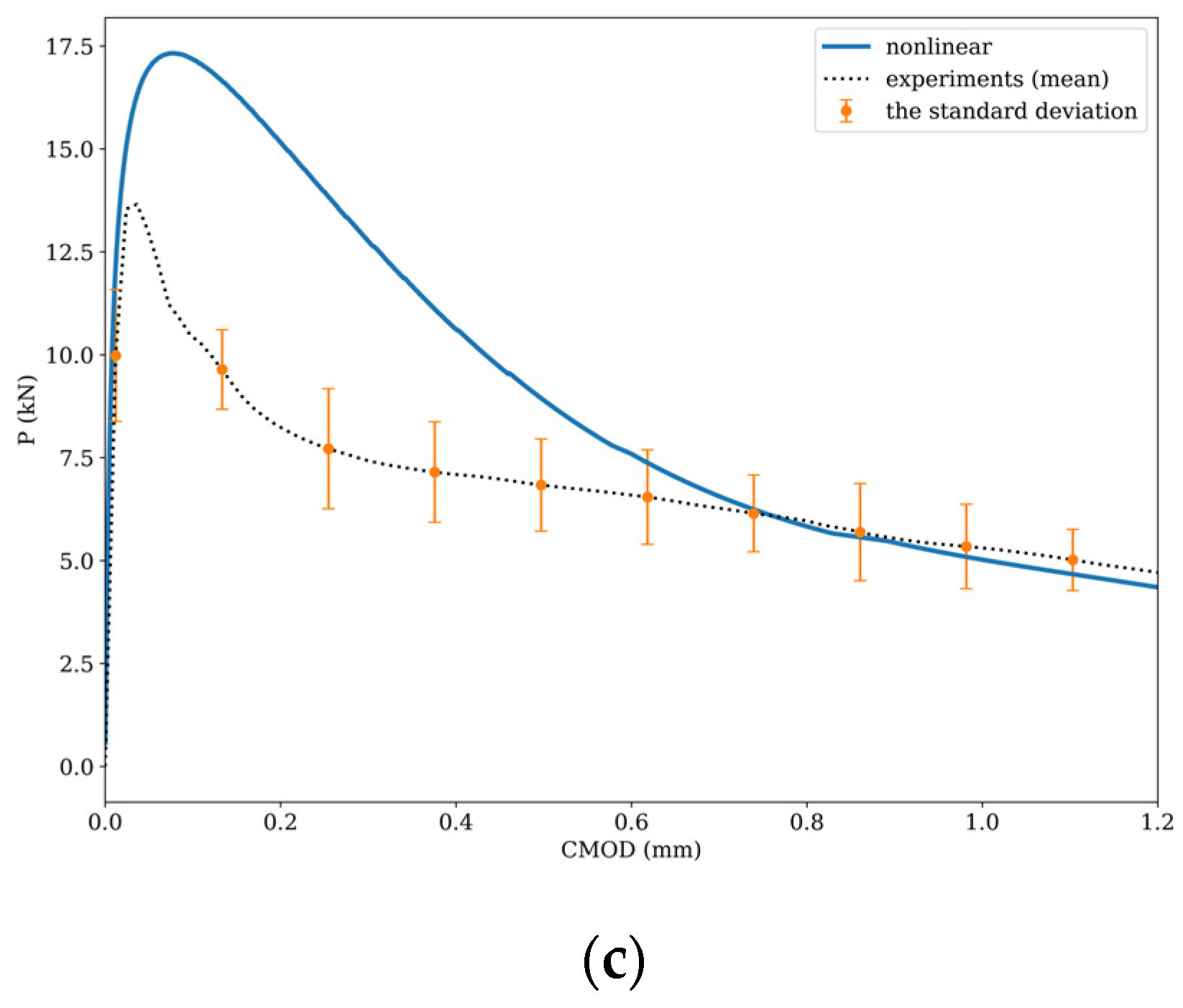
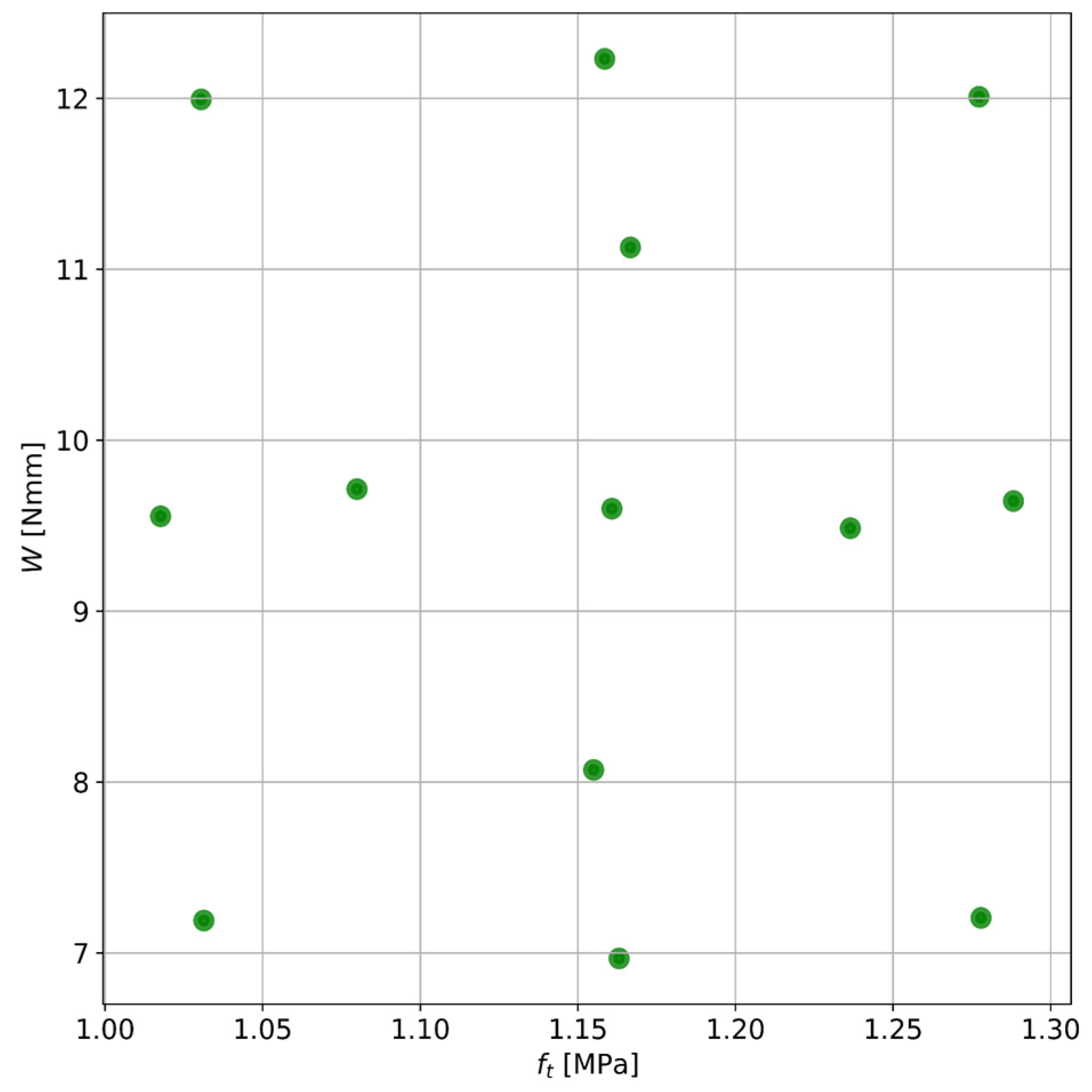

| Mix | BF (kg) | CEMII/B-S 52.5 N | Pigment | Aggregates 0–8 mm | Aggregates 8–22.4 mm | Dynamon SX-23 (kg) | Dynamon SX-23 (% of Binder) |
|---|---|---|---|---|---|---|---|
| A0 | 0 | 350 | 7 | 1279 | 656 | 3.68 | 1.03 |
| A1 | 6.7 | 350 | 7 | 1271 | 652 | 4.36 | 1.22 |
| A2 | 10.8 | 350 | 7 | 1270 | 652 | 4.36 | 1.22 |
| Mix | Fresh Density (kg · m−3) | Air Content (%) | Slump (mm) | Hardened Density (kg · m−3) | Compressive Strength (MPa) | E-Modulus (GPa) |
|---|---|---|---|---|---|---|
| A0 | 2466 | 2.0 | 210 | 2460 | 64.4 | 28.7 |
| A1 | 2429 | 2.4 | 200 | 2440 | 64.1 | 29.5 |
| A2 | 2460 | 2.0 | 210 | 2420 | 60.3 | 29.8 |
| Case | The Number of Elements in the FE Model | The Number of Nodes | Pmax (kN) |
|---|---|---|---|
| M1 | 8600 | 3891 | 9.79 |
| M2 | 2456 | 1025 | 9.83 |
| M3 | 749 | 274 | 9.98 |
| OSA (%) | FE Modeling | Experiments | ||
|---|---|---|---|---|
| Pmax (kN) | δPmax (mm) | Pmax (kN) (stdev) | δPmax (mm) (stdev) | |
| 10 | 10.72 | 0.094 | 9.93 (0.16) | 0.05 (0.004) |
| 15 | 10.23 | 0.104 | 10.45 (0.33) | 0.05 (0.006) |
| 30 | 9.75 | 0.10 | 9.97 (0.42) | 0.05 (0.007) |
| OSA (%) | FE Modeling | Experiments | ||
|---|---|---|---|---|
| Pmax (kN) | δPmax (mm) | Pmax (kN) (stdev) | δPmax (mm) (stdev) | |
| 10 | 7.82 | 0.104 | 7.44 (0.64) | 0.14 (0.04) |
| 20 | 7.73 | 0.112 | 7.90 (0.67) | 0.16 (0.05) |
| 30 | 9.91 | 0.104 | 9.64 (0.59) | 0.15 (0.05) |
| Polynomial | Cross-Validation Error | R2 |
|---|---|---|
| 1st order | 10.65% | 0.993 |
| 2nd order | 1.17% | 0.999 |
| 3rd order | 0.47% | 0.999 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Upnere, S.; Novakova, I.; Jekabsons, N.; Krasnikovs, A.; Macanovskis, A. Bridging Law Application to Fracture of Fiber Concrete Containing Oil Shale Ash. Buildings 2023, 13, 1868. https://doi.org/10.3390/buildings13071868
Upnere S, Novakova I, Jekabsons N, Krasnikovs A, Macanovskis A. Bridging Law Application to Fracture of Fiber Concrete Containing Oil Shale Ash. Buildings. 2023; 13(7):1868. https://doi.org/10.3390/buildings13071868
Chicago/Turabian StyleUpnere, Sabine, Iveta Novakova, Normunds Jekabsons, Andrejs Krasnikovs, and Arturs Macanovskis. 2023. "Bridging Law Application to Fracture of Fiber Concrete Containing Oil Shale Ash" Buildings 13, no. 7: 1868. https://doi.org/10.3390/buildings13071868
APA StyleUpnere, S., Novakova, I., Jekabsons, N., Krasnikovs, A., & Macanovskis, A. (2023). Bridging Law Application to Fracture of Fiber Concrete Containing Oil Shale Ash. Buildings, 13(7), 1868. https://doi.org/10.3390/buildings13071868






