Vibration of Timber and Hybrid Floors: A Review of Methods of Measurement, Analysis, and Design
Abstract
:1. Introduction
1.1. Industry Challenge
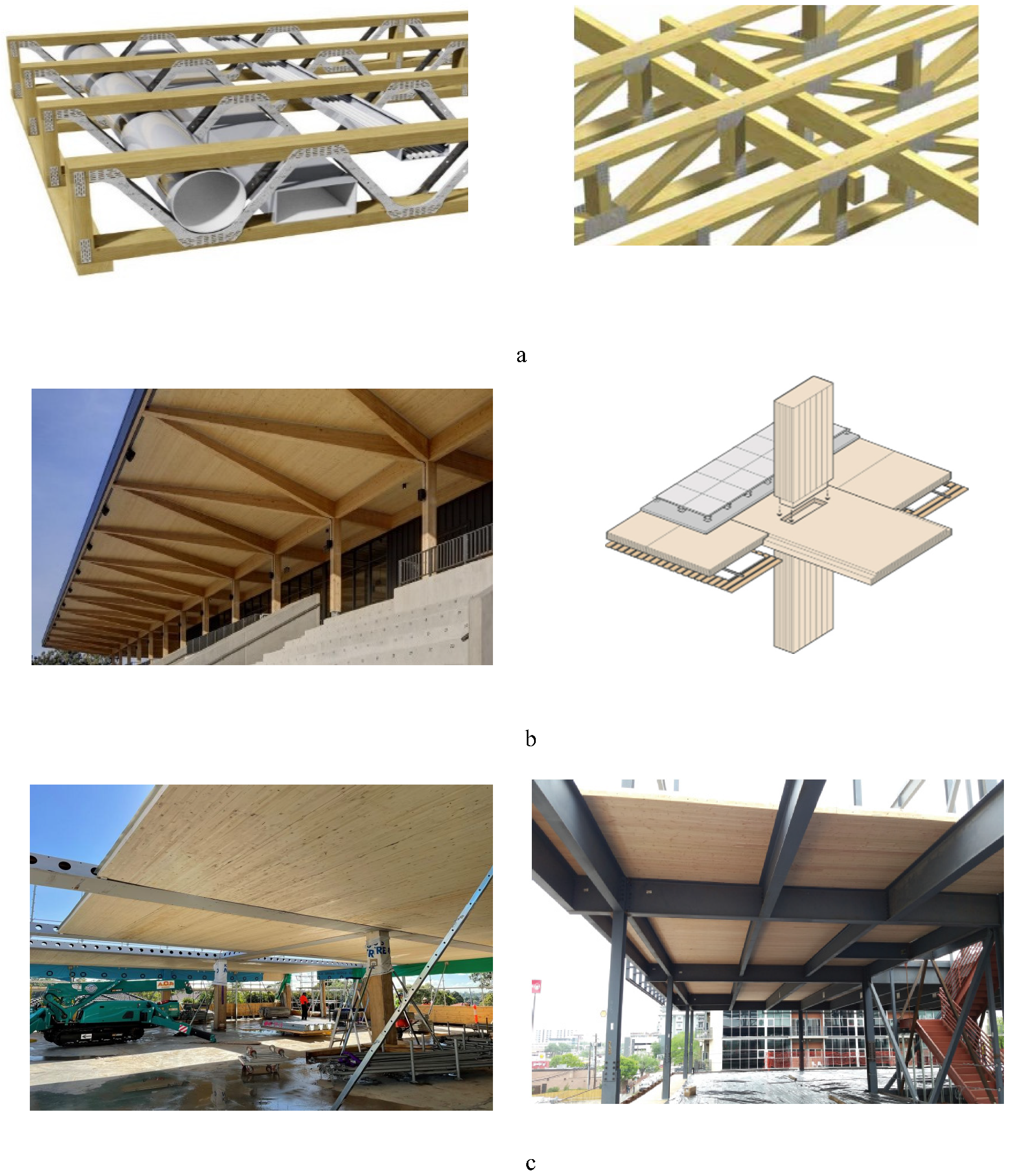
1.2. Long Span Timber Floor Systems
2. Systems View of Vibration: Evaluation, Perceptibility, and Comfort
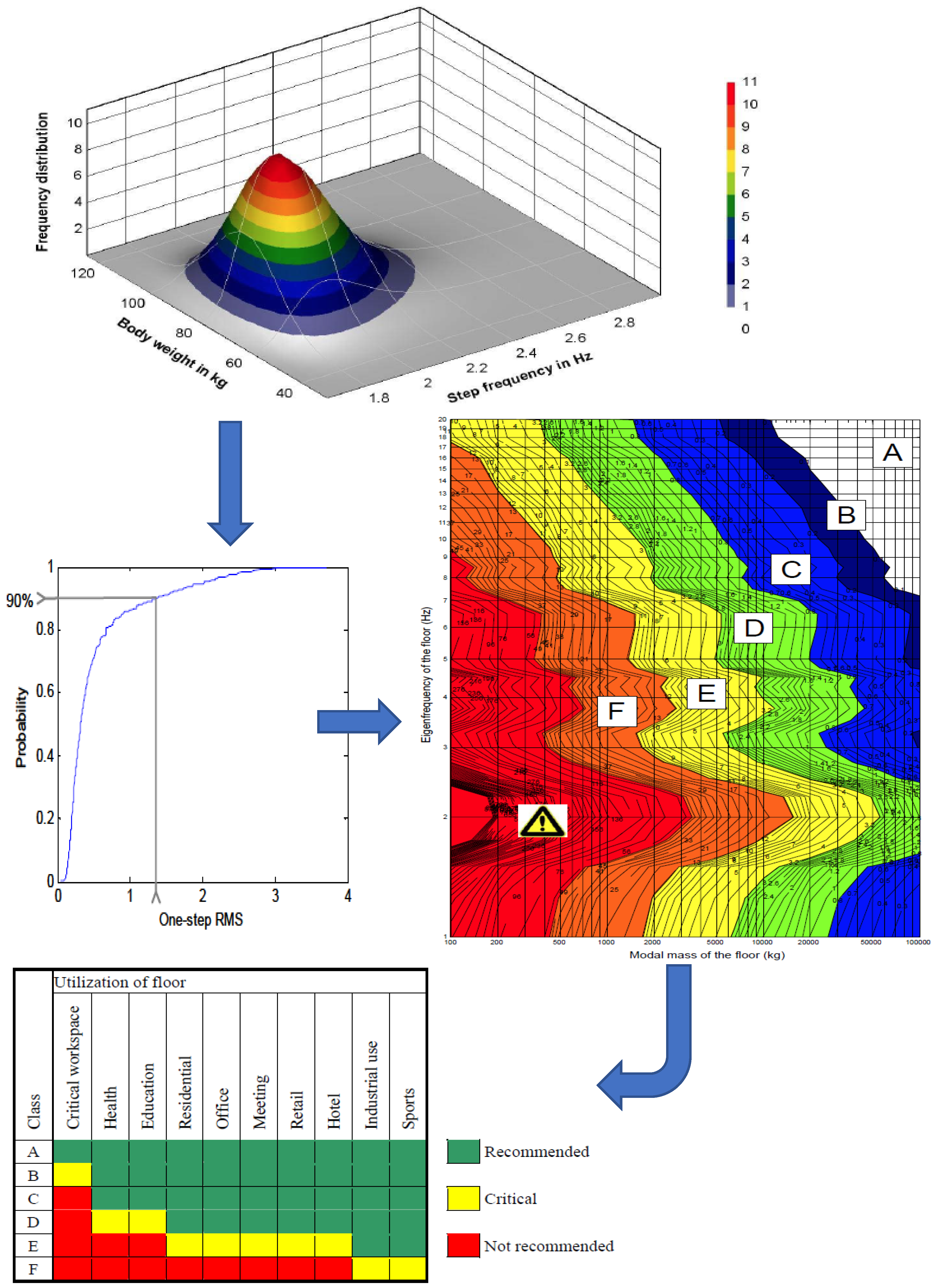
2.1. Vibration Evaluation According to ISO 2631-1 (AS 2670.1)
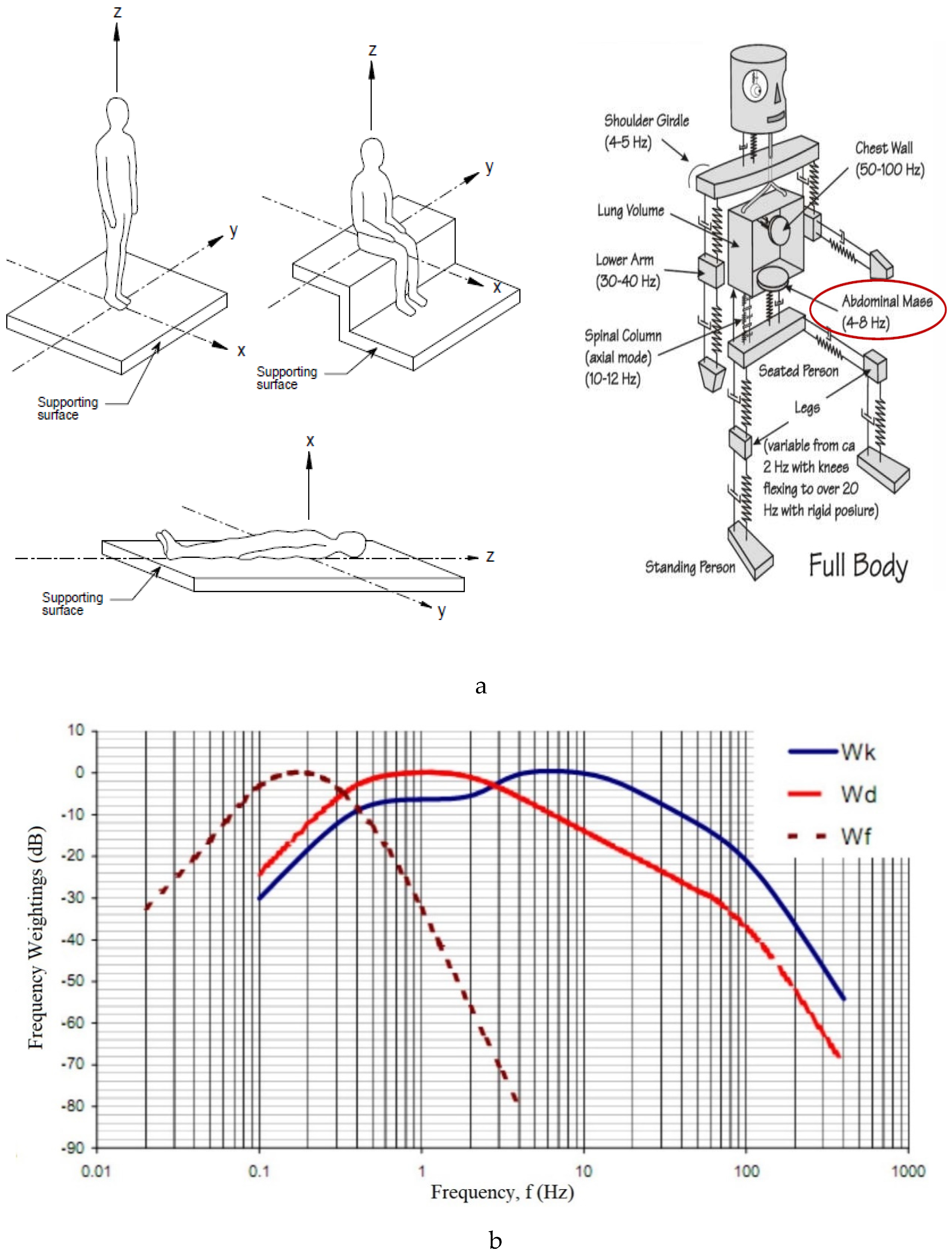
| Criterion | Position | Wd | Kx | Wd | Ky | Wk | Kz |
|---|---|---|---|---|---|---|---|
| Comfort | Seated (translational vibration) | ✓ | 1.0 | ✓ | 1.0 | ✓ | 1.0 |
| Seated (rotary vibration) | ✓ | 0.4 | ✓ | 0.5 | ✓ | 0.4 | |
| Standing | ✓ | 1.0 | ✓ | 1.0 | ✓ | 1.0 | |
| Recumbent | ✓ | 1.0 | ✓ | 1.0 | ✓ | 1.0 | |
| Perception | All | ✓ | 1.0 | ✓ | 1.0 | ✓ | 1.0 |
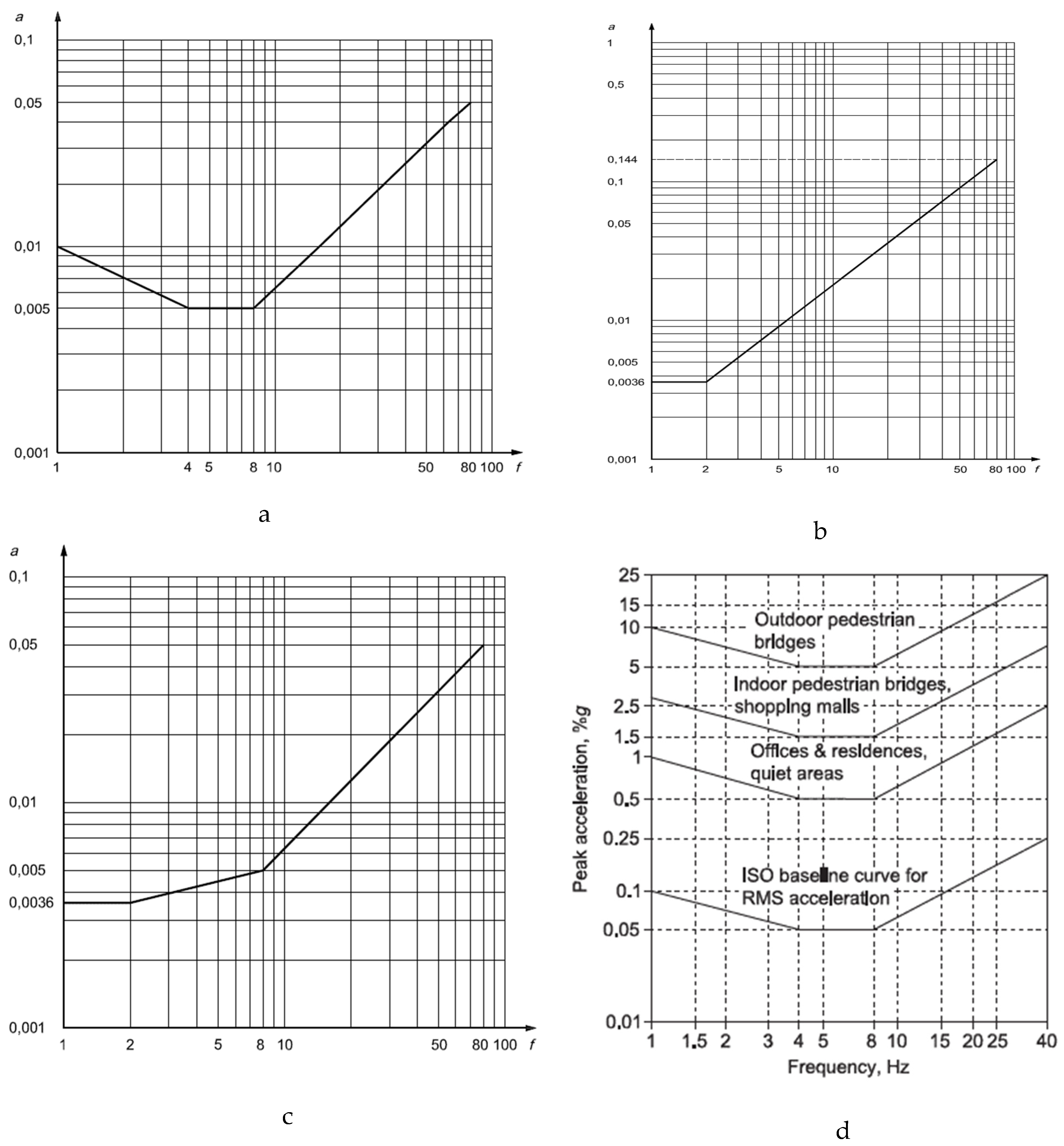
2.2. Vibration Criteria in ISO 10137
| Multiplying Factors to Base Curves in Figure 4 | |||
|---|---|---|---|
| Building Usage | Time | Continuous and Intermittent Vibration | Impulsive Vibration |
| Residential | Day | 2–4 | 30–90 |
| Night | 1.4 | 1.4–20 | |
| Office and School | Day | 4 * | 60–128 |
| Night | 4 * | 60–128 | |
| Vibration Dose Values (m/s1.75) in Equation (4) from BS 6472 [36] | |||
| Building usage | Adverse comment unlikely | Adverse comment possible | Adverse comment probable |
| Residential 16 h day | 0.2–0.4 | 0.4–0.8 | 0.8–1.6 |
| Residential 8 h night | 0.13 | 0.26 | 0.51 |
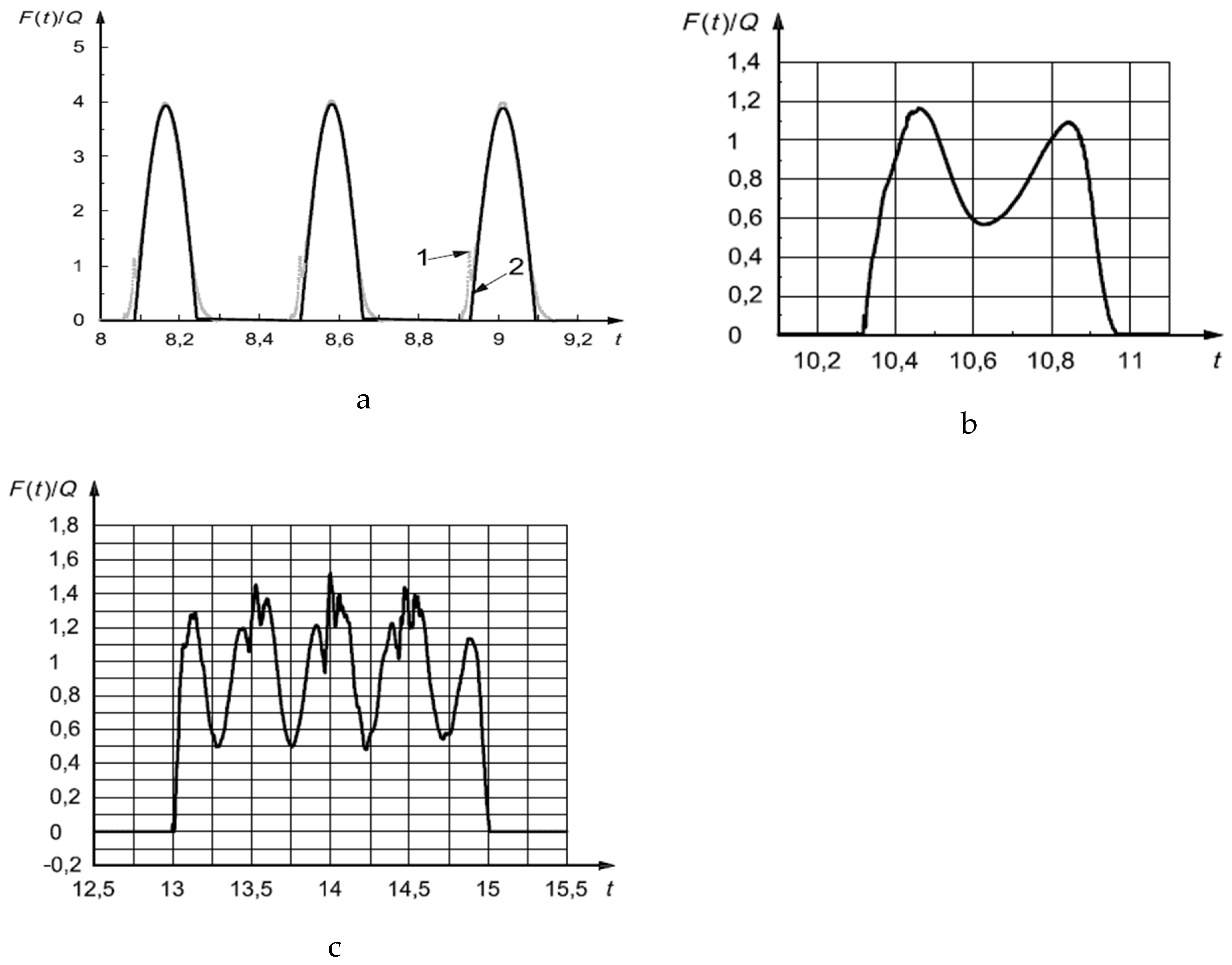
3. Dynamic Actions Applied to the Floor
| ISO 10137 [31] | CCIP-016 [22] | SCI-P354 [21] | AISC DG 11 [23] | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Harmonic Number n | Forcing Frequency fw (Hz) | Fourier Coefficient αn | Harmonic Number n | Forcing Frequency fw (Hz) | Fourier Coefficient αn | Harmonic Number n | Forcing Frequency fw (Hz) | Fourier Coefficient αn | Harmonic Number n | Forcing Frequency fw (Hz) | Fourier Coefficient αn |
| 1 | 1.2 to 2.4 | 0.37f − 0.37 | 1 | 1–2.8 | 0.41f − 0.3895 < 0.56 | 1 | 1.8–2.2 | 0.436f − 0.4142 | 1 | 1.6–2.2 | 0.5 |
| 2 | 2.4 to 4.8 | 0.1 | 2 | 2–5.6 | 0.069 + 0.0056f | 2 | 3.6–4.4 | 0.0738 + 0.012f | 2 | 3.2–4.4 | 0.2 |
| 3 | 3.6 to 7.2 | 0.06 | 3 | 3–8.4 | 0.033 + 0.0064f | 3 | 5.4–6.6 | 0.0364 + 0.021f | 3 | 4.8–6.6 | 0.1 |
| 4 | 4.8 to 9.6 | 0.06 | 4 | 4–11.2 | 0.013 + 0.0065f | 4 | 7.2–8.8 | 0.014 + 0.028f | 4 | 6.4–8.8 | 0.05 |
| 5 | 6.0 to 12.0 | 0.06 | >4 | >11.2 | 0 | >4 | >8.8 | 0 | |||
4. Floor Dynamics
| Standard/Guideline | Low-Frequency Floor | High-Frequency Floor |
|---|---|---|
| ISO 10137 [31] | 8 Hz < fn < 10 Hz or smaller | 10 Hz < fn |
| SCI-P354 [21] | fn < 10 Hz | 10 Hz < fn |
| CCIP-016 [22] | fn < 10.5 Hz | 10.5 Hz < fn |
| AISC DG11 [23] | fn < 9 Hz | 9 Hz < fn |
| Toratti and Talja [40] | fn < 10 Hz | 10 Hz < fn |
| BS 6472-1:2008 [34] | 7 Hz < fn < 10 Hz or smaller | 10 Hz < fn |
| Allen and Murray [43] | fn < 9 Hz | 9 Hz < fn |
| Wyatt and Dier [44] | fn < 7 Hz | 7 Hz < fn |
| Ohlsson [45] | fn < 8 Hz | 8 Hz < fn |
4.1. Frequency, Mode Shape, and Modal Mass in One-Way and Two-Way Spanning Floor Systems
4.2. Modal Damping
| Floor Type | EN 5 [14] | UK NA to EN 5 [61] | ISO 10137 [31] | HIVOSS [62] | Hamm et al. [63] |
|---|---|---|---|---|---|
| Timber floor | 1% | 2% | 1% | 1% | |
| Wood joist floor | 1% to 5.5% | ||||
| Plain glulam floor with sound insulation | 2% | 2% | |||
| Girder floors and nail laminated floors * | 3% | 3% |
5. Vibration Design Methods
5.1. Rules of Thumb
5.2. Empirical and Simplified Analytical Methods
5.2.1. Empirical Method in ISO/TR21136
5.2.2. Hamm et al.
5.2.3. Combined Frequency, Deflection, and Impulse Velocity Method in EN 5:2004
5.2.4. Vibration-Controlled Span Method in CSA 086:19
5.2.5. One Step Root Mean Square Method in HIVOSS:2007
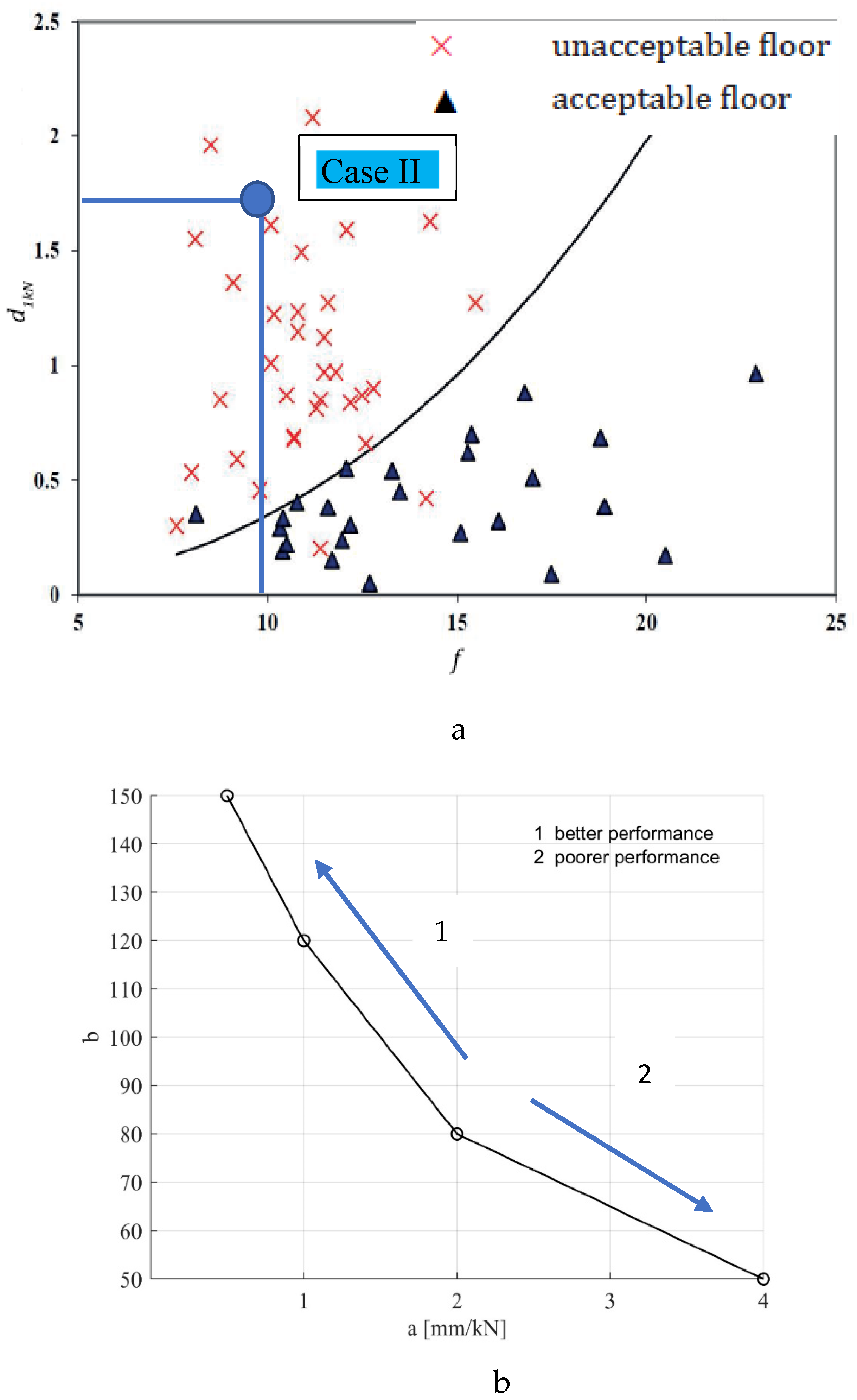
5.2.6. Floor Performance vs. Floor Usage in prEN 1995-1-1: 2025 (Final Draft)
| Floor Performance Levels | ||||||
|---|---|---|---|---|---|---|
| Criteria | Level I | Level II | Level III | Level IV | Level V | Level VI |
| d1kN (mm) ≤ | 0.25 | 0.25 | 0.5 | 0.8 | 1.2 | 1.6 |
| Response factor (R) | 4 | 8 | 12 | 20 | 30 | 40 |
| Floor Usage | Quality Choice | Base Choice | Economy Choice | |||
| Multi-family residential | Levels I, II, III | Level IV | Level V | |||
| Single-family residential | Levels I, II, III, IV | Level V | Level VI | |||
| Office | Levels I, II, III | Level IV | Level V | |||
5.3. Modal Superposition Methods
| CCIP-016 [22] | ||||
|---|---|---|---|---|
| Low-Frequency Floor | High-Frequency Floor | |||
| Floor Use | Peak Acceleration | Response Factor, R | VRMS (m/s) | Response Factor, R |
| Commercial (offices, retail, restaurants, and airports) | 0.57% g | 8 | 8 × 10−4 | 8 |
| Residential (day) | 0.28–0.57% g | 4 to 8 | 4–8 × 10−4 | 4 to 8 |
| Premium quality office, open office with busy corridors near midspan, heavily trafficked public areas with seating | 0.28% g | 4 | 4 × 10−4 | 4 |
| Residential (night) | 0.2% g | 2.8 | - | - |
| Hospitals and critical work areas | 0.071% g | 1 | - | - |
| AISC DG 11 [23] | ||||
| Low-Frequency Floor | High-Frequency Floor | |||
| Floor Use | Peak Acceleration | Response Factor, R | VRMS (m/s) | Response Factor, R |
| Outdoor pedestrian bridges | 5% g | 70 | - | - |
| Indoor pedestrian bridges, shopping malls | 1.5% g | 21 | - | - |
| Offices, residences, quiet areas | 0.5% g | 7 | - | - |
| Ordinary workshops | - | - | 8 × 10−4 | 8 |
| Offices | - | - | 4 × 10−4 | 4 |
| Residences | - | - | 2 × 10−4 | 2 |
| Hospital patient rooms | - | - | 1.5 × 10−4 | 1.5 |
5.3.1. CCIP-016
- Find the frequency, fn, modal mass, m*n, and modal damping, ζm, of each mode. The mode shape values at the excitation, μen, and response, μrn, locations in each mode on the floor are also needed. While this is normally conducted through FEA, it can also be calculated using simple equations such as those provided in Equations (17) and (18).
- Calculate the harmonic forcing frequency, fh = h fw, for harmonic numbers, h, from 1 to 4, and the harmonic force, Fh, from coefficients in Table 3 and using Equation (8) at each harmonic.
- Find the real and imaginary parts of the acceleration from Equation (36).
- Sum the real and imaginary responses in all modes and find the magnitude of the acceleration at the harmonic, ah, by summing the square root of the real and imaginary accelerations at this harmonic.
- Convert ah to a response factor, Rh, for this harmonic by dividing ah by the base acceleration, aR = 1,h (m/s2), given in Equation (37) for the corresponding fh.
- Find the total response factor, R, which is the “square root sum of the squares” combination of the response factor for each of the four harmonics.
- The impulsive footfall force, FI, in Ns is calculated from Equation (9) [22] for all modes with frequencies up to twice the fundamental frequency of the floor.
- Find the peak velocity of each mode, vn, and the time history of the velocity response, vn(t), over the period of one footfall, Tw, from Equation (38).
- Add the velocity response in each mode, vn(t), in the time domain to find the total response, v(t) (m/s).
- Calculate the RMS velocity (VRMS) and divide it by the baseline RMS velocity, VR = 1 (m/s), at the fundamental frequency, f1, to determine the response factor, R, and compare it against the target values in Table 7 for the desired floor use (see Equation (38)).
5.3.2. SCI-P354

5.3.3. American Institute of Steel Construction AISC/CISC DG 11
5.3.4. Harmonized Peak Acceleration and VDV Approach
5.4. Time History Analysis Method
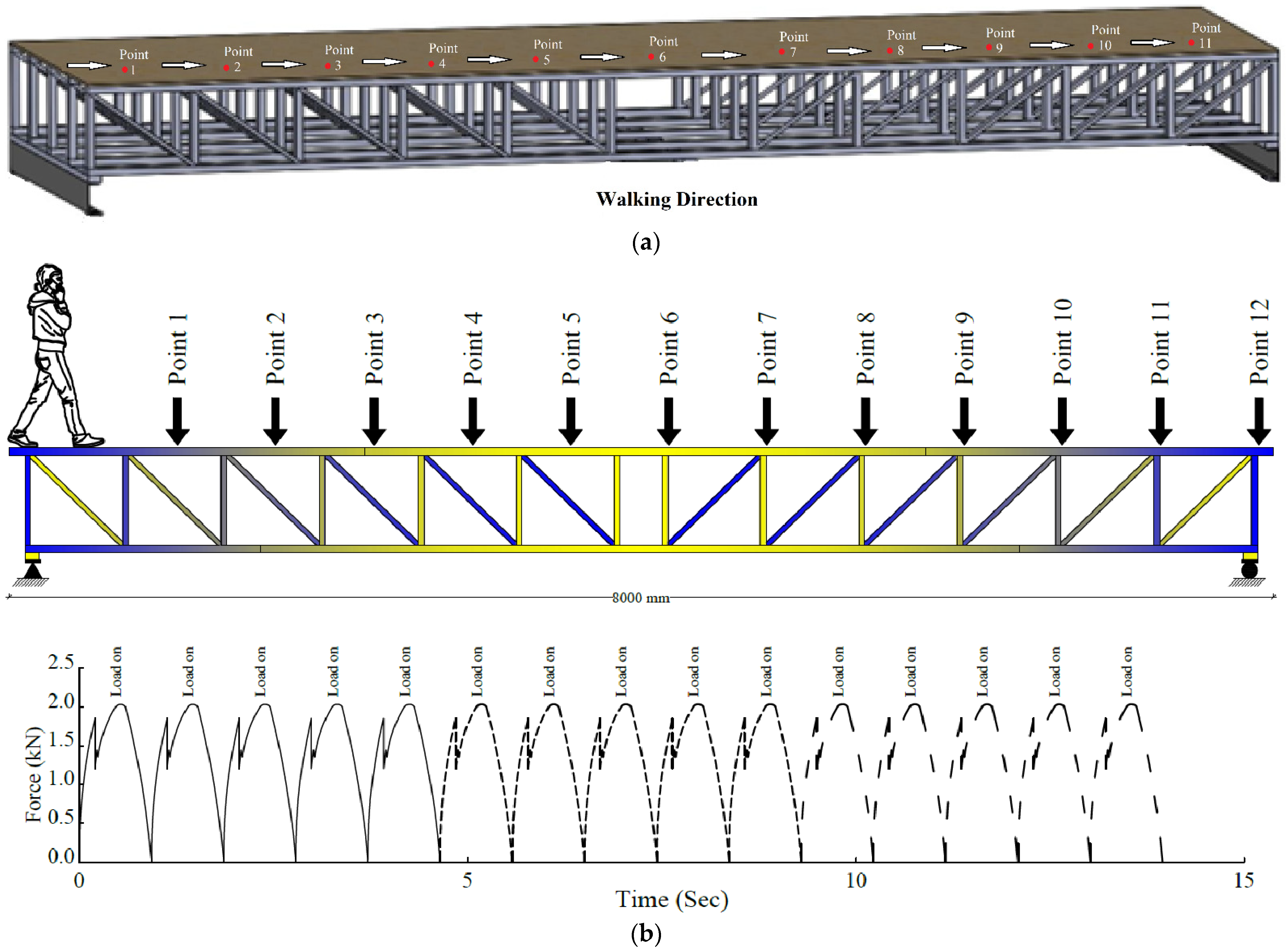
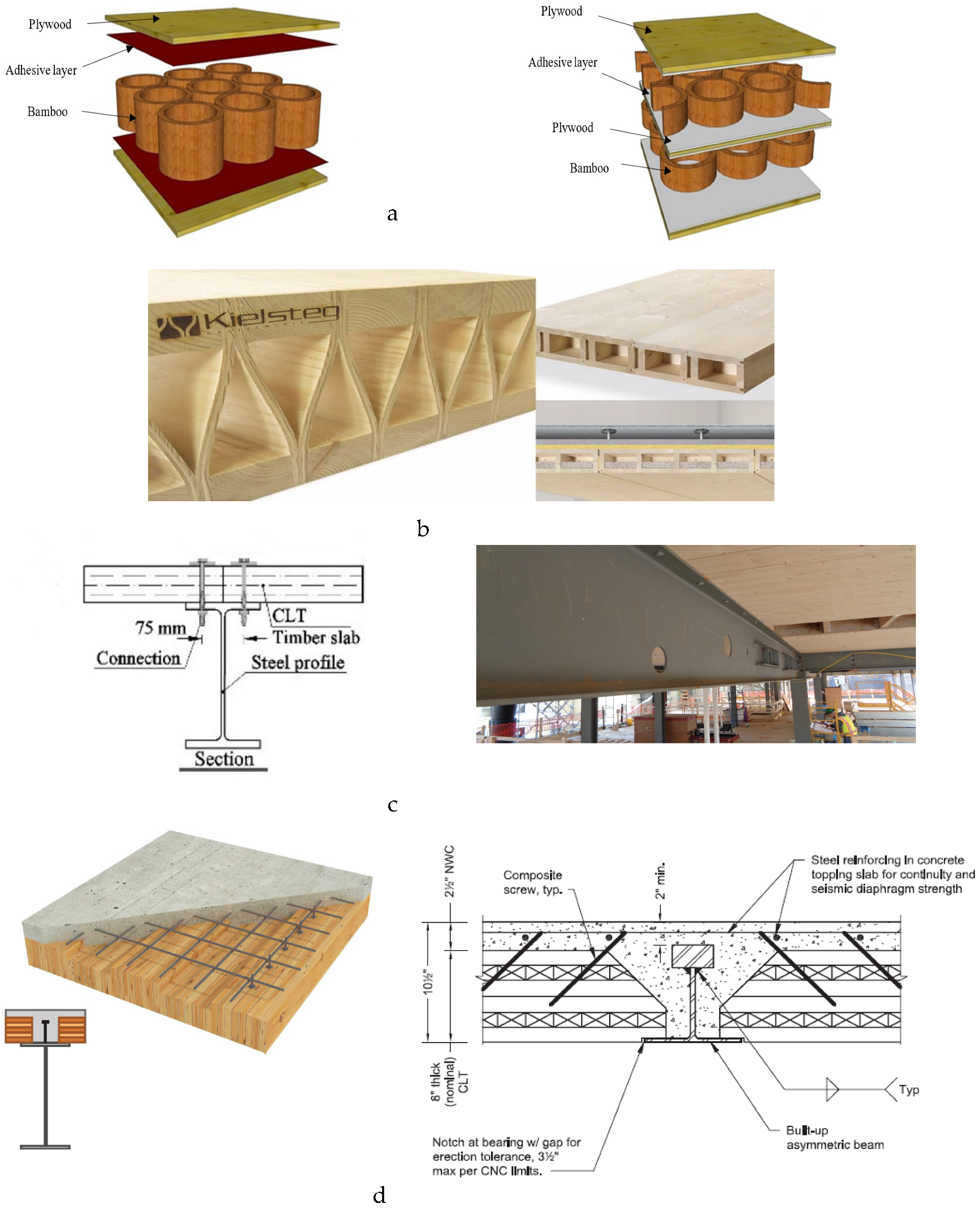
6. Hybrid Floor Systems
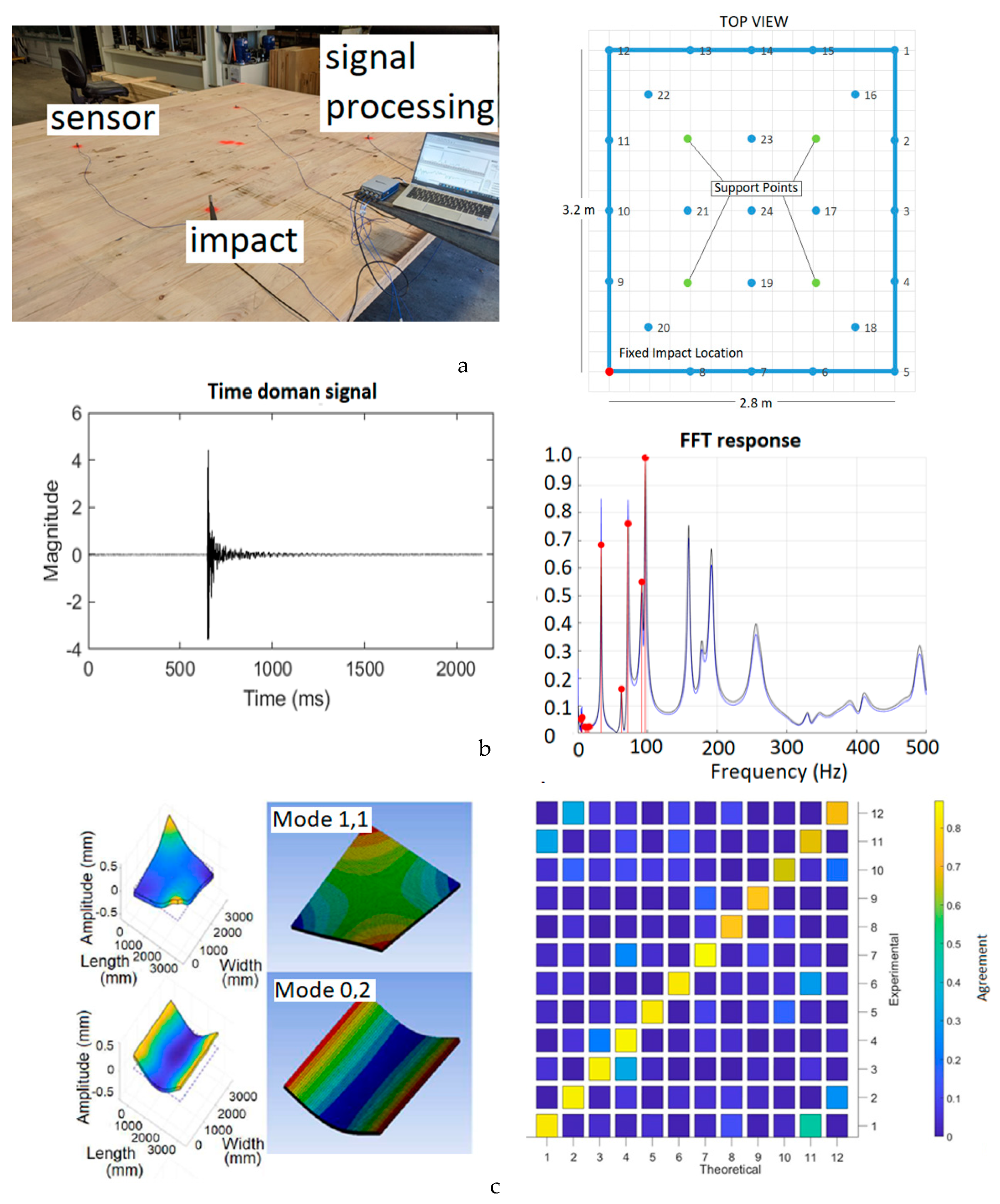
7. Case Study I: EMA and NMA of a CLT Floor
| Rank | Mode Shape (i,j) | EMA Frequency f EMA (Hz) | NMA Frequency f NMA (Hz) | Difference |
|---|---|---|---|---|
| 1 | 1,1 | 11.7 | 10.0 | 15% |
| 2 | 0,2 | 16.5 | 15.4 | 7% |
| 3 | 1,2 | 27.9 | 25.1 | 10% |
| 4 | 2,0 | 34.2 | 28.8 | 16% |
| 5 | 2,1 | 40.6 | 34.7 | 15% |
| 6 | 0,3 | 44.7 | 42.3 | 5% |
| 7 | 2,2 | 55.3 | 50.7 | 8% |
| 8 | 1,3 | 58.4 | 51.0 | 13% |
| 9 | 3,0 | 85.6 | 75.1 | 12% |
| 10 | 2,3 | 88.3 | 76.2 | 14% |
| 11 | 3,1 | 94.0 | 79.9 | 15% |
| 12 | 0,4 | 112.8 | 82.2 | 27% |
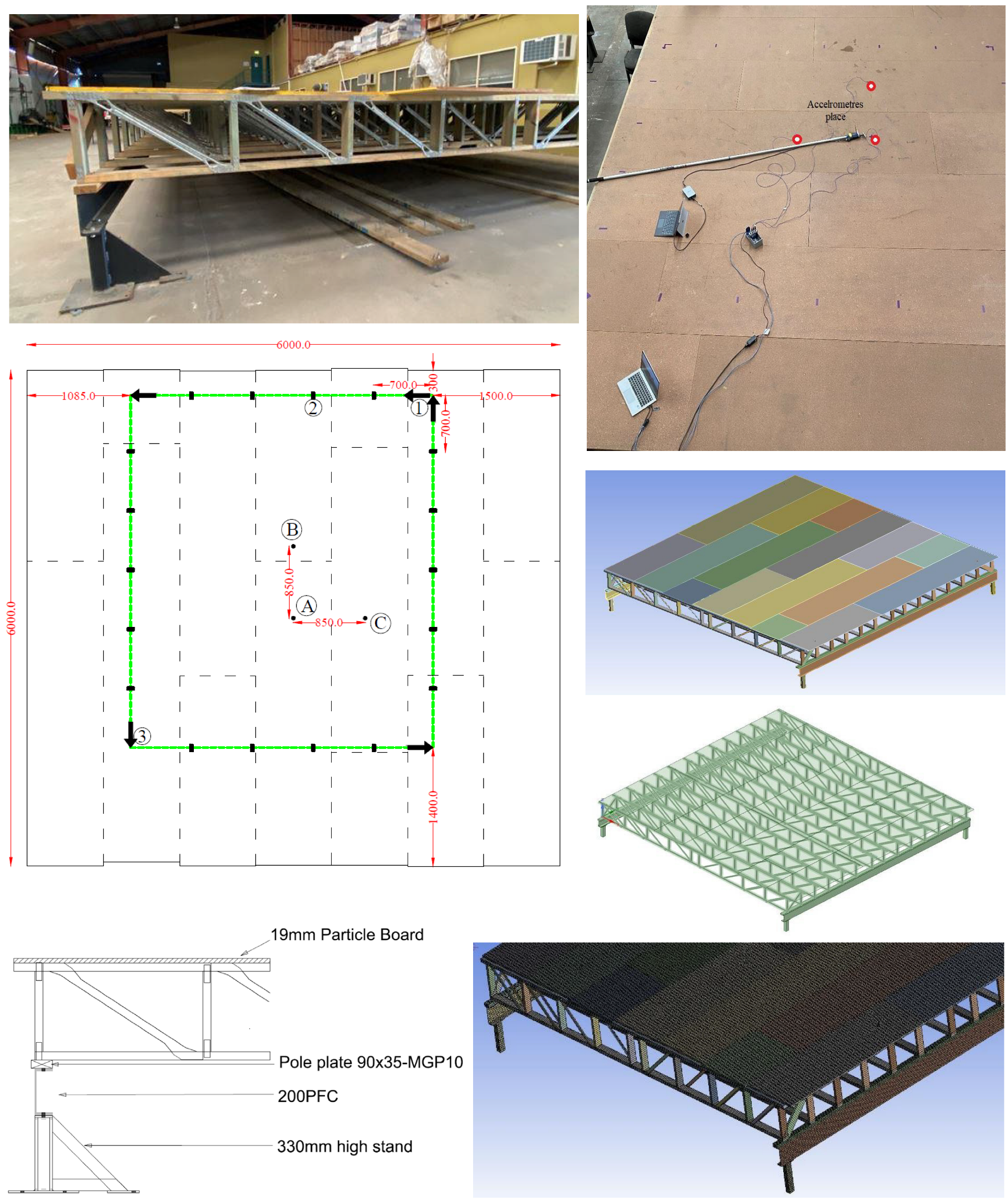
8. Case Study II: EMA and NMA of a 6 m × 6 m Cassette Floor
8.1. The Floor System and FEA Model
| Property | MGP 12 | MGP 10 | Particle Board |
|---|---|---|---|
| Density (kg/m3) | 594 | 550 | 748 |
| EL (MPa) | 12,700 | 10,000 | 3000 |
| ER (MPa) | 1435 | 1130 | 3000 |
| ET (MPa) | 991 | 780 | 3000 |
| GLR (MPa) | 1029 | 810 | 1360 |
| GLT (MPa) | 165 | 130 | 1360 |
| GRT (MPa) | 1041 | 820 | 1360 |
| μLR | 0.292 | 0.292 | 0.103 |
| μLT | 0.382 | 0.382 | 0.103 |
| μRT | 0.328 | 0.328 | 0.103 |
8.2. Dynamic Properties
| Accelerometer A | Accelerometer B | Accelerometer C | Damping | |||||
|---|---|---|---|---|---|---|---|---|
| favg (Hz) | CoV | favg (Hz) | CoV | favg (Hz) | CoV | ζ | CoV | |
| 1 | 9.08 | 0.59% | 9.08 | 0.01% | 9.08 | 0.01% | 0.90% | 0.14% |
| 2 | 17.05 | 3.77% | 16.68 | 2.81% | 16.77 | 0.50% | 1.08% | 0.66% |
| 3 | 17.84 | 0.01% | 17.45 | 2.65% | 17.98 | 0.31% | 1.03% | 0.17% |
| 4 | 19.41 | 0.01% | 19.49 | 0.19% | 19.40 | 0.34% | 0.84% | 0.55% |
| 5 | 20.98 | 0.01% | 21.03 | 0.20% | 21.04 | 0.24% | 0.91% | 0.14% |
| 6 | 22.01 | 0.26% | 22.31 | 0.33% | 22.23 | 0.37% | 0.89% | 0.14% |
| 7 | 24.86 | 0.01% | 23.69 | 2.41% | 23.55 | 0.40% | 0.95% | 0.21% |
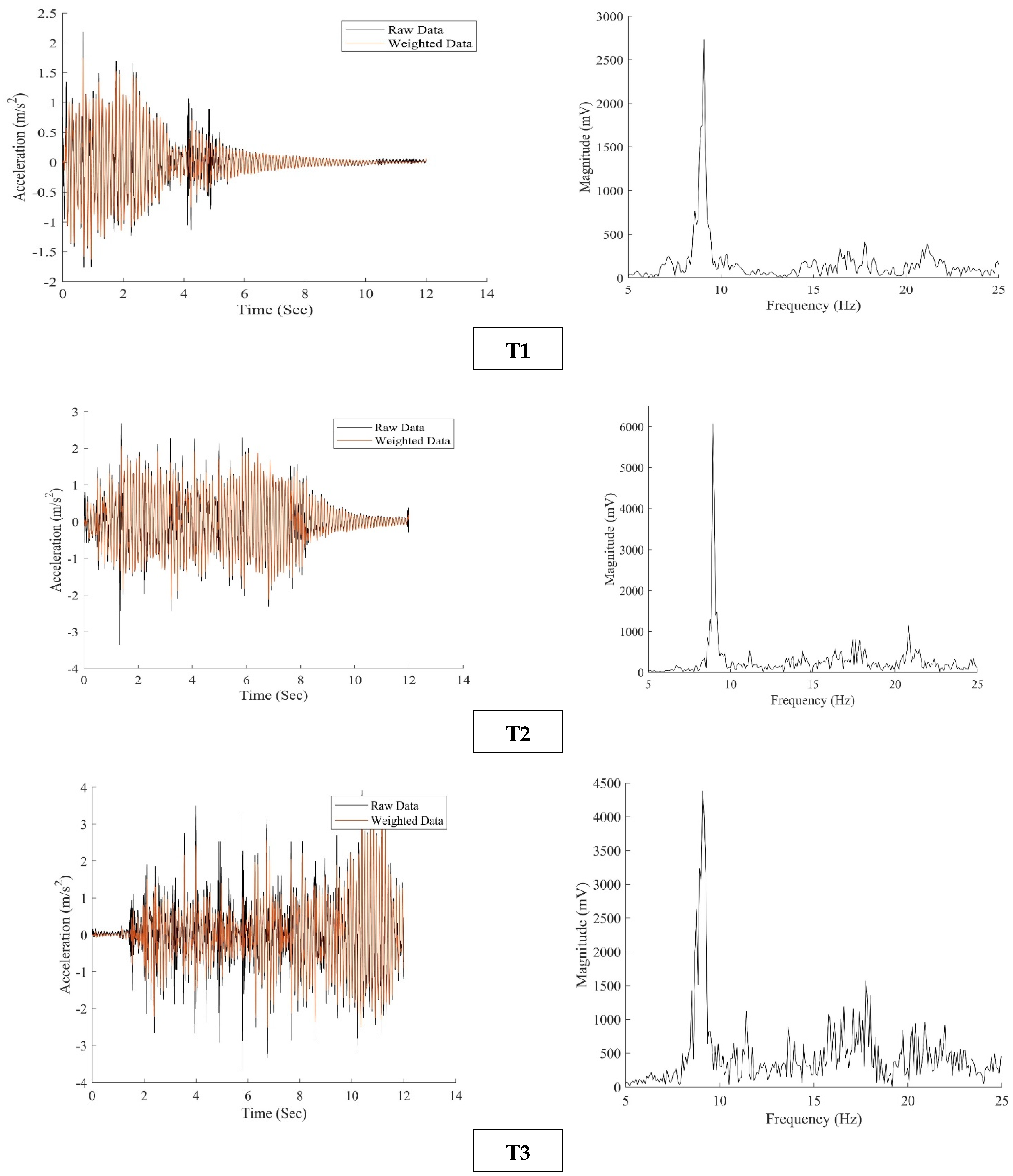
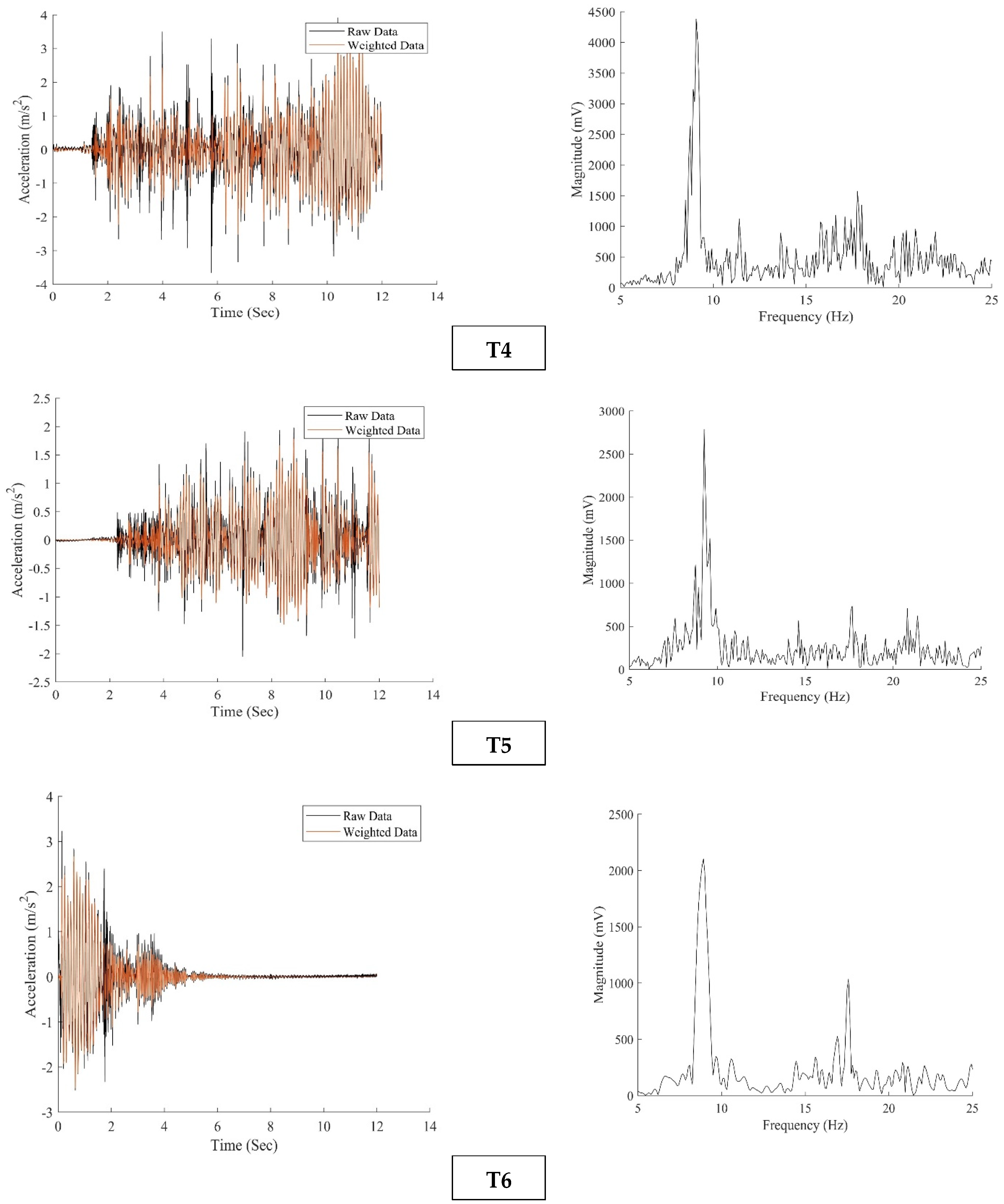
8.3. Walking Tests and Evaluation to ISO 2631.2 and BS 6472-1
| Walking Configuration | ||||||
|---|---|---|---|---|---|---|
| fw (Hz) | Walker 1 (80 kg) | Walker 2 (75 kg) | Single Walker W1 @ St. 1 | Double Walkers W1 Start @ St.1 W2 Start @ St. 2 | Double Walkers W1 Start @ St.1 W2 Start @ St. 3 | |
| T1 | 1.80 | ✓ | - | ✓ | - | - |
| T2 | 2.25 | ✓ | - | ✓ | - | - |
| T3 | 1.80 | ✓ | ✓ | - | ✓ | - |
| T4 | 2.25 | ✓ | ✓ | - | ✓ | - |
| T5 | 1.80 | ✓ | ✓ | - | - | ✓ |
| T6 | 2.25 | ✓ | ✓ | - | - | ✓ |
| Vibration Response Parameters | ||||||
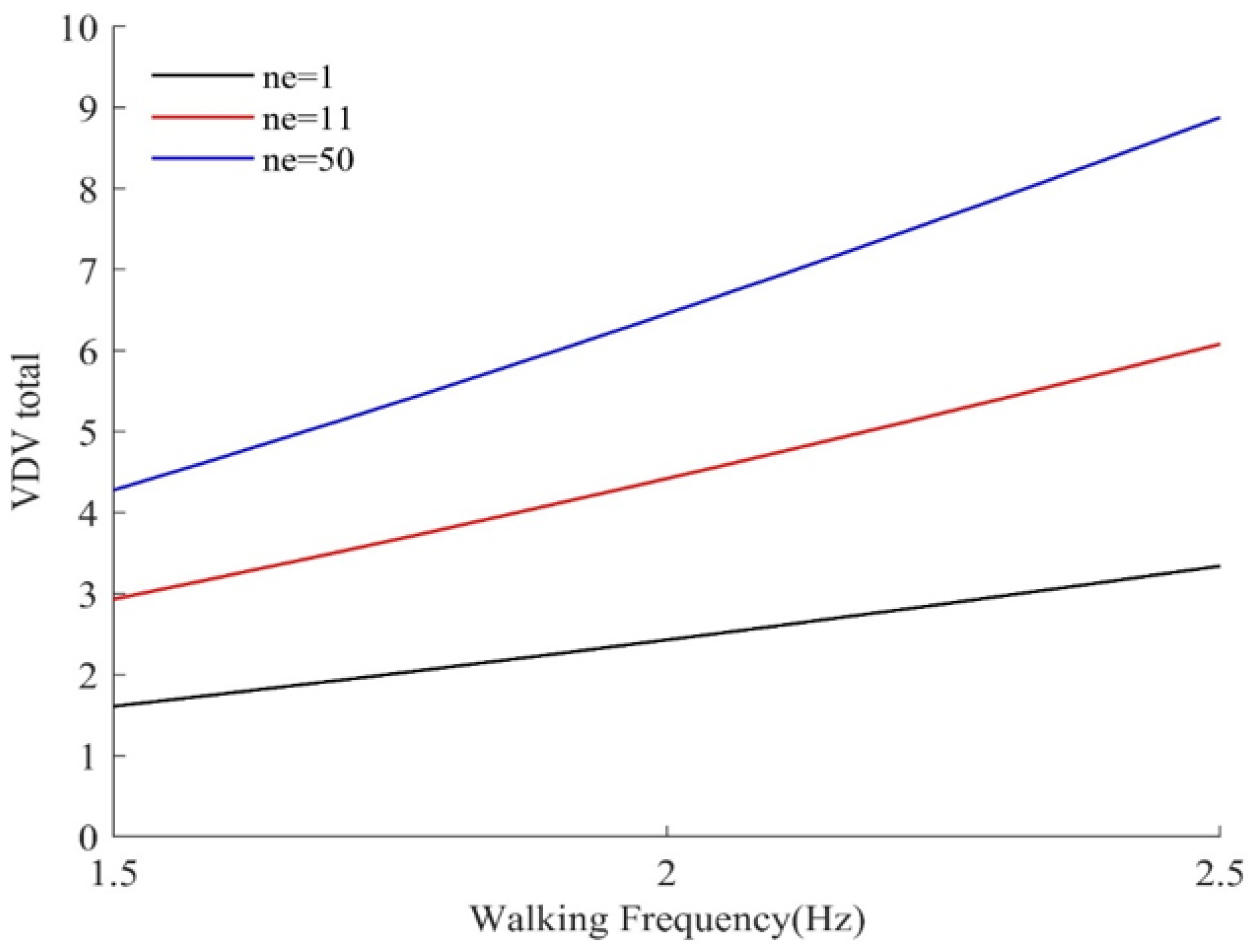
| aw (m/s2) Equation (2) | aw,max (m/s2) | MTVV (m/s2) Equation (3) | VDV (m/s1.75) Equation (4) | Equation (5) | Max number of events, ne Equation (43) | |
| T1 | 0.75 | 1.75 | 0.75 | 0.92 | No | 11 |
| T2 | 0.91 | 2.05 | 0.91 | 1.10 | No | 10 |
| T3 | 1.17 | 2.66 | 1.17 | 1.39 | No | 10 |
| T4 | 1.54 | 3.72 | 1.54 | 1.89 | No | 11 |
| T5 | 0.78 | 1.79 | 0.78 | 0.92 | No | 9 |
| T6 | 1.29 | 2.66 | 1.29 | 1.52 | No | 9 |
8.4. Vibration Design to AS 1170.0 and IRC
8.5. Vibration Design Based on Empirical and Simplified Analytical Methods
8.5.1. Simplified Method in ISO/TR 21136
8.5.2. Empirical Method of Hamm et al.
8.5.3. Combined Frequency, Deflection, and Impulse Velocity in EN 1995-1-1:2004
8.5.4. Vibration-Controlled Span in CSA 086:19
8.5.5. One Step Root Mean Square Method (HIVOSS)
8.5.6. Floor Performance vs. Floor Usage in prEN 1995-1-1: 2025 (Final Draft)
8.6. Vibration Design Based on the Modal Superposition Methods
8.6.1. AISC/CISC DG 11
8.6.2. CCIP-016
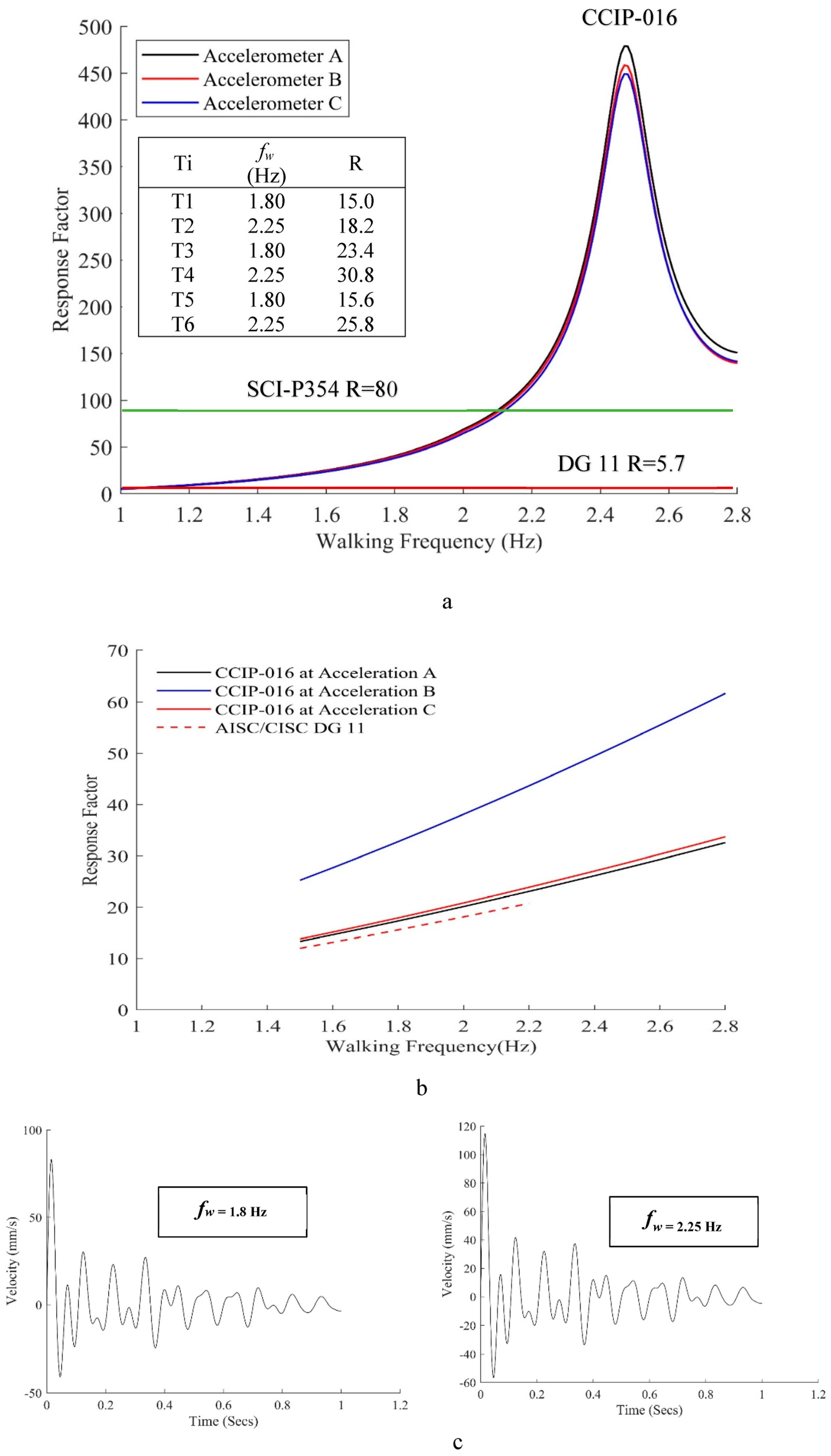
8.6.3. SCI-P354
8.6.4. Harmonized Peak Acceleration and VDV Approach
9. Conclusions and Suggestions for Future Work
- Measure and formulate load functions of continuous and impulsive excitations as well as rhythmic activities, tailored for long-span timber floors. The existing dynamic vertical forces are based on measurement of footfall forces using force plates and on stiff grounds. Furthermore, these force models do not account for the human-induced response between the walker and the floor, such as the feed-back phenomenon observed between the user and the structure with the Millennium Bridge in [98]. These models can be improved by using more accurate measurement equipment such as digital pressure mats. In laboratory testing, the walker can be placed in a virtual reality (VR) environment using VR goggles to achieve more realistic walking dynamics.
- Characterize the dynamic properties (frequencies, modal mass, mode shape, and damping) and response to vibration of long-span floor panels (i) in the laboratory environment, and (ii) floor systems in selected constructed or completed buildings. This will help in understanding the difference between a slab design analogy and the actual performance of the floor within the mass timber or light-frame structural system.
- Develop experimentally validated analytical models that can reliably predict the dynamic properties and vibration response of the floor systems.
- Assess occupant comfort with different floor usages and identify acceptance criteria for the investigated floor systems. Selected completed buildings with long-span timber floors, an office floor for instance, can be instrumented and monitored during working hours, and the occupants’ experiences can be collected from surveys. There will be vast benefit in gathering the data from field tests and occupant surveys to establish an international database for researchers and practitioners worldwide for vibration design of long-span timber floor systems.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yu, M.; Wiedmann, T.; Crawford, R.; Tait, C. The carbon footprint of Australia’s construction sector. Procedia Eng. 2017, 180, 211–220. [Google Scholar] [CrossRef]
- Australian Industry Skills Committee. Construction; Australian Industry Skills Committee: Canberra, Australia, 2022. [Google Scholar]
- Mai, K.Q.; Park, A.; Nguyen, K.T.; Lee, K. Full-scale static and dynamic experiments of hybrid CLT–concrete composite floor. Constr. Build. Mater. 2018, 170, 55–65. [Google Scholar]
- Hassanieh, A.; Valipour, H.; Bradford, M. Experimental and numerical investigation of short-term behaviour of CLT-steel composite beams. Eng. Struct. 2017, 144, 43–57. [Google Scholar] [CrossRef]
- Carradine, D. Multi-Storey Light Timber-Framed Buildings in New Zealand—Engineering Design; BRANZ: Judgeford, New Zealand, 2019. [Google Scholar]
- Masaeli, M.; Gilbert, B.P.; Karampour, H.; Underhill, I.D.; Lyu, C.; Gunalan, S. Experimental assessment of the timber beam-to-column connections: The scaling effect. In Proceedings of the World Conference on Timber Engineering 2021, WCTE 2021, Santiago, Chile, 9–12 August 2021. [Google Scholar]
- TTW. Atlassian Sydney Headquarters. Available online: http://www.ttw.com.au/projects/atlassian-sydney-headquarters (accessed on 9 February 2023).
- United Nations. Department of Economic and Social Affairs, The 2030 Agenda for Sustainable Development. Available online: https://www.un.org/en/development/desa/publications/global-sustainable-development-report-2015-edition.html (accessed on 9 February 2023).
- WoodWorks. U.S. Mass Timber Construction Manual. Available online: https://www.woodworks.org/resources/u-s-mass-timber-construction-manual/ (accessed on 9 February 2023).
- Bazli, M.; Heitzmann, M.; Ashrafi, H. Long-span timber flooring systems: A systematic review from structural performance and design considerations to constructability and sustainability aspects. J. Build. Eng. 2022, 48, 103981. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Peer, A. Wood Products Manufacturing Optimization: A Survey. IEEE Access 2022, 10, 121653–121683. [Google Scholar] [CrossRef]
- Hu, L.J.; Chui, Y.H.; Onysko, D.M. Vibration serviceability of timber floors in residential construction. Prog. Struct. Eng. Mater. 2001, 3, 228–237. [Google Scholar] [CrossRef]
- Pavic, A. Results of IStructE 2015 survey of practitioners on vibration serviceability. In Proceedings of the SECED 2019 Conference: Earthquake Risk and Engineering towards a Resilient Word, London, UK, 9–10 September 2019. [Google Scholar]
- Dolan, J.; Murray, T.; Johnson, J.; Runte, D.; Shue, B. Preventing annoying wood floor vibrations. J. Struct. Eng. 1999, 125, 19–24. [Google Scholar] [CrossRef]
- EN 1995-1-1; Eurocode 5: Design of Timber Structures—Part 1-1: General—Common Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- CSA 086:19; Engineering Design in Wood. National Standard of Canada: Ottawa, ON, Canada, 2019.
- Hu, L. Serviceability design criteria for commercial and multi-family floors. In Canadian Forest Service Report; Forintek Canada Corporation: Sainte-Foy, QC, Canada, 2000. [Google Scholar]
- Chang, W.; Goldsmith, T.; Harris, R. A new design method for timber floors–peak acceleration approach. In Proceedings of the International Network on Timber Engineering Research Meeting 2018, Tallinn, Estonia, 13–16 August 2018. [Google Scholar]
- AS/NZS 1170.0; Structural Design Actions, Part 0: General Principles. Australian Standard: Sydney, Australia, 2002.
- CEAS. Consulting Engineering Advancement Society. Available online: https://ceas.co.nz/ (accessed on 9 February 2023).
- Smith, A.L.; Hicks, S.J.; Devine, P.J. Design of Floors for Vibration: A New Approach; Steel Construction Institute Ascot: Berkshire, UK, 2007. [Google Scholar]
- Concrete Centre. A Design Guide for Footfall Induced Vibration of Structures; Concrete Centre: London, UK, 2006. [Google Scholar]
- Murray, T.; Allen, D.; Ungar, E.; Davis, D.B. Steel Design Guide Series 11: Vibrations of Steel-Framed Structural Systems Due to Human Activity; American Institute of Steel Construction: Chicago, IL, USA, 2016; Available online: https://www.aisc.org/Design-Guide-11-Vibrations-of-Steel-Framed-Structural-Systems-Due-to-Human-Activity-Second-Edition (accessed on 1 June 2023).
- Feldmann, M.; Heinemeyer, C.; Butz, C.; Caetano, E.; Cunha, A.; Galanti, F.; Goldack, A.; Hechler, O.; Hicks, S.; Keil, A. Design of Floor Structures for Human Induced Vibrations. JRC–ECCS Joint Report 2009. Available online: https://publications.jrc.ec.europa.eu/repository/bitstream/JRC55118/jrc_design_of_floor100208.pdf (accessed on 1 June 2023).
- Ebrahimpour, A.; Sack, R.L. A review of vibration serviceability criteria for floor structures. Comput. Struct. 2005, 83, 2488–2494. [Google Scholar] [CrossRef]
- Sitharam, T.; Sebastian, R.; Fazil, F. Vibration isolation of buildings housed with sensitive equipment using open trenches–case study and numerical simulations. Soil Dyn. Earthq. Eng. 2018, 115, 344–351. [Google Scholar] [CrossRef]
- Sanayei, M.; Zhao, N.; Maurya, P.; Moore, J.A.; Zapfe, J.A.; Hines, E.M. Prediction and mitigation of building floor vibrations using a blocking floor. J. Struct. Eng. 2012, 138, 1181–1192. [Google Scholar] [CrossRef]
- Wiss, J.F.; Parmelee, R.A. Human perception of transient vibrations. J. Struct. Div. 1974, 100, 773–787. [Google Scholar] [CrossRef]
- Chopra, A.K. Dynamics of Structures; Pearson Education India: Chennai, India, 2007. [Google Scholar]
- ISO 2631-1–AS 2670.1; Evaluation of Human Exposure to Whole-Body Vibration—Part 1: General Requirements. Australian Standard: Sydney, Australia, 2001.
- ISO 10137; Basis for Design of Structures-Serviceability of Buildings and Walkways against Vibrations. International Standards Organisation: Geneva, Switzerland, 2007.
- AS ISO 2631.2; Mechanical Vibration and Shock—Evaluation of Human Exposure to Wholebody Vibration, Part 2: Vibration in Buildings (1 Hz to 80 Hz). Australian Standard: Sydney, Australia, 2014.
- BS 6472; Guide to the Evaluation of Human Exposure to Vibration in Buildings (1Hz to 80 Hz). British Standards Institution: London, UK, 1992.
- BS 6472-1; Guide to Evaluation of Human Exposure to Vibration in Buildings. British Standards Institution: London, UK, 2008.
- Sedlacek, G.; Heinemeyer, C.; Butz, C.; Veiling, B.; Waarts, P.; Van Duin, F.; Hicks, S.; Devine, P.; Demarco, T. Generalisation of criteria for floor vibrations for industrial, office, residential and public building and gymnastic halls. EUR 2006, 1–343. Available online: https://op.europa.eu/en/publication-detail/-/publication/a2fc45db-6b9a-49e6-9f36-72fc25a8eded/language-en (accessed on 1 June 2023).
- Kerr, S.C. Human Induced Loading on Staircases; University of London: London, UK; University College London: London, UK, 1999; Available online: https://discovery.ucl.ac.uk/id/eprint/1318004/1/312827.pdf (accessed on 1 June 2023).
- Wheeler, J.E. Prediction and control of pedestrian-induced vibration in footbridges. J. Struct. Div. 1982, 108, 2045–2065. [Google Scholar] [CrossRef]
- Basaglia, B.M.; Li, J.; Shrestha, R.; Crews, K. Response prediction to walking-induced vibrations of a long-span timber floor. J. Struct. Eng. 2021, 147, 04020326. [Google Scholar] [CrossRef]
- Ji, T.; Pachi, A. Frequency and velocity of people walking. Struct. Eng. 2005, 84, 36–40. [Google Scholar]
- Toratti, T.; Talja, A. Classification of human induced floor vibrations. Build. Acoust. 2006, 13, 211–221. [Google Scholar] [CrossRef]
- Rahbar Ranji, A.; Rostami Hoseynabadi, H. A semi-analytical solution for forced vibrations response of rectangular orthotropic plates with various boundary conditions. J. Mech. Sci. Technol. 2010, 24, 357–364. [Google Scholar] [CrossRef]
- El-Dardiry, E.; Ji, T. Modelling of the dynamic behaviour of profiled composite floors. Eng. Struct. 2006, 28, 567–579. [Google Scholar] [CrossRef]
- Allen, D.; Murray, T. Design criterion for vibrations due to walking. Eng. J. 1993, 30, 117–129. [Google Scholar]
- Wyatt, T.; Dier, A. Floor serviceability under dynamic loading. In Proceedings of the International Symposium “Building in Steel-The Way Ahead”; ECCS Publication: Stratford-upon-Avon, UK, 1989; pp. 19–20. Available online: https://www.google.com.au/books/edition/Building_in_Steel/o95RAAAAMAAJ?hl=en (accessed on 1 June 2023).
- Ohlsson, S. Ten years of floor vibration research—A review of aspects and some results. In Proceedings of the Symposium/Workshop on Serviceability of Buildings (Movements, Deformations, Vibrations), Ottawa, ON, Canada, 16 May 1988; pp. 419–434. [Google Scholar]
- Karnovsky, I.A.; Lebed, O.I. Formulas for Structural Dynamics: Tables, Graphs and Solutions; McGraw-Hill Education: New York, NY, USA, 2001. [Google Scholar]
- Middleton, C.; Brownjohn, J. Response of high frequency floors: A literature review. Eng. Struct. 2010, 32, 337–352. [Google Scholar] [CrossRef]
- Basaglia, B.M. Dynamic Behaviour of Long-Span Timber Ribbed-Deck Floors. Ph.D. Thesis, University of Technology Sydney, Ultimo, Australia, 2019. [Google Scholar]
- Stürzenbecher, R.; Hofstetter, K.; Eberhardsteiner, J. Structural design of Cross Laminated Timber (CLT) by advanced plate theories. Compos. Sci. Technol. 2010, 70, 1368–1379. [Google Scholar] [CrossRef]
- Karacabeyli, E.; Gagnon, S. Canadian CLT Handbook; FPInnovations: Pointe-Claire, QC, Canada, 2019; Volume 1, Available online: https://web.fpinnovations.ca/wp-content/uploads/clt-handbook-complete-version-en-low.pdf (accessed on 1 June 2023).
- Fink, G.; Honfi, D.; Köhler, J.; Dietsch, P. Basis of design principles for timber structures. In A State-of-the-Art Report by COST Action FP1402/WG1; Shaker: Aachen, Germany, 2018; Available online: https://www.cost.eu/uploads/2018/11/Basis-of-Design-Principles-for-Timber-Structures.pdf (accessed on 1 June 2023).
- Jarnerö, K.; Brandt, A.; Olsson, A. Vibration properties of a timber floor assessed in laboratory and during construction. Eng. Struct. 2015, 82, 44–54. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory, Practice and Application; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- ISO 18324; Timber Structure—Test Methods—Floor Vibration Performance. International Standards Organisation: Geneva, Switzerland, 2016.
- Labonnote, N. Damping in Timber Structures; NTNU-trykk: Trondheim, Norway, 2012. [Google Scholar]
- Rijal, R.; Samali, B.; Shrestha, R.; Crews, K. Experimental and analytical study on dynamic performance of timber floor modules (timber beams). Constr. Build. Mater. 2016, 122, 391–399. [Google Scholar] [CrossRef]
- Conta, S.; Homb, A. Sound radiation of hollow box timber floors under impact excitation: An experimental parameter study. Appl. Acoust. 2020, 161, 107190. [Google Scholar] [CrossRef]
- Ouis, D. On the frequency dependence of the modulus of elasticity of wood. Wood Sci. Technol. 2002, 36, 335–346. [Google Scholar] [CrossRef]
- Craik, R.J.; Barry, P.J. The internal damping of building materials. Appl. Acoust. 1992, 35, 139–148. [Google Scholar] [CrossRef]
- Lin, R.; Zhu, J. On the relationship between viscous and hysteretic damping models and the importance of correct interpretation for system identification. J. Sound Vib. 2009, 325, 14–33. [Google Scholar] [CrossRef]
- Code, B. International Residential Code; International Energy: Paris, France, 2018. [Google Scholar]
- RFS2-CT-2007-00033; Design of Footbridges Guideline. Human Induced Vibrations of Steel Structures. HIVOSS: Luxembourg, 2009.
- Hamm, P.; Richter, A.; Winter, S. Floor vibrations–new results. In Proceedings of the 11th World Conference on Timber Engineerig (WCTE2010), Riva del Garda, Italy, 20–24 June 2010. [Google Scholar]
- AS/NZS 1170.1; Structural Design Actions, Part 1: Permanent, Imposed and Other Actions. Australian Standard: Sydney, Australia, 2002.
- Woeste, F.; Dolan, J. Design to minimize annoying wood-floor vibrations. Struct. Eng. 2007, 8, 24–27. [Google Scholar]
- ISO/TR 21136; Timber Structures—Vibration Performance Criteria for Timber Floors. International Standards Organisation: Geneva, Switzerland, 2017.
- Onysko, D. Serviceability Criteria for Residential Floors Based on a Field Study of Consumer Response; FPInnovations: Ottawa, ON, Canada, 1986; Available online: https://library.fpinnovations.ca/en/permalink/fpipub38186 (accessed on 1 June 2023).
- Smith, I.; Chui, Y.H. Design of lightweight wooden floors to avoid human discomfort. Can. J. Civ. Eng. 1988, 15, 254–262. [Google Scholar] [CrossRef]
- Hu, L. Serviceability of Next Generation Wood Buildings: Laboratory Study of Vibration Performance of Cross-Laminated-Timber (CLT) Floors; FPinnovations: Québec, QC, Canada, 2013; Available online: https://library.fpinnovations.ca/en/permalink/fpipub39703 (accessed on 1 June 2023).
- prEN 1995-1-1:2025; Vibrations. European Committee for Standardization: Brussels, Belgium, 2025.
- EN 16929; Test Methods—Timber Floors—Determination of Vibration Properties. European Committee for Standardization: Brussels, Belgium, 2018.
- ASHRAE. Noise and Vibration Control; Handbook A49; ASHRAE: Peachtree Corners, GA, USA, 2019. [Google Scholar]
- Ellis, B. Serviceability Evaluation of Floor Vibration Induced by Walking Loads; Structural Engineer: London, UK, 2001. [Google Scholar]
- Rainer, J.; Pernica, G.; Allen, D.E. Dynamic loading and response of footbridges. Can. J. Civ. Eng. 1988, 15, 66–71. [Google Scholar] [CrossRef] [Green Version]
- Huang, H.; Gao, Y.; Chang, W.-S. Human-induced vibration of cross-laminated timber (CLT) floor under different boundary conditions. Eng. Struct. 2020, 204, 110016. [Google Scholar] [CrossRef]
- Wang, C.; Chang, W.-S.; Yan, W.; Huang, H. Predicting the human-induced vibration of cross laminated timber floor under multi-person loadings. Structures 2020, 29, 65–78. [Google Scholar] [CrossRef]
- Chen, F.; Li, Z.; He, M.; Wang, Y.; Shu, Z.; He, G. Seismic performance of self-centering steel-timber hybrid shear wall structures. J. Build. Eng. 2021, 43, 102530. [Google Scholar] [CrossRef]
- Schneider, J.; Tannert, T.; Tesfamariam, S.; Stiemer, S. Experimental assessment of a novel steel tube connector in cross-laminated timber. Eng. Struct. 2018, 177, 283–290. [Google Scholar] [CrossRef]
- Karampour, H.; Bourges, M.; Gilbert, B.P.; Bismire, A.; Bailleres, H.; Guan, H. Compressive behaviour of novel timber-filled steel tubular (TFST) columns. Constr. Build. Mater. 2020, 238, 117734. [Google Scholar] [CrossRef]
- Darzi, S.; Karampour, H.; Gilbert, B.P.; Bailleres, H. Numerical study on the flexural capacity of ultra-light composite timber sandwich panels. Compos. Part B Eng. 2018, 155, 212–224. [Google Scholar] [CrossRef]
- Darzi, S.; Karampour, H.; Bailleres, H.; Gilbert, B.P.; McGavin, R.L. Experimental study on bending and shear behaviours of composite timber sandwich panels. Constr. Build. Mater. 2020, 259, 119723. [Google Scholar] [CrossRef]
- Trummer, A.; Krestel, S.; Aicher, S. KIELSTEG-Defining the design parameters for a lightweight wooden product. In Proceedings of the World Conference on Timber Engineering: WCTE 2016, Vienna, Austria, 22–25 August 2016. [Google Scholar]
- Skidmore, O.M.S. Timber Tower Research Project. Available online: https://www.som.com/research/timber-tower-research/ (accessed on 25 January 2023).
- Auclair, S.C.; Hu, L.; Gagnon, S.; Mohammad, M. Effect of type of lateral load resisting system on the natural frequencies of mid-to high-rise wood buildings. In Proceedings of the WCTE 2018, World Conference on Timber Engineering, Seoul, Republic of Korea, 20–23 August 2018. [Google Scholar]
- Jiang, Y.; Crocetti, R. CLT-concrete composite floors with notched shear connectors. Constr. Build. Mater. 2019, 195, 127–139. [Google Scholar] [CrossRef]
- CSA 086-14; Supplement: Engineering Design in Wood. Canadian Standards Association: Toronto, ON, Canada, 2016.
- Council Binational Softwood Lumber. Nail-Laminated Timber: US Design & Construction Guide; Council Binational Softwood Lumber: Surrey, BC, Canada, 2017; Available online: https://info.thinkwood.com/nlt-design-and-construction-guide-u.s.-version-think-wood-0 (accessed on 25 January 2023).
- Barber, D.; Blount, D.; Hand, J.J.; Roelofs, M.; Wingo, L.; Woodson, J.; Yang, F. Design Guide 37: Hybrid Steel Frames with Wood Floors; The American Institute of Steel Construction: Chicago, IL, USA, 2022. [Google Scholar]
- Bitter, R.; Mohiuddin, T.; Nawrocki, M. LabVIEW: Advanced Programming Techniques; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- MathWorks. MATLAB: The Language of Technical Computing: Computation, Visualization, Programming: Installation Guide for UNIX Version 5; MathWorks: Natwick, MA, USA, 2022. [Google Scholar]
- DeSalvo, G.J.; Swanson, J.A. ANSYS Engineering Analysis System: User’s Manual; Swanson Analysis Systems: Houston, TX, USA, 2022. [Google Scholar]
- Brancheriau, L.; Baillères, H. Natural vibration analysis of clear wooden beams: A theoretical review. Wood Sci. Technol. 2002, 36, 347–365. [Google Scholar] [CrossRef]
- Faircloth, A.; Brancheriau, L.; Karampour, H.; So, S.; Bailleres, H.; Kumar, C. Experimental modal analysis of appropriate boundary conditions for the evaluation of cross-laminated timber panels for an in-line approach. For. Prod. J. 2021, 71, 161–170. [Google Scholar] [CrossRef]
- Nemli, G.; Aydın, A. Evaluation of the physical and mechanical properties of particleboard made from the needle litter of Pinus pinaster Ait. Ind. Crops Prod. 2007, 26, 252–258. [Google Scholar] [CrossRef]
- AS 1720.1; Timber Structures—Part 1: Design Methods. Australian Standard: Sydney, Australia, 2010.
- Ross, R. Wood Handbook: Wood as an Engineering Material; Forest Products Laboratory: Madison, WI, USA, 1987. Available online: https://www.fpl.fs.usda.gov/documnts/fplgtr/fplgtr282/fpl_gtr282.pdf (accessed on 25 January 2023).
- AS 4100; Steel Structures. Australian Standard: Sydney, Australia, 2020.
- Dallard, P.; Fitzpatrick, A.; Flint, A.; Le Bourva, S.; Low, A.; Ridsdill Smith, R.; Willford, M. The London millennium footbridge. Struct. Eng. 2001, 79, 17–21. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karampour, H.; Piran, F.; Faircloth, A.; Talebian, N.; Miller, D. Vibration of Timber and Hybrid Floors: A Review of Methods of Measurement, Analysis, and Design. Buildings 2023, 13, 1756. https://doi.org/10.3390/buildings13071756
Karampour H, Piran F, Faircloth A, Talebian N, Miller D. Vibration of Timber and Hybrid Floors: A Review of Methods of Measurement, Analysis, and Design. Buildings. 2023; 13(7):1756. https://doi.org/10.3390/buildings13071756
Chicago/Turabian StyleKarampour, Hassan, Farid Piran, Adam Faircloth, Nima Talebian, and Dane Miller. 2023. "Vibration of Timber and Hybrid Floors: A Review of Methods of Measurement, Analysis, and Design" Buildings 13, no. 7: 1756. https://doi.org/10.3390/buildings13071756
APA StyleKarampour, H., Piran, F., Faircloth, A., Talebian, N., & Miller, D. (2023). Vibration of Timber and Hybrid Floors: A Review of Methods of Measurement, Analysis, and Design. Buildings, 13(7), 1756. https://doi.org/10.3390/buildings13071756