Performance of Novel U-Connector in CFS Truss-to-Column Bolted Connection under Axial Force
Abstract
:1. Introduction
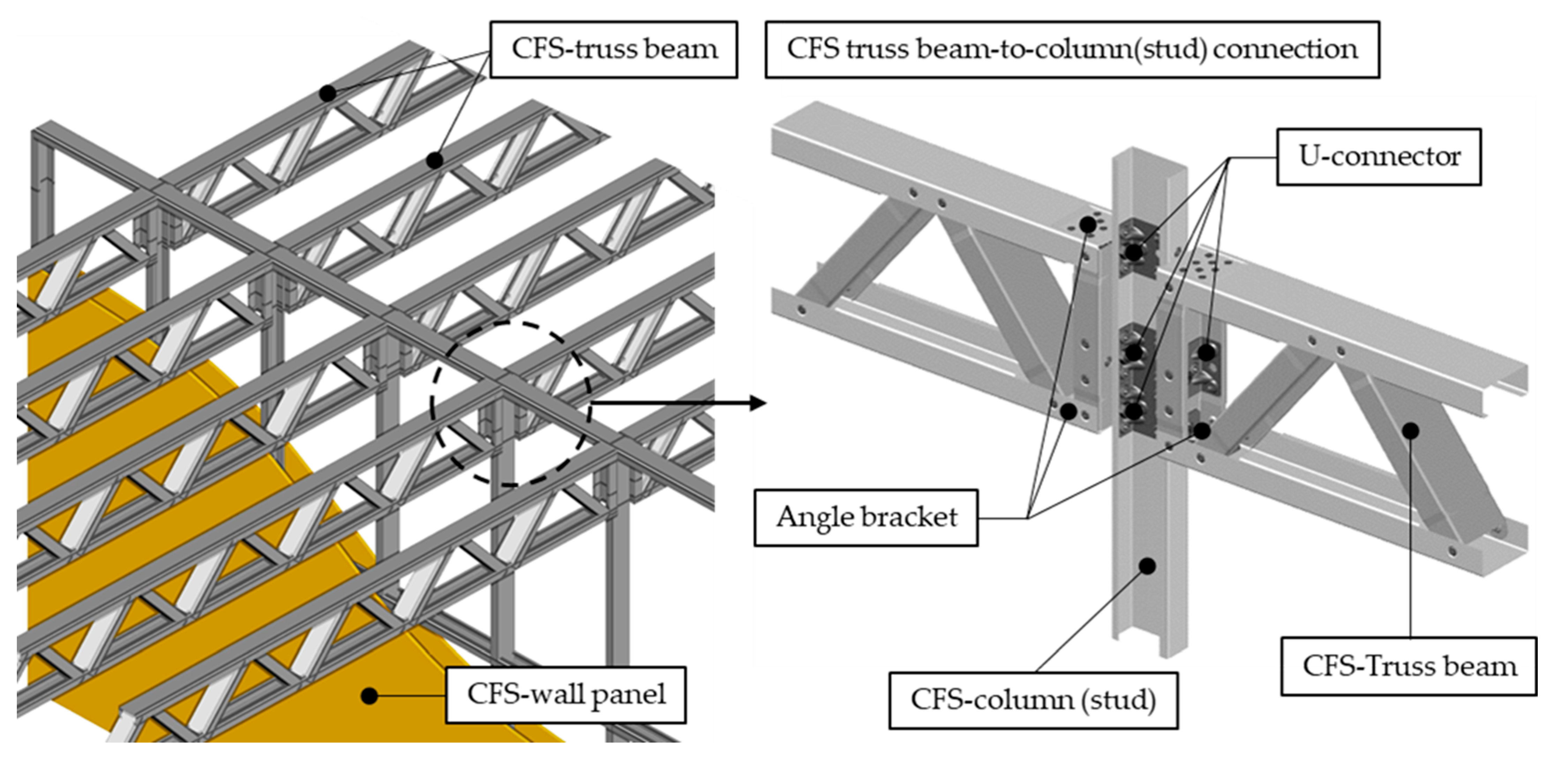
2. Experimental Study
2.1. Test Specimen
2.2. Material Properties
2.3. Test Setup and Measuring Equipment
3. Test Results
3.1. Load-Bearing Capacity
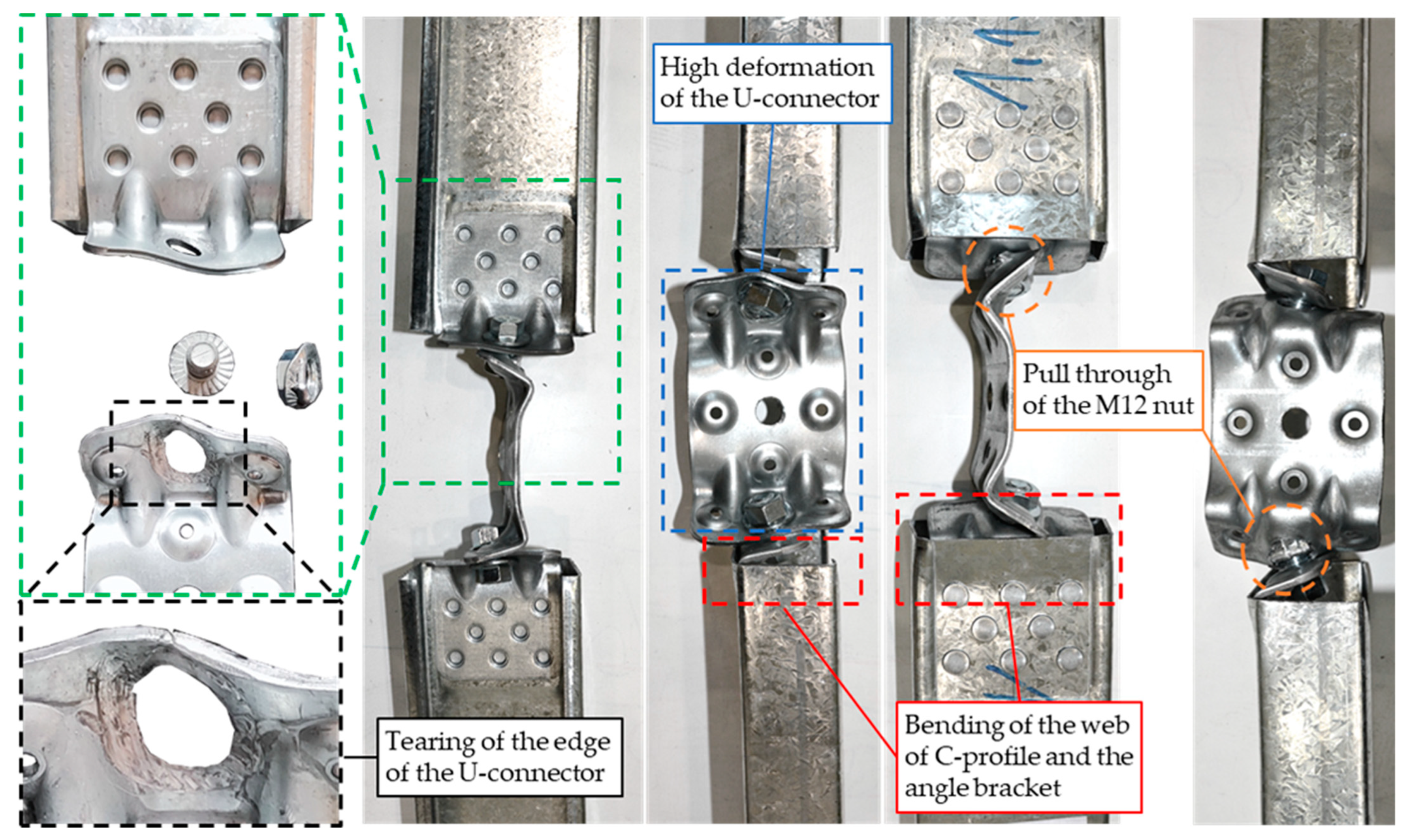
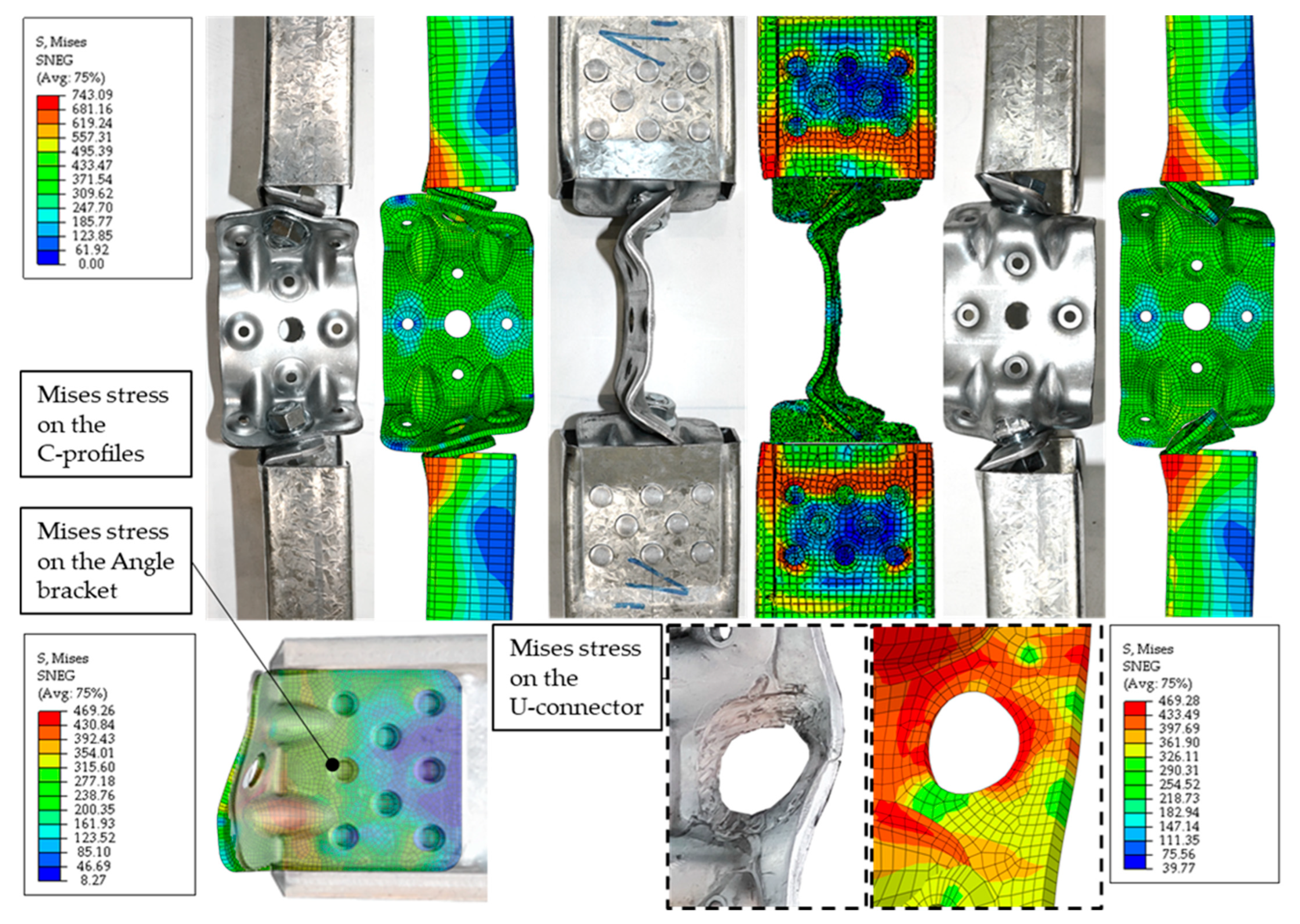
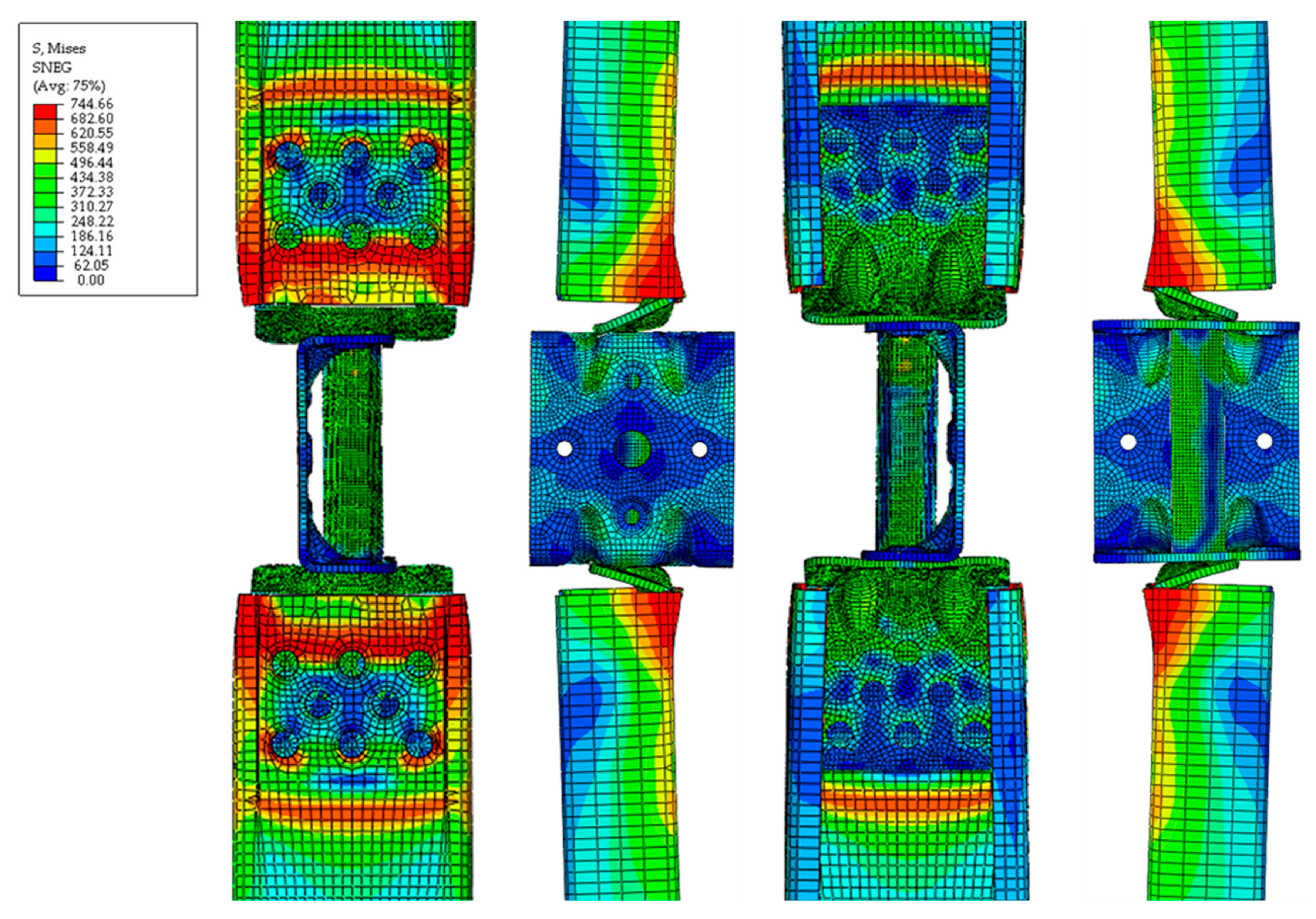
3.2. Failure Mode
4. Numerical Simulation
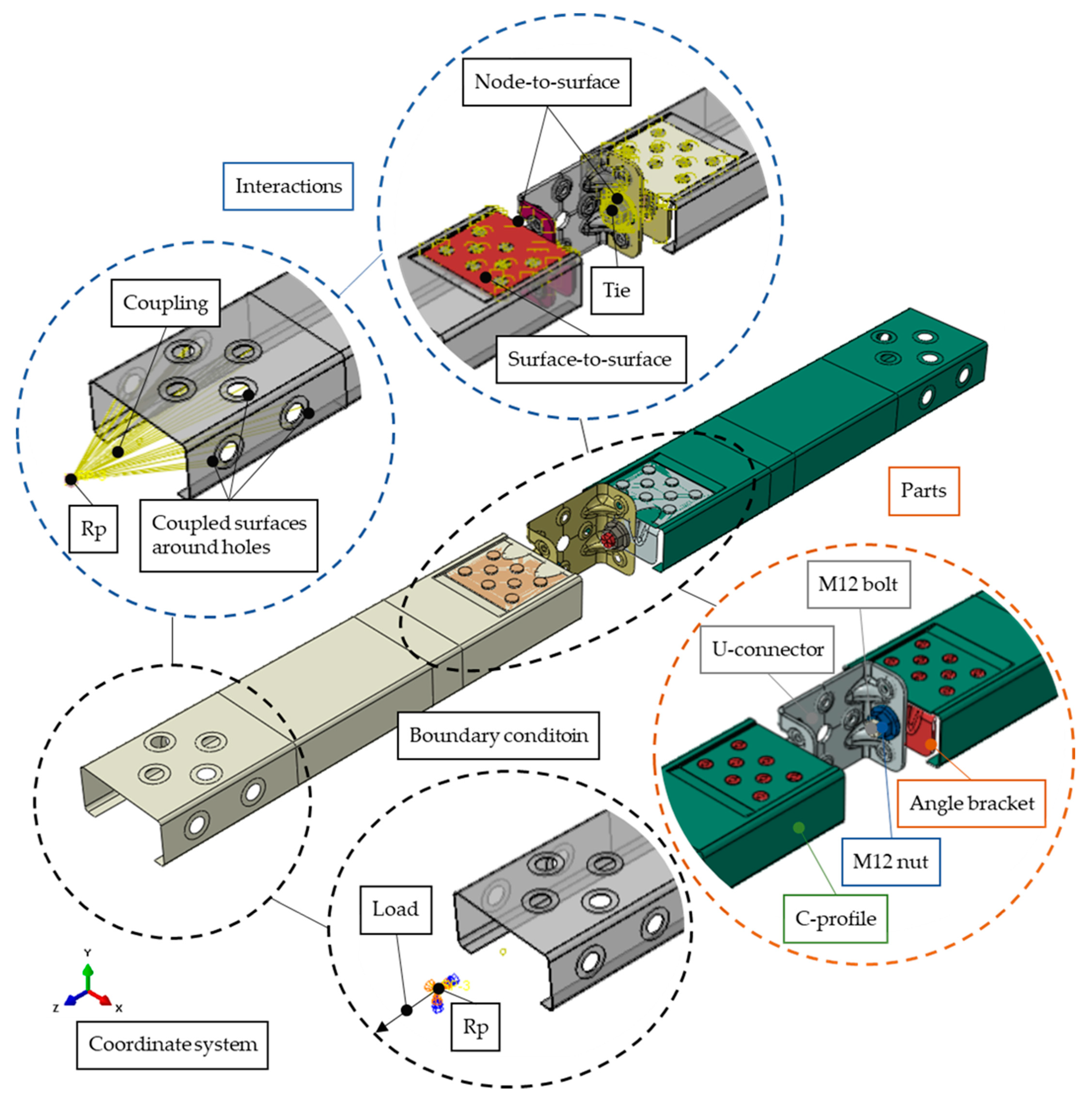
4.1. Finite Element Model
4.1.1. Geometry
4.1.2. Interactions and Boundary Conditions
4.1.3. Material Models
4.1.4. Element Type and Mesh Size
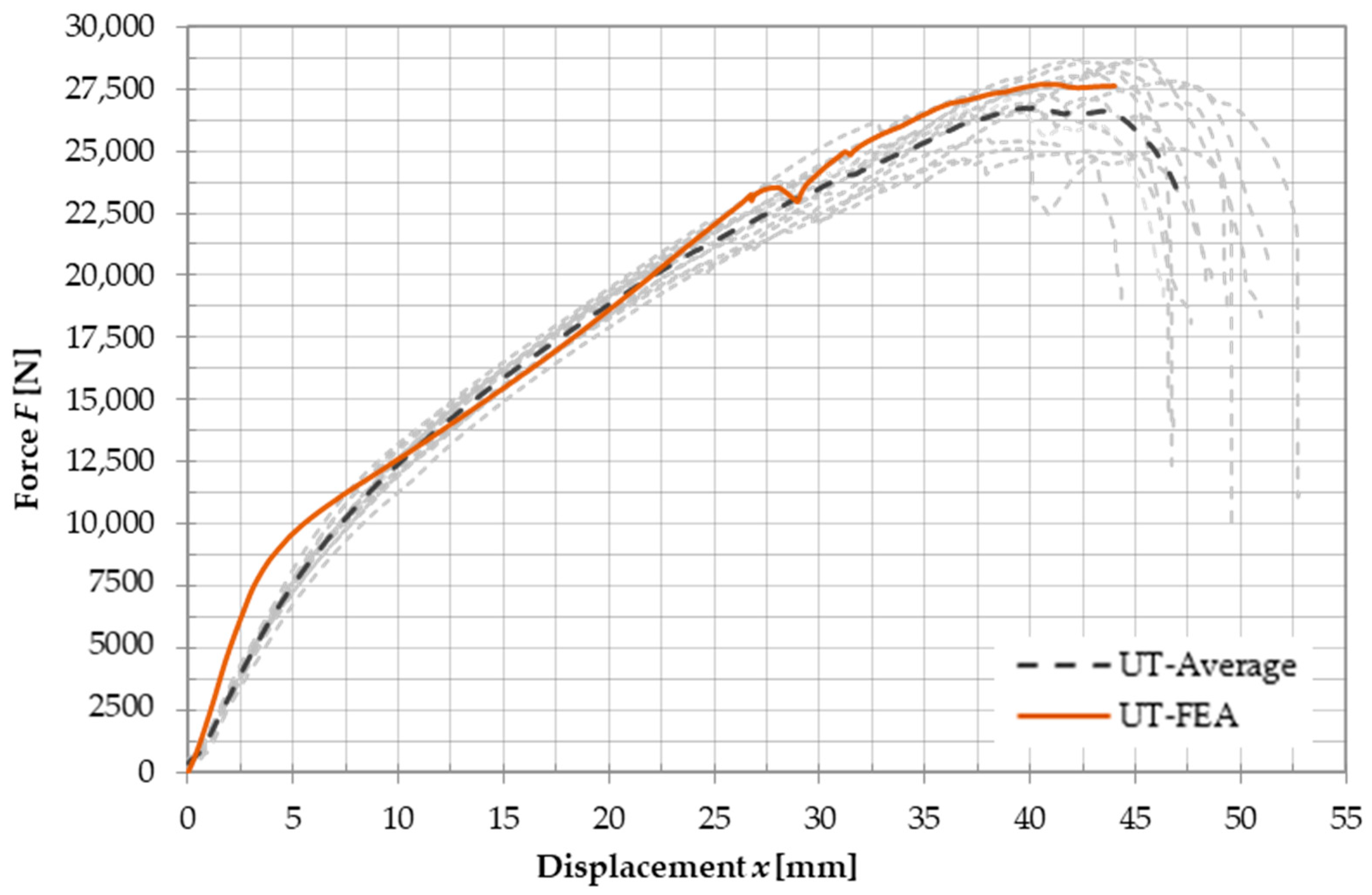
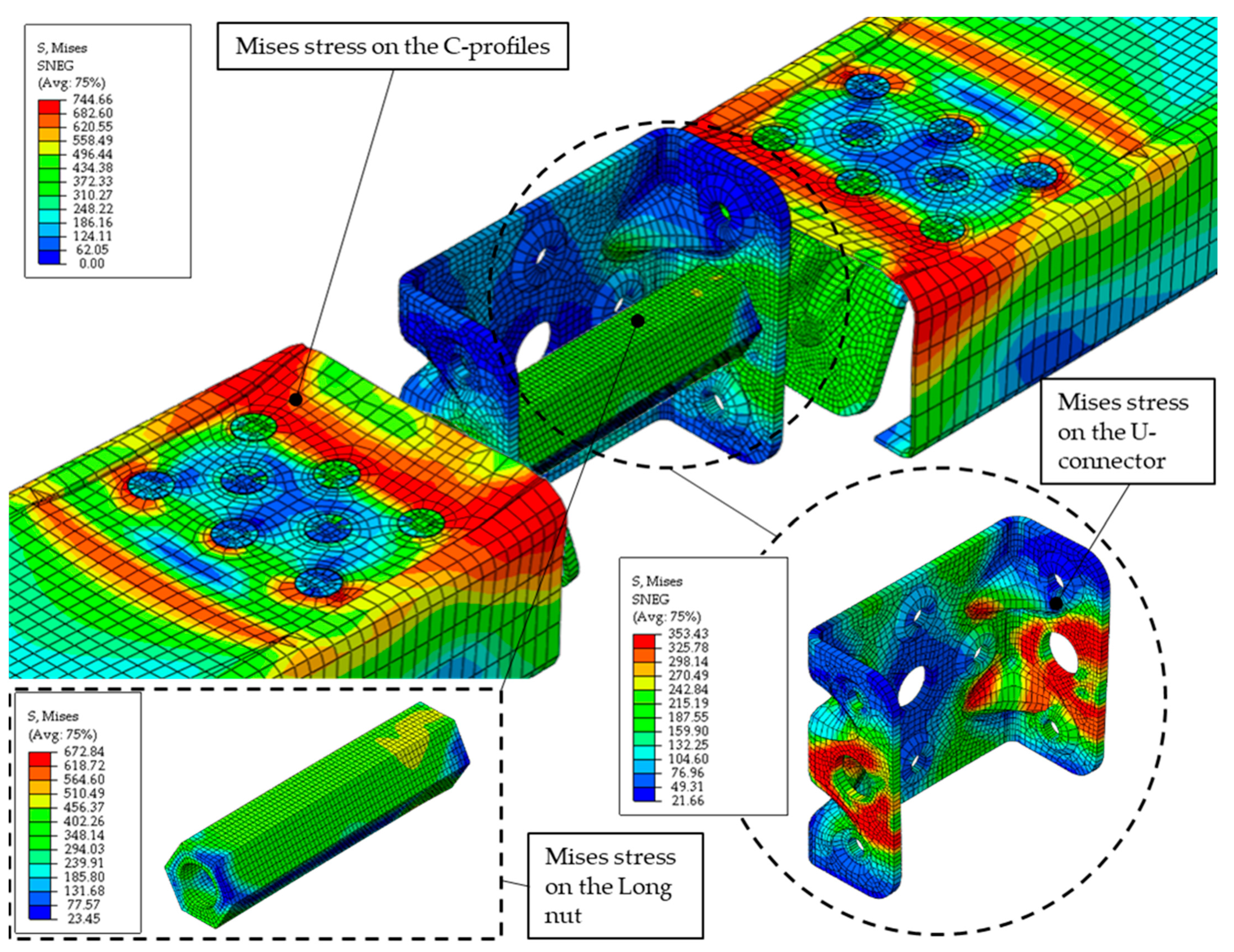
4.1.5. Validation of Numerical Model
4.2. Numerical Simulation of CFS Connection under Compression Loading
4.3. Optimisation of CFS Connection under Compression Loading
Finite Element Modelling
4.4. Comparison of the Optimised Results with the Experimentally Tested and the FEA Obtained Ones
5. Conclusions
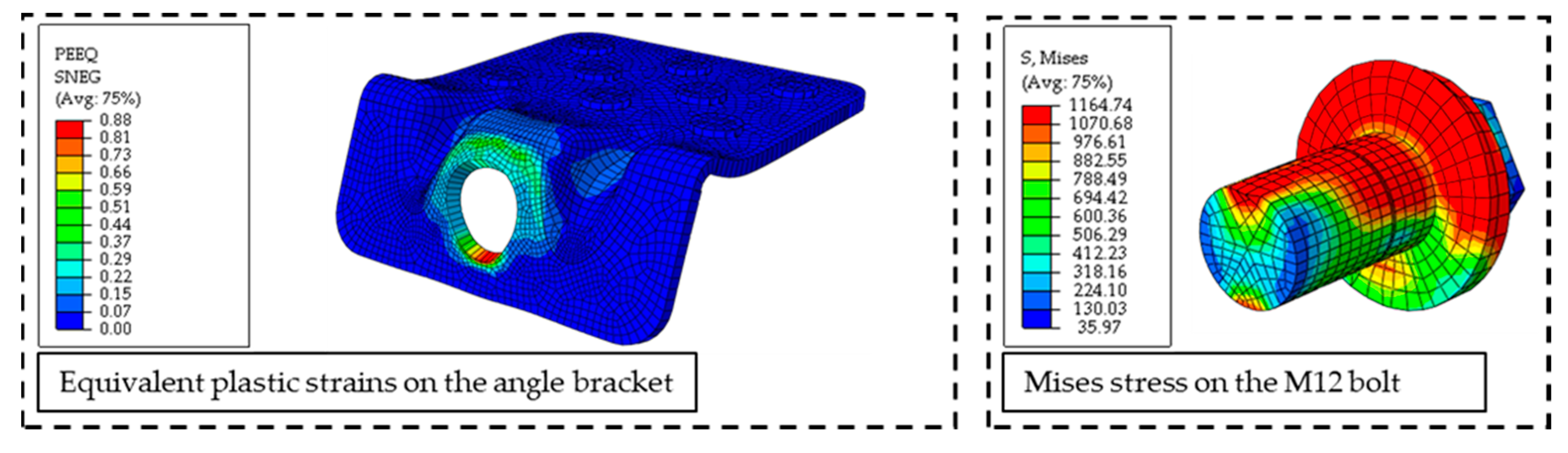
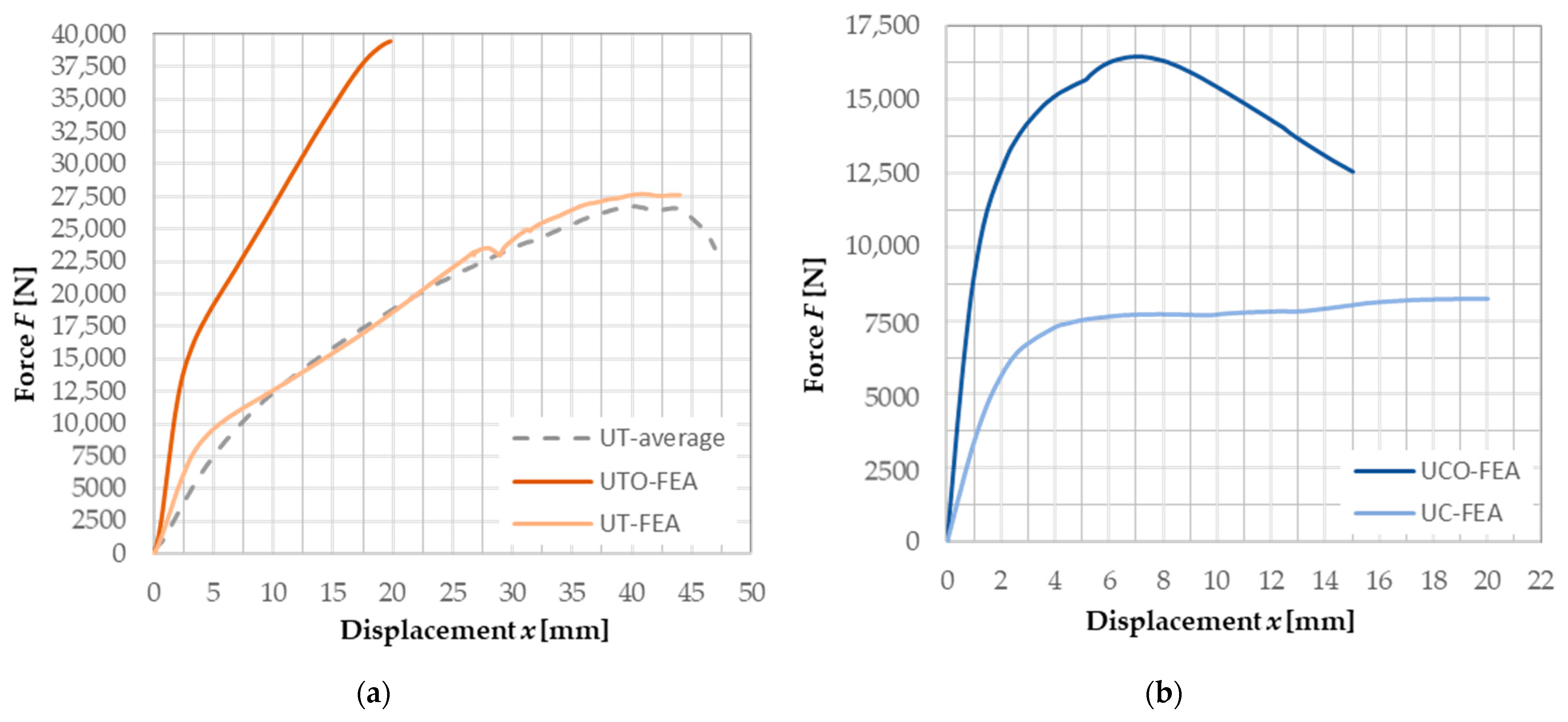
- The results of the experimental tests conducted in this study show that the U-connector exhibits unfavourable behaviour when subjected to loads higher than 7500 N. In particular, the U-connector experienced high plastic deformation, which eventually led to its failure at an average load value of 26,737.56 N with a corresponding displacement of 40.27 mm. The main failure mechanism in the tension component was the tearing of the flange edge of the U-connector, which led to a complete loss of load-bearing capacity.
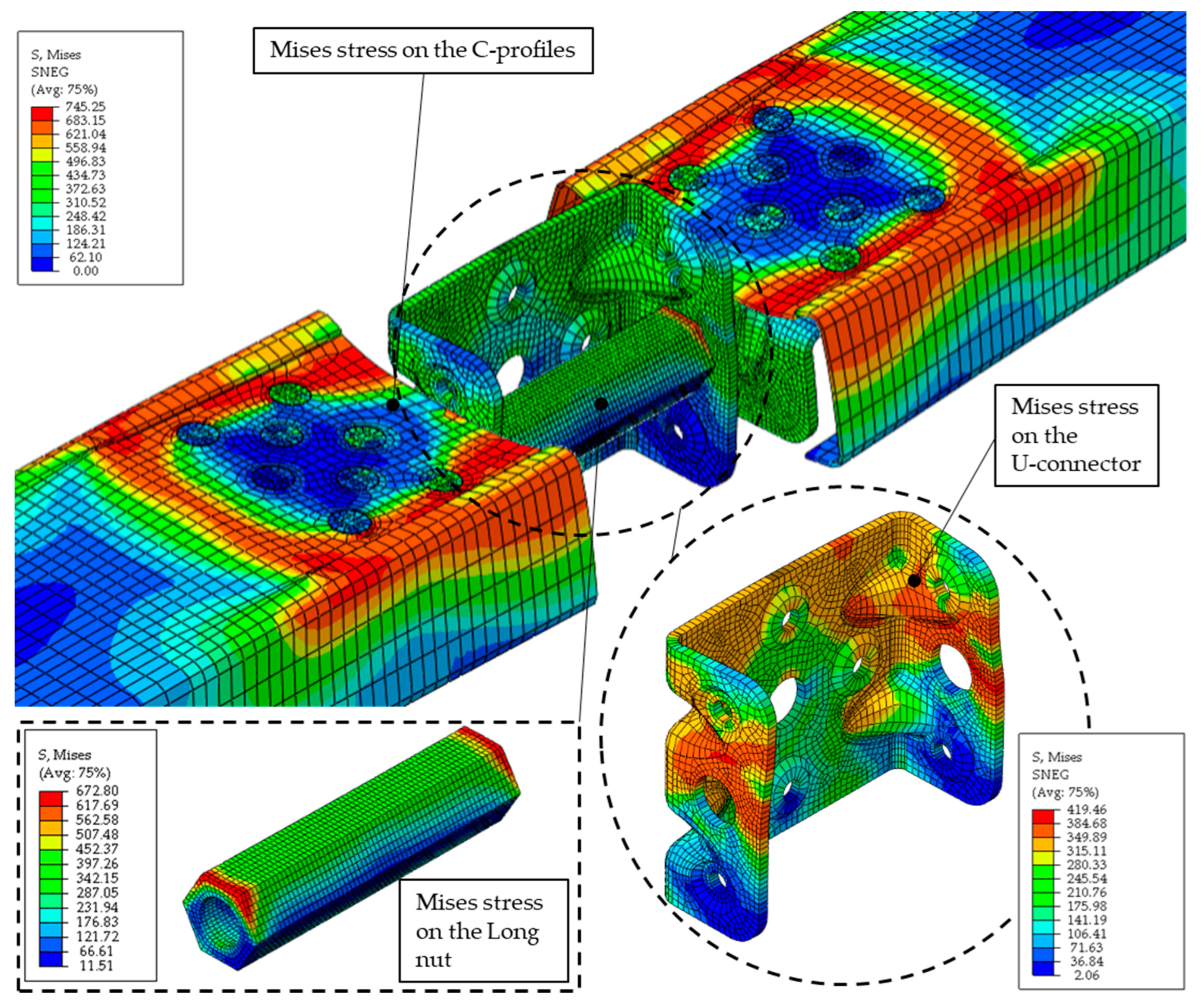
- By using one longer nut instead of two, a geometric optimisation of the proposed connection was achieved, resulting in significantly more favourable behaviour. The optimised connection design allowed full utilisation of the angle brackets with direct load transfer through the long nut. This behaviour of the optimised connection is similar to that of the previously tested anchored angle bracket.
- Although the numerical simulation carried out in this study gave a slightly higher ultimate load of 39,452.40 N, the load before the start of the pull-through failure, it is recommended to use the ultimate load value that was experimentally obtained in the previous work [19].
- The numerical analysis of the optimised tensile component carried out in this study supports the original assumption that a non-uniform stress distribution occurs under the bolt head. This is due to the greater stiffness of the upper part of the flange of the angle bracket, which leads to a concentration of stresses in this area.
- Numerical analysis of the compression component showed a lower load capacity, with most of the deformation occurring in the U-connector. As the deformation increased, contact occurred between the edge of the C-profile and the U-connector, resulting in damage to the C-section.
- The proposed compression component without the long nut is only suitable for light-load applications involving truss beams with compression forces not exceeding approximately 5000 N.
- The use of a long nut in the compression component significantly reduced the deformations in the U-connector and allowed the angle brackets to be fully utilised with an ultimate load value of 16,456.30 N. However, the bending of the angle brackets was the main failure mechanism leading to high plastic deformation of the C-profile flanges. Future work needs to confirm that this effect is insignificant as the vertical element of the truss beam constrains these flanges.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liang, H.; Roy, K.; Fang, Z.; Lim, J.B.P. A Critical Review on Optimization of Cold-Formed Steel Members for Better Structural and Thermal Performances. Buildings 2022, 12, 34. [Google Scholar] [CrossRef]
- Fan, J.; Li, T.; Sun, P.; Wang, D.; Chen, X. Experimental analysis of the truss-to-column connection in a modularized prefabricated steel structure. J. Build. Eng. 2022, 49, 104115. [Google Scholar] [CrossRef]
- Di Lorenzo, G.; De Martino, A. Earthquake response of cold-formed steel-based building systems: An overview of the current state of the art. Buildings 2019, 9, 228. [Google Scholar] [CrossRef] [Green Version]
- Lennon, R.; Pedreschi, R.; Sinha, B.P. Comparative study of some mechanical connections in cold formed steel. Constr. Build. Mater. 1999, 13, 109–116. [Google Scholar] [CrossRef]
- Davies, J.M. Recent research advances in cold-formed steel structures. J. Constr. Steel Res. 2000, 55, 267–288. [Google Scholar] [CrossRef]
- Usefi, N.; Ronagh, H.; Sharafi, P. Lateral performance of a new hybrid CFS shear wall panel for mid-rise construction. J. Constr. Steel Res. 2020, 168, 106000. [Google Scholar] [CrossRef]
- Ayatollahi, S.R.; Usefi, N.; Ronagh, H.; Izadinia, M.; Javaheri, M.R. Performance of gypsum sheathed CFS panels under combined lateral and gravity loading. J. Constr. Steel Res. 2020, 170, 106125. [Google Scholar] [CrossRef]
- Kyprianou, C.; Kyvelou, P.; Gardner, L.; Nethercot, D.A. Characterisation of Material and Connection Behaviour in Sheathed Cold-Formed SteelWall Systems—Part 2: Analytical Modelling. Structures 2021, 30, 1184–1199. [Google Scholar] [CrossRef]
- Wang, J.; Wang, W.; Xiao, Y.; Guo, L. Cyclic behavior tests and evaluation of CFS truss composite floors. J. Build. Eng. 2021, 35, 101974. [Google Scholar] [CrossRef]
- Dizdar, Ç.; Baran, E.; Topkaya, C. Strength and stiffness of floor trusses fabricated from cold-formed steel lipped channels. Eng. Struct. 2019, 181, 437–457. [Google Scholar] [CrossRef]
- Tian, L.-M.; Kou, Y.-F.; Hao, J.-P.; Zhao, L.-W. Flexural performance of a lightweight composite floor comprising cold-formed steel trusses and a composite mortar slab. Thin-Walled Struct. 2019, 144, 106361. [Google Scholar] [CrossRef]
- Bondok, D.H.; Salim, H.A. Failure Capacities of Cold-Formed Steel Roof Trusses End-Connections. Thin-Walled Struct. 2017, 121, 57–66. [Google Scholar] [CrossRef]
- Obeydi, M.; Daei, M.; Zeynalian, M.; Abbasi, M. Numerical Modeling on Thin-Walled Cold-Formed Steel Clip Angles Subjected to Pull-out Failures. Thin-Walled Struct. 2021, 164, 107716. [Google Scholar] [CrossRef]
- Wang, X.; Yuan, X.; Zeng, H.; Li, T.; Liang, Y.; Gao, X.; Yu, Y. Bearing capacity and failure mode of a light-steel tubular K-joint connected by a novel U-shape connector. Appl. Sci. 2021, 11, 8587. [Google Scholar] [CrossRef]
- Wang, X.; Liu, F.; Li, X.; Li, T.; Xu, X.; Shui, Z.; Wu, H. Static Bearing Capacity Investigation of a Novel Prefabricated Light-Steel Beam–Column Connection. Appl. Sci. 2022, 12, 4387. [Google Scholar] [CrossRef]
- Wang, X.; Wang, J.; Xu, M.; Tang, Y.; Li, D.M.; Wang, Q.; Cai, S.; Hu, S.Y.; Liao, C.Y.; Feng, J.S. Prefabricated tubular light roof truss assembled with screwed novel modular connectors: Full-scale test and FE modelling. Thin-Walled Struct. 2023, 182, 110229. [Google Scholar] [CrossRef]
- Tankova, T.; Craveiro, H.; Silva, L.C.; Ribeiro, F.F.; Simões, R.; Martins, C.; Costa, R.; Simões da Silva, L. Behaviour of plug-and-play joints between RHS columns and CFS trusses. Structures 2022, 41, 1719–1745. [Google Scholar] [CrossRef]
- Da Silva, L.S.; Silva, L.C.; Tankova, T.; Craveiro, H.D.; Simoes, R.; Costa, R.; D’Aniello, M.; Landolfo, R. Performance of modular hybrid cold-formed/tubular structural system. Structures 2021, 30, 1006–1019. [Google Scholar] [CrossRef]
- Lukačcević, L.; Krolo, P.; Bakran, A. Experimental Investigation of Novel Angle Bracket Connection in Cold-Formed Steel Structures. Buildings 2022, 12, 1115. [Google Scholar] [CrossRef]
- Abaqus/CAE User’s Manual, version 6.7; ABAQUS Inc.: Pawtucket, RI, USA, 2007.
- DIN 6921; Hexagon Flange Bolts. International Organization for Standardization: Geneva, Switzerland, 1983.
- EN 1661; Hexagon Nuts with Flange. European Committee for Standardization, CEN: Brussels, Belgium, 1996.
- ISO 6892-1:2019; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. The International Organization for Standardization: Geneva, Switzerland, 2019.
- Bolted Joint Analysis. Mechanicalc.com. Available online: https://mechanicalc.com/reference/bolted-joint-analysis (accessed on 8 February 2023).
- Pang, X.-P.; Hu, Y.; Tang, S.-L.; Xiang, Z.; Wu, G.; Xu, T.; Wang, X.-Q. Physical properties of high-strength bolt materials at elevated temperatures. Results Phys. 2019, 13, 102156. [Google Scholar] [CrossRef]
- Quan, G.; Ye, J.; Li, W. Computational modelling of Cold-formed steel lap joints with screw fasteners. Structures 2021, 33, 230–245. [Google Scholar] [CrossRef]
- Schafer, B.W.; Peköz, T. Computational modeling of cold-formed steel: Characterizing geometric imperfections and residual stresses. J. Constr. Steel Res. 1998, 47, 193–210. [Google Scholar] [CrossRef]
- EN 10277:2018; Bright Steel Products—Technical Delivery Conditions. European Committee for Standardization, CEN: Brussels, Belgium, 2018.
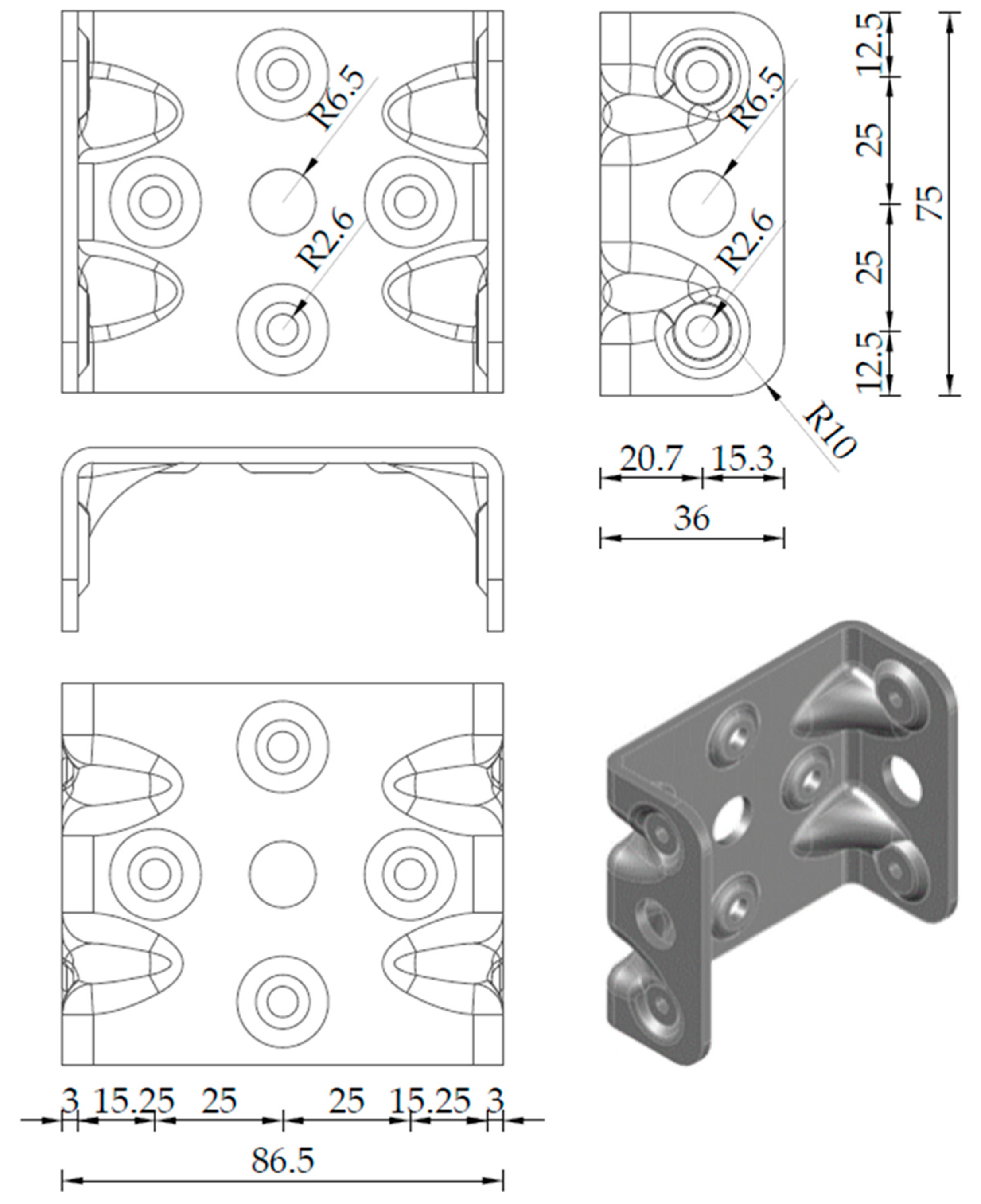

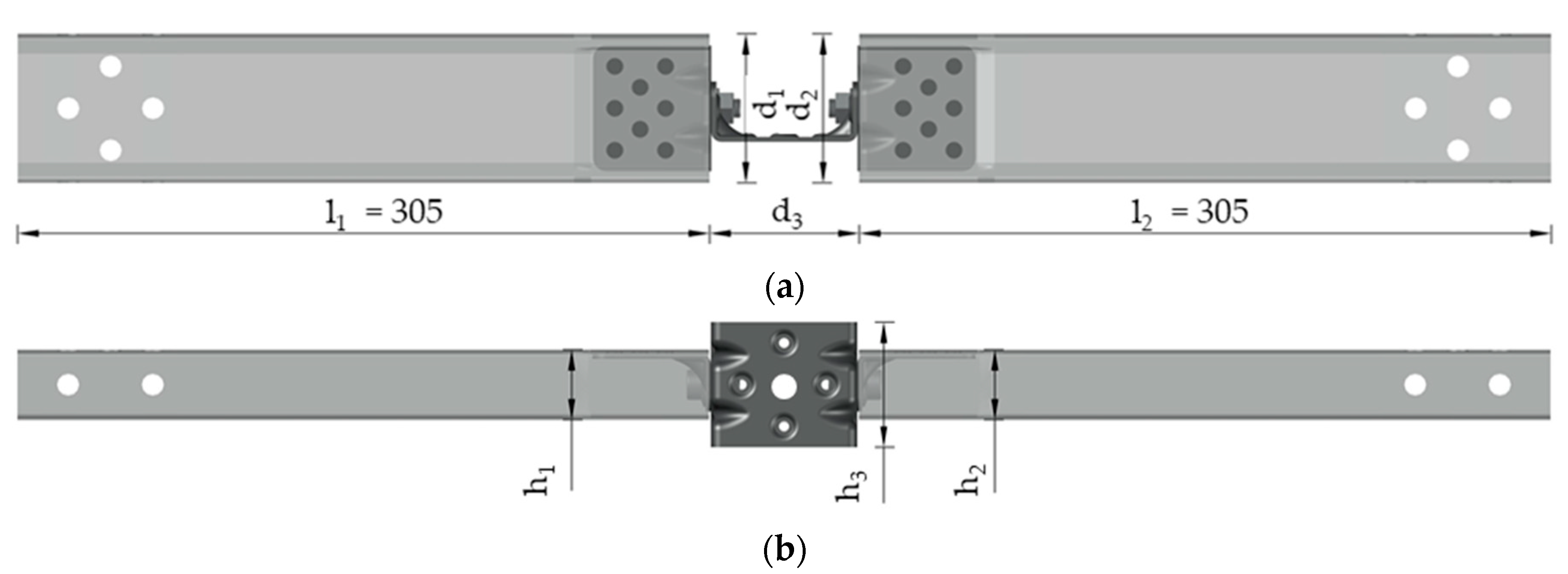
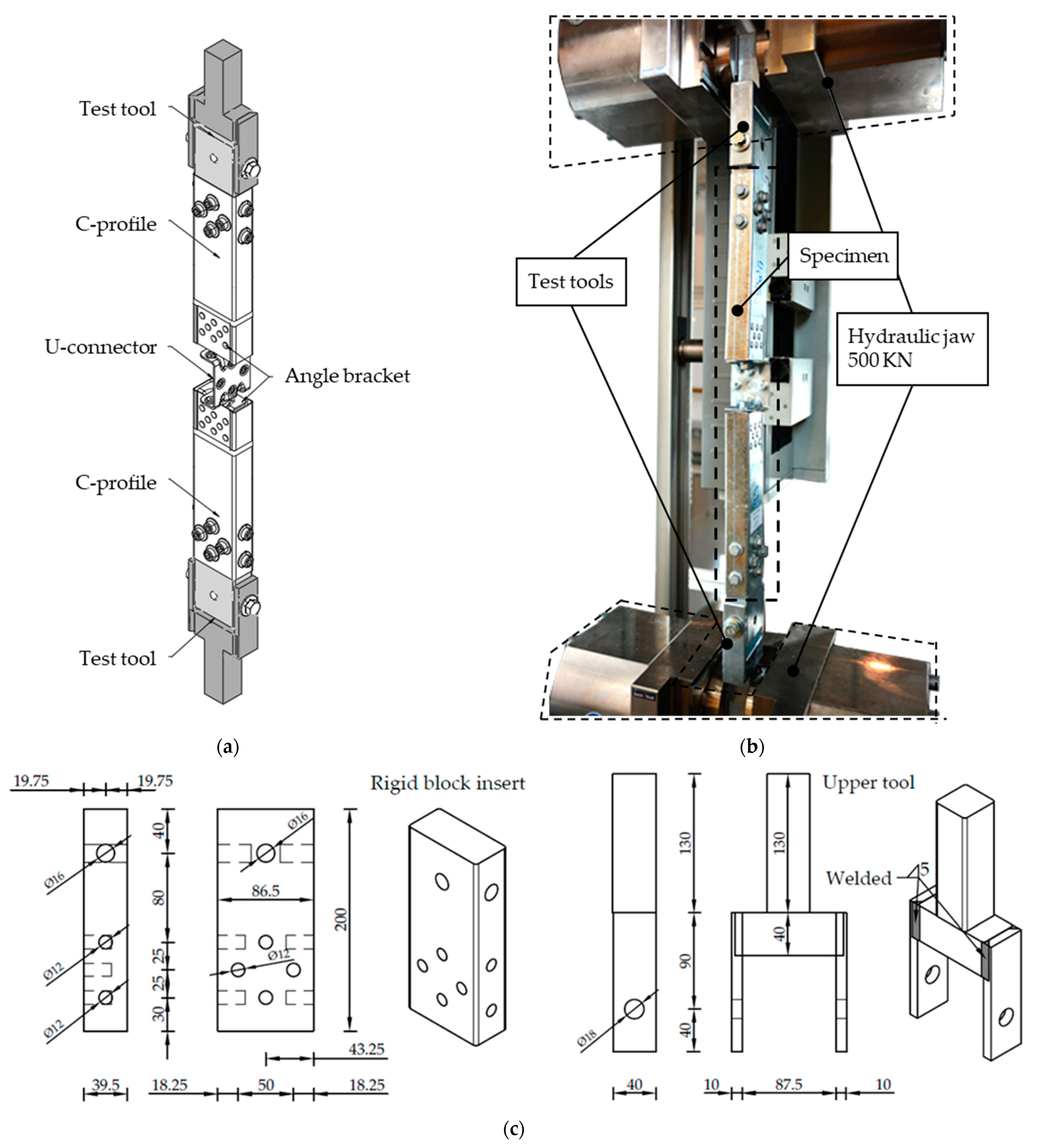






| Specimen | d1 [mm] | d2 [mm] | d3 [mm] | h1 [mm] | h2 [mm] | h3 [mm] |
|---|---|---|---|---|---|---|
| UT-1 | 92.38 | 92.70 | 85.94 | 42.43 | 41.98 | 74.84 |
| UT-2 | 92.37 | 92.13 | 86.07 | 42.66 | 42.27 | 74.81 |
| UT-3 | 92.51 | 92.63 | 85.95 | 42.45 | 42.60 | 74.70 |
| UT-4 | 91.62 | 91.89 | 85.98 | 42.41 | 42.39 | 75.10 |
| UT-5 | 92.18 | 92.04 | 85.97 | 42.10 | 42.12 | 75.06 |
| UT-6 | 92.67 | 92.27 | 86.08 | 42.50 | 42.40 | 74.74 |
| UT-7 | 92.17 | 92.41 | 86.07 | 41.97 | 42.49 | 74.84 |
| UT-8 | 91.92 | 92.06 | 86.09 | 42.30 | 42.52 | 75.14 |
| UT-9 | 92.27 | 92.31 | 86.20 | 42.42 | 42.49 | 74.69 |
| UT-10 | 92.36 | 92.19 | 86.04 | 41.69 | 42.45 | 74.80 |
| UT-11 | 92.69 | 92.28 | 85.94 | 42.27 | 42.39 | 74.80 |
| UT-12 | 92.14 | 92.02 | 86.04 | 42.30 | 42.30 | 74.84 |
| UT (Average) | 92.27 | 92.24 | 86.03 | 42.29 | 42.37 | 74.86 |
| Material | ||||||
|---|---|---|---|---|---|---|
| S550 GD (Average) | 183,737 | 697.22 | 704.98 | 0.39 | 7.41 | 8.89 |
| DX51D (Average) | 194,295 | 375.78 | 414.07 | 0.19 | 13.33 | 26.44 |
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] * |
|---|---|---|---|---|
| UT-1 | 27,102 | 39.10 | 1431 | 35.26 |
| UT-2 | 28,577 | 44.82 | 1567 | 37.36 |
| UT-3 | 27,379 | 39.94 | 1553 | 36.22 |
| UT-4 | 25,129 | 46.99 | 1374 | 37.10 |
| UT-5 | 28,594 | 42.75 | 1461 | 36.30 |
| UT-6 | 27,125 | 42.77 | 1651 | 36.45 |
| UT-7 | 27,836 | 46.98 | 1274 | 39.47 |
| UT-8 | 25,473 | 38.01 | 1368 | 35.83 |
| UT-9 | 28,739 | 45.27 | 1448 | 32.54 |
| UT-10 | 25,005 | 43.03 | 1434 | 37.68 |
| UT-11 | 28,717 | 42.15 | 1572 | 35.98 |
| UT-12 | 28,206 | 44.27 | 1334 | 37.55 |
| UT (Average) | 27,323 | 43.01 | 1455 | 36.48 |
| Standard deviation (RSD **) | 1410 (5.16%) | 2.89 (6.73%) | 112 (7.66%) | 1.66 (4.56%) |
| Specimen | d1 * [mm] | d2 * [mm] | d3 * [mm] | h1 * [mm] | h2 * [mm] | h3 * [mm] |
|---|---|---|---|---|---|---|
| UT-1 | 92.05 | 92.11 | 121.20 | 42.93 | 43.17 | 74.79 |
| UT-2 | 91.68 | 92.09 | 123.43 | 42.98 | 42.64 | 74.54 |
| UT-3 | 91.77 | 91.93 | 122.17 | 42.97 | 42.78 | 74.56 |
| UT-4 | 91.64 | 91.99 | 123.08 | 42.96 | 42.84 | 74.77 |
| UT-5 | 91.86 | 91.93 | 122.27 | 42.98 | 42.52 | 74.71 |
| UT-6 | 92.04 | 91.95 | 122.53 | 42.93 | 42.67 | 74.74 |
| UT-7 | 91.37 | 91.83 | 125.54 | 42.97 | 42.70 | 74.69 |
| UT-8 | 91.83 | 92.06 | 121.92 | 42.93 | 42.75 | 74.55 |
| UT-9 | 92.05 | 92.39 | 118.74 | 42.75 | 42.63 | 74.50 |
| UT-10 | 91.43 | 91.72 | 123.72 | 42.90 | 42.83 | 74.52 |
| UT-11 | 91.54 | 91.62 | 121.92 | 42.87 | 42.65 | 74.56 |
| UT-12 | 92.01 | 92.03 | 123.59 | 42.87 | 42.65 | 74.72 |
| UT (Average) | 91.77 | 91.97 | 122.51 | 42.92 | 42.74 | 74.64 |
| Material (Bolt Class) | S550 GD | DX51D | (8.8) | (10.9) | |||||
|---|---|---|---|---|---|---|---|---|---|
| Elastic | t [mm] | 1.15 | 3.00 | / | / | ||||
| E [N/mm2] | 183,737 | 199,105 | 211,700 | 214,500 | |||||
| ν [-] | 0.3 | 0.3 | 0.3 | 0.3 | |||||
| Plastic | σt [MPa] | εt [-] | σt [MPa] | εt [-] | σt [MPa] | εt [-] | σt [MPa] | εt [-] | |
| 1 (Yield stress) | 680.28 | 0 | 348.52 | 0 | 668.2 | 0 | 1085 | 0 | |
| 2 | 745.25 | 0.06839 | 356.6 | 0.0215 | 936.55 | 0.079 | 1163.63 | 0.0435 | |
| 3 | 400.56 | 0.0441 | |||||||
| 4 | 453.84 | 0.09616 | |||||||
| 5 | 493.02 | 0.19584 | |||||||
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] | A [Nmm] |
|---|---|---|---|---|---|
| UT-average | 26,737 | 40.27 | 1455 | 36.48 | 874,934.5 |
| UT-FEA | 27,685 | 40.55 | 1998 | 36.91 | 830,171.4 |
| Ratio | 1.04 | 1.01 | 1.37 | 1.01 | 0.95 |
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] |
|---|---|---|---|---|
| UC-FEA | 7696 | 8.25 | 2912 | 18.73 |
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] |
|---|---|---|---|---|
| UTO-FEA | 39,452 | 19.79 | 7215 | 0.17 |
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] |
|---|---|---|---|---|
| UT-average | 26,737 | 40.27 | 1455 | 36.48 |
| UT-FEA | 27,684 | 40.55 | 1998 | 36.91 |
| UTO-FEA | 39,452 | 19.79 | 7215 | 0.17 |
| Specimen | Fmax [N] | x [mm] | K [N/mm] | Δl [mm] |
|---|---|---|---|---|
| UC-FEA | 7696 | 8.25 | 2912 | 18.73 |
| UCO-FEA | 16,456 | 7.03 | 7586 | 1.38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lukačević, L.; Krolo, P.; Bakran, A.; Palijan, I. Performance of Novel U-Connector in CFS Truss-to-Column Bolted Connection under Axial Force. Buildings 2023, 13, 1623. https://doi.org/10.3390/buildings13071623
Lukačević L, Krolo P, Bakran A, Palijan I. Performance of Novel U-Connector in CFS Truss-to-Column Bolted Connection under Axial Force. Buildings. 2023; 13(7):1623. https://doi.org/10.3390/buildings13071623
Chicago/Turabian StyleLukačević, Lazar, Paulina Krolo, Antonio Bakran, and Ivan Palijan. 2023. "Performance of Novel U-Connector in CFS Truss-to-Column Bolted Connection under Axial Force" Buildings 13, no. 7: 1623. https://doi.org/10.3390/buildings13071623
APA StyleLukačević, L., Krolo, P., Bakran, A., & Palijan, I. (2023). Performance of Novel U-Connector in CFS Truss-to-Column Bolted Connection under Axial Force. Buildings, 13(7), 1623. https://doi.org/10.3390/buildings13071623







