Compatible Truss-Arch Model for Predicting the Shear Strength of Steel Shape-Reinforced Concrete (SRC) Beams
Abstract
:1. Introduction
2. Proposed Model
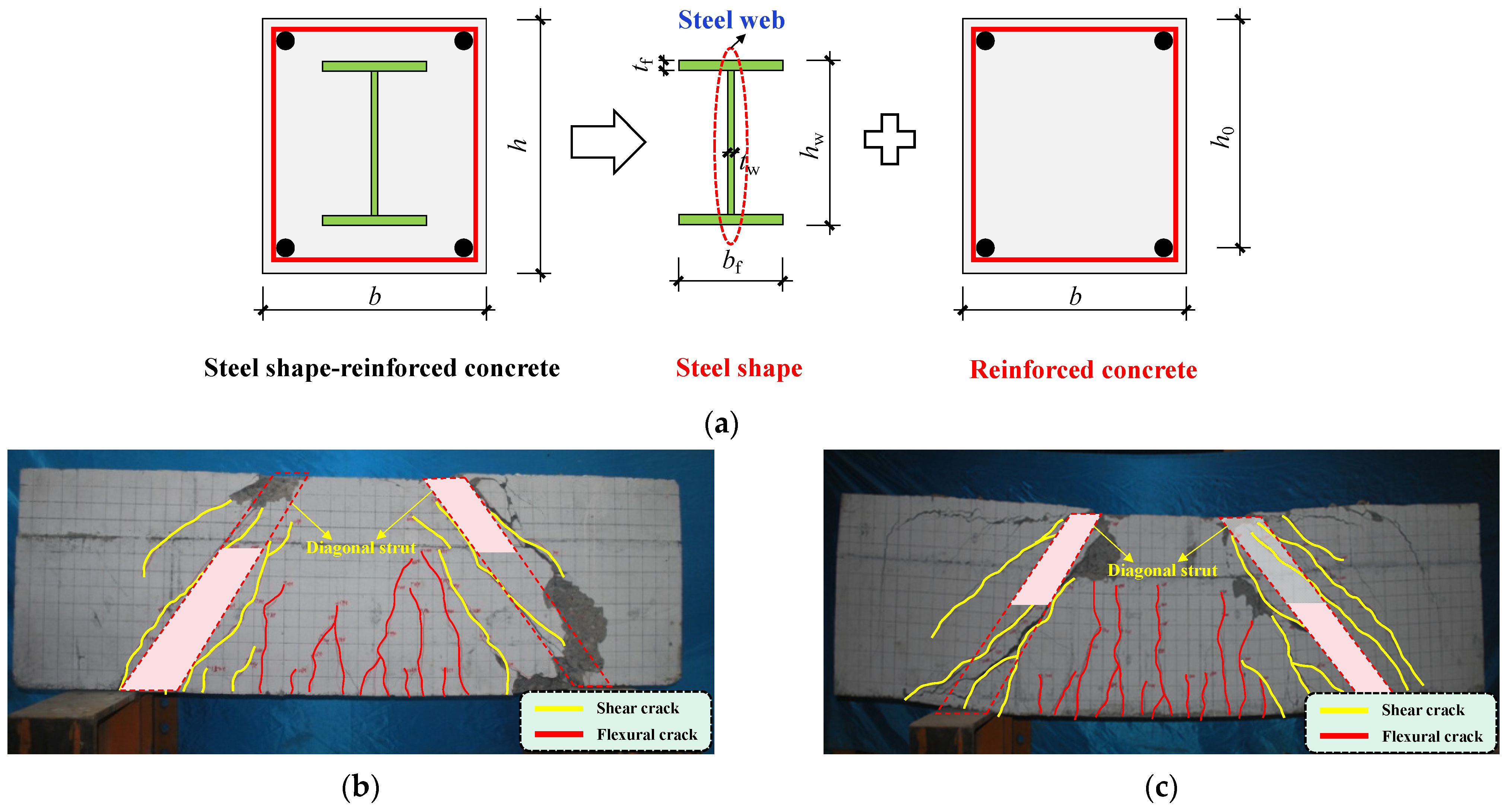
2.1. Model Description
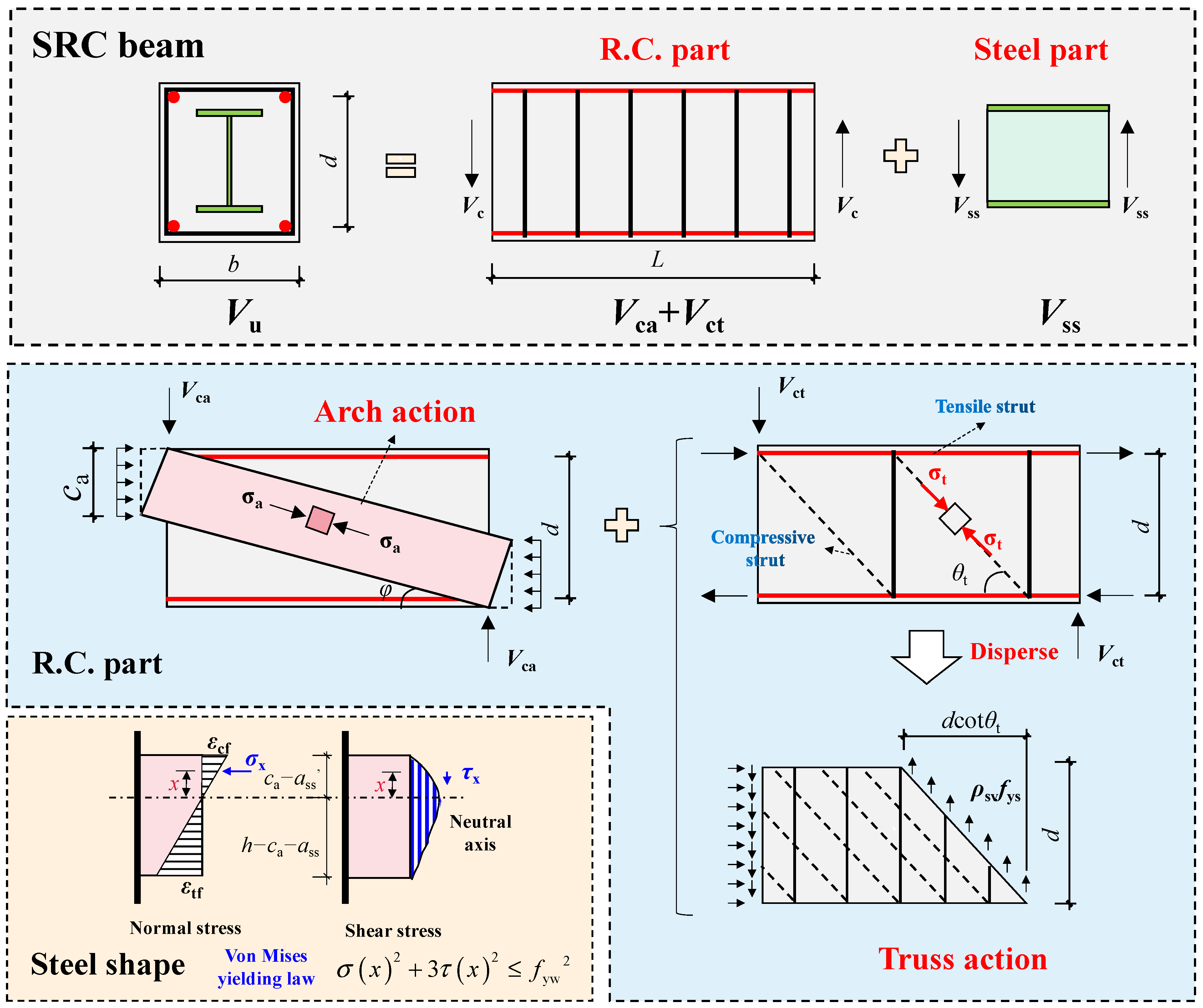
2.2. Truss-Arch Modeling of Composite Truss
2.3. Stress Decomposition of Steel Shape
2.4. Calculation Process
- (1)
- Input the geometric dimensions, reinforcement details, and the properties of concrete and steel;
- (2)
- Calculate the shear resistance of the truss and arch actions Vct + Vca by Equations (1)–(7);
- (3)
- Calculate the shear resistance of the vertical steel web Vss by Equations (9)–(15);
- (4)
- Calculate the overall shear strength of SRC beams by Vct + Vca + Vss.
3. Results and Discussions
3.1. Test Database
- (1)
- The compressive strength of concrete varies from 23.3 MPa to 46.6 MPa;
- (2)
- The yield strength of steel web varies from 265 MPa to 332 MPa;
- (3)
- The steel shape ratio varies from 2.16% to 6.62%;
- (4)
- The shear span-to-depth ratio varies from 0.84 to 2.00;
- (5)
- The width of the cross-section varies from 150 mm to 450 mm;
- (6)
- The height of the cross-section varies from 240 mm to 650 mm;
- (7)
- The stirrup ratio varies from 0.00% to 0.52%.
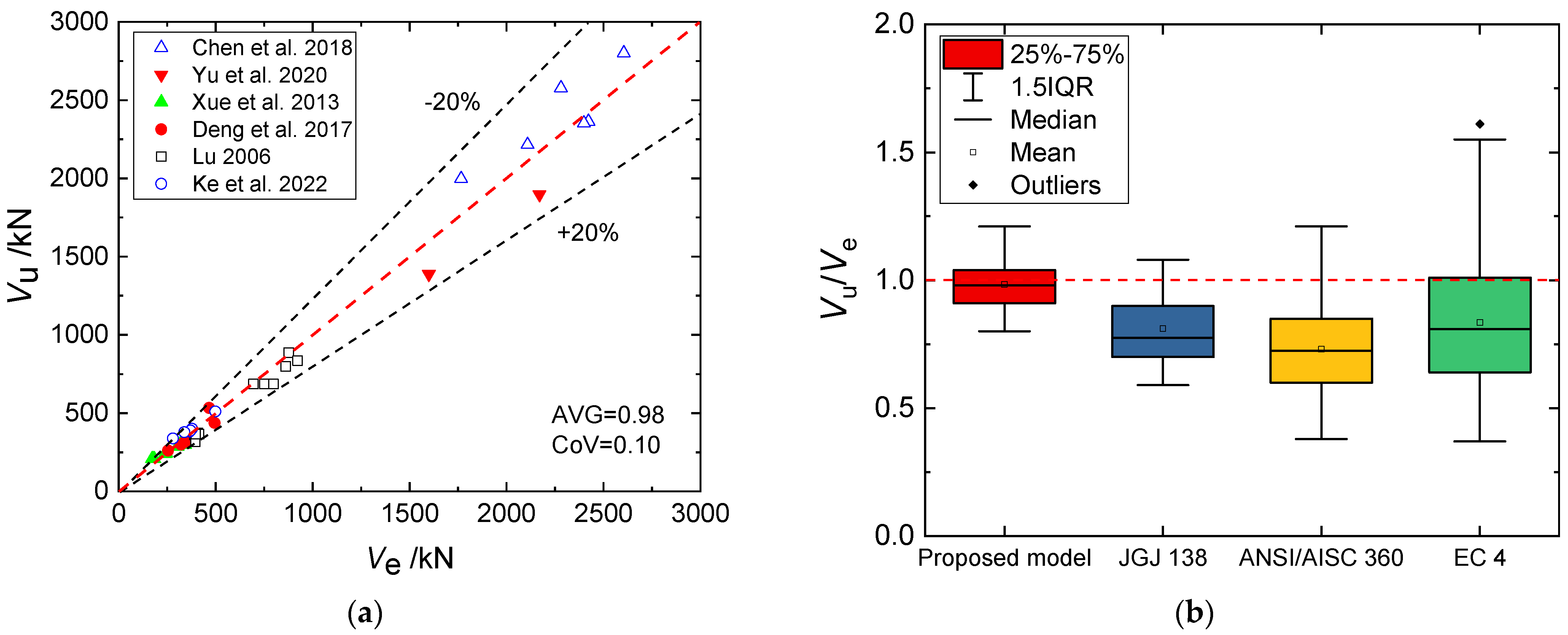
3.2. Comparison between Tested and Calculated Results
4. Conclusions
- (1)
- Multiple shear mechanisms, which consist of a vertical steel web and a composite truss, exist to resist the applied shear load. In the proposed model, the shear strength of the composite truss is evaluated using the traditional truss-arch model, and a stress decomposition based on von Mises yielding criterion is conducted within the steel shape to decouple its shear contribution. Finally, the total shear strength can be determined by superimposing the shear contributions of these two mechanisms;
- (2)
- Through verification with 50 available test results for SRC beams, the proposed model demonstrated its superiority. The predictions generated by the proposed model (with an AVG of 0.98 and a CoV of 0.10) exhibited significantly better agreement with the test results when compared with existing shear equations. For instance, the JGJ 138 equation had an AVG of 0.81 and a CoV of 0.18, the ANSI/AISC 360 equation had values of 0.73 and 0.27, and the Eurocode 4 equation had values of 0.74 and 0.31, indicating that the established model can effectively and reliably predict the shear strength of SRC beams.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lai, B.L.; Zhang, M.Y.; Zheng, X.F.; Chen, Z.P.; Zheng, Y.Y. Experimental Study on the Axial Compressive Behaviour of Steel Reinforced Concrete Composite Columns with Stay-in-Place ECC Jacket. J. Build. Eng. 2023, 68, 106174. [Google Scholar] [CrossRef]
- Lai, B.L.; Yang, L.; Xiong, M.X. Numerical Simulation and Data-Driven Analysis on the Flexural Performance of Steel Reinforced Concrete Composite Members. Eng. Struct. 2021, 247, 113200. [Google Scholar] [CrossRef]
- Lai, B.L.; Tan, W.K.; Feng, Q.T.; Venkateshwaran, A. Numerical Parametric study on the Uniaxial and Biaxial Compressive Behavior of H-shaped Steel Reinforced Concrete Composite Beam-Columns. Adv. Struct. Eng. 2022, 25, 2641–2661. [Google Scholar] [CrossRef]
- JGJ 138-2016; Code for Design of Composite Structures. China Architecture & Building Press: Beijing, China, 2016.
- ANSI/AISC 360-16; Specification for Structural Steel Buildings. American Institute of Steel Construction: Chicago, IL, USA, 2016.
- BS EN 1994-1-1:2004; Eurocode 4: Design of Composite Steel and Concrete Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- Xue, Y.; Shang, C.; Yang, Y.; Yu, Y. Prediction of Lateral Load-Displacement Curve of Concrete-Encased Steel Short Columns Under Shear Failure. Eng. Struct. 2022, 262, 114375. [Google Scholar] [CrossRef]
- Chen, C.C.; Lin, K.T.; Chen, Y.J. Behavior and Shear Strength of Steel Shape Reinforced Concrete Deep Beams. Eng. Struct. 2018, 175, 425–435. [Google Scholar] [CrossRef]
- Hwang, S.J.; Lee, H.J. Strength Prediction for Discontinuity Regions by Softened Strut-and-Tie model. J. Struct. Eng. 2002, 128, 1519–1526. [Google Scholar] [CrossRef]
- Lu, W.Y. Shear Strength Prediction for Steel Reinforced Concrete Deep Beams. J. Constr. Steel Res. 2006, 62, 933–942. [Google Scholar] [CrossRef]
- Yu, Y.; Yang, Y.; Xue, Y.; Liu, Y. Shear Behavior and Shear Capacity Prediction of Precast Concrete-Encased Steel Beams. Steel Compos. Struct. 2020, 36, 261–272. [Google Scholar]
- Deng, M.; Ma, F.; Li, B.; Liang, X. Analysis on Shear Capacity of SRC Deep Beams based on Modified Strut-and-Tie Model. Eng. Mech. 2017, 34, 95–103. [Google Scholar]
- Ke, X.J.; Tang, Z.K.; Yang, C.H. Shear Bearing Capacity of Steel-Reinforced Recycled Aggregate Concrete Short Beams based on Modified Compression Field Theory. Structures 2022, 45, 645–658. [Google Scholar] [CrossRef]
- Chen, B.Q.; Zeng, L.; Liu, C.J.; Mo, J. Study on Shear Behavior and Bearing Capacity of Steel Reinforced Concrete Deep Beams. Eng. Mech. 2022, 39, 215–224. [Google Scholar]
- Bentz, E.C.; Vecchio, F.J.; Collins, M.P. Simplified Modified Compression Field Theory for Calculating Shear Strength of Reinforced Concrete Elements. ACI. Struct. J. 2006, 103, 614–624. [Google Scholar]
- Ichinose, T. A Shear Design Equation for Ductile R/C Members. Earthq. Eng. Struct. D 1992, 21, 197–214. [Google Scholar] [CrossRef]
- ACI 318-14; Building Code Requirements for Structural Concrete. American Concrete Institute: Farmington Hills, MI, USA, 2014.
- Kim, J.H.; Mander, J.B. Influence of Transverse Reinforcement on Elastic Shear Stiffness of Cracked Concrete Elements. Eng. Struct. 2007, 29, 1798–1807. [Google Scholar] [CrossRef]
- Pan, Z.; Li, B. Truss-Arch Model for Shear Strength of Shear-Critical Reinforced Concrete Columns. J. Struct. Eng. 2013, 139, 548–560. [Google Scholar]
- Horne, M.R. Plastic Theory of Structures; Pergamon Press: Surrey, UK, 1979. [Google Scholar]
- Choi, K.K.; Park, H.G.; Wight, J.K. Unified Shear Strength Model for Reinforced Concrete Beams-Part I: Development. ACI Struct. J. 2007, 104, 142–152. [Google Scholar]
- Choi, K.K.; Park, H.G. Unified Shear Strength Model for Reinforced Concrete Beams-Part II: Verification and Simplified Method. ACI Struct. J. 2007, 104, 153–161. [Google Scholar]
- Xue, J.; Wang, X.; Ma, H.; Lin, J.; Chen, Z. Experimental Study on Shear Performance of Steel Reinforced Recycled Aggregate Concrete Beams. Build. Struct. 2013, 43, 69–72. [Google Scholar]
- Barkhordari, M.S.; Feng, D.C.; Tehranizadeh, M. Efficiency of Hybrid Algorithms for Estimating the Shear Strength of Deep Reinforced Concrete Beams. Period. Polytech. Civ. Eng. 2022, 66, 398–410. [Google Scholar] [CrossRef]
- Xue, Y.; Yang, Y.; Yu, Y. Shear Strength Model for Steel Reinforced Concrete Composite Members: Short Columns and Deep Beams. Eng. Struct. 2020, 216, 110748. [Google Scholar] [CrossRef]
| Ref. | Specimen ID | Steel Shape | fyw/MPa | ρss /% | L/mm | b/mm | h/mm | ρsv /% | ρsl /% | fc/MPa | fys/MPa | fyw/MPa | Ve/kN | Vu/kN |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| [8] | BH1 | H450 × 200 × 9 × 14 | 312 | 3.80 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 312 | 2423 | 2364 |
| BH2 | H390 × 200 × 10 × 16 | 330 | 4.03 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 330 | 2399 | 2353 | |
| BH3 | H300 × 200 × 13 × 15 | 313 | 3.84 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 313 | 2109 | 2216 | |
| BH4 | H200 × 200 × 20 × 12 | 280 | 3.36 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 280 | 1766 | 1999 | |
| BWH2 | H390 × 200 × 10 × 16 | 330 | 4.03 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 330 | 2399 | 2353 | |
| BWH2A | H390 × 200 × 15 × 16 | 312 | 4.76 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 312 | 2281 | 2578 | |
| BWH2B | H390 × 200 × 20 × 16 | 303 | 5.48 | 460 | 450 | 550 | / | 1.32 | 40.10 | / | 303 | 2605 | 2802 | |
| [10] | SRC1-00 | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | / | 1.45 | 28.90 | / | 332 | 772 | 686 |
| SRC1-00-T | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | / | 1.45 | 28.90 | / | 332 | 751 | 686 | |
| SRC1-00-E | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | / | 1.45 | 28.90 | / | 332 | 799 | 686 | |
| SRC1-00-D | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | / | 1.45 | 28.90 | / | 332 | 695 | 686 | |
| SRC1-50 | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | 0.09 | 1.45 | 27.70 | 380 | 332 | 861 | 798 | |
| SRC1-25 | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | 0.18 | 1.45 | 32.20 | 380 | 332 | 877 | 885 | |
| SRC1-17 | H300 × 150 × 6.5 × 9 | 332 | 2.16 | 975 | 350 | 600 | 0.26 | 1.45 | 28.90 | 380 | 332 | 923 | 835 | |
| D1-N | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 24.50 | 407 | 325 | 408 | 373 | |
| D2-FS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 23.90 | 407 | 325 | 415 | 366 | |
| D3-WS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 23.90 | 407 | 325 | 395 | 366 | |
| DB1-15-NS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 23.30 | 407 | 325 | 391 | 359 | |
| DB2-15-NS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 24.50 | 407 | 325 | 409 | 373 | |
| DB3-NTNS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | / | 0.36 | 23.70 | / | 325 | 396 | 316 | |
| DB4-15-FS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 23.90 | 407 | 325 | 414 | 366 | |
| DB5-15-WS | H198 × 99 × 4.5 × 7 | 325 | 3.16 | 338 | 200 | 350 | 0.52 | 0.36 | 23.90 | 407 | 325 | 398 | 366 | |
| [11] | PPSRC1 | H500 × 200 × 9 × 14 | 317 | 3.37 | 650 | 450 | 650 | 0.16 | 0.84 | 24.30 | 393 | 317 | 2170 | 1898 |
| PPSRC2 | H500 × 200 × 9 × 14 | 317 | 3.37 | 975 | 450 | 650 | 0.16 | 0.84 | 24.30 | 393 | 317 | 1600 | 1388 | |
| [12] | B1-1.5 | I16 | 312 | 4.97 | 390 | 200 | 260 | 0.28 | 1.21 | 27.09 | 298 | 312 | 304 | 287 |
| B1-1.5p | I16 | 312 | 4.97 | 390 | 200 | 260 | 0.28 | 1.21 | 39.47 | 298 | 312 | 367 | 341 | |
| B2-1.0 | I16 | 312 | 4.97 | 260 | 200 | 260 | 0.28 | 1.21 | 34.23 | 298 | 312 | 495 | 437 | |
| B2-1.5 | I16 | 312 | 4.97 | 390 | 200 | 260 | 0.28 | 1.21 | 34.23 | 298 | 312 | 342 | 315 | |
| B2-2.0 | I16 | 312 | 4.97 | 520 | 200 | 260 | 0.28 | 1.21 | 34.23 | 298 | 312 | 255 | 261 | |
| B3-1.0 | I16 | 312 | 5.13 | 260 | 200 | 260 | 0.28 | 1.21 | 44.98 | 298 | 312 | 467 | 533 | |
| B3-1.5 | I16 | 312 | 5.13 | 390 | 200 | 260 | 0.28 | 1.21 | 44.98 | 298 | 312 | 373 | 383 | |
| B3-2.0 | I16 | 312 | 5.13 | 520 | 200 | 260 | 0.28 | 1.21 | 44.98 | 298 | 312 | 322 | 300 | |
| [13] | SRRAC-1 | I16 | 265 | 6.62 | 342 | 150 | 300 | 0.05 | 1.13 | 30.00 | 393 | 265 | 379 | 400 |
| SRRAC-2 | I16 | 265 | 6.62 | 342 | 150 | 300 | 0.05 | 1.13 | 26.40 | 393 | 265 | 366 | 382 | |
| SRRAC-3 | I16 | 265 | 6.62 | 228 | 150 | 300 | 0.05 | 1.13 | 27.60 | 393 | 265 | 499 | 510 | |
| SRRAC-4 | I16 | 265 | 6.62 | 342 | 150 | 300 | 0.05 | 1.13 | 27.60 | 393 | 265 | 371 | 388 | |
| SRRAC-5 | I14 | 265 | 6.62 | 456 | 150 | 300 | 0.05 | 1.13 | 27.60 | 393 | 265 | 280 | 338 | |
| SRRAC-6 | I16 | 283 | 5.78 | 342 | 150 | 300 | 0.05 | 1.13 | 27.60 | 393 | 283 | 339 | 378 | |
| [23] | SRRC1 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 34.31 | 339 | 327 | 319 | 305 |
| SRRC2 | I14 | 327 | 4.92 | 336 | 180 | 240 | 0.31 | 1.18 | 34.31 | 339 | 327 | 239 | 239 | |
| SRRC3 | I14 | 327 | 4.92 | 432 | 180 | 240 | 0.31 | 1.18 | 34.31 | 339 | 327 | 184 | 207 | |
| SRRC4 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 35.26 | 339 | 327 | 343 | 309 | |
| SRRC5 | I14 | 327 | 4.92 | 336 | 180 | 240 | 0.31 | 1.18 | 35.26 | 339 | 327 | 245 | 242 | |
| SRRC6 | I14 | 327 | 4.92 | 432 | 180 | 240 | 0.31 | 1.18 | 35.26 | 339 | 327 | 172 | 207 | |
| SRRC7 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 36.03 | 339 | 327 | 325 | 313 | |
| SRRC8 | I14 | 327 | 4.92 | 336 | 180 | 240 | 0.31 | 1.18 | 36.03 | 339 | 327 | 245 | 245 | |
| SRRC9 | I14 | 327 | 4.92 | 432 | 180 | 240 | 0.31 | 1.18 | 36.03 | 339 | 327 | 178 | 208 | |
| SRRC10 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 44.20 | 339 | 327 | 368 | 359 | |
| SRRC11 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 46.61 | 393 | 327 | 368 | 373 | |
| SRRC12 | I14 | 327 | 4.92 | 240 | 180 | 240 | 0.31 | 1.18 | 33.20 | 393 | 327 | 343 | 299 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Xue, Y.; Liu, Y.; Yu, Y. Compatible Truss-Arch Model for Predicting the Shear Strength of Steel Shape-Reinforced Concrete (SRC) Beams. Buildings 2023, 13, 1391. https://doi.org/10.3390/buildings13061391
Zhang X, Xue Y, Liu Y, Yu Y. Compatible Truss-Arch Model for Predicting the Shear Strength of Steel Shape-Reinforced Concrete (SRC) Beams. Buildings. 2023; 13(6):1391. https://doi.org/10.3390/buildings13061391
Chicago/Turabian StyleZhang, Xu, Yicong Xue, Yaping Liu, and Yunlong Yu. 2023. "Compatible Truss-Arch Model for Predicting the Shear Strength of Steel Shape-Reinforced Concrete (SRC) Beams" Buildings 13, no. 6: 1391. https://doi.org/10.3390/buildings13061391
APA StyleZhang, X., Xue, Y., Liu, Y., & Yu, Y. (2023). Compatible Truss-Arch Model for Predicting the Shear Strength of Steel Shape-Reinforced Concrete (SRC) Beams. Buildings, 13(6), 1391. https://doi.org/10.3390/buildings13061391






