Study on Failure Performance of the Thin-Walled Steel-Reinforced Concrete Pier under Low Cyclic Loading
Abstract
:1. Introduction
2. Experimental Procedure
2.1. Design of Specimens
2.2. The Mechanical Performance of the Used Materials
2.3. The Monitored Indicators and Layout of Sensors
2.3.1. Cracks Monitoring
2.3.2. Strain Measurement
2.3.3. Displacement Measurement
2.4. Test Setup
3. Analysis and Discussion of Experimental Results
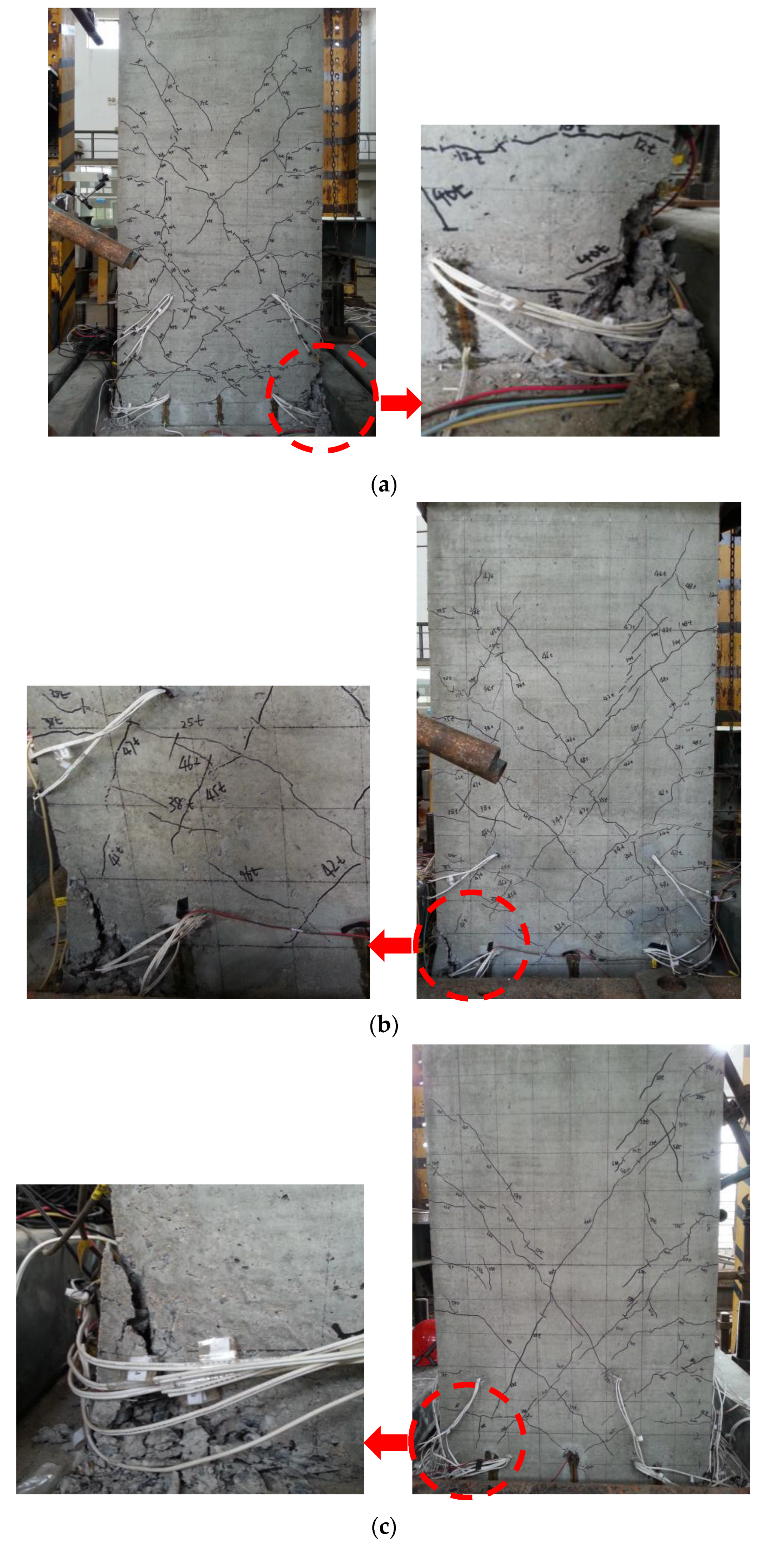
3.1. The Measured Load and Failure Mode
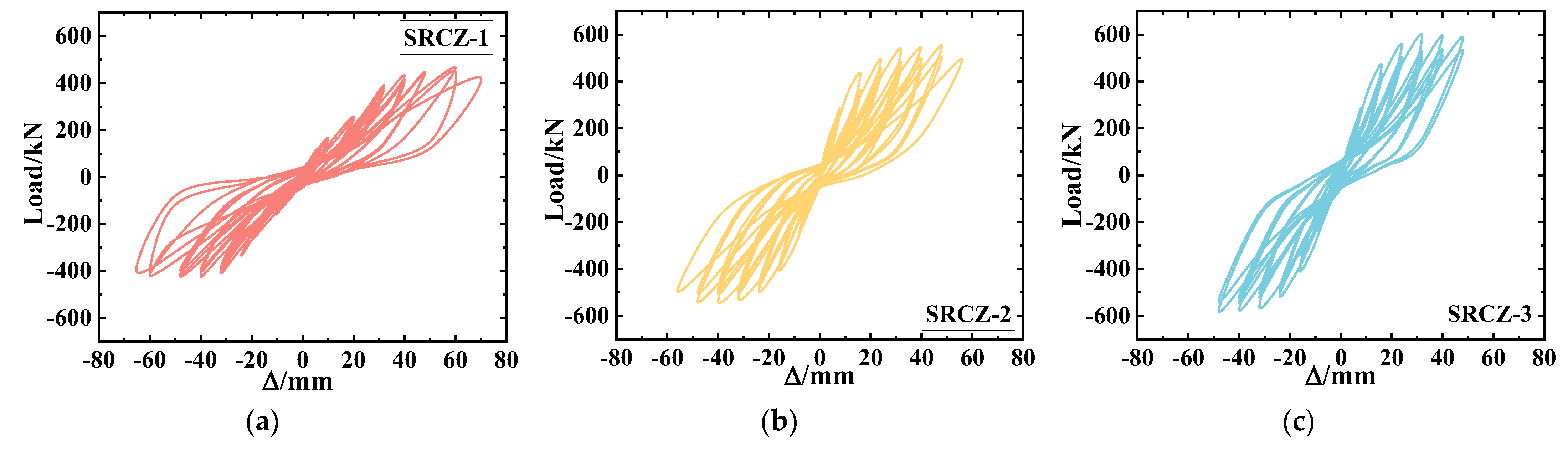
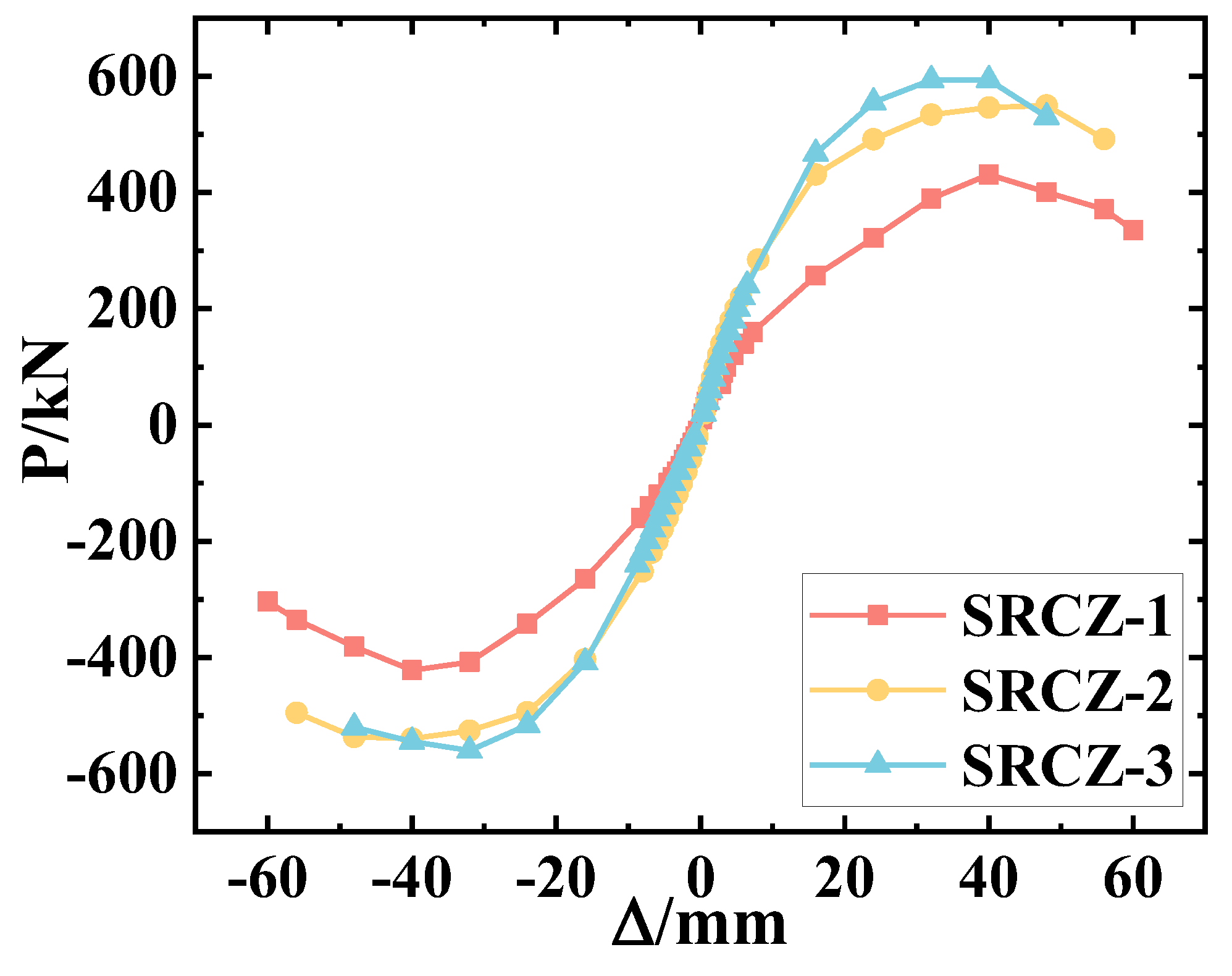
3.2. Load–Displacement Curves
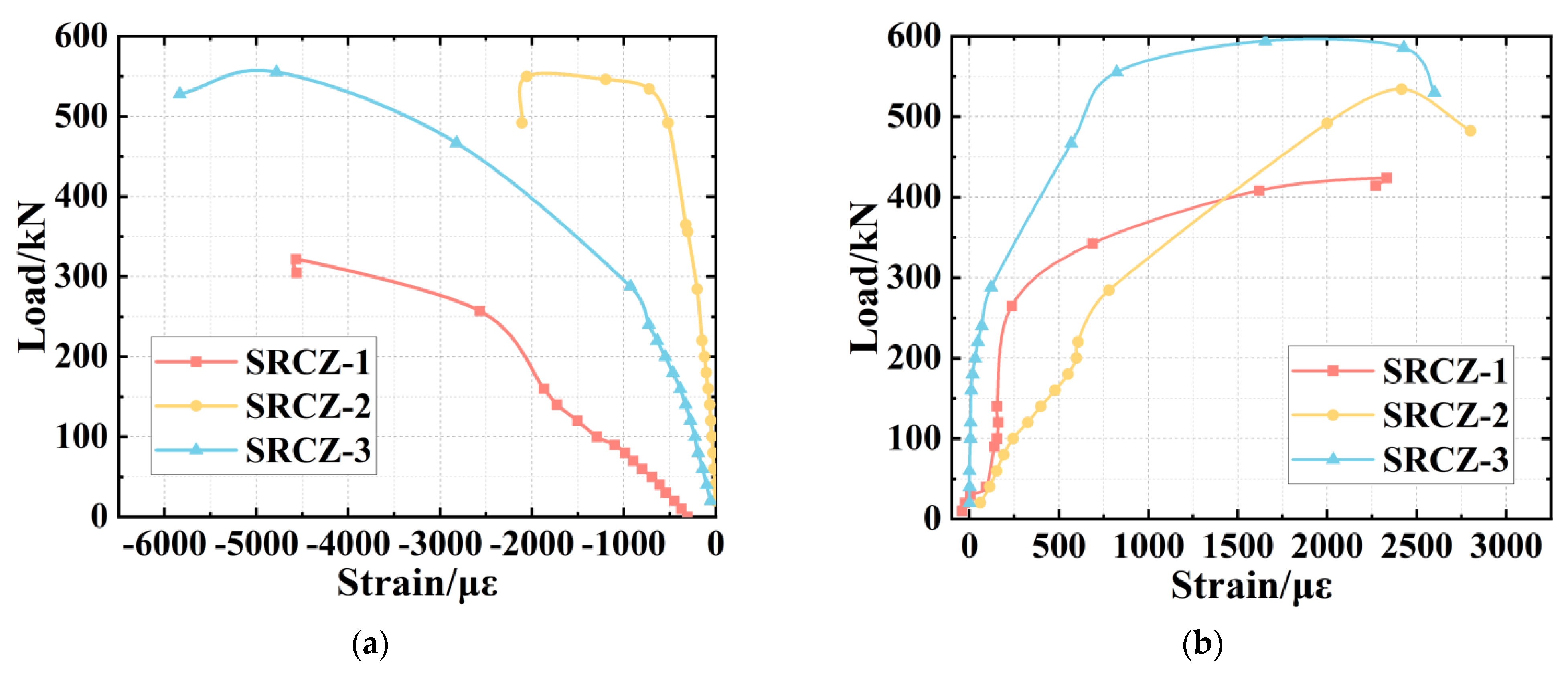
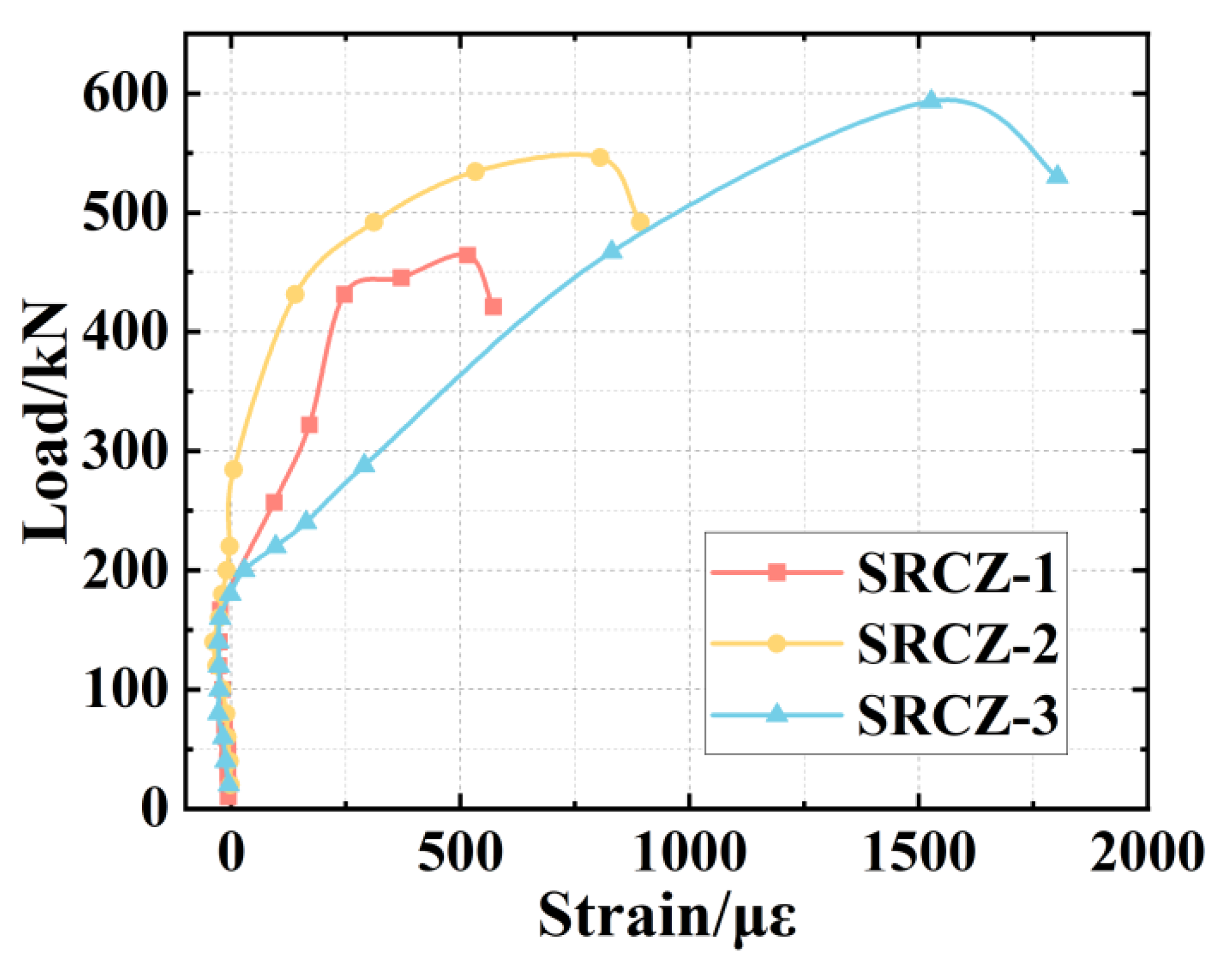
3.3. Load–Strain Curves
3.4. Ductility Results
4. Nonlinear Finite Element Analysis
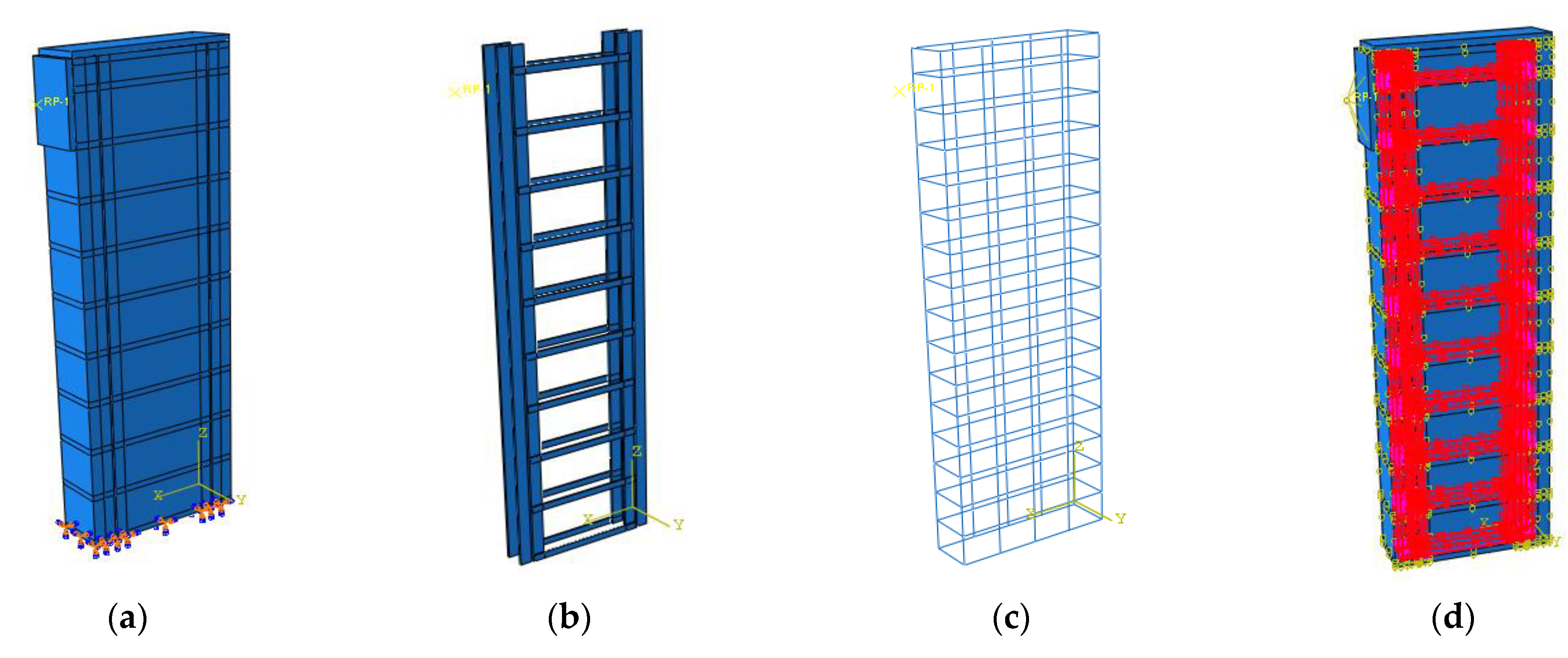
4.1. Finite Element Model of the Test Specimen
4.1.1. Material Parameter and Element Type
4.1.2. Geometric Model, Contact and Boundary Conditions
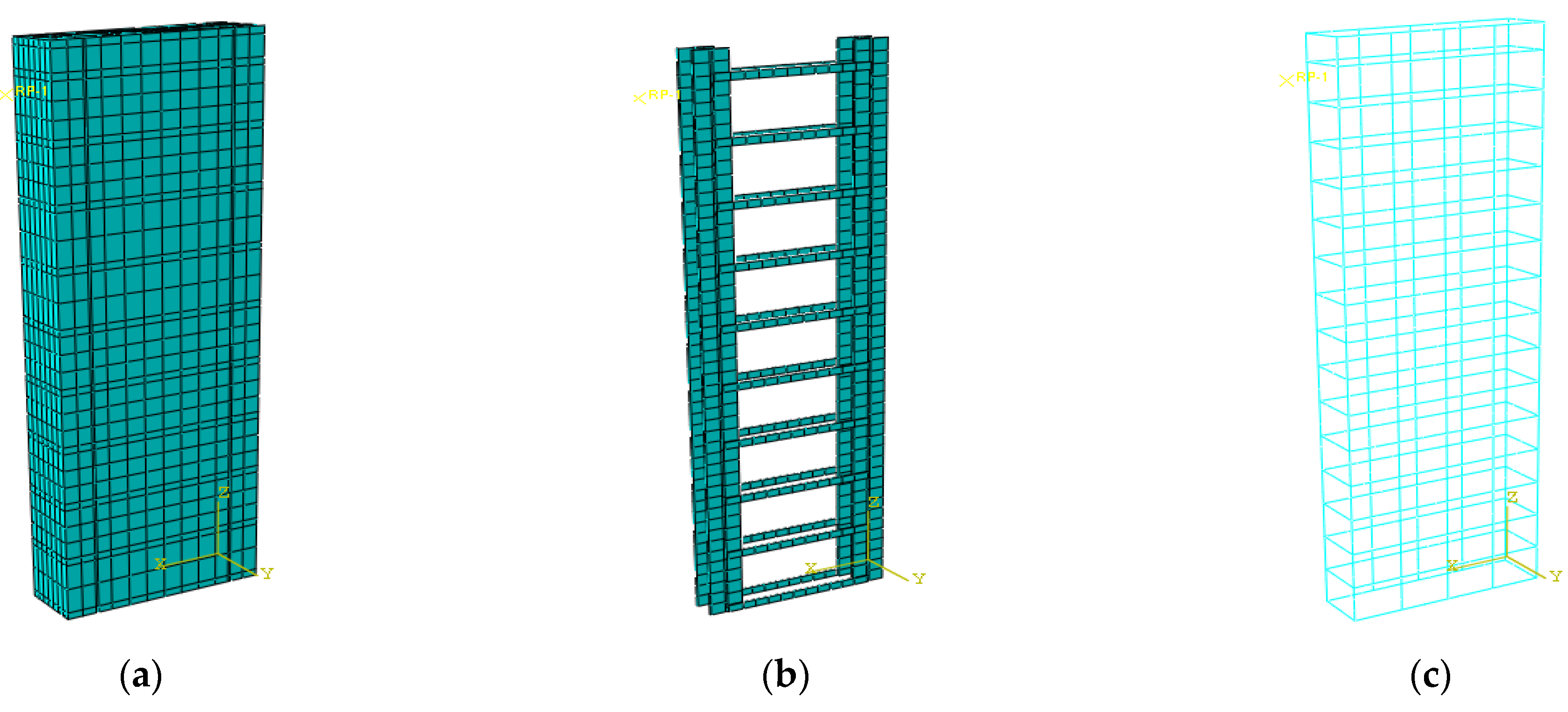
4.1.3. Meshing
4.2. The Comparative Study of Experimental and Analytical Results
4.2.1. The Ultimate Load
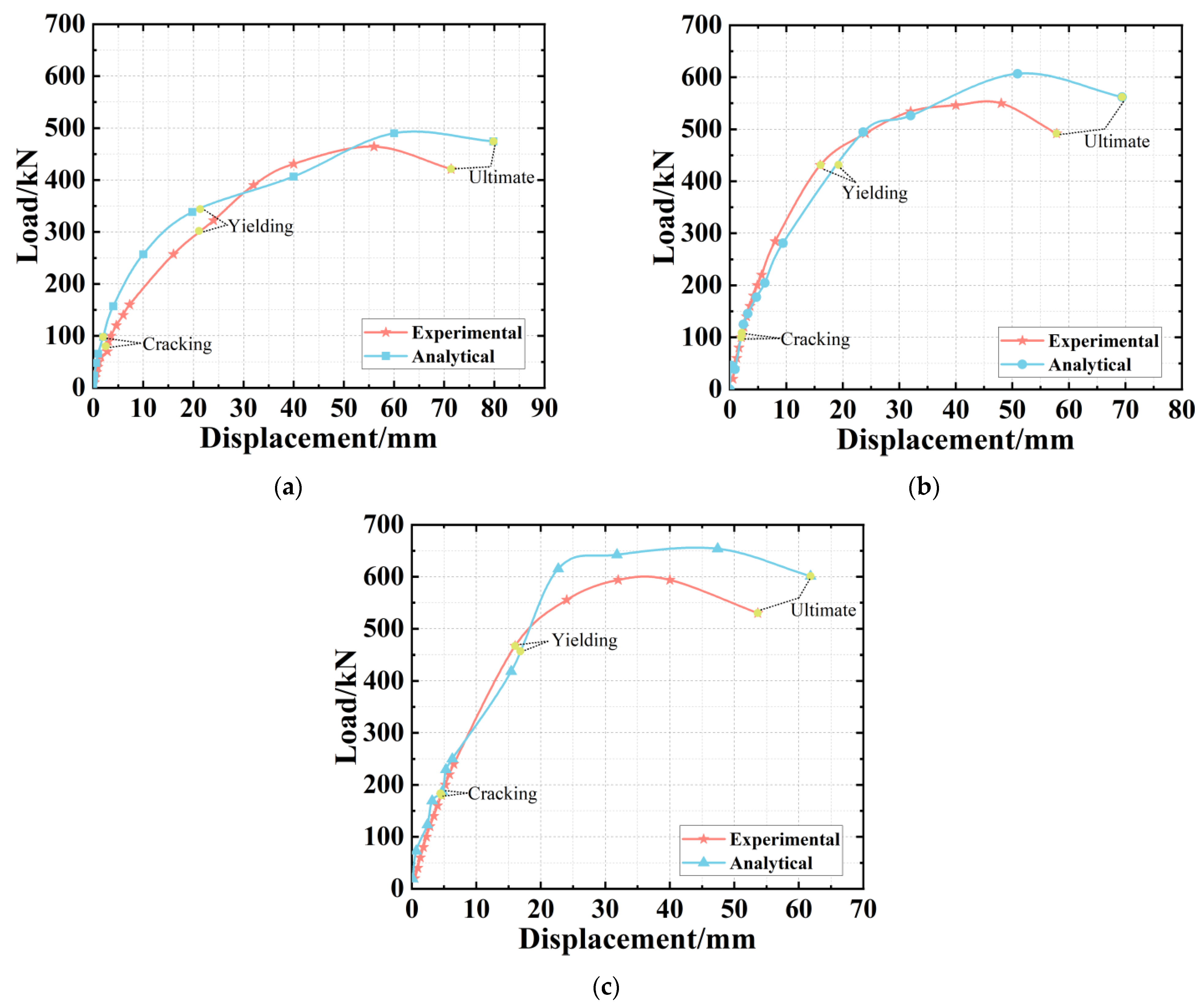
4.2.2. The Load–Displacement Curve
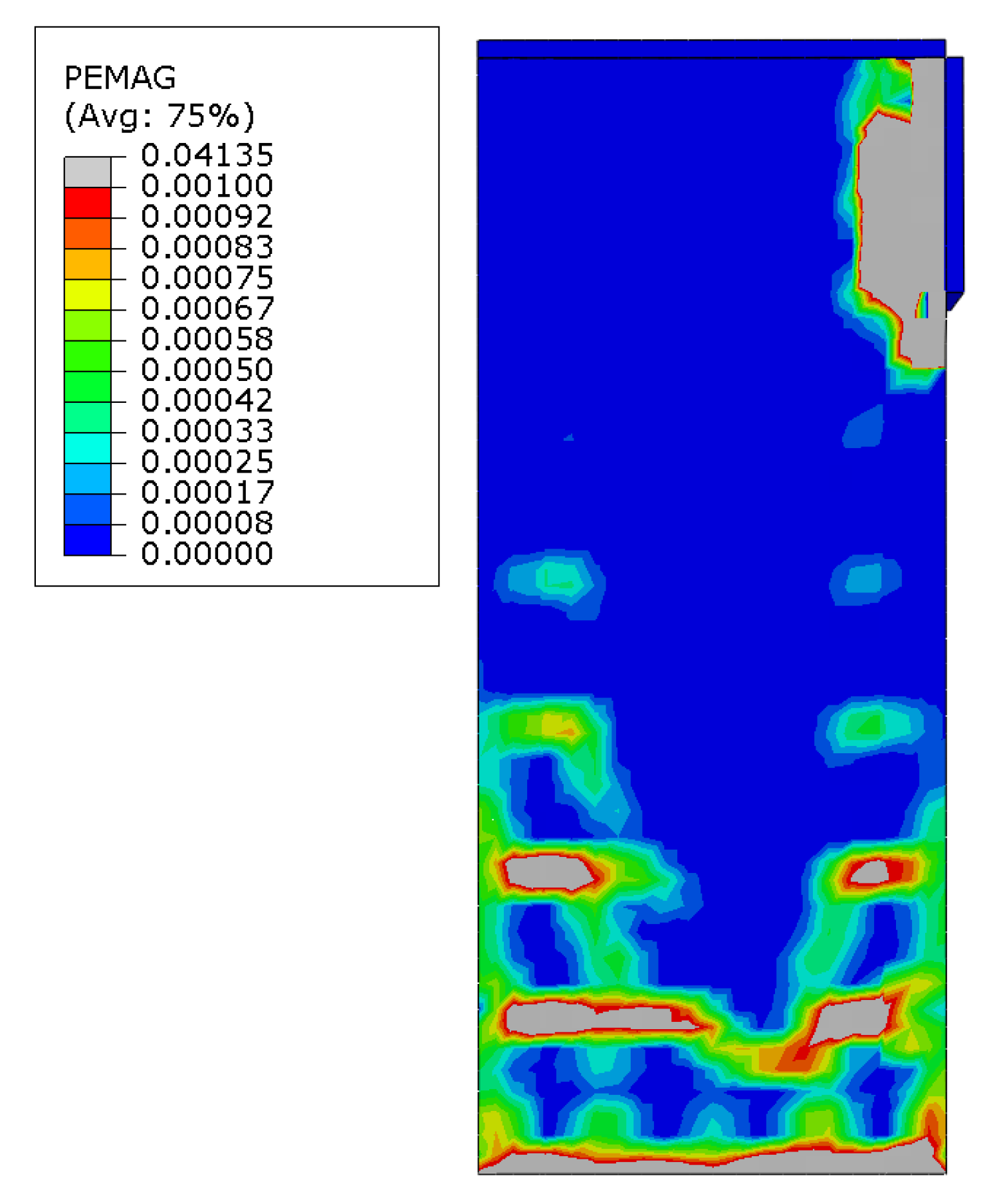
4.2.3. The Failure Mode and Crack Development
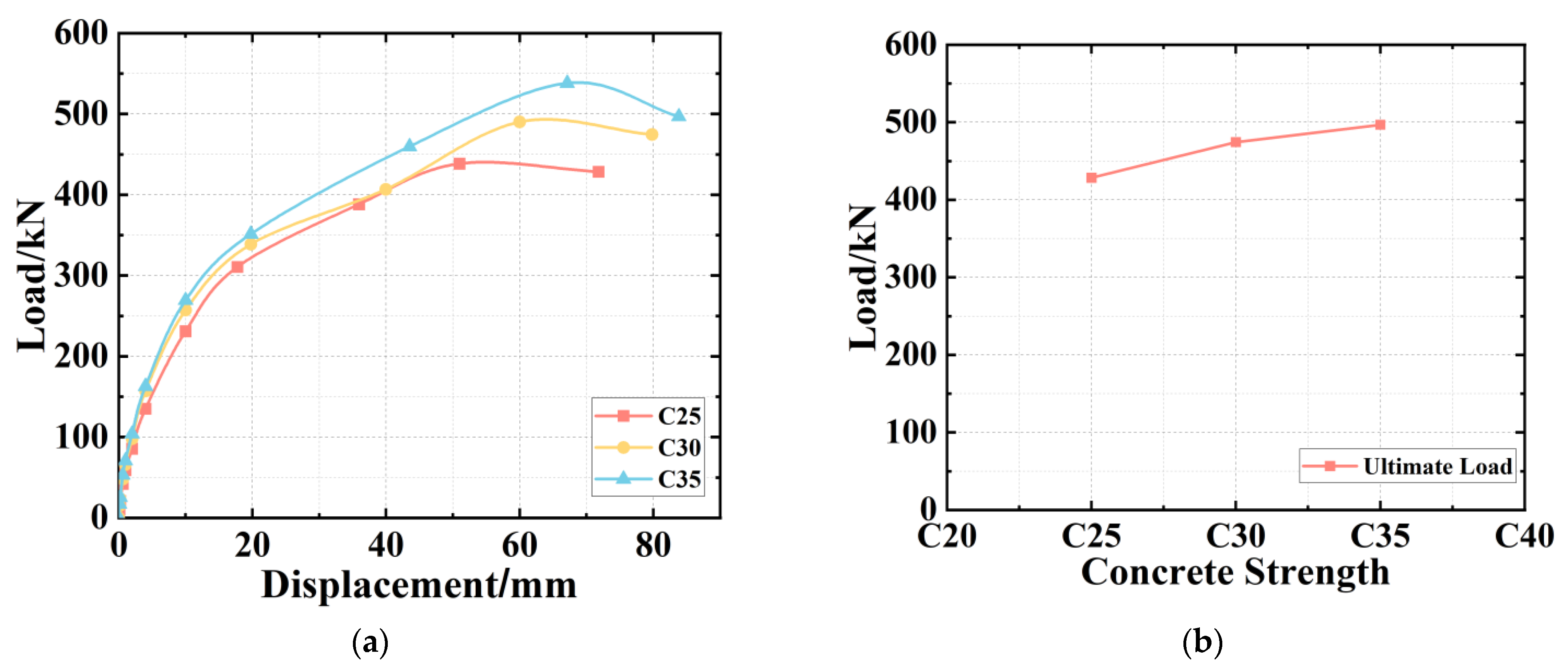
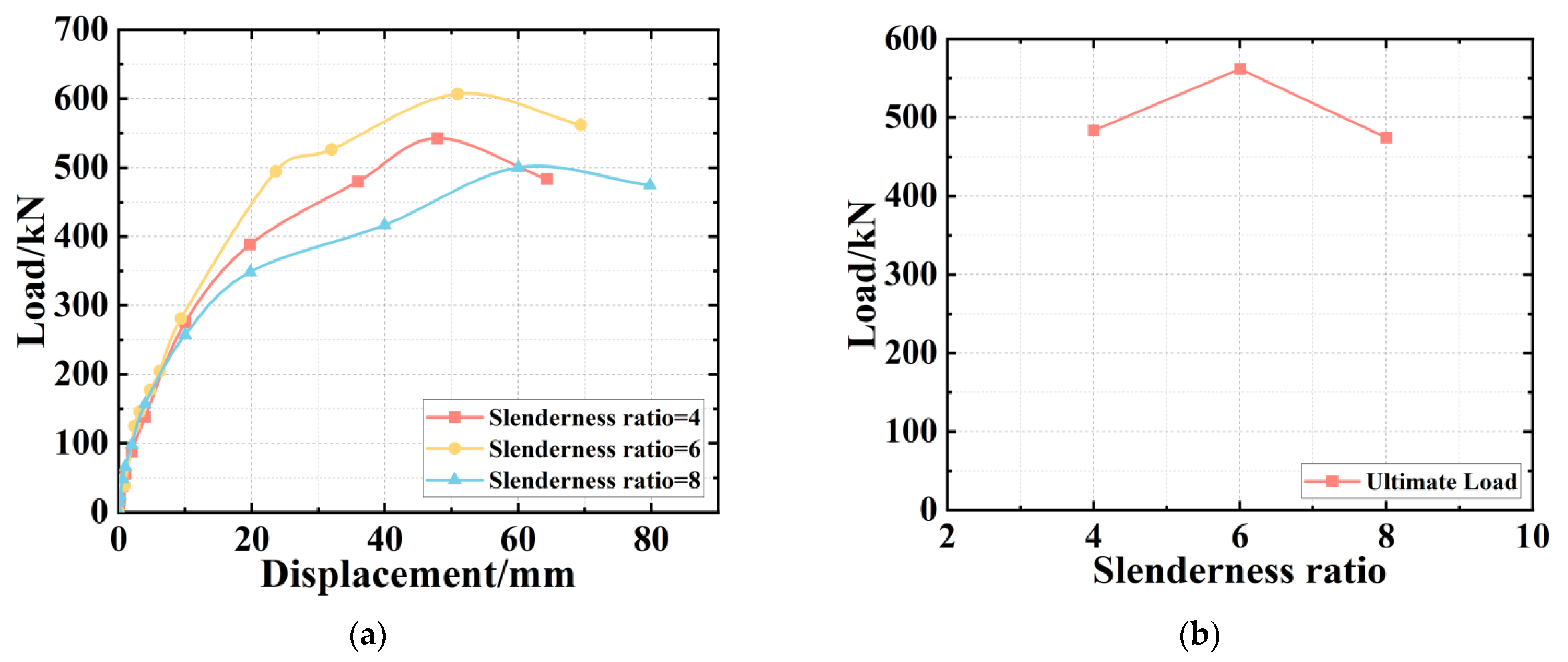
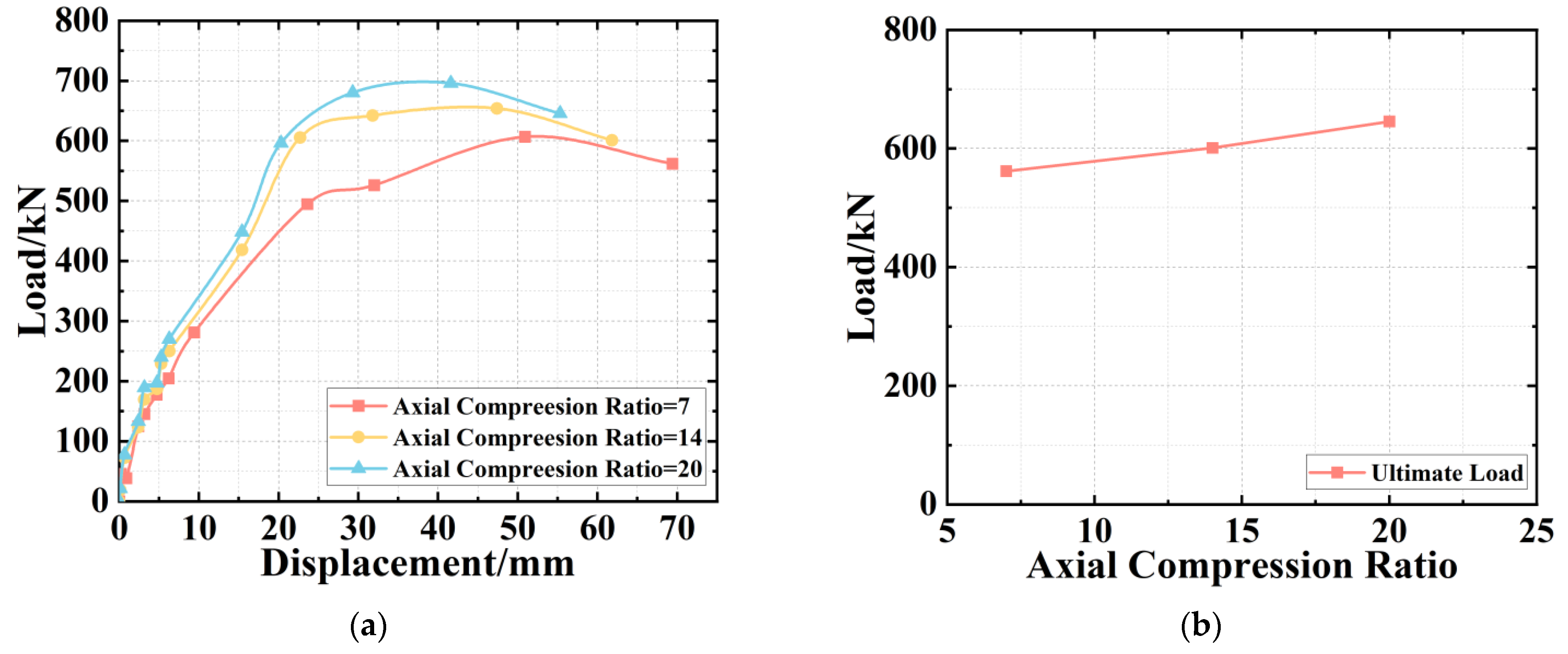
4.2.4. Extended Numerical Analysis on the Designed Parameters of a Thin-Walled Steel-Reinforced Concrete Pier
- (1)
- The Concrete Strength
- (2)
- The DWR
- (3)
- The ACR
5. Discussion on the Effects of ACR and DWR on the Failure Performance
5.1. The Effects of ACR
5.2. The Effects of DWR
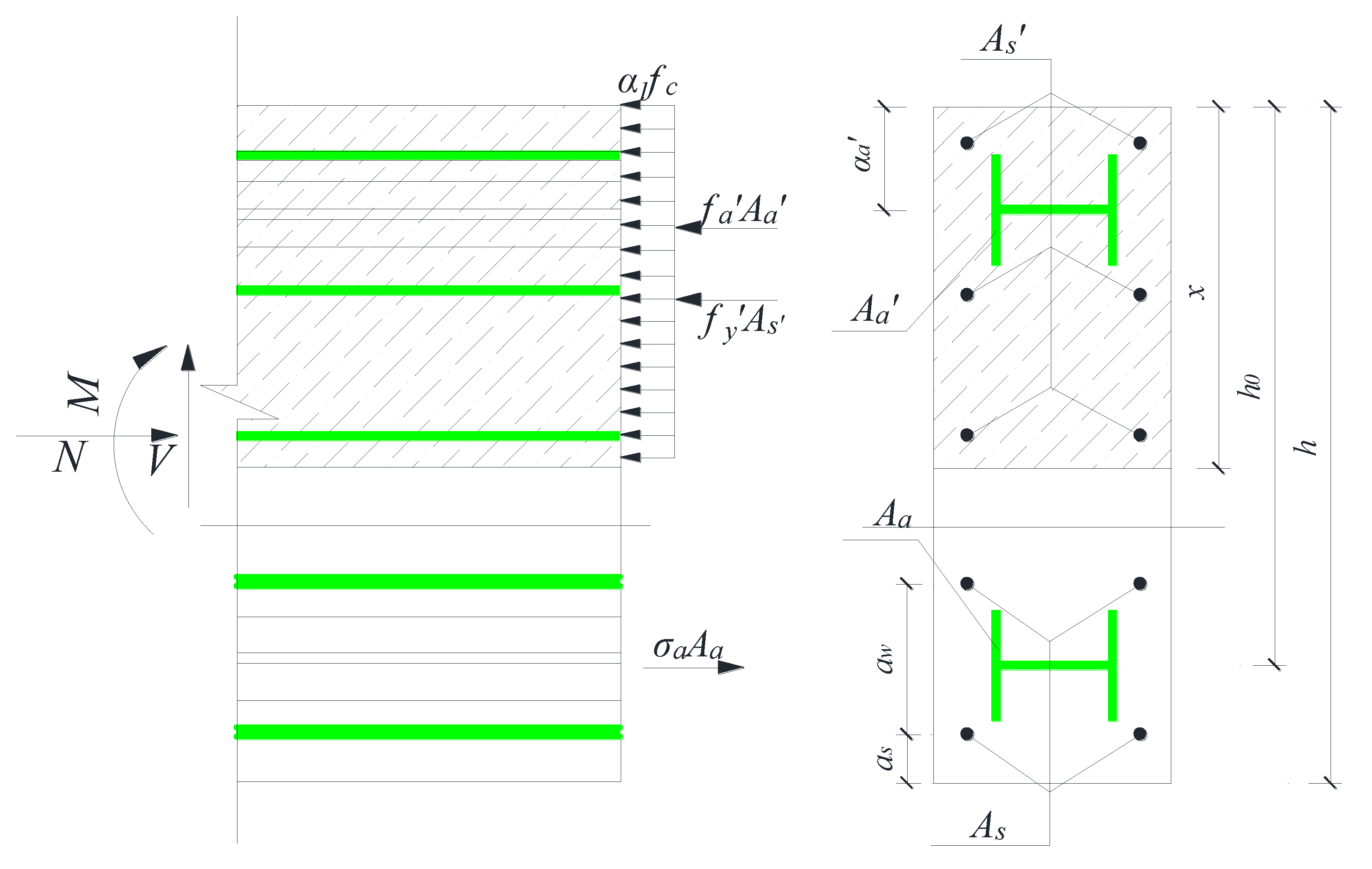
6. Horizontal Ultimate Bearing Capacity Equation for Thin-Walled Steel-Reinforced Concrete Pier
7. Conclusions and Outlooks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yuan, X.J.; Liu, F. Comparing Hysteretic Behavior of Flexible Piers with Different Stirrup Ratio and Slenderness Ratio. Adv. Mater. Res. 2011, 261–263, 576–580. [Google Scholar] [CrossRef]
- Pinto, A.V.; Molina, J.; Tsionis, G. Cyclic tests on large-scale models of existing bridge piers with rectangular hollow cross-section. Earthq. Eng. Struct. Dyn. 2003, 32, 1995–2012. [Google Scholar] [CrossRef]
- Lehman, D.; Moehle, J.; Mahin, S.; Calderone, A.; Henry, L. Experimental Evaluation of the Seismic Performance of Reinforced Concrete Bridge Columns. J. Struct. Eng. 2004, 130, 869–879. [Google Scholar] [CrossRef]
- Zhang, Q.; Wei, Z.Y.; Gu, X.L. Confinement behavior and stress–strain response of square concrete columns strengthened with carbon textile reinforced concrete (CTRC) composites. Eng. Struct. 2022, 266, 114592. [Google Scholar] [CrossRef]
- Zhang, Q.; Zheng, N.H.; Gu, X.L. Study of the confinement performance and stress-strain response of RC columns with corroded stirrups. Eng. Struct. 2022, 266, 114476. [Google Scholar] [CrossRef]
- Yeh, Y.K.; Mo, Y.L.; Yang, C.Y. Seismic performance of hollow circular bridge piers. Struct. J. 2001, 98, 862–871. [Google Scholar]
- Yeh, Y.-K.; Mo, Y.L.; Yang, C.Y. Seismic Performance of Rectangular Hollow Bridge Columns. J. Struct. Eng. 2002, 128, 60–68. [Google Scholar] [CrossRef]
- Mo, Y.L.; Nien, I.C. Seismic Performance of Hollow High-Strength Concrete Bridge Columns. J. Bridge Eng. 2002, 7, 338–349. [Google Scholar] [CrossRef]
- Mo, Y.L.; Yeh, Y.-K.; Hsieh, D.M. Seismic Retrofit of Hollow Rectangular Bridge Columns. J. Compos. Constr. 2004, 8, 43–51. [Google Scholar] [CrossRef]
- Mo, Y.L.; Wong, D.C.; Maekawa, K. Seismic performance of hollow bridge columns. Struct. J. 2003, 100, 337–348. [Google Scholar]
- Tirasit, P.; Kawashima, K. Seismic Performance of Square Reinforced Concrete Columns under Combined Cyclic Flexural and Torsional Loadings. J. Earthq. Eng. 2007, 11, 425–452. [Google Scholar] [CrossRef]
- Chang, S.-Y. Experimental Studies of Reinforced Concrete Bridge Columns under Axial Load Plus Biaxial Bending. J. Struct. Eng. 2010, 136, 12–25. [Google Scholar] [CrossRef]
- Zhang, Q.; Gong, J.-X.; Zhang, Y.-Q. Lateral-Load Behavior Prediction and Pushover Analysis of Reinforced Concrete Columns Including Shear Effects. Adv. Struct. Eng. 2013, 16, 741–758. [Google Scholar] [CrossRef]
- Zhao, Y.; Jiang, H.Y.; Gu, J.; Wang, R.Q. Seismic Performance of Reinforced Concrete Rectangular Hollow Bridge Piers. Adv. Mater. Res. 2013, 859, 95–99. [Google Scholar] [CrossRef]
- Cassese, P.; Ricci, P.; Verderame, G.M. Experimental study on the seismic performance of existing reinforced concrete bridge piers with hollow rectangular section. Eng. Struct. 2017, 144, 88–106. [Google Scholar] [CrossRef]
- Su, J.; Wang, J.; Li, Z.; Liang, X. Effect of reinforcement grade and concrete strength on seismic performance of reinforced concrete bridge piers. Eng. Struct. 2019, 198, 109512. [Google Scholar] [CrossRef]
- Roderick, J.W.; Rogers, D.F. Load Carrying Capacity of Simple Composite Columns. J. Strl. Div. 1969, 95, 209–228. [Google Scholar] [CrossRef]
- Bridge, R.Q. Design Of Composite Columns–Steel, Concrete or Composite Approach? Compos. Construct. Steel Concr. 2011, 6, 276–290. [Google Scholar]
- Johansson, M.; Gylltoft, K. Mechanical Behavior of Circular Steel–Concrete Composite Stub Columns. J. Struct. Eng. 2002, 128, 1073–1081. [Google Scholar] [CrossRef]
- Zhou, X.; Liu, J. Seismic behavior and strength of tubed steel reinforced concrete (SRC) short columns. J. Constr. Steel Res. 2010, 66, 885–896. [Google Scholar] [CrossRef]
- Gan, D.; Guo, L.; Liu, J.; Zhou, X. Seismic behavior and moment strength of tubed steel reinforced-concrete (SRC) beam-columns. J. Constr. Steel Res. 2011, 67, 1516–1524. [Google Scholar] [CrossRef]
- Chang, X.; Wei, Y.-Y.; Yun, Y.-C. Analysis of steel-reinforced concrete-filled-steel tubular (SRCFST) columns under cyclic loading. Constr. Build. Mater. 2012, 28, 88–95. [Google Scholar] [CrossRef]
- Nie, J.-G.; Wang, Y.-H.; Fan, J.-S. Experimental research on concrete filled steel tube columns under combined compression-bending-torsion cyclic load. Thin. Wall. Struct. 2013, 67, 1–14. [Google Scholar] [CrossRef]
- Naito, H.; Akiyama, M.; Suzuki, M. Ductility Evaluation of Concrete-Encased Steel Bridge Piers Subjected to Lateral Cyclic Loading. J. Bridge. Eng. 2011, 16, 72–81. [Google Scholar] [CrossRef]
- Zhang, S.A.; Zhao, Z.Z.; He, X.G. Flexural Behavior of SRC Columns under Axial and Bilateral Loading. Appl. Mech. Mater. 2012, 166, 3383–3390. [Google Scholar] [CrossRef]
- Zhu, W.; Jia, J.; Gao, J.; Zhang, F. Experimental study on steel reinforced high-strength concrete columns under cyclic lateral force and constant axial load. Eng. Struct. 2016, 125, 191–204. [Google Scholar] [CrossRef]
- Yin, F.; Wang, R.-W.; Cao, W.-L.; Zhao, Y.; Song, Y. Experimental and analytical research on steel reinforced high-strength concrete columns with different steel sections. Structures 2021, 34, 4350–4363. [Google Scholar] [CrossRef]
- Cao, X.; Wu, L.; Li, Z. Behaviour of steel-reinforced concrete columns under combined torsion based on ABAQUS FEA. Eng. Struct. 2020, 209, 109980. [Google Scholar] [CrossRef]
- Yang, B.S.; Song, S.H. Study on the Factors Affecting the Anti-Seismic Performance of Steel Reinforced High-Strengh Concrete Columns. Adv. Mater. Res. 2012, 535–537, 1842–1846. [Google Scholar] [CrossRef]
- Fang, L.; Zhang, B.; Jin, G.-F.; Li, K.-W.; Wang, Z.-L. Seismic behavior of concrete-encased steel cross-shaped columns. J. Constr. Steel Res. 2015, 109, 24–33. [Google Scholar] [CrossRef]
- Wang, Q.; Shi, Q.; Tao, Y. Experimental and numerical studies on the seismic behavior of steel reinforced concrete compression-bending members with new-type section steel. Adv. Struct. Eng. 2016, 19, 255–269. [Google Scholar] [CrossRef]
- Yang, Y.; Xue, Y.; Yu, Y.; Gao, F. Experimental study on seismic performance of partially precast steel reinforced concrete columns. Eng. Struct. 2018, 175, 63–75. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, C.; Chang, H.; Xue, Y. Experimental study on seismic performance of steel reinforced high-strength concrete composite columns. IOP Conf. Ser. Earth Environ. Sci. 2021, 676, 012065. [Google Scholar] [CrossRef]
- Xu, W.; Ma, B.; Duan, X.; Li, J. Experimental investigation of seismic behavior of UHPC connection between precast columns and footings in bridges. Eng. Struct. 2021, 239, 112344. [Google Scholar] [CrossRef]
- Wang, J.; Shen, Y.; Li, G.; Chen, M.; Fan, C. Quasi-Full-Scale Experimental Study on Bridge Precast Concrete Columns under Static Loading. J. Bridge Eng. 2021, 26, 04021031. [Google Scholar] [CrossRef]

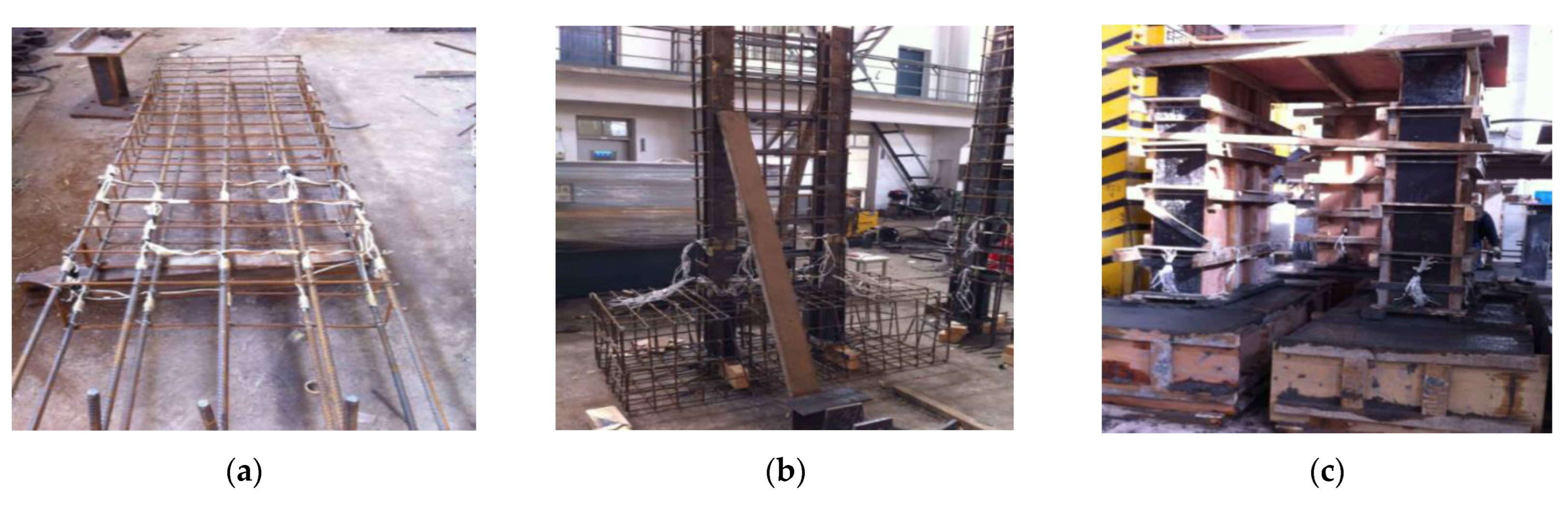
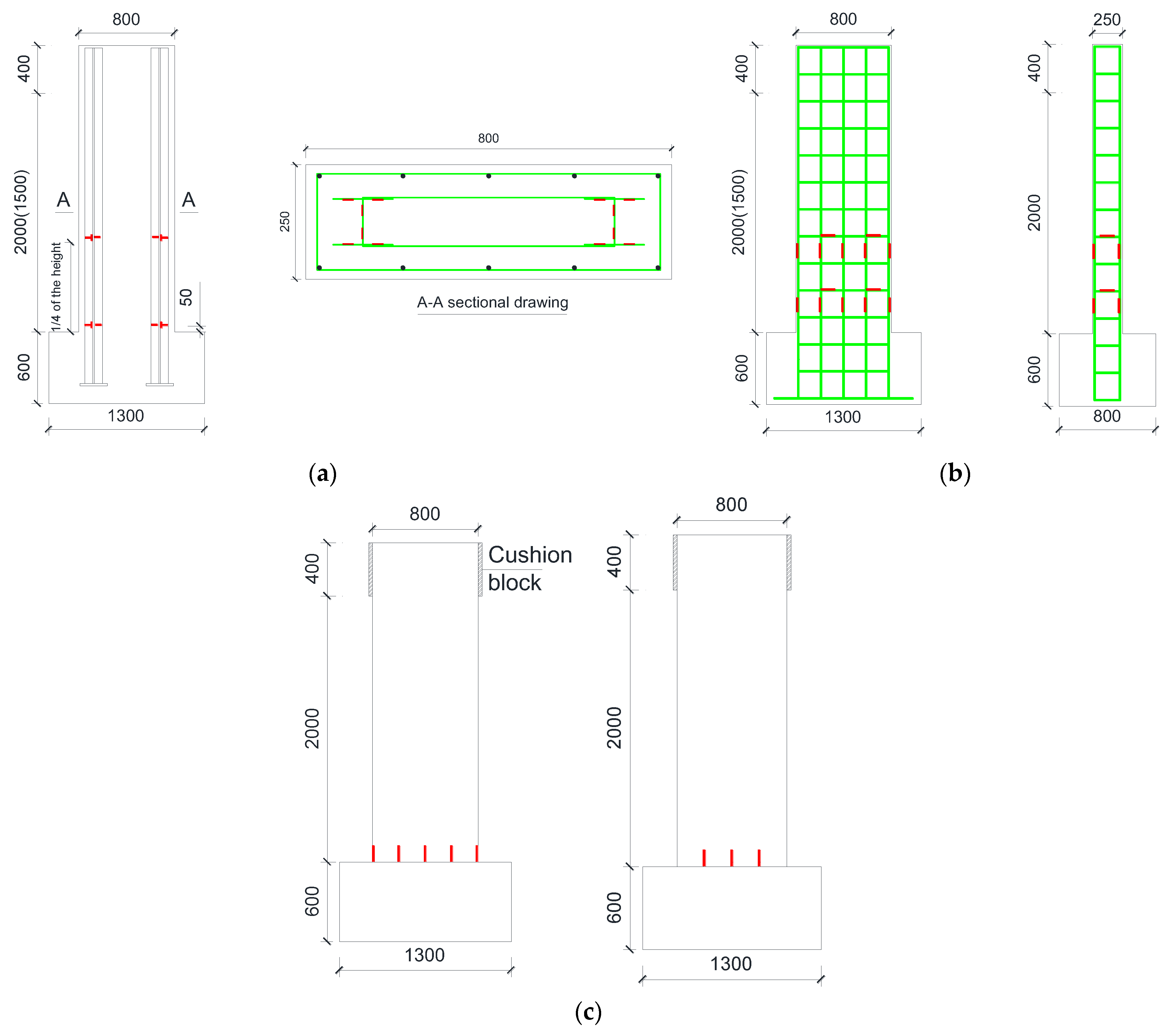
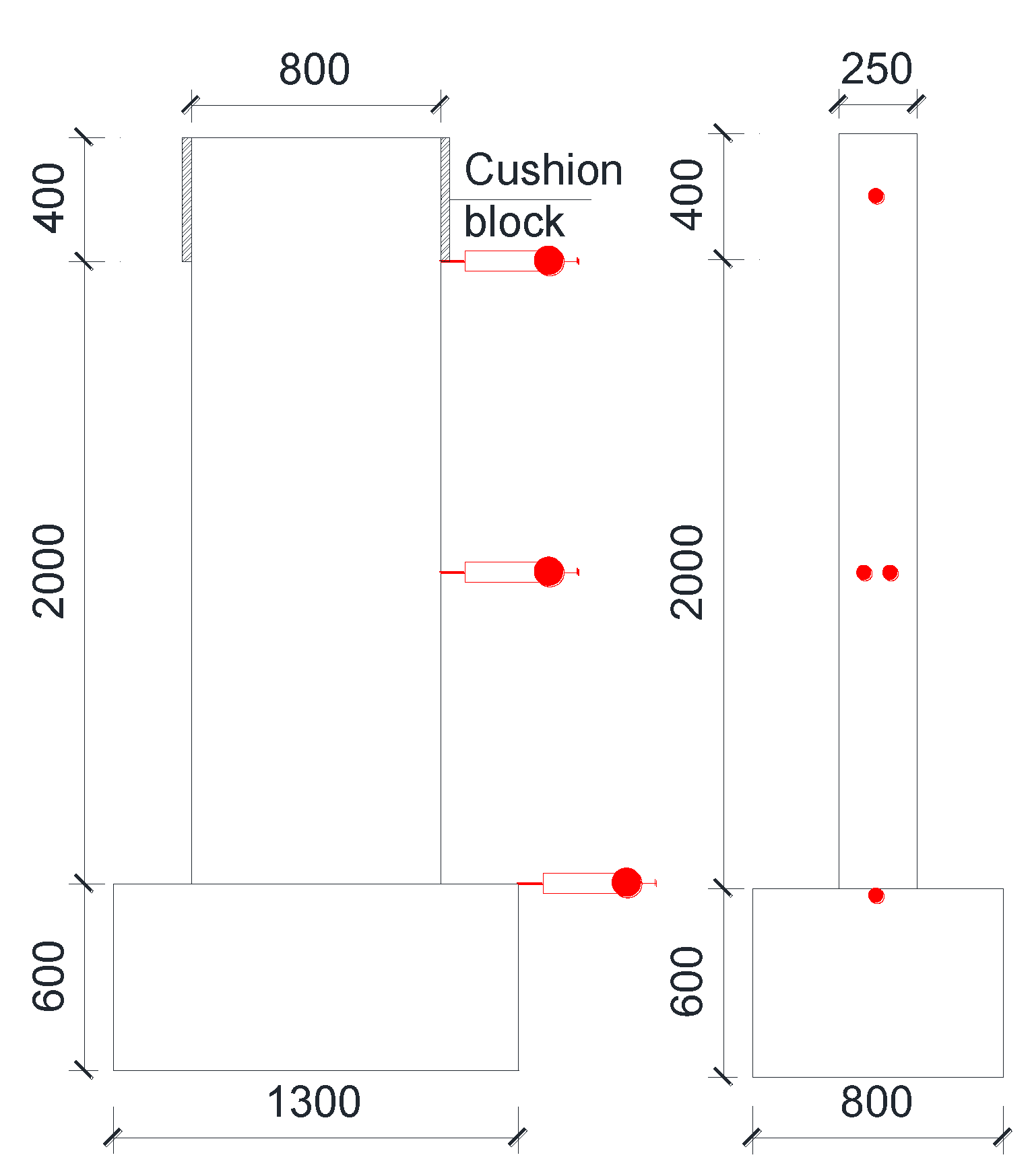


| Specimen No. | Length/mm | ACR/% | Steel Ratio/% | DWR |
|---|---|---|---|---|
| SRCZ-1 | 2000 | 7 | 3.2 | 8 |
| SRCZ-2 | 1500 | 7 | 3.2 | 6 |
| SRCZ-3 | 1500 | 14 | 3.2 | 6 |
| Strength/MPa | Yielding Strength | Ultimate Strength | Yielding Strain | Elastic Modulus | |
|---|---|---|---|---|---|
| Item | |||||
| Steel | Flange | 303.6 | 442.5 | 1430 × 10−6 | 2.35 × 105 |
| Web | 296.2 | 427.8 | 1380 × 10−6 | 2.23 × 105 | |
| Longitudinal reinforcement | 417.4 | 593.7 | 1530 × 10−6 | 2.01 × 105 | |
| Hooped reinforcement | 409.2 | 586.6 | 1360 × 10−6 | 2.03 × 105 | |
| Concrete | Specimen No. | SRCZ-1 | SRCZ-2 | SRCZ-3 | |
| Compressive Strength/MPa | 33.27 | 33.66 | 33.42 | ||
| Specimen No. | DWR | ACR/% | Load/kN | Displacement/mm | Failure Mode | ||||
|---|---|---|---|---|---|---|---|---|---|
| SRCZ-1 | 8 | 7 | 80 | 321.93 | 421.03 | 2.51 | 21.08 | 71.36 | bending-failure |
| SRCZ-2 | 6 | 7 | 100 | 431.06 | 491.85 | 2.02 | 17.06 | 57.82 | bending-failure |
| SRCZ-3 | 6 | 14 | 180 | 466.91 | 530.02 | 4.61 | 13.74 | 53.63 | bending-failure |
| Specimen No. | ACR/% | DDC | DWR | YDDR | UDDR |
|---|---|---|---|---|---|
| SRCZ-1 | 7 | 3.39 | 8 | 0.0105 | 0.0357 |
| SRCZ-2 | 7 | 3.97 | 6 | 0.0114 | 0.0452 |
| SRCZ-3 | 14 | 3.90 | 6 | 0.0092 | 0.0357 |
| Average value | 0.01 | 0.039 |
| Specimen No. | DWR | ACR/% | Ultimate Load | Analytical/Experimental | Ultimate Displacement | Analytical/Experimental | ||
|---|---|---|---|---|---|---|---|---|
| Analytical | Experimental | Analytical | Experimental | |||||
| SRCZ-1 | 8 | 7 | 474.30 | 421.03 | 1.13 | 79.80 | 71.36 | 1.12 |
| SRCZ-2 | 6 | 7 | 561.85 | 491.85 | 1.14 | 69.40 | 57.82 | 1.20 |
| SRCZ-3 | 6 | 14 | 601.02 | 530.02 | 1.14 | 61.81 | 53.63 | 1.15 |
| Specimen No. | Shear-to-Span Ratio | ACR | |||
|---|---|---|---|---|---|
| Ref. [28]-1 | 2 | 0.3 | 117 | 101.9 | 1.15 |
| Ref. [28]-2 | 2 | 0.5 | 124 | 109.5 | 1.13 |
| Ref. [28]-3 | 2 | 0.4 | 116 | 105.8 | 1.10 |
| Average | 1.13 | ||||
| Mean Square Error | 0.021 |
| Specimen No. | DWR | ACR | /kN | /kN | /kN | ||
|---|---|---|---|---|---|---|---|
| SRCZ-1 | 8 | 7 | 421.03 | 443.31 | 474.30 | 0.95 | 1.07 |
| SRCZ-2 | 6 | 7 | 491.85 | 468.69 | 561.85 | 1.05 | 1.20 |
| SRCZ-3 | 6 | 14 | 530.02 | 480.69 | 601.02 | 1.10 | 1.25 |
| Average Mean Square Error | 1.03 | 1.17 | |||||
| 0.062 | 0.076 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Xu, B.; Liu, Q.; Gu, J. Study on Failure Performance of the Thin-Walled Steel-Reinforced Concrete Pier under Low Cyclic Loading. Buildings 2022, 12, 1412. https://doi.org/10.3390/buildings12091412
Chen H, Xu B, Liu Q, Gu J. Study on Failure Performance of the Thin-Walled Steel-Reinforced Concrete Pier under Low Cyclic Loading. Buildings. 2022; 12(9):1412. https://doi.org/10.3390/buildings12091412
Chicago/Turabian StyleChen, Huihui, Bing Xu, Qin Liu, and Jianfeng Gu. 2022. "Study on Failure Performance of the Thin-Walled Steel-Reinforced Concrete Pier under Low Cyclic Loading" Buildings 12, no. 9: 1412. https://doi.org/10.3390/buildings12091412
APA StyleChen, H., Xu, B., Liu, Q., & Gu, J. (2022). Study on Failure Performance of the Thin-Walled Steel-Reinforced Concrete Pier under Low Cyclic Loading. Buildings, 12(9), 1412. https://doi.org/10.3390/buildings12091412









