Evaluating Timoshenko Method for Analyzing CLT under Out-of-Plane Loading
Abstract
:1. Introduction
2. Analytical Models for Deflection Calculation in CLT Panels
2.1. Timoshenko Method
2.2. Shear Form Factor
3. Finite Element Modelling
3.1. Material Model
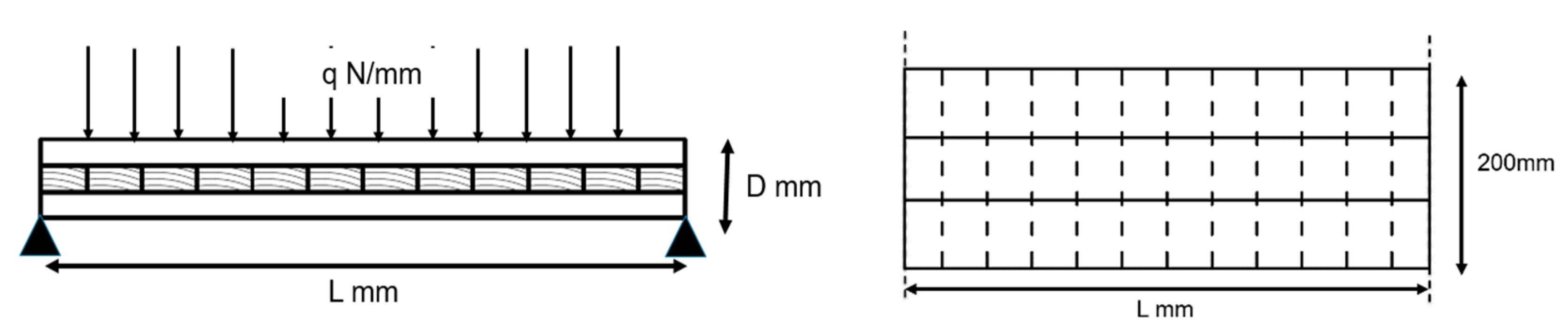
3.2. Geometry and Boundary Conditions
3.3. Material Properties
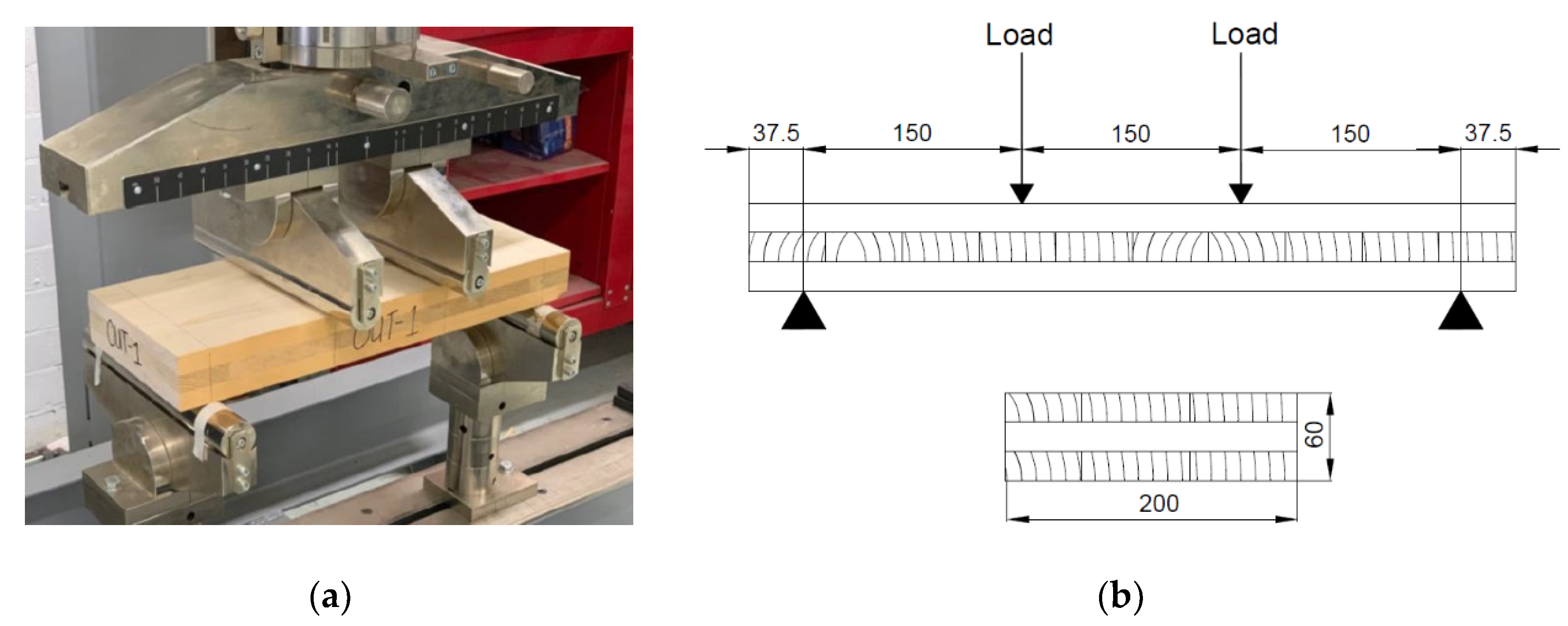
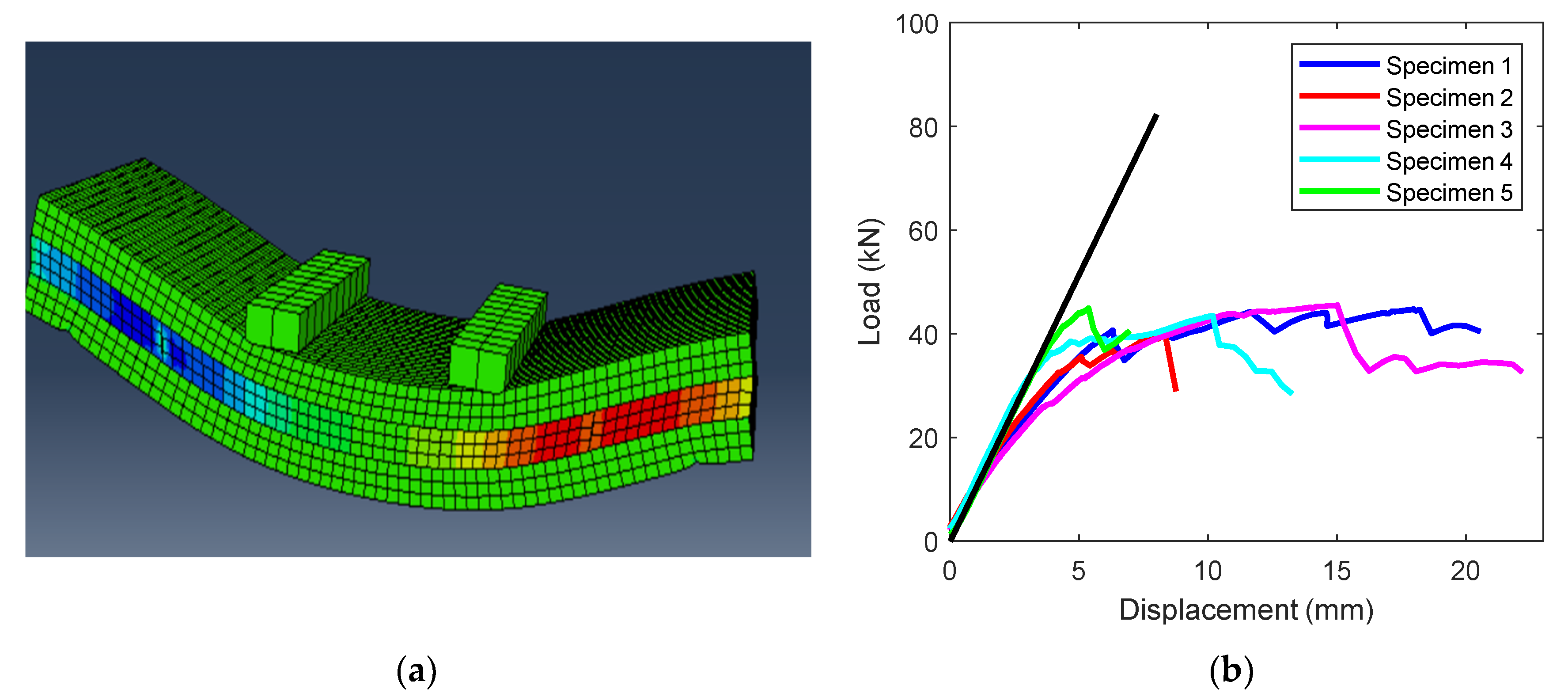
3.4. Verification of the Numerical Modelling Technique
3.5. Numerical Modelling of Glue Laminated Timber (GLT)
4. Reassessing the Suitability of Timoshenko Method for CLT Panels
4.1. Comparison between Timoshenko Method and CLT Panels
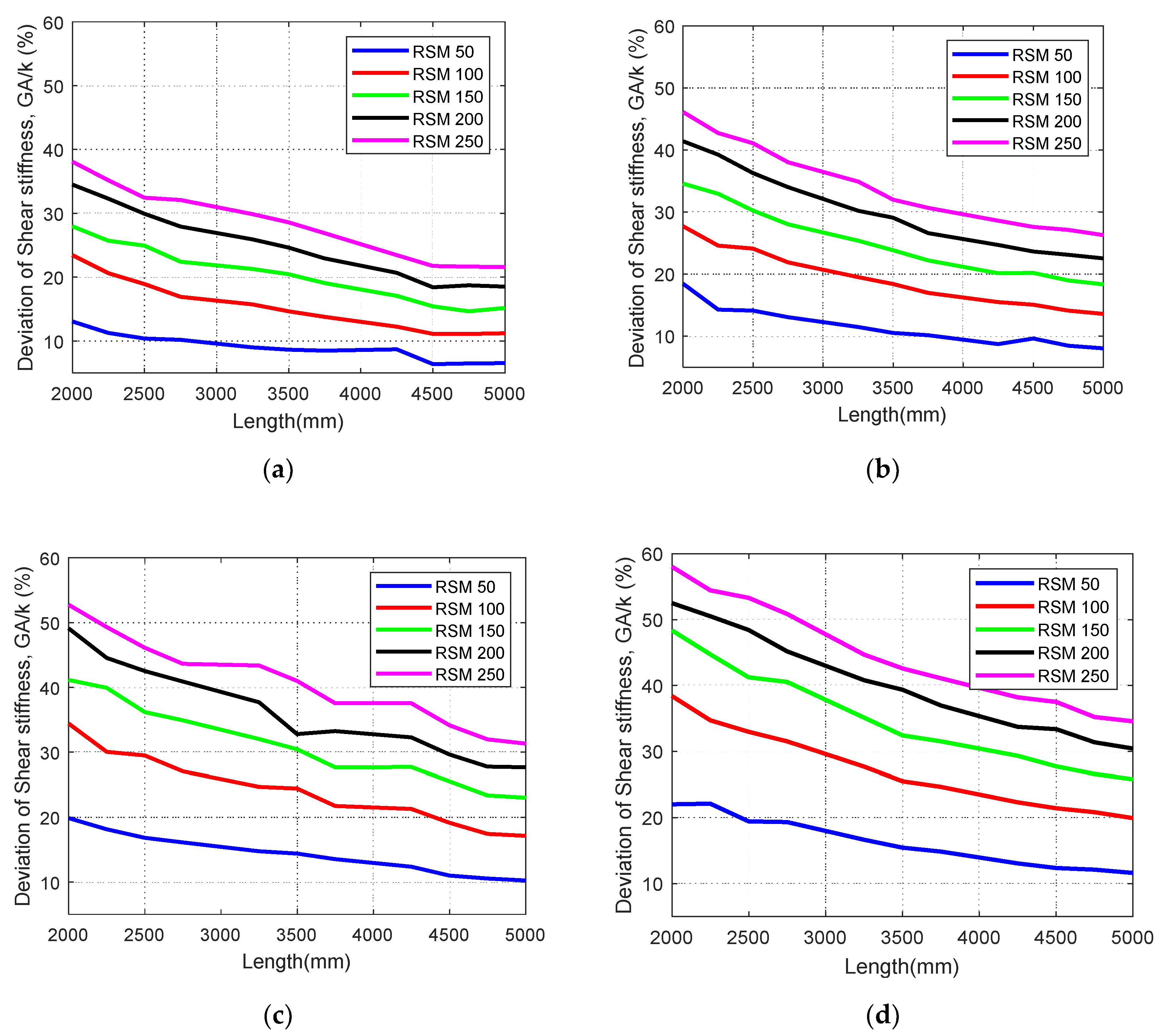
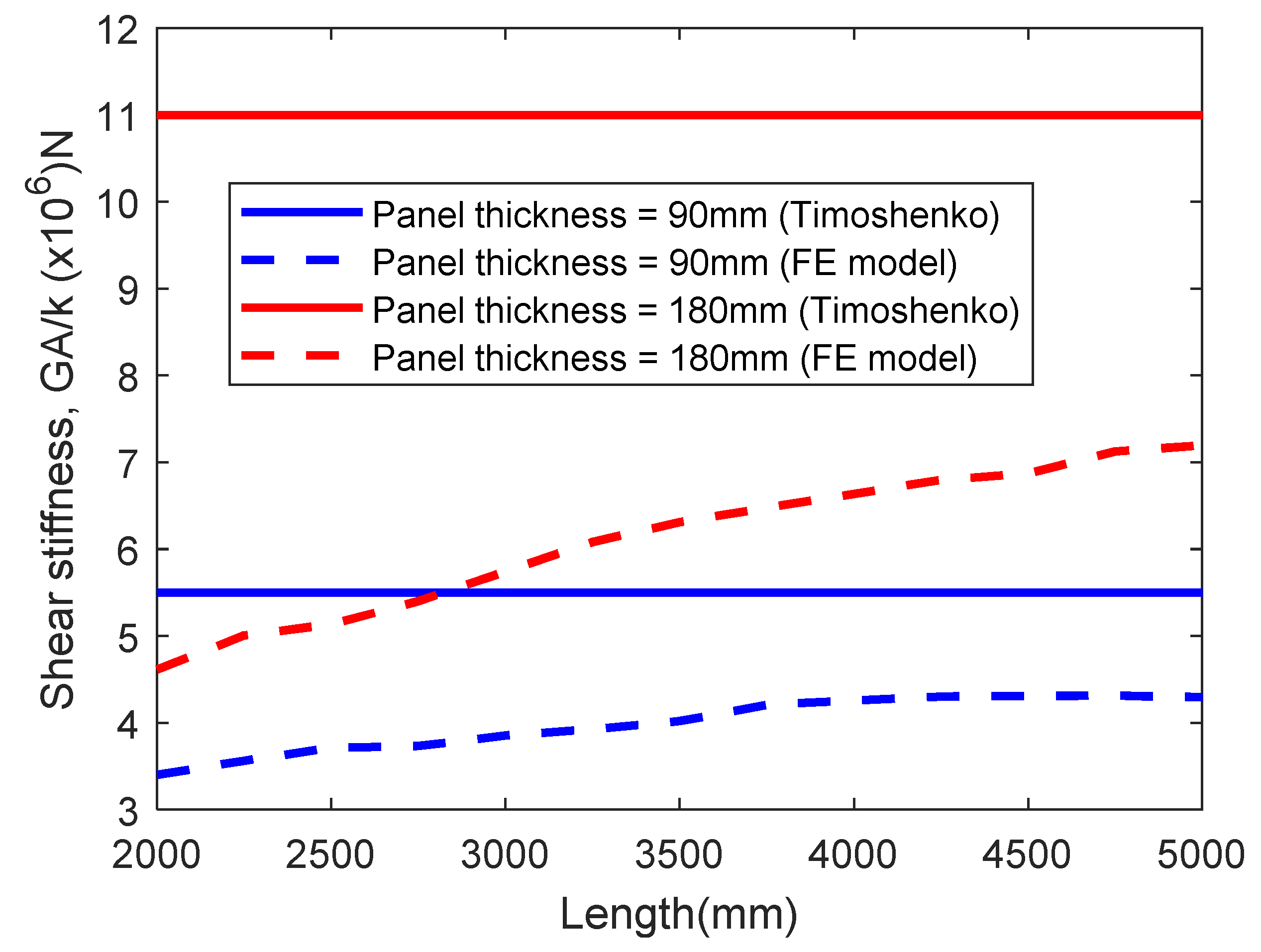
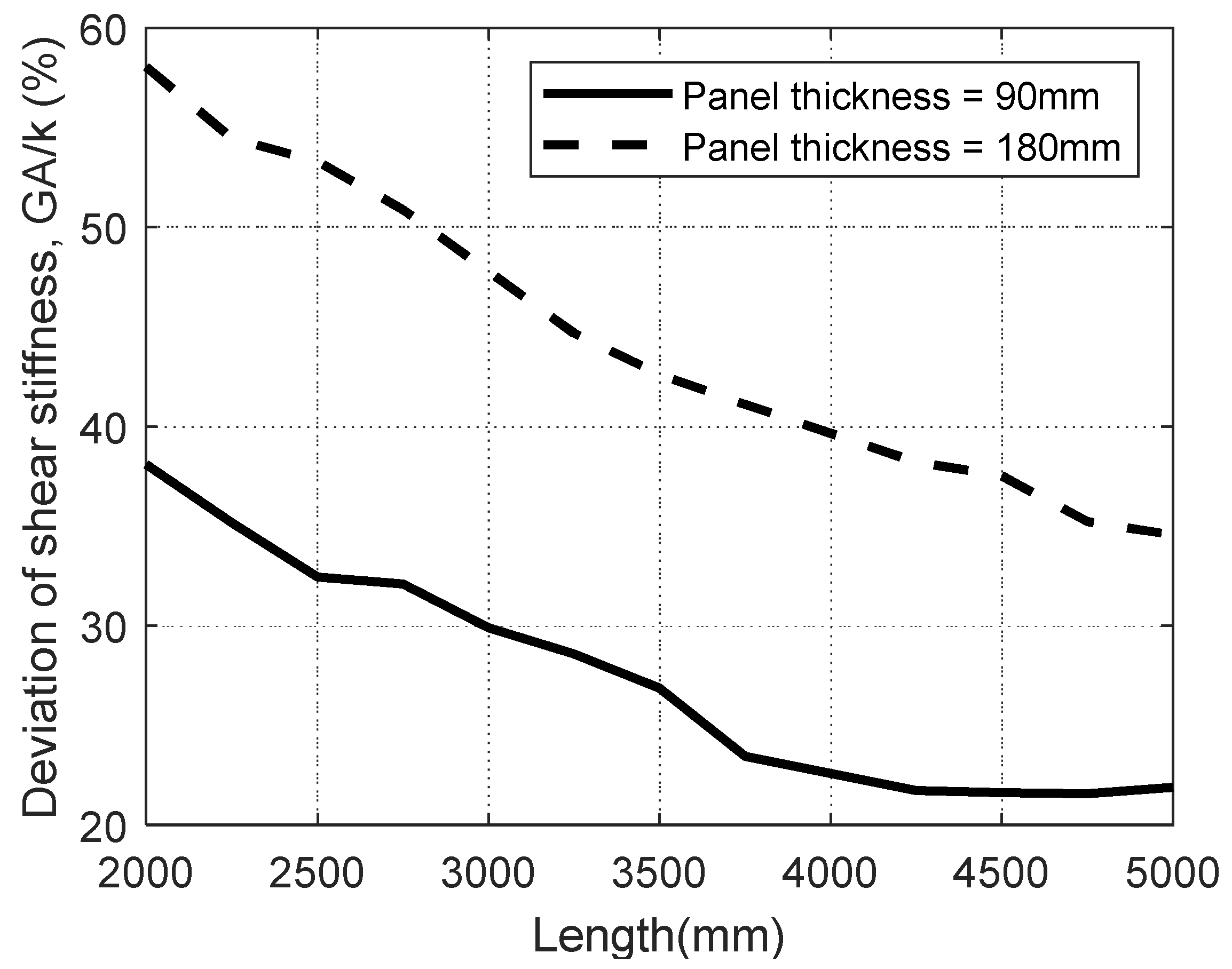
4.2. Parameters Affecting the Performance of Timoshenko Method
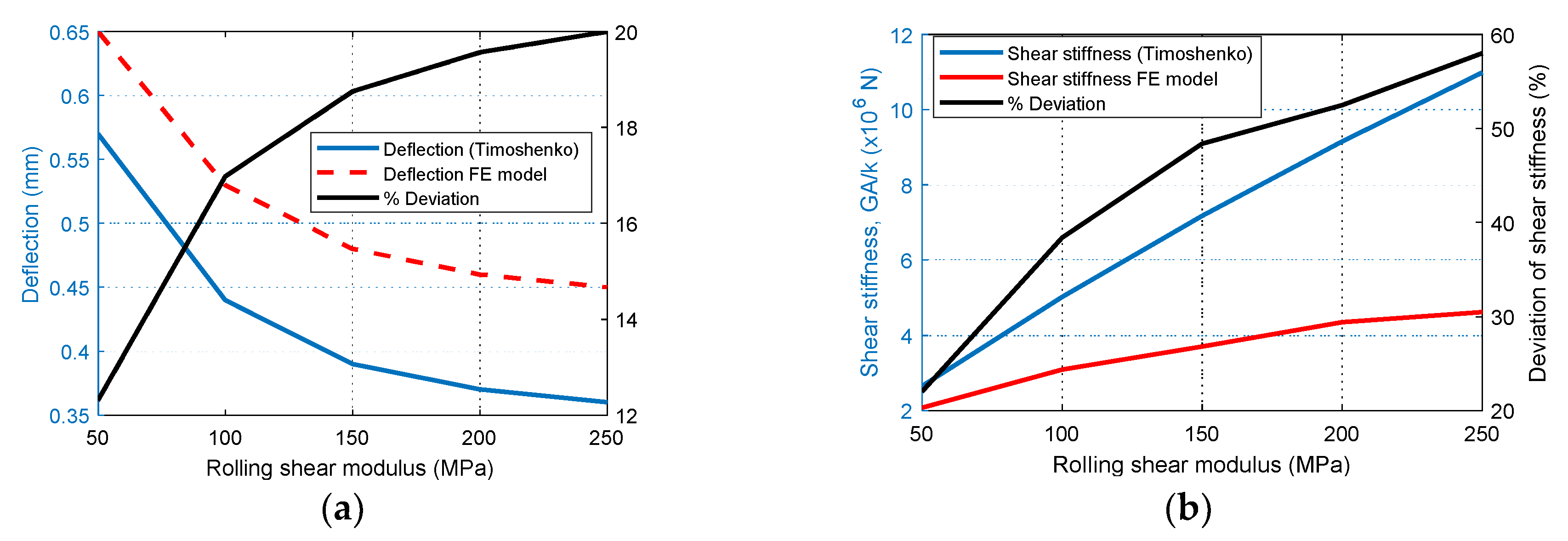
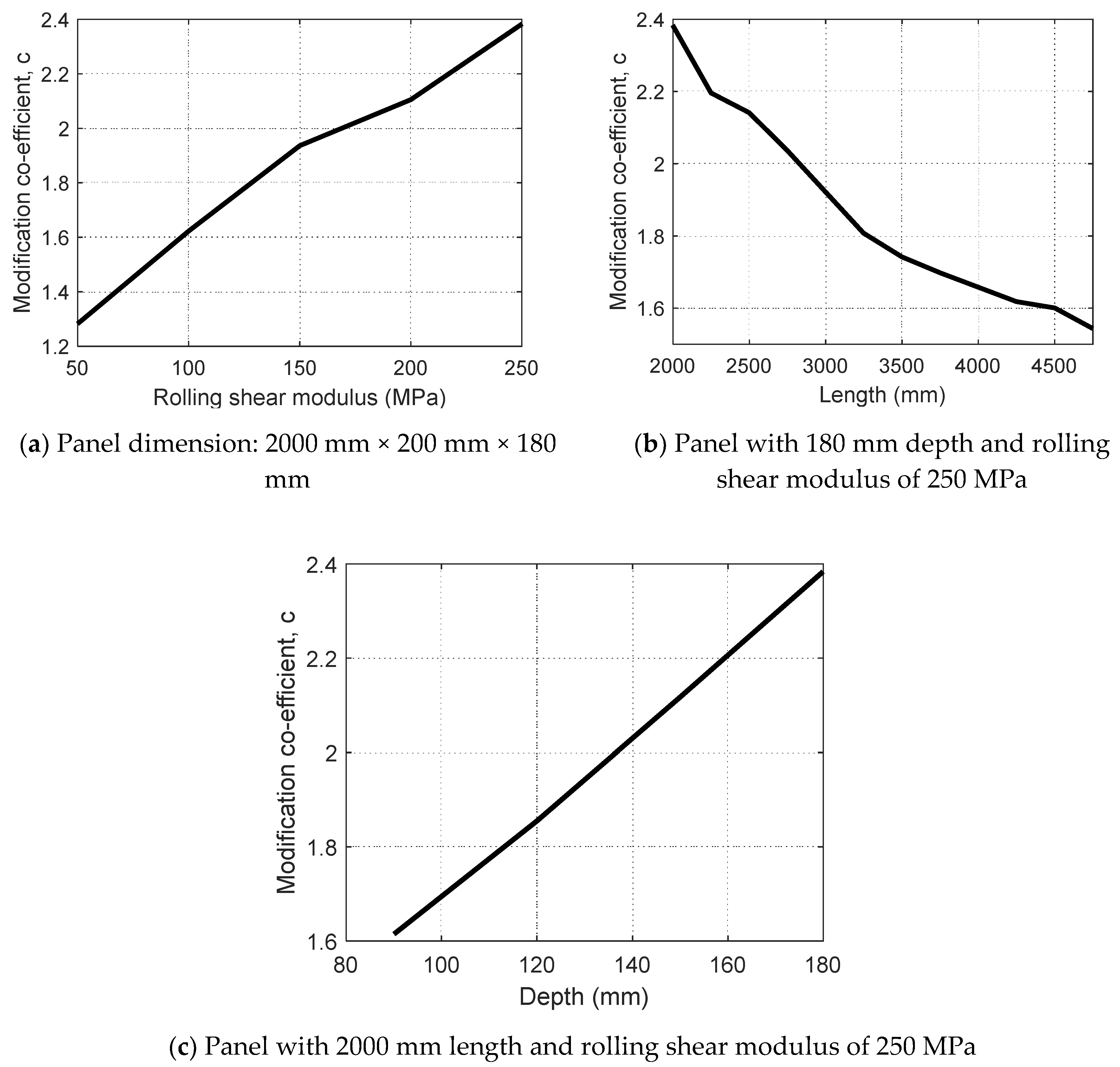
4.3. Effect of Rolling Shear Modulus
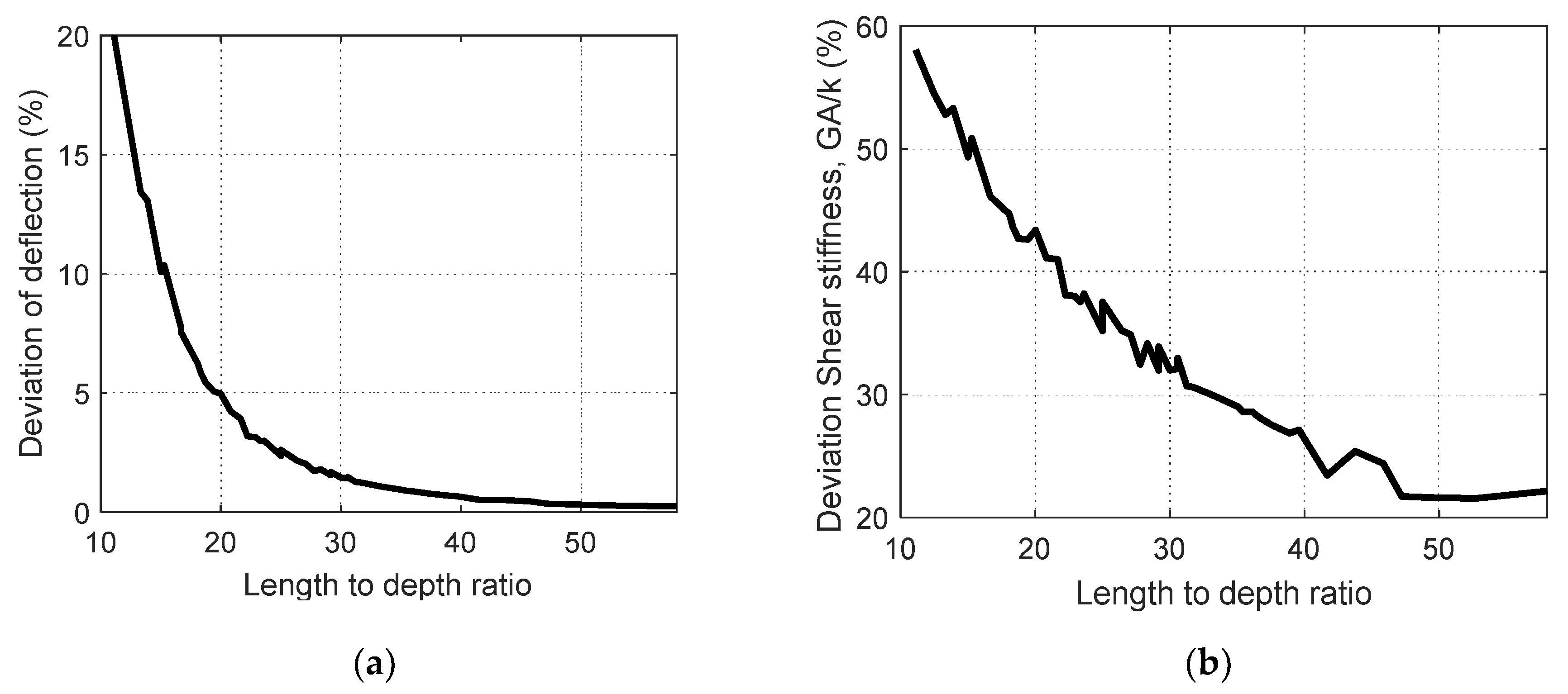
4.4. Effect of Length and Depth
5. Modified Shear Form Factor
5.1. Modification Co-Efficient for Shear Form Factor
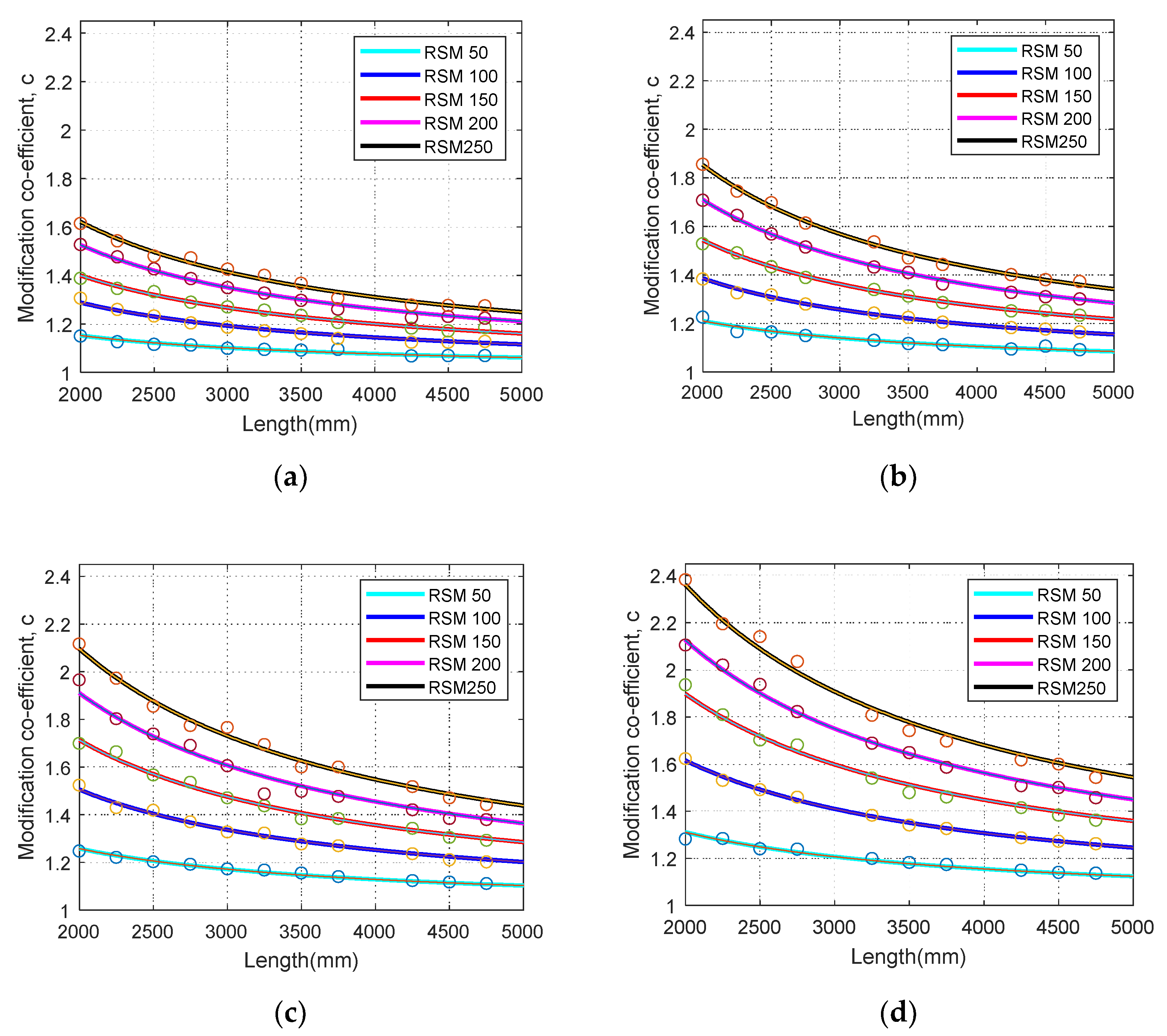
5.2. Formulating Equation for Modification Co-Efficient
5.3. Relationship between Modification Factor, Length and Rolling Shear Modulus
5.4. Validation of the Proposed Factor
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Symbols and Abbreviations
| LVL | Laminated veneer lumber |
| GLT | Glued laminated timber |
| CLT | Cross-laminated timber |
| LSL | Laminated strand veneer |
| k | Shear form factor |
| UDL | Uniformly distributed loading |
| EL | Longitudinal elastic modulus |
| ER | Radial elastic modulus |
| ET | Tangential elastic modulus |
| Poisson’s ratio in longitudinal–tangential plane | |
| Poisson’s ratio in longitudinal–radial plane | |
| Poisson’s ratio in radial–tangential plane | |
| GLT | Shear modulus in longitudinal–tangential plane |
| GLR | Shear modulus in longitudinal–radial plane |
| GRT | Shear modulus in radial–tangential plane |
| L/D ratio | Span-to-depth ratio |
| Effective bending stiffness of the panel | |
| Effective shear stiffness of the panel | |
| q | Uniformly distributed load |
| L | Span of the panel |
| w | Mid-span deflection of the panel |
| Shear form factor estimated from FE models | |
| c | Modification co-efficient |
| ws | Mid-span shear free deflection |
| wm | Mid-span deflection from modified Timoshenko method |
References
- Manninen, H. Long-Term Outlook for Engineered Wood Products in Europe; European Forest Institute: Joensuu, Finland, 2014. [Google Scholar]
- Brandner, R. Production and Technology of Cross Laminated Timber (CLT): A state-of-the-art Report. In Focus Solid Timber Solution-European Conference on Cross Laminated Timber (CLT); University of Bath: Bath, UK, 2013; pp. 3–36. [Google Scholar]
- Christovasilis, I.; Brunetti, C.; Follesa, M.; Nocetti, M.; Vassallo, D. Evaluation of the mechanical properties of cross laminated timber with elementary beam theories. Constr. Build. Mater. 2016, 122, 202–213. [Google Scholar] [CrossRef]
- Li, X.; Ashraf, M.; Subhani, M.; Kremer, P.; Kafle, B.; Ghabraie, K. Experimental and numerical study on bending properties of heterogeneous lamella layups in cross laminated timber using Australian Radiata Pine. Constr. Build. Mater. 2020, 247, 118525. [Google Scholar] [CrossRef]
- Li, X.; Ashraf, M.; Subhani, M.; Kremer, P. Experimental Investigation and Numerical Modelling of Mechanical Properties of CLTs. In SEMC 2019: Advances in Engineering Materials, Structures and Systems: Innovations, Mechanics and Applications, Proceedings of the 7th International Conference on Structural Engineering, Mechanics and Computation (SEMC 2019), Cape Town, South Africa, 2–4 September 2019; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Blass, H.J.; Fellmoser, P. Design of solid wood panels with cross layers. In Proceedings of the 8th World Conference on Timber Engineering, Lahti, Finland, 14–17 June 2004. [Google Scholar]
- Aicher, S.; Dill-Langer, G.; Höfflin, L. Effect of Polar Anisotropy of Wood Loaded Perpendicular to Grain. J. Mater. Civ. Eng. 2001, 13, 2–9. [Google Scholar] [CrossRef]
- Niederwestberg, J.; Zhou, J.; Chui, Y.-H. Mechanical Properties of Innovative, Multi-Layer Composite Laminated Panels. Building 2018, 8, 142. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Fu, H.; Gong, M.; Luo, J.; Dong, W.; Wang, T.; Chui, Y.H. Planar shear and bending properties of hybrid CLT fabricated with lumber and LVL. Constr. Build. Mater. 2017, 151, 172–177. [Google Scholar] [CrossRef]
- Thomas, R.E.; Buehlmann, U. Using Low-Grade Hardwoods for CLT Production: A Yield Analysis. In 6th International Scientific Conference on Hardwood Processing: Proceedings, Proceedings of the 6th International Scientific Conference on Hardwood Processing, Lahti, Finland, 25–28 September 2017; Möttönen, V., Heinonen, E., Eds.; Natural Resources Institute of Finland: Helsinki, Findland, 2017; pp. 199–206. [Google Scholar]
- Ehrhart, T.; Brandner, R. Rolling shear: Test configurations and properties of some European soft- and hardwood species. Eng. Struct. 2018, 172, 554–572. [Google Scholar] [CrossRef]
- Aicher, S.; Christian, Z.; Hirsch, M. Rolling shear modulus and strength of beech wood laminations. Holzforschung 2016, 70, 773–781. [Google Scholar] [CrossRef]
- Li, X.; Subhani, M.; Ashraf, M.; Kafle, B.; Kremer, P. A Current-State-of-the-Art on Design Rules vs Test Resistance of Cross Laminated Timber Members Subjected to Transverse Loading. In CIGOS 2019, Innovation for Sustainable Infrastructure; Springer: Singapore, 2020; pp. 185–190. [Google Scholar]
- Bajzecerová, V. Bending Stiffness of CLT-Concrete Composite Members—Comparison of Simplified Calculation Methods. Procedia Eng. 2017, 190, 15–20. [Google Scholar] [CrossRef]
- Porteous, J.; Ross, P.; Gulvanessian, H. Designers’ Guide to Eurocode 5: Design of Timber Buildings; ICE Publishing: London, UK, 2012. [Google Scholar]
- Sandoli, A.; Calderoni, B. The Rolling Shear Influence on the Out-of-Plane Behavior of CLT Panels: A Comparative Analysis. Building 2020, 10, 42. [Google Scholar] [CrossRef] [Green Version]
- Jeleč, M.; Varevac, D.; Rajčić, V. Cross-laminated timber (CLT)—A state of the art report. Građevinar Časopis Hrvatskog Saveza Građevinskih Inženjera 2018, 70, 75. [Google Scholar]
- Thiel, A.; Schickhofer, G. CLTdesigner—A software tool for designing cross laminated timber elements: 1D-plate-design. In Proceedings of the World Conference on Timber Engineering, Riva del Garda, Italy, 20–24 June 2010. [Google Scholar]
- Brandner, R.; Flatscher, G.; Ringhofer, A.; Schickhofer, G.; Thiel, A. Cross laminated timber (CLT): Overview and development. Eur. J. Wood Wood Prod. 2016, 74, 331–351. [Google Scholar] [CrossRef]
- Wallner-Novak, M.; Koppelhuber, J.; Pock, K. Cross-Laminated Timber Structural Design—Basic Design and Engineering Principles According to Eurocode; ProHolz: Innsbruck, Austria, 2014. [Google Scholar]
- Brandner, R.; Tomasi, R.; Moosbrugger, T.; Serrano, E.; Dietsch, P. Properties, Testing and Design of Cross Laminated Timber; A State-of-the-Art Report by COST Action FP1402/WG2; Shaker Verlag: Aachen, Germany, 2018. [Google Scholar]
- Gagnon, S.; Pirvu, C. CLT Handbook: Cross-Laminated Timber; FPInnovations: Pointe-Claire, QC, Canada, 2011. [Google Scholar]
- Timoshenko, S. LXVI. On the correction for shear of the differential equation for transverse vibrations of prismatic bars. London, Edinburgh, Dublin Philos. Mag. J. Sci. 1921, 41, 744–746. [Google Scholar] [CrossRef] [Green Version]
- Mindlin, R.D. Thickness-Shear and Flexural Vibrations of Crystal Plates. J. Appl. Phys. 1951, 22, 316–323. [Google Scholar] [CrossRef]
- Niederwestberg, J.; Zhou, J.; Chui, Y.-H. Comparison of Theoretical and Laboratory Out-of-Plane Shear Stiffness Values of Cross Laminated Timber Panels. Building 2018, 8, 146. [Google Scholar] [CrossRef] [Green Version]
- Barbero, E.J.; Lopez-Anido, R.A.; Davalos, J.F. On the Mechanics of Thin-Walled Laminated Composite Beams. J. Compos. Mater. 1993, 27, 806–829. [Google Scholar] [CrossRef]
- Madabhusi-Raman, P.; Davalos, J.F. Static shear correction factor for laminated rectangular beams. Compos. Part B Eng. 1996, 27, 285–293. [Google Scholar] [CrossRef]
- Augustin, M.; Schickhofer, G. BSPhandbuch: Holz-Massivbauweise in Brettsperrholz; Verlag der Technischen Universität Graz: Graz, Austria, 2009. [Google Scholar]
- Green, D.W.; Winandy, J.E.; Kretschmann, D.E. Mechanical Properties of Wood. In Wood Handbook; Department of Agrieculture, Fofest Service, Products Laboratory: Madison, WI, USA, 1999. [Google Scholar]
- Davalos, J.F.; Loferski, J.R.; Holzer, S.M.; Yadama, V. Transverse Isotropy Modeling of 3-D Glulam Timber Beams. J. Mater. Civ. Eng. 1991, 3, 125–139. [Google Scholar] [CrossRef]
- CEN 338. Structural Timber–Strength Classes; CEN: Brussels, Belgium, 2009. [Google Scholar]
- Perret, O.; Lebée, A.; Douthe, C.; Sab, K. Equivalent stiffness of timber used in CLT: Closed-form estimates and numerical validation. Eur. J. Wood Wood Prod. 2019, 77, 367–379. [Google Scholar] [CrossRef] [Green Version]
- Ehrhart, T.; Brandner, R.; Schickhofer, G.; Frangi, A. Rolling Shear Properties of Some European Timber Species with Focus on Cross Laminated Timber (CLT): Test Configuration and Parameter Study. In International Network on Timber Engineering Research: Proceedings of Meeting 48; Timber Scientific Publishing, KIT Holzbau und Baukonstruktionen: Graz, Austria, 2015. [Google Scholar]
- Keunecke, D.; Hering, S.; Niemz, P. Three-dimensional elastic behaviour of common yew and Norway spruce. Wood Sci. Technol. 2008, 42, 633–647. [Google Scholar] [CrossRef] [Green Version]
- Neuhaus, F. Helmuth: Elastizitätszahlen von Fichtenholz in Abhängigkeit von der Holzfeuchtigkeit; Institut für konstruktiven Ingenieurbau, Ruhr-Universität: Bochum, Germany, 1981. [Google Scholar]
- EN 16351:2015: Timber Structures—Cross Laminated Timber—Requirements; BSI Standards Publication: London, UK, 2015; pp. 1–103.
- Lahr, F.A.R.; Nogueira, M.C.D.J.A.; De Araujo, V.A.; Vasconcelos, J.S.; Christoforo, A.L. Physical-mechanical characterization of eucalyptus urophylla wood. Engenharia Agrícola 2017, 37, 900–906. [Google Scholar] [CrossRef] [Green Version]
- Crovella, P.; Smith, W.; Bartczak, J. Experimental verification of shear analogy approach to predict bending stiffness for softwood and hardwood cross-laminated timber panels. Constr. Build. Mater. 2019, 229, 116895. [Google Scholar] [CrossRef]
- Sikora, K.S.; McPolin, D.O.; Harte, A.M. Effects of the thickness of cross-laminated timber (CLT) panels made from Irish Sitka spruce on mechanical performance in bending and shear. Constr. Build. Mater. 2016, 116, 141–150. [Google Scholar] [CrossRef] [Green Version]
- Liao, Y.; Tu, D.; Zhou, J.; Zhou, H.; Yun, H.; Gu, J.; Hu, C. Feasibility of manufacturing cross-laminated timber using fast-grown small diameter eucalyptus lumbers. Constr. Build. Mater. 2017, 132, 508–515. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |






| Elastic Property | Direction | Transversely Isotropic Material |
|---|---|---|
| Elastic Modulus | Longitudinal direction | EL |
| Radial direction | ER = ET | |
| Tangential direction | ||
| Poisson’s ratio | Characterizing tangential normal strain to longitudinal normal strain | = |
| Characterizing radial normal strain to longitudinal normal strain | ||
| Characterizing normal tangential strain to radial strain | = | |
| Shear modulus | Longitudinal–tangential plane | GLT = GLR |
| Longitudinal–radial plane | ||
| Radial–tangential plane |
| Characteristic Mechanical Property | Elastic Modulus (MPa) | Poisson’s Ratio | Shear Modulus (MPa) | |||||
|---|---|---|---|---|---|---|---|---|
| EL | ER/ET | GLR | GLT | GRT | ||||
| Longitudinal layer | 11,600 | 370 | 0.014 | 0.014 | 0.21 | 690 | 690 | 50 |
| Cross-layer | 11,600 | Constrained * | 0.014 | 0.014 | 0.21 | 690 | 690 | 50–250 |
| Characteristic Mechanical Property | Elastic Modulus (MPa) | Poisson’s Ratio | Shear Modulus (MPa) | |||||
|---|---|---|---|---|---|---|---|---|
| EL | ER/ET | GLR | GLT | GRT | ||||
| Longitudinal layer | 11,000 | 370 | 0.014 | 0.014 | 0.21 | 690 | 690 | 50 |
| Engineered Wood Product | Elastic Modulus (MPa) | Shear Modulus (MPa) | ||||
|---|---|---|---|---|---|---|
| Layer 1 E1 | Layer 2 E2 | Layer 3 E3 | Layer 1 G1 | Layer 2 G2 | Layer 3 G3 | |
| CLT | 11,600 | 370 | 11,600 | 690 | 50 | 690 |
| GLT | 11,600 | 11,600 | 11,600 | 690 | 690 | 690 |
| Dimension (L × W × D) (mm × mm × mm) | Deflection (Timoshenko) (mm) | Deflection (Model) (mm) | Error (%) |
|---|---|---|---|
| 1026 × 200 × 60 | 0.57 | 0.55 | 3.5 |
| 1800 × 200 × 120 | 0.68 | 0.68 | 0 |
| 1800 × 200 × 90 | 1.57 | 1.54 | 1.9 |
| 2500 × 200 × 120 | 2.45 | 2.40 | 2 |
| Species | EL (MPa) | ER/ET (MPa) | GLT/GLR (MPa) | GRT (MPa) | Reference |
|---|---|---|---|---|---|
| Irish sitka (Softwood) | 9900 | 772.2 | 633.6 | 61.88 3 | [29] |
| White pine (Softwood) | 8900 | 694.2 | 462.8 | 44.5 3 | [29] |
| Eucalyptus urophylla (Hardwood) | 13,391.7 | 640.70 | 897.24 1 | 267.83 2 | [37] |
| Red Maple (Hardwood) | 11,300 | 1582 | 1502 | 203.4 2 | [29] |
| White Ash (Hardwood) | 12,000 | 1536 | 1308 | 216 2 | [29] |
| Species | Geometry (l × w × h) (mm) | a (mm) | EIglobal (×1010 N/mm2) | Experimental vs. Analytical Predictions | |
|---|---|---|---|---|---|
| δTM/δTest * | δmTM/δTest | ||||
| Irish Sitka [40] | 1440 × 270 × 60 | 540 | 3.69 | 0.939 | 0.963 |
| 1728 × 288 × 72 | 648 | 6.64 | 0.917 | 0.941 | |
| 2880 × 584 × 120 | 1080 | 63.61 | 0.936 | 0.962 | |
| 1440 × 584 × 120 | 360 | 39.44 | 0.806 | 0.922 | |
| Eucalyptus urophylla [40] | 1620 × 305 × 54 | 540 | 4.59 | 0.786 | 0.938 |
| White pine [38] | 2992.5 × 300 × 105 | 997.5 | 17.5 | 0.812 | 0.824 |
| Red Maple [38] | 2992.5 × 300 × 105 | 997.5 | 28.6 | 0.944 | 0.960 |
| White Ash [38] | 2992.5 × 300 × 105 | 997.5 | 30.6 | 0.952 | 0.970 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, M.T.; Ashraf, M.; Ghabraie, K.; Subhani, M. Evaluating Timoshenko Method for Analyzing CLT under Out-of-Plane Loading. Buildings 2020, 10, 184. https://doi.org/10.3390/buildings10100184
Rahman MT, Ashraf M, Ghabraie K, Subhani M. Evaluating Timoshenko Method for Analyzing CLT under Out-of-Plane Loading. Buildings. 2020; 10(10):184. https://doi.org/10.3390/buildings10100184
Chicago/Turabian StyleRahman, MD Tanvir, Mahmud Ashraf, Kazem Ghabraie, and Mahbube Subhani. 2020. "Evaluating Timoshenko Method for Analyzing CLT under Out-of-Plane Loading" Buildings 10, no. 10: 184. https://doi.org/10.3390/buildings10100184
APA StyleRahman, M. T., Ashraf, M., Ghabraie, K., & Subhani, M. (2020). Evaluating Timoshenko Method for Analyzing CLT under Out-of-Plane Loading. Buildings, 10(10), 184. https://doi.org/10.3390/buildings10100184








