Effect of Rise and Fall Time on Dwell Fatigue Behavior of a High Strength Titanium Alloy
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bania, P.J.; Eylon, D. Fatigue crack propagation of titanium alloys under dwell-time conditions. Metall. Trans. A 1978, 9, 847–855. [Google Scholar] [CrossRef]
- Lefranc, P.; Sarrazin-Baudoux, C.; Doquet, V.; Petit, J. Investigation of the dwell period’s influence on the fatigue crack growth of a titanium alloy. Scr. Mater. 2009, 60, 281–284. [Google Scholar] [CrossRef]
- Wang, F.; Cui, W. Experimental investigation on dwell-fatigue property of Ti-6Al-4V ELI used in deep-sea manned cabin. Mater. Sci. Eng. A 2015, 642, 136–141. [Google Scholar] [CrossRef]
- Qiu, J.; Ma, Y.; Lei, J.; Liu, Y.; Huang, A.; Rugg, D.; Yang, R. A Comparative study on dwell fatigue of Ti-6Al-2Sn-4Zr-xMo (x = 2 to 6) alloys on a microstructure-normalized basis. Metall. Mater. Trans. A 2014, 45, 6075–6087. [Google Scholar] [CrossRef]
- Shen, W.; Soboyejo, W.O.; Soboyejo, A.B.O. An investigation on fatigue and dwell-fatigue crack growth in Ti-6Al-2Sn-4Zr-2Mo-0.1Si. Mech. Mater. 2004, 36, 117–140. [Google Scholar] [CrossRef]
- Zheng, Z.; Balint, D.S.; Dunne, F.P.E. Mechanistic basis of temperature-dependent dwell fatigue in titanium alloys. J. Mech. Phys. Solids 2017, 107, 185–203. [Google Scholar] [CrossRef]
- Song, Z.; Hoeppner, D.W. Dwell time effects on the fatigue behavior of titanium alloys. Int. J. Fatigue 1988, 10, 211–218. [Google Scholar] [CrossRef]
- Bache, M.R. A review of dwell sensitive fatigue in titanium alloys: The role of microstructure, texture and operating conditions. Int. J. Fatigue 2003, 25, 1079–1087. [Google Scholar] [CrossRef]
- Evans, W.J.; Gostelow, C.R. The effect of hold time on the fatigue properties of a β-processed titanium alloy. Metall. Trans. A 1979, 10, 1837–1846. [Google Scholar] [CrossRef]
- Bache, M.R.; Cope, M.; Davies, H.M.; Harrison, G. Dwell sensitive fatigue in a near alpha titanium alloy at ambient temperature. Int. J. Fatigue 1997, 19, S83–S88. [Google Scholar] [CrossRef]
- Evans, W.J. Time dependent effects in fatigue of titanium and nickel alloys. Fatigue Fract. Eng. Mater. Struct. 2004, 27, 543–557. [Google Scholar] [CrossRef]
- Takezono, S.; Satoh, M. Effect of stress frequency on fatigue crack propagation in titanium. J. Eng. Mater. Technol. ASME 1982, 104, 257–261. [Google Scholar] [CrossRef]
- Dahal, J.; Maciejewski, K.; Ghonem, H. Loading frequency and microstructure interactions in intergranular fatigue crack growth in a disk Ni-based superalloy. Int. J. Fatigue 2013, 57, 93–102. [Google Scholar] [CrossRef]
- Morrissey, R.J.; McDowell, D.L. Frequency and stress ratio effects in high cycle fatigue of Ti-6Al-4V. Int. J. Fatigue 1999, 21, 679–685. [Google Scholar] [CrossRef]
- Guennec, B.; Ueno, A.; Sakai, T.; Takanashi, M.; Itabashi, Y. Effect of the loading frequency on fatigue properties of JIS S15C low carbon steel and some discussions based on micro-plasticity behavior. Int. J. Fatigue 2014, 66, 29–38. [Google Scholar] [CrossRef]
- Beretta, S.; Ghidini, A.; Lombardo, F. Fracture mechanics and scale effects in the fatigue of railway axles. Eng. Fract. Mech. 2005, 72, 195–208. [Google Scholar] [CrossRef]
- Furuya, Y. Specimen size effects on gigacycle fatigue properties of high-strength steel under ultrasonic fatigue testing. Scr. Mater. 2008, 58, 1014–1017. [Google Scholar] [CrossRef]
- Sun, C.; Song, Q. A method for predicting the effects of specimen geometry and loading condition on fatigue strength. Metals 2018, 8, 811. [Google Scholar] [CrossRef]
- Song, Z.; Hoeppner, D.W. Size effect on the fatigue behaviour of IMI 829 titanium alloy under dwell conditions. Int. J. Fatigue 1989, 11, 85–90. [Google Scholar] [CrossRef]
- Taylor, J.R. An Introduction to Error Analysis, 2nd ed.; University Science Books: Sausalito, CA, USA, 1997. [Google Scholar]
- Yang, L.; Liu, J.; Chen, Z.; Wang, Q.; Yang, R. Effect of loading waveform on fatigue damage behavior of Ti-60 alloy. Chin. J. Nonferrous Metals 2010, 20, S487–S490. [Google Scholar]


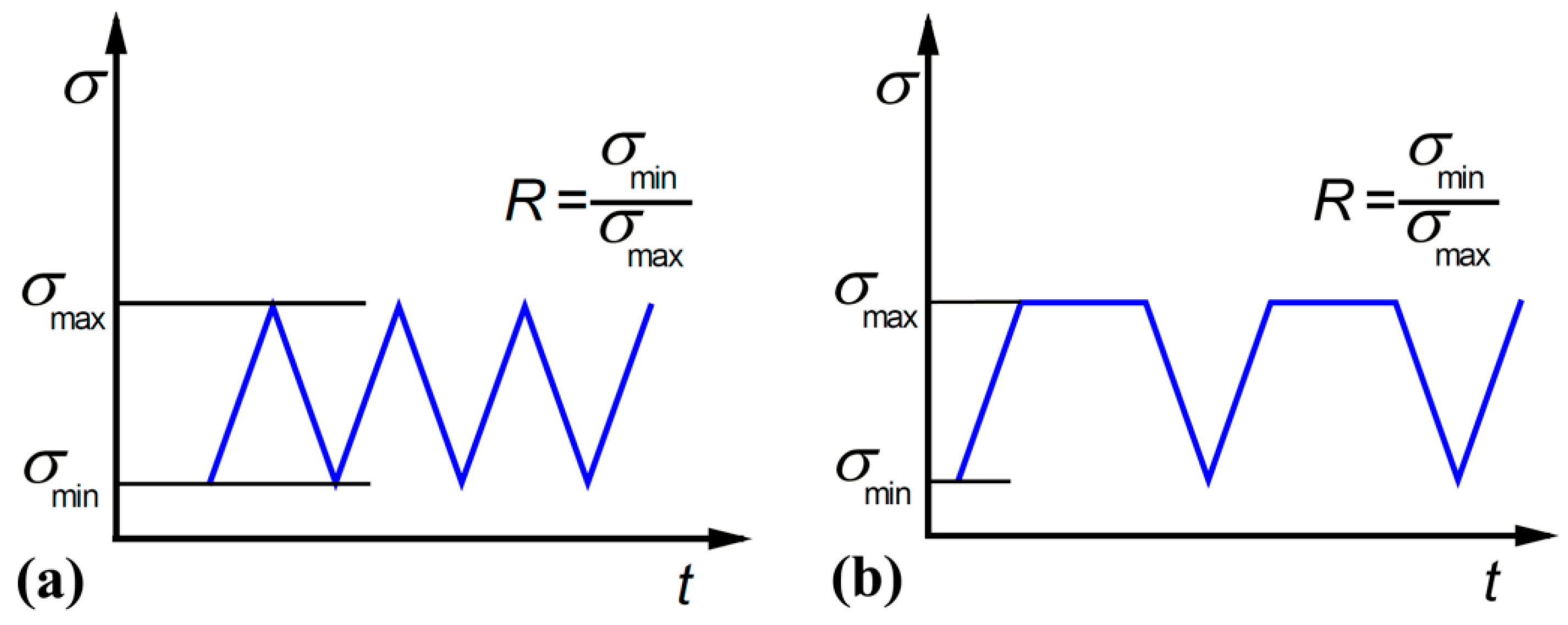
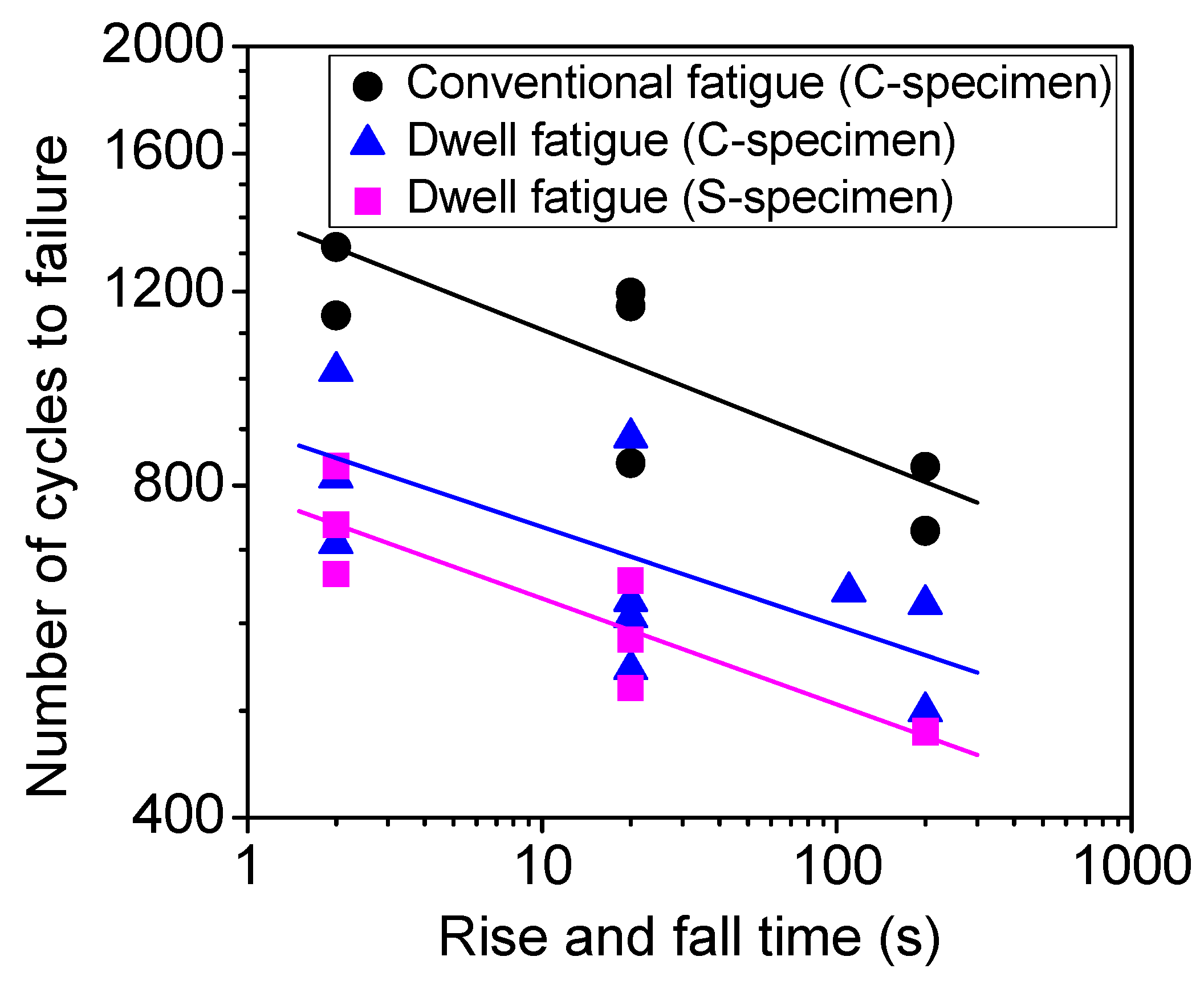

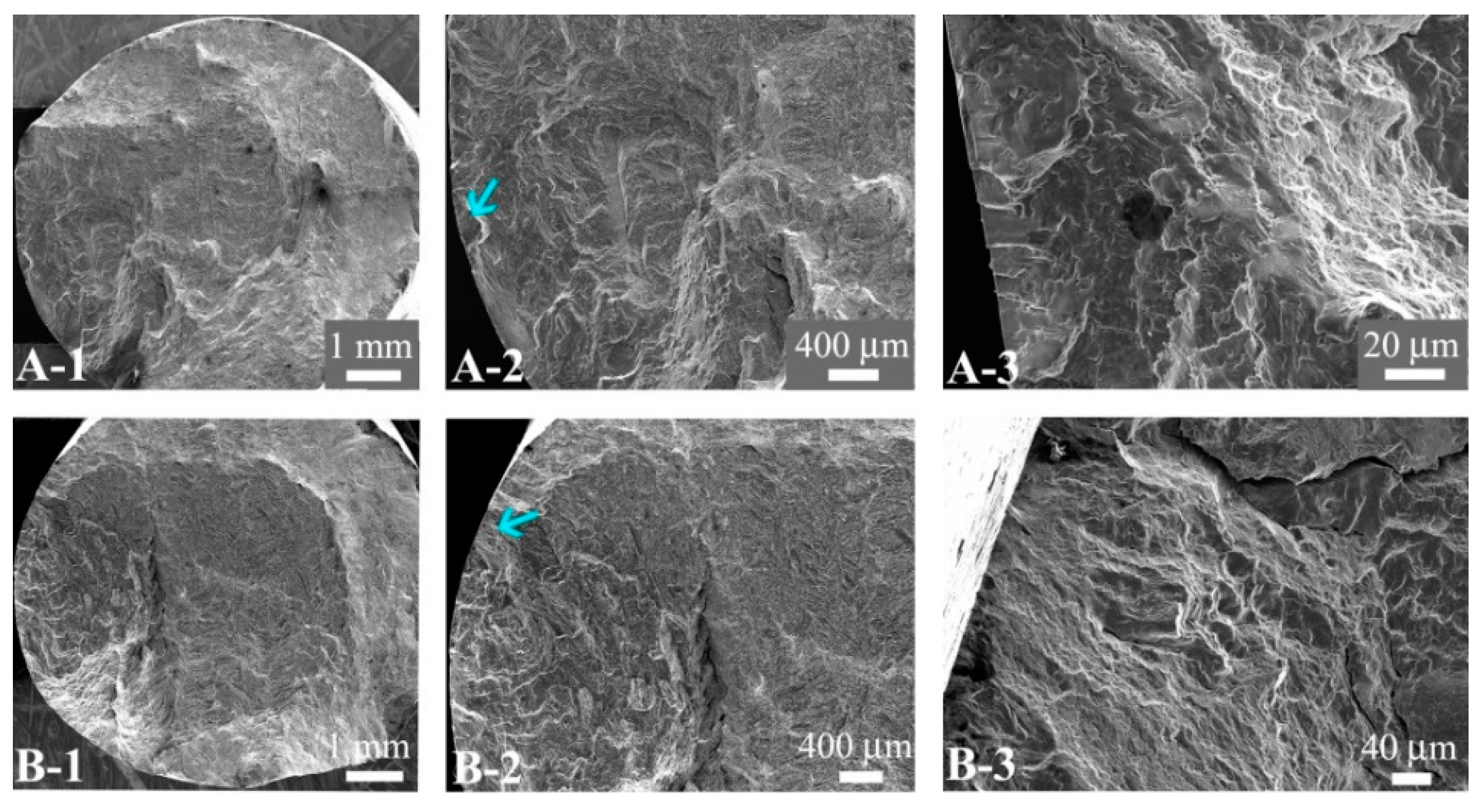
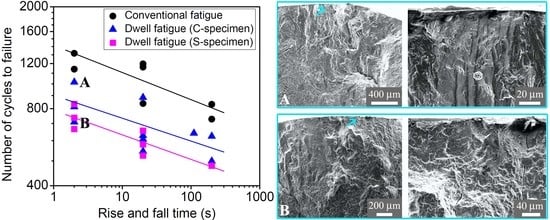
| Specimen No. | Specimen Type | Maximum Stress/MPa | Stress Ratio R | Rise Time/s | Fall Time/s | Dwell Time/s | Fatigue Life/cyc |
|---|---|---|---|---|---|---|---|
| 1 | C−specimen | 929.1 | −1 | 2 | 2 | 60 | 1016 |
| 2 | C−specimen | 929.1 | −1 | 2 | 2 | 60 | 813 |
| 3 | C−specimen | 929.1 | −1 | 2 | 2 | 60 | 709 |
| 4 | C−specimen | 929.1 | −1 | 20 | 20 | 60 | 884 |
| 5 | C−specimen | 929.1 | −1 | 20 | 20 | 60 | 628 |
| 6 | C−specimen | 929.1 | −1 | 20 | 20 | 60 | 607 |
| 7 | C−specimen | 929.1 | −1 | 20 | 20 | 60 | 545 |
| 8 | C−specimen | 929.1 | −1 | 110 | 110 | 60 | 641 |
| 9 | C−specimen | 929.1 | −1 | 200 | 200 | 60 | 499 |
| 10 | C−specimen | 929.1 | −1 | 200 | 200 | 60 | 624 |
| 11 | C−specimen | 929.1 | −1 | 2 | 2 | 0 | 1141 |
| 12 | C−specimen | 929.1 | −1 | 2 | 2 | 0 | 1316 |
| 13 | C−specimen | 929.1 | −1 | 20 | 20 | 0 | 1197 |
| 14 | C−specimen | 929.1 | −1 | 20 | 20 | 0 | 1163 |
| 15 | C−specimen | 929.1 | −1 | 20 | 20 | 0 | 838 |
| 16 | C−specimen | 929.1 | −1 | 200 | 200 | 0 | 832 |
| 17 | C−specimen | 929.1 | −1 | 200 | 200 | 0 | 728 |
| 18 | S−specimen | 929.1 | −1 | 2 | 2 | 60 | 665 |
| 19 | S−specimen | 929.1 | −1 | 2 | 2 | 60 | 833 |
| 20 | S−specimen | 929.1 | −1 | 2 | 2 | 60 | 737 |
| 21 | S−specimen | 929.1 | −1 | 20 | 20 | 60 | 656 |
| 22 | S−specimen | 929.1 | −1 | 20 | 20 | 60 | 524 |
| 23 | S−specimen | 929.1 | −1 | 20 | 20 | 60 | 580 |
| 24 | S−specimen | 929.1 | −1 | 200 | 200 | 60 | 479 |
| 25 | S−specimen | 929.1 | −1 | 200 | 200 | 60 | 478 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Q.; Li, Y.; Wang, L.; Huang, R.; Sun, C. Effect of Rise and Fall Time on Dwell Fatigue Behavior of a High Strength Titanium Alloy. Metals 2019, 9, 914. https://doi.org/10.3390/met9080914
Song Q, Li Y, Wang L, Huang R, Sun C. Effect of Rise and Fall Time on Dwell Fatigue Behavior of a High Strength Titanium Alloy. Metals. 2019; 9(8):914. https://doi.org/10.3390/met9080914
Chicago/Turabian StyleSong, Qingyuan, Yanqing Li, Lei Wang, Ruxu Huang, and Chengqi Sun. 2019. "Effect of Rise and Fall Time on Dwell Fatigue Behavior of a High Strength Titanium Alloy" Metals 9, no. 8: 914. https://doi.org/10.3390/met9080914
APA StyleSong, Q., Li, Y., Wang, L., Huang, R., & Sun, C. (2019). Effect of Rise and Fall Time on Dwell Fatigue Behavior of a High Strength Titanium Alloy. Metals, 9(8), 914. https://doi.org/10.3390/met9080914