Modeling Thermomechanical Stress with H13 Tool Steel Material Response for Rolling Die under Hot Milling
Abstract
1. Introduction
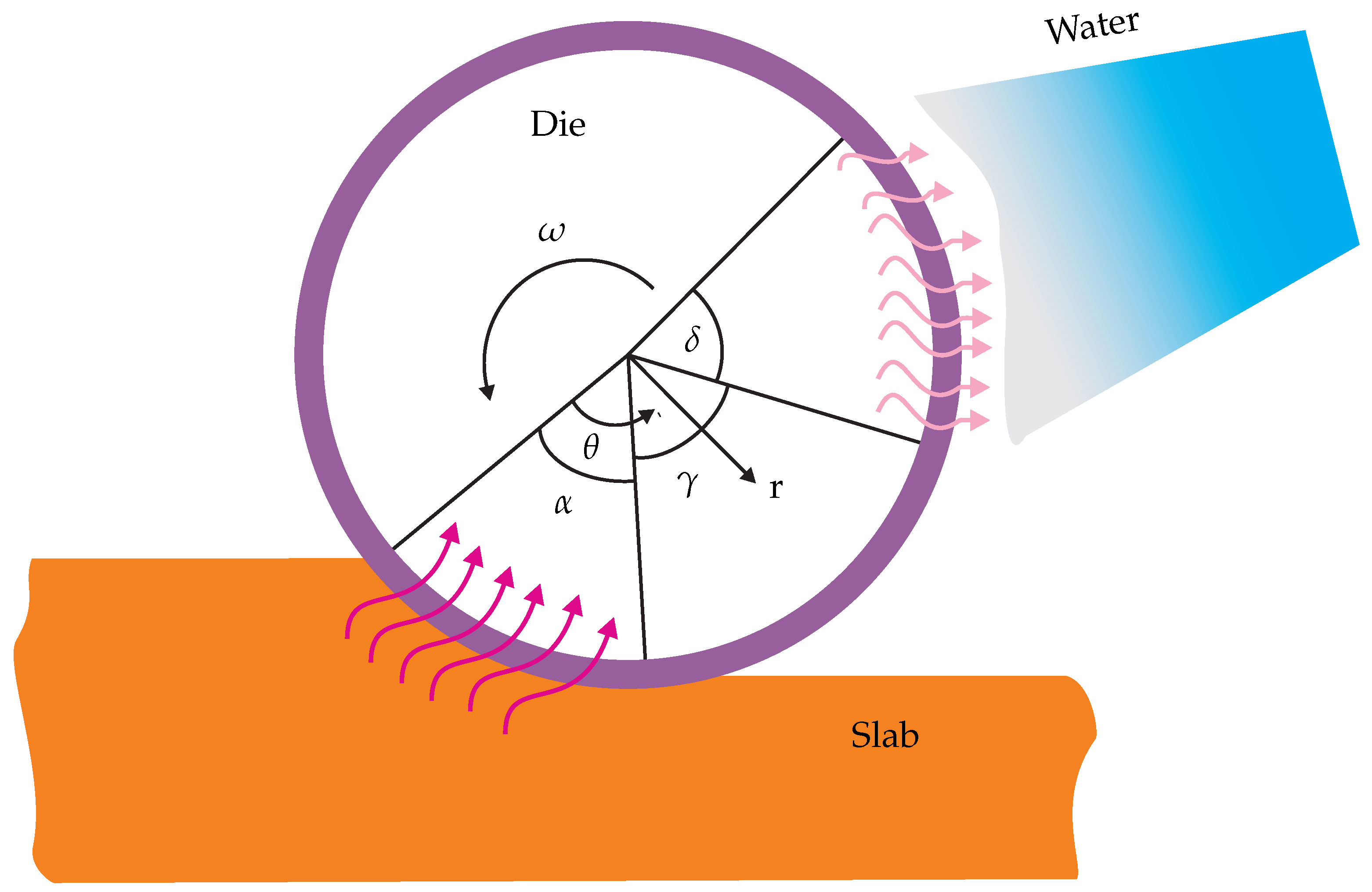
2. Temperature Distribution in Hot Rolling Process
- Gain during the deformation of a hot strip due to heat conduction
- Loss due to water cooling
- Loss due to radiation
- Loss due to convection, and
- Gain due to friction.
2.1. Temperature Gain Due to Conduction to the Hot Slab
2.2. Temperature Loss Due to Water Cooling
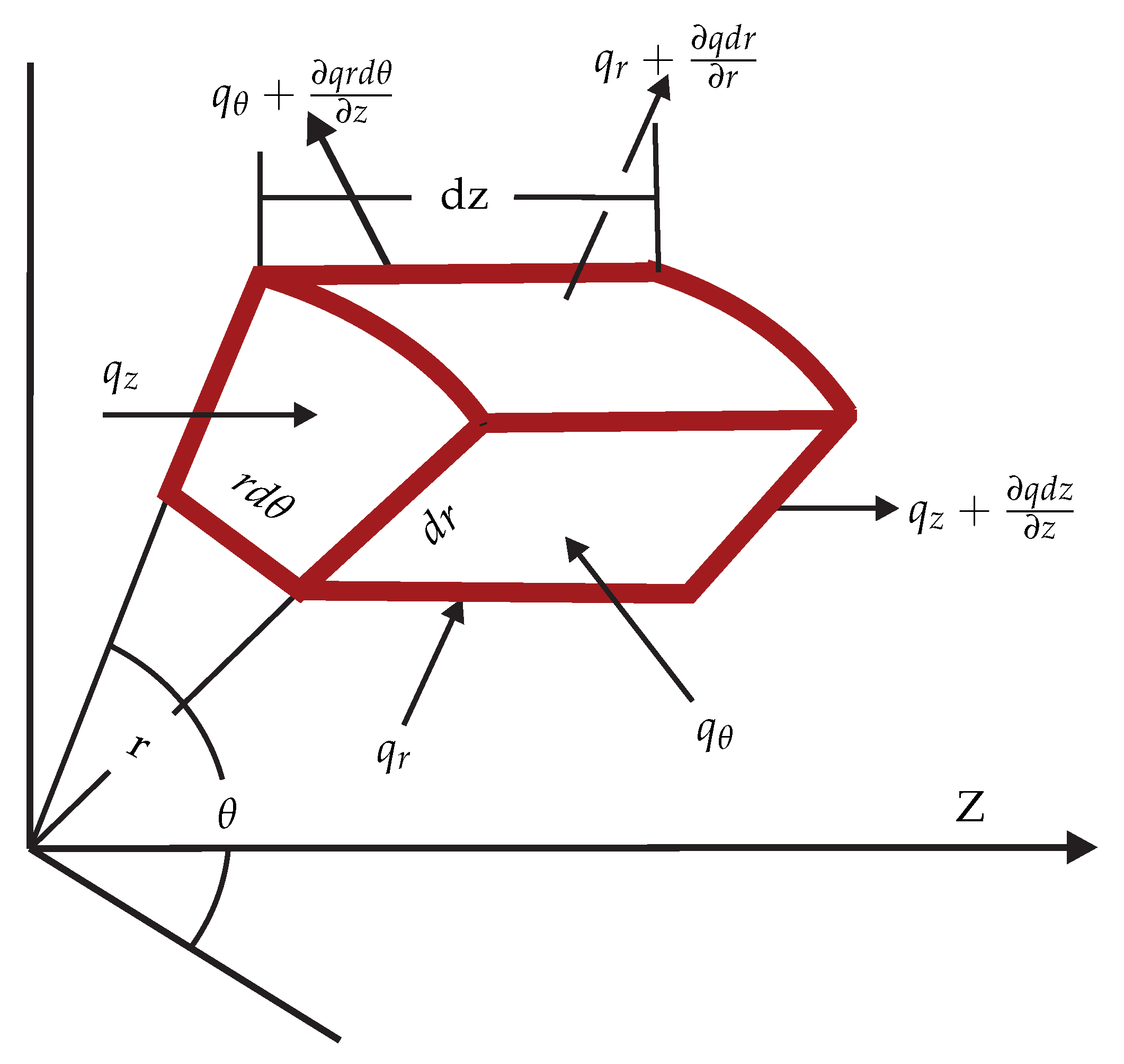
2.3. Temperature Gradient
3. Thermal Stresses in Hot Rolling Die
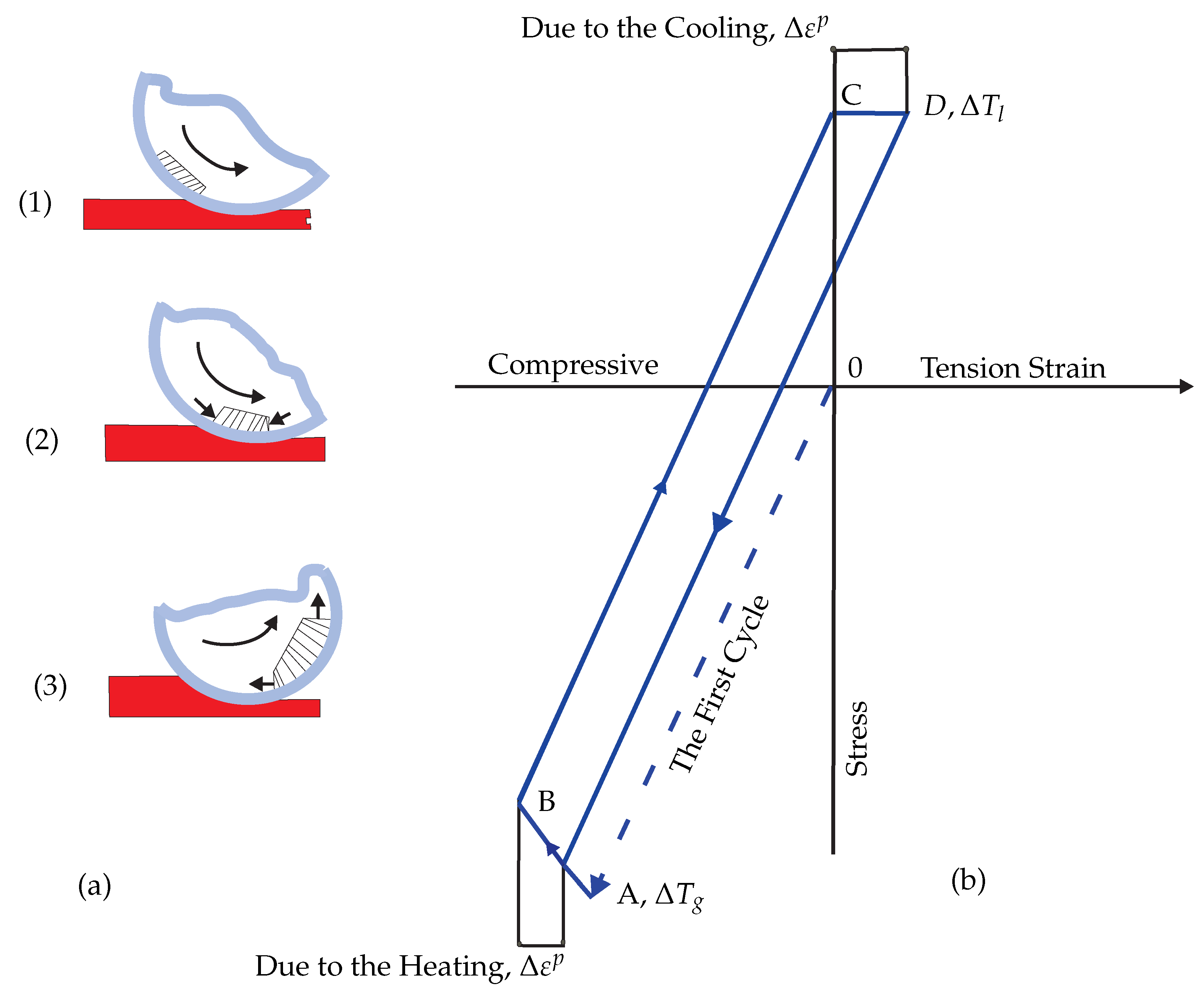
- The circumferential stress is equal in magnitude to the yield stress during plastic deformation, and
- The maximum circumferential strains are unaltered by the occurrence of the plastic deformation. The plastic strain amplitude of the hysteresis loop can be expressed by
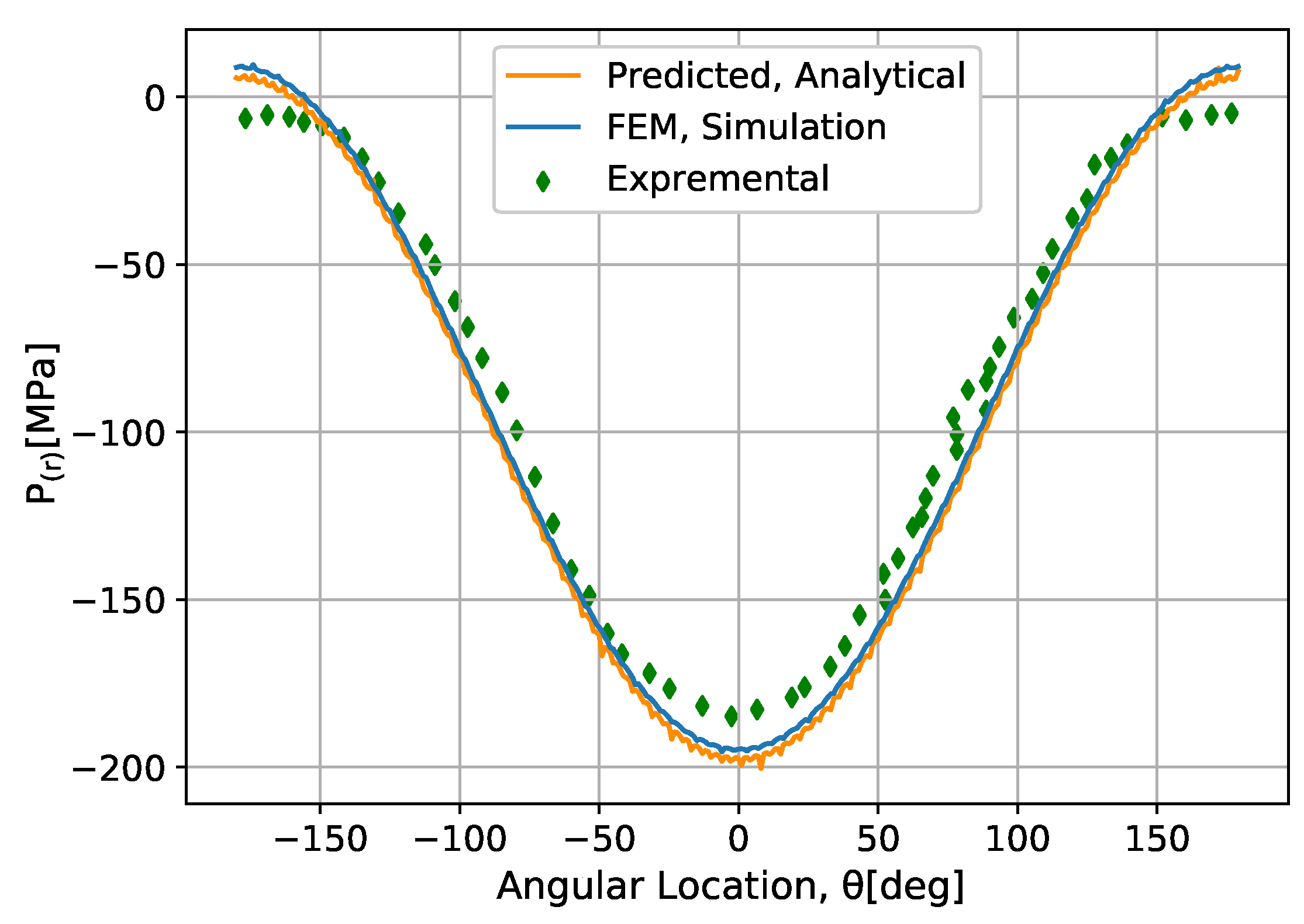
4. Mechanical Stresses and Constitutive Equation for Rolling Die
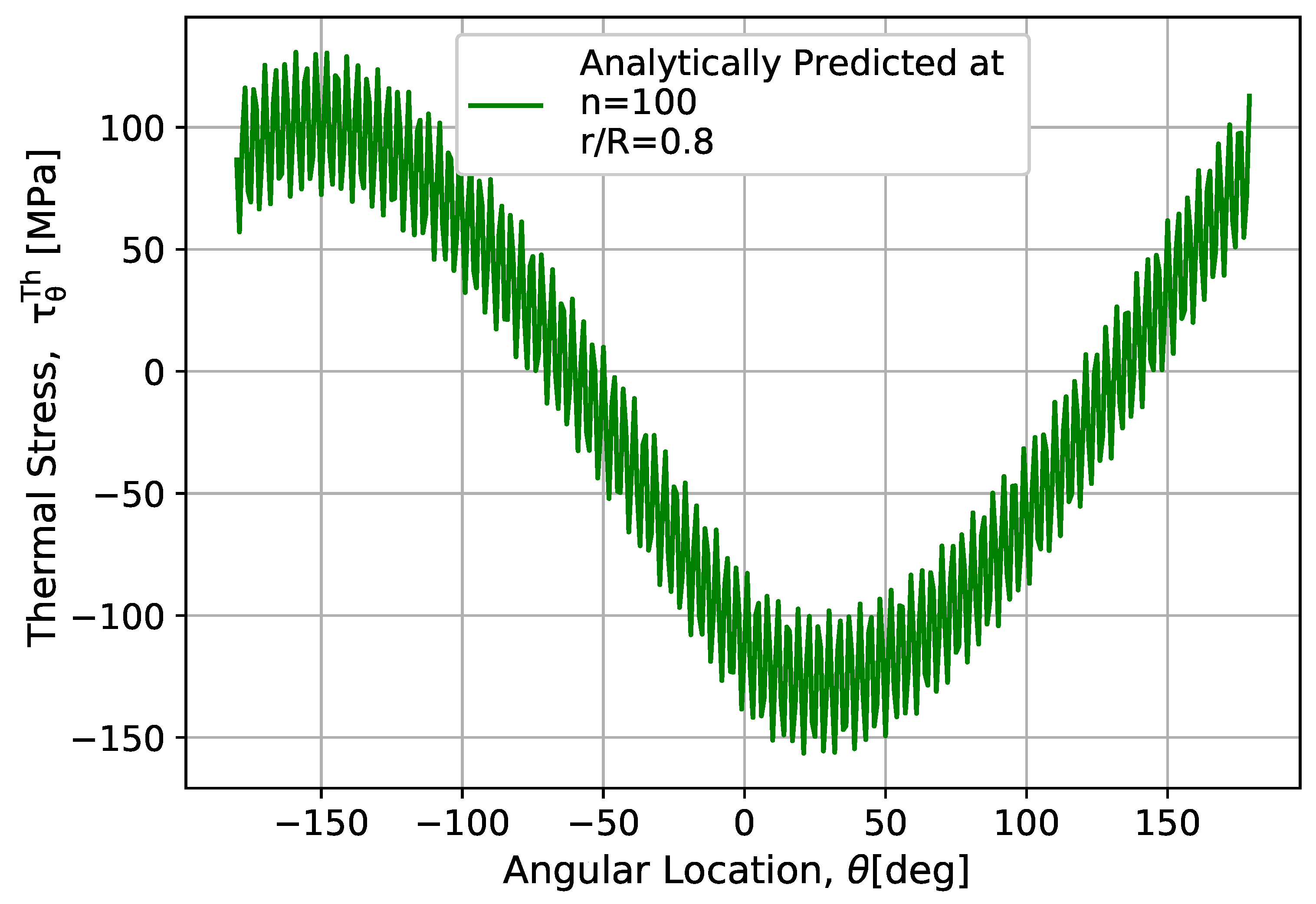
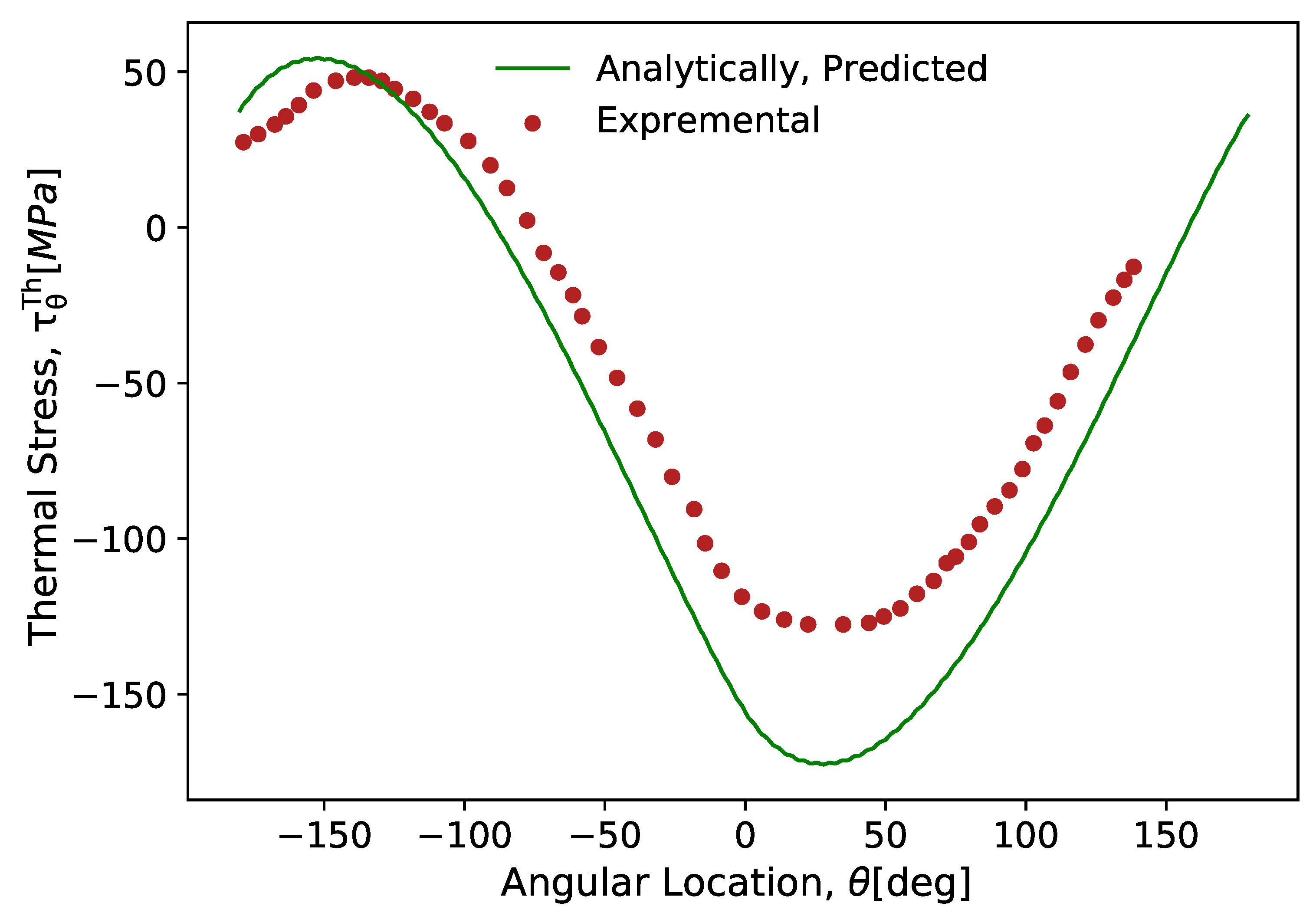
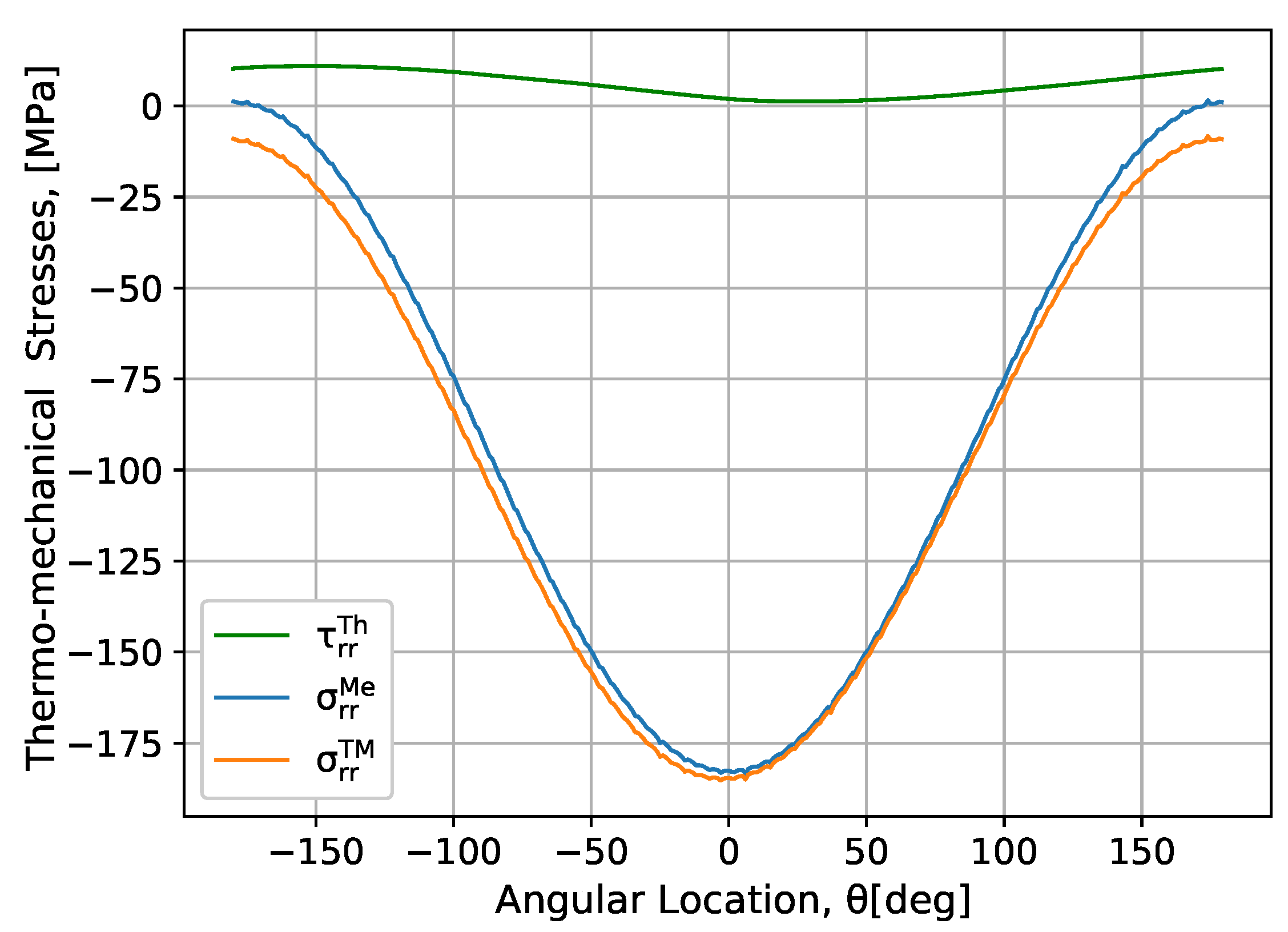
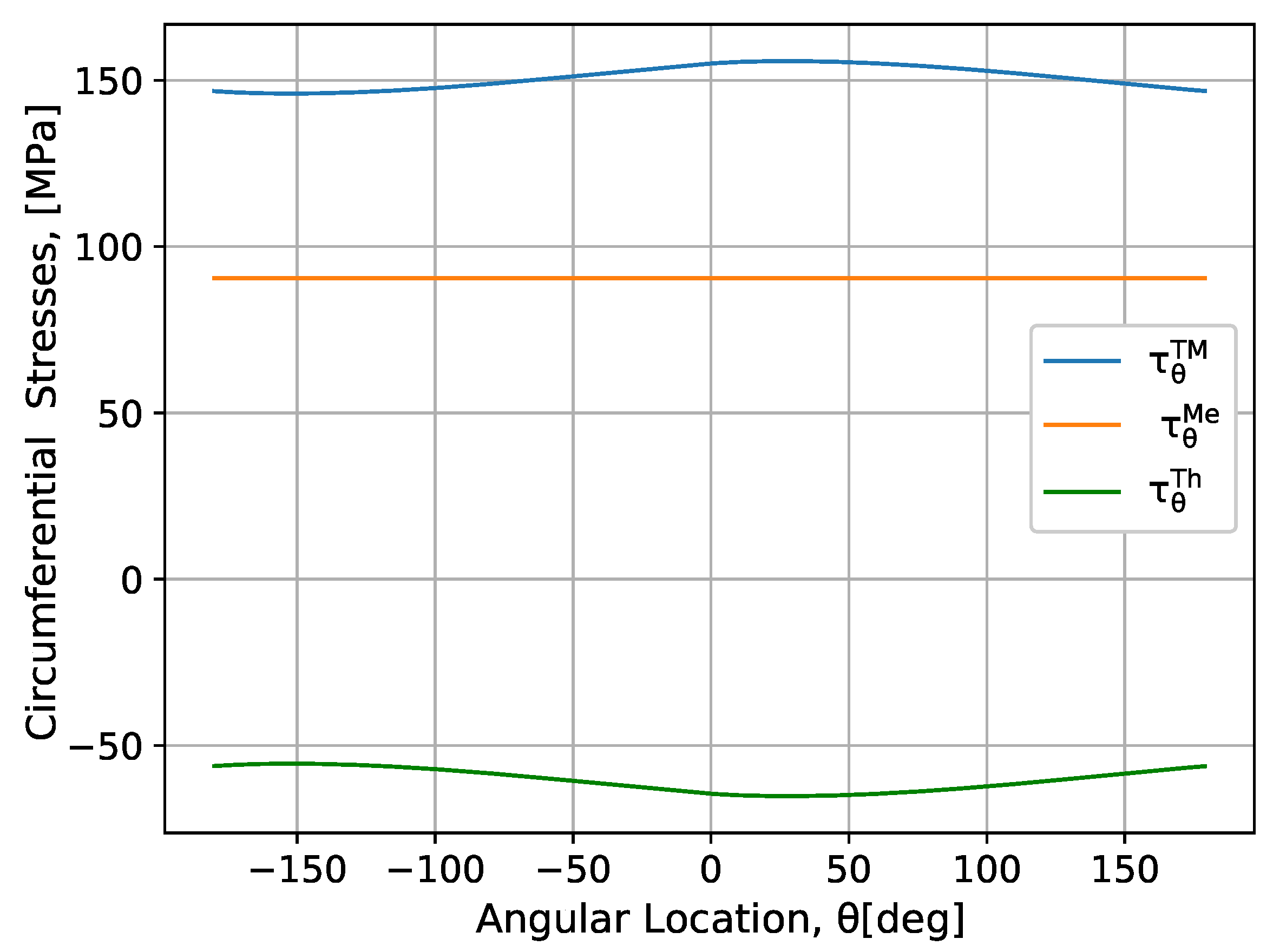
5. Thermomechanical Stress
6. The Effect of Temperature on the H13 Tool Steel Material
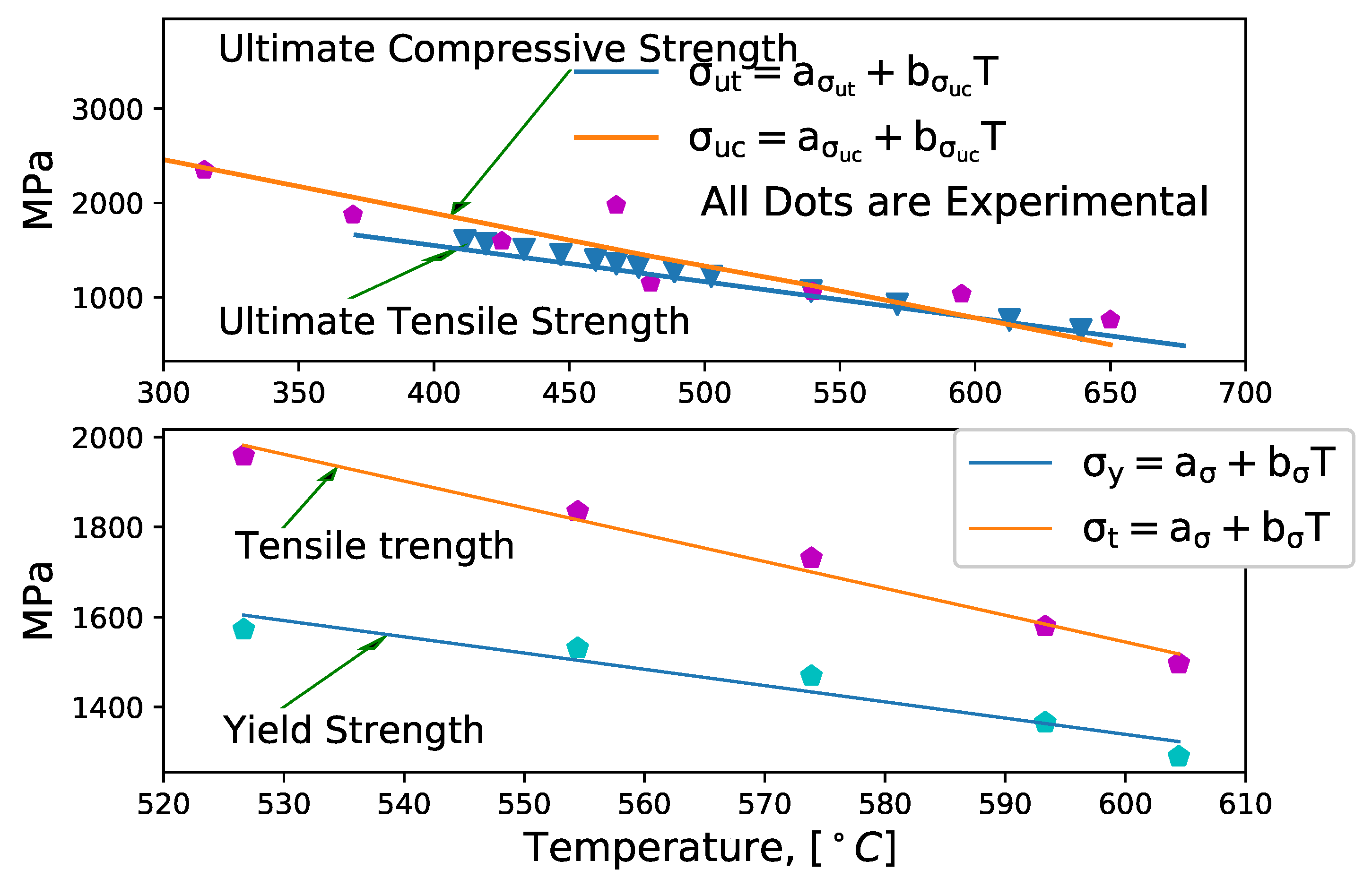
6.1. Mechanical Properties of H13 Tool Steels
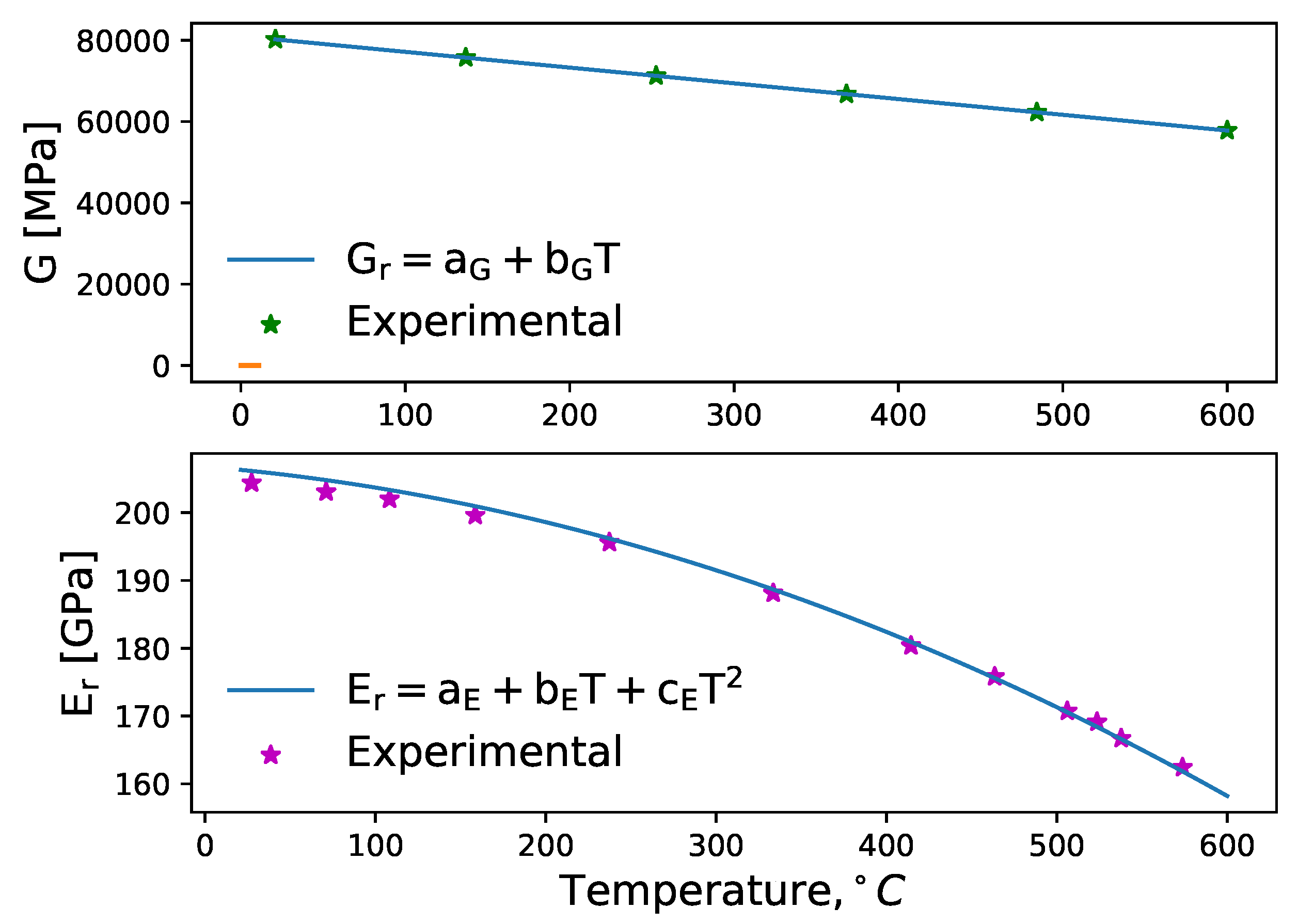
6.2. Thermal Effect on H13 Tool Steel
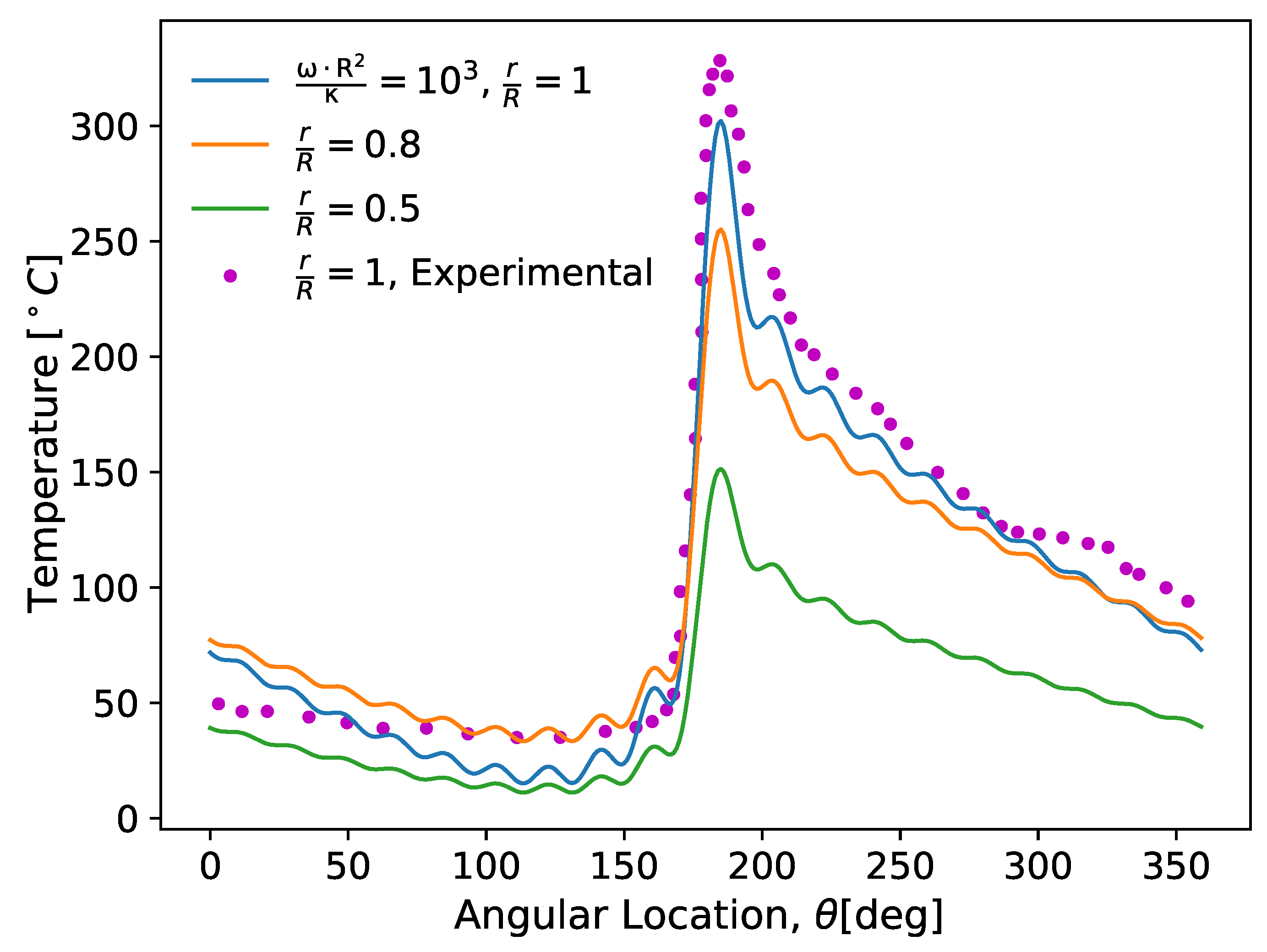
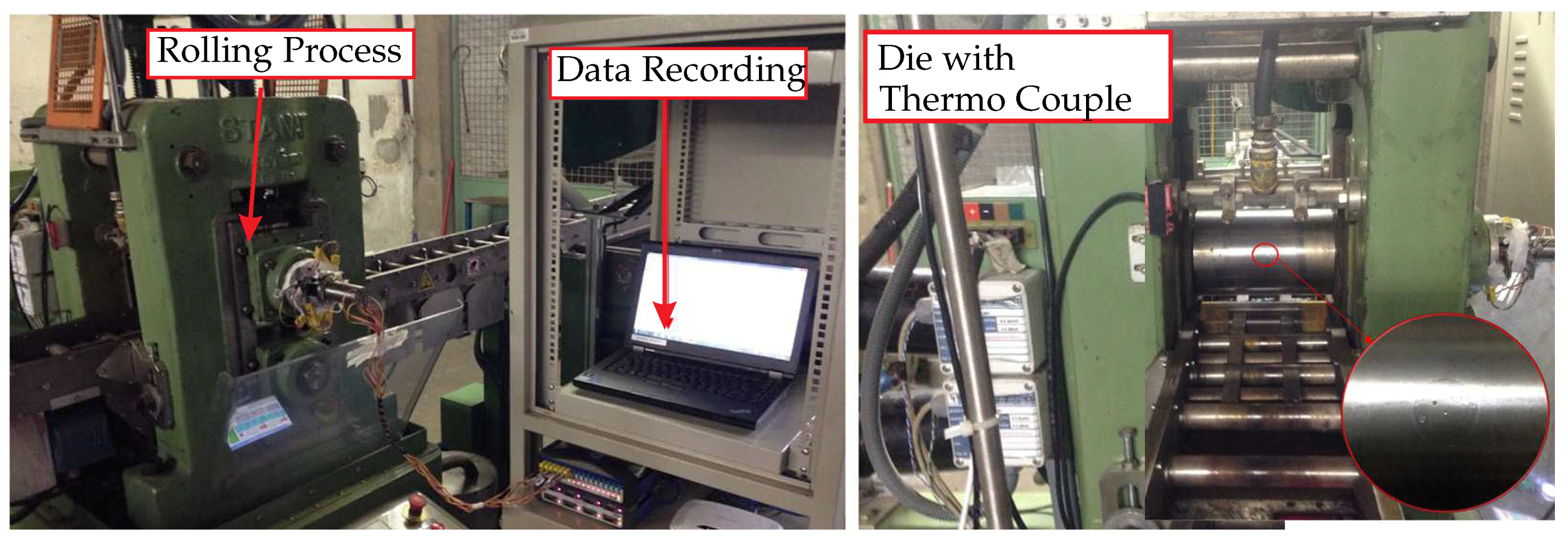
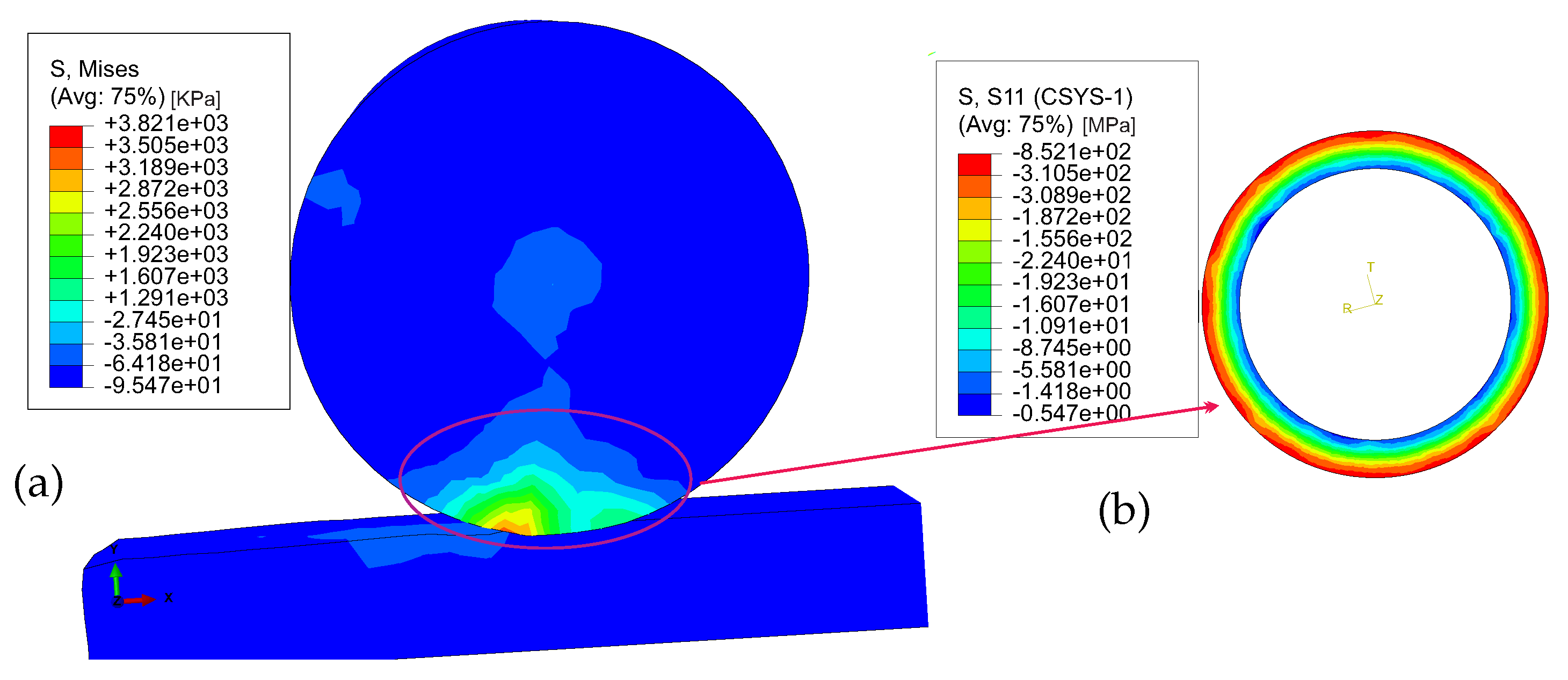
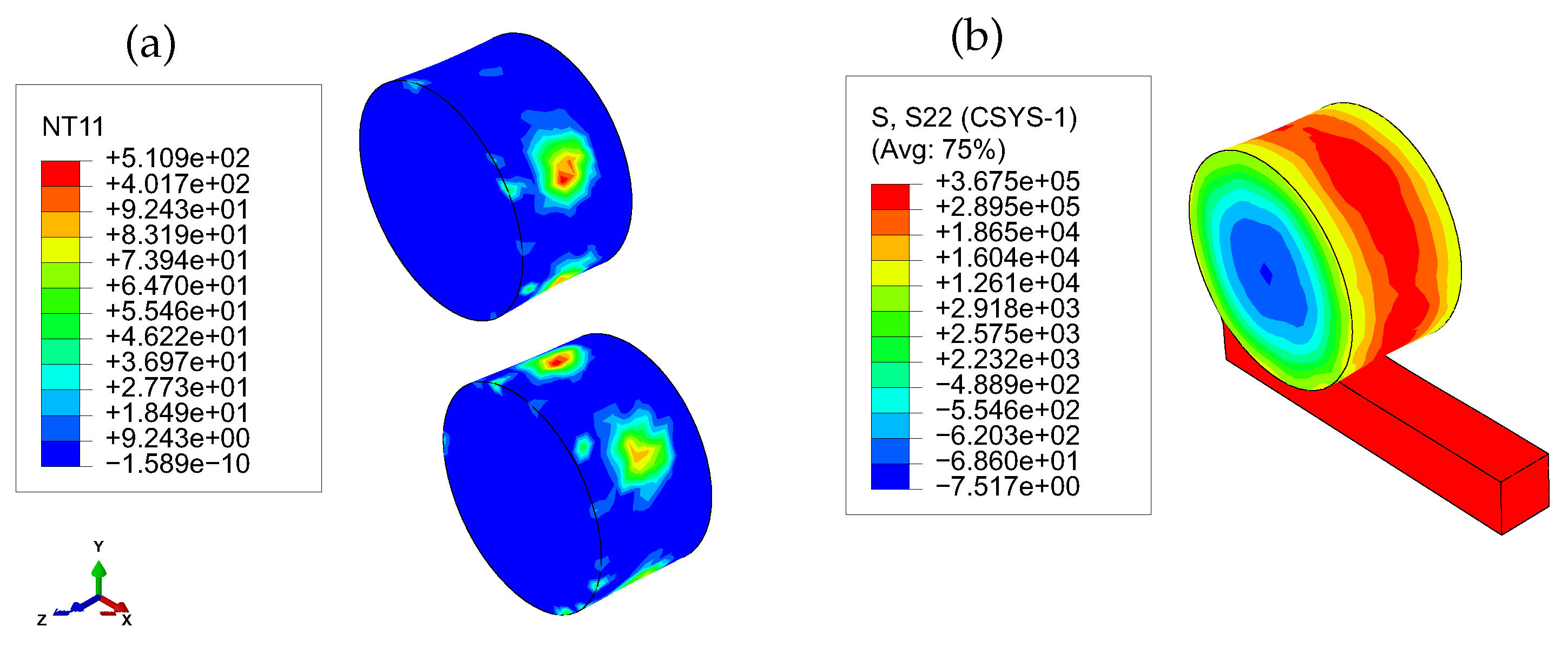
7. Finite Element Simulation
- Material property specification
- (a)
- Specifying the known H13 tool steel material properties for the rolling die
- (b)
- Specifying A36 mild steel material properties (obtained from Reference [35] for the slab)
- After the material properties are collected, the constitutive equations are implemented in ABAQUS via user-defined material model (UMAT) with the following steps:
- (a)
- A proper definition of the constitutive model,
- (b)
- A definition of the state variables,
- (c)
- Transforming the constitutive equation that perform the proper integration scheme,
- (d)
- A calculation of the Jacobian,
- (e)
- Code developing in the FORTRAN software,
- (f)
- Connecting the written code to the ABAQUS subroutine.
8. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
Appendix A.
- The thermal stress part in the angular direction , where , , , , , and are given byandwhere , t is time or temporary coordinate , and is the rotation period of a rolling die
- The thermal strain parts in the direction , where , , , , , and are given
Appendix B. Parameters in the Curve-Fit of Material Properties Used in Simulation
- (a)
- Shear modulus, GPaGPa, GPa, GPa
- (b)
- Young’s modulus, GPaGPa, GPaGPa, GPa
- (c)
- Yield strength MPa, valid only when T CMPa, MPaMPa
- (d)
- Tensile strength MPa, valid only when T CMPa, MPaMPa
- (e)
- Ultimate tensile strength MPa, valid only when T CMPa, MPaMPa
- (f)
- Ultimate compressive strength MPa, valid only when T CMPa, MPa,MPa
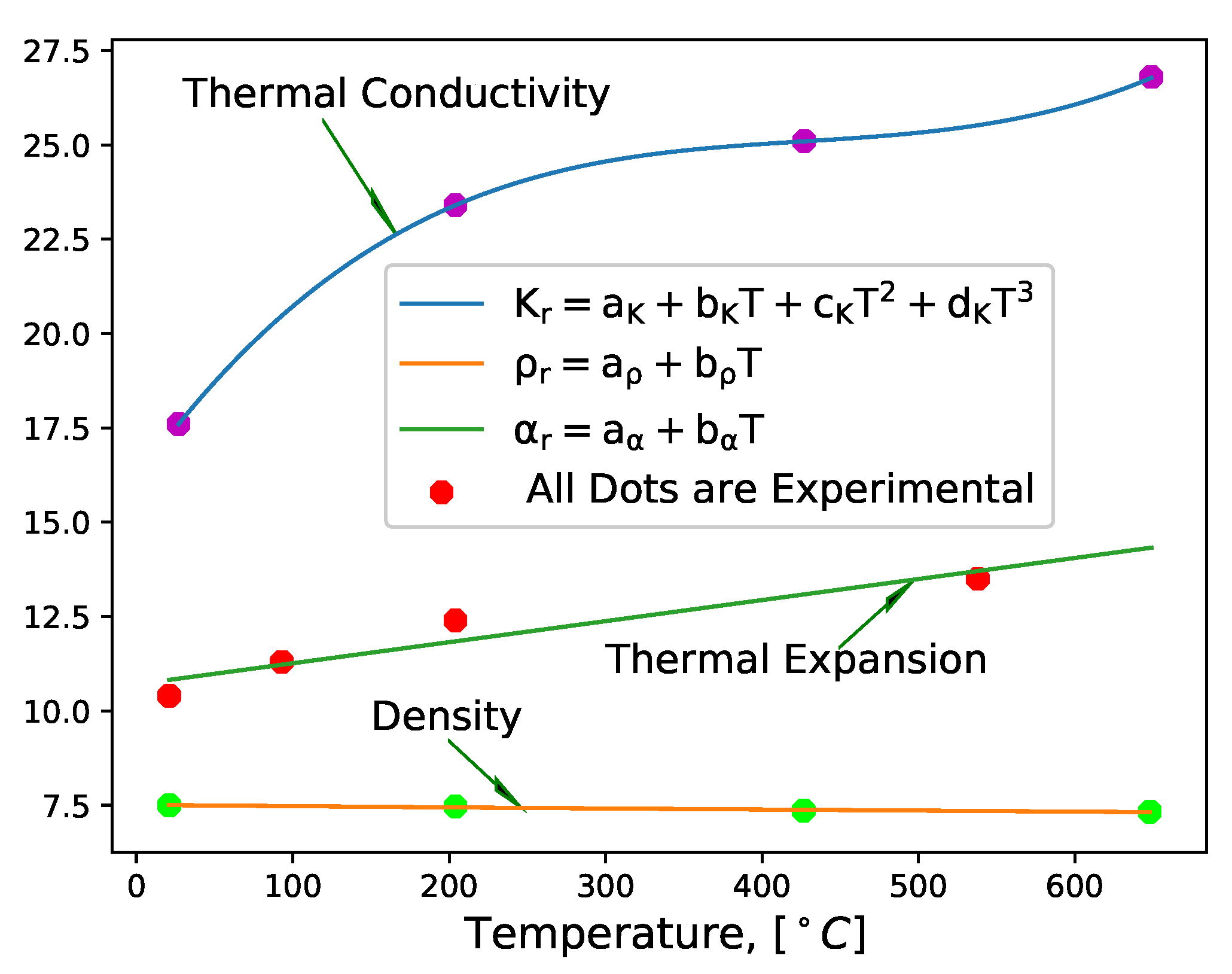
- The thermal properties of H13 steel from Figure 14
- (a)
- Density, g/ccg/cc, g/cc,g/cc
- (b)
- Thermal conductivity, W/mCW/mC, W/mCW/mCW/mCW/m C
- (c)
- Thermal expansion coefficient, mm/mm Cmm/mm Cmm/mm Cmm/mm C
References
- Shigeru, O.; Yosuke, N.; Thoshiyuki, S. Progress and Prospect of Rolling Technology; Nippon Steel Technical Report; UDC 621: Tokyo, Japan, 2012. [Google Scholar]
- Mercado-Solis, D.; Talamantes-Silva, J.; Hernandez-Rodriguez, A.; Beynon, H. Modelling surface thermal damage to hot mill rolls. Wear 2007, 263, 1560–1567. [Google Scholar] [CrossRef]
- Tseng, A.; Lin, H.; Gunderia, S.; Ni, S. Roll cooling and its relationship to roll life. Metall. Trans. A 1989, 20. [Google Scholar] [CrossRef]
- Sheikh, H. Thermal analysis of hot strip rolling using finite element and upper bound methods. Appl. Math. Modell. 2009, 33, 2187–2195. [Google Scholar] [CrossRef]
- Benasciutti, D.; Brusa, E.; Bazzaro, E. Finite elements prediction of thermal stresses in work roll of hot rolling mills. Procedia Eng. 2010, 2, 707–716. [Google Scholar] [CrossRef]
- Sonali, K. Effect of Temperature on Thermal and Mechanical Properties of High Strength Steel A325 and a490 Bolts. Master’s Thesis, Michigan State University, East Lansing, MI, USA, 22 April 2012. [Google Scholar]
- Der-Form, C. Thermal stresses in work rolls during the rolling of metal strip. J. Mate. Proc. Technol. 1999, 94, 45–51. [Google Scholar] [CrossRef]
- Galantucci, M.; Tricarico, L. Thermo-mechanical simulation of a rolling Process with an FEM approach. J. Mater. Process. Technol. 1999, 92–93, 494–501. [Google Scholar] [CrossRef]
- Dunckelmeyer, M.; Krempaszky, C.; Werner, E.; Hein, G.; Schorkhuber, K. Microstructure analysis of a banded work roll with comparison between damage appearance and thermo-mechanical considerations. In Proceedings of the International Doctoral Seminar, Bratislava, Slovakia, 16–19 May 2010. [Google Scholar]
- Sun, D.; Zhao, J.; Chen, L. Finite element analysis of temperature and crown of rolls in hot strip rolling. Appl. Mech. Mater. 2009, 16–19, 575–579. [Google Scholar] [CrossRef]
- Hongchun, L.; Jiang, Z.; Tieu, A.; Sun, W. Analysis of premature failure of work rolls in a cold strip plant. Wear 2007, 263, 1442–1446. [Google Scholar] [CrossRef]
- Sonboli, A.; Serajzadeh, S. Prediction of thermal stresses and temperature field in work rolls during hot strip rolling process. Mater. Sci. Technol. 2010, 26, 343–351. [Google Scholar] [CrossRef]
- Atack, A.; Robinson, S. An investigation into the control of thermal camber by spray cooling when hot rolling aluminum. J. Mate Proc. Technol. 1994, 45, 125–130. [Google Scholar] [CrossRef]
- Chen, W.; Samarasekera, V.; Kumar, A.; Hawbolt, B. Mathematical modeling of heat flow and deformation during rough rolling. Ironmaking Steelmaking 1993, 20, 113–125. [Google Scholar]
- Carslaw, H.; Jaeger, J. Conduction of Heat in Solids, 2nd ed.; Clarendon Press: Oxford, UK, 1959. [Google Scholar]
- Patula, E. Steady-state temperature distribution in a rotating roll subject to surface heat fluxes and conwective cooling. J. Heat Transfer 1981, 103, 36–41. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- Braga, A.; Lima, L.; GonÇalves, A.; Boccalini, M.; Souza, R. Coupled experimental-numerical analysis of Wear in hotrolling Mills. In Proceedings of the 10th International Tooling Conference, Bratislava, Slovakia, 4–7 October 2016. [Google Scholar]
- Raudensky, M.; Horsky, J.; Ondrouskova, J.; Vervaet, B. Measurement of thermal load on working rolls during Hot rolling. Steel Res. Int. 2013, 84, 269–275. [Google Scholar] [CrossRef]
- Zahradník, R.; Hrabovský. Study of the Work Roll Cooling in Hot Rolling Process with Regard on Service Life; Metallurgia Italiana. Available online: http://www.1391-Article%20Text-5287-1-10-20150225.pdf (accessed on 27 February 2019).
- Tseng, A.; Tong, V.; Raudensky, M.; Chen, C. Thermal Expansion and Crown Evaluations of Rolls in Rolling Processes. Steel Res. 1996, 67, 188–199. [Google Scholar] [CrossRef]
- Pérez, A.; Corral, L.; Fuentes, R.; Colás, R. Computer simulation of the thermal behaviour of a work roll during hot rolling of steel strip. J. Mater. Process. Technol. 2004, 153, 894–899. [Google Scholar] [CrossRef]
- Richman, M. Book Review: Juran’s Quality Handbook, 7th ed.; McGraw-Hill: New York, NY, USA, 2016; ISBN 9780070331761. [Google Scholar]
- Robert, W. Hot Rolling of Steel Manufacturing Engineering and Materials Processing; Marcel Dekker: New York, NY, USA, 1983. [Google Scholar]
- Mesay, A.; Altenbach, H. Numerical modeling rolling contact problem and elasticity deformation of rolling die under hot milling. Metals 2019, 9, 226. [Google Scholar] [CrossRef]
- Jeswiet, J.; Rice, W. The design of a sensor for measuring normal pressure and friction stress in the roll gap during cold rolling. In Proceedings of the 10th North American Manufacturing Research Conference Proceedings, Hamilton, ON, Canada, 24–25 May 1982; pp. 130–134. [Google Scholar]
- Weisz-Patrault, D.; Ehrlacher, A.; Legrand, N. A new sensor for the evaluation of contact stress by inverse analysis during steel strip rolling. J. Mater. Process. Technol. 2011, 211, 1500–1509. [Google Scholar] [CrossRef]
- Goswami, T. Creep-Fatigue Behaviour and Life Prediction. Ph.D. Thesis, University of Wollongong, Wollongong, Australia, 1998. Available online: https://ro.uow.edu.au/cgi/viewcontent.cgi? (accessed on 26 March 2019).
- Davis, J. Metal Handbook Desk Edition; ASM International: New York, NY, USA, 1998; pp. 265–289. [Google Scholar]
- AZoM. Tool Steels Molybdenum High-Speed Steels; AZO Materials: London, UK, 2016. [Google Scholar]
- Engerg, G.; Larsson, L. Elevated-Temperature Low Cycle and Thermomechanical Fatigue Properies of AISI H13 Hotwork Tool Steel; ASTM STP 942; ASTM International: West Conshohocken, PA, USA, 1988; pp. 565–577. [Google Scholar] [CrossRef]
- Steiner, R. ASM Handbook, Volume 1: Properties and Selection: Irons, Steels; ASM International: West Conshohocken, PA, USA, 1990; ISBN 978-0-87170-377-4. [Google Scholar]
- Harvey, D. Editor, Engineering Properties of Steels; American Society for Metals: Metals Park, OH, USA, 1982. [Google Scholar]
- Hudson. Tool Steel and High Speed Steel for Manufacturers; Tool Steel Corporation: London, UK; Available online: https://www.hudsontoolsteel.com/technical-data/steelH3 (accessed on 27 February 2019).
- Mesay, T. Modelling plastic deformation of A36 mild steel under extrusion process. Int. J. Eng. Res. 2014, 3, 1551–1555. [Google Scholar]
- Bower, A. EN234FEA. Computational Methods in Structural and Solid Mechanics; School of Engineering Brown University: Manchester, UK, 2012; Available online: http://www.brown.edu/Departments/Engineering/Courses/En2340 (accessed on 27 February 2019).

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tolcha, M.A.; Lemu, H.G. Modeling Thermomechanical Stress with H13 Tool Steel Material Response for Rolling Die under Hot Milling. Metals 2019, 9, 495. https://doi.org/10.3390/met9050495
Tolcha MA, Lemu HG. Modeling Thermomechanical Stress with H13 Tool Steel Material Response for Rolling Die under Hot Milling. Metals. 2019; 9(5):495. https://doi.org/10.3390/met9050495
Chicago/Turabian StyleTolcha, Mesay Alemu, and Hirpa Gelgele Lemu. 2019. "Modeling Thermomechanical Stress with H13 Tool Steel Material Response for Rolling Die under Hot Milling" Metals 9, no. 5: 495. https://doi.org/10.3390/met9050495
APA StyleTolcha, M. A., & Lemu, H. G. (2019). Modeling Thermomechanical Stress with H13 Tool Steel Material Response for Rolling Die under Hot Milling. Metals, 9(5), 495. https://doi.org/10.3390/met9050495




