Tensile Creep Characterization and Prediction of Zr-Based Metallic Glass at High Temperatures
Abstract
:1. Introduction
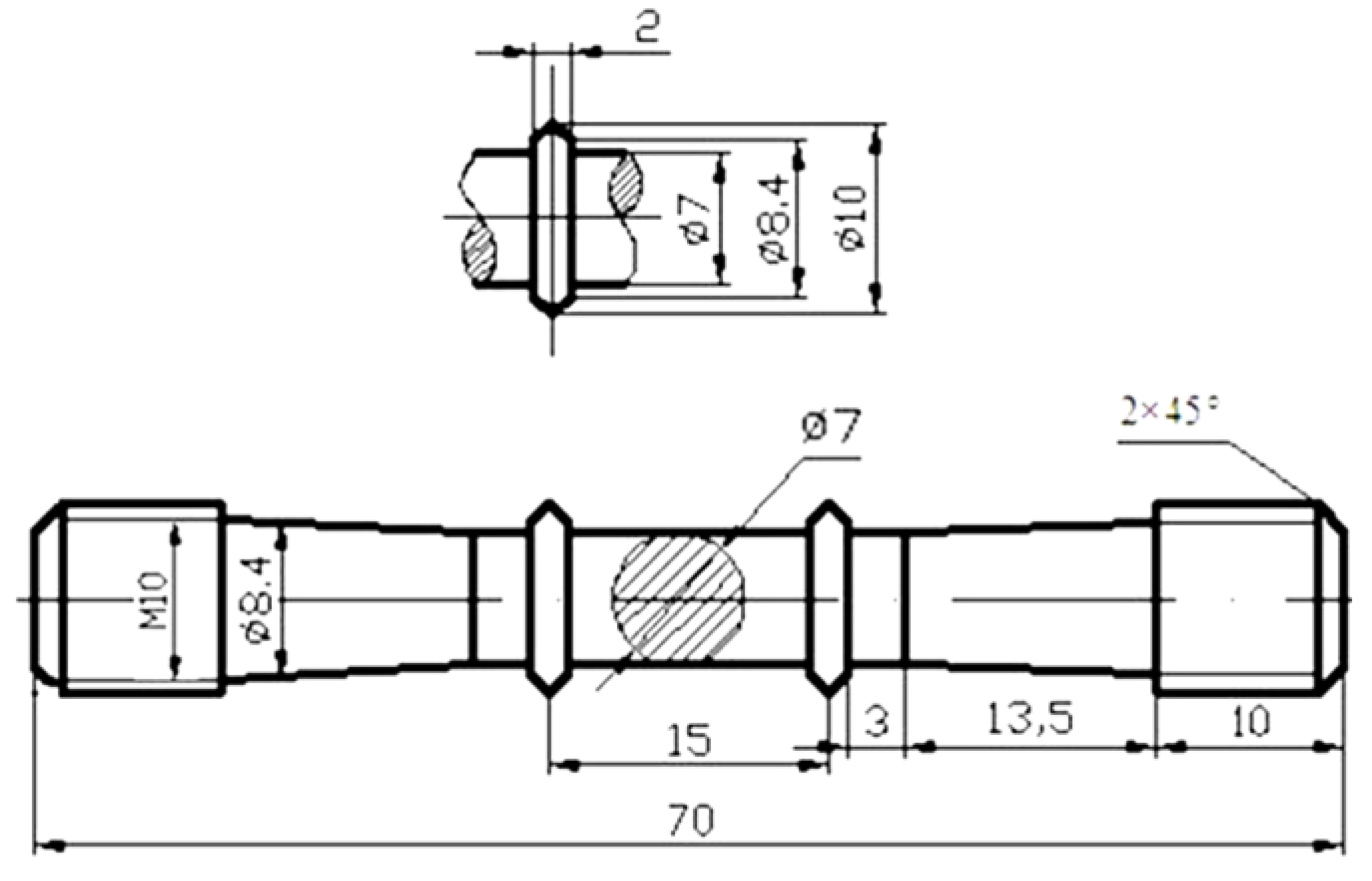
2. Materials and Methods
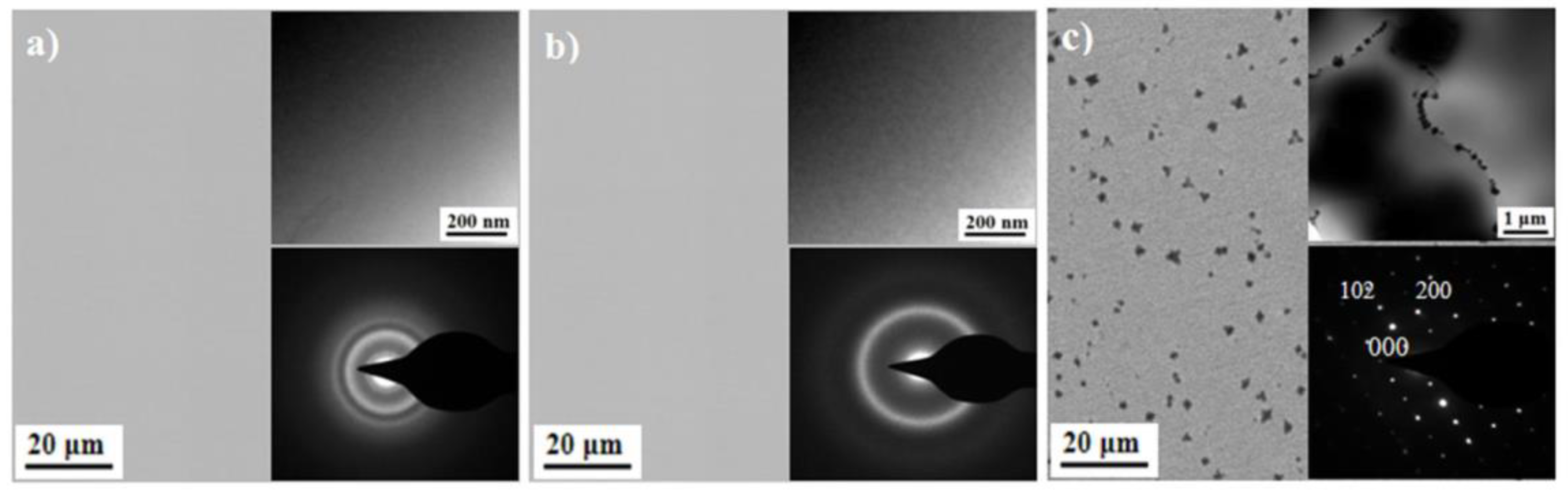
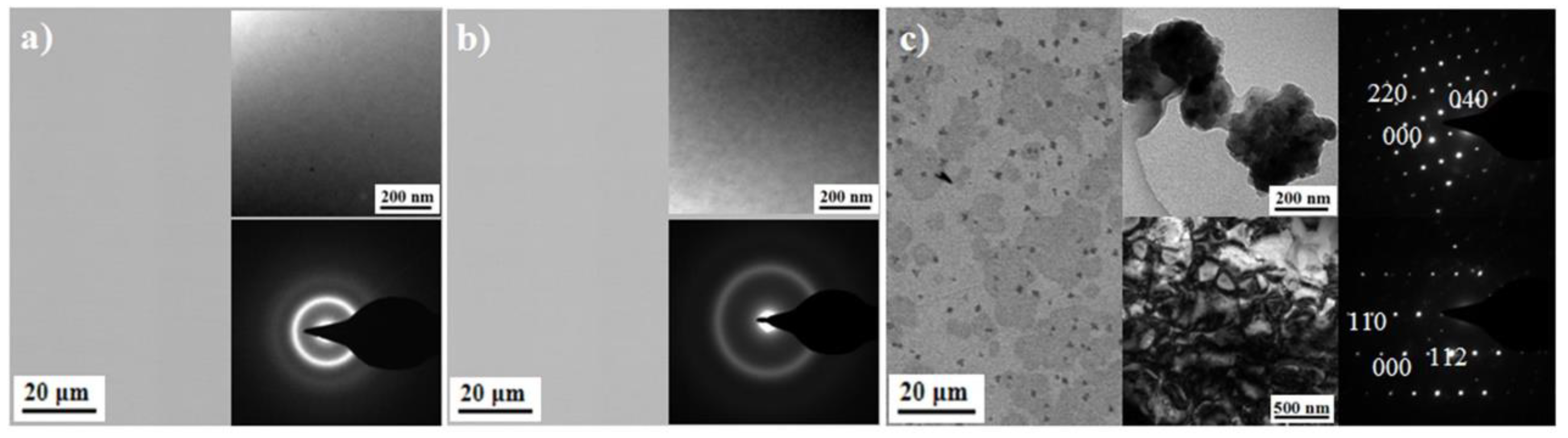
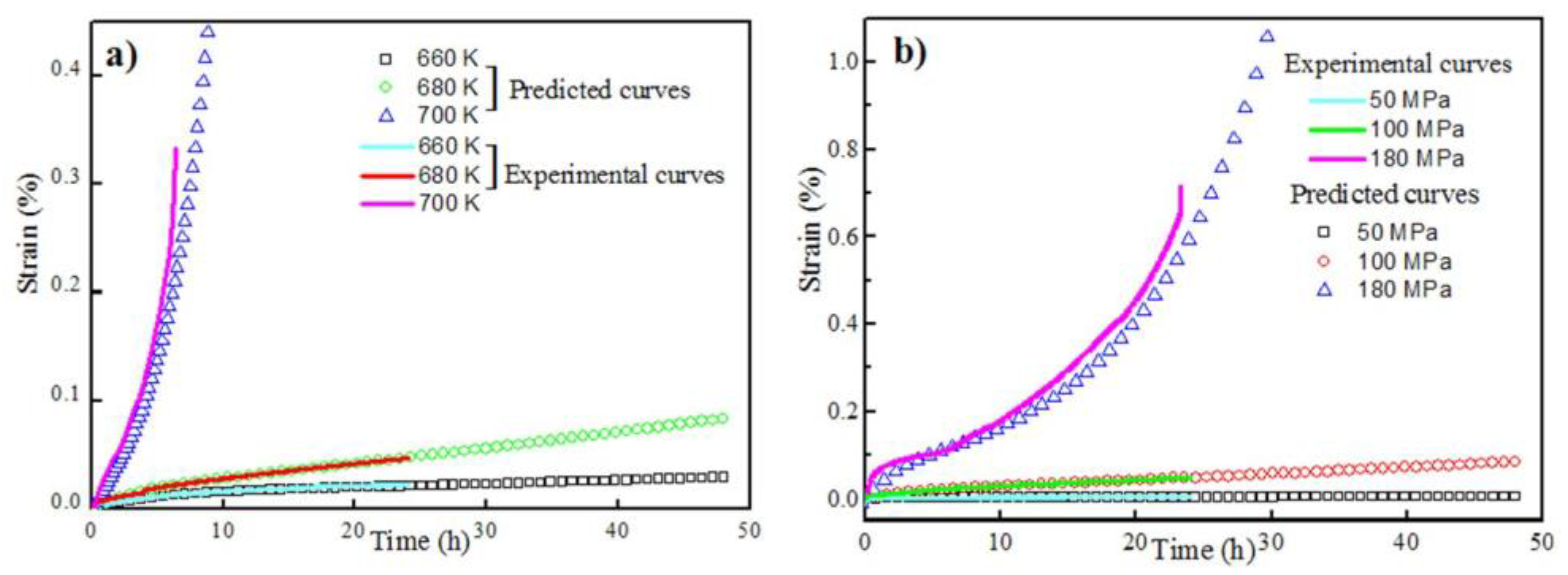
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Johnson, W.L. Bulk Glass-Forming Metallic Alloys: Science and Technology. MRS Bull. 1999, 24, 42–56. [Google Scholar] [CrossRef]
- Zhang, Z.F.; He, G.; Eckert, J.; Schultz, L. Fracture Mechanisms in Bulk Metallic Glassy Materials. Phys. Rev. Lett. 2003, 91, 045505. [Google Scholar] [CrossRef] [PubMed]
- Wang, G.; Fan, H.B.; Huang, Y.J.; Shen, J.; Chen, Z.H. A new TiCuHfSi bulk metallic glass with potential for biomedical applications. Mater. Des. 2014, 54, 251–255. [Google Scholar] [CrossRef]
- Liang, D.D.; Wei, X.S.; Chang, C.T.; Li, J.W.; Shen, J. Effect of W addition on the glass forming ability and mechanical properties of Fe-based metallic glass. J. Alloy. Compd. 2018, 731, 1146–1150. [Google Scholar] [CrossRef]
- Li, J.C.; Chen, X.W.; Huang, F.L. On the mechanical properties of particle reinforced metallic glass matrix composites. J. Alloy. Compd. 2018, 737, 271–294. [Google Scholar] [CrossRef]
- Huang, Y.J.; Shen, J.; Chiu, Y.L.; Chen, J.J.J.; Sun, J.F. Indentation creep of an Fe-based bulk metallic glass. Intermetallics 2009, 17, 190–194. [Google Scholar] [CrossRef]
- Yu, P.F.; Feng, S.D.; Xu, G.S.; Guo, X.L.; Wang, Y.Y.; Zhao, W.; Qi, L.; Li, G.; Liaw, P.K.; Liu, R.P. Room-temperature creep resistance of Co-based metallic glasses. Scr. Mater. 2014, 90–91, 45–48. [Google Scholar] [CrossRef]
- Gong, P.; Jin, J.S.; Deng, L.; Wang, S.B.; Gu, J.L.; Yao, K.F.; Wang, X.Y. Room temperature nanoindentation creep behavior of TiZrHfBeCu(Ni) high entropy bulk metallic glasses. Mater. Sci. Eng. A 2017, 688, 174–179. [Google Scholar] [CrossRef]
- Zhang, M.; Chen, Y.; Wei, D.; Dai, L.H. Extraordinary creep relaxation time in a La-based metallic glass. J. Mater. Sci. 2018, 53, 2956–2964. [Google Scholar] [CrossRef]
- Zhang, T.H.; Ye, J.H.; Feng, Y.H.; Ma, Y. On the spherical nanoindentation creep of metallic glassy thin films at room temperature. Mater. Sci. Eng. A 2017, 685, 294–299. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.H.; Huang, J.C.; Du, X.H.; Wang, X. Time-dependent creep behavior of amorphous ZrCu and nanocrystalline Zr thin films—A comparison. Intermetallics 2016, 68, 101–106. [Google Scholar] [CrossRef]
- Wang, C.; Cao, Q.P.; Wang, X.D.; Zhang, D.X.; Qu, S.X.; Jiang, J.Z. Time-dependent shear transformation zone in thin film metallic glasses revealed by nanoindentation creep. J. Alloy Compd. 2017, 696, 239–245. [Google Scholar] [CrossRef]
- Qiao, J.C.; Pelletier, J.M.; Blandin, J.J.; Gravier, S. High temperature deformation in a lanthanum based bulk metallic glass showing a pronounced secondary relaxation. Mater. Sci. Eng. A 2013, 586, 57–61. [Google Scholar] [CrossRef]
- Zhang, C.; Qiao, J.C.; Pelletier, J.M.; Yao, Y. Thermal activation in the Zr65Cu18Ni7Al10 metallic glass by creep deformation and stress relaxation. Scr. Mater. 2016, 113, 180–184. [Google Scholar] [CrossRef]
- Kassner, M.E.; Smith, K.; Eliasson, V. Creep in amorphous metals. J. Mater. Res. Technol. 2015, 4, 100–107. [Google Scholar] [CrossRef]
- Li, F.C.; Xie, Y.; Song, M.; Ni, S.; Guo, S.F.; Liao, X.Z. A detailed appraisal of the stress exponent used for characterizing creep behavior in metallic glasses. Mater. Sci. Eng. A 2016, 654, 53–59. [Google Scholar] [CrossRef]
- Galano, M.; Rubiolo, G.H. Creep behaviour of a FeSi-base metallic glass containing nanocrystals. Scr. Mater. 2003, 48, 617–622. [Google Scholar] [CrossRef]
- Omprakash, C.M.; Kumar, A.; Srivathsa, B.; Satyanarayana, D.V.V. Prediction of creep curves of high temperature alloys using θ projection concept. Proccdia Eng. 2013, 55, 756–759. [Google Scholar] [CrossRef]
- Alipour, R.; Farokhi Nejad, A. Creep behaviour characterisation of a ferritic steel alloy based on the modified theta-projection data at an elevated temperature. Int. J. Mater. Res. 2016, 107, 406–412. [Google Scholar] [CrossRef]
- Xie, L.J.; Ning, D.; Yang, Y.Z. Experimental study on creep characterization and lifetime estimation of RPV material at 723-1023 K. J. Mater. Eng. Perform. 2017, 26, 643–652. [Google Scholar] [CrossRef]
- Kim, W.G.; Kim, S.H.; Lee, C.B. Long-term creep characterization of Gr.91 steel by modified creep constitutive equations. Metall. Mater. Int. 2011, 17, 497–504. [Google Scholar] [CrossRef]
- Lin, Y.C.; Xia, Y.C.; Ma, X.S.; Jiang, Y.Q.; Chen, M.S. High temperature creep behavior of Al-Cu-Mg alloy. Mater. Sci. Eng. A 2012, 550, 125–130. [Google Scholar] [CrossRef]
- Lin, Y.C.; Xia, Y.C.; Chen, M.S.; Jiang, Y.Q.; Li, L.T. Modeling the creep behavior of 2024-T3 Al alloy. Comp. Mater. Sci. 2013, 67, 243–248. [Google Scholar] [CrossRef]
- Sun, Y.J.; Qu, D.D.; Huang, Y.J.; Liss, K.-D.; Wei, X.S.; Xing, D.W.; Shen, J. Zr–Cu–Ni–Al bulk metallic glasses with superhigh glass-forming ability. Acta Mater. 2009, 57, 1290–1299. [Google Scholar] [CrossRef]
- Argon, A.S. Plastic deformation in metallic glasses. Acta Metall. 1979, 27, 47–58. [Google Scholar] [CrossRef]
- Johnson, W.L.; Samwer, K. A universal criteria for plastic yielding of metallic glasses with a (T/Tg)2/3 temperature dependence. Phys. Rev. Lett. 2005, 95, 195501. [Google Scholar] [CrossRef] [PubMed]
- Schuh, C.A.; Hufnagel, T.C.; Ramamurty, U. Mechanical behavior of amorphous alloys. Acta Mater. 2007, 55, 4067–4109. [Google Scholar] [CrossRef]
- Huang, Y.J.; Shen, J.; Sun, J.F.; Zhang, Z.F. Enhanced strength and plasticity of a Ti-based metallic glass at cryogenic temperatures. Mater. Sci. Eng. A 2008, 498, 203–207. [Google Scholar] [CrossRef]
- Jiang, W.H.; Jiang, F.; Liu, F.X.; Choo, H.; Liaw, P.K.; Qiu, K.Q. Temperature dependence of serrated flows in compression in a bulk-metallic glass. Appl. Phys. Lett. 2006, 89, 261909. [Google Scholar] [CrossRef]
- Yu, P.; Chan, K.C.; Chen, W.; Xia, L. Low-temperature mechanical properties of Ce68Al10Cu20Co2 bulk metallic glass. Philos. Mag. Lett. 2011, 91, 70–77. [Google Scholar] [CrossRef]
- Jiang, W.C.; Zhang, Y.C.; Luo, Y.; Gong, J.M.; Tu, S.T. Creep analysis of solid oxide fuel cell with bonded compliant steel design. J. Power Source 2013, 243, 913–918. [Google Scholar] [CrossRef]
- Yang, B.; Xuan, F.Z. Creep behavior of subzones in a CrMoV weldment characterized by the in-situ creep test with miniature specimens. Mater. Sci. Eng. A 2018, 723, 148–156. [Google Scholar] [CrossRef]
- Zhao, J.F.; Gong, J.D.; Saboo, A.; Dunand, D.C.; Olson, G.B. Dislocation-based modeling of long-term creep behaviors of Grade 91 steels. Acta Mater. 2018, 149, 19–28. [Google Scholar] [CrossRef]
- Miresmaeili, S.M.; Nami, B. Impression creep behavior of Al–1.9%Ni–1.6%Mn–1%Mg alloy. Mater. Des. 2014, 56, 286–290. [Google Scholar] [CrossRef]
- Wilshire, B.; Burt, H. Creep data prediction for Aluminium air frame alloy. Mater. Sci. Forum 2003, 426–432, 261–266. [Google Scholar] [CrossRef]
- Tian, S.G.; Lv, X.X.; Yu, H.C.; Wang, Q.; Jiao, Z.H.; Sun, H.F. Creep behavior and deformation feature of TiAl-Nb alloy with various states at high temperature. Mater. Sci. Eng. A 2016, 651, 490–498. [Google Scholar] [CrossRef]
- Evans, R.W. A constitutive model for the high-temperature creep of particle-hardened alloys based on the θ projection method. Proc. R. Soc. A Math. Phys. 2000, 456, 835–868. [Google Scholar] [CrossRef]
- Williams, S.J.; Bache, M.R.; Wilshire, B. 25 Year Perspective Recent developments in analysis of high temperature creep and creep fracture behaviour. Mater. Sci. Technol. 2010, 26, 1329–1336. [Google Scholar] [CrossRef]
- Zheng, W. Dynamic Mechanical Behavior of ZrCuNiAl Bulk Metallic Glass. Ph.D. Thesis, Harbin Institute of Technology, Harbin, China, 2011. [Google Scholar]
- Kim, J.J.; Choi, Y.; Suresh, S.; Argon, A.S. Nanocrystallization during nanoindentation of a bulk amorphous metal alloy at room temperature. Science 2002, 295, 654–657. [Google Scholar] [CrossRef] [PubMed]
- Na, Y.S.; Lee, J.H. Interpretation of viscous deformation of bulk metallic glasses based on the Nabarro–Herring creep model. J. Mater. Proc. Technol. 2007, 187–188, 786–790. [Google Scholar] [CrossRef]
- Argon, A.S.; Shi, L.T. Development of visco-plastic deformation in metallic glasses. Acta Metall. 1983, 31, 499–507. [Google Scholar] [CrossRef]
- Kato, H.; Kawamura, Y.; Chen, H.S.; Inoue, A. A fictive stress model calculation of nonlinear viscoelastic behaviors in a Zr-based glassy alloy: Stress growth and relaxation. J. Appl. Phys. 2000, 39, 5184–5187. [Google Scholar] [CrossRef]
- Khonik, V.A. The kinetics of irreversible structural relaxation and homogeneous plastic flow of metallic glasses. Phys. Status Solidi A 2000, 177, 173–189. [Google Scholar] [CrossRef]
- Spaepen, F.; Turnbull, D. A mechanism for the flow and fracture of metallic glasses. Scr. Metall. Mater. 1974, 8, 563–568. [Google Scholar] [CrossRef]
- Lund, A.C.; Schuh, C.A. The mohr-coulomb criterion from unit shear processes in metallic glass. Intermetallics 2004, 12, 1159–1165. [Google Scholar] [CrossRef]
- Greer, A.L.; Cheng, Y.Q.; Ma, E. Shear bands in metallic glasses. Mater. Sci. Eng. R Rep. 2013, 74, 71–132. [Google Scholar] [CrossRef]
- Li, J.; Spaepen, F.; Hufnagel, T.C. Nanometre-scale defects in shear bands in a metallic glass. Philos. Mag. A 2002, 82, 2623–2630. [Google Scholar] [CrossRef]
- Li, J.; Wang, Z.L.; Hufnagel, T.C. Characterization of nanometer-scale defects in metallic glasses by quantitative high-resolution transmission electron microscopy. Phys. Rev. B 2002, 65, 144201. [Google Scholar] [CrossRef]
- Nieh, T.G.; Wadsworth, J. Homogeneous deformation of bulk metallic glasses. Scr. Mater. 2006, 54, 387–392. [Google Scholar] [CrossRef]
- Yavari, A.R.; Moulec, A.L.; Inoue, A.; Nishiyama, N.; Lupu, N.; Matsubara, E.; Botta, W.J.; Vaughan, G.; Michiel, M.D.; Kvick, A. Excess free volume in metallic glasses measured by X-ray diffraction. Acta Mater. 2005, 53, 1611–1619. [Google Scholar] [CrossRef]
- Jiang, W.H.; Atzmon, M. Mechanically-assisted nanocrystallization and defects in amorphous alloys: A high-resolution transmission electron microscopy study. Scr. Mater. 2006, 54, 333–336. [Google Scholar] [CrossRef]
- Spaepen, F. A microscopic mechanism for steady state inhomogeneous flow in metallic glasses. Acta Metall. 1977, 25, 407–415. [Google Scholar] [CrossRef] [Green Version]
- Han, Z.; Wu, W.F.; Li, Y.; Wei, Y.G.; Gao, H.J. An instability index of shear band for plasticity in metallic glasses. Acta Mater. 2009, 57, 1367–1372. [Google Scholar] [CrossRef]
- Ghidelli, M.; Idrissi, H.; Gravier, S.; Blandin, J.-J.; Raskin, J.-P.; Schryvers, D.; Pardoen, T. Homogeneous flow and size dependent mechanical behavior in highly ductile Zr65Ni35 metallic glass films. Acta Mater. 2017, 131, 246–259. [Google Scholar] [CrossRef]
- Huang, Y.J.; Shen, J.; Sun, J.F. Bulk metallic glasses: Smaller is softer. Appl. Phys. Lett. 2007, 90, 081919. [Google Scholar] [CrossRef]
- Huang, Y.J.; Chiu, Y.L.; Shen, J.; Sun, Y.; Chen, J.J.J. Mechanical performance of metallic glasses during nanoscratch tests. Intermetallics 2010, 18, 1056–1061. [Google Scholar] [CrossRef]
- Huang, Y.J.; Shen, J.; Chen, J.J.J.; Sun, J.F. Critical cooling rate and thermal stability for a Ti–Zr–Ni–Cu–Be metallic glass. J. Alloy. Compd. 2009, 477, 920–924. [Google Scholar] [CrossRef]

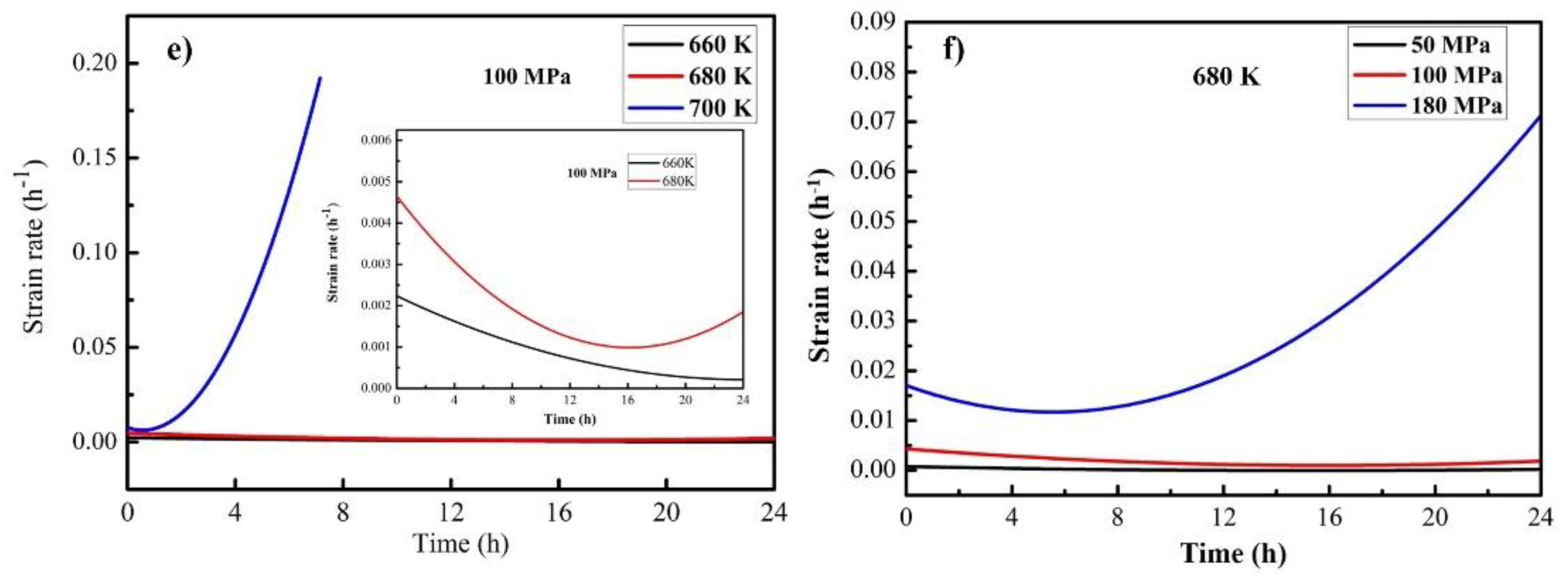


| 660 K 100 MPa σ/σy = 0.0931 | 680 K 100 MPa σ/σy = 0.1127 | 700 K 100 MPa σ/σy = 0.1269 | |||||||||
| θ1 | θ2 | θ3 | θ4 | θ1 | θ2 | θ3 | θ4 | θ1 | θ2 | θ3 | θ4 |
| 0.00954 | 0.2551 | 0.0714 | 0.007342 | 0.01382 | 0.3183 | 0.0595 | 0.015 | 0.01753 | 0.3667 | 0.04207 | 0.3271 |
| 680 K 50 MPa σ/σy = 0.0564 | 680 K 100 MPa σ/σy = 0.1127 | 680 K 180 MPa σ/σy = 0.2029 | |||||||||
| θ1 | θ2 | θ3 | θ4 | θ1 | θ2 | θ3 | θ4 | θ1 | θ2 | θ3 | θ4 |
| 0.01467 | 0.2491 | 0.0459 | 0.001464 | 0.01682 | 0.3183 | 0.0565 | 0.015 | 0.07778 | 0.4358 | 0.05218 | 0.09878 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Pan, D.; Shi, X.; Huttula, M.; Cao, W.; Huang, Y. Tensile Creep Characterization and Prediction of Zr-Based Metallic Glass at High Temperatures. Metals 2018, 8, 457. https://doi.org/10.3390/met8060457
Wang G, Pan D, Shi X, Huttula M, Cao W, Huang Y. Tensile Creep Characterization and Prediction of Zr-Based Metallic Glass at High Temperatures. Metals. 2018; 8(6):457. https://doi.org/10.3390/met8060457
Chicago/Turabian StyleWang, Gang, Daoyuan Pan, Xinying Shi, Marko Huttula, Wei Cao, and Yongjiang Huang. 2018. "Tensile Creep Characterization and Prediction of Zr-Based Metallic Glass at High Temperatures" Metals 8, no. 6: 457. https://doi.org/10.3390/met8060457
APA StyleWang, G., Pan, D., Shi, X., Huttula, M., Cao, W., & Huang, Y. (2018). Tensile Creep Characterization and Prediction of Zr-Based Metallic Glass at High Temperatures. Metals, 8(6), 457. https://doi.org/10.3390/met8060457





