Formation of Deformation-Induced Products in a Metastable-β Titanium Alloy during High Temperature Compression
Abstract
1. Introduction
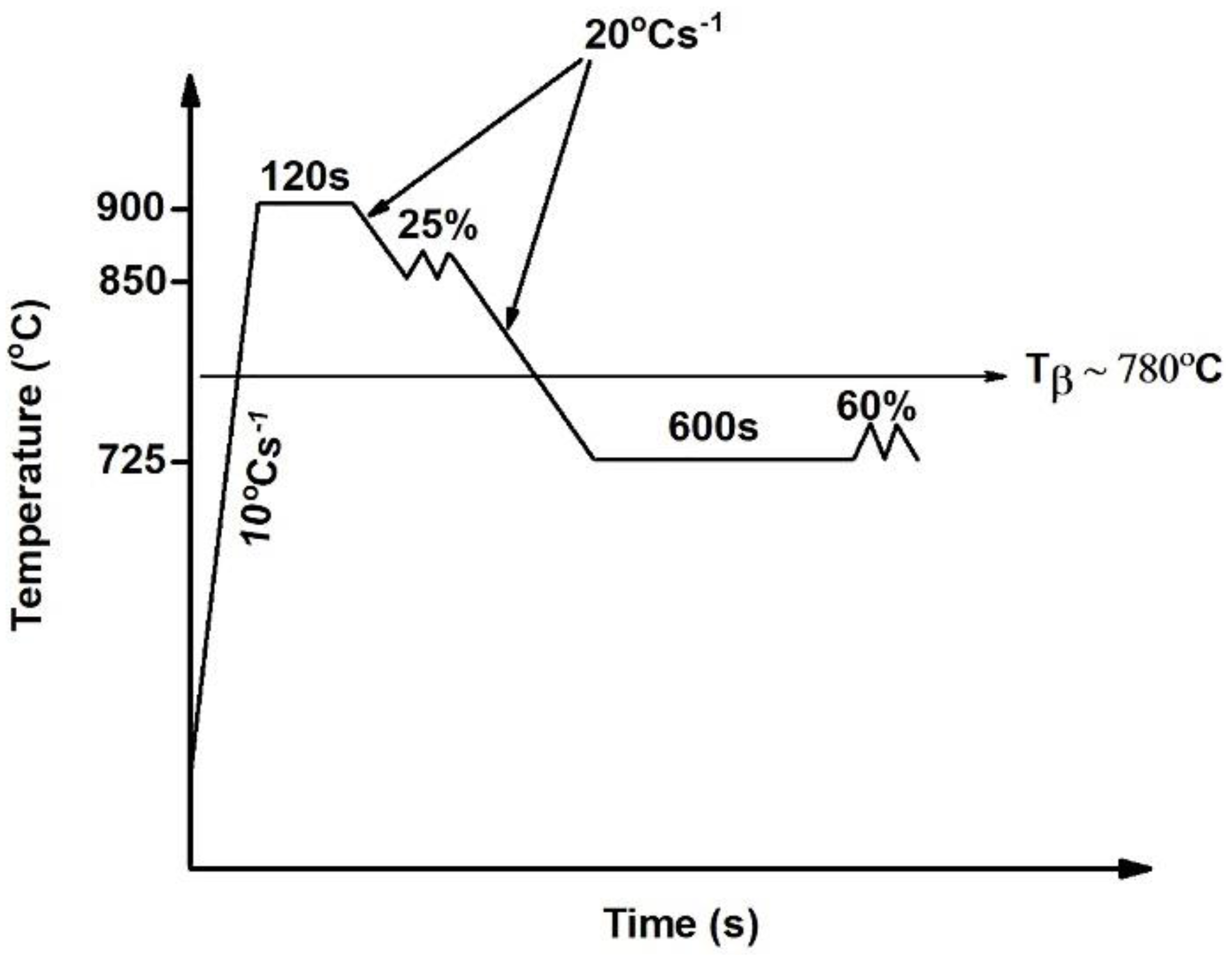
2. Materials and Methods
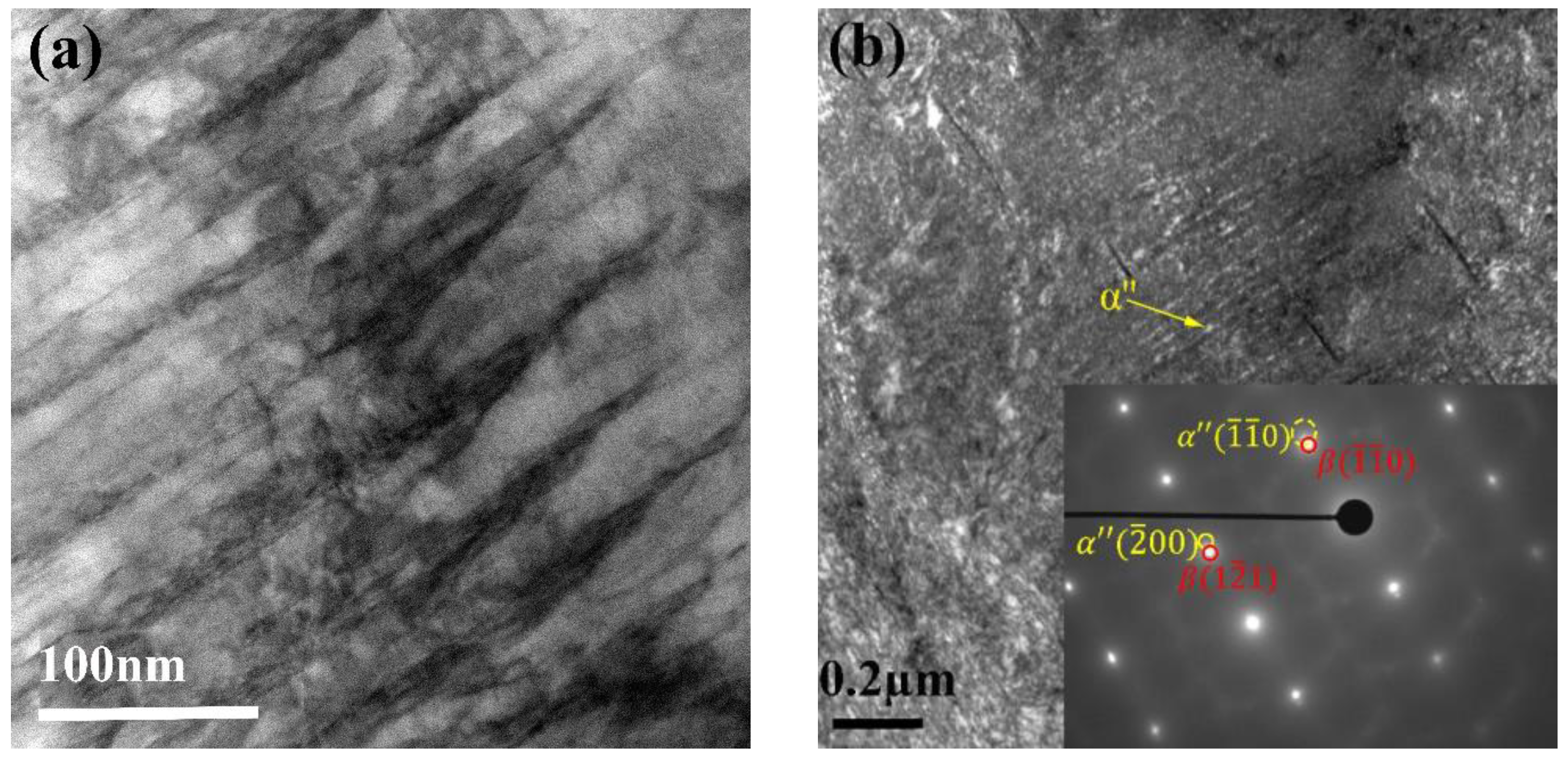
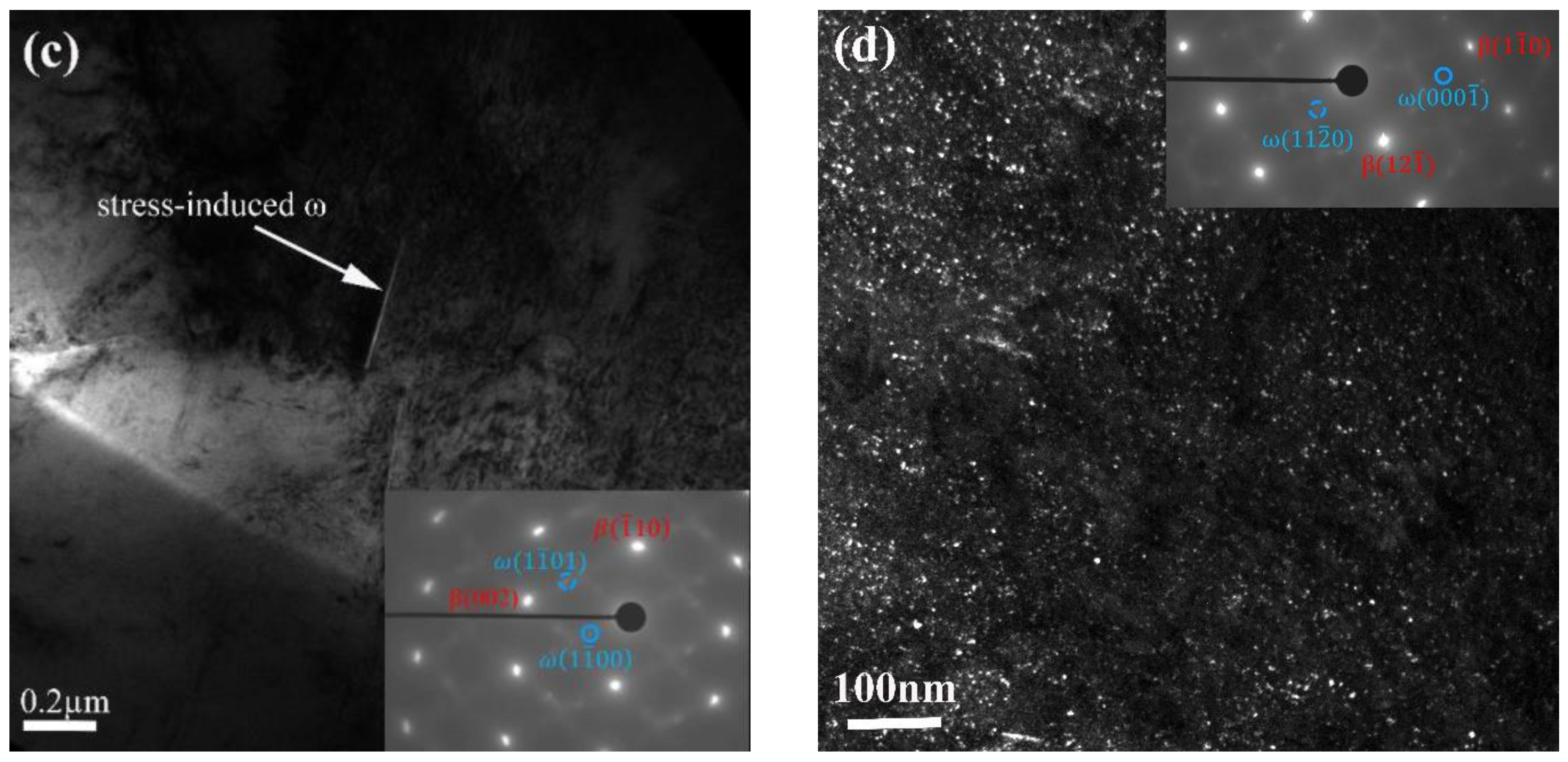
3. Results
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Peters, J.; Lütjering, G.; Nalla, R.; Altenberger, I.; Ritchie, R. High Cycle Fatigue of Beta-Titanium Alloys. In Proceedings of the Eighth International Fatigue Congress, Stockholm, Sweden, 3–7 June 2002. [Google Scholar]
- Jha, S.K.; Ravichandran, K.S. High-cycle fatigue resistance in beta-titanium alloys. JOM 2000, 52, 30–35. [Google Scholar] [CrossRef]
- Coakley, J.; Vorontsov, V.A.; Jones, N.G.; Radecka, A.; Bagot, P.A.; Littrell, K.C.; Heenan, R.K.; Hu, F.; Magyar, A.P.; Bell, D.C. Precipitation processes in the Beta-Titanium alloy Ti–5Al–5Mo–5V–3Cr. J. Alloys Compd. 2015, 646, 946–953. [Google Scholar] [CrossRef]
- Kent, D.; Wang, G.; Wang, W.; Dargusch, M. Influence of ageing temperature and heating rate on the properties and microstructure of β Ti alloy, Ti–6Cr–5Mo–5V–4Al. Mater. Sci. Eng. A 2012, 531, 98–106. [Google Scholar] [CrossRef]
- Nag, S.; Banerjee, R.; Srinivasan, R.; Hwang, J.; Harper, M.; Fraser, H. ω-Assisted nucleation and growth of α precipitates in the Ti–5Al–5Mo–5V–3Cr–0.5 Fe β titanium alloy. Acta Mater. 2009, 57, 2136–2147. [Google Scholar] [CrossRef]
- Fan, J.; Kou, H.; Lai, M.-J.; Tang, B.; Chang, H.; Li, J. Characterization of hot deformation behavior of a new near beta titanium alloy: Ti-7333. Mater. Des. 2013, 49, 945–952. [Google Scholar] [CrossRef]
- Jackson, M.; Dashwood, R.; Flower, H.; Christodoulou, L. The microstructural evolution of near beta alloy Ti-10V-2Fe-3Al during subtransus forging. Metall. Mater. Trans. A 2005, 36, 1317–1327. [Google Scholar] [CrossRef]
- Fan, J.; Kou, H.; Lai, M.; Tang, B.; Chang, H.; Li, J. Hot deformation mechanism and microstructure evolution of a new near β titanium alloy. Mater. Sci. Eng. A 2013, 584, 121–132. [Google Scholar] [CrossRef]
- Jones, N.; Jackson, M. On mechanism of flow softening in Ti–5Al–5Mo–5V–3Cr. Mater. Sci. Technol. 2011, 27, 1025–1032. [Google Scholar] [CrossRef]
- Warchomicka, F.; Poletti, C.; Stockinger, M. Study of the hot deformation behaviour in Ti–5Al–5Mo–5V–3Cr–1Zr. Mater. Sci. Eng. A 2011, 528, 8277–8285. [Google Scholar] [CrossRef]
- Lei, L.; Huang, X.; Wang, M.; Wang, L.; Qin, J.; Li, H.; Lu, S. Effect of hot compressive deformation on the martensite transformation of Ti–10V–2Fe–3Al titanium alloy. Mater. Sci. Eng. A 2011, 530, 591–601. [Google Scholar] [CrossRef]
- Jones, N.; Dashwood, R.; Dye, D.; Jackson, M. The flow behavior and microstructural evolution of Ti-5Al-5Mo-5V-3Cr during subtransus isothermal forging. Metall. Mater. Trans. A 2009, 40, 1944–1954. [Google Scholar] [CrossRef]
- Zhao, J.; Zhong, J.; Yan, F.; Chai, F.; Dargusch, M. Deformation behaviour and mechanisms during hot compression at supertransus temperatures in Ti-10V-2Fe-3Al. J. Alloys Compd. 2017, 710, 616–627. [Google Scholar] [CrossRef]
- Furuhara, T.; Poorganji, B.; Abe, H.; Maki, T. Dynamic recovery and recrystallization in titanium alloys by hot deformation. JOM 2007, 59, 64–67. [Google Scholar] [CrossRef]
- Quan, G.-Z.; Lv, W.-Q.; Liang, J.-T.; Pu, S.-A.; Luo, G.-C.; Liu, Q. Evaluation of the hot workability corresponding to complex deformation mechanism evolution for Ti–10V–2Fe–3Al alloy in a wide condition range. J. Mater. Process. Technol. 2015, 221, 66–79. [Google Scholar] [CrossRef]
- Balasubrahmanyam, V.; Prasad, Y. Hot deformation mechanisms in metastable beta titanium alloy Ti–10V–2Fe–3Al. Mater. Sci. Technol. 2001, 17, 1222–1228. [Google Scholar] [CrossRef]
- Akanuma, T.; Matsumoto, H.; Sato, S.; Chiba, A.; Inagaki, I.; Shirai, Y.; Maeda, T. Enhancement of athermal α″ martensitic transformation in Ti–10V–2Fe–3Al alloy due to high-speed hot deformation. Scr. Mater. 2012, 67, 21–24. [Google Scholar] [CrossRef]
- Ahmed, M.; Wexler, D.; Casillas, G.; Ivasishin, O.M.; Pereloma, E.V. The influence of β phase stability on deformation mode and compressive mechanical properties of Ti–10V–3Fe–3Al alloy. Acta Mater. 2015, 84, 124–135. [Google Scholar] [CrossRef]
- Duerig, T.; Albrecht, J.; Richter, D.; Fischer, P. Formation and reversion of stress induced martensite in Ti-10V-2Fe-3Al. Acta Metall. 1982, 30, 2161–2172. [Google Scholar] [CrossRef]
- Ahmed, M.; Wexler, D.; Casillas, G.; Savvakin, D.G.; Pereloma, E.V. Strain rate dependence of deformation-induced transformation and twinning in a metastable titanium alloy. Acta Mater. 2016, 104, 190–200. [Google Scholar] [CrossRef]
- Zhang, L.C.; Zhou, T.; Aindow, M.; Alpay, S.P.; Blackburn, M.J.; Wu, M.H. Nucleation of stress-induced martensites in a Ti/Mo-based alloy. J. Mater. Sci. 2005, 40, 2833–2836. [Google Scholar] [CrossRef]
- Grosdidier, T.; Philippe, M.-J. Deformation induced martensite and superelasticity in a β-metastable titanium alloy. Mater. Sci. Eng. A 2000, 291, 218–223. [Google Scholar] [CrossRef]
- Sun, F.; Zhang, J.; Marteleur, M.; Gloriant, T.; Vermaut, P.; Laillé, D.; Castany, P.; Curfs, C.; Jacques, P.; Prima, F. Investigation of early stage deformation mechanisms in a metastable β titanium alloy showing combined twinning-induced plasticity and transformation-induced plasticity effects. Acta Mater. 2013, 61, 6406–6417. [Google Scholar] [CrossRef]
- Marteleur, M.; Sun, F.; Gloriant, T.; Vermaut, P.; Jacques, P.J.; Prima, F. On the design of new β-metastable titanium alloys with improved work hardening rate thanks to simultaneous TRIP and TWIP effects. Scr. Mater. 2012, 66, 749–752. [Google Scholar] [CrossRef]
- Savvakin, D.G.; Carman, A.; Ivasishin, O.M.; Matviychuk, M.V.; Gazder, A.A.; Pereloma, E.V. Effect of iron content on sintering behavior of Ti-V-Fe-Al near-β titanium alloy. Metall. Mater. Trans. A 2012, 43, 716–723. [Google Scholar] [CrossRef]
- Guo, Z.; Malinov, S.; Sha, W. Modelling beta transus temperature of titanium alloys using artificial neural network. Comput. Mater. Sci. 2005, 32, 1–12. [Google Scholar] [CrossRef]
- Polmear, I. Light Metals: From Traditional Alloys to Nanocrystals, 4th ed.; Elsevier’s Science and Technology: Oxford, UK, 2006; pp. 299–335. [Google Scholar]
- Cullity, B.D.; Stock, S. Elements of X-ray Diffraction; Pearson Education Limited: Essex, UK, 2014. [Google Scholar]
- Wang, Z.-J.; Qiang, H.-F.; Wang, X.-R.; Guang, W. Constitutive model for a new kind of metastable β titanium alloy during hot deformation. Trans. Nonferrous Met. Soc. China 2012, 22, 634–641. [Google Scholar] [CrossRef]
- Balasubrahmanyam, V.; Prasad, Y. Deformation behaviour of beta titanium alloy Ti–10V–4.5 Fe–1.5 Al in hot upset forging. Mater. Sci. Eng. A 2002, 336, 150–158. [Google Scholar] [CrossRef]
- Jackson, M.; Jones, N.; Dye, D.; Dashwood, R. Effect of initial microstructure on plastic flow behaviour during isothermal forging of Ti–10V–2Fe–3Al. Mater. Sci. Eng. A 2009, 501, 248–254. [Google Scholar] [CrossRef]
- Zhao, J.; Zhong, J.; Zhou, M.; Chai, F.; Yan, F. The effect of alpha phase on flow softening and deformation of Ti–10V–2Fe–3Al. Mater. Sci. Technol. 2017, 33, 1993–2003. [Google Scholar] [CrossRef]
- Warner, C.P. Mechanisms of Strain Localization in Plane Strain Compression of Aluminum and Aluminum Alloys. Ph.D. Thesis, University of Pennsylvania, Philadelphia, PA, USA, 1997. Disertation available from ProQuest AAI9727310. [Google Scholar]
- Barriobero-Vila, P.; Requena, G.; Warchomicka, F.; Stark, A.; Schell, N.; Buslaps, T. Phase transformation kinetics during continuous heating of a β-quenched Ti–10V–2Fe–3Al alloy. J. Mater. Sci. 2015, 50, 1412–1426. [Google Scholar] [CrossRef]
- Cai, M.-H.; Lee, C.-Y.; Kang, S.; Lee, Y.-K. Fine-grained structure fabricated by strain-induced martensite and its reverse transformations in a metastable β titanium alloy. Scr. Mater. 2011, 64, 1098–1101. [Google Scholar] [CrossRef]
- De Fontaine, D.; Paton, N.; Williams, J. The omega phase transformation in titanium alloys as an example of displacement controlled reactions. Acta Metall. 1971, 19, 1153–1162. [Google Scholar] [CrossRef]
- Furuhara, T.; Maki, T.; Makino, T. Microstructure control by thermomechanical processing in β-Ti–15–3 alloy. J. Mater. Process. Technol. 2001, 117, 318–323. [Google Scholar] [CrossRef]
- Ahmed, M.; Li, T.; Casillas, G.; Cairney, J.M.; Wexler, D.; Pereloma, E.V. The evolution of microstructure and mechanical properties of Ti–5Al–5Mo–5V–2Cr–1Fe during ageing. J. Alloys Compd. 2015, 629, 260–273. [Google Scholar] [CrossRef]
- Grosdidier, T.; Roubaud, C.; Philippe, M.-J.; Combres, Y. The deformation mechanisms in the β-metastable β-Cez titanium alloy. Scr. Mater. 1997, 36, 21–28. [Google Scholar] [CrossRef]
- Neelakantan, S.; San Martin, D.; Rivera-Diaz-del-Castillo, P.; Van der Zwaag, S. Plasticity induced transformation in a metastable β Ti-1023 alloy by controlled heat treatments. Mater. Sci. Technol. 2009, 25, 1351–1358. [Google Scholar] [CrossRef]
- Joshi, V.A. Titanium Alloys: An Atlas of Structures and Fracture Features; Taylor & Francis: Boca Raton, FL, USA, 2006. [Google Scholar]
- Liu, Y.; Yang, H. The concern of elasticity in stress-induced martensitic transformation in NiTi. Mater. Sci. Eng. A 1999, 260, 240–245. [Google Scholar] [CrossRef]
- Bhattacharjee, A.; Bhargava, S.; Varma, V.; Kamat, S.; Gogia, A. Effect of β grain size on stress induced martensitic transformation in β solution treated Ti–10V–2Fe–3Al alloy. Scr. Mater. 2005, 53, 195–200. [Google Scholar] [CrossRef]
- Cheng, G.; Yuan, H.; Jian, W.; Xu, W.; Millett, P.; Zhu, Y. Deformation-induced ω phase in nanocrystalline Mo. Scr. Mater. 2013, 68, 130–133. [Google Scholar] [CrossRef]
- Hsiung, L.; Lassila, D. Shock-induced deformation twinning and omega transformation in tantalum and tantalum–tungsten alloys. Acta Mater. 2000, 48, 4851–4865. [Google Scholar] [CrossRef]
- Sleeswyk, A. ½ Screw dislocations and the nucleation of {112} twins in the Bcc Lattice. Philos. Mag. 1963, 8, 1467–1486. [Google Scholar] [CrossRef]
- Lagerlof, K. On deformation twinning in bcc metals. Acta Metall. Mater. 1993, 41, 2143–2151. [Google Scholar] [CrossRef]
- Seeger, A.; Schiller, P. The formation and diffusion of kinks as the fundamental process of dislocation movement in internal friction measurements. Acta Metall. 1962, 10, 348–357. [Google Scholar] [CrossRef]
- Ogawa, K. Edge dislocations dissociated in {112} planes and twinning mechanism of bcc metals. Philos. Mag. 1965, 11, 217–233. [Google Scholar] [CrossRef]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Samiee, A.; Casillas, G.; Ahmed, M.; Savvakin, D.G.; Naseri, R.; Pereloma, E. Formation of Deformation-Induced Products in a Metastable-β Titanium Alloy during High Temperature Compression. Metals 2018, 8, 100. https://doi.org/10.3390/met8020100
Samiee A, Casillas G, Ahmed M, Savvakin DG, Naseri R, Pereloma E. Formation of Deformation-Induced Products in a Metastable-β Titanium Alloy during High Temperature Compression. Metals. 2018; 8(2):100. https://doi.org/10.3390/met8020100
Chicago/Turabian StyleSamiee, Alireza, Gilberto Casillas, Mansur Ahmed, Dmytro G. Savvakin, Ryan Naseri, and Elena Pereloma. 2018. "Formation of Deformation-Induced Products in a Metastable-β Titanium Alloy during High Temperature Compression" Metals 8, no. 2: 100. https://doi.org/10.3390/met8020100
APA StyleSamiee, A., Casillas, G., Ahmed, M., Savvakin, D. G., Naseri, R., & Pereloma, E. (2018). Formation of Deformation-Induced Products in a Metastable-β Titanium Alloy during High Temperature Compression. Metals, 8(2), 100. https://doi.org/10.3390/met8020100





