Critical Distance Default Values for Structural Steels and a Simple Formulation to Estimate the Apparent Fracture Toughness in U-Notched Conditions
Abstract
1. Introduction
2. The Theory of Critical Distances
3. Materials and Methods
4. Derivation of Default Values of L and Apparent Fracture Toughness Estimations
- The LM predictions derived from the proposed default values of the material critical distance (Ld) capture a significant part of the physics of the notch effect, given that the LM prediction adequately follows the tendency of the experimental results, which have been obtained for a wide variety of structural steels and conditions. The results are particularly accurate considering fitting Equations (11) and (12) and the average value of the material fracture toughness (Kmat) for each particular steel and working temperature. These fitting equations significantly reduce the conservatism obtained from the lower bound values proposed in [31].
- The most conservative results have been obtained in Lower Shelf conditions when using the lower bound value of m (m = 1.6). Even under such circumstances, the resulting apparent fracture toughness estimations may be significantly higher than the corresponding fracture toughness obtained in cracked conditions, so the potential reduction of conservatism is still important.
- If the LM evaluations are to be used in structural integrity assessments, although the use of Kmat (average value of the material fracture toughness obtained in cracked conditions) captures most of the notch effect adequately, it may be unsafe on many occasions due to the high scatter of the fracture processes. This means that it sometimes provides apparent fracture toughness values higher than those measured experimentally (see Figure 4 and Figure 6). In order to provide a fracture analysis tool to be used in structural integrity assessments, it is necessary to propose a methodology that is capable of providing safe predictions of the apparent fracture toughness. With this purpose, it is proposed to use a 95% confidence level value of the fracture toughness (Kmat,0.95).
- Thus, the most accurate, yet conservative, methodology for the apparent fracture toughness estimation arises from the combination of Kmat,0.95 (as the material fracture toughness) and the m values derived from Equations (11) and (12), for LS and DBTZ conditions respectively.
5. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Taylor, D. The Theory of Critical Distances: A New Perspective in Fracture Mechanics; Elsevier: Oxford, UK, 2007; ISBN 978-008044478-9. [Google Scholar]
- Cicero, S.; Madrazo, V.; Carrascal, I.A.; Cicero, R. Assessment of notched structural components using failure assessment diagrams and the theory of critical distances. Eng. Fract. Mech. 2011, 78, 2809–2825. [Google Scholar] [CrossRef]
- Cicero, S.; Madrazo, V.; Carrascal, I.A. Analysis of notch effect in PMMA using the theory of critical distances. Eng. Fract. Mech. 2012, 86, 56–72. [Google Scholar] [CrossRef]
- Niu, L.S.; Chehimi, C.; Pluvinage, G. Stress field near a large blunted V notch and application of the concept of notch stress intensity factor to the fracture of very brittle materials. Eng. Fract. Mech. 1994, 49, 325–335. [Google Scholar]
- Pluvinage, G. Fatigue and fracture emanating from notch; the use of the notch stress intensity factor. Nucl. Eng. Des. 1998, 185, 173–184. [Google Scholar] [CrossRef]
- Dugdale, D.S. Yielding of steel sheets containing slits. J. Mech. Phys. Solids 1960, 8, 100–108. [Google Scholar] [CrossRef]
- Gómez, F.J.; Elices, M.; Valiente, A. Cracking in PMMA containing U-shaped notches. Fat. Frac. Eng. Mat. Struct. 2000, 23, 795–803. [Google Scholar] [CrossRef]
- Weibull, W. The phenomenon of rupture in solids. Proc. R. Swed. Inst. Eng. Res. 1939, 153, 1–55. [Google Scholar]
- Beremin, F.M.; Pineau, A.; Mudry, F.; Devaux, J.C.; D’Escatha, Y.; Ledermann, P. A local criterion for cleavage fracture of a nuclear pressure vessel steel. Metall. Trans. A 1983, 14A, 2277–2287. [Google Scholar] [CrossRef]
- Ritchie, R.O.; Knott, J.F.; Rice, J.R. On the relationship between critical tensile stress and fracture toughness in mild steel. J. Mech. Phys. Solids 1973, 21, 395–410. [Google Scholar] [CrossRef]
- Sih, G.C. Strain-energy-density factor applied to mixed mode crack problems. Int. J. Fract. 1974, 10, 305–321. [Google Scholar] [CrossRef]
- Berto, F.; Lazzarin, P. Recent developments in brittle and quasi-brittle failure assessment of engineering materials by means of local approaches. Mater. Sci. Eng. R 2014, 75, 1–48. [Google Scholar] [CrossRef]
- Neuber, H. Theory of Notch Stresses: Principles for Exact Calculation of Strength with Reference to Structural form and Material; Springer: Berlin, Germany, 1958. [Google Scholar]
- Peterson, R.E. Notch sensitivity. In Metal Fatigue; McGraw Hill: New York, NY, USA, 1959; pp. 293–306. [Google Scholar]
- Susmel, L.; Taylor, D. On the use of the Theory of Critical Distances to predict failures in ductile metallic materials containing different geometrical features. Eng. Fract. Mech. 2008, 75, 4410–4421. [Google Scholar] [CrossRef]
- Susmel, L.; Taylor, D. An elasto-plastic reformulation of the Theory of Critical Distances to estimate lifetime of notched components failing in the low/medium-cycle fatigue regime. J. Eng. Mater. Technol. 2010, 132, 0210021–0210028. [Google Scholar] [CrossRef]
- Taylor, D. A mechanistic approach to critical-distance methods in notch fatigue. Fatig. Fract. Eng. Mater. Struct. 2001, 24, 215–224. [Google Scholar] [CrossRef]
- Creager, M.; Paris, P.C. Elastic field equations for blunt cracks with reference to stress corrosion cracking. Int. J. Fract. 1967, 3, 247–252. [Google Scholar] [CrossRef]
- Madrazo, V.; Cicero, S.; García, T. Assessment of notched structural steel components using failure assessment diagrams and the theory of critical distances. Eng. Fail. Anal. 2014, 36, 104–120. [Google Scholar] [CrossRef]
- Anderson, T.L. Fracture Mechanics: Fundamentals and Applications, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- BS 7910. Guide to Methods for Assessing the Acceptability of Flaws in Metallic Structures; British Standards Institution: London, UK, 2013. [Google Scholar]
- Kocak, M.; Webster, S.; Janosch, J.J.; Ainsworth, R.A.; Koers, R. (Eds.) FITNET Fitness-for-Service (FFS) Procedure—Volume 1; GKSS Forschungzscentrum: Geesthacht, Germany, 2008. [Google Scholar]
- Cicero, S.; Madrazo, V.; García, T. Analysis of notch effect in the apparent fracture toughness and the fracture micromechanisms of ferritic–pearlitic steels operating within their lower shelf. Eng. Fail. Anal. 2014, 36, 322–342. [Google Scholar] [CrossRef]
- Cicero, S.; García, T.; Madrazo, V. Application and validation of the Notch Master Curve in medium and high strength structural steels. J. Mech. Sci. Tech. 2015, 29, 4129–4142. [Google Scholar] [CrossRef]
- ASTM E1820-09e1. Standard Test Method for Measurement of Fracture Toughness; American Society for Testing and Materials: Philadelphia, PA, USA, 2009. [Google Scholar]
- Quinn, G.D.; Bradt, R.C. On the Vickers Indentation Fracture Test. J. Am. Ceram. Soc. 2007, 90, 673–680. [Google Scholar] [CrossRef]
- Akono, A.T.; Randall, N.X.; Ulm, F.J. Experimental determination of the fracture toughness via microscratch tests: Application to polymers, ceramics, and metals. J. Mater. Res. 2012, 27, 485–493. [Google Scholar] [CrossRef]
- Akono, A.T.; Alm, F.J. An improved technique for characterizing the fracture toughness via scratch test experiments. Wear 2014, 313, 117–124. [Google Scholar] [CrossRef]
- Sola, R.; Giovanardi, R.; Parigi, G.; Varonesi, P. A Novel Method for Fracture Toughness Evaluation of Tool Steels with Post-Tempering Cryogenic Treatment. Metals 2017, 7, 75. [Google Scholar] [CrossRef]
- Lacalle, R.; Álvarez, J.A.; Gutiérrez-Solana, F. Use of Small Punch Notched Specimens in the Determination of Fracture Toughness. ASME Press. Vessels Pip. Conf. 2008, 6, 1363–1369. [Google Scholar] [CrossRef]
- Fuentes, J.D.; Cicero, S.; Procopio, I. Some default values to estimate the critical distance and their effect on structural integrity assessments. Theor. Appl. Fract. Mech. 2017, 90, 204–212. [Google Scholar] [CrossRef]
- Taylor, D.; Cornetti, P.; Pugno, N. The fracture mechanics of finite crack extension. Eng. Fract. Mech. 2005, 72, 1021–1038. [Google Scholar] [CrossRef]
- Susmel, L.; Taylor, D. The Theory of Critical Distances as an alternative experimental strategy for the determination of KIC and ΔKth. Eng. Fract. Mech. 2010, 77, 1492–1501. [Google Scholar] [CrossRef]
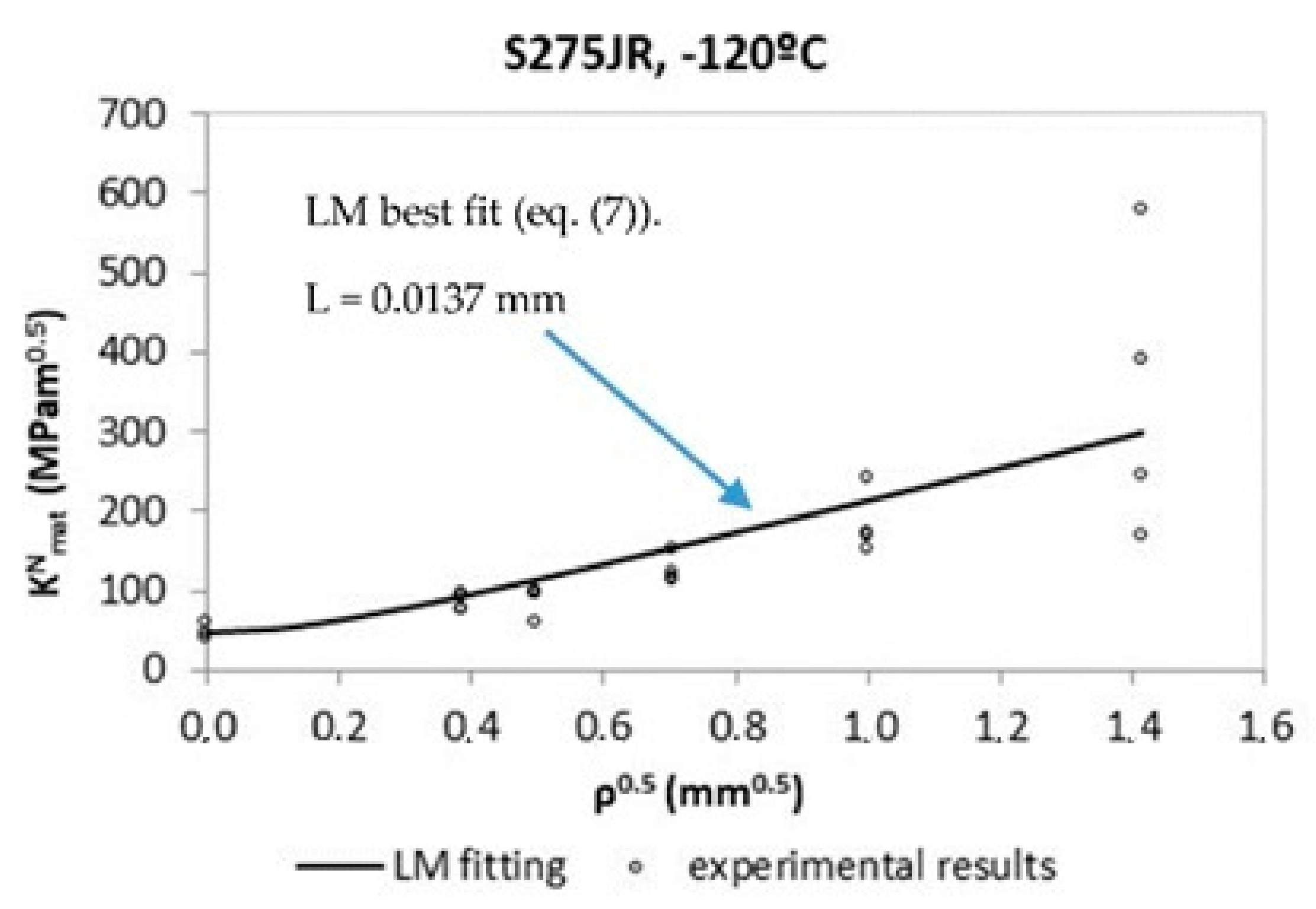
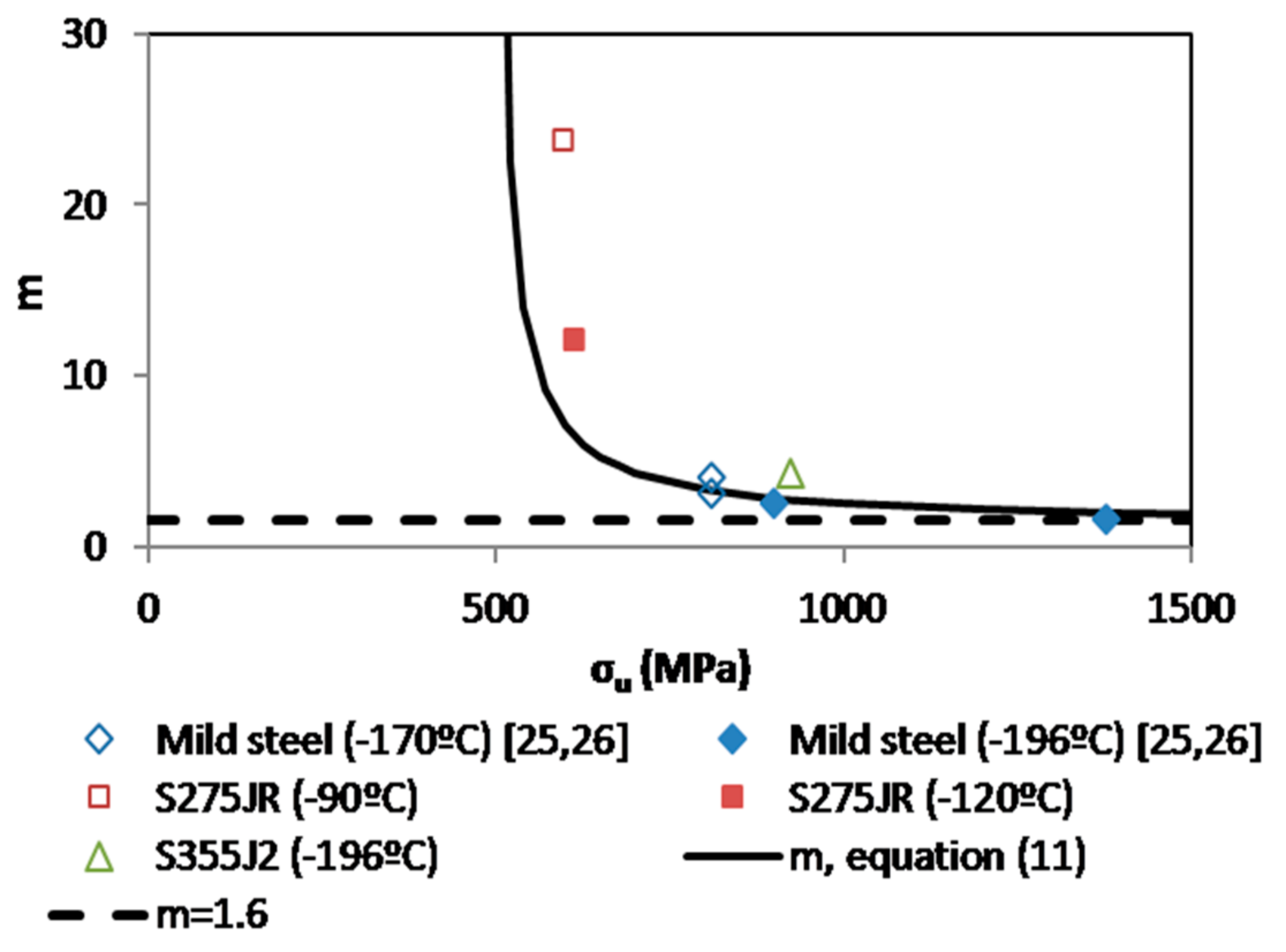
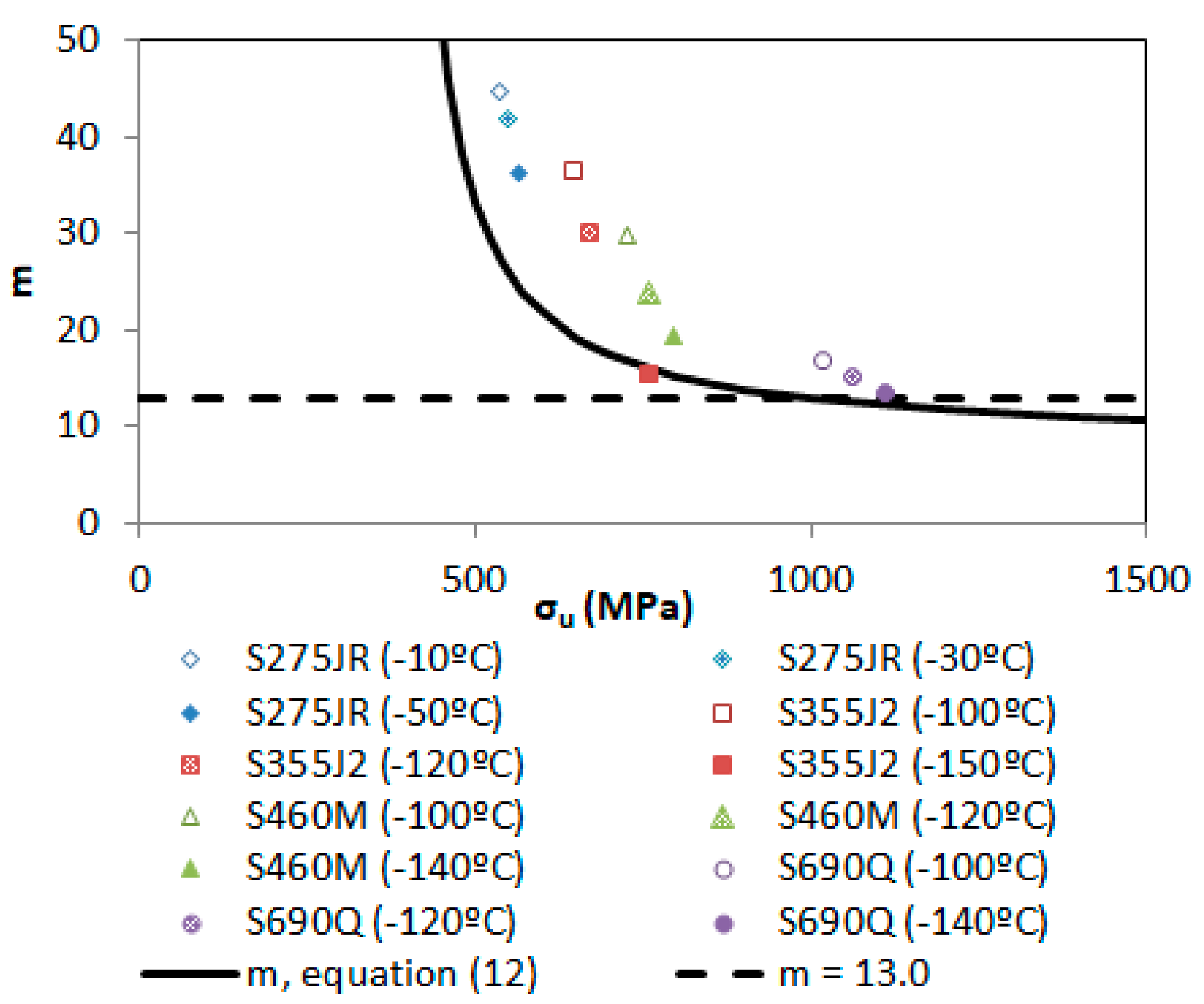


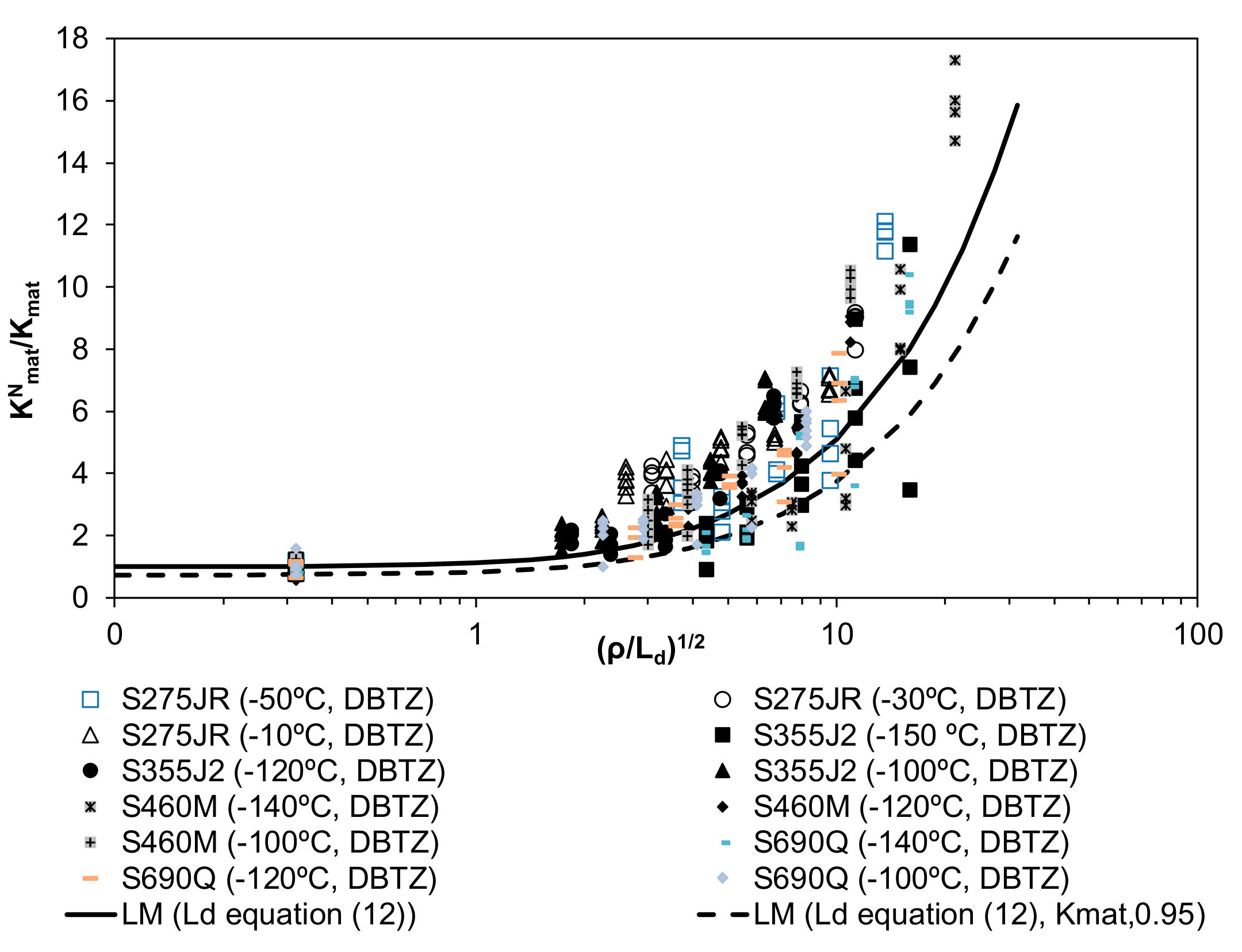

| Steel | Number of Tests | Kmat (MPa·m1/2) | L (mm) | σu (MPa) | σ0 (MPa) |
|---|---|---|---|---|---|
| S275JR (−120 °C, LS) | 23 | 48.80 | 0.0137 | 614 | 7438 |
| S275JR (−90 °C, LS) | 24 | 62.72 | 0.0062 | 597 | 14,211 |
| S275JR (−50 °C, DBTZ) | 24 | 80.60 | 0.0049 | 565 | 20,543 |
| S275JR (−30 °C, DBTZ) | 24 | 100.70 | 0.0061 | 549 | 23,003 |
| S275JR (−10 °C, DBTZ) | 34 | 122.80 | 0.0083 | 536 | 24,048 |
| S355J2 (−196 °C, LS) | 24 | 31.27 | 0.0198 | 923 | 3965 |
| S355J2 (−150 °C, DBTZ) | 21 | 60.56 | 0.0084 | 758 | 11,789 |
| S355J2 (−120 °C, DBTZ) | 22 | 146.60 | 0.0168 | 672 | 20,179 |
| S355J2 (−100 °C, DBTZ) | 35 | 157.40 | 0.0140 | 647 | 23,734 |
| S460M (−140 °C, DBTZ) | 24 | 45.60 | 0.0028 | 795 | 15,375 |
| S460M (−120 °C, DBTZ) | 24 | 88.29 | 0.0075 | 759 | 18,189 |
| S460M (−100 °C, DBTZ) | 33 | 88.58 | 0.0053 | 727 | 21,708 |
| S690Q (−140 °C, DBTZ) | 24 | 69.11 | 0.0069 | 1112 | 14,844 |
| S690Q (−120 °C, DBTZ) | 24 | 103.80 | 0.0131 | 1061 | 16,180 |
| S690Q (−100 °C, DBTZ) | 34 | 125.40 | 0.0170 | 1016 | 17,159 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cicero, S.; Fuentes, J.D.; Procopio, I.; Madrazo, V.; González, P. Critical Distance Default Values for Structural Steels and a Simple Formulation to Estimate the Apparent Fracture Toughness in U-Notched Conditions. Metals 2018, 8, 871. https://doi.org/10.3390/met8110871
Cicero S, Fuentes JD, Procopio I, Madrazo V, González P. Critical Distance Default Values for Structural Steels and a Simple Formulation to Estimate the Apparent Fracture Toughness in U-Notched Conditions. Metals. 2018; 8(11):871. https://doi.org/10.3390/met8110871
Chicago/Turabian StyleCicero, Sergio, Juan Diego Fuentes, Isabela Procopio, Virginia Madrazo, and Pablo González. 2018. "Critical Distance Default Values for Structural Steels and a Simple Formulation to Estimate the Apparent Fracture Toughness in U-Notched Conditions" Metals 8, no. 11: 871. https://doi.org/10.3390/met8110871
APA StyleCicero, S., Fuentes, J. D., Procopio, I., Madrazo, V., & González, P. (2018). Critical Distance Default Values for Structural Steels and a Simple Formulation to Estimate the Apparent Fracture Toughness in U-Notched Conditions. Metals, 8(11), 871. https://doi.org/10.3390/met8110871






