A Simple Calibration Method to Consider Plastic Deformation Influence on X-ray Elastic Constant Based on Peak Width Variation
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Composition, Microstructure, and Macroscopic Mechanical Properties (For Confidentiality Reasons, This Section Only Contains Limited Information)
2.2. Test Specimens
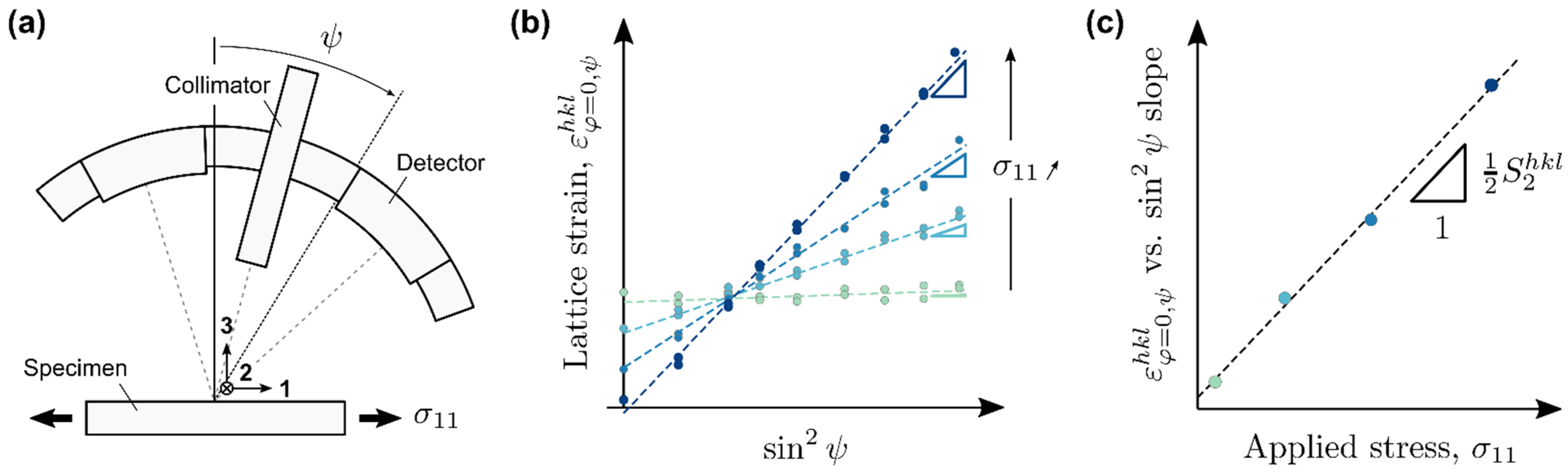
2.3. Procedure for Determining X-ray Elastic Constants
2.3.1. Preliminaries
2.3.2. Detailed Procedure
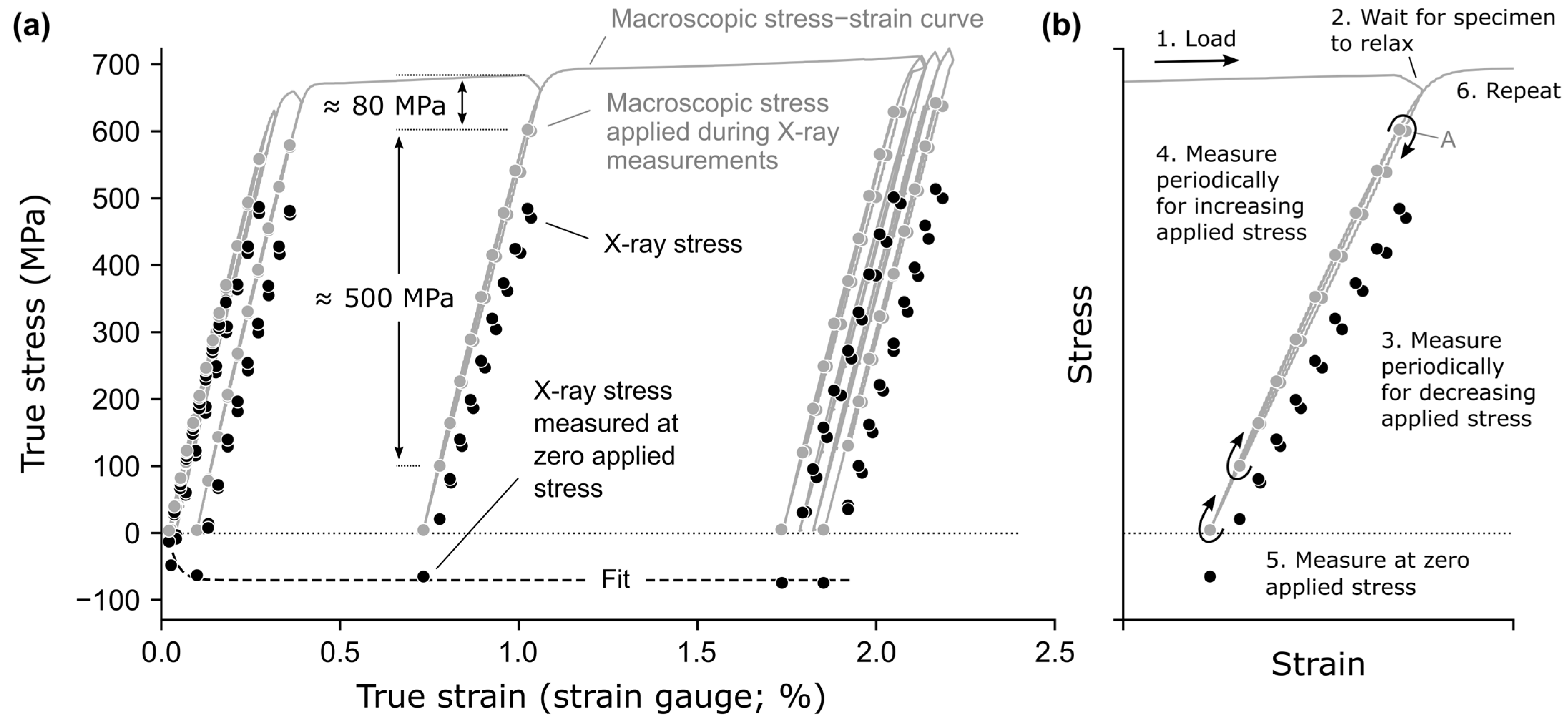
- Whereas ASTM-E1426-14 recommends using rectangular specimens with a length between grips at least four times the width and a width-to-thickness ratio less than eight, the tensile specimen’s geometry described in Section 2.2 was used due to size and load constraints dictated by the micro-tensile stage used to load the specimen.
- Whereas ASTM-E1426-14 recommends measuring stresses experienced by the irradiated volume using calibrated strain gauges located as close as possible to the X-ray spot, the applied loads were measured using the micro-tensile stage load cell. This was because the steels’ Young’s modulus was known to decrease by several percentage points with plastic deformation [28]. Using strain gauges to measure stresses would have required re-calibrating the strain gauge at each plastic deformation level, which would have considerably lengthened measurements.
- Whereas ASTM-E1426-14 recommends that applied stresses range from 10% to 70% of the macroscopic yield stress, a constant range of 500 MPa was sampled, with the higher applied stress equal to the macroscopic yield stress minus 80 MPa. This procedure was chosen to minimize micro-plasticity artifacts that appear at the end of the unloading on this material and that can be observed as the stress–strain curve forms an open loop.
2.4. X-ray Stress Measurements
2.4.1. Setup and Diffraction Conditions
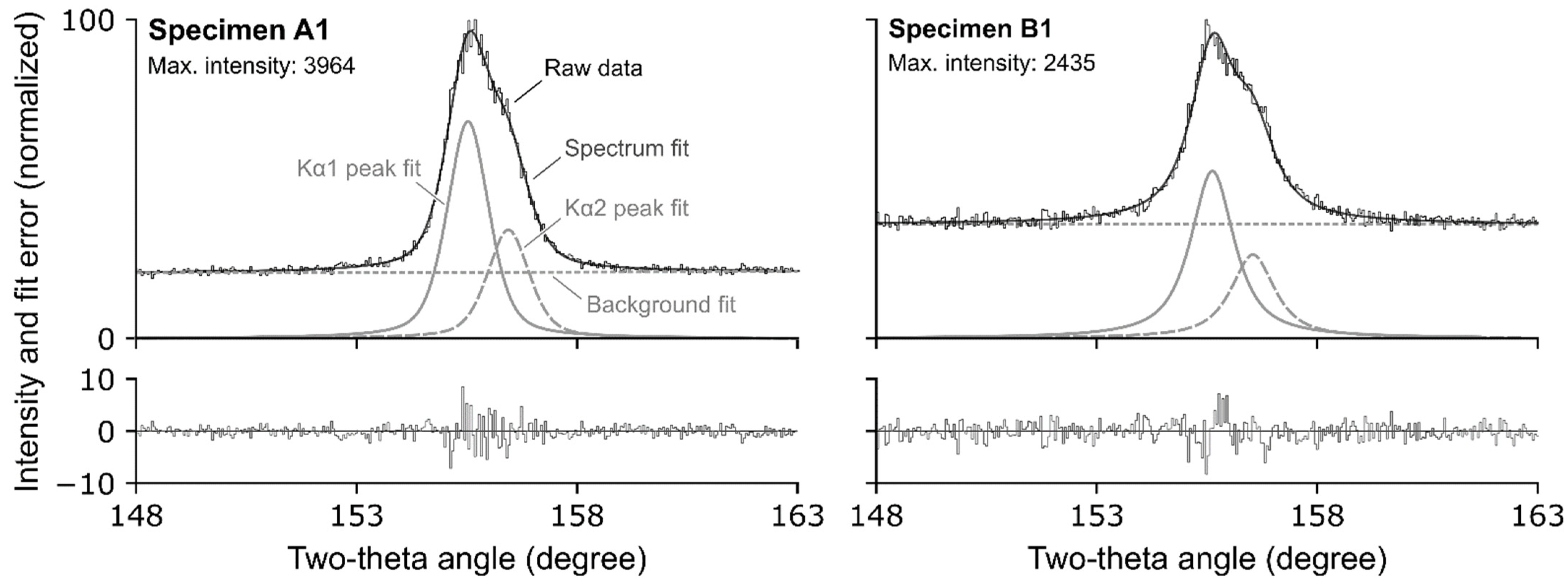
2.4.2. Peak Fitting
2.4.3. X-ray Stress Computations
3. Results
- -
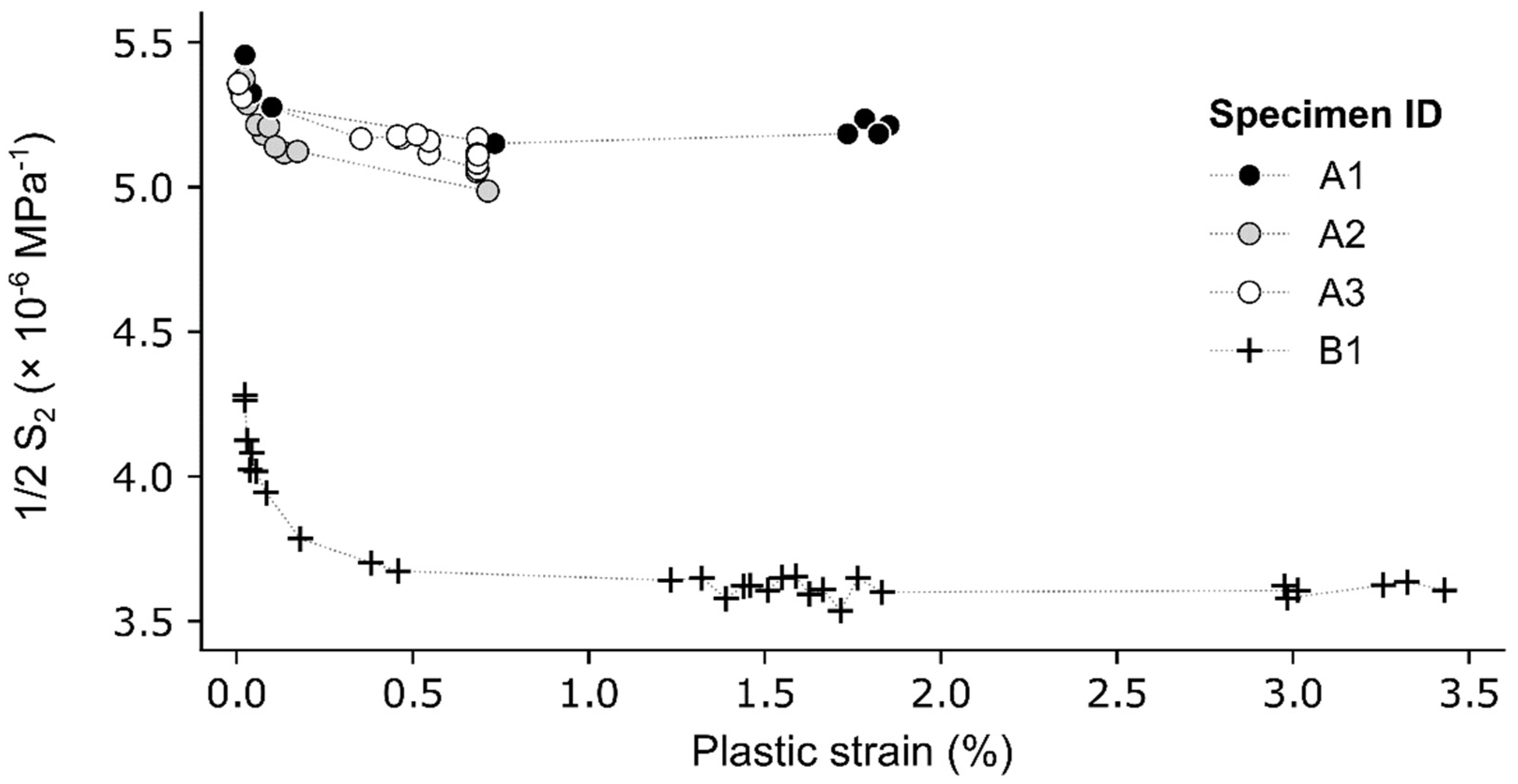
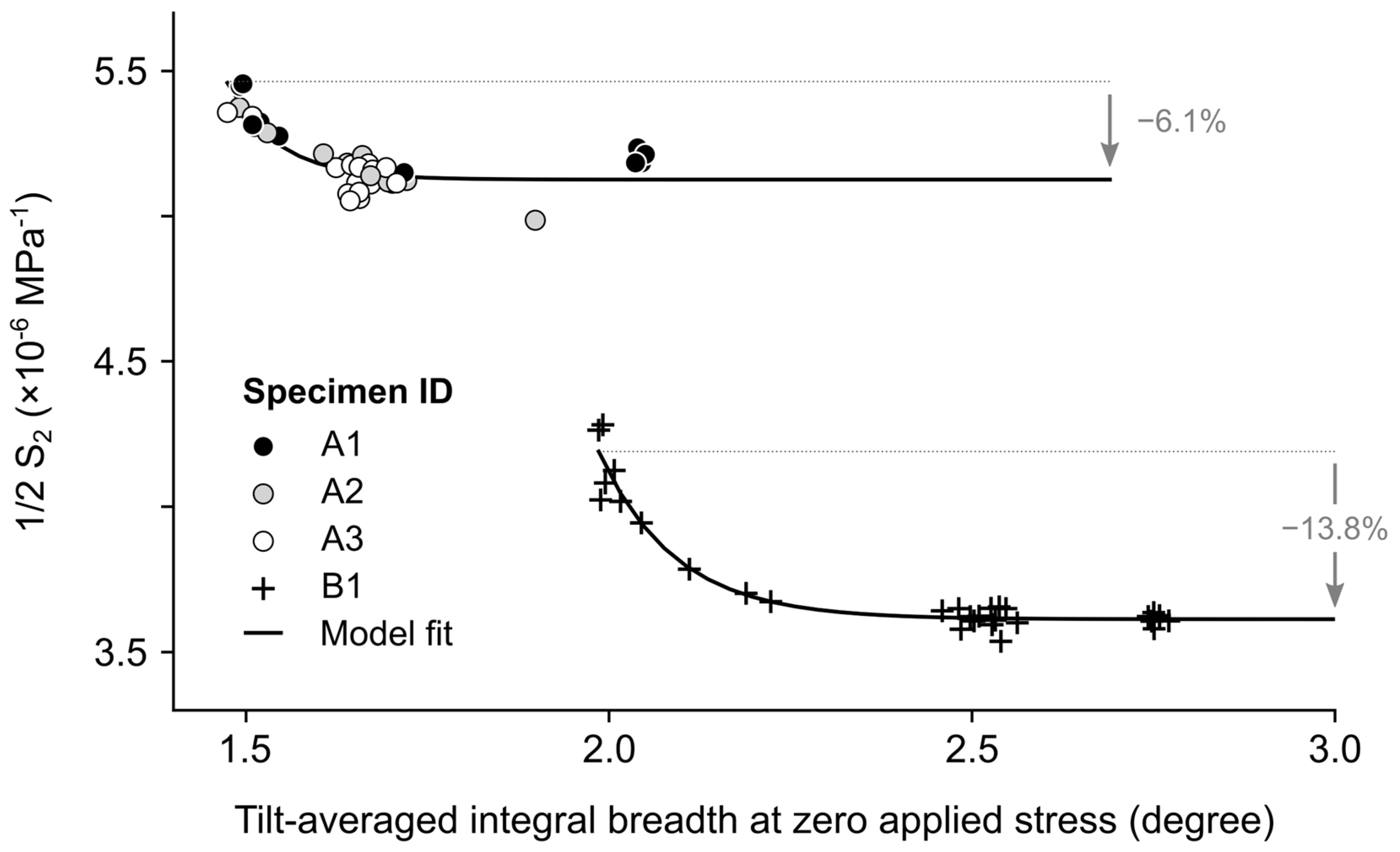
- For electropolished specimens, the constant is initially close to values reported in the literature, and then decreases by about 6% after 2% added plastic strain.
- -
- For as-annealed specimens, the constant is initially about 25% lower than the values from the literature, at about 4.3 × 10−6 MPa−1, and it further decreases by about 13%, down to 3.75 × 10−6 MPa−1.
4. Discussion
- -
- is the value assessed on the first load of the test;
- -
- is the value assessed on the last unload of the test;
- -
- is the tilt-averaged integral breadth at the start of the test.
- -
- Black dots use the constant textbook value MPa−1 and represent the case where no calibration test is performed (i.e., the same value for all specimens).
- -
- Grey dots represent the standard case where a calibration test is performed on each specimen, and stresses are calculated using the experimentally determined value obtained at the beginning of each test (i.e., a different value for each specimen).
- -
- White dots are obtained with the methodology proposed in this study: values are computed with Equation (10), using the parameters listed in Table 3 (i.e., a different value for each specimen and each plastic strain level).
- -
- Whether it is a constant or variable parameter with plastic strain, the effect of taking into account the calibrated values is more important than other stress measurement uncertainties.
- -
- This study is based on the experimental observation that the parameter depends on plastic strain. In order to propose a practical solution, the peak width is used as a variable instead of plastic strain to describe the XEC evolution. However, it induces a secondary dependence of the XEC on the stress level as the peak width depends on both plastic strain and stress levels (see Figure 8). Figure 10 proves that the methodology does not suffer from this bias for the studied configurations.
- -
- Stress values can be used to validate process simulation models. In this case, also, absolute macroscopic stress values are of interest and constants should be corrected.
- -
- More critically, stresses can be used for design purposes. The mean stress effect determines fatigue life [29,30], corrosion cracking [31,32], and fracture failure [33,34]. The corresponding design criteria are generally calibrated on simple specimens designed for laboratory testing. In the case where the surface and plastic strain states of the calibration specimen differ from those of the application cases, different constants should be used for the stress evaluation of the two configurations, which is not generally done as it is assumed that the material is the same.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schajer, G.S. Practical Residual Stress Measurement Methods; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Song, X.; Feih, S.; Zhai, W.; Sun, C.-N.; Li, F.; Maiti, R.; Wei, J.; Yang, Y.; Oancea, V.; Romano Brandt, L. Advances in additive manufacturing process simulation: Residual stresses and distortion predictions in complex metallic components. Mater. Des. 2020, 193, 108779. [Google Scholar] [CrossRef]
- Srivastava, S.; Garg, R.K.; Sharma, V.S.; Sachdeva, A. Measurement and Mitigation of Residual Stress in Wire-Arc Additive Manufacturing: A Review of Macro-Scale Continuum Modelling Approach. Arch. Comput. Methods Eng. 2021, 28, 3491–3515. [Google Scholar] [CrossRef]
- NF EN 15305; Non-Destructive Testing—Test Method For Residual Stress Analysis By X-ray Diffraction. AFNOR: Paris, France, 2009.
- ASTM E2860; Standard Test Method for Residual Stress Measurement by X-ray Diffraction for Bearing Steels. ASTM International: West Conshohocken, PA, USA, 2021.
- SAE J784A-1971; Residual Stress Measurement by X-ray Diffraction. ANSI: Washington, DC, USA, 1971.
- François, M. Unified description for the geometry of X-ray stress analysis: Proposal for a consistent approach. J. Appl. Crystallogr. 2008, 41, 44–55. [Google Scholar] [CrossRef]
- Welzel, U.; Ligot, J.; Lamparter, P.; Vermeulen, A.C.; Mittemeijer, E.J. Stress analysis of polycrystalline thin films and surface regions by X-ray diffraction. J. Appl. Crystallogr. 2005, 38, 1–29. [Google Scholar] [CrossRef]
- Hauk, V. 2.13—X-ray elastic constants (XEC). In Structural and Residual Stress Analysis by Nondestructive Methods; Hauk, V., Ed.; Elsevier Science B.V.: Amsterdam, The Netherlands, 1997; pp. 279–336. [Google Scholar] [CrossRef]
- Liu, L.; Chen, Q.; Zhu, C.; Chen, K. A new model for evaluating the diffraction elastic constants of WC-Co. Mater. Sci. Technol. 2020, 36, 334–341. [Google Scholar] [CrossRef]
- ASTM-E1426-14; Standard Test Method for Determining the X-ray Elastic Constants for Use in the Measurement of Residual Stress Using X-ray Diffraction Techniques. ASTM International: West Conshohocken, PA, USA, 2019.
- Marion, R.H.; Cohen, J.B. The Need for Experimentally Determined X-ray Elastic Constants. Adv. X-ray Anal. 1976, 20, 355–367. [Google Scholar] [CrossRef]
- Mareau, C. The dependence of X-ray elastic constants with respect to the penetration depth. J. Appl. Crystallogr. 2023, 56, 1446–1455. [Google Scholar] [CrossRef] [PubMed]
- Esquivel, A.L. X-ray Diffraction Study of the Effects of Uniaxial Plastic Deformation on Residual Stress Measurements. Adv. X-ray Anal. 1968, 12, 269–300. [Google Scholar] [CrossRef]
- Taira, S.; Hayashi, K.; Watase, Z. X-ray Investigation on the Deformation of Polycrystalline Metals. J. Soc. Mater. Sci. Jpn. 1968, 17, 1151–1157. [Google Scholar] [CrossRef]
- Taira, S.; Hayashi, K.; Koe, S. X-ray Investigation of Deformation of Polycrystalline Metals. J. Soc. Mater. Sci. Jpn. 1970, 19, 447–454. [Google Scholar] [CrossRef][Green Version]
- Dölle, H.; Hauk, V.; Kloth, H.; Over, H.; Wichert, W. Röntgenographische Elastizitätskonstanten von Stählen bei ein- und zweiachsiger Zugbeanspruchung in Abhängigkeit von der plastischen Dehnung. Arch. Für Eisenhüttenwes. 1977, 48, 601–605. [Google Scholar] [CrossRef]
- Evenschor, P.; Hauk, V.; Kockelmann, H.; Sesemann, H. Die röntgenographischen Elastizitätskonstanten von plastisch verformtem Kupfer verschiedener Textur. Int. J. Mater. Res. 1974, 65, 726–729. [Google Scholar] [CrossRef]
- Prümmer, R. Der Zusammenhang Zwischen Gitterdehnungen und Lastspannungen Zugverformter Stähle und Dessen Aus-Wirkung auf Röntgenographische Eigenspannungbestimmungen. Ph.D. Thesis, Universität Karlsruhe, Karlsruhe, Germany, 1967. [Google Scholar]
- Noyan, I.C.; Cohen, J.B. Residual Stress: Measurement by Diffraction and Interpretation; Springer: Berlin, Germany, 1987. [Google Scholar]
- Withers, P. Residual Stresses: Measurement by Diffraction. Encycl. Mater. Sci. Technol. 2001, 8158–8169. [Google Scholar] [CrossRef]
- Cullity, B.D. Elements of X-ray Diffraction; Addison-Wesley Publishing Company: Boston, MA, USA, 1956. [Google Scholar]
- Hutchings, M.T.; Withers, P.J.; Holden, T.M.; Lorentzen, T. Introduction to the Characterization of Residual Stress by Neutron Diffraction; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar] [CrossRef]
- Bahadur, A.; Chowdhury, S.G.; Kumar, B.R. Evaluation of changes in X-ray elastic constants and residual stress as a function of cold rolling of austenitic steels. Mater. Sci. Technol. 2004, 20, 387–392. [Google Scholar] [CrossRef]
- Iadicola, M.A. Gnäupel-Herold Effective X-ray elastic constant measurement for in situ stress measurement of biaxially strained AA5754-O. Mater. Sci. Eng. A 2012, 545, 168–175. [Google Scholar] [CrossRef]
- Gautier, E. Plasticité Cyclique Multiaxiale en Zone Confinée: Approche Théorique et Expérimentale. Ph.D. Thesis, École nationale supérieure de techniques avancées Bretagne, Brest, France, 2023. [Google Scholar]
- Prevey, P.S. A Method of Determining the Elastic Properties of Alloys in Selected Crystallographic Directions for X-ray Diffraction Residual Stress Measurement. Adv. X-ray Anal. 1976, 20, 345–354. [Google Scholar] [CrossRef][Green Version]
- Yoshida, F.; Uemori, T.; Fujiwara, K. Elastic–plastic behavior of steel sheets under in-plane cyclic tension–compression at large strain. Int. J. Plast. 2002, 18, 633–659. [Google Scholar] [CrossRef]
- Ince, A. A generalized mean stress correction model based on distortional strain energy. Int. J. Fatigue 2017, 104, 273–282. [Google Scholar] [CrossRef]
- Bercelli, L.; Levieil, B.; Doudard, C.; Malek, B.; Bridier, F.; Ezanno, A. Use of infrared thermography to model the effective stress ratio effect on fatigue crack growth in welded T-joints. Eng. Fract. Mech. 2023, 279, 109061. [Google Scholar] [CrossRef]
- Raja, V.S.; Shoji, T. (Eds.) Stress Corrosion Cracking; Woodhead Publishing Series in Metals and Surface Engineering; Woodhead Publishing: Cambridge, UK, 2011. [Google Scholar] [CrossRef]
- Dhondt, M.; Shubina, V.; Homette, R.; Chirani, S.A.; Calloch, S. Effect of mechanical (monotonic and cyclic) stress on the corrosion resistance of chromium-plated steel rods. Procedia Eng. 2018, 213, 598–605. [Google Scholar] [CrossRef]
- Prime, M.B.; DeWald, A.T.; Hill, M.R.; Clausen, B.; Tran, M. Forensic determination of residual stresses and KI from fracture surface mismatch. Eng. Fract. Mech. 2014, 116, 158–171. [Google Scholar] [CrossRef]
- Altaf Husain, S.; Kreiml, P.; Trost, C.O.W.; Faurie, D.; Cordill, M.J.; Renault, P.-O. Residual stress and thickness effects on fracture behavior of trilayer films during uniaxial loading. Thin Solid Films 2024, 788, 140173. [Google Scholar] [CrossRef]





| C | S | P | Ti | Mn | Ni | Cr | Mo | Fe |
|---|---|---|---|---|---|---|---|---|
| balance |
| Specimen ID | Condition |
|---|---|
| A1 | Annealed and electropolished |
| A2 | Annealed and electropolished |
| A3 | Annealed and electropolished |
| B1 | As-annealed |
| Surface Condition/Parameter | (×10−6 MPa−1) | (×10−6 MPa−1) | (Degree) | (Degree−1) |
|---|---|---|---|---|
| Electropolished | 5.459 | 5.126 | 1.475 | 14.33 |
| As-annealed | 4.189 | 3.612 | 1.986 | 9.52 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gautier, E.; Faucheux, P.; Levieil, B.; Barrallier, L.; Calloch, S.; Doudard, C. A Simple Calibration Method to Consider Plastic Deformation Influence on X-ray Elastic Constant Based on Peak Width Variation. Metals 2024, 14, 62. https://doi.org/10.3390/met14010062
Gautier E, Faucheux P, Levieil B, Barrallier L, Calloch S, Doudard C. A Simple Calibration Method to Consider Plastic Deformation Influence on X-ray Elastic Constant Based on Peak Width Variation. Metals. 2024; 14(1):62. https://doi.org/10.3390/met14010062
Chicago/Turabian StyleGautier, Ewann, Pierre Faucheux, Bruno Levieil, Laurent Barrallier, Sylvain Calloch, and Cédric Doudard. 2024. "A Simple Calibration Method to Consider Plastic Deformation Influence on X-ray Elastic Constant Based on Peak Width Variation" Metals 14, no. 1: 62. https://doi.org/10.3390/met14010062
APA StyleGautier, E., Faucheux, P., Levieil, B., Barrallier, L., Calloch, S., & Doudard, C. (2024). A Simple Calibration Method to Consider Plastic Deformation Influence on X-ray Elastic Constant Based on Peak Width Variation. Metals, 14(1), 62. https://doi.org/10.3390/met14010062





