Correlation Modeling between Morphology and Compression Behavior of Closed-Cell Al Foams Based on X-ray Computed Tomography Observations
Abstract
:1. Introduction
2. Materials and Methods
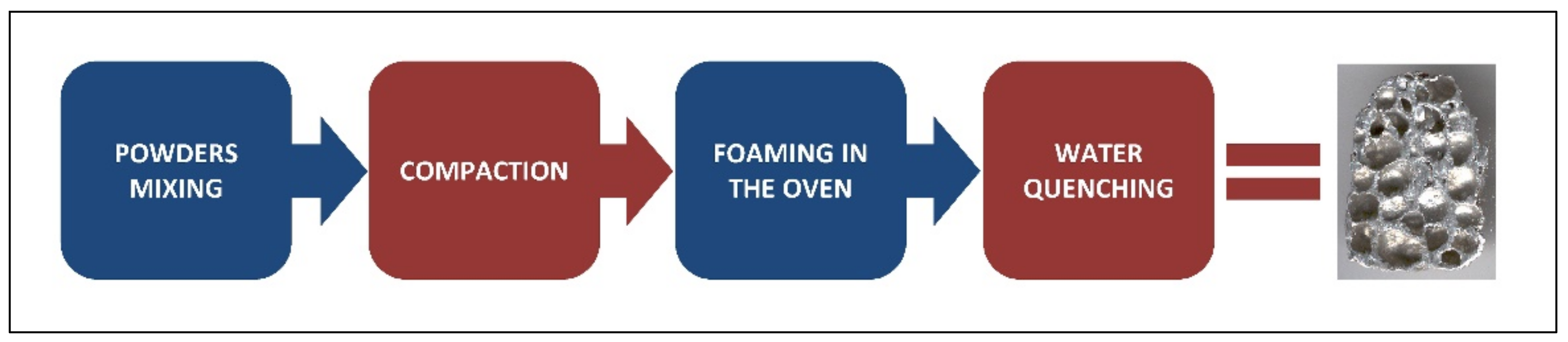
2.1. Specimens Production
2.2. Compression Tests
2.3. Tomographic Observations and Morphology Analysis
3. Theoretical Modeling
3.1. Morphology–Behavior Correlation Model Setting
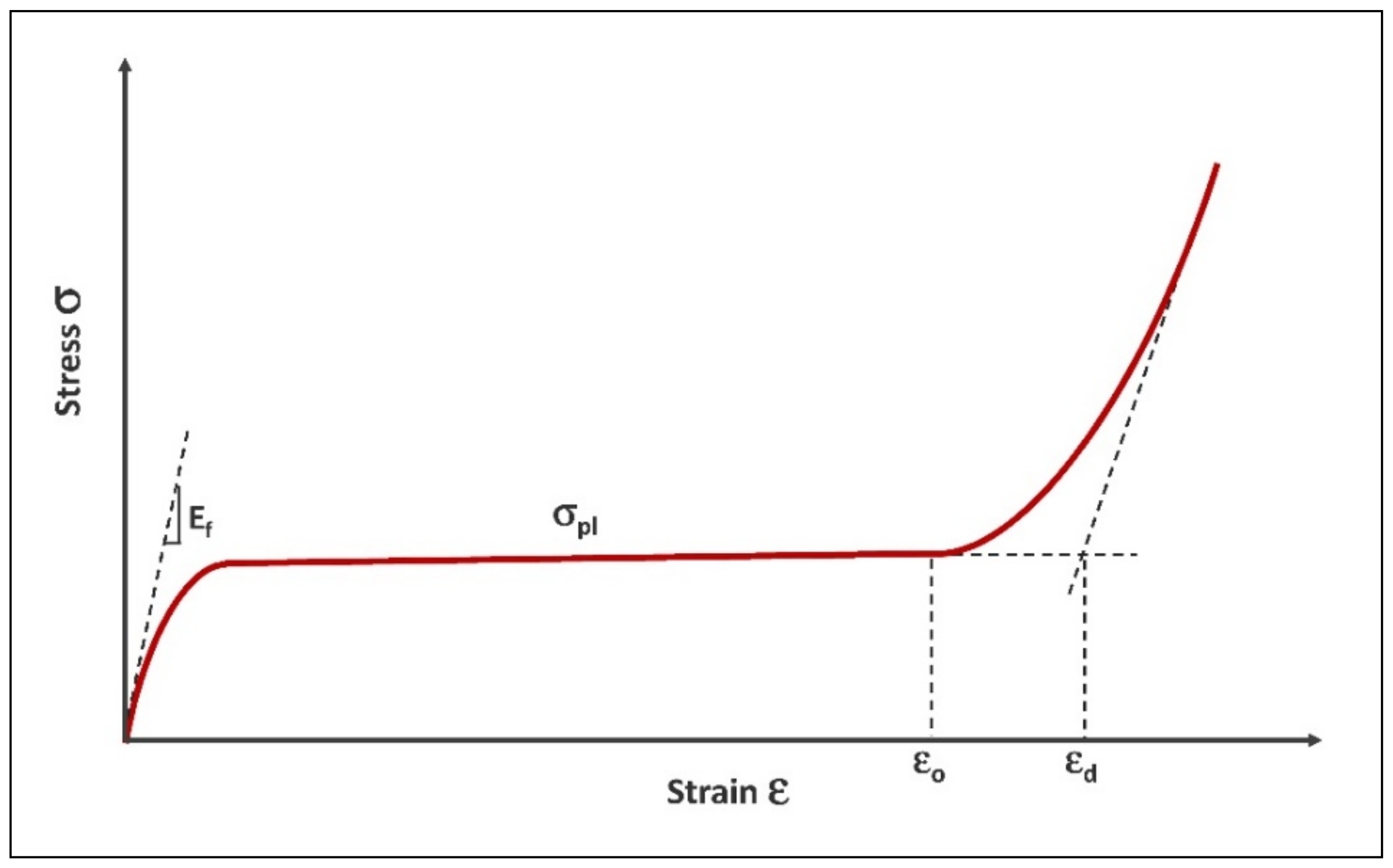
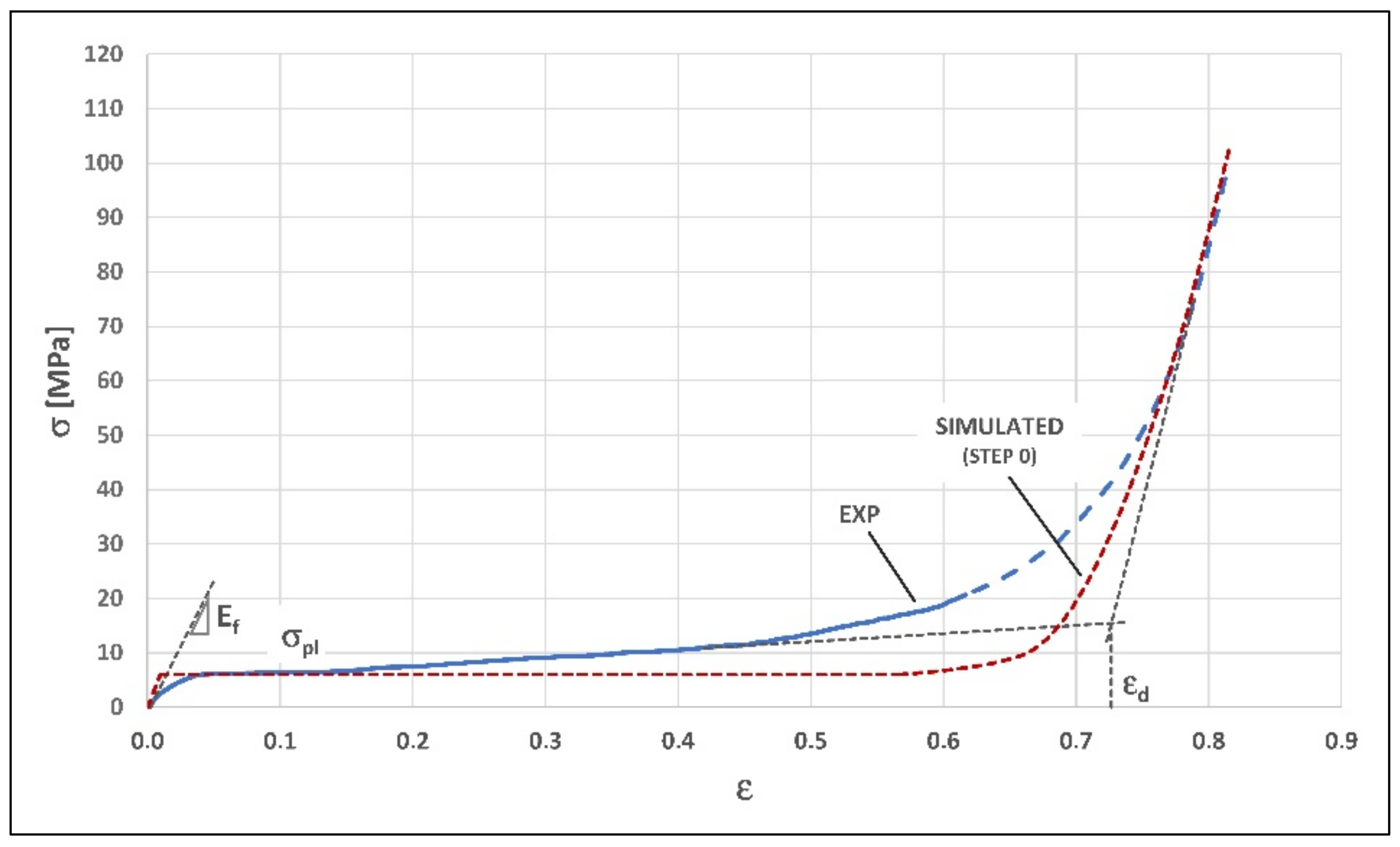
- Linear elastic regime at low stresses, for which the value of Young’s modulus Ef can be defined as the initial slope of the curve.
- Plastic collapse regime, associated with a long plateau at a stabilization stress value σpl, that is due to the combination between different collapse mechanisms at the cell walls (elastic buckling, plastic hinging, brittle crushing), depending on the nature of the material.
- Densification regime, with an interruption of the plateau region due to structure crushing and compacting, and rapid rise in stress with further strain; this region is usually characterized by a deformation parameter (densification strain εd).
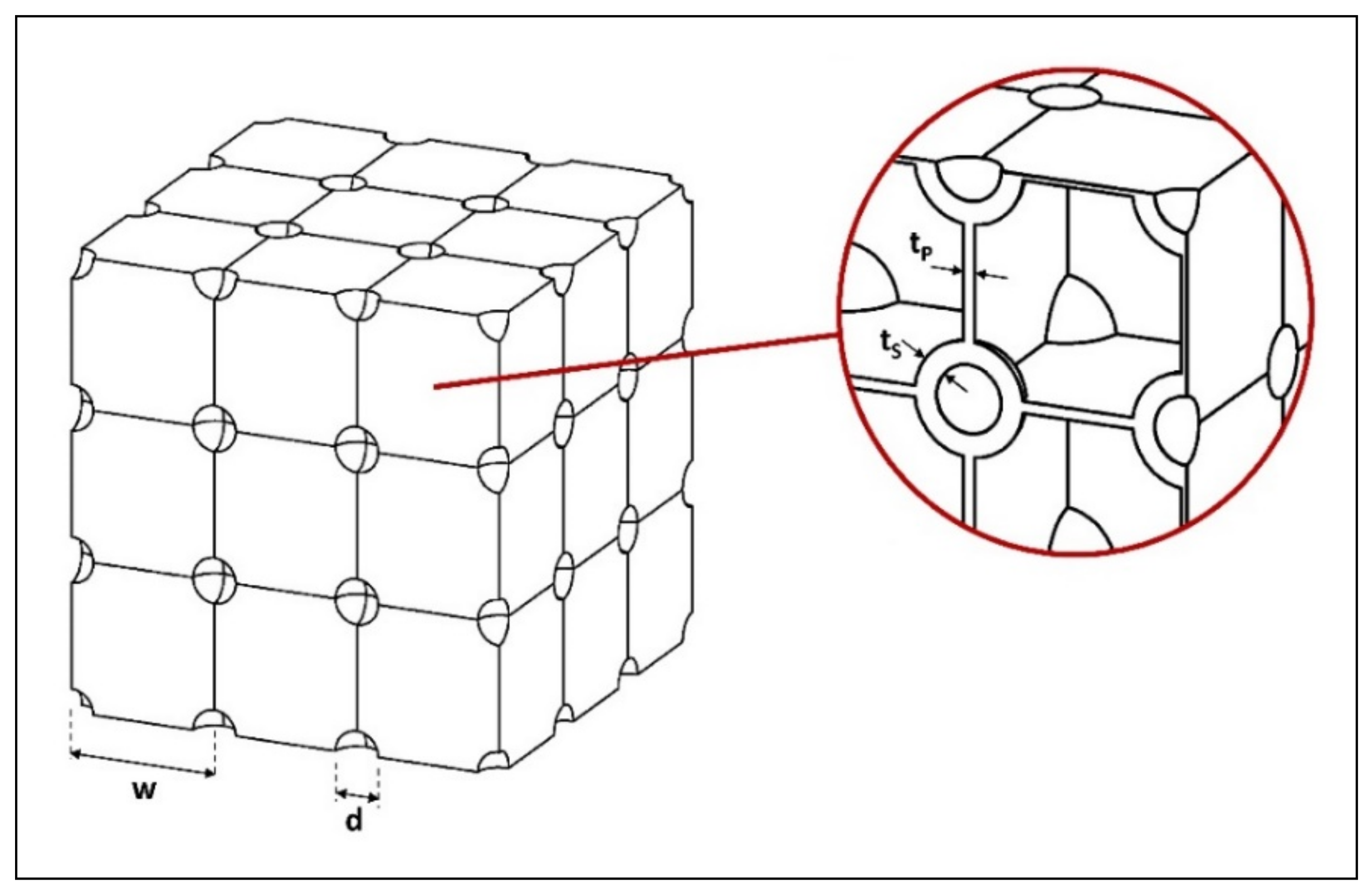
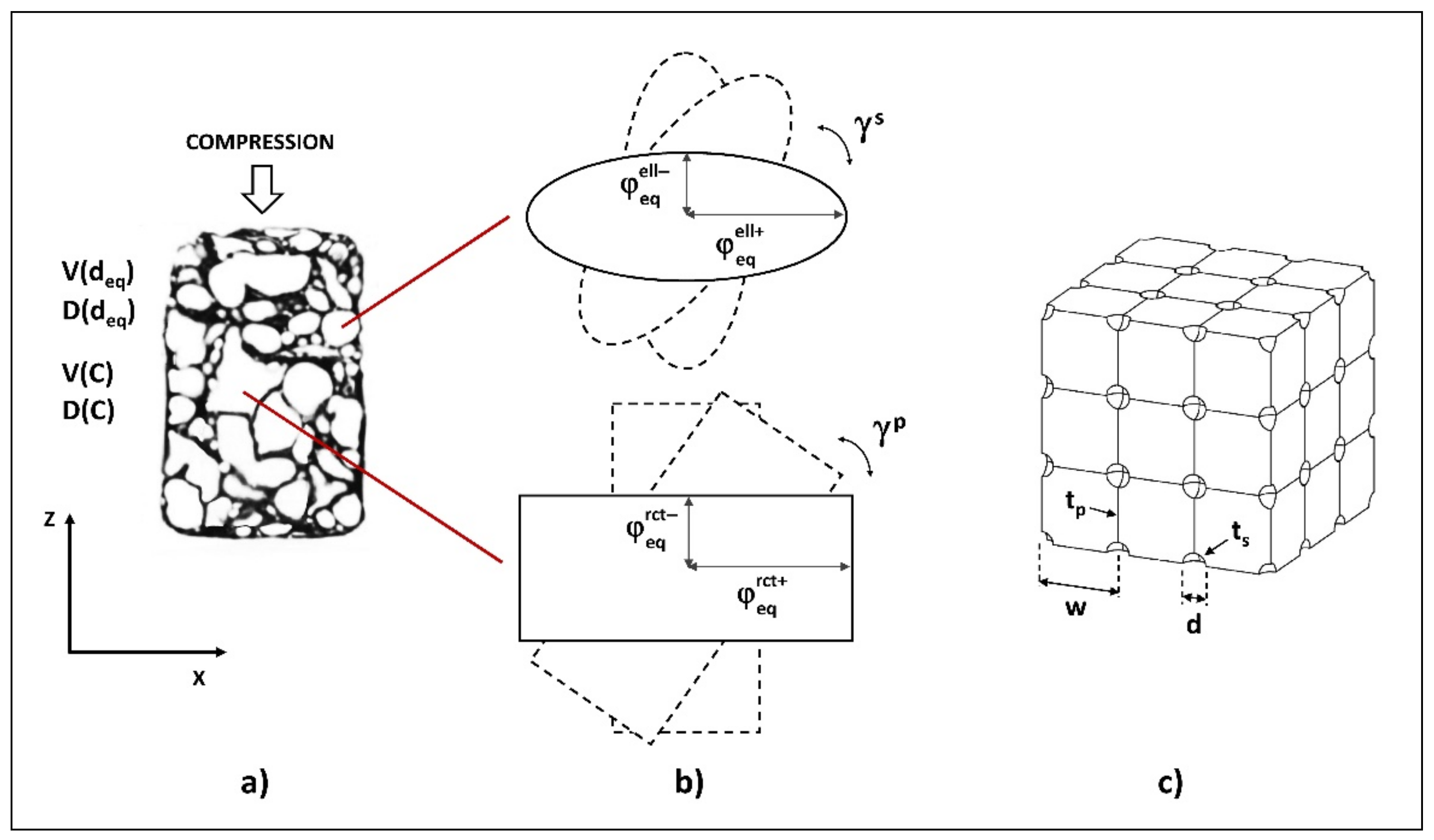
3.2. Cellular Structure Modeling
- The cells can be divided into two size classes (large and small cells).
- The smaller cells have high circularity and are characterized by spheroidal shapes; the larger cells tend to lose circularity and are characterized by prismatic shape.
- The wall thicknesses of the smaller cells are greater than the larger ones.
3.2.1. Stage 1
3.2.2. Stage 2
3.2.3. Stage 3
3.3. Simulation of Compression Behavior
3.4. Fitting of the Correlation Model
- γp and γs in Equations (12) and (13) (coefficients simulating the inclination of elliptical and rectangular sections of cells in the intermediate virtual model of cellular structure).
- λ in Equation (15) (ratio between the thickness of spherical and prismatic cells).
- c1, c2, c3 in Equations (16)–(18) (coefficients of stress–strain curve parameters).
- ξ and ψ in Equation (23) (coefficients of post-plateau compression curve).
4. Application and Discussion of Results
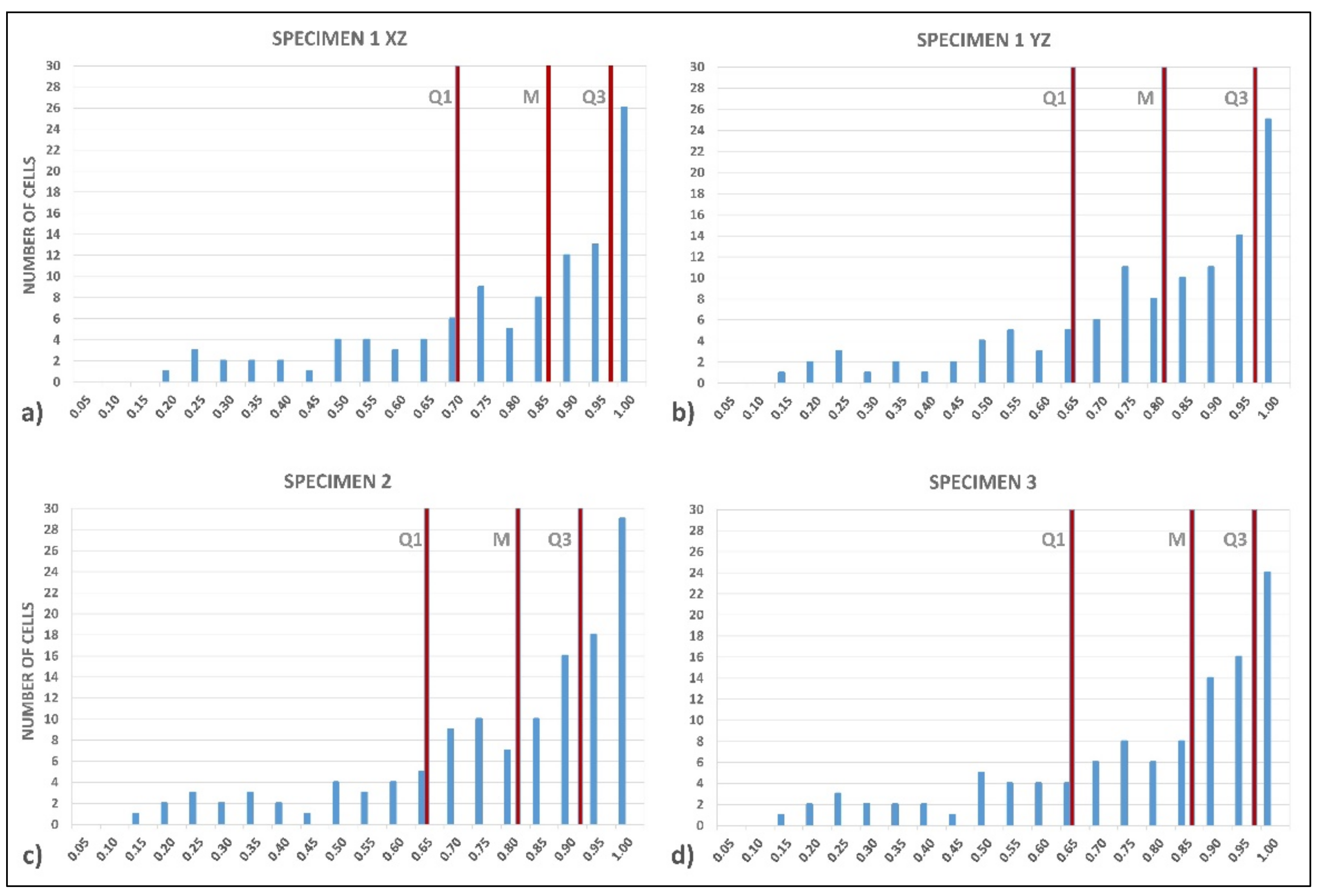
4.1. Compression Test and Morphology Analysis
- The sections present cells diversified according to different size classes, which can be reduced to small and large cells.
- The former have greater circularity and tend to spheroidal shape, whereas the latter lose circularity, and tend to a prismatic shape.
- The wall thicknesses of the smaller cells are generally greater than the larger ones.
4.2. Correlation Model Fitting and Mechanical Behavior Simulation
- to calculate the value of the relative density ρf/ρs by means of the Equation (3).
- to simulate the compression stress–strain curve by calculating the parameters Ef, σpl, εo, εd using the most suitable equations that correlate them to relative density, among those collected in the Section 3.3.
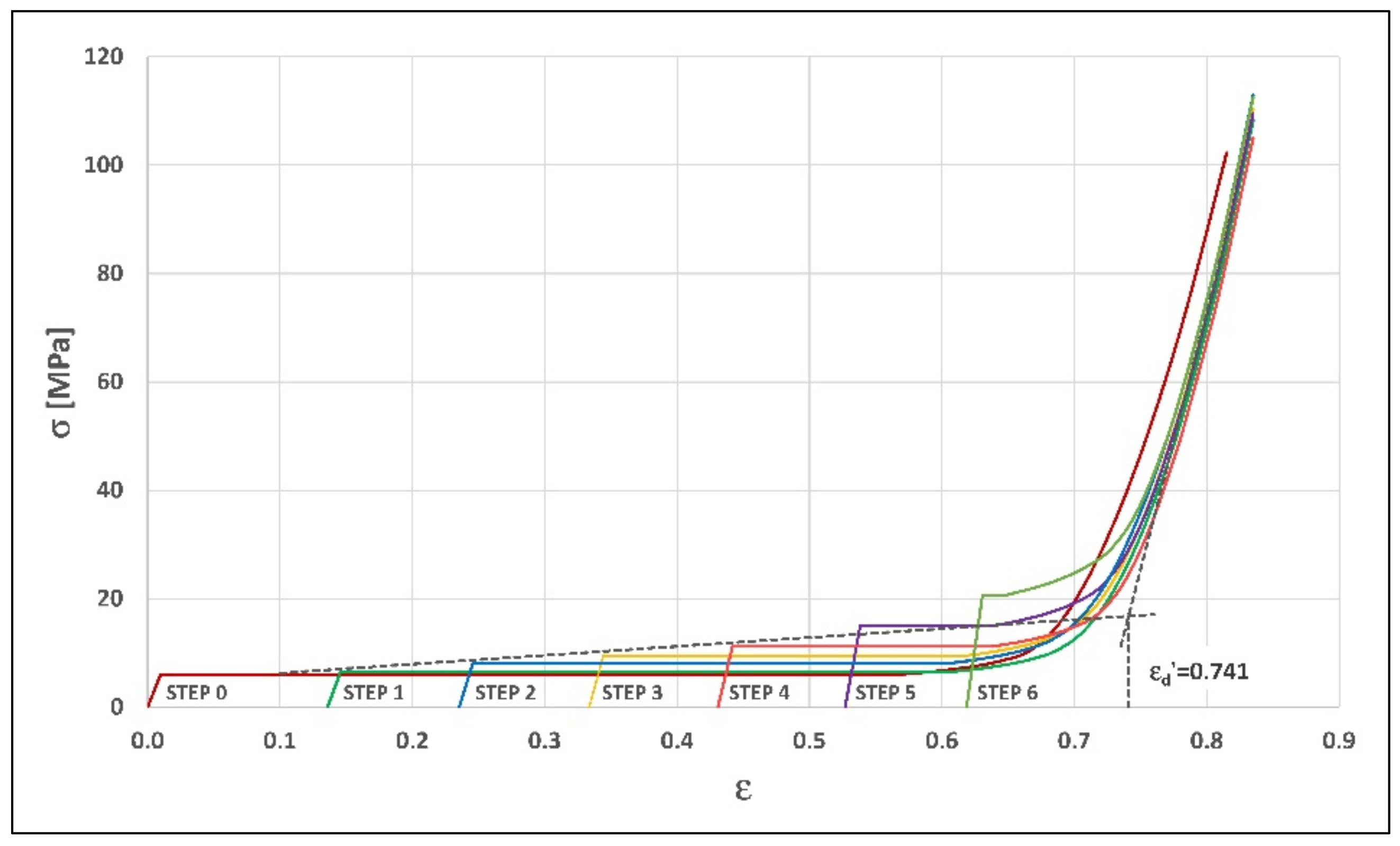
- By enveloping these curves, it is possible to define the rise trend of the plateau stress.
- The sections of the curves in densification regime tend to converge.
- The elastic regime at low stresses is assumed to be represented by the linear regime simulated for Step 0 morphology.
- The plastic collapse regime is obtained by enveloping the start points of the plateau of the simulated curves in Figure 11.
- The start point of the plateau for Step 6 simulated curve is assumed as the end point of plastic collapse regime and start point of post-plateau densification regime, so that the corresponding strain is assumed as densification onset strain εo’ of the enveloped stress–strain curve.
- The densification regime is obtained by iteration of densification curve calculation, starting from ε = εo’ and imposing εd = εd’ in Equation (23).
4.3. Robustness of the Correlation Model
- The morphological properties of closed-cell structures of metal foams that affect the mechanical behavior are similar between longitudinal sections, while they change significantly with respect to cross sections [38].
- The process of translating the local morphological parameters of the real sections into geometric parameters of the virtual model of the cellular structure, described in detail in Section 3, attenuates the effects of the local morphological characteristics.
4.4. Predictive Use of the Fitted Model
- The predictive values of the relative densities ρf/ρs for the subsequent compression steps were calculated by applying the strain-dependent law determined in the previous case by interpolation (Table 3, column 3), modulated with respect to the value of the relative density at Step 0 of the similar foams.
- The parameters for the stress–strain curves at each compression step were calculated using the aforementioned Equations (16), (18), (21)–(23), with the predictive values of relative density ρf/ρs, and the fitted values in Table 2 for c1, c3, ξ, ψ.
4.5. Effectiveness of the Model, Limitations, and Field of Application
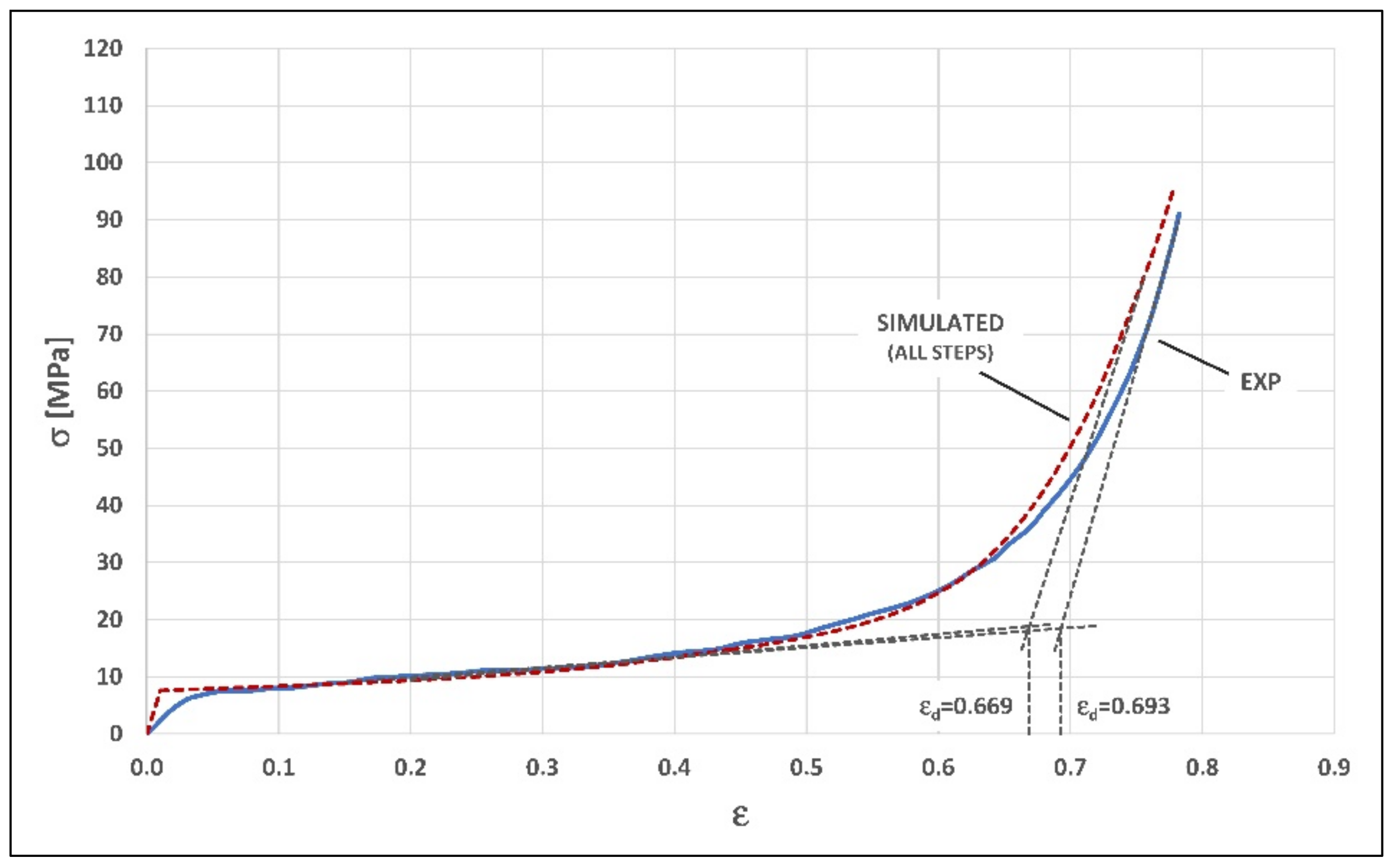
- Considering only the starting morphological properties, corresponding to Step 0 pre-compression state (Step 0 curve such as in Figure 10 for 0.4% TiH2 foam).
- Constructing the final compression curves according to the procedure defined in Section 4.2, that allowed to obtain the theoretical curves in Figure 12, Figure 15 and Figure 16 (all steps curves, obtained taking into account the evolution of the compression process up to collapse in fitting the model).
- As expected, the most accurate theoretical curve (whose compression parameters have the lowest error values compared to the experimental ones) is the all steps curve corresponding to the 4% TiH2 foam (Figure 12), which was entirely fitted on the corresponding experimental curve.
- In all cases, the improvement in accuracy due to fit the correlation model between morphology and mechanical behavior according to several compression steps (obtaining the all steps curves), compared to the results obtained by a morphological analysis limited to just one compression state, such as the initial pre-compression one (obtaining Step 0 curves), is clearly represented by the marked reductions in the corresponding errors with respect to the experimental curves (confirming what was anticipated in Section 4.2 regarding the construction of the compression curves).
- No analytical model in the literature was taken into consideration, due to the limited accuracy in the estimation of the compression parameters [18]; for this reason, the comparison was made with some of the numerical models presented in the introduction (Section 1), and chosen so to diversify the comparison and cover the main modeling types.
- Also in this case the compression parameters taken into consideration were the mean plateau stress σpl and the densification strain εd; while for the former it was always possible to extract the data from the literature, regarding the latter it must be pointed out that some models fail to provide a sufficiently clear reconstruction of the densification curve, so the corresponding densification strain cannot be defined (therefore in this cases it is missing in the comparison table).
- Only the percentage errors between the simulated and experimental values were used as significant indicators for the comparison, because they allow to quantify the accuracy of the models in the simulation of mechanical behavior, regardless of the properties of the cellular structure analyzed; furthermore, since it is clearly evident that the accuracy of the models varies with the relative density of the foam, it was considered appropriate to report this data as well.
- As representative models based on polyhedra, the cruciform-hemisphere model proposed by Meguid et al. [17] (NM3), and the Hasan’s weaker-cruciform-stronger-hemisphere model [19] (NM4); the latter was also assumed as reference model to set the preliminary ordered structure of the foam in the present study.
- As an example of advanced tessellation-based numerical modeling, the irregular Voronoi model implemented by Vengatachalam et al. [30] (NM5).
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Banhart, J. Manufacture, characterisation and application of cellular metals and metal foams. Prog. Mater. Sci. 2001, 46, 559–632. [Google Scholar] [CrossRef]
- Nazir, A.; Abate, K.M.; Kumar, A.; Jeng, J.-Y. A state-of-the-art review on types, design, optimization, and additive manufacturing of cellular structures. Int. J. Adv. Manuf. Tech. 2019, 104, 3489–3510. [Google Scholar] [CrossRef]
- Andrews, E.; Sanders, W.; Gibson, L.J. Compressive and tensile behaviour of aluminum foams. Mat. Sci. Eng. A 1999, 270, 113–124. [Google Scholar] [CrossRef]
- McCullough, K.Y.G.; Fleck, N.A.; Ashby, M.F. Uniaxial stress-strain behavior of aluminium alloy foams. Acta Mater. 1999, 47, 2323–2330. [Google Scholar] [CrossRef]
- Papadopoulus, D.P.; Konstantinidis, I.C.; Papanastasious, N.; Skolianos, S.; Tsipas, D.N. Mechanical properties of Al metal foams. Mater. Lett. 2004, 58, 2574–2578. [Google Scholar] [CrossRef]
- Paul, A.; Ramamurty, U. Strain rate sensitivity of a closed-cell aluminum foam. Mat. Sci. Eng. A 2000, 281, 1–7. [Google Scholar] [CrossRef]
- Shen, J.; Lu, G.; Ruan, D. Compressive behaviour of closed-cell aluminium foams at high strain rates. Compos. Part B Eng. 2010, 41, 678–685. [Google Scholar] [CrossRef]
- Arshad, A.B.; Nazir, A.; Jeng, J.-Y. The effect of fillets and crossbars on mechanical properties of lattice structures fabricated using additive manufacturing. Int. J. Adv. Manuf. Tech. 2020, 111, 931–943. [Google Scholar] [CrossRef]
- Ashby, M.F.; Evans, A.; Fleck, N.A.; Gibson, L.J.; Hutchinson, J.W.; Wadley, H.N.J. Metal Foams: A Design Guide; Butterworth-Heinemann: Oxford, UK, 2010. [Google Scholar]
- Yu, H.; Guo, Z.; Li, B.; Yao, G.; Luo, H.; Liu, Y. Research into the effect of cell diameter of aluminium foam on its compressive and energy absorption properties. Mat. Sci. Eng. A 2007, 454-455, 542–546. [Google Scholar] [CrossRef]
- Campana, F.; Pillone, D. Effect of wall microstructure and morphometric parameters on the crush behaviour of Al alloy foams. Mat. Sci. Eng. A 2008, 479, 58–64. [Google Scholar] [CrossRef]
- Mu, Y.; Yao, G.; Liang, L.; Luo, H.; Zu, G. Deformation mechanims of closed-cell aluminum foam in compression. Scripta Mater. 2010, 63, 629–632. [Google Scholar] [CrossRef]
- Song, H.W.; He, Q.J.; Xie, J.J.; Tobota, A. Fracture mechanisms and size effects of brittle metallic foams: In situ compression test inside SEM. Compos. Sci. Technol. 2008, 68, 2441–2450. [Google Scholar] [CrossRef] [Green Version]
- Hanssen, A.G.; Hopperstad, O.S.; Langseth, M.; Ilstad, H. Validation of constitutive models applicable to aluminium foams. Int. J. Mech. Sci. 2002, 44, 359–406. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Santosa, S.; Wierzbicki, T. On the modeling of crush behavior of a closed-cell aluminum foam structure. J. Mech. Phys. Solids 1998, 46, 645–669. [Google Scholar] [CrossRef]
- Meguid, S.A.; Cheon, S.S.; El-Abbasi, N. FE modelling of deformation localization in metallic foams. Finite Elem. Anal. Des. 2002, 38, 631–643. [Google Scholar] [CrossRef]
- Olurin, O.B.; Fleck, N.A.; Ashby, M.F. Deformation and fracture of aluminium foams. Mat. Sci. Eng. A 2000, 291, 136–146. [Google Scholar] [CrossRef] [Green Version]
- Hasan, M.A. An improvement model for FE modeling and simulation of closed cell Al-alloy foams. Adv. Mater. Sci. Eng. 2010, 567390. [Google Scholar]
- Konstantinidis, I.C.; Papadopoulus, D.P.; Lefakis, H.; Tsipas, D.N. Model for determining mechanical properties of aluminum closed-cell foams. Theor. Appl. Fract. Mech. 2005, 43, 157–167. [Google Scholar] [CrossRef]
- Kadkhodapour, J.; Raeisi, S. Micro–macro investigation of deformation and failure in closed-cell aluminum foams. Comp. Mater. Sci. 2014, 83, 137–148. [Google Scholar] [CrossRef]
- Kulcsár, K.; Kónya, J. Evaluation of closed and open-cell structural lattices with Finite Element Analysis. Acta Mater. Trans. 2020, 3, 26–32. [Google Scholar] [CrossRef]
- Sun, Y.; Zhang, X.; Shao, Z.; Li, Q.M. Image-based correlation between the meso-scale structure and deformation of closed-cell foam. Mat. Sci. Eng. A 2017, 688, 27–39. [Google Scholar] [CrossRef] [Green Version]
- Liu, C.; Zhang, Y.X.; Yang, C. Numerical modelling of mechanical behaviour of aluminium foam using a representative volume element method. Int. J. Mech. Sci. 2016, 118, 155–165. [Google Scholar] [CrossRef]
- Ghazi, A.; Tiago, C.; Sonon, B.; Berke, P.; Massart, T.J. Efficient computational modelling of closed cell metallic foams using a morphologically controlled shell geometry. Int. J. Mech. Sci. 2020, 168, 105298. [Google Scholar] [CrossRef] [Green Version]
- Redenbach, C.; Shklyar, I.; Andrä, H. Laguerre tessellations for elastic stiffness simulations of closed foams with strongly varying cell sizes. Int. J. Eng. Sci. 2012, 50, 70–78. [Google Scholar] [CrossRef]
- Li, L.; Xue, P.; Chen, Y.; Butt, H.S.U. Insight into cell size effects on quasi-static and dynamic compressive properties of 3D foams. Mat. Sci. Eng. A 2015, 636, 60–69. [Google Scholar] [CrossRef]
- Shi, X.; Liu, S.; Nie, H.; Lu, G.; Li, Y. Study of cell irregularity effects on the compression of closed-cell foams. Int. J. Mech. Sci. 2018, 135, 215–225. [Google Scholar] [CrossRef]
- Su, B.-Y.; Huang, C.-M.; Sheng, H.; Jang, W.-Y. The effect of cell-size dispersity on the mechanical properties of closed-cell aluminum foam. Mater. Charact. 2018, 135, 203–212. [Google Scholar] [CrossRef]
- Vengatachalam, B.; Poh, L.H.; Liu, Z.S.; Qin, Q.H.; Swaddiwudhiponga, S. Three dimensional modelling of closed-cell aluminium foams with predictive macroscopic behaviour. Mech. Mater. 2019, 136, 103067. [Google Scholar] [CrossRef]
- Novak, N.; Vesenjak, M.; Duarte, I.; Tanaka, S.; Hokamoto, K.; Krstulovic-Opara, L.; Guo, B.; Chen, P. Compressive behaviour of closed-cell aluminium foam at different strain rates. Materials 2019, 12, 4108. [Google Scholar] [CrossRef] [Green Version]
- Sharma, V.; Zivic, F.; Grujovic, N.; Babcsan, N.; Babcsan, J. Numerical modeling and experimental behavior of closed-cell aluminum foam fabricated by the gas blowing method under compressive loading. Materials 2019, 12, 1582. [Google Scholar] [CrossRef] [Green Version]
- Costanza, G.; Gusmano, G.; Montanati, R.; Tata, M.E.; Ucciardello, N. Effect of powder mix composition on Al foam morphology. Proc. Inst. Mech. Eng. Part L J. Mater. 2008, 222, 131–140. [Google Scholar] [CrossRef]
- Ou, X.; Zhang, X.; Lowe, T.; Blanc, R.; Norouzi Rad, M.; Wang, Y.; Batail, N.; Pham, C.; Shokri, N.; Garforth, A.A.; et al. X-ray micro computed tomography characterization of cellular SiC foams for their applications in chemical engineering. Mater. Charact. 2017, 123, 20–28. [Google Scholar] [CrossRef]
- Wang, N.; Maire, E.; Chenga, Y.; Amani, Y.; Li, Y.; Adrien, J.; Chen, X. Comparison of aluminium foams prepared by different methods using X-ray tomography. Mater. Charact. 2018, 138, 296–307. [Google Scholar] [CrossRef]
- Garcia-Moreno, F.; Mukherjee, M.; Jiménez, C.; Rack, A.; Banhart, J. Metal foaming investigated by X-ray radioscopy. Metals 2012, 2, 10–21. [Google Scholar] [CrossRef] [Green Version]
- Maire, E.; Elmoutaouakkil, A.; Fazekas, A.; Salvo, L. In situ X-ray tomography measurements of deformation in cellular solids. MRS Bull. 2003, 28, 284–289. [Google Scholar] [CrossRef]
- Benouali, A.H.; Froyen, L.; Dillard, T.; Forest, S.; N’Guyen, F. Investigation on the influence of cell shape anisotropy on the mechanical performance of closed cell aluminium foams using micro-computed tomography. J. Mater. Sci. 2005, 40, 5801–5811. [Google Scholar] [CrossRef]
- Ulbin, M.; Vesenjak, M.; Borovinšek, M.; Duarte, I.; Higa, Y.; Shimojima, K.; Ren, Z. Detailed analysis of closed-cell aluminum alloy foam internal structure changes during compressive deformation. Adv. Eng. Mater. 2018, 20, 1800164. [Google Scholar] [CrossRef]
- Costanza, G.; Mantineo, F.; Missori, S.; Sili, A.; Tata, M.E. Characterization of the compressive behaviour of an Al foam by X-ray computerized tomography. In Light Metals 2012; Suarez, C., Ed.; John Wiley & Sons: New York, NY, USA, 2012; pp. 533–536. [Google Scholar]
- Costanza, G.; Mantineo, F.; Sili, A.; Tata, M.E. Characterization of Cu tube filled with Al alloy foam by means of X-ray computer tomography. In TMS 2014 Annual Meeting Supplemental Proceedings; John Wiley & Sons: Hoboken, NJ, USA, 2014; pp. 613–619. [Google Scholar]
- Costanza, G.; Sili, A.; Tata, M.E. Mechanical characterization of AISI 316 tubes filled with Al alloy foams. Metall. Ital. 2015, 3, 9–14. [Google Scholar]
- Park, C.; Nutt, S.R. Effect of process parameters on steel foam synthesis. Mat. Sci. Eng. A 2001, 297, 62–68. [Google Scholar] [CrossRef]
- Bonaccorsi, L.; Garescì, F.; Giacobbe, F.; Freni, F.; Mantineo, F.; Montanini, R.; Sili, A. Applications in metallurgy of X-ray computed tomography with variable focal spot-size and infrared thermography. Metall. Ital. 2013, 7/8, 33–40. [Google Scholar]
- Chan, K.C.; Xie, L.S. Dependency of densification properties on cell topology of metal foams. Scripta Mater. 2003, 48, 1147–1152. [Google Scholar] [CrossRef]
- Simone, A.E.; Gibson, L.J. Effects of solid distribution on the stiffness and strength of metallic foams. Acta Mater. 1998, 46, 2139–2150. [Google Scholar] [CrossRef]
- Simone, A.E.; Gibson, L.J. Aluminium foam produced by liquid-state processed. Acta Mater. 1998, 46, 3109–3123. [Google Scholar] [CrossRef]
- Byakova, A.; Gnyloskurenko, S.; Nakamura, T. The role of foaming agent and processing route in the mechanical performance of fabricated aluminum foams. Metals 2012, 2, 95–112. [Google Scholar] [CrossRef] [Green Version]
- Jang, W.-Y.; Hsieh, W.-Y.; Miao, C.-C.; Yen, Y.-C. Microstructure and mechanical properties of ALPORAS closed-cell aluminium foam. Mater. Charact. 2015, 107, 228–238. [Google Scholar] [CrossRef]
- Khezrzadeh, O.; Mirzaee, O.; Emadoddin, E.; Linul, E. Anisotropic compressive behavior of metallic foams under extreme temperature conditions. Materials 2020, 13, 2329. [Google Scholar] [CrossRef] [PubMed]
- Banhart, J.; Baumeister, J. Deformation characteristics of metal foams. J. Mater. Sci. 1998, 33, 1431–1440. [Google Scholar] [CrossRef]
- Wu, G.H.; Dou, Z.Y.; Sun, D.L.; Jiang, L.T.; Ding, B.S.; He, B.F. Compression behaviors of cenosphere–pure aluminum syntactic foams. Scripta Mater. 2007, 56, 221–224. [Google Scholar] [CrossRef]

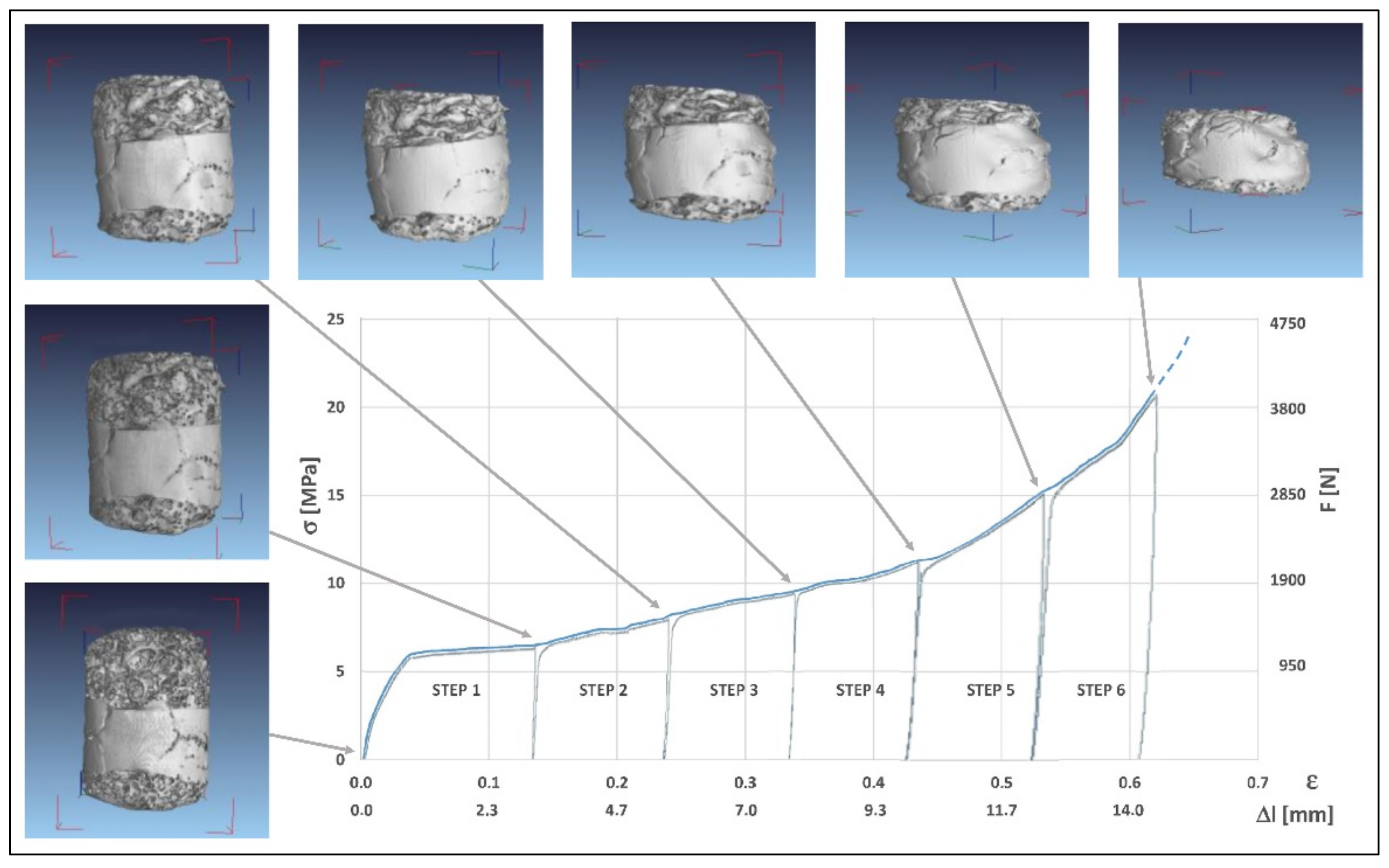
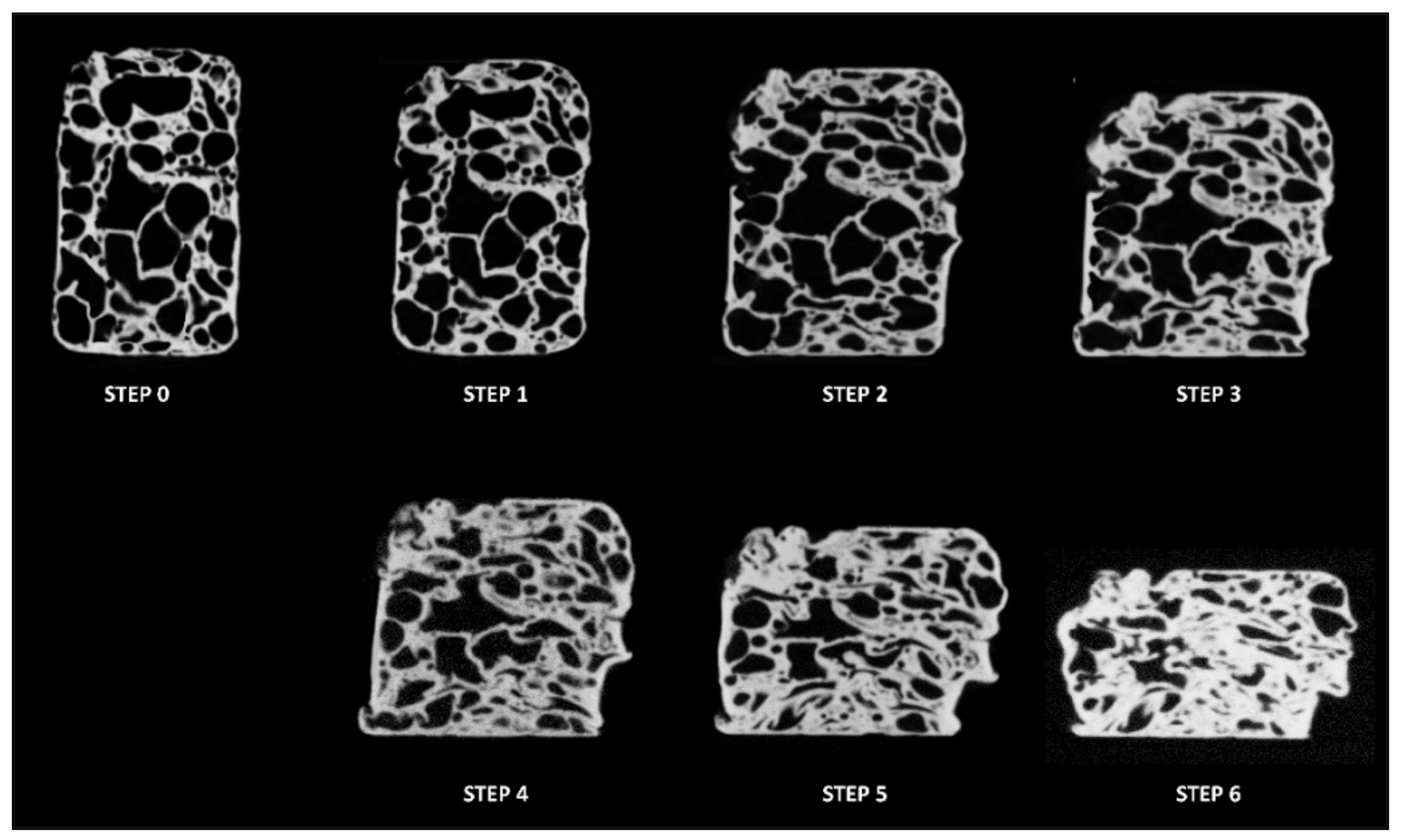
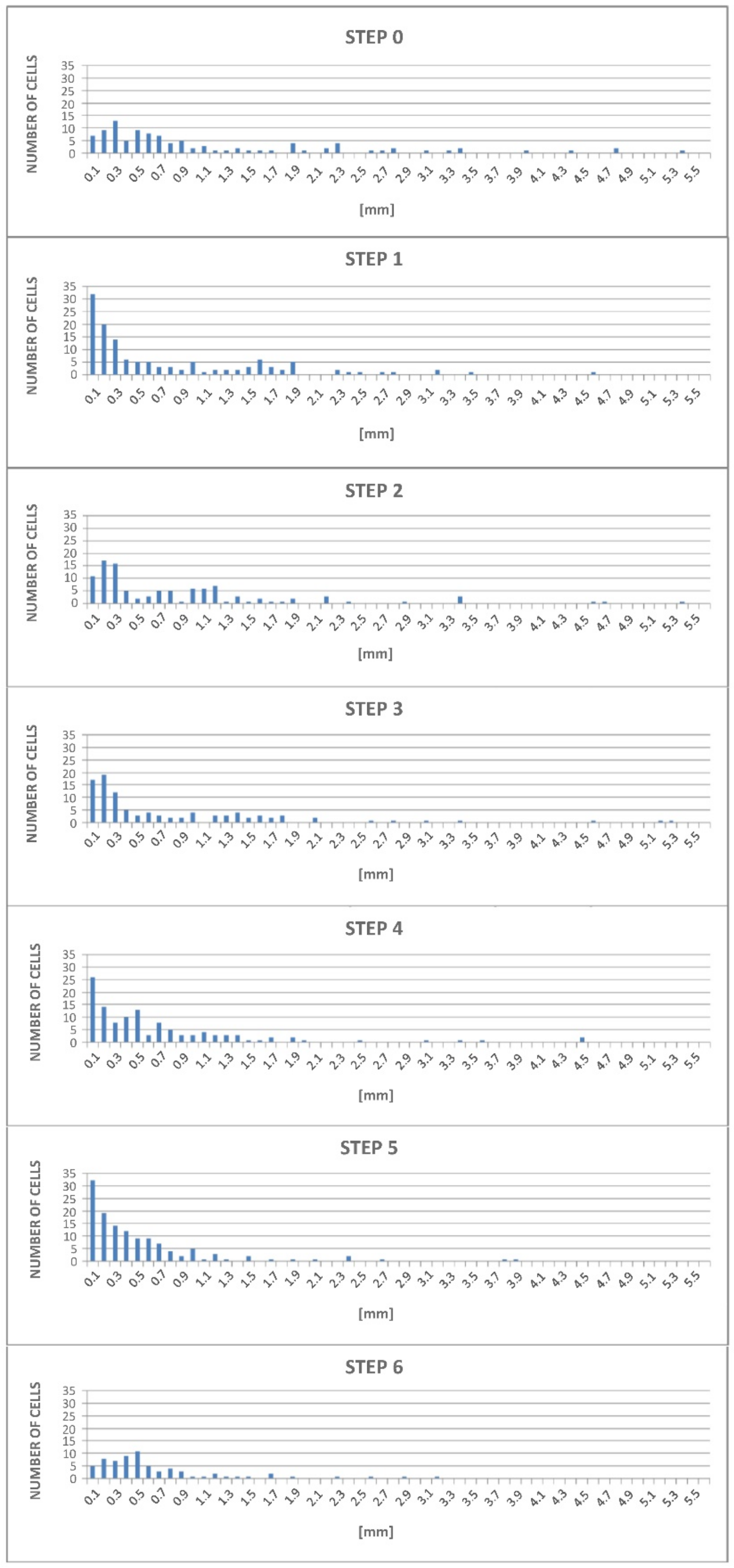
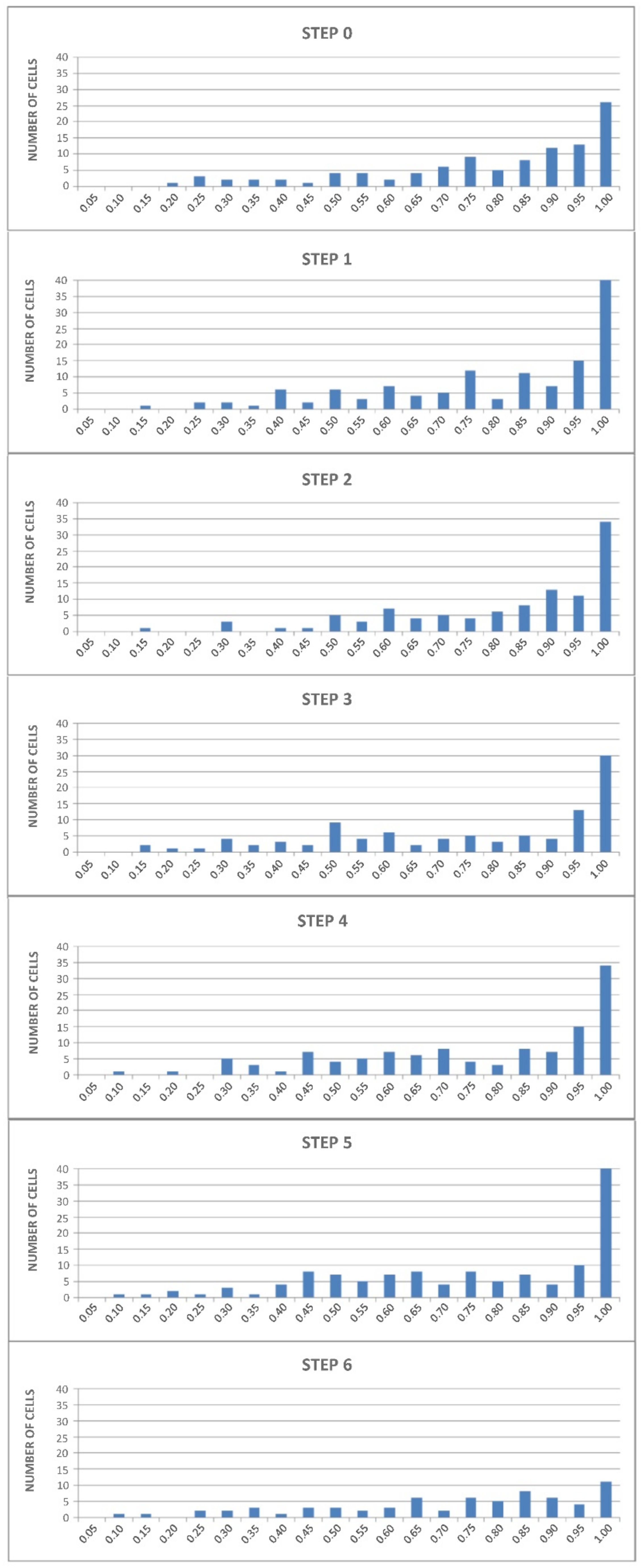



| Steps | Deformation (%) | Initial Height (mm) | Final Height (mm) | Initial Diam (mm) | Final Diam (mm) |
|---|---|---|---|---|---|
| Starting Condition (Step 0) | - | - | 23.33 | - | 15.35 |
| Compression (Step 1) | 13.6 | 23.33 | 20.16 | 15.35 | 15.55 |
| Compression (Step 2) | 23.5 | 20.16 | 17.84 | 15.55 | 15.58 |
| Compression (Step 3) | 33.3 | 17.84 | 15.55 | 15.58 | 16.19 |
| Compression (Step 4) | 43.1 | 15.55 | 13.28 | 16.19 | 16.39 |
| Compression (Step 5) | 52.7 | 13.28 | 11.04 | 16.39 | 17.14 |
| Compression (Step 6) | 61.9 | 11.04 | 8.90 | 17.14 | 18.11 |
| Steps | Fitted Coefficient of Correlation Model | Global Parameters of Structure | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| γp | γs | λ | c1 | c3 | ξ | ψ | w [μm] | d [μm] | tp [μm] | ts [μm] | |
| Step 0 | 0.87 | 1.04 | 2.87 | 0.10 | 0.96 | 1.94 | 0.40 | 1206 | 165 | 68 | 195 |
| Step 1 | 1.02 | 1.12 | 3.27 | 0.10 | 0,98 | 2.11 | 0.44 | 1465 | 195 | 82 | 266 |
| Step 2 | 1.52 | 1.16 | 3.86 | 0.10 | 1.00 | 2.13 | 0.43 | 1828 | 190 | 106 | 409 |
| Step 3 | 1.57 | 1.12 | 4.23 | 0.10 | 0.97 | 2.04 | 0.40 | 1897 | 173 | 113 | 478 |
| Step 4 | 1.42 | 1.08 | 4.71 | 0.10 | 0.96 | 1.99 | 0.37 | 1539 | 148 | 91 | 426 |
| Step 5 | 1.44 | 1.14 | 5.34 | 0.10 | 1.00 | 1.90 | 0.35 | 1075 | 115 | 63 | 337 |
| Step 6 | 1.49 | 1.20 | 5.46 | 0.10 | 0.97 | 1.81 | 0.32 | 1210 | 123 | 78 | 424 |
| Steps | Relative Density | Parameters of Simulated Curve | |||||
|---|---|---|---|---|---|---|---|
| ε | ρf/ρs (Equation (3)) | ρf/ρs (Interpolation) | Ef [GPa] | σpl [MPa] | εd | εo | |
| Step 0 | 0.000 | 0.217 | 0.886ε3 − 0.161ε2 + 0.156ε + 0.215 (R2 = 0.997) | 0.62 | 6.01 | 0.701 | 0.567 |
| Step 1 | 0.136 | 0.228 | 0.66 | 6.50 | 0.729 | 0.606 | |
| Step 2 | 0.235 | 0.260 | 0.78 | 8.02 | 0.728 | 0.603 | |
| Step 3 | 0.333 | 0.287 | 0.89 | 9.44 | 0.738 | 0.617 | |
| Step 4 | 0.431 | 0.318 | 1.02 | 11.18 | 0.754 | 0.638 | |
| Step 5 | 0.527 | 0.381 | 1.31 | 15.02 | 0.758 | 0.640 | |
| Step 6 | 0.619 | 0.461 | 1.71 | 20.65 | 0.769 | 0.648 | |
| Sections | Relative Density | Parameters of Simulated Curve | |||
|---|---|---|---|---|---|
| ρf/ρs | Ef [GPa] | σpl [MPa] | εd | εo | |
| Specimen 1 XZ | 0.217 | 0.62 | 6.01 | 0.701 | 0.567 |
| Specimen 1 YZ | 0.213 | 0.61 | 5.84 | 0.706 | 0.574 |
| Sections | Relative Density | Parameters of Simulated Curve | |||
|---|---|---|---|---|---|
| ρf/ρs | Ef [GPa] | σpl [MPa] | εd | εo | |
| Specimen 1 | 0.217 | 0.62 | 6.01 | 0.701 | 0.567 |
| Specimen 2 | 0.224 | 0.65 | 6.33 | 0.691 | 0.552 |
| Specimen 3 | 0.212 | 0.60 | 5.80 | 0.707 | 0.576 |
| Mean Value | 0.218 | 0.62 | 6.05 | 0.700 | 0.565 |
| Standard Dev | 0.006 | 0.03 | 0.27 | 0.008 | 0.012 |
| Foam | Type of Data | σpl [MPa] | εd |
|---|---|---|---|
| 4% TiH2 | Experimental | 8.50 | 0.727 |
| Step 0 Curve | 6.00 (29.4%) | 0.701 (3.6%) | |
| All Steps Curve | 8.20 (3.5%) | 0.715 (1.6%) | |
| 1% TiH2 | Experimental | 15.80 | 0.605 |
| Step 0 Curve | 9.70 (38.6%) | 0.581 (3.9%) | |
| All Steps Curve | 13.27 (16.0%) | 0.598 (1.1%) | |
| 2% TiH2 | Experimental | 11.20 | 0.693 |
| Step 0 Curve | 7.58 (32.3%) | 0.655 (5.5%) | |
| All Steps Curve | 9.97 (11.0%) | 0.669 (3.5%) |
| Model | Specifications | ρf/ρs | σpl (%) | εd (%) | Ref. |
|---|---|---|---|---|---|
| PAM | 4% TiH2 | 0.217 | 3.5 | 1.6 | |
| 1% TiH2 | 0.294 | 16.0 | 1.1 | ||
| 2% TiH2 | 0.251 | 11.0 | 3.5 | ||
| NM1 | Circular 1 | 0.600 | 27.2 | – | [20] |
| Elliptic | 0.600 | 4.3 | – | ||
| Rectangular | 0.570 | 35.8 | – | ||
| Square | 0.610 | 68.5 | – | ||
| NM2 | Multicell 2 | 0.083 | 3.4 | 5.9–7.3 | [21] |
| 0.109 | 7.4 | 4.0–4.5 | |||
| NM3 | Uniform Density 3 | 0.100 | 50.0 | – | [17] |
| Randomized Density 4 | 0.081-0.143 | 6.5 | – | ||
| NM4 | WCSH Model 5 | 0.120 | 32.0 | – | [19] |
| 0.150 | 16.0 | – | |||
| 0.200 | 6.0 | – | |||
| NM5 | Voronoi Model | 0.117 | 12.0 | 3.5 | [30] |
| 0.172 | 11.0 | 12.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Costanza, G.; Giudice, F.; Sili, A.; Tata, M.E. Correlation Modeling between Morphology and Compression Behavior of Closed-Cell Al Foams Based on X-ray Computed Tomography Observations. Metals 2021, 11, 1370. https://doi.org/10.3390/met11091370
Costanza G, Giudice F, Sili A, Tata ME. Correlation Modeling between Morphology and Compression Behavior of Closed-Cell Al Foams Based on X-ray Computed Tomography Observations. Metals. 2021; 11(9):1370. https://doi.org/10.3390/met11091370
Chicago/Turabian StyleCostanza, Girolamo, Fabio Giudice, Andrea Sili, and Maria Elisa Tata. 2021. "Correlation Modeling between Morphology and Compression Behavior of Closed-Cell Al Foams Based on X-ray Computed Tomography Observations" Metals 11, no. 9: 1370. https://doi.org/10.3390/met11091370
APA StyleCostanza, G., Giudice, F., Sili, A., & Tata, M. E. (2021). Correlation Modeling between Morphology and Compression Behavior of Closed-Cell Al Foams Based on X-ray Computed Tomography Observations. Metals, 11(9), 1370. https://doi.org/10.3390/met11091370








