Fatigue Damage of an Asperity in Frictionless Normal Contact with a Rigid Flat
Abstract
1. Introduction
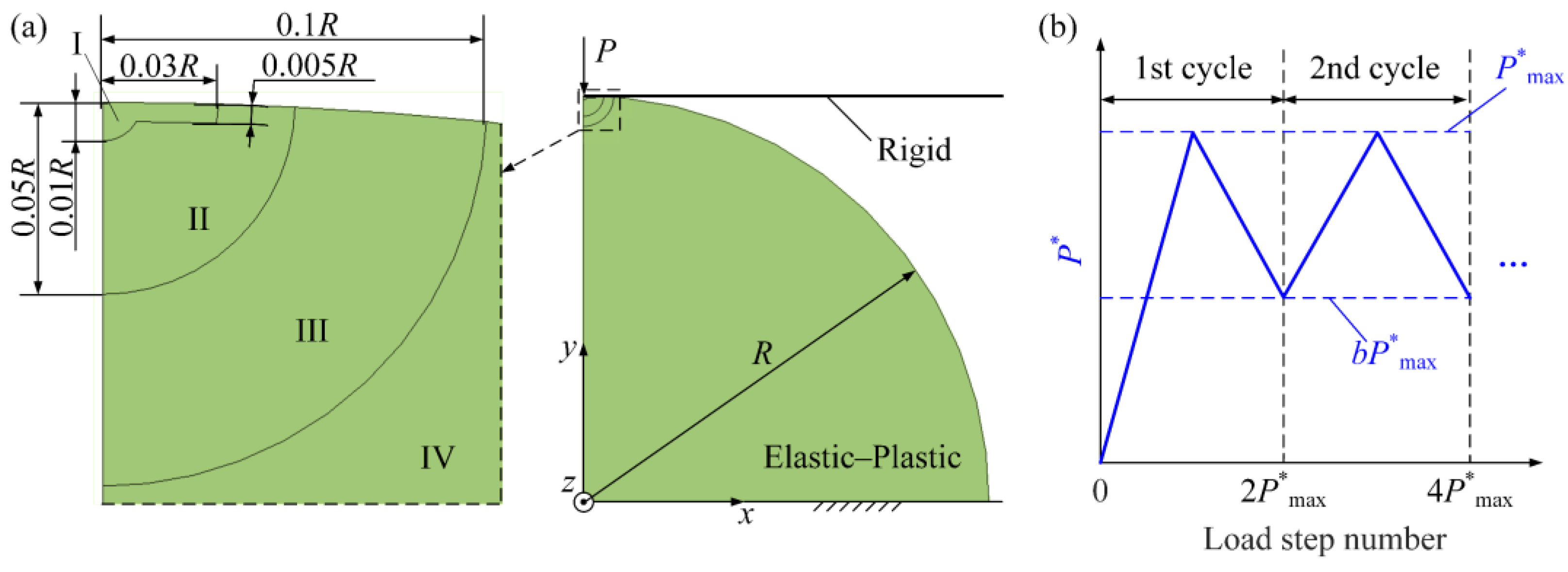
2. Elastic–Plastic Spherical Contact
3. Finite Element Model
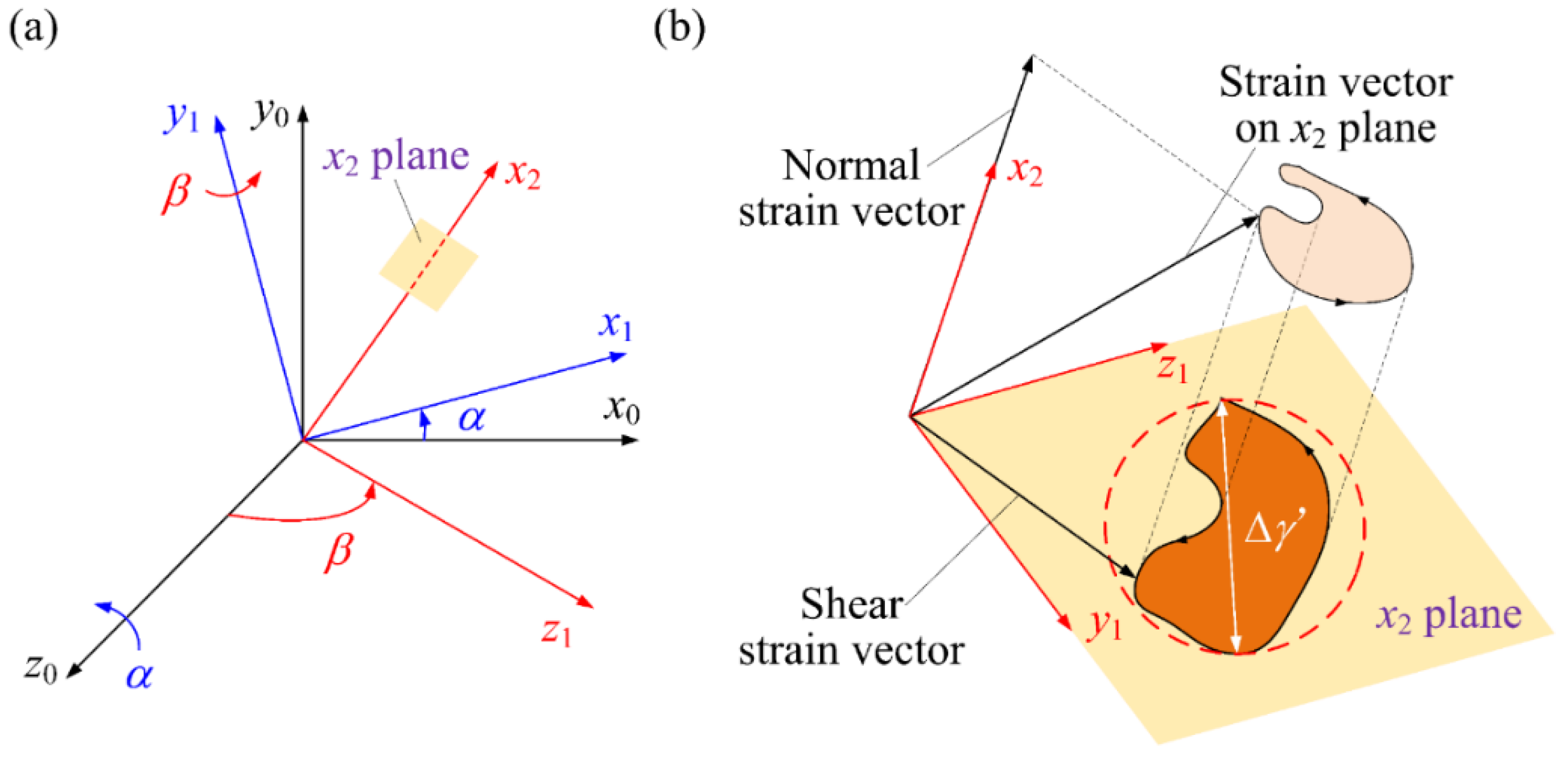
4. Fatemi-Socie Multiaxial Fatigue Criterion
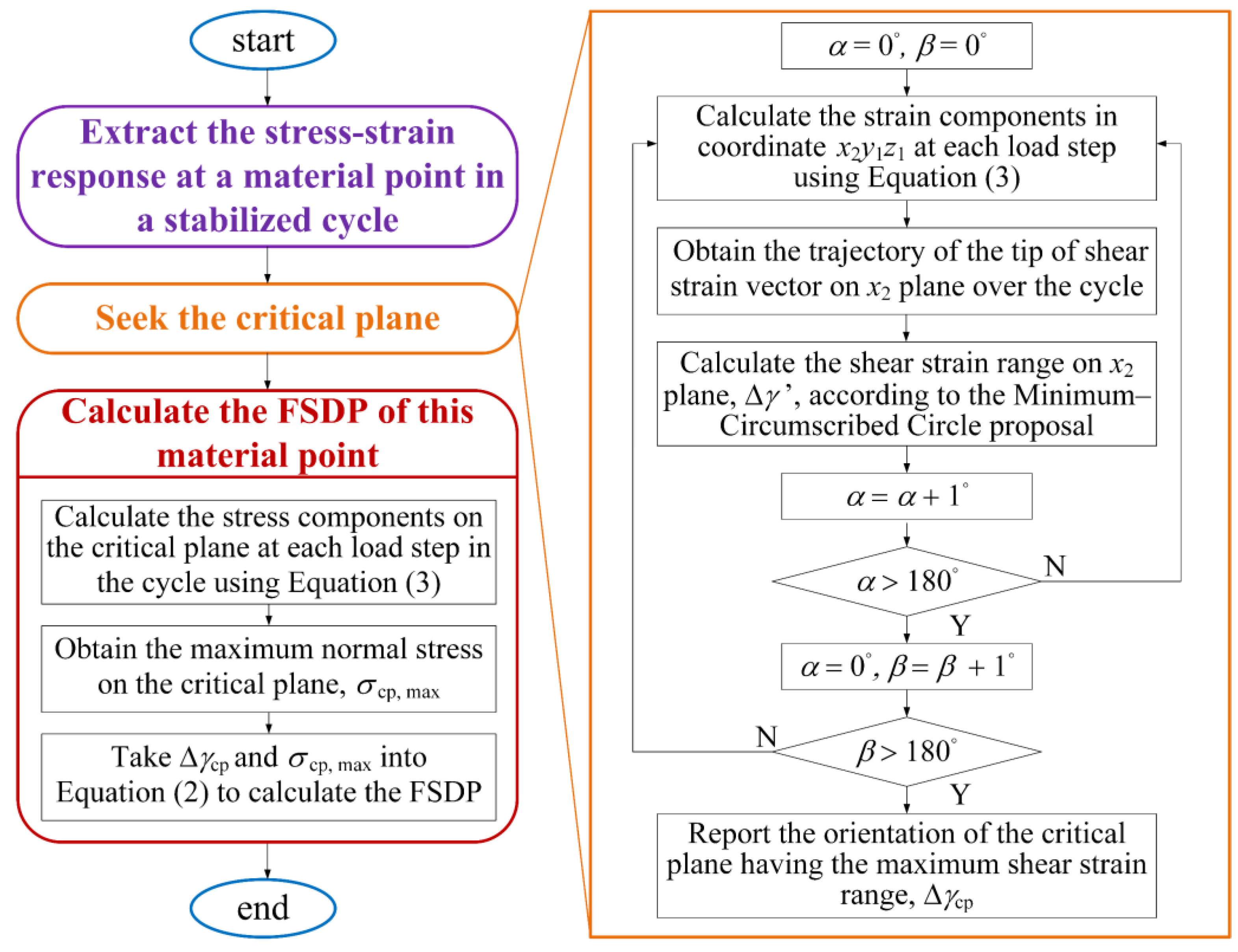
5. Framework of Determining the Critical Plane and FSDP
- 1.
- Extract the stabilized stress–strain response in shakedown state at each material point over one cycle from the FE model (see Section 3 for details);
- 2.
- Seek the critical plane at each material point.
- 3.
- Calculate the FSDP at each material point.
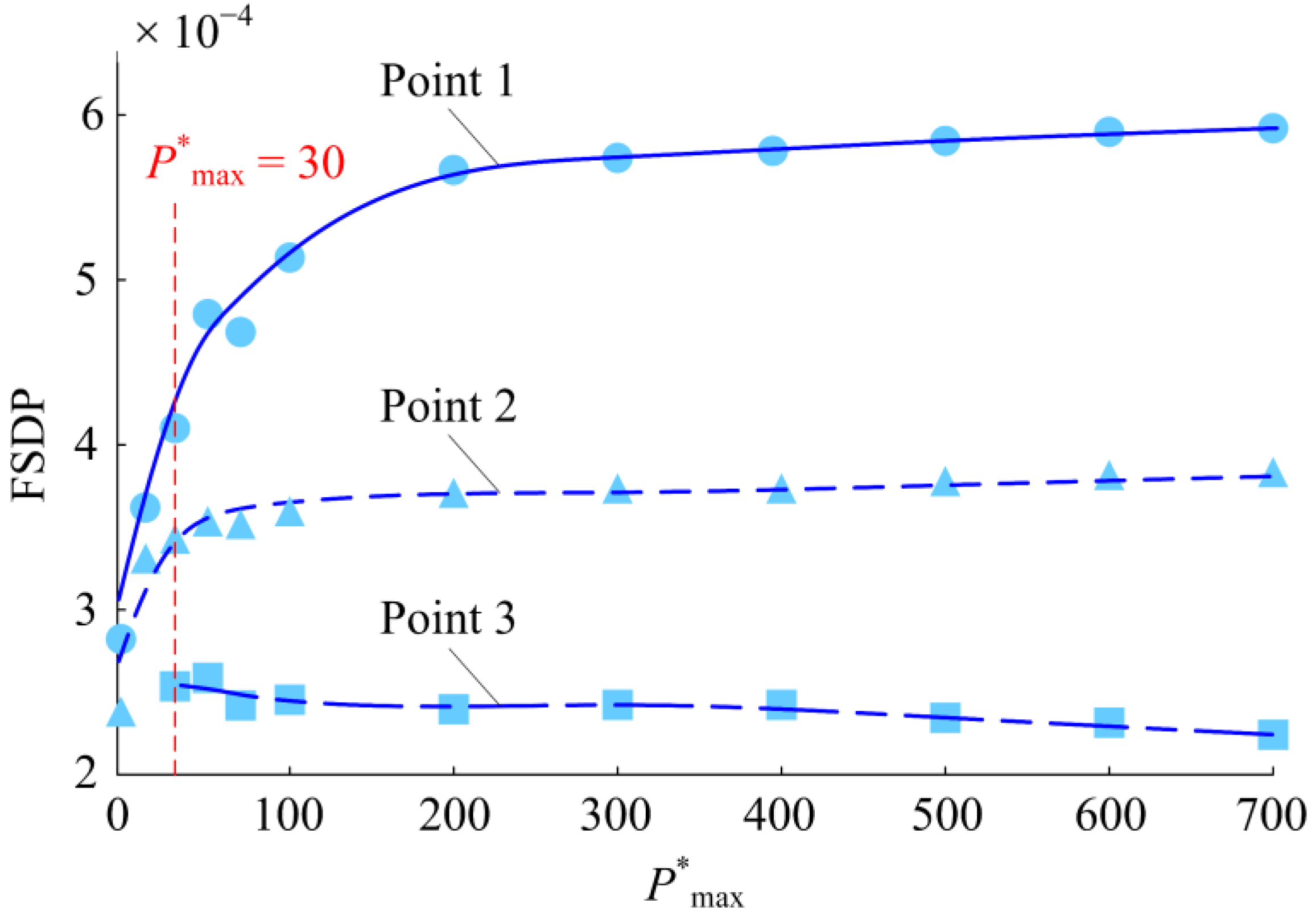
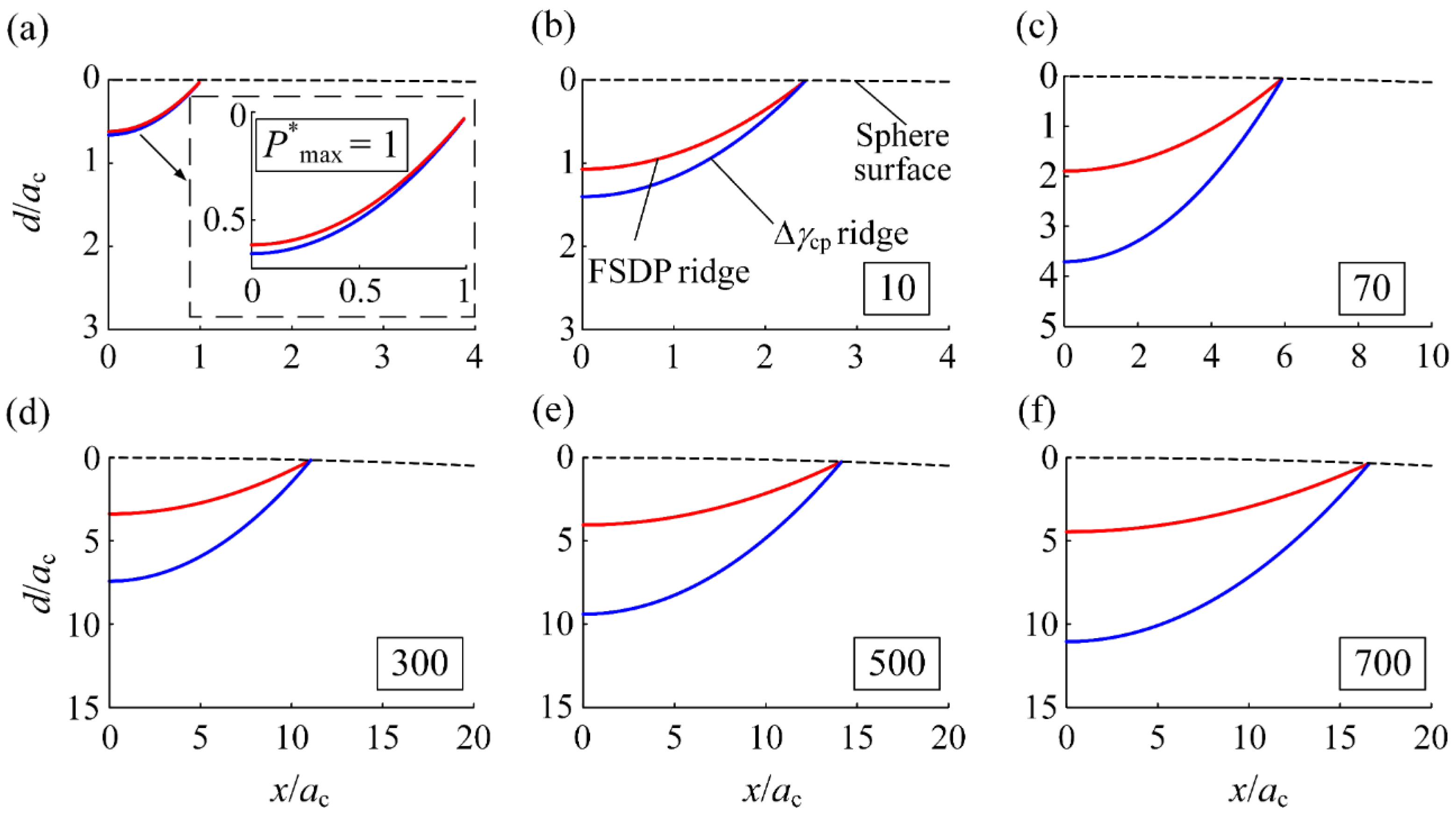
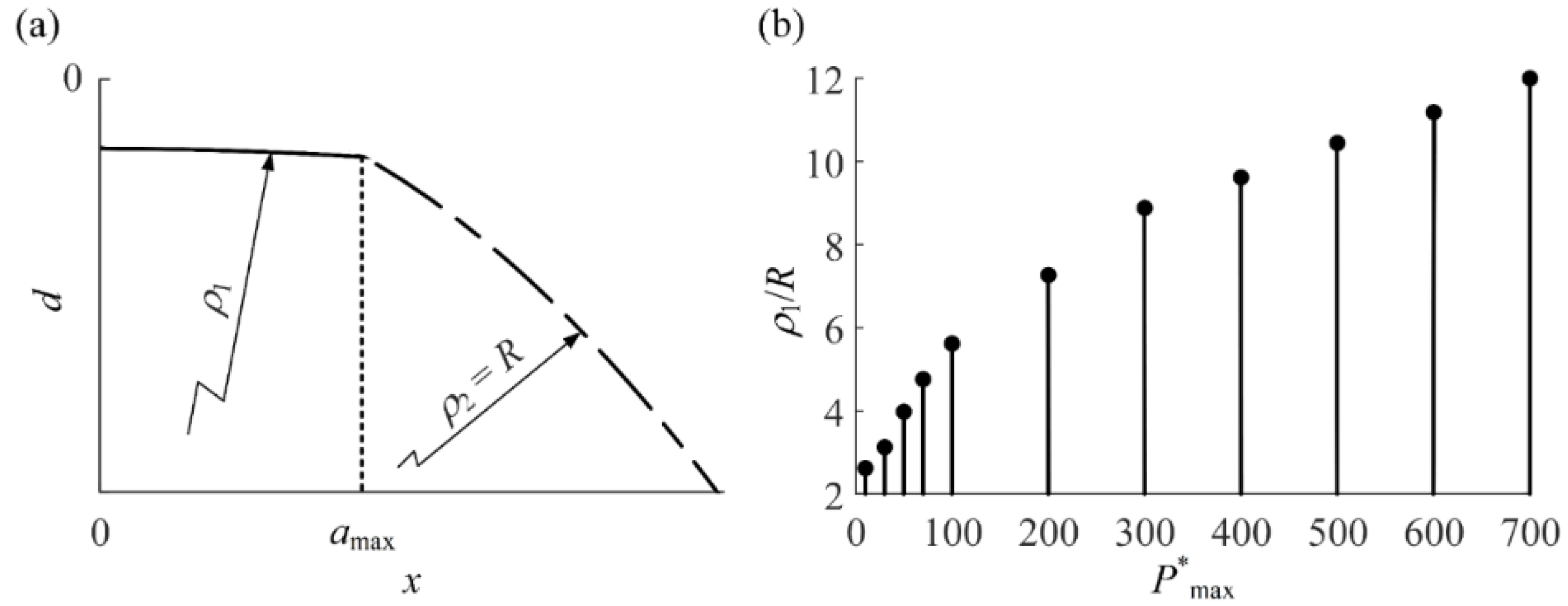
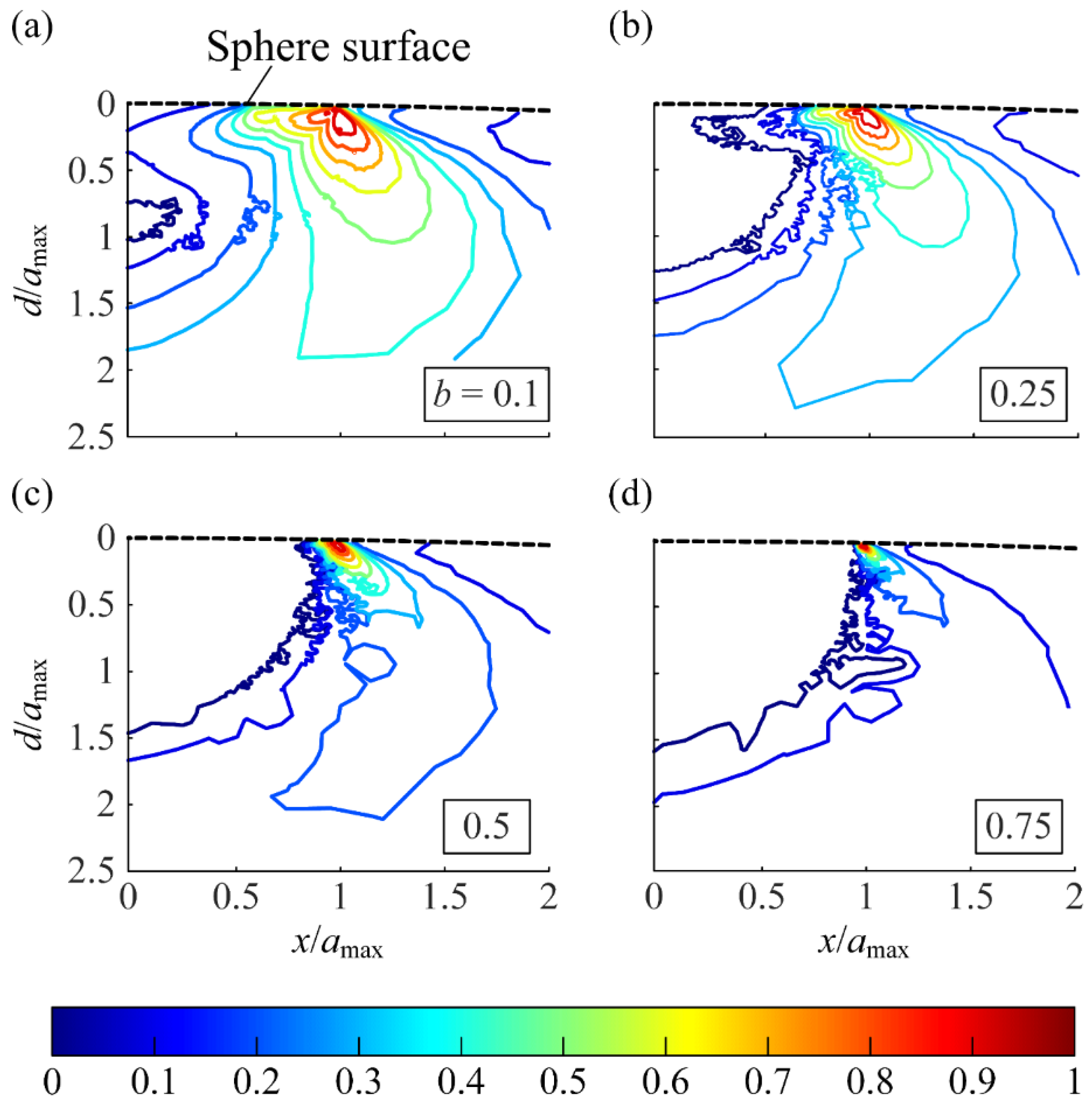
6. Results and Discussion
6.1. Shakedown Analysis
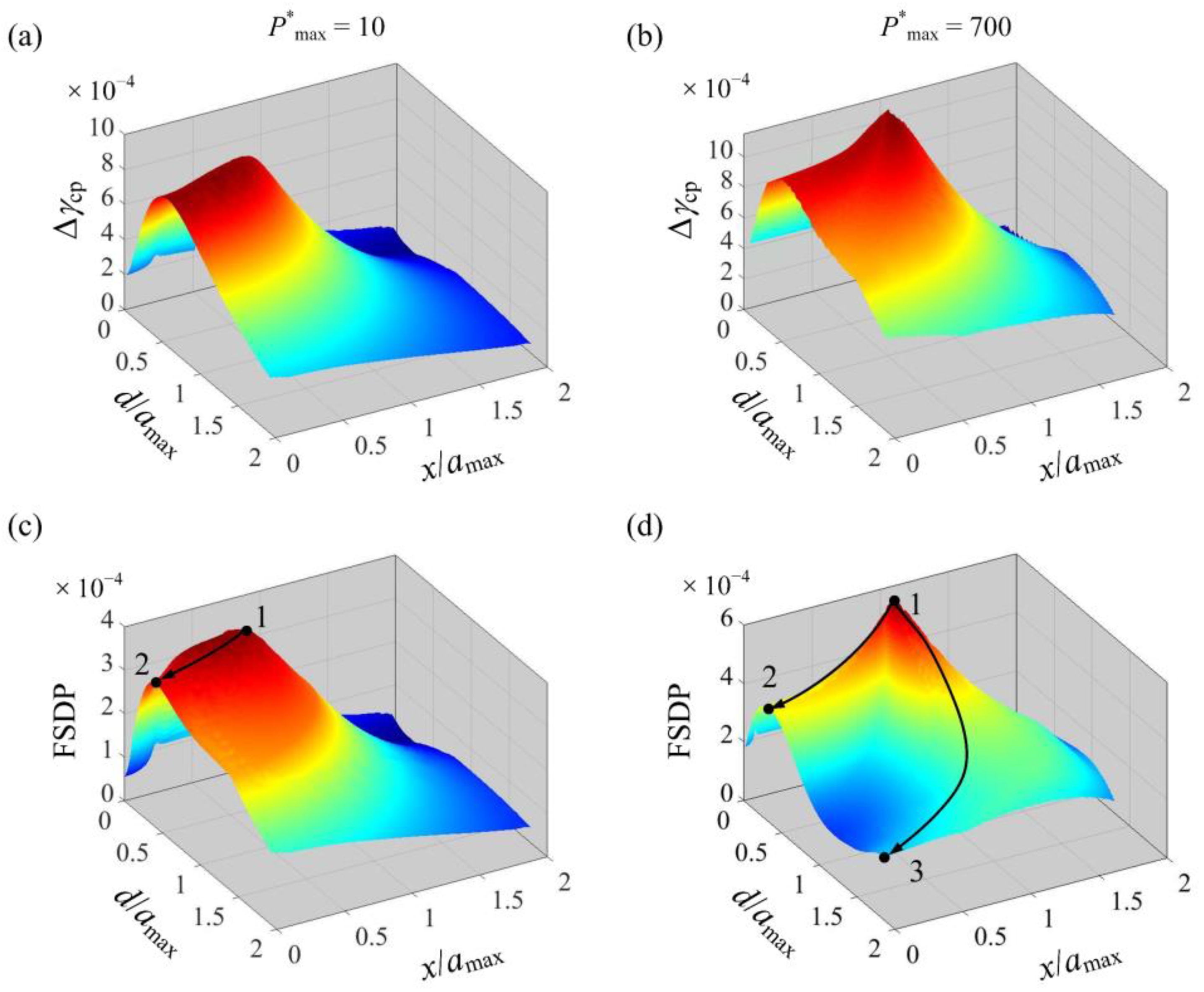
6.2. Complete Unloading Cases (b = 0)
6.3. Partial Unloading Cases (b ≠ 0)
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | contact area |
| a | contact radius |
| d | depth measured from the sphere summit |
| E | Young’s modulus |
| P | contact load |
| R | radius of the spherical asperity |
| Y | yield strength |
| FSDP | Fatemi-Socie damage parameter |
| critical plane | the plane that has the maximum shear strain range over a cycle |
| α, β | Euler angle that describes the orientation of a plane |
| εpeq | equivalent plastic strain |
| Δγ | shear strain range on a plane over a cycle |
| Δγcp | shear strain range on the critical plane over a cycle |
| v | Poisson’s ratio |
| σcp,max | maximum normal stress on the critical plane over a cycle |
| ω | interference |
| Subscripts: | |
| c | critical values of contact parameters at elasticity terminus |
| cp | critical plane |
| max | maximum value over a cycle |
| Superscripts: | |
| max | maximum value among all the material points |
| * | dimensionless contact parameters normalized by corresponding critical values |
| ’ | entities after coordinate transformation |
Appendix A
| P*max | Maximum Errors (%) | |||
|---|---|---|---|---|
| σx | σy | σz | σxy | |
| 10 | 1.85 | 0.63 | 8.03 | 0.73 |
| 50 | 2.64 | 0.65 | 4.54 | 0.81 |
| 100 | 2.9 | 0.64 | 3.22 | 0.78 |
| 300 | 2.64 | 0.34 | 2.74 | 1.07 |
| 700 | 2.76 | 0.34 | 2.82 | 0.58 |
| P*max | R2 Goodness-of-Fit for the Loading Process | R2 Goodness-of-Fit for the Unloading Process | ||
|---|---|---|---|---|
| A* vs. ω * | P* vs. ω * | A* vs. ω * | P* vs. ω * | |
| 10 | 0.978 | 0.931 | 0.932 | 0.972 |
| 50 | 0.970 | 0.970 | 0.946 | 0.961 |
| 100 | 0.959 | 0.982 | 0.952 | 0.955 |
| 300 | 0.930 | 0.972 | 0.957 | 0.954 |
| 700 | 0.936 | 0.942 | 0.943 | 0.974 |
| P*max | Difference in the Maximum FSDP (%) | |
|---|---|---|
| 1st Iteration | 2nd Iteration | |
| 10 | 4.966 | 5.215 |
| 50 | 0.881 | 1.116 |
| 100 | 0.052 | 2.695 |
| 300 | 1.039 | 3.999 |
| 700 | 1.19 | 1.607 |
References
- Manam, N.S.; Harun, W.S.W.; Shri, D.N.A.; Ghani, S.A.C.; Kurniawan, T.; Ismail, M.H.; Ibrahim, M.H.I. Study of corrosion in biocompatible metals for implants: A review. J. Alloys Compd. 2017, 701, 698–715. [Google Scholar] [CrossRef]
- Nečas, D.; Usami, H.; Niimi, T.; Sawae, Y.; Křupka, I.; Hartl, M. Running-in friction of hip joint replacements can be significantly reduced: The effect of surface-textured acetabular cup. Friction 2020, 8, 1137–1152. [Google Scholar] [CrossRef]
- Tang, Z.; Zhou, D.; Jia, T.; Pan, D.; Zhang, C. Investigation of lubricant transfer and distribution at head/disk interface in air-helium gas mixtures. Friction 2019, 7, 564–571. [Google Scholar] [CrossRef]
- Cao, W.; Ren, S.; Pu, W.; Xiao, K. Microstress cycle and contact fatigue of spiral bevel gears by rolling-sliding of asperity contact. Friction 2020, 8, 1083–1101. [Google Scholar] [CrossRef]
- Hu, S.; Huang, W.; Brunetiere, N.; Liu, X.; Wang, Y. Truncated separation method for characterizing and reconstructing bi-Gaussian stratified surfaces. Friction 2017, 5, 32–44. [Google Scholar] [CrossRef]
- Patil, D.B.; Eriten, M. Frictional energy dissipation in spherical contacts under presliding: Effect of elastic mismatch, plasticity and phase difference in loading. ASME J. Appl. Mech. 2015, 82, 011005. [Google Scholar] [CrossRef]
- Jackson, R.; Chusoipin, I.; Green, I. A finite element study of the residual stress and deformation in hemispherical contacts. ASME J. Tribol. 2005, 127, 484–493. [Google Scholar] [CrossRef]
- Etsion, I.; Kligerman, Y.; Kadin, Y. Unloading of an elastic-plastic loaded spherical contact. Int. J. Solids Struct. 2005, 42, 3716–3729. [Google Scholar] [CrossRef]
- Zhao, J.H.; Nagao, S.; Zhang, Z.L. Loading and unloading of a spherical contact: From elastic to elasticperfectly plastic materials. Int. J. Mech. Sci. 2012, 56, 70–76. [Google Scholar] [CrossRef]
- Zhao, B.; Zhang, S.; Wang, Q.F.; Zhang, Q.; Wang, P. Loading and unloading of a power-law hardening spherical contact under stick contact condition. Int. J. Mech. Sci. 2015, 94–95, 20–26. [Google Scholar] [CrossRef]
- Jana, T.; Mitra, A.; Sahoo, P. Unloading analysis of elastically and plastically graded hemispherical contact with rigid flat. Tribol. Int. 2020, 142, 105973. [Google Scholar] [CrossRef]
- Kadin, Y.; Kligerman, Y.; Etsion, I. Multiple loading-unloading of an elastic-plastic spherical contact. Int. J. Solids Struct. 2006, 43, 7119–7127. [Google Scholar] [CrossRef][Green Version]
- Zait, Y.; Zolotarevsky, V.; Kligerman, Y.; Etsion, I. Multiple normal loading-unloading cycles of a spherical contact under stick contact condition. ASME J. Tribol. 2010, 132, 041401. [Google Scholar] [CrossRef]
- Wang, J.; Li, Q.; Yang, C.; Zhou, C. Repeated loading model for elastic–plastic contact of geomaterial. Adv. Mech. Eng. 2018, 10, 1–15. [Google Scholar] [CrossRef]
- Xu, H.; Komvopoulos, K. Fracture mechanics analysis of asperity cracking due to adhesive normal contact. Int. J. Fract. 2013, 181, 273–283. [Google Scholar] [CrossRef]
- Xu, H.; Komvopoulos, K. A fracture mechanics analysis of asperity cracking due to sliding contact. Int. J. Solids Struct. 2019, 171, 1–9. [Google Scholar] [CrossRef]
- Zhao, B.; Shen, F.; Cui, Y.; Xie, Y.; Zhou, K. Damage analysis for an elastic-plastic body in cylindrical contact with a rigid plane. Tribol. Int. 2017, 115, 18–27. [Google Scholar] [CrossRef]
- Green, I. Poisson ratio effects and critical values in spherical and cylindrical hertzian contacts. Int. J. Appl. Mech. Eng. 2005, 10, 451–462. [Google Scholar]
- Jackson, R.L.; Green, I. A finite element study of elasto-plastic hemispherical contact against a rigid flat. J. Tribol. 2005, 127, 343–354. [Google Scholar] [CrossRef]
- Chen, Z.; Goltsberg, R.; Etsion, I. A universal model for a frictionless elastic-plastic coated spherical normal contact with moderate to large coating thicknesses. Tribol. Int. 2017, 114, 485–493. [Google Scholar] [CrossRef]
- Chen, Z.; Jiang, Y.; Tong, Z.; Tong, S.; Tan, J. Fatigue analysis of spherical contact subjected to cyclic elastic-plastic normal loading. J. Tribol. 2021, 1–20. [Google Scholar] [CrossRef]
- Tong, Z.; Xin, J.; Tong, S.; Yang, Z.; Zhao, J.; Mao, J. Internal flow structure, fault detection, and performance optimization of centrifugal pumps. J. Zhejiang Univ. A 2020, 21, 85–117. [Google Scholar] [CrossRef]
- Zhao, B.; Xu, H.; Lu, X.; Ma, X.; Shi, X.; Dong, Q. Contact behaviors of a power-law hardening elastic–plastic asperity with soft coating flattened by a rigid flat. Int. J. Mech. Sci. 2019, 152, 400–410. [Google Scholar] [CrossRef]
- Fatemi, A.; Shamsaei, N. Multiaxial fatigue: An overview and some approximation models for life estimation. Int. J. Fatigue 2011, 33, 948–958. [Google Scholar] [CrossRef]
- Fatemi, A.; Socie, D.F. A critical plane approach to multiaxial fatigue damage including out-of-phase loading. Fatigue Fract. Eng. Mater. Struct. 1988, 11, 149–165. [Google Scholar] [CrossRef]
- Wang, W.; Liu, H.; Zhu, C.; Du, X.; Tang, J. Effect of the residual stress on contact fatigue of a wind turbine carburized gear with multiaxial fatigue criteria. Int. J. Mech. Sci. 2019, 151, 263–273. [Google Scholar] [CrossRef]
- Vijay, A.; Sadeghi, F. A continuum damage mechanics framework for modeling the effect of crystalline anisotropy on rolling contact fatigue. Tribol. Int. 2019, 140, 105845. [Google Scholar] [CrossRef]
- Papadopoulos, I.V. Critical plane approaches in high-cycle fatigue: On the definition of the amplitude and mean value of the shear stress acting on the critical plane. Fatigue Fract. Eng. Mater. Struct. 1998, 21, 269–285. [Google Scholar] [CrossRef]
- Liu, Z.; Tsang, K.S.; Liu, Y.; Pang, J.H.L. Finite element and experimental study on multiaxial fatigue analysis of rail clip failures. Fatigue Fract. Eng. Mater. Struct. 2020, 43, 2390–2401. [Google Scholar] [CrossRef]
- Tong, Z.; Cheng, Z.; Tong, S. A review on the development of compressed air energy storage in China: Technical and economic challenges to commercialization. Renew. Sustain. Energy Rev. 2021, 135, 110178. [Google Scholar] [CrossRef]
- Chen, Y.; Tong, Z.; Zheng, Y.; Samuelson, H.; Norford, L. Transfer learning with deep neural networks for model predictive control of HVAC and natural ventilation in smart buildings. J. Clean. Prod. 2020, 254, 119866. [Google Scholar] [CrossRef]
- Socie, D.F.; Marquis, G.B. Multiaxial Fatigue; Society of Automotive Engineers: Warrendale, PA, USA, 2000. [Google Scholar]
- Matecka, J.; Rozumek, D. Metallographic and Mechanical Research of the O–Ti2AlNb Alloy. Materials 2020, 13, 3006. [Google Scholar] [CrossRef]
- Fischer-Cripps, A.C. Introduction of Contact Mechanics, 2nd ed.; Springer: Sydney, NSW, Australia, 2007; ISBN 9780387681870. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Z.; Jiang, Y.; Tong, Z.; Tong, S. Fatigue Damage of an Asperity in Frictionless Normal Contact with a Rigid Flat. Metals 2021, 11, 545. https://doi.org/10.3390/met11040545
Chen Z, Jiang Y, Tong Z, Tong S. Fatigue Damage of an Asperity in Frictionless Normal Contact with a Rigid Flat. Metals. 2021; 11(4):545. https://doi.org/10.3390/met11040545
Chicago/Turabian StyleChen, Zhou, Yibo Jiang, Zheming Tong, and Shuiguang Tong. 2021. "Fatigue Damage of an Asperity in Frictionless Normal Contact with a Rigid Flat" Metals 11, no. 4: 545. https://doi.org/10.3390/met11040545
APA StyleChen, Z., Jiang, Y., Tong, Z., & Tong, S. (2021). Fatigue Damage of an Asperity in Frictionless Normal Contact with a Rigid Flat. Metals, 11(4), 545. https://doi.org/10.3390/met11040545






