Atmospheric Corrosion Sensor Based on Strain Measurement with Active–Dummy Fiber Bragg Grating Sensors
Abstract
1. Introduction
2. Materials and Methods
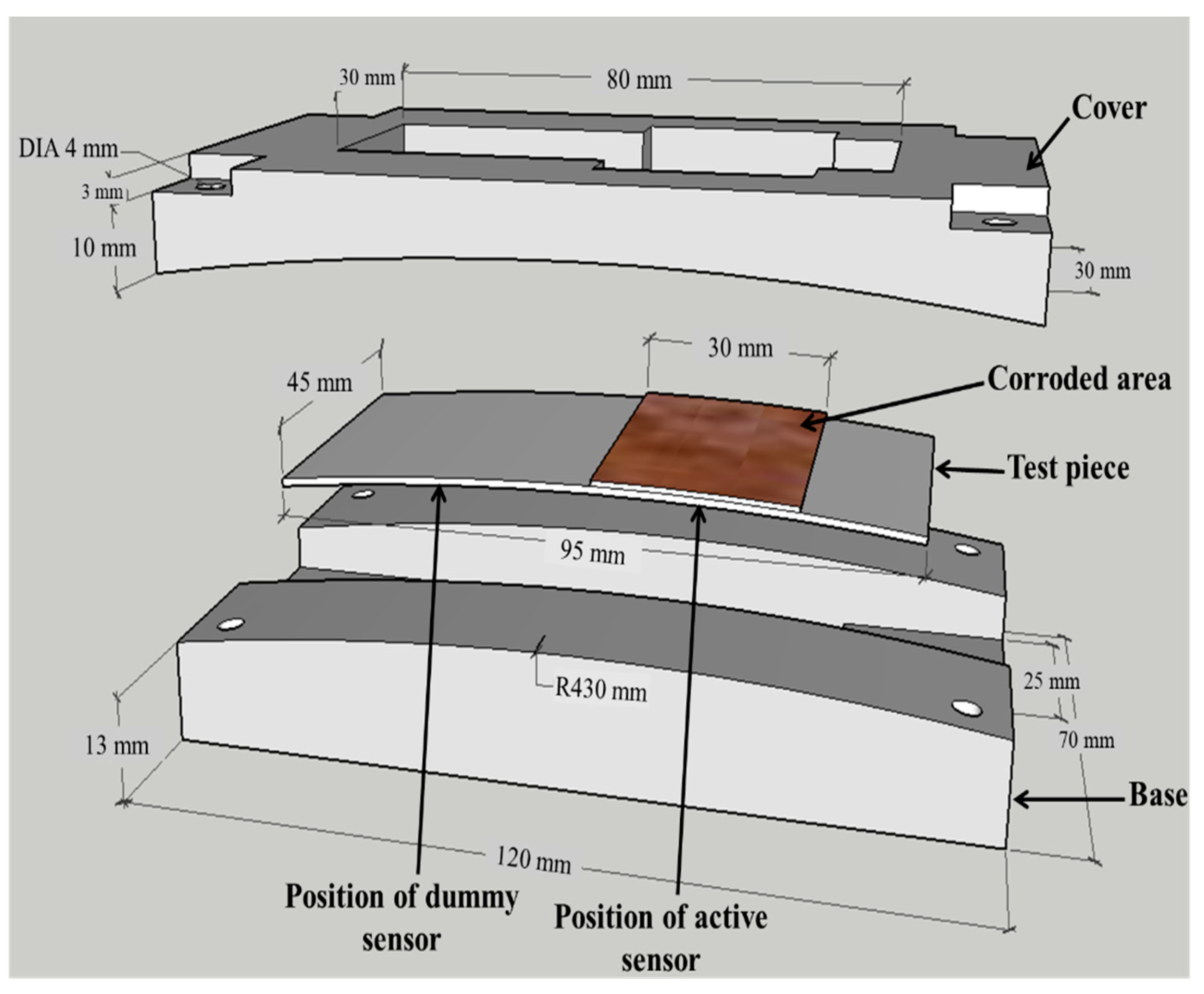
2.1. Experimental Apparatus
2.2. Measurement Using the Active–Dummy Method
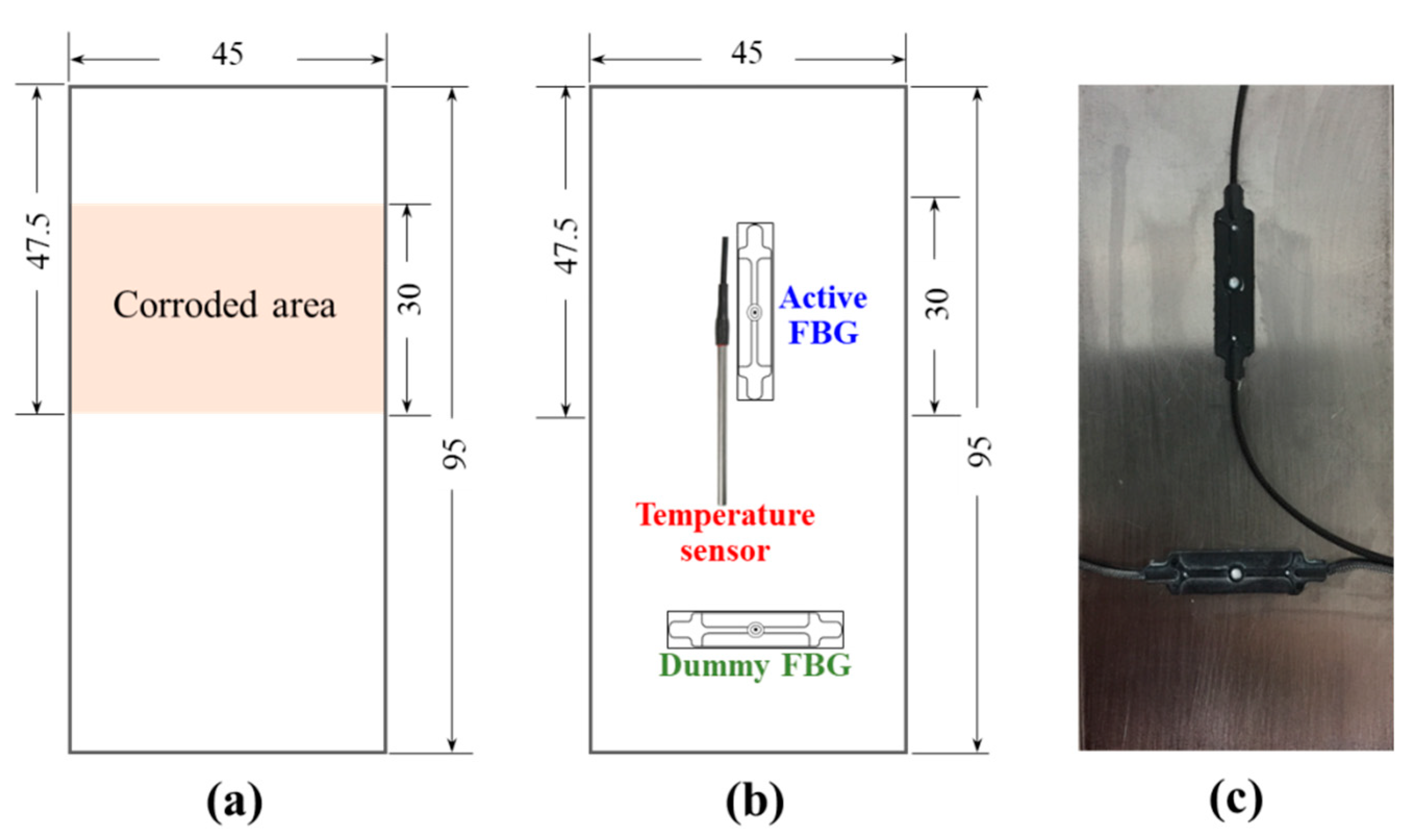
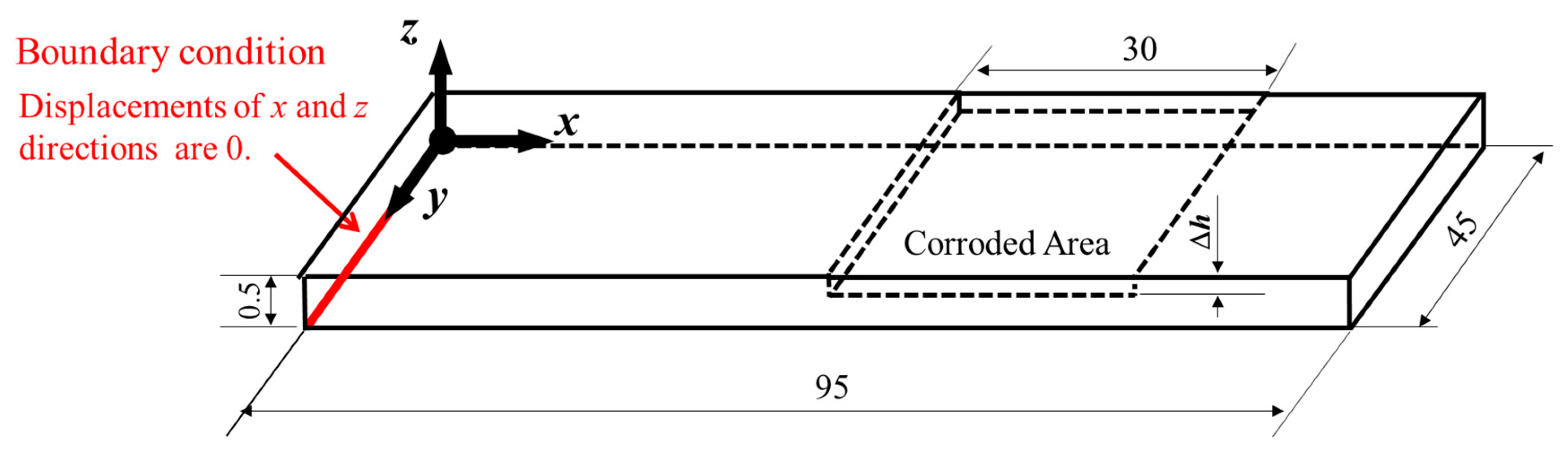
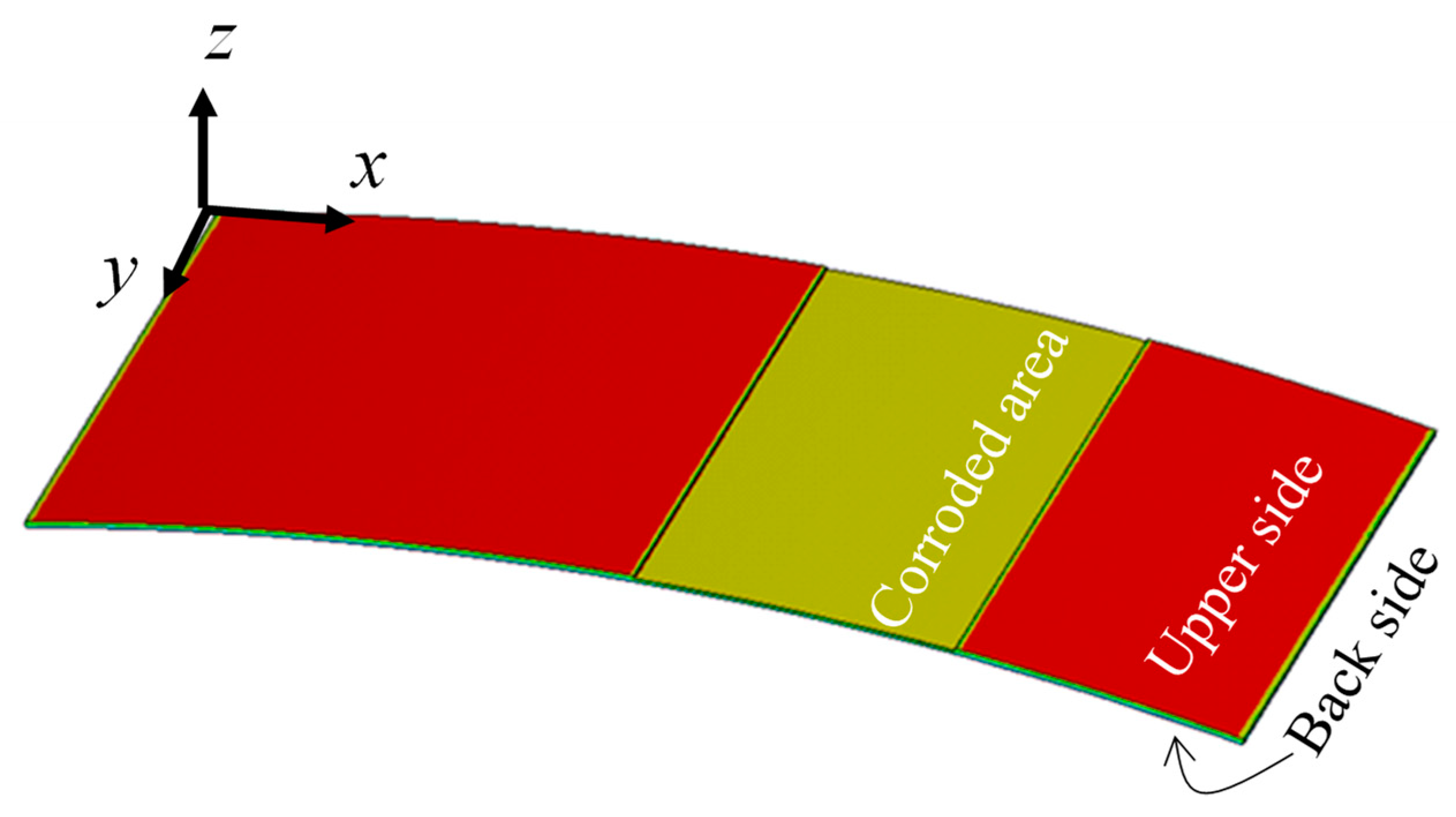
2.3. Configuration of FBG Sensors on the Test Piece
2.4. Compensation of Thermal Strain
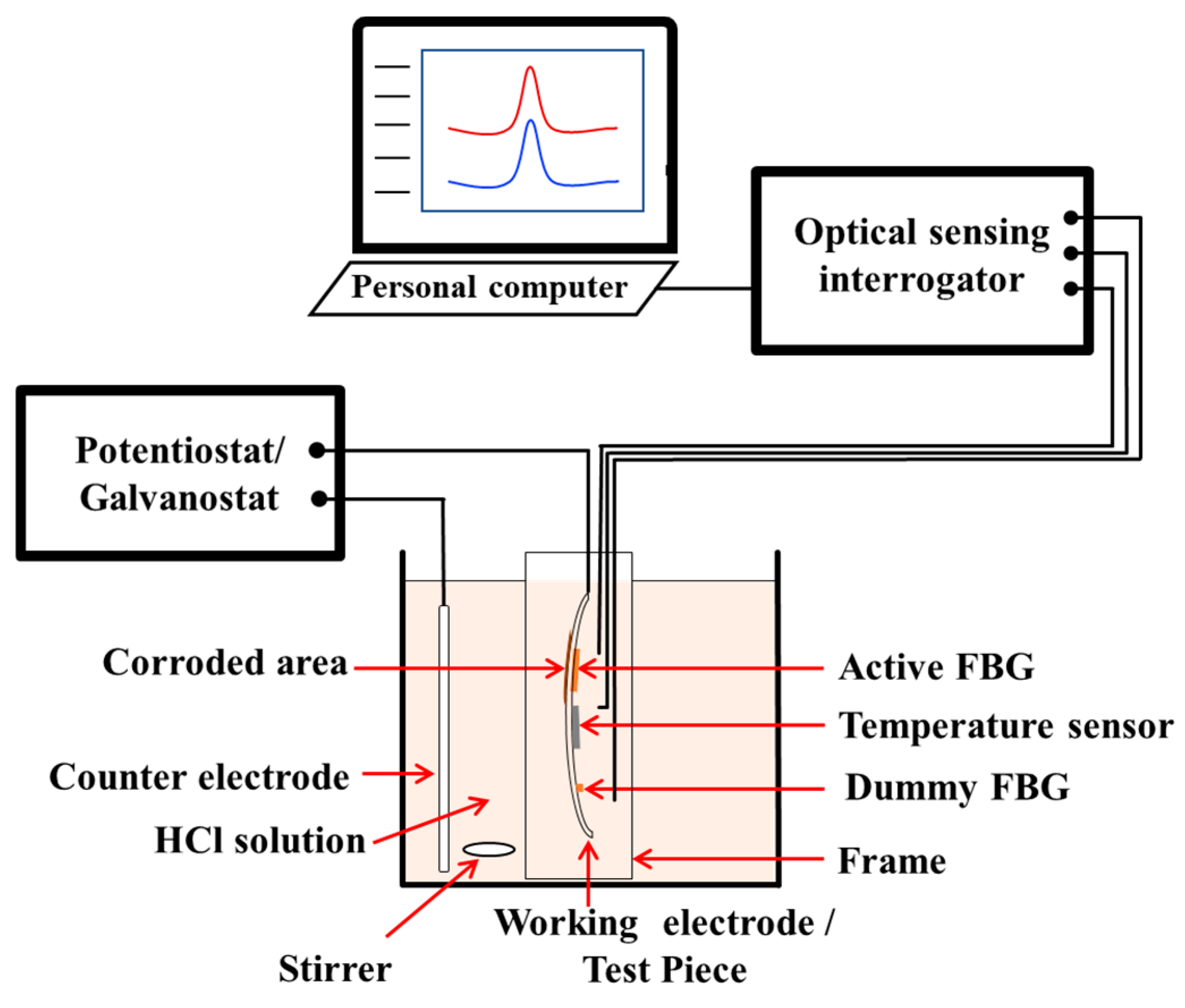
2.5. Accelerated Corrosion Using Galvanostatic Electrolysis
3. Result
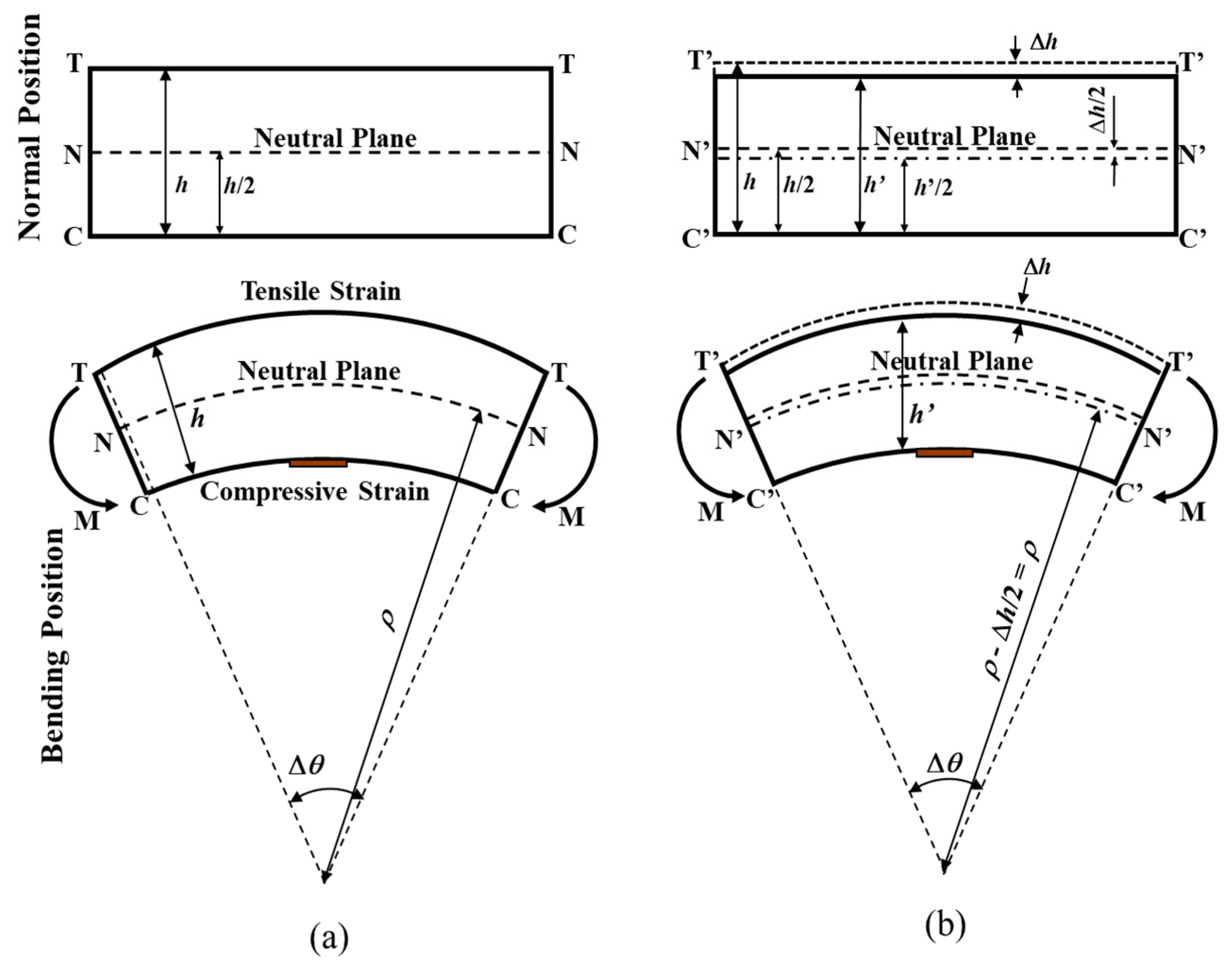
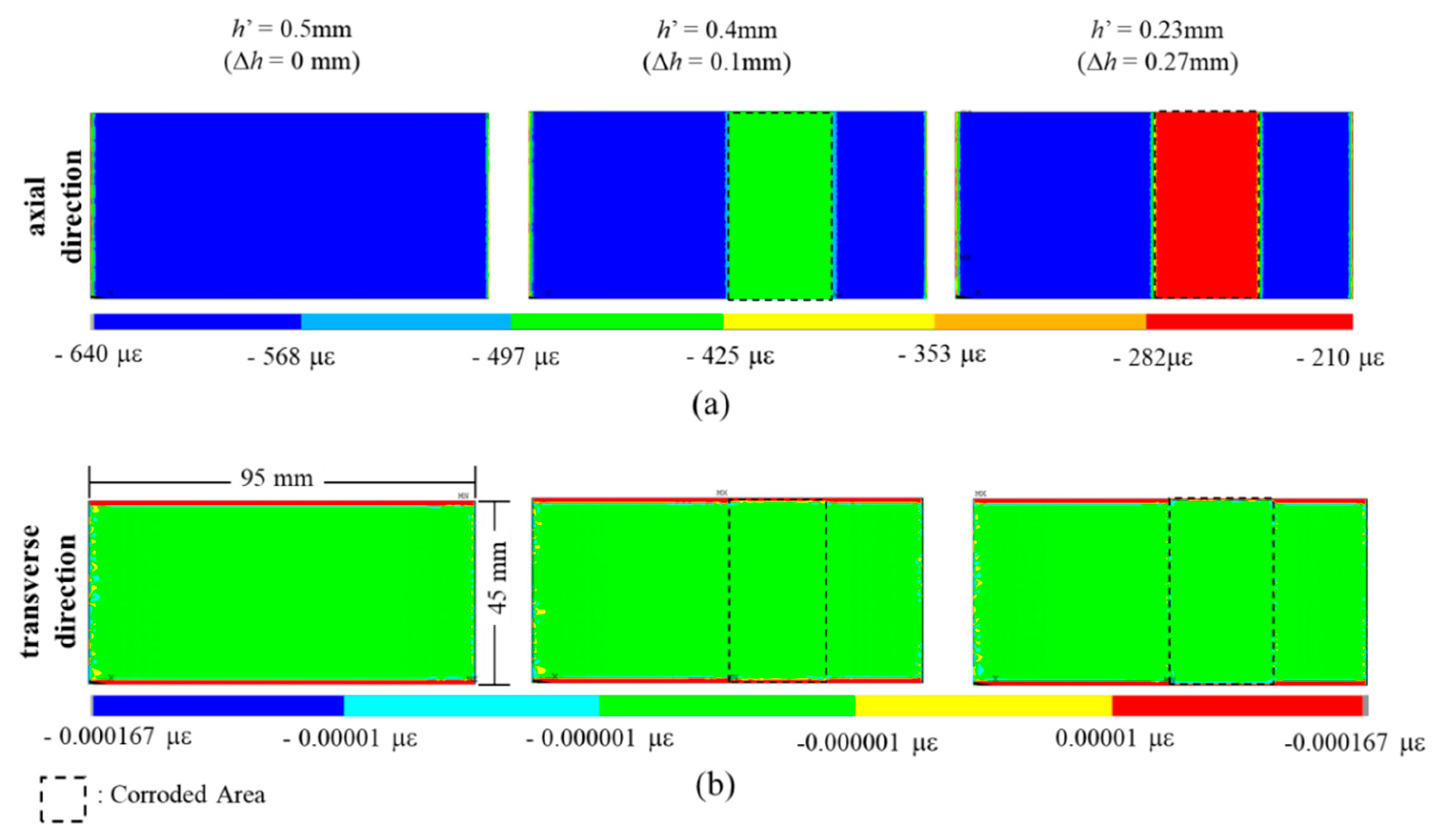
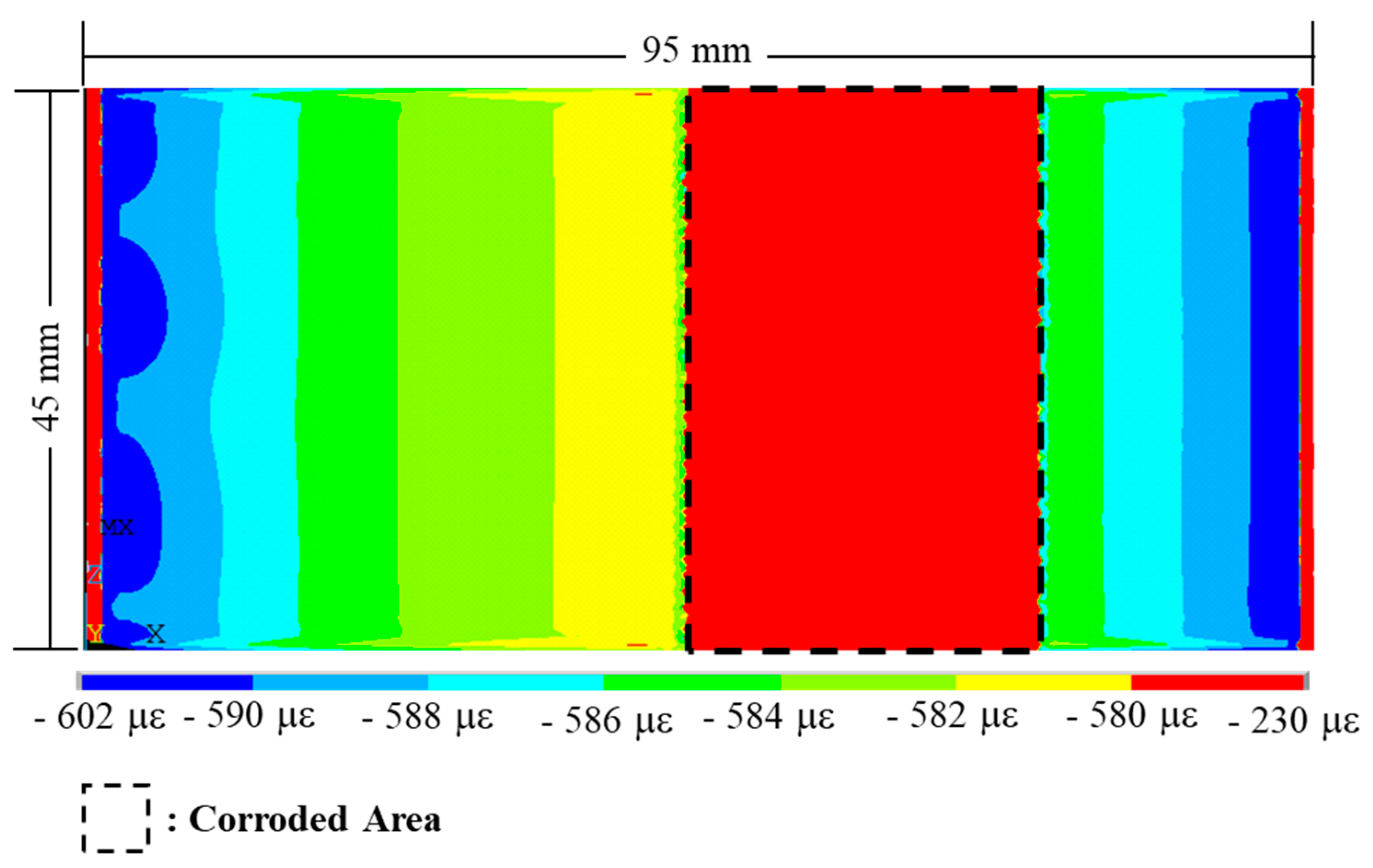
3.1. Numerical Analysis
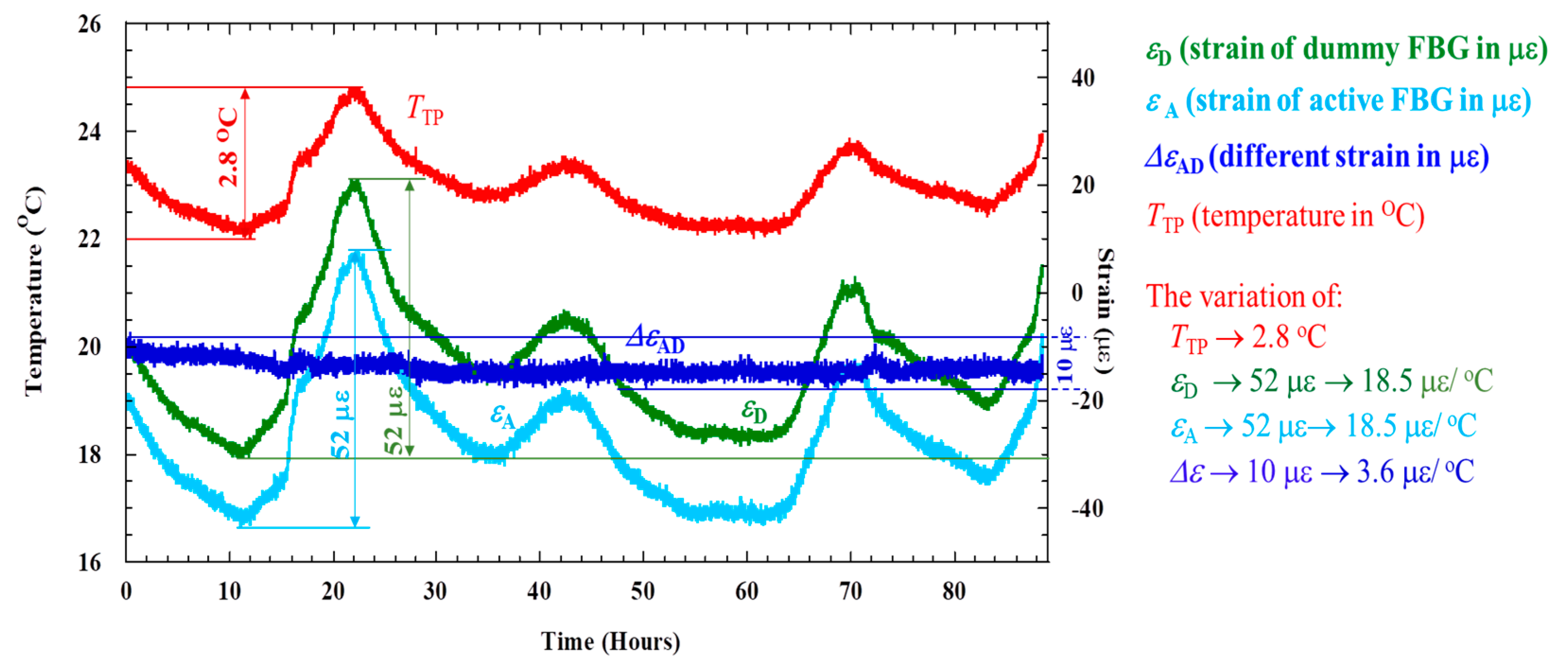
3.2. Thermal Strain Compensation
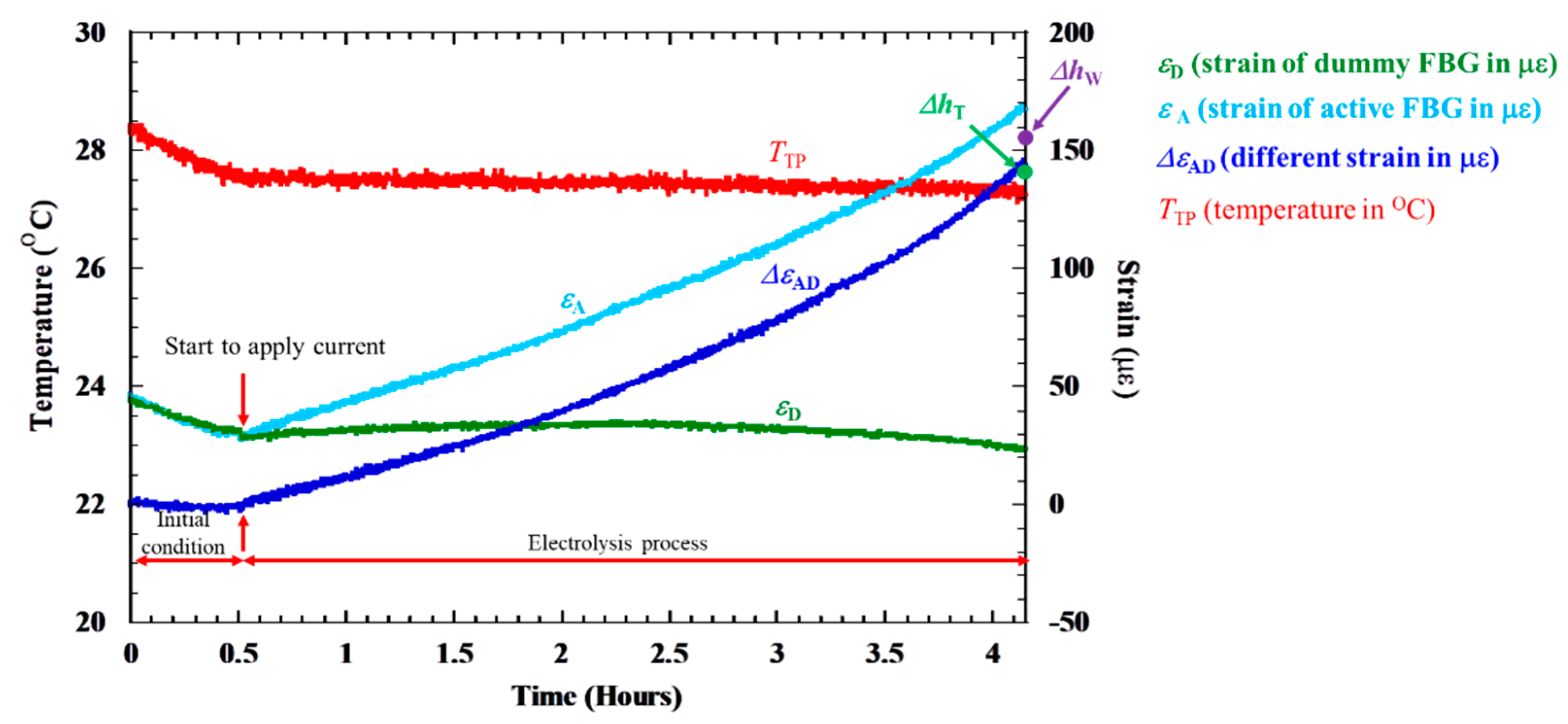
3.3. Galvano-Static Electrolysis Experiment
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Cai, H.T.; Faisal, R.M.A.; Yu, G.S.; Boon, K.Y. Non-destructive fiber Bragg grating based sensing system: Early corrosion detection for structural health monitoring. Sens. Act. A Phys. 2017, 268, 61–67. [Google Scholar]
- Muhammad, R.; Syarizal, F.; Syifaul, H.; Juendri, S.; Ahmad, K.A. Atmospheric corrosion of structural steels exposed in the 2004 tsunami-affected areas of Aceh. Int. J. Automot. Mech. Eng. 2013, 7, 1014–1022. [Google Scholar] [CrossRef]
- Tadashi, S.; Akira, T.; To, D. Atmospheric corrosion behaviours of steels in Japan. Zair. Kank. 2016, C-111, 285–288. [Google Scholar]
- Isao, S.; Ayaka, O.; Masayuki, I.; Kunihiro, W.; Yafusumi, A. Screen printed atmospheric corrosion monitoring sensor based on electrochemical impedance spectroscopy. Sens. Actuators B Chem. 2009, 139, 292–297. [Google Scholar] [CrossRef]
- Chungling, L.; Yuantai, M.; Ying, L.; Fuhui, W. EIS monitoring study of atmospheric corrosion under variable relative humidity. Cor. Sci. 2010, 52, 3677–3686. [Google Scholar] [CrossRef]
- Naoya, K.; Masatoshi, H.; Toshirou, Y.; Hiroshi, K.; Kazumi, M.; Yukihisa, K.; Shinji, O. Atmospheric corrosion sensor based on strain measurement. Meas. Sci. Technol. 2016, 28, 015106. [Google Scholar]
- Nining, P.; Naoya, K.; Shinji, O.; Hiroshi, K. Development of amplifier circuit by active dummy method for atmospheric corrosion monitoring in steel based on strain measurement. Metals 2018, 8, 5. [Google Scholar]
- Nining, P.; Naoya, K.; Shinji, O.; Hiroshi, K.; Yukihisa, K. Atmospheric Corrosion Sensor Based on Strain Measurement with an Active Dummy Circuit Method in Experiment with Corrosion Products. Metal 2019, 9, 579. [Google Scholar]
- Sascha, L.; Philipp, L.; Mario, W.; Katerina, K.; Monika, S.; Elke, T.; Heike, M.; Berhane, G.; Johannes, C.M. Polymer Optical Fiber Sensors for Distributed Strain Measurement and Application in Structural Health Monitoring. IEEE Sens. J. 2009, 9, 1330–1338. [Google Scholar]
- Drake, D.A.; Sullivan, R.W.; Wilson, J.C. Distributed Strain Sensing from Different Optical Fiber. Inventions 2018, 3, 67. [Google Scholar] [CrossRef]
- Libo, Y.; Jun, Y.; Limin, Z.; Wei, J.; Xiaoli, D. Low-coherence Michelson interferometric fiber-optic multiplexed strain sensor array: A minimum configuration. Appl. Opt. 2004, 43, 3211–3216. [Google Scholar] [CrossRef]
- Kamal, R.; Ajay, S. Strain Measurement with Fiber Mach-Zehnder Interferometer Using Spatial Phase Measurement Techniques. Int. J. Sci. Tech. Res. 2019, 8, 1617–1622. [Google Scholar]
- Genda, C.; Hai, X.; Ying, H.; Zhi, Z.; Yinan, Z. A Novel Long-Period Fiber Grating Optical Sensor for Large Strain Measurement. Proc. SPIE 2009, 7292, 12. [Google Scholar] [CrossRef]
- Jian, N.W.; Jaw, L.T. Feasibility of Fiber Bragg Grating and Long-Period Fiber Grating Sensors under Different Environmental Conditions. Sensors 2010, 10, 10105–10127. [Google Scholar] [CrossRef]
- Carlos, R.; Filipe, C.; Carlos, F.; Joaqium, F. FBG based strain monitoring in the rehabilitation of a centenary metallic bridge. Eng. Struct. 2012, 44, 281–290. [Google Scholar]
- Sherif, Y.; Taha, L.; Mohamed, H.; Mohammad, H. Monitoring of strain induced by heat of hydration, cyclic and dynamic loads in concrete structures using fiber-optics sensors. Measurement 2014, 52, 33–46. [Google Scholar]
- Li, H.N.; Ren, L.; Li, D.S.; Yi, T.H. Design and Applications of Fiber BRAGG Grating Sensors for Structural Health Monitoring. In Proceedings of the 2013 World Congress on Advances in Structural Engineering and Mechanics (ASEM13), Jeju, Korea, 8–12 September 2013; pp. 2215–2221. [Google Scholar]
- Hong-lin, L.; Zheng-wei, Z.; Yong, Z.; Bang, L.; Feng, X. Experimental study on an FBG strain sensor. Opt. Fiber Tech. 2018, 40, 144–151. [Google Scholar]
- Hideaki, M.; Kohei, O.; Nozomi, K.; Kazura, K.; Hirotaka, I. Strain monitoring and defect detection in welded joints by using fiber-optic distributed sensors with high spatial resolution. E-J. Adv. Maintenance 2011, 2, 191–199. [Google Scholar]
- Michael, C.E.; Sunny, K.; Stefano, T.; Kotekar, P.M.; Richards, W.L.; Gregory, P.C. Strain measurement validation of embedded fiber bragg gratings. Int. J. Optomech. 2010, 4, 22–33. [Google Scholar]
- Wenbin, H.; Likang, D.; Cheng, Z.; Donglai, G.; Yinquan, Y.; Ning, M.; Wei, C. Optical fiber polarizer with Fe-C film for corrosion monitoring. IEEE Sens. J. 2017, 17, 6904–6910. [Google Scholar]
- Wenbin, H.; Hanli, C.; Minghong, Y.; Xinglin, T.; Ciming, Z.; Wei, C. Fe-C-coated fibre Bragg grating sensor for steel corrosion monitoring. Cor. Sci. 2011, 53, 1933–1938. [Google Scholar]
- Ning, Z.; Wei, C.; Xing, Z.; Wenbin, H.; Min, G. Optical sensor for steel corrosion monitoring based on etched fiber bragg grating sputtered with iron film. IEEE Sens. J. 2015, 15, 3551–3556. [Google Scholar]
- Rosdi, H.; Muhammad, H.A.B.; Katrina, D.; Faisal, R.M.A. Optical-based sensors for monitoring corrosion of reinforcement rebar via an etched cladding Bragg grating. Sensors 2012, 12, 15820–15826. [Google Scholar]
- Omar, A.; Hwa, K.C.; Muhammad, R.I.; Kok-Sing, L.; Chee, G.T. Monitoring corrosion process of reinforced concrete structure using FBG strain sensor. IEEE Trans. Instrum. Meas. 2017, 66, 2148–2155. [Google Scholar]
- Simon, K.T.G.; Su, E.T.; Basheer, P.A.; Muhammed, B.; Tong, S.; Kenneth, T.V.G. Monitoring of corrosion in structural reinforcing bars: Performance comparison using in situ fiber-optic and electric Wire Strain Gauge Systems. IEEE Sens. J. 2009, 9, 1494–1502. [Google Scholar]
- Wen, C.; Dong, X. Modification of the wavelength-strain coefficient of FBG for the prediction of steel bar corrosion embedded in concrete. Opt. Fib. Tech. 2012, 18, 47–50. [Google Scholar]
- Khalil, A.H.; Nader, V.; Paul, R.; Lydia, L.; Oleg, S. Strain based FBG sensor for real-time corrosion rate monitoring in pre-stressed structures. Sens. Act. B Chem. 2016, 236, 276–285. [Google Scholar]
- Marcelo, M.W.; Regina, C.S.B.A.; Bessie, A.R.; Fabio, V.B.D.N. A Guide to Fiber Bragg Grating Sensors. Intech 2013, 24. [Google Scholar] [CrossRef]
- Jigou, L.; Cornelia, S.H.; Gunter, B. Dynamic strain measurement with a fibre Bragg grating sensor system. Measurement 2002, 32, 151–161. [Google Scholar]
- Hang-yin, L.; Kin-tak, L.; Li, C.; Wei, J. Viability of using an embedded FBG sensor in a composite structure for dynamic strain measurement. Measurement 2006, 39, 328–334. [Google Scholar]
- Rosa, A.P.H.; Ricardo, M.A.; Susana, F.S.; Martin, B.; Kay, S.; Jens, K.; Manuel, L.A.; Jose, L.S.; Orlanclo, F. Simultaneous measurement of strain and temperature based on clover microstructured fiber loop mirror. Measurement 2015, 65, 50–53. [Google Scholar]
- Jianping, H.; Zhi, Z.; Jinping, O. Simultaneous measurement of strain and temperature using a hybrid local and distributed optical fiber sensing system. Measurement 2014, 47, 698–706. [Google Scholar]


| h [mm] | Δh [mm] | Strain (με) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Corroded Area | Uncorroded Area | |||||||||||
| Axial Direction | Axial Direction | Transverse Direction | ||||||||||
| εC at 300 K | ΔεC at 310 K | ΔεUC at 300 K | ΔεUC at 310 K | ΔεUC at 300 K | ΔεUC at 310 K | |||||||
| 0.5 | 0 | −584 | 0 | −467 | 0 | 117 | −584 | −467 | 117 | 0 | 117 | 117 |
| 0.45 | 0.05 | −525 | 59 | −408 | 59 | 117 | - | |||||
| 0.4 | 0.1 | −466 | 118 | −349 | 118 | 117 | ||||||
| 0.35 | 0.15 | −408 | 176 | −291 | 176 | 117 | ||||||
| 0.3 | 0.2 | −350 | 234 | −233 | 234 | 117 | ||||||
| 0.25 | 0.25 | −292 | 292 | −175 | 292 | 117 | ||||||
| 0.23 | 0.27 | −268 | 316 | −151 | 316 | 117 | ||||||
| 0.2 | 0.3 | −233 | 351 | −116 | 351 | 117 | ||||||
| Based on Strain Measurement (Δh) | Based on Actual Thickness (ΔhT) | Based on Weight Loss (ΔhW) | |
|---|---|---|---|
| Thickness (μm) | 123 | 120 | 132 |
| Difference (%) | - | 2.5 | 6.8 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Purwasih, N.; Shinozaki, H.; Okazaki, S.; Kihira, H.; Kuriyama, Y.; Kasai, N. Atmospheric Corrosion Sensor Based on Strain Measurement with Active–Dummy Fiber Bragg Grating Sensors. Metals 2020, 10, 1076. https://doi.org/10.3390/met10081076
Purwasih N, Shinozaki H, Okazaki S, Kihira H, Kuriyama Y, Kasai N. Atmospheric Corrosion Sensor Based on Strain Measurement with Active–Dummy Fiber Bragg Grating Sensors. Metals. 2020; 10(8):1076. https://doi.org/10.3390/met10081076
Chicago/Turabian StylePurwasih, Nining, Hiroki Shinozaki, Shinji Okazaki, Hiroshi Kihira, Yukihisa Kuriyama, and Naoya Kasai. 2020. "Atmospheric Corrosion Sensor Based on Strain Measurement with Active–Dummy Fiber Bragg Grating Sensors" Metals 10, no. 8: 1076. https://doi.org/10.3390/met10081076
APA StylePurwasih, N., Shinozaki, H., Okazaki, S., Kihira, H., Kuriyama, Y., & Kasai, N. (2020). Atmospheric Corrosion Sensor Based on Strain Measurement with Active–Dummy Fiber Bragg Grating Sensors. Metals, 10(8), 1076. https://doi.org/10.3390/met10081076







