On Waviness and Two-Sided Surface Features in Thermal Elastohydrodynamically Lubricated Line Contacts
Abstract
1. Introduction
2. Methodology
2.1. Elastohydrodynamic Model
2.2. Thermal Model
2.3. Lubricant Properties
2.4. Numerical Approach
3. Results and Discussion
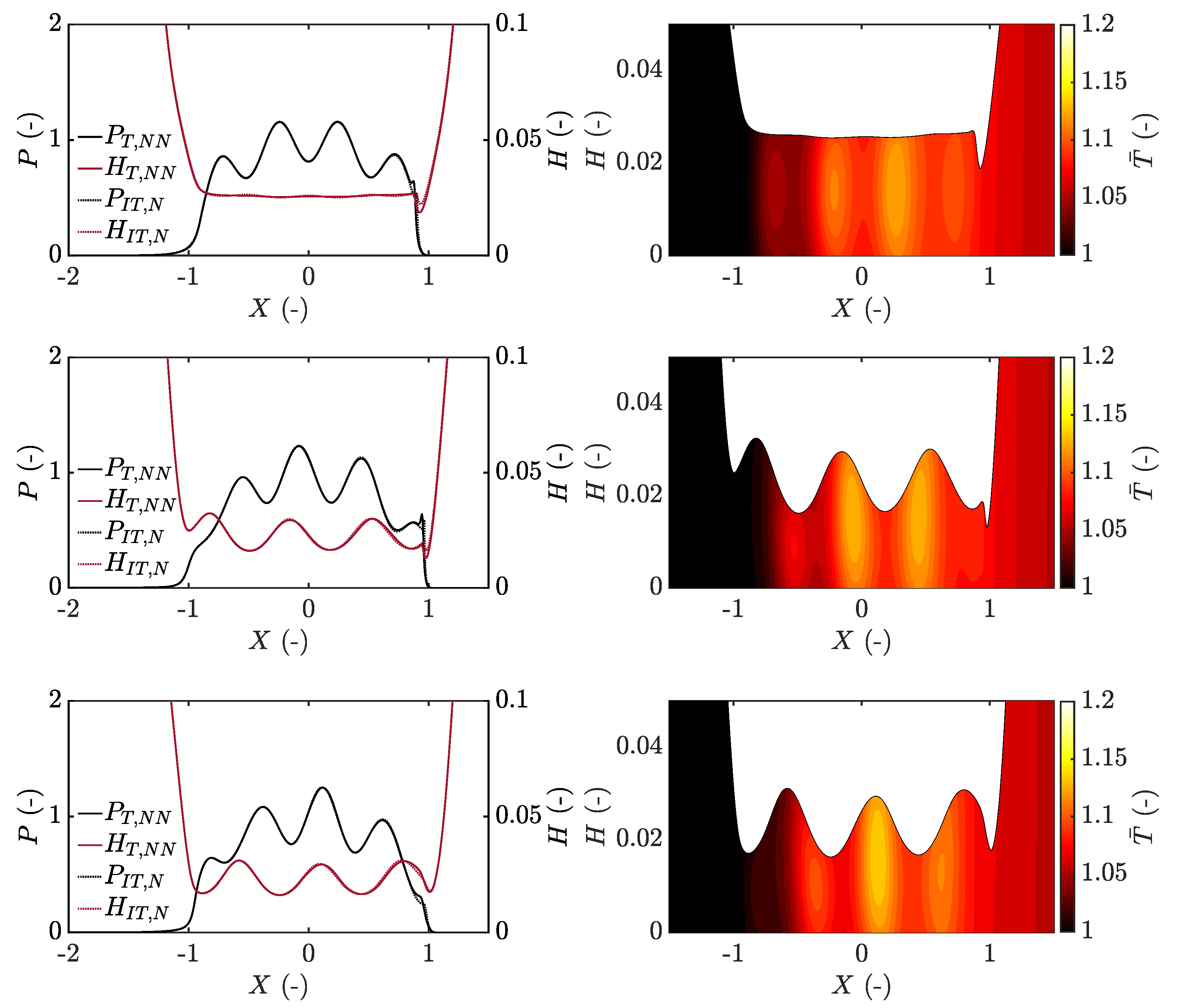
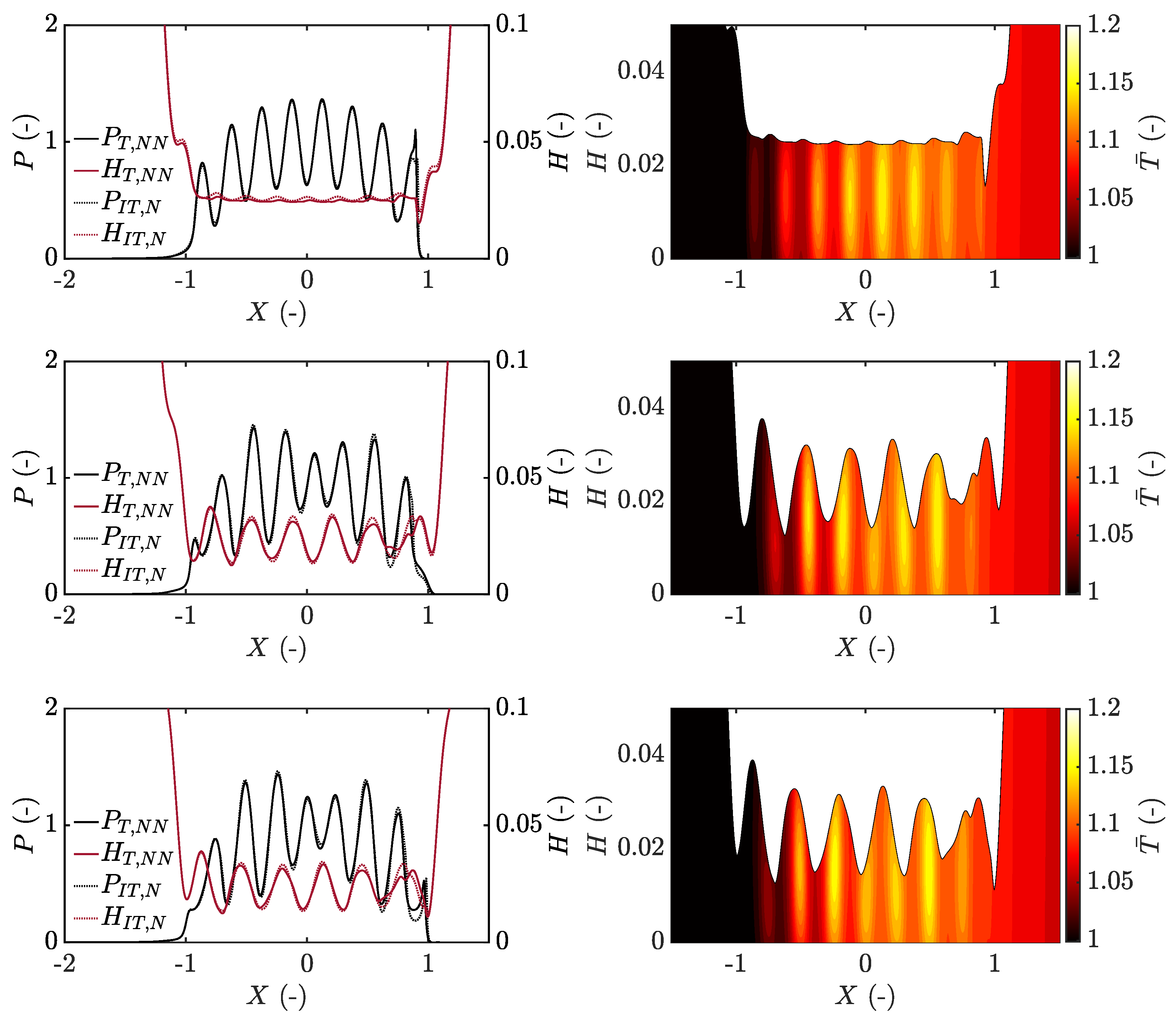
3.1. Sinusoidal Roughness
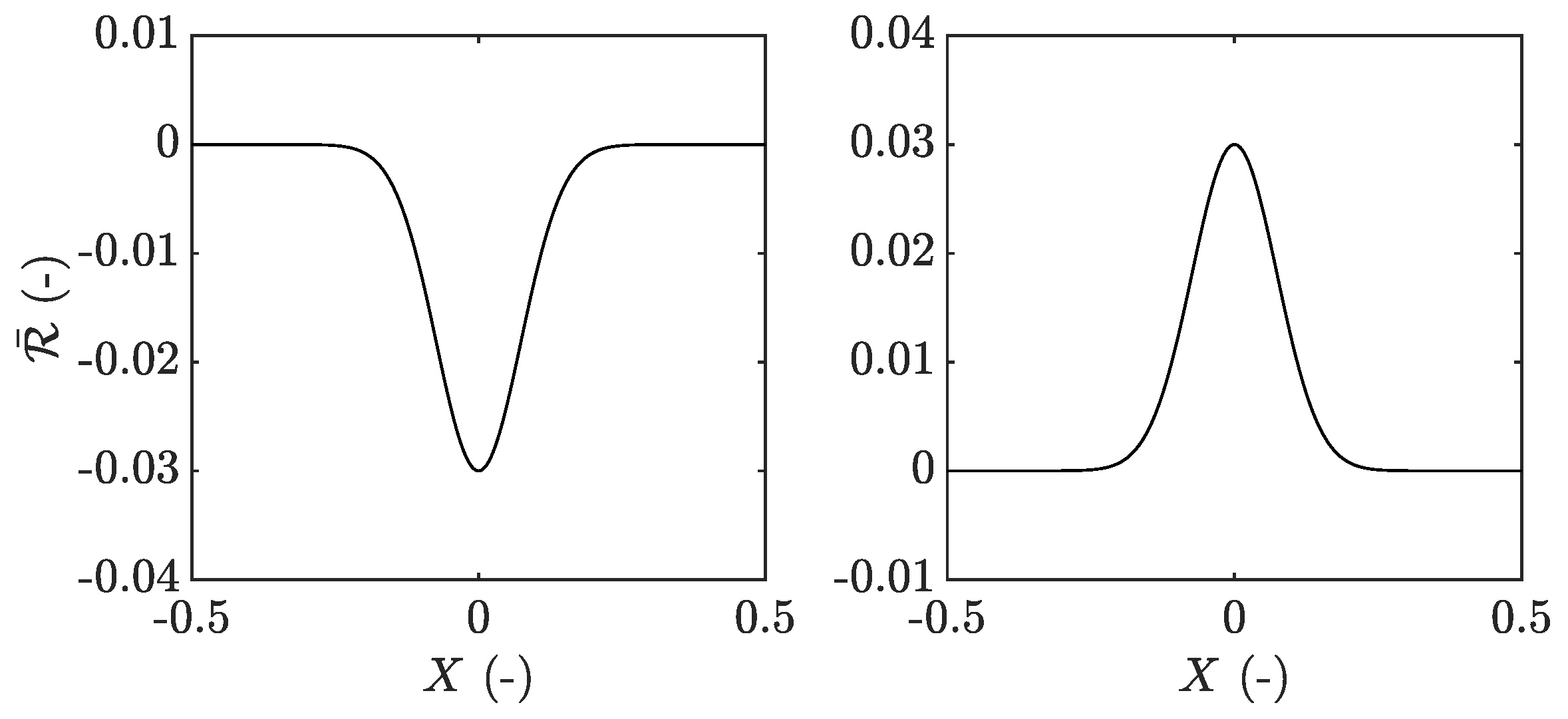
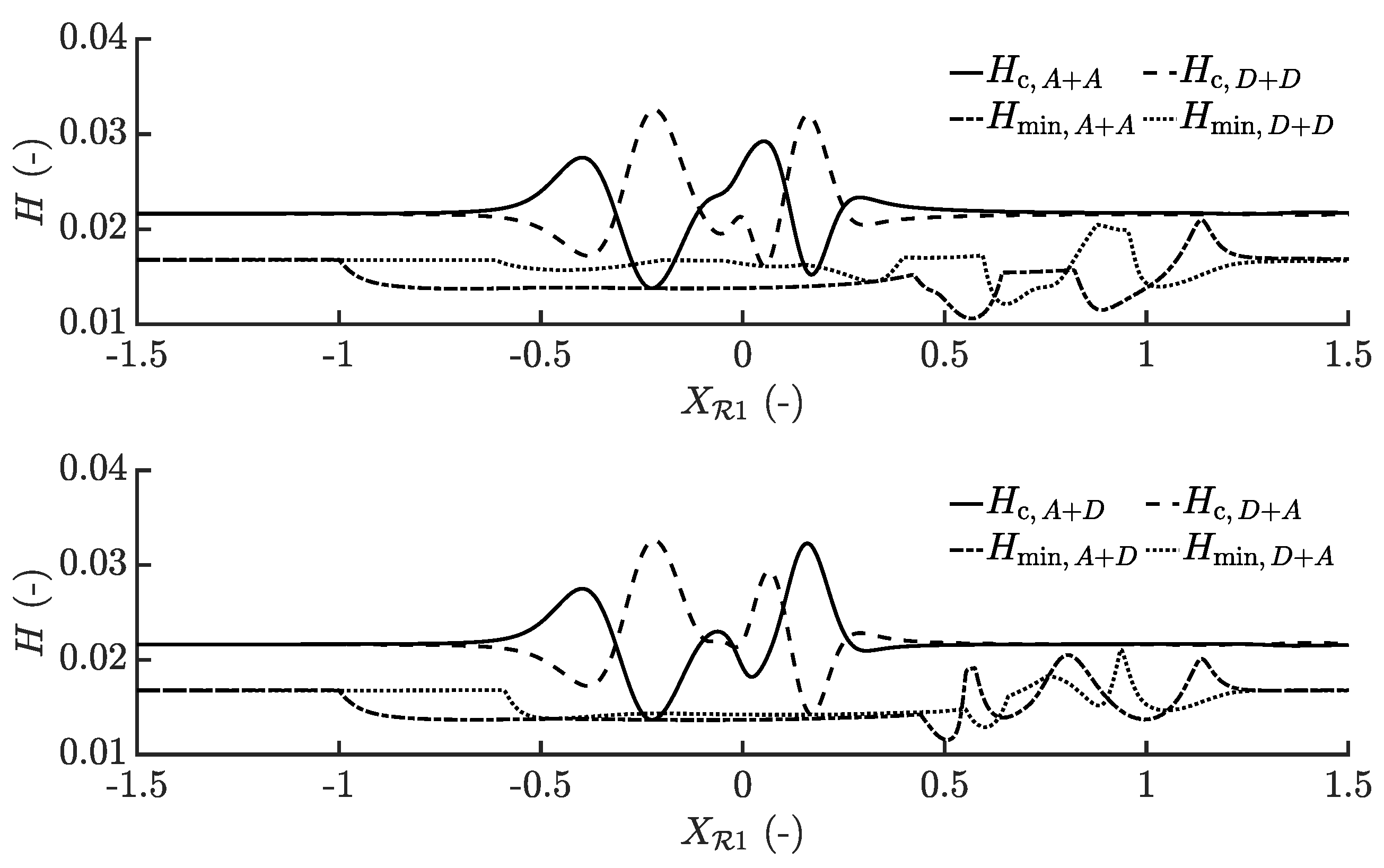
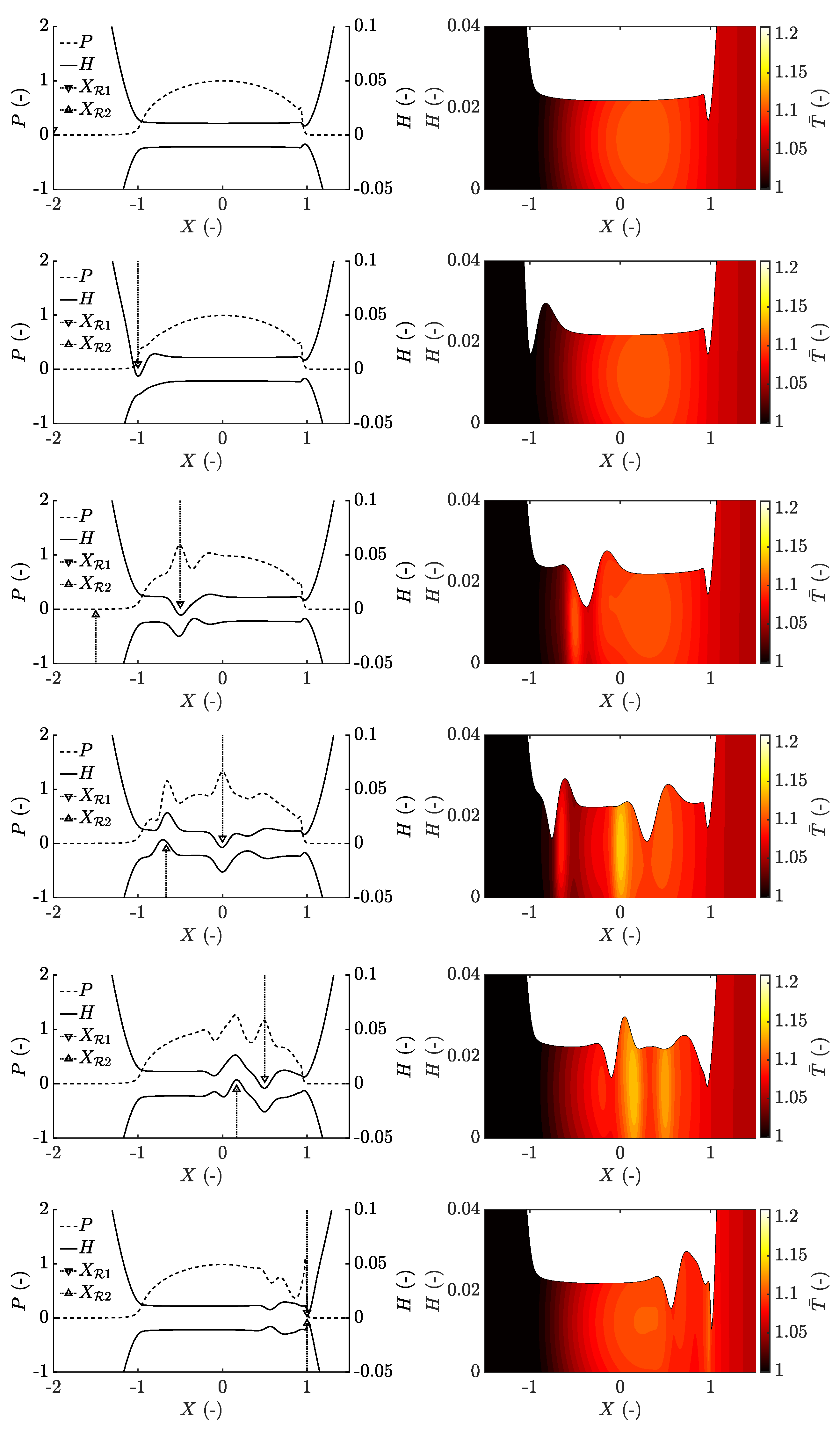
3.2. Two-Sided Surface Features
- an asperity on the slower surface overtaken by an asperity on the faster surface (),
- a dent on the slower surface overtaken by a dent on the faster surface (),
- an asperity on the slower surface overtaken by a dent on the faster surface (),
- a dent on the slower surface overtaken by an asperity on the faster surface ().
4. Conclusions
- (i)
- In case of sinusoidal roughness, differences in outlet film thickness between the thermal non-Newtonian and isothermal Newtonian approaches were for all studied wavelengths observed. These differences were explained by a reduced viscosity in the contact inlet due to shear thinning and shear heating, and further resulted in the isothermal Newtonian approach to overestimate the minimum film thickness by up to .
- (ii)
- Differences in the high pressure region were only found to be significant in case of short wavelength roughness. These differences were explained by a reduced viscosity in the contact inlet due to shear thinning and shear heating, further disturbing the formation of the complementary function. This resulted in central film thickness estimations to differ between and by using the thermal non-Newtonian approach compared to the isothermal Newtonian approach.
- (iii)
- Following the relatively small influence on pressure by the complementary function, only small differences in maximum pressure between the thermal non-Newtonian and isothermal Newtonian approaches were noticed.
- (iv)
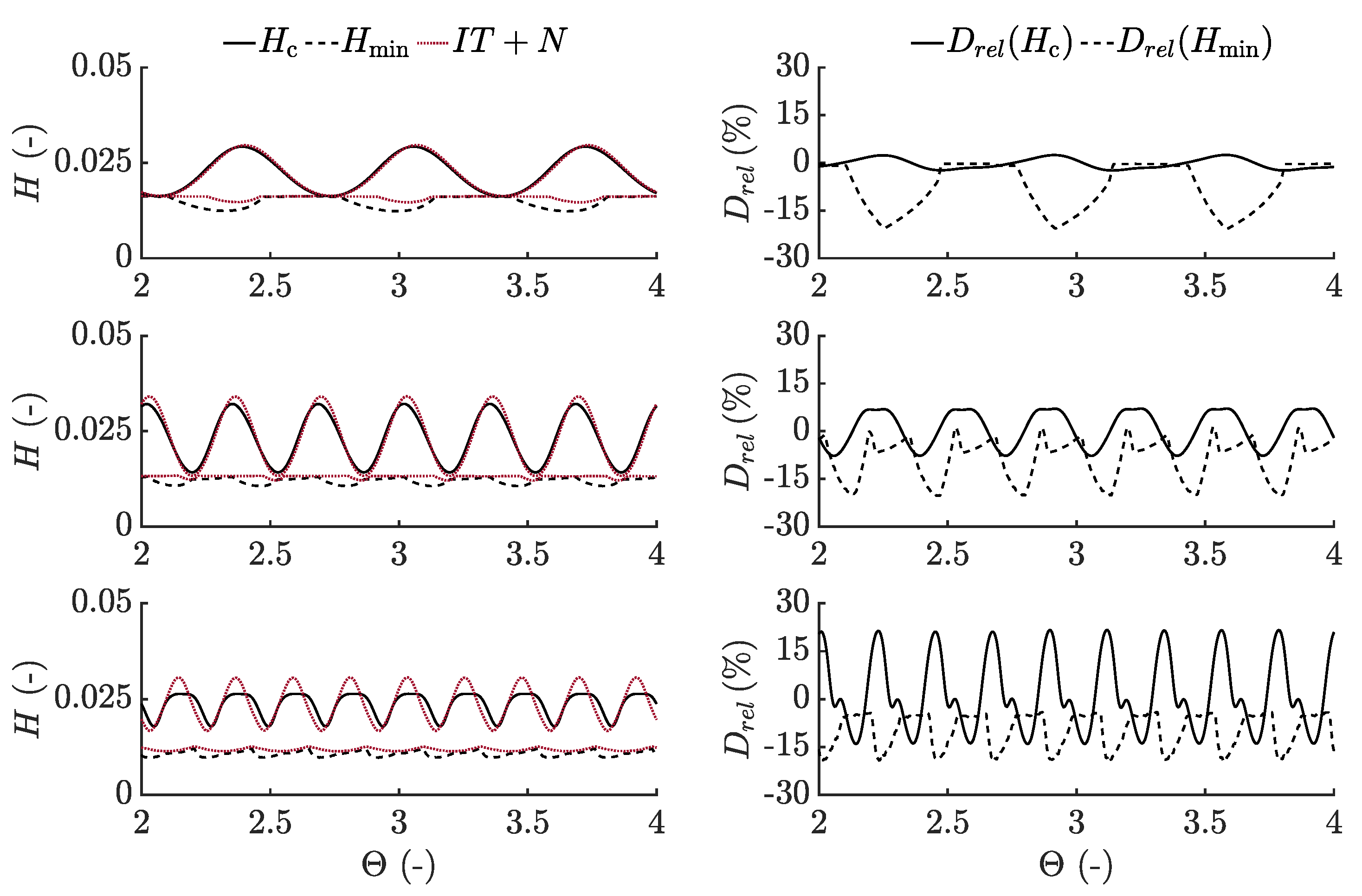
- In case of two-sided surface features overtaking within the contact, it was found that an interference between the film thickness perturbations did not occur due to the complementary function being generated in the inlet of the contact and travelling with the speed of the lubricant. On the other hand, due to the particular integral being directly connected to the roughness feature, an interference between the pressure and temperature variations were obvious.
- (v)
- In case of the overtaking event occurring in the inlet of the contact, one single complementary function was formed with larger amplitude than the other cases. Moreover, if overtaking instead took place in the outlet of the contact, a significantly reduced film thickness was noted.
- (vi)
- For the studied cases, it can be concluded that a thermal non-Newtonian approach is required for quantitatively accurate outlet film thickness predictions and may also be necessary for accurate predictions of film thickness within the contact if the complementary function is affected during formation. Otherwise, in the case of long wavelength roughness or low sliding conditions, an isothermal approach may be sufficient due to the inlet dominated film formation.
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Conductivity scaling parameter |
| Amplitude of surface roughness | |
| Dimensionless amplitude of surface roughness, | |
| a | Hertzian contact radius |
| Thermal expansivity | |
| Fragility parameter in viscosity equation | |
| Parameter for calculation of heat capacity | |
| Parameter in conductivity function | |
| Volumetric heat capacity of lubricant | |
| Specific heat capacity of lubricant | |
| Specific heat capacity of upper- and lower solid | |
| Young’s modulus of upper- and lower body, respectively | |
| F | Applied load per unit width |
| Shear thinning parameter | |
| g | Thermodynamic interaction parameter |
| h | Lubricant film thickness |
| Rigid body displacement | |
| H | Dimensionless lubricant film thickness |
| Isothermal bulk modulus at and zero absolute temperature | |
| Pressure rate of change of | |
| Thermal conductivity of lubricant | |
| Thermal conductivity of upper- and lower solid | |
| m | Parameter in heat capacity function |
| n | Shear thinning parameter |
| Maximum Hertzian pressure (1D) | |
| p | Hydrodynamic pressure in lubricant film |
| P | Dimensionless hydrodynamic pressure |
| q | Coefficient in conductivity scaling parameter |
| R | Equivalent radius of curvature |
| Radius of upper- and lower body, respectively | |
| s | Exponent in conductivity model |
| Visualised upper- and lower surface, respectively | |
| Slide-to-roll ratio | |
| Temperature and reference temperature, respectively | |
| Dimensionless temperature | |
| t | Time |
| Deformation in x and z, respectively | |
| Mean entrainment velocity of lubricant | |
| Speed of upper- and lower surface, respectively | |
| Lubricant velocity in -plane | |
| V | Volume of lubricant |
| Volume of lubricant at and , respectively | |
| Spatial coordinates | |
| Current- and initial position of roughness, respectively | |
| Dimensionless spatial coordinates | |
| Current- and initial dimensionless position of asperity, respectively | |
| Temperature coefficient | |
| Dimensionless shear dependent lubricant viscosity | |
| Shear dependent lubricant viscosity | |
| Lubricant resultant shear rate and shear rate in -plane, respectively | |
| Relaxation time at and ambient pressure | |
| Wavelength of asperity | |
| Dimensionless wavelength of asperity | |
| Lubricant Newtonian- and reference viscosity, respectively | |
| Extrapolated viscosity to infinite temperature | |
| Possion’s ratio of upper- and lower body, respectively | |
| Scaling parameters for viscosity | |
| Geometry of surface roughness | |
| Dimensionless geometry of surface roughness | |
| Dimensionless lubricant density | |
| Density of upper- and lower body | |
| Lubricant density and reference density, respectively | |
| Stress tensor | |
| Shear stress | |
| Dimensionless time |
References
- Morales-Espejel, G.E. Surface roughness effects in elastohydrodynamic lubrication: A review with contributions. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2014, 228, 1217–1242. [Google Scholar] [CrossRef]
- Lubrecht, A.A.; Napel, W.E.T.; Bosma, R. Multigrid, an alternative method for calculating film thickness and pressure profiles in elastohydrodynamically lubricated line contacts. J. Tribol. 1986, 108, 551–556. [Google Scholar] [CrossRef]
- Lubrecht, A.A.; Ten Napel, W.E.; Bosma, R. Multigrid, an alternative method of solution for two-dimensional elastohydrodynamically lubricated point contact calculations. J. Tribol. 1987, 109, 437–443. [Google Scholar] [CrossRef]
- Lubrecht, A.A. The Numerical Solution of the Elastohydrodynamically Lubricated Line- and Point Contact Problem, Using Multigrid Techniques. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 1987. [Google Scholar]
- Venner, C.; Lubrecht, A.; ten Napel, W.E. Numerical simulation of the overrolling of a surface feature in an EHL line contact. J. Tribol. 1991, 113, 777–783. [Google Scholar] [CrossRef]
- Venner, C.H. Multilevel Solution of the EHL Line and Point-Contact Problems. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 1991. [Google Scholar]
- Venner, C.H.; Lubrecht, A.A. Transient analysis of surface features in an EHL line contact in the case of sliding. J. Tribol. 1994, 116, 186–193. [Google Scholar] [CrossRef]
- Venner, C.H.; Lubrecht, A.A. Numerical simulation of a transverse ridge in a circular EHL contact under rolling/sliding. J. Tribol. 1994, 116, 751–761. [Google Scholar] [CrossRef]
- Ai, X.; Cheng, H.S. The Influence of Moving Dent on Point EHL Contacts. Tribol. Trans. 1994, 37, 323–335. [Google Scholar] [CrossRef]
- Venner, C.H.; Lubrecht, A.A. Numerical Simulation of Waviness in A Circular Ehl Contact, Under Rolling/Sliding. Tribol. Ser. 1995, 30, 259–272. [Google Scholar] [CrossRef]
- Venner, C.; Lubrecht, A.A. Numerical analysis of the influence of waviness on the film thickness of a circular EHL contact. J. Tribol. 1996, 118, 153–161. [Google Scholar] [CrossRef]
- Venner, C.H.; Morales-Espejel, G.E. Amplitude reduction of small-amplitude waviness in transient elastohydrodynamically lubricated line contacts. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1999, 213, 487–502. [Google Scholar] [CrossRef]
- Wedeven, L.D. Influence of debris dent on EHD lubrication. ASLE Trans. 1978, 21, 41–52. [Google Scholar] [CrossRef][Green Version]
- Wedeven, L.D.; Cusano, C. Elastohydrodynamic film thickness measurements of artificially produced surface dents and grooves. ASLE Trans. 1979, 22, 369–381. [Google Scholar] [CrossRef]
- Kaneta, M.; Sakai, T.; Nishikawa, H. Optical interferometric observations of the effects of a bump on point contact EHL. J. Tribol. 1992, 114, 779–784. [Google Scholar] [CrossRef]
- Kaneta, M.; Sakai, T.; Nishikawa, H. Effects of surface roughness on point contact EHL. Tribol. Trans. 1993, 36, 605–612. [Google Scholar] [CrossRef]
- Dundurs, J.; Tsai, K.C.; Keer, L.M. Contact between elastic bodies with wavy surfaces. J. Elast. 1973, 3, 109–115. [Google Scholar] [CrossRef]
- Manners, W. Partial contact between elastic surfaces with periodic profiles. Proc. R. Soc. Math. Phys. Eng. Sci. 1998, 454, 3203–3221. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Adams, G.G. Steady-state frictional sliding of two elastic bodies with a wavy contact interface. J. Tribol. 2000, 122, 490–495. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Johnson, K.L. The behaviour of transverse roughness in sliding elastohydrodynamically lubricated contacts. Wear 1992, 153, 107–117. [Google Scholar] [CrossRef]
- Greenwood, A.; Morales-Espejel, G.E. The behaviour of transverse roughness in EHL contacts. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1994, 208, 121–132. [Google Scholar] [CrossRef]
- Morales-Espejel, G.E. Elastohydrodynamic lubrication of smooth and rough surfaces. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 1993. [Google Scholar]
- Morales-Espejel, G.E.; Félix-Quiñonez, A. Kinematics of two-sided surface features in elastohydrodynamic lubrication. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1999, 213, 95–108. [Google Scholar] [CrossRef]
- Venner, C.H.; Couhier, F.; Lubrecht, A.A.; Greenwood, J.A. Amplitude reduction of waviness in transient EHL line contacts. Tribol. Ser. 1997, 32, 103–112. [Google Scholar]
- Lubrecht, A.A.; Graille, D.; Venner, C.H.; Greenwood, J.A. Waviness amplitude reduction in EHL line contacts under rolling-sliding. J. Tribol. 1998, 120, 705–709. [Google Scholar] [CrossRef]
- Lubrecht, A.A.; Venner, C. Elastohydrodynamic lubrication of rough surfaces. Proc. Inst. Mech. Eng. Part J. Eng. 1999, 213, 397–404. [Google Scholar] [CrossRef]
- Chapkov, A.D.; Venner, C.H.; Lubrecht, A.A. Roughness amplitude reduction under non-Newtonian EHD lubrication conditions. J. Tribol. 2006, 128, 753–760. [Google Scholar] [CrossRef]
- Hooke, C.J. Surface roughness modification in elastohydrodynamic line contacts operating in the elastic piezoviscous regime. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1998, 212, 145–162. [Google Scholar] [CrossRef]
- Hooke, C.J. The behaviour of low-amplitude surface roughness under line contacts. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1999, 213, 275–285. [Google Scholar] [CrossRef]
- Hooke, C.J. The behaviour of low-amplitude surface roughness under line contacts: Non-Newtonian fluids. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2000, 214, 253–265. [Google Scholar] [CrossRef]
- Hooke, G.J. Roughness in rolling-sliding elastohydrodynamic lubricated contacts. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2006, 220, 259–271. [Google Scholar] [CrossRef]
- Hooke, C.J.; Morales-Espejel, G.E. Analysis of general low-amplitude roughness in rolling–sliding elastohydrodynamic contacts including thermal effects. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2019, 233, 1648–1660. [Google Scholar] [CrossRef]
- Hooke, C.J.; Morales-Espejel, G.E. Rapid analysis of low-amplitude sinusoidal roughness in rolling-sliding elastohydrodynamic contacts including thermal effects. Proc. Inst. Mech. Eng. Part J. Mech. Eng. Sci. 2018, 232, 1690–1706. [Google Scholar] [CrossRef]
- Šperka, P.; Křupka, I.; Hartl, M. Experimental study of real roughness attenuation in concentrated contacts. Tribol. Int. 2010, 43, 1893–1901. [Google Scholar] [CrossRef]
- Šperka, P.; Křupka, I.; Hartl, M. Experimental study of real roughness attenuation in rolling/sliding concentrated contacts. Tribol. Int. 2012, 46, 14–21. [Google Scholar] [CrossRef]
- Šperka, P.; Křupka, I.; Hartl, M. Prediction of real rough surface deformation in pure rolling EHL contact: Comparison with experiment. Tribol. Trans. 2012, 55, 698–704. [Google Scholar] [CrossRef]
- Šperka, P.; Křupka, I.; Hartl, M. Experimental Study of Roughness Effect in a Rolling-Sliding EHL Contact. Part I: Roughness Deformation. Tribol. Trans. 2016, 59, 267–276. [Google Scholar] [CrossRef]
- Šperka, P.; Křupka, I.; Hartl, M. Experimental Study of Roughness Effect in a Rolling-Sliding EHL Contact. Part II: Complementary Effects. Tribol. Trans. 2016, 59, 277–285. [Google Scholar] [CrossRef]
- Félix-Quiñonez, A.; Ehret, P.; Summers, J.L. New Experimental Results of a Single Ridge Passing Through an EHL Conjunction. J. Tribol. 2003, 125, 252. [Google Scholar] [CrossRef]
- Morales-Espejel, G.E.; Wemekamp, A.W.; Félix-Quiñonez, A. Micro-geometry effects on the sliding friction transition in elastohydrodynamic lubrication. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2010, 224, 621–637. [Google Scholar] [CrossRef]
- Hooke, C.J.; Li, K.Y.; Morales-Espejel, G. Rapid calculation of the pressures and clearances in rough, rolling-sliding elastohydrodynamically lubricated contacts. Part 1: Low-amplitude, sinusoidal roughness. Proc. Inst. Mech. Eng. Part J. Mech. Eng. Sci. 2007, 221, 535–549. [Google Scholar] [CrossRef]
- Wang, J.; Venner, C.H.; Lubrecht, A.A. Amplitude reduction in EHL line contacts under rolling sliding conditions. Tribiol. Int. 2011, 44, 1997–2001. [Google Scholar] [CrossRef]
- Habchi, W.; Eyheramendy, D.; Vergne, P.; Morales-Espejel, G. A full-system approach of the elastohydrodynamic line/point contact problem. J. Tribol. 2008, 130, 021501. [Google Scholar] [CrossRef]
- Habchi, W.; Eyheramendy, D.; Bair, S.; Vergne, P.; Morales-Espejel, G. Thermal elastohydrodynamic lubrication of point contacts using a Newtonian/generalized Newtonian lubricant. Tribol. Lett. 2008, 30, 41–52. [Google Scholar] [CrossRef]
- Habchi, W. A Full-System Finite Element Approach to Elastohydrodynamic Lubrication Problems: Application to Ultra-Low-Viscosity Fluids. Ph.D. Thesis, University of Lyon, Lyon, France, 2008. [Google Scholar]
- Habchi, W.; Vergne, P.; Bair, S.; Andersson, O.; Eyheramendy, D.; Morales-Espejel, G.E. Influence of pressure and temperature dependence of thermal properties of a lubricant on the behaviour of circular TEHD contacts. Tribol. Int. 2010, 43, 1842–1850. [Google Scholar] [CrossRef]
- Habchi, W. A numerical model for the solution of thermal elastohydrodynamic lubrication in coated circular contacts. Tribol. Int. 2014, 73, 57–68. [Google Scholar] [CrossRef]
- Habchi, W. Coupling strategies for finite element modeling of thermal elastohydrodynamic lubrication problems. J. Tribol. 2017, 139, 041501. [Google Scholar] [CrossRef]
- Lohner, T.; Ziegltrum, A.; Stahl, K. Engineering software solution for thermal elastohydrodynamic lubrication using multiphysics software. Adv. Tribol. 2016, 2016, 1–13. [Google Scholar] [CrossRef]
- Ziegltrum, A.; Lohner, T.; Stahl, K. TEHL Simulation on the influence of lubricants on the frictional losses of DLC coated gears. Lubricants 2018, 6, 17. [Google Scholar] [CrossRef]
- Hultqvist, T.; Vrcek, A.; Marklund, P.; Prakash, B.; Larsson, R. Transient analysis of surface roughness features in thermal elastohydrodynamic contacts. Tribol. Int. 2020, 141, 105915. [Google Scholar] [CrossRef]
- Dowson, D.; Whitaker, A.V. A Numerical Procedure for the Solution of the Elastohydrodynamic Problem of Rolling and Sliding Contacts Lubricated by a Newtonian Fluid. Proc. Inst. Mech. Eng. Conf. Proc. 1965, 180, 57–71. [Google Scholar] [CrossRef]
- Kim, K.H.; Sadeghi, F. Three-dimensional temperature distribution in EHD lubrication: Part I—Circular contact. J. Tribol. 1992, 114, 32–41. [Google Scholar] [CrossRef]
- Kaneta, M.; Matsuda, K.; Wang, J.; Yang, P. Numerical Study on Effect of Dimples on Tribo-Characteristics in Non-Newtonian Thermal Elastohydrodynamic Lubrication Point Contacts With Different Mechanical and Thermal Properties. J. Tribol. 2020, 142, 1–11. [Google Scholar] [CrossRef]
- Almqvist, A. On the effects of surface roughness in lubrication. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2006. [Google Scholar] [CrossRef]
- Habchi, W. Finite Element Modeling of Elastohydrodynamic Lubrication Problems; Wiley Blackwell: Hoboken, NJ, USA, 2018. [Google Scholar]
- Dowson, D. A generalized Reynolds equation for fluid-film lubrication. Int. J. Mech. Sci. 1962, 4, 159–170. [Google Scholar] [CrossRef]
- Yang, P.; Wen, S. A generalized Reynolds equation for non-Newtonian thermal elastohydrodynamic lubrication. J. Tribol. 1990, 112, 631–636. [Google Scholar] [CrossRef]
- Raisin, J.; Fillot, N.; Dureisseix, D.; Vergne, P.; Lacour, V. Characteristic times in transient thermal elastohydrodynamic line contacts. Tribiol. Int. 2015, 82, 472–483. [Google Scholar] [CrossRef]
- Popovici, G.; Venner, C.H.; Lugt, P.M. Effects of load system dynamics on the film thickness in EHL contacts during start up. J. Tribol. 2004, 126, 258–266. [Google Scholar] [CrossRef][Green Version]
- Wu, S.R. A penalty formulation and numerical approximation of the Reynolds-Hertz problem of elastohydrodynamic lubrication. Int. J. Eng. Sci. 1986, 24, 1001–1013. [Google Scholar] [CrossRef]
- Björling, M.; Habchi, W.; Bair, S.; Larsson, R.; Marklund, P. Friction reduction in elastohydrodynamic contacts by thin-layer thermal insulation. Tribol. Lett. 2014, 53, 477–486. [Google Scholar] [CrossRef]
- Bair, S. Reference liquids for quantitative elastohydrodynamics: Selection and rheological characterization. Tribol. Lett. 2006, 22, 197–206. [Google Scholar] [CrossRef]
- Bair, S. High-Pressure Rheology for Quantitative Elastohydrodynamics; Elsevier: Amsterdam, The Netherlands, 2007; Volume 54. [Google Scholar]
- COMSOL Multiphysics® v. 5.5; COMSOL AB: Stockholm, Sweden, 2020.
- MathWorks. Matlab and Statistic Toolbox Release 2018; MathWorks: Natick, MA, USA, 2018. [Google Scholar]
- Habchi, W.; Vergne, P. On the compressive heating/cooling mechanism in thermal elastohydrodynamic lubricated contacts. Tribiology Int. 2015, 88, 143–152. [Google Scholar] [CrossRef]
- Hertz, H. Uber die Berührung fester Elastischer Körper. J. Die Reine Und Angew. Math. 1881, 92, 156–171. [Google Scholar]
- Couhier, F. Influence des Rugosités de Surface sur les Mécanismes de Lubrification du Contact élastohydrodynamique Cylindre-plan. Ph.D. Thesis, Lyon, INSA, Villeurbanne, France, 1996. [Google Scholar]
- Venner, C.H.; Bos, J. Effects of lubricant compressibility on the film thickness in EHL line and circular contacts. Wear 1994, 173, 151–165. [Google Scholar] [CrossRef]
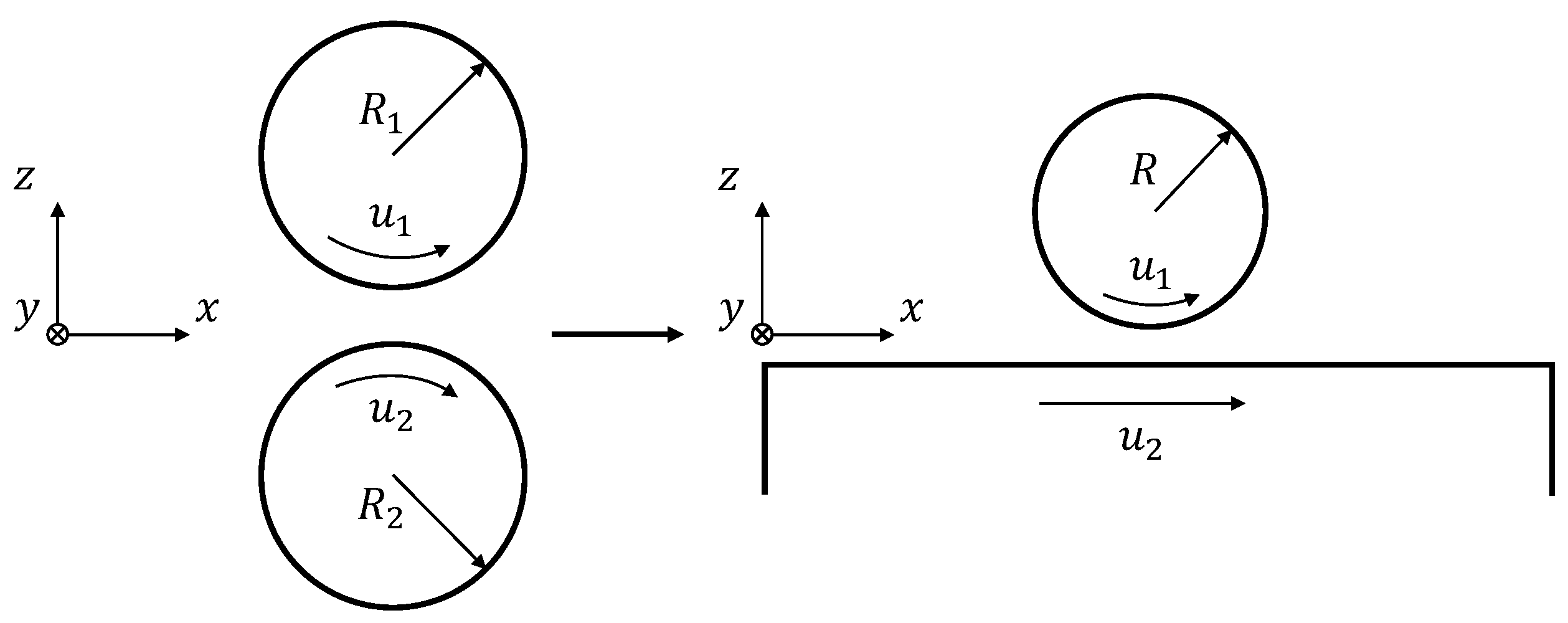








| Parameter | Value | Unit | Parameter | Value | Unit |
|---|---|---|---|---|---|
| 206 | F | ||||
| 7850 | |||||
| − | R | 15 | |||
| 45 | 40 | ||||
| 450 | 1 | ||||
| a | − |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hultqvist, T.; Vrček, A.; Marklund, P.; Larsson, R. On Waviness and Two-Sided Surface Features in Thermal Elastohydrodynamically Lubricated Line Contacts. Lubricants 2020, 8, 64. https://doi.org/10.3390/lubricants8060064
Hultqvist T, Vrček A, Marklund P, Larsson R. On Waviness and Two-Sided Surface Features in Thermal Elastohydrodynamically Lubricated Line Contacts. Lubricants. 2020; 8(6):64. https://doi.org/10.3390/lubricants8060064
Chicago/Turabian StyleHultqvist, Tobias, Aleks Vrček, Pär Marklund, and Roland Larsson. 2020. "On Waviness and Two-Sided Surface Features in Thermal Elastohydrodynamically Lubricated Line Contacts" Lubricants 8, no. 6: 64. https://doi.org/10.3390/lubricants8060064
APA StyleHultqvist, T., Vrček, A., Marklund, P., & Larsson, R. (2020). On Waviness and Two-Sided Surface Features in Thermal Elastohydrodynamically Lubricated Line Contacts. Lubricants, 8(6), 64. https://doi.org/10.3390/lubricants8060064






