The Influence of Surface Texturing on the Frictional Behaviour of Parallel Sliding Lubricated Surfaces under Conditions of Mixed Lubrication
Abstract
1. Introduction
2. Materials and Methods
3. Mathematical Solution and Modelling
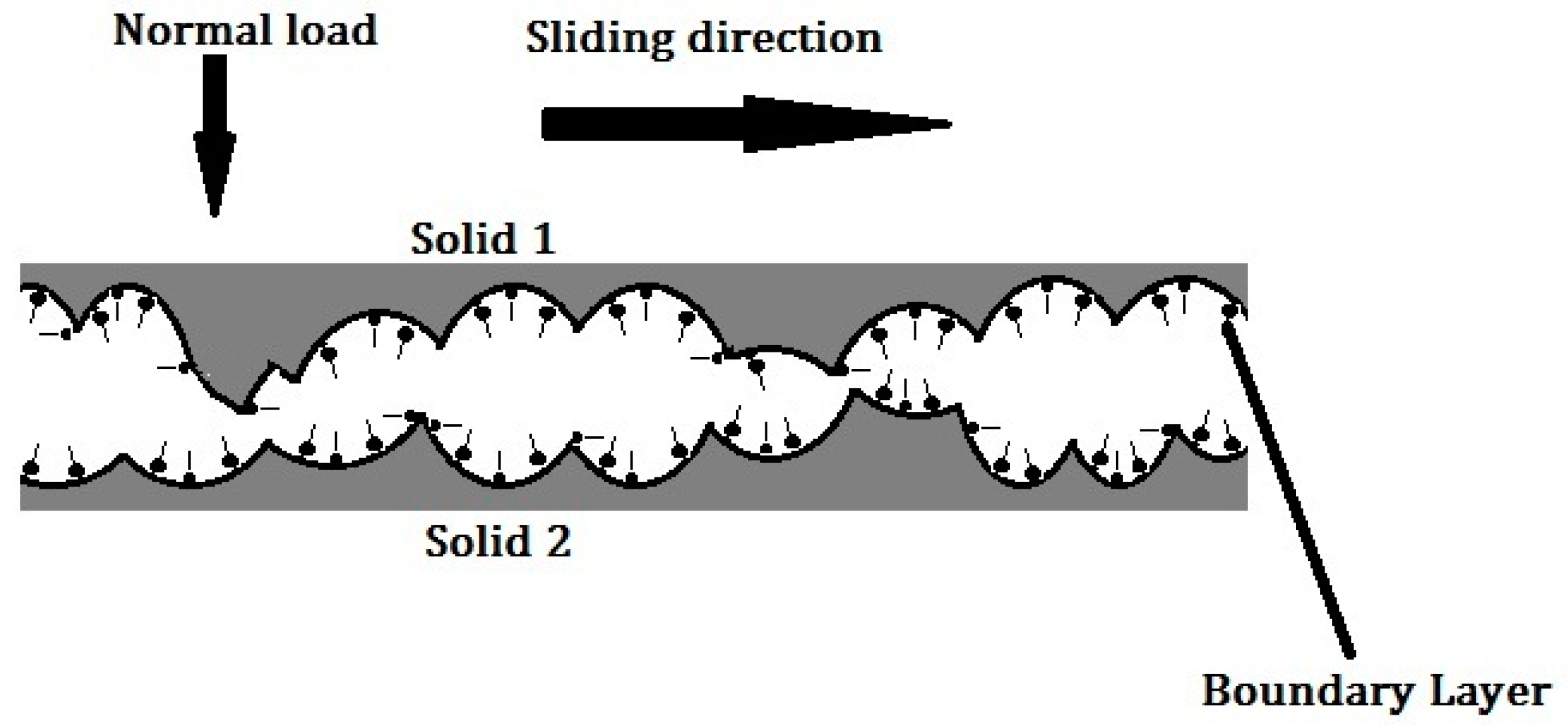
Deterministic Asperity Model:
- The first equation is Equation (2) ().
- The Reynolds equation.
- III.
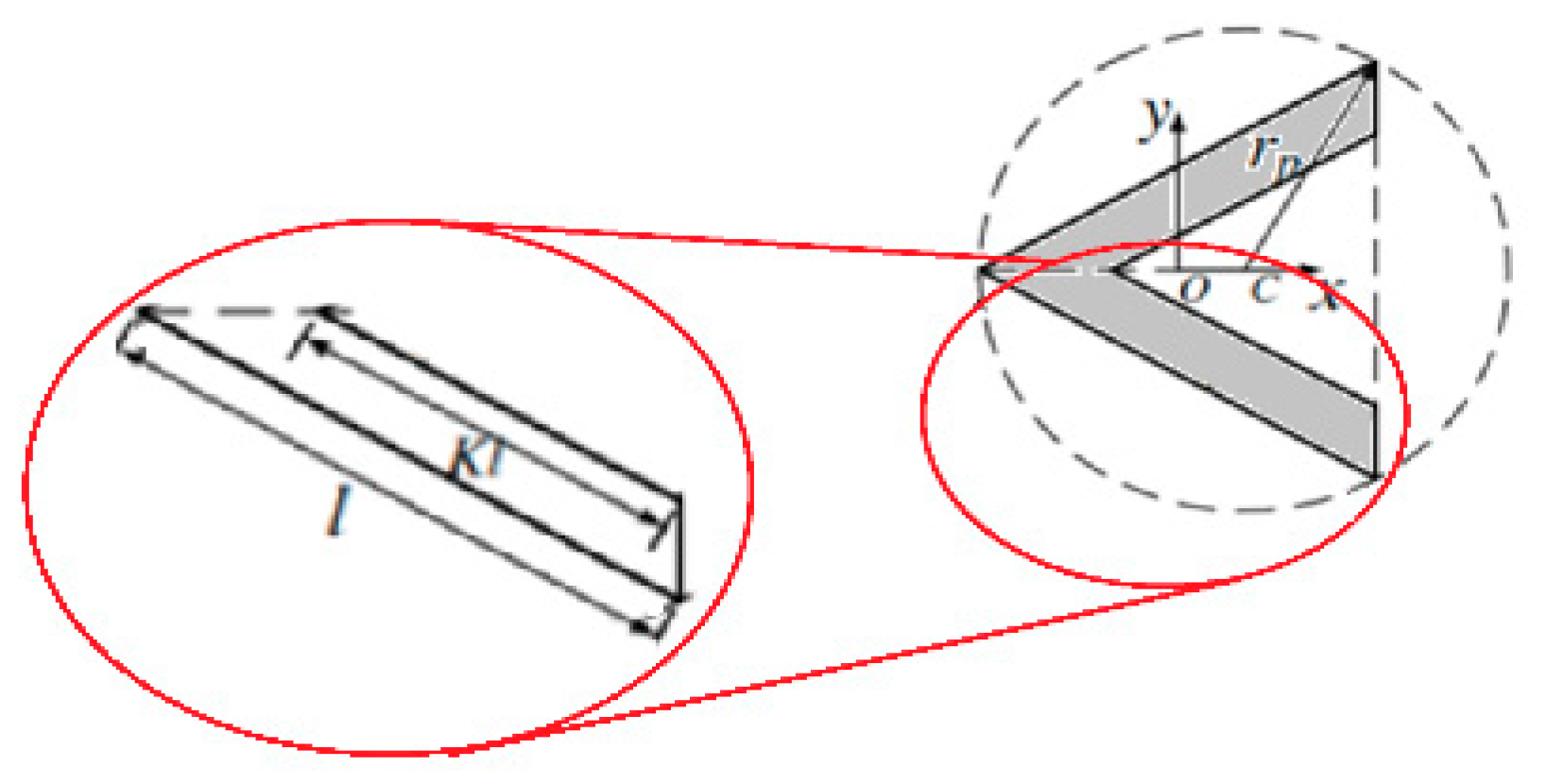
- The third equation is the force carried by asperities:in which is the combined elasticity modulus, and is the reduced radius of the cylinder. The reduced elastic modulus is given by:where and .
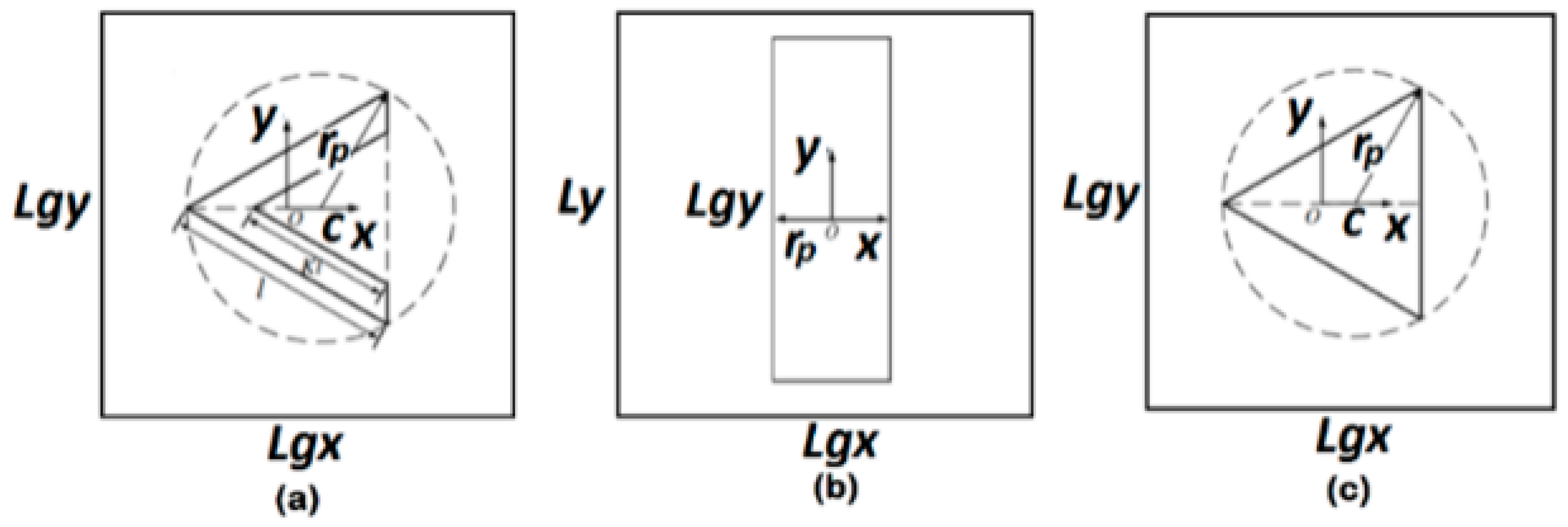
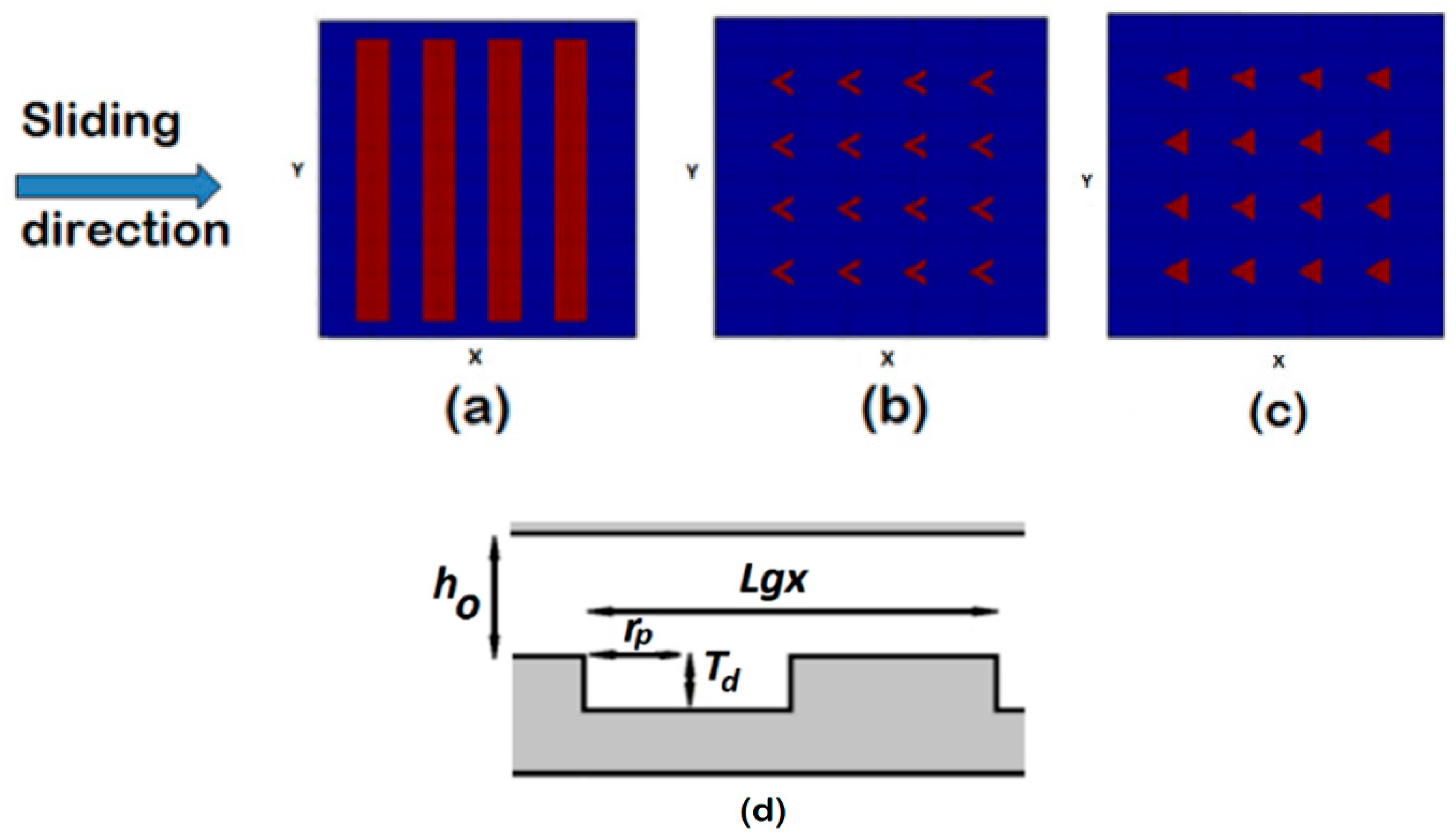
4. Problem Definition and its Solution
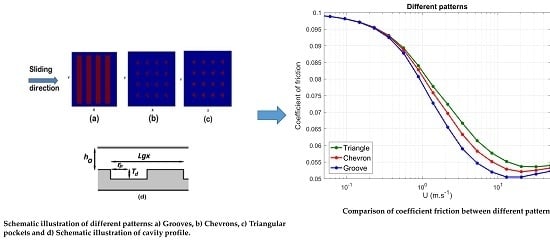
4.1. Comparison of Patterns
4.2. Property Effects
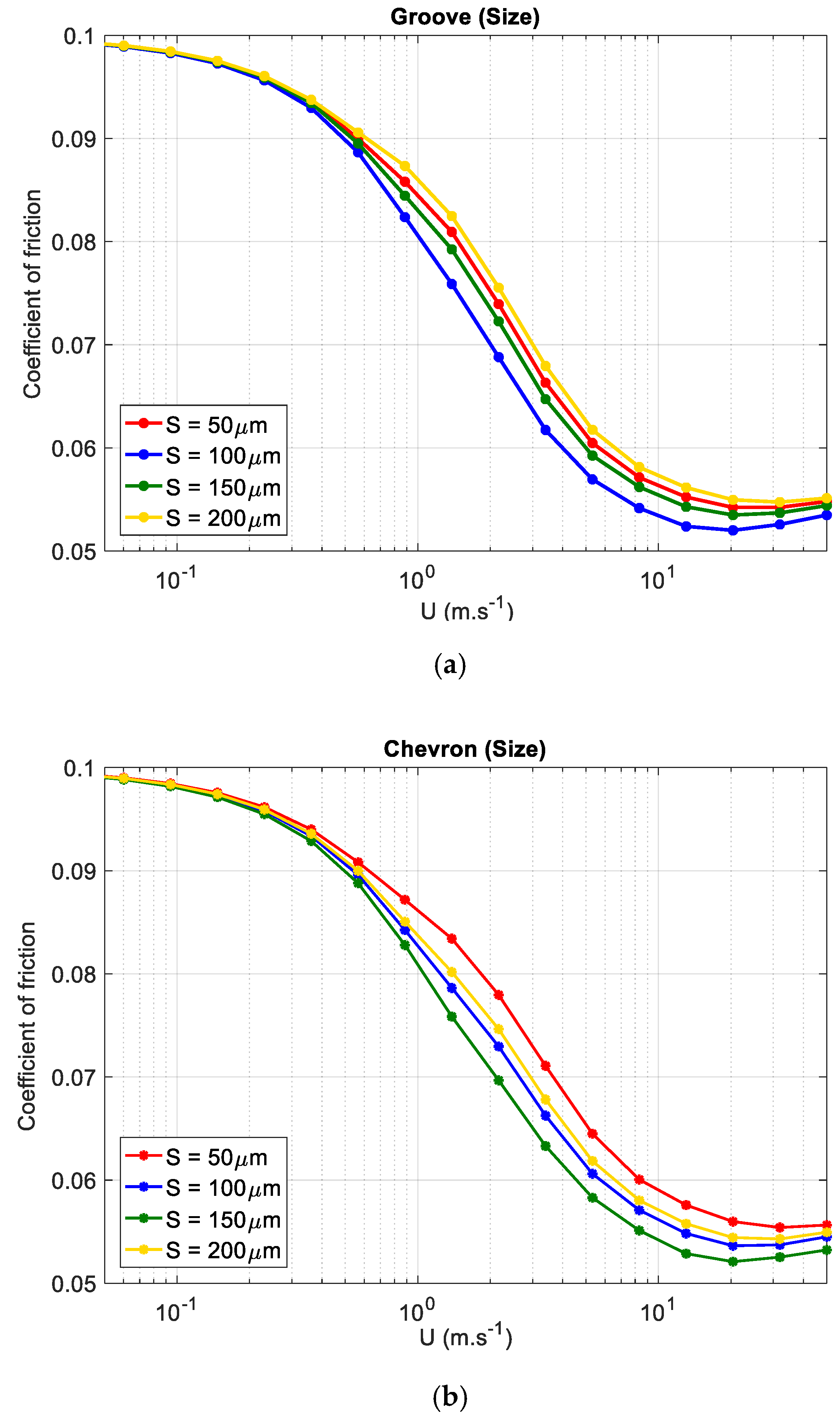
4.2.1. The Effect of Cavity Size (S)
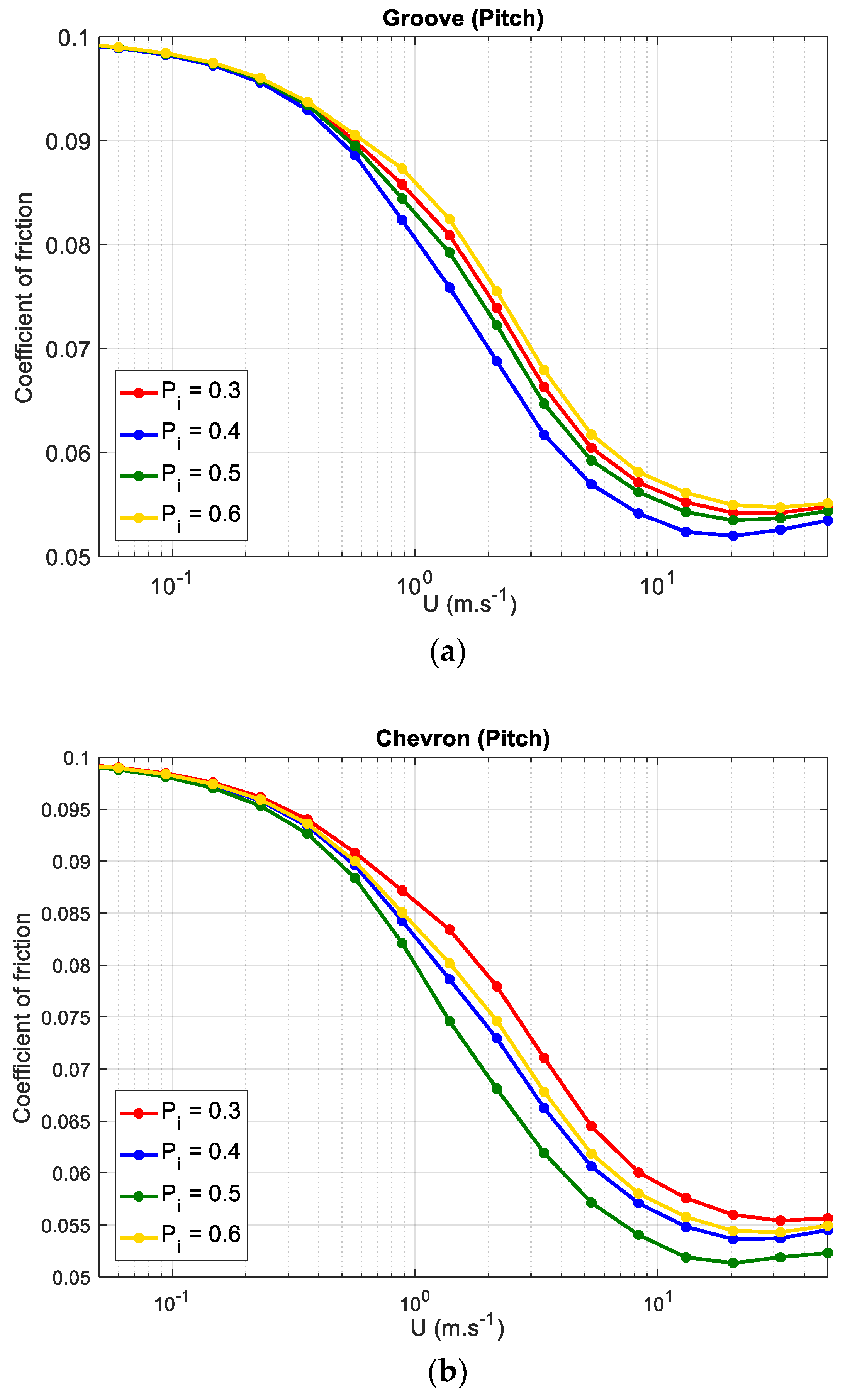
4.2.2. The Effect of Texture Pitch ()
5. Conclusions
- Of the patterns studied, the groove pattern shows the lowest coefficient of friction. When the distances between texture cells are the same (the pitch is constant), the groove pattern is more successful in generating a load-carrying capacity, because of the higher textured area fraction per unit area. Chevrons are more beneficial than triangular pockets.
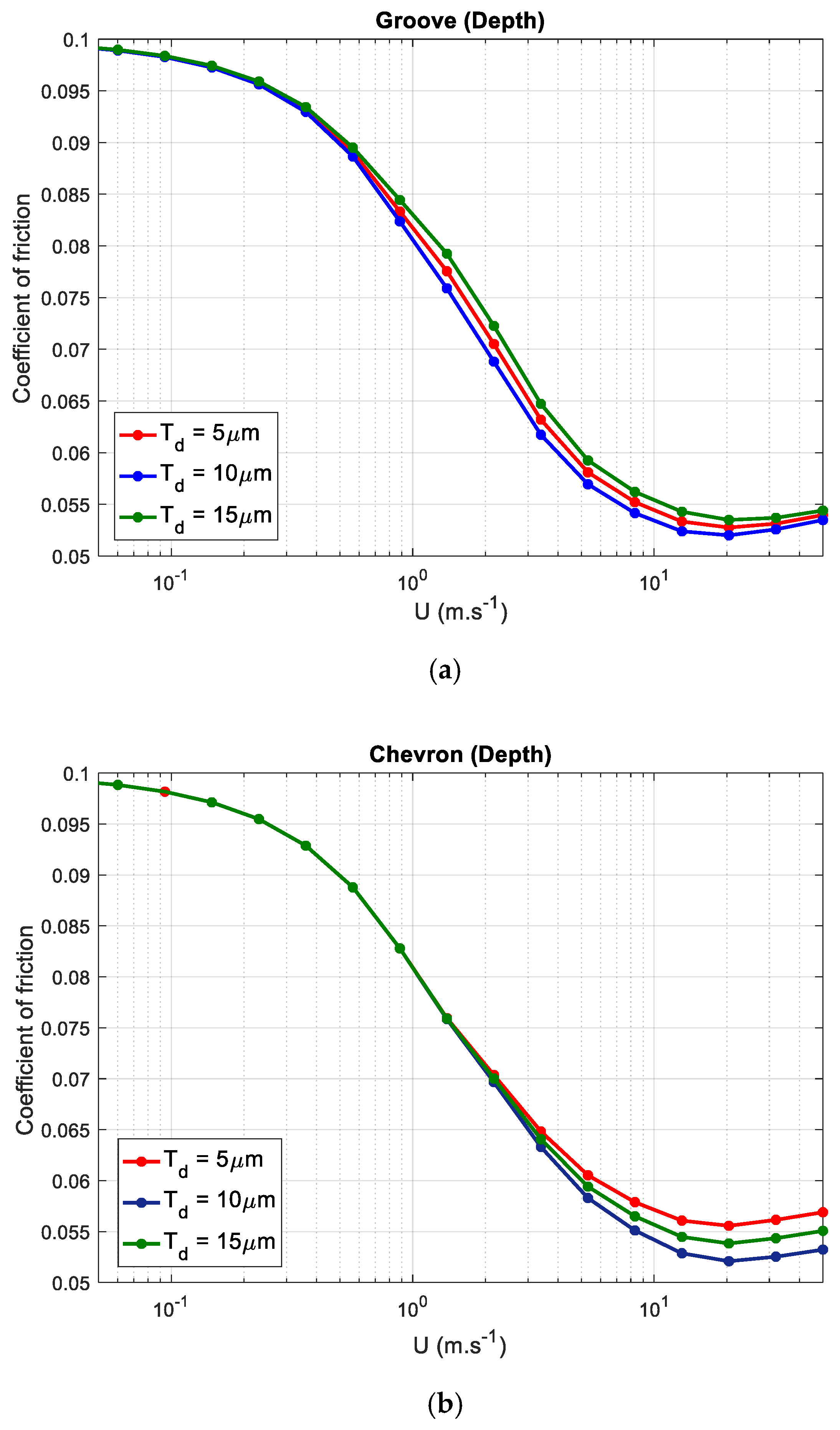
- For both chevron and groove patterns, texturing parameters such as depth, pitch, and size values have an influence on the hydrodynamic lubrication component in the mixed lubrication regime.
- An increase of the texture depth to a specific value of this parameter for different patterns leads to a reduction of coefficients of friction due to an increase in film thickness.
- In the case of a groove pattern, the optimum cavity sizes is approximately 100 µm, and in the case of chevrons, the optimum cavity size is approximately .
- The optimum depth for different patterns is in the range of .
- The optimum value for the texture pitch is for the groove pattern, and for the chevron patterned surfaces.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| η | Dynamic viscosity | Pa·s |
| Density | ||
| Cavitation dimensionless variable | ||
| Adaption parameter for hydrodynamic component in ML | ||
| Adaption parameter for asperity contact | ||
| Poisson’s ratio | ||
| Shear stress of asperity contact | ||
| Real area of asperity contact | ||
| Separation | ||
| Elasticity modulus | ||
| Reduced elasticity modulus | ||
| Coefficient of friction | ||
| Coefficient of friction in BL regime | ||
| Elrod switch function | ||
| Friction force | ||
| Hydrodynamic friction force | ||
| Dimensionless total normal load | ||
| Normal load on the contact | ||
| Load carried by the asperities | ||
| Load carried by the hydrodynamic component | ||
| Film thickness | ||
| Minimum film thickness | ||
| Dimensionless local depth of textured surface | ||
| Texture cell length in the x-direction | ||
| Textured area in the x-direction | ||
| Textured area in the y-direction | ||
| Texture pitch | ||
| Pressure | ||
| Dimensionless pressure | ||
| Average contact pressure | ||
| Ambient pressure | ||
| Cavitation pressure | ||
| Total pressure | ||
| Asperity pressure | ||
| Hydrodynamic pressure | ||
| Cavity characteristic radius | ||
| Reduced radius of cylinder | ||
| Cavity size = | ||
| Texture depth | ||
| Sum velocity | ||
| Dimensionless Cartesian coordinate | ||
| Dimensionless Cartesian coordinate | ||
| compliance of an asperity | ||
| Asperity height |
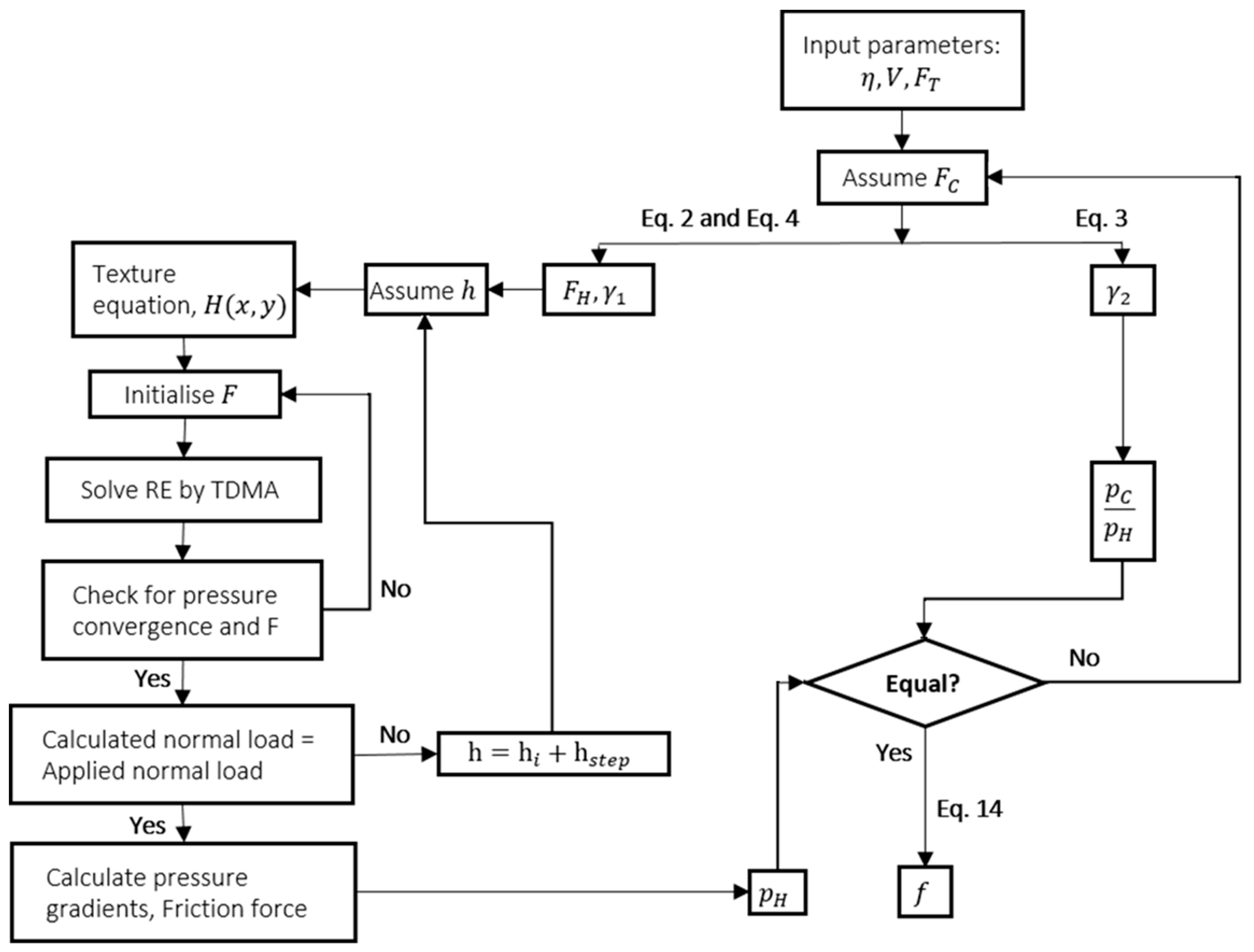
Appendix A. Mixed Lubrication Model Algorithm
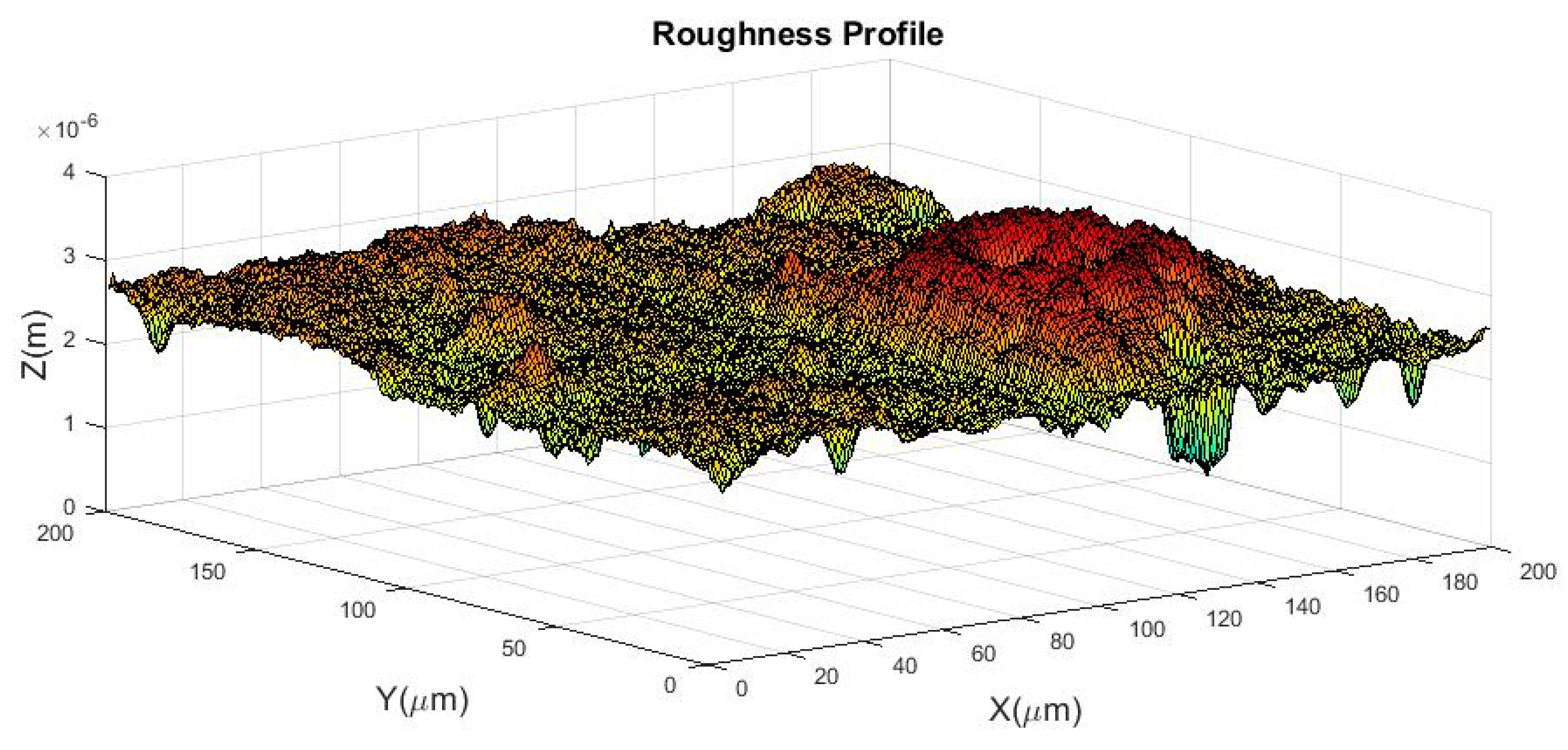
Appendix B. Determination of Roughness Parameters
Roughness Measurement
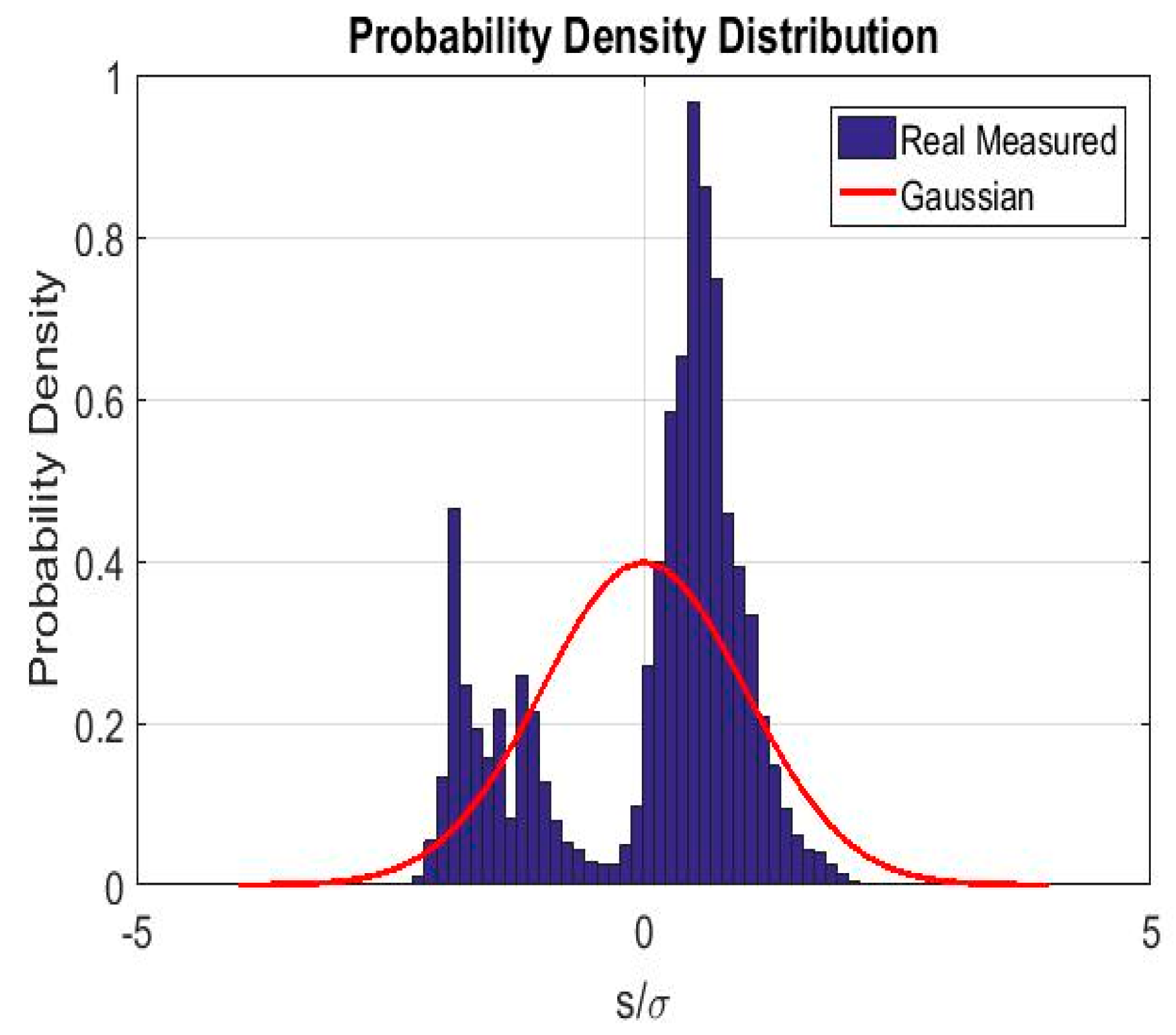
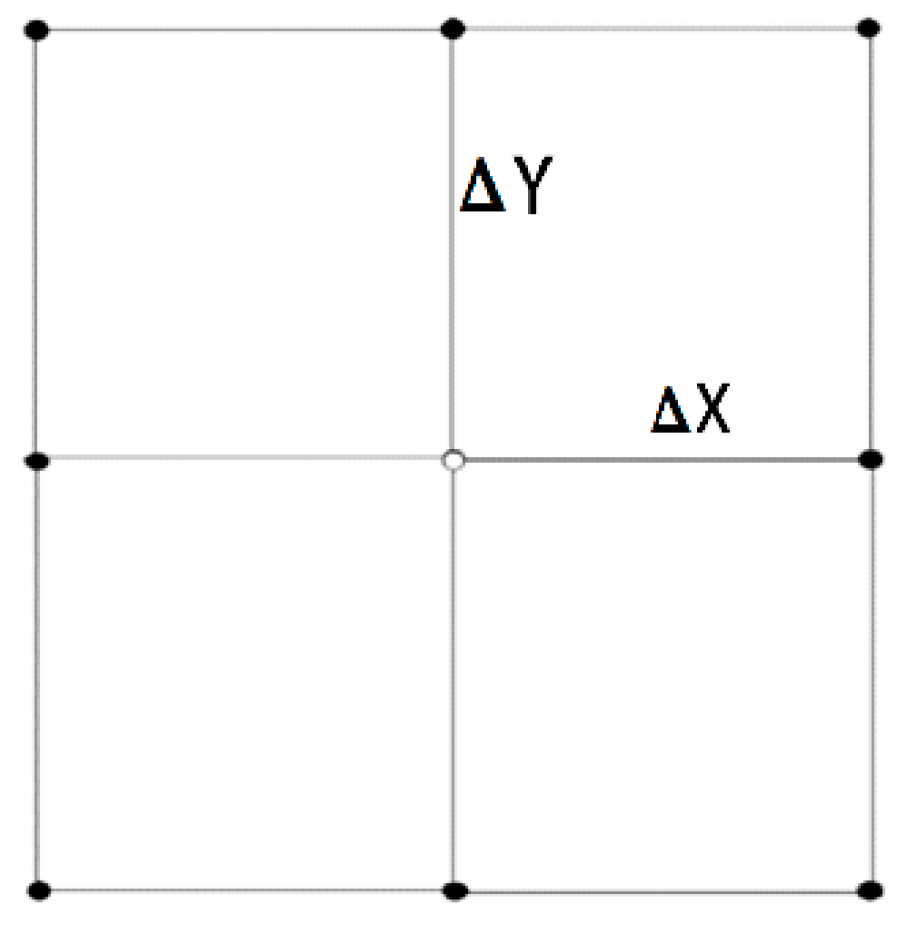
References
- Hirst, W. Scuffing and its prevention. Chart Mech. Eng. 1974, 21, 88–92. [Google Scholar]
- Wakuda, M.; Yamauchi, Y.; Kanzaki, S.; Yasuda, Y. Effect of surface texturing on friction reduction between ceramic and steel materials under lubricated sliding contact. Wear 2003, 254, 356–363. [Google Scholar] [CrossRef]
- Wang, W.-Z.; Huang, Z.; Shen, D.; Kong, L.; Li, S. The effect of triangle-shaped surface textures on the performance of the lubricated point-contacts. J. Tribol. 2013. [Google Scholar] [CrossRef]
- Baumgart, P.; Krajnovich, D.J.; Nguyen, T.A.; Tam, A.G. A new laser texturing technique for high performance magnetic disk drives. IEEE Trans. Magn. 1995, 31, 2946–2951. [Google Scholar] [CrossRef]
- Geiger, M.; Roth, S.; Becker, W. Influence of laser-produced microstructures on the tribological behaviour of ceramics. Surf. Coat. Technol. 1998, 100–101, 17–22. [Google Scholar] [CrossRef]
- Steinhoff, K.; Rasp, W.; Pawelski, O. Development of deterministic-stochastic surface structures to improve the tribological conditions of sheet forming processes. J. Mater. Process. Technol. 1996, 60, 355–361. [Google Scholar] [CrossRef]
- Etsion, I.; Burstein, L. A Model for Mechanical Seals with Regular Microsurface Structure. Tribol. Trans. 1996, 39, 677–683. [Google Scholar] [CrossRef]
- Etsion, I.; Kligerman, Y.; Halperin, G. Analytical and Experimental Investigation of Laser-Textured Mechanical Seal Faces. Tribol. Trans. 1999, 42, 511–516. [Google Scholar] [CrossRef]
- Kovalchenko, A.; Ajayi, O.; Erdemir, A.; Fenske, G.; Etsion, I. The effect of laser surface texturing on transitions in lubrication regimes during unidirectional sliding contact. Tribol. Int. 2005, 38, 219–225. [Google Scholar] [CrossRef]
- Dobrica, M.B.; Fillon, M.; Pascovici, M.D.; Cicone, T. Optimizing surface texture for hydrodynamic lubricated contacts using a mass-conserving numerical approach. Proc. Inst. Mech. Eng. 2010, 224, 737–750. [Google Scholar] [CrossRef]
- Zhu, D.; Hu, Y.-Z. A computer program package for the prediction of EHL and mixed lubrication characteristics, friction, subsurface stresses and flash temperatures based on measured 3-d surface roughness. Tribol. Trans. 2001, 44, 383–390. [Google Scholar] [CrossRef]
- Hu, Y.Z.; Zhu, D. A full numerical solution to the mixed lubrication in point contacts. J. Tribol. 2000, 122, 1–9. [Google Scholar] [CrossRef]
- Zhu, D.; Cheng, H.S.; Hamrock, B.J. Effect of surface roughness on pressure spike and film constriction in elastohydrodynamically lubricated line contacts. Tribol. Trans. 1990, 33, 267–273. [Google Scholar] [CrossRef]
- Hamilton, D.B.; Walowit, J.A.; Allen, C.M. A theory of lubrication by microirregularities. J. Basic Eng. 1966, 88, 177–185. [Google Scholar] [CrossRef]
- Ronen, A.; Etsion, I.; Kligerman, Y. Friction-reducing surface-texturing in reciprocating automotive components. Tribol. Trans. 2001, 44, 359–366. [Google Scholar] [CrossRef]
- Galda, L.; Pawlus, P.; Sep, J. Dimples shape and distribution effect on characteristics of Stribeck curve. Tribol. Int. 2009, 42, 1505–1512. [Google Scholar] [CrossRef]
- Qiu, Y.; Khonsari, M.M. Experimental investigation of tribological performance of laser textured stainless steel rings. Tribol. Int. 2011, 44, 635–644. [Google Scholar] [CrossRef]
- Kovalchenko, A.; Ajayi, O.; Erdemir, A.; Fenske, G.; Etsion, I. The effect of laser texturing of steel surfaces and speed-load parameters on the transition of lubrication regime from boundary to hydrodynamic. Tribol. Trans. 2004, 47, 299–307. [Google Scholar] [CrossRef]
- Raeymaekers, B.; Etsion, I.; Talke, F.E. Enhancing tribological performance of the magnetic tape/guide interface by laser surface texturing. Tribol. Lett. 2007, 27, 89–95. [Google Scholar] [CrossRef]
- Wang, X.; Kato, K.; Adachi, K.; Aizawa, K. Loads carrying capacity map for the surface texture design of SiC thrust bearing sliding in water. Tribol. Int. 2003, 36, 189–197. [Google Scholar] [CrossRef]
- Wang, X.; Kato, K.; Adachi, K.; Aizawa, K. The effect of laser texturing of SiC surface on the critical load for the transition of water lubrication mode from hydrodynamic to mixed. Tribol. Int. 2001, 34, 703–711. [Google Scholar] [CrossRef]
- Costa, H.L.; Hutchings, I.M. Hydrodynamic lubrication of textured steel surfaces under reciprocating sliding conditions. Tribol. Int. 2007, 40, 1227–1238. [Google Scholar] [CrossRef]
- Wang, Q.J.; Zhu, D. Virtual texturing: Modeling the performance of lubricated contacts of engineered surfaces. J. Tribol. 2005, 127, 722–728. [Google Scholar] [CrossRef]
- Ren, N.; Nanbu, T.; Yasuda, Y.; Zhu, D.; Wang, Q. Micro textures in concentrated-conformal-contact lubrication: Effect of distribution patterns. Tribol. Lett. 2007, 28, 275–285. [Google Scholar] [CrossRef]
- Rahmani, R.; Shirvani, A.; Shirvani, H. Optimization of partially textured parallel thrust bearings with square-shaped micro-dimples. Tribol. Trans. 2007, 50, 401–406. [Google Scholar] [CrossRef]
- Pascovici, M.D.; Cicone, T.; Fillon, M.; Dobrica, M.B. Analytical investigation of a partially textured parallel slider. Proc. Inst. Mech. Eng. 2009, 223, 151–158. [Google Scholar] [CrossRef]
- Wang, X.; Adachi, K.; Otsuka, K.; Kato, K. Optimization of the surface texture for silicon carbide sliding in water. Appl. Surf. Sci. 2006, 253, 1282–1286. [Google Scholar] [CrossRef]
- Wang, X.; Kato, K. Improving the anti-seizure ability of SiC seal in water with RIE texturing. Tribol. Lett. 2003, 14, 275–280. [Google Scholar] [CrossRef]
- Nakano, M.; Korenaga, A.; Korenaga, A.; Miyake, K.; Murakami, T.; Ando, Y.; Usami, H.; Sasaki, S. Applying micro-texture to cast iron surfaces to reduce the friction coefficient under lubricated conditions. Tribol. Lett. 2007, 28, 131–137. [Google Scholar] [CrossRef]
- Raeymaekers, B.; Etsion, I.; Talke, F.E. A model for magnetic tape/guide friction reduction by laser surface texturing. Tribol. Lett. 2007, 28, 9–17. [Google Scholar] [CrossRef]
- Kligerman, Y.; Etsion, I. Analysis of the hydrodynamic effects in a surface textured circumferential gas seal. Tribol. Trans. 2001, 44, 472–478. [Google Scholar] [CrossRef]
- Stribeck, R. Die Wesentlichen Eigenschaften der Gleit- Und Rollenlager (The Basic Properties of Sliding and Rolling Bearings). Z. Ver. Deutsch. Ing. 1902, 46, 1341–1348. [Google Scholar]
- Hersey, M.D. The laws of lubrication of horizontal journal bearings. J. Wash. Acad. Sci. 1914, 4, 542–552. [Google Scholar]
- Lenning, R.L. The transition from boundary to mixed friction. Lubr. Eng. 1960, 16, 575. [Google Scholar]
- Schipper, D.J. Transitions in the Lubrication of Concentrated Contacts. Ph.D. Theses, University of Twente, Enschede, Sweden, 1988. [Google Scholar]
- Patir, N.; Cheng, H.S. Application of Average Flow Model to Lubrication Between Rough Sliding Surfaces. J. Lubr. Technol. 1978. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Lubr. Technol. 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Johnson, K.L.; Greenwood, J.A.; Poon, S.Y. A simple theory of asperity contact in elastohydro-dynamic lubrication. Wear 1972, 19, 91–108. [Google Scholar] [CrossRef]
- Gelinck, E.R.M.; Schipper, D.J. Calculation of Stribeck curves for line contacts. Tribol. Int. 2000, 33, 175–181. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Williamson, J.B.P. Contact of Nominally Flat Surfaces. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1966, 295, 300–319. [Google Scholar] [CrossRef]
- Shi, F.; Salant, R.F. A mixed soft elastohydrodynamic lubrication model with interasperity cavitation and surface shear deformation. J. Tribol. 2000, 122, 308–316. [Google Scholar] [CrossRef]
- Jakobsson, B.; Floberg, L. The Finite Journal Bearing, Considering Vaporization: (Das Gleitlager von Endlicher Breite mit Verdampfung); Gumpert: Göteborg, Sweden, 1957. [Google Scholar]
- Olsson, K.O. Cavitation in Dynamically Loaded Bearings; Chalmers University of Technology: Göteborg, Sweden, 1965. [Google Scholar]
- Coyne, J.C.; Elrod, J.H.G. Conditions for the rupture of a lubricating film. Part I Theor. Model. J. Lubr. Technol. 1970, 92, 451–456. [Google Scholar]
- Faraon, I.C. Mixed Lubricated Line Contacts, Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2005. [Google Scholar]
- Bijani, D.; Deladi, L.E.; Schipper, D.J. The Influence of Surface Texturing on the Film Thickness in Parallel Sliding Surfaces. In Proceedings of the 20th International Colloquium Tribology, Stuttgart/Ostfildern, Germany, 12–14 January 2016. [Google Scholar]
- Xiong, S.; Wang, Q.J. Steady-state hydrodynamic lubrication modeled with the payvar-salant mass conservation model. J. Tribol. 2012. [Google Scholar] [CrossRef]
- Qiu, M.; Minson, B.R.; Raeymaekers, B. The effect of texture shape on the friction coefficient and stiffness of gas-lubricated parallel slider bearings. Tribol. Int. 2013, 67, 278–288. [Google Scholar] [CrossRef]
- Qiu, M.; Delic, A.; Raeymaekers, B. The effect of texture shape on the load-carrying capacity of gas-lubricated parallel slider bearings. Tribol. Lett. 2012, 48, 315–327. [Google Scholar] [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow, Series in Computational Methods in Mechanics and Thermal Sciences; Patankar, S.V., Ed.; Hemisphere Pub. Corp.: Washington, DC, USA, 1980. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics—The Finite Volume Method; Longman Group Ltd.: Harlow, UK, 1995. [Google Scholar]
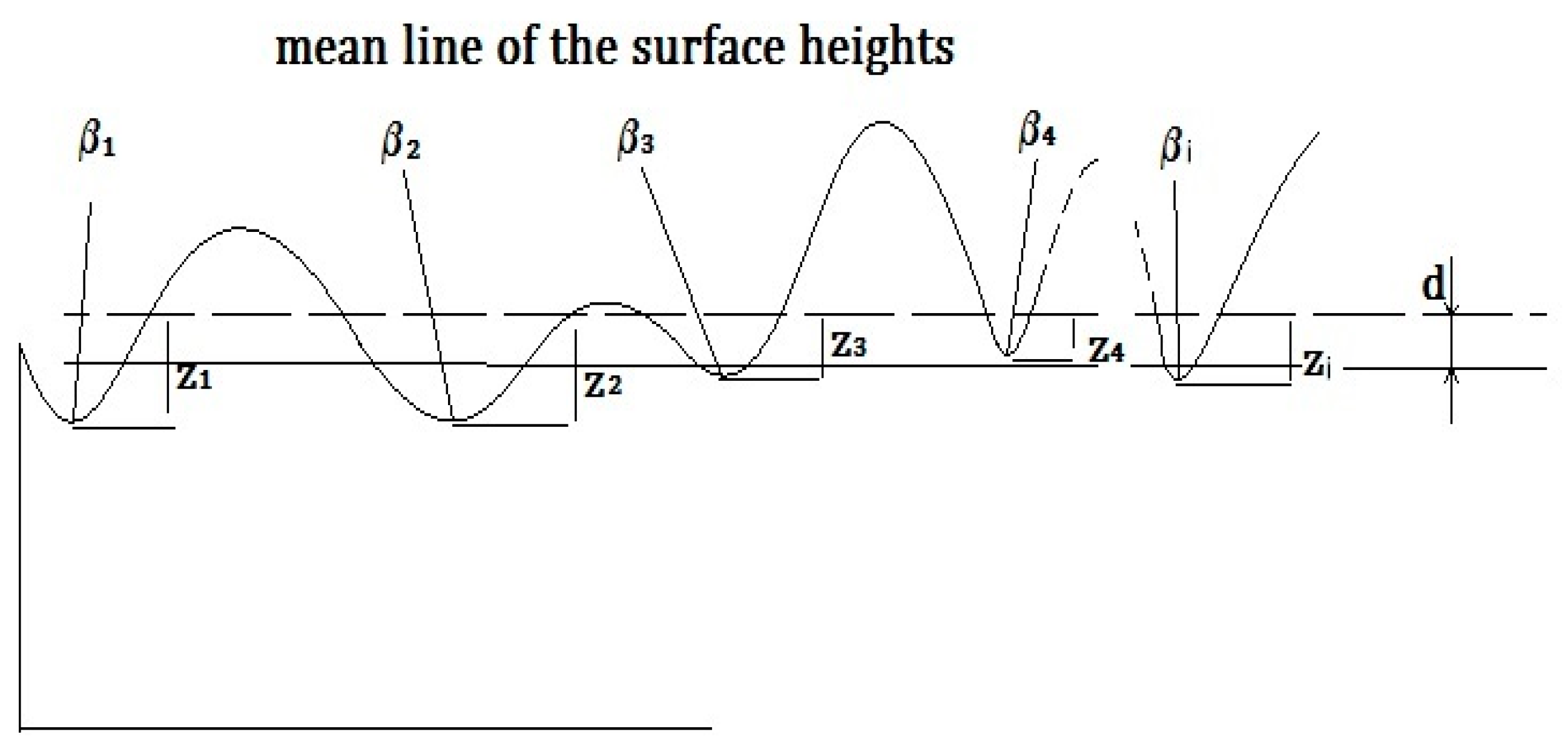

| Parameter | Value |
|---|---|
| Normal load | |
| Average contact pressure | |
| Dynamic viscosity | |
| 210 GPa | |
| 0.3 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bijani, D.; Deladi, E.L.; Akchurin, A.; De Rooij, M.B.; Schipper, D.J. The Influence of Surface Texturing on the Frictional Behaviour of Parallel Sliding Lubricated Surfaces under Conditions of Mixed Lubrication. Lubricants 2018, 6, 91. https://doi.org/10.3390/lubricants6040091
Bijani D, Deladi EL, Akchurin A, De Rooij MB, Schipper DJ. The Influence of Surface Texturing on the Frictional Behaviour of Parallel Sliding Lubricated Surfaces under Conditions of Mixed Lubrication. Lubricants. 2018; 6(4):91. https://doi.org/10.3390/lubricants6040091
Chicago/Turabian StyleBijani, Dariush, Elena L. Deladi, Aydar Akchurin, Matthijn B. De Rooij, and Dirk J. Schipper. 2018. "The Influence of Surface Texturing on the Frictional Behaviour of Parallel Sliding Lubricated Surfaces under Conditions of Mixed Lubrication" Lubricants 6, no. 4: 91. https://doi.org/10.3390/lubricants6040091
APA StyleBijani, D., Deladi, E. L., Akchurin, A., De Rooij, M. B., & Schipper, D. J. (2018). The Influence of Surface Texturing on the Frictional Behaviour of Parallel Sliding Lubricated Surfaces under Conditions of Mixed Lubrication. Lubricants, 6(4), 91. https://doi.org/10.3390/lubricants6040091