A Simulation Study for the Design of Membrane Restrictor in an Opposed-Pad Hydrostatic Bearing to Achieve High Static Stiffness
Abstract
:1. Introduction
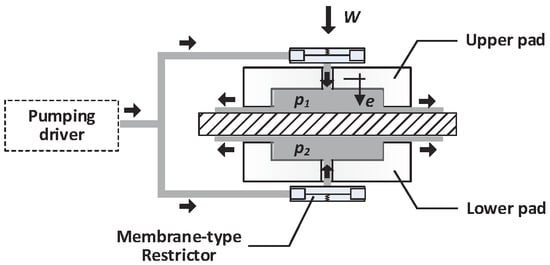
2. Bearing Models for the Opposed-Pad Configuration
3. The Effects of Restrictor Design on Bearing Performance
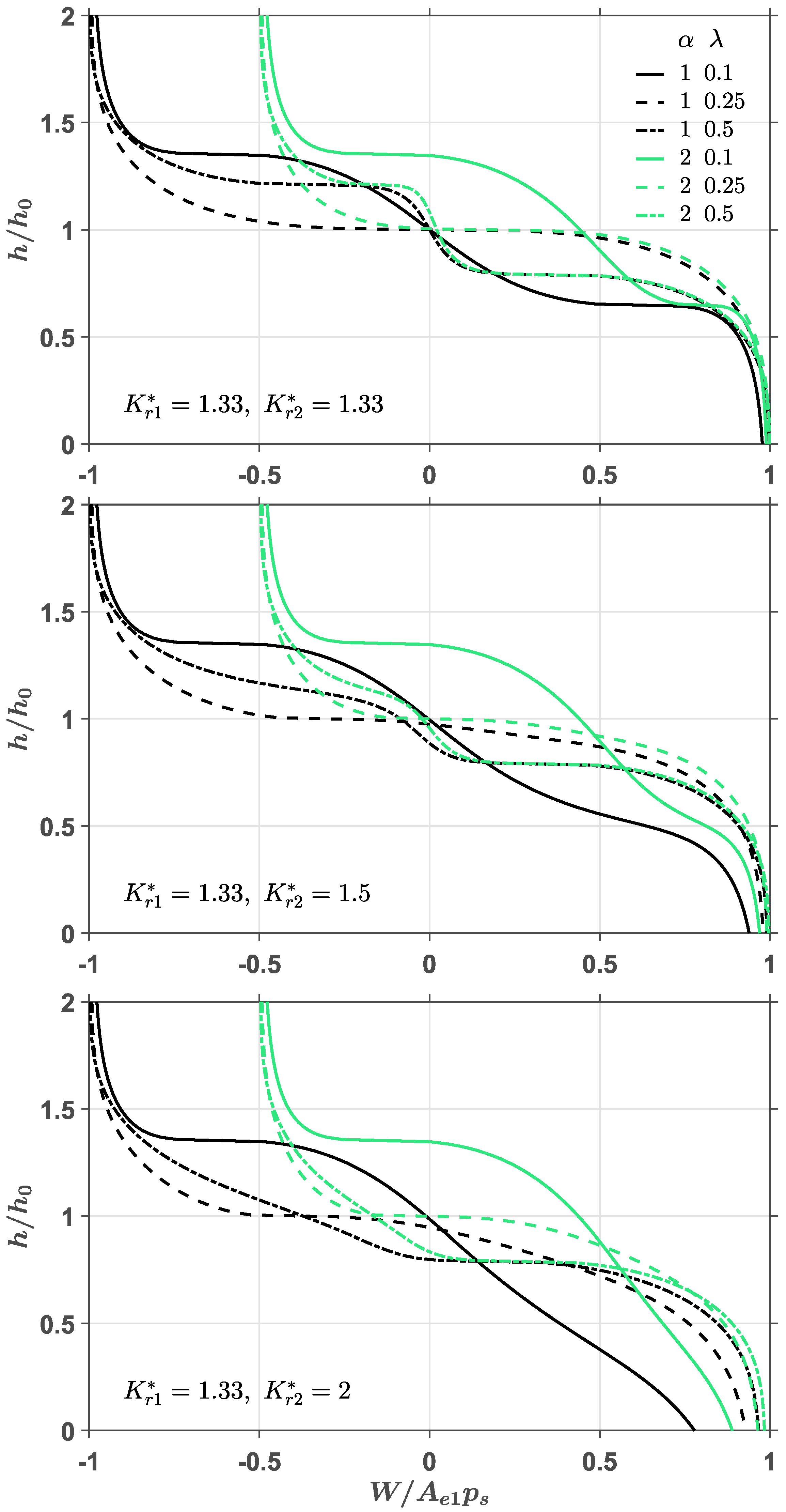
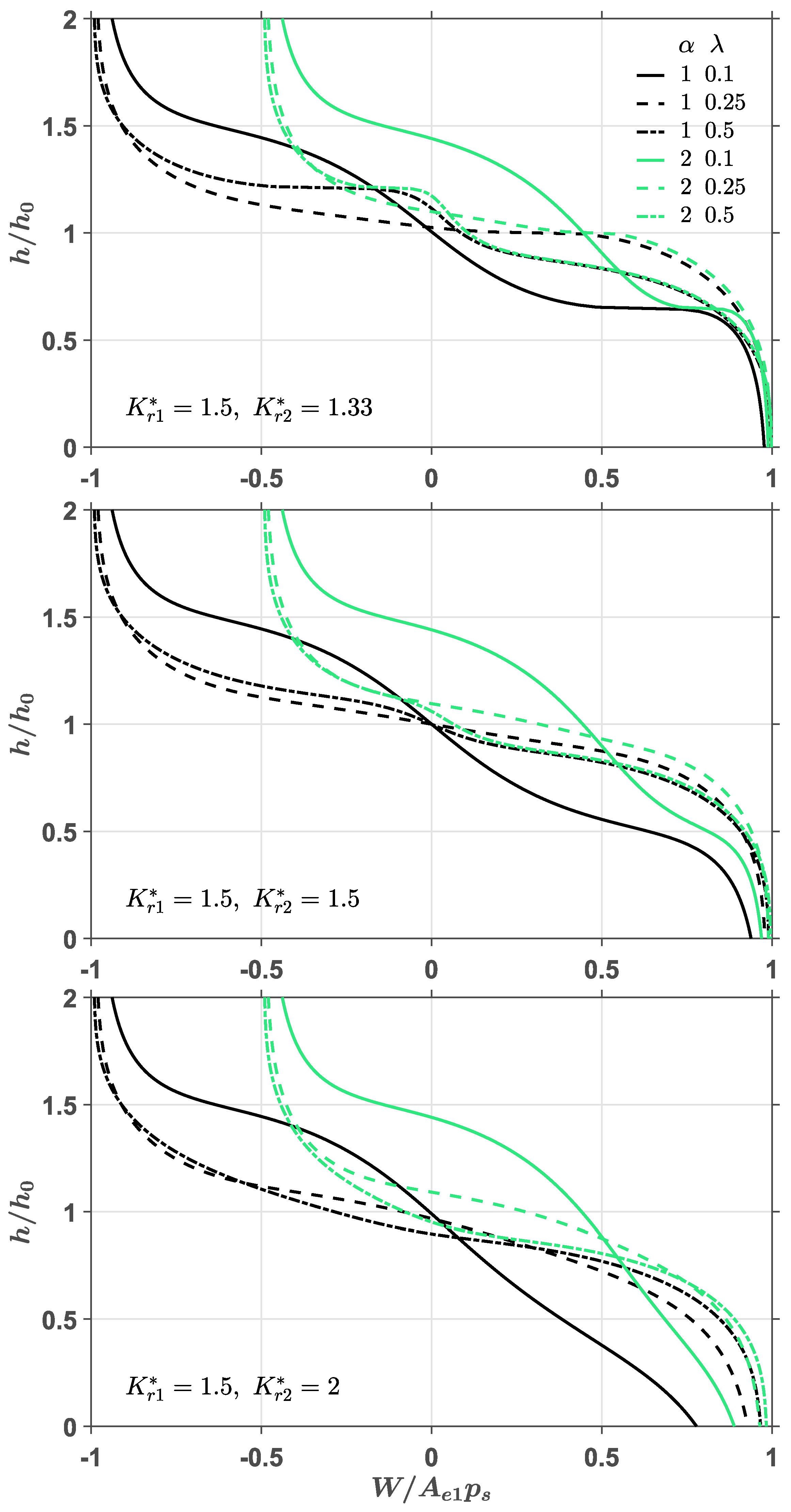
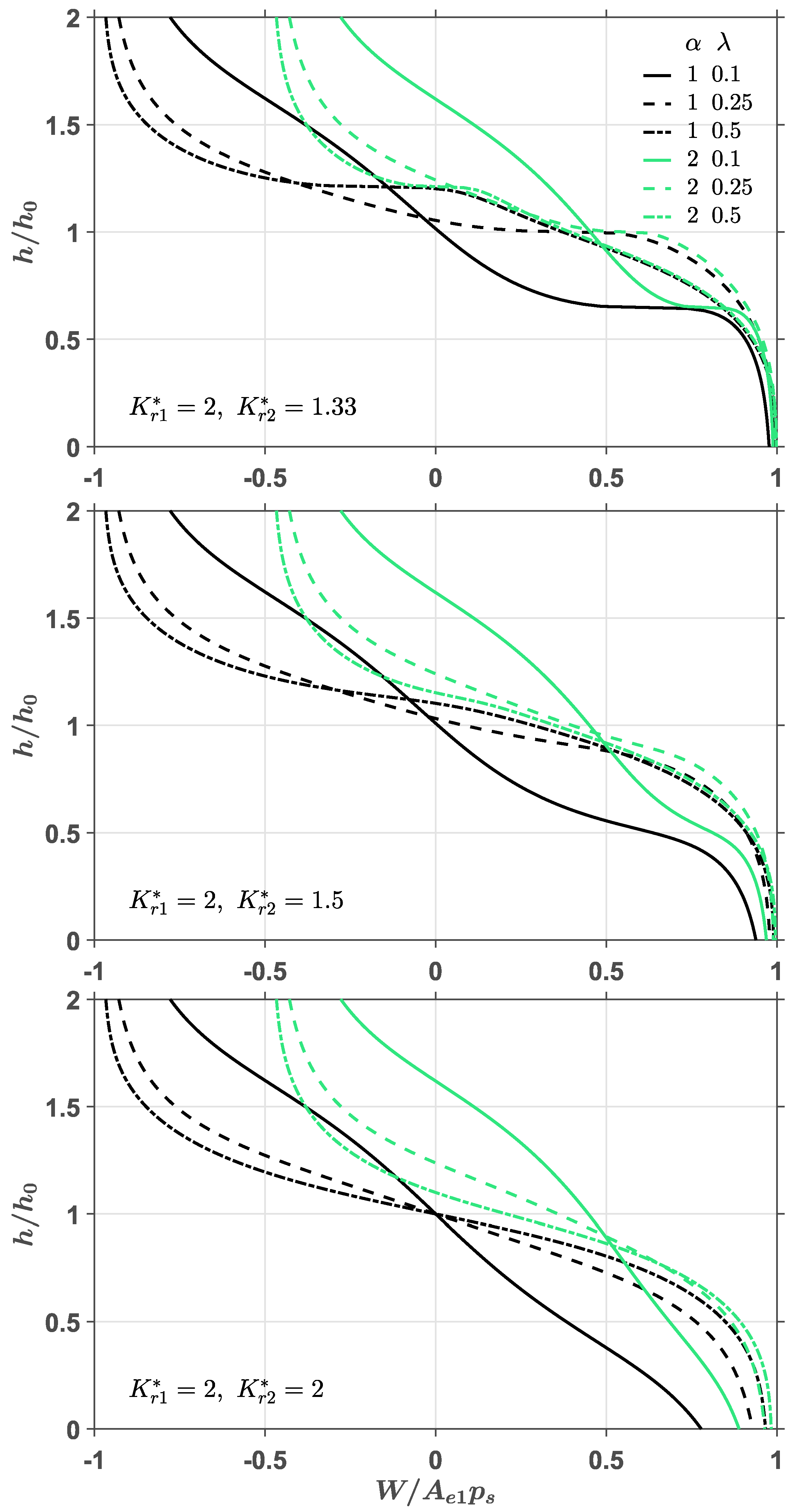
- A consistent, relatively good performance can be observed for a dimensionless membrane stiffness, , of 1.33 and a design restriction ratio, , of 0.25. In this case, the bearing clearance is maintained at an almost constant level over a wide range of the dimensionless loading. In addition, the system performance for the opposed-pad bearing is less sensitive to the change of membrane stiffness, compared with that for the single-pad case presented in Reference [14].
- Within a small eccentricity range, i.e., , the benefit of choosing the design restriction ratio is also significant. For the case of zero eccentricity, a good bearing stiffness can generally be obtained even if the dimensionless stiffness of the membrane is not the optimal value of 1.33.
- As shown in Figure 4, it is interesting to find that the displacement of the bearing is dominated by the value of when the dimensionless loading is positive and is small (e.g., ). This phenomenon is also revealed in Figure 5 and Figure 6. After carefully examining the variation of pressure in both pads, it is found that when the dimensionless loading is positive, the pressure in the upper pad, for all loading cases, is maintained at a high level, while the pressure in the lower pad is significantly changed as the loading is changed. This is because when a smaller design restriction ratio is adopted, the flow resistance of restrictor is smaller and hence a higher recess pressure p will appear, even when no load was applied (). The increase in loading will result in a decrease in bearing clearance ratio () and hence a lower resistance of the lower pad. However, the increase in upper pad pressure is limited since the upper pad pressure is already quite high when no load is applied. Therefore, the lower pad dominates the performance of the bearing. Similar phenomenon can be observed when the dimensionless loading is negative.
- From Figure 4, Figure 5 and Figure 6, for the case , it is found that when the dimensionless load is positive, the effects of on the clearance ratio of the bearing increases as a larger is adopted (e.g., ). When , the value of still dominates the performance of the system within the positive load range. However, the effects of appear. This is because when a larger design restriction ratio is adopted, the flow resistance of restrictor is larger, and hence a lower pressure on the upper pad will be present when no load is applied (). Increasing the loading will decrease the clearance ratio and, hence, leads to a higher resistance of the upper pad. Therefore, the increase in the upper pad pressure is larger since the upper pad pressure is lower than the previous case when no load is applied.
- A further increase in the design restriction ratio generally increases the dominance of in the positive load range. It becomes difficult to identify the optimal parameter combinations such that the system can have the best performance. The dimensionless membrane stiffness of 1.33 for both pads is no longer the optimal solution, when the applied load is small. A small load change makes a big clearance change; it is indicated that the bearing stiffness is quite low at this loading range. Instead, the dimensionless membrane stiffness of 2.00 for both pads can provide a more consistent bearing stiffness over a wide load range.
- Comparing the results with the effective area ratio (depicted as green lines), it is shown that similar trends and conclusions can be derived for both cases. The major difference is the loading range for negative loading is roughly half of that for positive loading. This is so because the effective area of the lower pad is only half of the effective area of the upper pad.
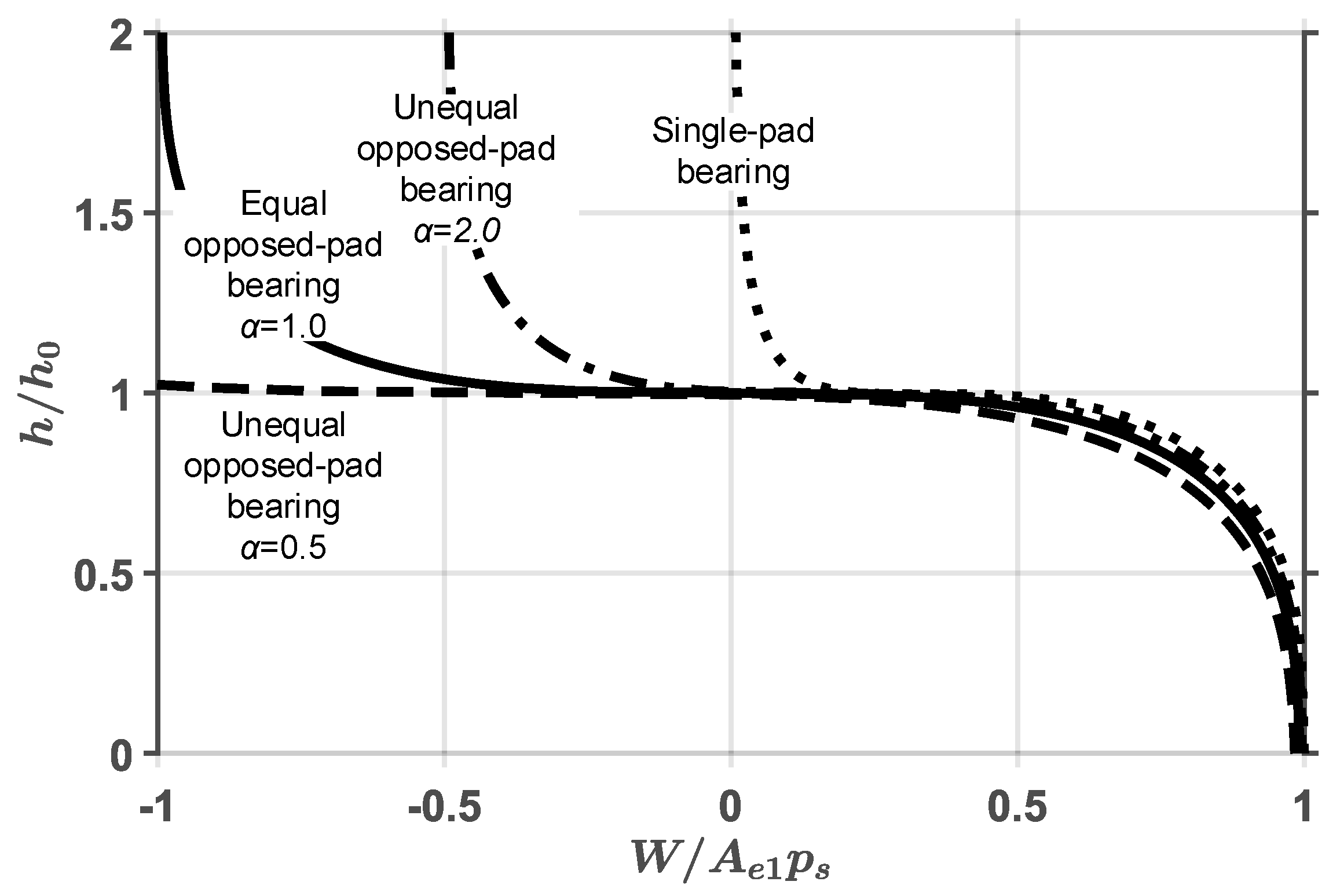
- As shown in Figure 7, the opposed-pad bearing can withstand two principal forces coming from two opposite directions, and the bearing will have great stiffness even when the bearing load approaches zero. However the single-pad bearing can withstand only a principal force in one direction, and the bearing stiffness will be dramatically degraded when the bearing load becomes small.
- Decreasing the effective area ratio , generally increases the applicable working range of the bearing, i.e., it makes the flat region of the curve wider, however the eccentricity at a high loading condition, , is increased. For the single-pad bearing, the flat region of the curve is between = 0.2∼0.5. For the unequal opposed-pad bearing (), the flat region of the curve is increased to 0∼0.5. For the equal opposed-pad bearing (), the flat region becomes much wider, and extends to −0.4∼0.4.
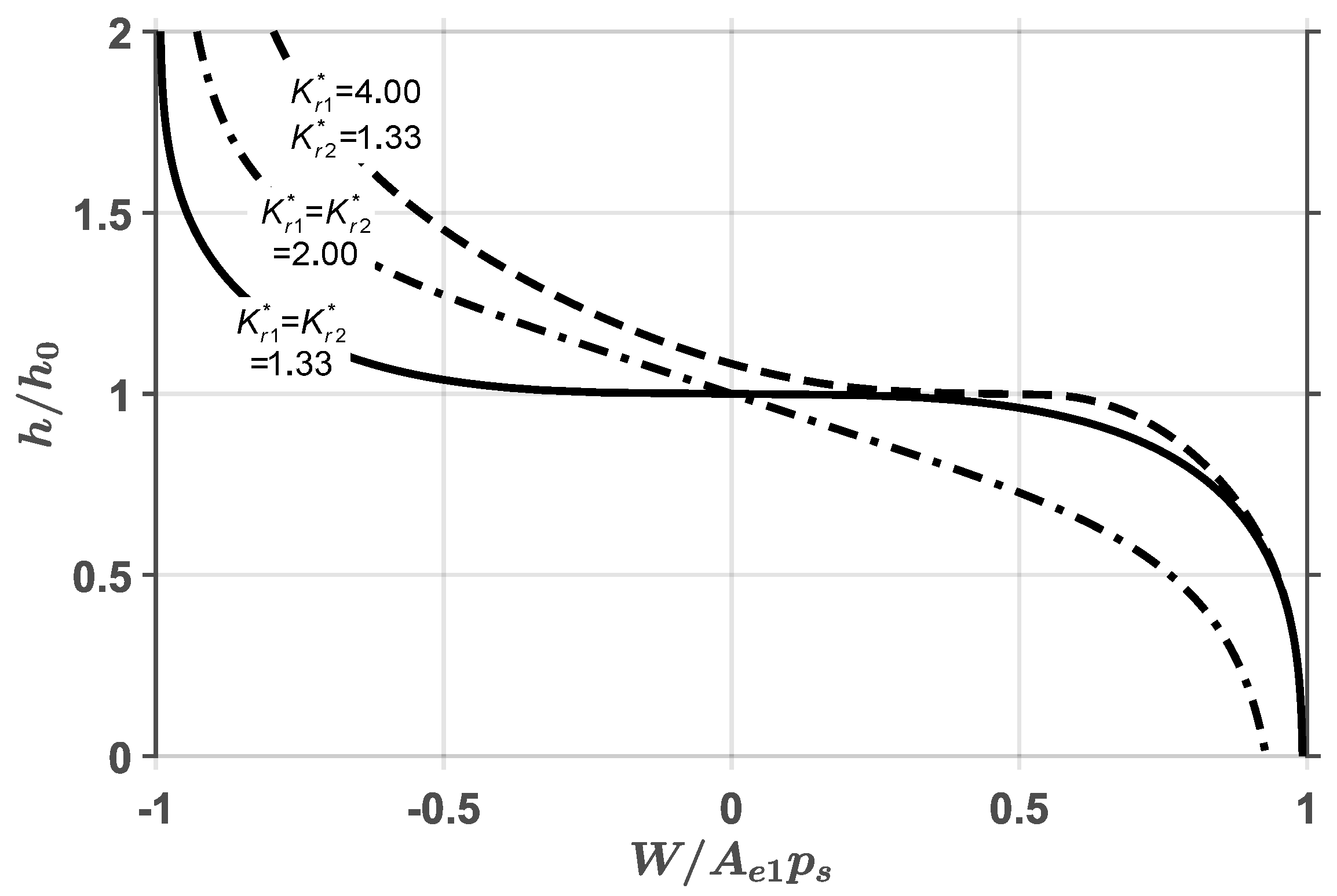
- Based on the examined results shown in Figure 4, Figure 5 and Figure 6, when the design parameters for both pads are given by and , the bearing performance is generally good. However, for some special loading demands, e.g., the bearing is designed to withstand high loads, different design parameters for each pad may help to fulfill the requirements. The chance for further improvements of the bearing performance for high loading demands is shown in Figure 8. From this figure, when the bearing load is high, it is observed that when different design parameters for each pad are given, higher bearing stiffness can be obtained. The calculation results show that the parameter combination of and has better bearing stiffness within the load range 0.3∼0.6.
4. Design Procedure
- Give the maximum positive load ()
- Give the maximum negative load ()
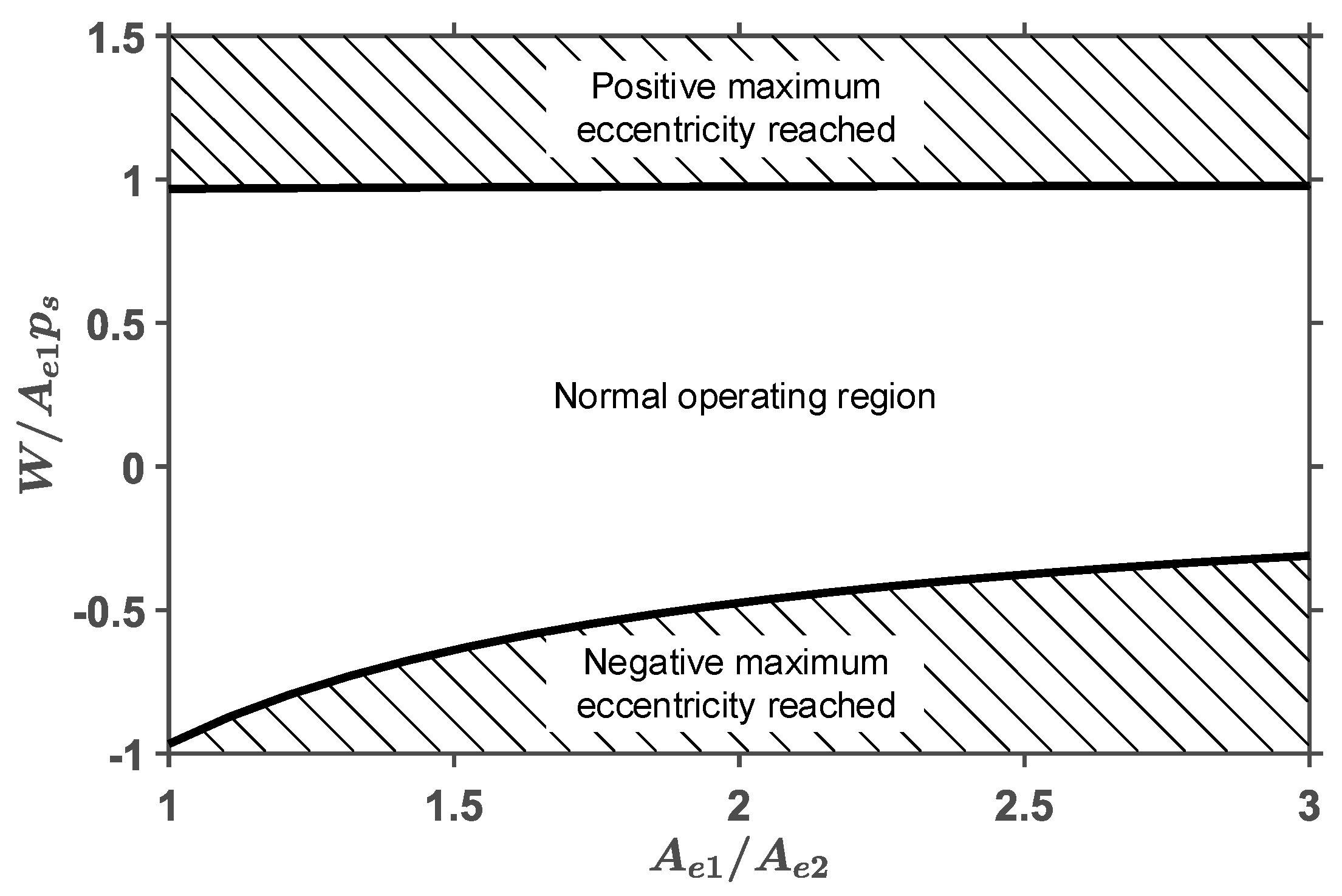
- Determine the area ratio ()Having given a maximum positive load and a maximum negative load , read an appropriate area ratio from Figure 10.
- Decide on a supply pressure ()For given a high supply pressure: a high bearing capacity, a high minimal bearing stiffness, and a small required bearing area will be obtained. However, a high pumping power follows.
- Determine the effective areas ( and )When the maximum load (or ), the supply pressure , and the area ratio are known, the effective areas of the bearing can be determined from Figure 9.
- Decide on a reference clearance ()When the bearing speed is very low, there is no evidence that the bearing performance can benefit by a large value of the reference clearance. Large pad clearance means high lubricant flow rate, therefore the pumping power is likely to be high. For the purpose of decreasing the power consumption, a small clearance is suggested. The small clearance should be consistent with capabilities and economics of manufacturing.
- Determine the minimal bearing stiffness ()With the area ratio , the minimal stiffness of the bearing under the designed operating work range can be determined from Figure 11.
- Check the minimal bearing stiffness ()If the minimal stiffness is not greater than the specified value, consider decreasing the reference clearance in step 6; the bearing stiffness is generally inversely proportional to the bearing clearance. Some issues, however, will follow when the reference clearance is set too small. Smaller clearance will require better surface finish, which necessitates longer manufacturing time and higher production cost. Even when the bearing works at a low rotating speed, making the clearance extremely small also leads to high viscous friction and high lubricant temperature. Gradually decreasing the reference clearance is therefore, not always a feasible option. Instead, one can consider increasing the supply pressure in step 4 to improve the minimal stiffness.
- Determine the dimensionless effective area ()The dimensionless effective area (a.k.a. bearing area, or area shape factor) is a loading-capacity factor related to the geometry of the bearing pad, such as its length-to-width ratio, fillet, and recess dimension, etc. Depending on applications, many different geometries have been introduced, and detailed calculations of can be found in many early reports [1,2,3]. For designing convenience, a dimensionless effective area will be given for both pads.
- Determine the minimal required projected areas ( and )When the effective areas and , and the dimensionless effective area are known, the minimal required projected areas can be determined. The term minimum indicates that a smaller size of the area should not be given.
- Determine the actual bearing areas ( and )The actual bearing area for the upper pad should be chosen as and conform to the size limits in engineering practices. Then the actual bearing area for the lower pad can be calculated with the area ratio such that . Generally, larger bearing area leads to better bearing load capacity, however, a higher power loss coming from the viscous friction will follow.
- Determine the bearing dimensions
- Determine the dimensionless flow resistance ()Similar to the dimensionless effective area , the dimensionless flow resistance () is a flow factor related to the geometry of the bearing pad. The dimensionless flow resistance can be calculated by the reported models from References [1,3]. Sometimes it will be presented in a inversely proportional form (); in terms of the flow shape factor [2].
- Decide on a radius ratio of the restricting plane ()
- Determine the assembling clearance of membrane ()
- Determine the minimal membrane stiffness ()The minimal requirement for the membrane stiffness is given to ensure the dimensionless membrane stiffness is larger than the optimal value of 1.33. The reason for this is to avoid an operating instability without compromising the bearing load capacity. Theoretically, a negative bearing stiffness appears when the dimensionless membrane stiffness is smaller than 1.33 [14].When the dimensionless membrane stiffness , the supply pressure , the effective area of the restricting plane , and the assembling clearance of the membrane are given, then the minimal membrane stiffness can be determined as:For maximizing the bearing stiffness at zero eccentricity, it is suggested that .
- Decide on the inner radius of the restricting plane ()
- Determine the outer radius of the restricting plane ()
- Determine the actual membrane stiffness ()The effective stiffness of a circular membrane plate (diaphragm) can generally be determined by the deformation of the membrane with an applied force. The deformation can be estimated by the equations for plates and shells [15]. When a plate material is chosen, the elasticity modulus and the Poisson’s ratio of the membrane plate is determined. With this information and the dimensions of the plate, including and , the membrane stiffness can be calculated. In engineering practice, making the membrane stiffness match the optimal one is trivial. In order to obtain a great bearing stiffness without compromising the bearing load capacity, a larger membrane stiffness, i.e., , should be given.
- Revise the supply pressure ()When the actual membrane stiffness is given, the actual supply pressure, needed for making the restrictor operate with good design features, should be modified to:
- Making the temperature rise of the lubricant small.
- Making the viscosity variations small. (Large viscosity variations will make pressure control difficult.)
- Making the thermal distortions of the bearing structure small.
- Making the time for temperature stabilization of the bearing structure short.
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Projected area of pad |
| Effective area of pad | |
| Effective area of upper pad (suffix “1” denotes upper pad) | |
| Effective area of lower pad (suffix “2” denotes lower pad) | |
| Dimensionless effective area of pad (=) | |
| Effective friction area of pad | |
| Effective area of restricting plane | |
| e | Eccentricity |
| h | Clearance |
| Reference clearance | |
| Stiffness of membrane | |
| Dimensionless stiffness of membrane (=) | |
| p | Recess pressure |
| Pressure ratio (=) | |
| Supply pressure | |
| Inner radius of the restricting plane | |
| Outer radius of the restricting plane | |
| Dimensionless flow resistance of pad (=) | |
| Flow resistance of pad at a reference configuration | |
| Flow resistance of restrictor | |
| Flow resistance of restrictor when no force is exerted on it (i.e., ) | |
| W | Bearing load |
| Positive maximum load | |
| Negative maximum load | |
| x | Deformation of membrane |
| Effective area ratio (=) | |
| Radius ratio of restricting plane (=) | |
| Eccentricity ratio (=) | |
| Design restriction ratio (=) | |
| Assembling clearance of membrane | |
| Deformation ratio of membrane (=) |
References
- Bassani, R.; Piccigallo, B. Hydrostatic Lubrication; Elsevier: Amsterdam, The Netherlands, 1992. [Google Scholar]
- Rowe, W.B. Hydrostatic, Aerostatic and Hybrid Bearing Design; Butterworth-Heinemann: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of Fluid Film Lubrication; Marcel Dekker: New York, NY, USA, 2004. [Google Scholar]
- Mohsin, M.E. The use of controlled restrictors for compensating hydrostatic bearings. In Advances in Machine Tool Design and Research, Proceedings of the 3rd International MDTR Conference, Birmingham, UK, September 1962; Pergamon Press: New York, NY, USA, 1962; pp. 429–442. [Google Scholar]
- DeGast, J.G.C. A new type of controlled restrictor (M.D.R.) for double film hydrostatic bearings and its application to high-precision machine tools. In Advances in Machine Tool Design and Research, Proceedings of the 7th International MDTR Conference, Birmingham, UK, September 1966; Pergamon Press: New York, NY, USA, 1966; pp. 273–298. [Google Scholar]
- Mohsin, M.E.; Morsi, S.A. The dynamic stiffness of controlled hydrostatic bearings. J. Lubr. Technol. 1969, 91, 597–608. [Google Scholar] [CrossRef]
- Rowe, W.B.; O’Donoghue, J.P. Diaphragm valves for controlling opposed pad bearings. Proc. Inst. Mech. Eng. 1969, 184, 1–9. [Google Scholar]
- Cusano, C. Characteristics of externally pressurized journal bearings with membrance-type variable-flow restrictors as compensating elements. Proc. Inst. Mech. Eng. 1974, 188, 527–536. [Google Scholar]
- Phalle, V.M.; Sharma, S.C.; Jain, S.C. Influence of wear on the performance of a 2-lobe multirecess hybrid journal bearing system compensated with membrane restrictor. Tribol. Int. 2011, 44, 380–395. [Google Scholar] [CrossRef]
- Liu, Z.; Pan, W.; Lu, C.; Zhang, Y. Numerical analysis on the static performance of a new piezoelectric membrane restrictor. Ind. Lubr. Tribol. 2016, 68, 521–529. [Google Scholar] [CrossRef]
- O’Donoghue, J.P.; Rowe, W.B. Hydrostatic bearing design. Tribology 1969, 2, 25–71. [Google Scholar] [CrossRef]
- O’Donoghue, J.P.; Rowe, W.B. Hydrostatic bearing design: Supplement for unequal opposed pads. Tribology 1969, 2, 225–232. [Google Scholar] [CrossRef]
- Rowe, W.B.; O’Donoghue, J.P.; Cameron, A. Optimization of externally pressurized bearings for minimum power and low temperature. Tribology 1970, 3, 153–157. [Google Scholar] [CrossRef]
- Lai, T.H.; Chang, T.Y.; Yang, Y.L.; Lin, S.C. Parameters design of a membrane-type restrictor with single-pad hydrostatic bearing to achieve high static stiffness. Tribol. Int. 2017, 107, 206–212. [Google Scholar] [CrossRef]
- Timoshenko, S.; Woinowsky Krieger, S. Theory of Plates and Shells; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
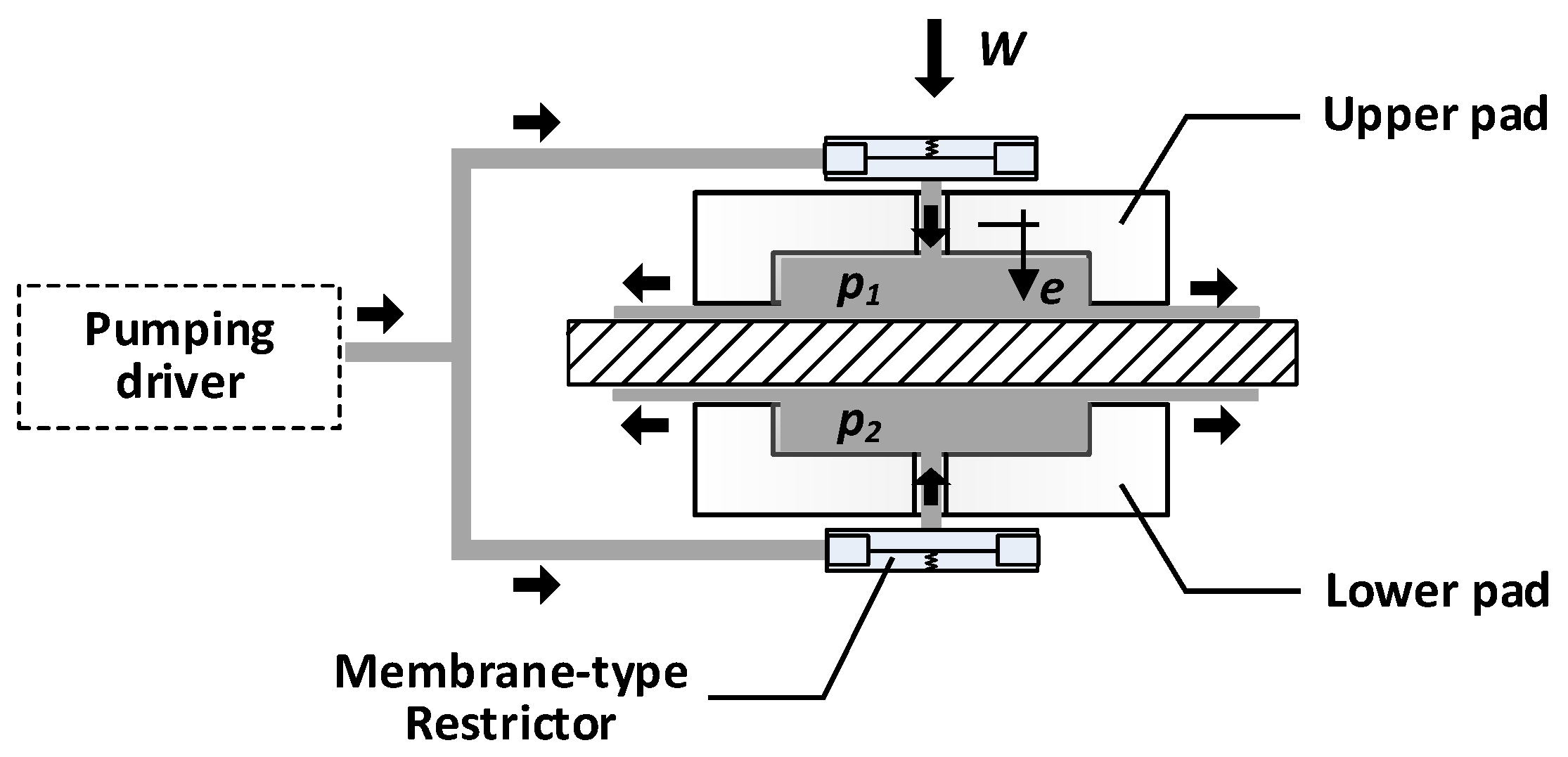
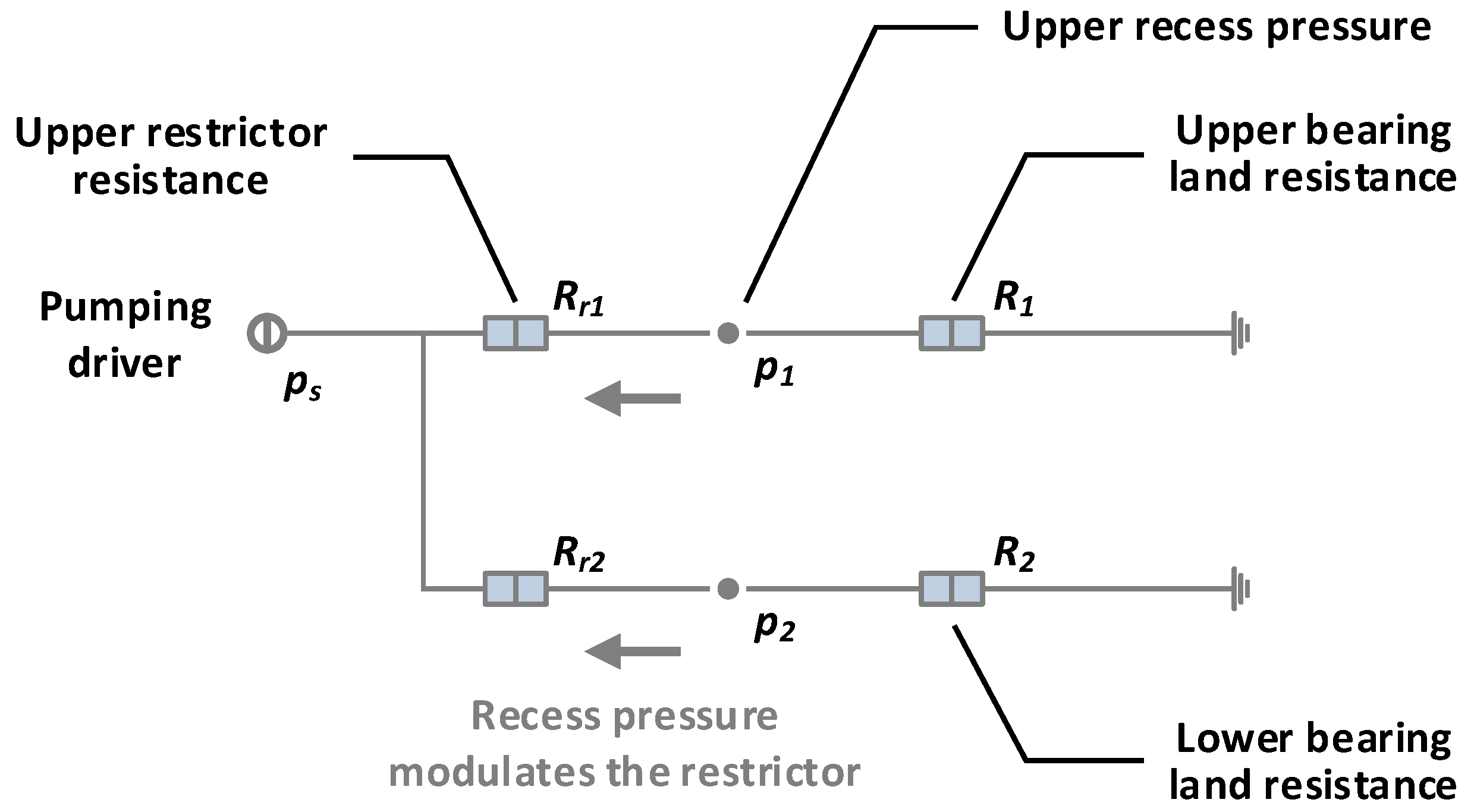



| Parameters | Levels |
|---|---|
| Effective area ratio () | 1.0, 2.0 |
| Dimensionless stiffness of upper membrane () | 1.33, 1.50, 2.00 |
| Dimensionless stiffness of lower membrane () | 1.33, 1.50, 2.00 |
| Design restriction ratio () | 0.1, 0.25, 0.5 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lai, T.-H.; Lin, S.-C. A Simulation Study for the Design of Membrane Restrictor in an Opposed-Pad Hydrostatic Bearing to Achieve High Static Stiffness. Lubricants 2018, 6, 71. https://doi.org/10.3390/lubricants6030071
Lai T-H, Lin S-C. A Simulation Study for the Design of Membrane Restrictor in an Opposed-Pad Hydrostatic Bearing to Achieve High Static Stiffness. Lubricants. 2018; 6(3):71. https://doi.org/10.3390/lubricants6030071
Chicago/Turabian StyleLai, Ta-Hua, and Shih-Chieh Lin. 2018. "A Simulation Study for the Design of Membrane Restrictor in an Opposed-Pad Hydrostatic Bearing to Achieve High Static Stiffness" Lubricants 6, no. 3: 71. https://doi.org/10.3390/lubricants6030071
APA StyleLai, T.-H., & Lin, S.-C. (2018). A Simulation Study for the Design of Membrane Restrictor in an Opposed-Pad Hydrostatic Bearing to Achieve High Static Stiffness. Lubricants, 6(3), 71. https://doi.org/10.3390/lubricants6030071