Study on the Influence of Inertial Force on the Performance of Aerostatic Thrust Bearings

Abstract

Firstly, the Reynolds equation considering gas inertia force is theoretically deduced in the cylindrical coordinate system, and then a mathematical model of aerostatic thrust bearing with three degrees of freedom (3-DOF) is constructed. Secondly, the Reynolds equation and velocity control equation are solved by the finite difference method (FDM), and the characteristics of gas pressure and velocity distribution in the gas film under steady-state conditions are revealed. On this basis, in the single-factor analysis, the bearing capacity error and recovery torque error caused by the inertia force term are quantitatively analyzed. It is found that the bearing rotating speed has a significant influence on the inertial force error, and the bearing radius also has a certain influence on the inertial force error, while the initial clearance, gas supply pressure, and torsion angle have relatively little influence on the inertial force error. Finally, in the multi-factor analysis, the sample regression equation of relative error of bearing capacity and relative error of restoring torque is established by using the multiple regression analysis method. By comparing the estimated values with the simulation results, the validity of the constructed regression equation is verified.

1. Introduction

Aerostatic bearing is widely used in various high-precision and high-speed equipment because of its advantages of high precision, high speed, low friction, and no pollution [1,2]. As the main component of ultra-precision machine tools, the performance of the aerostatic motorized spindle directly affects the surface quality and machining accuracy of the machined workpiece [3,4]. With the increasingly stringent requirements for the rotational speed and stability of the spindle in the product manufacturing process, the performance of the gas bearing also proposes higher requirements. Determining how to calculate the static and dynamic characteristics of gas bearing more accurately and establish a mathematical model more in line with the real working conditions is very important for the performance evaluation and structural design of the aerostatic motorized spindle [5]. In the previous numerical calculation of aerostatic bearing, gas inertia force, as a small factor affecting the performance of aerostatic bearing, is often ignored [6,7]. Under specific structural parameters and application conditions, ignoring the influence of inertia force may cause a large calculation error.

Relevant scholars have carried out some research on the influence of inertial force on the performance of gas bearings. Among them, Brand [8] concluded through dimensional analysis that when the corrected Reynolds number approaches or exceeds 1, the inertial force will have an important influence on aerostatic radial bearings. However, the research results presented by Gargiulo [9] indicated that even if the corrected Reynolds number reaches 1, the inertia correction coefficient of pressure distribution remains less than 0.3%, suggesting that the inertia force is still not a significant factor for gas bearings. Launder et al. [10,11] proposed two numerical calculation methods, which were, respectively, employed to calculate the laminar and turbulent inertial flow problems within the film of a finite-width thrust bearing. By comparing their findings with the results of various theories, the validity of the proposed methods was substantiated, and the significance of incorporating fluid inertial effects into lubrication analysis was underscored. Mori et al. [12,13] deduced the fluid control equation in the cylindrical coordinate system considering the gas extrusion effect and inertia force and studied the influence of inertia force on the gas velocity and pressure distribution characteristics in the solution domain of aerostatic thrust bearing. The results showed that the velocity distribution curve in the gas film changed from parabolic to elliptic function after considering the inertia force, which made the gas film pressure distribution change to some extent, but the bearing performance changed little. Hashimoto and Wada [14,15] studied the influence of turbulence and inertia force on the thrust bearing performance, and the results showed that under certain conditions, inertia force had a significant impact on the static characteristics of the bearing, and the theoretical calculation was verified by experimental results. Stolarski et al. [16] derived the Reynolds equation with the inertia term and used the concept of average inertia to deal with the inertia force in the thickness direction of the gas film, pointing out that the modified Reynolds equation was discretized by the control volume method, and at the same time, the convergence result of the gas film could be easily obtained by combining the Newton–Raphson iteration method. Yang [17] took the plane gas thrust bearing with spiral groove as the research object, solved the Reynolds equation with gas inertia force by finite element method, and studied the influence of bearing structure parameters on its bearing performance. Shen et al. [18] studied the influence of gas inertia effect and end face inclination on the steady and dynamic characteristics of ultra-high-speed spiral groove end face seal. The results showed that under ultra-high-speed conditions, the leakage rate of dry gas seal was significantly reduced and the rigid-leakage ratio was significantly increased, while the opening force, gas film stiffness, and dynamic characteristic coefficient changed little.

To sum up, although the existing literature includes research on the effect of inertia force on the performance of gas bearings, most of the work still stays in qualitative analysis, and even the results obtained in some research are contradictory, such as [8,9]. For different structural parameters, working parameters, and gas parameters of bearings, the quantitative analysis of the influence of inertia force on the performance of bearings is still quite lacking. In addition, some scholars [19,20] simplified the theoretical model in the previous research, ignoring the rotational freedom of the bearing, which made the model different from the real working state of the gas bearing. Therefore, aiming at the shortcomings of previous research, this paper takes aerostatic thrust bearing as the research object and obtains the Reynolds equation considering gas inertia force in the cylindrical coordinate system through theoretical derivation. At the same time, a 3-DOF gas film model of thrust bearing is established by fully considering the influence of bearing rotation freedom. The Reynolds equation and velocity control equation are solved by the FDM, and the pressure and velocity distribution of gas in the gas film considering the gas inertia force term are obtained. In addition, the influence of bearing initial clearance, radius, rotating speed, gas supply pressure, and torsion angle on bearing capacity error and recovery torque error caused by inertia force is quantitatively analyzed. On this basis, the sample regression equation of bearing capacity relative error and recovery torque relative error under the influence of multiple factors is obtained by using the method of multiple regression analysis, and the effectiveness of the equation is verified.

2. Mathematical Modeling

The structural schematic diagram of the aerostatic thrust bearing studied in this paper is shown in Figure 1. From the figure, it can be seen that the solution domain of the thrust bearing is circular, so the pressure and velocity distribution of gas in the gas film can be obtained by solving the fluid control equation in the cylindrical coordinate system.

2.1. Velocity Control Equation

According to the related literature [14], in the cylindrical coordinate system, the equation of motion considering the inertial force can be expressed as

$$\left\{\begin{array}{l}\rho \left({\displaystyle \frac{𝜕v_{\mathrm{r}}}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\mathrm{r}}}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_r}{r𝜕\theta }}+w{\displaystyle \frac{𝜕v_r}{𝜕z}}-{\displaystyle \frac{v_{\theta }^2}{r}}\right)+{\displaystyle \frac{𝜕p}{𝜕r}}={\displaystyle \frac{𝜕}{𝜕z}}\left(\eta {\displaystyle \frac{𝜕v_r}{𝜕z}}\right)\\ \rho \left({\displaystyle \frac{𝜕v_{\theta }}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\theta }}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_{\theta }}{r𝜕\theta }}+w{\displaystyle \frac{𝜕v_{\theta }}{𝜕z}}+{\displaystyle \frac{v_r{v}_{\theta }}{r}}\right)+{\displaystyle \frac{𝜕p}{r𝜕\theta }}={\displaystyle \frac{𝜕}{𝜕z}}\left(\eta {\displaystyle \frac{𝜕v_{\theta }}{𝜕z}}\right)\\ {\displaystyle \frac{𝜕p}{𝜕z}}=0\end{array}\right.$$

(1)

By integrating z on both sides of the first equation and the second equation of Equation (1), Equation (2) can be obtained:

$$\left\{\begin{array}{l}{\displaystyle \frac{\rho }{2\eta }}\left({\displaystyle \frac{𝜕v_r}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_r}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_r}{r𝜕\theta }}-{\displaystyle \frac{v_{\theta }^2}{r}}\right)z^2+{\displaystyle \frac{\rho }{\eta }}w{v}_{r}z+{\displaystyle \frac{1}{2\eta }}{\displaystyle \frac{𝜕p}{𝜕r}}{z}^2+c_{1}z+c_2=v_r\\ {\displaystyle \frac{\rho }{2\eta }}\left({\displaystyle \frac{𝜕v_{\theta }}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\theta }}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_{\theta }}{r𝜕\theta }}+{\displaystyle \frac{v_r{v}_{\theta }}{r}}\right)z^2+{\displaystyle \frac{\rho }{\eta }}w{v}_{\theta }z+{\displaystyle \frac{1}{2\eta }}{\displaystyle \frac{𝜕p}{r𝜕\theta }}{z}^2+c_{1}z+c_2=v_{\theta }\end{array}\right.$$

(2)

We introduce velocity boundary conditions:

$$z=0,v_r=v_{r1},v_{\theta }=v_{\theta 1};z=h,v_r=v_{r2},v_{\theta }=v_{\theta 2}$$

(3)

And bring it into Equation (2) to obtain the velocity control equation of gas in the gas film:

$$\left\{\begin{array}{l}{v}_r=-{\displaystyle \frac{\rho }{2\eta }}\left({\displaystyle \frac{𝜕v_r}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_r}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_r}{r𝜕\theta }}-{\displaystyle \frac{v_{\theta }^2}{r}}\right)z\left(h-z\right)-{\displaystyle \frac{1}{2\eta }}{\displaystyle \frac{𝜕p}{𝜕r}}z\left(h-z\right)+{\displaystyle \frac{v_{r2}-v_{r1}}{h}}z+v_{r1}\\ v_{\theta }=-{\displaystyle \frac{\rho }{2\eta }}\left({\displaystyle \frac{𝜕v_{\theta }}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\theta }}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_{\theta }}{r𝜕\theta }}+{\displaystyle \frac{v_r{v}_{\theta }}{r}}\right)z\left(h-z\right)-{\displaystyle \frac{1}{2\eta }}{\displaystyle \frac{𝜕p}{r𝜕\theta }}z\left(h-z\right)+{\displaystyle \frac{v_{\theta 2}-v_{\theta 1}}{h}}z+v_{\theta 1}\end{array}\right.$$

(4)

Then, the average velocity equation of gas in the gas film can be expressed as

$$\left\{\begin{array}{l}{v}_{ra}={\displaystyle {\int }_0^h{v}_{r}dz}/h=-{\displaystyle \frac{\rho }{12\eta }}{h}^2\left({\displaystyle \frac{𝜕v_r}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_r}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_r}{r𝜕\theta }}-{\displaystyle \frac{v_{\theta }^2}{r}}\right)-{\displaystyle \frac{1}{12\eta }}{h}^2{\displaystyle \frac{𝜕p}{𝜕r}}+{\displaystyle \frac{v_{r2}+v_{r1}}{2}}\\ v_{\theta a}={\displaystyle {\int }_0^h{v}_{\theta }dz/h}=-{\displaystyle \frac{\rho }{12\eta }}{h}^2\left({\displaystyle \frac{𝜕v_{\theta }}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\theta }}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_{\theta }}{r𝜕\theta }}+{\displaystyle \frac{v_r{v}_{\theta }}{r}}\right)-{\displaystyle \frac{1}{12\eta }}{h}^2{\displaystyle \frac{𝜕p}{r𝜕\theta }}+{\displaystyle \frac{v_{\theta 2}+v_{\theta 1}}{2}}\end{array}\right.$$

(5)

We introduce dimensionless parameters:

$$r=lR,\mathrm{z}=lZ,p=p_{0}P,h=h_{m}H,v_r=v_m{V}_r,v_{\theta }=v_m{V}_{\theta },t=l/v_{m}T$$

(6)

Substituting Equation (6) into Equation (4) and Equation (5), respectively, the dimensionless velocity equation and dimensionless average velocity equation can be obtained, as shown in Equations (7) and (8):

$$\left\{\begin{array}{l}{V}_r=-{\displaystyle \frac{{\rho }_a{p}_0{h}_m^2}{2\eta p_a}}{\displaystyle \frac{v_m}{l}}P\left({\displaystyle \frac{𝜕V_r}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_r}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_r}{R𝜕\theta }}-{\displaystyle \frac{V_{\theta }^2}{R}}\right)Z\left(H-Z\right)-{\displaystyle \frac{p_0{h}_m^2}{2\eta l{v}_m}}{\displaystyle \frac{𝜕P}{𝜕R}}Z\left(H-Z\right)+{\displaystyle \frac{V_{r2}-V_{r1}}{H}}Z+V_{r1}\\ V_{\theta }=-{\displaystyle \frac{{\rho }_a{p}_0{h}_m^2}{2\eta p_a}}{\displaystyle \frac{v_m}{l}}P\left({\displaystyle \frac{𝜕V_{\theta }}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_{\theta }}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_{\theta }}{R𝜕\theta }}+{\displaystyle \frac{V_r{V}_{\theta }}{R}}\right)Z\left(H-Z\right)-{\displaystyle \frac{p_0{h}_m^2}{2\eta l{v}_m}}{\displaystyle \frac{𝜕P}{R𝜕\theta }}Z\left(H-Z\right)+{\displaystyle \frac{V_{\theta 2}-V_{\theta 1}}{H}}Z+V_{\theta 1}\end{array}\right.$$

(7)

$$\left\{\begin{array}{l}{V}_{ra}=-{\displaystyle \frac{{\rho }_a{p}_0{h}_m^2}{12\eta p_a}}{\displaystyle \frac{v_m}{l}}P{H}^2\left({\displaystyle \frac{𝜕V_r}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_r}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_r}{R𝜕\theta }}-{\displaystyle \frac{V_{\theta }^2}{R}}\right)-{\displaystyle \frac{p_0{h}_m^2}{12\eta l{v}_m}}{H}^2{\displaystyle \frac{𝜕P}{𝜕R}}\\ V_{\theta a}=-{\displaystyle \frac{{\rho }_a{p}_0{h}_m^2}{12\eta p_a}}{\displaystyle \frac{v_m}{l}}P{H}^2\left({\displaystyle \frac{𝜕V_{\theta }}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_{\theta }}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_{\theta }}{R𝜕\theta }}+{\displaystyle \frac{V_r{V}_{\theta }}{R}}\right)-{\displaystyle \frac{p_0{h}_m^2}{12\eta l{v}_m}}{H}^2{\displaystyle \frac{𝜕P}{R𝜕\theta }}+{\displaystyle \frac{V_{\theta 1}}{2}}\end{array}\right.$$

(8)

2.2. Reynolds Equation

In the cylindrical coordinate system, the continuous equation of gas in the gas film can be expressed as

$${\displaystyle \frac{𝜕\left(\rho h\right)}{𝜕t}}+{\displaystyle \frac{1}{r}}{\displaystyle \frac{𝜕}{𝜕r}}\left(\rho r{\displaystyle {\int }_0^h{v}_{r}dz}\right)+{\displaystyle \frac{1}{r}}{\displaystyle \frac{𝜕}{𝜕\theta }}\left(\rho {\displaystyle {\int }_0^h{v}_{\theta }dz}\right)-\rho w{\delta }_i=0$$

(9)

where δ_i is the Kronecker function, which is 1 at the orifice nodes and 0 at the other nodes. By bringing Equation (4) into Equation (9), the general Reynolds equation in the cylindrical coordinate system can be obtained:

$$\begin{array}{l}{\displaystyle \frac{𝜕}{r𝜕r}}\left(r{h}^3{\displaystyle \frac{𝜕p^2}{𝜕r}}\right)+{\displaystyle \frac{𝜕}{r^2𝜕\theta }}\left(h^3{\displaystyle \frac{𝜕p^2}{𝜕\theta }}\right)+{\displaystyle \frac{24\eta p_a}{{\rho }_a}}\rho w{\delta }_i=-2{\displaystyle \frac{{\rho }_a}{p_a}}{\displaystyle \frac{𝜕}{r𝜕r}}\left(p^{2}r{h}^3\left({\displaystyle \frac{𝜕v_r}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_r}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_r}{r𝜕\theta }}-{\displaystyle \frac{v_{\theta }^2}{r}}\right)\right)\\ -2{\displaystyle \frac{{\rho }_a}{p_a}}{\displaystyle \frac{𝜕}{r𝜕\theta }}\left(p^2{h}^3\left({\displaystyle \frac{𝜕v_{\theta }}{𝜕t}}+v_r{\displaystyle \frac{𝜕v_{\theta }}{𝜕r}}+v_{\theta }{\displaystyle \frac{𝜕v_{\theta }}{r𝜕\theta }}+{\displaystyle \frac{v_r{v}_{\theta }}{r}}\right)\right)+24\eta {\displaystyle \frac{𝜕}{𝜕t}}\left(ph\right)+12\eta \left(v_{r2}+v_{r1}\right){\displaystyle \frac{𝜕}{r𝜕r}}\left(rph\right)+12\eta \left(v_{\theta 2}+v_{\theta 1}\right){\displaystyle \frac{𝜕}{r𝜕\theta }}\left(ph\right)\end{array}$$

(10)

Meanwhile, dimensionless Equation (6) is brought into Equation (10) to obtain the dimensionless Reynolds Equation (11):

$$\begin{array}{l}{\displaystyle \frac{𝜕}{R𝜕R}}\left(R{H}^3{\displaystyle \frac{𝜕P^2}{𝜕R}}\right)+{\displaystyle \frac{𝜕}{R^2𝜕\theta }}\left(H^3{\displaystyle \frac{𝜕P^2}{𝜕\theta }}\right)+Q{\delta }_i=-\sigma {\displaystyle \frac{𝜕}{R𝜕R}}\left(P^{2}R{H}^3\left({\displaystyle \frac{𝜕V_r}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_r}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_r}{R𝜕\theta }}-{\displaystyle \frac{V_{\theta }^2}{R}}\right)\right)\\ -\sigma {\displaystyle \frac{𝜕}{R𝜕\theta }}\left(P^2{H}^3\left({\displaystyle \frac{𝜕V_{\theta }}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_{\theta }}{𝜕R}}+V_{\theta }{\displaystyle \frac{𝜕V_{\theta }}{R𝜕\theta }}+{\displaystyle \frac{V_r{V}_{\theta }}{R}}\right)\right)+2\Lambda {\displaystyle \frac{𝜕}{𝜕T}}\left(PH\right)+\Lambda \left(V_{r2}+V_{r1}\right){\displaystyle \frac{𝜕}{R𝜕R}}\left(RPH\right)+\Lambda \left(V_{\theta 2}+V_{\theta 1}\right){\displaystyle \frac{𝜕}{R𝜕\theta }}\left(PH\right)\end{array}$$

(11)

where the expressions of Q, Λ, and σ are

$$Q={\displaystyle \frac{24\eta p_a\dot{m}}{{\rho }_a{p}_0^2{h}_m^{3}Rd\theta dR}},\Lambda ={\displaystyle \frac{12\eta v_{m}l}{p_0{h}_m^2}},\sigma ={\displaystyle \frac{2{\rho }_a{v}_m^2}{p_a}}$$

(12)

To facilitate the solution, the Reynolds equation in the cylindrical coordinate system is transformed by conformal transformation, so that the left end of the equation is transformed into the same form as that in the rectangular coordinate system. The conformal transformation equation is

$$\xi =\ln (R)$$

(13)

By substituting Equation (13) into Equation (11), the dimensionless Reynolds equation after coordinate transformation can be obtained, as shown in Equation (14):

$$\begin{array}{l}{\displaystyle \frac{𝜕}{𝜕\xi }}\left(H^3{\displaystyle \frac{𝜕P^2}{𝜕\xi }}\right)+{\displaystyle \frac{𝜕}{𝜕\theta }}\left(H^3{\displaystyle \frac{𝜕P^2}{𝜕\theta }}\right)+R^{2}Q{\delta }_i=-\sigma {\displaystyle \frac{𝜕}{𝜕\xi }}\left(P^{2}R{H}^3\left({\displaystyle \frac{𝜕V_r}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_r}{R𝜕\xi }}+V_{\theta }{\displaystyle \frac{𝜕V_r}{R𝜕\theta }}-{\displaystyle \frac{V_{\theta }^2}{R}}\right)\right)\\ -\sigma R{\displaystyle \frac{𝜕}{𝜕\theta }}\left(P^2{H}^3\left({\displaystyle \frac{𝜕V_{\theta }}{𝜕T}}+V_r{\displaystyle \frac{𝜕V_{\theta }}{R𝜕\xi }}+V_{\theta }{\displaystyle \frac{𝜕V_{\theta }}{R𝜕\theta }}+{\displaystyle \frac{V_r{V}_{\theta }}{R}}\right)\right)+2\Lambda R^2{\displaystyle \frac{𝜕}{𝜕T}}\left(PH\right)+\Lambda V_{\theta 1}R{\displaystyle \frac{𝜕}{𝜕\theta }}\left(PH\right)\end{array}$$

(14)

According to the method of the average inertia term mentioned in [14,16], the velocity term in Equation (14) is calculated by the average velocity in this paper.

2.3. Flow Control Equation

According to [21], the mass flow rate of gas entering the gas film from a single orifice can be expressed as follows:

$$\mathrm{m}=A{p}_s\Phi \sqrt{{\displaystyle \frac{2{\rho }_a}{p_a}}}\psi$$

(15)

$$\psi =\left\{\begin{array}{c}{\left[{\displaystyle \frac{k}{k-1}}\left({\beta }^{2/k}-{\beta }^{(k+1)/k}\right)\right]}^{1/2}\beta ={\displaystyle \frac{p_d}{p_s}}>{\beta }_k\\ {\left[{\displaystyle \frac{k}{2}}{\left({\displaystyle \frac{2}{k+1}}\right)}^{(k+1)/(k-1)}\right]}^{1/2}\beta ={\displaystyle \frac{p_d}{p_s}}⩽{\beta }_k\\ {\beta }_k={\left({\displaystyle \frac{2}{k+1}}\right)}^{k/(k-1)}\end{array}\right.$$

(16)

where Φ is the mass flow coefficient, which is usually taken as 0.8.

In the process of using the aerostatic motorized spindle, when the spindle is subjected to external force, it will cause its translation and rotation in space, which will lead to a certain degree of inclination of the thrust collar in three-dimensional space.

Figure 2 shows the space attitude diagram of the thrust collar in an inclined state. In the frame of the coordinate system established in Figure 2, the gas film thickness of the thrust bearing can be expressed as

$$h=h_m+r(-{\phi }_x\sin \theta +{\phi }_y\cos \theta )$$

(17)

Its dimensionless form can be expressed as

$$H=1+r(-{\phi }_x\sin \theta +{\phi }_y\cos \theta )/h_m$$

(18)

By solving Equation (8) and Equation (14) jointly, the pressure value of each node in the solution domain can be obtained, and by integrating the pressure, the axial bearing capacity F_z, recovery torque M_x around the x-axis, and recovery torque M_y around the y-axis of thrust bearing can be obtained. The expressions are as follows:

$$\left\{\begin{array}{l}{F}_z=p_0{l}^2{\displaystyle \underset{R,\theta }{\iint }(P-p_a/p_0)RdRd\theta }\\ M_x=p_0{l}^3{\displaystyle \underset{R,\theta }{\iint }-(P-p_a/p_0)R^2\sin \theta dRd\theta }\\ M_y=p_0{l}^3{\displaystyle \underset{R,\theta }{\iint }(P-p_a/p_0)R^2\cos \theta dRd\theta }\end{array}\right.$$

(19)

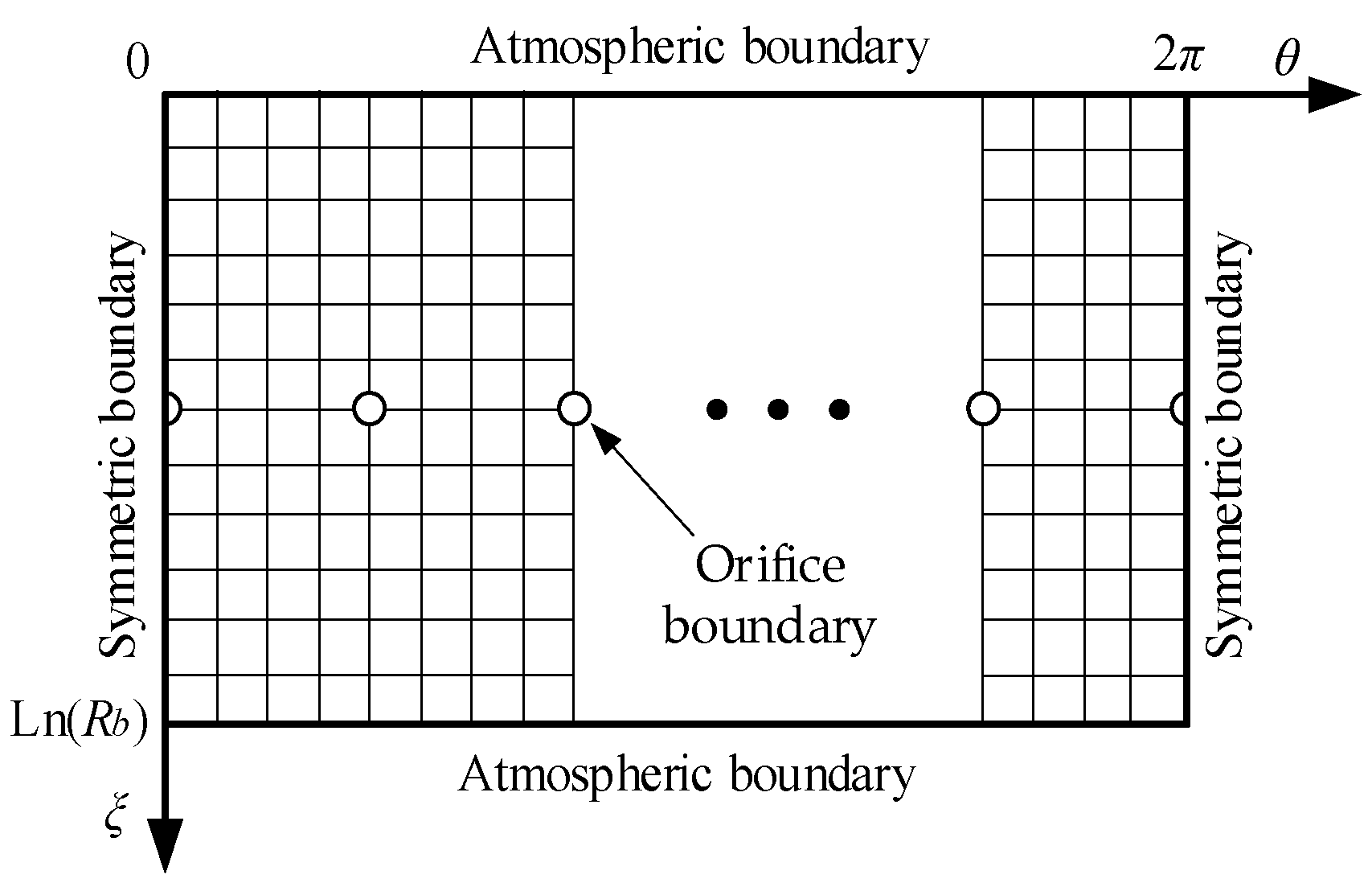
3. Numerical Calculation and Verification

3.1. Numerical Calculation

The solution domain of aerostatic thrust bearing studied in this paper is circular. After the conformal transformation of coordinates by Equation (13), the solution domain of the thrust bearing is transformed into a rectangular region, as shown in Figure 3. Figure 3 contains three boundary types, namely, atmospheric boundary, orifice boundary, and symmetrical boundary. The boundary conditions on different boundaries are as follows:

$$\left\{\begin{array}{l}P=P_a\left(\mathrm{at}\mathrm{the}\mathrm{atmospheric}\mathrm{boundary}\right)\\ P=P_d\left(\mathrm{at}\mathrm{the}\mathrm{orifice}\mathrm{boundary}\right)\\ {\displaystyle \frac{𝜕P}{𝜕\theta }}=0\left(\mathrm{at}\mathrm{the}\mathrm{symmetrical}\mathrm{boundary}\right)\end{array}\right.$$

(20)

Using the central difference scheme in the finite difference method to discretize Equations (8) and (14), their difference equations can be obtained, respectively, and the velocity and pressure of each node in the solution domain can be obtained by solving the difference equations through the successive overrelaxation method. Figure 4 shows the specific solution flow.

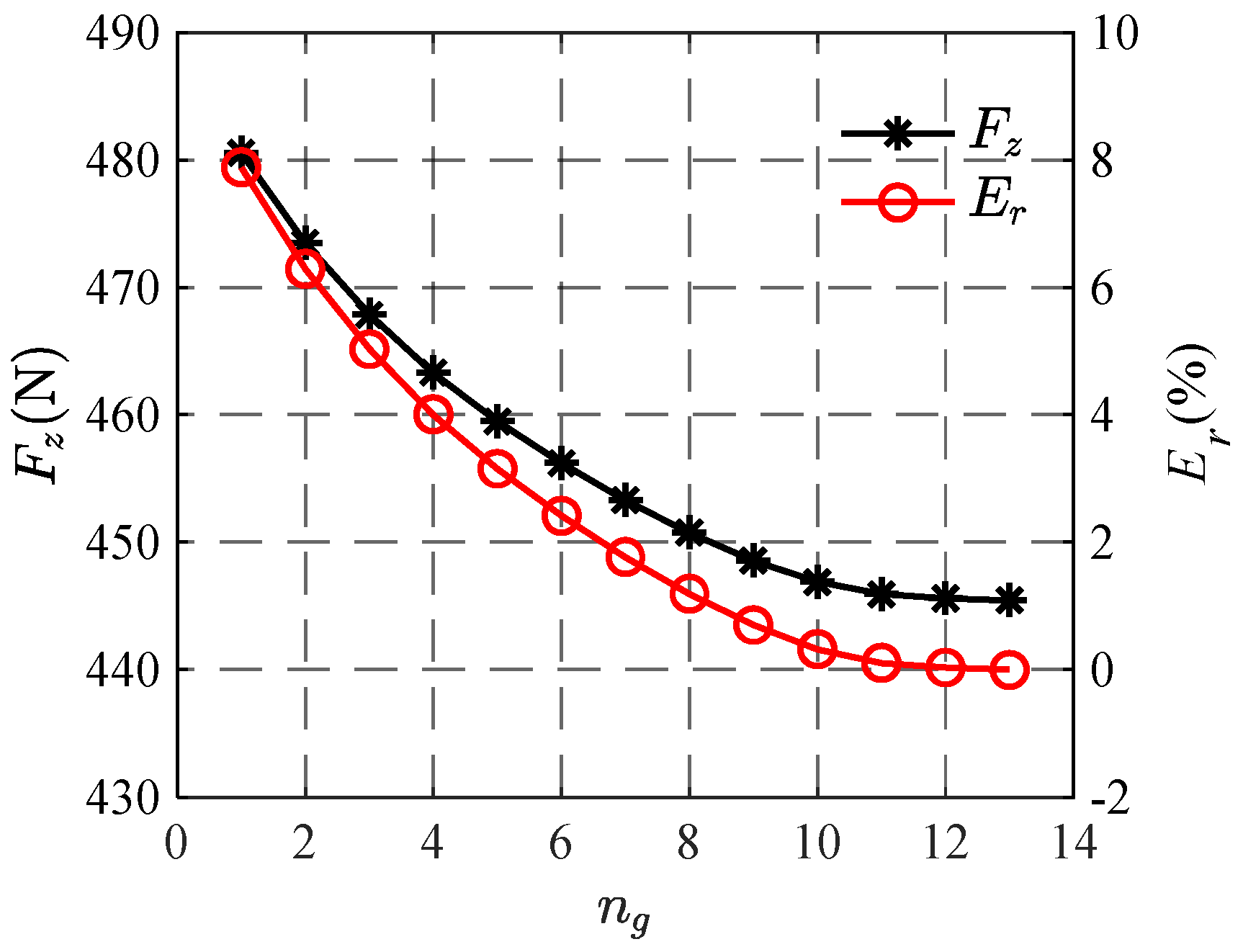
3.2. Grid Independence Verification

In the process of numerical calculation, the number of grids is very important for the accuracy of the solution. Too few grids will lead to too much deviation between the simulation results and the exact solution, which cannot meet the requirements of the solution accuracy, while too many grids will lead to a too-long calculation time and reduce the solution efficiency. Therefore, it is necessary to verify the independence of grids to determine the necessary number of grids in the solution process. The main structural parameters and working parameters of the aerostatic thrust bearing studied in this paper are shown in

Table 1. Parameters of aerostatic thrust bearing.

Parameter	Value
Internal radius (ra)	20 mm
External radius (rb)	45 mm
Distribution circle radius (rc)	30 mm
Initial clearance (hm)	20 μm
Orifice diameter (d)	0.2 mm
Orifice number (n)	16
Ambient pressure (pa)	0.1 Mpa
Gas supply pressure (ps)	0.5 Mpa
Gas viscosity (η)	1.82 × 10−5 Ns/m2
Ambient gas density (ρa)	1.204 kg/m3
Ratio of specific heat (k)	1.4
Rotating speed (N)	5 × 104 rpm

.

When φ_x and φ_y are both 0, the bearing capacity value obtained by numerical calculation is used as the judgment basis to verify the irrelevance of the grid in the solution domain. The initial grid number n_θ × n_r is set to 80 × 15, and the grid refinement factor n_g is the encryption multiple of the grid in a single direction. By densifying the grids in the solution domain, the bearing capacity obtained by numerical calculation gradually converges, as shown in Figure 5. Based on the final convergence result, when the relative error of bearing capacity E_r reaches the preset error threshold of 2%, it is considered that the number of grids can meet the requirements of solution accuracy. According to Figure 5, it can be seen that when the grid refinement factor increases to 7, the relative error of bearing capacity is less than the established error threshold. Therefore, the final determined n_θ × n_r is 560 × 105.

3.3. Contrast Verification

To verify the correctness of the above solution process, the following is compared with the simulation results in the existing published literature. The simulation results obtained by the solution program used in this paper are compared with those obtained by Shi et al. in the published literature [22]. The structural parameters and working parameters of the bearing are shown in

Table 2. Bearing parameters in reference [22].

Parameter	Value
Internal radius (ra)	10 mm
External radius (rb)	40 mm
Distribution circle radius (rc)	25 mm
Initial clearance (hm)	18.67 μm
Orifice diameter (d)	0.2 mm
Orifice number (n)	6
Ambient pressure (pa)	0.1 Mpa
Gas supply pressure (ps)	0.6 Mpa
Gas viscosity (η)	1.82 × 10−5 Ns/m2
Ambient gas density (ρa)	1.204 kg/m3
Ratio of specific heat (k)	1.4
Rotating speed (N)	0

, and the comparison of the results is shown in Figure 6. When comparing Figure 6a,b, it can be seen that the pressure distribution curve obtained by the simulation program in this paper is consistent with the pressure distribution curve obtained in [22], and the numerical value is basically the same. Therefore, the validity of the solution program in this paper can be verified.

4. Results and Analysis

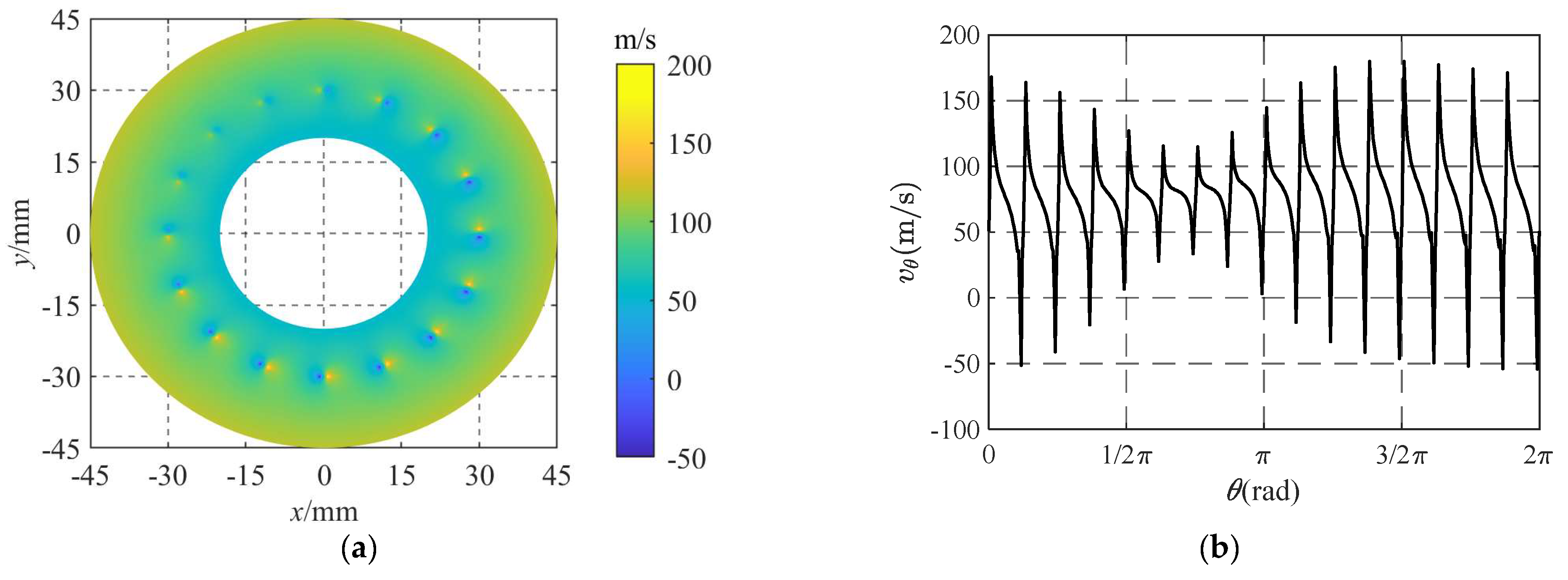
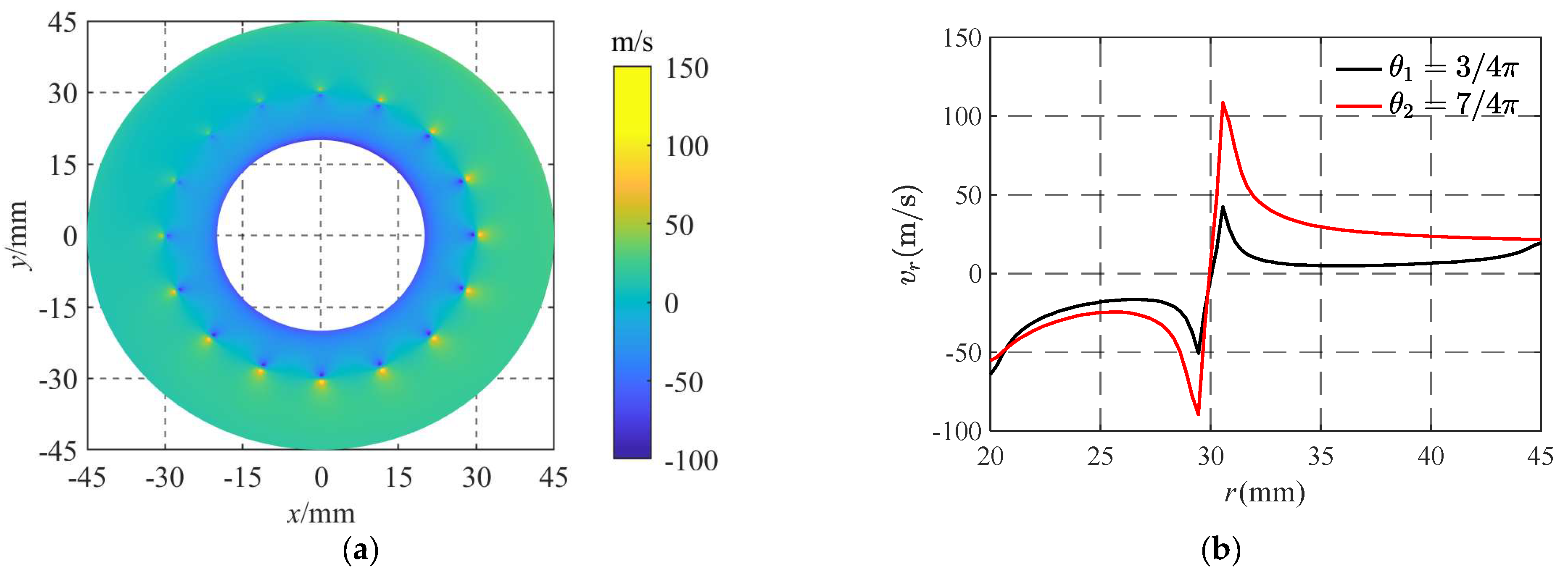
4.1. Static Characteristics Analysis

In the actual working process of the aerostatic motorized spindle, the spindle will produce translation and rotation after being forced, which will change the spatial position of the thrust collar. This change will directly affect the gas film distribution of the thrust bearing and then have an important impact on the pressure and velocity distribution of gas in the gas film. The parameters of aerostatic thrust bearings studied in this paper are shown in Table 1. When the values of φ_x and φ_y are both 2 × 10⁻⁴ rad, the gas pressure distribution in the gas film obtained by the numerical solution is shown in Figure 7, and the circumferential average velocity distribution and the radial average velocity distribution of the gas in the film are shown in Figure 8 and Figure 9, respectively.

As can be seen from Figure 7a, the torsion angle of the thrust collar will seriously affect the pressure distribution of gas in the gas film. Because the torsion angles of the thrust collar on the x-axis and the y-axis are both positive values, the gas film thickness is the smallest at θ = 3/4π, and the gas film pressure near this angle is the largest, while the gas film thickness at θ = 7/4π is the largest and the gas film pressure near this angle is the smallest. In addition, because the rotation direction of the thrust collar is counterclockwise, under the influence of the dynamic pressure effect, the high-pressure region in the gas film will shift to a certain extent in the direction of smaller θ. As shown in Figure 7b, under the same gas supply pressure, the pressure behind the orifice at θ = 3/4π is close to 0.5 Mpa, while the pressure behind the orifice at θ = 7/4π is only about 0.25 Mpa. This shows that the film thickness at the orifice has a significant influence on the pressure behind the orifice. Specifically, the smaller the film thickness at the orifice, the greater the pressure, and vice versa.

As shown in Figure 8a, with the increase in the gas film radius, the circumferential average velocity of gas in the gas film shows a trend of increasing gradually. At the orifice node, because the high-pressure gas flows into the gas film through the orifice, it will quickly spread around, which leads to a significant increase in the gas velocity gradient near the orifice, thus causing an obvious sudden change in the circumferential average velocity. In addition, combined with Figure 8b, it can be further observed that there is a positive correlation between the velocity variation amplitude at the orifice and the film thickness. Specifically, at θ = 3/4π, the film thickness is the smallest, and the velocity variation amplitude is also the smallest. At θ = 7/4π, the film thickness is the largest, and the velocity variation amplitude is also the largest. This phenomenon shows that the film thickness has an important influence on the gas flow characteristics.

As shown in Figure 9a, the radial average velocity of gas is different under different angular coordinates. Generally speaking, the change amplitude of the radial average velocity of the gas is relatively small near θ = 3/4π, but it increases significantly near θ = 7/4π. In addition, it can be observed from the figure that the radial average velocity of the gas in the gas film changes in the flow direction at the orifice distribution circle. The concrete manifestations are as follows: for the nodes whose radial coordinates are greater than the radius of the distribution circle, the radial velocity is positive, indicating that the gas flows outward; for the nodes whose radial coordinates are smaller than the radius of the distribution circle, the radial velocity is negative, indicating that the gas flows inward. Figure 9b further shows the distribution characteristics of gas radial average velocity along radial coordinates at θ₁ = 3/4π and θ₂ = 7/4π. It can be seen that the radial average velocity suddenly changes at r = 30 mm due to the diffusion of gas into the gas film, and the suddenly changed velocity values are located on both sides of the 0 velocity line. By comparing the radial velocity curves at θ₁ and θ₂, it can be found that the radial average velocity at θ₁ is lower than that at θ₂, which indicates that the radial velocity distribution of gas is also positively correlated with the bearing gas film thickness.

Combined with the analysis results of Figure 7, Figure 8 and Figure 9, it can be seen that the torsion angle of the thrust collar has a significant influence on the pressure distribution and velocity distribution of the aerostatic thrust bearing. Therefore, in the simulation calculation, the influence of the rotational degree of freedom of the thrust collar on the gas flow characteristics must be fully considered.

4.2. Single-Factor Analysis of Inertial Force Error

In the analysis of aerostatic bearings, the gas inertial force is considered to describe the flow behavior of gas in the bearing gap more accurately, especially at high speed or acceleration. The introduction of inertial force will increase the complexity of the model, but it can reflect the dynamic characteristics of the fluid more truly, thus improving the accuracy and reliability of the analysis. According to the description in [21], the influence of gas inertia force on the performance of aerostatic bearing can be roughly evaluated by multiplying the gas Reynolds number in the gas film by the ratio of gas film thickness to bearing radius, and its expression is

$${\mathrm{Re}}^{\ast }=\mathrm{Re}\times \lambda ={\displaystyle \frac{\rho v{h}^2}{\eta r}}$$

(21)

Although this evaluation method cannot accurately calculate the size of bearing performance error caused by inertial force term, it can be seen from its expression that the size of inertial force error is mainly related to key parameters such as gas density, velocity, gas film thickness, and bearing geometry size. Among them, the gas density and velocity are mainly determined by the gas supply pressure and rotating speed, while the gas film thickness is influenced by the initial clearance of the bearing and the torsion angle of the thrust collar. Therefore, the gas supply pressure, rotational speed, gas film thickness, torsion angle, and bearing radius are the main influencing factors in this paper, and we quantitatively study the influence of inertia force term on bearing performance.

The relative error of bearing performance caused by gas inertia force term can be expressed as

$$\left\{\begin{array}{l}{E}_{\mathrm{Fz}}=\left(F_{\mathrm{z}1}-F_{\mathrm{z}2}\right)/F_{\mathrm{z}2}\\ E_{\mathrm{Mx}}=\left(M_{\mathrm{x}1}-M_{\mathrm{x}2}\right)/M_{\mathrm{x}2}\\ E_{\mathrm{My}}=\left(M_{\mathrm{y}1}-M_{\mathrm{y}2}\right)/M_{\mathrm{y}2}\end{array}\right.$$

(22)

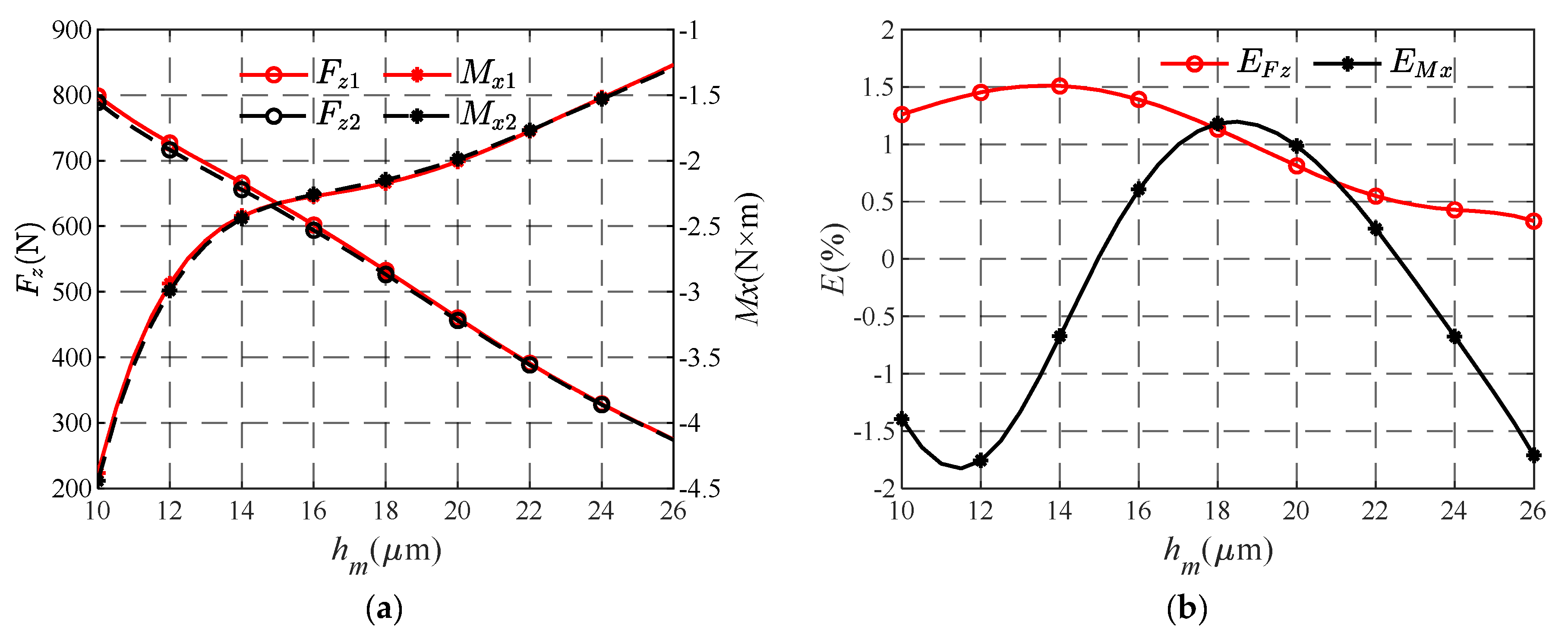
4.2.1. Influence of Initial Clearance on Inertial Force Error

For aerostatic bearings, the gas film thickness has a very important influence on the performance of bearings. When the value of φ_x is 1 × 10⁻⁴ rad and the value of φ_y is 0, other parameters are shown in Table 1. The curves of axial bearing capacity F_z and torque around the x-axis M_x with the initial clearance obtained by numerical calculation are shown in Figure 10a. The curves of the relative error of bearing capacity E_Fz and the relative error of torque around the x-axis E_Mx caused by inertia force with the initial clearance are shown in Figure 10b.

As can be seen from Figure 10a, with the increase in initial clearance, the axial bearing capacity F_z gradually decreases, and the value of F_z1 is always slightly larger than F_z2, but the gap between them gradually becomes smaller and tends to overlap. Observing the change curve of torque around the x-axis M_x, it can be found that because φ_x is positive, the thrust bearing will produce recovery torque that makes the thrust collar rotate around the x-axis in the opposite direction, so the torque produced by the thrust bearing is negative. At the same time, observing the change of torque, it can be found that with the increase in initial clearance, the recovery torque produced by the thrust bearing decreases gradually, and the reduction rate of M_x is faster when the initial clearance is less than 14 μm, slower when the initial clearance is between 14 μm and 20 μm and increases again when the initial clearance is greater than 20 μm, and decreases linearly. When comparing the two curves of M_x1 and M_x2, it can be seen that the two curves corresponding to M_x1 and M_x2 basically coincide, which shows that the inertia force error caused by the change of bearing initial clearance is not large.

As can be seen from Figure 10b, with the change in initial clearance, the overall E_Fz curve shows a decreasing trend. When the initial clearance is 14 μm, the E_Fz reaches the maximum value, which is about 1.5%. At the same time, the E_Mx curve fluctuates in a certain range with the change in initial clearance, but the fluctuation amplitude of its relative error never exceeds 2%. The comprehensive evaluation of the influence of initial clearance on E_Fz and E_Mx curves shows that the change in initial clearance has a relatively small influence on the inertial force error, which does not exceed the preset error threshold of 2%.

4.2.2. Influence of Bearing Radius on Inertial Force Error

The size of the bearing will affect the Reynolds number of the gas in the gas film. When the difference between the inner and outer radius of the bearing is constant, the outer radius r_b of the bearing increases from 35 mm to 80 mm. The curves of F_z and M_x with the bearing radius are shown in Figure 11a, and the curves of E_Fz and E_Mx with the bearing radius are shown in Figure 11b.

Figure 11a reveals the influence of bearing radius on F_z and M_x performance parameters. Specifically, with the increase in bearing radius, the F_z curve shows a nearly linear growth trend. By further comparing the F_z1 and F_z2 curves, it can be found that when the bearing radius is small, they almost coincide, but as the bearing radius increases, the gap between them gradually expands. When observing the change law of the M_x curve, it can be seen that M_x increases with the increase in bearing radius, and the growth rate is gradually accelerated. When comparing the two curves of M_x1 and M_x2, it can be found that the variation rules of the two curves are the same and the numerical differences are small. However, as the bearing radius increases, the absolute error between them also gradually becomes obvious. The main reason for the above changes is that with the increase in bearing size, not only does the bearing area of the gas bearing increase but the bearing is also affected by the dynamic pressure effect, resulting in a significant increase in bearing capacity and recovery torque. In addition, the increase in bearing radius leads to the increase in Reynolds number of gas in the gas film, and then the absolute error of bearing performance parameters caused by inertial force term increases gradually. Therefore, the curve difference between ignoring inertia force and considering inertia force is gradually obvious.

In Figure 11b, the value of E_Fz increases with the increase in bearing radius, but the growth rate gradually decreases. When the bearing radius is greater than 60 mm, the value of E_Fz will be greater than 2%. It can be seen that the relative error of bearing capacity caused by inertia force is already large. With the increase in bearing radius, E_Mx first increases and then decreases. When the outer radius of the bearing is 65 mm, E_Mx reaches the maximum value, which is about 2.2%, and when the radius of the bearing outer circle is between 60 mm and 80 mm, the values of E_Mx all exceed 2%. The comprehensive analysis in Figure 11 shows that the bearing size has a great influence on the inertial force error, and the larger the bearing size, the greater the error caused by the inertial force term.

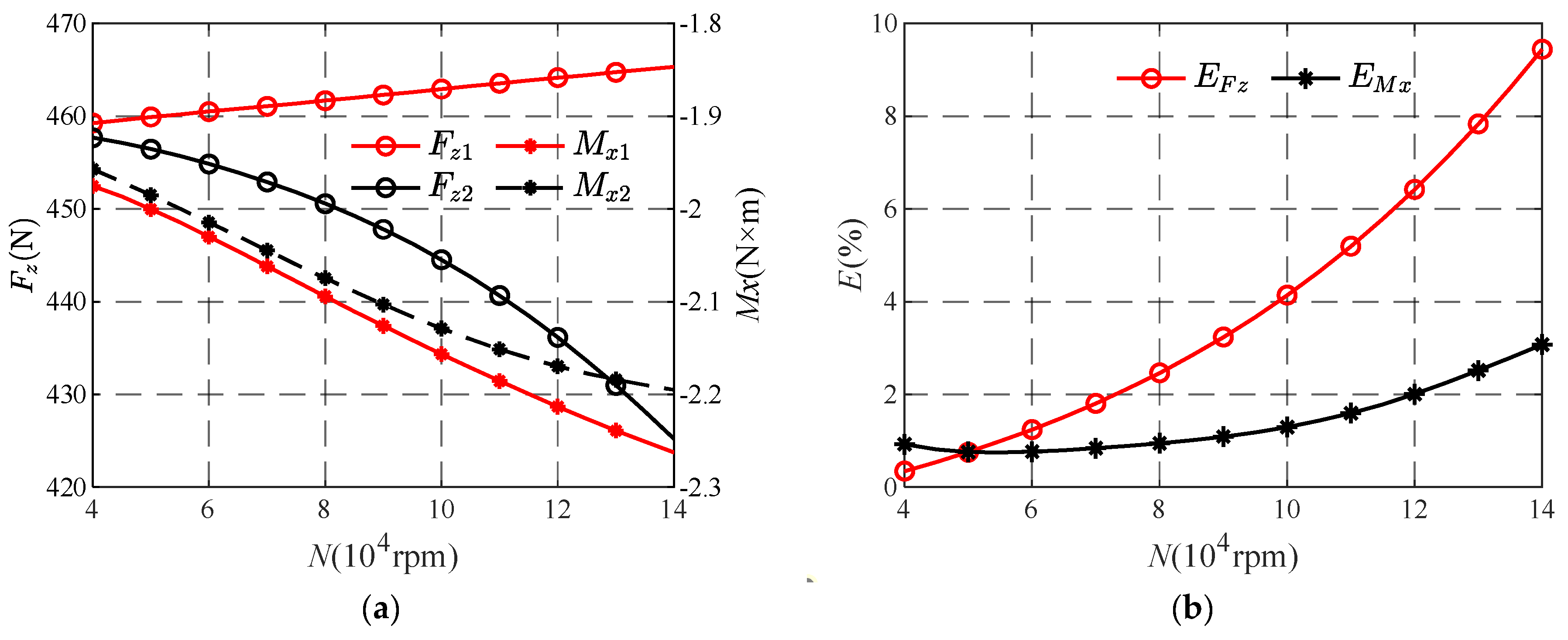
4.2.3. Influence of Rotating Speed on Inertial Force Error

The bearing rotating speed has a great influence on the Reynolds number of the gas in the gas film. When the rotating speed gradually increases from 40,000 rpm to 140,000 rpm, the curves of F_z and M_x with the rotating speed are shown in Figure 12a, and the curves of E_Fz and E_Mx with the rotating speed are shown in Figure 12b.

As can be seen from Figure 12a, there is a very obvious difference between the two curves corresponding to F_z1 and F_z2. Specifically, with the increase in rotational speed, the value of F_z1 is always greater than the value of F_z2, F_z1 increases slowly in a straight line, while F_z2 shows a downward trend, and the rate of decline gradually accelerates. When comparing the two curves of M_x1 and M_x2, it can be seen that the values of M_x1 and M_x2 increase with the increase in rotational speed, and in the process of change, M_x1 is always greater than M_x2. In addition, the M_x1 curve almost grows linearly, while the growth rate of the corresponding curve of M_x2 gradually slows down. This paper holds that the main reason for this difference is that when the bearing speed is high, the bearing is greatly influenced by the inertial force term, which makes the dynamic characteristics of gas flow more complicated. The change in gas flow characteristics will change the distribution of gas pressure to a certain extent, resulting in uneven distribution of gas film pressure, thus reducing the bearing capacity and having a certain impact on the recovery torque of the bearing.

Figure 12b shows the changes of E_Fz and E_Mx with the rotating speed. It can be seen that both curves increase with the increase in rotating speed. Among them, the growth rate of the E_Fz curve is relatively fast, and with the increase in rotational speed, the growth rate gradually accelerates. When the rotational speed exceeds 7 × 10⁴ rpm, the E_Fz value will exceed 2%, indicating that the inertial force has a great influence on the bearing capacity error. In contrast, the growth rate of the E_Mx curve is relatively small. When the rotation speed is less than 8 × 10⁴ rpm, the E_Mx value is relatively stable and remains at about 1%. However, as the rotation speed continues to increase, the growth rate of the E_Mx curve gradually accelerates, and when the rotation speed is greater than 12 × 10⁴ rpm, the value exceeds 2%. Through the influence of the inertial force on the bearing capacity and torque of the gas bearing, it can be seen that the bearing speed has a significant effect on the inertial force error. Therefore, when the bearing speed is high, the influence of the inertial force term cannot be ignored.

4.2.4. Influence of Gas Supply Pressure on Inertial Force Error

The gas supply pressure will affect the pressure and velocity distribution of gas in the gas film. When the gas supply pressure is increased from 0.3 Mpa to 0.7 Mpa, the curves of F_z and M_x with the gas supply pressure are shown in Figure 13a, and the curves of E_Fz and E_Mx with the gas supply pressure are shown in Figure 13b.

In Figure 13a, both F_z and M_x increase linearly with the increase in gas supply pressure. It can be seen that there is only a small difference between the bearing capacity and torque under the condition of considering inertia force and not considering inertia force, indicating that the influence of inertia force term on bearing performance is always small in the process of bearing gas supply pressure change. As can be seen from Figure 13b, the E_Fz curve decreases continuously with the increase in gas supply pressure. When the gas supply pressure is 0.3 Mpa, the value of E_Fz is the largest, but it is still less than 2.5%. When the gas supply pressure exceeds 0.6 Mpa, E_Fz even appears negative, indicating that when the gas supply pressure of the bearing is large enough, the bearing capacity under the condition of considering the gas inertia force is slightly larger than that without considering the inertia force. At the same time, it can be seen from the curve of E_Mx that with the increase in gas supply pressure, E_Mx first increases and then gradually decreases. When the gas supply pressure is 0.45 Mpa, E_Mx reaches the maximum value, but it still does not exceed 1%. According to the influence of inertia force on the absolute error and relative error of bearing capacity and torque under different gas supply pressures, it can be seen that the change in gas supply pressure has little influence on inertia force and very limited influence on bearing performance.

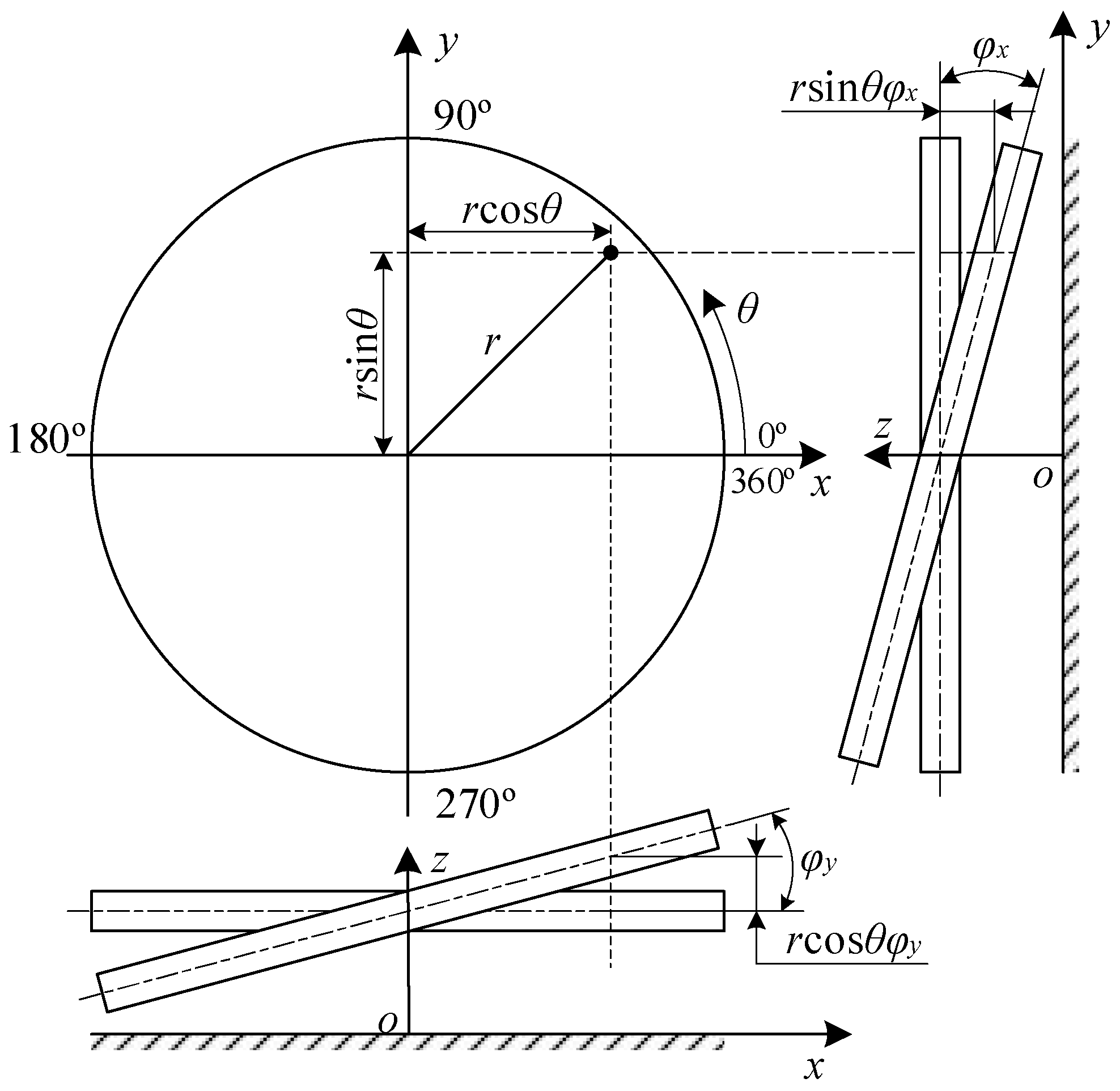
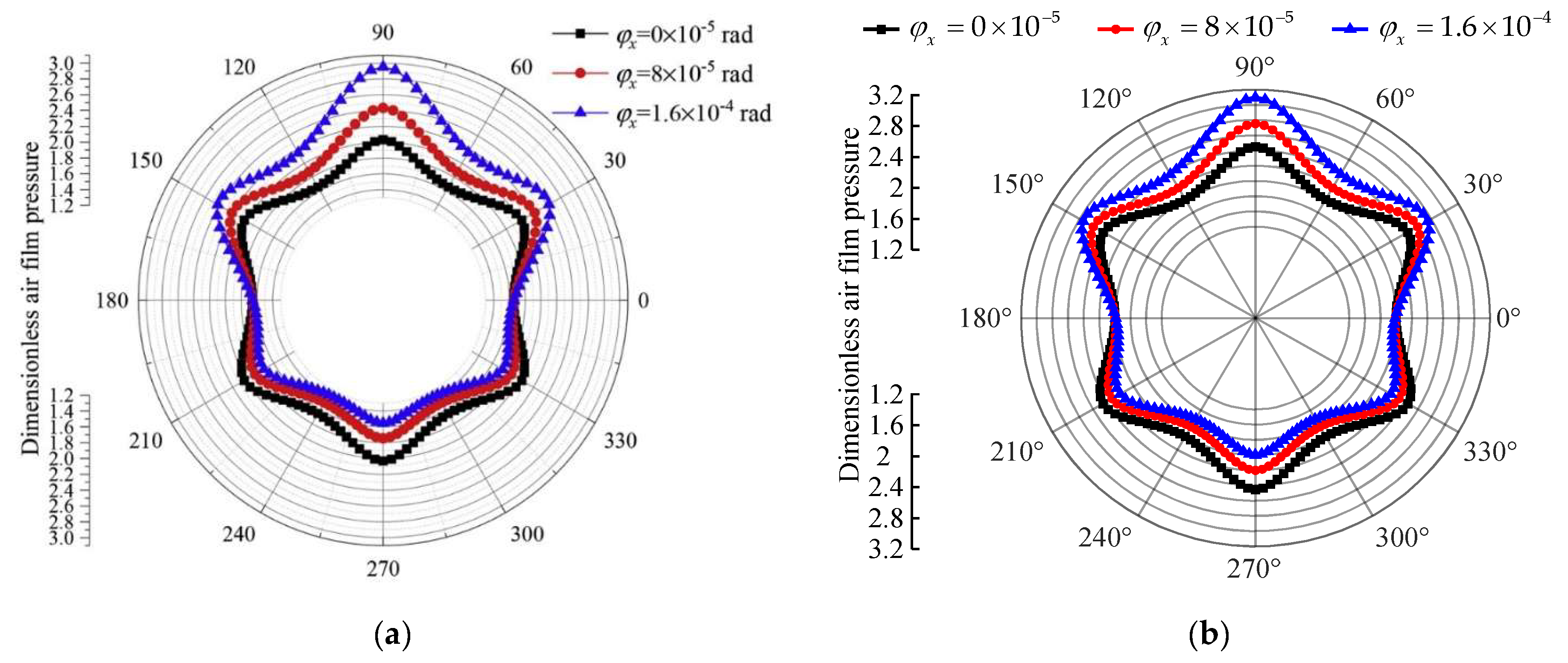
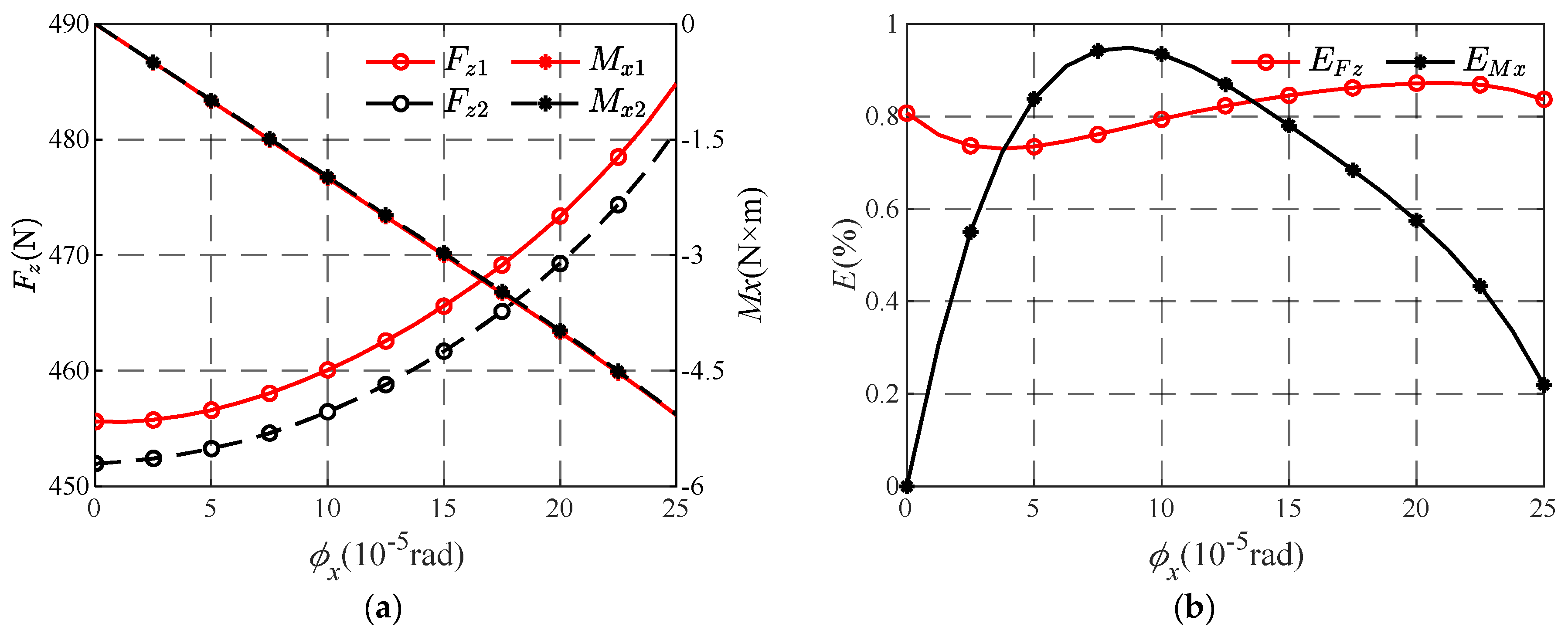
4.2.5. Influence of Torsion Angle on Inertial Force Error

The spatial attitude of the thrust collar will directly affect the gas film thickness distribution of the bearing, and it will also affect the pressure and velocity distribution of the gas in the gas film to a certain extent, thus affecting the magnitude of the inertia force term. With the torsion angle of the thrust collar as a variable, when the torsion angle φ_x increases from 0 to 2.5 × 10⁻⁴ rad, the curves of F_z and M_x with torsion angle are shown in Figure 14a, and the curves of E_Fz and E_Mx with torsion angle are shown in Figure 14b.

As can be seen from Figure 14a, with the increase in the torsion angle of the thrust collar, the bearing capacity F_z gradually increases, and its growth rate also gradually increases. When comparing the two curves of F_z1 and F_z2, it can be seen that the value of F_z1 is always greater than F_z2 in the process of torsion angle change, and the absolute error between them has little change. It can be seen that the bearing capacity decreases after increasing the influence of the inertia force term, and in the process of torsion angle change, the change in bearing capacity error caused by inertial force is small. When observing the change curve of M_x, it can be found that with the increase in torsion angle, the value of M_x increases rapidly along the straight line, and the two curves of M_x1 and M_x2 almost coincide, indicating that the absolute error between the two is very small. As can be seen from Figure 14b, with the increase in torsion angle, the value of E_Fz is stable at about 0.8%, while the value of E_Mx increases first and then decreases gradually. When the torsion angle is 7.5 × 10⁻⁵ rad, E_Mx reaches the maximum value, but its maximum value is still less than 1%. It can be seen that the inertia force error caused by the torsion angle of the thrust collar is very limited. When considering the influence of gas inertia force on the absolute error and relative error of bearing capacity and torque under different torsion angles, it can be concluded that the change of torsion angle has little influence on the inertia force term.

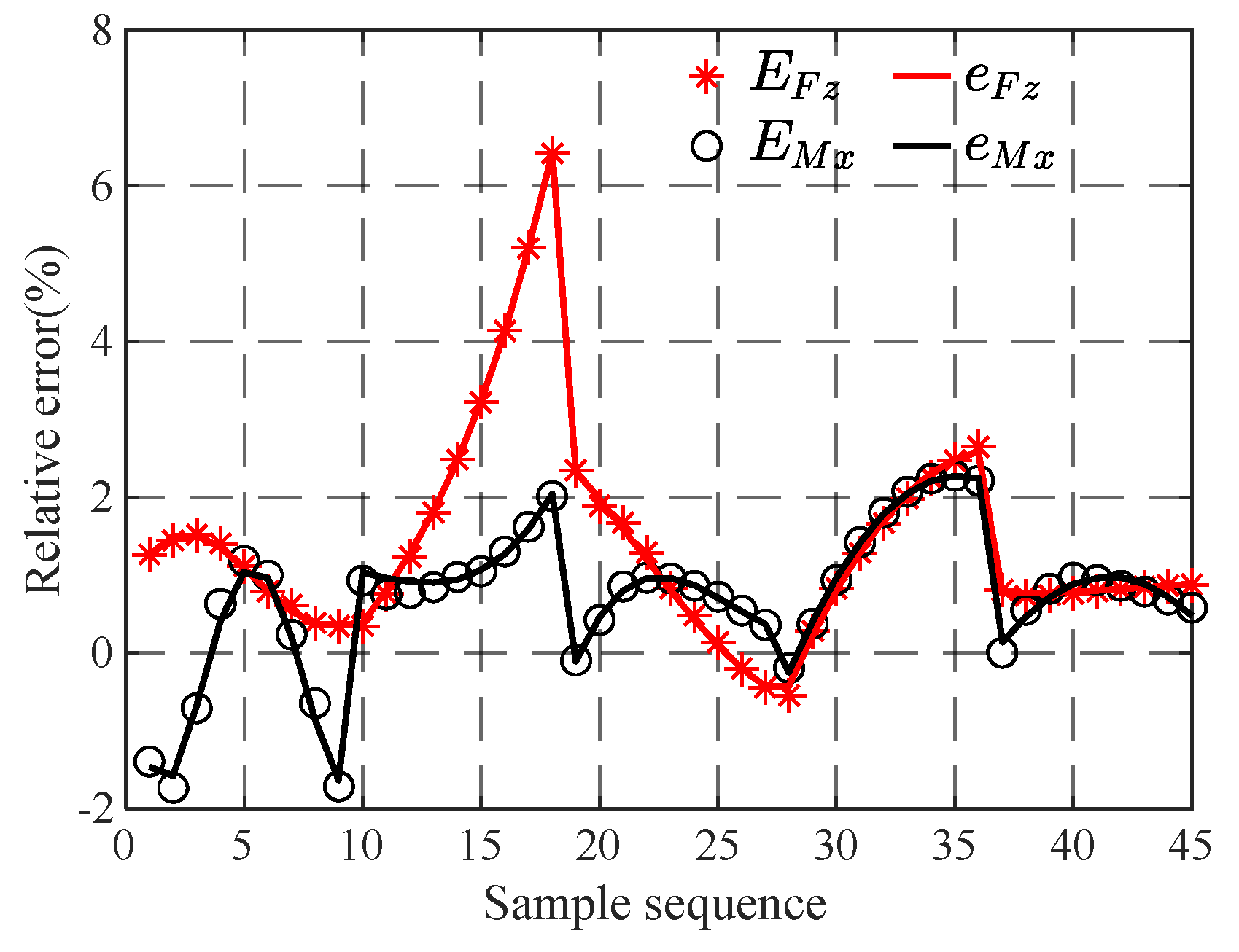
4.3. Multiple Regression Analysis of Relative Error

From the above single-factor simulation results, it can be seen that the errors of bearing capacity and torque caused by gas inertia force are influenced by multiple factors. To evaluate the relative errors of bearing capacity and torque caused by inertia force under the joint influence of different structural parameters and working parameters, taking the above single-factor simulation results as samples, the first 9 simulation results of each group are taken to form a database of 45 units. Through regression analysis, the sample regression equation of the relative error of bearing capacity and the relative error of torque under the influence of multiple factors is obtained, and the validity of the regression equation is verified.

4.3.1. Establishment of Regression Equation

Figure 15 shows the values of each factor in 45 samples and the relative errors corresponding to each sample. According to the different linear relationship between the relative error of bearing capacity and various factors, and the different linear relationship between the relative error of recovery torque and various factors, the regression model is shown in Equation (23):

$$\left\{\begin{array}{l}{e}_{Fz}=a_0+a_1{h}_m+a_2{h}_m^2+a_3{h}_m^3+a_4{r}_b+a_5{r}_b^2+a_6{r}_b^3\\ +a_{7}N+a_8{N}^2+a_9{p}_0+a_{10}{p}_0^2+a_{11}{p}_0^3+a_{12}{\phi }_x+a_{13}{\phi }_x^2\\ e_{Tx}=b_0+b_1{h}_m+b_2{h}_m^2+b_3{h}_m^3+b_4{h}_m^4+b_5{r}_b+b_6{r}_b^2+b_7{r}_b^3\\ +b_{8}N+b_9{N}^2+b_{10}{p}_0+b_{11}{p}_0^2+b_{12}{p}_0^3+b_{13}{\phi }_x+b_{14}{\phi }_x^2\end{array}\right.$$

(23)

where e_Fz and e_Mx are estimated values of E_Fz and E_Mx, respectively; a₀~a₁₃ are the regression coefficient of e_Fz, and b₀~b₁₄ are the regression coefficient of e_Mx.

Through the multiple regression analysis of the results in the sample database, and expressed in the above form, the specific values of each regression coefficient can be obtained. The sample regression equation finally obtained by calculation is shown in Equation (23), and the corresponding goodness of fits are 0.999 and 0.997, respectively. At the same time, as can be seen from Figure 16, the relative error value calculated by the sample regression equation is very consistent with the numerical calculation results, which reflects the rationality of the regression model.

$$\left\{\begin{array}{l}{e}_{Fz}=-17.651+1.313h_m+-0.076h_m^2+0.001h_m^3+0.502r_b-0.035r_b^2+0.004r_b^3\\ +0.488N-0.006N^2+0.359p_0-0.258p_0^2+0.019p_0^3-0.019{\phi }_x+0.003{\phi }_x^2\\ e_{Mx}=46.285-17.622h_m+1.596h_m^2-0.060h_m^3+0.001h_m^4+0.084r_b-0.038r_b^2+0.003r_b^3\\ +0.279N-0.002N^2+6.133p_0-1.039p_0^2+0.055p_0^3+0.149{\phi }_x-0.007{\phi }_x^2\end{array}\right.$$

(24)

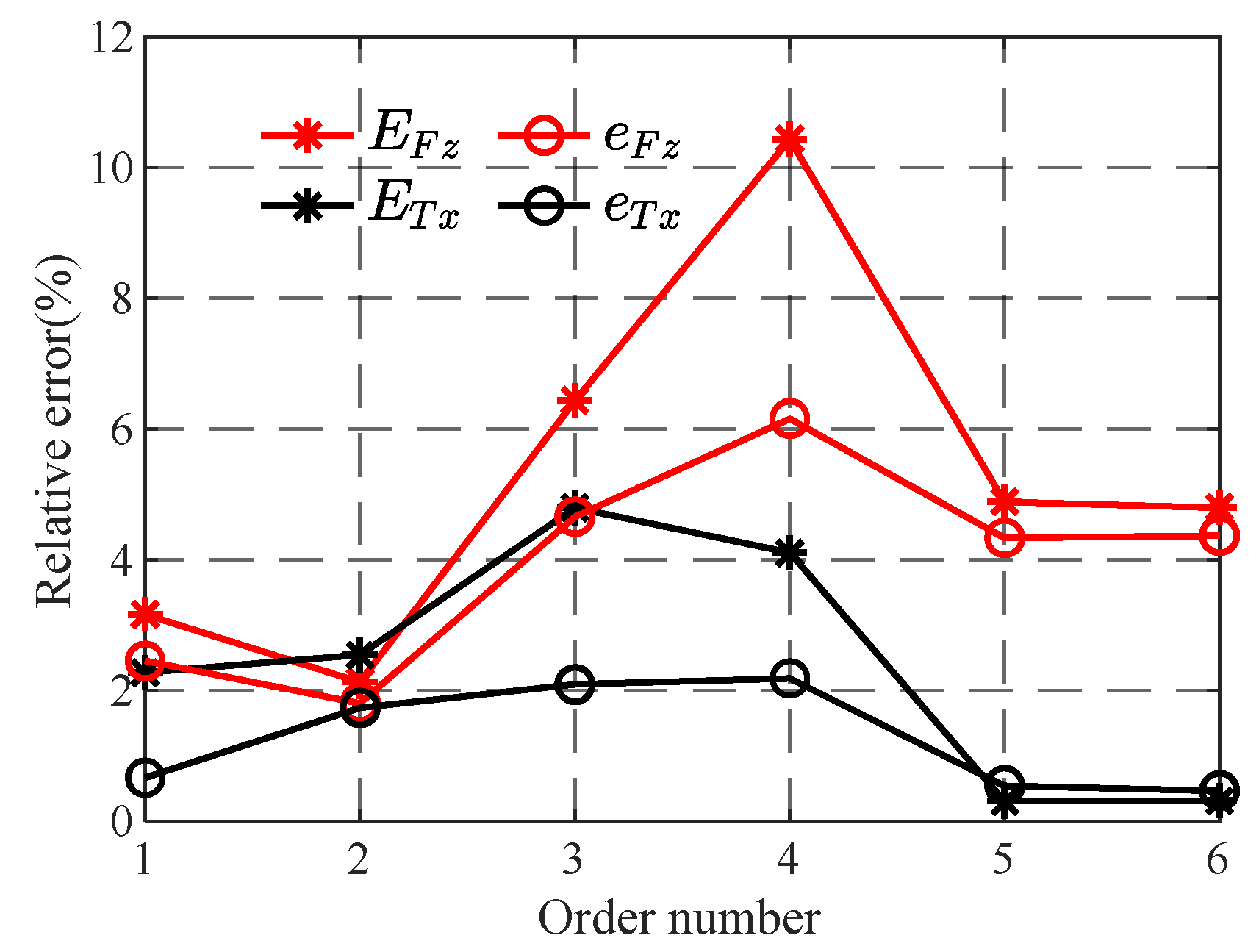
4.3.2. Validation of Regression Equation

To verify the validity of the regression equation, six groups of bearing parameters were selected as verification samples. The accuracy and reliability of the sample regression equation were evaluated by comparing the relative error estimated by the regression equation with the numerical simulation results; see

Table 3. Values of variables in validation samples.

Order Number	hm (μm)	rb (mm)	N (104 rpm)	p0 (bar)	φx (10−5 rad)
1	15	60	6	6	10
2	20	60	6	6	10
3	20	60	10	6	10
4	20	60	10	4	10
5	20	40	10	4	10
6	20	40	10	4	15

for the specific values of the relevant independent variables in the verification sample.

It can be seen from Figure 17 that the curves of e_Fz and e_Mx calculated by the sample regression equation are basically the same as the corresponding curves of e_Fz and e_Mx calculated by numerical calculation., but there are still some differences in values, and the results calculated by sample regression equation are generally smaller than those calculated by numerical calculation. The main reason for the above differences is that the number of samples in the process of solving the regression equation is small, which leads to a certain deviation between the calculation results of the regression equation and the numerical calculation results. When comparing the two curves of E_Fz and e_Fz, it can be seen that the biggest difference between them appears in the fourth group of samples, and the difference is about 5%. At the same time, when observing E_Mx and e_Mx curves, we can see that the biggest difference between them appears in the third group of samples, and the difference is about 3%. It can be seen that although there are some differences between the relative error of regression analysis and numerical calculation, the error between them is not large, which can explain the effectiveness of the sample regression equation.

5. Conclusions

In this paper, firstly, the Reynolds equation in the cylindrical coordinate system was derived theoretically, and a mathematical model of 3-DOF aerostatic thrust bearing was established under the condition of fully considering the rotational freedom of the bearing. Secondly, the finite difference method was used to numerically calculate the bearing in the inclined state of the thrust collar, and the pressure and velocity distribution characteristics of the gas in the gas film under a steady state were obtained. In the single-factor analysis, the effects of initial clearance, radius, rotational speed, gas supply pressure, and torsion angle on the bearing capacity and recovery torque were studied. Finally, using the method of multiple regression analysis, the sample regression equations of relative error of bearing capacity and relative error of restoring torque were obtained, and the effectiveness of the regression equations was verified by simulation results. The main conclusions of this study are as follows:

(1)

The degree of freedom of bearing rotation will seriously affect the thickness distribution of the gas film, and then change the pressure and velocity distribution characteristics of gas in the gas film. At the same time, the smaller the film thickness, the greater the pressure, and the smaller the circumferential and meridional velocity changes at this position.

(2)

In single-factor analysis, it was found that the inertia force error caused by the change of bearing speed is the largest, and when the rotating speed exceeds 7 × 10⁴ rpm, the bearing capacity error will exceed 2%. The bearing radius also has a certain influence on the inertial force error; when it exceeds 60 mm, the relative error of the bearing capacity and the error of the recovery torque will exceed 2%. In addition, the research shows that the inertia force error caused by changing the bearing initial clearance, gas supply pressure, and torsion angle is small.

(3)

The sample regression equations of relative error of bearing capacity and relative error of torque were obtained by multiple regression analysis, and the estimated value of relative error obtained by verifying the regression equation in the sample was compared with the simulation value. The results show that the variation law of the estimated value and the simulation value is the same, and there are some differences in numerical values, but the error range is small, which can prove the effectiveness of the sample regression equation.