Study on Traction Characteristics of Point Contact State under Oil–Air Lubrication
Abstract
1. Introduction
2. Oil–Air Lubrication Traction Test
2.1. Test Apparatus
2.2. Test Scheme
3. Oil–Air Lubrication Flow Field Simulation
3.1. Multiphase Flow Model and Governing Equation
3.2. Oil–Air Lubrication Flow Field Calculation Domain Model
4. Traction Test and Simulation Result
4.1. The Quantitative Valve Is 0.05 mL/Time
4.2. The Quantitative Valve Is 0.2 mL/Time
4.3. Comparison between Experimental Results and Simulation Results
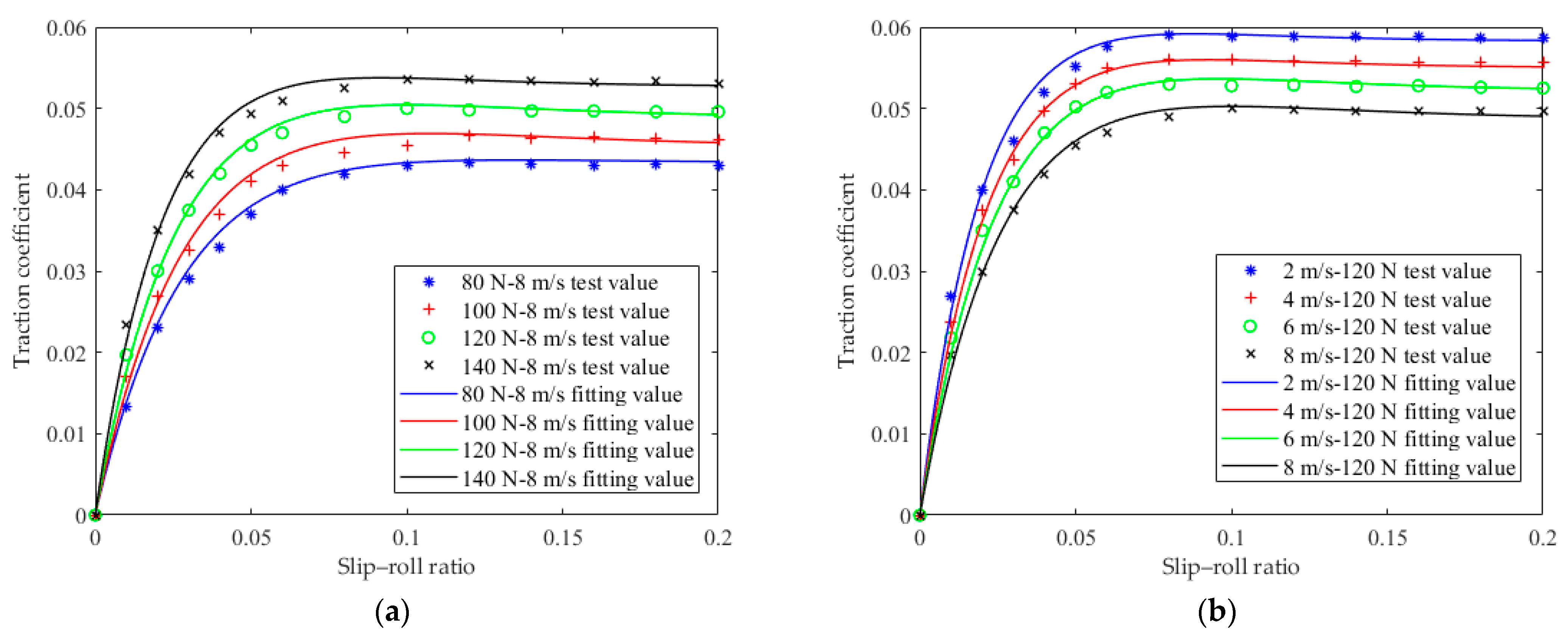
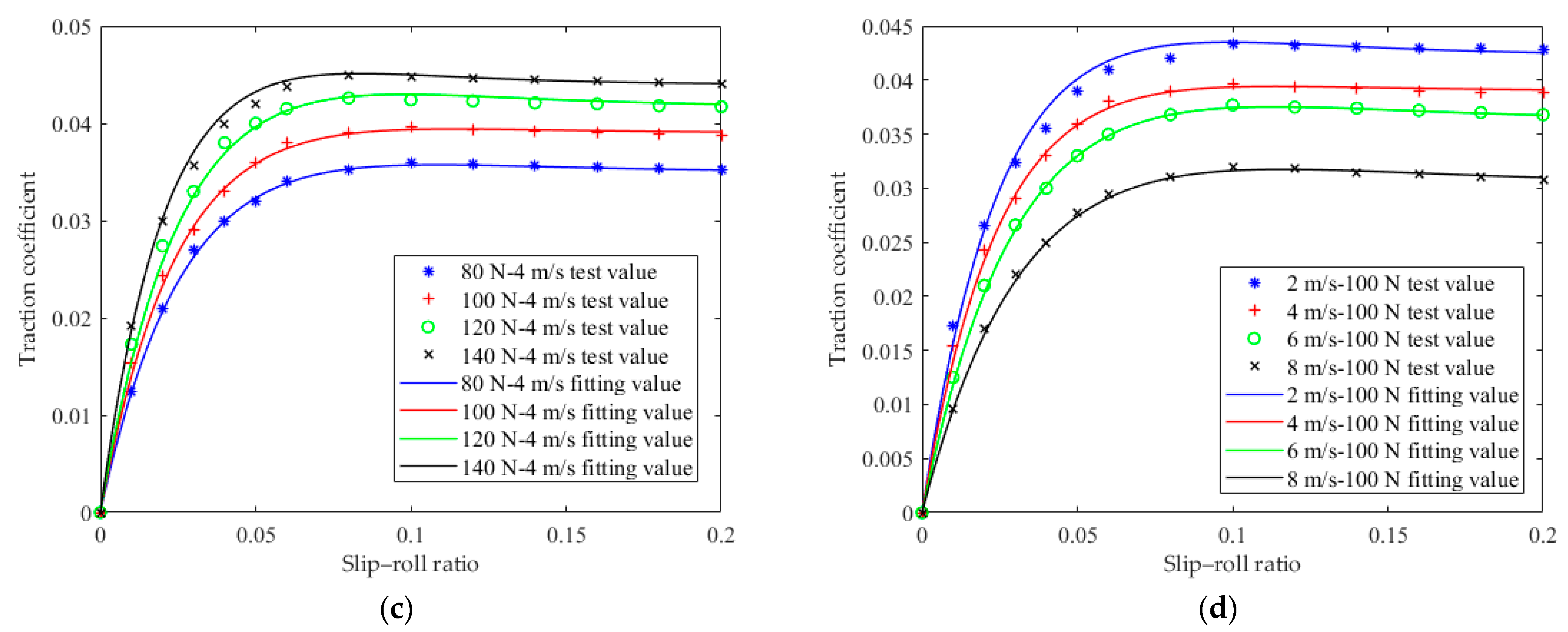
5. Analysis of the Four-Parameter Exponential Model
6. Conclusions
- (1)
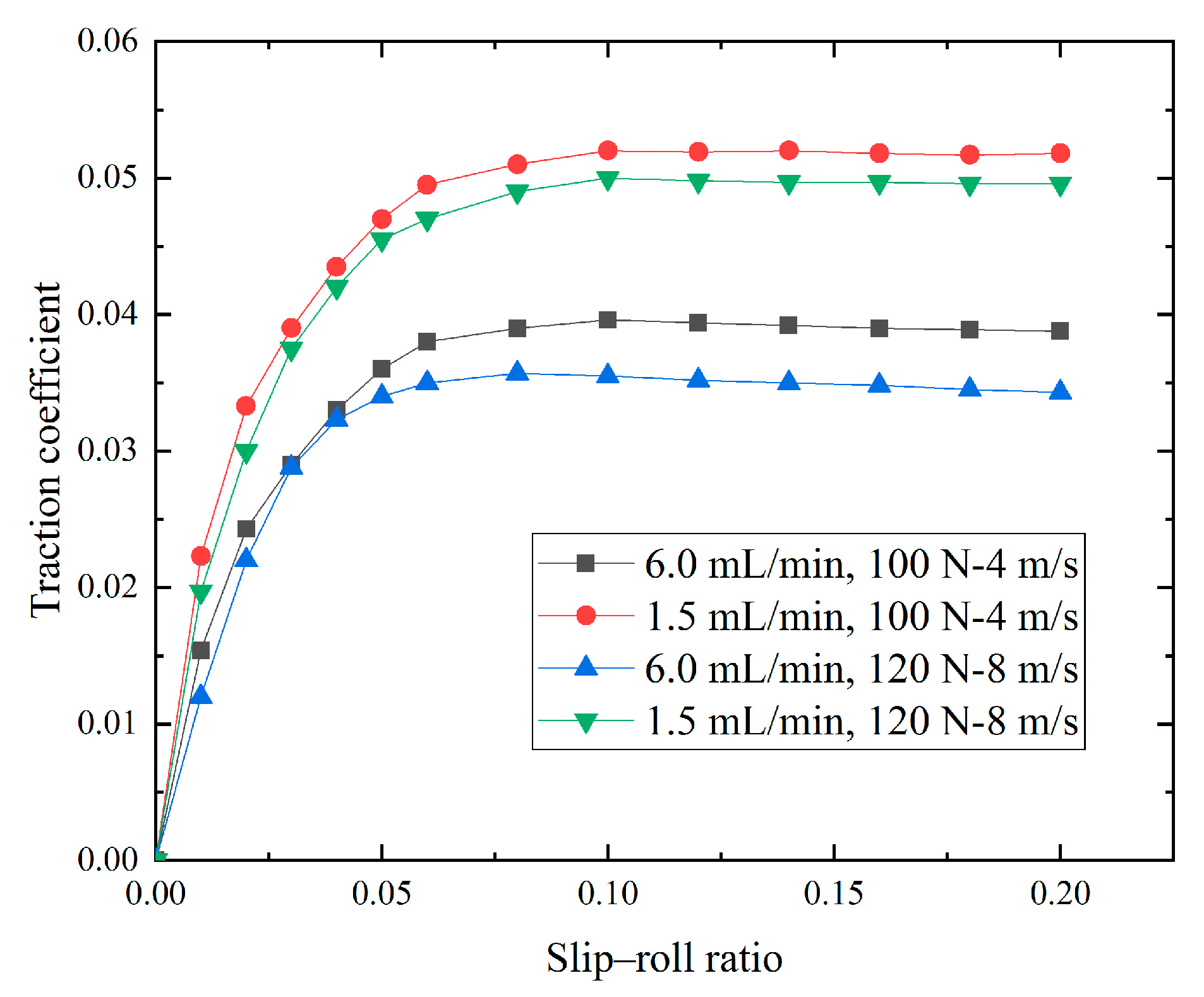
- The traction characteristics of oil–air lubrication were tested under different quantitative valve working conditions of 1.5 mL/min and 6 mL/min using the traction power testing machine. The curve of the traction coefficient varying with the slip–roll ratio was obtained. The experimental results show that under the condition of 12 m/s air-speed, the traction coefficient of 6 mL/min oil supply is smaller than that of 1.5 mL/min oil supply. When the entrainment speed is constant, the traction coefficient increases with the increase of the load. When the load is constant, the traction coefficient decreases with the increase of entrainment speed.
- (2)
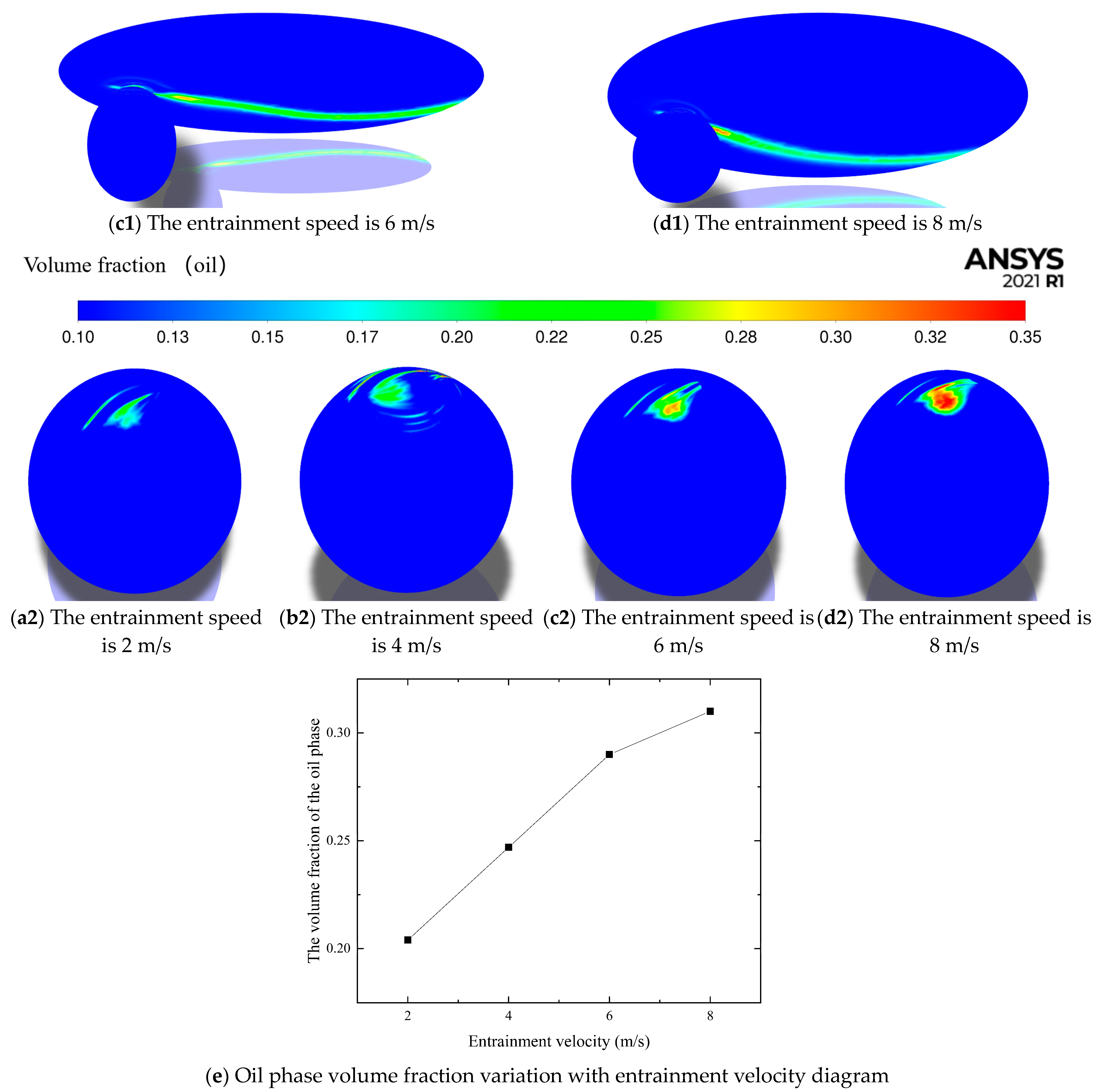
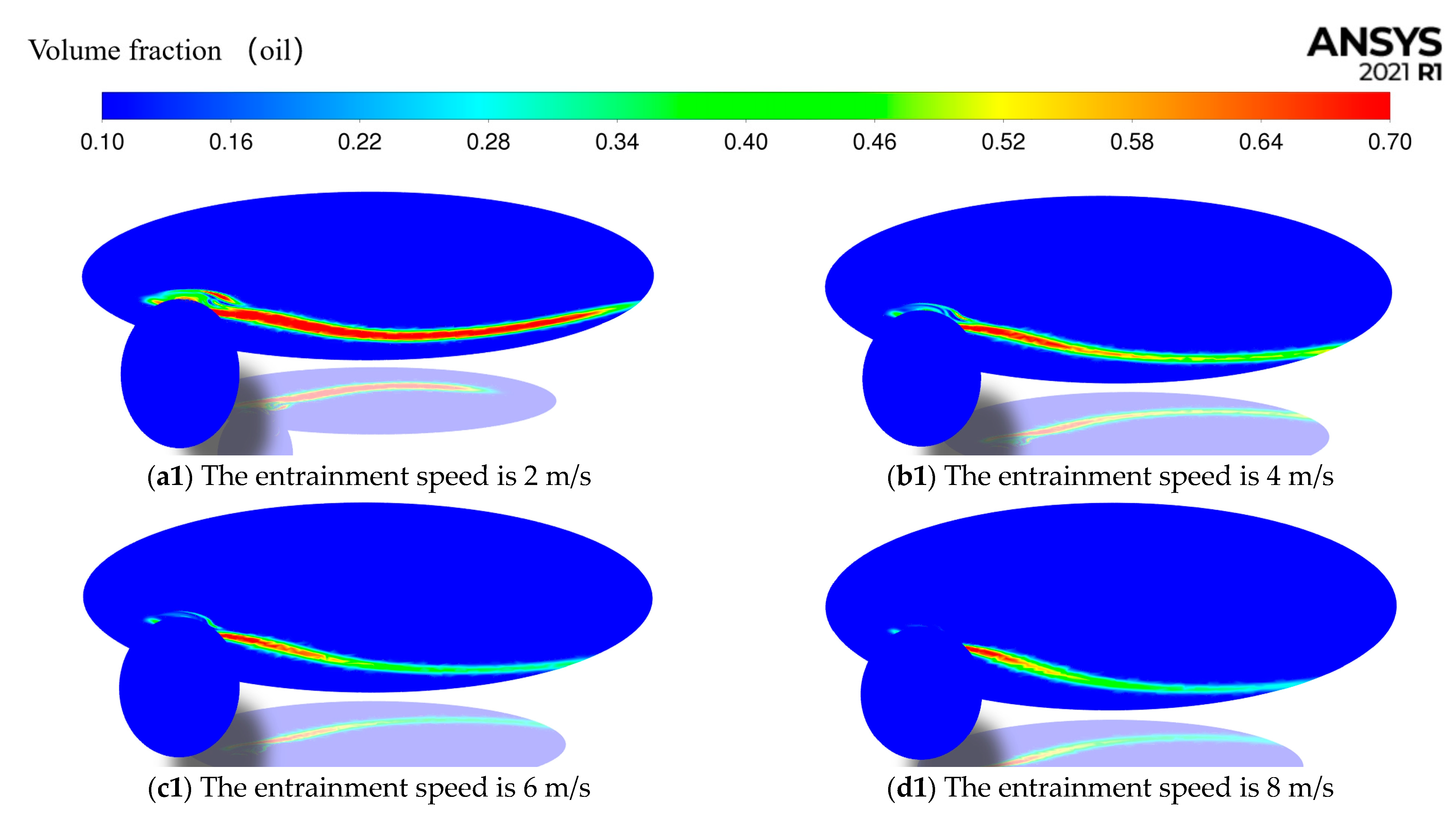
- The oil–air lubrication flow field simulation model was established, and the oil supply conditions of 1.5 mL/min and 6 mL/min were simulated and analyzed. With the increase of entrainment speed, the oil volume fraction on the spherical surface of the contact region also increases. Furthermore, under the oil supply condition of 6 mL/min, the oil volume fraction on the spherical surface of the contact region exceeds that observed at 1.5 mL/min.
- (3)
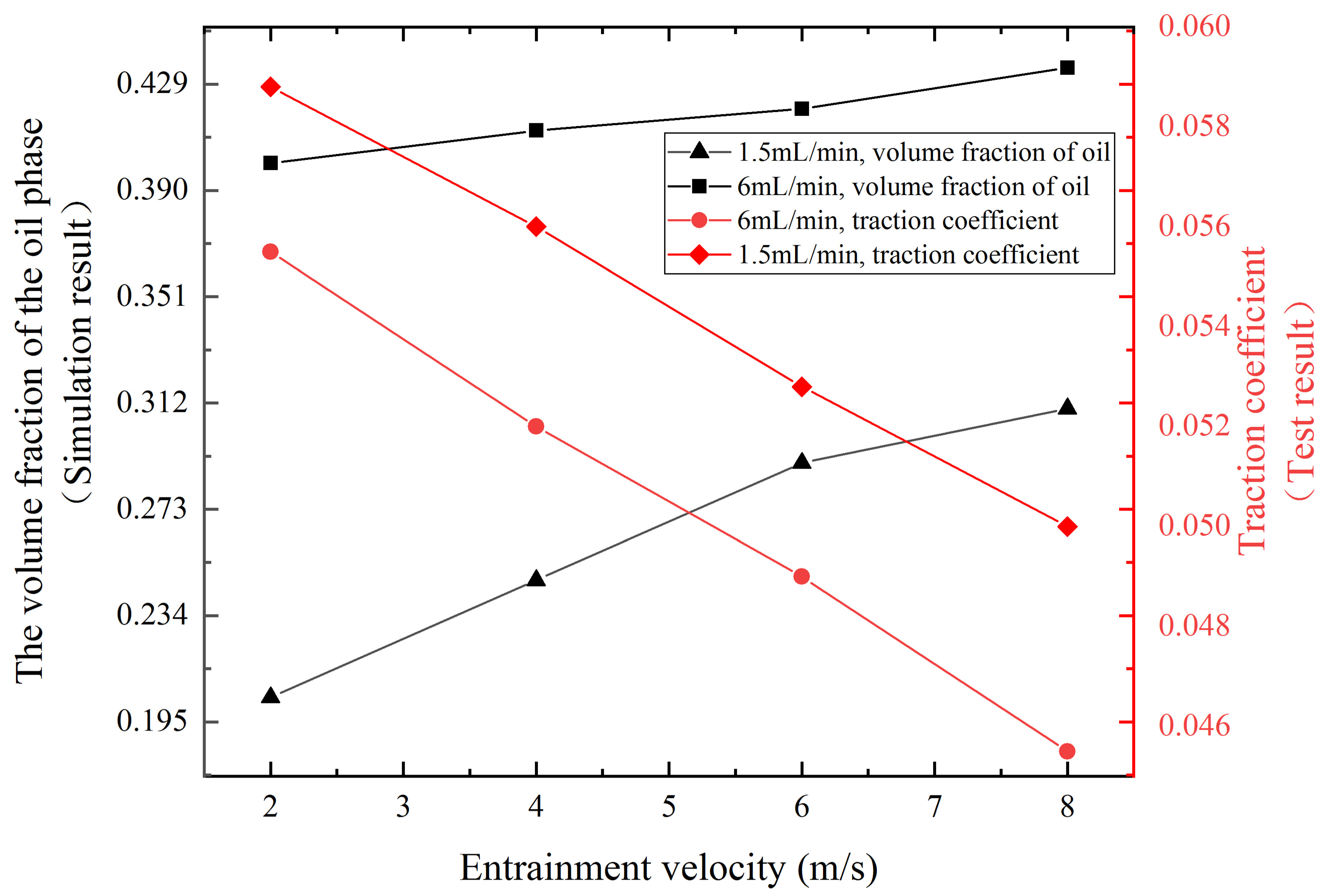
- Fluid simulation analysis is closely related to the results of the traction test. When the slip–roll ratio is 0.1, the traction coefficient shows a downward trend with the change of the entrainment speed from 2 to 8 m/s, and the volume fraction of lubricating oil on the spherical surface of the contact area gradually increases.
- (4)
- The four-parameter exponential model was used for the fitting analysis of the test data, and it was found that the theoretically predicted value of the four-parameter exponential model was in good agreement with the experimental value, and the fitting accuracy was above 0.95.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yao, J.; Kong, X.D.; Meng, Z.Y.; Ai, C.; Yu, B. Research on Flow Characteristics of Air-oil Two Phase Annular Flow for Oil-air Lubrication. Lubr. Eng. 2012, 37, 65–69. [Google Scholar]
- Yao, J. A New Type of Lubrication System—Oil-Air Lubrication System. Lubr. Eng. 1996, 6, 56–57. [Google Scholar]
- Zeng, Q.; Liu, C.; Zhang, J. Application Status and Study of Oil-air Lubrication System for High Speed Rolling Bearing. Lubr. Eng. 2015, 40, 103–108. [Google Scholar] [CrossRef]
- Yang, H.; Liu, H. Turbolub Oil-air Lubrication Technology (5). Lubr. Eng. 2003, 5, 92–95, 91. [Google Scholar] [CrossRef]
- Su, B.; Li, H.; Zhang, G.; Liu, F.; Cui, Y. Study on Cage Stability of Solid-Lubricated Angular Contact Ball Bearings in an Ultra-Low Temperature Environment. Lubricants 2024, 12, 124. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, B.; Feng, Q. Research on Critical Technology of Elastohydrodynamic Traction Experiment System. J. Luoyang Inst. Sci. Technol. (Soc. Sci. Ed.) 2003, 18, 23–27. [Google Scholar] [CrossRef]
- Qiang, X.; Li, J.; Niu, Y.; Wu, L. The Discussing on Traction Force of Elastohydrodynamic Lubricants under Line Contact. J. Zhongyuan Univ. Technol. 2001, 12, 27–28, 39. [Google Scholar] [CrossRef]
- Liu, K.; Bing, L.; Liu, X.; Wang, W. Experimental Study on EHL Friction under Big Slide-roll Ratio Conditions. China Mech. Eng. 2008, 19, 2573–2576. [Google Scholar]
- Guo, K.; Yuan, S.; Shao, Z. Study on Oil-Air Two-Phase Flow Inside the High-Speed Bearing Cavity with Jet Lubrication. Trans. Beijing Inst. Technol. 2012, 32, 1022–1025, 1041. [Google Scholar] [CrossRef]
- Wu, L.; Tong, B.; Guo, D.; Ma, L.; Hu, X. Influence of Oil—Air Lubrication Flow Behavior on Frictional Characteristics of Point Contact. Tribology 2018, 38, 700–710. [Google Scholar] [CrossRef]
- Liu, C.; Li, X.; Guo, F.; Li, S.; Wan, Y. Experimental Observation of EHL Behavior under Single Oil Droplet Supply. Tribology 2017, 37, 340–347. [Google Scholar] [CrossRef]
- Tan, H.; Yang, P.; Yin, C. Analysis of the Oil Starvation in EHL Point Contacts under a Special Oil-supply Condition. Tribology 2007, 27, 357–361. [Google Scholar] [CrossRef]
- Duan, J.; Zhang, D. Lubricated Sliding-rolling of a Ball-on-disc Configuration at Low Speed and Light Load. Tribology 2010, 30, 431–436. [Google Scholar] [CrossRef]
- Hu, Z.; Li, S.; Chen, P.; Wang, Y.; Gu, J.; Fu, L. Analysis of Lubricating Status in Ultra High-speed Spindle Bearings. Lubr. Eng. 2009, 34, 31–35, 40. [Google Scholar]
- Liu, J.; Yang, P.; Ma, Z.; Li, M.; Jin, X.; Gao, J. Experimental and Numerical Observation of Oil-Feeding Temperature on Lubrication States. Tribology 2024, 44, 40–48. [Google Scholar] [CrossRef]
- Jeng, Y.R.; Gao, C.C. Investigation of the ball-bearing temperature rise under an oil-air lubrication system. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2001, 215, 139–148. [Google Scholar] [CrossRef]
- Li, L.; Liu, S.G.; Wang, J.L. The Research on Oil-Air Lubrication and Oil Lubrication in the Sliding Bearing. Key Eng. Mater. 2013, 572, 393–396. [Google Scholar] [CrossRef]
- Lee, J.-H.; Lee, J.-H.; Yang, S.-H. Thermal Error Modeling of a Horizontal Machining Center Using Fuzzy Logic Strategy. J. Manuf. Process. 2001, 3, 120–127. [Google Scholar] [CrossRef]
- Yang, J.; Yuan, J.; Ni, J. Thermal error mode analysis and robust modeling for error compensation on a CNC turning center. Int. J. Mach. Tools Manuf. 1999, 39, 1367–1381. [Google Scholar] [CrossRef]
- Meng, H.C.; Ludema, K.C. Wear models and predictive equations: Their form and content. Wear 1995, 181–183, 443–457. [Google Scholar] [CrossRef]
- Jiang, S.; Mao, H. Investigation of the High Speed Rolling Bearing Temperature Rise With Oil-Air Lubrication. J. Tribol. 2011, 133, 021101. [Google Scholar] [CrossRef]
- Kostal, D.; Sperka, P.; Svoboda, P.; Krupka, I.; Hartl, M. Influence of Lubricant Inlet Film Thickness on Elastohydrodynamically Lubricated Contact Starvation. J. Tribol. 2017, 139, 051503. [Google Scholar] [CrossRef]
- Li, X.M.; Guo, F.; Wong, P.L.; Zhao, Y. Regulation of lubricant supply by wettability gradient in rolling EHL contacts. Tribol. Int. 2018, 120, 565–574. [Google Scholar] [CrossRef]
- van Emden, E.; Venner, C.H.; Morales-Espejel, G.E. Aspects of flow and cavitation around an EHL contact. Tribol. Int. 2016, 95, 435–448. [Google Scholar] [CrossRef]
- Nogi, T.; Shiomi, H.; Matsuoka, N. Starved Elastohydrodynamic Lubrication With Reflow in Elliptical Contacts. J. Tribol. 2017, 140, 011501. [Google Scholar] [CrossRef]
- Nogi, T. Film Thickness and Rolling Resistance in Starved Elastohydrodynamic Lubrication of Point Contacts with Reflow. J. Tribol. 2015, 137, 041502. [Google Scholar] [CrossRef]
- Chan, R.T.F.; Martinez-Botas, R.; Gohar, R.J.L.S. Isoviscous flow past a rigid sphere partially immersed in a thin oil film. Lubr. Sci. 2007, 19, 197–212. [Google Scholar] [CrossRef]
- Pemberton, J.; Cameron, A. A mechanism of fluid replenishment in elastohydrodynamic contacts. Wear 1976, 37, 185–190. [Google Scholar] [CrossRef]
- Riva, E.; Del Col, D. Numerical Simulation of Laminar Liquid Film Condensation in a Horizontal Circular Minichannel. J. Heat Transf. 2012, 134, 051019. [Google Scholar] [CrossRef]
- Chiu, Y.P. An Analysis and Prediction of Lubricant Film Starvation in Rolling Contact Systems. ASLE Trans. 1974, 17, 22–35. [Google Scholar] [CrossRef]
- Lin, Y.; Hu, Z.; Xiong, C.; Zang, M.; Jia, Y.; Chen, Y.; Luo, D.; Zhao, F. Research of Flow Field Simulation for Lubrication System and Effect Evaluation on a 7-Speed Dual Clutch Transmission. In Proceedings of the FISITA 2012 World Automotive Congress; Springer: Berlin/Heidelberg, Germany, 2013; pp. 285–298. [Google Scholar]
- Zeng, Q.; Zhang, J.; Hong, J.; Liu, C. A comparative study on simulation and experiment of oil-air lubrication unit for high speed bearing. Ind. Lubr. Tribol. 2016, 68, 325–335. [Google Scholar] [CrossRef]
- Liu, C.; Tong, B.; Zhang, G.; Xu, P.m.; Wang, W.; Liu, K. Effect of air supply speed on point contact sliding wear characteristics under oil-air lubrication conditions. Lubr. Sci. 2019, 31, 273–284. [Google Scholar] [CrossRef]
- Szydlo, Z. Effective oil/air ratio in industrial oil mist lubricating systems. Ind. Lubr. Tribol. 2007, 59, 4–11. [Google Scholar] [CrossRef]
- ISO-3448; Industrial Liquid Lubricants—ISO Vicosity Classification. International Organization for Standardization: Geneva, Switzerland, 1992.
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]

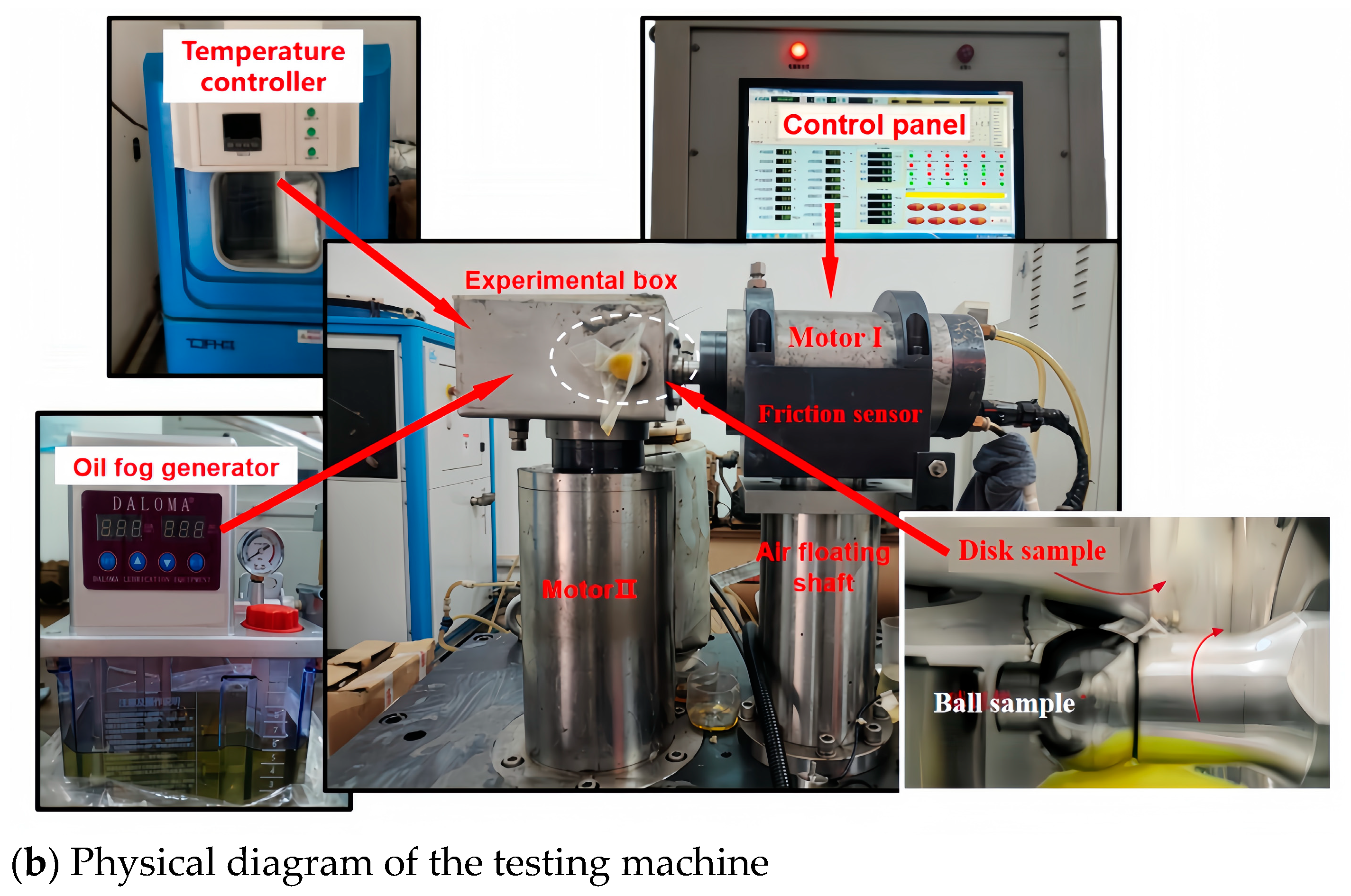

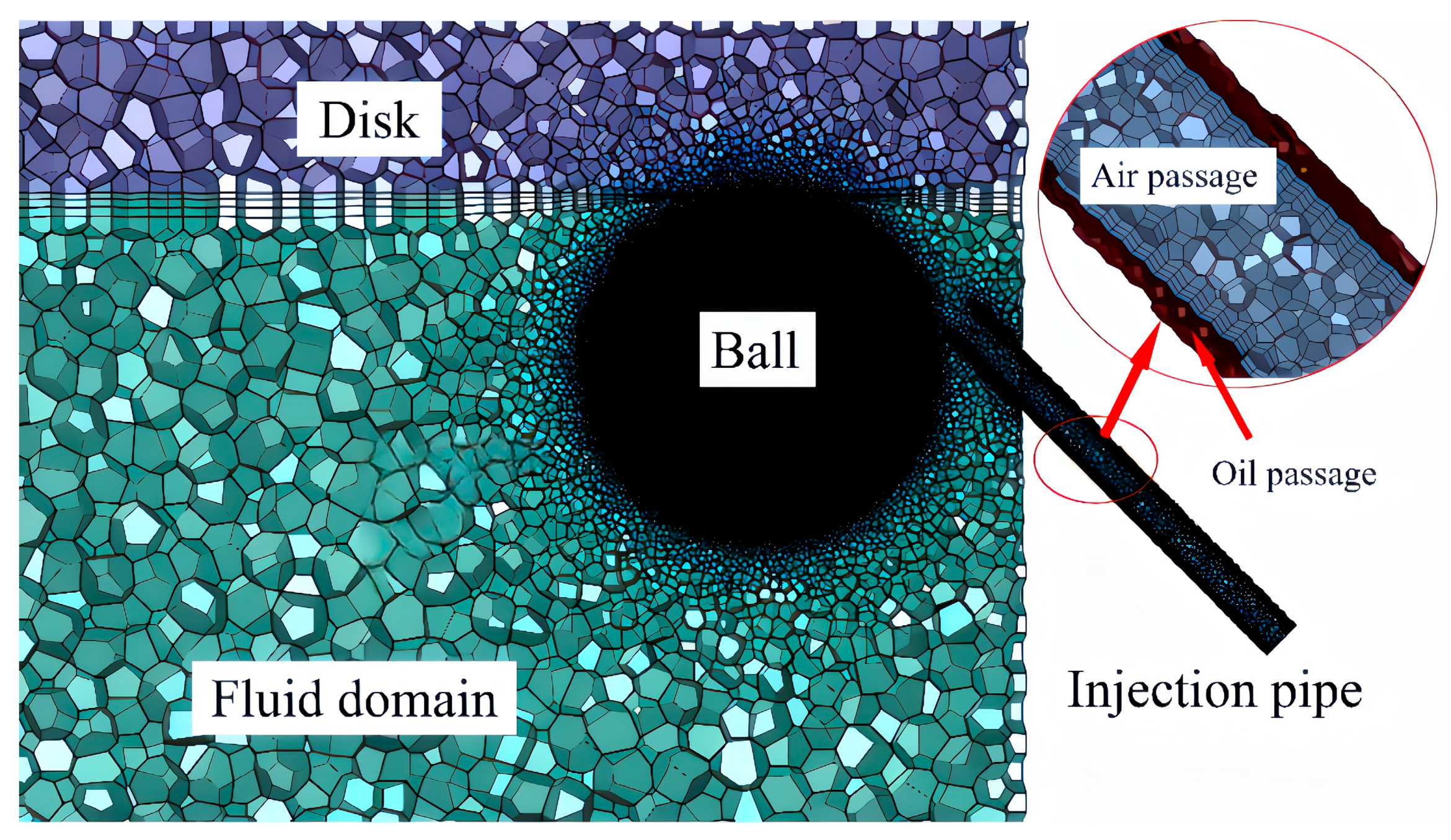
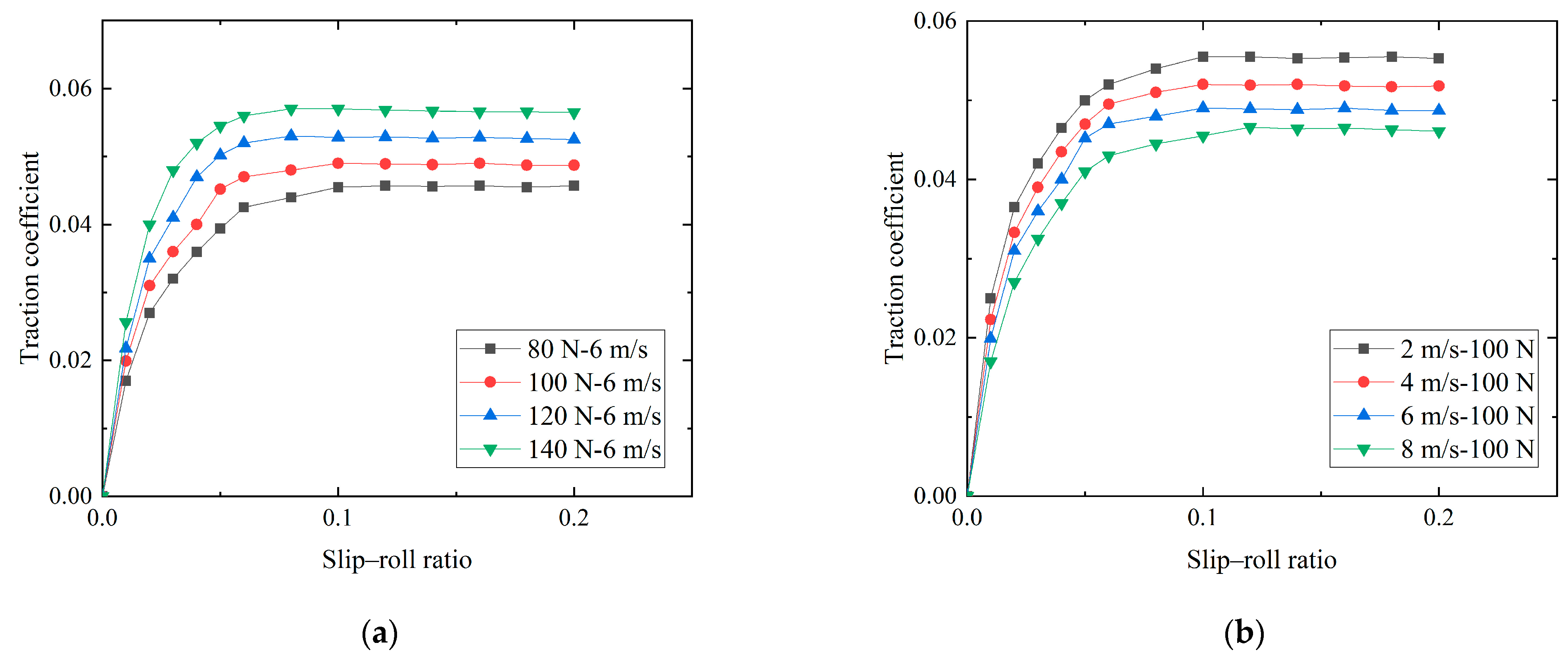
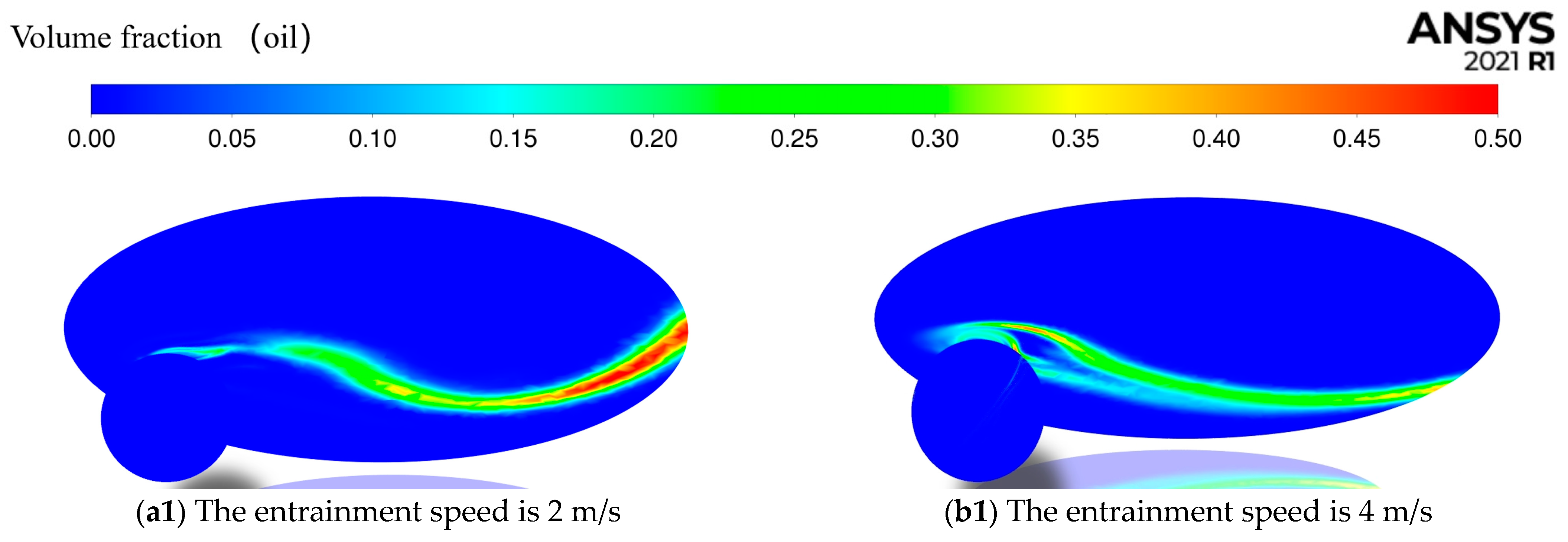


| Condition Parameter | Symbol | Range of Value |
|---|---|---|
| Entrainment speed of ball and disk contact area | 1~50 m/s | |
| Speed range of electric spindle I | 0~48,000 r/min | |
| Speed range of electric spindle II | 0~24,000 r/min | |
| Test temperature range | T | −175~150 °C |
| Slip–roll ratio range | S | 0~0.35 |
| Loading range | W1 | 0~500 N |
| Hertz contact stress range | W2 | 0~3.5 GPa |
| Phase | Parameter | Density | Dynamic Viscosity |
|---|---|---|---|
| air | 12 m/s | 1.128 kg/m3 | 1.7894 10−2 mPa·s |
| oil | 1.2 (6.0) mL/min | 865 kg/m3 | 4.325 mPa·s |
| Working Condition N-m/s | A | B | C | D | Residual Standard Deviation | Determination Coefficient |
|---|---|---|---|---|---|---|
| 80-2 | −0.0498 | −0.0001 | 44.1272 | 0.051 | 0.0009 | 0.9982 |
| 100-2 | −0.0539 | −0.001 | 50.6598 | 0.0551 | 0.0011 | 0.9979 |
| 120-2 | −0.0583 | 0 | 55.5239 | 0.0589 | 0.0008 | 0.9989 |
| 140-2 | −0.0634 | 0.5096 | 50.1188 | 0.0638 | 0.0006 | 0.9994 |
| 80-4 | −0.0481 | 0.2968 | 36.0932 | 0.0489 | 0.009 | 0.9982 |
| 100-4 | −0.051 | 0 | 49.1317 | 0.0518 | 0.0008 | 0.9988 |
| 120-4 | −0.0554 | 0.682 | 39.819 | 0.0557 | 0.0005 | 0.9995 |
| 140-4 | −0.0611 | 0.4426 | 54.5941 | 0.0609 | 0.0008 | 0.9991 |
| 80-6 | −0.0451 | 0 | 41.4334 | 0.0457 | 0.0007 | 0.9989 |
| 100-6 | −0.0484 | 0.0001 | 47.4736 | 0.049 | 0.0008 | 0.9985 |
| 120-6 | −0.0523 | 0.7195 | 36.8474 | 0.0522 | 0.0005 | 0.9995 |
| 140-6 | −0.0566 | 0.7457 | 45.6521 | 0.0566 | 0.0002 | 0.9999 |
| 80-8 | −0.0423 | 0.4791 | 24.6241 | 0.0425 | 0.0004 | 0.9997 |
| 100-8 | −0.0459 | −0.0001 | 41.8755 | 0.0466 | 0.0005 | 0.9994 |
| 120-8 | −0.0495 | 0.3292 | 39.7291 | 0.0498 | 0.0005 | 0.9993 |
| 140-8 | 0.0529 | 0 | 53.4028 | 0.0533 | 0.0004 | 0.9997 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, B.; Li, H.; Ren, J.; Gong, Z. Study on Traction Characteristics of Point Contact State under Oil–Air Lubrication. Lubricants 2024, 12, 299. https://doi.org/10.3390/lubricants12090299
Su B, Li H, Ren J, Gong Z. Study on Traction Characteristics of Point Contact State under Oil–Air Lubrication. Lubricants. 2024; 12(9):299. https://doi.org/10.3390/lubricants12090299
Chicago/Turabian StyleSu, Bing, Han Li, Jiongli Ren, and Zeyu Gong. 2024. "Study on Traction Characteristics of Point Contact State under Oil–Air Lubrication" Lubricants 12, no. 9: 299. https://doi.org/10.3390/lubricants12090299
APA StyleSu, B., Li, H., Ren, J., & Gong, Z. (2024). Study on Traction Characteristics of Point Contact State under Oil–Air Lubrication. Lubricants, 12(9), 299. https://doi.org/10.3390/lubricants12090299





