Theoretical Model Development and Mixed Lubrication Analyses of Rolling Piston Type Rotary Compressors: A Review
Abstract
1. Introduction
2. Model Development and Analyses
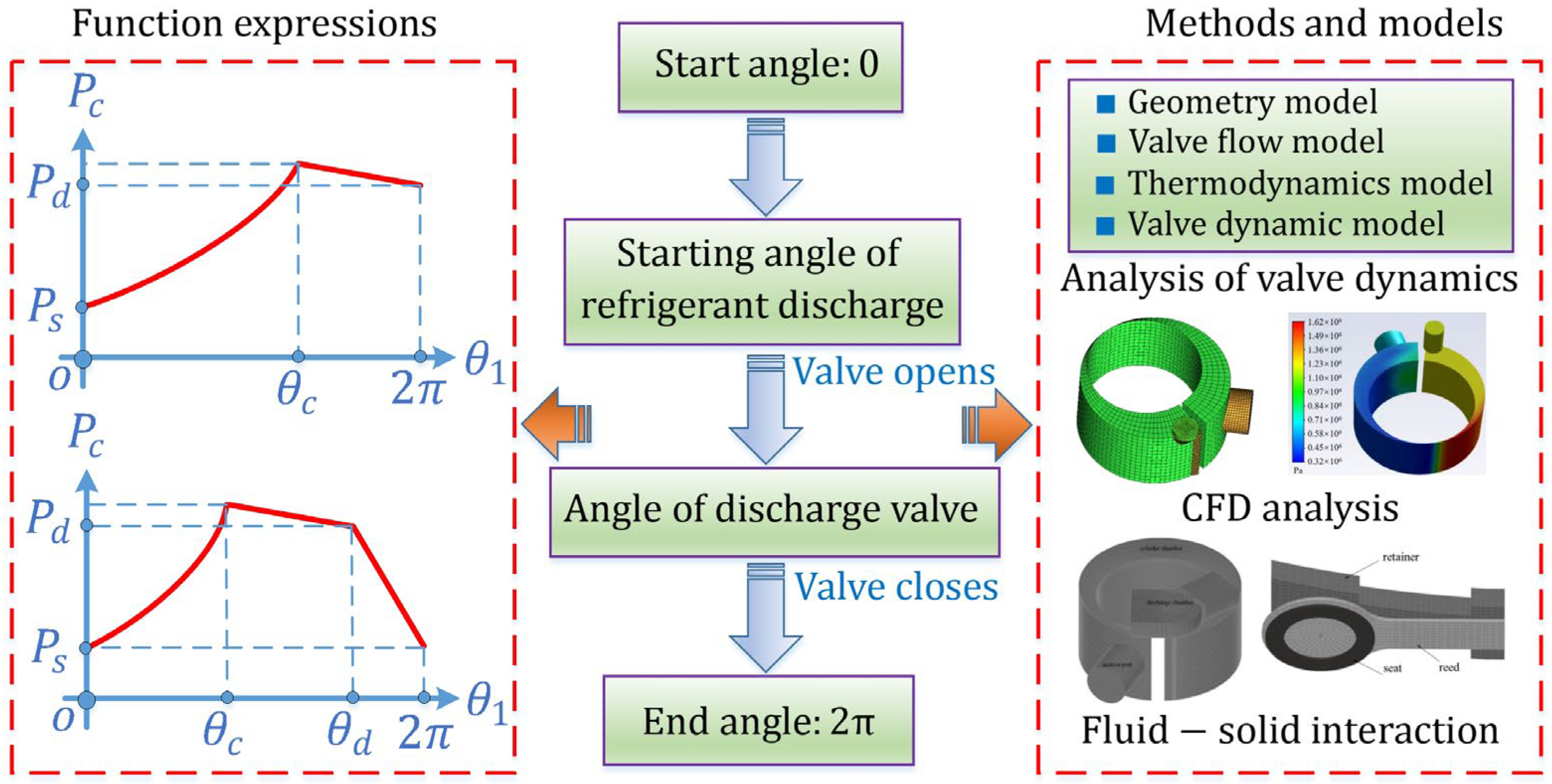
2.1. Determination of Compression Pressure
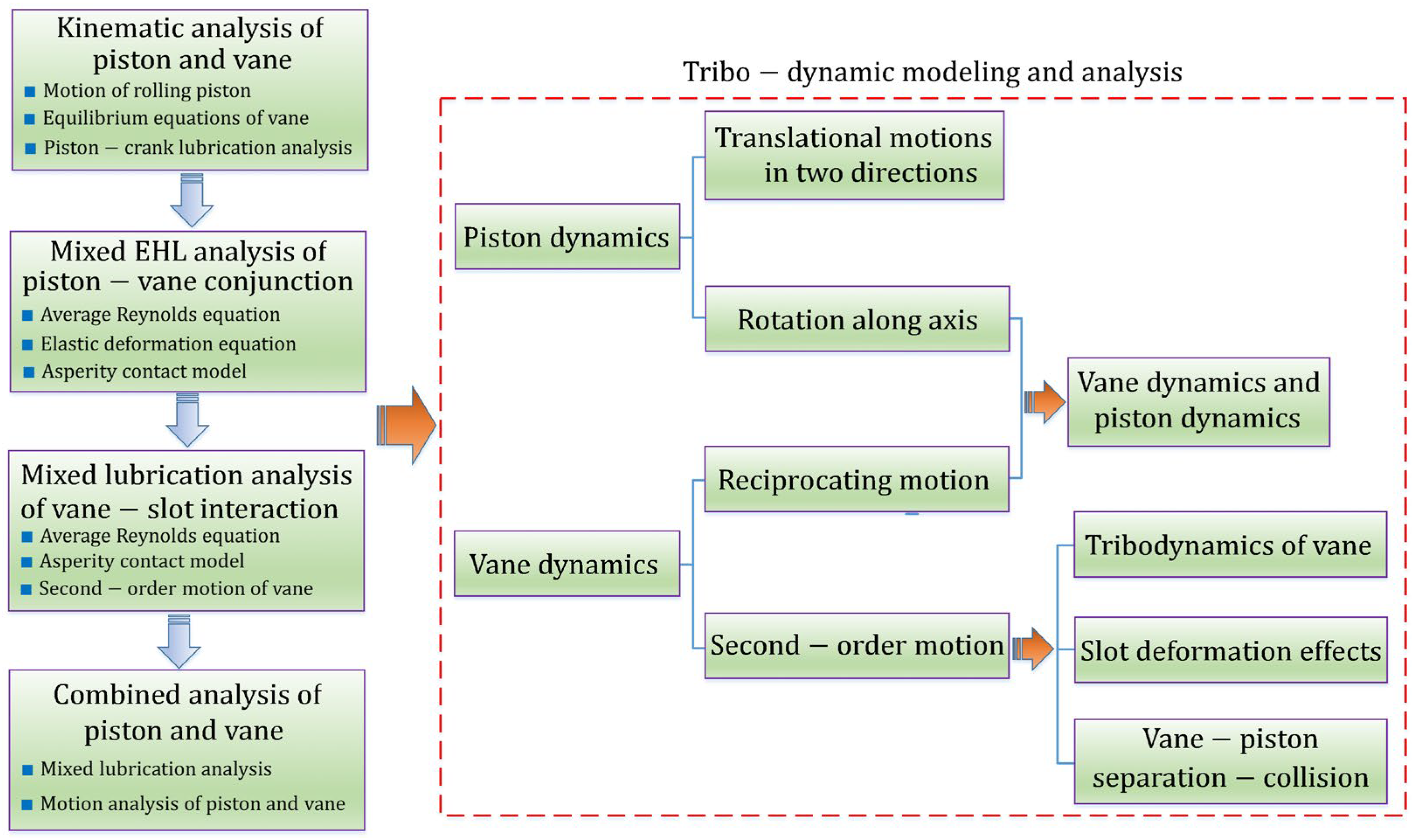
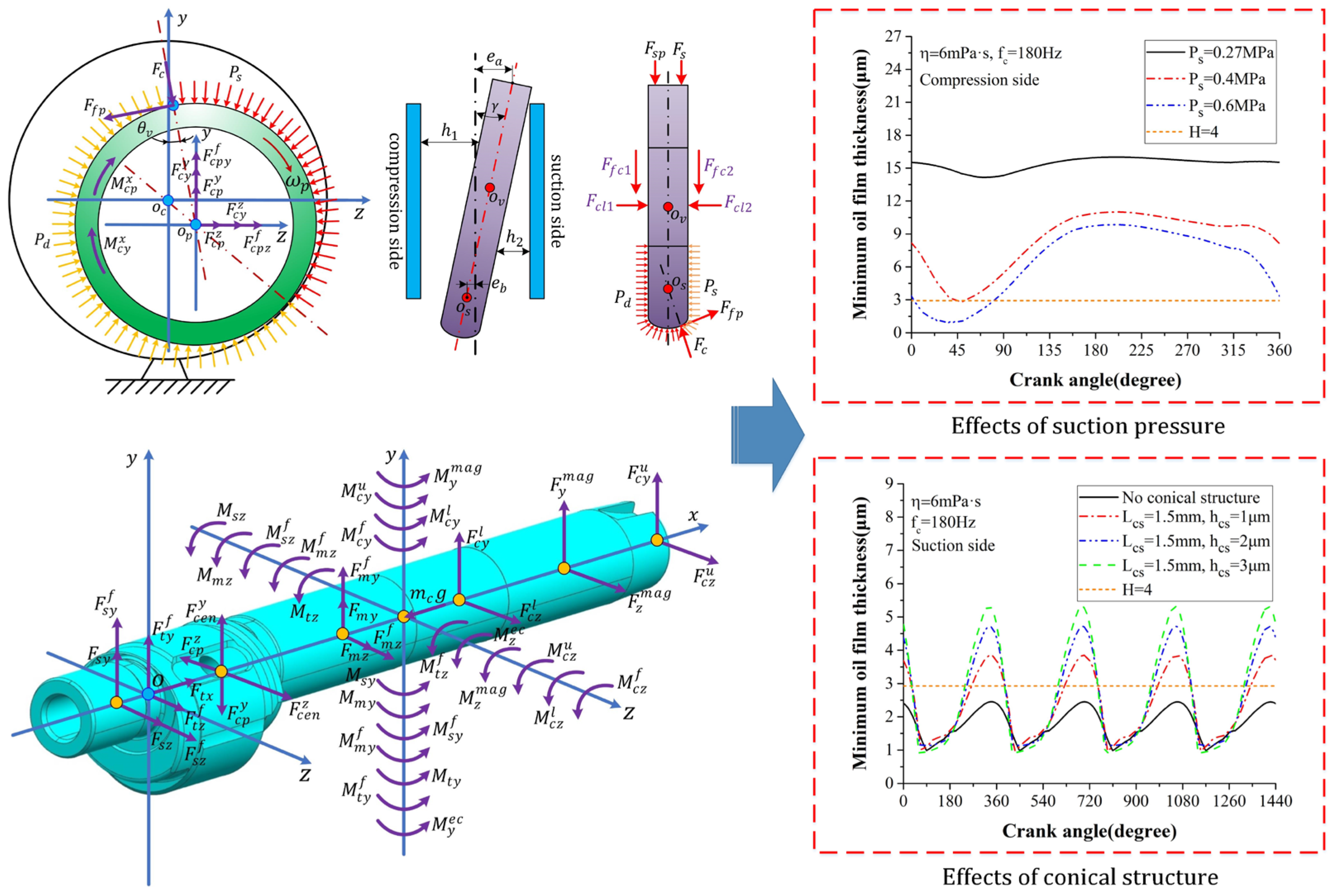
2.2. Sliding Vane and Rolling Piston
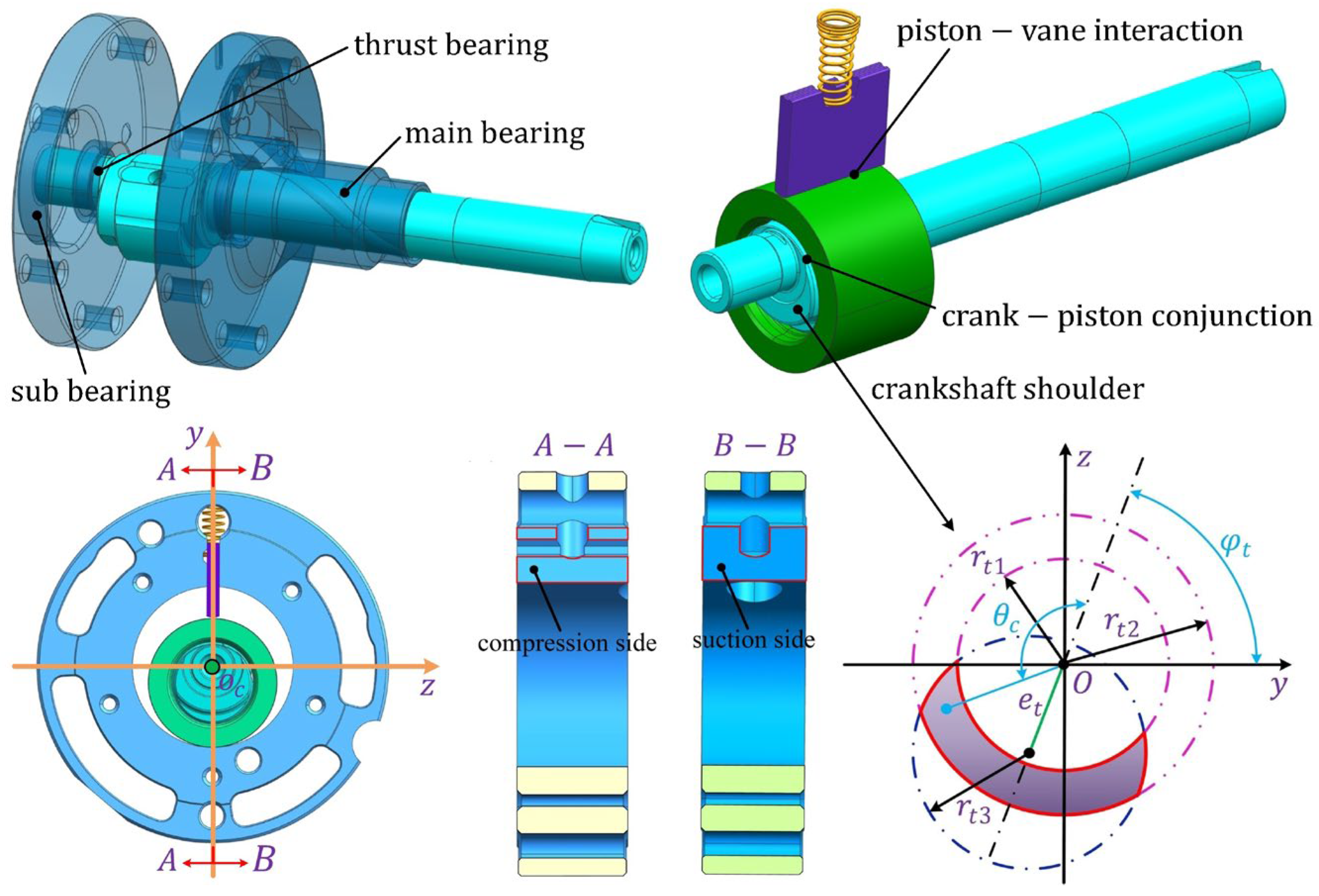
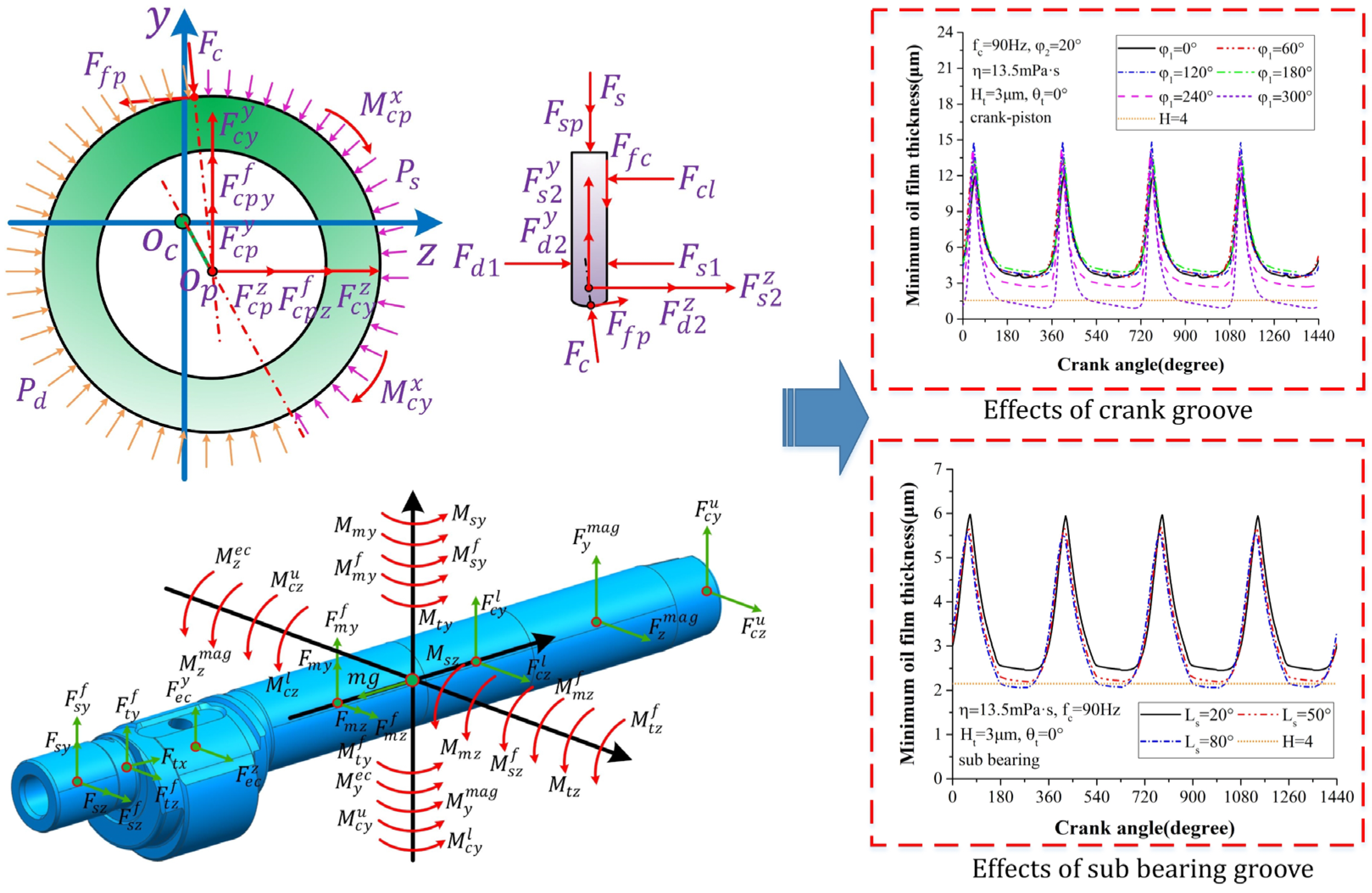
2.3. Crankshaft
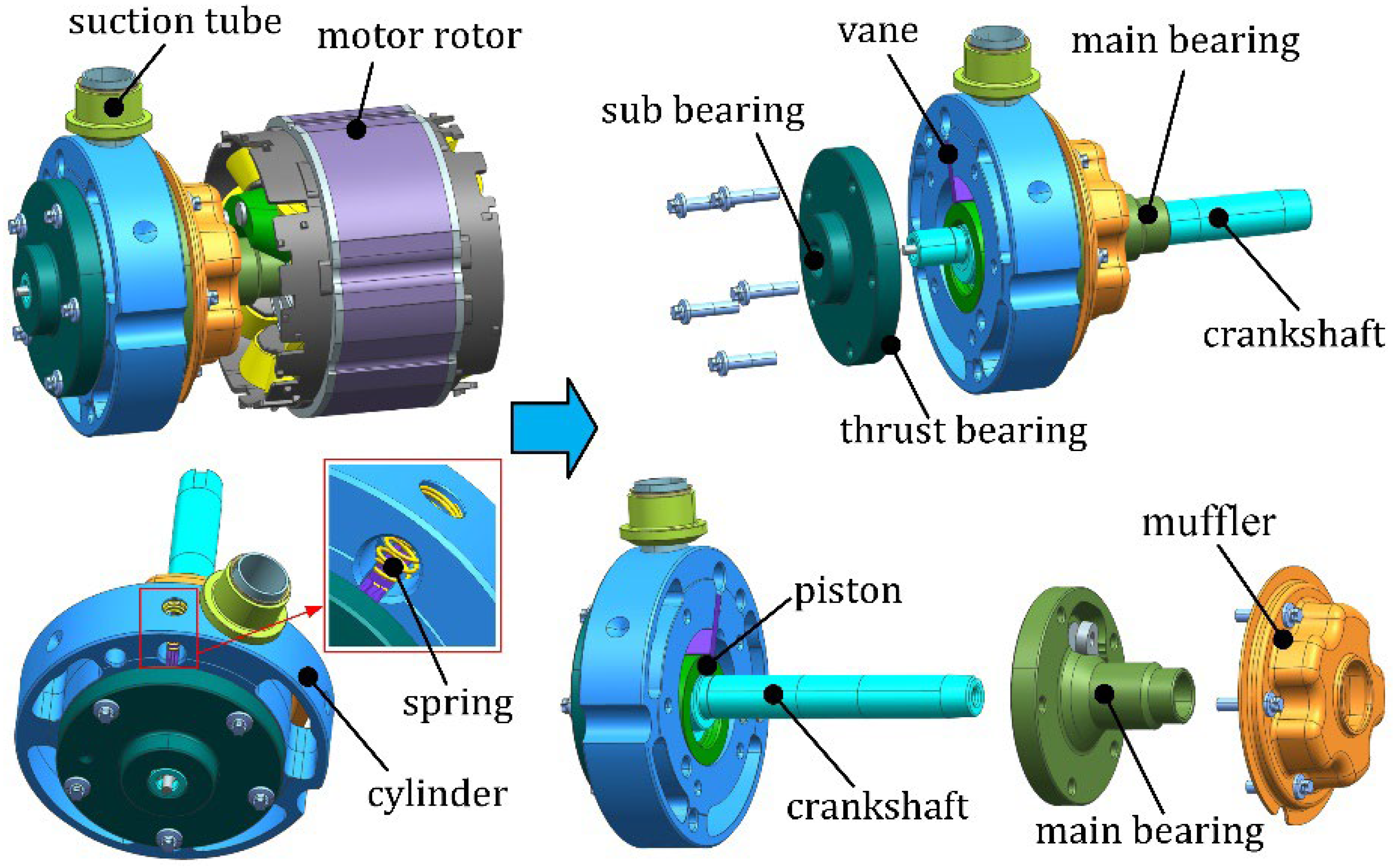
2.4. Whole Compressor
3. Influencing Factors and Problems
4. Conclusions
- (1)
- To provide the pressure boundary conditions, the three-segment function of the compression pressure is widely adopted in current models. To consider the effects of supercharging, thermodynamics, and valve dynamics, more comprehensive analyses of the compression process have been conducted. However, the corresponding methods and models are much more complex, limiting their applications in mixed lubrication analysis.
- (2)
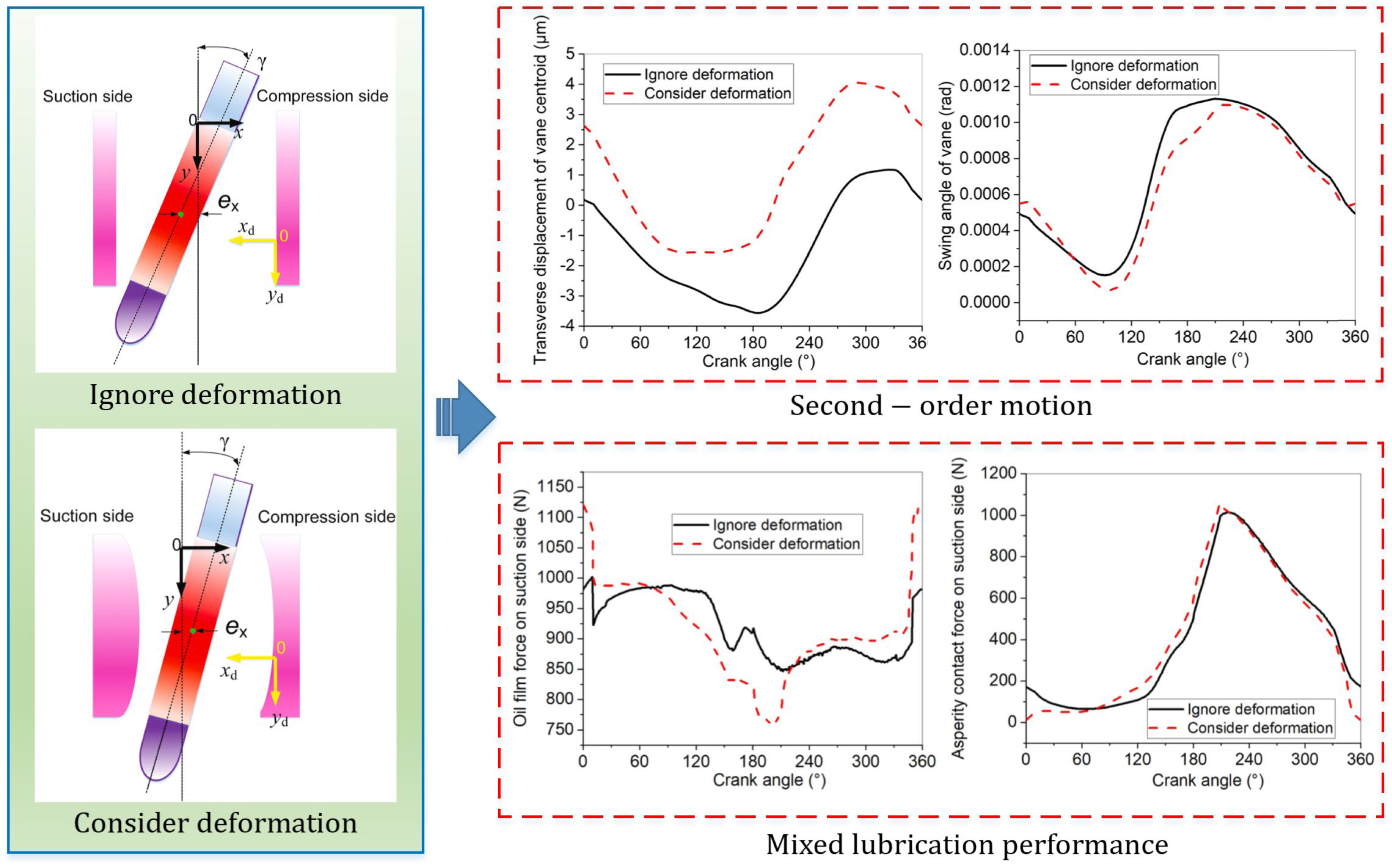
- Considering the disadvantages of the theoretical models of a part of the moving components or a part of the friction pairs, tribodynamic modeling of the whole compressor has been implemented in recent years. To conduct the modeling study of the vane–piston system and the crankshaft separately, decoupling of the moving components needs to be achieved, resulting in the extra assumptions. To improve decoupling model accuracy, second-order motion, elastic deformation, and groove distribution have been considered in the previous studies. With the increasing effects of multibody coupling, the modeling and analysis of the whole compressor is the preference.
- (3)
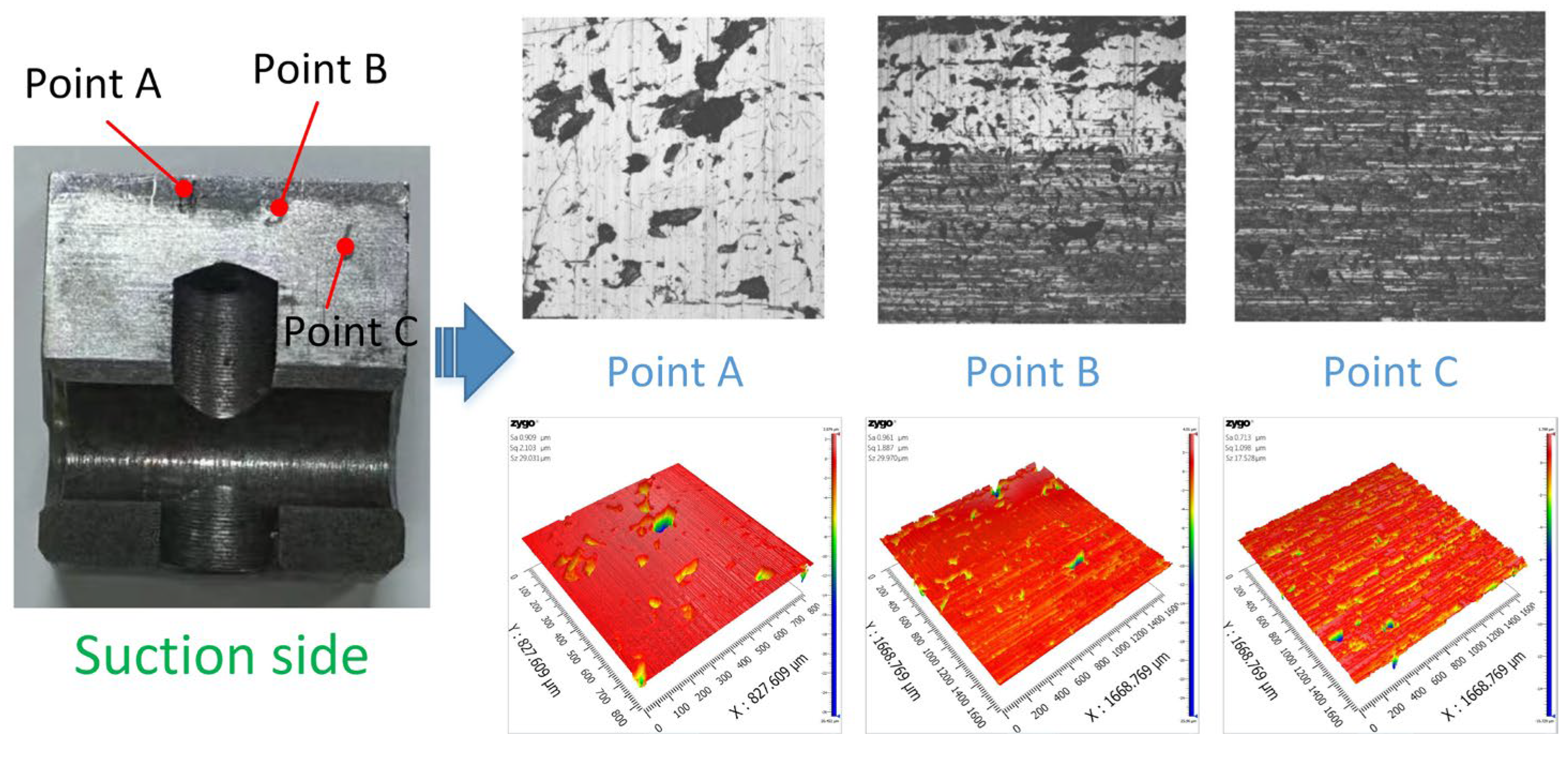
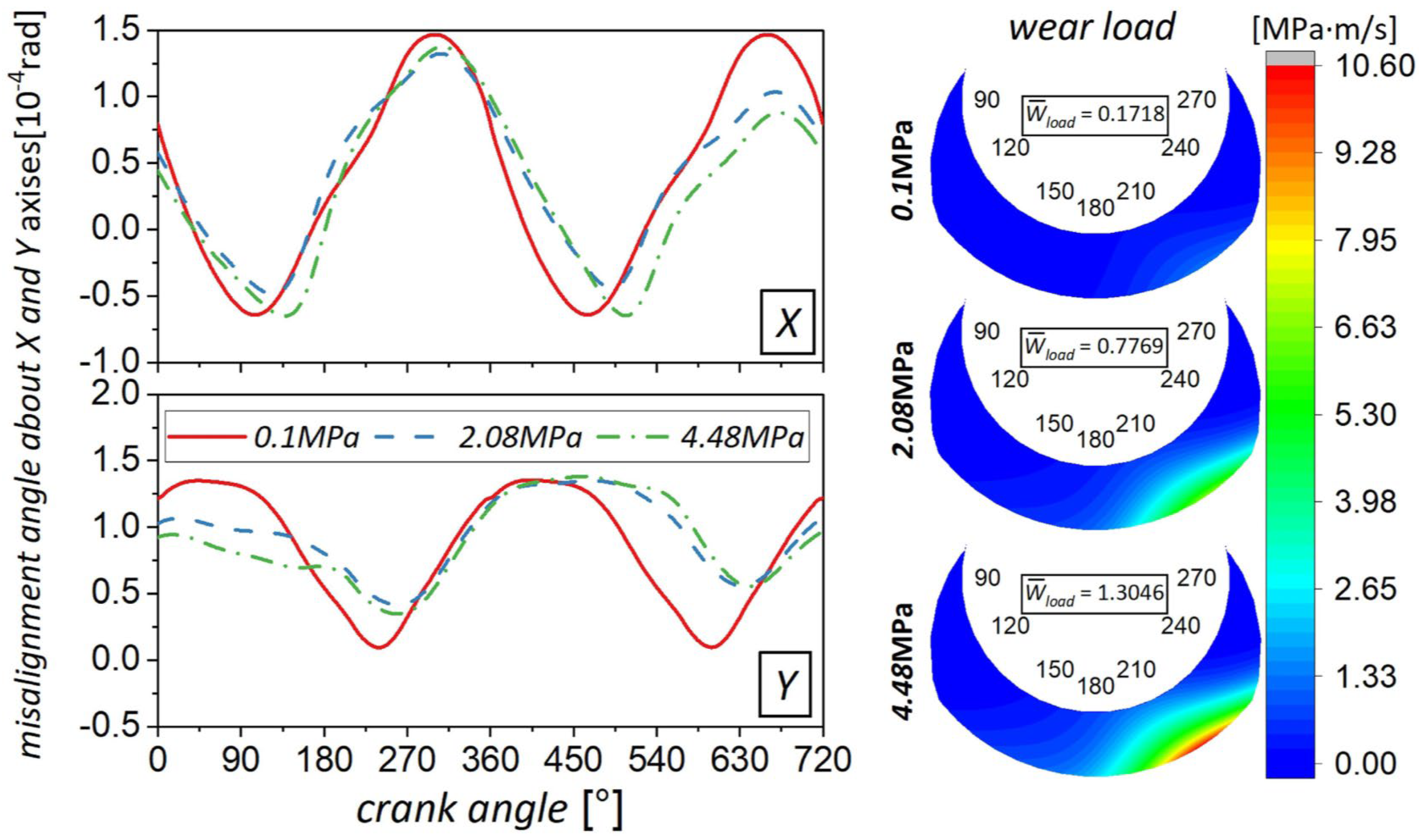
- Based on mixed lubrication analyses, various optimal designs of the compressor have been achieved. Optimized textures can greatly reduce the contact forces and wear of the thrust bearing under a high working frequency. An optimal vertical groove can reduce the friction loss and wear of the vane–slot conjunction. Proper conical structure on the vane slot of the suction side can greatly reduce the maximum wear load of the vane slot. In addition, optimally smoothed surface roughness can improve the ability of the lubrication film formation of journal bearings.
- (4)
- To reveal the service performance of the rotary compressor comprehensively, some important influencing factors need to be further analyzed in the future. Starved lubrication analysis of the compressor is full of challenges and has not been carried out so far. The coupling analysis of elastic deformation and multibody movements for the whole compressor needs to be addressed more. The thermal performance analysis of friction pairs under mixed lubrication regime is also very limited. During variable-speed processes, the lubrication and wear characteristics of the rotary compressor have not been investigated theoretically, which is also an important topic for the future study.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| correction coefficient | |
| compliance matrix value | |
| oil film thickness | |
| supplied lubricant film thickness | |
| oil film thickness at the inlet | |
| oil film pressure | |
| boundary pressure | |
| contact pressure | |
| compression pressure | |
| discharge pressure | |
| suction pressure | |
| oil supply flow rate | |
| lubricant flow rate | |
| journal radius | |
| time | |
| sliding speed | |
| compression chamber volume | |
| suction chamber volume | |
| position parameters | |
| inlet position | |
| cavity fraction | |
| elastic deformation | |
| grid area | |
| viscosity of lubricant | |
| starting angle of refrigerant discharge | |
| angle of discharge valve | |
| crank angle | |
| specific heat ratio of refrigerant gas | |
| density of the lubricant | |
| composite roughness | |
| contact factor | |
| shear flow factor | |
| pressure flow factors | |
| rotation speed |
References
- Wen, C.; Liu, Z.; Wen, X.; Gao, K.; Guan, J.; Bai, P.; Meng, Y.; Tian, Y. Dynamic modeling and characteristics analysis of sliding vane in a high-speed rotary compressor considering groove distribution and multi-body coupling. Tribol. Int. 2024, 194, 109505. [Google Scholar] [CrossRef]
- Wen, C.; Liu, Z.; Bai, P.; Guan, J.; Gao, K.; Wen, X.; Tian, Y. Modeling and performance analysis of rotary compressor considering multi-body coupling and bearing deformation. Tribol. Int. 2023, 189, 108981. [Google Scholar] [CrossRef]
- Aw, K.T.; Ooi, K.T. A Review on Sliding Vane and Rolling Piston Compressors. Machines 2021, 9, 125. [Google Scholar] [CrossRef]
- Wang, C.; Wu, J.; Du, Y.; Lei, B. Lubricating condition evaluation of the rotary compressor under high ambient temperature. Int. J. Refrig. 2020, 118, 220–229. [Google Scholar] [CrossRef]
- Pandeya, P.N.; Soedel, W. Rolling Piston Type Rotary Compressors with Special Attention to Friction and Leakage. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 19–21 July 1978. [Google Scholar]
- Wang, C.; Zhang, S.; Lei, B.; Cheng, J.; Wu, J. Analysis on influence factors of back pressure in an asymmetrical algebraic scroll compressor. Int. J. Refrig. 2022, 138, 97–107. [Google Scholar] [CrossRef]
- Cheng, S.; Feng, Y.; Wang, K.; Meng, X. Tribo-dynamics modeling and analysis of key friction pairs in scroll compressor with floating fixed scroll design. Eng. Appl. Comput. Fluid Mech. 2022, 16, 2270–2285. [Google Scholar] [CrossRef]
- Pont, A.; López, J.; Rigola, J.; Pérez-Segarra, C.D. Numerical dynamic analysis of reciprocating compressor mechanism. Parametric studies for optimization purposes. Tribol. Int. 2017, 105, 1–14. [Google Scholar] [CrossRef]
- Cho, J.R.; Moon, S.J. A numerical analysis of the interaction between the piston oil film and the component deformation in a reciprocating compressor. Tribol. Int. 2005, 38, 459–468. [Google Scholar] [CrossRef]
- Bianchi, G.; Cipollone, R. Theoretical modeling and experimental investigations for the improvement of the mechanical efficiency in sliding vane rotary compressors. Appl. Energy 2015, 142, 95–107. [Google Scholar] [CrossRef]
- Gu, H.; Ye, F.; Chen, Y.; Wu, J.; Li, W.; Sundén, B. Performance investigation and design optimization of novel rotating-cylinder sliding vane rotary compressors. Int. J. Refrig. 2022, 142, 137–147. [Google Scholar] [CrossRef]
- Meng, X.; Qi, Y.; Sheng, L.; Li, Q.; Su, L. Investigations on efficiency improvement of rolling piston type rotary compressor with a new-designed cylinder. Appl. Therm. Eng. 2023, 222, 119920. [Google Scholar] [CrossRef]
- Shiga, T.; Ishijima, K.; Sakainc, M.; Chu, I. Analysis of The Rolling-Piston Type Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 19–21 July 1978. [Google Scholar]
- Tanaka, H.; Ishijima, K. Noise and Efficiency of Rolling Piston Type Refrigeration Compressor for Household Refrigerator and Freazer. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 1 January 1980. [Google Scholar]
- Imaichi, K.; Fukushima, M.; Muramatsu, S.; Ishii, N. Vibration Analysis of Rotary Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 1 January 1982. [Google Scholar]
- Huang, Z.; Jiang, W.; Zhang, H.; Liu, C.; Jin, H.; Zhou, Y.; He, J. An effective experimental method for identifying radiated noise of different angular ranges for the rolling-piston compressor. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2008, 222, 2409–2417. [Google Scholar] [CrossRef]
- Wu, J.H.; Shi, H.Y.; Zhang, S.; Li, J.C.; Lei, B.W.; Du, Y.J. Experimental and numerical analysis of the performance of the R290 rotary compressor used in a split-room air conditioner at high ambient temperature. Sci. Technol. Built Environ. 2020, 27, 226–239. [Google Scholar] [CrossRef]
- Li, R.; Jing, L.; Meng, X.; Liu, Z.; Zhang, R. Numerical analysis of vane–slot friction pair in a rolling piston compressor considering deformation and groove design. Tribol. Int. 2021, 162, 107124. [Google Scholar] [CrossRef]
- Liu, R.; Jing, L.; Meng, X.; Lyu, B. Mixed elastohydrodynamic analysis of a coupled journal-thrust bearing system in a rotary compressor under high ambient pressure. Tribol. Int. 2021, 159, 106943. [Google Scholar] [CrossRef]
- Mi, J.; Meng, Y. Numerical Analyses of Hydrodynamic Lubrication and Dynamics of the Rolling Piston and Crankshaft in a Rotary Compressor. Tribol. Trans. 2014, 57, 1136–1147. [Google Scholar] [CrossRef]
- Padhy, S.K.; Scheldorf, G.O. Wear and Tribodynamics of a Rolling Piston Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 19–22 July 1994. [Google Scholar]
- Wu, J. Dynamic Analysis of Roller and Vane of Inverter Controlled Rotary Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- Lee, Y.-Z.; Oh, S.-D. Friction and wear of the rotary compressor vane–roller surfaces for several sliding conditions. Wear 2003, 255, 1168–1173. [Google Scholar] [CrossRef]
- Wu, J.; Shi, H.; Li, J. Analysis on the reliability of R290 rotary compressor in the high ambient temperature. Int. J. Refrig. 2020, 110, 132–141. [Google Scholar] [CrossRef]
- Ito, Y.; Hattori, H.; Miura, K. Lubricating characteristics and motion characteristics of a rolling piston for rotary compressors in air conditioners. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2014, 228, 984–996. [Google Scholar] [CrossRef]
- Lyu, B.; Jing, L.; Meng, X.; Liu, R. Texture Optimization and Verification for the Thrust Bearing Used in Rotary Compressors Based on a Transient Tribo-Dynamics Model. J. Tribol. 2022, 144, 1–19. [Google Scholar] [CrossRef]
- Yoshimura, T.; Ono, K.; Inagaki, K.; Kotsuka, H.; Korenaga, A. Analysis of Lubricating Cliaracteristics of Rotary Compressors for Domestic Refrigerators. Trans. ASME 1999, 121, 510–516. [Google Scholar] [CrossRef]
- Wu, J.; Chen, A. A new structure and theoretical analysis on leakage and performance of an oil-free R290 rolling piston compressor. Int. J. Refrig. 2015, 49, 110–118. [Google Scholar] [CrossRef]
- Wu, J.; Hu, J.; Chen, A.; Mei, P.; Zhou, X.; Chen, Z. Numerical analysis of temperature distribution of motor-refrigerant in a R32 rotary compressor. Appl. Therm. Eng. 2016, 95, 365–373. [Google Scholar] [CrossRef]
- Gasche, J.L.; Ferreira, R.T.S.; Prata, A.T. Two-Phase Flow of Oil-Refrigerant Mixture Through the Radial Clearance in Rolling Piston Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- Cho, I.-S.; Oh, S.-H.; Jung, J.-Y. Lubrication Characteristics Between the Vane and the Rolling Piston in a Rotary Compressor Used for Refrigeration and Air-Conditioning Systems. KSME Int. J. 2001, 15, 562–568. [Google Scholar] [CrossRef]
- Ooi, K.T.; Wong, T.N.; Kwek, E.C. A Real Gas Simulation of a Refrigeration Compressor and its Performance Comparison for CFCs and Non-CFCs. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1992. [Google Scholar]
- Hwang, S.; Kim, K.; Lee, S.; Kim, J.; Park, S. Development of the high efficiency rolling piston type rotary compressor for alternative refrigerant R410a. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1998. [Google Scholar]
- Fang, X.; Lin, J.; Ma, X. Simulation study on compression characteristics of low GWP refrigerants in the cylinder of rotary compressors. Appl. Therm. Eng. 2021, 193, 117056. [Google Scholar] [CrossRef]
- Cai, D.; He, G.; Yokoyama, T.; Tian, Q.; Yang, X.; Pan, J. Simulation and comparison of leakage characteristics of R290 in rolling piston type rotary compressor. Int. J. Refrig. 2015, 53, 42–54. [Google Scholar] [CrossRef]
- Geng, K.; Yan, C.; Wei, W.; Shi, D.; Zheng, X.; Lei, Y. Numerical and experimental investigation of vane–piston separation–collision in variable-speed compressors. Int. J. Refrig. 2022, 139, 148–157. [Google Scholar] [CrossRef]
- Cho, I.-S.; Baek, I.-H.; Oh, S.-H.; Jung, J.-Y. Friction characteristics between vane and rolling piston in a rotary compressor used for refrigeration and air-conditioning systems. KSTLE Int. J. 2008, 9, 17–21. [Google Scholar]
- Jeon, H.-G.; Oh, S.-D.; Lee, Y.-Z. Friction and wear of the lubricated vane and roller materials in a carbon dioxide refrigerant. Wear 2009, 267, 1252–1256. [Google Scholar] [CrossRef]
- Ahn, H.J.; Han, D.C.; Hwang, I.S. A built-in bearing sensor to measure the shaft motion of a small rotary compressor for air conditioning. Tribol. Int. 2003, 36, 561–572. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Lubr. Technol. 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, M.S. Application of Average Flow Model to Lubrication Between Rough Sliding Surfaces. J. Lubr. Technol. 1979, 101, 220–229. [Google Scholar] [CrossRef]
- Gu, C.; Meng, X.; Xie, Y.; Zhang, D. Mixed lubrication problems in the presence of textures: An efficient solution to the cavitation problem with consideration of roughness effects. Tribol. Int. 2016, 103, 516–528. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Tripp, J.H. The contact of two nominally flat rough surfaces. Proc. Inst. Mech. Eng. 1970, 185, 625–634. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. A finite element based elastic-plastic model for the contact of rough surfaces. Tribol. Trans. 2003, 46, 383–390. [Google Scholar] [CrossRef]
- Wen, C.; Meng, X.; Xie, Y.; Liu, R.; Kong, X.; Li, R.; Liu, Z.; Fang, C. Online measurement of piston-assembly friction with wireless IMEP method under fired conditions and comparison with numerical analysis. Measurement 2021, 174, 109009. [Google Scholar] [CrossRef]
- Gu, C.; Meng, X.; Xie, Y.; Kong, X. Performance of Surface Texturing During Start-Up Under Starved and Mixed Lubrication. J. Tribol. 2017, 139, 011702. [Google Scholar] [CrossRef]
- Jakobsson, B.; Floberg, L. The finite journal bearing considering vaporization. Trans. Chalmers Univ. Technol. 1957, 190, 1–116. [Google Scholar]
- Olsson, K. Cavitation in dynamically loaded bearings. Trans. Chalmers Univ. Technol. 1965, 308, 1–60. [Google Scholar]
- Zhang, J.; Meng, Y. Direct Observation of Cavitation Phenomenon and Hydrodynamic Lubrication Analysis of Textured Surfaces. Tribol. Lett. 2012, 46, 147–158. [Google Scholar] [CrossRef]
- Lyu, B.; Meng, X.; Zhang, R.; Wen, C. A deterministic contact evolution and scuffing failure analysis considering lubrication deterioration due to temperature rise under heavy loads. Eng. Fail. Anal. 2021, 123, 105276. [Google Scholar] [CrossRef]
- Almqvist, A. Homogenization of the Reynolds equation governing hydrodynamic flow in a rotating device. J. Tribol. 2011, 133, 021705. [Google Scholar] [CrossRef]
- Almqvist, A.; Fabricius, J.; Spencer, A.; Wall, P. Similarities and differences between the flow factor method by Patir and Cheng and homogenization. J. Tribol. 2011, 133, 031702. [Google Scholar] [CrossRef]
- Ito, Y.; Hattori, H.; Miura, K.; Hirayama, T. Mixed Lubrication Analysis of Vane Sliding Surface in Rotary Compressor Mechanisms. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 17–20 July 2006. [Google Scholar]
- Hattori, H.; Kawashima, N. Dynamic Analysis of a Rotor-Journal Bearing System for Twin Rotary Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 17–20 July 1990. [Google Scholar]
- Mi, J.; Meng, Y. THD Analysis of Rolling Piston and Journal Bearings in Rotary Compressors. Tribol. Trans. 2016, 59, 195–207. [Google Scholar] [CrossRef]
- Liu, Y.; Kosco, J. Vane Dynamics Analysis of a Tilted Vane Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1998. [Google Scholar]
- Okada, K.; Kuyama, K. Motion of Rolling Piston in Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 1 January 1982; pp. 178–184. [Google Scholar]
- Yanagisawa, T.; Shimizu, T.; Chu, I.; Ishijima, K. Motion Analysis of Rolling Piston in Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 1 January 1982. [Google Scholar]
- Tanaka, S.; Kyogoku, K.; Nakahara, T. Lubrication Characteristics of Refrigerating/Air Conditioning Rotary Compressor: Mixed Lubrication Analysis on Vane Tip. Jpn. J. Tribol. 1996, 41, 253–268. [Google Scholar]
- Liu, Z.; Soedel, W. Performance Study of a Variable Speed Compressor with Special Attention to Supercharging Effect. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 19–22 July 1994. [Google Scholar]
- Deng, W.-J.; Zhang, Y.-l.; Zhu, Z.-P.; Yue, X.-J.; Ba, D.-C.; Che, S.-G. Fluid–solid coupling numerical simulation for the performance prediction and valve dynamic analysis of a rotary compressor. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 6928–6938. [Google Scholar] [CrossRef]
- Liu, Z.; Soedel, W. Using a Gas Dynamic Model to Predict the Supercharging Phenomenon in a Variable Speed Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 19–22 July 1994. [Google Scholar]
- Geng, W.; Liu, C.; Wang, Y. The Performance Optimization of Rolling Piston Compressors Based on CFD Simulation. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 2004. [Google Scholar]
- Liu, C.H.; Geng, W. Research on Suction Performance of Two-Cylinder Rolling Piston Type Rotary Compressors Based on CFD Simulation. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 2004. [Google Scholar]
- Guo, N.; Lin, J.; Wu, J. Simulation on two-phase refrigerant compression in the cylinder of rotary compressors using CFD method. Sci. Rep. 2024, 14, 6075. [Google Scholar] [CrossRef] [PubMed]
- Brancher, R.D.; Deschamps, C.J. Modeling of Rolling-Piston Compressors with Special Attention to the Suction and Discharge Processes. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 2014. [Google Scholar]
- Ooi, K.T.; Wong, T.N. A computer simulation of a rotary compressor for household refrigerators. Appl. Therm. Eng. 1996, 17, 65–78. [Google Scholar] [CrossRef]
- Chae, H.M.; Kim, C.N. A numerical study with FSI mode on the characteristics of pressure fluctuation and discharge valve motion in rotary compressors with single and dual muffler. Int. J. Precis. Eng. Manuf. 2010, 11, 589–596. [Google Scholar] [CrossRef]
- Tan, Q.; Pan, S.-l.; Feng, Q.-k.; Yu, X.-l.; Wang, Z.-l. Fluid–structure interaction model of dynamic behavior of the discharge valve in a rotary compressor. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2014, 229, 280–289. [Google Scholar] [CrossRef]
- Yu, X.; Tan, Q.; Ren, Y.; Jia, X.; Jin, L. Numerical Study of the Reed Valve Impact in the Rotary Compressor by FSI Model. Energy Procedia 2017, 105, 4890–4897. [Google Scholar] [CrossRef]
- Ito, Y.; Hattori, H.; Miura, K. Numerical Analysis for Rotating Motion of a Rolling Piston in Rotary Compressors—Effective Factors for Characteristics of Rotating Motion of a Rolling Piston. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 2010. [Google Scholar]
- Xu, J.; Yu, B.; Yang, O.X.; Ding, S.P.; Zhao, H.H. Research on motion and friction of rolling piston in rotary compressor. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1180, 012047. [Google Scholar] [CrossRef]
- Tanaka, S.; Nakahara, T.; Kyogoku, K. Mixed Lubrication Analysis of Vane Tip in Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- Cho, I.-S.; Jung, J.-Y. The influence of vane on the lubrication characteristics between the vane and rolling piston of a rotary compressor. J. Mech. Sci. Technol. 2006, 20, 2242–2249. [Google Scholar] [CrossRef]
- Yang, Z.; Guo, H.; Jiang, B.; Zhu, B. Numerical Analysis of Friction power and Wear of Vane in Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 9–12 July 2018. [Google Scholar]
- Ito, Y.; Hattori, H.; Miura, K. Mixed Lubrication Analysis of Vane Sliding Surface in Rotary Compressor Mechanisms- Influences of Elastic Deformation at Surface End of Vane Slot. Tribol. Online 2009, 4, 96–102. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Shimizu, T. Friction losses in rolling piston type rotary compressors. Int. J. Refrig. 1985, 8, 159–165. [Google Scholar] [CrossRef]
- Padhy, S.K. On the Dynamics of a Rotary Compressor: Part 1-Mathematical Modeling. Adv. Des. Autom. 1993, 65, 207–217. [Google Scholar]
- Minami, K.; Hattori, H.; Hayano, M. Lubrication Analysis of Rotary Compressors for HFC Refrigerants. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1998. [Google Scholar]
- Geng, K.; Geng, A.; Wang, X.; Zheng, X.; Wei, W.; Zhao, T.; Lei, Y.; He, Y. Frictional characteristics of the vane–chute pair in a rolling piston compressor based on the second-order motion. Tribol. Int. 2019, 133, 111–125. [Google Scholar] [CrossRef]
- Padhy, S.K. On the dynamics of a rotary compressor: Part 2- Experimental validation and sensitivity analysis. Adv. Des. Autom. 1993, 65, 219–227. [Google Scholar]
- Lin, J.; Guo, N.; Hong, L.; Wu, J.; Jiang, J.; Zhao, T. Simulation of single and two-phase refrigerant compression in rotary compressors. Appl. Therm. Eng. 2022, 211, 118465. [Google Scholar] [CrossRef]
- Zhang, A.; Sun, H. Dynamics Analysis of a Rolling Rotor Compressor Considering the Electromagnetic Force. J. Comput. Electron. Inf. Manag. 2023, 10, 91–94. [Google Scholar] [CrossRef]
- Seve, F.; Berlioz, A.; Dufour, R.; Dufour, R.; Peyaud, F. Balancing of a Variable Speed Rotary Compressor: Experimental and Numerical Investigations. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- Wang, Z.; Yu, X.; Liu, F.; Feng, Q.; Tan, Q. Dynamic analyses for the rotor-journal bearing system of a variable speed rotary compressor. Int. J. Refrig. 2013, 36, 1938–1950. [Google Scholar] [CrossRef]
- Park, Y.C. Transient analysis of a variable speed rotary compressor. Energy Convers. Manag. 2010, 51, 277–287. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, J.; Xie, F.; Chen, A.; Li, Y. Dynamic behaviors of the crankshafts in single-cylinder and twin-cylinder rotary compressors. Int. J. Refrig. 2014, 47, 36–45. [Google Scholar] [CrossRef]
- Hirayama, T.; Ito, Y.; Shida, S.; Kawabe, I.; Hirano, K. Development of large capacity rotary compressor with three cylinders. First report: Prototype designs and their efficiencies. Int. J. Refrig. 2021, 130, 278–287. [Google Scholar] [CrossRef]
- Hirayama, T.; Shida, S.; Hirano, K.; Ito, Y.; Monasry, J.F. Development of large capacity rotary compressor with three cylinders. Second report: Vibration and sound. Int. J. Refrig. 2021, 130, 261–270. [Google Scholar] [CrossRef]
- Dufour, R.; Charreyron, M.; Gerard, M. Dynamics Prediction of Refrigerant Rotary Compressor Crankshaft. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1998. [Google Scholar]
- Fu, Y.; Zhou, X.; Guo, H.; Mei, P. Lubrication Analysis of Journal Bearings in R410A Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 11–14 July 2016. [Google Scholar]
- Yu, B.; Yang, O.; Xu, J.; Wei, H.; Zhao, H. Numerical Analysis and Optimization for Hydrodynamic Lubrication in Journal Bearings of Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 24–28 May 2021. [Google Scholar]
- Hirayama, T.; Miura, K.; Hattori, H.; Ito, Y. Numerical Analysis for Mixed Lubrication in Journal Bearings of Rotary Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 17–20 July 2006. [Google Scholar]
- Xie, F.; Zhang, H.; Wu, J.; Ma, W.; Liu, C. Dynamic Analysis of a Rotor-Journal Bearing System of Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 17–20 July 2006. [Google Scholar]
- Kitsunai, Y.; Matsui, M.; Oyagi, S. High Efficiency Development of a Rotary Compressor by Clarification of its Shaft Dynamic Motion. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 2010. [Google Scholar]
- Meier, A.M.; Tada, M.P.; Espindola, G. Compressor Friction Losses due to Oil Viscosity and Asperity Contact Surfaces: A numerical study on crankshaft bearings lengths, clearances and roughness parameters. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 9–12 July 2018. [Google Scholar]
- Jorgensen, S.H.; Nissen, H.S. Mechanical Loss Model of Rolling Piston Rotary Compressor with Special Importance Attached to Journal Bearing. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 11–13 July 1984. [Google Scholar]
- Yang, J. Mechanical Loss Analysis Of Inverter Controlled Two Cylinders Type Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 16–19 July 2002. [Google Scholar]
- Han, H.-S.; Hwang, S.-W. Vibration Analysis of a Rotary Compressor. Int. J. Precis. Eng. Manuf. 2004, 5, 43–53. [Google Scholar]
- Pinninti, R.R. Rotary Behavior of Crank Shaft and Piston of the Compressor Housing. Int. J. Adv. Eng. Res. Technol. 2014, 2, 359–367. [Google Scholar]
- Kima, H.J.; Lancey, T.W. Numerical study on the lubrication oil distribution in a refrigeration rotary compressor. Int. J. Refrig. 2003, 26, 800–808. [Google Scholar] [CrossRef]
- Padhy, S.K. Lubrication analysis of a rolling piston rotary compressor Part 1: Mathematical modelling. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 1994, 208, 83–94. [Google Scholar] [CrossRef]
- Padhy, S.K. Lubrication analysis of a rolling piston rotary compressor Part 2: Experimental validation and results. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 1994, 208, 95–104. [Google Scholar] [CrossRef]
- Chen, H.; Liang, H.; Wang, W.; Zhang, S. Investigation on the oil transfer behaviors and the air-oil interfacial flow patterns in a ball bearing under different capillary conditions. Friction 2022, 11, 228–245. [Google Scholar] [CrossRef]
- Yan, B.; Dong, L.; Yan, K.; Chen, F.; Zhu, Y.; Wang, D. Effects of oil-air lubrication methods on the internal fluid flow and heat dissipation of high-speed ball bearings. Mech. Syst. Signal Process. 2021, 151, 107409. [Google Scholar] [CrossRef]
- Wu, C.; Zheng, L. An Average Reynolds Equation Lubrication with a Contact Factor. Trans. ASME 1989, 111, 188–191. [Google Scholar] [CrossRef]
- Liu, R.; Meng, X.; Cui, Y. Influence of numerous start-ups and stops on tribological performance evolution of engine main bearings. Int. J. Engine Res. 2018, 21, 1362–1380. [Google Scholar] [CrossRef]
- Liu, Z.; Meng, X.; Wen, C.; Yu, S.; Zhou, Z. On the oil-gas-solid mixed bearing between compression ring and cylinder liner under starved lubrication and high boundary pressures. Tribol. Int. 2019, 140, 105869. [Google Scholar] [CrossRef]
- Gu, C.; Meng, X.; Xie, Y.; Yang, Y. Effects of surface texturing on ring/liner friction under starved lubrication. Tribol. Int. 2016, 94, 591–605. [Google Scholar] [CrossRef]
- Woodward, W.S.; Paul, B. Contact Stresses for Closely Conforming Bodies—Application to Cylinders and Spheres; U.S. Department of Transportation: Washington, DC, USA, 1976.
- Xiang, G.; Han, Y.; Wang, J.; Wang, J.; Ni, X. Coupling transient mixed lubrication and wear for journal bearing modeling. Tribol. Int. 2019, 138, 1–15. [Google Scholar] [CrossRef]
- Meng, Y.; Mi, J. Numerical analyses of THD lubrication and dynamics of rolling piston and bearings in a rotary compressor. In Positive Displacement Machines; Elsevier: Amsterdam, The Netherlands, 2019; pp. 225–261. [Google Scholar]
- Lin, J.; Lian, Y.; Wu, J. Numerical investigation on vapor-liquid two-phase compression in the cylinder of rotary compressors. Appl. Therm. Eng. 2020, 170, 115022. [Google Scholar] [CrossRef]
- Nagatomo, S.; Kato, S. Estimation of the Starting Torque of Refrigerant Rotary Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 1 January 1980. [Google Scholar]
- Yanagisawa, T.; Shimizu, T.; Horioka, T. A Study on Starting Characteristics of a Rolling Piston Type Rotary Compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 4–7 August 1986. [Google Scholar]
- Ishii, N.; Imaichi, K.; Muramatsu, S.; Fukushima, M.; Matsunaga, H. The Study of Rolling Piston, Rotary Compressor Dynamic Behavior When Stopping to Reduce Noise and Vibration Level. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 11–13 July 1984. [Google Scholar]
- Lin, J.; Wu, J.; Zhang, Z.; Chen, Z.; Xie, J.; Lu, J. Experimental investigation of startup characteristics of R290 rotary compressor under low ambient temperature heating condition. Int. J. Refrig. 2017, 77, 128–135. [Google Scholar] [CrossRef]
- Wu, J.; Lin, J.; Zhang, Z.; Chen, Z.; Xie, J.; Lu, J. Experimental investigation on cold startup characteristics of a rotary compressor in the R290 air-conditioning system under cooling condition. Int. J. Refrig. 2016, 65, 209–217. [Google Scholar] [CrossRef]
- Guo, N.; Lin, J.; Wu, J.; Zhao, Y. Transient thermal analysis of the rotary compressor during startup process. Int. J. Refrig. 2024, 157, 34–42. [Google Scholar] [CrossRef]
- Zhao, Y.; Du, Y.; Lin, J.; Guo, N.; Wu, J. Start-up characteristics of a R290 rotary compressor for an air source heat pump under low ambient temperature condition. Int. J. Refrig. 2024, 159, 385–394. [Google Scholar] [CrossRef]

| Type | Year | Reference | Research Content and Findings |
|---|---|---|---|
| 1 | 1978 | Pandeya et al. [5] |
|
| 1982 | Okada et al. [58] |
| |
| 1982 | Yanagisawa et al. [59] |
| |
| 2010 | Ito et al. [72] |
| |
| 2021 | Xu et al. [73] |
| |
| 2 | 1999 | Yoshimura et al. [27] |
|
| 2000 | Tanaka et al. [74] |
| |
| 2001 | Cho et al. [31] |
| |
| 2006 | Cho et al. [75] |
| |
| 3 | 2006 | Ito et al. [54] |
|
| 2009 | Ito et al. [77] |
| |
| 2018 | Yang et al. [76] |
| |
| 4 | 1985 | Yanagisawa et al. [78] |
|
| 2014 | Ito et al. [25] |
| |
| 5 | 1993 | Padhy [79] |
|
| 1998 | Minami et al. [80] |
| |
| 2019 | Geng et al. [81] |
| |
| 2021 | Li et al. [18] |
| |
| 2022 | Geng et al. [36] |
|
| Type | Year | Reference | Research Content and Findings |
|---|---|---|---|
| 1 | 1990 | Hattori et al. [55] |
|
| 1998 | Dufour et al. [91] |
| |
| 2000 | Seve et al. [85] |
| |
| 2023 | Zhang et al. [84] |
| |
| 2 | 2016 | Fu et al. [92] |
|
| 2021 | Yu et al. [93] |
| |
| 2006 | Hirayama et al. [94] |
| |
| 2006 | Xie et al. [95] |
| |
| 2010 | Kitsunai et al. [96] |
| |
| 2014 | Zhang et al. [88] |
| |
| 2018 | Meier et al. [97] |
| |
| 2020 | Wang et al. [4] |
| |
| 2021 | Liu et al. [19] |
| |
| 2022 | Lyu et al. [26] |
|
| Type | Year | Reference | Research Content and Findings |
|---|---|---|---|
| 1 | 1984 | Jorgensen et al. [98] |
|
| 2002 | Jun et al. [99] |
| |
| 2 | 2004 | Han et al. [100] |
|
| 2014 | Venugopal et al. [101] |
| |
| 3 | 2014 | Mi et al. [20] |
|
| 2016 | Mi et al. [56] |
| |
| 2023 | Wen et al. [2] |
| |
| 2024 | Wen et al. [1] |
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, C.; Bai, P.; Zhang, H.; Zhang, S.; Meng, X.; Meng, Y.; Tian, Y. Theoretical Model Development and Mixed Lubrication Analyses of Rolling Piston Type Rotary Compressors: A Review. Lubricants 2024, 12, 273. https://doi.org/10.3390/lubricants12080273
Wen C, Bai P, Zhang H, Zhang S, Meng X, Meng Y, Tian Y. Theoretical Model Development and Mixed Lubrication Analyses of Rolling Piston Type Rotary Compressors: A Review. Lubricants. 2024; 12(8):273. https://doi.org/10.3390/lubricants12080273
Chicago/Turabian StyleWen, Chengwei, Pengpeng Bai, Hang Zhang, Shuaijun Zhang, Xianghui Meng, Yonggang Meng, and Yu Tian. 2024. "Theoretical Model Development and Mixed Lubrication Analyses of Rolling Piston Type Rotary Compressors: A Review" Lubricants 12, no. 8: 273. https://doi.org/10.3390/lubricants12080273
APA StyleWen, C., Bai, P., Zhang, H., Zhang, S., Meng, X., Meng, Y., & Tian, Y. (2024). Theoretical Model Development and Mixed Lubrication Analyses of Rolling Piston Type Rotary Compressors: A Review. Lubricants, 12(8), 273. https://doi.org/10.3390/lubricants12080273