Heat and Mass Transformation of Casson Hybrid Nanofluid (MoS2 + ZnO) Based on Engine Oil over a Stretched Wall with Chemical Reaction and Thermo-Diffusion Effect
Abstract
1. Introduction
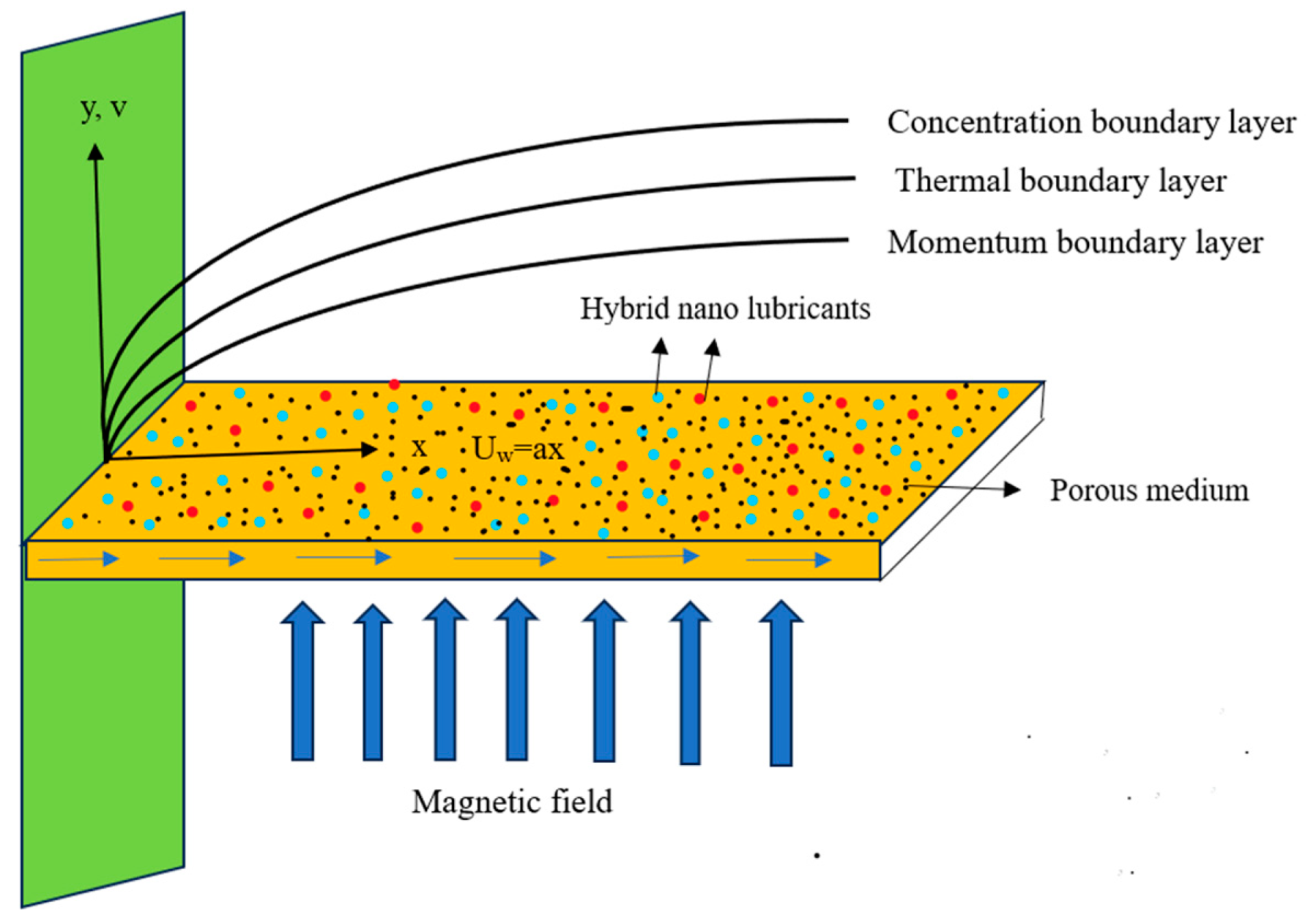
2. Materials and Methods
Similarity Variables
3. Numerical Procedure
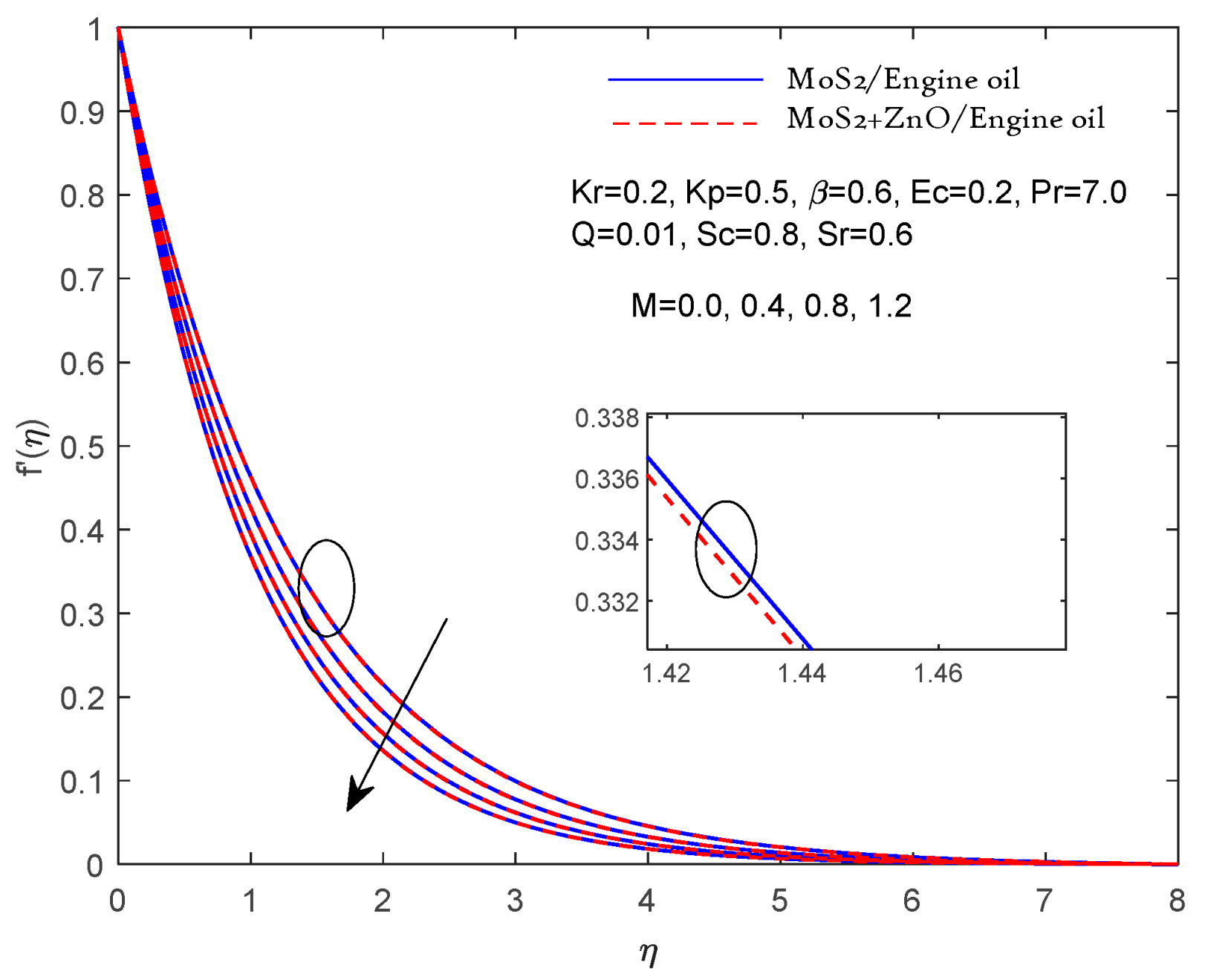
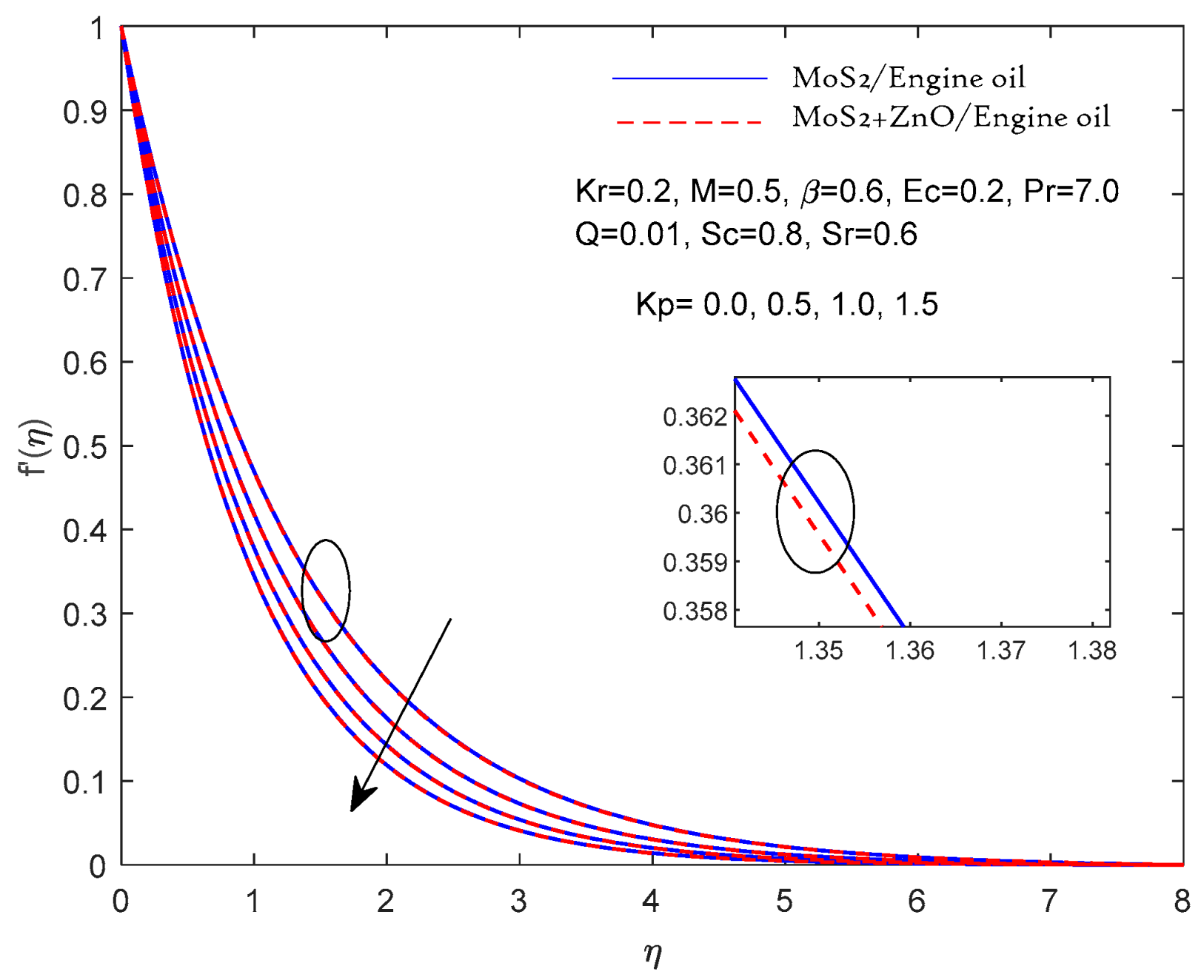
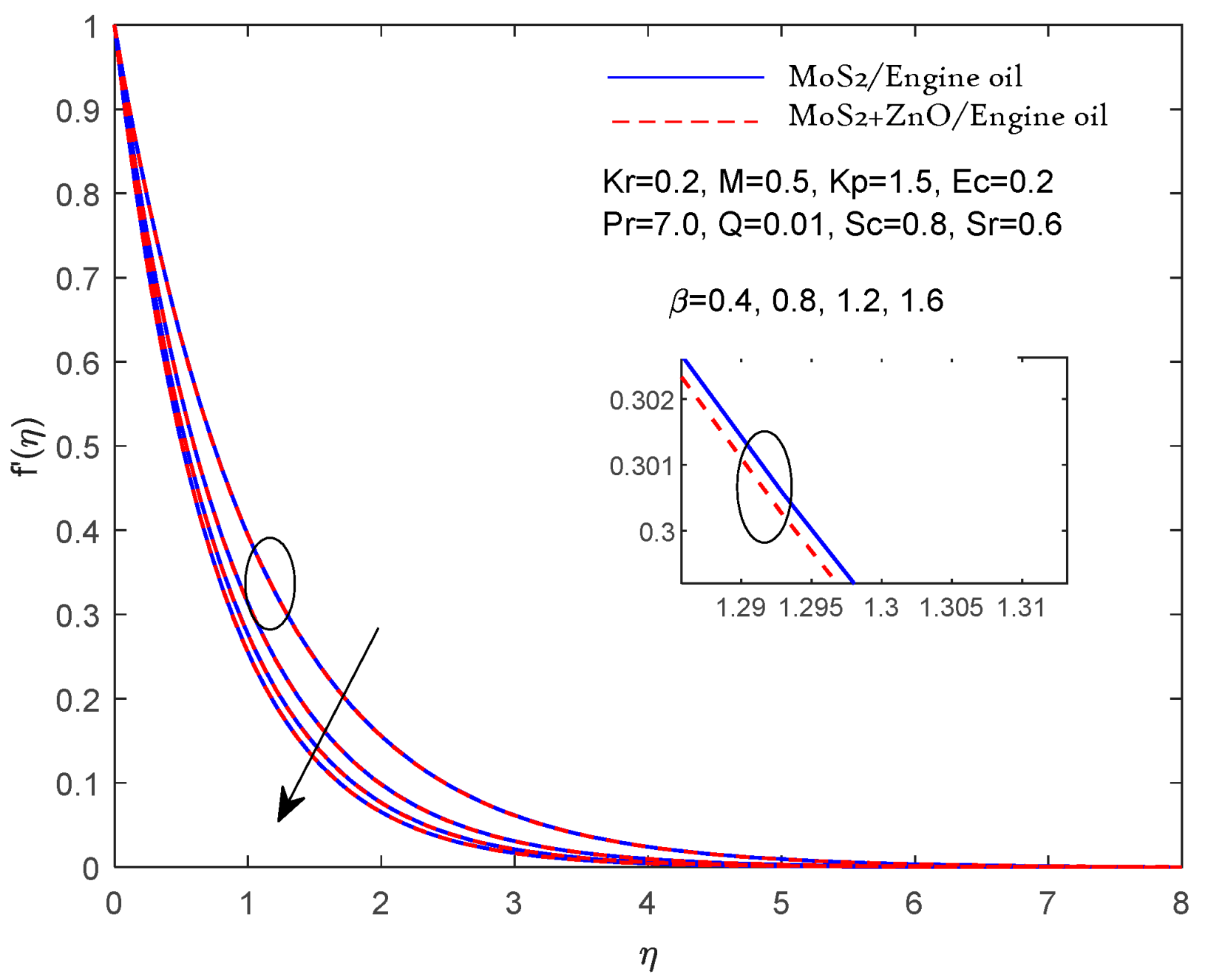
4. Results and Discussion
- (i)
- MoS2/engine oil (nanolubricant);
- (ii)
- ZnO + MoS2/engine oil (hybrid nanolubricant).
5. Conclusions
- The velocity profile is diminished against the values of M, Kp, and β;
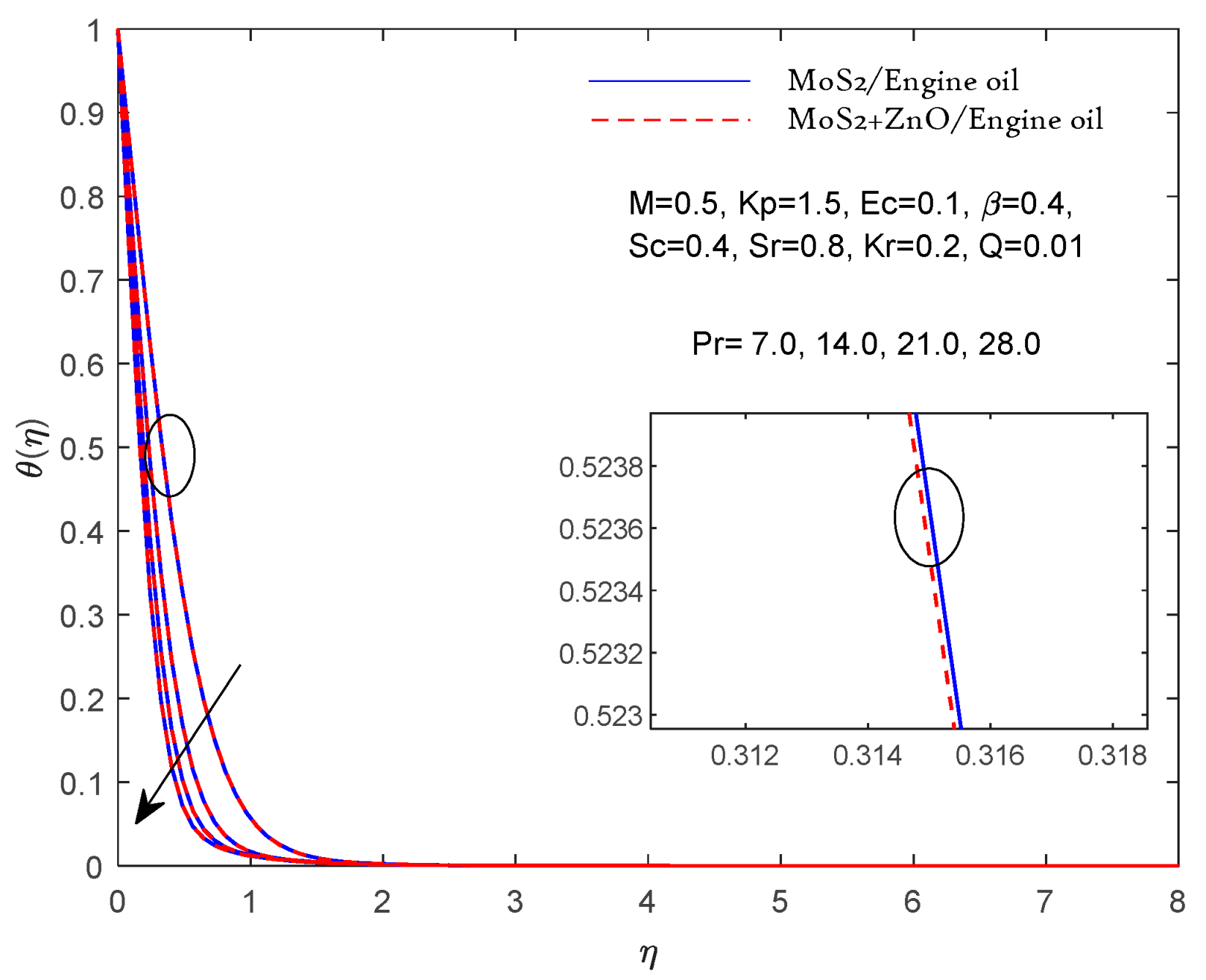
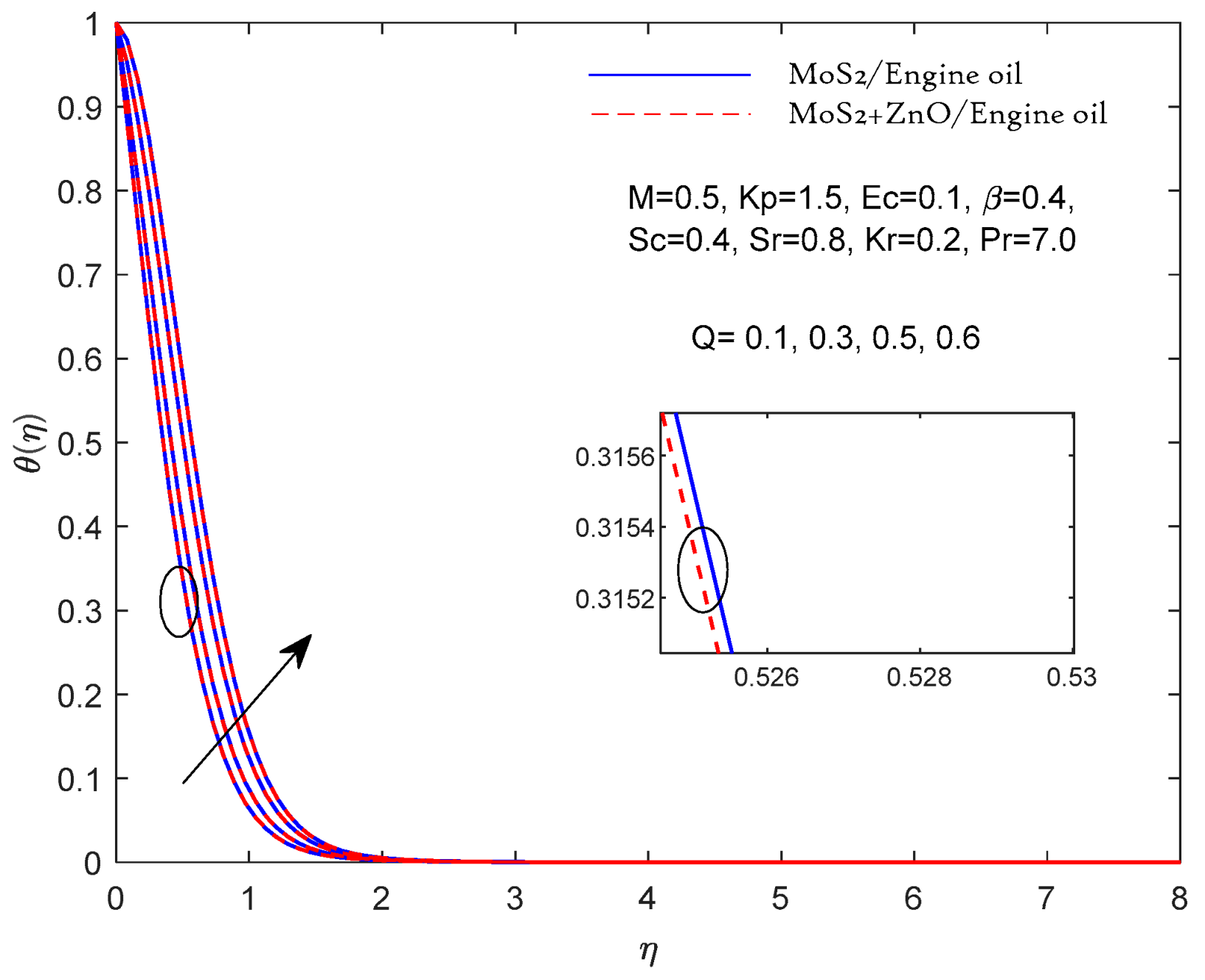
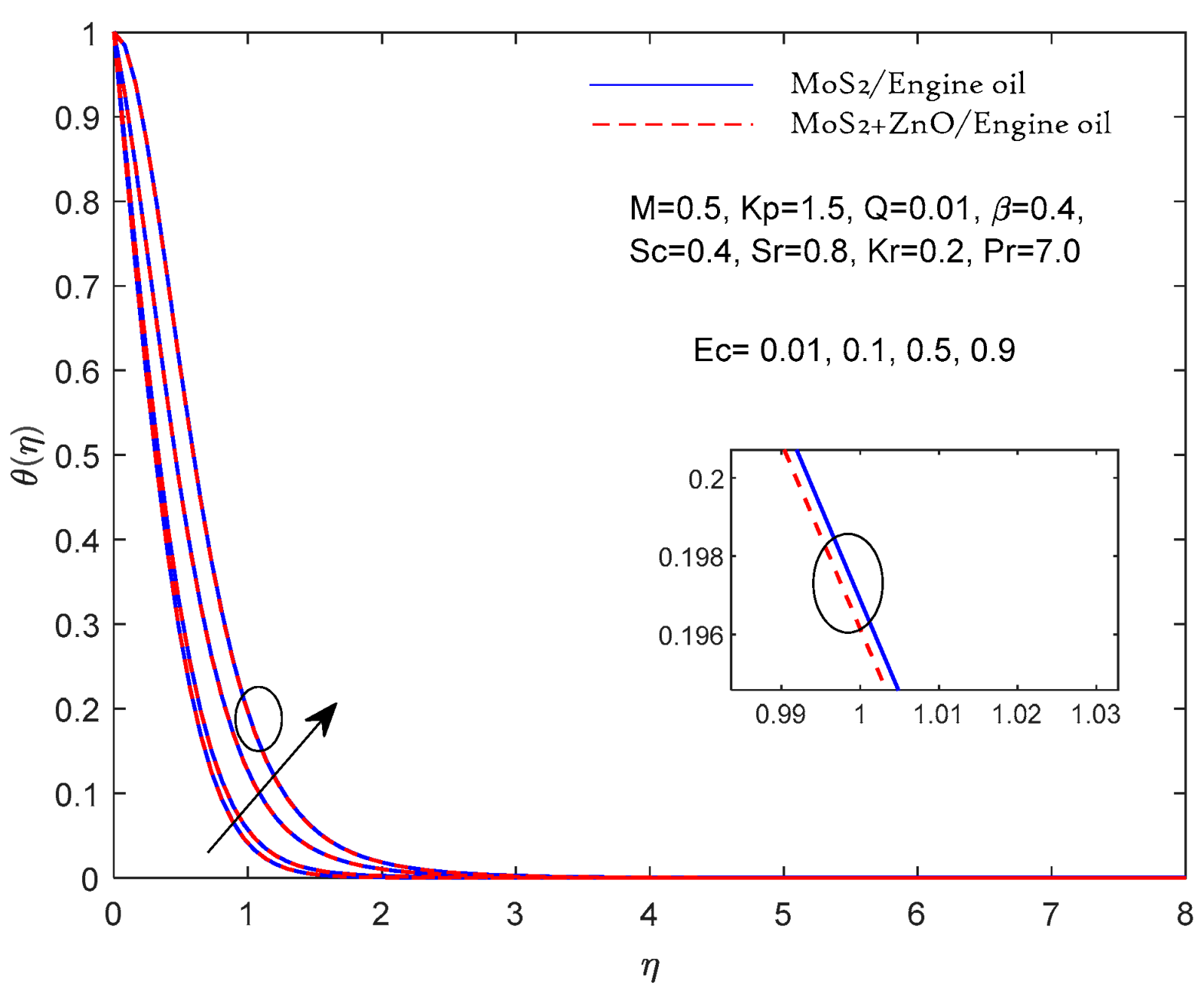
- The temperature profile rises in line with increased Ec and Q, and reciprocally goes down in line with Pr;
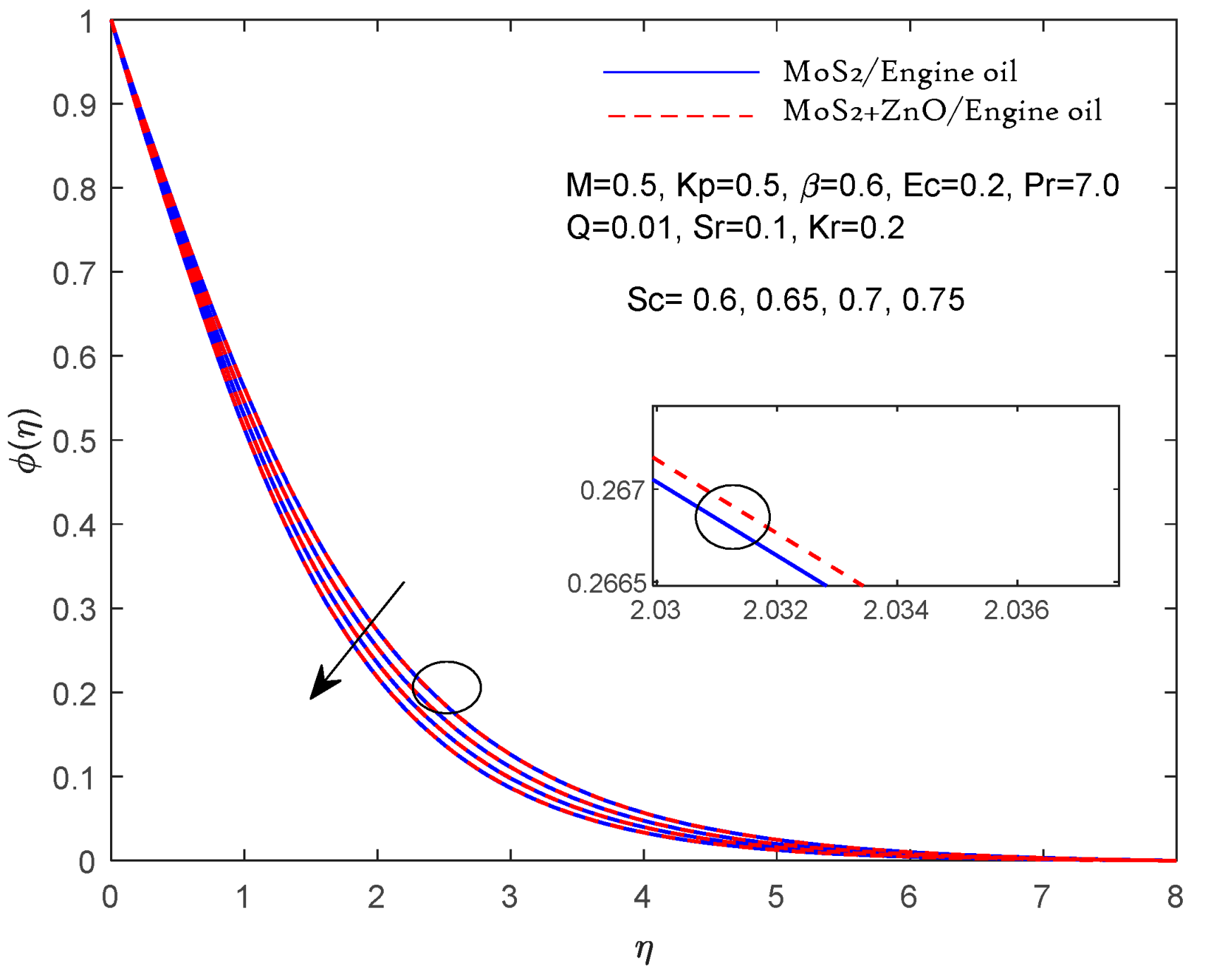
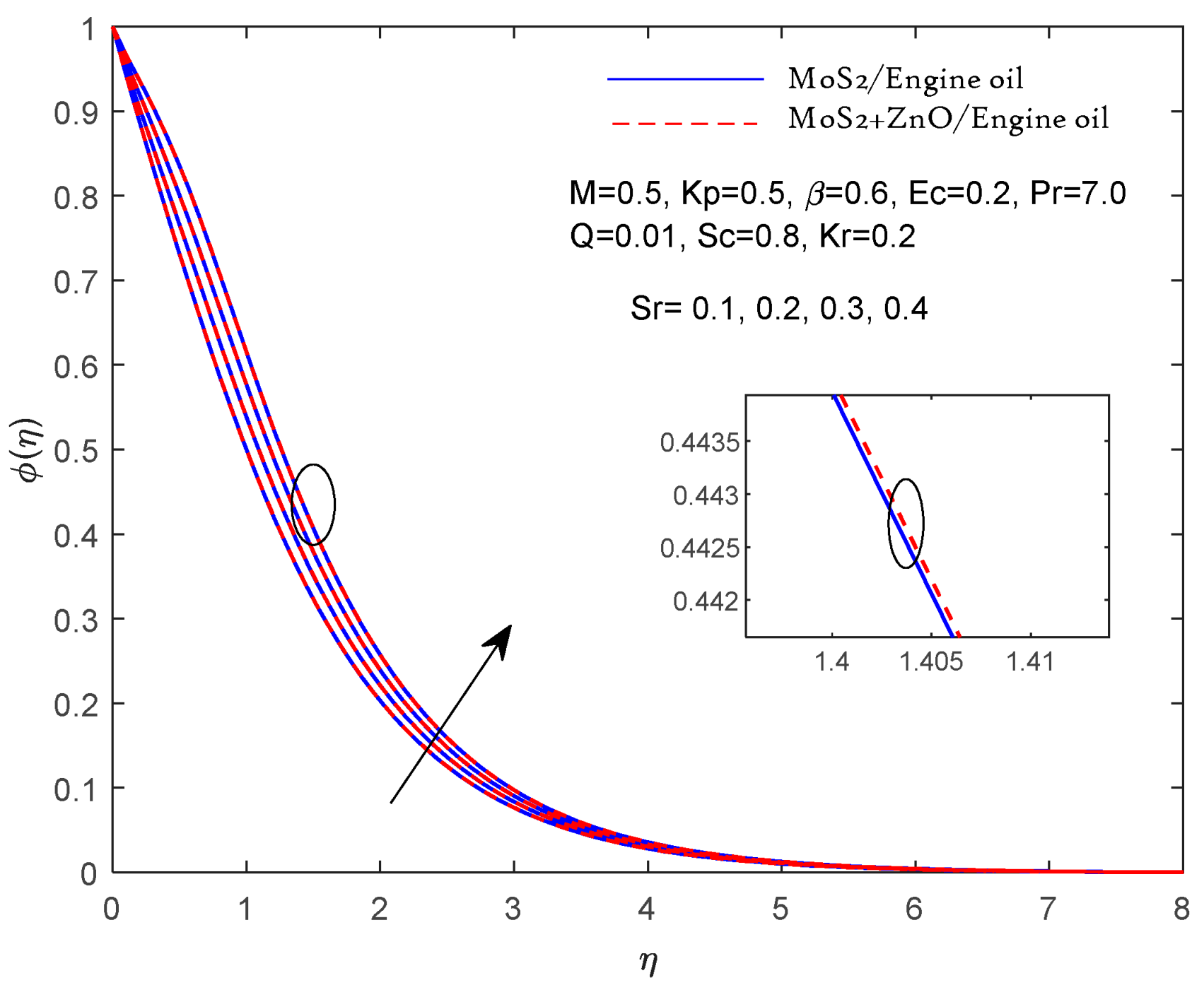
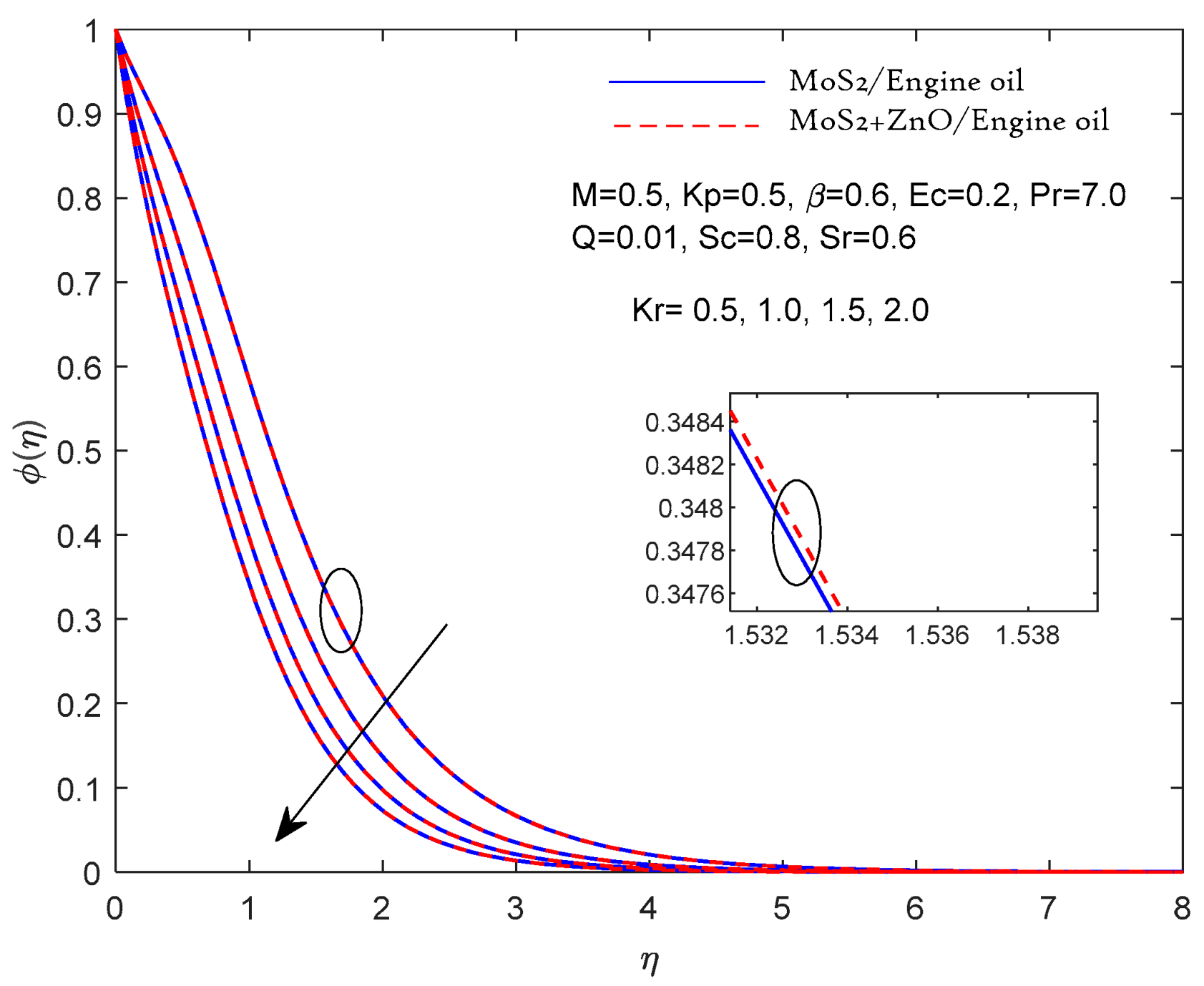
- The concentration profile is incremented with the value of Sr and decremented with the value of Sc and Kr;
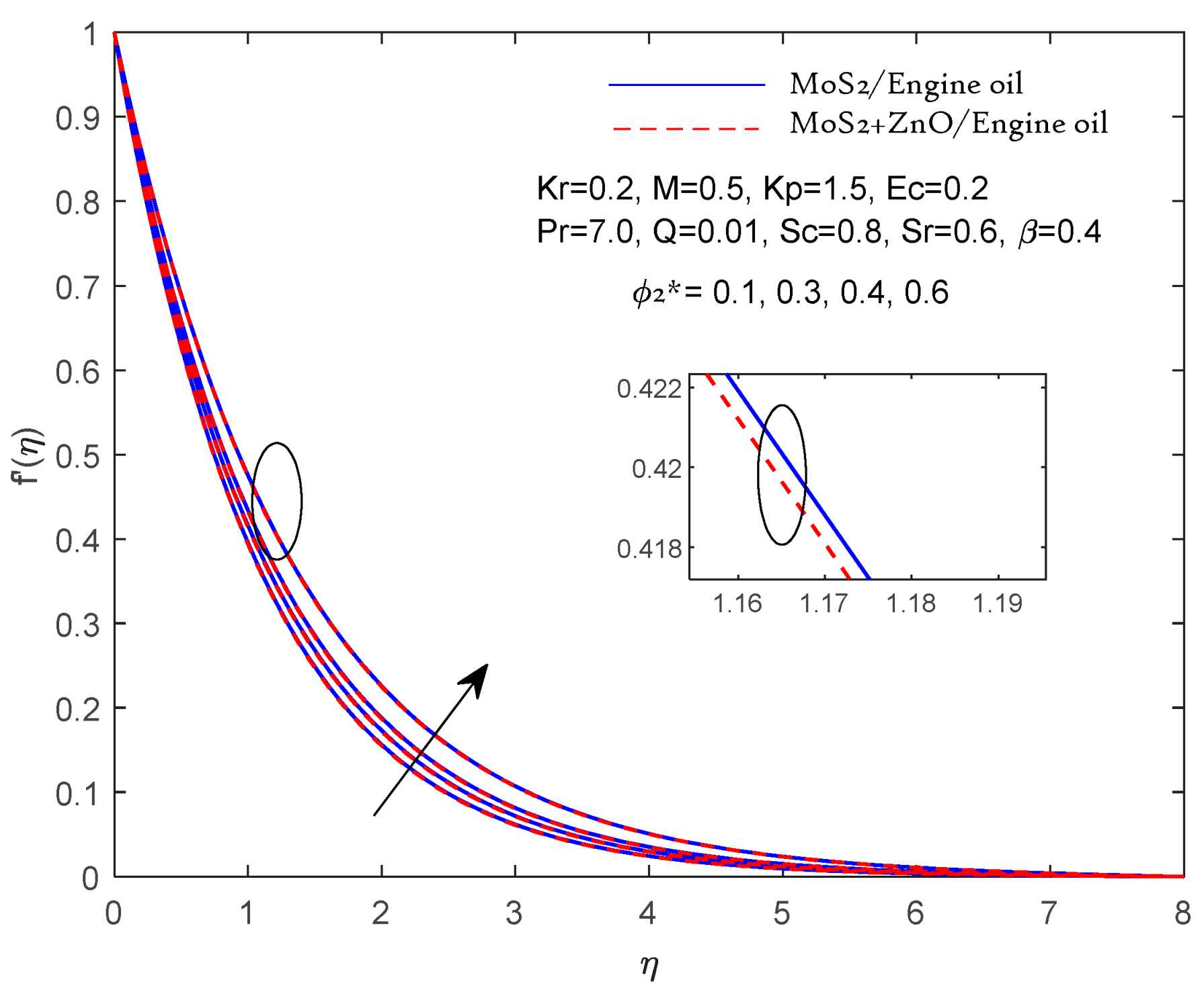
- The velocity f′ (η) becomes slower with the increasing value of , whereas the temperature increase when = 0.6 becomes slower.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Umair, K.; Aurang, Z.; Anuar, I. Impact of Thermal and Activation Energies on Glauert Wall Jet (WJ) Heat and Mass Transfer Flows Induced by ZnO-SAE50 Nano Lubricants with Chemical Reaction: The Case of Brinkman-Extended Darcy Model. Lubricants 2023, 11, 22. [Google Scholar] [CrossRef]
- Zia, U.; Ahmad, H.; Musaad, S.A.; Nifeen, H.A.; Sana, S. Significance of Temperature-Dependent Density on Dissipative and Reactive Flows of Nanofluid along Magnetically Driven Sheet and Applications in Machining and Lubrications. Lubricants 2023, 11, 410. [Google Scholar] [CrossRef]
- Zawar, H.; Fahad, A.; Ayaz, M.; Saeed, I. Significance of Slips and Convective Conditions towards the Non-Newtonian Hybrid Nanofluid Flow over a Bi-Directional Stretching Surface. Int. J. Thermofluids 2023, 2023, 100537. [Google Scholar] [CrossRef]
- Najiyah, S.K.; Norihan, M.A.; Ioan, P.; Roslinda, N.; Ezad, H.H.; Nadihah, W. Three-Dimensional Hybrid Nanofluid Flow and Heat Transfer pasta Permeable Stretching/Shrinking Sheet with Velocity Slip and Convective Condition. Chin. J. Phys. 2020, 66, 157–171. [Google Scholar] [CrossRef]
- Bilal, S.; Khalil, U.R.; Malik, M.Y.; Arif, H.; Mair, K. Effects of temperature dependent conductivity and absorptive/generative heat transfer on MHD three dimensional flow of Williamson fluid due to bidirectional non-linear stretching surface. Results Phys. 2017, 7, 204–212. [Google Scholar] [CrossRef]
- Ramzan, M.; Abdullah, D.; Anwar, S.; Poom, K.; Wiboonsak, W. MHD flow of micropolar and Williamson fluids over a bi-directional stretching sheet. Eur. Phys. J. Plus 2022, 137, 869. [Google Scholar] [CrossRef]
- Anuar, J.; Roslinda, N.; Ioan, P. Three-Dimensional Magnetohydrodynamic Mixed Convection Flow of Nanofluids over a Nonlinearly Permeable Stretching/Shrinking Sheet with Velocity and Thermal Slip. Appl. Sci. 2018, 8, 1128. [Google Scholar] [CrossRef]
- Ishtiaq, K.; Amin, U.R.; Abdullah, D.; Saeed, I.; Aiman, Z. Second-order slip flow of a magnetohydrodynamic hybrid nanofluid past a bi-directional stretching surface with thermal convective and zero mass flux conditions. Adv. Mech. Eng. 2023, 15, 16878132221149894. [Google Scholar] [CrossRef]
- Tanzila, H.; Nadeem, S. Heat transfer enhancement with Ag–CuO/water hybrid nanofluid. Results Phys. 2017, 7, 2317–2324. [Google Scholar] [CrossRef]
- Awatif, A.; Haneen, H.; Muhammad, B.; Aatif, A. Numerical study of Williamson hybrid nanofluid flow with thermal characteristics past over an extending surface. Heat Transf. 2022, 2022, 6641–6655. [Google Scholar] [CrossRef]
- Umadevi, S.S.; Anjalidevi, S.P. Heat Transfer Enhancement of Cu—Al2O3/Water Hybrid Nanofluid Flow over a Stretching Sheet. Niger. Math. Soc. 2017, 36, 419–433. Available online: https://ojs.ictp.it/jnms/index.php/jnms/article/view/147/47 (accessed on 1 March 2024).
- Ram Prakash, S.; Debasish, G.; Kalidas, D. Comparative study on hybrid nanofluid flow of Ag–CuO/H2O over a curved stretching surface with Soret and Dufour effects. Heat Transf. 2022, 51, 6365–6383. [Google Scholar] [CrossRef]
- Revathi, G.; Sajja, V.S.; Babu, M.J.; Raju, C.S.K.; Shehzad, S.A.; Bapanayya, C. Entropy optimization in hybrid radiative nanofluid (CH3OH + SiO2 + Al2O3) flow by a curved stretching sheet with cross-diffusion effects. Appl. Nanosci. 2021, 13, 337–351. [Google Scholar] [CrossRef]
- Motahar, R.; Anindita, B.; Amalendu, R.; Raghunath, P. Soret and Dufour effects on entropy generation for AIN and Al2O3 Hybrid Nanofluid Flow over past a stretching sheet in porous. Indian Soc. Theor. Appl. Mech. 2019, 2019, 9–12. Available online: https://www.researchgate.net/publication/338855239 (accessed on 1 March 2024).
- Asmat, U.Y.; Nadeem, S.; Wen-Hua, H.; Imran, S.; Sohaib, A.; Sajjad, H. Thermal charactristics for the flow of Williamson hybrid nanofluid (MoS2 + ZnO) based with engine oil over a streched sheet. Case Stud. Therm. Eng. 2021, 26, 101196. [Google Scholar] [CrossRef]
- Shami, A.M.A.; Abbas, T.; Al-Zubaidi, A.; Sami, U.K.; Saleem, S. Analytical assessment of heat transfer due to Williamson hybrid nanofluid (MoS2 + ZnO) with engine oil base material due to stretched sheet. Case Stud. Therm. Eng. 2023, 15, 103593. [Google Scholar] [CrossRef]
- Sreedevi, P.; Sudarsana, P.R.; Chamkha, A. Heat and mass transfer analysis of unsteady hybrid nanofluid flow over a stretching sheet with thermal radiation. SN Appl. Sci. 2020, 2, 1222. [Google Scholar] [CrossRef]
- Mubashar, A.; Fahad, M.A.; Ali, H.; Qusain, H.; Abdullah, A.; Sayed, M.E.; Zubair, A.; Laila, A.A.; Ahmed, M.G. Effect of inclined magnetic field on radiative heat and mass transfer in chemically reactive hybrid nanofluid flow due to dual stretching. Sci. Rep. 2023, 13, 7828. [Google Scholar] [CrossRef] [PubMed]
- Yu-Ming, C.; Muhammad, D.I.; Muhammad, I.A.; Ali, A.; Ferial, G. Influence of hybrid nanofluids and heat generation on coupled heat and mass transfer flow of a viscous fluid with novel fractional derivative. J. Therm. Anal. Calorim. 2021, 144, 2057–2077. [Google Scholar] [CrossRef]
- Tassaddiq, A.; Khan, S.; Bilal, M.; Gul, T.; Mukhtar, S.; Shah, Z.; Bonyah, E. Heat and mass transfer together with hybrid nanofluid flow over a rotating disk. AIP Adv. 2020, 10, 055317. [Google Scholar] [CrossRef]
- Shanmugapriya, M.; Sundareswaran, R.; Senthil Kumar, P. Heat and Mass Transfer Enhancement of MHD Hybrid Nanofluid Flow in the Presence of Activation Energy. Int. J. Chem. Eng. 2021, 2021, 9473226. [Google Scholar] [CrossRef]
- Sohail, A.; Kashif, A.; Muhammad, R.; Muhammad, A. Heat and mass transfer attributes of copper–aluminum oxide hybrid nanoparticles flow through a porous medium. Case Stud. Therm. Eng. 2021, 25, 100932. [Google Scholar] [CrossRef]
- Abdulmajeed, A. Numerical study on heat and mass transport enhancement in MHD Williamson fluid via hybrid nanoparticles. Alex. Eng. J. 2022, 16, 8343–8354. [Google Scholar] [CrossRef]
- Gohar; Tahir, S.K.; Ndolane, S.; Abir, M.; Ameni, B. Heat and Mass Transfer of the Darcy-Forchheimer Casson Hybrid Nanofluid Flow due to an Extending Curved Surface. J. Nanomater. 2022, 2022, 3979168. [Google Scholar] [CrossRef]
- Ali, F.; Zaiba, A.; Faizana, M.; Zafara, S.S.; Shalan, A.; Nehad, A.S.; Jae, D.C. Heat and mass exchanger analysis for Ree-Eyring hybrid nanofluid through a stretching sheet utilizing the homotopy perturbation method. Case Stud. Therm. Eng. 2024, 54, 104014. [Google Scholar] [CrossRef]
- Wei-Feng, X.; Shafiq, A.; Naveed, K.M.; Hijaz, A.; Aysha, R.; Jamel, B.; Tuan, N.G. Heat and mass transfer analysis of nonlinear mixed convective hybrid nanofluid flow with multiple slip boundary conditions. Case Stud. Therm. Eng. 2022, 32, 101893. [Google Scholar] [CrossRef]
- Saeed, D.; Mohammadreza, N.R.; Ioan, P. A novel hybridity model for TiO2-CuO/water hybrid nanofluid flow over a static/moving wedge or corner. Sci. Rep. 2019, 9, 16290. [Google Scholar] [CrossRef]
- Saqib, M.; Ilyas, K.; Sharidan, S. Application of fractional differential equations to heat transfer in hybrid nanofluid: Modeling and solution via integral transforms. Adv. Differ. Equ. 2019, 2019, 52. [Google Scholar] [CrossRef]
- Jun, Z.; Yingru, L.; Yongyong, H.; Jianbin, L. In Situ Green Synthesis of the New Sandwichlike Nanostructure of Mn3O4/Graphene as Lubricant Additives. ACS Appl. Mater. Interfaces 2019, 11, 36931–36938. [Google Scholar] [CrossRef] [PubMed]
- Vicki, W.V.; Praveen Kumar, K.; Prabhakar, S.; Husna, N.; Abdullah, M.Z. Viscosity and rheological behavior of Al2O3-Fe2O3/water-EG based hybrid nanofluid: A new correlation based on mixture ratio. J. Mol. Liq. 2023, 375, 121365. [Google Scholar] [CrossRef]
- Jongsuk, L.; Sejin, L.; Chungyeon, C.; Sunghan, K. Experimental study and modeling of the energy density and time-dependent rheological behavior of carbon nanotube nanofluids with sonication. Int. J. Heat Mass Transf. 2022, 192, 122941. [Google Scholar] [CrossRef]
- Seyed, B.M.; Saeed, Z.H.; Patrice, E. Viscosity, tribological and physicochemical features of ZnO and MoS2 diesel oil-based nanofluids: An experimental study. Fuel 2021, 293, 120481. [Google Scholar] [CrossRef]

| Physical Properties | MoS2 | ZnO | Engine Oil |
|---|---|---|---|
| Ρ (kg·m−3) | 5060 | 5600 | 884 |
| Cp/j (kg·k) | 397.21 | 495.2 | 1910 |
| W.k/m.k | 904.4 | 13 | 0.144 |
| Properties | Nanofluid | Hybrid Nanofluid |
|---|---|---|
| μ (viscosity) | ||
| ρ (density) | ||
| ρCp (heat capacity) | ||
| k (thermal conductivity) |
| Pr | Asmat Ullah et al. [15] | Shami A.M. et al. [16] | P. Shreedevi et al. [17] | Our Results |
|---|---|---|---|---|
| 2.0 | 0.9112 | 0.91138 | 0.911341 | 0.911325 |
| 6.13 | 1.7597 | 1.75965 | 1.759676 | 1.759671 |
| 7.0 | 1.8953 | 1.8955 | 1.895397 | 1.895393 |
| 20.0 | 3.3540 | - | 3.353915 | 3.353921 |
| M | Kp | β | |
|---|---|---|---|
| 0.0 | 0.5 | 0.5 | 0.7256 |
| 0.5 | 0.8227 | ||
| 1.0 | 0.9097 | ||
| 1.5 | 0.1 | 0.9191 | |
| 0.3 | 0.9547 | ||
| 0.5 | 0.9889 | ||
| 0.5 | 0.1 | 0.5170 | |
| 0.2 | 0.6993 | ||
| 0.3 | 0.8228 |
| Pr | Q | Ec | |
|---|---|---|---|
| 7.0 | 0.01 | 0.1 | 1.5082 |
| 8.0 | 1.6176 | ||
| 10.0 | 1.8150 | ||
| 7.0 | 0.02 | 1.4899 | |
| 0.05 | 1.4341 | ||
| 0.09 | 1.3574 | ||
| 0.01 | 0.15 | 1.3899 | |
| 0.2 | 1.2715 | ||
| 0.25 | 1.1532 |
| Pr | Ec | Sc | Sr | Kr | Q | |
|---|---|---|---|---|---|---|
| 6.3 | 0.1 | 0.4 | 0.8 | 0.2 | 0.01 | 0.0643 |
| 7.0 | 0.0396 | |||||
| 7.5 | 0.0227 | |||||
| 7.0 | 0.2 | 0.1128 | ||||
| 0.25 | 0.1494 | |||||
| 0.3 | 0.1860 | |||||
| 0.1 | 2.5 | 0.0735 | ||||
| 3.0 | 0.0624 | |||||
| 3.5 | 0.0511 | |||||
| 0.4 | 0.5 | 0.1848 | ||||
| 0.6 | 0.1364 | |||||
| 0.7 | 0.0880 | |||||
| 0.8 | 0.3 | 0.1002 | ||||
| 0.4 | 0.1541 | |||||
| 0.5 | 0.2031 | |||||
| 0.2 | 0.02 | 0.0453 | ||||
| 0.04 | 0.0568 | |||||
| 0.06 | 0.0685 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Madiwal, S.; Naduvinamani, N.B. Heat and Mass Transformation of Casson Hybrid Nanofluid (MoS2 + ZnO) Based on Engine Oil over a Stretched Wall with Chemical Reaction and Thermo-Diffusion Effect. Lubricants 2024, 12, 221. https://doi.org/10.3390/lubricants12060221
Madiwal S, Naduvinamani NB. Heat and Mass Transformation of Casson Hybrid Nanofluid (MoS2 + ZnO) Based on Engine Oil over a Stretched Wall with Chemical Reaction and Thermo-Diffusion Effect. Lubricants. 2024; 12(6):221. https://doi.org/10.3390/lubricants12060221
Chicago/Turabian StyleMadiwal, Shreedevi, and Neminath B. Naduvinamani. 2024. "Heat and Mass Transformation of Casson Hybrid Nanofluid (MoS2 + ZnO) Based on Engine Oil over a Stretched Wall with Chemical Reaction and Thermo-Diffusion Effect" Lubricants 12, no. 6: 221. https://doi.org/10.3390/lubricants12060221
APA StyleMadiwal, S., & Naduvinamani, N. B. (2024). Heat and Mass Transformation of Casson Hybrid Nanofluid (MoS2 + ZnO) Based on Engine Oil over a Stretched Wall with Chemical Reaction and Thermo-Diffusion Effect. Lubricants, 12(6), 221. https://doi.org/10.3390/lubricants12060221






