Steady-State Temperature Field and Rolling Resistance Characteristics of Low-Speed and Low-Load Capacity Non-Pneumatic Tires
Abstract
:1. Introduction
2. Research Method
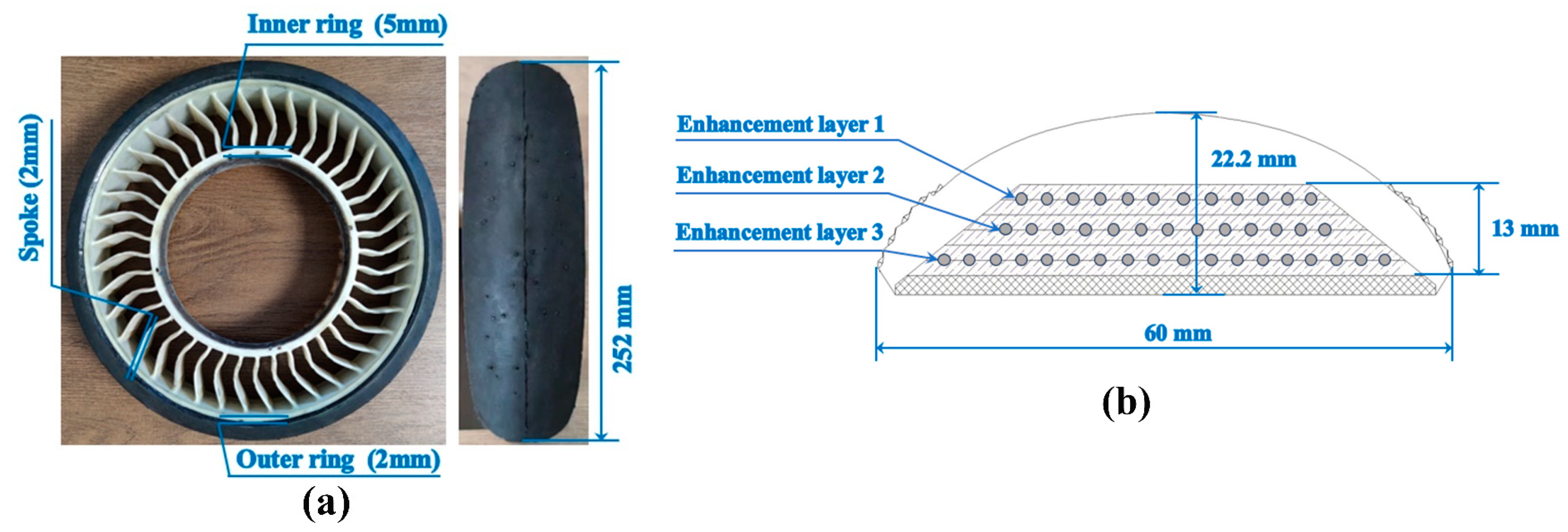
2.1. Structure of the LSL-Tire
2.2. Hysteresis Effect of Viscoelastic Materials
2.3. Rolling Resistance of the LSL-Tire
2.4. Heat Generation Rate of Element
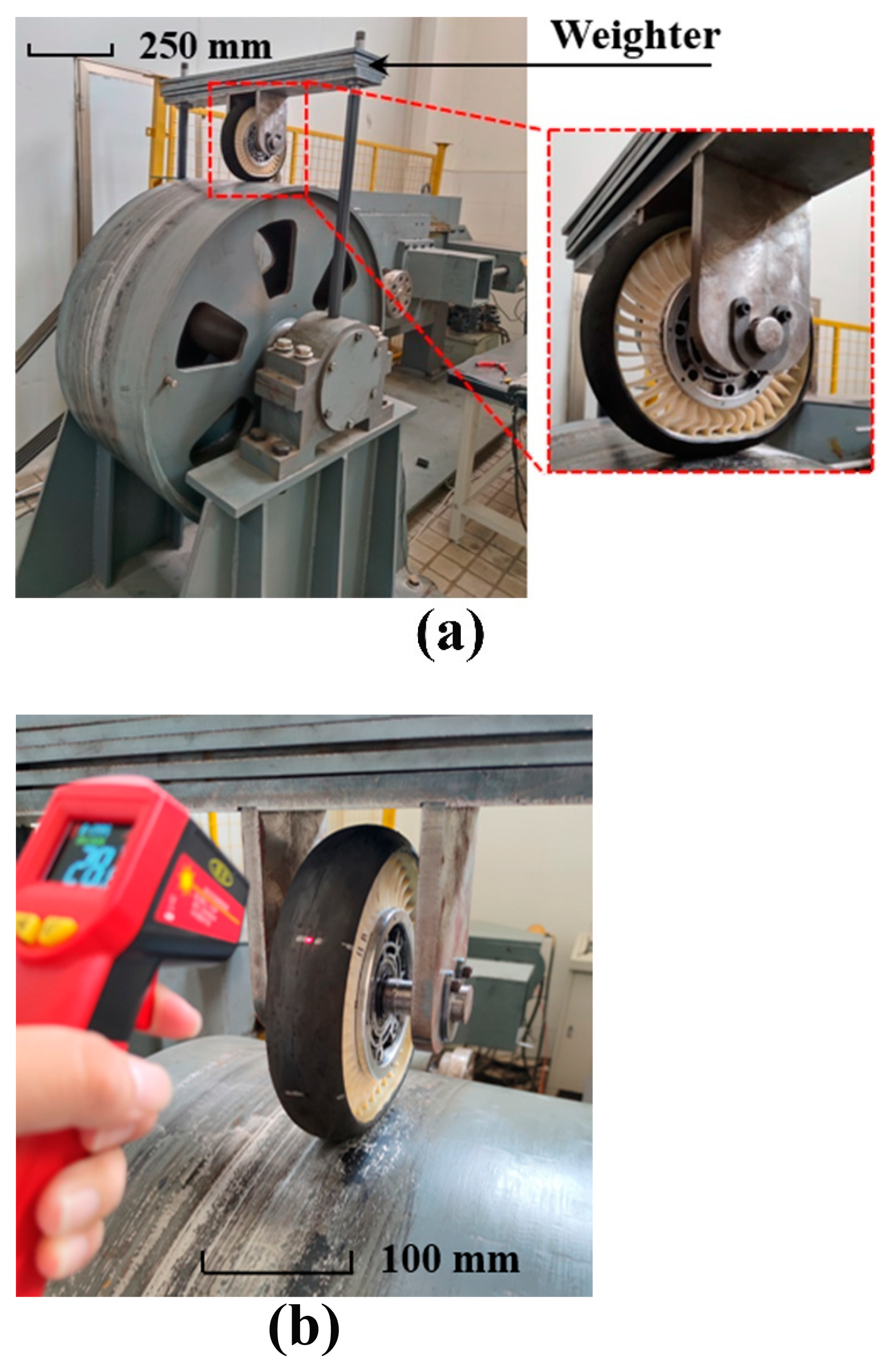
2.5. Steady-State Temperature Field Test of the LSL-Tire
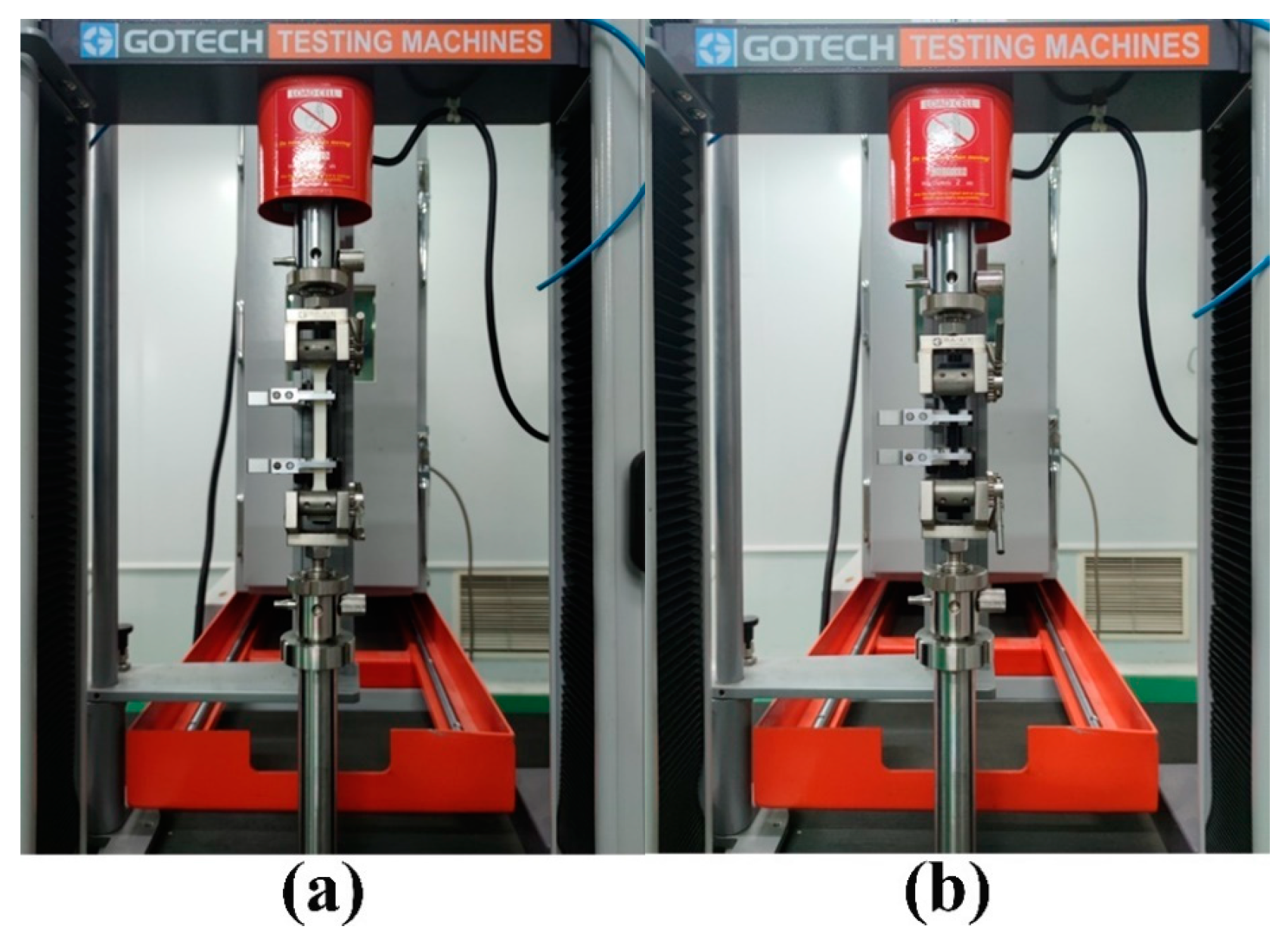
2.6. Mechanical Properties Test of Materials
2.7. Thermodynamic Parameters and Density Test of Materials
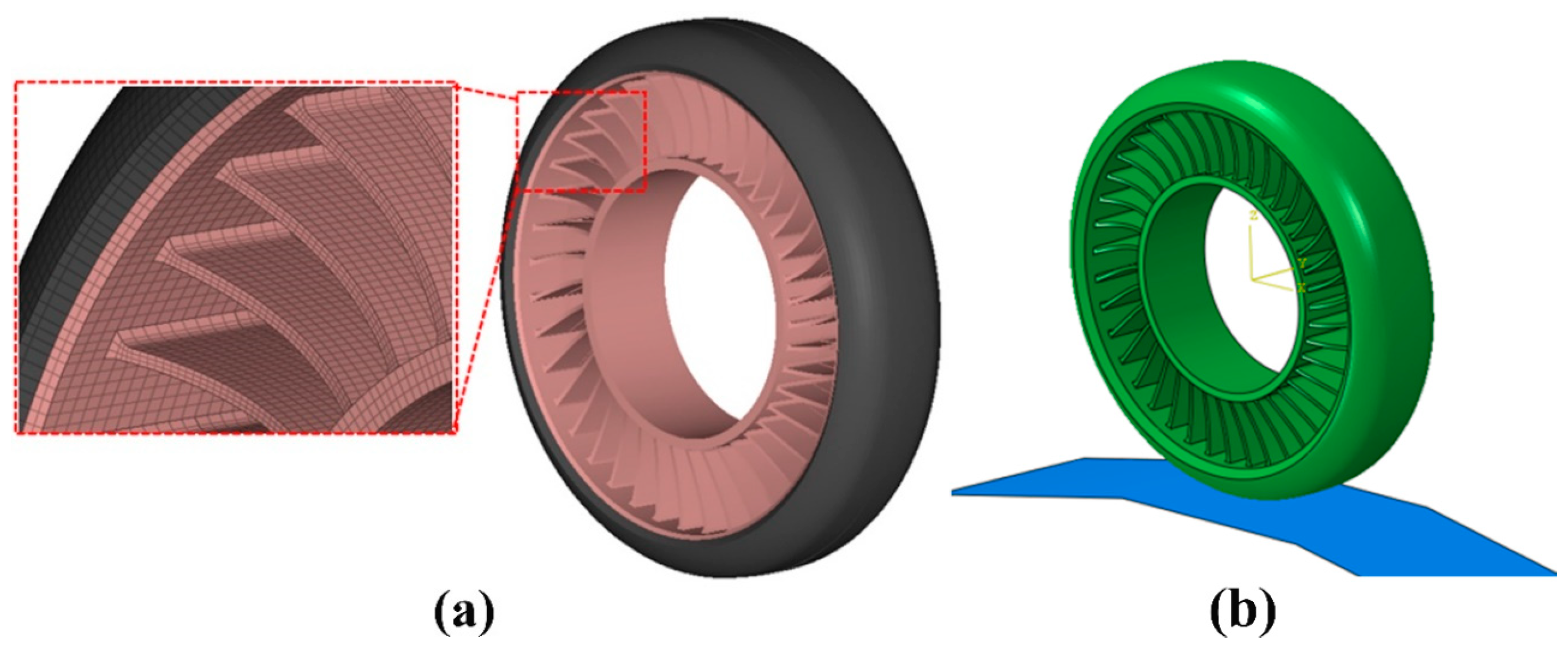
2.8. Structural Deformation Analysis
2.9. Heat Transfer Analysis
3. Results and Discussion
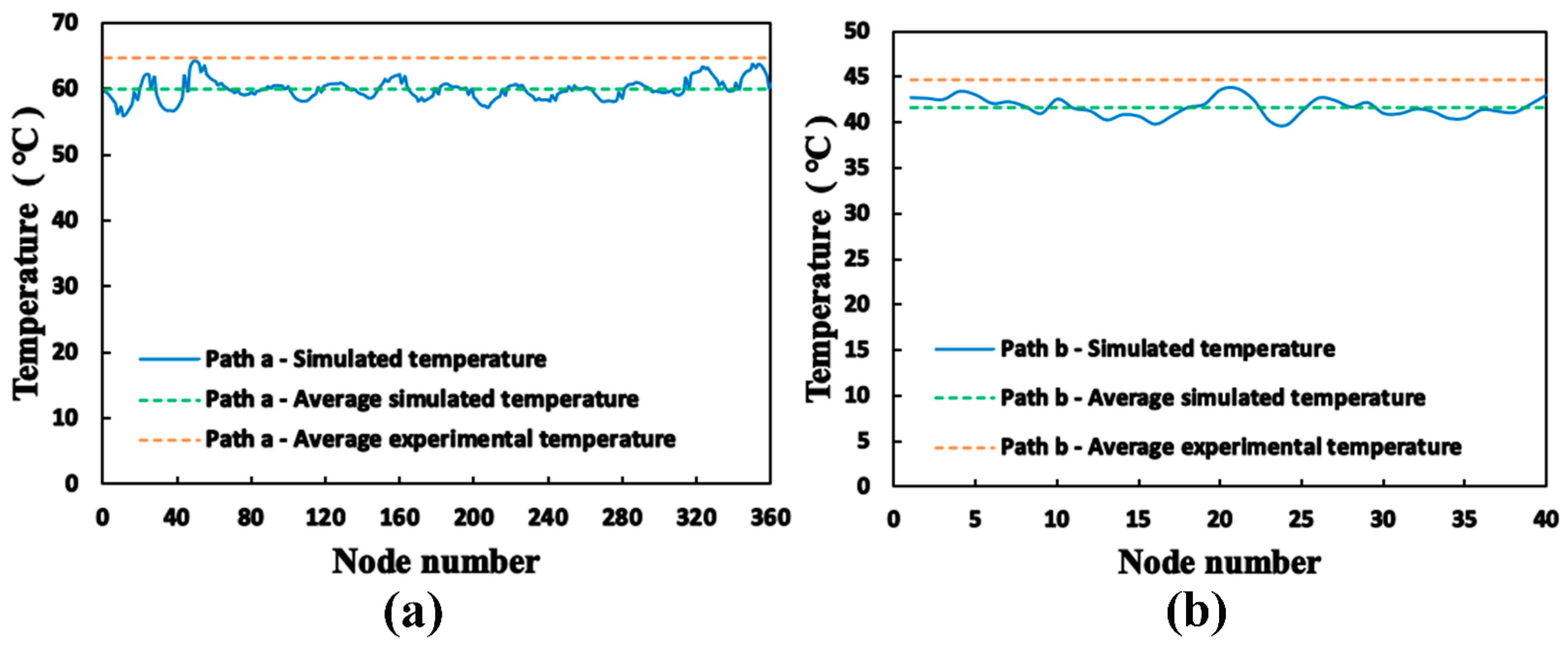
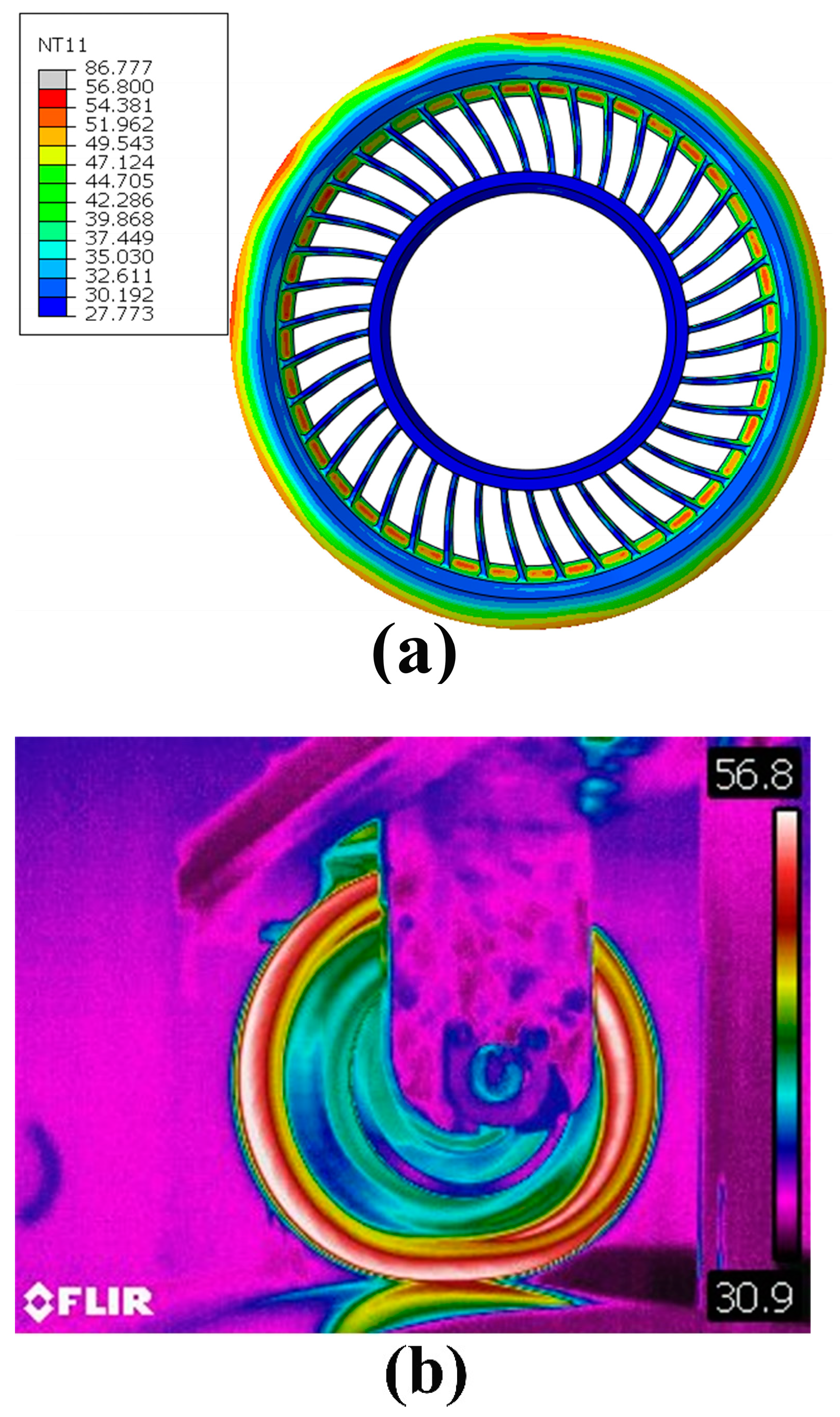
3.1. Steady-State Temperature Field Results of the LSL-Tire
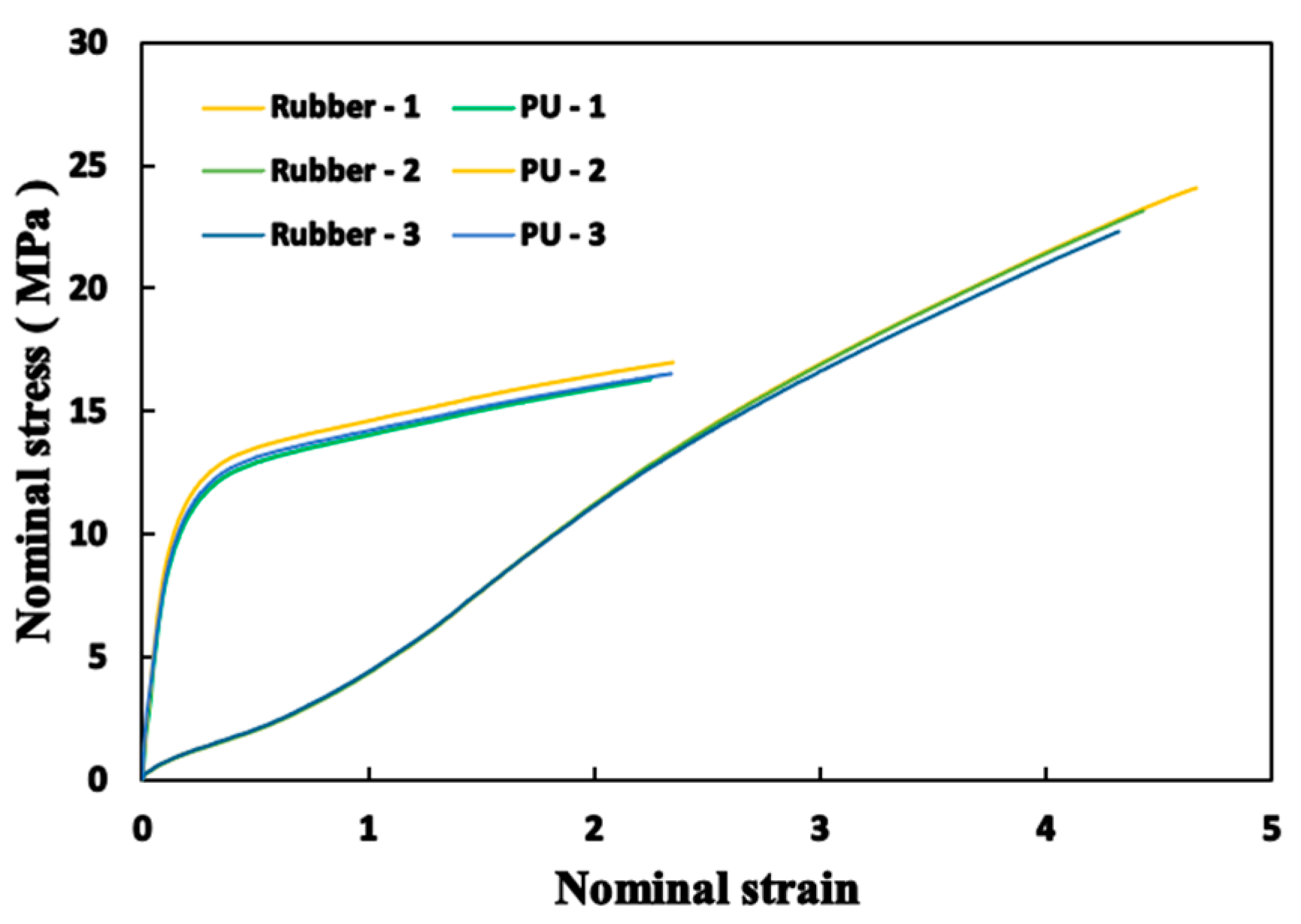
3.2. Mechanical Properties Results of Materials
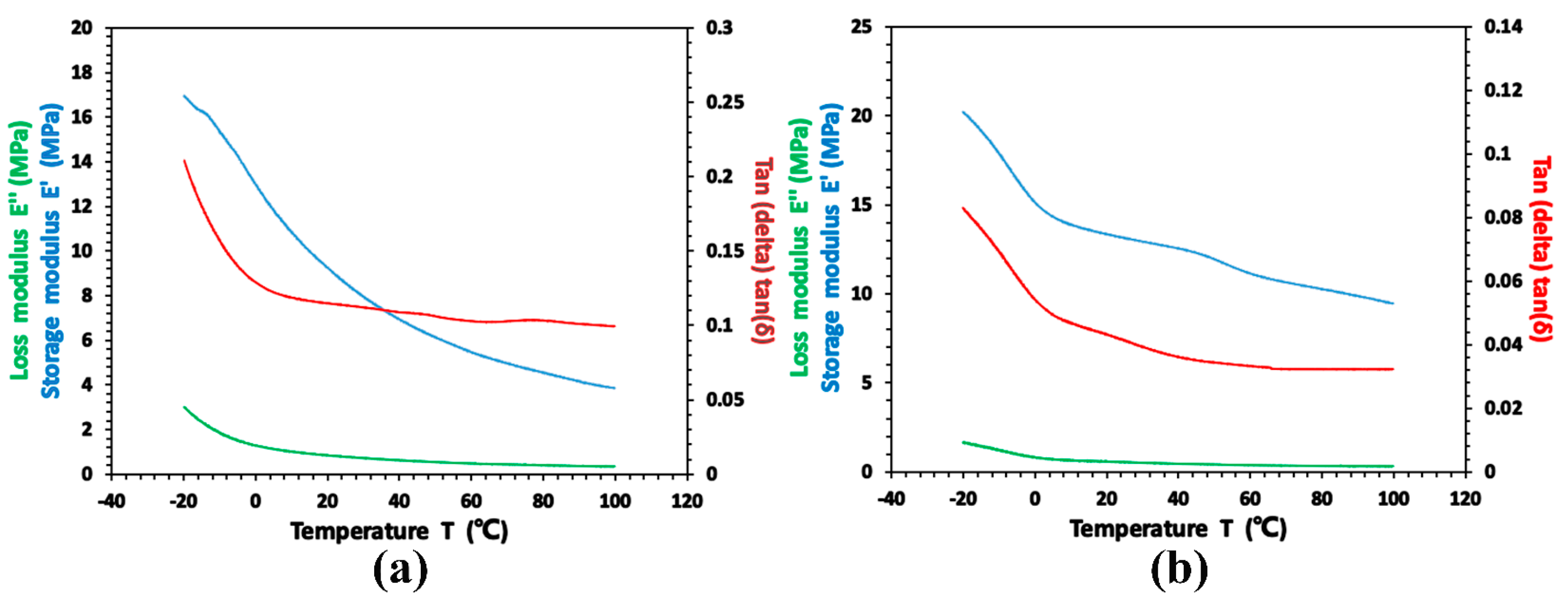
3.3. Thermodynamic Parameters and Density Results of Materials
3.4. Validation of the FE Model
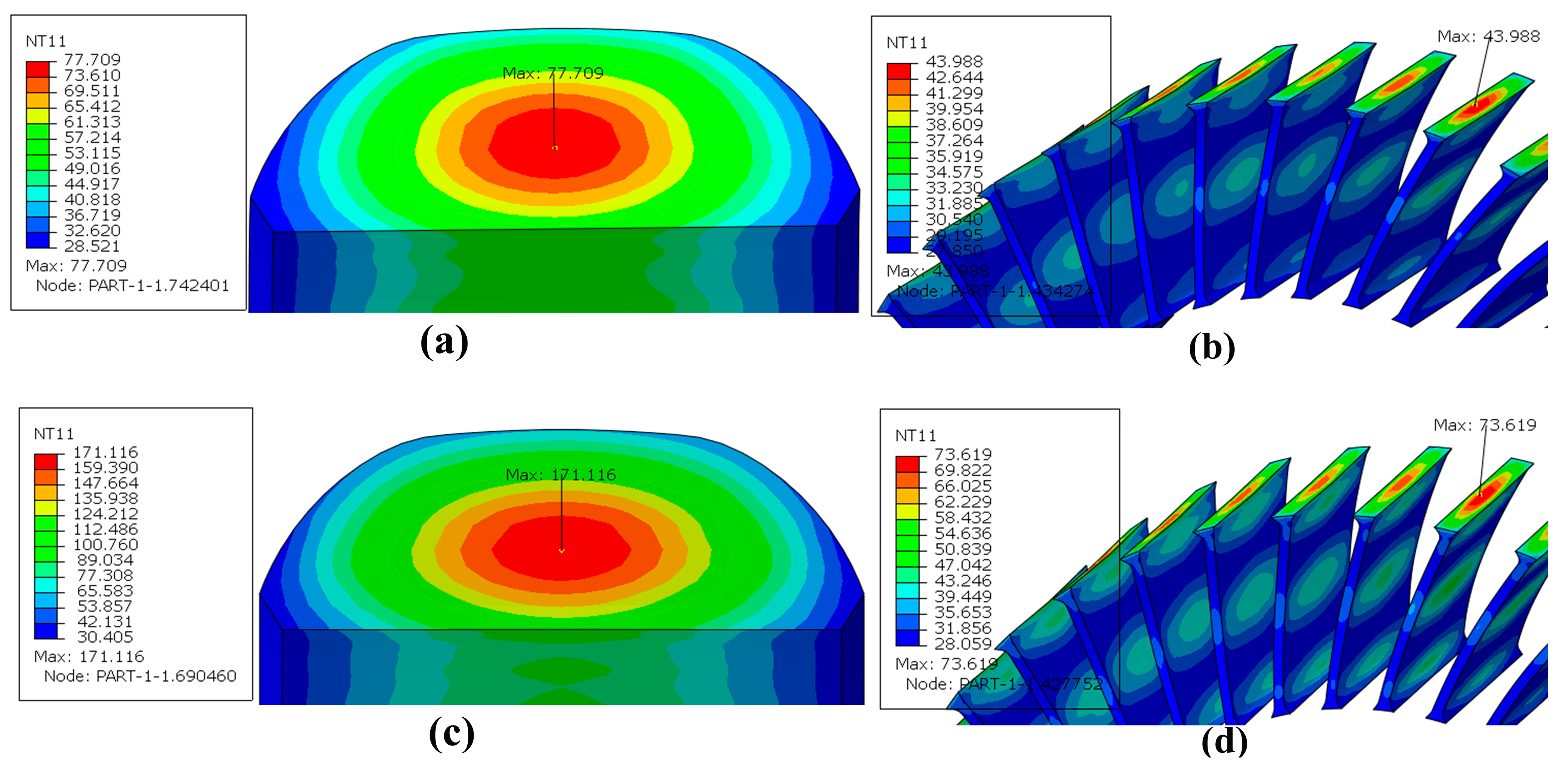
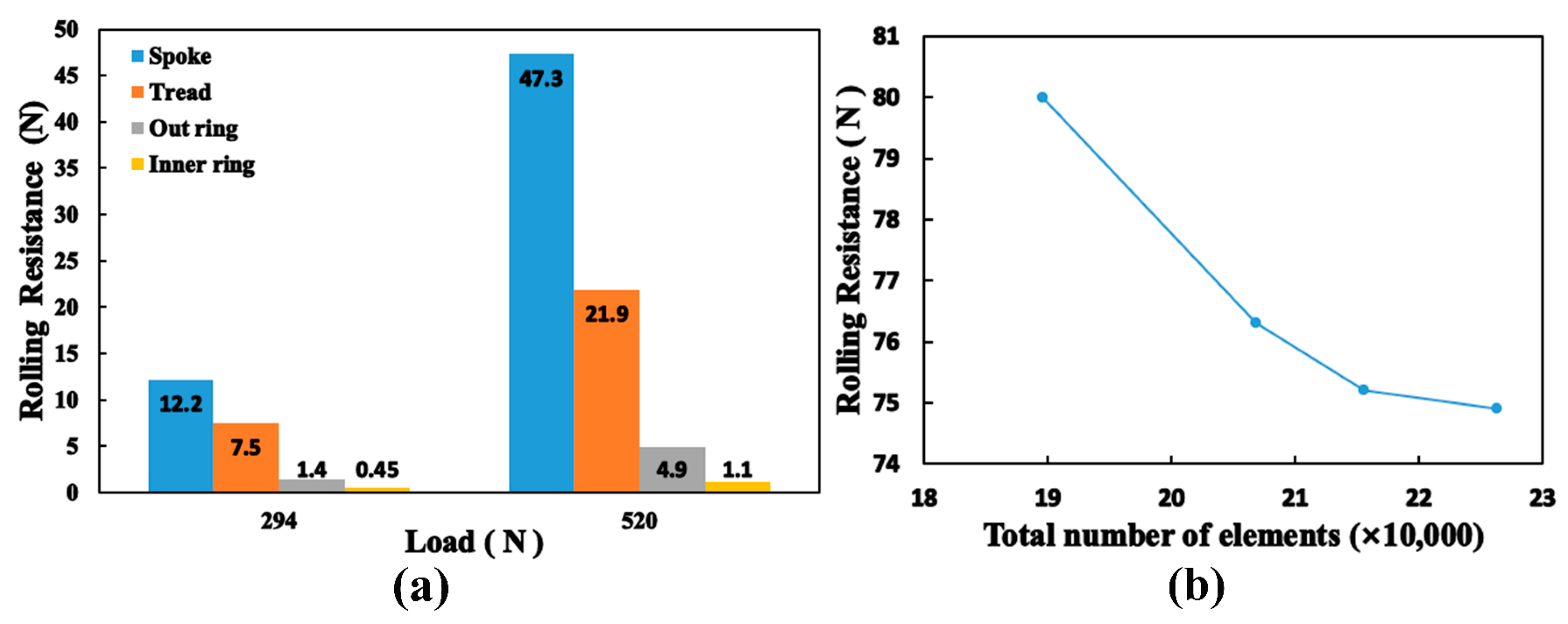
3.5. Steady-State Temperature Field and Rolling Resistance of the LSL-Tire under Different Loads
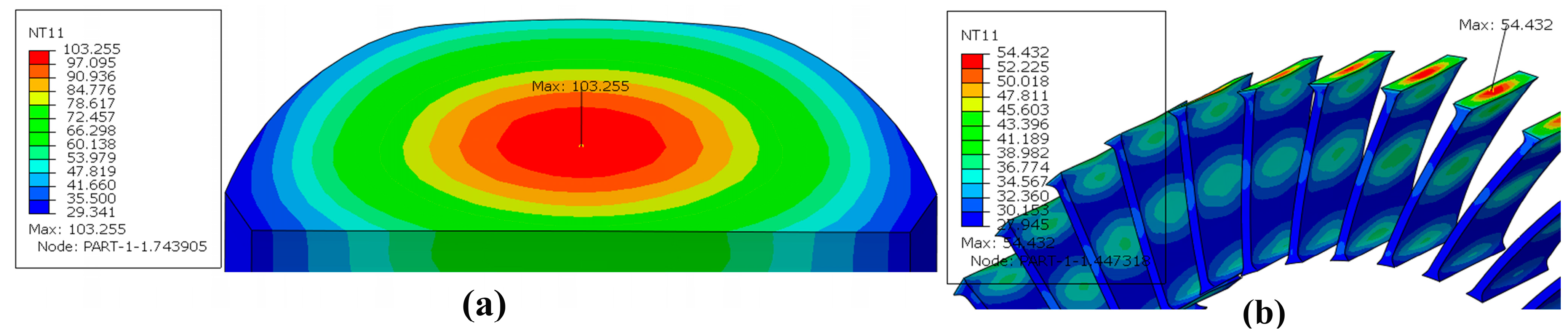
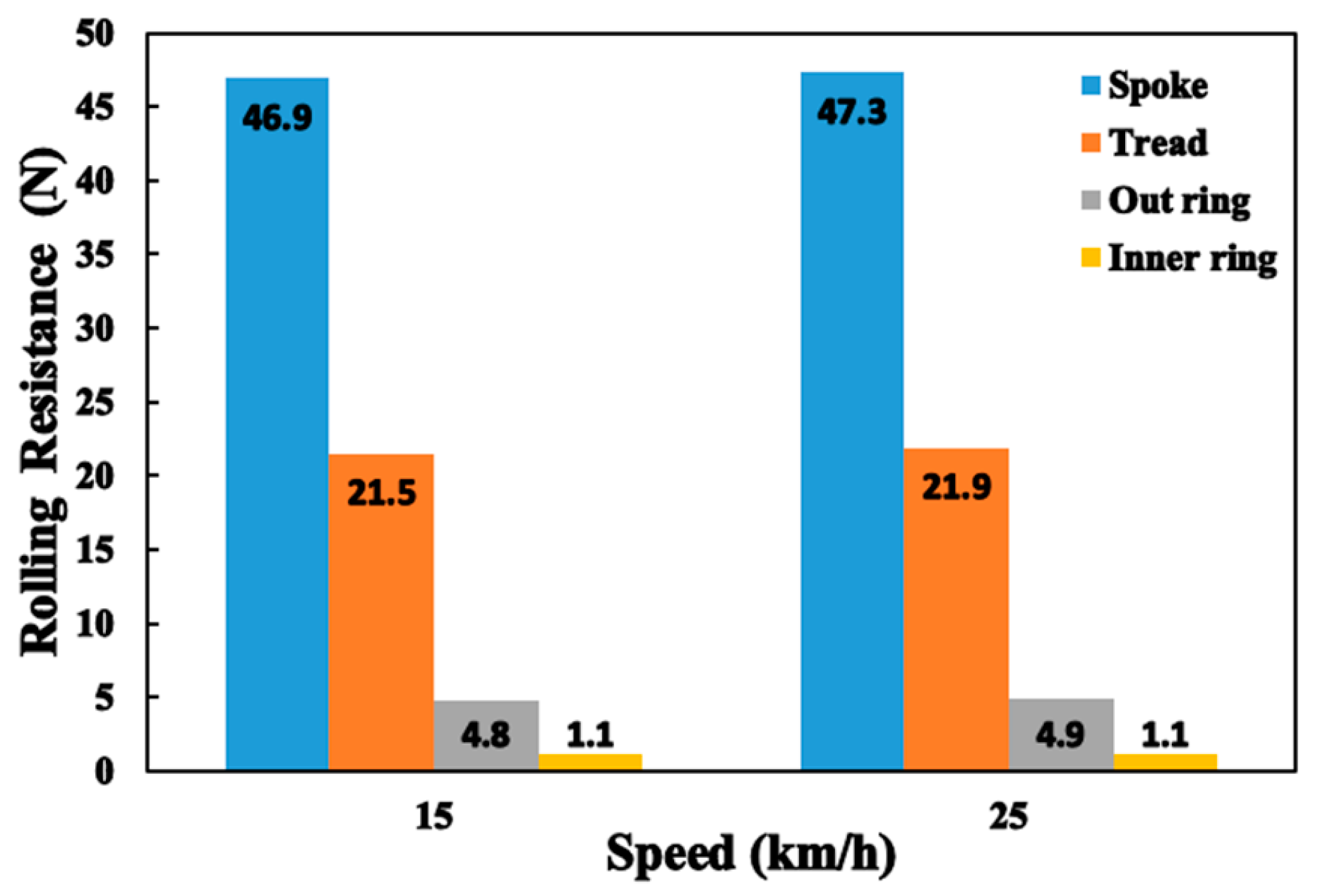
3.6. Steady-State Temperature Field and Rolling Resistance of the LSL-Tire under Different Speeds
4. Conclusions
- (1)
- Based on the SSTF experimental data of the LSL-tire, the accuracy of the solution strategy is verified, whose prediction accuracy pertaining to the SSTF of the tread outer surface center and the spoke outer surface center are 92.44% and 93.06%, respectively.
- (2)
- The SSTF of each part of the LSL-tire increases significantly with increases in load and velocity. The highest temperature point of the LSL-tire is located in the center of the tread, and the highest temperature point of the spokes is located in the center of the joint between spokes and the outer ring.
- (3)
- The RR of the LSL-tire increases significantly with increases in load, and the spokes exert the most considerable effect on the RR, followed by the tread.
- (4)
- The RR of the LSL-tire increases marginally with velocity.
- (5)
- The SSTF solution strategy proposed herein differs from the existing SSTF research methods; the strategy does not assume that the steady-state temperature of each radial cross-section of the tire is the same.
- (6)
- The SSTF and hysteresis energy loss solution strategy proposed herein can theoretically be applied to any object composed of viscoelastic materials, not only to tires.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hall, D.E.; Moreland, J.C. Fundamentals of Rolling Resistance. Rubber Chem. Technol. 2001, 74, 525–539. [Google Scholar]
- Xiong, Y. In-Plane Tire Deformation Measurement Using a Multi-Laser Sensor System. Ph.D. Thesis, Aalto University, Espoo, Finland, 2016. [Google Scholar]
- Shida, Z.; Koishi, M.; Kogure, T.; Kabe, K. A Rolling Resistance Simulation of Tires Using Static Finite Element Analysis. Tire Sci. Technol. 1999, 27, 84–105. [Google Scholar] [CrossRef]
- Koishi, M. Analysis of Rubber-Based Composite Materials Using Homogenization Method. Ph.D. Thesis, Yokohama National University, Yokohama, Japan, 1998. [Google Scholar]
- Lin, Y.J.; Hwang, S.J. Temperature prediction of rolling tires by computer simulation. Math. Comput. Simul. 2004, 67, 235–246. [Google Scholar]
- Tang, T.; Johnson, D.; Smith, R.E.; Felicelli, S.D. Numerical evaluation of the temperature field of steady-state rolling tires. Appl. Math. Model. 2013, 38, 1622–1637. [Google Scholar] [CrossRef]
- Li, F.Z.; Liu, F.; Liu, J.; Gao, Y.Y.; Lu, Y.L.; Chen, J.F.; Yang, H.B.; Zhang, L.Q. Thermo-mechanical coupling analysis of transient temperature and rolling resistance for solid rubber tire: Numerical simulation and experimental verification. Compos. Sci. Technol. 2018, 167, 404–410. [Google Scholar]
- Ulmer, J.D. Strain dependence of dynamic mechanical properties of carbon black-filled rubber compounds. Rubber Chem. Technol. 1996, 69, 15–47. [Google Scholar] [CrossRef]
- Saux, V.L.; Marco, Y.; Calloch, S.; Charrier, P.; Taveau, D. Heat build-up of rubber under cyclic loadings: Validation of an efficient demarch to predict the temperature fields. Rubber Chem. Technol. 2013, 86, 38–56. [Google Scholar] [CrossRef]
- Browne, A.L.; Wickliffe, L.E. Parametric study of convective heat transfer coefficients at the tire surface. Tire Sci. Technol. 1980, 8, 37–67. [Google Scholar] [CrossRef]
- He, H.; Liu, J.M.; Zhang, Y.R.; Han, X.; Mars, W.V.; Zhang, L.Q.; Li, F.Z. Heat Build-Up and Rolling Resistance Analysis of a Solid Tire: Experimental Observation and Numerical Simulation with Thermo-Mechanical Coupling Method. Polymers 2022, 14, 2210–2230. [Google Scholar]
- Ding, Y.M.; Wang, H.; ASCE, M.; Qian, J.Y.; Zhou, H.C. Evaluation of Tire Rolling Resistance from Tire-Deformable Pavement Interaction Modeling. J. Transp. Eng. Part B Pavements 2021, 147, 04021041. [Google Scholar]
- Park, J.W.; Jeong, H.Y. Finite element modeling for the cap ply and rolling resistance of tires. Int. J. Automot. Technol. 2022, 23, 1427–1436. [Google Scholar] [CrossRef]
- Cho, J.R.; Lee, H.W.; Jeong, W.B.; Jeong, K.M.; Kim, K.W. Numerical estimation of rolling resistance and temperature distribution of 3-D periodic patterned tire. Int. J. Solids Struct. 2013, 50, 86–96. [Google Scholar] [CrossRef]
- Cho, J.R.; Lee, H.W.; Jeong, W.B.; Jeong, K.M.; Kim, K.W. Finite element estimation of hysteretic loss and rolling resistance of 3-D patterned tire. Int. J. Mech. Mater. Des. 2013, 9, 355–366. [Google Scholar] [CrossRef]
- Lee, H.W.; Cho, J.R.; Jeong, W.B.; Jeong, K.M.; Kim, K.W. Mesh generation and hysteretic loss prediction of 3-D periodic patterned tire. Int. J. Automot. Technol. 2014, 15, 411–417. [Google Scholar] [CrossRef]
- Fu, H.X.; Liang, X.M.; Wang, Y.; Ku, L.Y.; Xiao, Z. Thermal Mechanical Coupling Analysis of a Flexible Spoke Non-pneumatic Tire. J. Mech. Eng. 2022, 68, 143–154. [Google Scholar] [CrossRef]
- Marin, M.; Chirila, A.; Öchsner, A.; Vlase, S. About finite energy solutions in thermoelasticity of micropolar bodies with voids. Bound. Value Probl. 2019, 2019, 89. [Google Scholar] [CrossRef]
- Kim, K.; Heo, H.; Uddin, M.; Ju, J.; Kim, D.M. Optimization of Nonpneumatic Tire with Hexagonal Lattice Spokes for Reducing Rolling Resistance; SAE Technical Paper; SAE: Warrendale, PA, USA, 2015. [Google Scholar] [CrossRef]
- Yoo, S.; Uddin, M.S.; Heo, H.; Ju, j.; Kim, D.M.; Choi, S.J. Deformation and Heat Generation in a Nonpneumatic Tire with Lattice Spokes; SAE Technical Paper; SAE: Warrendale, PA, USA, 2015. [Google Scholar] [CrossRef]
- Yoo, S.; Uddin, M.S.; Heo, H.; Ju, j.; Choi, S.J. Thermoviscoelastic modeling of a nonpneumatic tire with a lattice spoke. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2017, 231, 241–252. [Google Scholar] [CrossRef]
- Jin, X.C.; Hou, C.; Fan, X.L.; Sun, Y.L.; Lv, J.N.; Lu, C.S. Investigation on the static and dynamic behaviors of non-pneumatic tires with honeycomb spokes. Compos. Struct. 2018, 187, 27–35. [Google Scholar] [CrossRef]
- Veeramurthy, M. Modeling, Finite Element Analysis, and Optimization of Non-Pneumatic Tire (NPT) for the Minimization of Rolling Resistance. Master’s Thesis, Clemson University, Clemson, SC, USA, 2011. [Google Scholar]
- Veeramurthy, M.; Ju, J.; Thompson, L.L.; Summers, J.D. Optimization of a non-pneumatic tire for reduced rolling resistance. In Proceedings of the IDETC/CIE, Washington, DC, USA, 28–31 August 2011. [Google Scholar]
- Veeramurthy, M.; Ju, J.; Thompson, L.L.; Summers, J.D. Optimisation of geometry and material properties of a non-pneumatic tyre for reducing rolling resistance. Int. J. Veh. Des. 2014, 66, 193–216. [Google Scholar] [CrossRef]
- Aldhufairi, H.S.; Essa, K.E. Tire rolling-resistance computation based on material viscoelasticity representation. Adv. Automot. Eng. 2019, 1, 167–183. [Google Scholar]
- Aldhufairi, H.; Olatunbosun, R.; Essa, K. Determination of a Tyre’s Rolling Resistance Using Parallel Rheological Framework; SAE Technical Paper; SAE: Warrendale, PA, USA, 2019. [Google Scholar]
- Kiran, M.; Aswath, M.; Shishir, D.A.; Ponangi, B.R.; Bhanumurthy, R. Effect of Material Models on Rolling Resistance of Non-pneumatic Tires with Hexagonal Spokes. SAE Int. J. Commer. Veh. 2023, 16, 33–47. [Google Scholar] [CrossRef]
- GB/T 42825-2023; General Technical Specification of Electric Scooters. Standards Press of China: Beijing, China, 2023.
- Garman, C.M.R.; Como, S.G.; Campbell, I.C.; Wishart, J.; O’Brien, K.; Lean, S.M. Micro-Mobility Vehicle Dynamics and Rider Kinematics during Electric Scooter Riding; SAE Technical Paper; SAE: Warrendale, PA, USA, 2020. [Google Scholar] [CrossRef]
- T/ZZB 1970-2020; Electric Balancing Car Tires. Market Supervision Administration of Zhejiang Province: Hangzhou, China, 2020.
- Nie, Y.Q.; Meng, G.W. Mechanics of Materials; China Machine Press: Beijing, China, 2009. [Google Scholar]
- Wu, W.T. Simulation Analysis of Tire Temperature Field. Master’s Thesis, Jilin University, Changchun, China, 2017. [Google Scholar]
- GB/T 1040.2-2022; Plastics-Determination of Tensile Properties-Part 2: Test Conditions for Moulding and Extrusion Plastics. Standards Press of China: Beijing, China, 2022.
- GB/T 528-2009; Rubber, Vulcanized or Thermoplastic-Determination of Tensile Stress-Strain Properties. Standards Press of China: Beijing, China, 2009.
- GB/T 40396-2021; Test Method for Glass Transition Temperature of Polymer Matrix Composites-Dynamic Mechanical Analysis (DMA). Standards Press of China: Beijing, China, 2021.
- GB/T 19466.4-2016; Plastics-Differential Scanning Calorimetry (DSC)-Part 4: Determination of Specific Heat Capacity. Standards Press of China: Beijing, China, 2016.
- GB/T 22588-2008; Determination of Thermal Diffusivity or Thermal Conductivity by the Flash Method. Standards Press of China: Beijing, China, 2008.
- Liu, W.; Liu, S.; Li, X.; Zhang, Q.; Wang, C.; Li, K. Static Stiffness Properties of High Load Capacity Non-Pneumatic Tires with Different Tread Structures. Lubricants 2023, 11, 180. [Google Scholar]





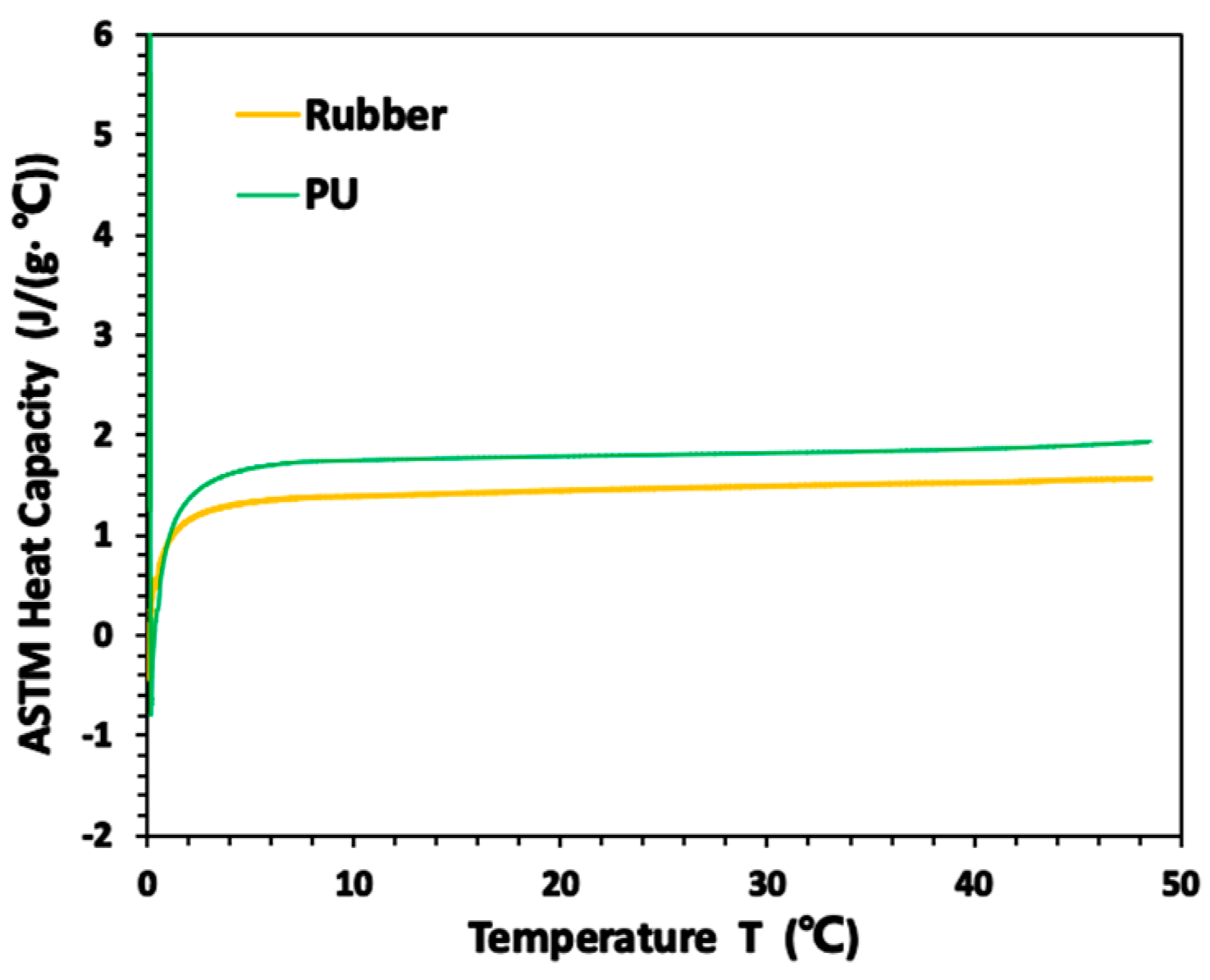



| Material | Specific Heat Capacity (J/(g·°C)) | Density (g/cm3) | Standard Deviation of Density | Thermal Conductivity (W/(m·k)) | Standard Deviation of Thermal Conductivity |
|---|---|---|---|---|---|
| Rubber | 1.476 | 1.206 | 0.12% | 0.310 | 0.04714% |
| PU | 1.805 | 1.135 | 0.16% | 0.220 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Liu, W.; Zhou, S.; Li, X.; Zhang, Q. Steady-State Temperature Field and Rolling Resistance Characteristics of Low-Speed and Low-Load Capacity Non-Pneumatic Tires. Lubricants 2023, 11, 402. https://doi.org/10.3390/lubricants11090402
Liu S, Liu W, Zhou S, Li X, Zhang Q. Steady-State Temperature Field and Rolling Resistance Characteristics of Low-Speed and Low-Load Capacity Non-Pneumatic Tires. Lubricants. 2023; 11(9):402. https://doi.org/10.3390/lubricants11090402
Chicago/Turabian StyleLiu, Shuo, Weidong Liu, Shen Zhou, Xiujuan Li, and Qiushi Zhang. 2023. "Steady-State Temperature Field and Rolling Resistance Characteristics of Low-Speed and Low-Load Capacity Non-Pneumatic Tires" Lubricants 11, no. 9: 402. https://doi.org/10.3390/lubricants11090402
APA StyleLiu, S., Liu, W., Zhou, S., Li, X., & Zhang, Q. (2023). Steady-State Temperature Field and Rolling Resistance Characteristics of Low-Speed and Low-Load Capacity Non-Pneumatic Tires. Lubricants, 11(9), 402. https://doi.org/10.3390/lubricants11090402





