Influence of Cavitation on the Heat Transfer of High-Speed Mechanical Seal with Textured Side Wall
Abstract
:1. Introduction
2. Numerical Models and Boundary Conditions
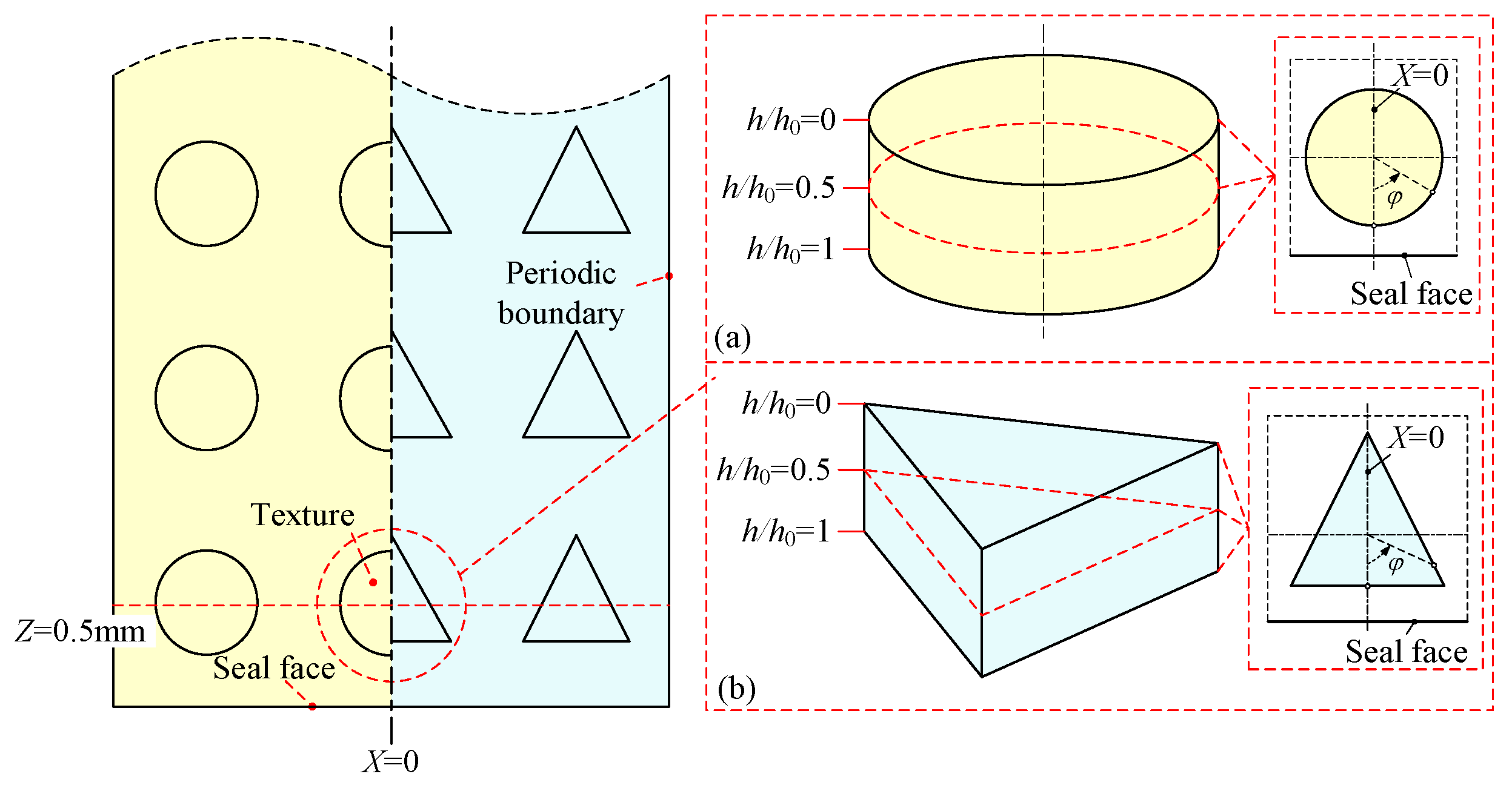
2.1. Geometric Model
2.2. Governing Equation
2.3. Boundary Conditions
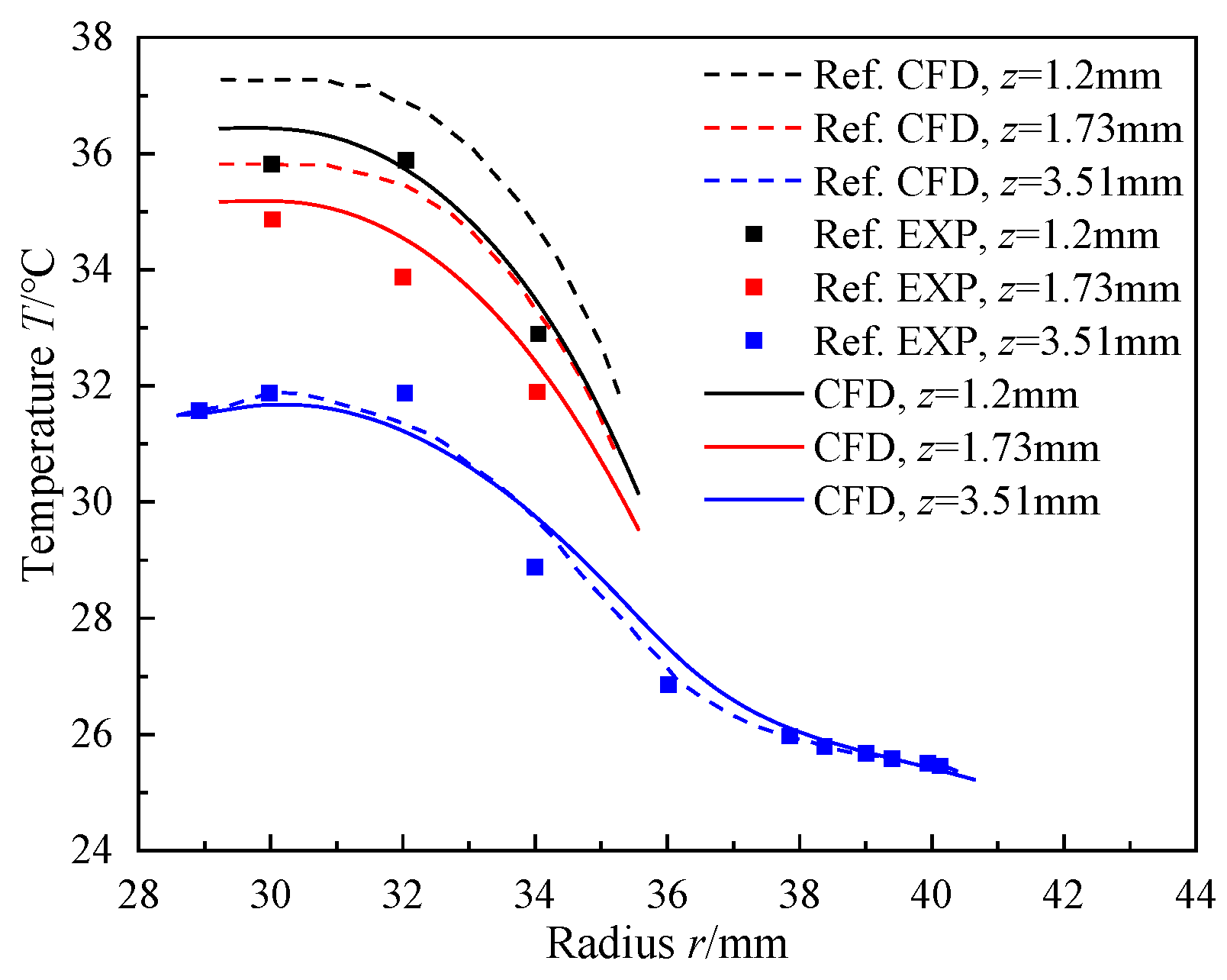
2.4. Grid Independence Validation and Model Validation
2.5. Sampling Location Description
3. Results and Discussion
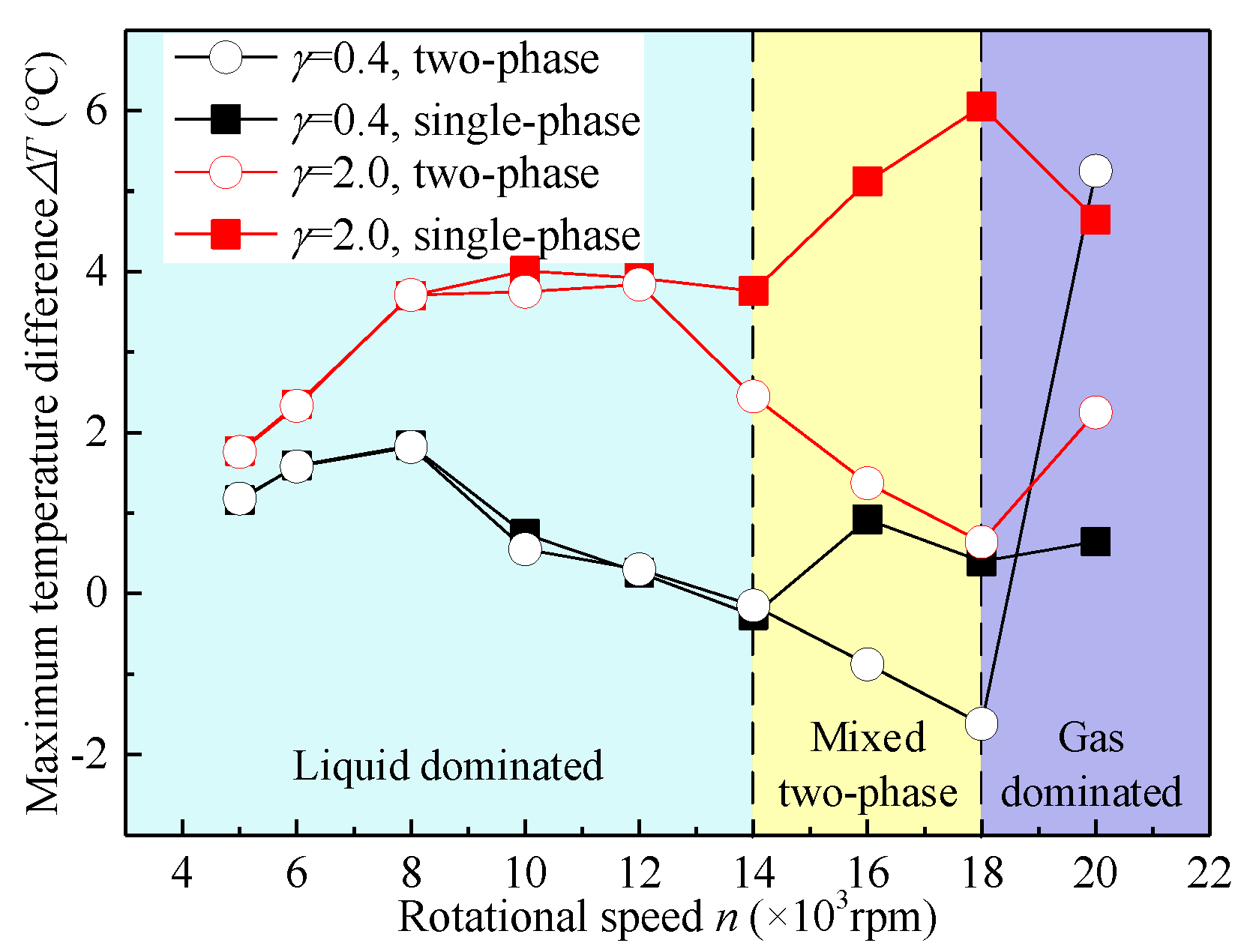
3.1. Influence of Rotational Speed on the Maximum Temperature of Seal Face
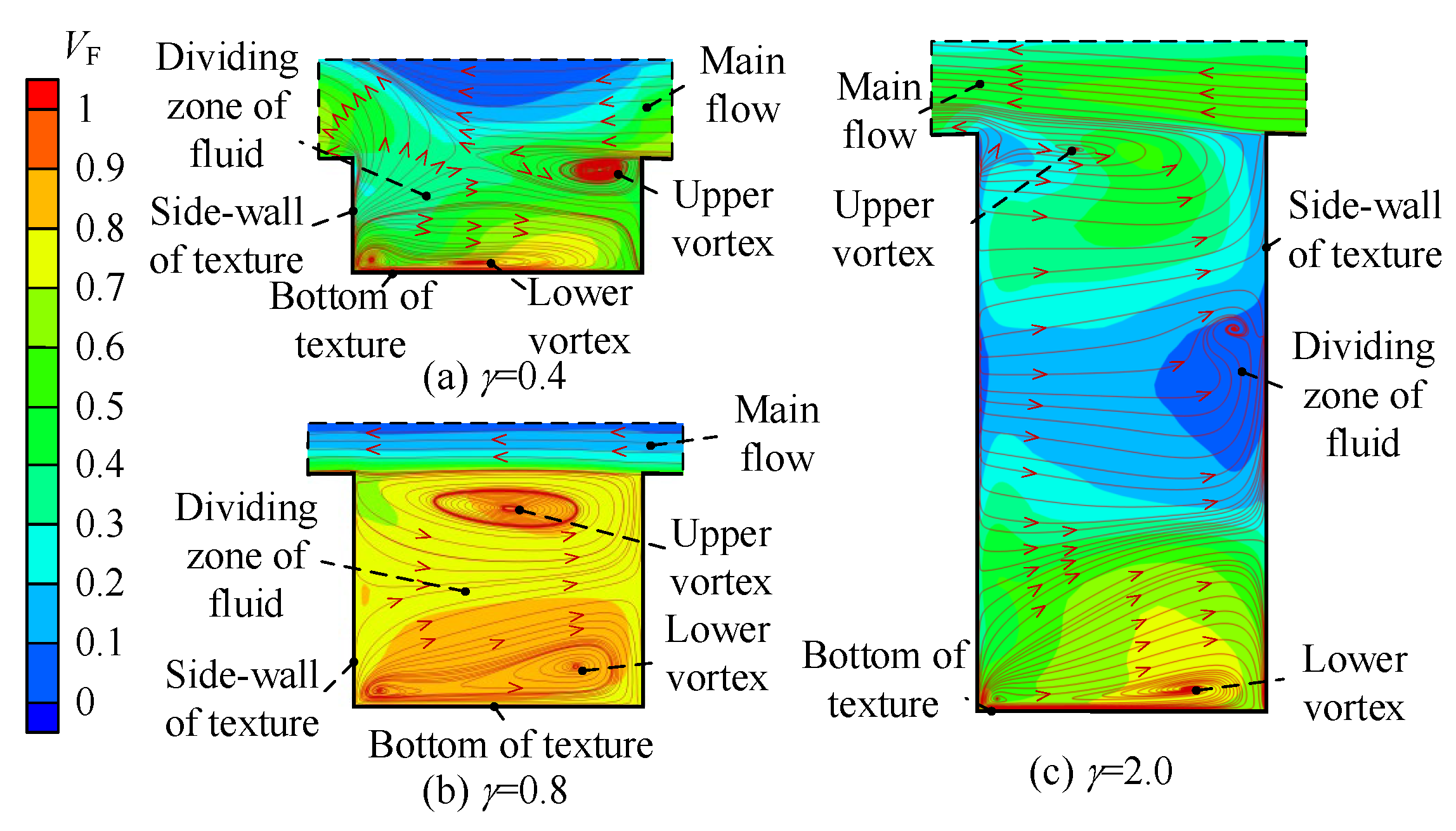
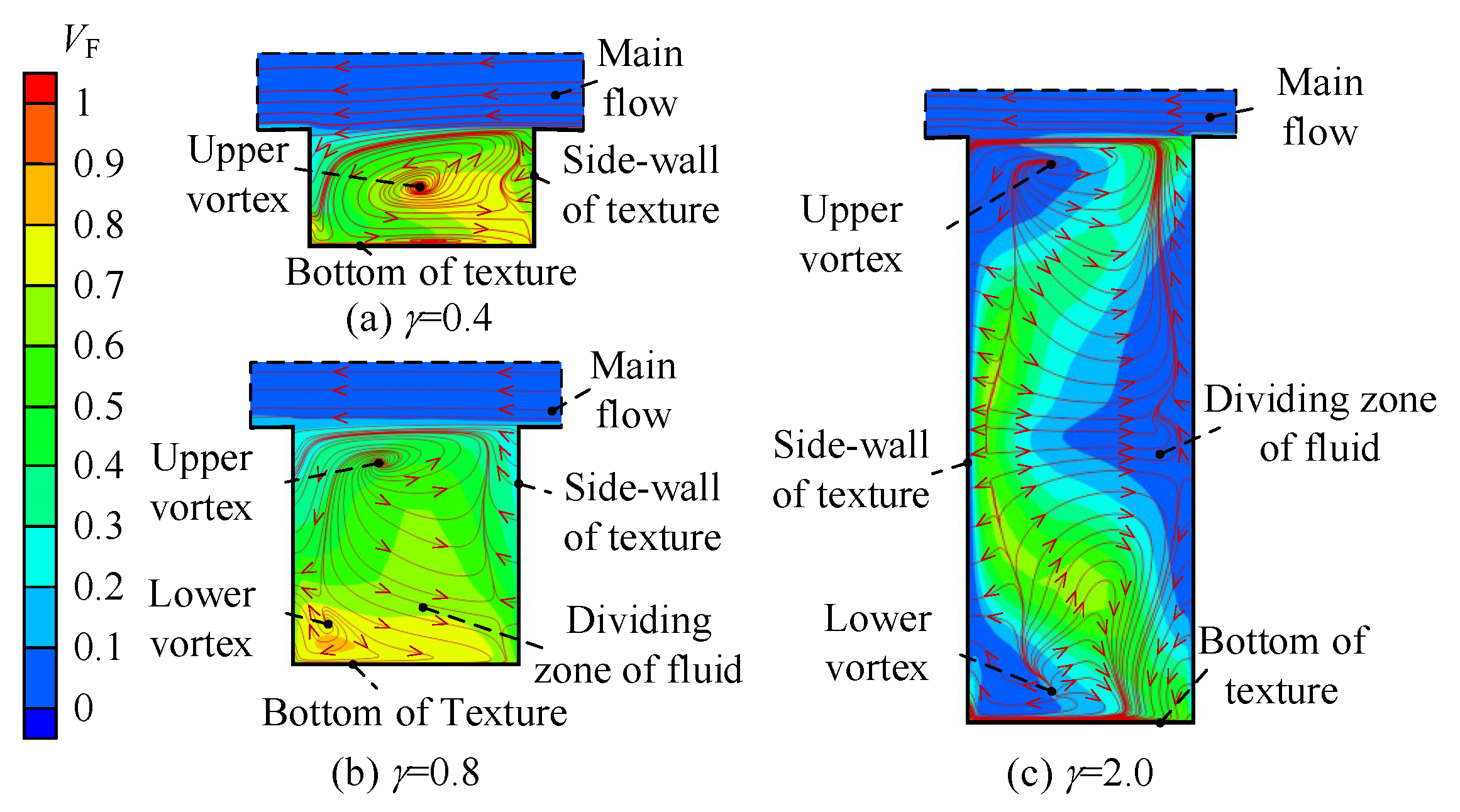
3.2. Influence of the Shape and the Depth-to-Diameter Ratio of Textures
4. Conclusions
- Cavitation intensity is related to the maximum temperature of the seal face. When the cavitation becomes stronger, the heat transfer of the surface is weakened, and the maximum temperature of the seal face increases;
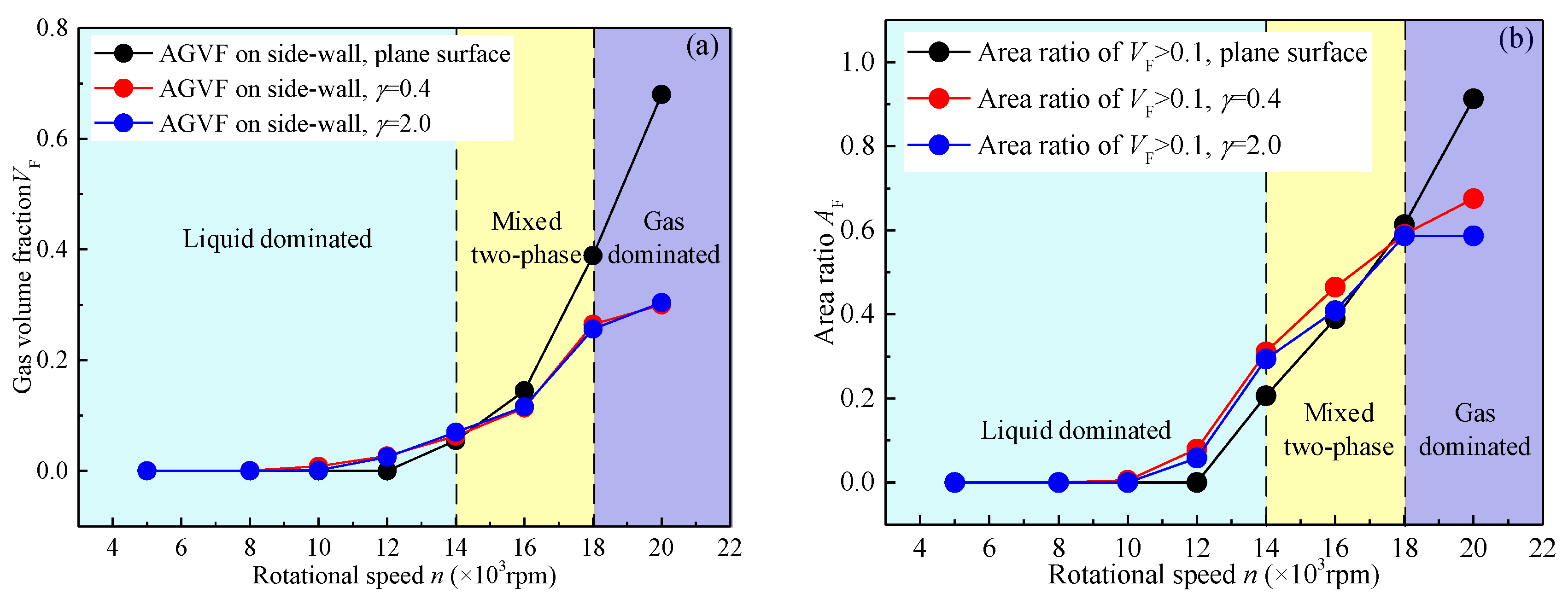
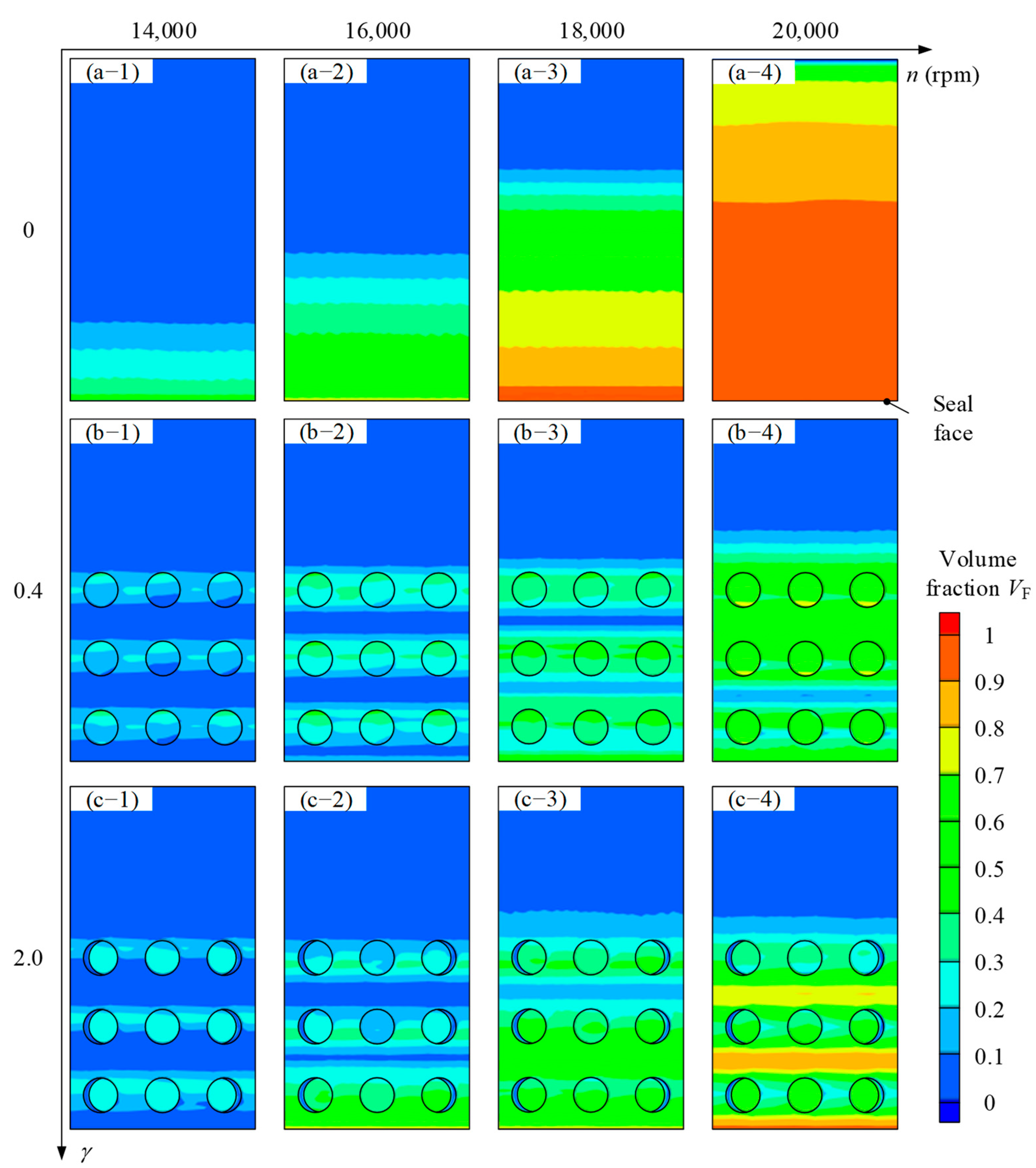
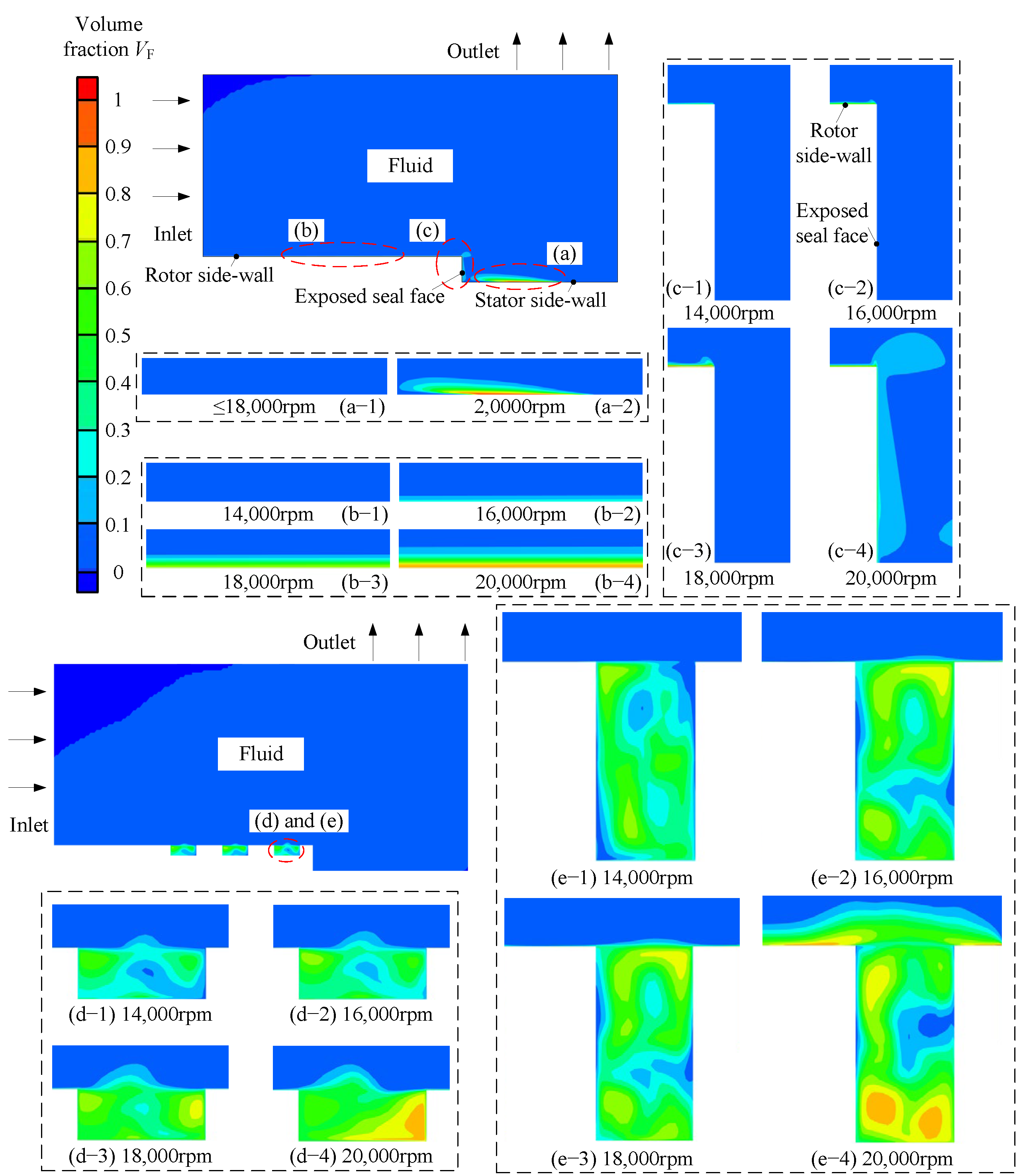
- The cavitation intensity of the side wall increases with the increase of rotational speed, but the law of cavitation intensity on three surfaces is different. Cavitation intensity on the plane surface occurs at the latest, but it grows fastest, leading to three stages of the gas phase: liquid-dominated, mixed two-phase, and gas-dominated. These stages cause a big difference in heat transfer;
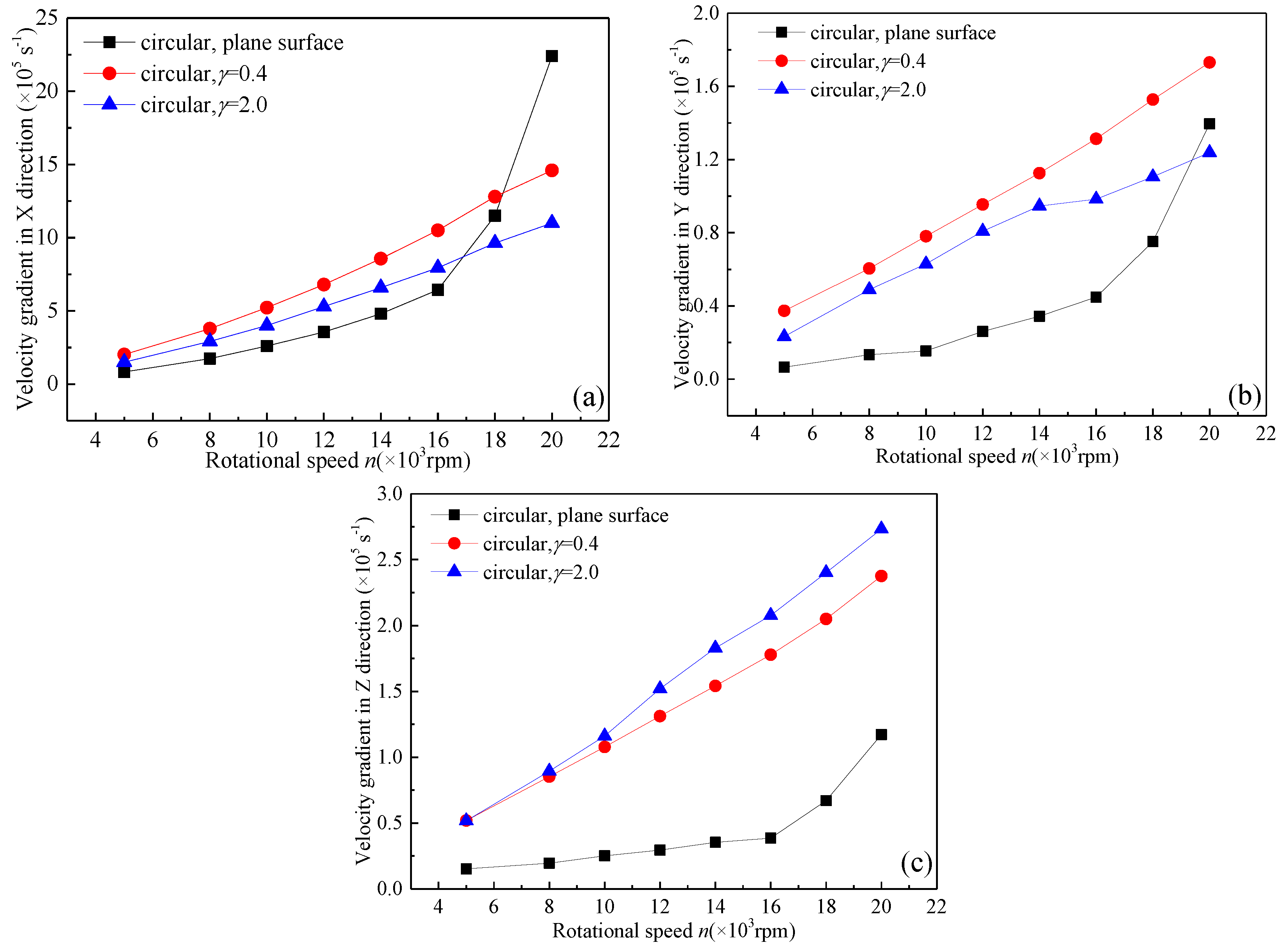
- Cavitation intensity can be explained by velocity gradient. The velocity gradient is positively correlated with turbulent kinetic energy dissipation. At first, the velocity gradient on textured surfaces is smaller than that on plane surfaces but soon catches up with the increase of rotational speed, and the change of cavitation intensity is also the same;
- The increase in depth-to-diameter ratio produces two opposite effects: (1) it increases the area of heat transfer, and (2) it causes fluid stratification in the textures. The first will enhance heat transfer, and the second will weaken heat transfer. In a deep texture (γ = 1.6 or 2.0), the first is dominant when the fluid is mainly liquid, and the second is dominant when the fluid is mainly gas. This is because the smaller specific heat capacity and thermal conductivity of the gas lead to poor heat transfer after stratification, but the liquid is less affected;
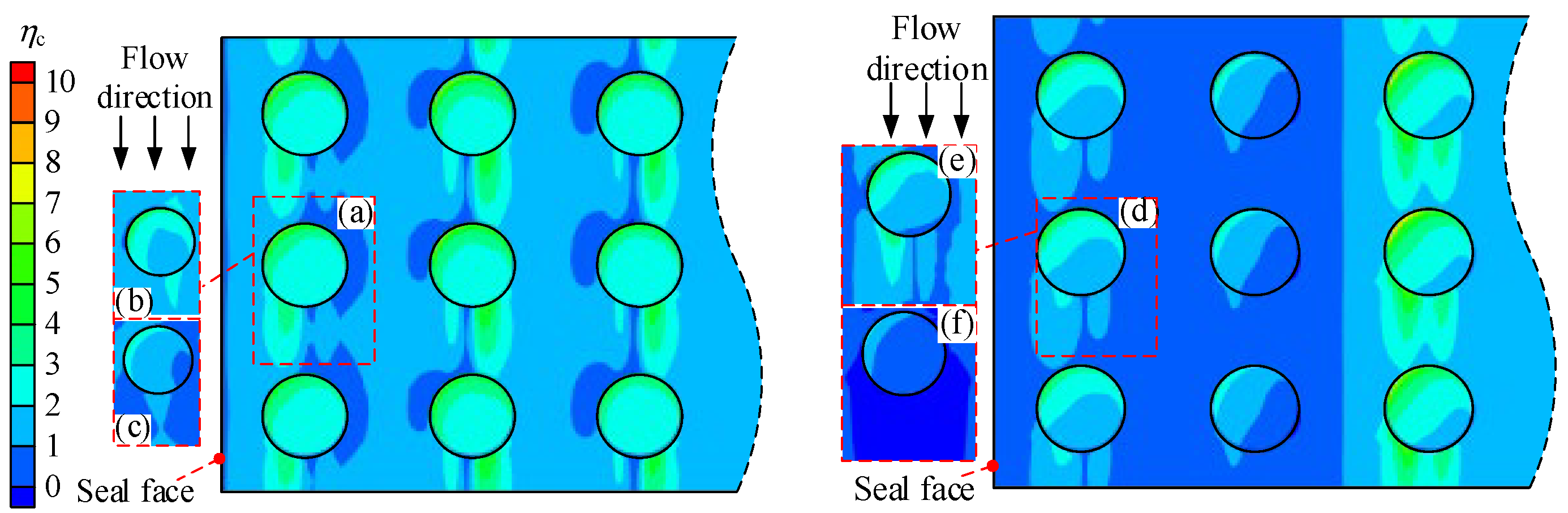
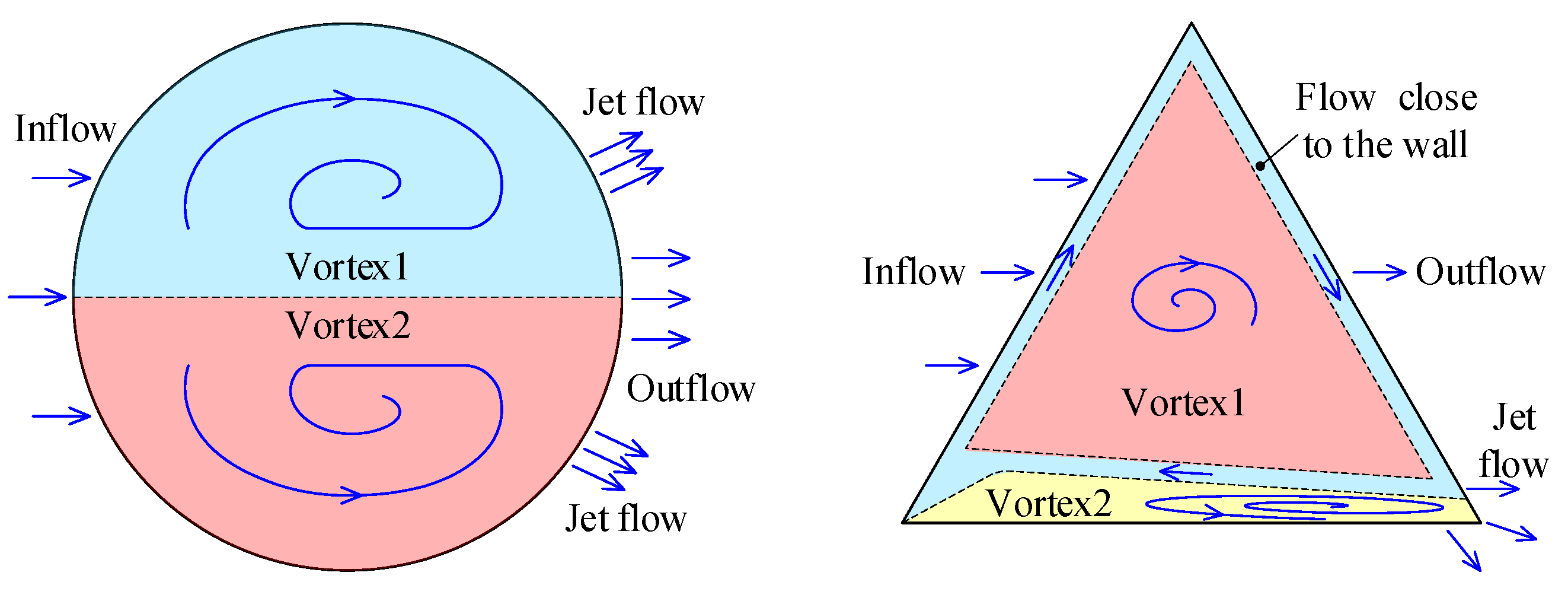
- The shape of textures will influence the flow field and pressure field on the textured side wall. First, the fluid stratification inside the triangle is more serious after the depth-to-diameter ratio increases. Second, if the two kinds of textures have similar hydraulic diameter and depth, the circular texture has a larger area of heat transfer. The first reason makes the shallow triangular texture perform better in multiphase, while the second reason makes the deep, circular texture perform better in single-phase;
- According to the previous conclusions, a deep, circular texture is recommended when cavitation will not occur (situations with high pressure and low speed), and a shallow triangular texture is better when cavitation occurs (situations with low pressure and high speed, such as a high-speed turbopump).
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| a | Axial gap of texture mm |
| b | Spacing to seal face mm |
| cp | Specific heat capacity J·kg−1·K−1 |
| d | Characteristic length of texture mm |
| d1 | Outer diameter of fluid zone mm |
| d2 | Outer diameter of seal face mm |
| d3 | Inner diameter of seal face mm |
| d4 | Outer diameter of stator mm |
| d5 | Inner diameter of stator mm |
| F | Model decision parameter |
| f | coefficient of friction |
| h0 | Depth of texture mm |
| k | Thermal conductivity W·m−1·K−1 |
| k0 | Turbulent kinetic energy m−2·s−2 |
| ld | Rotor length mm |
| ls | Stator length mm |
| n1 | Row number |
| n | rotational speed rpm |
| q | Heat flux on the wall W·m−2 |
| T | Ambient temperature K |
| ∆T | Maximum temperature difference °C |
| Tmax | Maximum temperature on the seal face of ring with cavitation °C |
| tmax | Maximum temperature on the seal face of ring without cavitation °C |
| u | Velocity in a certain direction m·s−1 |
| VF | Gas volume fraction |
| velocity vector m·s−1 | |
| X, Y, Z | Coordinate system directions mm |
| z | z-directions used in Ref. mm |
| y+ | Grid parameters of coupled wall |
| Simplification of nomenclature | |
| AGVF | Average gas volume fraction |
| FRT | First row of textures |
| LGVF | Local gas volume fraction |
| VG | Velocity gradient |
| Greek letters | |
| α | The ratio of shear stress to turbulent kinetic energy inside boundary layer |
| α0 | Circumferential gap of texture ° |
| β | Constant of dissipation term used in k-equation |
| Φ, Φ1, Φ2 | Intermediate values used in the calculation of each parameter |
| γ | Depth-to-diameter ratio of textures |
| η | Ratio of local Nu number and average Nu number on side wall |
| μ | Dynamic viscosity of fluid Pa·s |
| ρ | Density kg∙m−3 |
| σk | Constant of diffusion term used in k-equation |
| σω | Constant of diffusion term used in ω-equation |
| ν | Kinematic viscosity m2∙s−1 |
| ω | Vorticity s−1 |
| ω0 | Turbulent dissipation rate m2∙s−2 |
| Subscript | |
| g, l, m | Gas phase, liquid phase, and mixed phase |
| i, j | Component of the vector in the coordinate direction |
References
- Peng, X.; Jin, J.; Li, D.; Jiang, J.; Meng, X.; Li, J. Analysis of Face Temperature in Mechanical Seal Applied in the High Speed Turbo pump. Tribology 2019, 39, 313–318. [Google Scholar] [CrossRef]
- Cheng, W.; Meng, X.; Wang, Y.; Liang, Y.; Peng, X. Finite Element Model and Tribological Properties Analysis of Textured Mechanical Seals Considering Roughness Effect. Tribology 2019, 39, 523–530. [Google Scholar] [CrossRef]
- Luan, Z.; Khonsari, M. Heat transfer correlations for laminar flows within a mechanical seal chamber. Tribol. Int. 2008, 42, 770–778. [Google Scholar] [CrossRef]
- Qiu, Y.; Meng, X.; Liang, Y.; Peng, X. Thermal mixing effect and heat transfer in U-shaped notch on the mechanical seal face. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2020, 235, 1924–1936. [Google Scholar] [CrossRef]
- Chen, H.; Gui, K.; Li, X.; Han, T.; Xie, X.; Lu, J. Influences of Operating Parameters on Vaporization Characteristics and Properties of Liquid Films for Mechanical Seals. China Mech. Eng. 2021, 32, 2–11. [Google Scholar] [CrossRef]
- Wu, T.Y.T. Cavity and Wake Flows. Annu. Rev. Fluid Mech. 1972, 4, 243–284. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, J.; Shen, Z.; Chen, Y. Influence of Boundary Pressure Fluctuation on Cavitation and Performance of Liquid Film End Face Mechanical Seal. Chem. Ind. Eng. Prog. 2022, 41, 5175–5187. [Google Scholar] [CrossRef]
- Li, X.; Yang, Z.; Xu, J.; Chen, R.; Yang, H. The fractal leakage model of contact mechanical seals considering wear and thermal deformation. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 521. [Google Scholar] [CrossRef]
- Li, B.; Sun, J.; Zhu, S.; Fu, Y.; Zhao, X.; Wang, H.; Teng, Q.; Ren, Y.; Li, Y.; Zhu, G. Thermohydrodynamic lubrication analysis of misaligned journal bearing considering the axial movement of journal. Tribol. Int. 2019, 135, 397–407. [Google Scholar] [CrossRef]
- Wang, Q.; Hu, Y.-P.; Ji, H.-H. Leakage, heat transfer and thermal deformation analysis method for contacting finger seals based on coupled porous media and real structure models. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020, 234, 2077–2093. [Google Scholar] [CrossRef]
- Peng, C.; Ouyang, X.; Schmitz, K.; Wang, W.; Guo, S.; Yang, H. Investigation of the tribological performance of reciprocating seals in a wide temperature range. Proc. IMechE Part J J. Eng. Tribol. 2021, 235, 2396–2414. [Google Scholar] [CrossRef]
- Chávez, A.; De Santiago, O. Experimental measurements of the thermo elastic behavior of a dry gas seal operating with logarithmic spiral grooves of 11° and 15°. Proc. IMechE Part J J. Eng. Tribol. 2020, 235, 1807–1819. [Google Scholar] [CrossRef]
- Meng, X.; Zhao, W.; Shen, M.; Peng, X. Thermohydrodynamic analysis on herringbone-grooved mechanical face seals with a quasi-3D model. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2018, 232, 1402–1414. [Google Scholar] [CrossRef]
- Xiao, N.; Khonsari, M. Thermal performance of mechanical seals with textured side-wall. Tribol. Int. 2012, 45, 1–7. [Google Scholar] [CrossRef]
- Nian, X.; Khonsari, M.M. Improving Thermal Performance of Mechanical Seals with Surface Texturing. Proc. IMechE Part J J. Eng. Tribol. 2015, 229, 350–361. [Google Scholar] [CrossRef]
- Zhou, Y.; Peng, X.; Zhao, W.; Jiang, J.; Meng, X. Study on Heat Transfer Mechanism and Optimization of Circumferential Texture of Mechanical Seal. Tribology 2020, 4, 538–550. [Google Scholar] [CrossRef]
- Afanasyev, V.; Chudnovsky, Y.; Leontiev, A.; Roganov, P. Turbulent flow friction and heat transfer characteristics for spherical cavities on a flat plate. Exp. Therm. Fluid Sci. 1993, 7, 1–8. [Google Scholar] [CrossRef]
- Chyu, M.K.; Yu, Y.; Ding, H.; Downs, J.P.; Soechting, F.O. Concavity Enhanced Heat Transfer in an Internal Cooling Passage. In Proceedings of the ASME 1997 International Gas Turbine and Aeroengine Congress and Exhibition, Orlando, FL, USA, 2–5 June 1997. [Google Scholar] [CrossRef]
- Xu, L.; Wu, J.H.; Wang, Y.; Rafique, F.; Xu, J.; Yuan, X. Lubrication and stability enhancement by attaching superconducting magnetic force on non-contacting mechanical seals for reusable rocket turbopump. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 236, 892–907. [Google Scholar] [CrossRef]
- Elrod, H.G. A cavitation algorithm. J. Lubr. Technol. 1981, 103, 350–354. [Google Scholar] [CrossRef]
- Vijayaraghavan, D.; Keith, T.G. Development and Evaluation of a Cavitation Algorithm. Tribol. Trans. 1989, 32, 225–233. [Google Scholar] [CrossRef]
- Han, Y.; Meng, Q.; de Boer, G. Two-scale homogenization of hydrodynamic lubrication in a mechanical seal with isotropic roughness based on the Elrod cavitation algorithm. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 236, 359–385. [Google Scholar] [CrossRef]
- Guo, Q.; Zhou, L.; Wang, Z.; Liu, M.; Cheng, H. Numerical simulation for the tip leakage vortex cavitation. Ocean Eng. 2018, 151, 71–81. [Google Scholar] [CrossRef]
- Song, Y.; Gu, C.-W. Development and Validation of a Three-Dimensional Computational Fluid Dynamics Analysis for Journal Bearings Considering Cavitation and Conjugate Heat Transfer. J. Eng. Gas Turbines Power 2015, 137, 122502. [Google Scholar] [CrossRef]
- Ge, M.; Petkovšek, M.; Zhang, G.; Jacobs, D.; Coutier-Delgosha, O. Cavitation dynamics and thermodynamic effects at elevated temperatures in a small Venturi channel. Int. J. Heat Mass Transf. 2021, 170, 120970. [Google Scholar] [CrossRef]
- Ge, M.; Manikkam, P.; Ghossein, J.; Subramanian, R.K.; Coutier-Delgosha, O.; Zhang, G. Dynamic mode decomposition to classify cavitating flow regimes induced by thermodynamic effects. Energy 2022, 254, 124426. [Google Scholar] [CrossRef]
- Ge, M.; Zhang, G.; Petkovšek, M.; Long, K.; Coutier-Delgosha, O. Intensity and regimes changing of hydrodynamic cavitation considering temperature effects. J. Clean. Prod. 2022, 338, 130470. [Google Scholar] [CrossRef]
- Ge, M.; Sun, C.; Zhang, G.; Coutier-Delgosha, O.; Fan, D. Combined suppression effects on hydrodynamic cavitation performance in Venturi-type reactor for process intensification. Ultrason. Sonochemistry 2022, 86, 106035. [Google Scholar] [CrossRef]
- Li, W.; Yu, Z.; Kadam, S. An improved cavitation model with thermodynamic effect and multiple cavitation regimes. Int. J. Heat Mass Transf. 2023, 205, 123854. [Google Scholar] [CrossRef]
- Tian, L.M.; Ren, L.Q.; Liu, Q.P.; Han, Z.W.; Jiang, X. The mechanism of drag reduction around bodies of revolution using bionic non-smooth surfaces. J. Bionic Eng. 2007, 4, 109–116. [Google Scholar] [CrossRef]
- Zhao, J. Study of Drag Reduction Capability of the Dimple Bionic Non-Smooth Surface. Masteral Dissertation, Dalian University of Technology, Dalian, China, 2008. [Google Scholar]
- Tao, W. Numerical Heat Transfer; Xi’an Jiaotong University Press: Shaanxi, Xi’an, 2001. [Google Scholar]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Wang, Z. Parameter Modification of SST k-ω Turbulence Model Based on Bluff Body Wind Pressure Data. Masteral Dissertation, Harbin Institute of Technology, Harbin, China, 2020. [Google Scholar]
- Robinson, S.K. Coherent Motions in the Turbulent Boundary Layer. Annu. Rev. Fluid Mech. 1991, 23, 601–639. [Google Scholar] [CrossRef]
- Merati, P.; Okita, N.A.; Phillips, R.L.; Jacobs, L.E. Experimental and Computational Investigation of Flow and Thermal Behavior of a Mechanical Seal. Tribol. Trans. 1999, 42, 731–738. [Google Scholar] [CrossRef]
- Warda, H.; Adam, I.; Rashad, A.; Gamal-Aldin, M. Bi-directional integral pumping devices for dual mechanical seals: Influence of mesh type on accuracy of numerical simulations. Alex. Eng. J. 2018, 57, 3255–3264. [Google Scholar] [CrossRef]
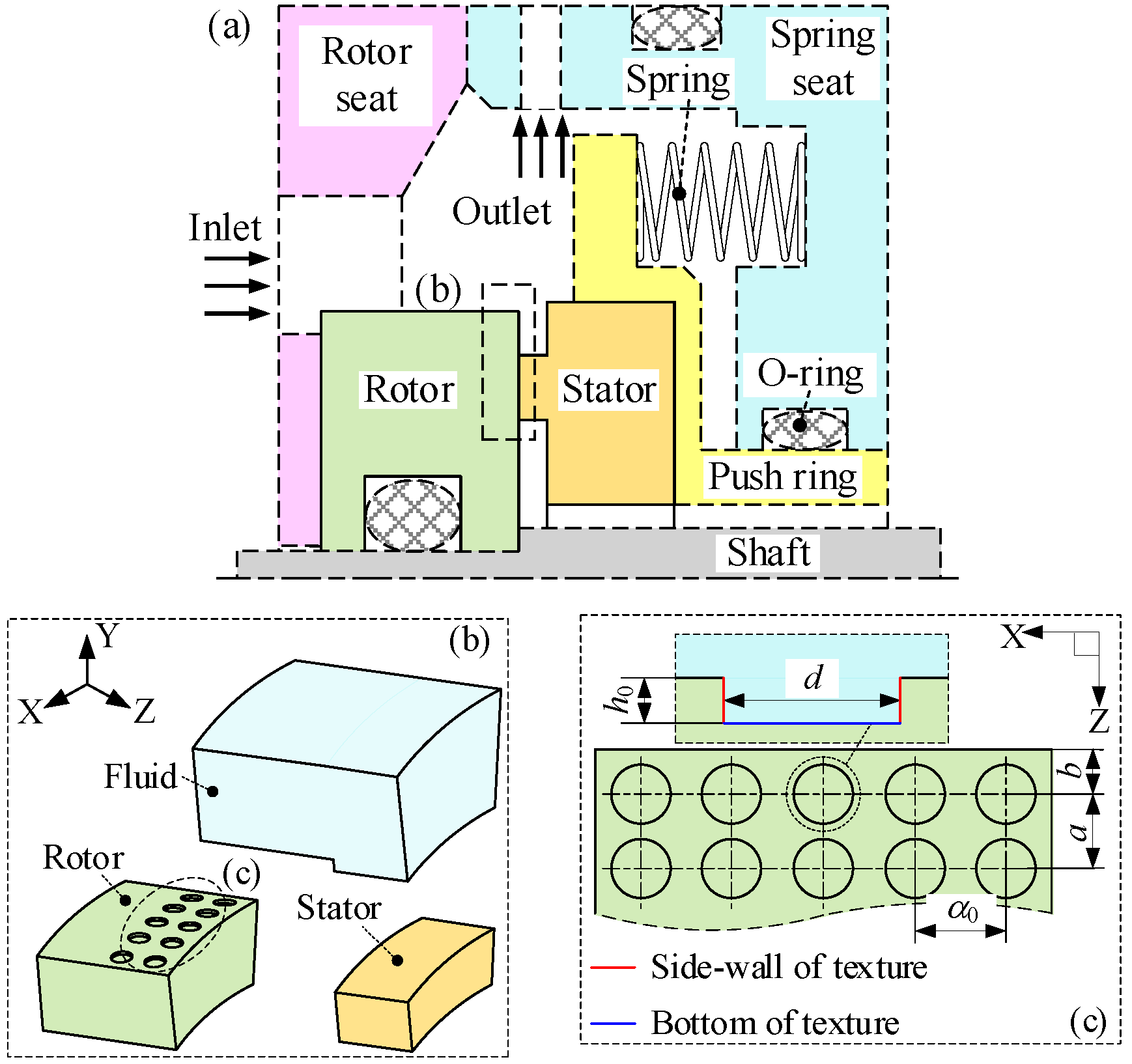
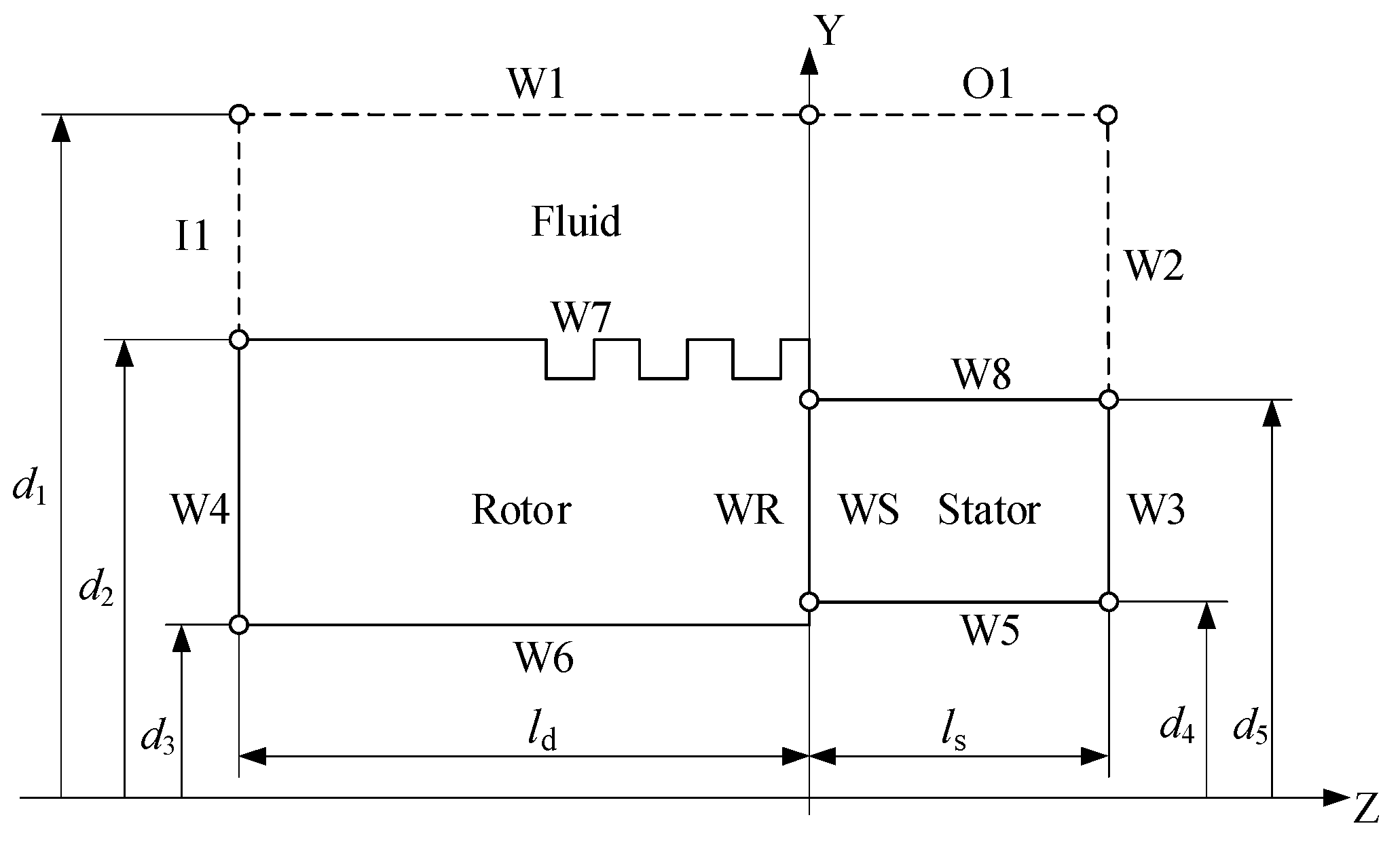
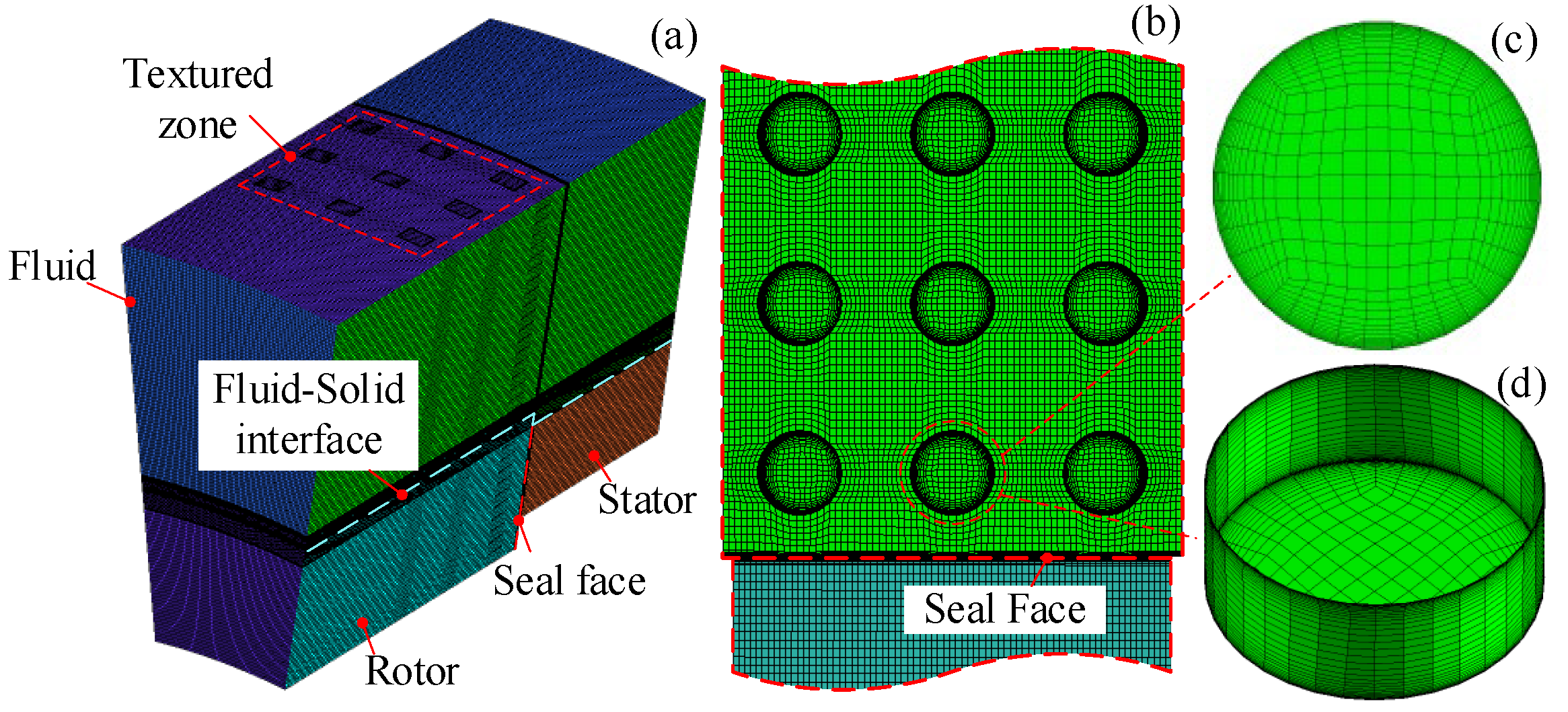
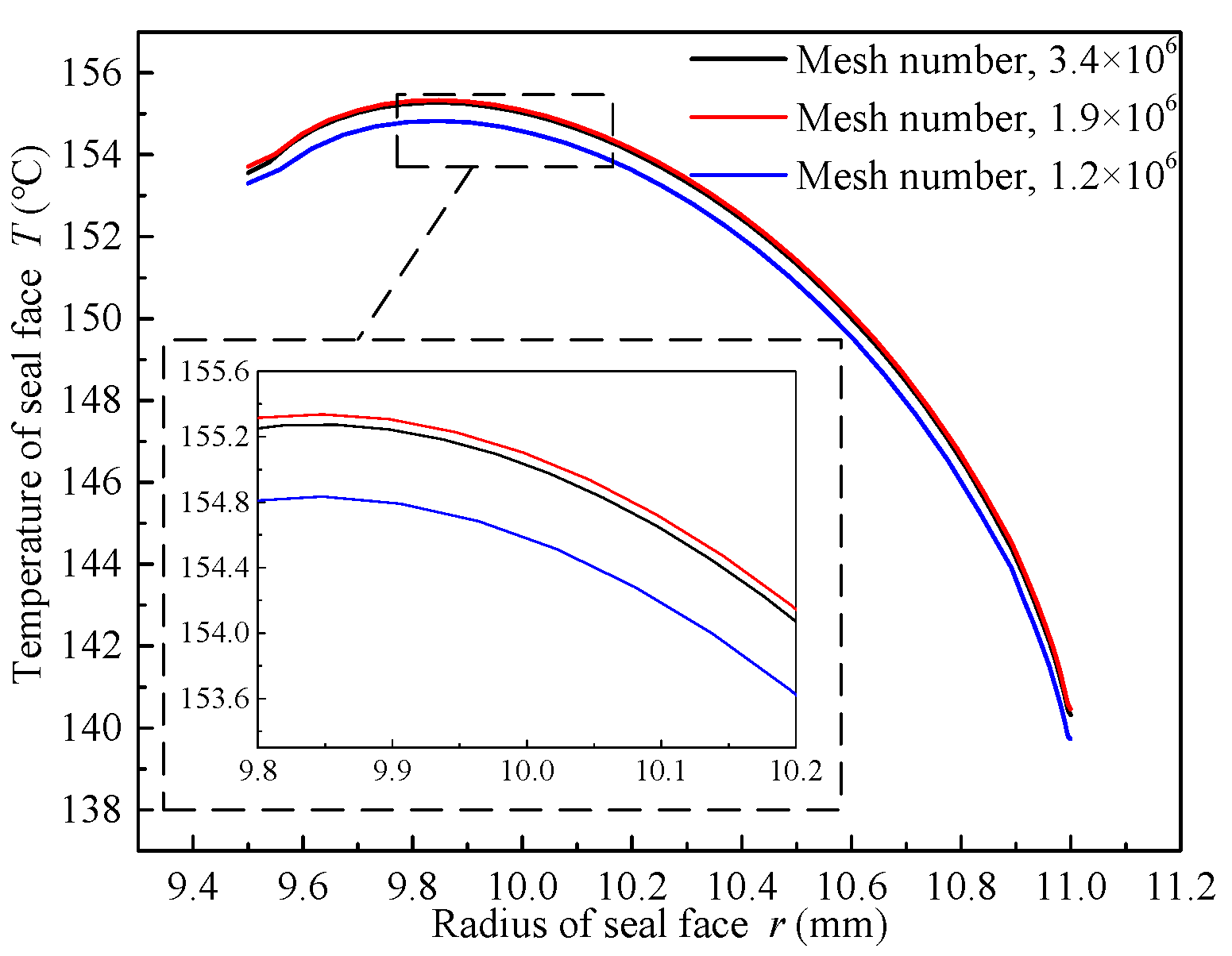


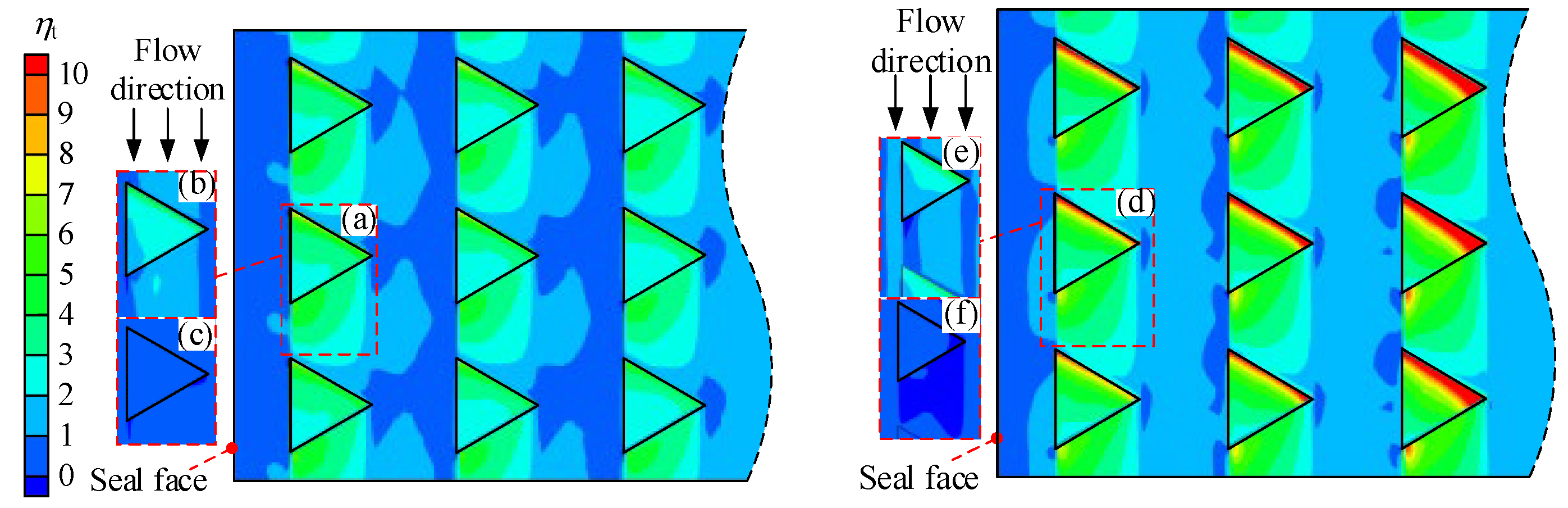
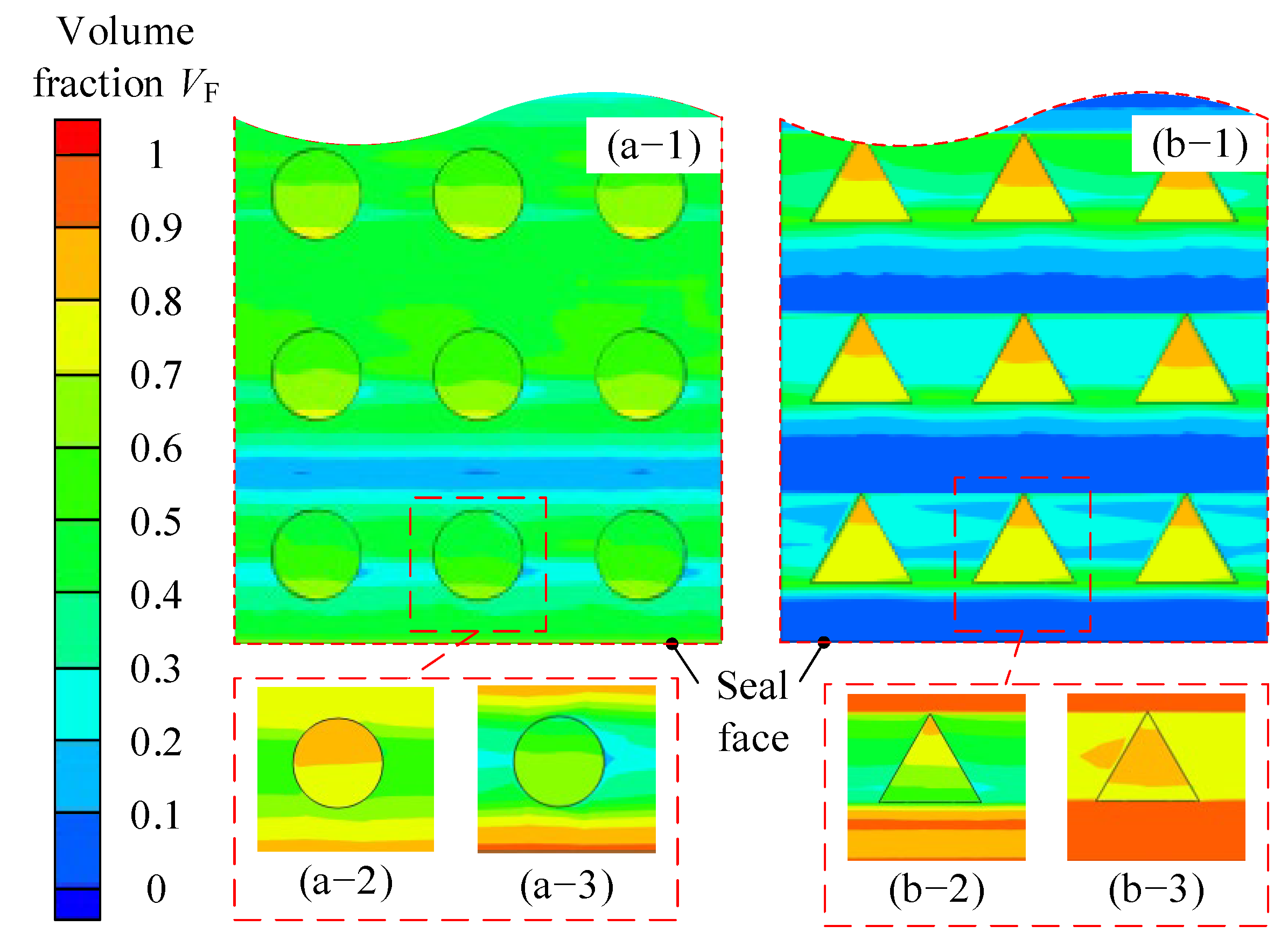
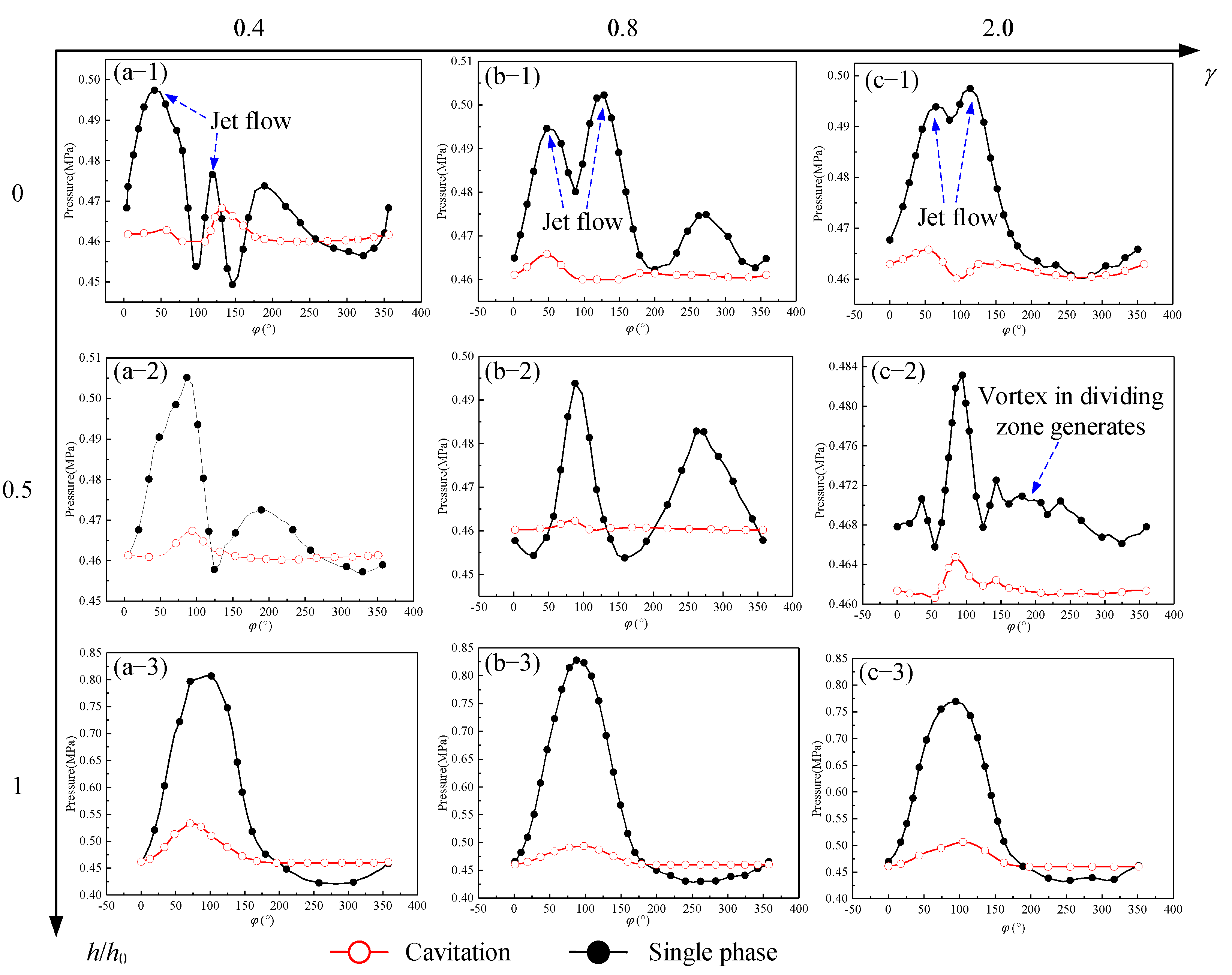
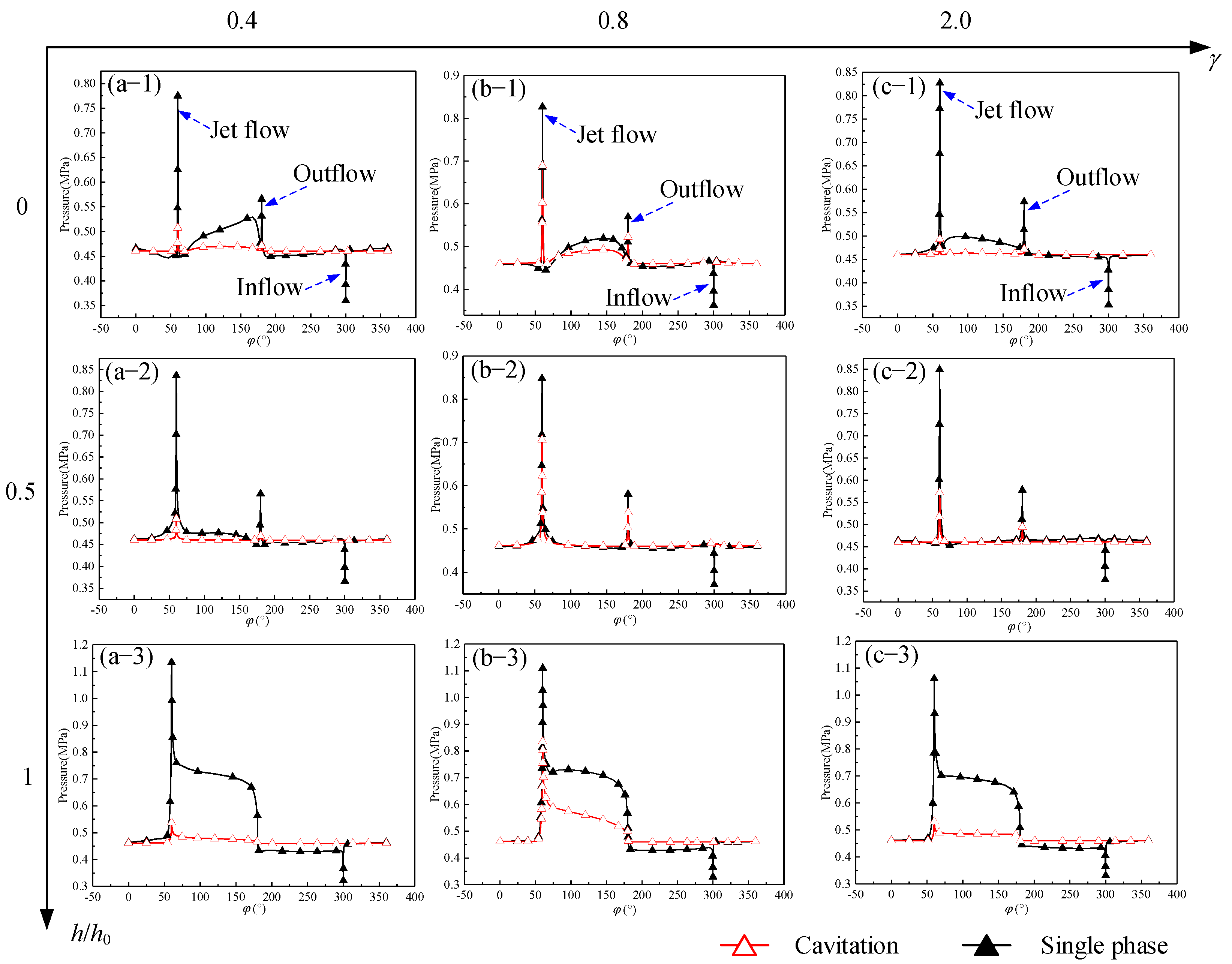

| Variable | Parameter Name | Value | Variable | Parameter Name | Value |
|---|---|---|---|---|---|
| d1 | Outer diameter of fluid zone/mm | 30 | d5 | Inner diameter of stator/mm | 19 |
| d2 | Outer diameter of seal face/mm | 23 | ld | Rotor length/mm | 5 |
| d3 | Inner diameter of seal face/mm | 18 | ls | Stator length/mm | 3 |
| d4 | Outer diameter of stator/mm | 22 | n1 | Row number | 3 |
| a | Axial gap of texture/mm | 1 | d | Characteristic length of texture/mm | 0.5 |
| b | Spacing to seal face/mm | 0.5 | h0 | Depth of texture/mm | 0.2~1 |
| α0 | Circumferential gap of texture/° | 4 |
| Boundary | Type | Thermal Condition | Comments |
|---|---|---|---|
| I1 | Velocity inlet | T = 413 K | vi = 0.9 m/s |
| O1 | Pressure outlet | T = 413 K | po = 0.47 MPa |
| W1–W2 | Wall, constant T | T = 413 K | / |
| W3–W6 | Wall, insulation | q = 0 W/m2 | / |
| WR | Wall, heat generation | User define function | Interface, f = 0.15 |
| WS | Wall, heat generation | User define function | Interface, f = 0.15 |
| W7–W8 | Wall, couple face | Coupled | n = 5000~20,000 rpm |
| Constant | ϕ1 | ϕ2 |
|---|---|---|
| σk | 1.176 | 1 |
| σω | 2 | 1.168 |
| β | 0.075 | 0.0828 |
| α | 0.31 | 0.31 |
| Material | Thermal Conductivity k (W·m−1·K−1) | Density ρ (kg·m−3) | Specific Heat Capacity cp (J·kg−1·K−1) | Viscosity μ (Pa·s) |
|---|---|---|---|---|
| 9 Cr18 | 30 | 10,200 | 279 | / |
| Carbon graphite | 51 | 1930 | 813.5 | / |
| Water (liquid) | 0.6 | 1000 | 4182 | 1.003 × 10−3 |
| Water (vapor) | 0.261 | 0.5542 | Piecewise–Polynomial | 1.34 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, M.; Peng, X.; Meng, X.; Jiang, J.; Ma, Y. Influence of Cavitation on the Heat Transfer of High-Speed Mechanical Seal with Textured Side Wall. Lubricants 2023, 11, 378. https://doi.org/10.3390/lubricants11090378
Yu M, Peng X, Meng X, Jiang J, Ma Y. Influence of Cavitation on the Heat Transfer of High-Speed Mechanical Seal with Textured Side Wall. Lubricants. 2023; 11(9):378. https://doi.org/10.3390/lubricants11090378
Chicago/Turabian StyleYu, Minfeng, Xudong Peng, Xiangkai Meng, Jinbo Jiang, and Yi Ma. 2023. "Influence of Cavitation on the Heat Transfer of High-Speed Mechanical Seal with Textured Side Wall" Lubricants 11, no. 9: 378. https://doi.org/10.3390/lubricants11090378
APA StyleYu, M., Peng, X., Meng, X., Jiang, J., & Ma, Y. (2023). Influence of Cavitation on the Heat Transfer of High-Speed Mechanical Seal with Textured Side Wall. Lubricants, 11(9), 378. https://doi.org/10.3390/lubricants11090378





