Analyzing the Fretting Fatigue of Bolt Joints by Experiments and Finite Element Analysis
Abstract
:1. Introduction
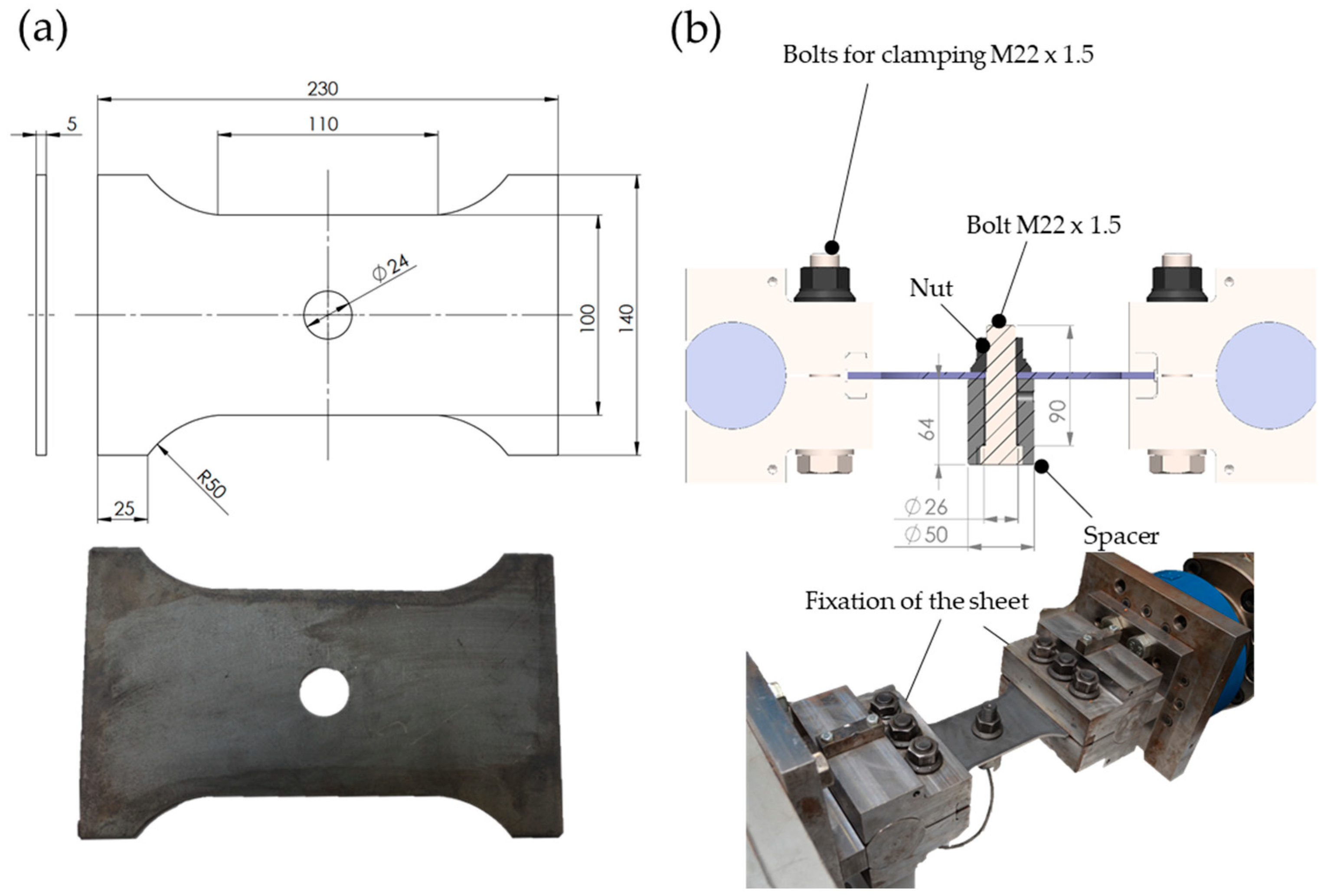
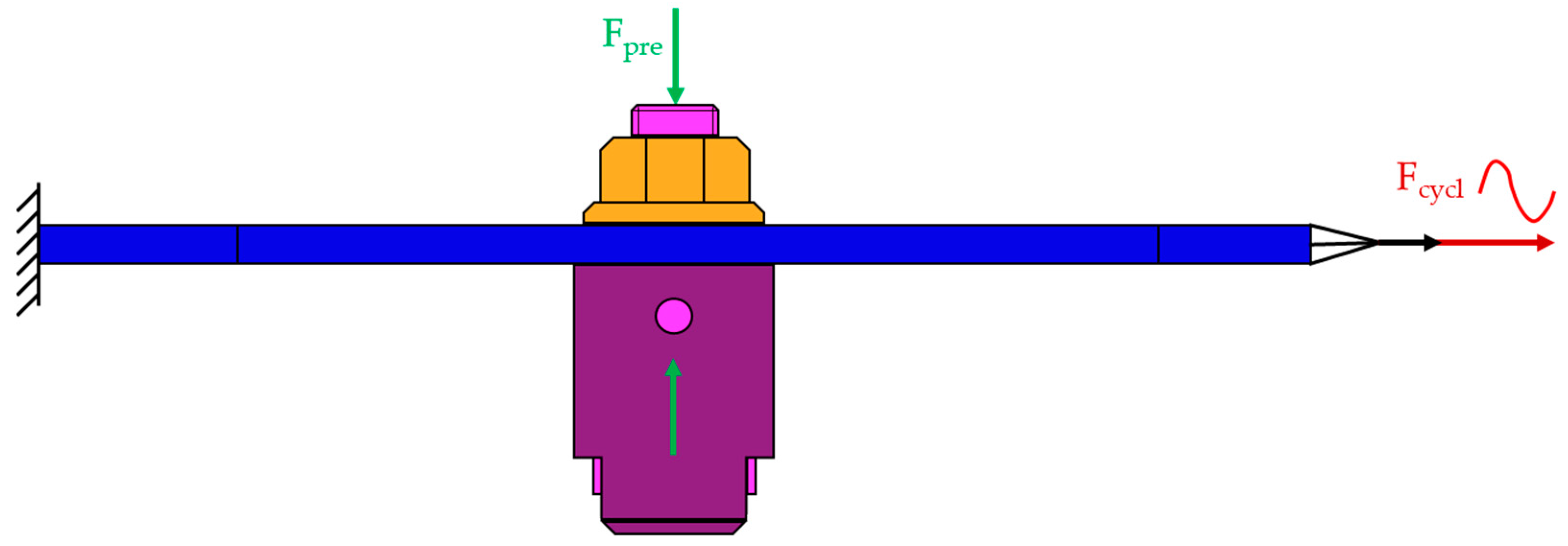
2. Materials and Methods
2.1. Experimental Setup
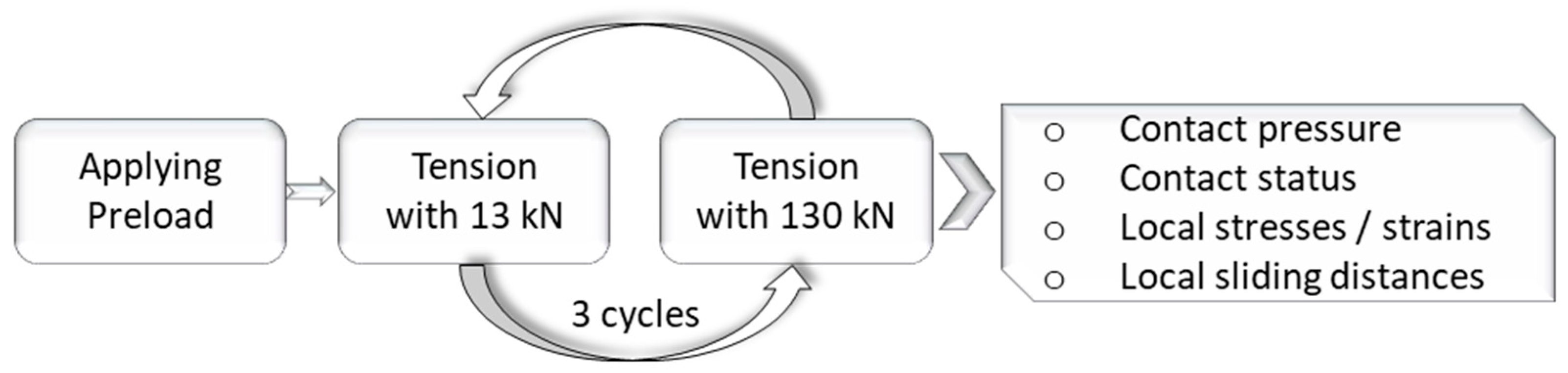
2.2. Finite Element Model
3. Results
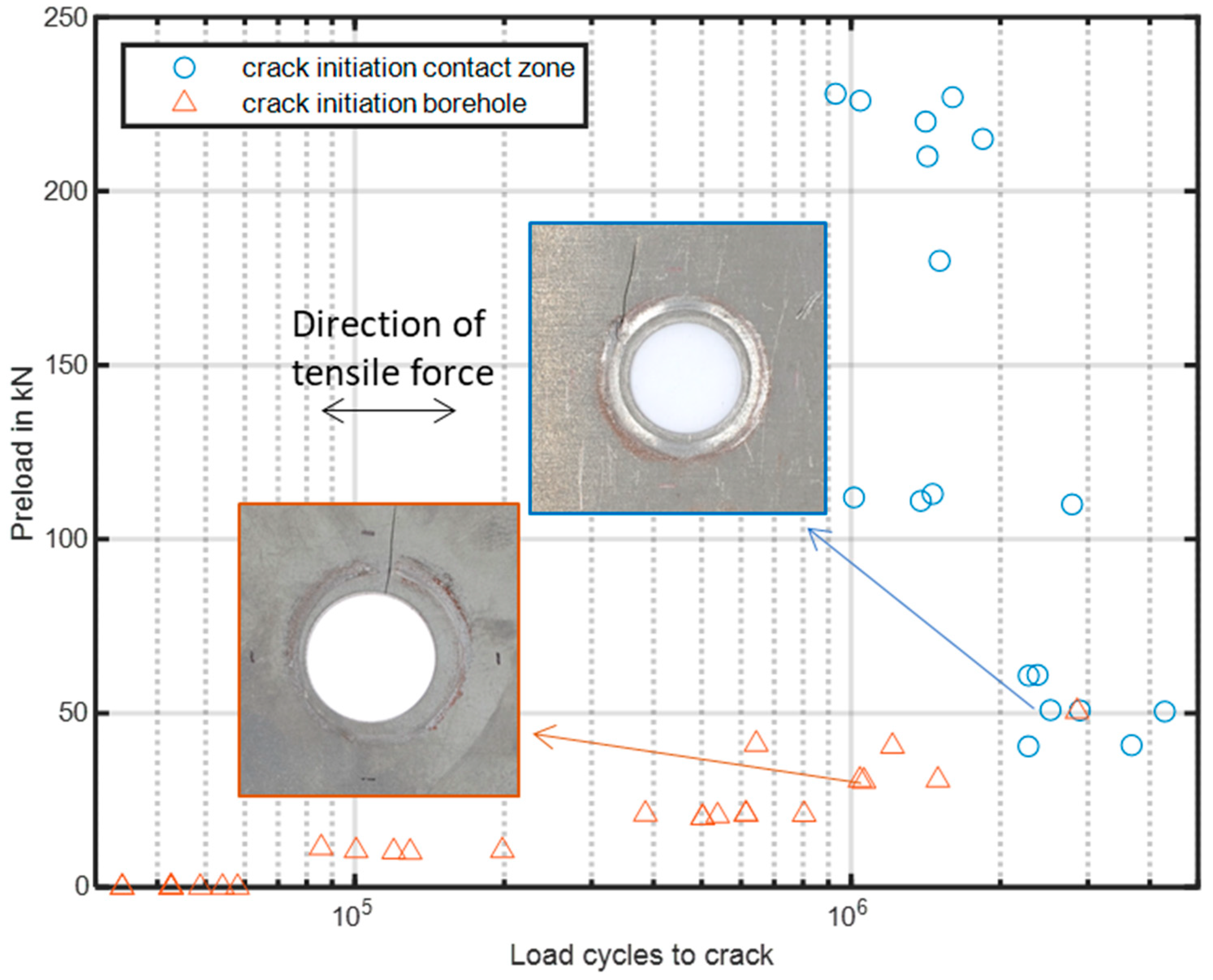
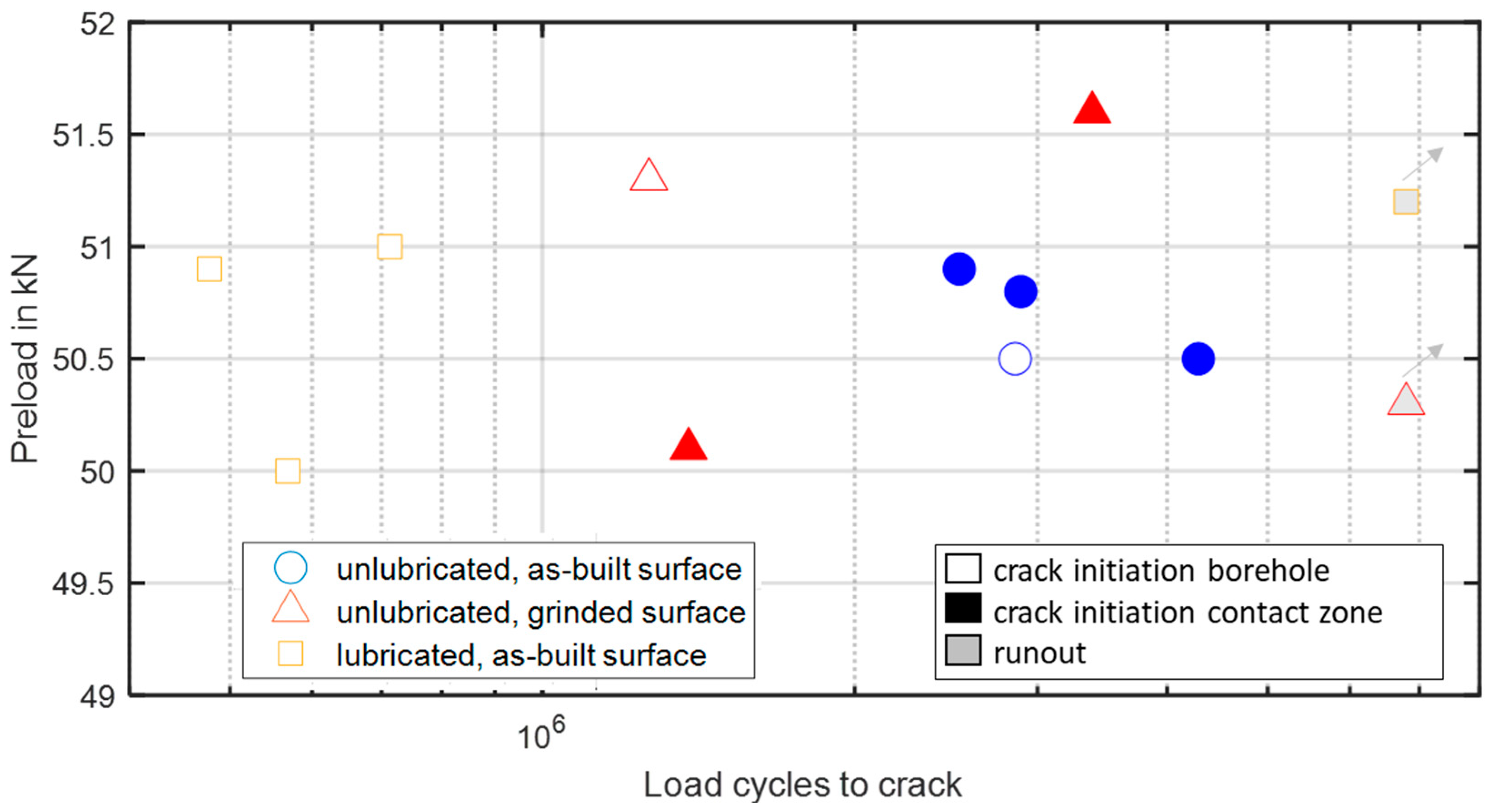
3.1. Experimental Results
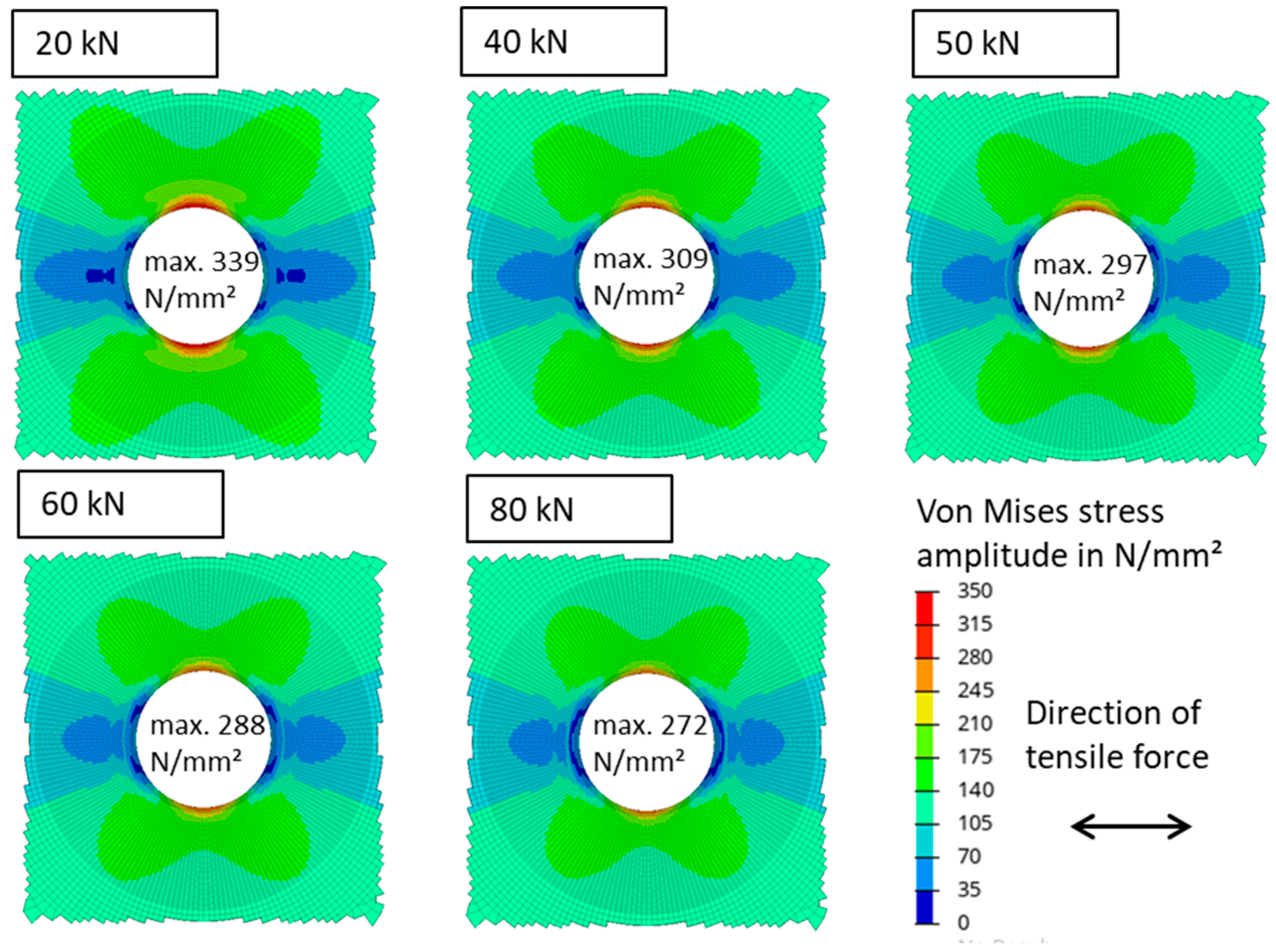
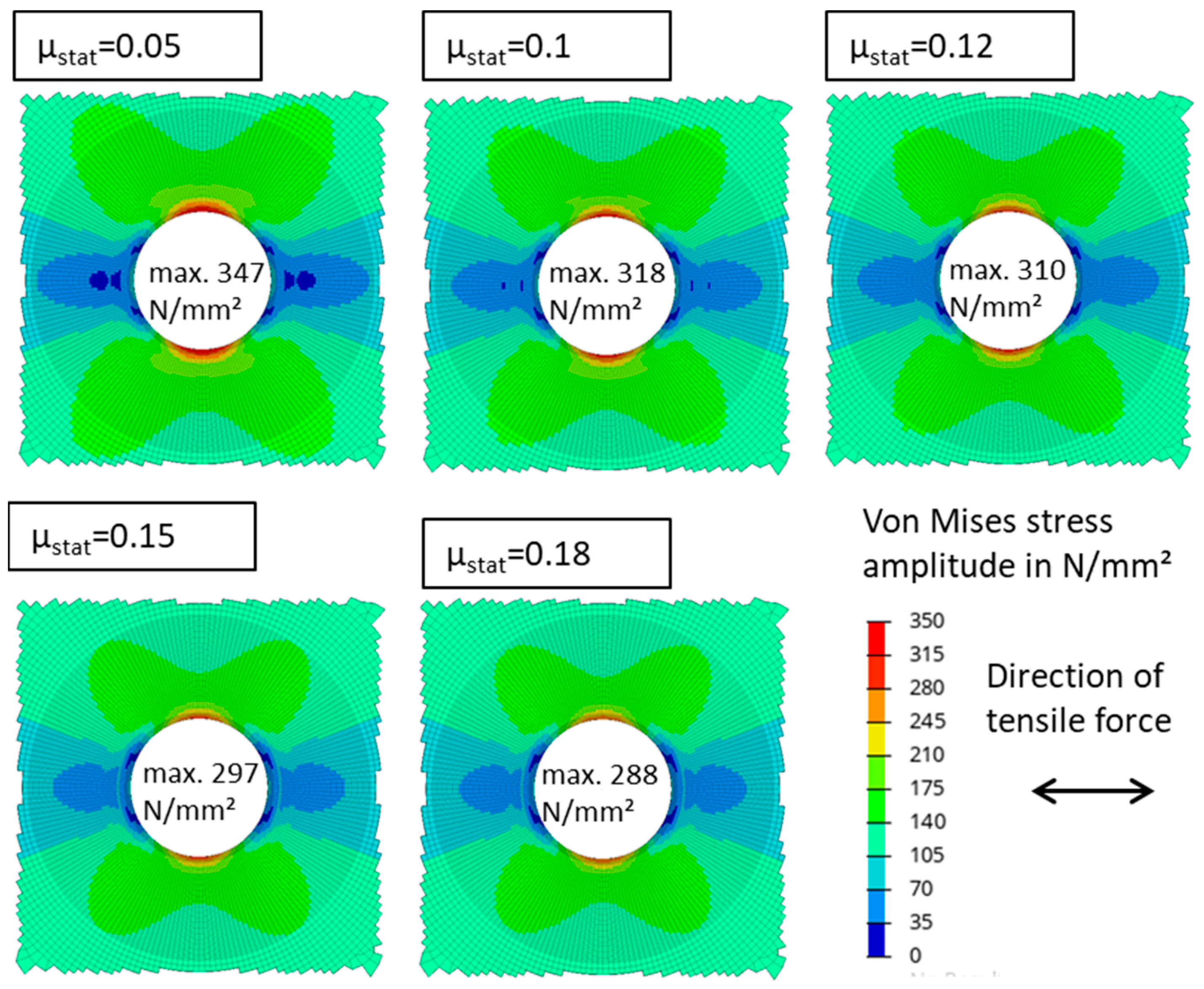
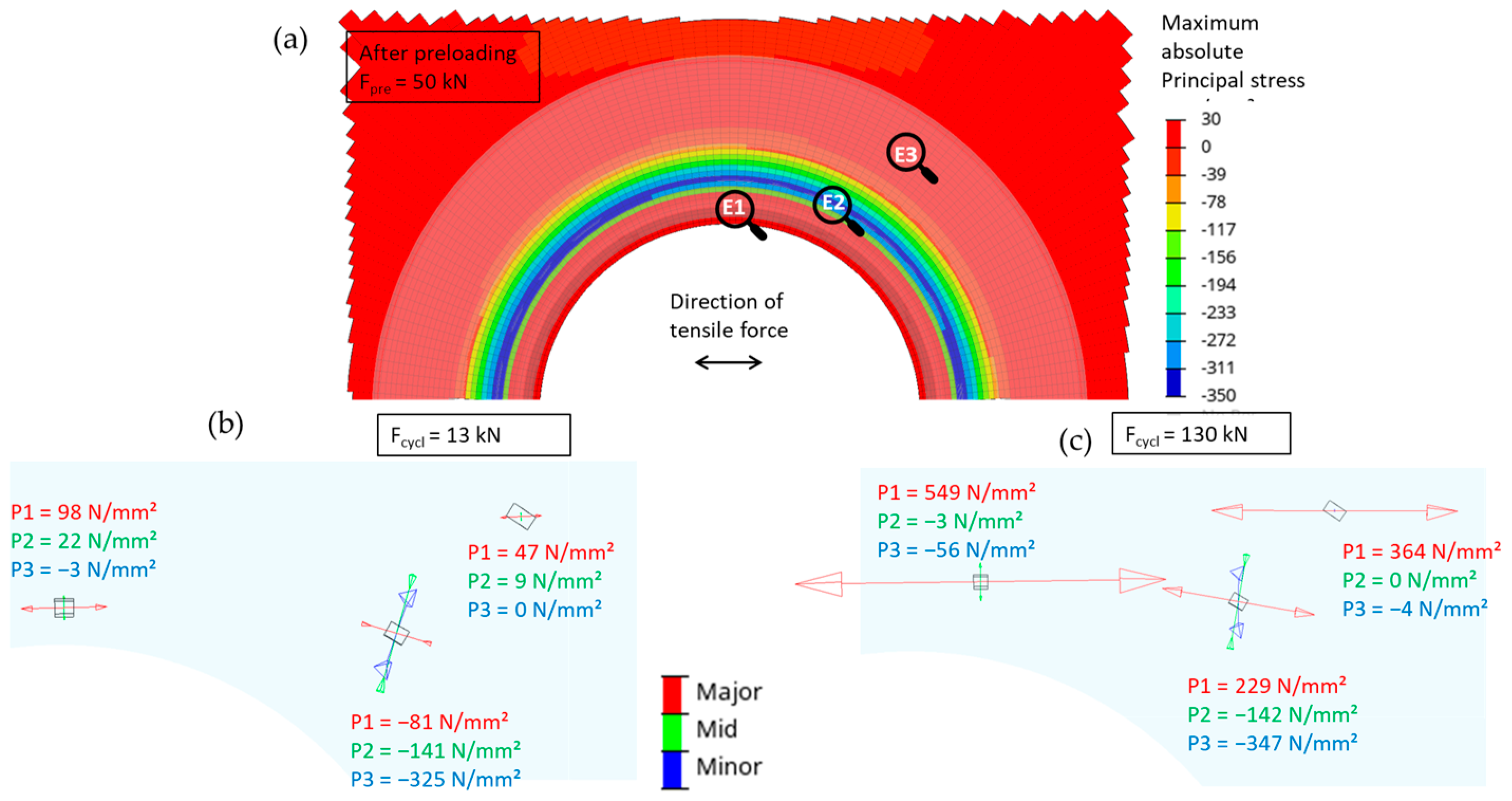
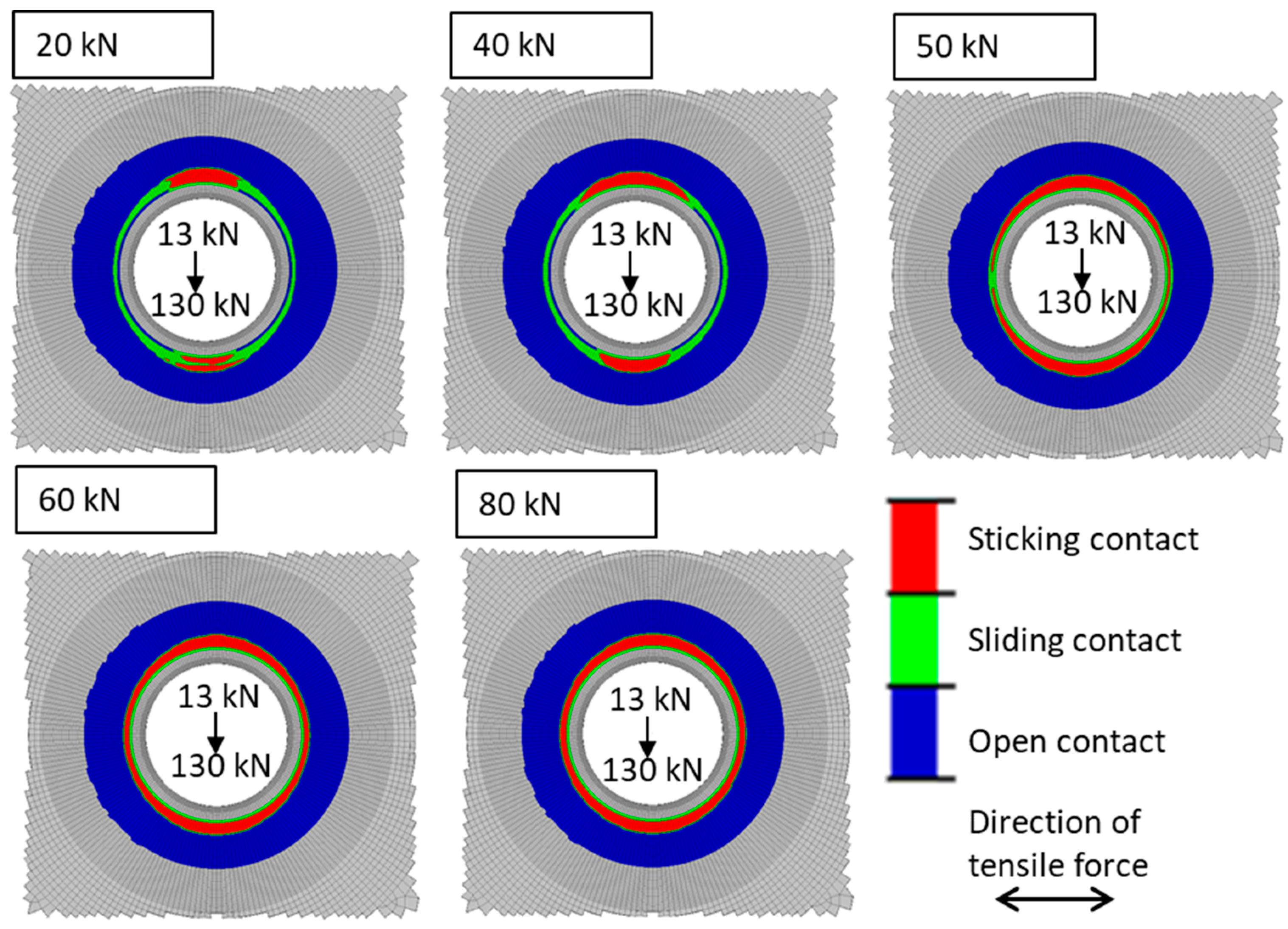
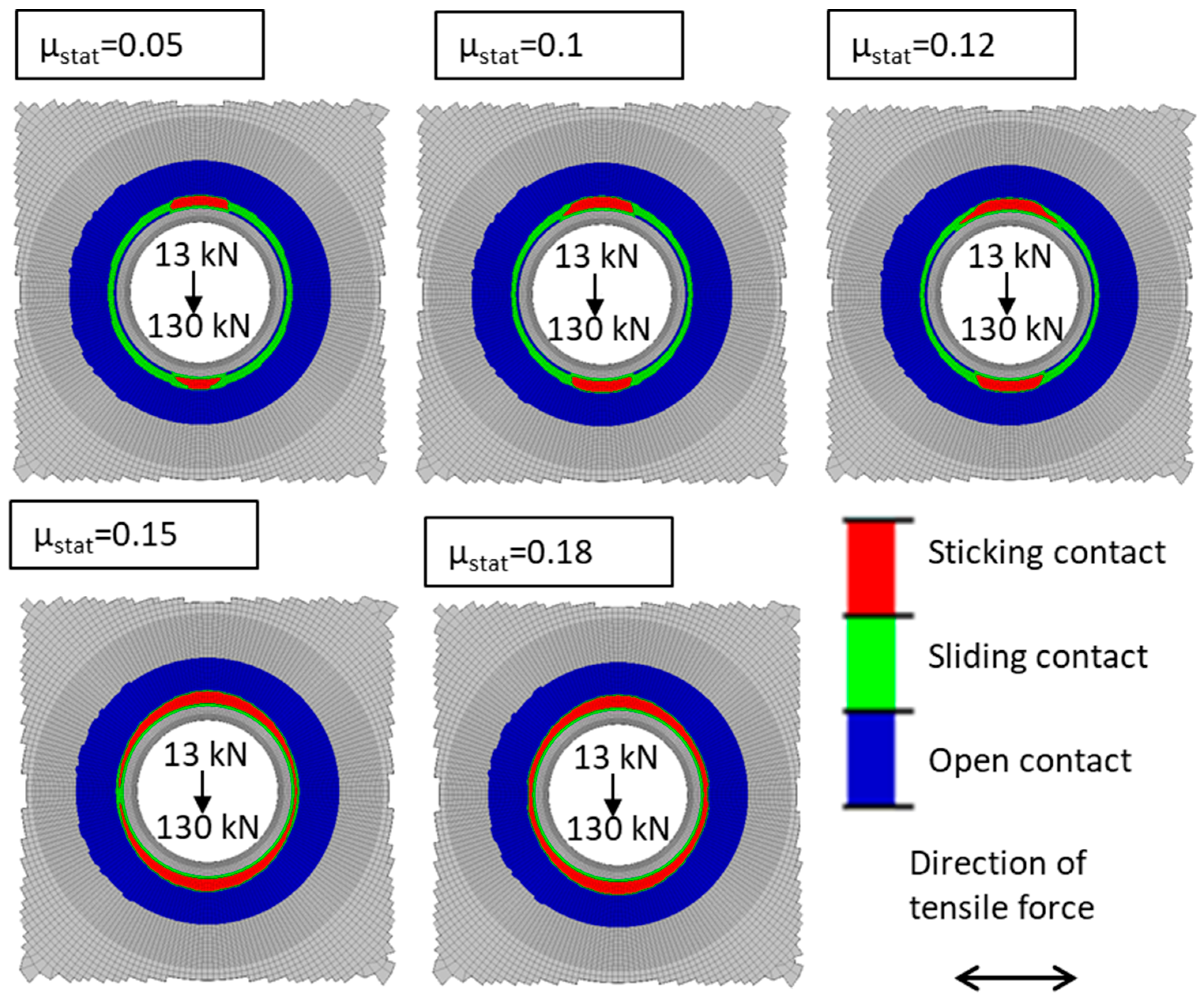
3.2. Numerical Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Test Number | Preload | Crack Initiation Location | Cycles to Crack |
|---|---|---|---|
| 1 | 0 kN | borehole | 57,995 |
| 2 | 0 kN | borehole | 42,600 |
| 3 | 0 kN | borehole | 42,694 |
| 4 | 0 kN | borehole | 33,955 |
| 5 | 0 kN | borehole | 42,545 |
| 6 | 0 kN | borehole | 48,777 |
| 7 | 0 kN | borehole | 54,094 |
| 8 | 10.2 kN | borehole | 119,732 |
| 9 | 10.1 kN | borehole | 129,211 |
| 10 | 10.5 kN | borehole | 198,211 |
| 11 | 11.3 kN | borehole | 85,515 |
| 12 | 20 kN | borehole | 501,977 |
| 13 | 21 kN | borehole | 613,971 |
| 14 | 20.9 kN | borehole | 804,070 |
| 15 | 20.5 kN | borehole | 538,255 |
| 16 | 30.8 kN | borehole | 1,041,874 |
| 17 | 30.8 kN | borehole | 1,496,038 |
| 18 | 30.5 kN | borehole | 1,061,404 |
| 19 | 41.1 kN | borehole | 643,995 |
| 20 | 40.8 kN | contact zone | 3,675,143 |
| 21 | 40.5 kN | contact zone | 2,276,980 |
| 22 | 40.5 kN | borehole | 1,211,595 |
| 23 | 50.5 kN | borehole | 2,855,086 |
| 24 | 50.8 kN | contact zone | 2,891,121 |
| 25 | 50.5 kN | contact zone | 4,287,646 |
| 26 | 50.9 kN | contact zone | 2,522,088 |
| 27 | 60.8 kN | contact zone | 2,279,173 |
| 28 | 61 kN | contact zone | 2,375,510 |
| 29 | 111 kN | contact zone | 1,381,777 |
| 30 | 112 kN | contact zone | 1,013,565 |
| 31 | 226 kN | contact zone | 1,043,371 |
| 32 | 228 kN | contact zone | 930,606 |
| 33 | 227 kN | contact zone | 1,601,310 |
Appendix B
| Test Number | Setup | Crack Initiation Location | Cycles to Crack |
|---|---|---|---|
| 1 | unlubricated, as-built surface | contact zone | 2,891,121 |
| 2 | unlubricated, as-built surface | contact zone | 4,287,646 |
| 3 | unlubricated, as-built surface | contact zone | 2,522,088 |
| 4 | unlubricated, as-built surface | borehole | 2,855,086 |
| 5 | unlubricated, grinded surface | contact zone | 1,383,318 |
| 6 | unlubricated, grinded surfaceunlubricated, polished surface | borehole | 1,267,019 |
| 7 | unlubricated, grinded surface | contact zone | 3,387,754 |
| 8 | unlubricated, grinded surface | no crack | runout |
| 9 | lubricated, as-built surface | contact zone | 568,428 |
| 10 | lubricated, as-built surface | contact zone | 712,844 |
| 11 | lubricated, as-built surface | contact zone | 477,696 |
| 12 | lubricated, as-built surface | no crack | runout |
Appendix C
| Unlubricated, As-Built Surface | Unlubricated, Grinded Surface | Lubricated, As-Built Surface |
|---|---|---|
 Cycles to crack: 2891121 |  Cycles to crack: 1267019 |  Cycles to crack: 568428 |
Appendix D
| Criteria | Ideal Value | % Ideal | Fail Value | % Fail |
|---|---|---|---|---|
| Aspect ratio | 1.000 | 99.135 | >8.0 | 0.002 |
| Jacobian | 1.000 | 14.396 | <0.5 | 0.001 |
| Skew angle | 0° | 21.227 | >60° | 0.000 |
| Warpage angle | 0° | 16.527 | >20° | 0.000 |
References
- Knothe, W.; Schumacher, J.; Streicher, M.; Fischer, G. Interactions Between Wheel and Hub—Developments and Potentials at Commercial Vehicles. Mater. Test. 2008, 50, 12–19. [Google Scholar] [CrossRef]
- Wöllner, U.; Szlosarek, R.; Kröger, M. Fatigue behavior of agricultural rims under rotating bending load. Fatigue Fract. Eng. Mater. Struct. 2022, 45, 2143–2158. [Google Scholar] [CrossRef]
- Benhamena, A.; Amrouche, A.; Talha, A.; Benseddiq, N. Effect of contact forces on fretting fatigue behavior of bolted plates: Numerical and experimental analysis. Tribol. Int. 2012, 48, 237–245. [Google Scholar] [CrossRef]
- Chakherlou, T.N.; Oskouei, R.H.; Vogwell, J. Experimental and numerical investigation of the effect of clamping force on the fatigue behaviour of bolted plates. Eng. Fail. Anal. 2008, 15, 563–574. [Google Scholar] [CrossRef]
- Chakherlou, T.N.; Alvandi-Tabrizi, Y.; Kiani, A. On the fatigue behavior of cold expanded fastener holes subjected to bolt tightening. Int. J. Fatigue 2011, 33, 800–810. [Google Scholar] [CrossRef]
- Esmaeili, F.; Chakherlou, T.N.; Zehsaz, M.; Hasanifard, S. Investigating the effect of clamping force on the fatigue life of bolted plates using volumetric approach. J. Mech. Sci. Technol. 2013, 27, 3657–3664. [Google Scholar] [CrossRef]
- Jiménez-Peña, C.; Talemi, R.H.; Rossi, B.; Debruyne, D. Investigations on the fretting fatigue failure mechanism of bolted joints in high strength steel subjected to different levels of pre-tension. Tribol. Int. 2017, 108, 128–140. [Google Scholar] [CrossRef]
- Ruiz, C.; Boddington, P.H.B.; Chen, K.C. An investigation of fatigue and fretting in a dovetail joint. Exp. Mech. 1984, 24, 208–217. [Google Scholar] [CrossRef]
- Juoksukangas, H.; Lehtovaara, A.; Mäntylä, A. Experimental and numerical investigation of fretting fatigue behavior in bolted joints. Tribol. Int. 2016, 103, 440–448. [Google Scholar] [CrossRef]
- Chakherlou, T.N.; Razavi, M.J.; Aghdam, A.B.; Abazadeh, B. An experimental investigation of the bolt clamping force and friction effect on the fatigue behavior of aluminum alloy 2024-T3 double shear lap joint. Mater. Des. 2011, 32, 4641–4649. [Google Scholar] [CrossRef]
- Jayaprakash, M.; Mutoh, Y.; Yoshii, K. Fretting Fatigue Behavior and Life Prediction of Automotive Steel Bolted Joint. Mater. Des. 2011, 32, 3911–3919. [Google Scholar] [CrossRef]
- Chakherlou, T.N.; Mirzajanzadeh, M.; Vogwell, J. Effect of hole lubrication on the fretting fatigue life of double shear lap joints: An experimental and numerical study. Eng. Fail. Anal. 2009, 16, 2388–2399 . [Google Scholar] [CrossRef]
- Oskouei, R.H.; Ibrahim, R.H. Improving fretting fatigue behaviour of Al 7075-T6 bolted plates using electroless Ni–P coatings. Int. J. Fatigue 2012, 44, 157–167. [Google Scholar] [CrossRef]
- Sun, Y.; Voyiadjis, G.Z.; Hu, W.; Shen, F.; Meng, Q. Fatigue and fretting fatigue life prediction of double-lap bolted joints using continuum damage mechanics-based approach. Int. J. Damage Mech. 2017, 26, 162–188. [Google Scholar] [CrossRef]
- Lemaitre, J.; Chaboche, J.L. Mechanics of Solid Materials, 1st ed.; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Chaboche, J.L. On some modifications of kinematic hardening to improve the description of ratcheting effects. Int. J. Plasticity 1991, 7, 661–678. [Google Scholar] [CrossRef]
- Venugopal Poovakaud, V.; Jiménez-Peña, C.; Talemi, R.; Coppieters, S.; Debruyne, D. Assessment of Fretting Fatigue in High Strength Steel Bolted Connections with Simplified Fe Modelling Techniques. Tribol. Int. 2020, 143, 106083. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Fini, S.; Olmi, G.; Robusto, F.; Scapecchi, C. Fretting Fatigue in Mechanical Joints: A Literature Review. Lubricants 2022, 10, 53. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Fini, S.; Mele, M.; Olmi, G.; Scapecchi, C.; Tariq, M.H.B. Failure of Threaded Connections: A Literature Review. Machines 2023, 11, 212. [Google Scholar] [CrossRef]
- Szlosarek, R.; Kröger, M. Fatigue behavior of bolted boreholes under various preloads. Mater. Test. 2022, 64, 195–201. [Google Scholar] [CrossRef]
- Szlosarek, R.; Kröger, M. Multiaxial fatigue crack initiation in bolted sheet material. Procedia Struct. Integr. 2023, 43, 41–46. [Google Scholar] [CrossRef]
- Fatemi, A.; Socie, D.F. A Critical Plane Approach to Multiaxial Fatigue Damage Including Out-Of-Phase Loading. Fatigue Fract. Eng. Mater. Struct. 1988, 11, 149–165. [Google Scholar] [CrossRef]
- Smith, K.N.; Topper, T.H.; Watson, P. A Stress–Strain Function for the Fatigue of Metals (Stress-Strain Function for Metal Fatigue Including Mean Stress Effect). J. Mater. 1970, 5, 767–778. [Google Scholar]
- Deutsches Institut für Normung, e. V (DIN). Disc Wheels for Motor Vehicles and Trailers—Part 3: Dimensions and Fastening Devices for Hub-Centering; DIN 74361-3; Deutsches Institut für Normung e. V (DIN): Berlin, Germany, 2023. [Google Scholar]
- Fernando, S. An engineering insight to the fundamental behaviour of tensile bolted joints. Steel Constr. 2001, 35, 1–13. [Google Scholar]
- Verein Deutscher Ingenieure (VDI). Systematic Calculation of High Duty Bolted Joints Joints with One Cylindrical Bolt; VDI 2230; Verein Deutscher Ingenieure (VDI): Düsseldorf, Germany, 2003. [Google Scholar]
- Nesládek, M.; Španiel, M.; Jurenka, J.; Růžička, J.; Kuželka, J. Fretting fatigue—Experimental and numerical approaches. Int. J. Fatigue 2012, 44, 61–73. [Google Scholar] [CrossRef]
- Mäntylä, A.; Juoksukangas, J.; Hintikka, J.; Frondelius, T.; Lehtovaara, A. FEM-Based Wear Simulation for Fretting Contacts. Raken. Mek. 2020, 53, 20–27. [Google Scholar] [CrossRef]
- Mäntylä, A.; Hintikka, J.; Frondelius, T.; Vaara, J.; Lehtovaara, A.; Juoksukangas, J. Prediction of Contact Condition and Surface Damage by Simulating Variable Friction Coefficient and Wear. Tribol. Int. 2020, 143, 106054. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szlosarek, R.; Holzmüller, P.; Kröger, M. Analyzing the Fretting Fatigue of Bolt Joints by Experiments and Finite Element Analysis. Lubricants 2023, 11, 348. https://doi.org/10.3390/lubricants11080348
Szlosarek R, Holzmüller P, Kröger M. Analyzing the Fretting Fatigue of Bolt Joints by Experiments and Finite Element Analysis. Lubricants. 2023; 11(8):348. https://doi.org/10.3390/lubricants11080348
Chicago/Turabian StyleSzlosarek, Robert, Paul Holzmüller, and Matthias Kröger. 2023. "Analyzing the Fretting Fatigue of Bolt Joints by Experiments and Finite Element Analysis" Lubricants 11, no. 8: 348. https://doi.org/10.3390/lubricants11080348
APA StyleSzlosarek, R., Holzmüller, P., & Kröger, M. (2023). Analyzing the Fretting Fatigue of Bolt Joints by Experiments and Finite Element Analysis. Lubricants, 11(8), 348. https://doi.org/10.3390/lubricants11080348





