Investigation on Tooth Surface Wear of Cycloid Drives Considering Tooth Profile Modifications
Abstract
:1. Introduction
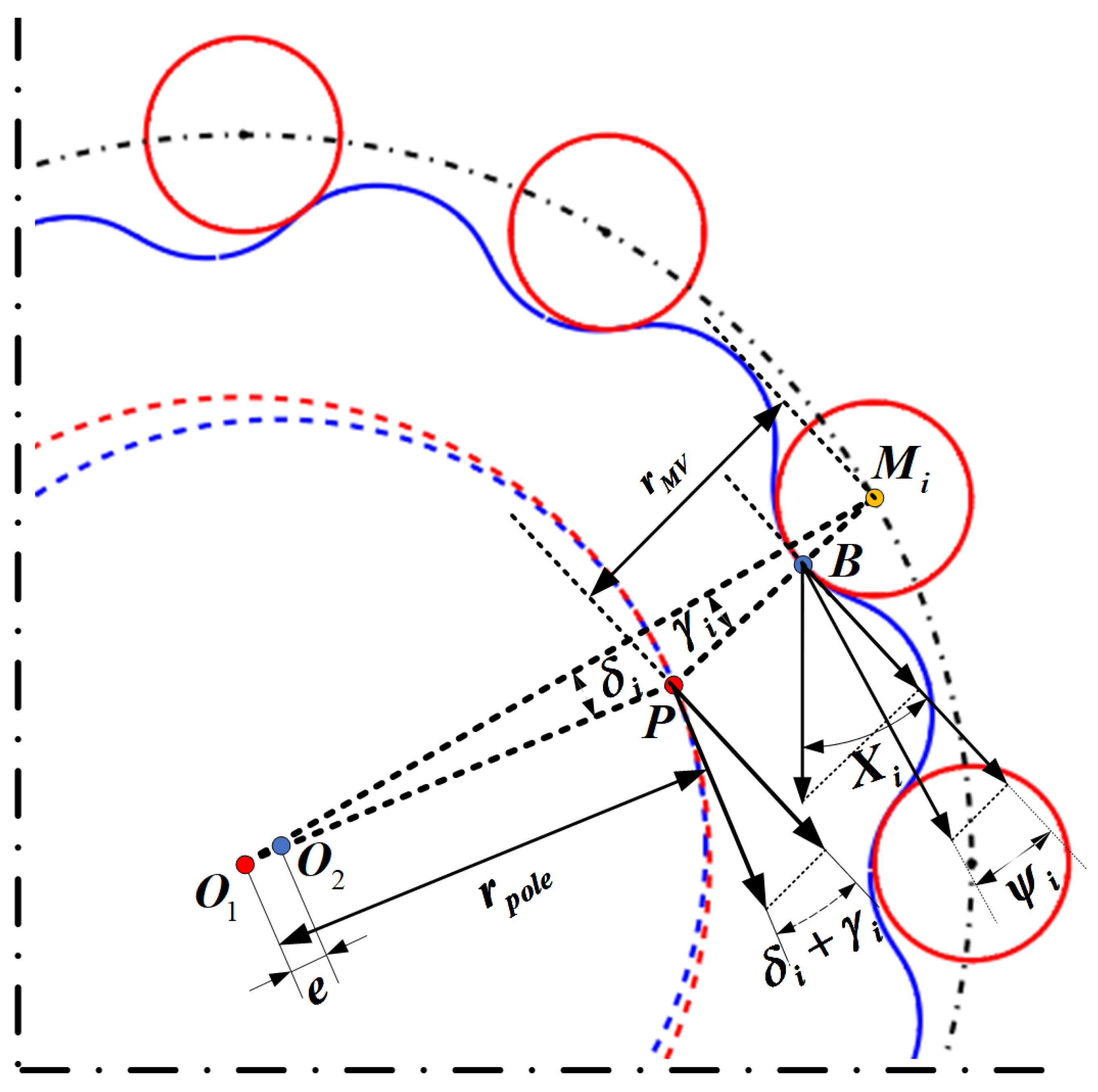
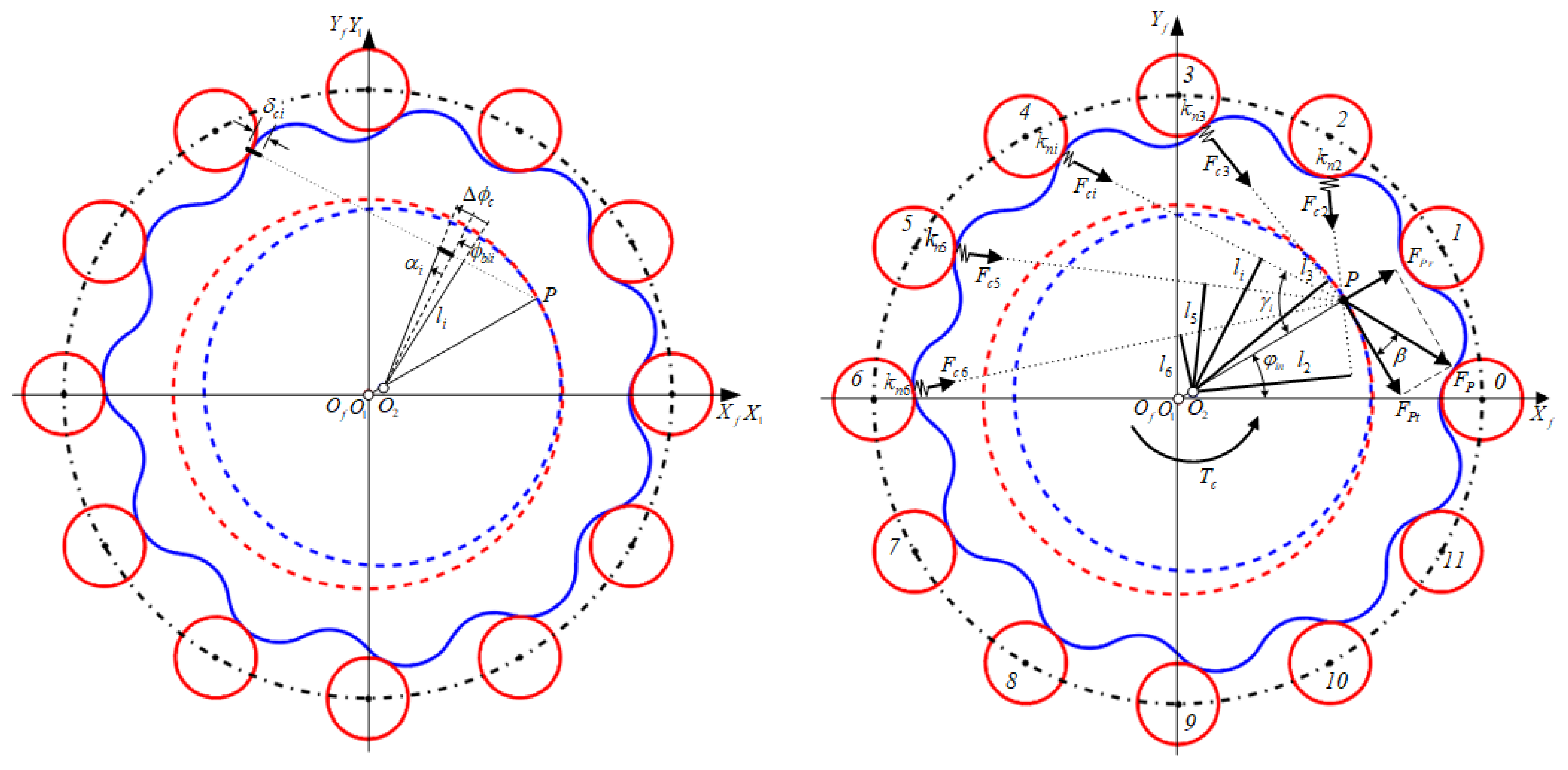
2. Loaded Tooth Contact Analysis
2.1. Tooth Contact Analysis
2.2. Relative Sliding Velocity
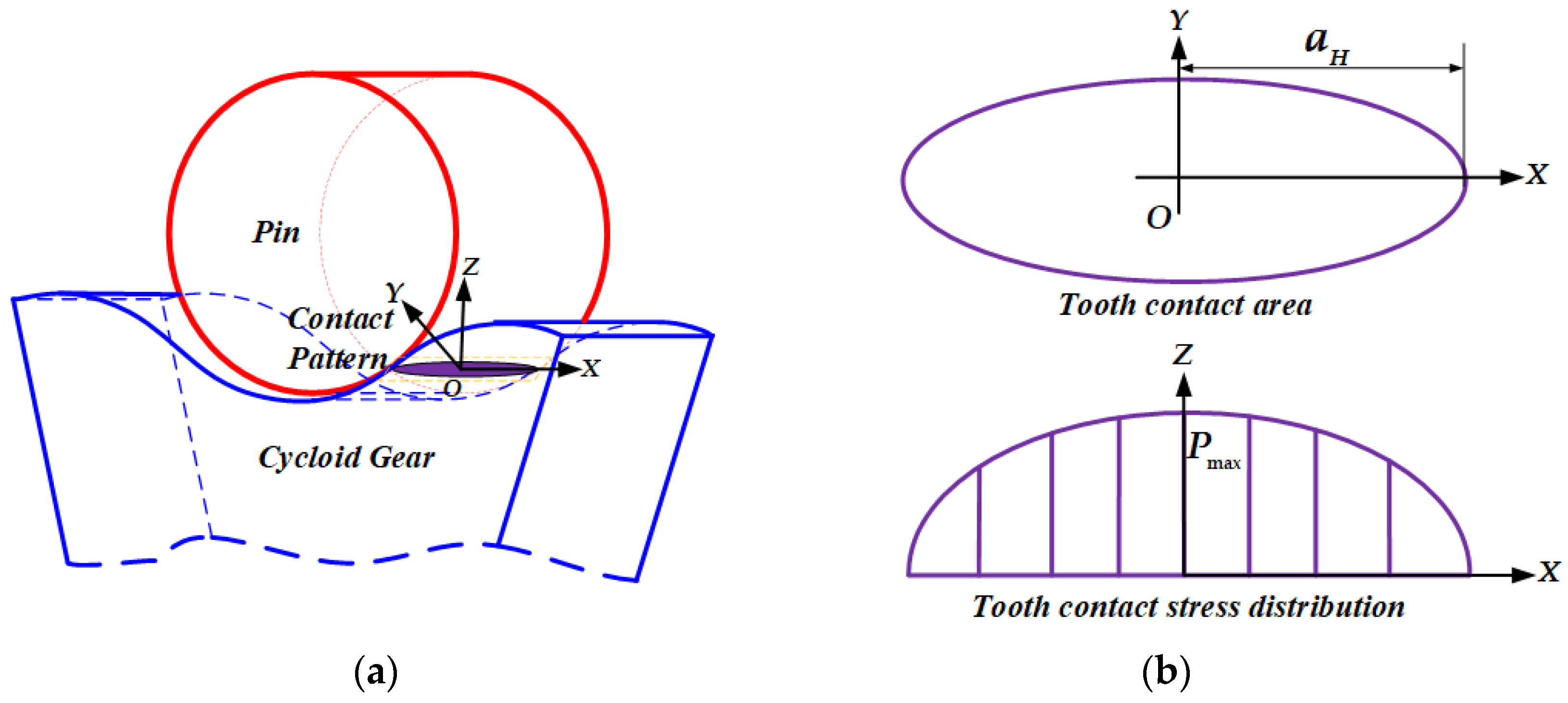
2.3. Tooth Contact Stress
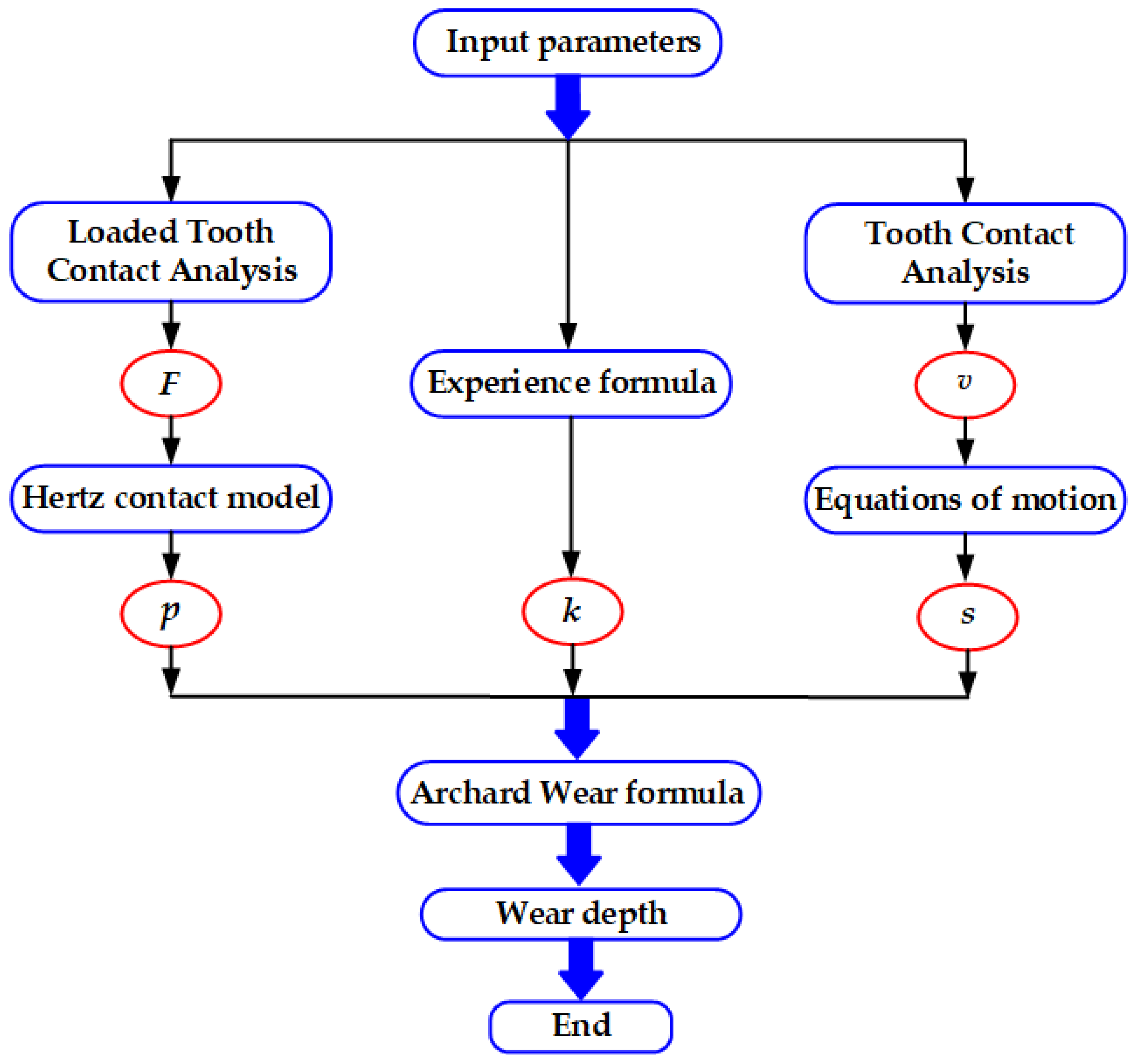
3. Wear Calculation of Tooth Surface
3.1. Wear Calculation Steps
3.2. Relative Sliding Distance
3.3. Wear Coefficient for the Contact Tooth Surface
3.4. Wear Depth Calculation
4. Wear Analysis and Discussion
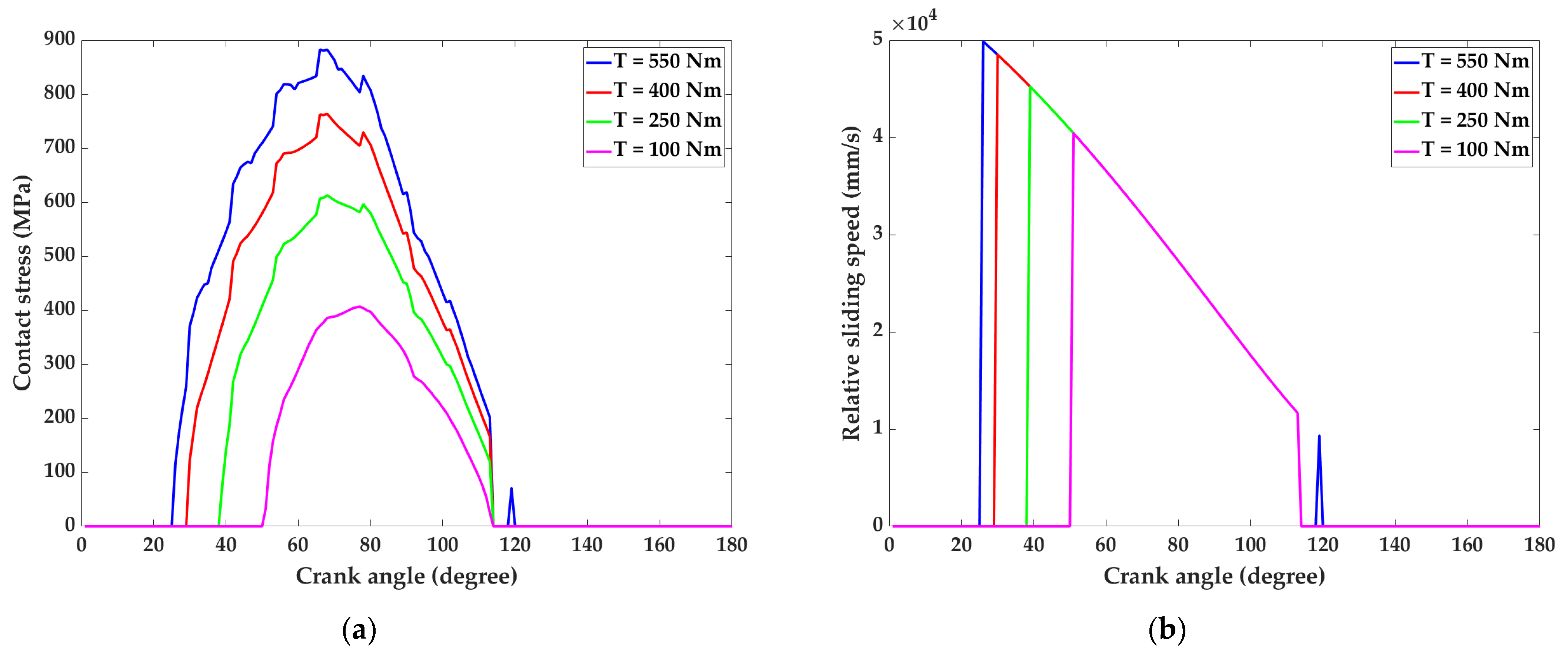
4.1. Tooth Contact Stress and Relative Sliding Speed
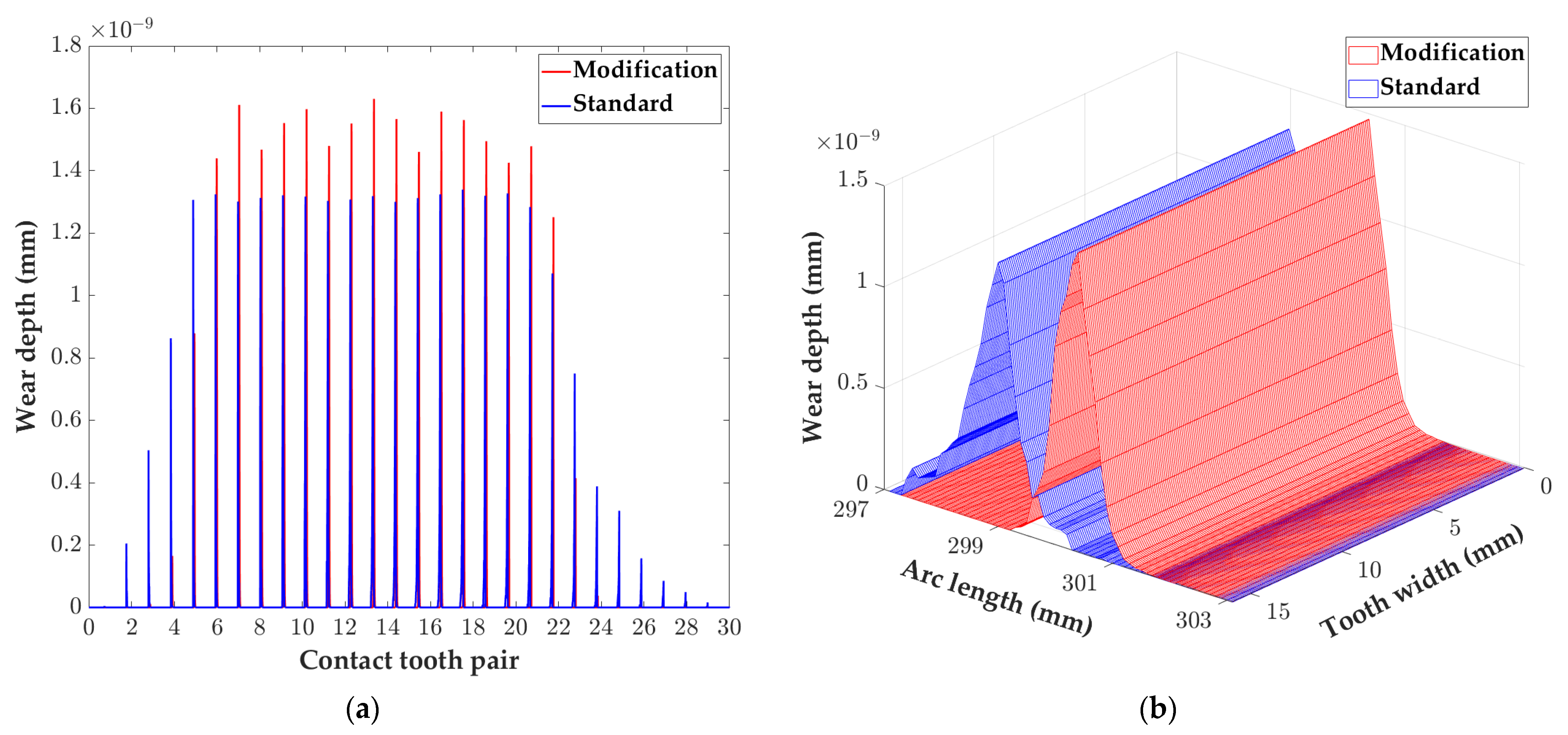
4.2. Effect of the Tooth Profile Modification
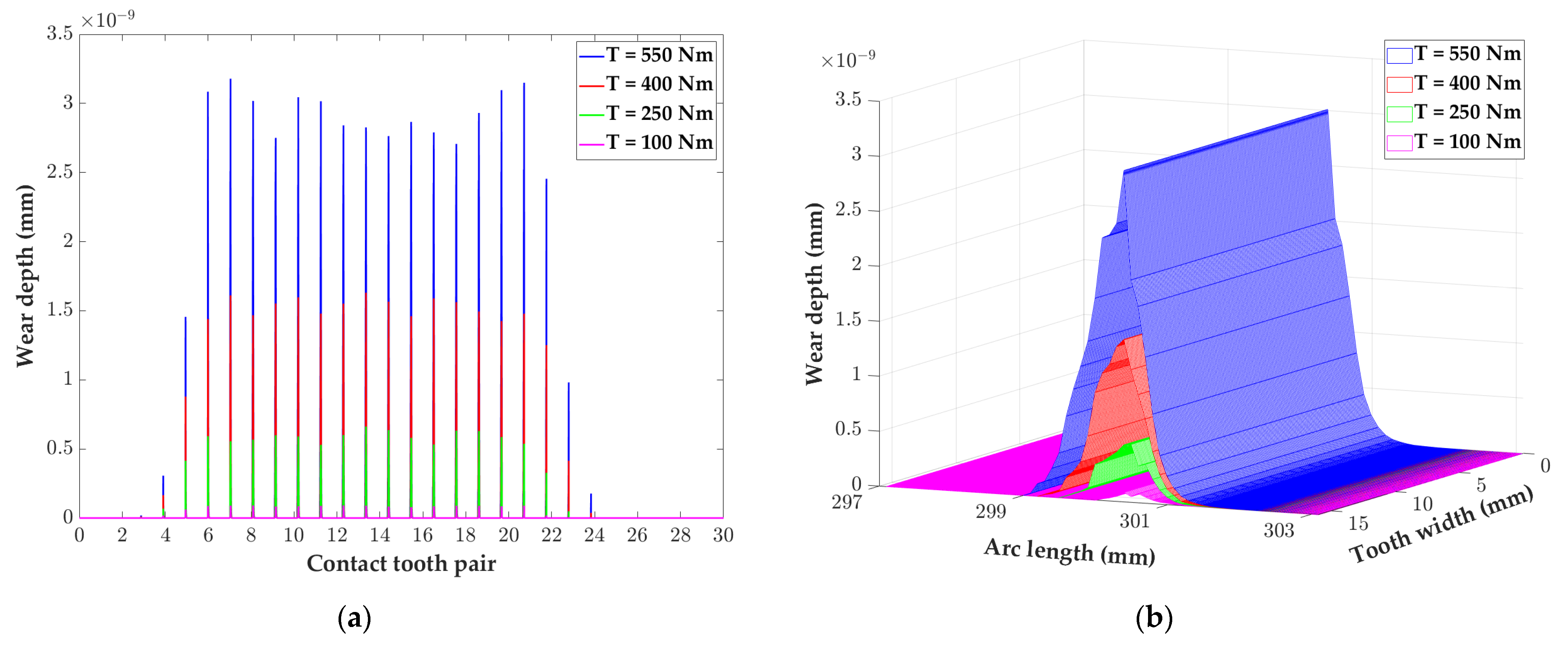
4.3. Effect of the Load Torque
4.4. Effect of Crank Shaft Rotation Speed
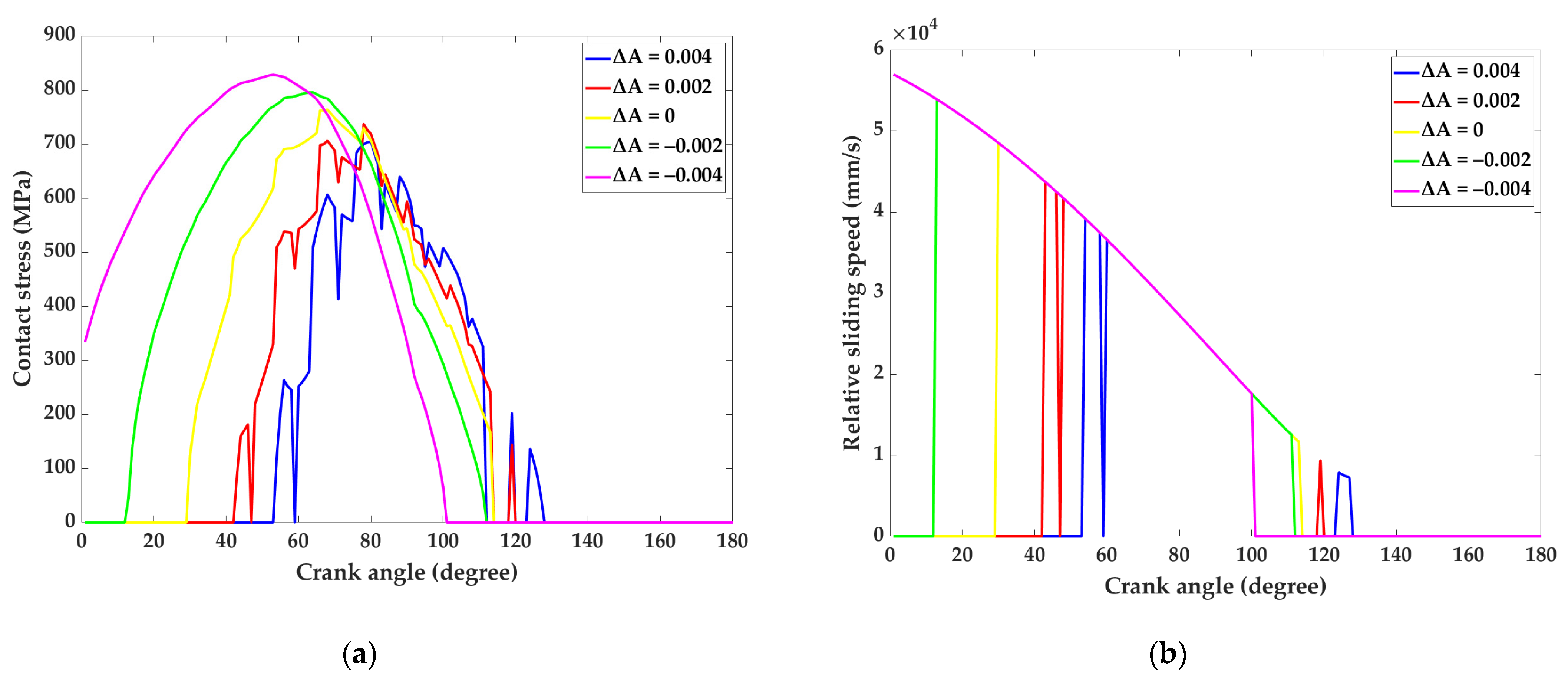
4.5. Effect of Different Eccentricity Errors
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wu, S.; Cheng, H.S. Sliding Wear Calculation in Spur Gears. J. Tribol. 1993, 115, 493–500. [Google Scholar] [CrossRef]
- Wu, S.; Cheng, H.S. A Sliding Wear Model for Partial-EHL Contacts. J. Tribol. 1991, 113, 134–141. [Google Scholar] [CrossRef]
- Flodin, A.; Andersson, S. Simulation of mild wear in spur gears. Wear 1997, 207, 16–23. [Google Scholar] [CrossRef]
- Flodin, A.; Andersson, S. A simplified model for wear prediction in helical gears. Wear 2001, 249, 285–292. [Google Scholar] [CrossRef]
- Flodin, A.; Andersson, S. Simulation of mild wear in helical gears. Wear 2000, 241, 123–128. [Google Scholar] [CrossRef]
- Park, D.; Kahraman, A. A surface wear model for hypoid gear pairs. Wear 2009, 267, 1595–1604. [Google Scholar] [CrossRef]
- Park, D.; Kolivand, M.; Kahraman, A. An approximate method to predict surface wear of hypoid gears using surface interpolation. Mech. Mach. Theory 2014, 71, 64–78. [Google Scholar] [CrossRef]
- Park, D.; Kolivand, M.; Kahraman, A. Prediction of surface wear of hypoid gears using a semi-analytical contact model. Mech. Mach. Theory 2012, 52, 180–194. [Google Scholar] [CrossRef]
- Yuksel, C.; Kahraman, A. Dynamic tooth loads of planetary gear sets having tooth profile wear. Mech. Mach. Theory 2004, 39, 695–715. [Google Scholar] [CrossRef]
- Imrek, H.; Düzcükoğlu, H. Relation between wear and tooth width modification in spur gears. Wear 2007, 262, 390–394. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Y.; Zhang, Q.; Qin, X.; Chen, K. Wear analysis and simulation of small module gear based on Archard model. Eng. Fail. Anal. 2023, 144, 26. [Google Scholar] [CrossRef]
- Zhao, Q.; Xing, Z.; Yuan, J.; Zhang, Z.; Zhu, J.; Jiang, H. An Improved Modeling and Numerical Analysis Method for Tooth Surface Wear of Double-Arc Harmonic Gears. Materials 2022, 15, 8869. [Google Scholar] [CrossRef]
- Onishchenko, V. Investigation of tooth wears from scuffing of heavy duty machine spur gears. Mech. Mach. Theory 2015, 83, 38–55. [Google Scholar] [CrossRef]
- Chernets, M.V. Prediction Method of Contact Pressures, Wear and Life of Worm Gears with Archimedean and Involute Worm, Taking Tooth Correction into Account. J. Frict. Wear 2019, 40, 342–348. [Google Scholar] [CrossRef]
- Kahraman, A.; Bajpai, P.; Anderson, N.E. Influence of Tooth Profile Deviations on Helical Gear Wear. J. Mech. Des. 2005, 127, 656–663. [Google Scholar] [CrossRef]
- Bajpai, P.; Kahraman, A.; Anderson, N.E. A Surface Wear Prediction Methodology for Parallel-Axis Gear Pairs. J. Tribol. 2004, 126, 597–605. [Google Scholar] [CrossRef]
- Li, G.; Wang, Z.H.; Zhu, W.D. Prediction of Surface Wear of Involute Gears Based on a Modified Fractal Method. J. Tribol. 2019, 141, 13. [Google Scholar] [CrossRef]
- Li, X.; Xu, J.; Yang, Z.; Chen, R.; Yang, H. The Influence of Tooth Surface Wear on Dynamic Characteristics of Gear-Bearing System Based on Fractal Theory. J. Comput. Nonlinear Dyn. 2020, 15, 10. [Google Scholar] [CrossRef]
- Zheng, F.; Zhang, J.; Yao, L.; Tan, R. Investigation on the wear of spur gears generated by modified cutter. Friction 2020, 9, 288–300. [Google Scholar] [CrossRef]
- Zhang, R.; Zhou, J.; Wei, Z. Study on transmission error and torsional stiffness of RV reducer under wear. J. Mech. Sci. Technol. 2022, 36, 4067–4081. [Google Scholar] [CrossRef]
- Li, X.; Li, C.; Wang, Y.; Chen, B.; Lim, T.C. Analysis of a Cycloid Speed Reducer Considering Tooth Profile Modification and Clearance-Fit Output Mechanism. J. Mech. Des. 2017, 139, 033303. [Google Scholar] [CrossRef]
- Li, X.; Chen, B.-K.; Wang, Y.-W.; Lim, T.C. Mesh stiffness calculation of cycloid-pin gear pair with tooth profile modification and eccentricity error. J. Cent. South Univ. 2018, 25, 1717–1731. [Google Scholar] [CrossRef]
- Janakiraman, V.; Li, S.; Kahraman, A. An Investigation of the Impacts of Contact Parameters on Wear Coefficient. J. Tribol. 2014, 136, 031602. [Google Scholar] [CrossRef]








| Parameter Name | Cycloid Gear | Pin |
|---|---|---|
| Number of teeth | 29 | 30 |
| Eccentricity /mm | 3 | |
| Radius of roller position /mm | 120 | |
| Radius of roller /mm | 8 | |
| Roller position modification amount /mm | 0.008 | |
| Roller radius modification amount /mm | 0.016 | |
| Tooth width /mm | 17 | 16 |
| Gear Material | GCr15 | GCr15 |
|---|---|---|
| Modulus of elasticity /GPa | 208 | 208 |
| Poisson’s ratio | 0.3 | 0.3 |
| Stress-viscosity factor /Pa·s | 1.48 × 10−2 | |
| Roughness of the cycloidal tooth surface /mm | 0.02 × 10−3 | |
| Roughness of the roller surface /mm | 0.02 × 10−3 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Yang, H.; Niu, W.; Guo, R.; Sun, L. Investigation on Tooth Surface Wear of Cycloid Drives Considering Tooth Profile Modifications. Lubricants 2023, 11, 323. https://doi.org/10.3390/lubricants11080323
Li X, Yang H, Niu W, Guo R, Sun L. Investigation on Tooth Surface Wear of Cycloid Drives Considering Tooth Profile Modifications. Lubricants. 2023; 11(8):323. https://doi.org/10.3390/lubricants11080323
Chicago/Turabian StyleLi, Xuan, Haidong Yang, Weilong Niu, Ran Guo, and Lining Sun. 2023. "Investigation on Tooth Surface Wear of Cycloid Drives Considering Tooth Profile Modifications" Lubricants 11, no. 8: 323. https://doi.org/10.3390/lubricants11080323
APA StyleLi, X., Yang, H., Niu, W., Guo, R., & Sun, L. (2023). Investigation on Tooth Surface Wear of Cycloid Drives Considering Tooth Profile Modifications. Lubricants, 11(8), 323. https://doi.org/10.3390/lubricants11080323






