Study on the Influence of Micro-Features in the Surface Topography of the Slider Raceway on the Dynamic Friction Factor of the Guide Rail Pair
Abstract
:1. Introduction
2. Mathematical Model
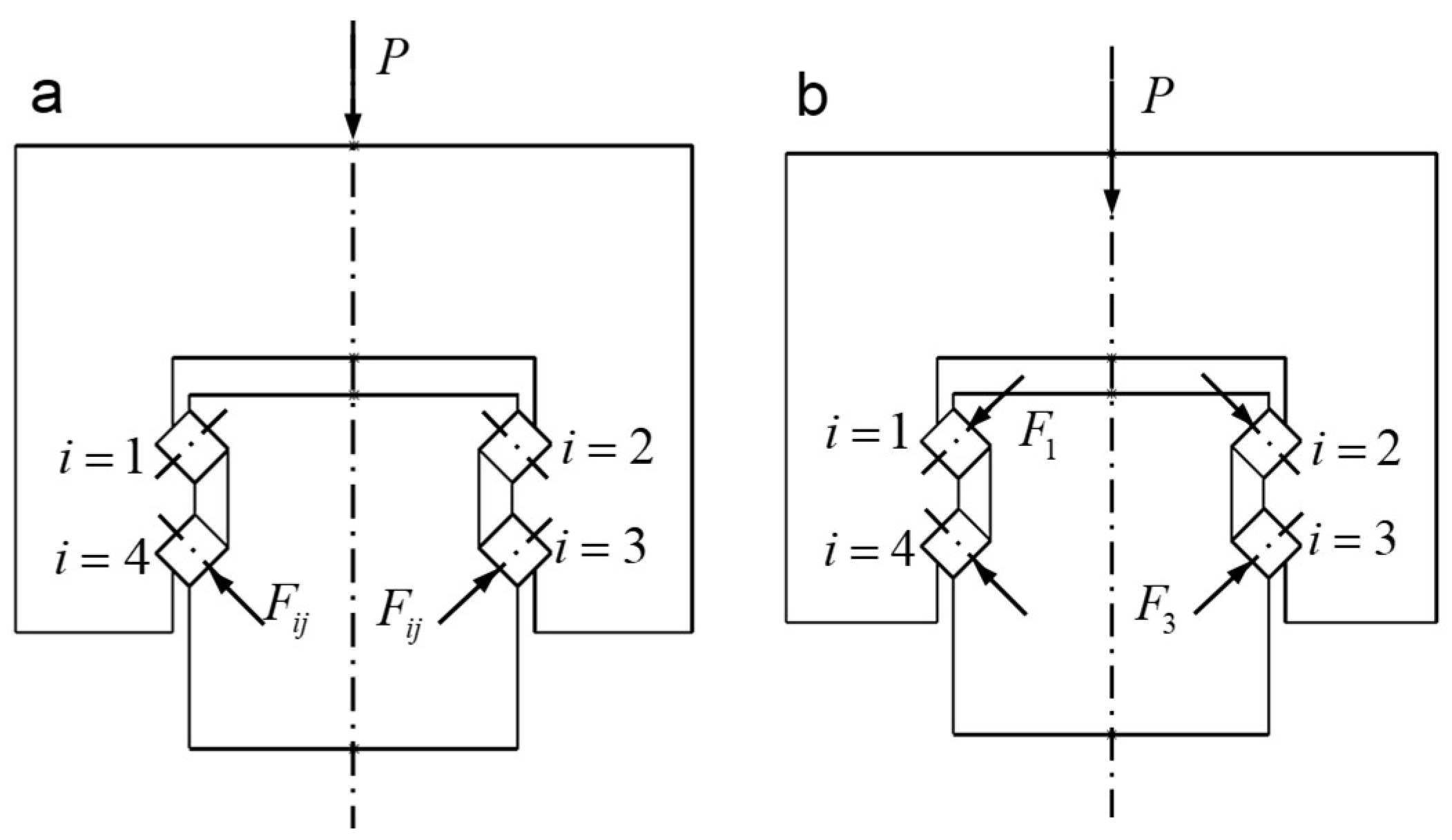
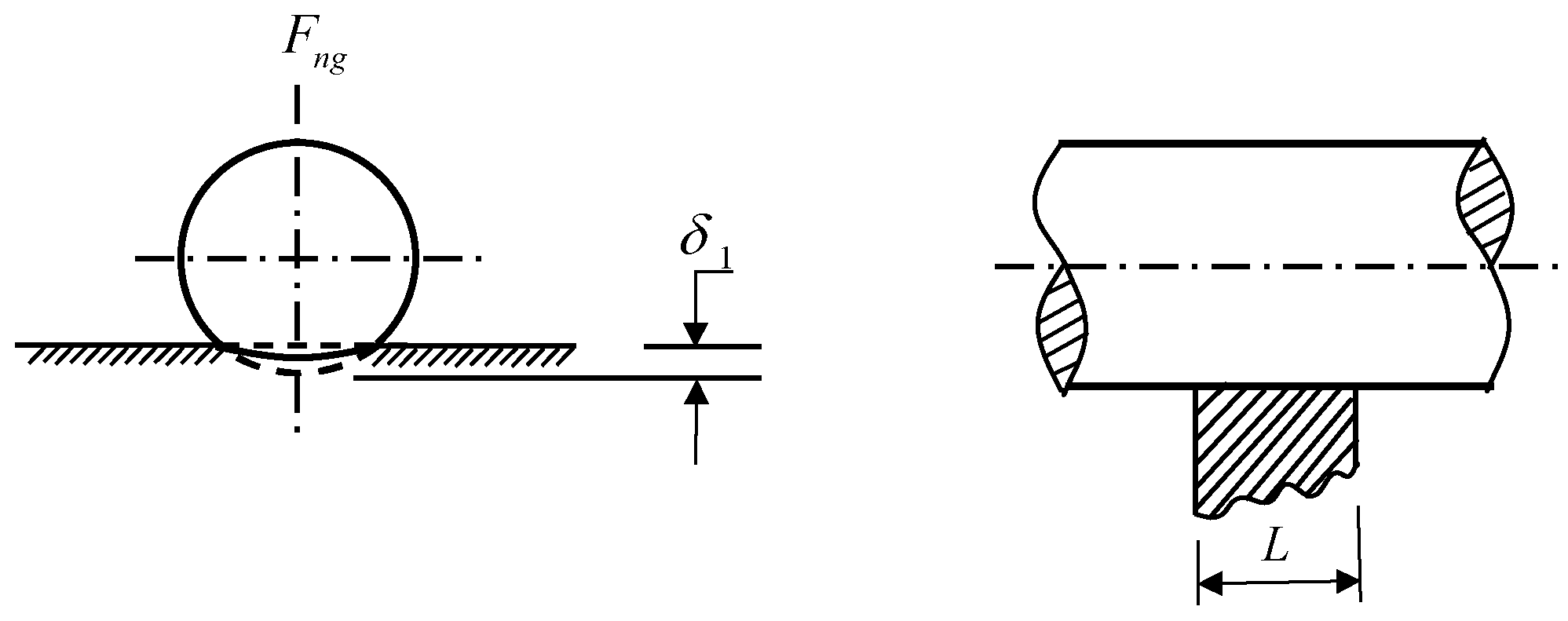
2.1. Friction Model of Guide Pair under Positive Pressure Load P
2.2. Dynamic Friction Factor Prediction Model of the Guide Rail
3. Experimental Research
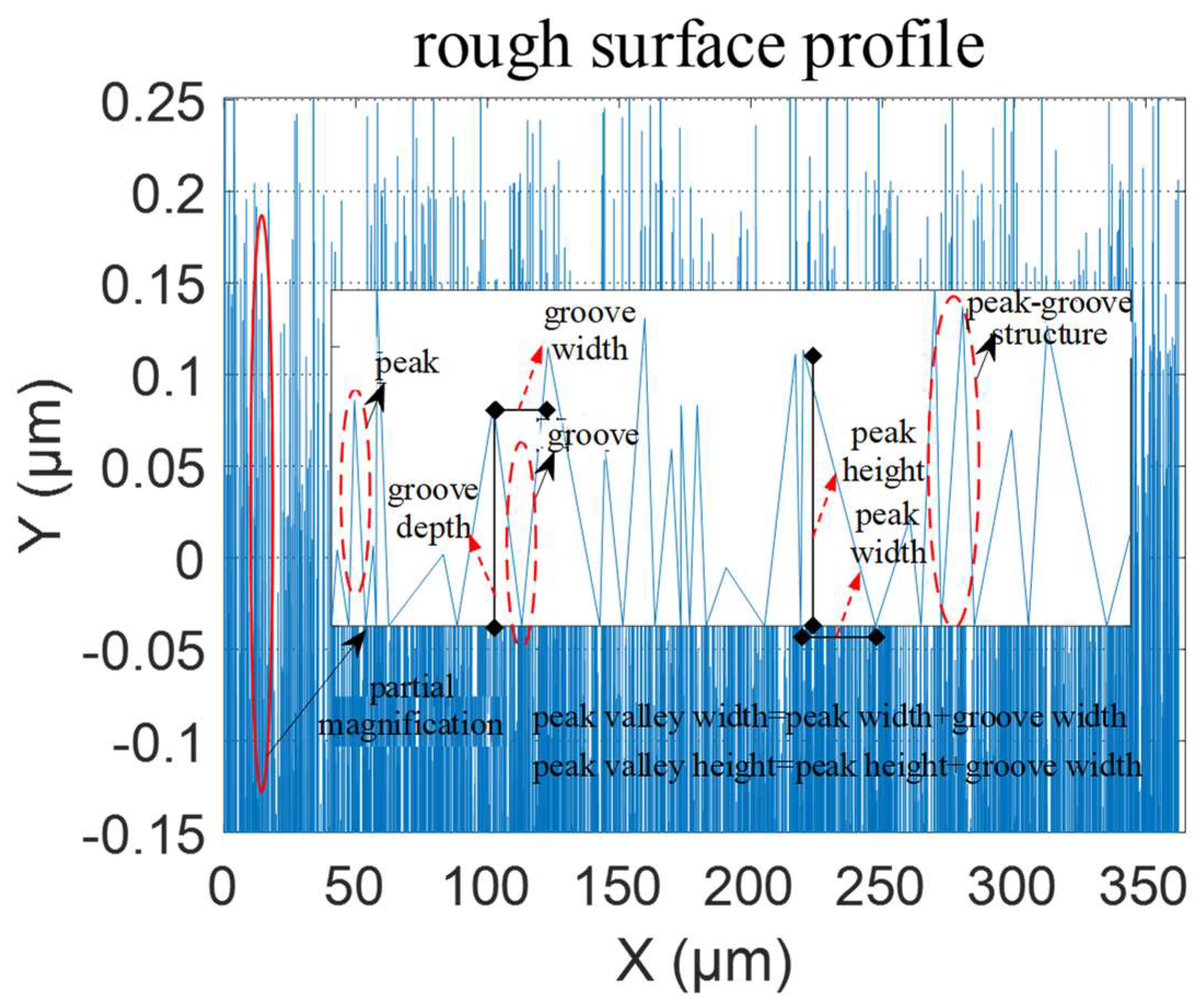
3.1. Materials
3.2. Experiments
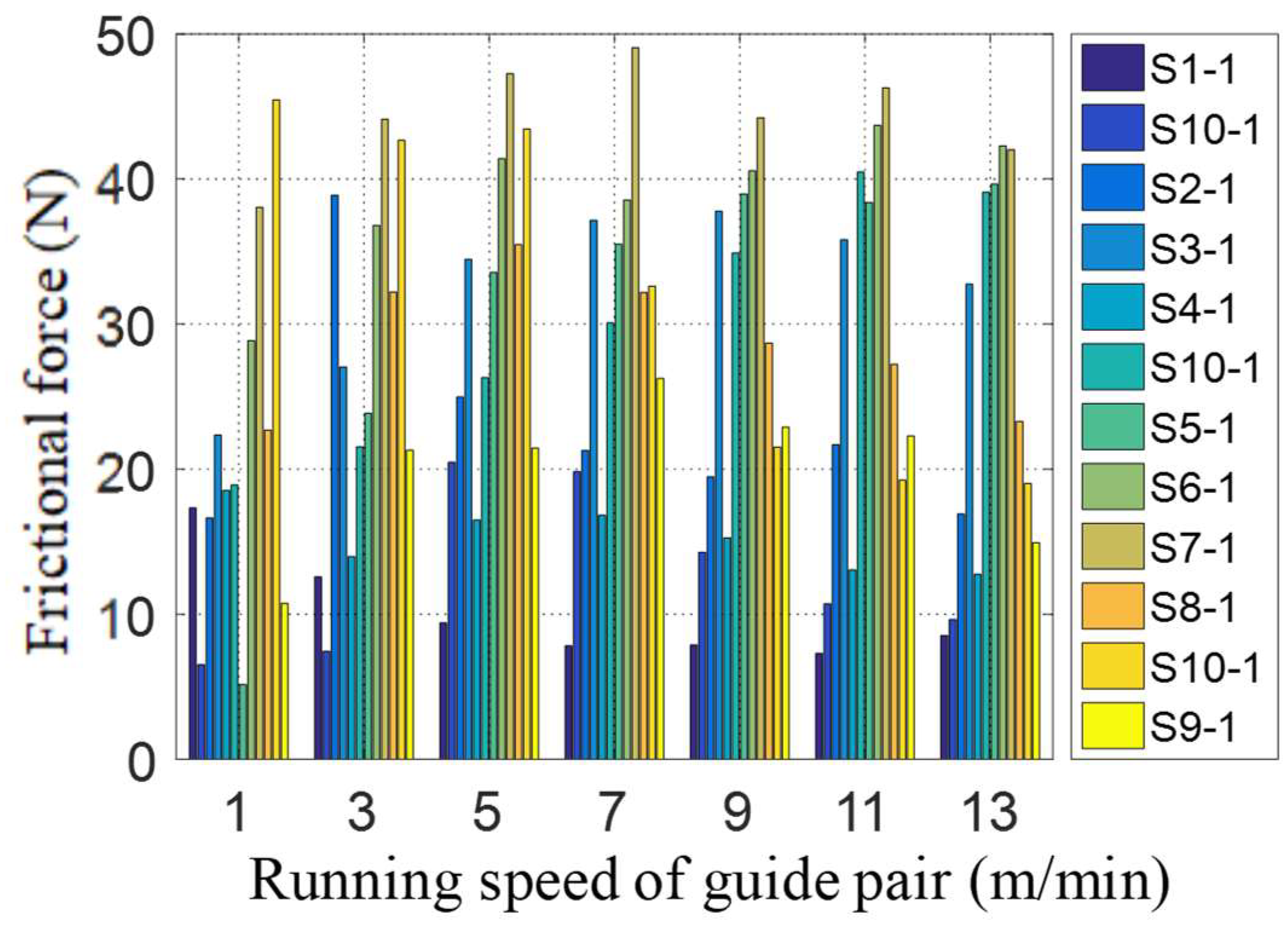
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Etsion, I.; Kligerman, Y.; Halperin, G. Analytical and experimental investigation of lase-textured mechanical seal faces. Tribol. Trans. 1999, 42, 511–516. [Google Scholar] [CrossRef]
- Etsion, I.; Halperin, G. A laser surface textured hydrostatic mechanical seal. Tribol. Trans. 2002, 45, 430–434. [Google Scholar] [CrossRef]
- Etsion, I.; Halperin, G.; Brizmer, V.; Kligerman, Y. Experimental investigation of laser surface textured parallel thrust bearings. Tribol. Lett. 2004, 17, 295–300. [Google Scholar] [CrossRef]
- Menezes, P.L.; Kishore; Kailas, S.V. Influence of surface texture on coefficient of friction and transfer layer formation during sliding of pure magnesium pin on 080 M40 (EN8) steel plate. Wear 2006, 261, 578–591. [Google Scholar] [CrossRef]
- Kim, B.; Chae, Y.H.; Choi, H.S. Effects of surface texturing on the frictional behavior of cast iron surfaces. Tribol. Int. 2014, 70, 128–135. [Google Scholar] [CrossRef]
- Costa, H.L.; Ardila, M.A.N.; Labiapari, W.S.; Silva, W.M.; de Mello, J.D.B. Effect of surface topography on the dynamics of the abrasive particles during micro-abrasion. Wear 2015, 324–325, 129–139. [Google Scholar] [CrossRef]
- Wakuda, M.; Yamauchi, Y.; Kanzaki, S.; Yasuda, Y. Effect of surface texturing on friction reduction between ceramic and steel materials under lubricated sliding contact. Wear 2003, 254, 356–363. [Google Scholar] [CrossRef]
- Magnus, C.; Gulenc, I.T.; Rainforth, W.M. Ambient dry sliding friction and wear behaviour of laser surface textured (LST) Ti3SiC2 MAX phase composite against hardened steel and alumina. Wear 2022, 490–491, 204184. [Google Scholar] [CrossRef]
- Grabon, W.; Pawlus, P.; Wos, S.; Koszela, W.; Wieczorowski, M. Effects of cylinder liner surface topography on friction and wear of liner-ring system at low temperature. Tribol. Int. 2018, 121, 148–160. [Google Scholar] [CrossRef]
- Yang, J.; Fu, H.; He, Y.; Gu, Z.; Fu, Y.; Ji, J.; Zhang, Y.; Zhou, Y. Investigation on friction and wear performance of volcano-shaped textured PVD coating. Surf. Coat. Technol. 2022, 431, 128044. [Google Scholar] [CrossRef]
- Wang, H.; He, D.; Wu, Y.; Xu, S. Study on wear state evaluation of friction stir welding tools based on image of surface topography. Measurement 2021, 186, 110173. [Google Scholar] [CrossRef]
- Pei, X.; Pu, W.; Zhang, Y.; Huang, L. Surface topography and friction coefficient evolution during sliding wear in a mixed lubricated rolling-sliding contact. Tribol. Int. 2019, 137, 303–312. [Google Scholar] [CrossRef]
- Zhu, D.; Martini, A.; Wang, W.; Hu, Y.; Lisowsky, B.; Wang, Q.J. Simulation of sliding wear in mixed lubrication. J. Tribol. 2007, 129, 544–552. [Google Scholar] [CrossRef]
- Saeidi, F.; Meylan, B.; Hoffmann, P.; Wasmer, K. Effect of surface texturing on cast iron reciprocating against steel under starved lubrication conditions: A parametric study. Wear 2016, 348–349, 17–26. [Google Scholar] [CrossRef] [Green Version]
- Yu, H.; Huang, W.; Wang, X. Dimple patterns design for different circumstances. Lubr. Sci. 2013, 25, 67–78. [Google Scholar] [CrossRef]
- Braun, D.; Greiner, C.; Schneider, J.; Gumbsch, P. Efficiency of laser surface texturing in the reduction of friction under mixed lubrication. Tribol. Int. 2014, 77, 142–147. [Google Scholar] [CrossRef]
- Segu, D.Z.; Kim, S.S. Influence on friction behavior of micro-texturing under lubricated non-conformal contact. Meccanica 2014, 49, 483–492. [Google Scholar] [CrossRef]
- Nakano, M.; Korenaga, A.; Korenaga, A.; Miyake, K.; Murakami, T.; Ando, Y.; Usami, H.; Sasaki, S. Applying micro-texture to cast iron surfaces to reduce the friction coefficient under lubricated conditions. Tribol. Lett. 2007, 28, 131–137. [Google Scholar] [CrossRef]
- Ren, N.; Nanbu, T.; Yasuda, Y.; Zhu, D.; Wang, Q. Micro textures in concentratedconformal-contact lubrication: Effect of distribution patterns. Tribol. Lett. 2007, 28, 275–285. [Google Scholar] [CrossRef]
- Andersson, P.; Koskinen, J.; Varjus, S.E.; Gerbig, Y.; Haefke, H.; Georgiou, S.; Zhmud, B.; Buss, W. Microlubrication effect by laser-textured steel surfaces. Wear 2007, 262, 369–379. [Google Scholar] [CrossRef]
- Wang, Z.; He, H.; Wu, S.; Xiang, J.; Ni, J. Effect of surface topography and wettability on friction properties of CFRPEEK. Tribol. Int. 2022, 171, 107573. [Google Scholar] [CrossRef]
- Liu, S. Mechanical Performance Analysis of Roller Linear Guide Pair. Ph.D. Dissertation, Huazhong University of Science and Technology, Wuhan, China, 2011. [Google Scholar]
- Johson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Harris, T.A.; Kotzalas, M.N. Polling Bearing Analysis: First Volume, 5th ed.; Taylor & Francis: Boca Raton, FL, USA, 2007. [Google Scholar]
- Kragelsky, I.V.; Dobychin, M.N.; Kombalov, V.S. Friction and Wear: Calculation Methods; Pergamon Press: Oxford, UK, 1982. [Google Scholar]
- Oh, K.J.; Khim, G.; Park, C.H.; Chung, S.C. Explicit modeling and investigation of friction forces in linear motion ball guides. Tribol. Int. 2019, 129, 16–28. [Google Scholar] [CrossRef]
- Cheng, D.J.; Park, T.J.; Kim, S.J. Improved friction model for the roller LM guide considering mechanics analysis. J. Mech. Sci. Technol. 2018, 32, 2723–2734. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, M.; Kong, D. Modeling and experimentation of the friction force of linear rolling guide pair considering oil film resistance. Chin. J. Eng. 2017, 39, 1718–1726. (In Chinese) [Google Scholar]
- Wang, Y.; Zu, L.; Ou, Y.; Feng, H. Relationship between frictional force and preload of linear rolling guide. Modul. Mach. Tool Autom. Manuf. Tech. 2018, 6, 12–14. (In Chinese) [Google Scholar]
- Ge, S.; Zhu, H. Fractals in Tribology; Mechanical Industry Press: Shanghai, China, 2005. [Google Scholar]
- Norden, B.N. On the Compression of a Cylinder in Contact with a Plane Surface; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1973. [Google Scholar]
- Hu, Y.; Gan, Z. Tolerance Fit and Measurement—Practical Planning Textbook for Vocational and Technical Colleges in the New Century; Tsinghua University Press: Beijing, China, 2005. [Google Scholar]
- Liu, J. Theory and Experiment of Rolling Linear Guideway. Ph.D. Dissertation, Huazhong University of Science and Technology, Wuhan, China, 1985. [Google Scholar]

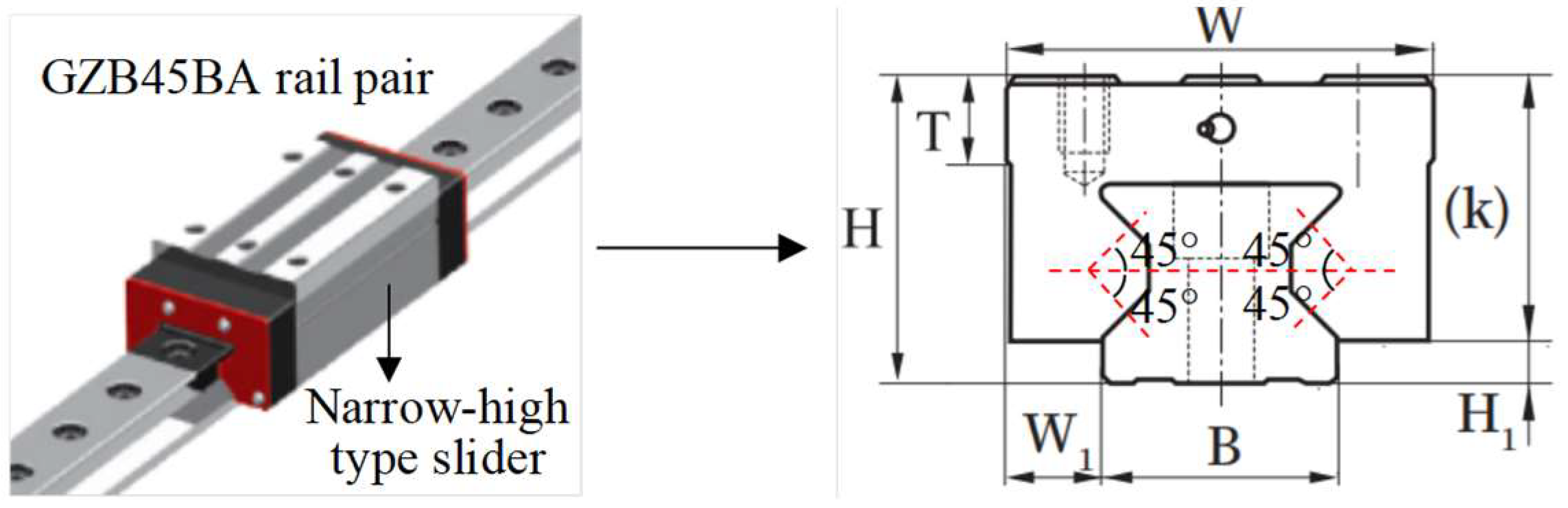
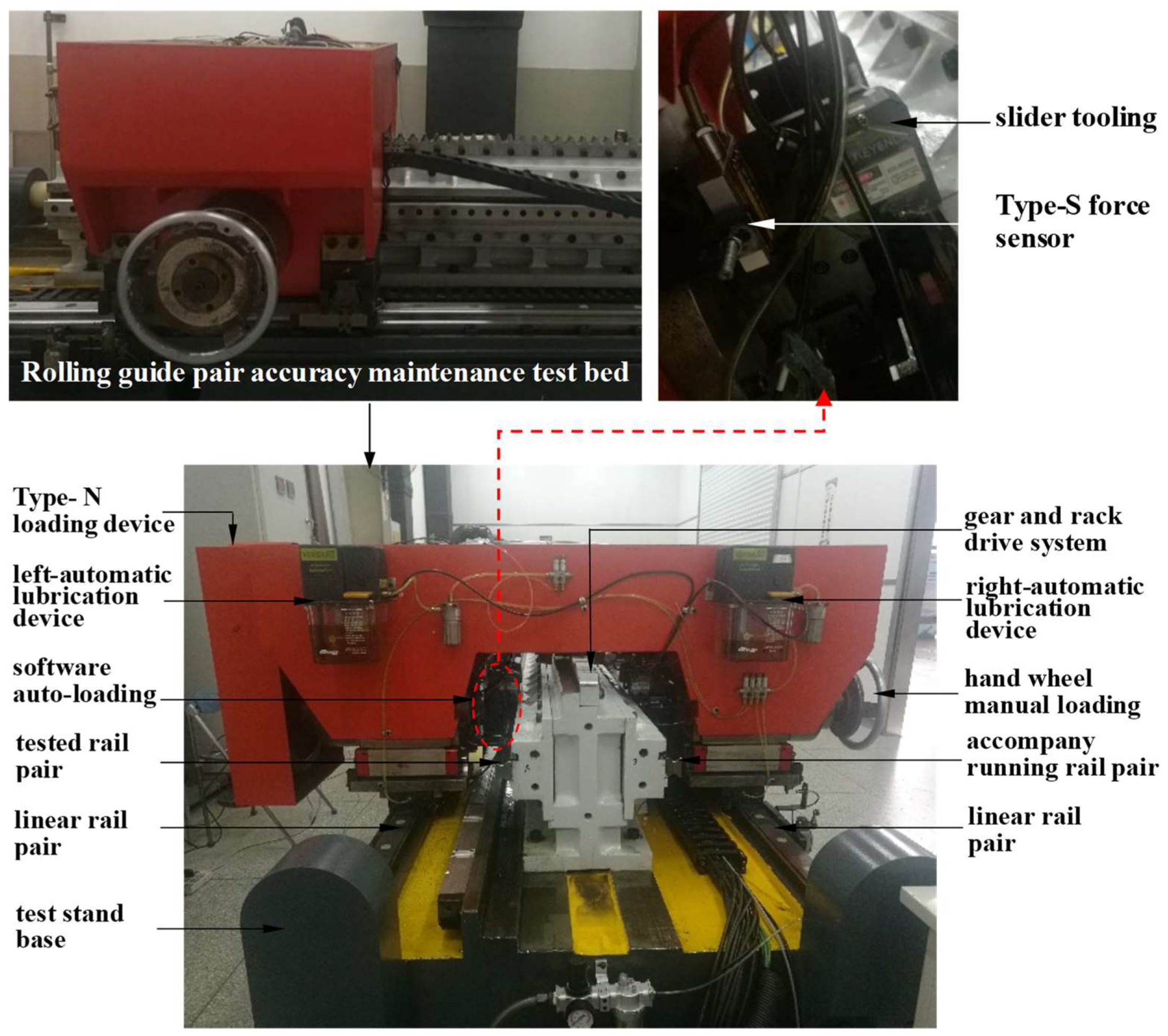

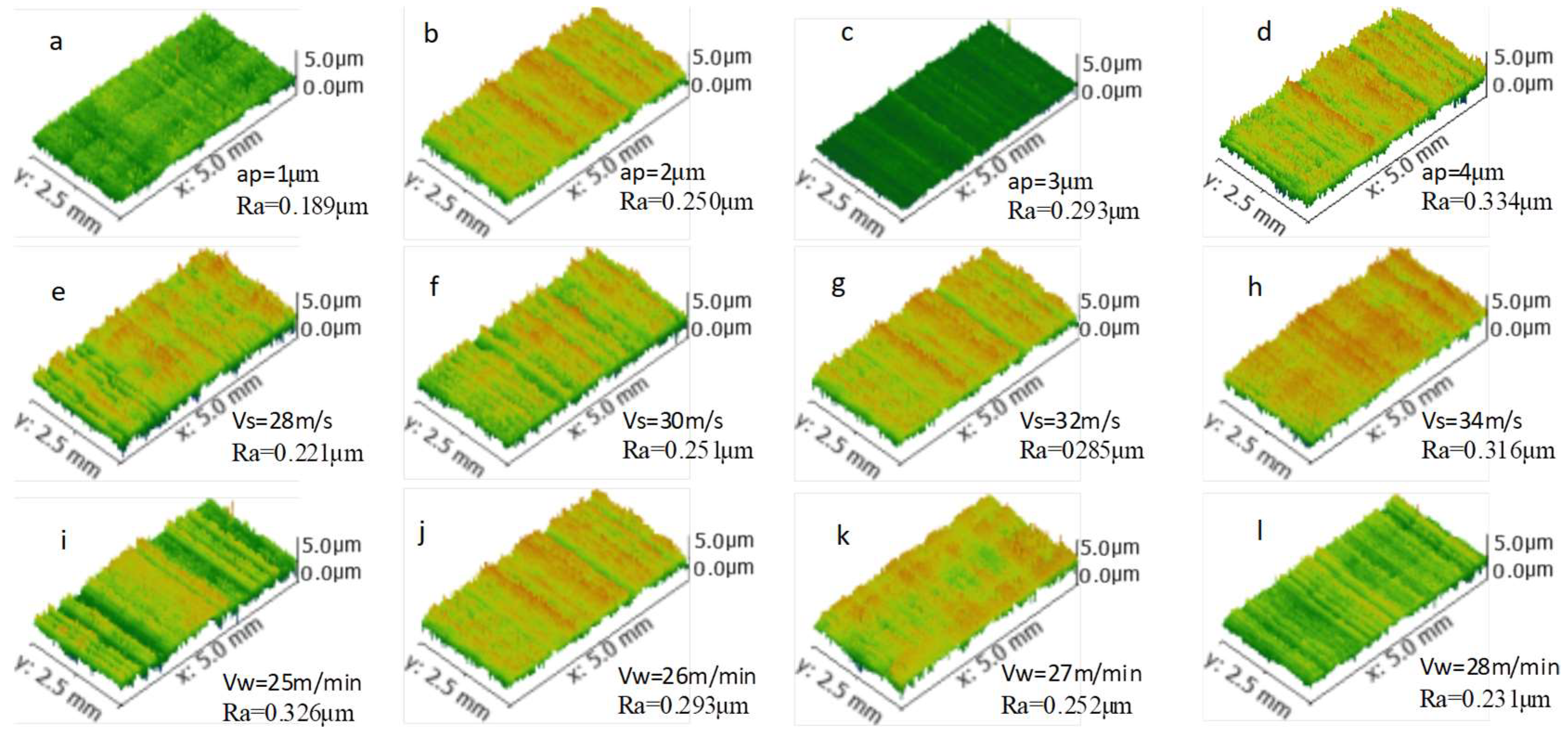

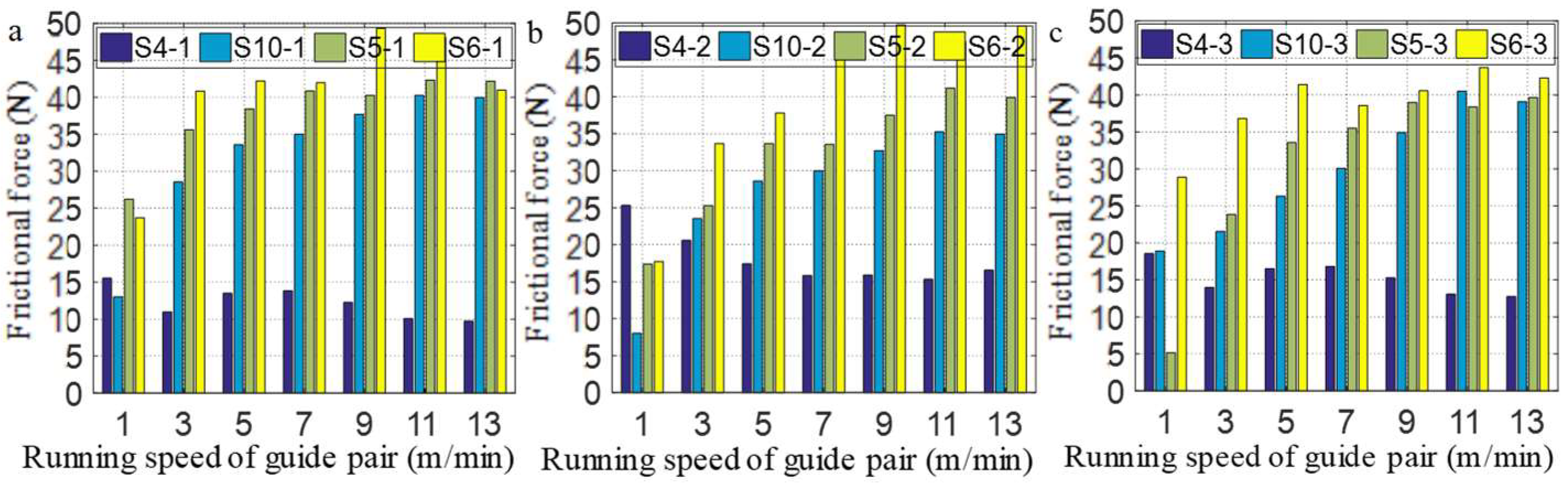

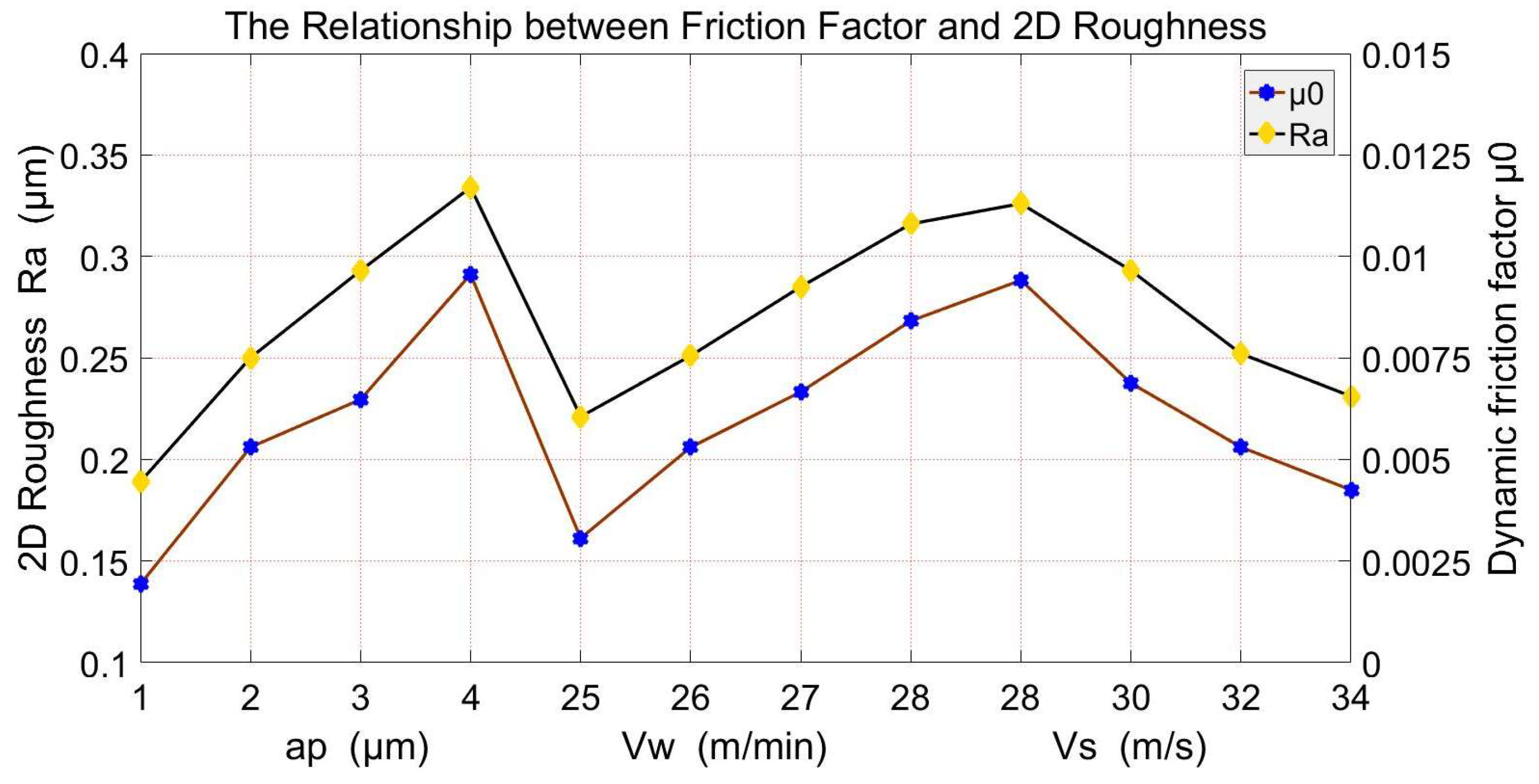
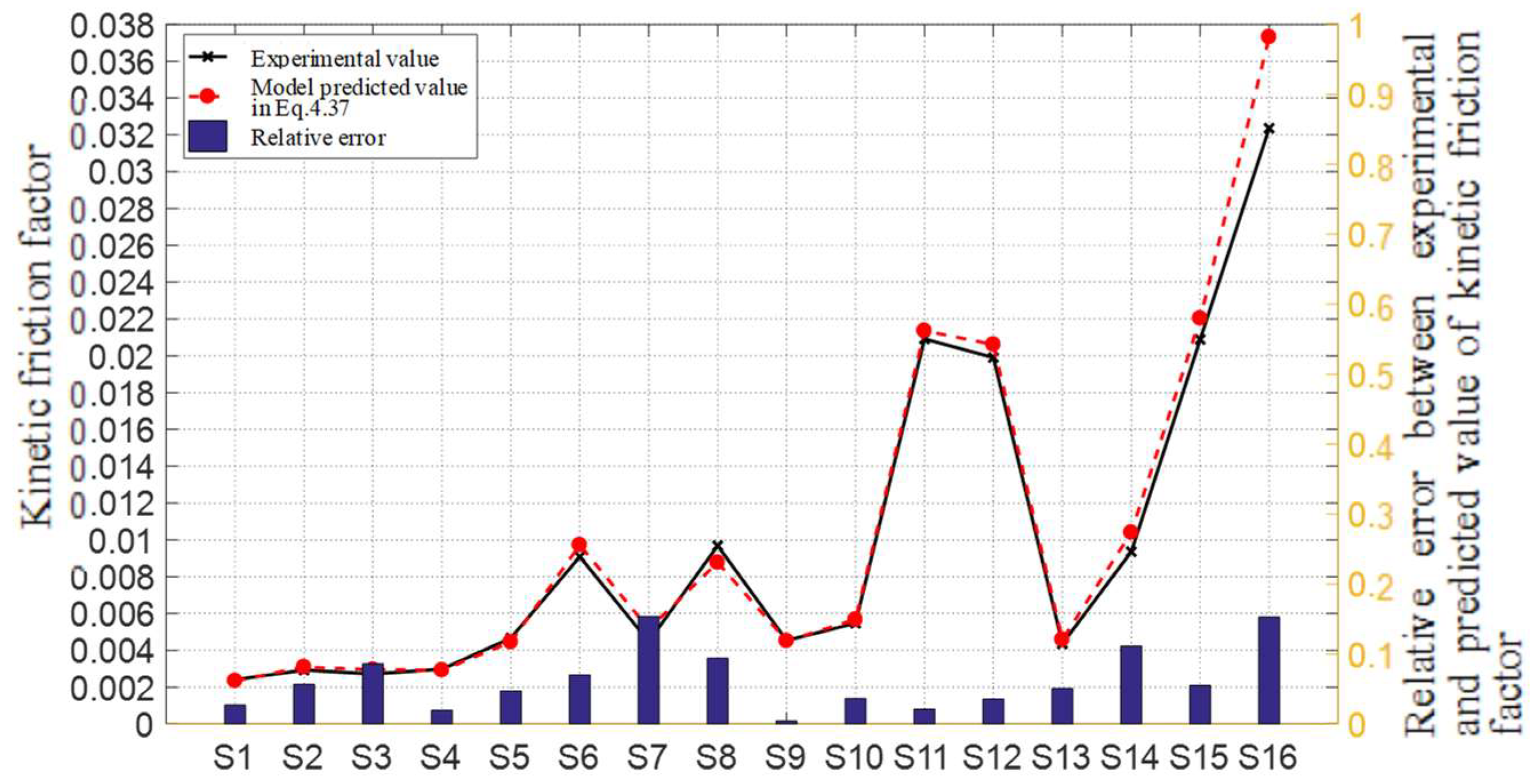
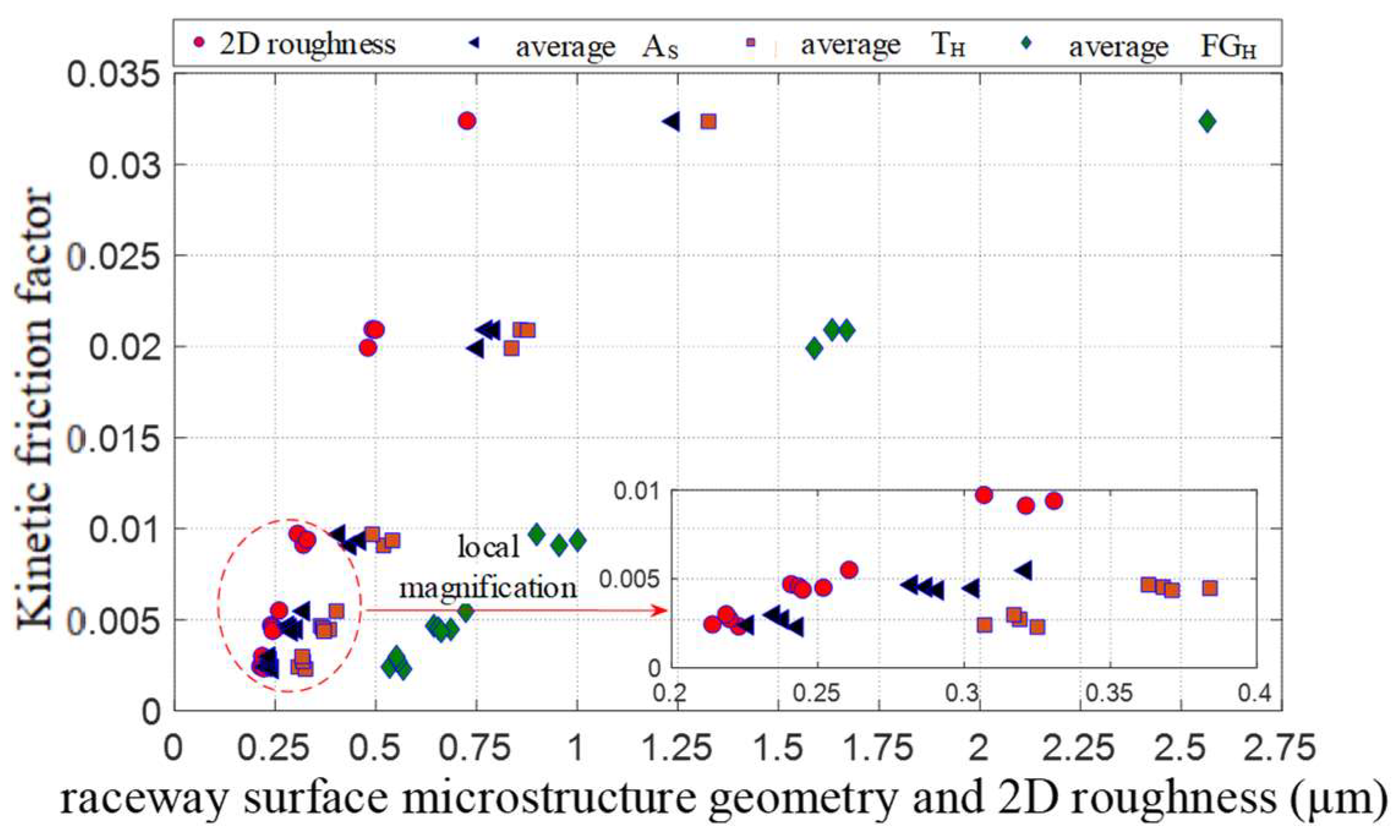
| Slider | Ra [μm] | AS | AW | ASW | TH | TW | THW | FGH | FGW | Test Value μ0 | Predictive Value μ0 | Residual |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| S1 | 0.189 | 0.214 | 0.295 | 2.740 | 0.295 | 0.295 | 1.444 | 0.509 | 0.589 | 0.001932 | 0.001910 | 2.1652 × 10−5 |
| S10 | 0.250 | 0.296 | 0.294 | 3.578 | 0.377 | 0.294 | 1.848 | 0.672 | 0.588 | 0.005299 | 0.004881 | 4.1800 × 10−4 |
| S2 | 0.293 | 0.377 | 0.294 | 4.312 | 0.458 | 0.294 | 2.245 | 0.835 | 0.587 | 0.006472 | 0.006594 | −1.2180 × 10−4 |
| S3 | 0.334 | 0.460 | 0.293 | 5.119 | 0.541 | 0.293 | 2.657 | 1.002 | 0.586 | 0.009554 | 0.009432 | 1.2180 × 10−4 |
| S4 | 0.221 | 0.239 | 0.295 | 2.952 | 0.319 | 0.294 | 1.564 | 0.559 | 0.589 | 0.003060 | 0.003162 | −1.0216 × 10−4 |
| S10 | 0.251 | 0.296 | 0.295 | 3.596 | 0.377 | 0.294 | 1.844 | 0.673 | 0.589 | 0.005299 | 0.005237 | 6.1534 × 10−5 |
| S5 | 0.285 | 0.352 | 0.294 | 4.083 | 0.434 | 0.294 | 2.119 | 0.786 | 0.588 | 0.006659 | 0.006749 | −8.9743 × 10−5 |
| S6 | 0.316 | 0.408 | 0.293 | 4.637 | 0.491 | 0.294 | 2.401 | 0.899 | 0.587 | 0.008409 | 0.008275 | 1.3363 × 10−4 |
| S7 | 0.326 | 0.436 | 0.293 | 4.880 | 0.520 | 0.294 | 2.541 | 0.955 | 0.587 | 0.009410 | 0.009427 | −1.6539 × 10−5 |
| S8 | 0.293 | 0.366 | 0.294 | 4.269 | 0.448 | 0.294 | 2.188 | 0.815 | 0.588 | 0.006875 | 0.007105 | −2.3007 × 10−4 |
| S10 | 0.252 | 0.296 | 0.295 | 3.570 | 0.377 | 0.295 | 1.842 | 0.673 | 0.589 | 0.005299 | 0.004674 | 6.2500 × 10−4 |
| S9 | 0.231 | 0.260 | 0.294 | 3.205 | 0.341 | 0.293 | 1.667 | 0.601 | 0.588 | 0.004248 | 0.004090 | 1.5772 × 10−4 |
| H | W1 | Slider | K | W | T | H1 | Slider Length | Raceway Length | B | E | Max. Length of Single Piece | Rated Dynamic Load (kN) | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| guide rail pair | 70 | 20.5 | 62 | 86 | 15 | 8 | 153 | 106 | guide rail | 45 | 38 | 6000 | 92.6 |
| No. | Vw [m/min] | Vs [m/s] | ap [μm] | Experimental Samples and Devices | |
|---|---|---|---|---|---|
| S1 | 25 | 32 | 1 | Grinding workpiece | GZB45BA |
| S2 | 25 | 32 | 3 | ||
| S3 | 25 | 32 | 4 | Workpiece material | GCr15 |
| S4 | 25 | 28 | 2 | Wheel type | 1A46X25X13-SA100K35 |
| S5 | 25 | 30 | 2 | Wheel material | alumina |
| S6 | 25 | 34 | 2 | Grinder | PLANMATHP408 |
| S7 | 26 | 32 | 2 | Grinding fluid | Fully synthetic water-soluble cutting fluid |
| S8 | 27 | 32 | 2 | ||
| S9 | 28 | 32 | 2 | ||
| S10 | 25 | 32 | 2 | ||
| Number of Trials | Ap [μm] | Vw [m/min] | Vs [m/s] | Number of Sliders |
|---|---|---|---|---|
| 1 | 2 | 25 | 28 | 3 |
| 2 | 2 | 28 | 30 | 3 |
| 3 | 2 | 31 | 32 | 3 |
| 4 | 2 | 34 | 34 | 3 |
| 5 | 3 | 25 | 30 | 3 |
| 6 | 3 | 28 | 28 | 3 |
| 7 | 3 | 31 | 34 | 3 |
| 8 | 3 | 34 | 32 | 3 |
| 9 | 4 | 25 | 32 | 3 |
| 10 | 4 | 28 | 34 | 3 |
| 11 | 4 | 31 | 28 | 3 |
| 12 | 4 | 34 | 30 | 3 |
| 13 | 5 | 25 | 34 | 3 |
| 14 | 5 | 28 | 32 | 3 |
| 15 | 5 | 31 | 30 | 3 |
| 16 | 5 | 34 | 28 | 3 |
| Slider | Ra [μm] | AS | AW | TH | TW | FGH | FGW | Experimental Value μ0 | Predictive Value μ0 | Residual | Relative Error |
|---|---|---|---|---|---|---|---|---|---|---|---|
| S1 | 0.2142 | 0.226 | 0.294 | 0.307 | 0.294 | 0.534 | 0.588 | 0.002408 | 0.002343 | 6.53 × 10−5 | 0.0271 |
| S2 | 0.2232 | 0.243 | 0.293 | 0.325 | 0.293 | 0.568 | 0.586 | 0.002927 | 0.003092 | −1.65 × 10−4 | 0.0564 |
| S3 | 0.2200 | 0.238 | 0.294 | 0.319 | 0.294 | 0.556 | 0.588 | 0.002718 | 0.002952 | −2.33 × 10−4 | 0.0859 |
| S4 | 0.2189 | 0.235 | 0.294 | 0.317 | 0.294 | 0.551 | 0.588 | 0.002978 | 0.002921 | 5.74 × 10−5 | 0.0193 |
| S5 | 0.2411 | 0.282 | 0.295 | 0.363 | 0.294 | 0.644 | 0.589 | 0.004668 | 0.004448 | 2.21 × 10−4 | 0.0472 |
| S6 | 0.3213 | 0.436 | 0.293 | 0.519 | 0.294 | 0.955 | 0.587 | 0.009087 | 0.009725 | −6.38 × 10−4 | 0.0702 |
| S7 | 0.2521 | 0.303 | 0.293 | 0.384 | 0.294 | 0.686 | 0.587 | 0.004473 | 0.005162 | −6.89 × 10−4 | 0.1539 |
| S8 | 0.3070 | 0.408 | 0.294 | 0.491 | 0.294 | 0.899 | 0.589 | 0.009685 | 0.008773 | 9.12 × 10−4 | 0.0941 |
| S9 | 0.2437 | 0.287 | 0.294 | 0.368 | 0.294 | 0.655 | 0.588 | 0.004537 | 0.004517 | 1.99 × 10−5 | 0.0044 |
| S10 | 0.2609 | 0.321 | 0.294 | 0.402 | 0.294 | 0.723 | 0.588 | 0.005473 | 0.005673 | −2.00 × 10−4 | 0.0366 |
| S11 | 0.4930 | 0.774 | 0.294 | 0.859 | 0.294 | 1.633 | 0.588 | 0.020916 | 0.021360 | −4.44 × 10−4 | 0.0212 |
| S12 | 0.4816 | 0.752 | 0.293 | 0.837 | 0.293 | 1.589 | 0.586 | 0.019904 | 0.020612 | −7.08 × 10−4 | 0.0356 |
| S13 | 0.2450 | 0.291 | 0.294 | 0.371 | 0.294 | 0.662 | 0.588 | 0.004360 | 0.004582 | −2.22 × 10−4 | 0.0509 |
| S14 | 0.3309 | 0.460 | 0.294 | 0.541 | 0.294 | 1.001 | 0.587 | 0.009357 | 0.010399 | −1.04 × 10−3 | 0.1114 |
| S15 | 0.5006 | 0.793 | 0.293 | 0.877 | 0.294 | 1.669 | 0.587 | 0.020889 | 0.022035 | −1.15 × 10−3 | 0.0549 |
| S16 | 0.7276 | 1.239 | 0.294 | 1.326 | 0.294 | 2.565 | 0.588 | 0.032362 | 0.037312 | −4.95 × 10−3 | 0.1530 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, M.; Hua, D.; Guo, X. Study on the Influence of Micro-Features in the Surface Topography of the Slider Raceway on the Dynamic Friction Factor of the Guide Rail Pair. Lubricants 2023, 11, 321. https://doi.org/10.3390/lubricants11080321
Kang M, Hua D, Guo X. Study on the Influence of Micro-Features in the Surface Topography of the Slider Raceway on the Dynamic Friction Factor of the Guide Rail Pair. Lubricants. 2023; 11(8):321. https://doi.org/10.3390/lubricants11080321
Chicago/Turabian StyleKang, Mingxia, Dezheng Hua, and Xiaoqiang Guo. 2023. "Study on the Influence of Micro-Features in the Surface Topography of the Slider Raceway on the Dynamic Friction Factor of the Guide Rail Pair" Lubricants 11, no. 8: 321. https://doi.org/10.3390/lubricants11080321
APA StyleKang, M., Hua, D., & Guo, X. (2023). Study on the Influence of Micro-Features in the Surface Topography of the Slider Raceway on the Dynamic Friction Factor of the Guide Rail Pair. Lubricants, 11(8), 321. https://doi.org/10.3390/lubricants11080321





