Numerical Analysis of the Mixed-Lubrication Performance of Staved Stern Tube Bearings Lubricated with Water
Abstract
1. Introduction
2. Methods and Material
2.1. Governing Equation
2.1.1. Hydrodynamic Pressure Model
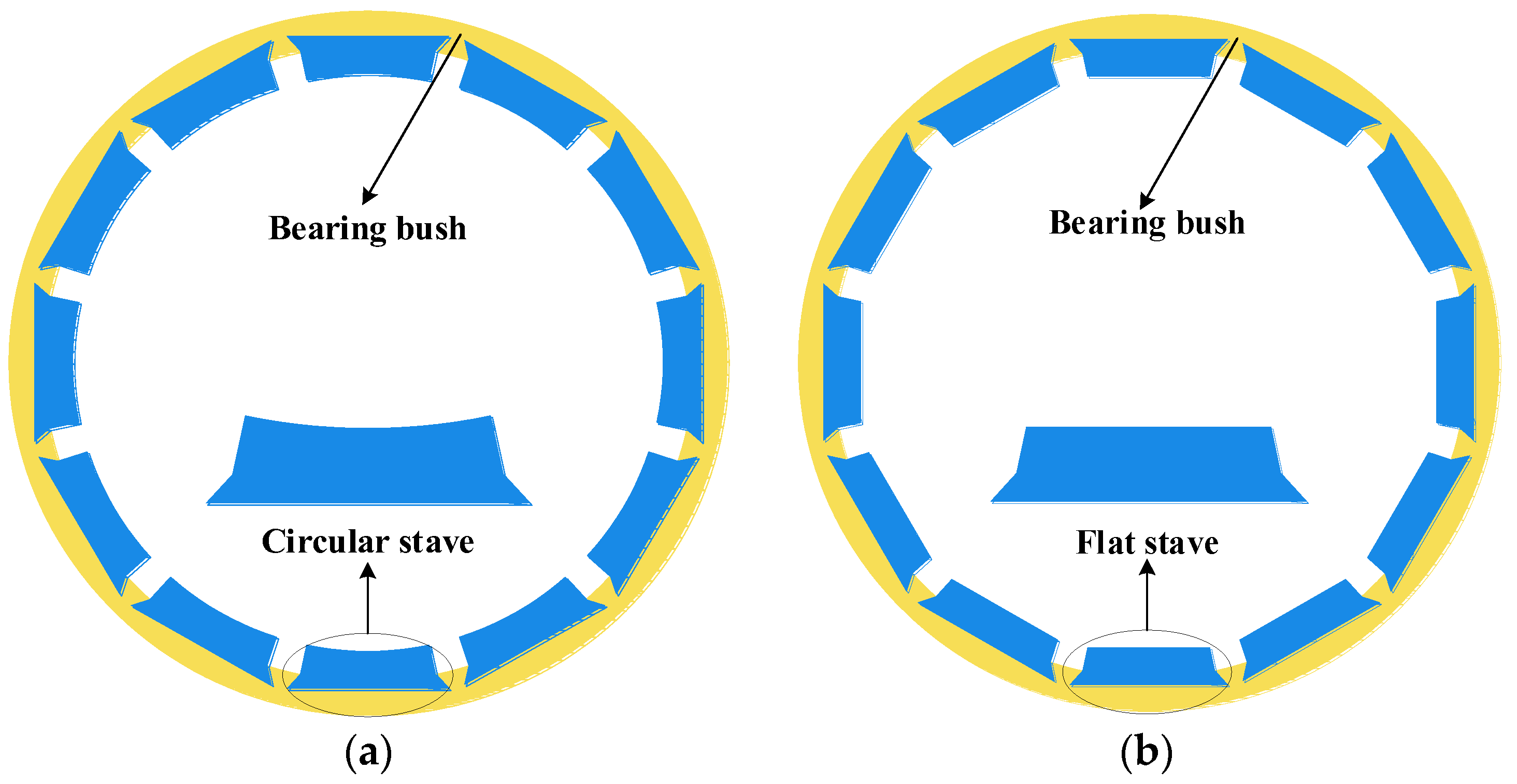
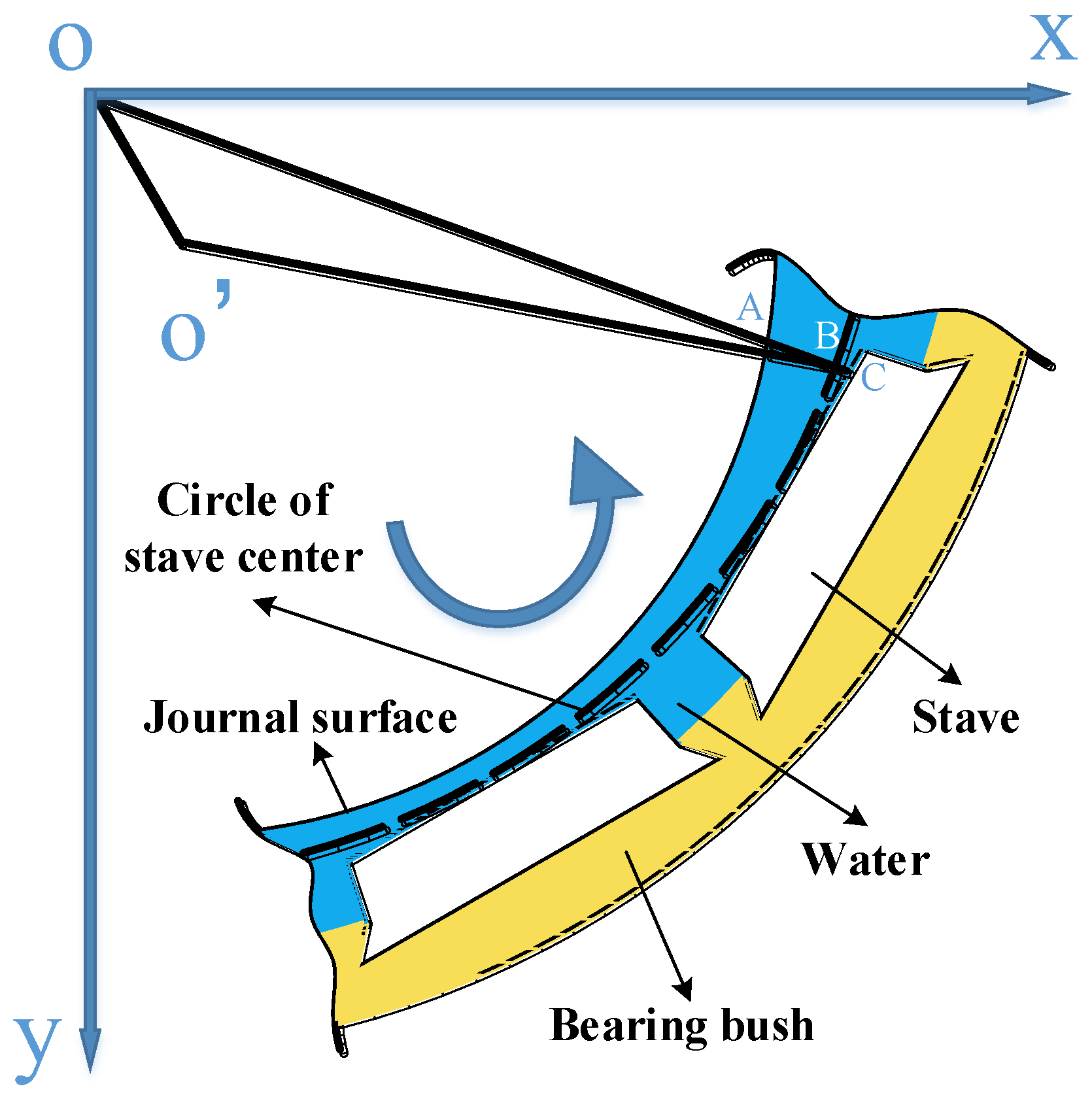
2.1.2. Geometrical Model of a Staved Bearing
2.1.3. Asperity Contact Model
2.2. Boundary Conditions
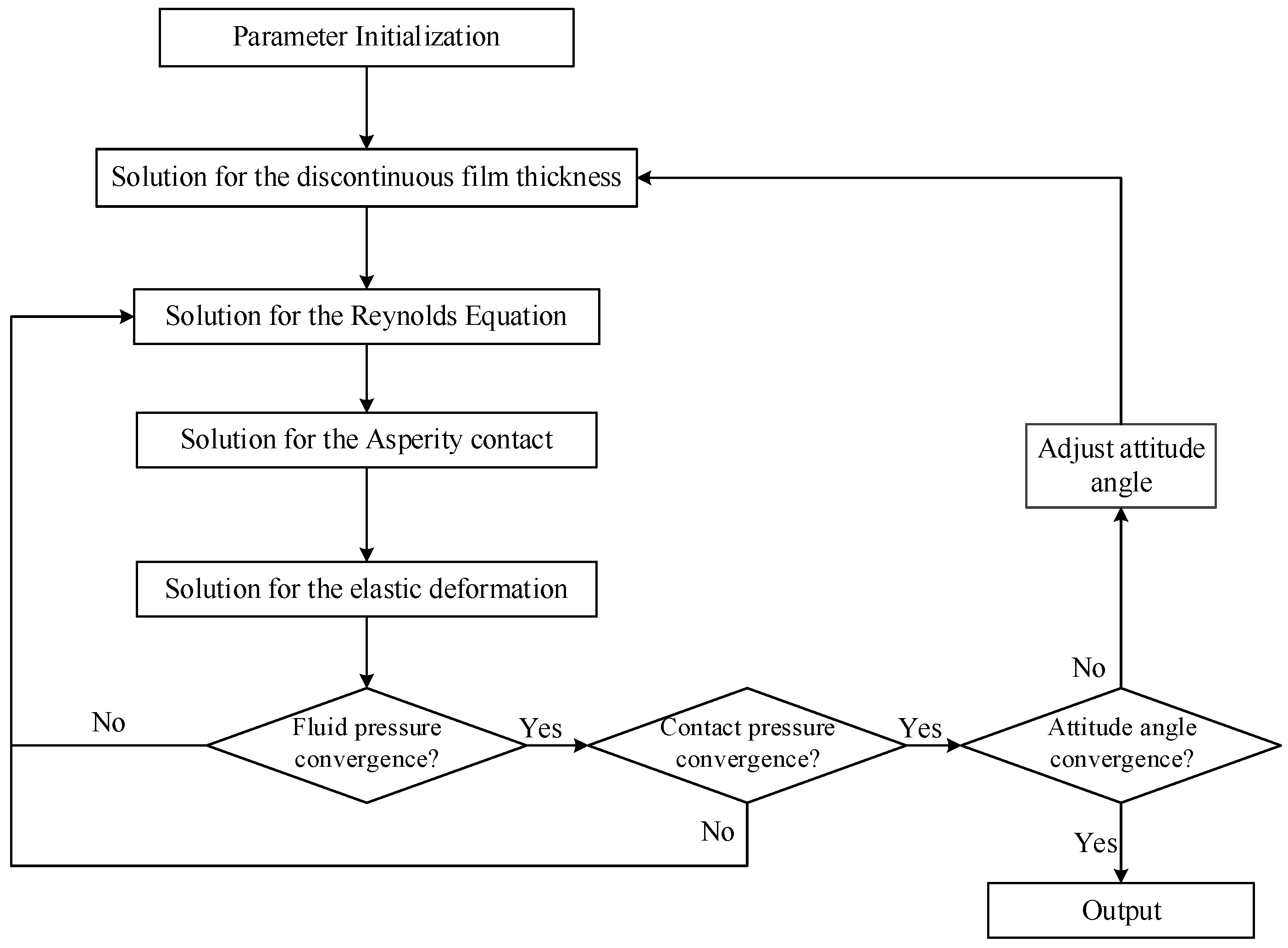
2.3. Numerical Procedure
3. Results and Discussion
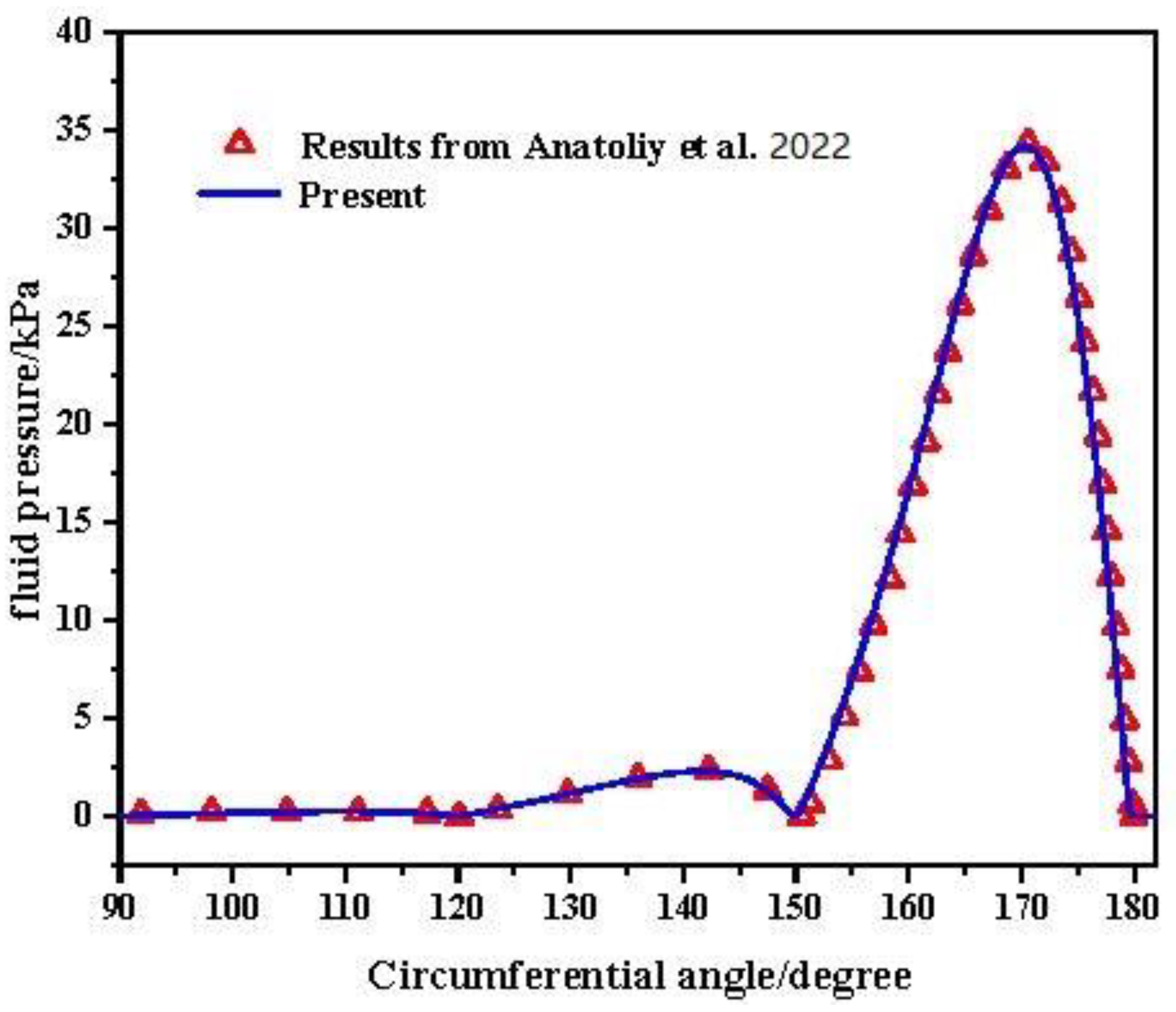
3.1. Verification
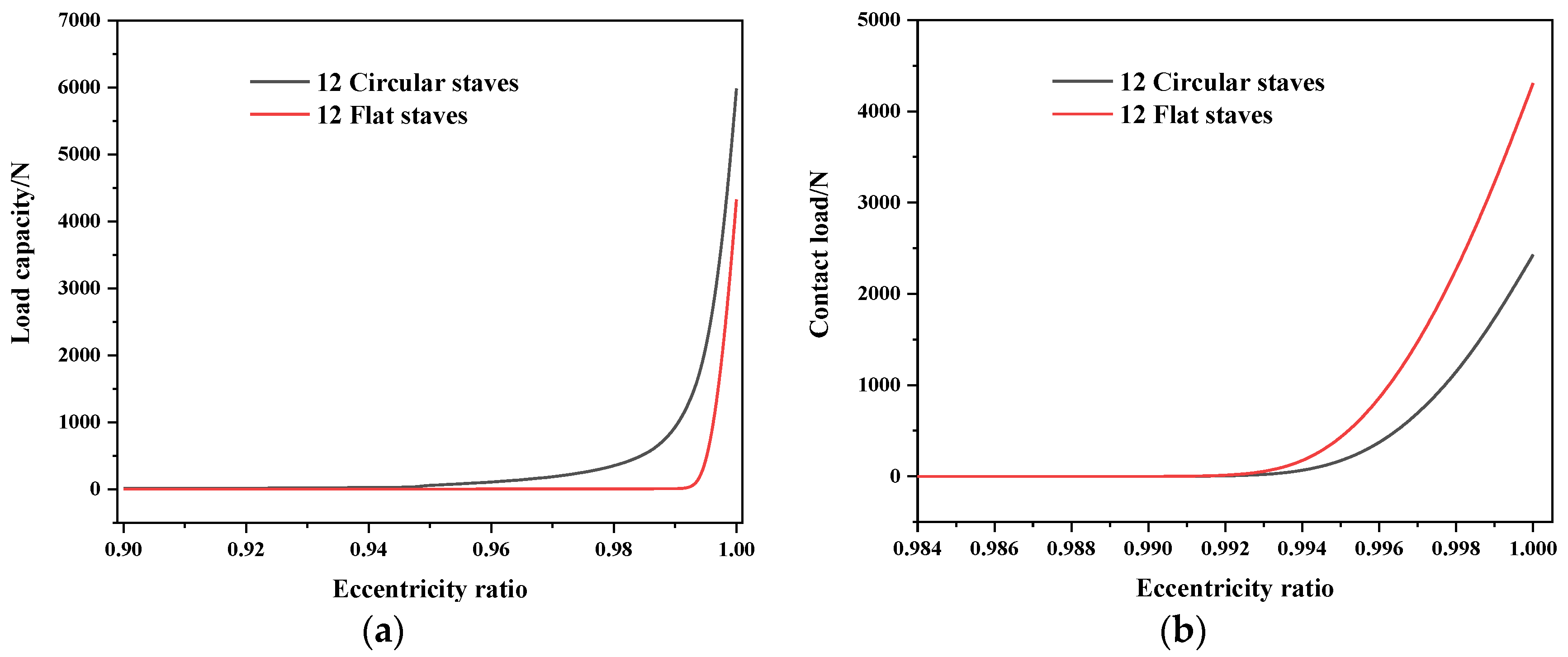
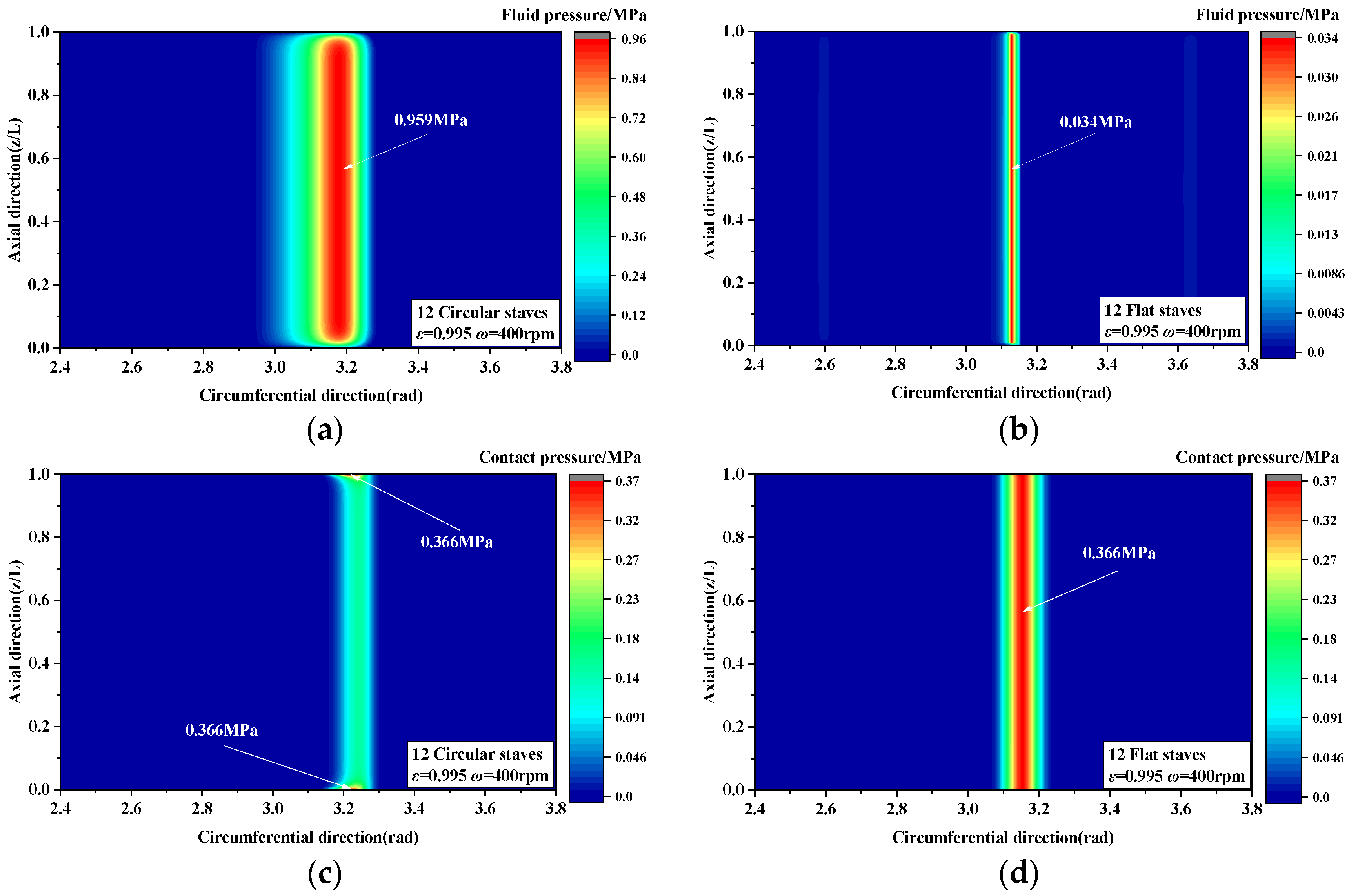
3.2. Performance Comparison between Bearings with Circular and Flat Staves
3.2.1. Load Capacity of the Staved Bearings
3.2.2. Mixed Lubrication Distributions of Staved Bearings
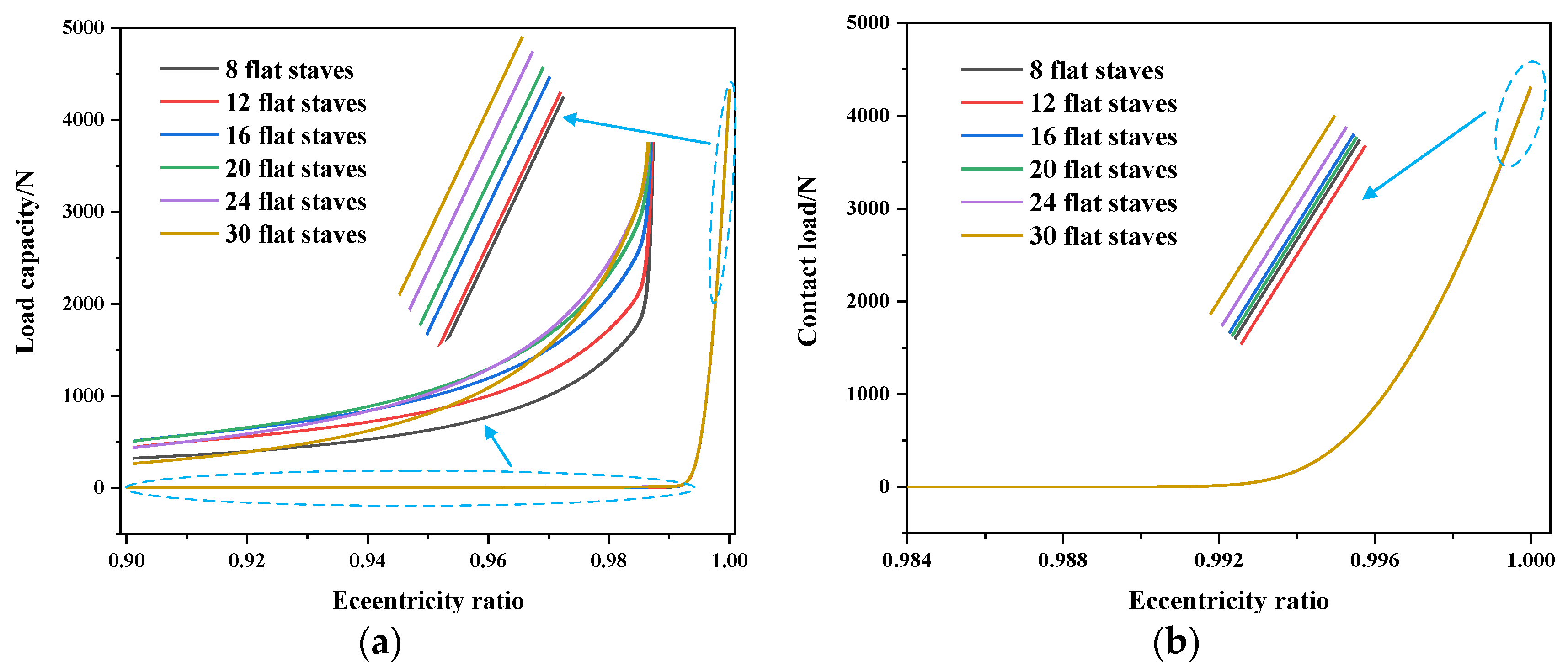
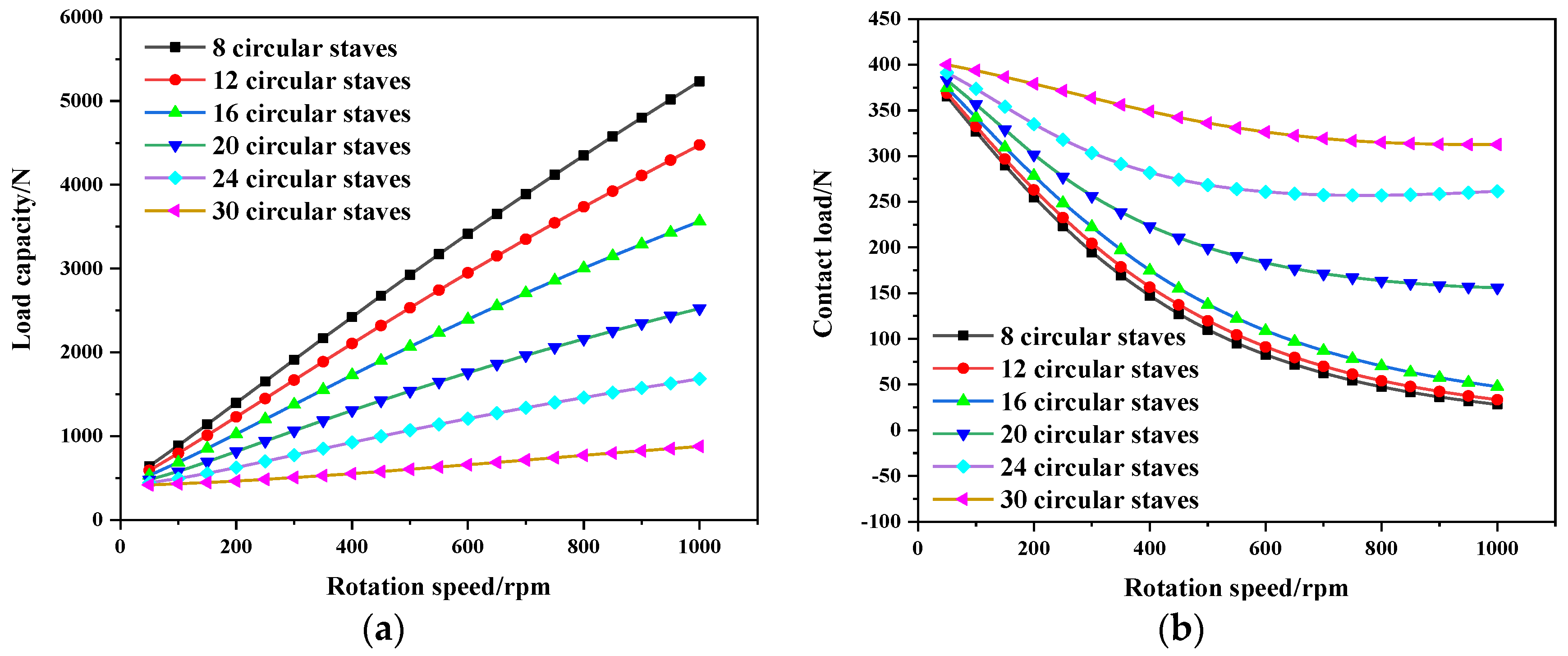
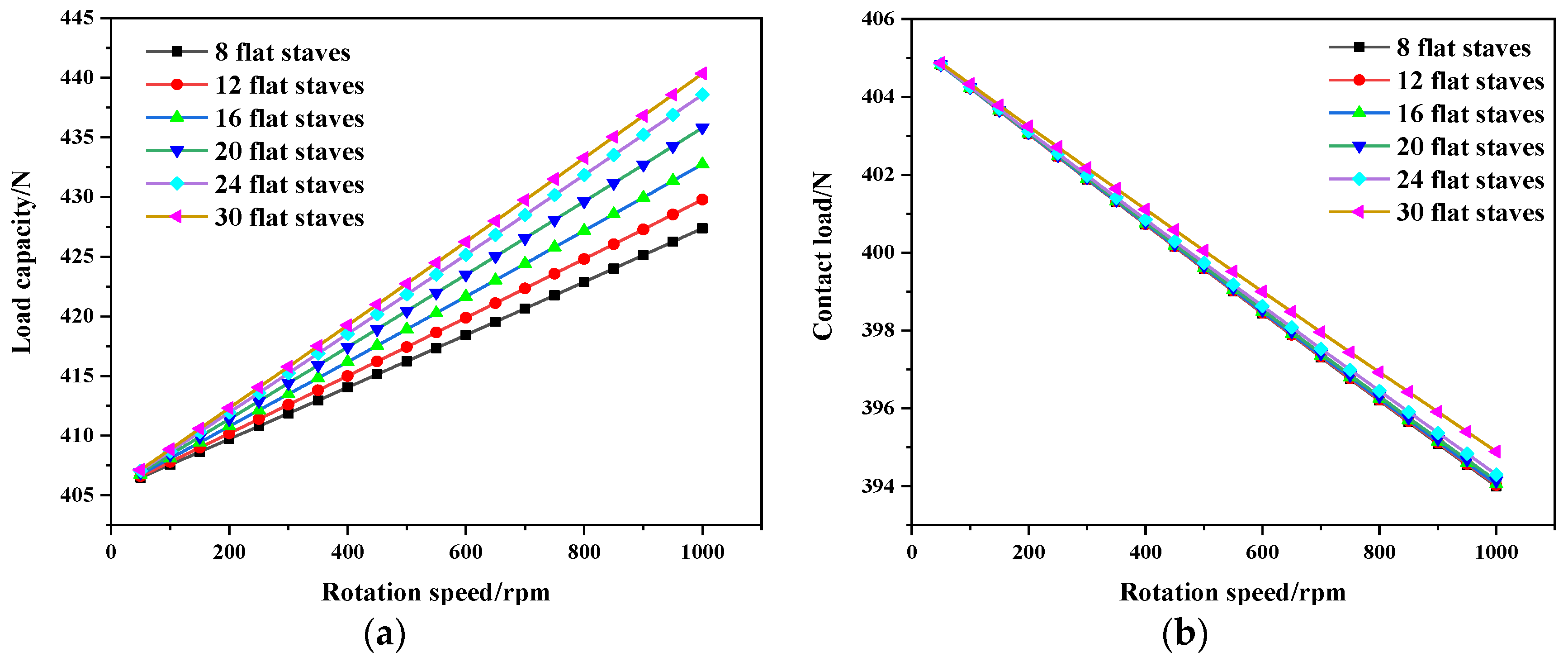
3.3. Effect of the Number of Staves on Mixed-Lubrication Performance
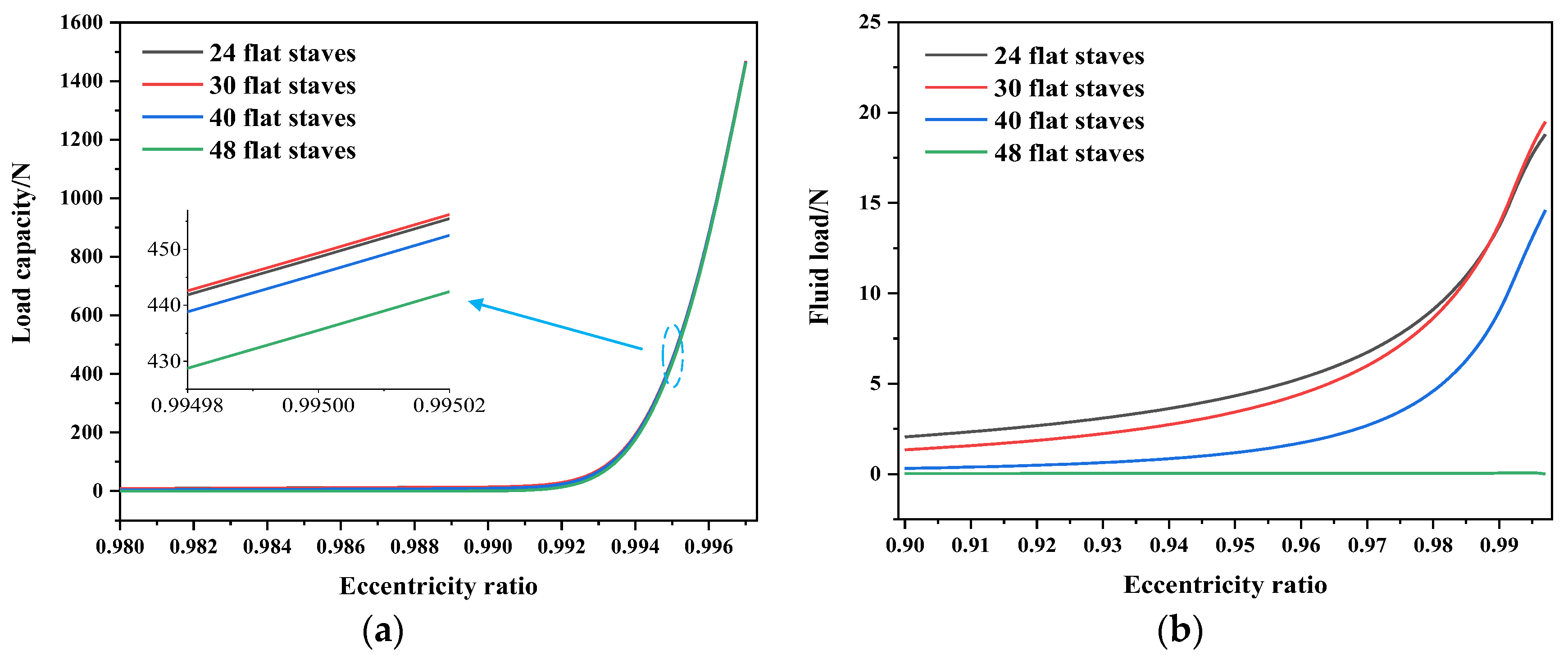
3.3.1. Under Different Eccentricity Ratios
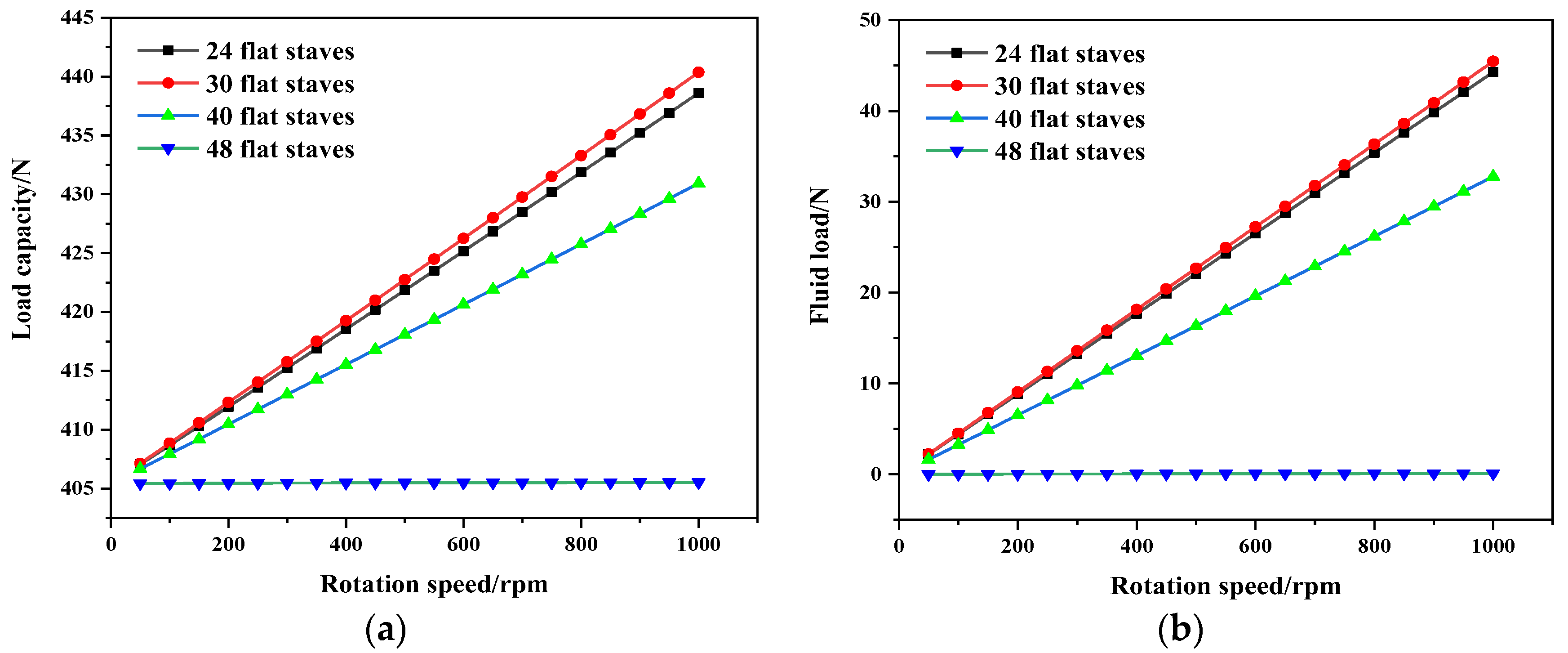
3.3.2. Under Different Rotation Speeds
3.3.3. Mechanism of Action of the Number of Staves on Lubrication Performance
3.4. Optimization of Number of Staves in Flat-Staved Bearings
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| C | bearing clearance, mm |
| ε | eccentricity ratio |
| θ | circumferential direction |
| z | axial direction |
| ψ | attitude angle, rad |
| RJ1 | inner radius of the journal bearing, mm |
| L | journal bearing width, mm |
| hJ | lubrication gap of the journal bearing, μm |
| Nst | number of staves |
| hp | geometry clearance, mm |
| δJ | journal bearing elastic deform, μm |
| phJ | hydrodynamic pressure of the Journal bearing, MPa |
| ρ | density of the water, kg/m3 |
| η | viscosity of the water, Pa·s |
| σ | composite roughness, μm |
| ϕθ | flow factor in the circumferential direction |
| ϕr | flow factor in the radial direction |
| ϕs | shear factor |
| ϕc | contact factor |
| ω | rotational speed, rad/s |
| pc | contact pressure, MPa |
| β | asperity curvature |
| D | asperity density |
| EJ | elastic modulus of the journal bearing, GPa |
| Es | elastic modulus of the shaft, GPa |
| νJ | Poisson ratio of the journal bearing |
| νs | Poisson ratio of the shaft |
| γ | surface orientation |
References
- Xiang, G.; Han, Y.; He, T.; Wang, J.; Xiao, K. A Dynamic Wear Model for Micro-Grooved Water-Lubricated Bearings Under Transient Mixed Lubrication Condition. J. Tribol. 2020, 142, 7101. [Google Scholar] [CrossRef]
- Prajapati, D.K.; Ramkumar, P. Surface topography effect on tribological performance of water-lubricated journal bearing under mixed-EHL regime. Surf. Topogr. Metrol. Prop. 2022, 10, 45022. [Google Scholar] [CrossRef]
- He, T.; Zou, D.; Lu, X.; Guo, Y.; Wang, Z.; Li, W. Mixed-lubrication analysis of marine stern tube bearing considering bending deformation of stern shaft and cavitation. Tribol. Int. 2014, 73, 108–116. [Google Scholar] [CrossRef]
- Lv, F.; Rao, Z.; Ta, N.; Jiao, C. Mixed-lubrication analysis of thin polymer film overplayed metallic marine stern bearing considering wall slip and journal misalignment. Tribol. Int. 2017, 109, 390–397. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, h.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Tribol. Trans. ASME 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Yang, H.; Zhang, Y.; Lu, L. Numerical investigation of after stern tube bearing during ship turning maneuver. J. Mar. Sci. Technol. 2019, 25, 707–717. [Google Scholar] [CrossRef]
- Huang, Q.; Yan, X. Impact factors on lubricant performance of stern bearing with misalignment angle induced by transverse vibration of shaft. Ocean Eng. 2020, 216, 108051. [Google Scholar] [CrossRef]
- Rossopoulos, G.N.; Papadopoulos, C.I.; Leontopoulos, C. Tribological comparison of an optimum single and double slope design of the stern tube bearing, case study for a marine vessel. Tribol. Int. 2020, 150, 106343. [Google Scholar] [CrossRef]
- Xie, Z.; Zhu, W. Theoretical and experimental exploration on the micro asperity contact load ratios and lubrication regimes transition for water-lubricated stern tube bearing. Tribol. Int. 2021, 164, 107105. [Google Scholar] [CrossRef]
- Liu, Q.; Ouyang, W.; Cheng, Q.; Li, J.; Cheng, Q.; Li, R. Influences of bidirectional shaft inclination on lubrication and dynamic characteristics of the water-lubricated stern bearing. Mech. Syst. Signal Process. 2021, 169, 108623. [Google Scholar] [CrossRef]
- He, T.; Xie, Z.; Ke, Z.; Dai, L.; Liu, Y.; Ma, C.; Jiao, J. Theoretical Study on the Dynamic Characteristics of Marine Stern Bearing Considering Cavitation and Bending Deformation Effects of the Shaft. Lubricants 2022, 10, 242. [Google Scholar] [CrossRef]
- Zhu, J.; Wei, G.; Peng, Z.; Xia, Z.; Zheng, L.; Zhu., h. Analysis of underwater explosion shock on ship shaft stern bearing lubrication characteristics under different bearing working conditions. Int. J. Nav. Arch. Ocean Eng. 2022, 14, 100444. [Google Scholar] [CrossRef]
- Wodtke, M.; Litwin, W. Water-lubricated stern tube bearing - experimental and theoretical investigations of thermal effects. Tribol. Int. 2020, 153, 106608. [Google Scholar] [CrossRef]
- Zhang, H.; Yuan, C.; Tan, Z. A novel approach to investigate temperature field evolution of water lubricated stern bearings (WLSBs) under hydrodynamic lubrication. Adv. Mech. Eng. 2021, 13, 1–15. [Google Scholar] [CrossRef]
- Zhou, G.; Wu, K.; Pu, W.; Li, P.; Han, Y. Tribological modification of hydrogenated nitrile rubber nanocomposites for water-lubricated bearing of ship stern shaft. Wear 2022, 504, 204432. [Google Scholar] [CrossRef]
- Han, H.S.; Lee, K.H. Experimental verification of the mechanism on stick-slip nonlinear friction induced vibration and its evaluation method in water-lubricated stern tube bearing. Ocean Eng. 2019, 182, 147–161. [Google Scholar] [CrossRef]
- Lin, C.-G.; Zou, M.-S.; Sima, C.; Liu, S.-X.; Jiang, L.-W. Friction-induced vibration and noise of marine stern tube bearings considering perturbations of the stochastic rough surface. Tribol. Int. 2018, 131, 661–671. [Google Scholar] [CrossRef]
- Hongling, Q.; Chang, Y.; Hefa, Z.; Xufei, L.; Zhixiong, L.; Xiang, X. Experimental analysis on friction-induced vibration of water-lubricated bearings in a submarine propulsion system. Ocean Eng. 2020, 203, 107239. [Google Scholar] [CrossRef]
- Jin, Y.; Liu, Z.; Zhou, X. Theoretical, numerical, and experimental studies on friction vibration of marine water-lubricated bearing coupled with lateral vibration. J. Mar. Sci. Technol. 2019, 25, 298–311. [Google Scholar] [CrossRef]
- Jin, Y.; Deng, T.; Liu, Z.; Zhou, J. Research on the influence of the normal vibration on the friction-induced vibration of the water-lubricated stern bearing. J. Vibroengineering 2020, 22, 762–772. [Google Scholar] [CrossRef]
- Lin, C.-G.; Zou, M.-S.; Zhang, H.C.; Qi, L.-B.; Liu, S.-X. Influence of different parameters on nonlinear friction-induced vibration characteristics of water lubricated stern bearings. Int. J. Nav. Arch. Ocean Eng. 2021, 13, 746–757. [Google Scholar] [CrossRef]
- Ning, C.; Hu, F.; Ouyang, W.; Yan, X.; Xu, D. Wear monitoring method of water-lubricated polymer thrust bearing based on ultrasonic reflection coefficient amplitude spectrum. Friction 2022, 11, 685–703. [Google Scholar] [CrossRef]
- Ouyang, W.; Liu, Q.; Xiao, J.; Huang, J.; Zhang, Z.; Wang, L. Experimental study on the distributed lubrication characteristics of full-size water-lubricated stern bearings under hull deformation. Ocean Eng. 2023, 267, 113226. [Google Scholar] [CrossRef]
- Cai, J.; Han, Y.; Xiang, G.; Wang, J.; Wang, L. Effects of wear and shaft-shape error defects on the tribo-dynamic response of water-lubricated bearings under propeller disturbance. Phys. Fluids 2022, 34, 077118. [Google Scholar] [CrossRef]
- Cai, J.; Han, Y.; Xiang, G.; Wang, C.; Wang, L.; Chen, S. Influence of the mass conservation cavitation boundary on the tribo-dynamic responses of the micro-groove water-lubricated bearing. Surf. Topogr. Metrol. Prop. 2022, 10, 045011. [Google Scholar] [CrossRef]
- Cai, J.; Xiang, G.; Li, S.; Guo, J.; Wang, J.; Chen, S.; Yang, T. Mathematical modeling for nonlinear dynamic mixed friction behaviors of novel coupled bearing lubricated with low-viscosity fluid. Phys. Fluids 2022, 34, 093612. [Google Scholar] [CrossRef]
- Xiang, G.; Yang, T.; Guo, J.; Wang, J.; Liu, B.; Chen, S. Optimization transient wear and contact performances of water-lubricated bearings under fluid-solid-thermal coupling condition using profile modification. Wear 2022, 502–503, 204379. [Google Scholar] [CrossRef]
- Chen, S.; Xiang, G.; Fillon, M.; Guo, J.; Wang, J.; Cai, J. On the tribo-dynamic behaviors during start-up of water lubricated bearing considering imperfect journal. Tribol. Int. 2022, 174, 107685. [Google Scholar] [CrossRef]
- Serdjuchenko, A.; Ursolov, A.; Batrak, Y. Asymptotic estimation of the fluid film pressure in non-metallic water-lubricated staved stern tube bearings. Tribol. Int. 2022, 175, 107798. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. A Finite Element Based Elastic-Plastic Model for the Contact of Rough Surfaces. Tribol. Trans. 2003, 46, 383–390. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. A Static Friction Model for Elastic-Plastic Contacting Rough Surfaces. J. Tribol. 2004, 126, 34–40. [Google Scholar] [CrossRef]
- Beheshti, A.; Khonsari, M. An engineering approach for the prediction of wear in mixed lubricated contacts. Wear 2013, 308, 121–131. [Google Scholar] [CrossRef]


| Parameters | Value |
|---|---|
| Journal bearing inner radius, RJ1 | 57.5 mm |
| Bearing width, L | 230 mm |
| Radius clearance, C | 0.5 mm |
| Journal bearing elastic modulus, EJ | 2.32 GPa |
| Journal bearing Poisson ratio, νJ | 0.327 |
| Composite roughness of journal and bearing, σs | 1.2 μm |
| Water viscosity, η | 8.49 × 10−4 Pa·s |
| Water density, ρ | 1000 kg/m3 |
| Shaft elastic modulus, Es | 210 GPa |
| Shaft Poisson ratio, νs | 0.3 |
| Surface orientation, γ | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, D.; Han, Y.; Yin, L.; Chen, Y. Numerical Analysis of the Mixed-Lubrication Performance of Staved Stern Tube Bearings Lubricated with Water. Lubricants 2023, 11, 168. https://doi.org/10.3390/lubricants11040168
Tang D, Han Y, Yin L, Chen Y. Numerical Analysis of the Mixed-Lubrication Performance of Staved Stern Tube Bearings Lubricated with Water. Lubricants. 2023; 11(4):168. https://doi.org/10.3390/lubricants11040168
Chicago/Turabian StyleTang, Dongxing, Yanfeng Han, Lei Yin, and Yi Chen. 2023. "Numerical Analysis of the Mixed-Lubrication Performance of Staved Stern Tube Bearings Lubricated with Water" Lubricants 11, no. 4: 168. https://doi.org/10.3390/lubricants11040168
APA StyleTang, D., Han, Y., Yin, L., & Chen, Y. (2023). Numerical Analysis of the Mixed-Lubrication Performance of Staved Stern Tube Bearings Lubricated with Water. Lubricants, 11(4), 168. https://doi.org/10.3390/lubricants11040168






