Explosion Mechanism of Lubricating Oil Droplets in High-Temperature and High-Pressure Combustion Environments
Abstract
:1. Introduction
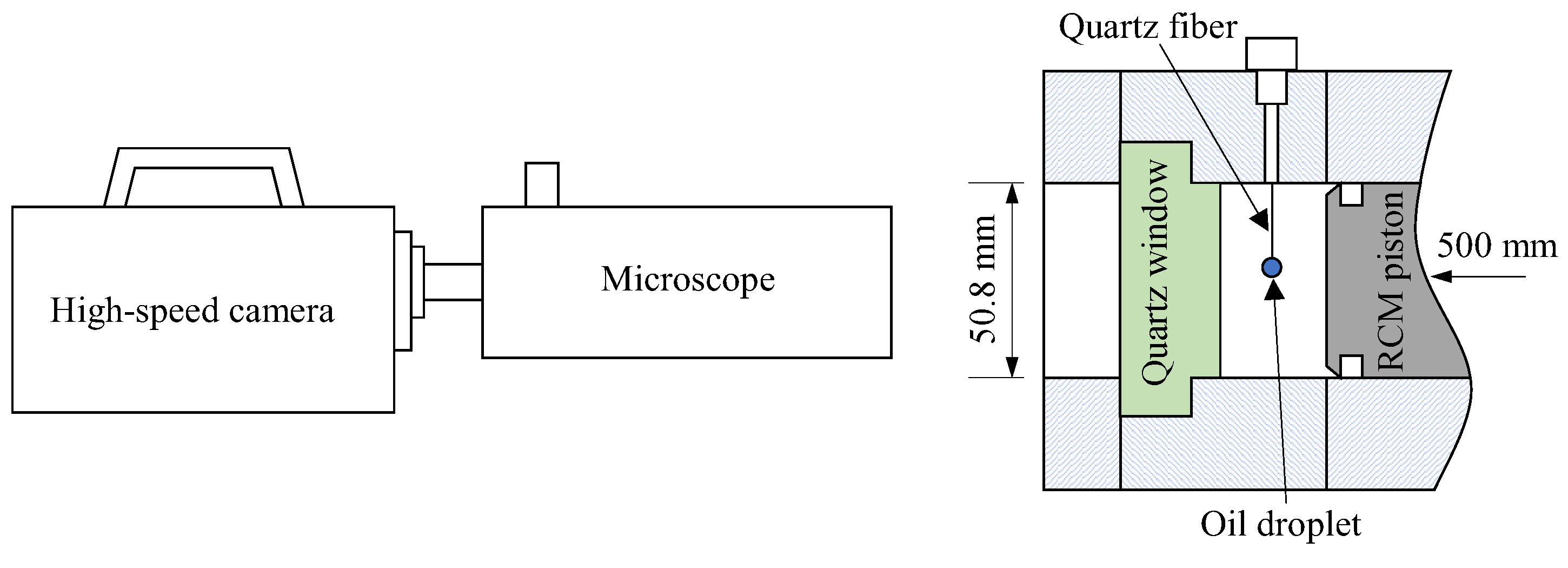
2. Experimental Setup
2.1. Experimental Apparatus
2.2. Data Processing
2.3. Properties of Oil and Ambient Gas
3. Results and Discussion
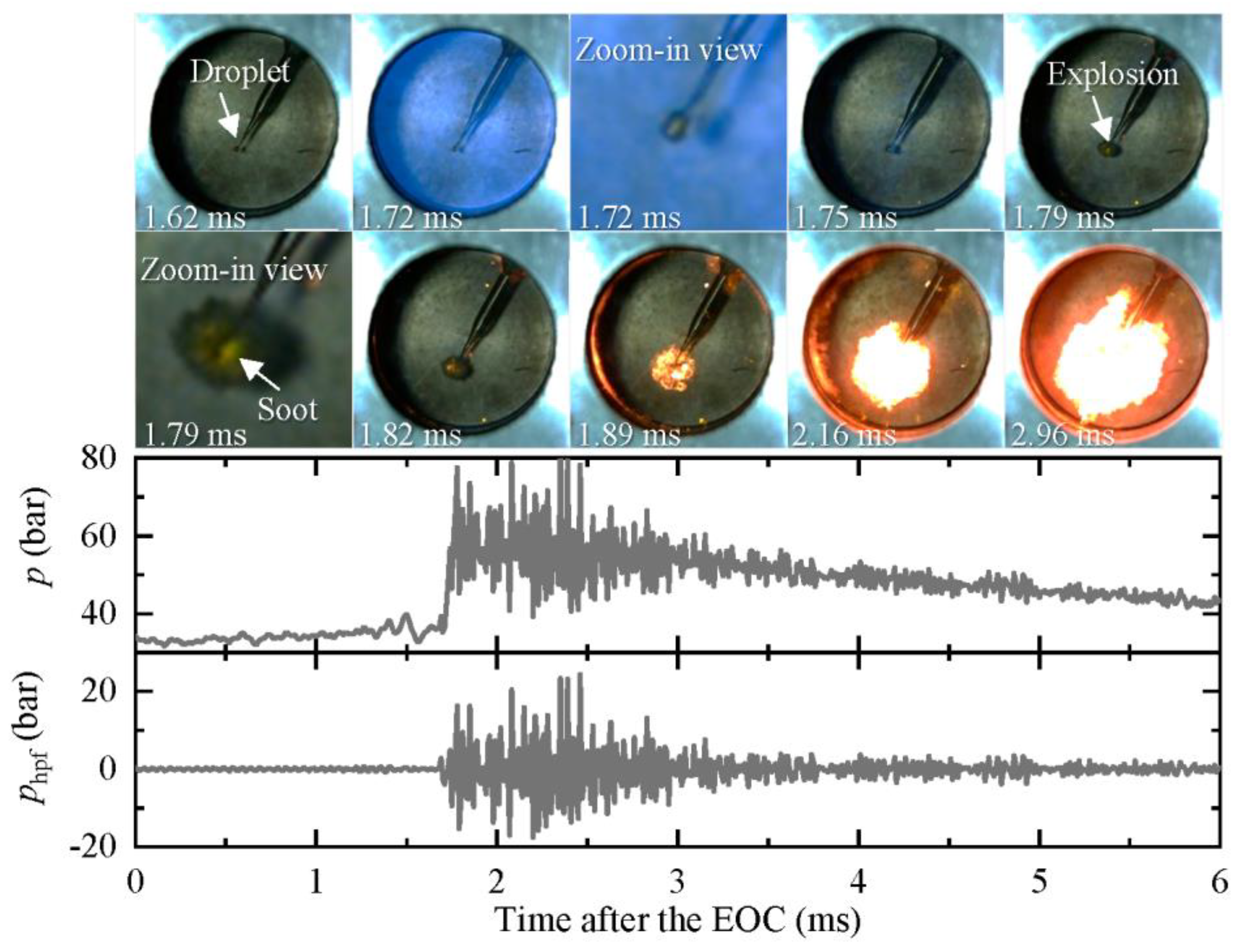
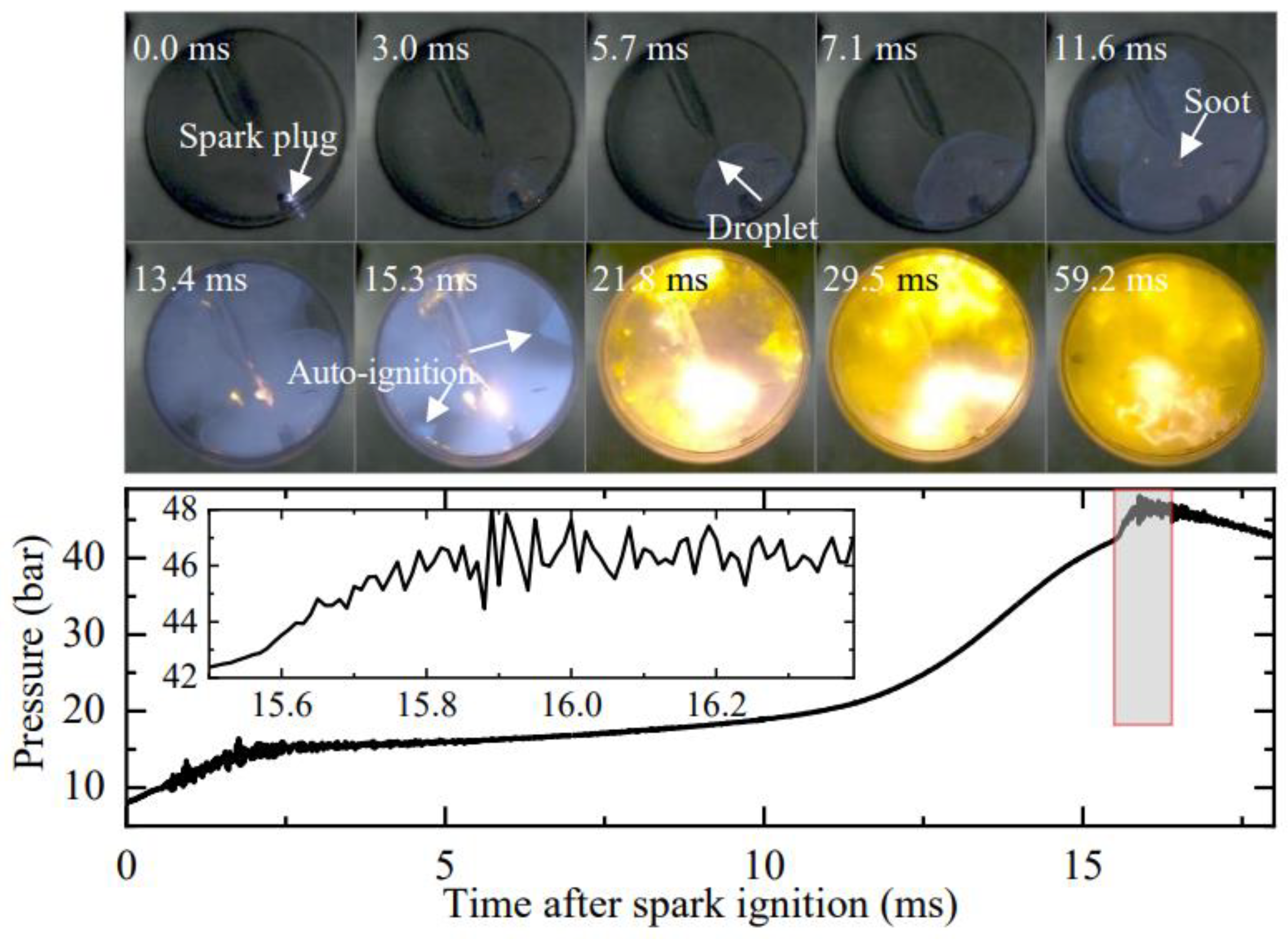
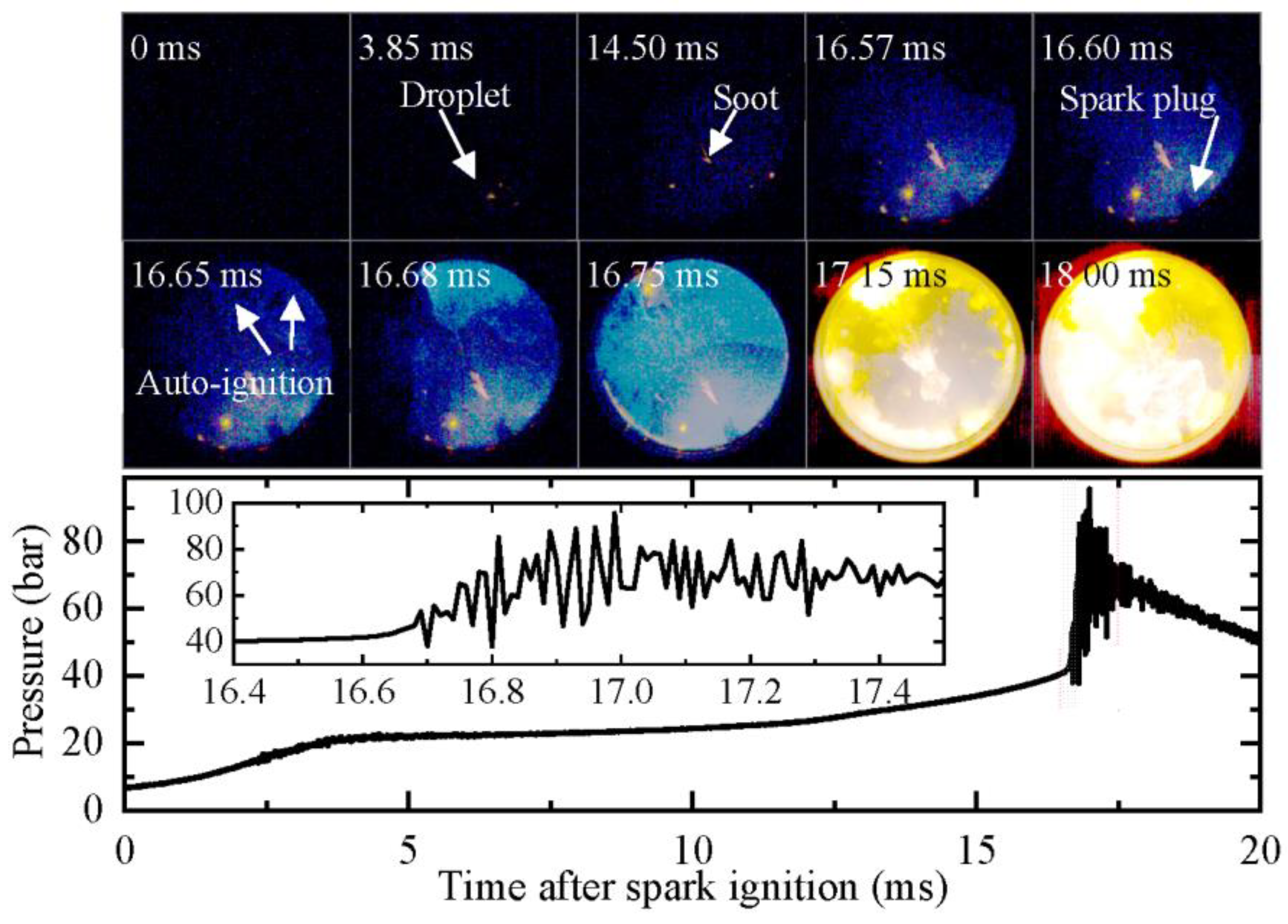
3.1. Ignition and Explosion of Lubricating Oil Droplets
3.2. Microphotography of Oil Droplet Explosion
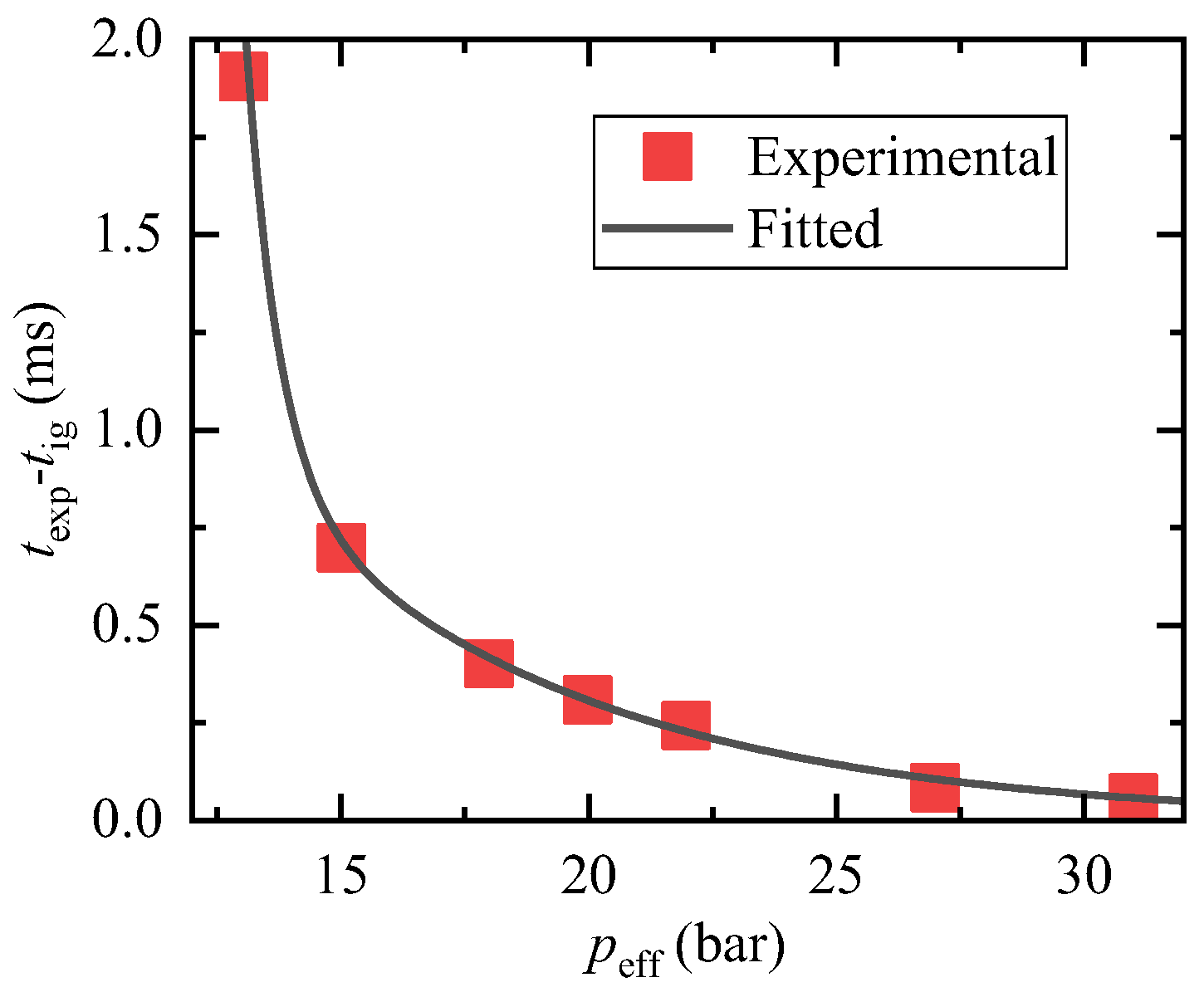
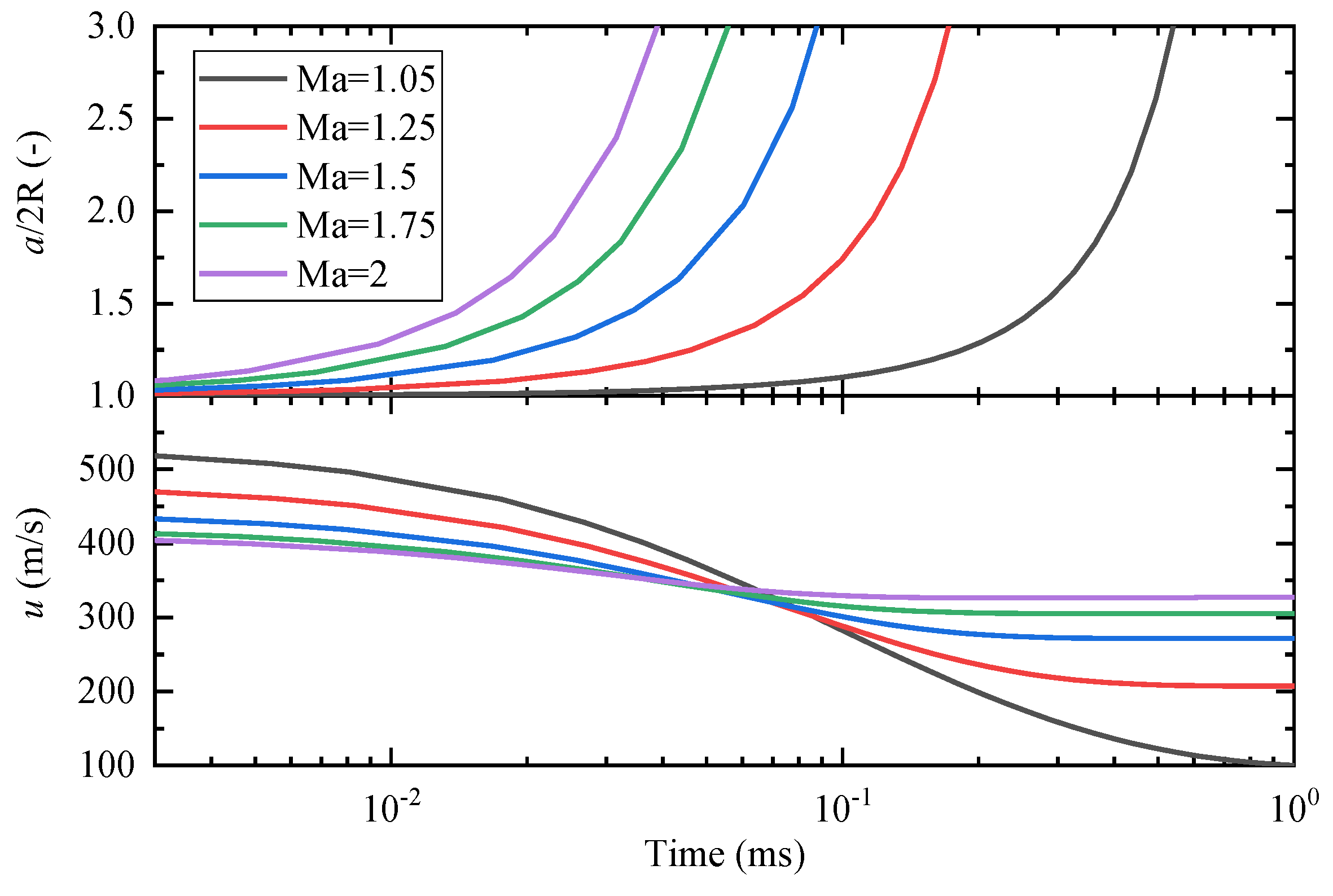
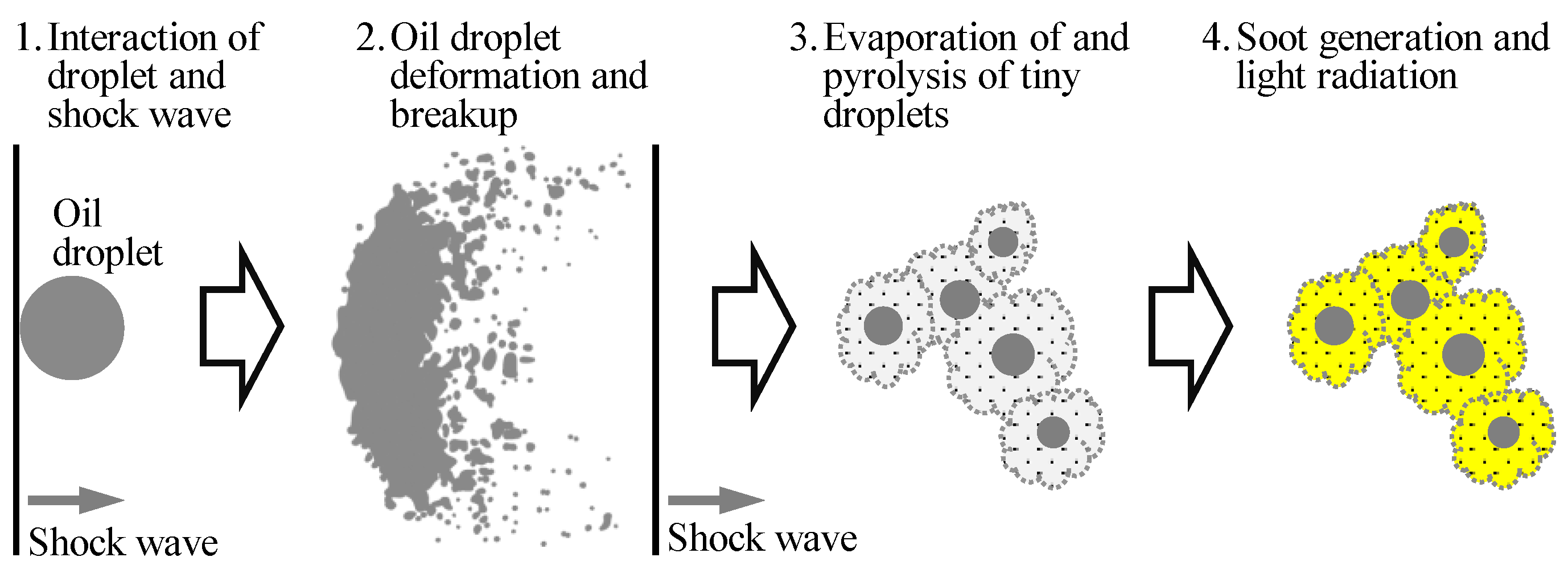
3.3. Mechanism of Oil Droplet Explosion
4. Conclusions
- Lubricating oil droplets can explode and develop into a soot cloud in HCCI-like combustion environments with a short ignition delay time. As the pressure of the ambient flammable gas increases, the oil droplet explodes more severely and earlier;
- The key factor in inducing oil droplet explosions is the shock waves generated by the combustion of the ambient gas. In cases of high temperature alone, without shock waves, the oil droplet cannot explode during the entire combustion process, even if surrounded by a flame;
- The mechanism of oil droplet explosion is as follows: the impact of shock waves on the droplet deforms and breaks up the oil droplet into many tiny droplets. The evaporation and pyrolysis of these tiny droplets in a high-temperature and high-pressure environment lacking oxygen generate a glowing soot cloud. The expansion of the soot cloud with oscillating shock waves and gas flow in the combustion chamber creates an explosion-like appearance.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Z.; Liu, H.; Reitz, R.D. Knocking combustion in spark-ignition engines. Prog. Energy Combust. Sci. 2017, 61, 78–112. [Google Scholar] [CrossRef]
- Rönn, K.; Swarts, A.; Kalaskar, V.; Alger, T.; Tripathi, R.; Keskiväli, J.; Kaario, O.; Santasalo-Aarnio, A.; Reitz, R.; Larmi, M. Low-speed pre-ignition and super-knock in boosted spark-ignition engines: A review. Prog. Energy Combust. Sci. 2023, 95, 101064. [Google Scholar] [CrossRef]
- Gong, Z.; Hu, M.; Fang, Y.; Zhang, D.; Feng, L. Mechanism study of natural gas pre-ignition induced by the auto-ignition of lubricating oil. Fuel 2022, 315, 123286. [Google Scholar] [CrossRef]
- Dahnz, C.; Han, K.-M.; Spicher, U.; Magar, M.; Schiessl, R.; Maas, U. Investigations on Pre-Ignition in Highly Supercharged SI Engines. SAE Int. J. Engines 2010, 3, 214–224. [Google Scholar] [CrossRef]
- Fujimoto, K.; Yamashita, M.; Hirano, S.; Kato, K.; Watanabe, I.; Ito, K. Engine Oil Development for Preventing Pre-Ignition in Turbocharged Gasoline Engine. SAE Int. J. Fuels Lubr. 2014, 7, 869–874. [Google Scholar] [CrossRef]
- Mayer, M.; Hofmann, P.; Geringer, B.; Williams, J.; Moss, J. Influence of Different Fuel Properties and Gasoline–Ethanol Blends on Low-Speed Pre-Ignition in Turbocharged Direct Injection Spark Ignition Engines. SAE Int. J. Engines 2016, 9, 841–848. [Google Scholar] [CrossRef]
- Kassai, M.; Torii, K.; Shiraishi, T.; Noda, T.; Goh, T.K.; Wilbrand, K.; Wakefield, S.; Healy, A.; Doyle, D.; Cracknell, R.; et al. Research on the Effect of Lubricant Oil and Fuel Properties on LSPI Occurrence in Boosted S. I. Engines; SAE Technical Paper: Warrendale, PA, USA, 2016; p. 2016-01-2292. [Google Scholar] [CrossRef]
- Han, K.; Pang, B.; Ma, X.; Chen, H.; Song, G.; Ni, Z. An experimental study of the burning characteristics of acetone–butanol–ethanol and diesel blend droplets. Energy 2017, 139, 853–861. [Google Scholar] [CrossRef]
- Wu, M.S.; Yang, S.I. Combustion characteristics of multi-component cedar bio-oil/kerosene droplet. Energy 2016, 113, 788–795. [Google Scholar] [CrossRef]
- Ambekar, A.; Maurya, A.K.; Chowdhury, A. Droplet combustion studies of nitromethane and its blends. Exp. Therm. Fluid Sci. 2018, 93, 431–440. [Google Scholar] [CrossRef]
- Glushkov, D.O.; Strizhak, P.A. Ignition of composite liquid fuel droplets based on coal and oil processing waste by heated air flow. J. Clean. Prod. 2017, 165, 1445–1461. [Google Scholar] [CrossRef]
- Kim, H.; Baek, S.W. Combustion of a single emulsion fuel droplet in a rapid compression machine. Energy 2016, 106, 422–430. [Google Scholar] [CrossRef]
- Ohtomo, M.; Miyagawa, H.; Koike, M.; Yokoo, N.; Nakata, K. Pre-Ignition of Gasoline-Air Mixture Triggered by a Lubricant Oil Droplet. SAE Int. J. Fuels Lubr. 2014, 7, 673–682. [Google Scholar] [CrossRef]
- Ohtomo, M.; Suzuoki, T.; Miyagawa, H.; Koike, M.; Yokoo, N.; Nakata, K. Fundamental analysis on auto-ignition condition of a lubricant oil droplet for understanding a mechanism of low-speed pre-ignition in highly charged spark-ignition engines. Int. J. Engine Res. 2018, 20, 292–303. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, D.; Fang, Y.; Song, M.; Gong, Z.; Feng, L. Experimental and numerical investigation of the auto-ignition characteristics of cylinder oil droplets under low-speed two-stroke natural gas engines in-cylinder conditions. Fuel 2022, 329, 125498. [Google Scholar] [CrossRef]
- Niegemann, P.; Herzler, J.; Fikri, M.; Schulz, C. Studying the influence of single droplets on fuel/air ignition in a high-pressure shock tube. Rev. Sci. Instrum. 2020, 91, 105107. [Google Scholar] [CrossRef] [PubMed]
- Yi, P.; Long, W.; Feng, L.; Wang, W.; Liu, C. An experimental and numerical study of the evaporation and pyrolysis characteristics of lubricating oil droplets in the natural gas engine conditions. Int. J. Heat Mass Transf. 2016, 103, 646–660. [Google Scholar] [CrossRef]
- Fei, S.; Wang, Z.; Qi, Y.; Wang, Y.; Zhang, H. Ignition of a Single Lubricating Oil Droplet in Combustible Ambient Gaseous Mixture under High-Temperature and High-Pressure Conditions. Combust. Sci. Technol. 2019, 191, 2033–2052. [Google Scholar] [CrossRef]
- He, X.; Donovan, M.T.; Zigler, B.T.; Palmer, T.R.; Walton, S.M.; Wooldridge, M.S.; Atreya, A. An experimental and modeling study of iso-octane ignition delay times under homogeneous charge compression ignition conditions. Combust. Flame 2005, 142, 266–275. [Google Scholar] [CrossRef]
- Kuti, O.A.; Yang, S.Y.; Hourani, N.; Naser, N.; Roberts, W.L.; Chung, S.H.; Sarathy, S.M. A fundamental investigation into the relationship between lubricant composition and fuel ignition quality. Fuel 2015, 160, 605–613. [Google Scholar] [CrossRef]
- Poplavski, S.V.; Minakov, A.V.; Shebeleva, A.A.; Boyko, V.M. On the interaction of water droplet with a shock wave: Experiment and numerical simulation. Int. J. Multiph. Flow 2020, 127, 103273. [Google Scholar] [CrossRef]
- Antonov, D.V.; Fedorenko, R.M.; Strizhak, P.A. Micro-Explosion Phenomenon: Conditions and Benefits. Energies 2022, 15, 7670. [Google Scholar] [CrossRef]
- Zhang, X.; Li, T.; Wang, B.; Wei, Y. Superheat limit and micro-explosion in droplets of hydrous ethanol-diesel emulsions at atmospheric pressure and diesel-like conditions. Energy 2018, 154, 535–543. [Google Scholar] [CrossRef]
- Sharma, S.; Chandra, N.K.; Basu, S.; Kumar, A. Advances in droplet aerobreakup. Eur. Phys. J. Spec. Top. 2022, 2022, 1–15. [Google Scholar] [CrossRef]
- Nicholls, J.A.; Ranger, A.A. Aerodynamic shattering of liquid drops. AIAA J. 1969, 7, 285–290. [Google Scholar] [CrossRef]
- Sharma, S.; Pratap Singh, A.; Srinivas Rao, S.; Kumar, A.; Basu, S. Shock induced aerobreakup of a droplet. J. Fluid Mech. 2021, 929, A27. [Google Scholar] [CrossRef]
- Lu, S.; Qin, Y. Deformation and breakup of droplets behind shock wave. Chin. J. High Press. Phys. 2000, 14, 151–154. [Google Scholar]
- Frolov, S.M. Modeling of Droplet Dformation and Breakup Conditions. In Proceedings of the 16th International Colloquium on the Dynamics of Explosions and Reactive Systems, Cracow, Poland, 3–8 August 1997; pp. 417–420.sa. [Google Scholar]
- Anderson, J. Fundamentals of Aerodynamics, 6th ed.; McGraw-Hill Education: New York, NY, USA, 2017. [Google Scholar]


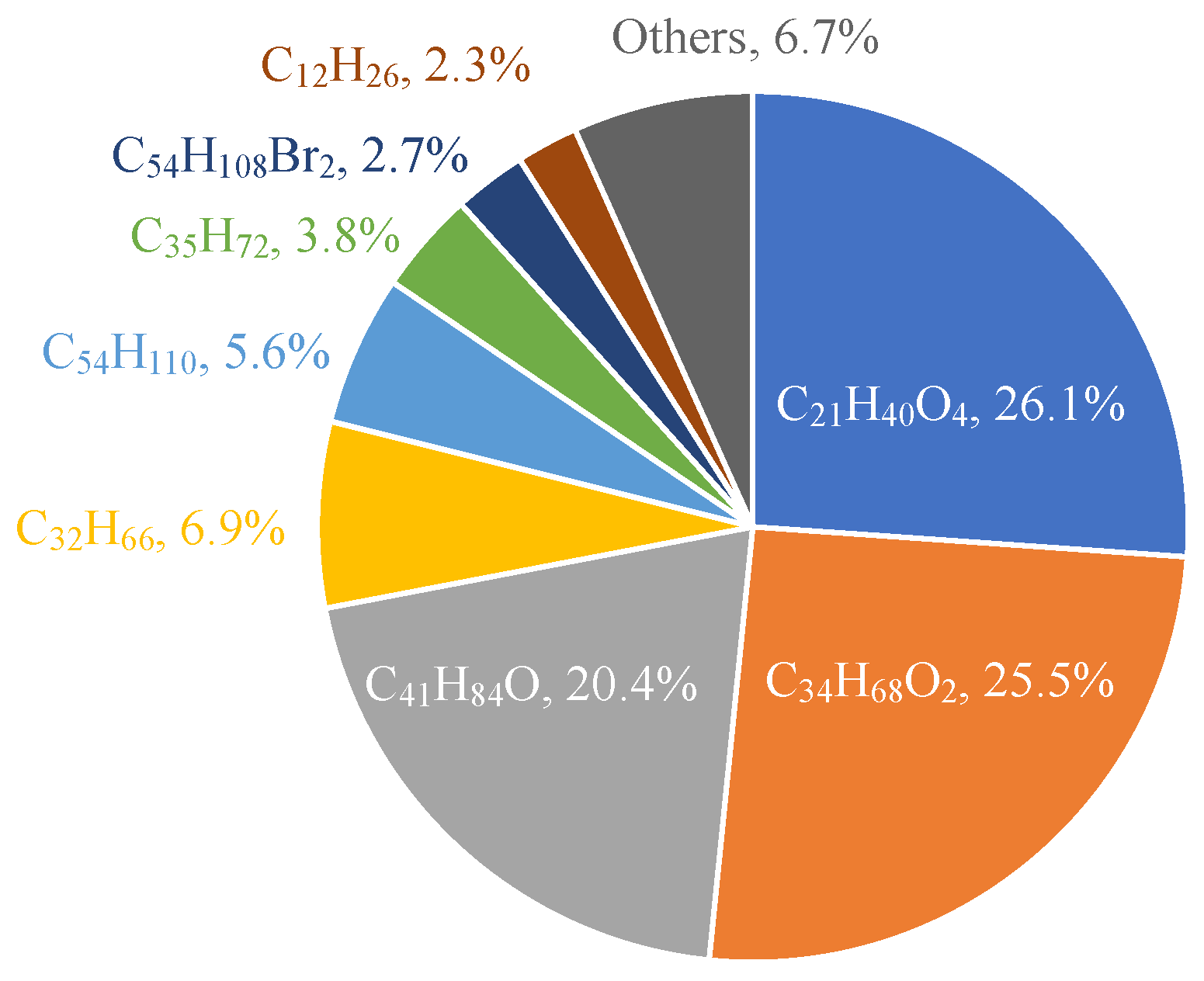




| Case | Factor | Method | peff/bar | Teff/K | Droplet Vol./μL |
|---|---|---|---|---|---|
| 1 | High temperature | Pure Ar compression | 23.0 | 1659 | 1.0 |
| 2 | High temperature | Low-pressure SI | 10.8 | 701 | 1.0 |
| 3 | Pressure wave | Medium-pressure SI | 15.7 | 703 | 1.0 |
| 4 | Pressure wave | High-pressure SI | 22.9 | 709 | 1.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, Y.; Fei, S.; Wang, Z. Explosion Mechanism of Lubricating Oil Droplets in High-Temperature and High-Pressure Combustion Environments. Lubricants 2023, 11, 118. https://doi.org/10.3390/lubricants11030118
Qi Y, Fei S, Wang Z. Explosion Mechanism of Lubricating Oil Droplets in High-Temperature and High-Pressure Combustion Environments. Lubricants. 2023; 11(3):118. https://doi.org/10.3390/lubricants11030118
Chicago/Turabian StyleQi, Yunliang, Shubo Fei, and Zhi Wang. 2023. "Explosion Mechanism of Lubricating Oil Droplets in High-Temperature and High-Pressure Combustion Environments" Lubricants 11, no. 3: 118. https://doi.org/10.3390/lubricants11030118
APA StyleQi, Y., Fei, S., & Wang, Z. (2023). Explosion Mechanism of Lubricating Oil Droplets in High-Temperature and High-Pressure Combustion Environments. Lubricants, 11(3), 118. https://doi.org/10.3390/lubricants11030118





