Impact of Gas Foil Bearings, Labyrinth Seals, and Impellers on the Critical Speed of Centrifugal Compressors for Fuel Cell Vehicles: A Comprehensive Investigation
Abstract
1. Introduction
2. Mathematical Model
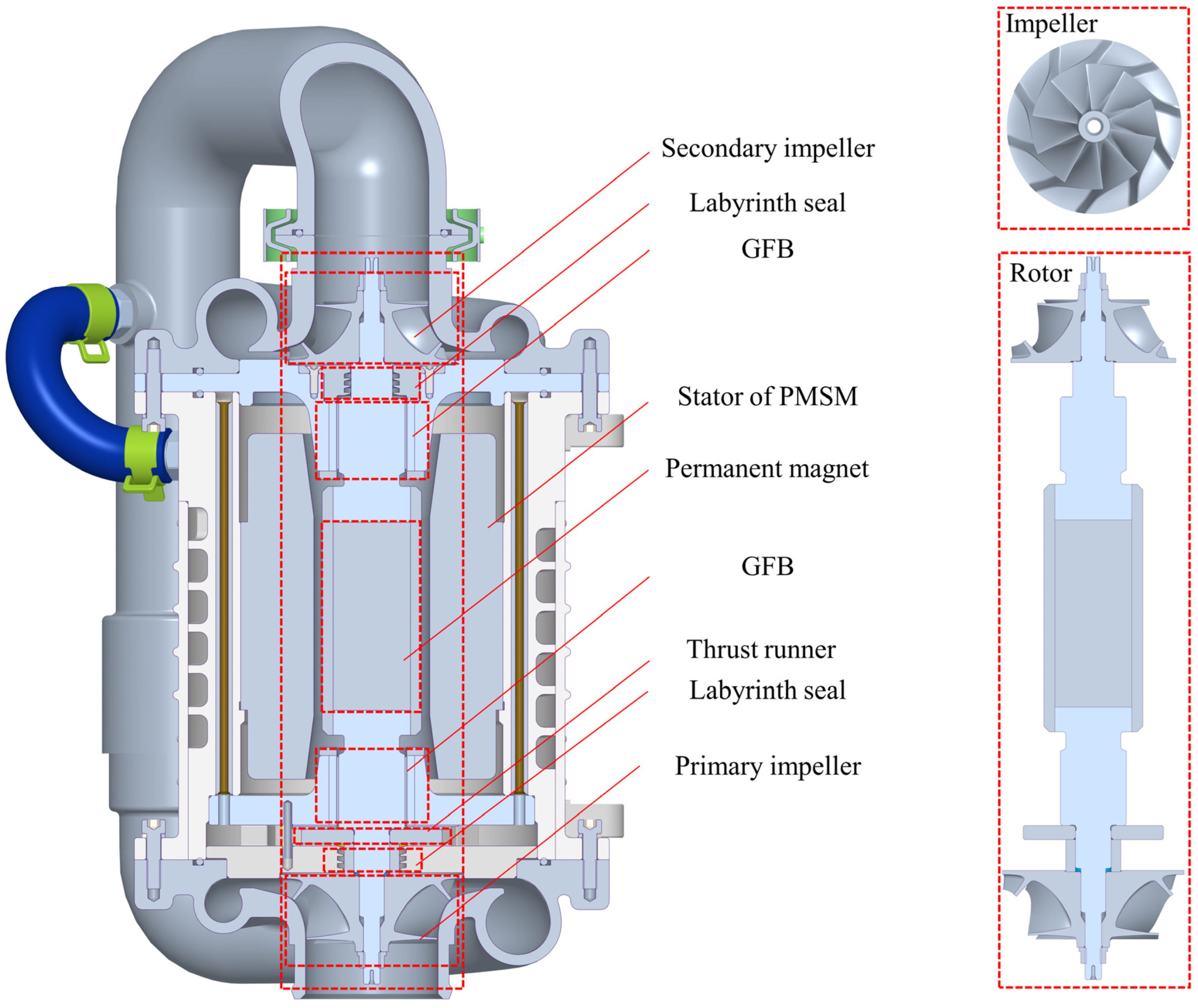
2.1. Rotordynamic Model
2.2. Rotordynamic Coefficient Model
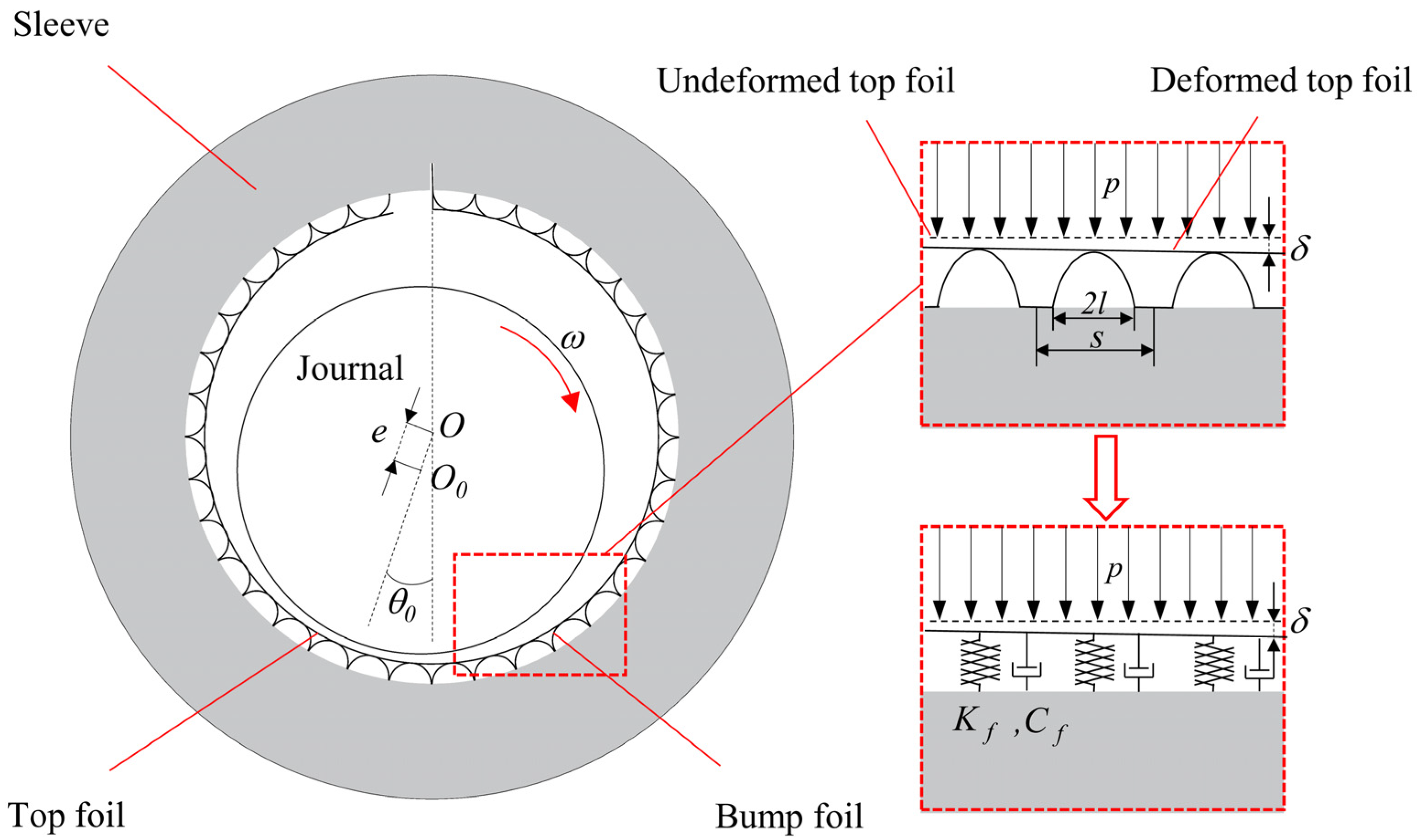
2.2.1. GFB Model
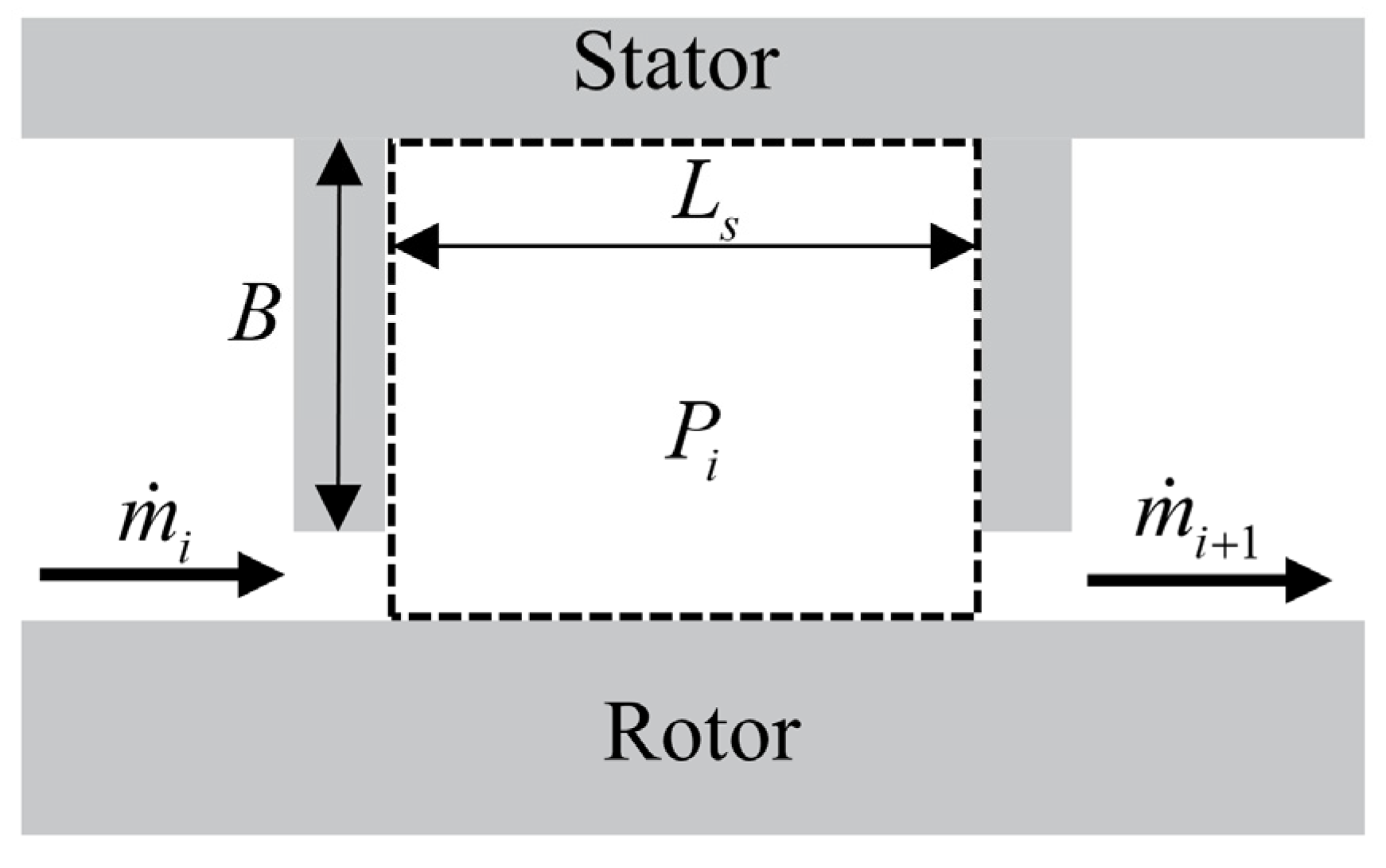
2.2.2. Labyrinth Seal Model
2.2.3. Centrifugal Impeller Model
3. Model Validation
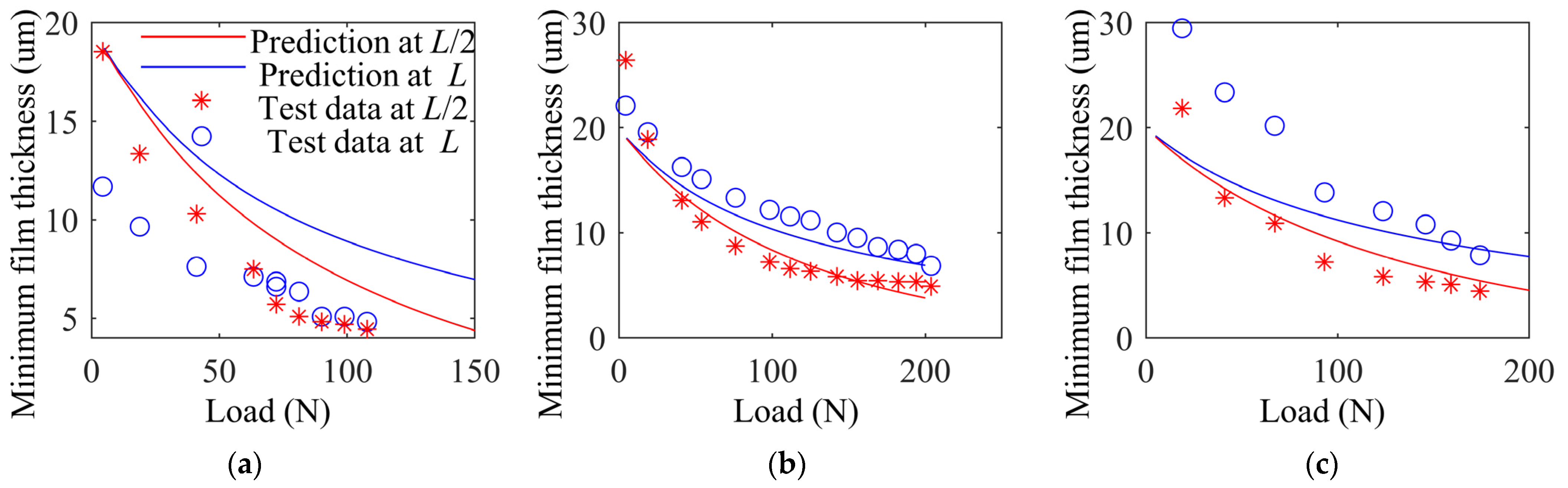
3.1. Validation of GFB Gas–Elastic Coupling Model
3.2. Validation of Critical Speed Calculation Model
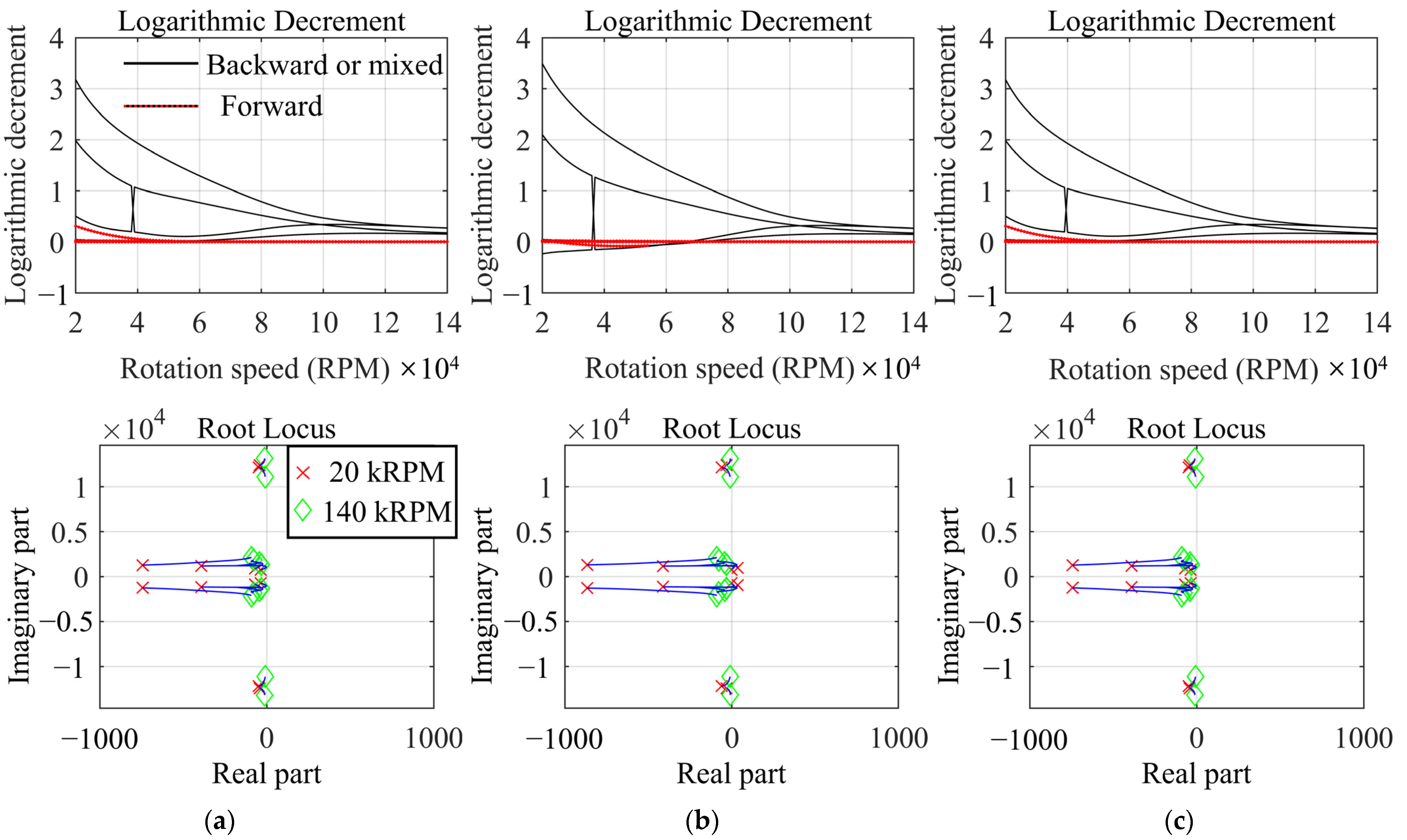
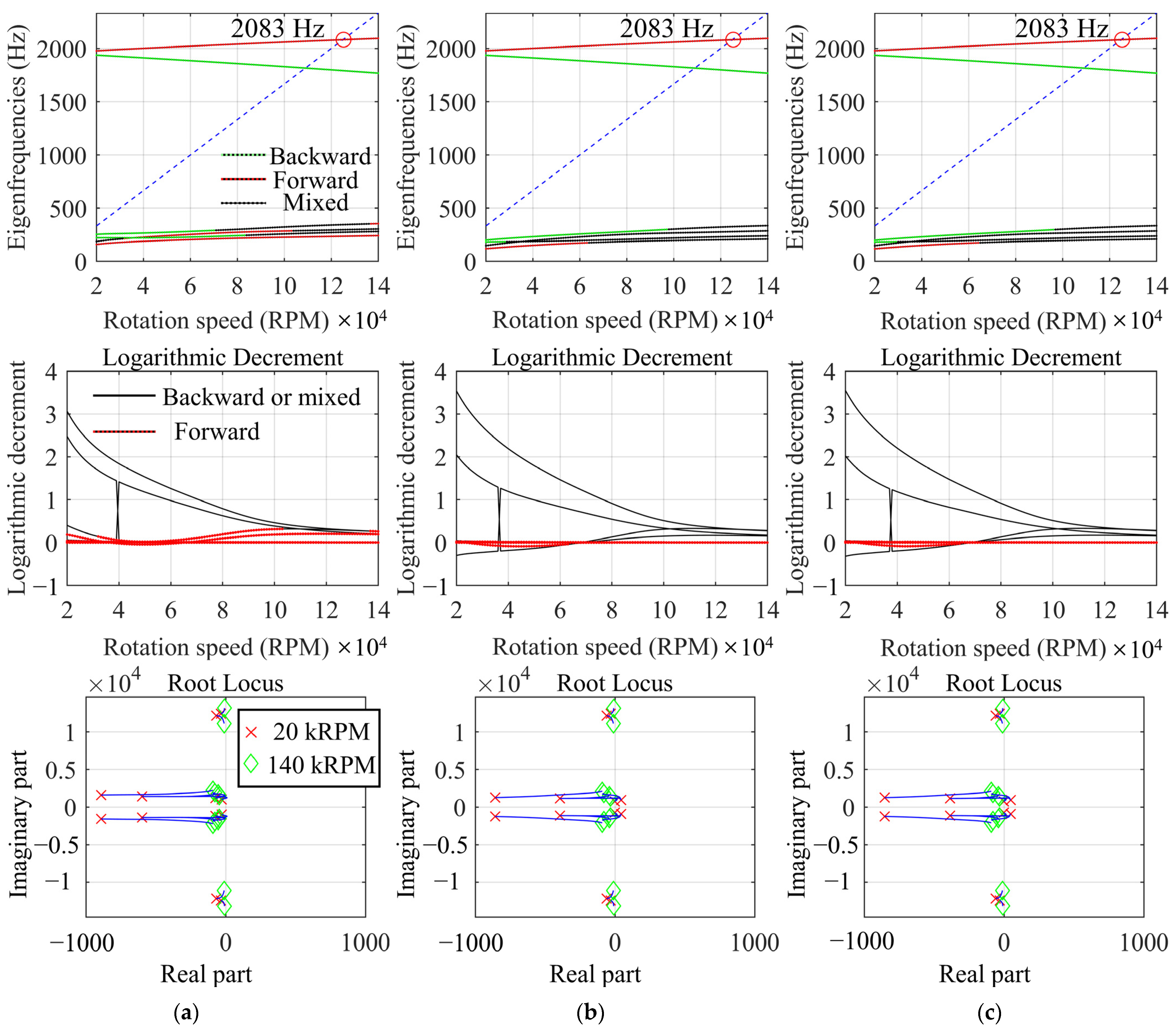
4. Results and Discussion
5. Conclusions
- 1
- A centrifugal compressor for fuel cell vehicles can be rationally designed to operate between speeds higher than the rigid mode and lower than the bending mode and to suppress the eigenfrequencies of the unstable rigid mode to less than half of the lift-off speed.
- 2
- The instability of the low frequencies’ rigid mode comes from the cross-coupling stiffness of the impellers, and reducing the bump foil structure stiffness can suppress the instability to some extent.
- 3
- The aerodynamic effects of the impellers and seals can be ignored when calculating the critical speed (or eigenfrequencies), and the GFBs can be reduced to a rigid gas bearing to reduce arithmetic costs.
- 4
- If the rigid mode frequencies obtained by simplified calculations in the manner described in Conclusion 3 are less than half of the lift-off speed, no stability judgment is required, and the compressor can be considered to operate stably between the lift-off speed and the bending critical speed.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, D.; Yang, L.; Yi, F.; Hu, L.; Yang, Q.; Zhou, J. Optimization of speed response of super-high-speed electric air compressor for hydrogen fuel cell vehicle considering the transient current. Int. J. Hydrog. Energy 2021, 46, 27183–27192. [Google Scholar] [CrossRef]
- Kim, J.-H.; Kim, D.-M.; Jung, Y.-H.; Lim, M.-S. Design of Ultra-High-Speed Motor for FCEV Air Compressor Considering Mechanical Properties of Rotor Materials. IEEE Trans. Energy Convers. 2021, 36, 2850–2860. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, Y.; Liu, G.; Yang, Q.; Li, L.; Gao, Z. Air and hydrogen supply systems and equipment for PEM fuel cells: A review. Int. J. Green Energy 2022, 19, 331–348. [Google Scholar] [CrossRef]
- Chen, S.; Zuo, S.; Wu, Z.; Liu, C. Comprehensive vibro-acoustic characteristics and mathematical modeling of electric high-speed centrifugal compressor surge for fuel cell vehicles at various compressor speeds. Mech. Syst. Signal Process. 2022, 178, 109311. [Google Scholar] [CrossRef]
- Shi, T.; Chen, Q.; Peng, X.; Feng, J.; Guo, Y. Multi-objective optimization of the oil-free centrifugal air compressor in hydrogen fuel cell vehicles based on grey relational analysis. Int. J. Hydrog. Energy 2023, 48, 26341–26355. [Google Scholar] [CrossRef]
- Ying, M.; Liu, X.; Zhang, C.; Wang, X.; Liu, Y.; Zhang, Y. The Two-Pad: A Novel Gas Foil Bearing for Fuel Cell Vehicles. Int. J. Energy Res. 2023, 2023, 5521171. [Google Scholar] [CrossRef]
- Friswell, M.I.; Penny, J.E.T.; Garvey, S.D.; Lees, A.W. Dynamics of Rotating Machines; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar] [CrossRef]
- Lund, J.W. Calculation of Stiffness and Damping Properties of Gas Bearings. J. Lubr. Technol. 1968, 90, 793–803. [Google Scholar] [CrossRef]
- Peng, J.-P.; Carpino, M. Calculation of Stiffness and Damping Coefficients for Elastically Supported Gas Foil Bearings. J. Tribol. 1993, 115, 20–27. [Google Scholar] [CrossRef]
- Peng, J.-P.; Carpino, M. Coulomb friction damping effects in elastically supported gas foil bearings. Tribol. Trans. 1994, 37, 91–98. [Google Scholar] [CrossRef]
- Carpino, M.; Talmage, G. Prediction of Rotor Dynamic Coefficients in Gas Lubricated Foil Journal Bearings with Corrugated Sub-Foils. Tribol. Trans. 2006, 49, 400–409. [Google Scholar] [CrossRef]
- Lee, N.S.; Choi, D.H.; Lee, Y.B.; Kim, T.H.; Kim, C.H. The influence of the slip flow on steady-state load capacity, stiffness and damping coefficients of elastically supported gas foil bearings. Tribol. Trans. 2002, 45, 478–484. [Google Scholar] [CrossRef]
- Kim, D. Parametric Studies on Static and Dynamic Performance of Air Foil Bearings with Different Top Foil Geometries and Bump Stiffness Distributions. J. Tribol. 2007, 129, 354–364. [Google Scholar] [CrossRef]
- Kim, T.; Andres, L. Heavily loaded gas foil bearings: A model anchored to test data. J. Eng. Gas Turbines Power-Trans. Asme 2008, 130. [Google Scholar] [CrossRef]
- Kim, T.; San Andres, L. Limits for high-speed operation of gas foil bearings. J. Tribol. Trans. Asme 2006, 128, 670–673. [Google Scholar] [CrossRef]
- Kim, T.H.; Andrés, L.S. Analysis of advanced gas foil bearings with piecewise linear elastic supports. Tribol. Int. 2007, 40, 1239–1245. [Google Scholar] [CrossRef]
- Larsen, J.S.; Hansen, A.J.; Santos, I.F. Experimental and theoretical analysis of a rigid rotor supported by air foil bearings. Mech. Ind. 2015, 16, 106. [Google Scholar] [CrossRef]
- von Osmanski, S.; Larsen, J.S.; Santos, I.F. The classical linearization technique’s validity for compliant bearings. In Mechanisms and Machine Science, Proceedings of the 10th International Conference on Rotor Dynamics–IFToMM, Rio de Janeiro, Brazil, 23–27 September 2018; Cavalca, K., Weber, H., Eds.; Springer: Cham, Switzerland, 2019; Volume 1, pp. 177–191. [Google Scholar]
- Bonello, P.; Pham, H.M. Nonlinear dynamic analysis of high speed oil-free turbomachinery with focus on stability and self-excited vibration. J. Tribol. 2014, 136, 41705. [Google Scholar] [CrossRef]
- Larsen, J.S.; Santos, I.F. On the nonlinear steady-state response of rigid rotors supported by air foil bearings-theory and experiments. J. Sound Vib. 2015, 346, 284–297. [Google Scholar] [CrossRef]
- Papafragkos, P.; Gavalas, I.; Raptopoulos, I.; Chasalevris, A. Optimizing energy dissipation in gas foil bearings to eliminate bifurcations of limit cycles in unbalanced rotor systems. Nonlinear Dyn. 2023, 111, 67–95. [Google Scholar] [CrossRef]
- Hoffmann, R.; Pronobis, T.; Liebich, R. Non-linear stability analysis of a modified gas foil bearing structure. In Mechanisms and Machine Science, Proceedings of the 9th IFToMM International Conference on Rotor Dynamics, Milan, Italy, 29 August–5 September 2015; Pennacchi, P., Ed.; Springer: Cham, Switzerland, 2015; pp. 1259–1276. [Google Scholar]
- Larsen, J.S.; Santos, I.F.; von Osmanski, S. Stability of rigid rotors supported by air foil bearings: Comparison of two fundamental approaches. J. Sound Vib. 2016, 381, 179–191. [Google Scholar] [CrossRef]
- Von Osmanski, S.; Larsen, J.S.; Santos, I.F. Multi-domain stability and modal analysis applied to Gas Foil Bearings: Three approaches. J. Sound Vib. 2020, 472, 115174. [Google Scholar] [CrossRef]
- Gupta, M.K.; Childs, D.W. Rotordynamic stability predictions for centrifugal compressors using a bulk-flow model to predict impeller shroud force and moment coefficients. J. Eng. Gas Turbines Power-Trans. Asme 2010, 132, 91402. [Google Scholar] [CrossRef]
- Zhang, D.; Lee, C.; Cave, M. A CFD study on the dynamic coefficients of labyrinth seals. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2012; Volume 44731, pp. 795–803. [Google Scholar]
- Ali, M.S.; Mortazavi, F.; Palazzolo, A. System Level Analysis of Compressor Eye-Labyrinth Seal Rotordynamic Forces: A Computational Fluid Dynamics Approach. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2021; Volume 85031, p. V09BT28A004. [Google Scholar]
- Song, J.; Kim, S.; Park, T.C.; Cha, B.J.; Lim, D.H.; Hong, J.S.; Lee, T.W.; Song, S.J. Non-Axisymmetric Flows and Rotordynamic Forces in an Eccentric Shrouded Centrifugal Compressor—Part 1: Measurement. J. Eng. Gas Turbines Power 2019, 141, 111014. [Google Scholar] [CrossRef]
- Song, J.; Song, S.J. Non-Axisymmetric Flows and Rotordynamic Forces in an Eccentric Shrouded Centrifugal Compressor—Part 2: Analysis. J. Eng. Gas Turbines Power 2019, 141, 111015. [Google Scholar] [CrossRef]
- San Andrés, L. A Review of Turbine and Compressor Aerodynamic Forces in Turbomachinery. Lubricants 2023, 11, 26. [Google Scholar] [CrossRef]
- Alford, J.S. Protecting Turbomachinery from Self-Excited Rotor Whirl. J. Eng. Power 1965, 87, 333–343. [Google Scholar] [CrossRef]
- Childs, D.W. Turbomachinery Rotordynamics with Case Studies; Minter Spring Publishing: New, York, NY, USA, 2013. [Google Scholar]
- Wachel, J.C.; Von Nimitz, W.W. Ensuring the Reliability of Offshore Gas Compression Systems. J. Pet. Technol. 1981, 33, 2252–2260. [Google Scholar] [CrossRef]
- Wachel, J.C. Rotordynamic instability field problems. In Proceedings of the NASA, Lewis Research Center Rotordyn, Instability Probl, in high-Performance Turbomachinery Workshop, San Antonio, TX, USA, 16–18 May 1982. [Google Scholar]
- Moore, J.J.; Ransom, D.L.; Viana, F. Rotordynamic Force Prediction of Centrifugal Compressor Impellers Using Computational Fluid Dynamics. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2007; Volume 47942, pp. 1023–1033. [Google Scholar]
- Moore, J.J.; Ransom, D.L. Refinement of Physics Based Approach Used in the Prediction of Impeller Rotordynamic Forces for Centrifugal Compressors. In Volume 5: Structures and Dynamics, Parts A and B; ASMEDC: Washington, DC, USA, 2008; pp. 1305–1309. [Google Scholar] [CrossRef]
- Iwatsubo, T. Evaluation of instability forces of labyrinth seals in turbines orcompressors. In Proceedings of the NASA. Lewis Res. Center Rotordyn. Instability Probl. in High-Performance Turbomachinery, Kobe, Japan, 12–14 May 1980; pp. 139–167. [Google Scholar]
- Childs, D.W.; Scharrer, J.K. An Iwatsubo-Based Solution for Labyrinth Seals: Comparison to Experimental Results. J. Eng. Gas Turbines Power 1986, 108, 325–331. [Google Scholar] [CrossRef][Green Version]
- Scharrer, J.K. Theory Versus Experiment for the Rotordynamic Coefficients of Labyrinth Gas Seals: Part I—A Two Control Volume Model. J. Vib. Acoust. 1988, 110, 270–280. [Google Scholar] [CrossRef]
- Nordmann, R.; Weiser, P. Evaluation of Rotordynamic Coefficients of Look-through Labyrinths by Means of a Three Volume Bulk Flow Model. In Proceedings of the NASA Lewis Research Center, Rotordynamic Instability Problems in High-Performance Turbomachinery 1990. Available online: https://ntrs.nasa.gov/citations/19920005138 (accessed on 28 October 2023).
- Moore, J.J. Three-Dimensional CFD Rotordynamic Analysis of Gas Labyrinth Seals. J. Vib. Acoust. 2003, 125, 427–433. [Google Scholar] [CrossRef]
- Pugachev, A.O.; Kleinhans, U.; Gaszner, M. Prediction of Rotordynamic Coefficients for Short Labyrinth Gas Seals Using Computational Fluid Dynamics. J. Eng. Gas Turbines Power 2012, 134, 62501. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of Fluid Film Lubrication; Marcel Dekker: New York, NY, USA, 2004. [Google Scholar]
- Heshmat, H.; Walowit, J.A.; Pinkus, O. Analysis of gas lubricated compliant thrust bearings. J. Tribol. 1983, 105, 638–646. [Google Scholar] [CrossRef]
- Neumann, K. Zur Frage der Verwendung von Durchblickdichtungen im Dampfturbinenbau. Maschinenbautechnik 1964, 13, 188–195. [Google Scholar]
- Blasius, H. Das Aehnlichkeitsgesetz bei Reibungsvorgängen in Flüssigkeiten. In Mitteilungen über Forschungsarbeiten auf dem Gebiete des Ingenieurwesens; Mitteilungen über Forschungsarbeiten auf dem Gebiete des Ingenieurwesens; Springer: Berlin/Heidelberg, Germany, 1913; Volume 131. [Google Scholar] [CrossRef]
- API 617; Axial and Centrifugal Compressors and Expander-Compressors for Petroleum, Chemical and gas Industry Services. Approved American National Standard: Washington, DC, USA, 2014.
- Ruscitto, D.; McCormick, J.; Gray, S. Hydrodynamic air lubricated compliant surface bearing for an automotive gas turbine engine. I. J. Bear. Perform. 1978, 7095892. [Google Scholar] [CrossRef]
- Andres, L.; Kim, T. Analysis of gas foil bearings integrating FE top foil models. Tribol. Int. 2009, 42, 111–120. [Google Scholar] [CrossRef]


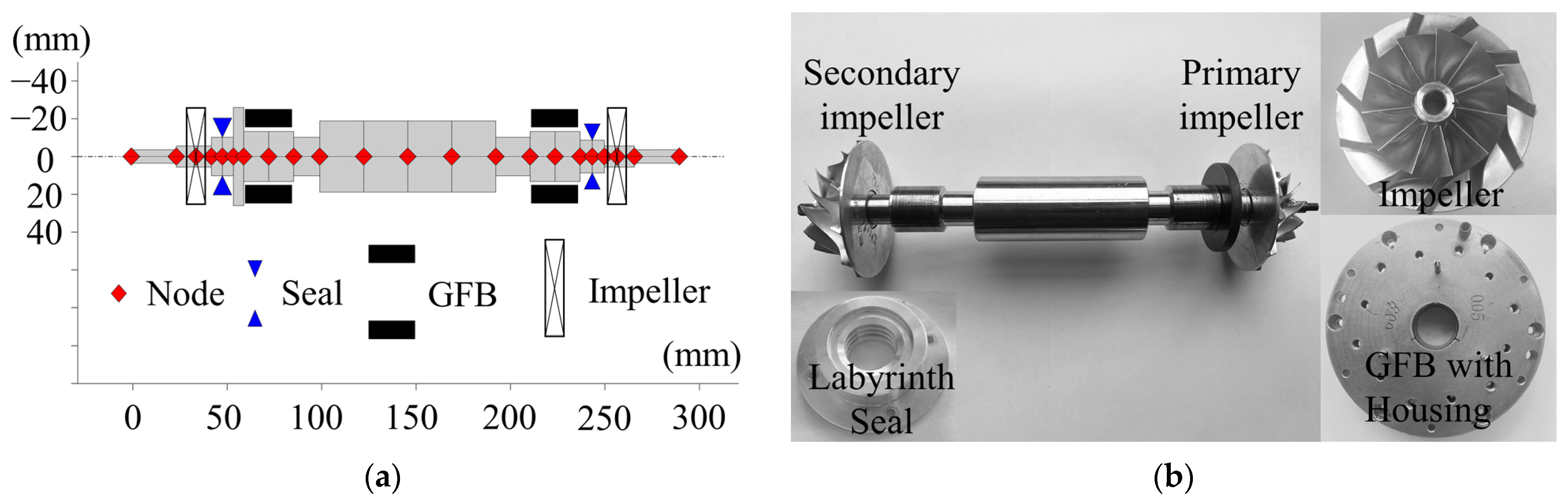


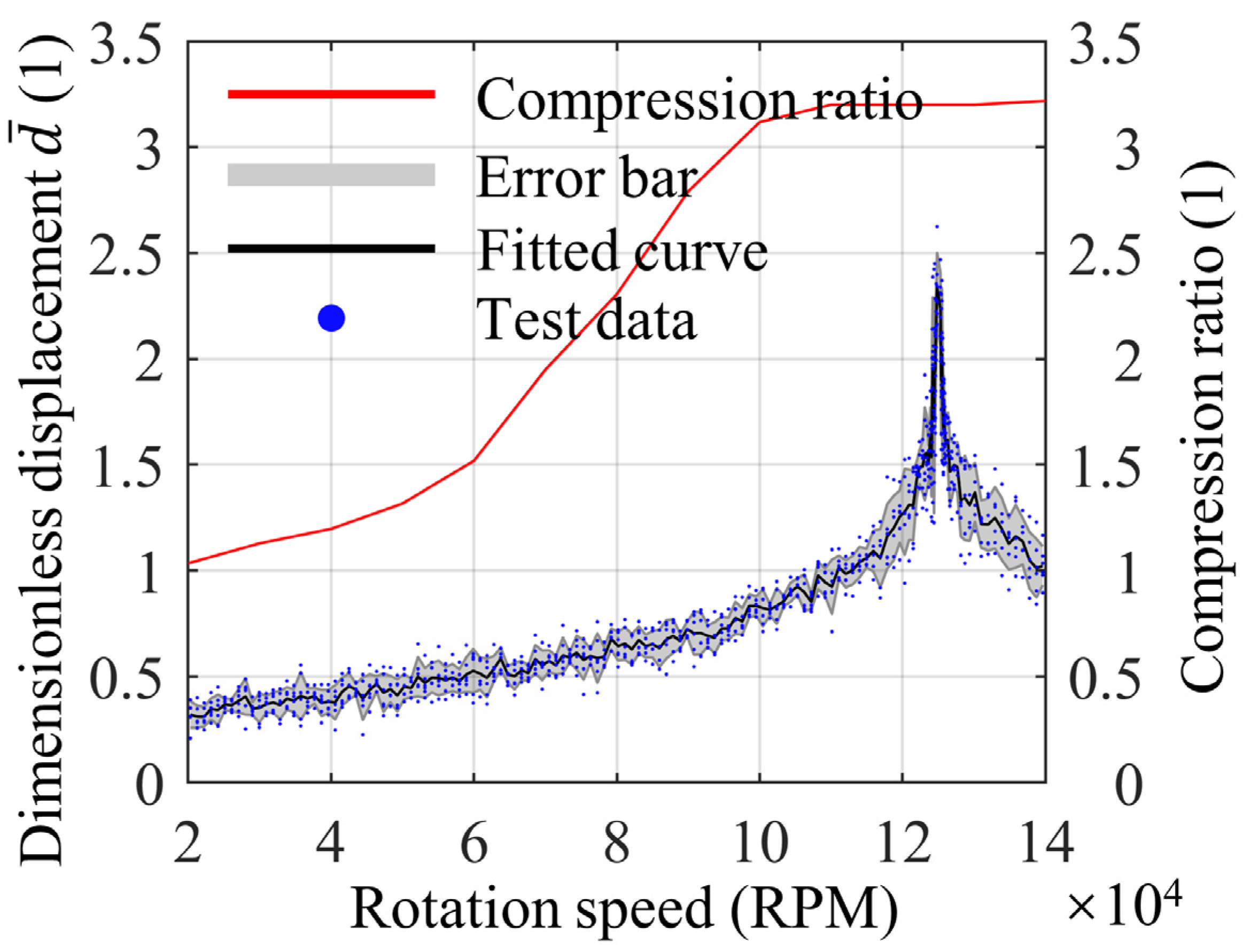
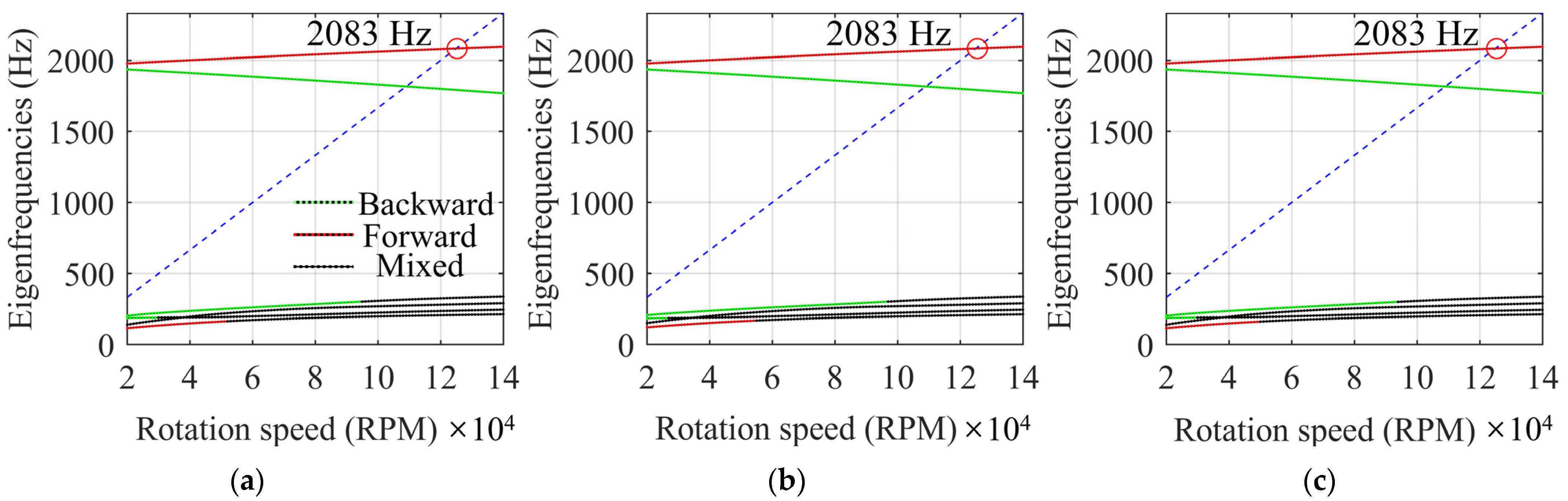
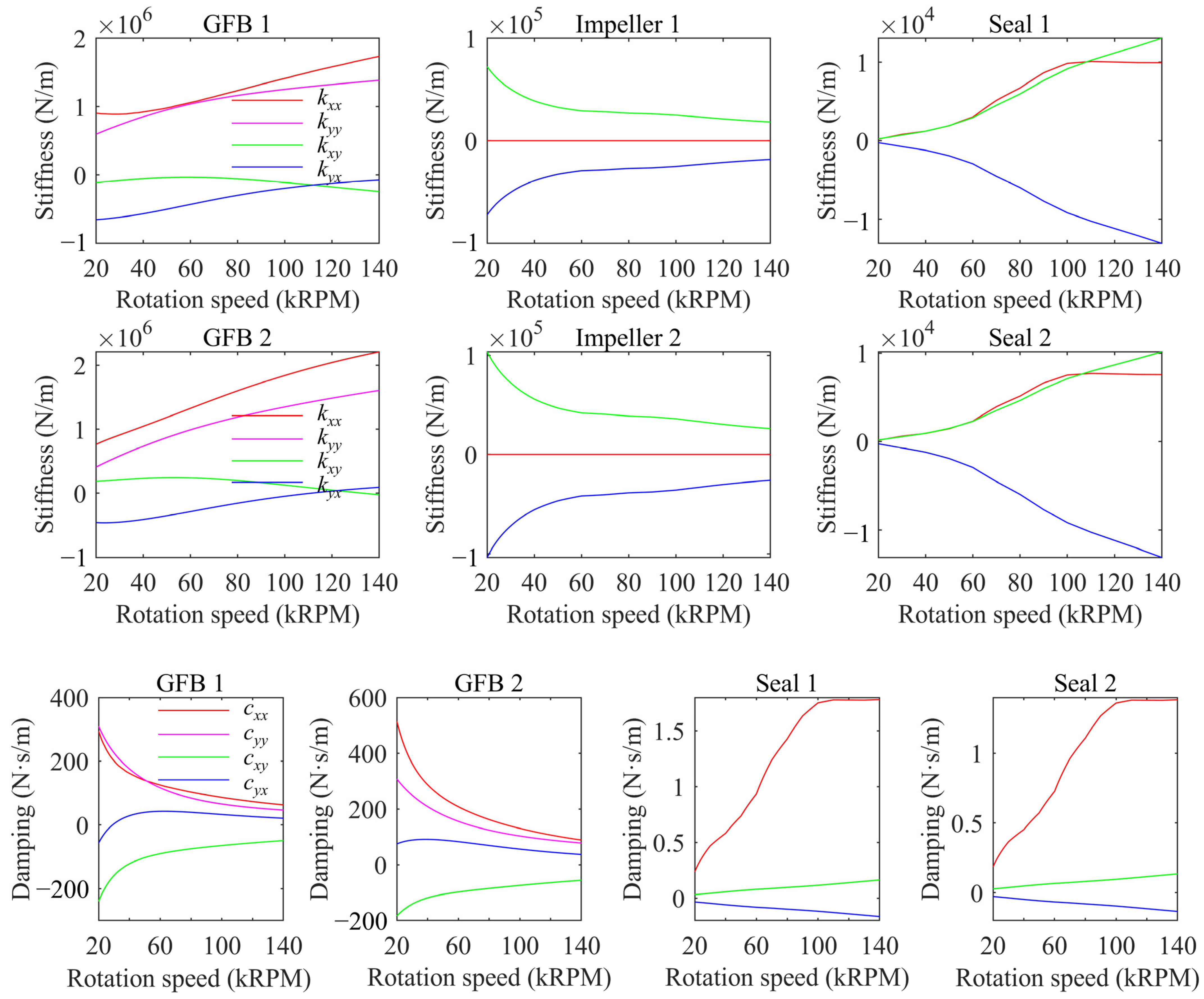

| Parameters | Value |
|---|---|
| Bearing inner radius, R0 | 19.05 mm |
| Bearing length, L0 | 38.1 mm |
| Radial clearance of GFB, C0 | 20 μm |
| Top foil thickness, ttop | 101.6 μm |
| Bump foil thickness, tbump | 101.6 μm |
| Bump pitch, s | 4.572 mm |
| Half bump length, l | 1.778 mm |
| Bump height, h | 0.508 mm |
| Bump foil Young’s modulus, Eb | 214 GPa |
| Bump foil Poisson’s ratio, νb | 0.29 |
| Parameters | Value |
|---|---|
| Compressor rated power, PW | 30 Kw |
| Rated speed, Ow | 100,000 rpm |
| Mass of rotor, m | 1426 g |
| Bearing inner radius, R1 | 13 mm |
| Bearing length, L1 | 26 mm |
| Radial clearance of GFB, C1 | 30 µm |
| Bump foil structural stiffness, kb | 1.745 × 1010 N/m3 |
| Top foil thickness, ttop | 0.124 mm |
| Maximum compression ratio, ρout/ρin | 3.97 |
| Maximum mass flow, mf | 0.19 kg/s |
| Primary impeller diameter, D1 | 72 mm |
| Secondary impeller diameter, D2 | 68 mm |
| Mass of primary impeller, m1 | 75 g |
| Mass of secondary impeller, m2 | 53 g |
| Primary impeller diffuser width, h1 | 4.2 mm |
| Secondary impeller diffuser width, h2 | 3.1mm |
| Labyrinth seal clearance, Cs | 0.25 mm |
| Primary labyrinth seal radius, Rs1 | 10 mm |
| Secondary labyrinth seal radius, Rs2 | 8.5 mm |
| Height of labyrinth seal strip, B | 2.25 mm |
| Pitch of seal strips, Ls | 1.5 mm |
| Number of cavities, NC | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ying, M.; Liu, X.; Zhang, Y.; Zhang, C. Impact of Gas Foil Bearings, Labyrinth Seals, and Impellers on the Critical Speed of Centrifugal Compressors for Fuel Cell Vehicles: A Comprehensive Investigation. Lubricants 2023, 11, 532. https://doi.org/10.3390/lubricants11120532
Ying M, Liu X, Zhang Y, Zhang C. Impact of Gas Foil Bearings, Labyrinth Seals, and Impellers on the Critical Speed of Centrifugal Compressors for Fuel Cell Vehicles: A Comprehensive Investigation. Lubricants. 2023; 11(12):532. https://doi.org/10.3390/lubricants11120532
Chicago/Turabian StyleYing, Ming, Xinghua Liu, Yue Zhang, and Chongbin Zhang. 2023. "Impact of Gas Foil Bearings, Labyrinth Seals, and Impellers on the Critical Speed of Centrifugal Compressors for Fuel Cell Vehicles: A Comprehensive Investigation" Lubricants 11, no. 12: 532. https://doi.org/10.3390/lubricants11120532
APA StyleYing, M., Liu, X., Zhang, Y., & Zhang, C. (2023). Impact of Gas Foil Bearings, Labyrinth Seals, and Impellers on the Critical Speed of Centrifugal Compressors for Fuel Cell Vehicles: A Comprehensive Investigation. Lubricants, 11(12), 532. https://doi.org/10.3390/lubricants11120532





