Simple Deconvolution Models for Evaluating the True Microhardness of Thin Nanostructured Coatings Deposited via an Advanced Physical Vapor Deposition Technique
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of the NTC Samples
2.2. Microindentation Hardness Testing Experiment
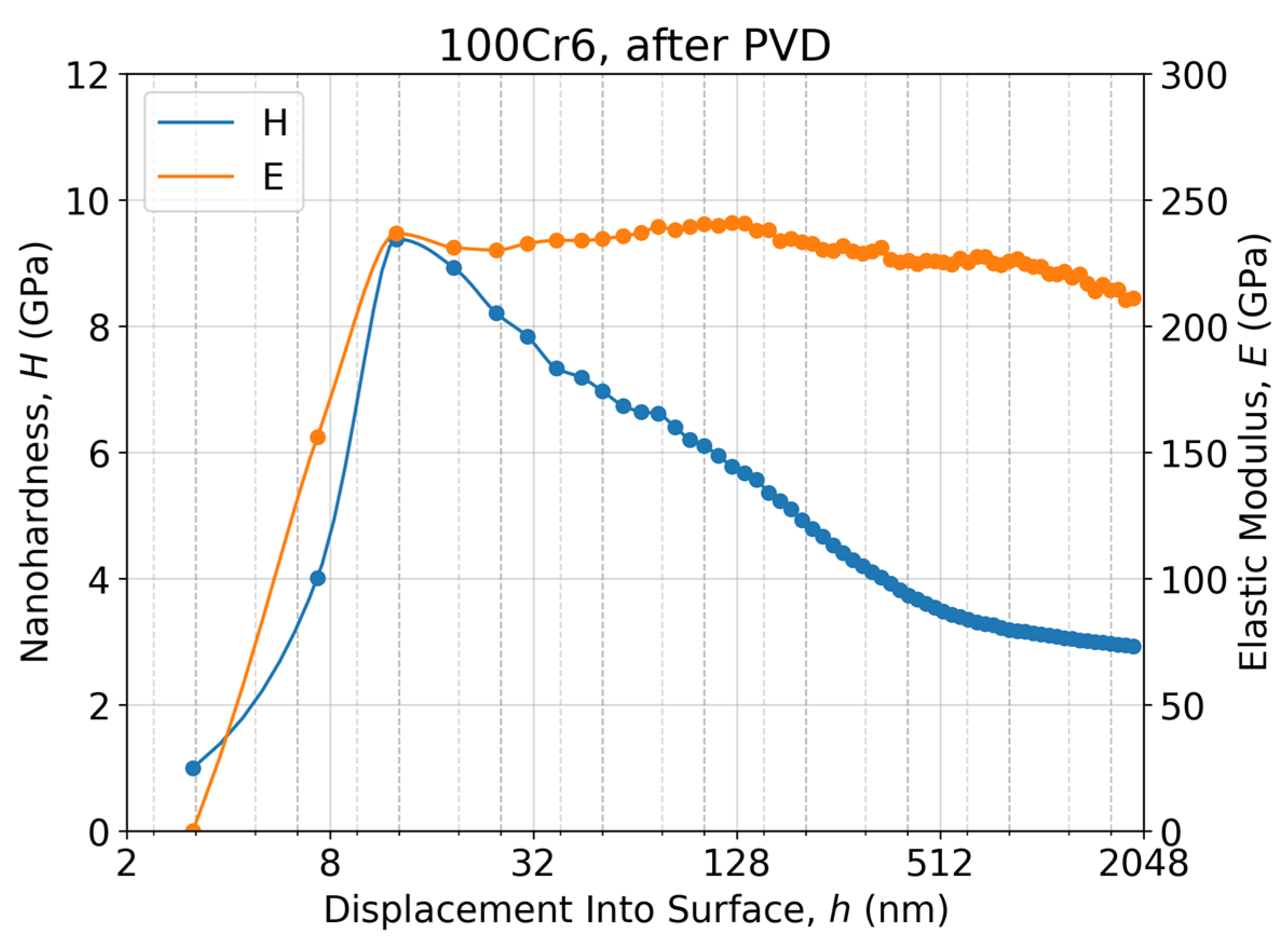
2.3. Nanoindentation Hardness Testing Experiment
2.4. Empirical Mathematical Models Applied for The Calculation of the Apparent Microhardness, Ha(h)
2.5. Determination of the Robustness of the Predicted True Hardness, Hf, of the Coated Film
3. Results and Discussion
3.1. Preparation and Characterization of the NTC Film Samples
3.2. Micromechanical Properties of the NTC Film Samples
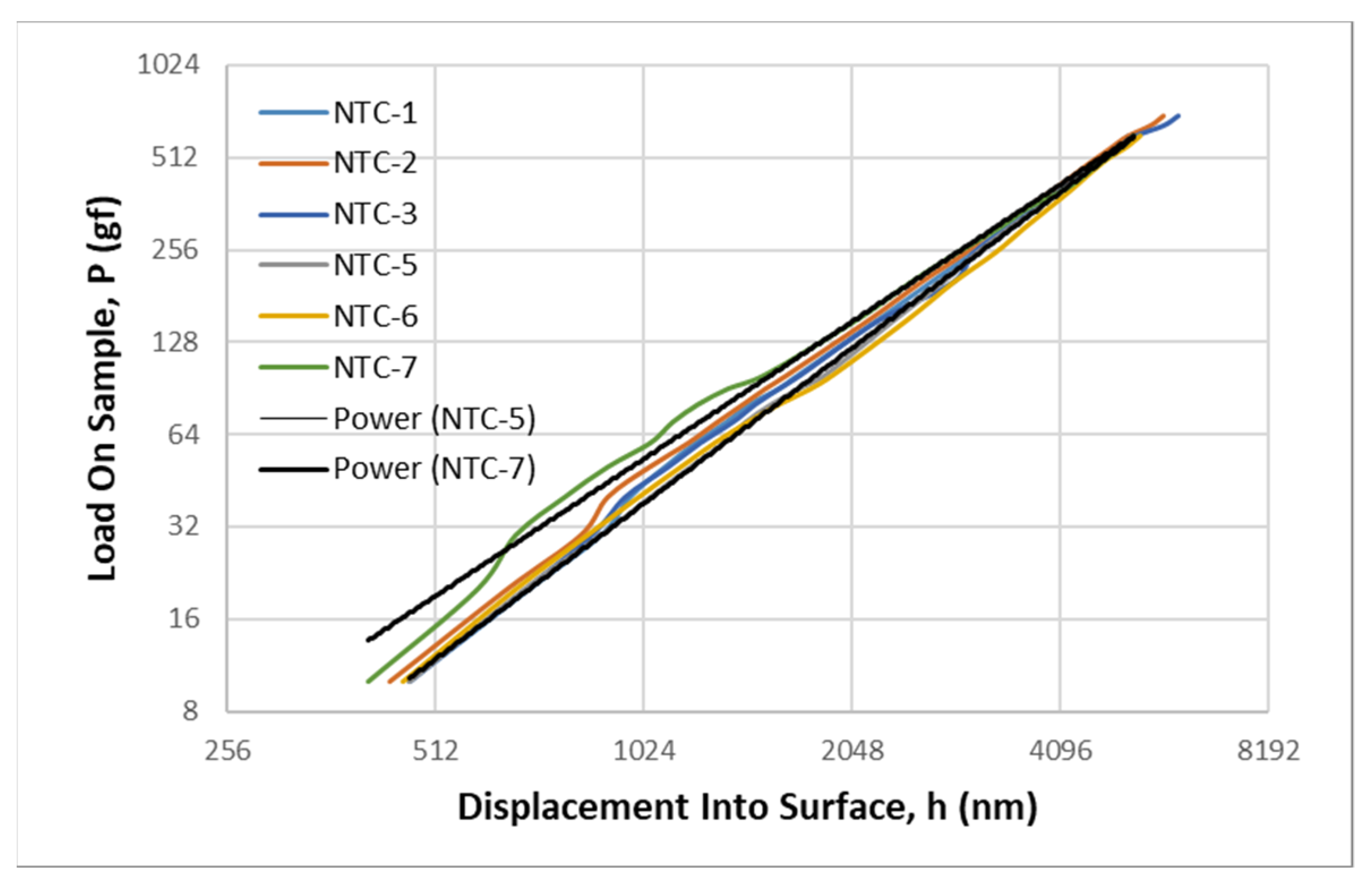
3.2.1. Microindentation Response Analysis Using the Load–Displacement Curves
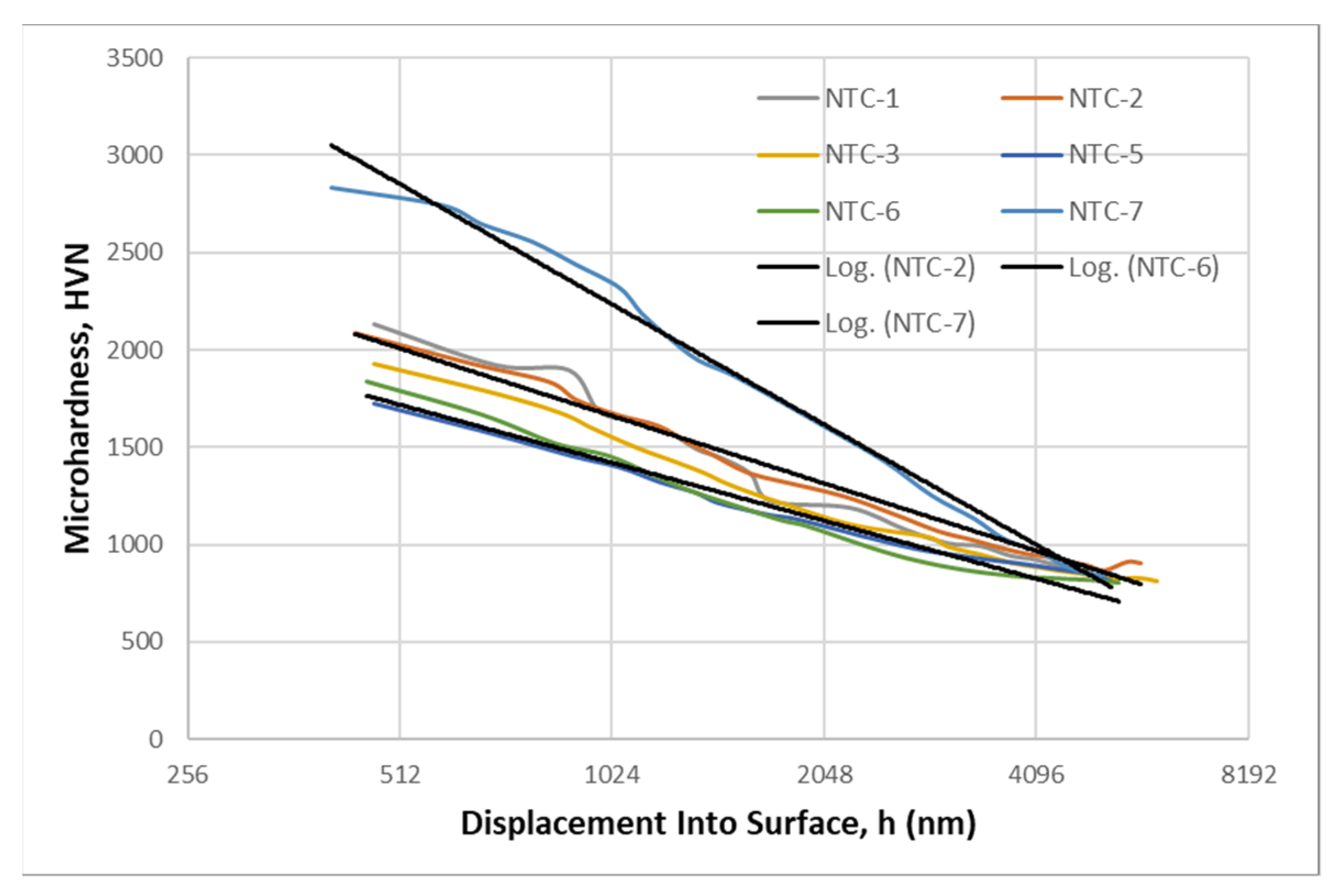
3.2.2. Microindentation Response Analysis Using the Hardness–Displacement Curves
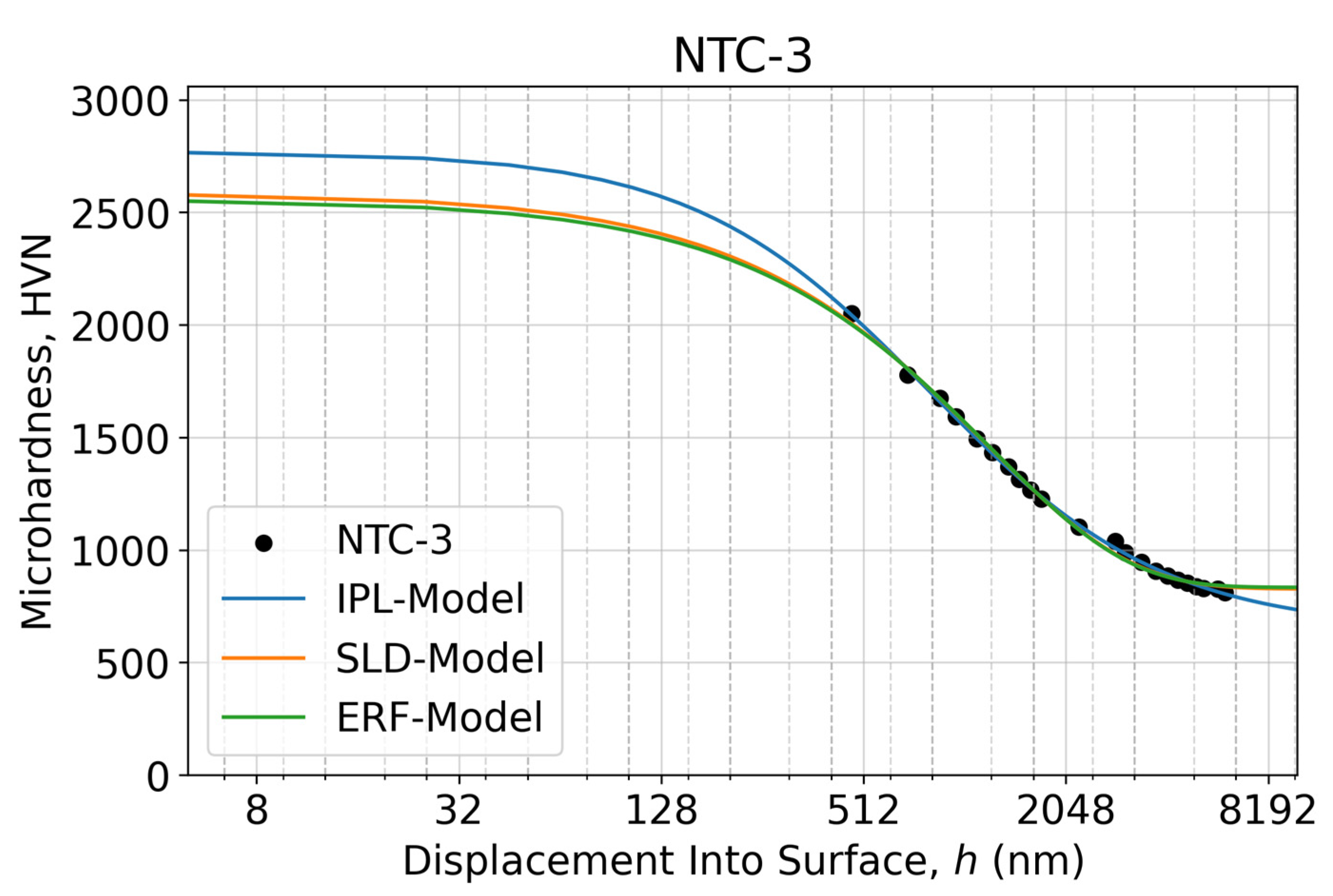
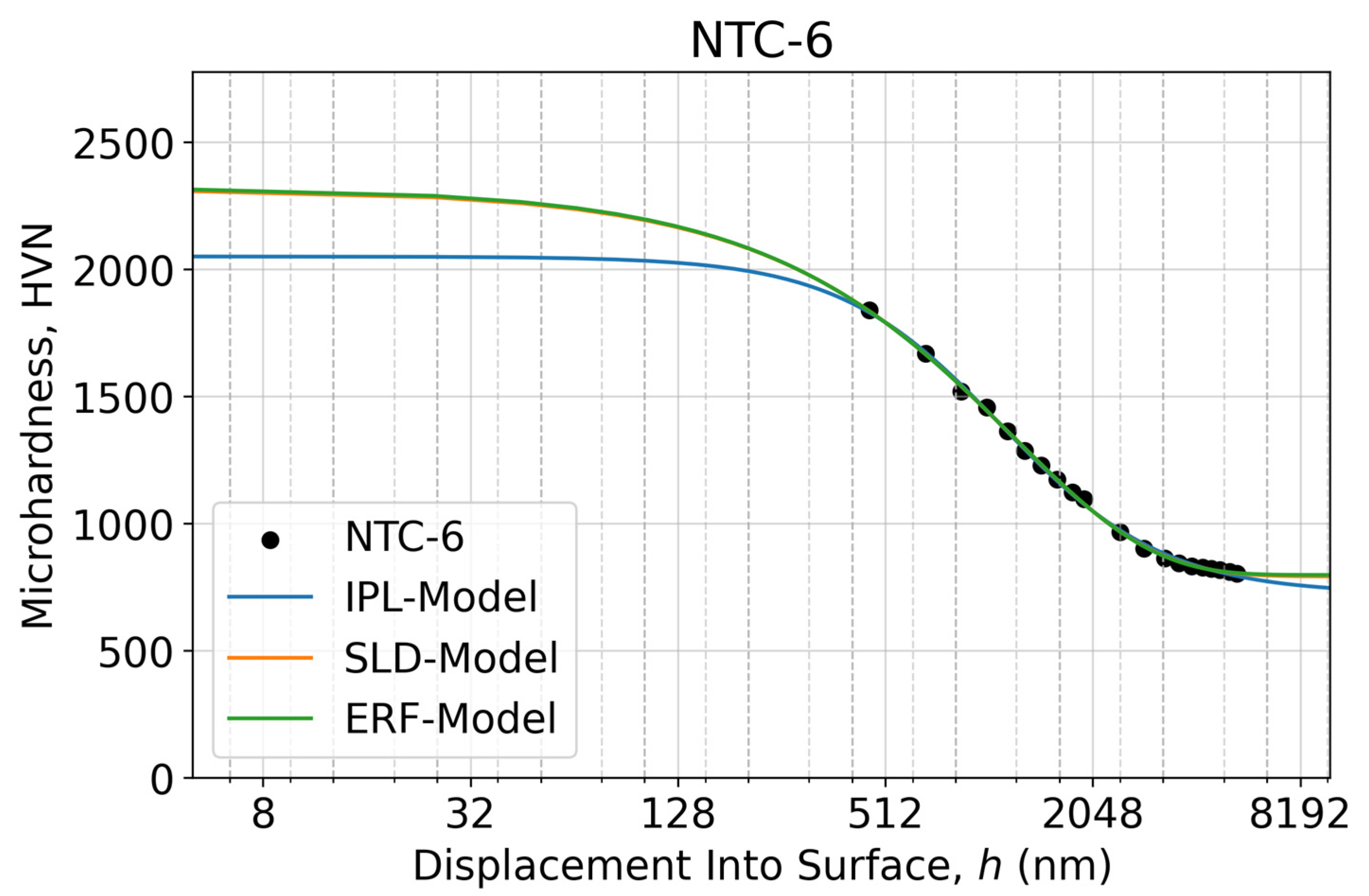
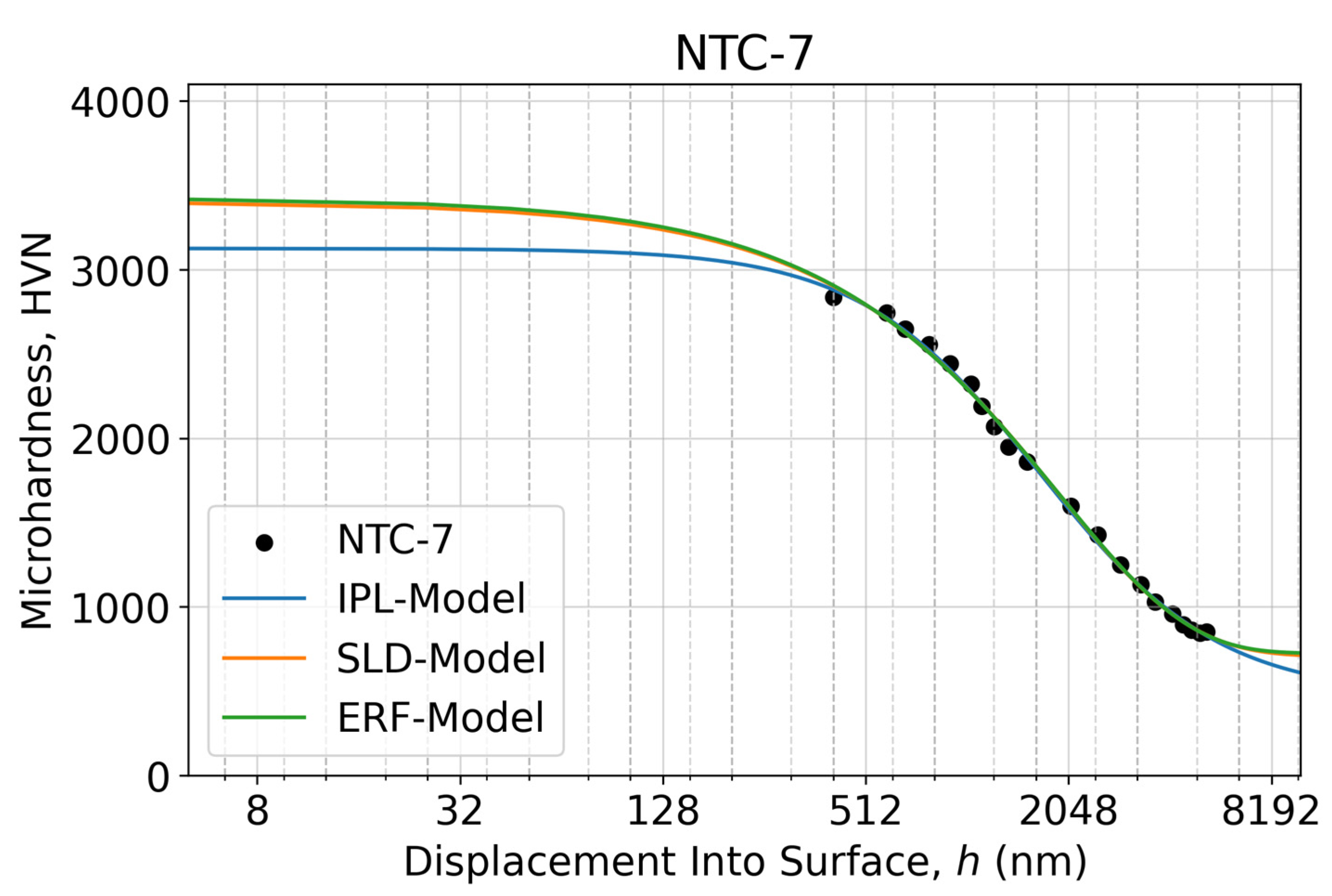
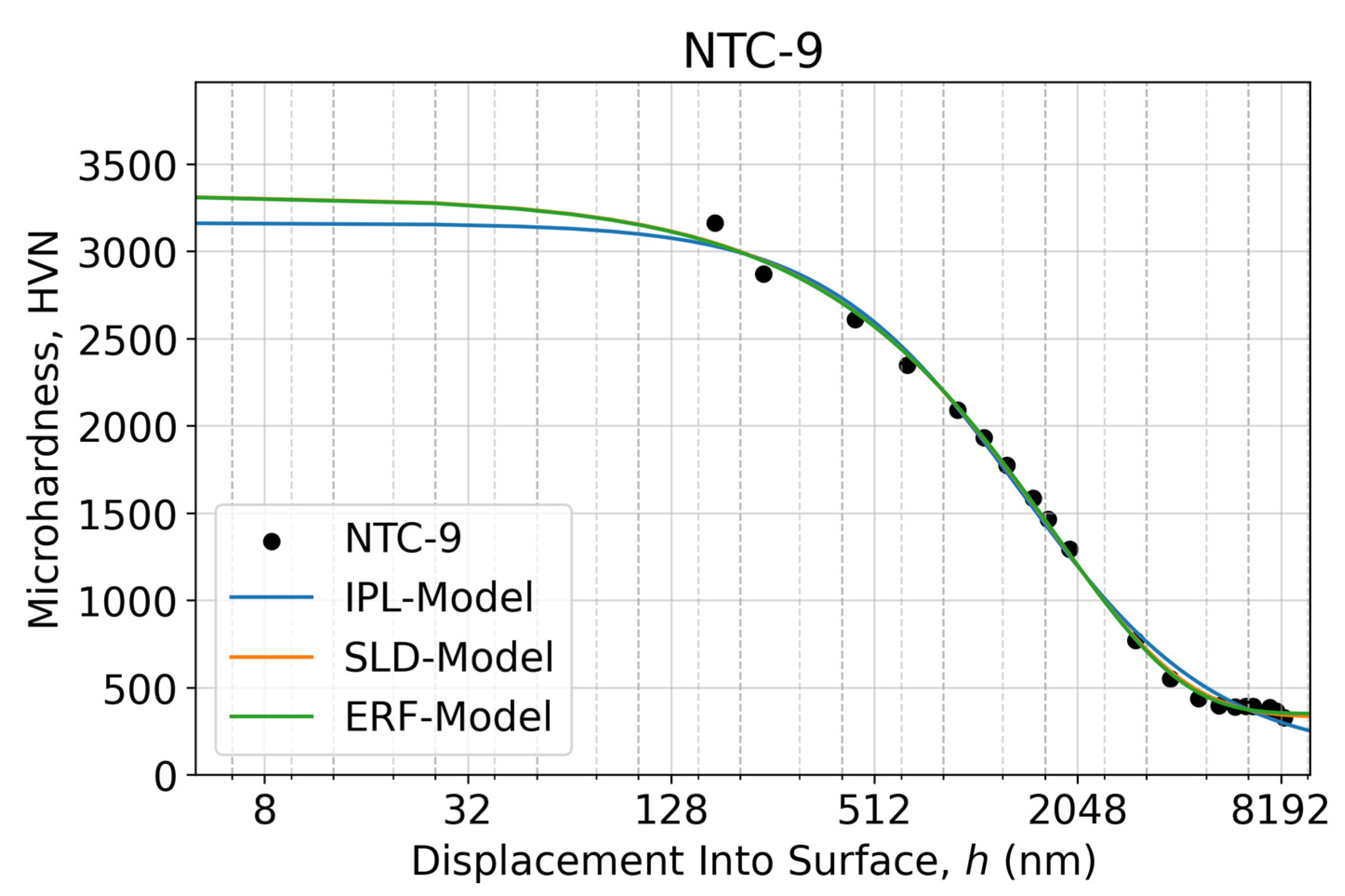
3.3. Mathematical Modelling of the Apparent Microhardness, Ha(h)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A


References
- Zhang, S.; Sun, D.; Fu, Y.; Du, H. Recent Advances of Superhard Nanocomposite Coatings: A Review. Surf. Coat. Technol. 2003, 167, 113–119. [Google Scholar] [CrossRef]
- Musil, J. Hard and Superhard Nanocomposite Coatings. Surf. Coat. Technol. 2000, 125, 322–330. [Google Scholar] [CrossRef]
- Musil, J.; Vlček, J. Magnetron Sputtering of Hard Nanocomposite Coatings and Their Properties. Surf. Coat. Technol. 2001, 142–144, 557–566. [Google Scholar] [CrossRef]
- Chang, Y.Y.; Lai, H.M. Wear Behavior and Cutting Performance of CrAlSiN and TiAlSiN Hard Coatings on Cemented Carbide Cutting Tools for Ti Alloys. Surf. Coat. Technol. 2014, 259, 152–158. [Google Scholar] [CrossRef]
- Miletić, A.; Panjan, P.; Škorić, B.; Čekada, M.; Dražič, G.; Kovač, J. Microstructure and Mechanical Properties of Nanostructured Ti–Al–Si–N Coatings Deposited by Magnetron Sputtering. Surf. Coat. Technol. 2014, 241, 105–111. [Google Scholar] [CrossRef]
- Farooq, S.A.; Raina, A.; Mohan, S.; Singh, R.A.; Jayalakshmi, S.; Haq, M.I.U. Nanostructured Coatings: Review on Processing Techniques, Corrosion Behaviour and Tribological Performance. Nanomaterials 2022, 12, 1323. [Google Scholar] [CrossRef] [PubMed]
- Bazhin, P.M.; Kostitsyna, E.V.; Stolin, A.M.; Chizhikov, A.M.; Bychkova, M.Y.; Pazniak, A. Nanostructured Ceramic Composite Rods: Synthesis, Properties and Application. Ceram. Int. 2019, 45, 9297–9301. [Google Scholar] [CrossRef]
- Wu, W.Y.; Ting, J.M. Growth and Characteristics of Metal-Containing Diamond-like Carbon Using a Self-Assembled Process. Carbon N. Y. 2006, 44, 1210–1217. [Google Scholar] [CrossRef]
- Persson, K.; Gåhlin, R. Tribological Performance of a DLC Coating in Combination with Water-Based Lubricants. Tribol. Int. 2003, 36, 851–855. [Google Scholar] [CrossRef]
- Maniks, J.; Mitin, V.; Kanders, U.; Kovalenko, V.; Nazarovs, P.; Baitimirova, M.; Meija, R.; Zabels, R.; Kundzins, K.; Erts, D. Deformation Behavior and Interfacial Sliding in Carbon/Copper Nanocomposite Films Deposited by High Power DC Magnetron Sputtering. Surf. Coat. Technol. 2015, 276, 279–285. [Google Scholar] [CrossRef]
- Das, J.; Linke, B. Evaluation and Systematic Selection of Significant Multi-Scale Surface Roughness Parameters (SRPs) as Process Monitoring Index. J. Mater. Process. Technol. 2017, 244, 157–165. [Google Scholar] [CrossRef]
- Pogrebnjak, A.; Smyrnova, K.; Bondar, O. Nanocomposite Multilayer Binary Nitride Coatings Based on Transition and Refractory Metals: Structure and Properties. Coatings 2019, 9, 155. [Google Scholar] [CrossRef]
- Kanders, U.; Lungevics, J.; Leitans, A.; Boiko, I.; Berzins, K.; Trubina, Z. Nanostructured TiAlSi-CN:Me/a-CN:Si3N4 Composite Coatings Deposited by Advanced PVD Technique. Solid State Phenom. 2021, 320, 37–42. [Google Scholar] [CrossRef]
- Das, P.; Anwar, S.; Bajpai, S.; Anwar, S. Structural and Mechanical Evolution of TiAlSiN Nanocomposite Coating under Influence of Si3N4 Power. Surf. Coat. Technol. 2016, 307, 676–682. [Google Scholar] [CrossRef]
- Liu, Y.; Yu, S.; Shi, Q.; Ge, X.; Wang, W. Multilayer Coatings for Tribology: A Mini Review. Nanomater 2022, 12, 1388. [Google Scholar] [CrossRef]
- Leitans, A.; Jansons, E.; Lungevics, J.; Kundzins, K.; Boiko, I.; Kanders, U.; Kovalenko, V.; Linins, O. Tribological and Micromechanical Properties of the Nanostructured Carbonitride/Nitride Coatings of Transition Metals Alloyed by Hf and Nb. Coatings 2023, 13, 552. [Google Scholar] [CrossRef]
- Plichta, T.; Zahradnicek, R.; Cech, V. Surface Topography Affects the Nanoindentation Data. Thin Solid Films 2022, 745, 139105. [Google Scholar] [CrossRef]
- Kulej, E.; Kucharska, B.; Pyka, G.; Gwoździk, M. Characterization of the Surface Topography and Nano-Hardness of Cu/Ni Multilayer Structures. Cent. Eur. J. Phys. 2011, 9, 1421–1425. [Google Scholar] [CrossRef]
- Wei, Z.; Zhang, G.; Chen, H.; Luo, J.; Liu, R.; Guo, S. A Simple Method for Evaluating Elastic Modulus of Thin Films by Nanoindentation. J. Mater. Res. 2009, 24, 801–815. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; McGurk, M.R.; Bull, S.J.; Page, T.F. On the Hardness of Coated Systems. Surf. Coat. Technol. 1998, 99, 171–183. [Google Scholar] [CrossRef]
- Iost, A.; Guillemot, G.; Rudermann, Y.; Bigerelle, M. A Comparison of Models for Predicting the True Hardness of Thin Films. Thin Solid Films 2012, 524, 229–237. [Google Scholar] [CrossRef]
- Doerner, M.F.; Nix, W.D. A Method for Interpreting the Data from Depth-Sensing Indentation Instruments. J. Mater. Res. 1986, 1, 601–609. [Google Scholar] [CrossRef]
- King, R.B. Elastic Analysis of Some Punch Problems for a Layered Medium. Int. J. Solids Struct. 1987, 23, 1657–1664. [Google Scholar] [CrossRef]
- Tuck, J.R.; Korsunsky, A.M.; Bhat, D.G.; Bull, S.J. Indentation Hardness Evaluation of Cathodic Arc Deposited Thin Hard Coatings. Surf. Coat. Technol. 2001, 139, 63–74. [Google Scholar] [CrossRef]
- Saha, R.; Nix, W.D. Effects of the Substrate on the Determination of Thin Film Mechanical Properties by Nanoindentation. Acta Mater. 2002, 50, 23–38. [Google Scholar] [CrossRef]
- Menčík, J.; Munz, D.; Quandt, E.; Weppelmann, E.R.; Swain, M.V. Determination of Elastic Modulus of Thin Layers Using Nanoindentation. J. Mater. Res. 1997, 12, 2475–2484. [Google Scholar] [CrossRef]
- Jansons, E.; Lungevics, J.; Kanders, U.; Leitans, A.; Civcisa, G.; Linins, O.; Kundzins, K.; Boiko, I. Tribological and Mechanical Properties of the Nanostructured Superlattice Coatings with Respect to Surface Texture. Lubricants 2022, 10, 285. [Google Scholar] [CrossRef]
- 6507-2:2018; Metallic materials—Vickers hardness test—Part 2: Verification and calibration of testing machines. International Organization for Standardization: Geneva, Switzerland, 2018.
- Oliver, W.C.; Pharr, G.M. Measurement of Hardness and Elastic Modulus by Instrumented Indentation: Advances in Understanding and Refinements to Methodology. J. Mater. Res. 2004, 19, 3–20. [Google Scholar] [CrossRef]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental Algorithms for Scientific Computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef]
- Efron, B.; Tibshirani, R.J. An Introduction to the Bootstrap. Teach. Stat. 1994, 23, 49–54. [Google Scholar] [CrossRef]
- Sneddon, I.N. The Relation between Load and Penetration in the Axisymmetric Boussinesq Problem for a Punch of Arbitrary Profile. Int. J. Eng. Sci. 1965, 3, 47–57. [Google Scholar] [CrossRef]
- Attaf, M.T. Connection between the Loading Curve Models in Elastoplastic Indentation. Mater. Lett. 2004, 58, 3491–3498. [Google Scholar] [CrossRef]
- Petrík, J.; Blaško, P.; Mihaliková, M.; Mikloš, V. The Relationship between the Deformation and the Indentation Size Effect (ISE). J. Appl. Res. Technol. 2018, 16, 267–275. [Google Scholar] [CrossRef]
- Broitman, E. Indentation Hardness Measurements at Macro-, Micro-, and Nanoscale: A Critical Overview. Tribol. Lett. 2017, 65, 23. [Google Scholar] [CrossRef]
- Chen, K.S.; Chen, T.C.; Ou, K.S. Development of Semi-Empirical Formulation for Extracting Materials Properties from Nanoindentation Measurements: Residual Stresses, Substrate Effect, and Creep. Thin Solid Films 2008, 516, 1931–1940. [Google Scholar] [CrossRef]
- Tayebi, N.; Polycarpou, A.A.; Conry, T.F. Effects of Substrate on Determination of Hardness of Thin Fillms by Nanoscratch and Nanoindentation Techniques. J. Mater. Res. 2004, 19, 1791–1802. [Google Scholar] [CrossRef]
- Chen, Y.; Guo, T.; Wang, J.; Pang, X.; Qiao, L. Effects of Orientation on Microstructure and Mechanical Properties of TiN/AlN Superlattice Films. Scr. Mater. 2021, 201, 113951. [Google Scholar] [CrossRef]
- Hahn, R.; Bartosik, M.; Soler, R.; Kirchlechner, C.; Dehm, G.; Mayrhofer, P.H. Superlattice Effect for Enhanced Fracture Toughness of Hard Coatings. Scr. Mater. 2016, 124, 67–70. [Google Scholar] [CrossRef]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar] [CrossRef]
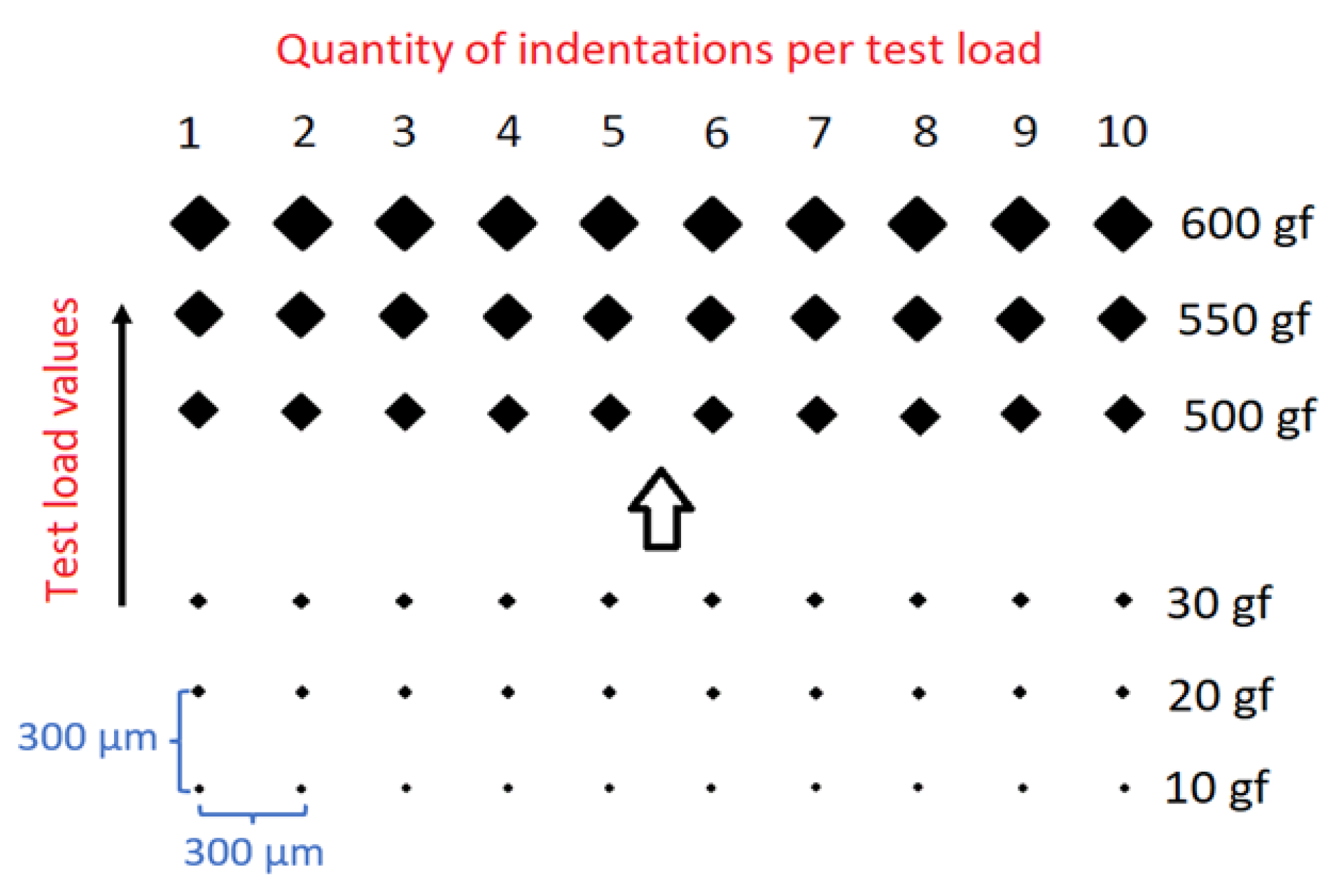




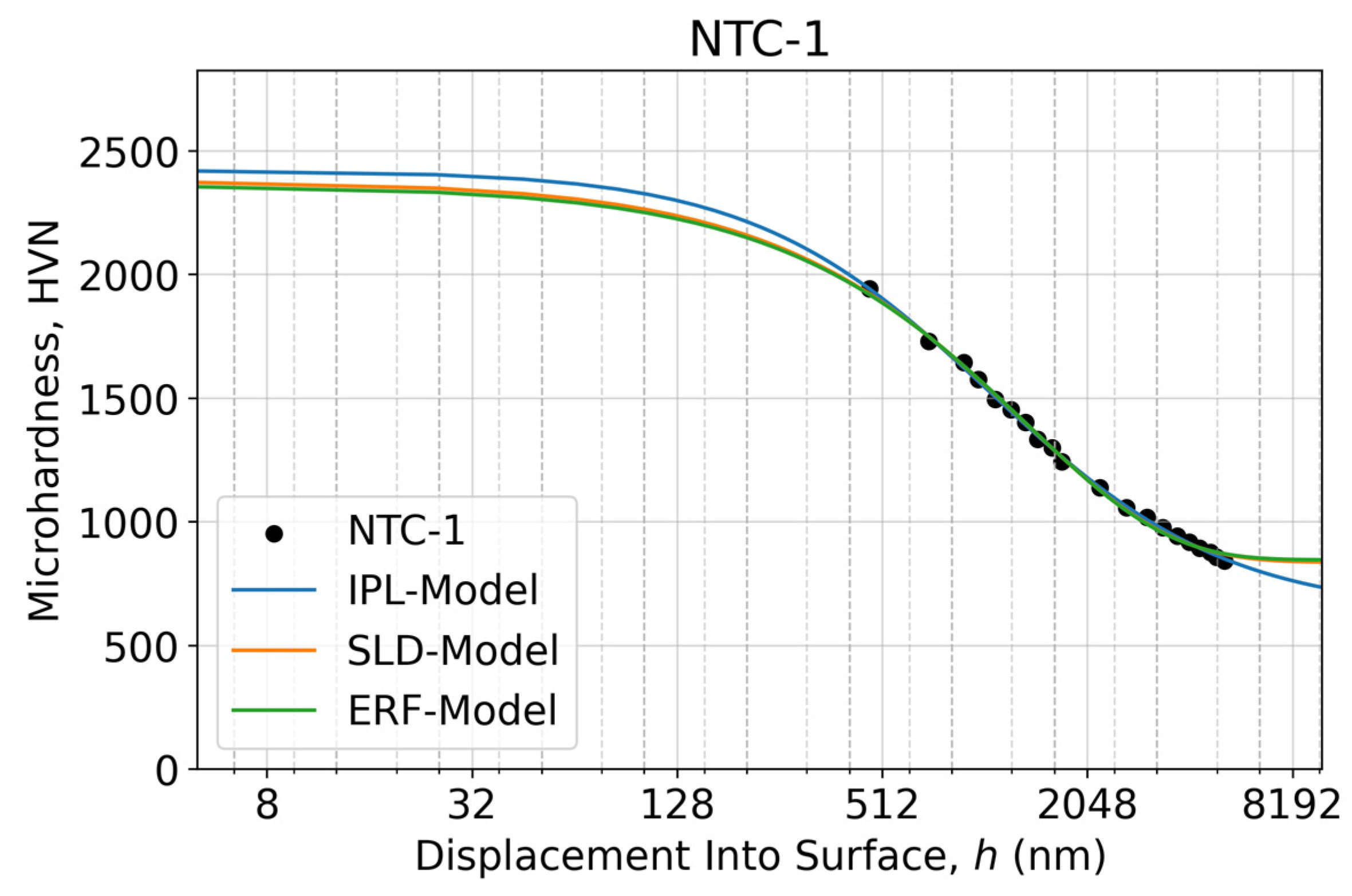
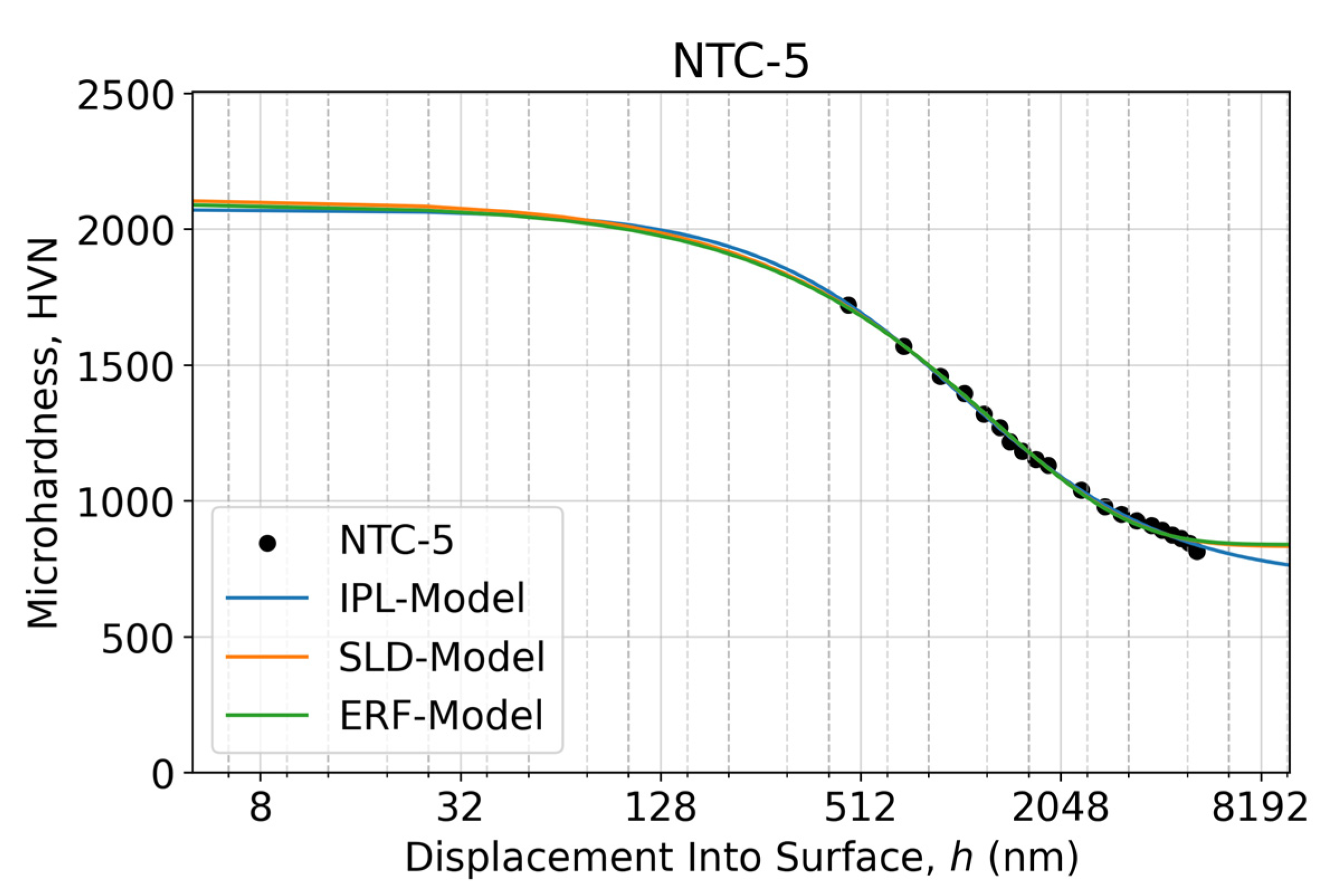
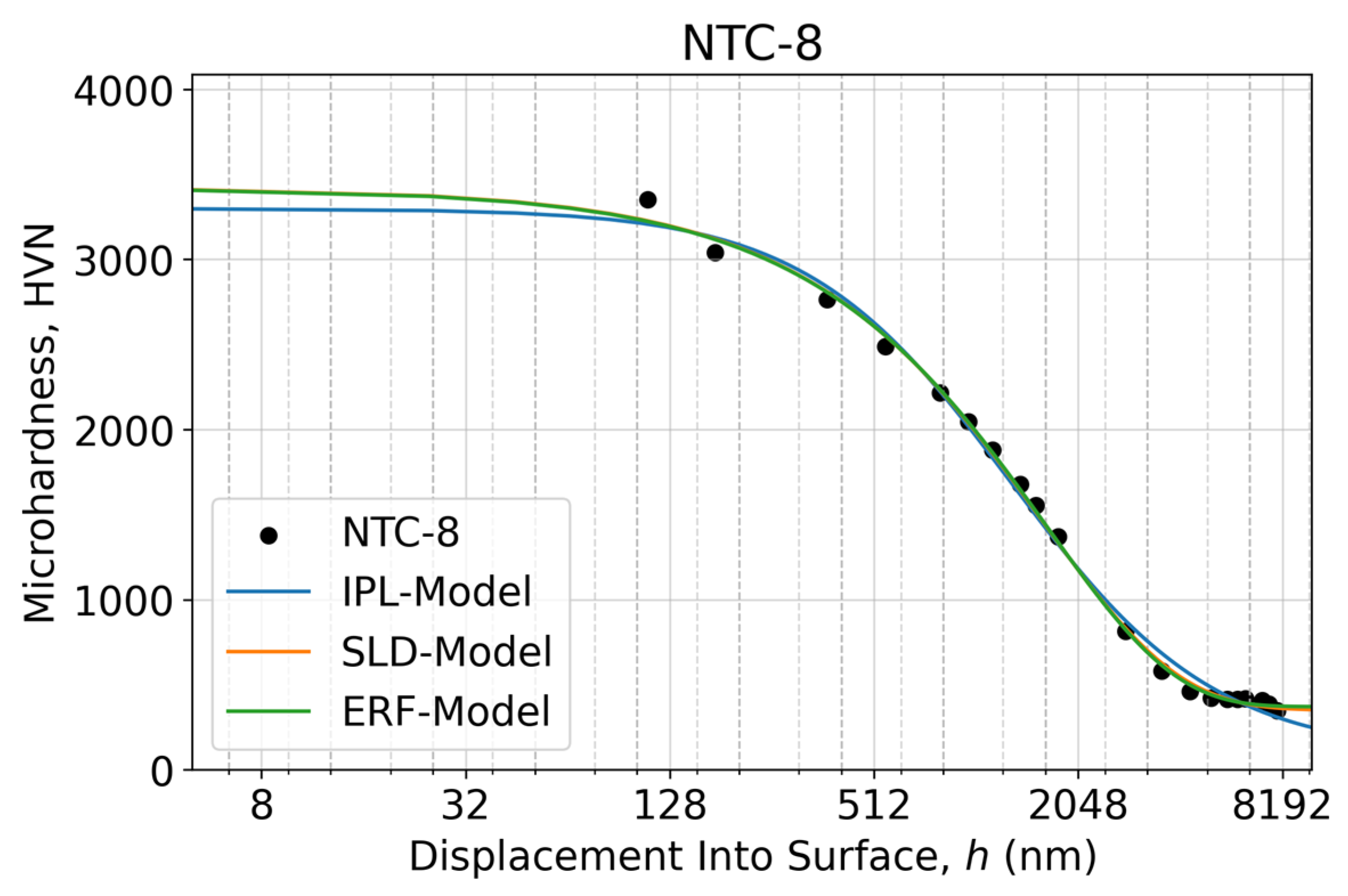

| Sample Label | Coating’s Bilayered Substructure 1 | Coating Thickness, t (nm) 2 | Indentation Depth, h (nm) at 100 gf 3 | Microhardness, HVN at 100 gf 4 |
|---|---|---|---|---|
| NTC-1 | {TiAlSiZr-N/W-CN} | 6200 ± 315 | 1720 ± 137 | 1244 ± 103 |
| NTC-2 | {TiAlSiNb-N/Ti-CN} | 5400 ± 270 | 1652 ± 132 | 1355 ± 109 |
| NTC-3 | {TiAlSiCr-N/Si-CN} | 5060 ± 250 | 1731 ± 138 | 1228 ± 98 |
| NTC-4 | {TiAlSi-N/TiNb-CN} | 4100 ± 190 | 2509 ±201 | 586 ± 47 |
| NTC-5 | {TiAlSi-N/TiNb-CN} | 3300 ± 160 | 1880 ± 150 | 1131 ± 91 |
| NTC-6 | {TiAlSi-N/TiNb-CN} | 2200 ± 110 | 1930 ± 154 | 1777 ± 142 |
| NTC-7 | {TiAlSi-N/TiHf-CN} | 4700 ± 130 | 1540 ± 123 | 1861 ± 149 |
| NTC-8 | {TiAlSi-N/TiHf-CN} | 6200 ± 315 | 1783 ± 142 | 1371 ± 110 |
| NTC-9 | {TiAlSi-N/TiHf-CN} | 6100 ± 300 | 1937 ± 154 | 1294 ± 104 |
| Sample | IPL-Model | SLD-Model | ERF-Model |
|---|---|---|---|
| NTC-1 | 107.9 | 248.4 | 284.2 |
| NTC-2 | 393.5 | 398.3 | 425.9 |
| NTC-3 | 130.9 | 453.8 | 531.3 |
| NTC-4 | 589 | 1753.6 | 2250.6 |
| NTC-5 | 85.6 | 177.5 | 201.4 |
| NTC-6 | 135.2 | 68.6 | 64.8 |
| NTC-7 | 1106.3 | 1373.3 | 1392.8 |
| NTC-8 | 4529.3 | 1991.5 | 1810.9 |
| NTC-9 | 3886.2 | 1780.5 | 1619.1 |
| Sample | Hf (HVN) | Hf2.5 (HVN) | Hf97.5 (HVN) |
|---|---|---|---|
| NTC-1 | 2397.4 | 2129 | 4848.3 |
| NTC-2 | 2462.7 | 2192.9 | 2898.9 |
| NTC-3 | 2738.8 | 2262.3 | 4650 |
| NTC-4 | 2242.6 | 2198.8 | 3773.1 |
| NTC-5 | 2081.4 | 1925.6 | 3612 |
| NTC-6 | 2029.7 | 1716.4 | 2143 |
| NTC-7 | 3137.2 | 2997.7 | 4635.3 |
| NTC-8 | 3297 | 2821.1 | 3490.9 |
| NTC-9 | 3162.4 | 2523.9 | 3403.5 |
| Sample | Hf (HVN) | Hf2.5 (HVN) | Hf97.5 (HVN) |
|---|---|---|---|
| NTC-1 | 2378.9 | 2235.1 | 2431.7 |
| NTC-2 | 2617.5 | 2501.1 | 2683.2 |
| NTC-3 | 2587 | 2334.8 | 2669.8 |
| NTC-4 | 2479.2 | 2337.6 | 4322.7 |
| NTC-5 | 2106.5 | 1990.6 | 2147.8 |
| NTC-6 | 2303.3 | 2050.6 | 2363.2 |
| NTC-7 | 3408.1 | 3230.4 | 3650.5 |
| NTC-8 | 3418.1 | 3075.1 | 3553 |
| NTC-9 | 3324 | 2924.5 | 3458.4 |
| Sample | Hf (HVN) | Hf2.5 (HVN) | Hf97.5 (HVN) |
|---|---|---|---|
| NTC-1 | 2357.5 | 2213.8 | 2418.3 |
| NTC-2 | 2601.2 | 2478.6 | 2664.6 |
| NTC-3 | 2558.1 | 2303.4 | 2652.4 |
| NTC-4 | 2559 | 2343.2 | 4146.2 |
| NTC-5 | 2092.3 | 1956.5 | 2131.4 |
| NTC-6 | 2314.1 | 2120.6 | 2341.8 |
| NTC-7 | 3421.7 | 3254.7 | 3603.2 |
| NTC-8 | 3413.2 | 3144.3 | 3545.7 |
| NTC-9 | 3313 | 3039.6 | 3450.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kanders, U.; Kanders, K.; Jansons, E.; Lungevics, J.; Sirants, R.; Leitans, A.; Boiko, I. Simple Deconvolution Models for Evaluating the True Microhardness of Thin Nanostructured Coatings Deposited via an Advanced Physical Vapor Deposition Technique. Lubricants 2023, 11, 501. https://doi.org/10.3390/lubricants11120501
Kanders U, Kanders K, Jansons E, Lungevics J, Sirants R, Leitans A, Boiko I. Simple Deconvolution Models for Evaluating the True Microhardness of Thin Nanostructured Coatings Deposited via an Advanced Physical Vapor Deposition Technique. Lubricants. 2023; 11(12):501. https://doi.org/10.3390/lubricants11120501
Chicago/Turabian StyleKanders, Uldis, Karlis Kanders, Ernests Jansons, Janis Lungevics, Raimonds Sirants, Armands Leitans, and Irina Boiko. 2023. "Simple Deconvolution Models for Evaluating the True Microhardness of Thin Nanostructured Coatings Deposited via an Advanced Physical Vapor Deposition Technique" Lubricants 11, no. 12: 501. https://doi.org/10.3390/lubricants11120501
APA StyleKanders, U., Kanders, K., Jansons, E., Lungevics, J., Sirants, R., Leitans, A., & Boiko, I. (2023). Simple Deconvolution Models for Evaluating the True Microhardness of Thin Nanostructured Coatings Deposited via an Advanced Physical Vapor Deposition Technique. Lubricants, 11(12), 501. https://doi.org/10.3390/lubricants11120501








