A Comparison of Hydrogen and Gasoline Piston Ring Simulations
Abstract
:1. Introduction
2. Methodology
3. Hydrodynamics
4. Lubricant Rheology
5. Asperity Contact Model
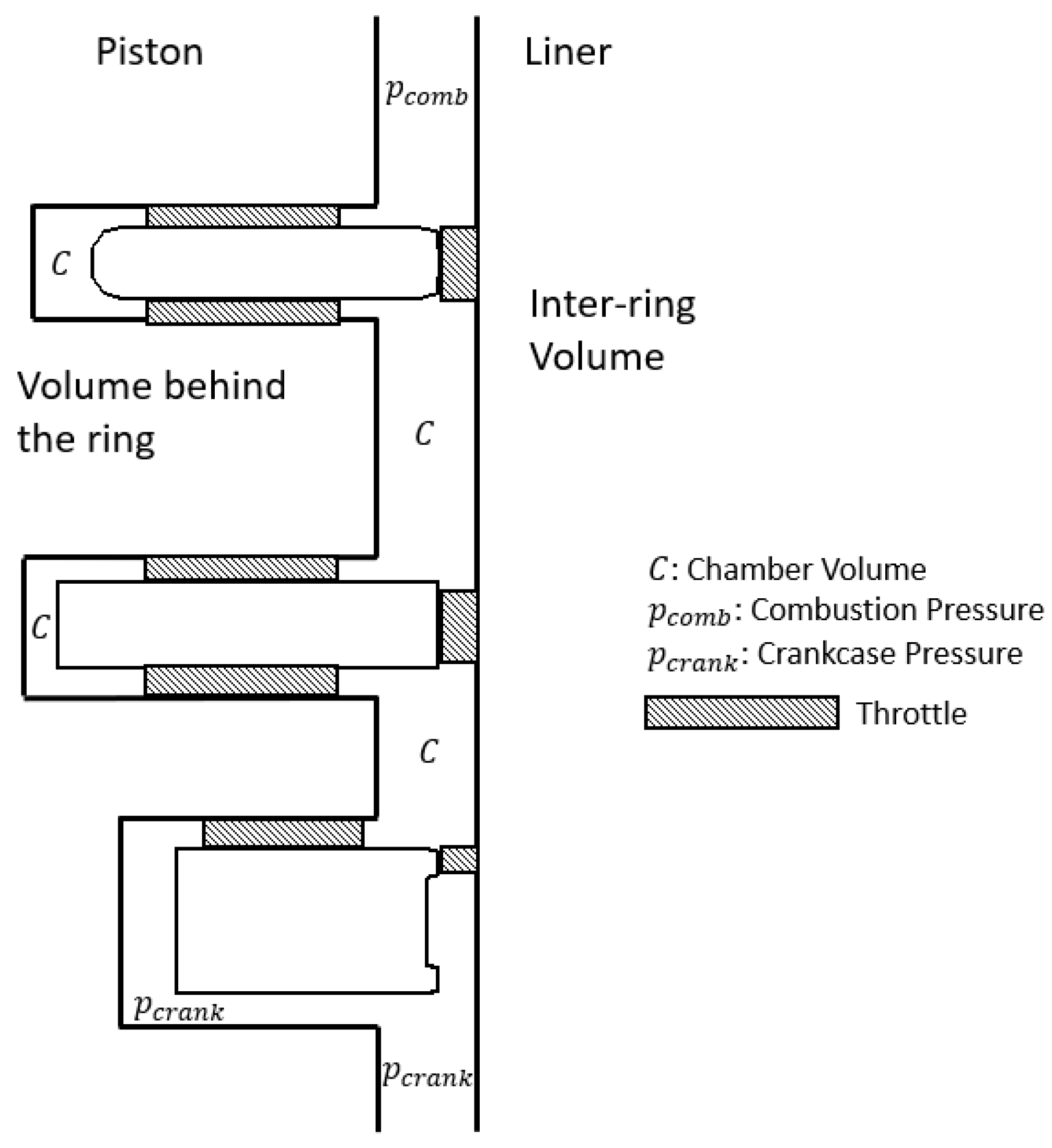
6. Gas Flow Model
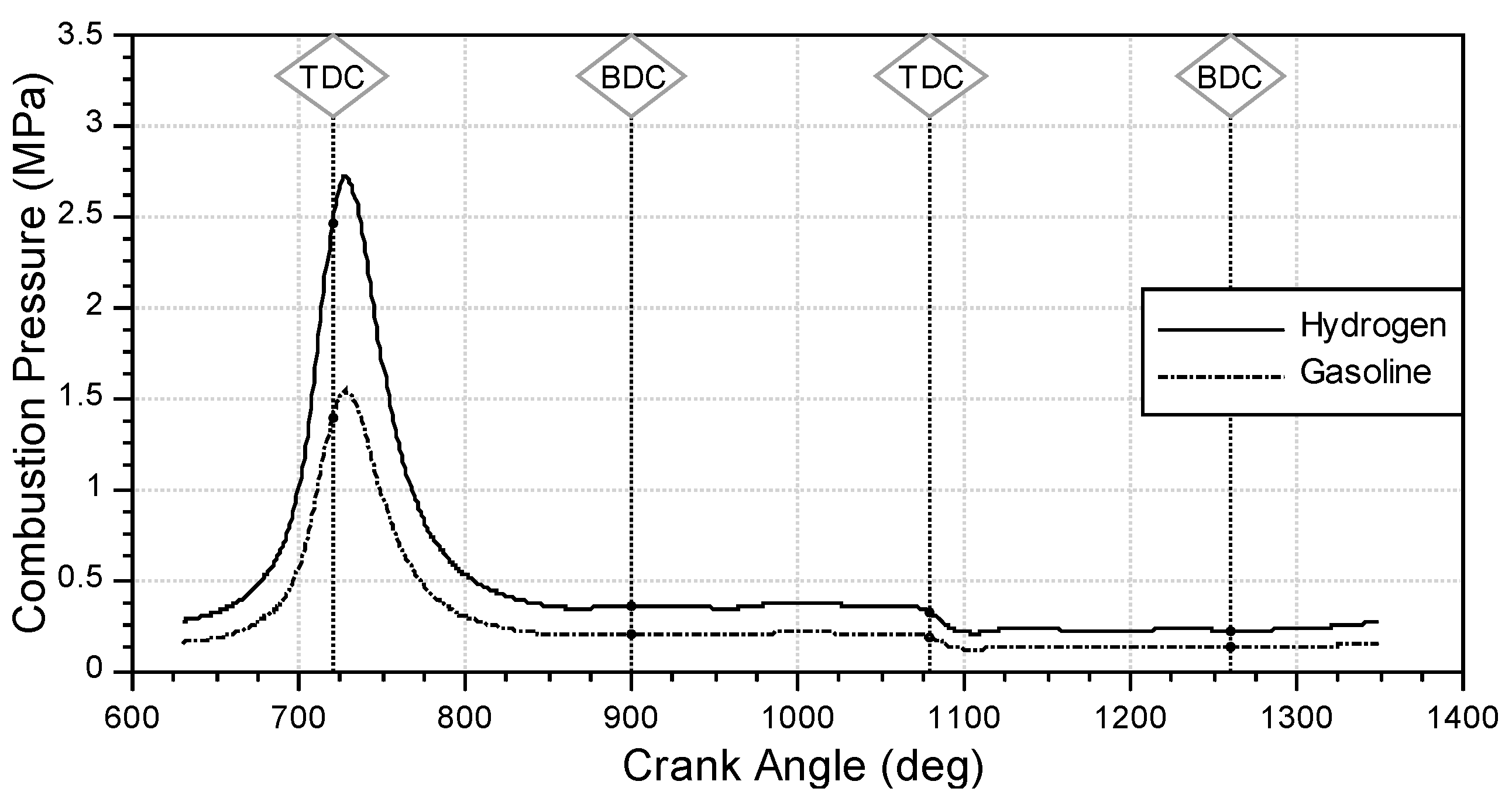
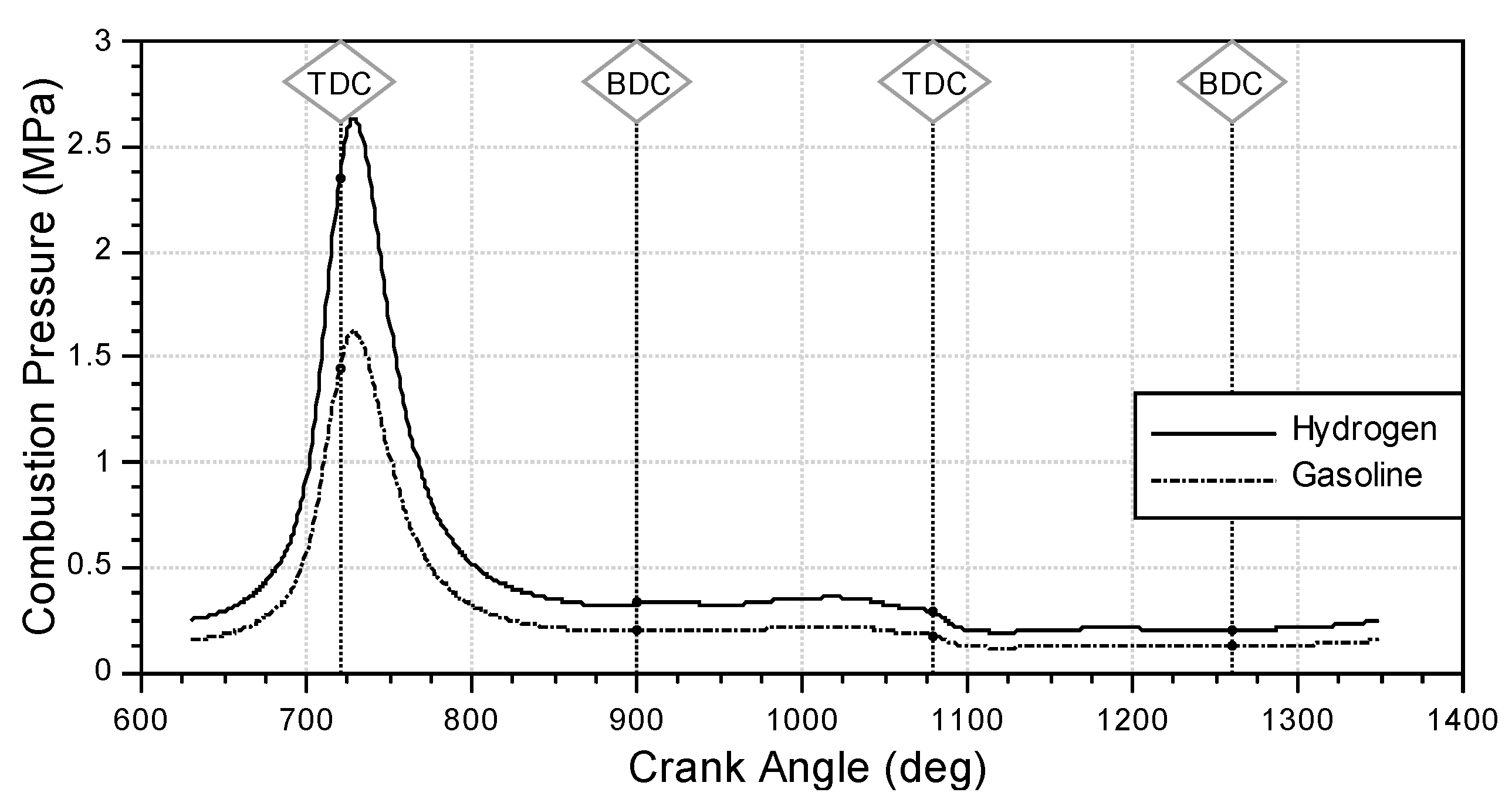
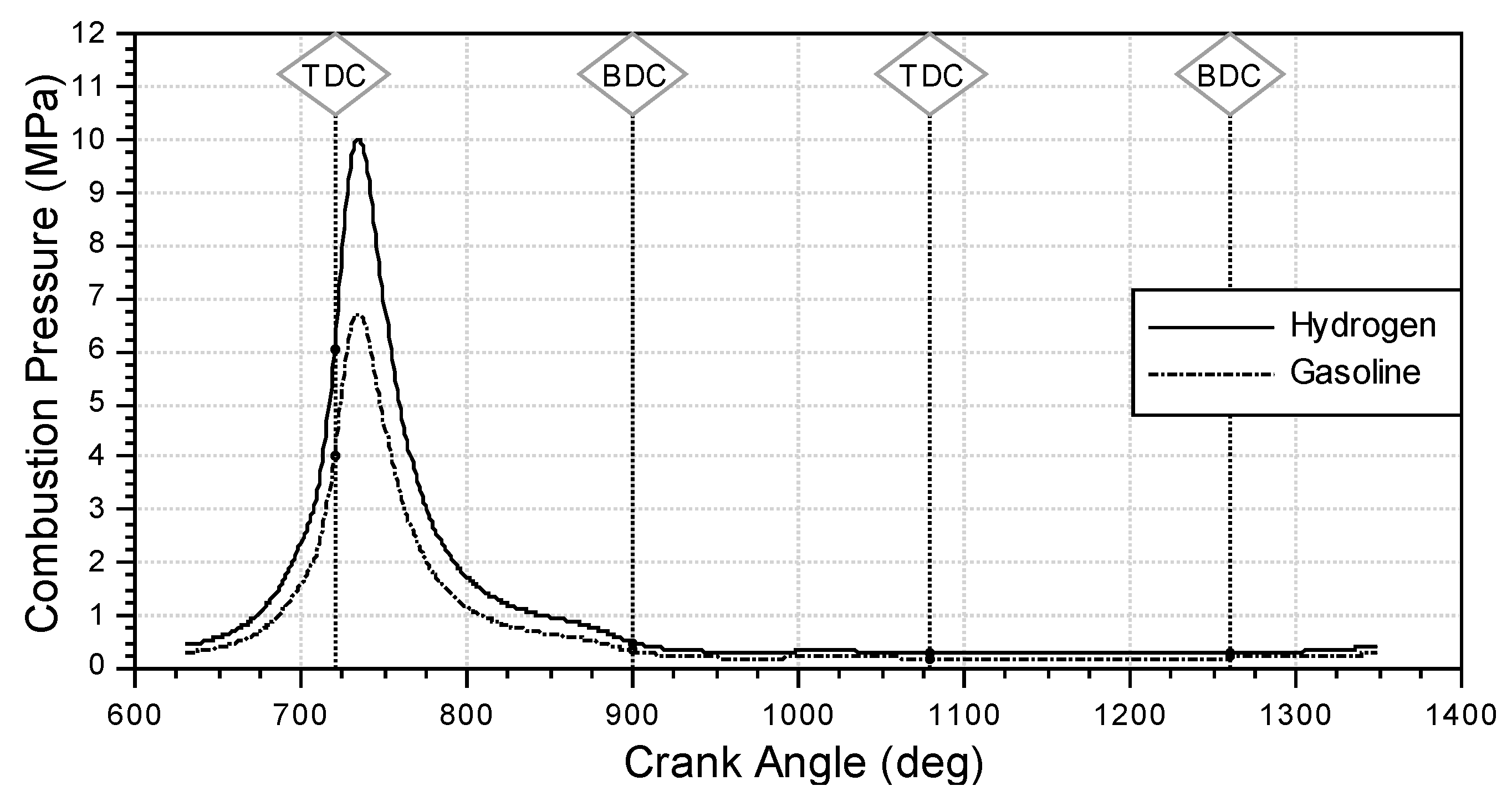
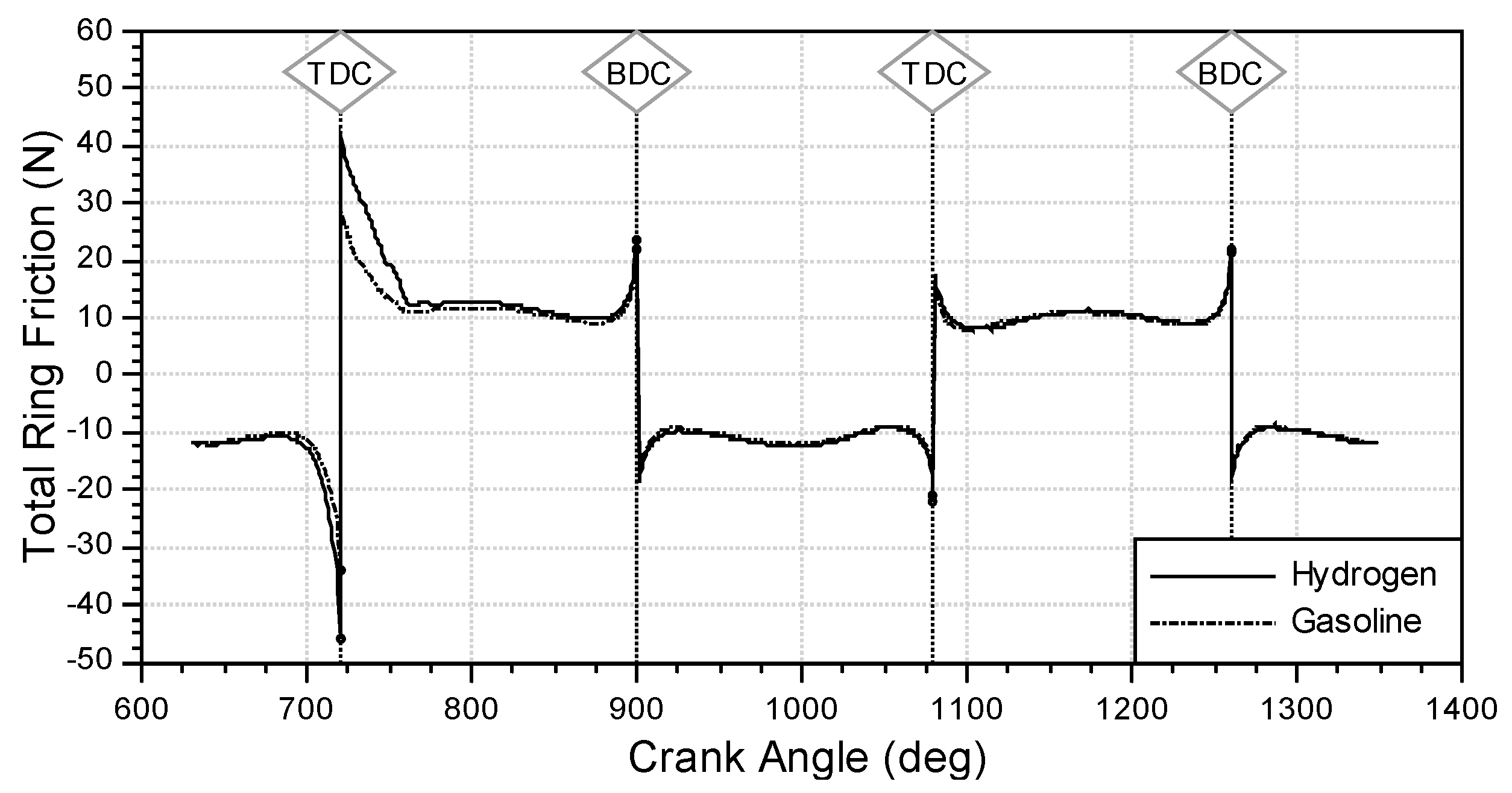
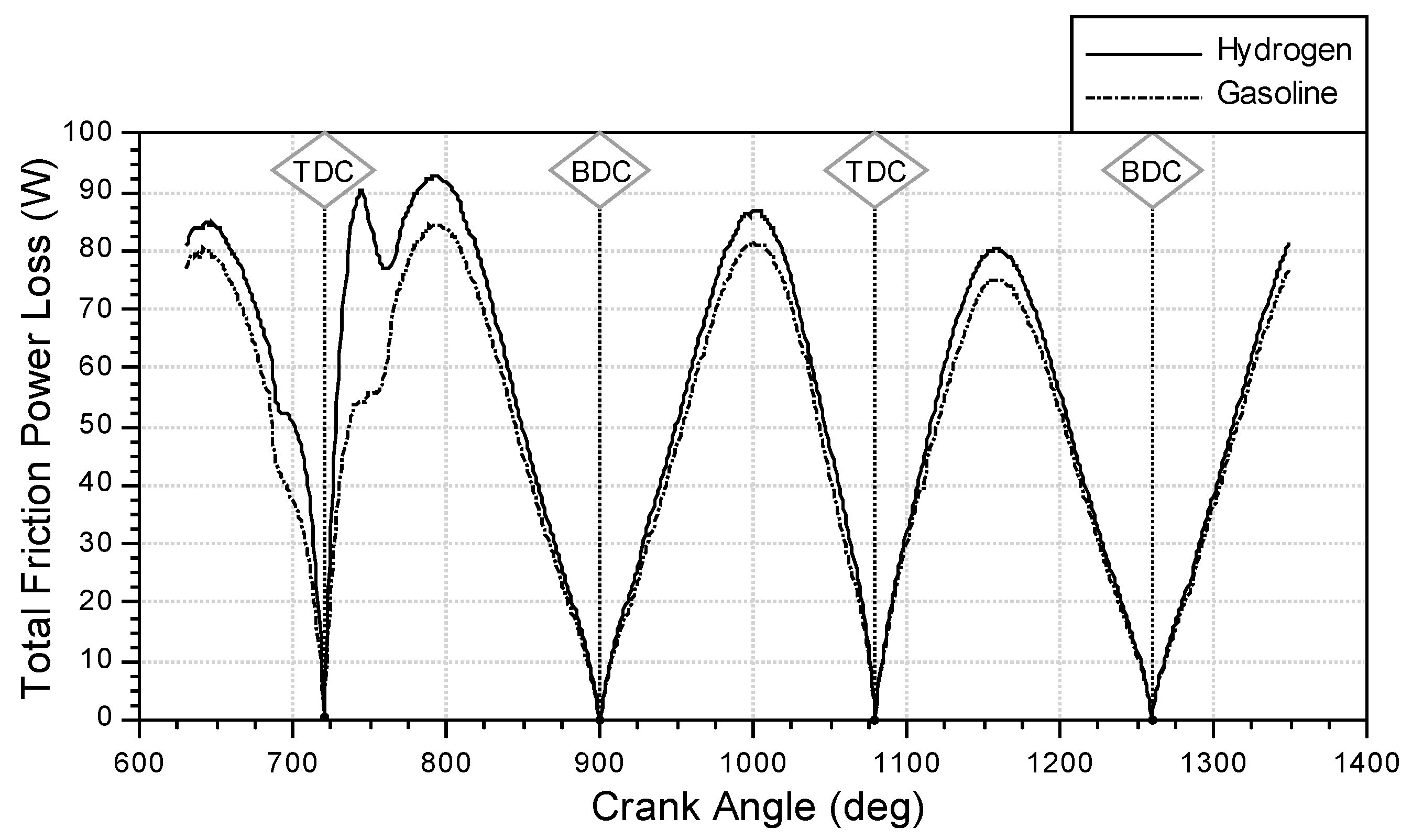
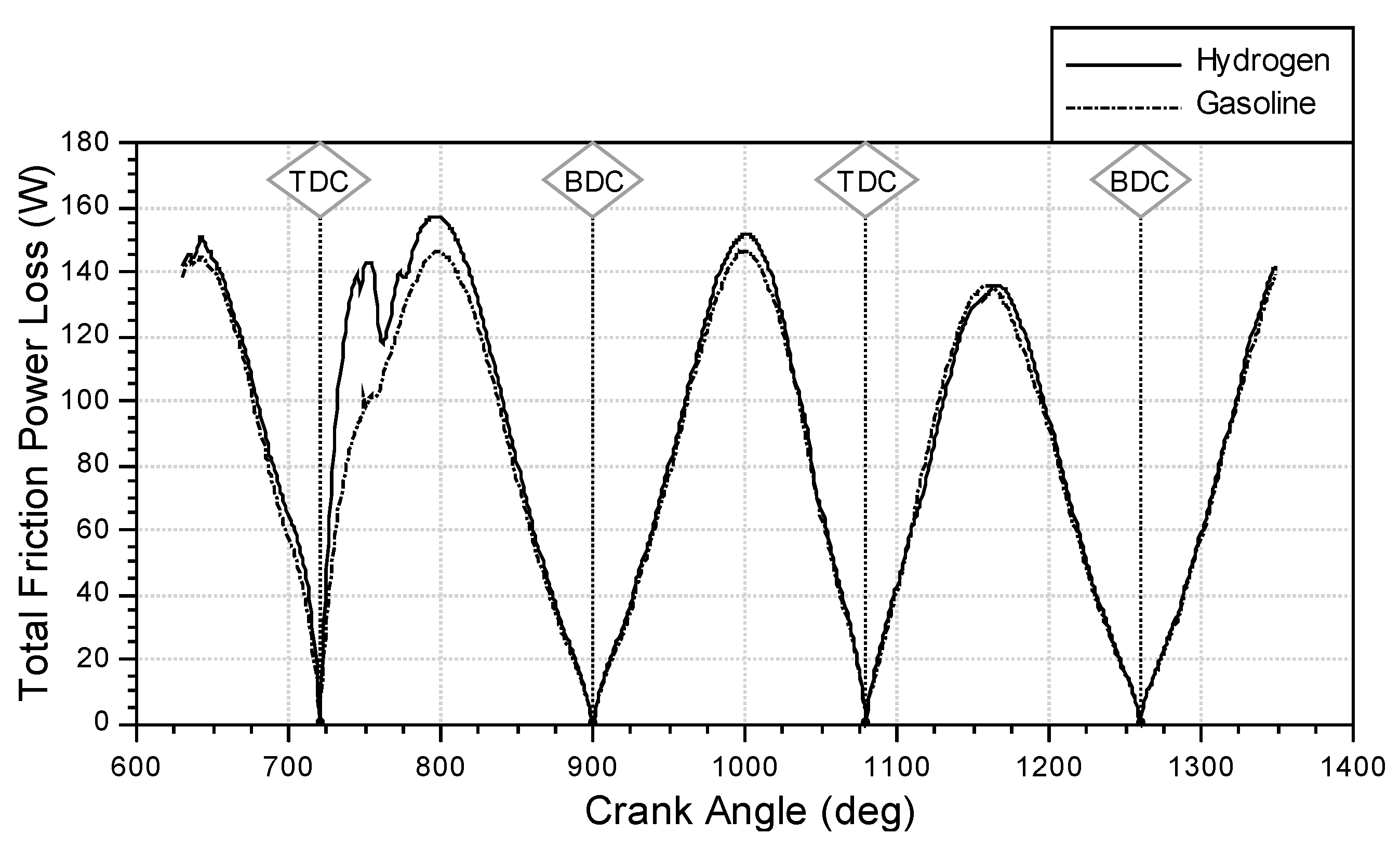
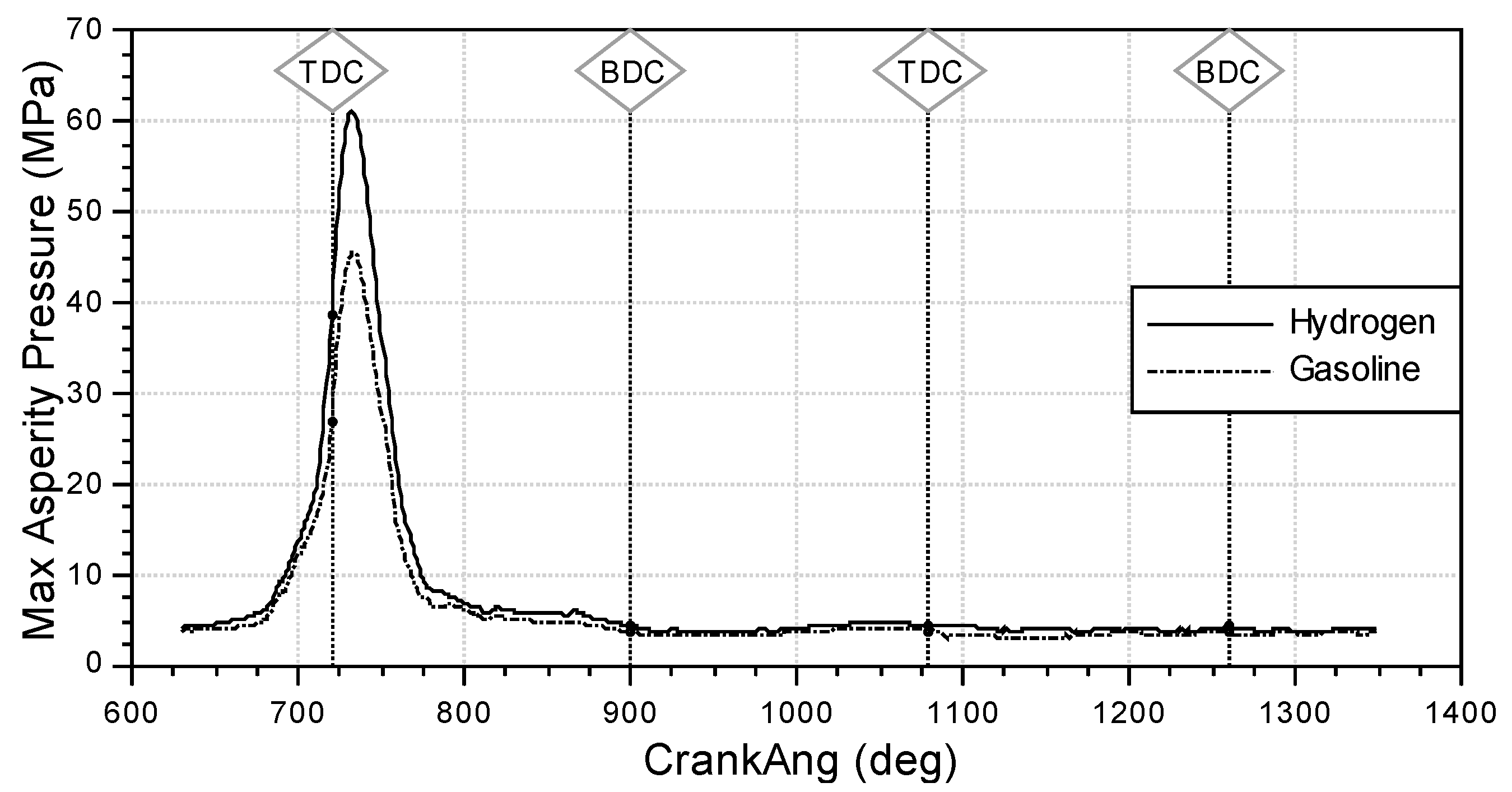
7. Results and Discussion
8. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Friction constants set to 2.718, 1000 and 1000, respectively | |
| Apparent contact area | |
| Cross-sectional area of gas flow | |
| Gas flow coefficient for ring end gap | |
| Composite Young’s modulus of elasticity | |
| Ring friction | |
| Boundary friction | |
| Function of gas mass flow | |
| Viscous friction | |
| Statistical function | |
| Lubricant film thickness | |
| Averaged lubricant film thickness | |
| Isentropic gas exponent | |
| Lubrication number (duty parameter) | |
| Reference length | |
| Gas mass flowrate | |
| Gas mass flowrate at ring end gap | |
| Change in gas mass | |
| Contact pressure | |
| Initial chamber pressure depending on stroke direction | |
| Asperity pressure | |
| Combustion pressure | |
| Crankcase pressure | |
| Chamber pressure | |
| Downstream pressure | |
| Pressure in the direction of lubricant film thickness | |
| Upstream pressure | |
| Pressure in the direction along the liner height | |
| Averaged pressure | |
| Asperity contact ratio | |
| Gas constant | |
| Time | |
| Lubricant oil temperature | |
| Chamber temperature | |
| Upstream temperature | |
| Sliding speed of surfaces 1 and 2 in direction | |
| Difference in sliding speed of surfaces 1 and 2 in direction | |
| Direction along the liner height | |
| Greek Symbols | |
| Asperity mean summit radius | |
| Number of asperities per unit area of contact | |
| Lubricant dynamic viscosity | |
| Fill ratio in Reynolds equation | |
| Asperity shear stress | |
| Dimensionless clearance height | |
| Friction coefficient | |
| Abrasive friction coefficient | |
| Adhesive friction coefficient | |
| Lubricant density | |
| Surface roughness (r.m.s.) | |
| Combined shear flow factor | |
| Pressure flow factor in direction | |
| Gas flow coefficient for orifice flow | |
References
- King, J. The King Review of Low-Carbon Cars: Part 1: The Potential for CO2 Reduction; Office of Public Sector Information, HM Treasury, HMSO: Richmond, UK, 2007. [Google Scholar]
- Capros, P.; De Vita, A.; Tasios, N.; Papadopoulos, D.; Siskos, P.; Apostolaki, E.; Zamapara, M.; Paroussos, L.; Fragiadakis, K.; Kouvaritakis, N.; et al. EU Energy, Transport and GHG Emissions—Trends to 2050. In European Commission, Directorate-General for Energy, Directorate-General for Climate Action and Directorate-General for Mobility and Transport; EU Publications: Luxembourg, 2013. [Google Scholar]
- Onorati, A.; Payri, R.; Vaglieco, B.M.; Agarwal, A.K.; Bae, C.; Bruneaux, G.; Canakci, M.; Gavaises, M.; Günthner, M.; Hasse, C.; et al. The role of hydrogen for future internal combustion engines. Int. J. Engine Res. 2022, 23, 529–540. [Google Scholar] [CrossRef]
- Ogden, J.M. Prospects for Building a Hydrogen Energy Infrastructure. Annu. Rev. Energy Environ. 1999, 24, 227–279. [Google Scholar] [CrossRef]
- Bockris, J.O. A Hydrogen Economy. In Comprehensive Treatise of Electrochemistry; Plenum Press: New York, NY, USA, 1981; pp. 505–526. [Google Scholar]
- Du, H.; Chen, Z.; Peng, B.; Southworth, F.; Ma, S.; Wang, Y. What drives CO2 emissions from the transport sector? A linkage analysis. Energy 2019, 175, 195–204. [Google Scholar] [CrossRef]
- Wróbel, K.; Wróbel, J.; Tokarz, W.; Lach, J.; Podsadni, K.; Czerwiński, A. Hydrogen Internal Combustion Engine Vehicles: A Review. Energies 2022, 15, 8937. [Google Scholar] [CrossRef]
- Sopena, C.; Diéguez, P.M.; Sáinz, D.; Urroz, J.C.; Guelbenzu, E.; Gandía, L.M. Conversion of a commercial spark ignition engine to run on hydrogen: Performance comparison using hydrogen and gasoline. Int. J. Hydrogen Energy 2010, 35, 1420–1429. [Google Scholar] [CrossRef]
- Sun, D.; Liu, F. Research on the Performance and Emission of a Port Fuel Injection Hydrogen Internal Combustion Engine. In Proceedings of the 2011 International Conference on Computer Distributed Control and Intelligent Environmental Monitoring, Changcha, China, 19–20 February 2011; pp. 963–966. [Google Scholar]
- Verhelst, S.; Sierens, R.; Verstraeten, S. A Critical Review of Experimental Research on Hydrogen Fueled SI Engines; Technical Paper 2006-01-0430; SAE: Warrendale, PA, USA, 2006; Volume 115, pp. 264–274. [Google Scholar]
- Verhelst, S.; Wallner, T. Hydrogen-fueled internal combustion engines. Prog. Energy Combust. Sci. 2009, 35, 490–527. [Google Scholar] [CrossRef]
- Stone, R. Introduction to Internal Combustion Engines; Springer: London, UK, 1999; Volume 3, pp. 1–16. [Google Scholar]
- Turner, J.W.G.; Popplewell, A.; Patel, R.; Johnson, T.R.; Darnton, N.J.; Richardson, S.; Bredda, S.W.; Tudor, R.J.; Bithell, C.I.; Jackson, R.; et al. Ultra Boost for Economy: Extending the Limits of Extreme Engine Downsizing. SAE Int. J. Engines 2014, 7, 387–417. [Google Scholar] [CrossRef]
- Lee, J.; Park, C.; Kim, Y.; Choi, Y.; Bae, J.; Lim, B. Effect of turbocharger on performance and thermal efficiency of hydrogen-fueled spark ignition engine. Int. J. Hydrogen Energy 2019, 44, 4350–4360. [Google Scholar] [CrossRef]
- Verhelst, S.; Sierens, R. Hydrogen engine-specific properties. Int. J. Hydrogen Energy 2001, 26, 987–990. [Google Scholar] [CrossRef]
- Allen, E.; Questa, H.; Walker, J.; Mohammadpour, M.; Bewsher, S.R.; Offner, G. Simulating the tribological performance of new water-based and mineral-oil-based lubricants for a journal bearing within an electrified powertrain. In Proceedings of the ÖTG-Symposium 2022, Wiener Neustadt, Austria, 15 September 2022. [Google Scholar]
- Wróblewski, P. Investigation of energy losses of the internal combustion engine taking into account the correlation of the hydrophobic and hydrophilic. Energy 2023, 264, 126002. [Google Scholar] [CrossRef]
- Dziubak, T.; Dziubak, S.D. A study on the effect of inlet air pollution on the engine component wear and operation. Energies 2022, 15, 1182. [Google Scholar] [CrossRef]
- Oh, S.; Park, C.; Nguyen, D.; Kim, S.; Kim, Y.; Choi, Y.; Lee, J. Investigation on the operable range and idle condition of hydrogen-fueled spark ignition engine for unmanned aerial vehicle (UAV). Energy 2021, 237, 121645. [Google Scholar] [CrossRef]
- Barthélémy, H. Compatibility of Metallic Materials with Hydrogen—Review of the present knowledge. In Proceedings of the 16th World Hydrogen Energy Conference, Lyon, France, 13–16 June 2006. [Google Scholar]
- Offner, G.; Knaus, O. A generic friction model for radial slider bearing simulation considering elastic and plastic deformation. Lubricants 2015, 3, 522–538. [Google Scholar] [CrossRef]
- AVL List GmbH. AVL List GmbH: AVL EXCITE Piston & Rings Theory: Version 2023; Software Documentation; AVL List GmbH: Graz, Austria, 2023. [Google Scholar]
- Herbst, H.; Priebsch, H. Simulation of piston ring dynamics and their effect on oil consumption. SAE Trans. 2000, 109, 862–873. [Google Scholar]
- Priebsch, H.-H.; Herbst, H.M. Simulation of Effects of Piston Ring Parameters on Ring Movement, Friction, Blow-by and LOC. MTZ Mot. Z. 1999, 60, 772–779. [Google Scholar]
- Götze, A.; Jaitner, D. Combined experimental and simulative approach for friction loss optimization od DLC coated piston rings. Automot. Engine Technol. 2022, 7, 283–293. [Google Scholar] [CrossRef]
- Mahmoud, K.G.; Knaus, O.; Parikyan, T.; Offner, G.; Sklepic, S. An integrated model for the performance of piston ring pack in internal combustion engines. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2018, 232, 371–384. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. An Average Flow Model for Determining Effects of Three-Dimensional Roughness on Partial Hydrodynamic Lubrication. J. Lubr. Technol. 1978, 100, 12–17. [Google Scholar] [CrossRef]
- Patir, N.; Cheng, H.S. Application of average flow model to lubrication between rough sliding surfaces. J. Lubr. Technol. 1979, 101, 220–229. [Google Scholar] [CrossRef]
- Jakobsson, B.; Floberg, L. The Finite Journal Bearing Considering Vaporization; Transactions of Chalmers University of Technology: Gothenburg, Sweden, 1957; Volume 190, pp. 1–116. [Google Scholar]
- Olsson, K.O. Cavitation in Dynamically Loaded Bearings; Transactions of Chalmers University of Technology: Gothenburg, Sweden, 1965; Volume 308. [Google Scholar]
- Greenwood, J.A.; Tripp, J.H. The elastic contact of rough spheres. J. Appl. Mech. 1967, 34, 153–159. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Tripp, J.H. The contact of two nominally flat rough surfaces. Proc. IMechE 1970, 185, 625–634. [Google Scholar] [CrossRef]
- Tian, T. Modeling the Performance of the Piston Ring-Pack in Internal Combustion Engines. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1997. [Google Scholar]







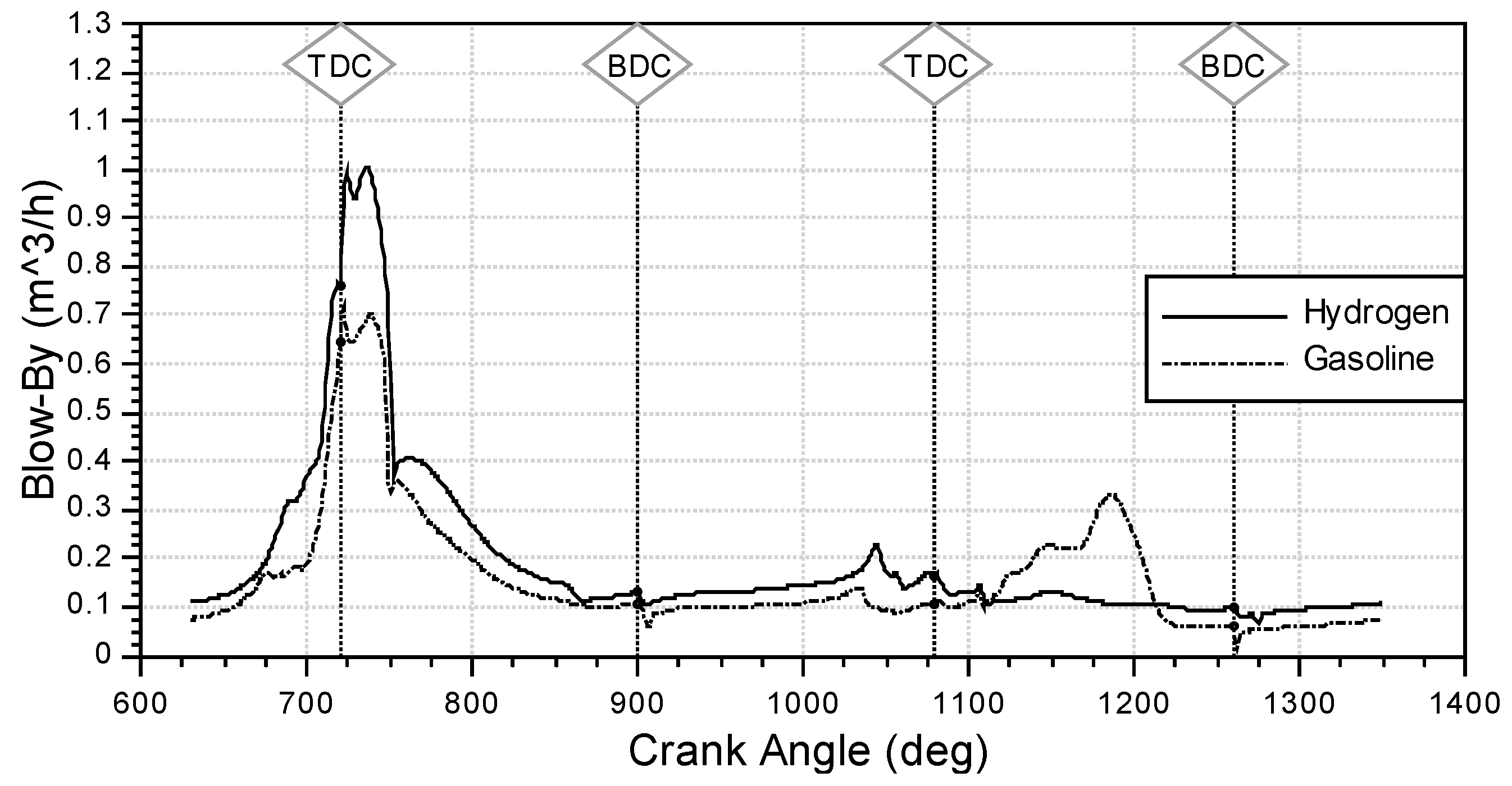
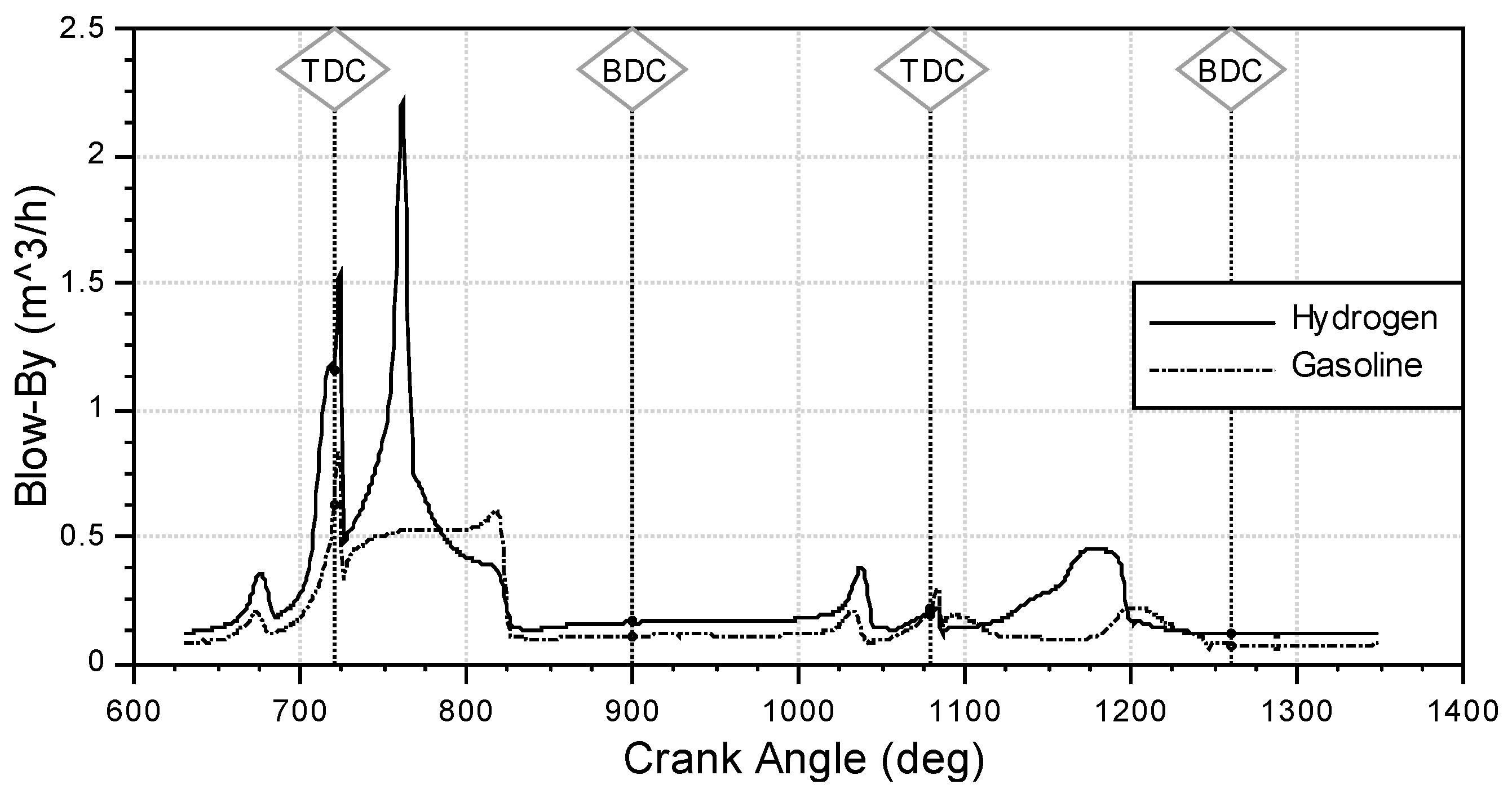
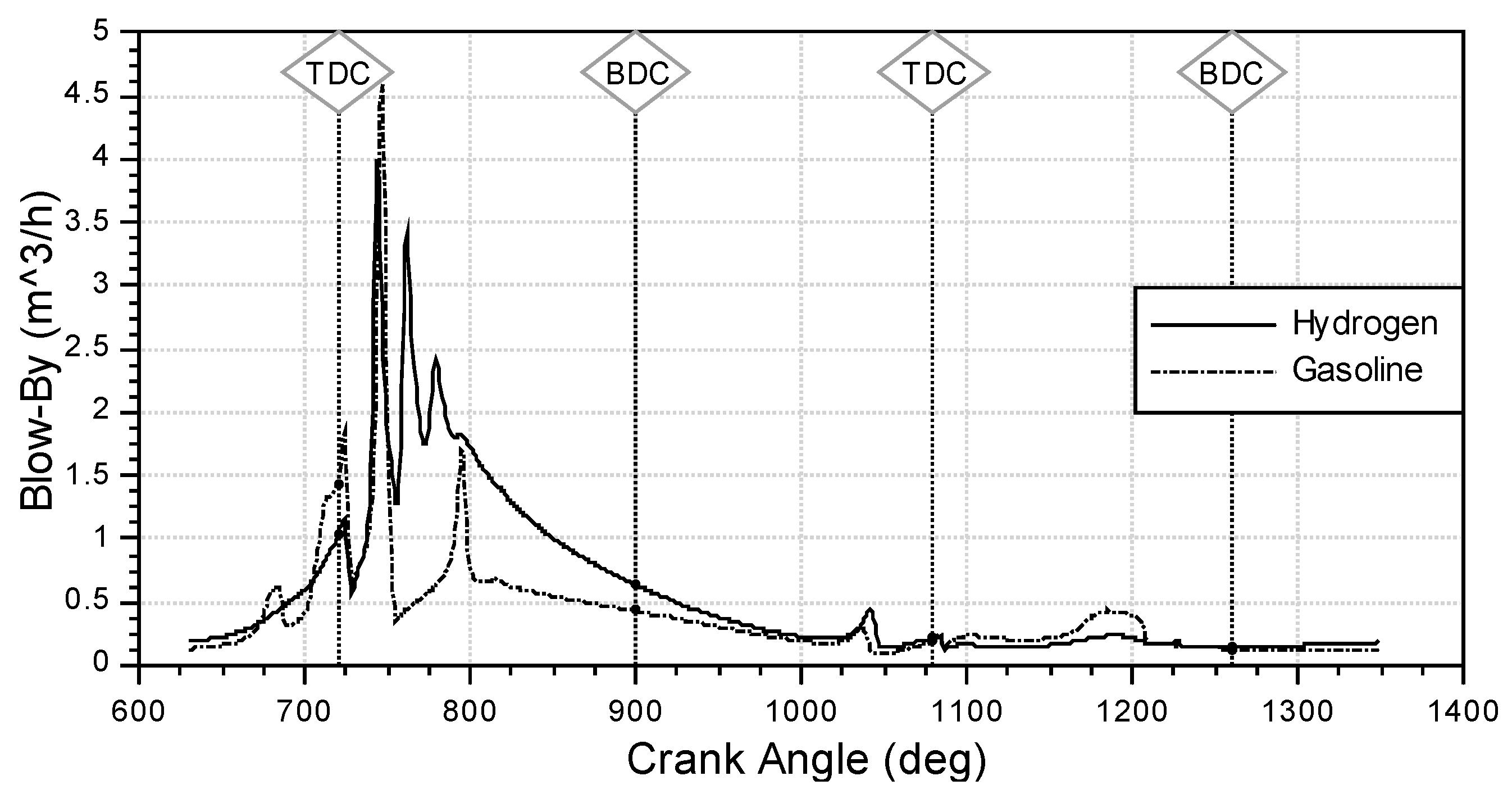
| Parameters | Values | Units |
|---|---|---|
| Density | 872 | kg/m3 |
| Molar Mass | 422 | kg/kmol |
| 3.314 × 10−5 | Pa·s | |
| 1384 | °C | |
| 146.9 | °C | |
| Temperature at top of Liner | 140 | °C |
| Temperature at bottom of Liner | 110 | °C |
| Parameters | Values | Units |
|---|---|---|
| Bore Diameter | 76 | mm |
| Crank Radius | 37.8 | mm |
| Conrod Length | 144 | mm |
| Engine Type | 4-Stroke | Not Applicable |
| Liner Height | 120 | mm |
| Parameters | Values | Units |
|---|---|---|
| Liner Material | Grey Cast Iron | N/A |
| Modulus of Elasticity of the Liner Material | 114 | GPa |
| Poisson Ratio of the Liner Material | 0.3 | [-] |
| Density of the Liner Material | 7200 | kg/m3 |
| Ring Material | Steel SAE 9254 | N/A |
| Modulus of Elasticity of the Ring Material | 203 | GPa |
| Poisson Ratio of the Ring Material | 0.3 | [-] |
| Density of the Ring Material | 7200 | kg/m3 |
| Abrasive friction coefficient | 0.02 | [-] |
| adhesive friction coefficient | 0.1 | [-] |
| Reference length | 10 | μm |
| Roughness parameter () | 0.04 | [-] |
| Measure of asperity gradient () | 0.001 | [-] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bewsher, S.R.; Offner, G. A Comparison of Hydrogen and Gasoline Piston Ring Simulations. Lubricants 2023, 11, 444. https://doi.org/10.3390/lubricants11100444
Bewsher SR, Offner G. A Comparison of Hydrogen and Gasoline Piston Ring Simulations. Lubricants. 2023; 11(10):444. https://doi.org/10.3390/lubricants11100444
Chicago/Turabian StyleBewsher, Stephen Richard, and Günter Offner. 2023. "A Comparison of Hydrogen and Gasoline Piston Ring Simulations" Lubricants 11, no. 10: 444. https://doi.org/10.3390/lubricants11100444
APA StyleBewsher, S. R., & Offner, G. (2023). A Comparison of Hydrogen and Gasoline Piston Ring Simulations. Lubricants, 11(10), 444. https://doi.org/10.3390/lubricants11100444






