Ramification of Hall and Mixed Convective Radiative Flow towards a Stagnation Point into the Motion of Water Conveying Alumina Nanoparticles Past a Flat Vertical Plate with a Convective Boundary Condition: The Case of Non-Newtonian Williamson Fluid
Abstract
1. Introduction
2. Problem Formulation
3. Results and Discussion
4. Conclusions
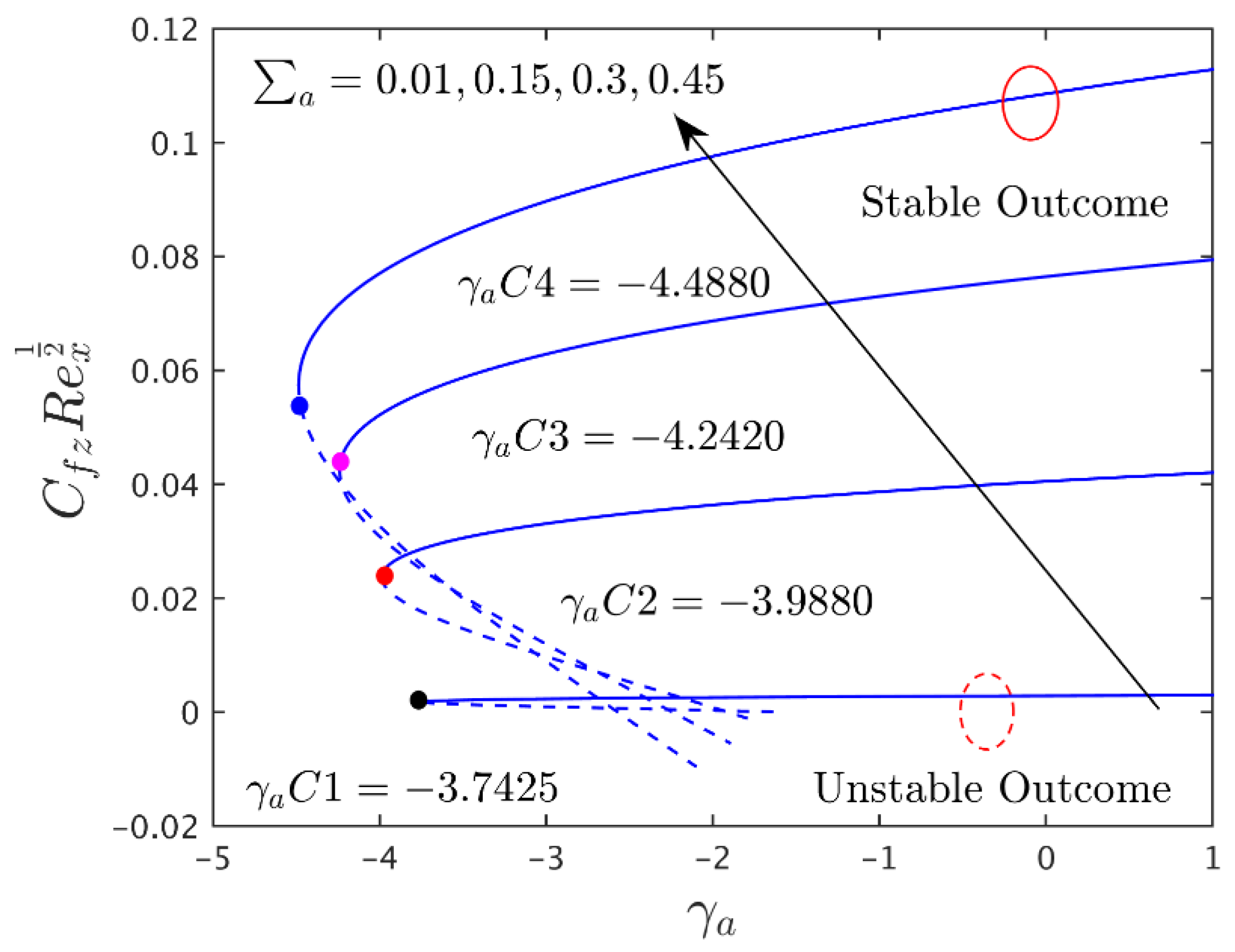
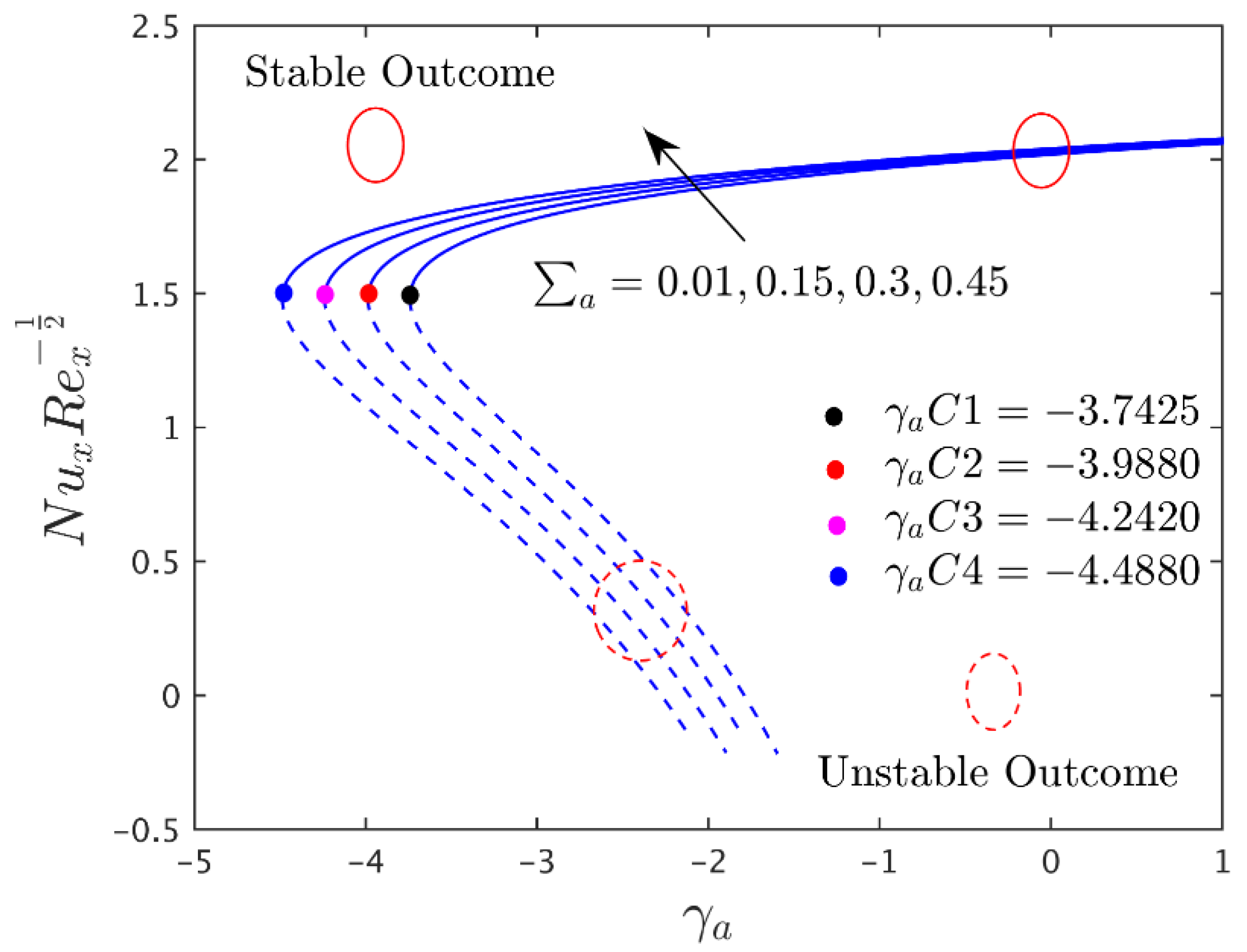
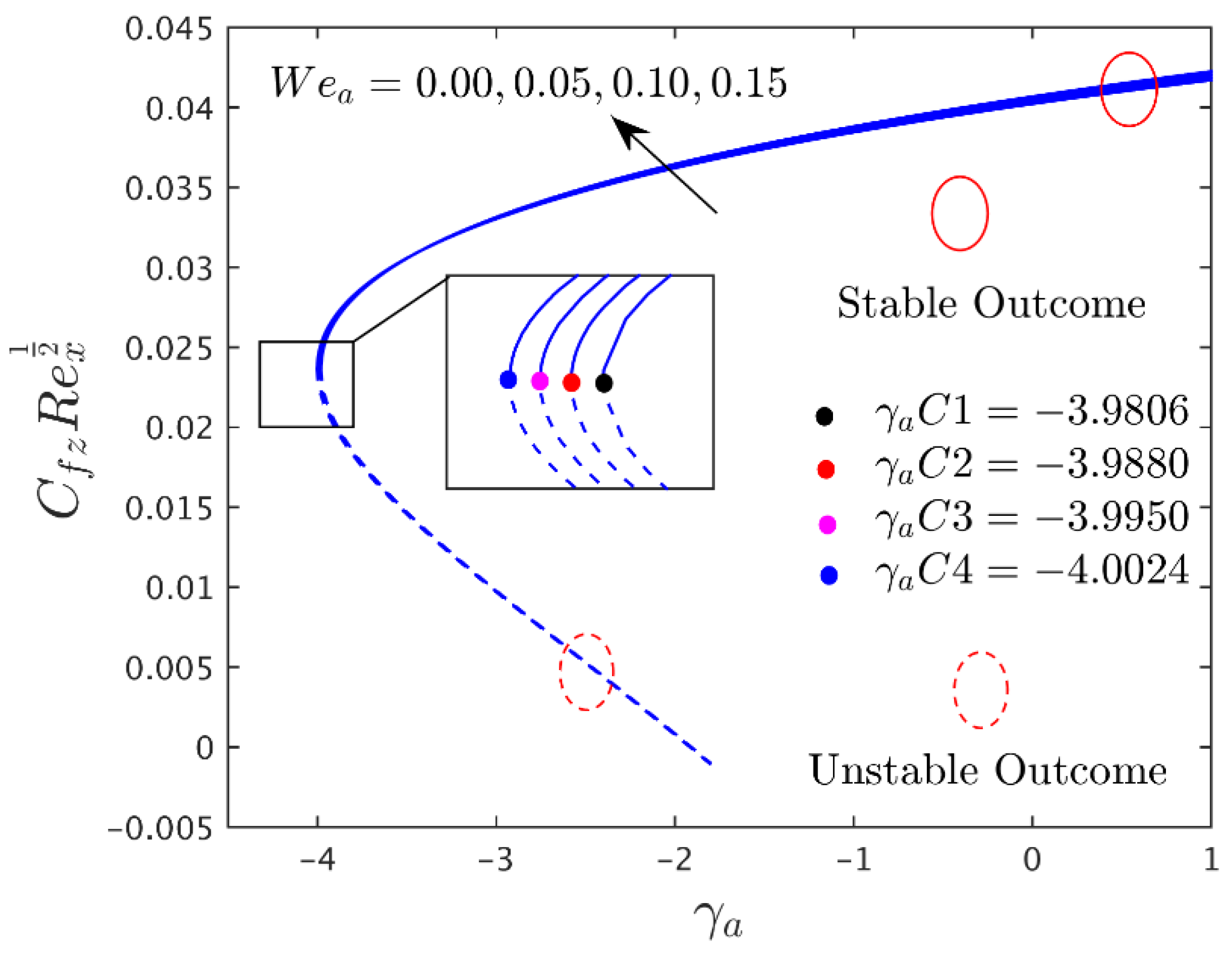
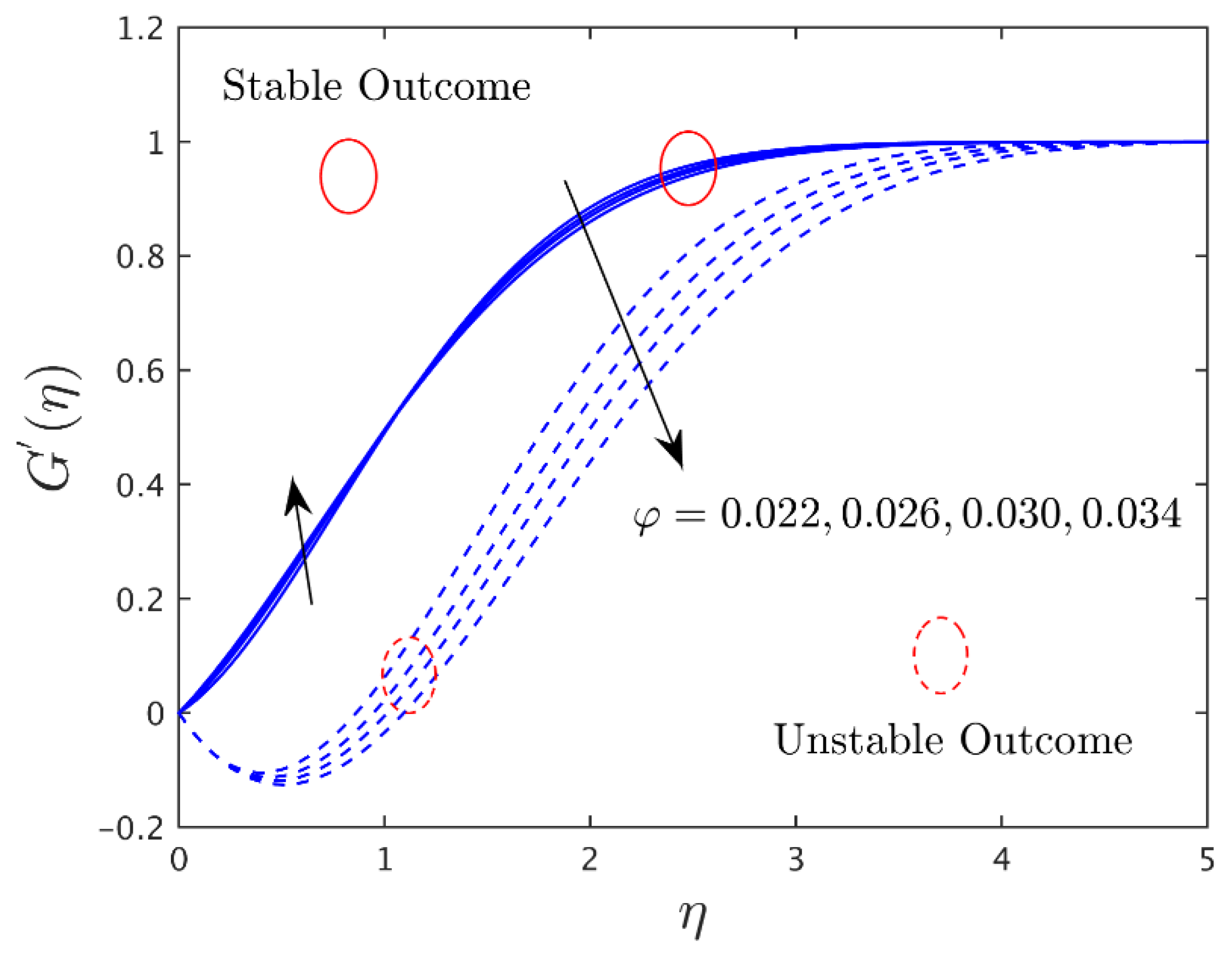
- For the stable branch solutions, the magnetic parameter causes an increase in the axial shear stress and the rate of heat transfer, while it has an entirely different effect on the branch of unstable solutions.
- Due to the higher values of the magnetic parameter, the transverse shear stress increases for upper and lower branches.
- Due to the bigger values of the magnetic parameter, the magnitude of the bifurcation values was increased.
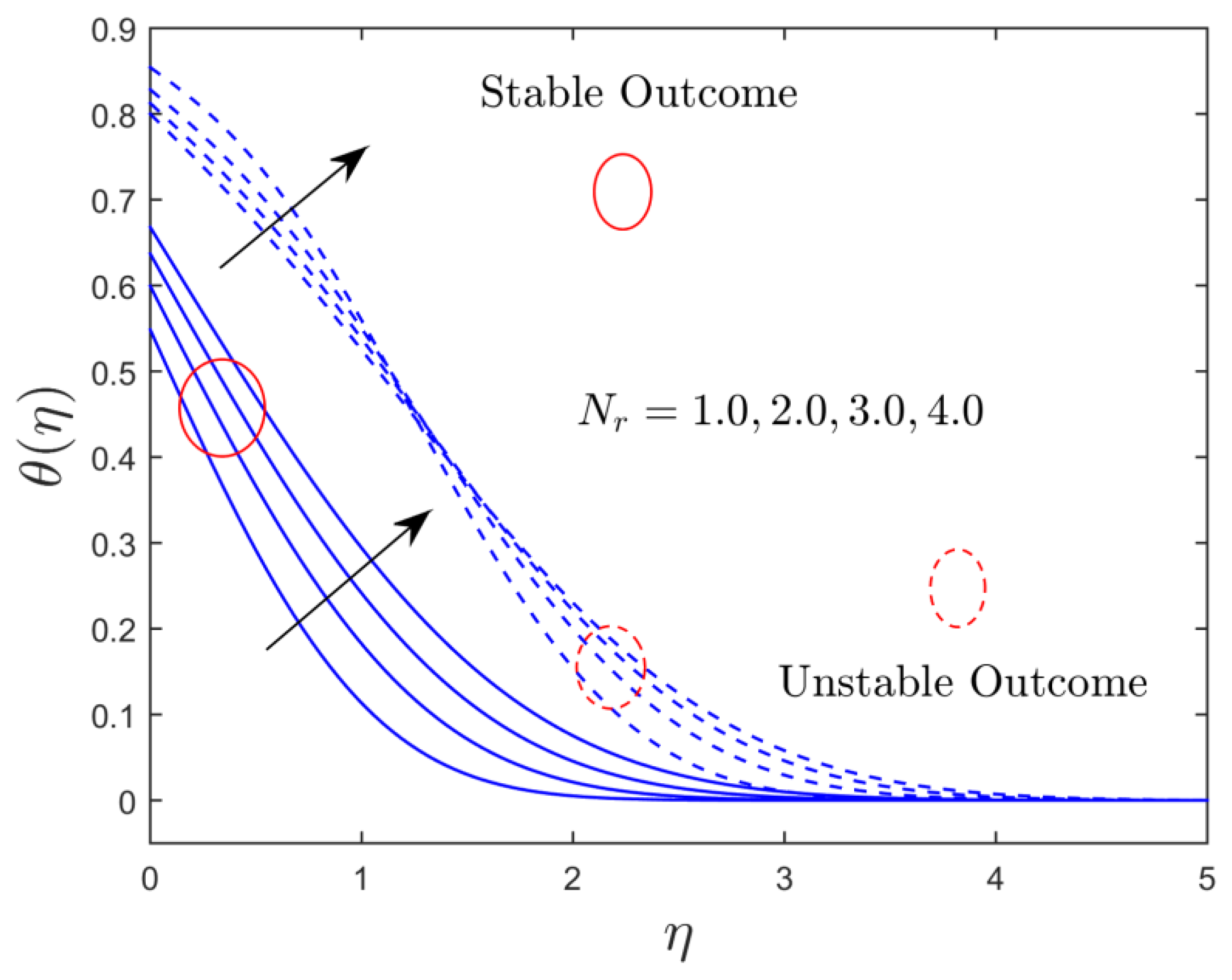
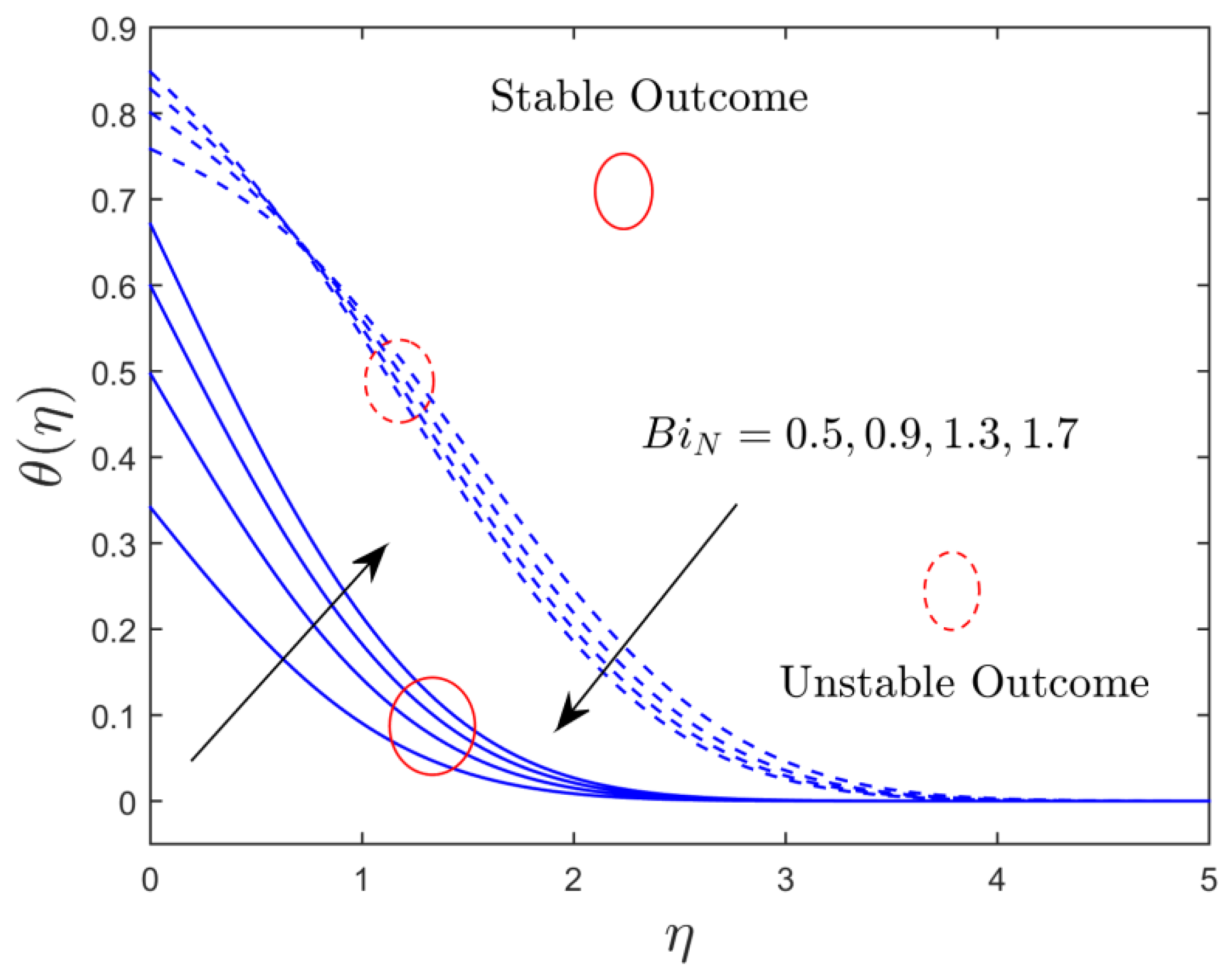
- The radiation parameter is more significantly impacted by the escalating temperature distribution in both solution branches.
- Due to the greater Hall parameter, the axial shear stress falls, while the transverse shear stress increases.
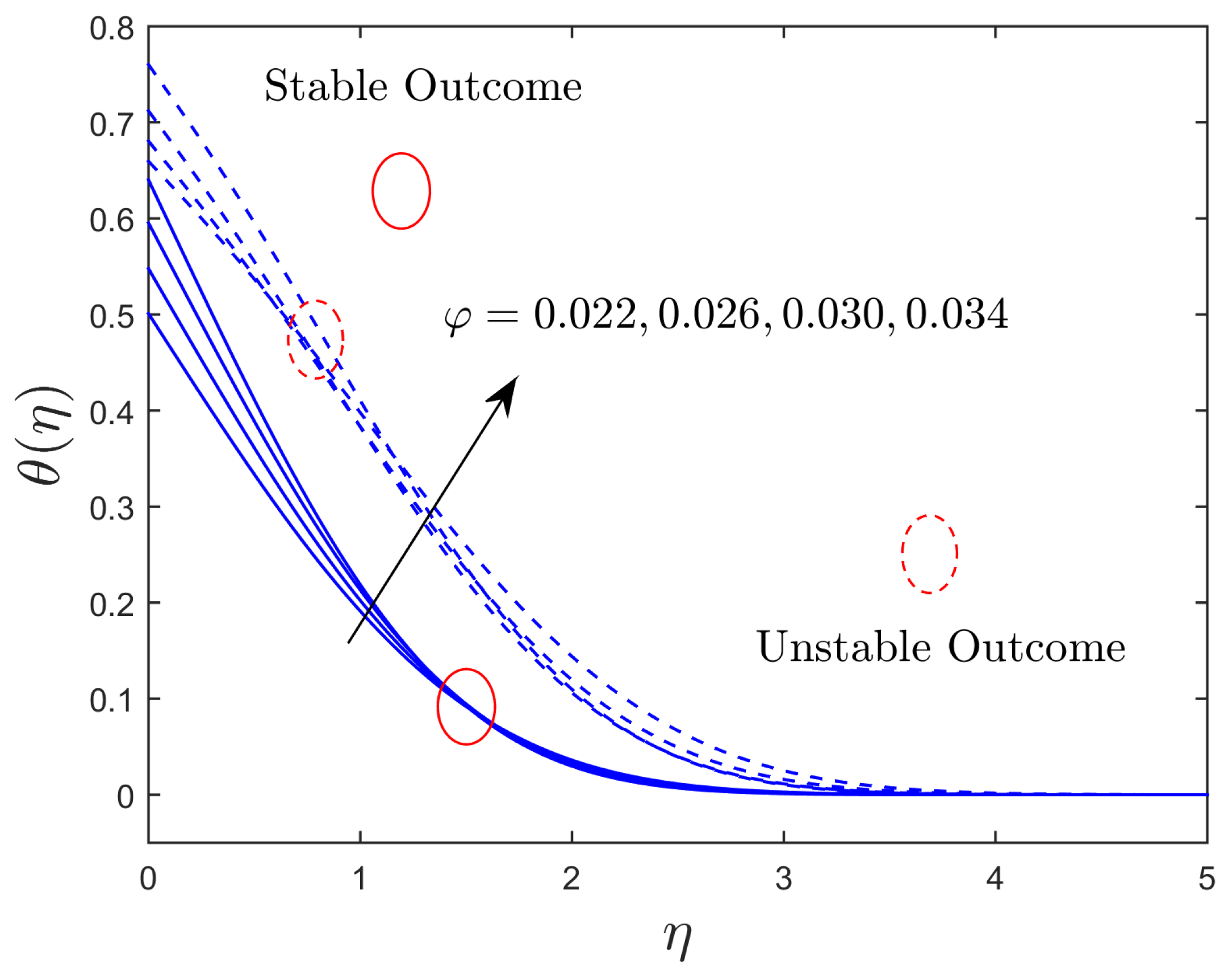
- The heat transfer rate is increased by the nanoparticles for stable branch solutions, while it is decreased for unstable branch solutions.
- The Williamson constraint augments the shear stresses, as well as the heat transfer.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Williamson, R.V. The flow of pseudoplastic materials. Ind. Eng. Chem. 1929, 21, 1108–1111. [Google Scholar] [CrossRef]
- Nadeem, S.; Hussain, S.T.; Lee, C. Flow of a Williamson fluid over a stretching sheet. Braz. J. Chem. Eng. 2013, 30, 619–625. [Google Scholar] [CrossRef]
- Hayat, T.; Shafiq, A.; Alsaedi, A. Hydromagnetic boundary layer flow of Williamson fluid in the presence of thermal radiation and Ohmic dissipation. Alex. Eng. J. 2016, 55, 2229–2240. [Google Scholar] [CrossRef]
- Shawky, H.M.; Eldabe, N.T.; Kamel, K.A.; Abd-Aziz, E.A. MHD flow with heat and mass transfer of Williamson nanofluid over stretching sheet through porous medium. Microsyst. Technol. 2018, 25, 1155–1169. [Google Scholar] [CrossRef]
- Kho, Y.B.; Hussanan, A.; Mohamed, M.K.A.; Salleh, M.Z. Heat and mass transfer analysis on flow of Williamson nanofluid with thermal and velocity slips: Buongiorno model. Propuls. Power Res. 2019, 8, 243–252. [Google Scholar] [CrossRef]
- Qureshi, M.A. Numerical simulation of heat transfer flow subject to MHD of Williamson nanofluid with thermal radiation. Symmetry 2020, 13, 10. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F. Cattaneo-Christov Double diffusion (CCDD) and magnetized stagnation point flow of non-Newtonian fluid with internal resistance of particles. Phys. Scr. 2020, 95, 125002. [Google Scholar] [CrossRef]
- Chandel, S.; Sood, S. Unsteady flow of Williamson fluid under the impact of prescribed surface temperature (PST) and prescribed heat flux (PHF) heating conditions over a stretching surface in a porous enclosure. Z. Angew. Math. Mech. 2022, 102, e202100128. [Google Scholar] [CrossRef]
- Choi, S.U.S. Enhancing Thermal Conductivity of Fluids with Nanoparticles. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, San Francisco, CA, USA, 12–17 November 1995; Volume 66, pp. 99–105. [Google Scholar]
- Xiao, B.; Wang, W.; Zhang, X.; Long, G.; Fan, J.; Chen, H.; Deng, L. A novel fractal solution for permeability and Kozeny-Carman constant of fibrous porous media made up of solid particles and porous fibers. Powder Technol. 2019, 349, 92–98. [Google Scholar] [CrossRef]
- Liang, M.; Fu, C.; Xiao, B.; Luo, L.; Wang, Z. A fractal study for the effective electrolyte diffusion through charged porous media. Int. J. Heat Mass Transf. 2019, 137, 365–371. [Google Scholar] [CrossRef]
- Ekiciler, R. Effects of novel hybrid nanofluid (TiO2–Cu/EG) and geometrical parameters of triangular rib mounted in a duct on heat transfer and flow characteristics. J. Therm. Anal. Calorim. 2021, 143, 1371–1387. [Google Scholar] [CrossRef]
- Sundar, L.S.; Ramana, E.V.; Said, Z.; Pereira, A.M.B.; Sousa, A.C.M. Heat transfer of rGO/Co3O4 hybrid nanomaterials-based nanofluids and twisted tape configuration in a tube. J. Therm. Sci. Eng. Appl. 2021, 13, 031004. [Google Scholar] [CrossRef]
- Masuda, H.; Ebata, A.; Teramae, K.; Hishinuma, N. Alteration of thermal conductivity and viscosity of liquid by dispersing ultra-fine particles. Netsu Bussei 1993, 7, 227–233. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles. In ASME International Mechanical Engineering Congress & Exposition, San Francisco, CA, USA, 12–17 November 1995; Argonne National Lab: Argonne, IL, USA, 1995. [Google Scholar]
- Bég, O.A.; Bakier, A.Y.; Prasad, V.R. Numerical modelling of non-similar mixed convection heat and species transfer along an inclined solar energy collector surface with cross diffusion effects. World J. Mech. 2011, 4, 185–196. [Google Scholar] [CrossRef]
- Anbuchezhian, N.; Srinivasan, K.; Chandrasekaran, K.; Kandasamy, R. Thermophoresis and Brownian motion effects on boundary layer flow of nanofluid in presence of thermal stratification due to solar energy. Appl. Math. Mech. English Ed. 2012, 33, 765–780. [Google Scholar] [CrossRef]
- Nasrin, R.; Alim, M.A. Performance of nanofluids on heat transfer in a wavy solar collector. Int. J. Eng. Sci. Technol. 2013, 5, 58–77. [Google Scholar] [CrossRef][Green Version]
- Kandasamy, R.; Muhaimin, I.; Rosmila, A.K. The performance evaluation of unsteady MHD non-Darcy nanofluid flow over a porous wedge due to renewable (solar) energy. Renew. Energy 2014, 64, 1–9. [Google Scholar] [CrossRef]
- Khan, J.A.; Mustafa, M.; Hayat, T.; Alsaedi, A. Numerical study of Cattaneo-Christov heat flux model for viscoelastic flow due to an exponentially stretching surface. PLoS ONE 2015, 10, e0137363. [Google Scholar]
- Shehzad, S.A.; Hayat, T.; Alsaedi, A.; Chen, B. A useful model for solar radiation. Energy Ecol. Environ. 2016, 1, 30–38. [Google Scholar] [CrossRef]
- Zeeshan, A.; Majeed, A. Non Darcy mixed convection flow of magnetic fluid over a permeable stretching sheet with ohmic dissipation. J. Magn. 2016, 21, 153–158. [Google Scholar] [CrossRef]
- Madhukesh, J.K.; Kumar, R.N.; Punith Gowda, R.J.; Prasannakumara, B.C.; Ramesh, G.K.; Khan, M.I.; Khan, S.U.; Chu, Y.-M. Numerical simulation of AA7072-AA7075/water-based hybrid nanofluid flow over a curved stretching sheet with Newtonian heating: A non-Fourier heat flux model approach. J. Mol. Liq. 2021, 335, 116103. [Google Scholar] [CrossRef]
- Gowda, R.J.P.; Kumar, R.N.; Aldalbahi, A.; Prasannakumara, B.C.; Rahimi-Gorji, M.; Rahaman, M. Thermophoretic particle deposition in time-dependent flow of hybrid nanofluid over rotating and vertically upward/downward moving disk. J. Mol. Liq. 2021, 22, 100864. [Google Scholar]
- Hamid, M.; Usman, M.; Haq, R.U.; Tian, Z. A Galerkin approach to analyze MHD flow of nanofluid along converging/diverging channels. Arch. Appl. Mech. 2021, 91, 1907–1924. [Google Scholar] [CrossRef]
- Waqas, H.; Yasmin, S.; Muhammad, T.; Imran, M. Flow and heat transfer of nanofluid over a permeable cylinder with nonlinear thermal radiation. J. Mater. Res. Technol. 2021, 14, 2579–2585. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Ishak, A.; Raizah, Z.; Galal, A.M. Analytical approach for a heat transfer process through nanofluid over an irregular porous radially moving sheet by employing KKL correlation with magnetic and radiation effects: Applications to thermal system. Micromachines 2022, 13, 1109. [Google Scholar] [CrossRef]
- Animasaun, I.L.; Yook, S.-J.; Muhammad, T.; Mathew, A. Dynamics of ternary-hybrid nanofluid subject to magnetic flux density and heat source or sink on a convectively heated surface. Surf. Interfaces 2022, 28, 101654. [Google Scholar] [CrossRef]
- Pavlov, K.B. Magnetohydrodynamic flow of an incompressible viscous fluid caused by deformation of a plane surface. Magnetohydrodynamics 1974, 10, 507–510. [Google Scholar]
- Salem, A.M.; El-Aziz, M.A. MHD-mixed convection and mass transfer from a vertical stretching sheet with diffusion of chemically reactive species and space- or temperature-dependent heat source. Can. J. Phys. 2007, 85, 359–373. [Google Scholar]
- Akbar, N.S.; Ebai, A.; Khan, Z.H. Numerical analysis of magnetic field effects on Eyring Powell fluid flow towards a stretching sheet. J. Magn. Magn. Mater. 2015, 382, 355–358. [Google Scholar] [CrossRef]
- Khan, M.; Hussain, M.; Azam, M.; Hashim. Magnetohydrodynamic flow of Carreau fluid over a convectively heated surface in the presence of non-linear radiation. J. Magn. Magn. Mater. 2016, 412, 63–68. [Google Scholar] [CrossRef]
- Moralesa, L.F.; Dasso, S.; Gomez, D.O.; Mininni, P. Hall effect on magnetic reconnection at the Earth's magnetopause. J. Atmosp. Solar-Terr. Phys. 2005, 67, 1821–1826. [Google Scholar] [CrossRef]
- Su, X.; Zheng, L. Hall effect on MHD flow and heat transfer of nanofluids over a stretching wedge in the presence of velocity slip and Joule heating. Central Euro. J. Phys. 2013, 11, 1694–1703. [Google Scholar] [CrossRef]
- Zaib, A.; Shafie, S. Thermal diffusion and diffusion thermo effects on unsteady MHD free convection flow over a stretching surface considering Joule heating and viscous dissipation with thermal stratification, chemical reaction and hall current. J. Frank. Inst. 2014, 351, 1268–1287. [Google Scholar] [CrossRef]
- Sreedevi, G.; Rao, R.R.; Prasada Rao, D.R.V.; Chamkha, A.J. Combined influence of radiation absorption and Hall current effects on MHD double-diffusive free convective flow past a stretching sheet. Ain Shams Eng. J. 2016, 7, 383–397. [Google Scholar] [CrossRef]
- Pal, D.; Mandal, G. Influence of Lorentz force and thermal radiation on heat transfer of nanofluids over a stretching sheet with velocity–thermal slip. Int. J. Appl. Comput. Math. 2017, 3, 3001–3020. [Google Scholar] [CrossRef]
- Khan, M.; Ali, W.; Ahmed, J. A hybrid approach to study the influence of Hall current in radiative nanofluid flow over a rotating disk. Appl. Nanosci. 2017, 10, 5167–5177. [Google Scholar] [CrossRef]
- Rana, P.; Mahanthesh, B.; Thriveni, K.; Muhammad, T. Significance of aggregation of nanoparticles, activation energy, and Hall current to enhance the heat transfer phenomena in a nanofluid: A sensitivity analysis. Waves Rand. Complex Media 2022, 1–23. [Google Scholar] [CrossRef]
- Rana, P.; Gupta, G. FEM solution to quadratic convective and radiative flow of Ag-MgO/H2O hybrid nanofluid over a rotating cone with Hall current: Optimization using response surface methodology. Math. Comp. Simul. 2022, 201, 121–140. [Google Scholar] [CrossRef]
- Aziz, A. A similarity solution for laminar thermal boundary layer over a flat plate with a convective surface boundary condition. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 1064–1068. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. Boundary layer flow of a nanofluid past a stretching sheet with a convective boundary condition. Int. J. Thermal Sci. 2010, 49, 1813–1820. [Google Scholar] [CrossRef]
- Ishak, A. Similarity solutions for flow and heat transfer over a permeable surface with a convective boundary condition. Appl. Math. Comp. 2010, 217, 837–842. [Google Scholar] [CrossRef]
- Yao, S.; Fang, T.; Zhong, Y. Heat transfer of a generalized stretching/shrinking wall problem with convective boundary conditions. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 752–760. [Google Scholar] [CrossRef]
- Rahman, M.M.; Merkin, J.H.; Pop, I. Mixed convection boundary-layer flow past a vertical flat plate with a convective boundary condition. Acta Mech. 2015, 226, 2441–2460. [Google Scholar] [CrossRef]
- Mustafa, M.; Khan, J.A.; Hayat, T.; Alsaedi, A. Simulations for Maxwell fluid flow past a convectively heated exponentially stretching sheet with nanoparticles. AIP Adv. 2015, 5, 037133. [Google Scholar] [CrossRef]
- Ibrahim, W.; Haq, R.U. Magnetohydrodynamic (MHD) stagnation point flow of nanofluid past a stretching sheet with convective boundary condition. J. Braz. Soc. Mech. Sci. Eng. 2015, 38, 1155–1164. [Google Scholar] [CrossRef]
- Makinde, O.D.; Khan, W.A.; Khan, Z.H. Stagnation point flow of MHD chemically reacting nanofluid over a stretching convective surface with slip and radiative heat. Proc. Inst. Mech E Part E J. Process Mech. Eng. 2017, 231, 695–703. [Google Scholar] [CrossRef]
- Abo-Eldahab, E.M.; El Aziz, M.A. Hall and Ion-Slip effects on MHD free convective heat generating flow past a semi-infinite vertical flat plate. Phys. Scr. A 2000, 61, 344. [Google Scholar] [CrossRef]
- Sutton, G.W.; Sherman, A. Engineering Magnetohydrodynamics; McGraw-Hill: New York, NY, USA, 1965. [Google Scholar]
- Takabi, B.; Salehi, S. Augmentation of the heat transfer performance of a sinusoidal corrugated enclosure by employing hybrid nanofluid. Adv. Mech. Eng. 2014, 2014, 147059. [Google Scholar] [CrossRef]
- Arifuzzaman, M.; Uddin, M.J. Convective flow of alumina–water nanofluid in a square vessel in presence of the exothermic chemical reaction and hydromagnetic field. Res. Eng. 2021, 10, 100226. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Hydromagnetic flow and heat transfer adjacent to a stretching vertical sheet. Heat Mass Transf. 2008, 44, 921. [Google Scholar] [CrossRef]
- Grubka, L.J.; Bobba, K.M. Heat transfer characteristics of a continuous, stretching surface with variable temperature. ASME J. Heat Transf. 1985, 107, 248–250. [Google Scholar] [CrossRef]
- Ali, M.E. On thermal boundary layer on a power-law stretched surface with suction or injection. Int. J. Heat Fluid Flow 1995, 16, 280–290. [Google Scholar] [CrossRef]
- Yih, K.A. Free convection effect on MHD coupled heat and mass transfer of a moving permeable vertical surface. Int. Commun. Heat Mass Transf. 1999, 26, 95–104. [Google Scholar] [CrossRef]





| Properties | |||||
|---|---|---|---|---|---|
| Water | 997.1 | 4179 | 0.613 | 5.5 × 10−6 | 21 |
| Alumina | 3970 | 765 | 40 | 3.5 × 107 | 0.85 |
| Ishak et al. [54] | Present Results | |||
|---|---|---|---|---|
| 0.00 | −0.5607 | 1.0873 | −0.560723 | 1.087336 |
| 0.01 | −0.5658 | 1.0863 | −0.565813 | 1.086323 |
| 0.04 | −0.5810 | 1.0833 | −0.581056 | 1.083329 |
| 0.25 | −0.6830 | 1.0630 | −0.683035 | 1.063014 |
| 1.00 | −1.0000 | 1.0000 | −1.000000 | 1.000000 |
| 4.00 | −1.8968 | 0.8311 | −1.896819 | 0.831129 |
| Pr | Grubka and Bobba [55] | Ali [56] | Yih [57] | Present |
|---|---|---|---|---|
| 0.01 | 0.0197 | - | 0.0197 | 0.019743 |
| 0.72 | 0.8086 | 0.8058 | 0.8086 | 0.808656 |
| 1.00 | 1.0000 | 0.9961 | 1.0000 | 1.00000 |
| 3.00 | 1.9237 | 1.9144 | 1.9237 | 1.923734 |
| 10.0 | 3.7207 | 3.7006 | 3.7207 | 3.720712 |
| 1000 | 12.2940 | - | 12.2940 | 12.294023 |
| Parameters | |||||
|---|---|---|---|---|---|
| Stable Branch Solution | Unstable Branch Solution | ||||
| 0.025 | 0.015 | 0.5 | 0.05 | 0.219198 | −0.599912 |
| 0.030 | - | - | - | 0.221735 | −0.606152 |
| 0.035 | - | - | - | 0.224611 | −0.612562 |
| 0.025 | 0.05 | 0.5 | 0.05 | 0.122133 | −0.540843 |
| - | 0.10 | - | - | 0.173385 | −0.573070 |
| - | 0.15 | - | - | 0.219198 | −0.599912 |
| 0.25 | 0.15 | 0.5 | 0.05 | 0.219198 | −0.599912 |
| - | - | 0.7 | - | 0.195805 | −0.586155 |
| - | - | 0.9 | - | 0.173778 | −0.572856 |
| 0.25 | 0.15 | 0.5 | 0.05 | 0.219198 | −0.599912 |
| - | - | - | 0.10 | 0.224448 | −0.598987 |
| - | - | - | 0.15 | 0.229606 | −0.597892 |
| Parameters | |||||
|---|---|---|---|---|---|
| Stable Branch Solution | Unstable Branch Solution | ||||
| 0.025 | 0.015 | 0.5 | 0.05 | 0.030567 | 0.014645 |
| 0.030 | - | - | - | 0.030987 | 0.014891 |
| 0.035 | - | - | - | 0.031418 | 0.015136 |
| 0.025 | 0.05 | 0.5 | 0.05 | 0.010414 | 0.006121 |
| - | 0.10 | - | - | 0.020619 | 0.010942 |
| - | 0.15 | - | - | 0.030567 | 0.014645 |
| 0.25 | 0.15 | 0.5 | 0.05 | 0.030567 | 0.014645 |
| - | - | 0.7 | - | 0.036079 | 0.018213 |
| - | - | 0.9 | - | 0.038359 | 0.020299 |
| 0.25 | 0.15 | 0.5 | 0.05 | 0.030567 | 0.014645 |
| - | - | - | 0.10 | 0.030573 | 0.014599 |
| - | - | - | 0.15 | 0.030578 | 0.014556 |
| Parameters | ||||
|---|---|---|---|---|
| Stable Branch Solution | Unstable Branch Solution | |||
| 0.025 | 2.0 | 1.3 | 1.732743 | 1.065175 |
| 0.030 | - | - | 1.728290 | 1.068399 |
| 0.035 | - | - | 1.723942 | 1.071205 |
| 0.025 | 1.0 | 1.3 | 1.284758 | 0.607092 |
| - | 1.5 | - | 1.524764 | 0.829616 |
| - | 2.0 | - | 1.732743 | 1.065175 |
| 0.25 | 2.0 | 0.5 | 0.963059 | 0.428501 |
| - | - | 0.9 | 1.330421 | 0.651489 |
| - | - | 1.3 | 1.732743 | 1.065175 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, U.; Zaib, A.; Ishak, A.; Waini, I.; M. Sherif, E.-S.; Boonsatit, N.; Pop, I.; Jirawattanapanit, A. Ramification of Hall and Mixed Convective Radiative Flow towards a Stagnation Point into the Motion of Water Conveying Alumina Nanoparticles Past a Flat Vertical Plate with a Convective Boundary Condition: The Case of Non-Newtonian Williamson Fluid. Lubricants 2022, 10, 192. https://doi.org/10.3390/lubricants10080192
Khan U, Zaib A, Ishak A, Waini I, M. Sherif E-S, Boonsatit N, Pop I, Jirawattanapanit A. Ramification of Hall and Mixed Convective Radiative Flow towards a Stagnation Point into the Motion of Water Conveying Alumina Nanoparticles Past a Flat Vertical Plate with a Convective Boundary Condition: The Case of Non-Newtonian Williamson Fluid. Lubricants. 2022; 10(8):192. https://doi.org/10.3390/lubricants10080192
Chicago/Turabian StyleKhan, Umair, Aurang Zaib, Anuar Ishak, Iskandar Waini, El-Sayed M. Sherif, Nattakan Boonsatit, Ioan Pop, and Anuwat Jirawattanapanit. 2022. "Ramification of Hall and Mixed Convective Radiative Flow towards a Stagnation Point into the Motion of Water Conveying Alumina Nanoparticles Past a Flat Vertical Plate with a Convective Boundary Condition: The Case of Non-Newtonian Williamson Fluid" Lubricants 10, no. 8: 192. https://doi.org/10.3390/lubricants10080192
APA StyleKhan, U., Zaib, A., Ishak, A., Waini, I., M. Sherif, E.-S., Boonsatit, N., Pop, I., & Jirawattanapanit, A. (2022). Ramification of Hall and Mixed Convective Radiative Flow towards a Stagnation Point into the Motion of Water Conveying Alumina Nanoparticles Past a Flat Vertical Plate with a Convective Boundary Condition: The Case of Non-Newtonian Williamson Fluid. Lubricants, 10(8), 192. https://doi.org/10.3390/lubricants10080192








