Radio Continuum Spectra of Planetary Nebulae
Abstract
1. Introduction
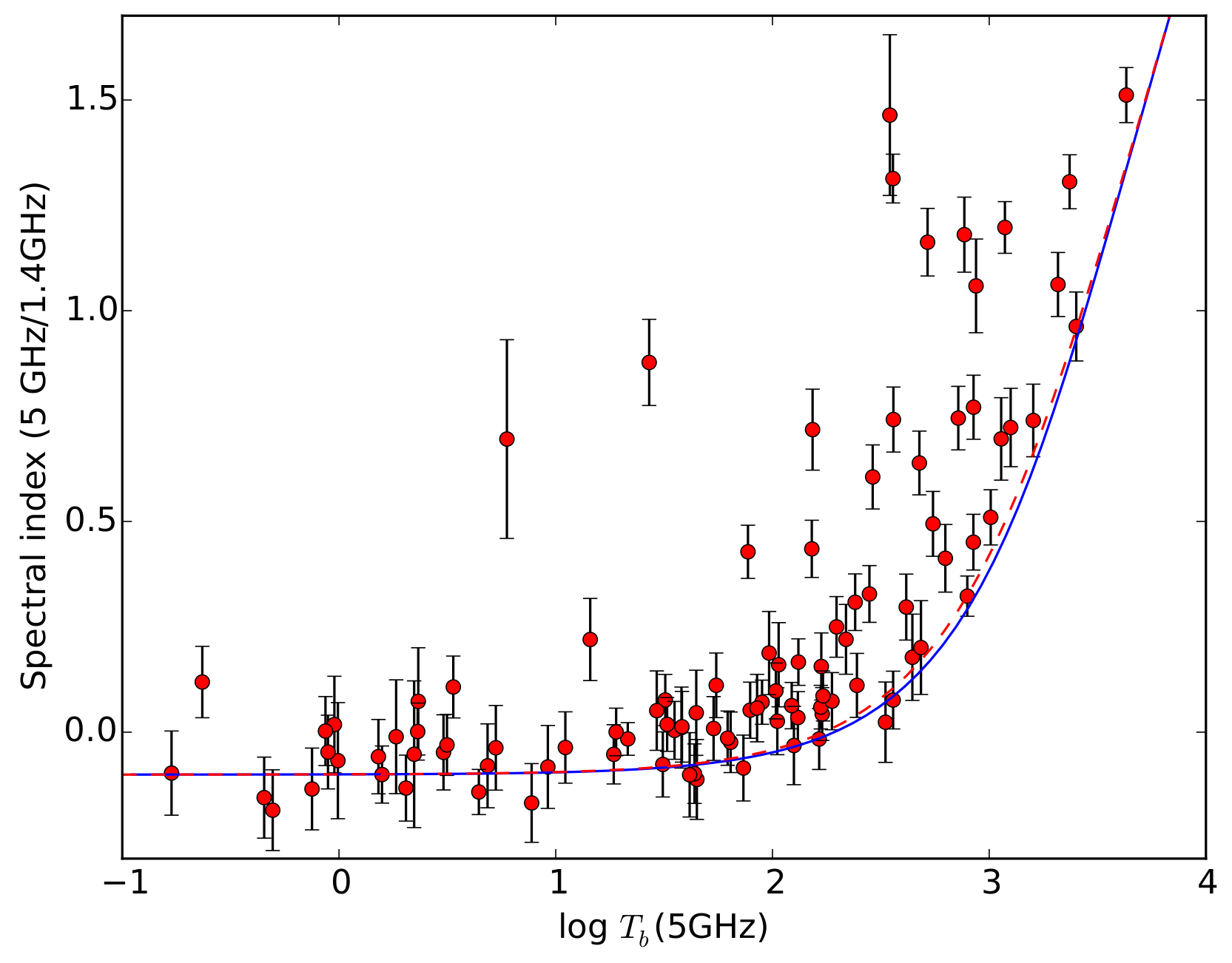
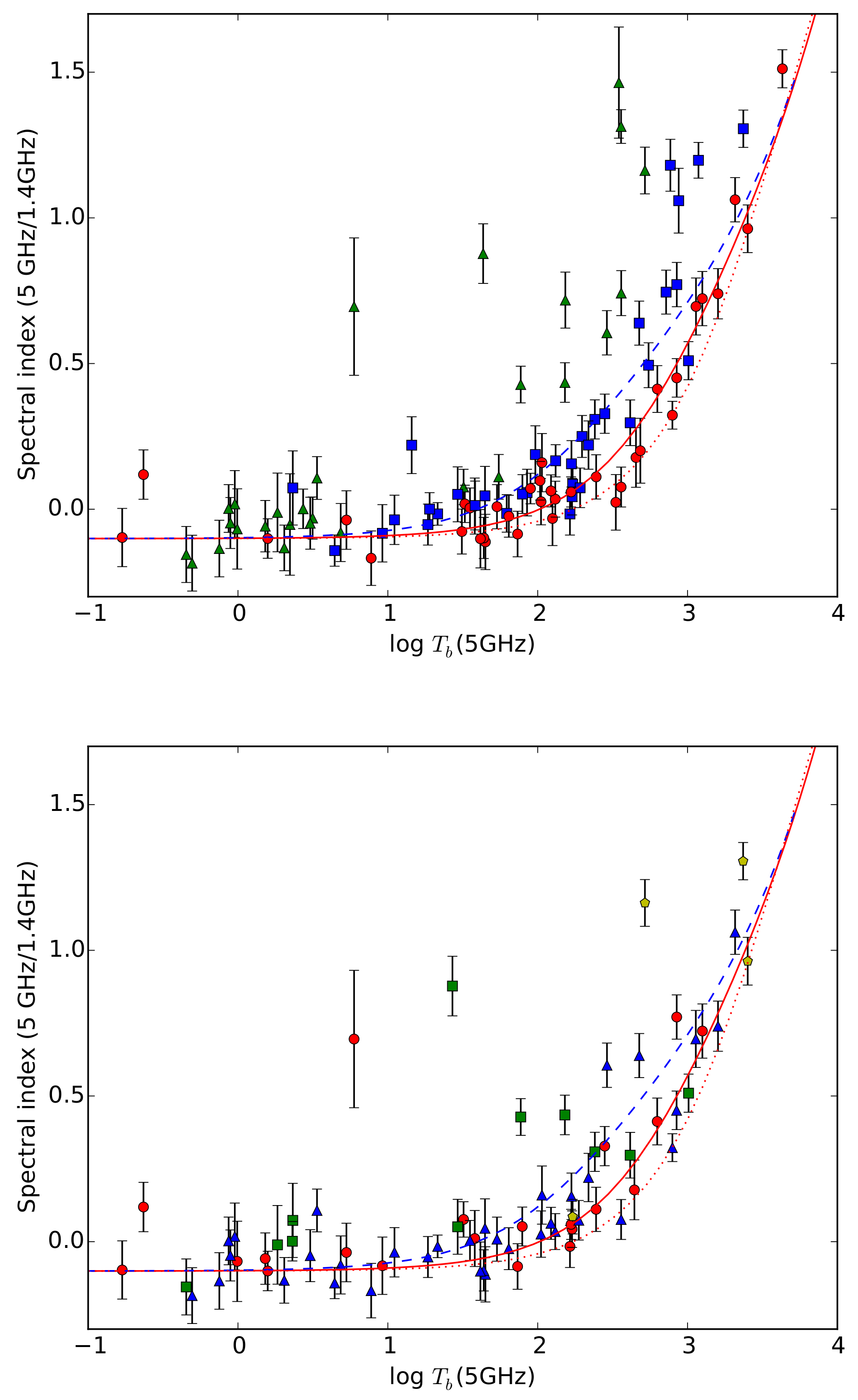
2. Methodology
3. Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Pazderska, B.M.; Gawroński, M.P.; Feiler, R.; Birkinshaw, M.; Browne, I.W.A.; Davis, R.; Kus, A.J.; Lancaster, K.; Lowe, S.R.; Pazderski, E.; et al. Survey of planetary nebulae at 30 GHz with OCRA-p. Astron. Astrophys. 2009, 498, 463. [Google Scholar] [CrossRef]
- Chhetri, R.; Ekers, R.D.; Kimball, A.; Miszalski, B.; Cohen, M.; Manick, R. High radio frequency sample of bright planetary nebulae in the Southern hemisphere detected in the AT20G survey. Mon. Not. R. Astron. Soc. 2007, 382, 1607. [Google Scholar] [CrossRef]
- Suárez, O.; Gómez, J.F.; Bendjoya, P.; Miranda, L.F.; Guerrero, M.A.; Uscanga, L.; Green, J.A.; Rizzo, J.R.; Ramos-Larios, G. Time-variable Non-thermal Emission in the Planetary Nebula IRAS 15103-5754. Astrophys. J. 2015, 806, 105. [Google Scholar] [CrossRef]
- Siódmiak, N.; Tylenda, R. An analysis of the observed radio emission from planetary nebulae. Astron. Astrophys. 2001, 373, 1032–1042. [Google Scholar] [CrossRef]
- Phillips, J.P. Density gradients in Galactic planetary nebulae. Mon. Not. R. Astron. Soc. 2007, 378, 231. [Google Scholar] [CrossRef]
- Hajduk, M.; van Hoof, P.A.; Śniadkowska, K.; Krankowski, A.; Błaszkiewicz, L.; Dąbrowski, B.; Zijlstra, A.A. Radio observations of planetary nebulae: No evidence for strong radial density gradients. Mon. Not. R. Astron. Soc. 2018, 479, 4931. [Google Scholar] [CrossRef]
- Olnon, F.M. Thermal bremsstrahlung radiospectra for inhomogeneous objects, with an application to MWC 349. Astron. Astrophys. 1975, 39, 217. [Google Scholar]
- Van Hoof, P.A.M.; Williams, R.J.R.; Volk, K.; Chatzikos, M.; Ferland, G.J.; Lykins, M.; Porter, R.L.; Wang, Y. Accurate determination of the free-free Gaunt factor—I. Non-relativistic Gaunt factors. Mon. Not. R. Astron. Soc. 2014, 444, 420. [Google Scholar] [CrossRef]
- Frew, D.J.; Parker, Q.A.; Bojičić, I.S. The Hα surface brightness-radius relation: A robust statistical distance indicator for planetary nebulae. Mon. Not. R. Astron. Soc. 2016, 455, 1459. [Google Scholar] [CrossRef]
- Cahn, J.H.; Kaler, J.B.; Stanghellini, L. A catalogue of absolute fluxes and distances of planetary nebulae. Astron. Astrophys. Suppl. Ser. 1992, 94, 399. [Google Scholar]
- Sandin, C.; Schönberner, D.; Roth, M.M.; Steffen, M.; Böhm, P.; Monreal-Ibero, A. Spatially resolved spectroscopy of planetary nebulae and their halos. I. Five galactic disk objects. Astron. Astrophys. 2004, 486, 545. [Google Scholar] [CrossRef]
- Masson, C.R. On the structure of ionization-bounded planetary nebulae. Astrophys. J. 1990, 348, 580. [Google Scholar] [CrossRef]
- Aaquist, O.B.; Kwok, S. Radio Morphologies of Planetary Nebulae. Astrophys. J. 1996, 462, 813. [Google Scholar] [CrossRef]
- Phillips, J.P. The relation between elemental abundances and morphology in planetary nebulae. Mon. Not. R. Astron. Soc. 2003, 340, 883. [Google Scholar] [CrossRef]
- Stanghellini, L.; Villaver, E.; Manchado, A.; Guerrero, M.A. The Correlations between Planetary Nebula Morphology and Central Star Evolution: Analysis of the Northern Galactic Sample. Astrophys. J. 2002, 576, 285. [Google Scholar] [CrossRef]
- Sahai, R.; Morris, M.R.; Villar, G.G. Young Planetary Nebulae: Hubble Space Telescope Imaging and a New Morphological Classification System. Astron. J. 2011, 141, 134. [Google Scholar] [CrossRef]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hajduk, M.; Van Hoof, P.A.M.; Sniadkowska, K.; Krankowski, A.; Błaszkiewicz, L.; Dabrowski, B.; Zijlstra, A.A. Radio Continuum Spectra of Planetary Nebulae. Galaxies 2019, 7, 6. https://doi.org/10.3390/galaxies7010006
Hajduk M, Van Hoof PAM, Sniadkowska K, Krankowski A, Błaszkiewicz L, Dabrowski B, Zijlstra AA. Radio Continuum Spectra of Planetary Nebulae. Galaxies. 2019; 7(1):6. https://doi.org/10.3390/galaxies7010006
Chicago/Turabian StyleHajduk, Marcin, Peter A. M. Van Hoof, Karolina Sniadkowska, Andrzej Krankowski, Leszek Błaszkiewicz, Bartosz Dabrowski, and Albert A. Zijlstra. 2019. "Radio Continuum Spectra of Planetary Nebulae" Galaxies 7, no. 1: 6. https://doi.org/10.3390/galaxies7010006
APA StyleHajduk, M., Van Hoof, P. A. M., Sniadkowska, K., Krankowski, A., Błaszkiewicz, L., Dabrowski, B., & Zijlstra, A. A. (2019). Radio Continuum Spectra of Planetary Nebulae. Galaxies, 7(1), 6. https://doi.org/10.3390/galaxies7010006





