Galactic Structures from Gravitational Radii
Abstract
:1. Introduction
- (i)
- using DM hypothesis in addition to Newtonian gravity (standard approach);
- (ii)
- modifying fundamental laws of dynamics or gravity (alternative approach).
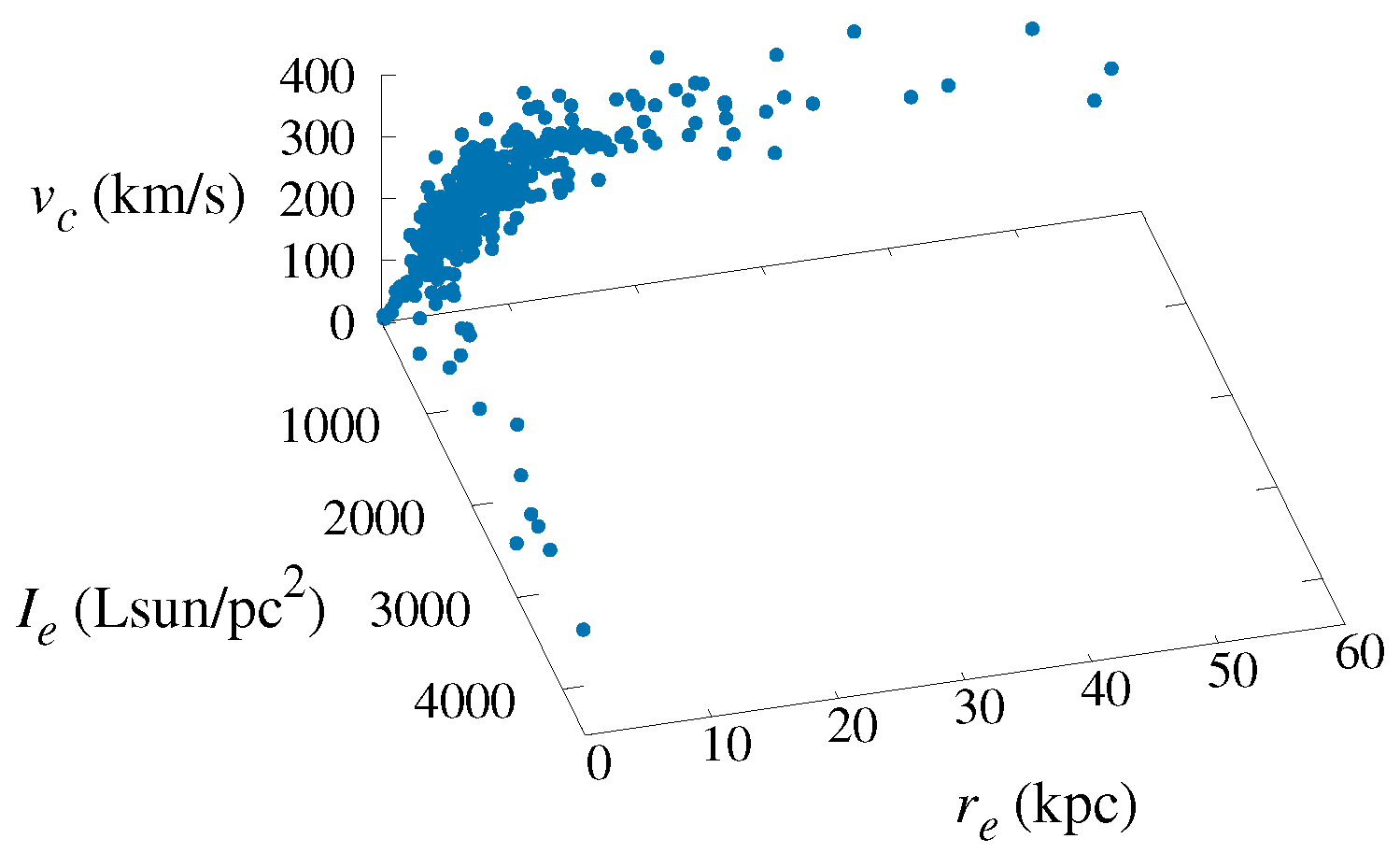
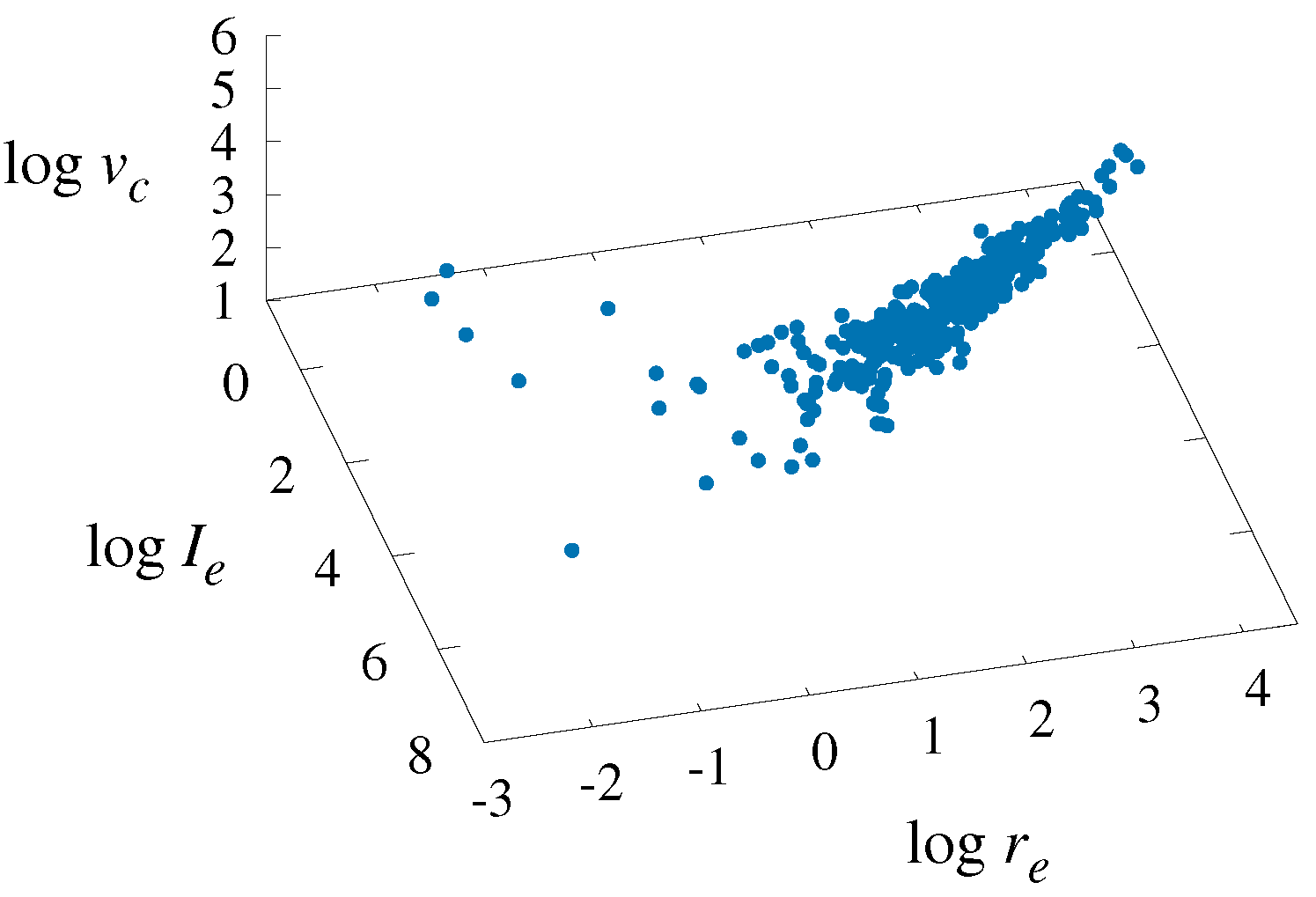
2. Fundamental Plane of Elliptical Galaxies and Gravity
2.1. Fundamental Plane of Ellipticals
2.2. Gravity and Dynamics of Stellar Systems
- addend with : correlation between and (—from potential)
- addend with : correlation between and (—virial velocity in )
- addend with : correlation between and (through the ratio).
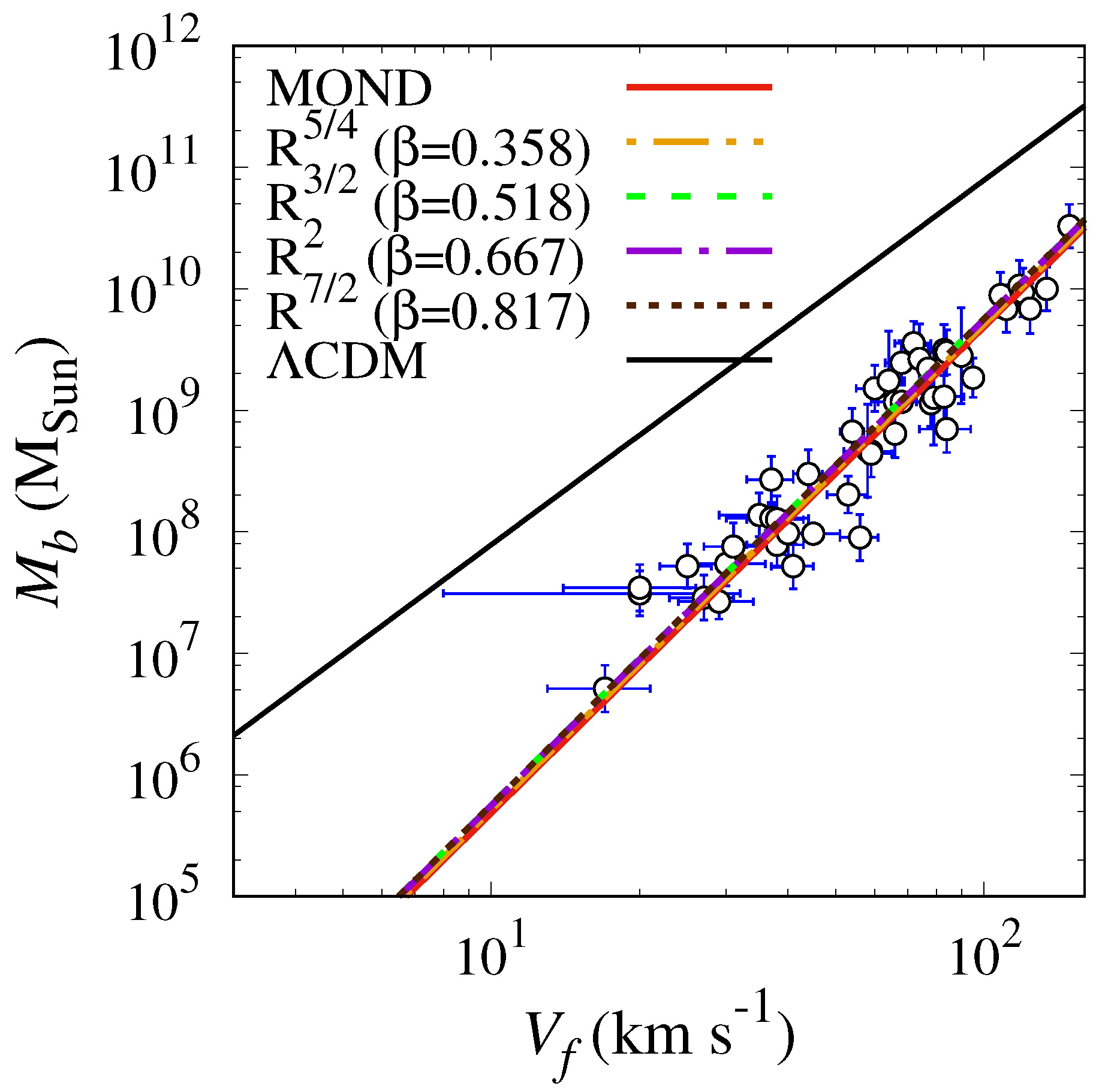
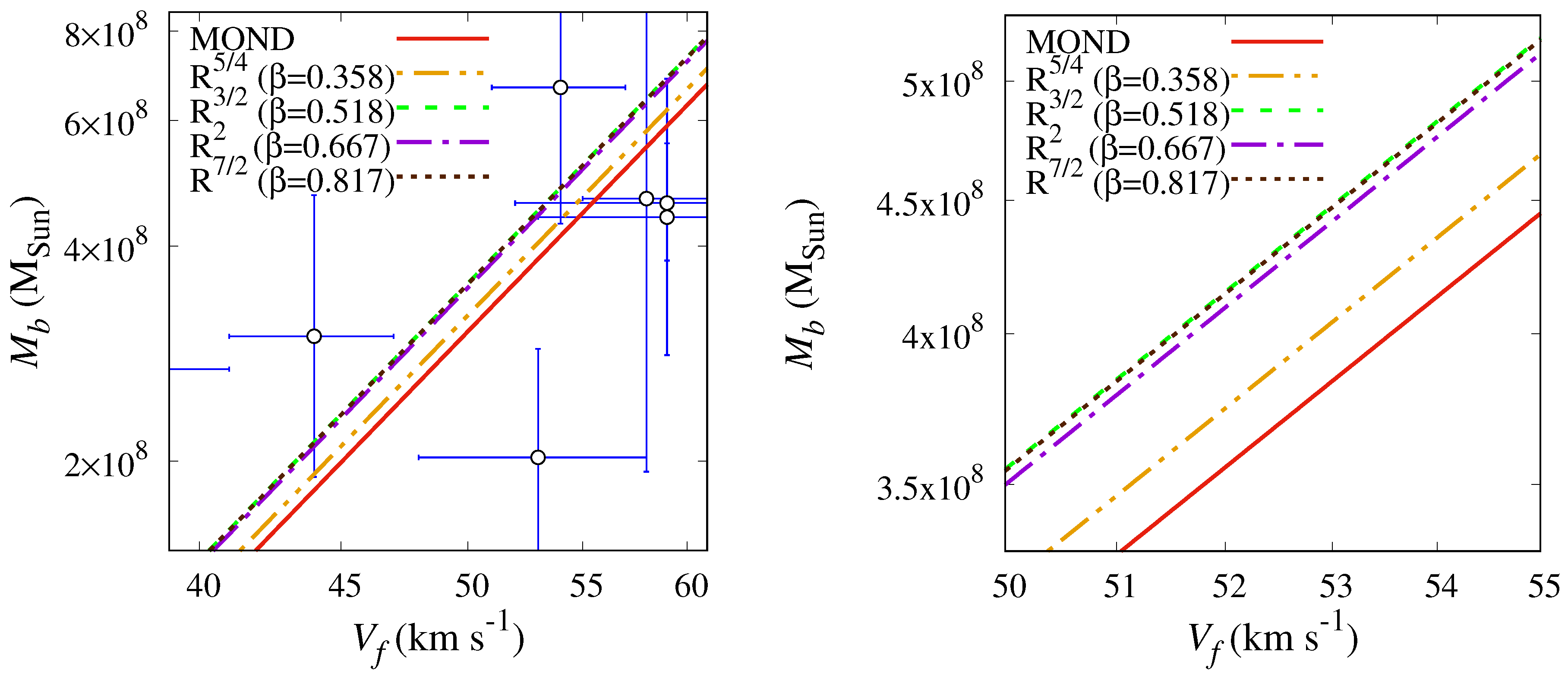
3. Explaining the Baryonic Tully-Fisher Relation with New Gravitational Radius
3.1. New Fundamental Gravitational Radius
3.2. Tully-Fisher Relation in MOND and f(R) Gravity
- (i)
- MOND: ;
- (ii)
- : ;We calculated BTFR for four cases of n: , which correspond to , respectively. —MOND acceleration constant for point source in infinity; a —constant for spiral systems. In the case of spiral galaxies, we have a instead of (empirical calibration is = 0.8a, as reported in [20]).
- (iii)
- CDM: .Formula for CDM is taken from the paper [21], Equation (13): .
4. Discussion and Conclusions
- We used power-law gravity to demonstrate the existence of a new fundamental gravitational radius.
- This radius plays an analog role, in the case of weak gravitational field at galactic scales (IR scales) as the Schwarzschild radius in the case of strong gravitational field in the vicinity of compact massive objects (UV scales).
- The radius emerges as a conserved quantity from Noether’s symmetries that exist for any power-law function.
- Using this new gravitational radius, gravity is able to explain the baryonic Tully-Fisher relation of gas-rich galaxies without DM hypothesis.
- MOND is a particular case of gravity in the weak field limit.
- The same radius is useful to address the FP of elliptical galaxies.
- The range (corresponding to ) is in a good agreement with observations. These values agree with observational constraints on obtained by fitting FP and MOND. We do not need DM to explain baryonic Tully-Fisher relation, and even more, CDM is not in satisfactory agreement with observations.
- For elliptical galaxies is proportional to .
- Considering the definition of , we can say that the effective radius (defined photometrically as the radius containing half of the luminosity of a galaxy) is led by gravity.
- In perspective, the whole galactic dynamics can be addressed by Extended Gravity.
- Work in progress for Faber-Jackson relation, galactic potentials, Boltzmann-Vlasov relation, and Virial Theorem.
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| BTFR | Baryonic Tully-Fisher relation |
| DM | Dark matter |
| ETGs | Extended Theories of Gravity |
| FP | Fundamental plane |
| GC | Galactic Center |
| GR | General Relativity |
| MOND | Modified Newtonian dynamics |
| STVG | Scalar-Tensor-Vector-Gravity |
References
- McGaugh, S.S.; Lelli, F.; Schombert, J.M. Radial acceleration relation in rotationally supported galaxies. Phys. Rev. Lett. 2016, 117, 201101. [Google Scholar] [CrossRef] [PubMed]
- Brownstein, J.R.; Moffat, J.W. The Bullet Cluster 1E0657-558 evidence shows modified gravity in the absence of dark matter. Mon. Not. R. Astron. Soc. 2007, 382, 29–47. [Google Scholar] [CrossRef]
- Israel, N.S.; Moffat, J.W. The Train Wreck Cluster Abell 520 and the Bullet Cluster 1E0657-558 in a Generalized Theory of Gravitation. arXiv, 2016; arXiv:1606.09128. [Google Scholar]
- Busarello, G.; Capaccioli, M.; Capozziello, S.; Longo, G.; Puddu, E. The relation between the virial theorem and the fundamental plane of elliptical galaxies. Astron. Astrophys. 1997, 320, 415–420. [Google Scholar]
- Burstein, B.; Bender, R.; Faber, S.M.; Nolthenius, R. Global relationships among the physical properties of stellar systems. Astron. J. 1997, 114, 1365–1392. [Google Scholar] [CrossRef]
- Capozziello, S.; Cardone, V.F.; Troisi, A. Low surface brightness galaxy rotation curves in the low energy limit of Rn gravity: No need for dark matter? Mon. Not. R. Astron. Soc. 2007, 375, 1423–1440. [Google Scholar] [CrossRef]
- Borka, D.; Jovanović, P.; Borka Jovanović, V.; Zakharov, A.F. Constraints on Rn gravity from precession of orbits of S2-like stars. Phys. Rev. D 2012, 85, 124004. [Google Scholar] [CrossRef]
- Borka, D.; Jovanović, P.; Borka Jovanović, V.; Zakharov, A.F. Orbital precession in Rn gravity: Simulations vs observations (the S2 star orbit case). In Proceedings of the Summer School and Conference on Modern Mathematical Physics, Belgrade, Serbia, 9–19 September 2012; Sveske fizičkih nauka (SFIN) 2013, XXVI Series A: Conferences No. A1. pp. 31–66. [Google Scholar]
- Zakharov, A.F.; Borka, D.; Borka Jovanović, V.; Jovanović, P. Constraints on Rn gravity from precession of orbits of S2-like stars: A case of a bulk distribution of mass. Adv. Space Res. 2014, 54, 1108–1112. [Google Scholar] [CrossRef]
- Borka, D.; Jovanović, P.; Borka Jovanović, V.; Zakharov, A.F. Chapter 9 in “Advances in General Relativity Research”; Williams, C., Ed.; Nova Science Publishers: Hauppauge, NY, USA, 2015; pp. 343–362. [Google Scholar]
- Borka Jovanović, V.; Capozziello, S.; Jovanović, P.; Borka, D. Recovering the fundamental plane of galaxies by f(R) gravity. Phys. Dark Univ. 2016, 14, 73–83. [Google Scholar] [CrossRef]
- Bender, R.; Burstein, D.; Faber, S.M. Dynamically hot galaxies. I. Structural properties. Astrophys. J. 1992, 399, 462–477. [Google Scholar] [CrossRef]
- Hernquist, L. An analytical model for spherical galaxies and bulges. Astrophys. J. 1990, 356, 359–364. [Google Scholar] [CrossRef]
- Frigerio Martins, C.; Salucci, P. Analysis of rotation curves in the framework of Rn gravity. Mon. Not. R. Astron. Soc. 2007, 381, 1103–1108. [Google Scholar] [CrossRef]
- Capozziello, S.; Jovanović, P.; Borka Jovanović, V.; Borka, D. Addressing the missing matter problem in galaxies through a new fundamental gravitational radius. J. Cosmol. Astropart. Phys. 2017, 6, 044. [Google Scholar] [CrossRef]
- McGaugh, S.S. A Novel Test of the Modified Newtonian Dynamics with Gas Rich Galaxies. Phys. Rev. Lett. 2011, 106, 121303. [Google Scholar] [CrossRef] [PubMed]
- Begum, A.; Chengalur, J.N.; Karachentsev, I.D.; Sharina, M.E. Baryonic Tully-Fisher relation for extremely low mass Galaxies. Mon. Not. R. Astron. Soc. 2008, 386, 138–144. [Google Scholar] [CrossRef]
- Stark, D.V.; McGaugh, S.S.; Swaters, R.A. A first attempt to calibrate the baryonic Tully-Fisher relation with gas-dominated galaxies. Astron. J. 2009, 138, 392–401. [Google Scholar] [CrossRef]
- Trachternach, C.; de Blok, W.J.G.; McGaugh, S.S.; van der Hulst, J.M.; Dettmar, R.-J. The baryonic Tully-Fisher relation and its implication for dark matter halos. Astron. Astrophys. 2009, 505, 577–587. [Google Scholar] [CrossRef]
- McGaugh, S.S. Erratum: Novel Test of Modified Newtonian Dynamics with Gas Rich Galaxies. Phys. Rev. Lett. 2011, 107, 229901. [Google Scholar] [CrossRef]
- McGaugh, S.S. The baryonic Tully-Fisher relation of gas-rich galaxies as a test of ΛCDM and MOND. Astron. J. 2012, 143, 40. [Google Scholar] [CrossRef]

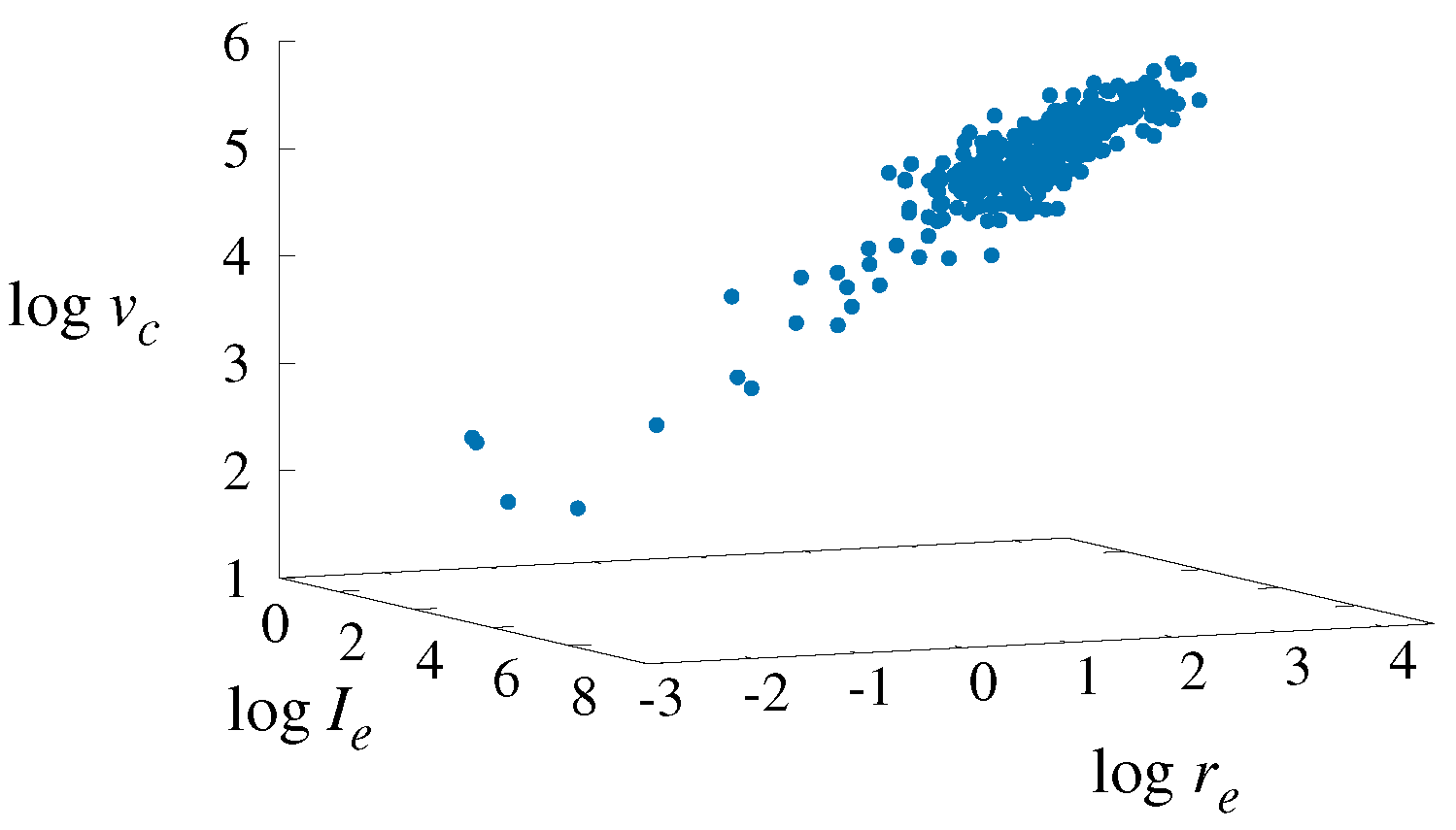


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capozziello, S.; Borka, D.; Borka Jovanović, V.; Jovanović, P. Galactic Structures from Gravitational Radii. Galaxies 2018, 6, 22. https://doi.org/10.3390/galaxies6010022
Capozziello S, Borka D, Borka Jovanović V, Jovanović P. Galactic Structures from Gravitational Radii. Galaxies. 2018; 6(1):22. https://doi.org/10.3390/galaxies6010022
Chicago/Turabian StyleCapozziello, Salvatore, Duško Borka, Vesna Borka Jovanović, and Predrag Jovanović. 2018. "Galactic Structures from Gravitational Radii" Galaxies 6, no. 1: 22. https://doi.org/10.3390/galaxies6010022
APA StyleCapozziello, S., Borka, D., Borka Jovanović, V., & Jovanović, P. (2018). Galactic Structures from Gravitational Radii. Galaxies, 6(1), 22. https://doi.org/10.3390/galaxies6010022






