The Cusp–Core Problem in Gas-Poor Dwarf Spheroidal Galaxies
Abstract
1. Introduction
1.1. Lambda Cold Dark Matter Paradigm and Its Dark Matter Cusps
1.2. The Historical Cusp–Core Problem
1.3. A Promising Solution for Gas-Rich Dwarf Galaxies
1.4. Review Plan
2. The Cusp–Core Problem in Gas-Poor Milky Way Satellites
2.1. Dynamical Models
2.2. Controversy on Producing DM Cores via Supernova Feedback
3. Solutions
3.1. Cusps to Cores
3.1.1. Mergers with Dwarf Galaxies
3.1.2. Globular Clusters and Gas Clumps
3.1.3. Globular Clusters Embedded in Dark Matter
3.1.4. Tidal Interactions
3.1.5. Cusp Regeneration
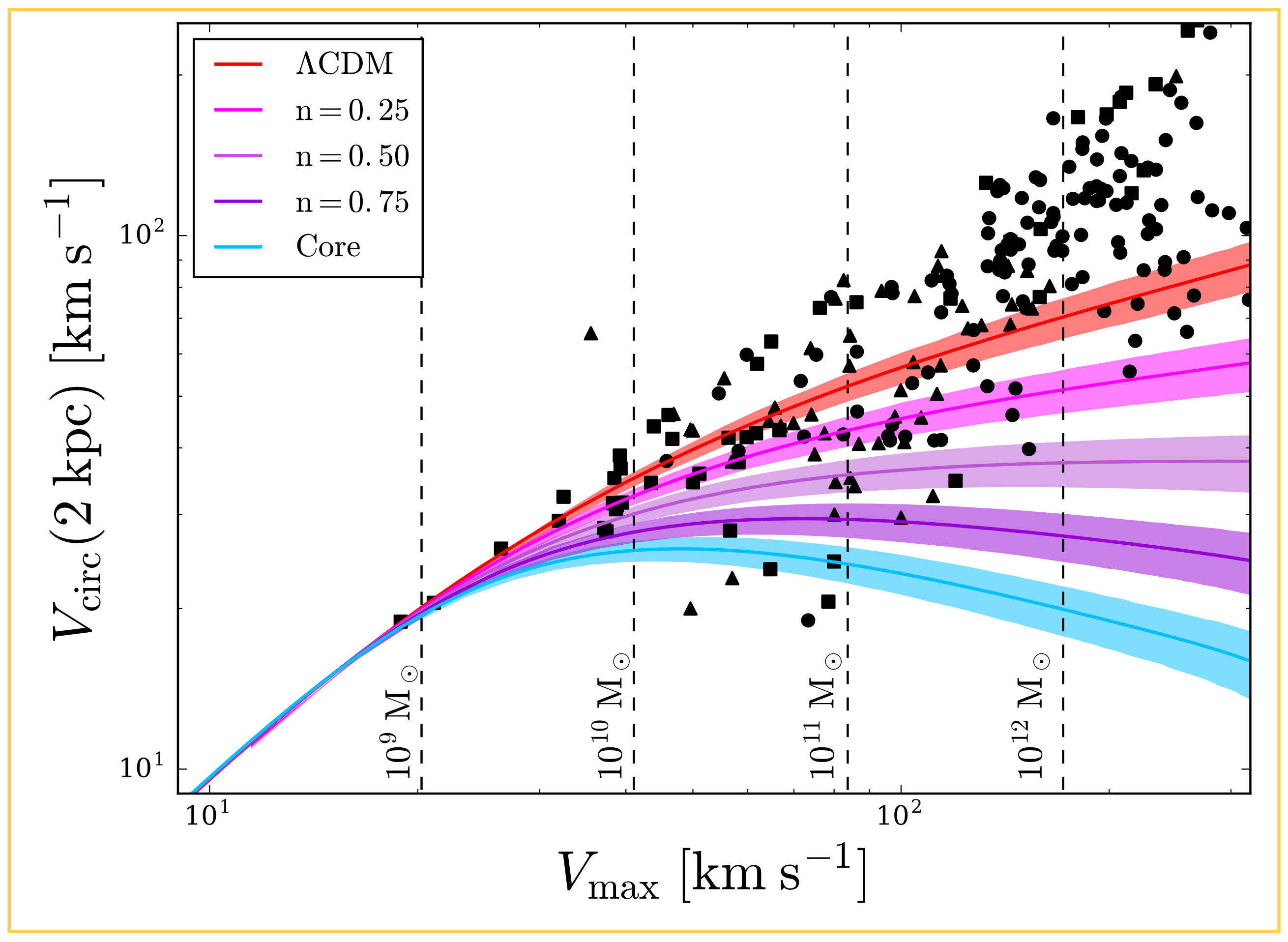
3.1.6. The Diversity Problem
3.2. Inherent Cores
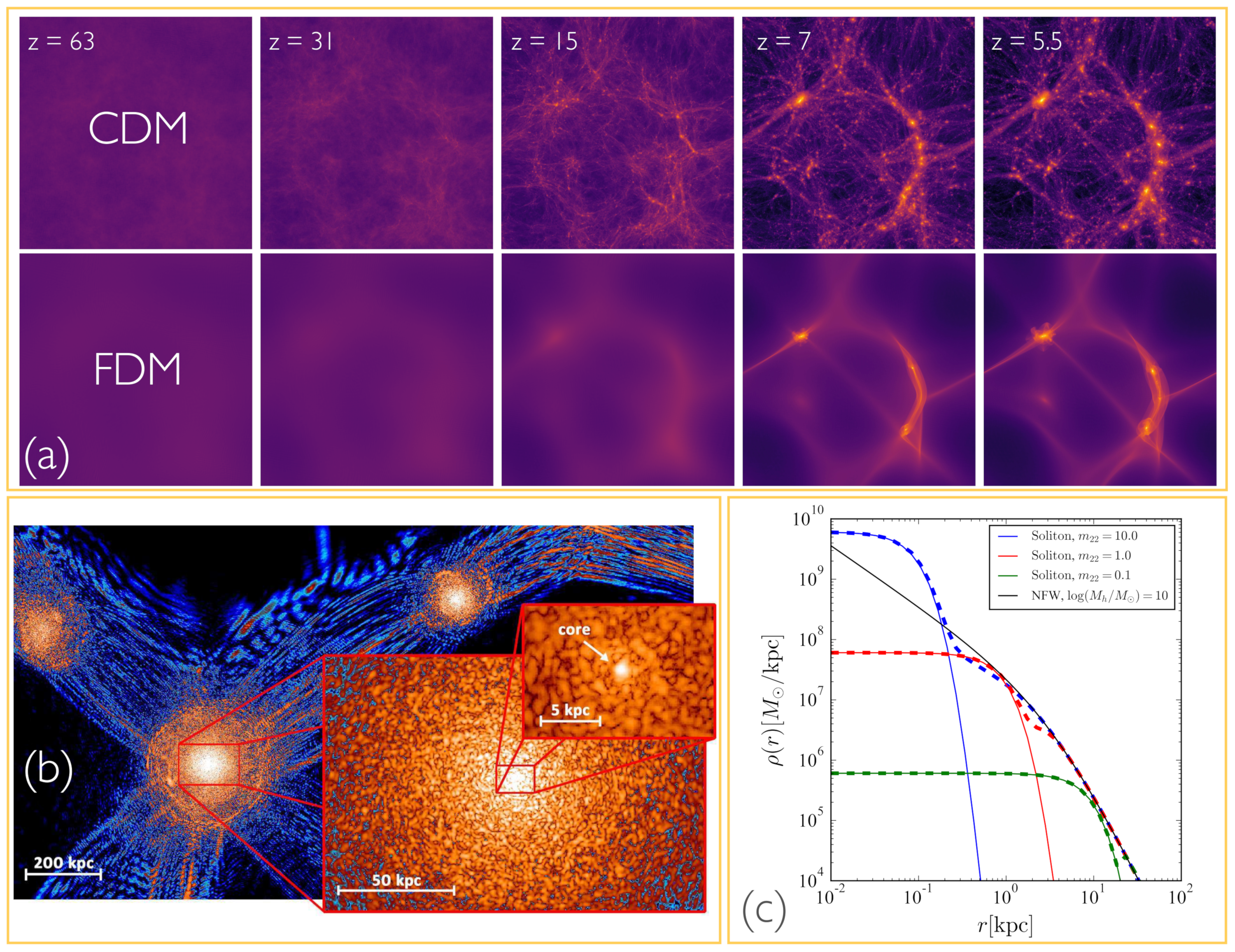
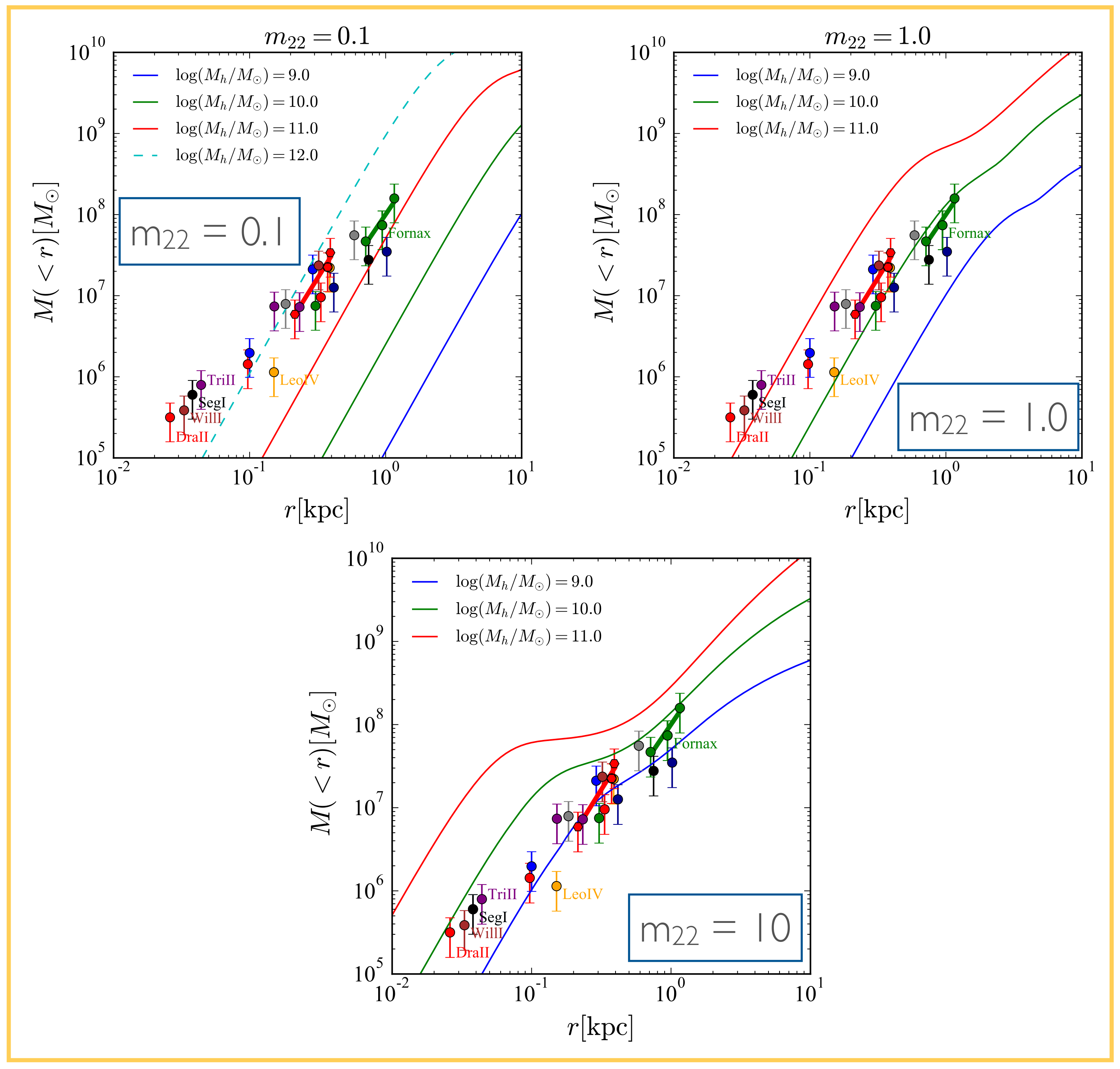
3.2.1. Fuzzy Dark Matter
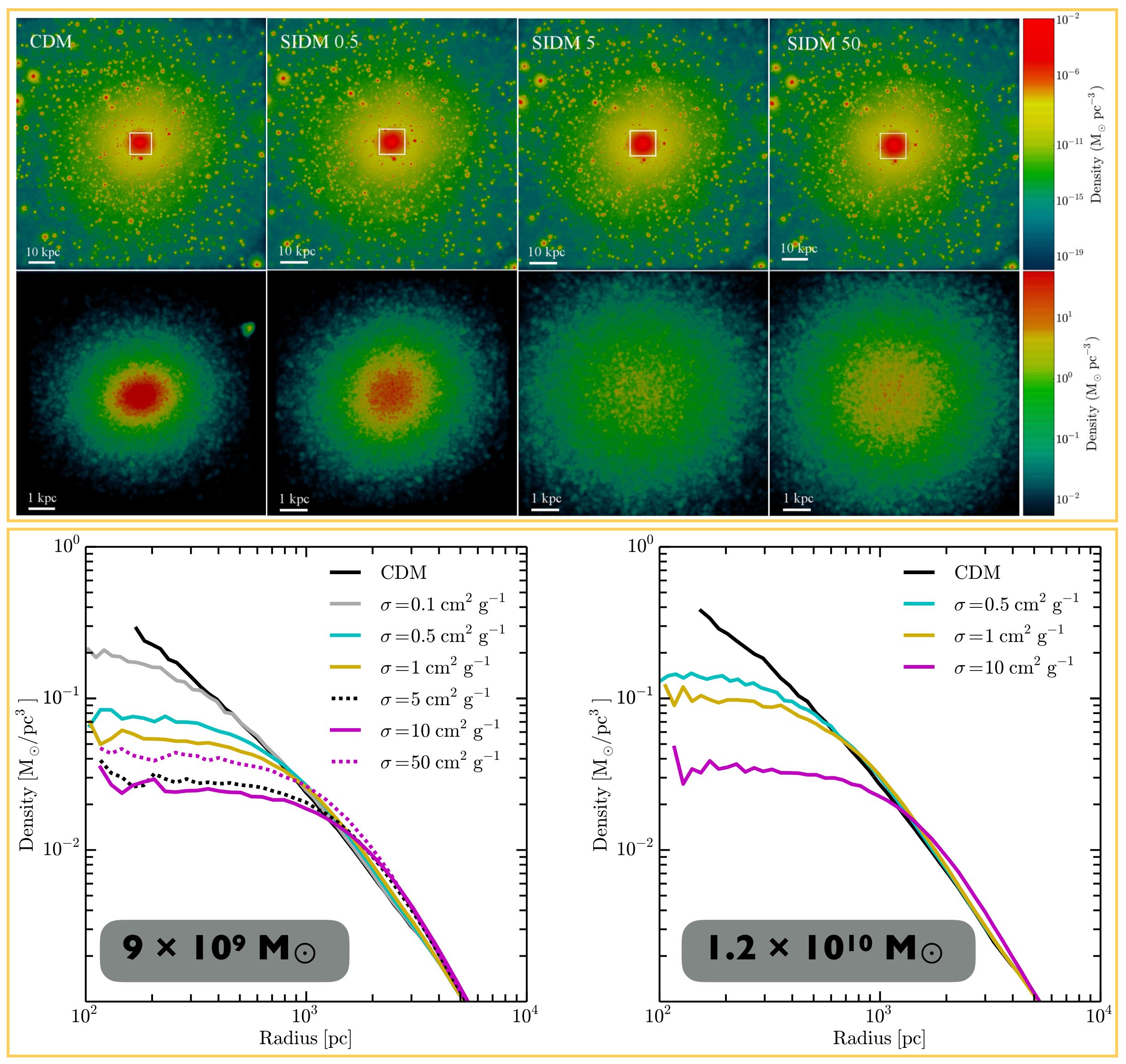
3.2.2. Self-Interacting Dark Matter
3.2.3. Primordial Black Holes as Dark Matter Candidates
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blumenthal, G.R.; Faber, S.M.; Primack, J.R.; Rees, M.J. Formation of galaxies and large-scale structure with cold dark matter. Nature 1984, 311, 517–525. [Google Scholar] [CrossRef]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Determination of Cosmological Parameters. Astrophys. J. Suppl. Ser. 2003, 148, 175–194. [Google Scholar] [CrossRef]
- Croft, R.A.C.; Weinberg, D.H.; Bolte, M.; Burles, S.; Hernquist, L.; Katz, N.; Kirkman, D.; Tytler, D. Toward a Precise Measurement of Matter Clustering: Lyα Forest Data at Redshifts 2-4. Astrophys. J. 2002, 581, 20–52. [Google Scholar] [CrossRef]
- Springel, V.; Frenk, C.S.; White, S.D.M. The large-scale structure of the Universe. Nature 2006, 440, 1137–1144. [Google Scholar] [CrossRef]
- Trujillo-Gomez, S.; Klypin, A.; Primack, J.; Romanowsky, A.J. Galaxies in ΛCDM with Halo Abundance Matching: Luminosity-Velocity Relation, Baryonic Mass-Velocity Relation, Velocity Function, and Clustering. Astrophys. J. 2011, 742, 16. [Google Scholar] [CrossRef]
- Frenk, C.S.; White, S.D.M.; Davis, M.; Efstathiou, G. The Formation of Dark Halos in a Universe Dominated by Cold Dark Matter. Astrophys. J. 1988, 327, 507. [Google Scholar] [CrossRef]
- Dubinski, J.; Carlberg, R.G. The Structure of Cold Dark Matter Halos. Astrophys. J. 1991, 378, 496. [Google Scholar] [CrossRef]
- Gelb, J.M.; Bertschinger, E. Cold Dark Matter. I. The Formation of Dark Halos. Astrophys. J. 1994, 436, 467. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Structure of Cold Dark Matter Halos. Astrophys. J. 1996, 462, 563. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. A Universal Density Profile from Hierarchical Clustering. Astrophys. J. 1997, 490, 493–508. [Google Scholar] [CrossRef]
- Fukushige, T.; Makino, J. On the Origin of Cusps in Dark Matter Halos. Astrophys. J. Lett. 1997, 477, L9–L12. [Google Scholar] [CrossRef]
- Moore, B.; Governato, F.; Quinn, T.; Stadel, J.; Lake, G. Resolving the Structure of Cold Dark Matter Halos. Astrophys. J. Lett. 1998, 499, L5–L8. [Google Scholar] [CrossRef]
- Navarro, J.F.; Ludlow, A.; Springel, V.; Wang, J.; Vogelsberger, M.; White, S.D.M.; Jenkins, A.; Frenk, C.S.; Helmi, A. The diversity and similarity of simulated cold dark matter haloes. Mon. Not. R. Astron. Soc. 2010, 402, 21–34. [Google Scholar] [CrossRef]
- Einasto, J. On the Construction of a Composite Model for the Galaxy and on the Determination of the System of Galactic Parameters. Tr. Astrofiz. Inst. Alma-Ata 1965, 5, 87–100. [Google Scholar]
- Graziani, L.; de Bennassuti, M.; Schneider, R.; Kawata, D.; Salvadori, S. The history of the dark and luminous side of Milky Way-like progenitors. Mon. Not. R. Astron. Soc. 2017, 469, 1101–1116. [Google Scholar] [CrossRef]
- Mateo, M. Strange Dark Matters in Nearby Dwarf Galaxies. In Proceedings of the Magellanic Clouds and Other Dwarf Galaxies, Bad Honnef, Germany, 19th–22 January 1998; pp. 53–66. Available online: https://ui.adsabs.harvard.edu/abs/1998mcdg.proc...53M/abstract (accessed on 27 October 2021).
- McConnachie, A.W. The Observed Properties of Dwarf Galaxies in and around the Local Group. Astron. J. 2012, 144, 4. [Google Scholar] [CrossRef]
- Moore, B. Evidence against dissipation-less dark matter from observations of galaxy haloes. Nature 1994, 370, 629–631. [Google Scholar] [CrossRef]
- Flores, R.A.; Primack, J.R. Observational and Theoretical Constraints on Singular Dark Matter Halos. Astrophys. J. Lett. 1994, 427, L1. [Google Scholar] [CrossRef]
- Burkert, A. The Structure of Dark Matter Halos in Dwarf Galaxies. Astrophys. J. Lett. 1995, 447, L25–L28. [Google Scholar] [CrossRef]
- Marchesini, D.; D’Onghia, E.; Chincarini, G.; Firmani, C.; Conconi, P.; Molinari, E.; Zacchei, A. Hα Rotation Curves: The Soft Core Question. Astrophys. J. 2002, 575, 801–813. [Google Scholar] [CrossRef]
- de Blok, W.J.G.; Bosma, A.; McGaugh, S. Simulating observations of dark matter dominated galaxies: Towards the optimal halo profile. Mon. Not. R. Astron. Soc. 2003, 340, 657–678. [Google Scholar] [CrossRef]
- Rhee, G.; Valenzuela, O.; Klypin, A.; Holtzman, J.; Moorthy, B. The Rotation Curves of Dwarf Galaxies: A Problem for Cold Dark Matter? Astrophys. J. 2004, 617, 1059–1076. [Google Scholar] [CrossRef]
- van den Bosch, F.C.; Swaters, R.A. Dwarf galaxy rotation curves and the core problem of dark matter haloes. Mon. Not. R. Astron. Soc. 2001, 325, 1017–1038. [Google Scholar] [CrossRef]
- Spekkens, K.; Giovanelli, R.; Haynes, M.P. The Cusp/Core Problem in Galactic Halos: Long-Slit Spectra for a Large Dwarf Galaxy Sample. Astron. J. 2005, 129, 2119–2137. [Google Scholar] [CrossRef]
- Walter, F.; Brinks, E.; de Blok, W.J.G.; Bigiel, F.; Kennicutt, R.C., Jr.; Thornley, M.D.; Leroy, A. THINGS: The H I Nearby Galaxy Survey. Astron. J. 2008, 136, 2563–2647. [Google Scholar] [CrossRef]
- Hunter, D.A.; Ficut-Vicas, D.; Ashley, T.; Brinks, E.; Cigan, P.; Elmegreen, B.G.; Heesen, V.; Herrmann, K.A.; Johnson, M.; Oh, S.H.; et al. Little Things. Astron. J. 2012, 144, 134. [Google Scholar] [CrossRef]
- Trachternach, C.; de Blok, W.J.G.; Walter, F.; Brinks, E.; Kennicutt, R.C., Jr. Dynamical Centers and Noncircular Motions in THINGS Galaxies: Implications for Dark Matter Halos. Astron. J. 2008, 136, 2720–2760. [Google Scholar] [CrossRef]
- Oh, S.H.; de Blok, W.J.G.; Walter, F.; Brinks, E.; Kennicutt, R.C., Jr. High-Resolution Dark Matter Density Profiles of THINGS Dwarf Galaxies: Correcting for Noncircular Motions. Astron. J. 2008, 136, 2761–2781. [Google Scholar] [CrossRef]
- de Blok, W.J.G.; Walter, F.; Brinks, E.; Trachternach, C.; Oh, S.H.; Kennicutt, R.C., Jr. High-Resolution Rotation Curves and Galaxy Mass Models from THINGS. Astron. J. 2008, 136, 2648–2719. [Google Scholar] [CrossRef]
- Oh, S.H.; de Blok, W.J.G.; Brinks, E.; Walter, F.; Kennicutt, R.C., Jr. Dark and Luminous Matter in THINGS Dwarf Galaxies. Astron. J. 2011, 141, 193. [Google Scholar] [CrossRef]
- Oh, S.H.; Hunter, D.A.; Brinks, E.; Elmegreen, B.G.; Schruba, A.; Walter, F.; Rupen, M.P.; Young, L.M.; Simpson, C.E.; Johnson, M.C.; et al. High-resolution Mass Models of Dwarf Galaxies from LITTLE THINGS. Astron. J. 2015, 149, 180. [Google Scholar] [CrossRef]
- Navarro, J.F.; Eke, V.R.; Frenk, C.S. The cores of dwarf galaxy haloes. Mon. Not. R. Astron. Soc. 1996, 283, L72–L78. [Google Scholar] [CrossRef]
- Ciardi, B.; Ferrara, A. The First Cosmic Structures and Their Effects. Space Sci. Rev. 2005, 116, 625–705. [Google Scholar] [CrossRef]
- White, S.D.M.; Frenk, C.S. Galaxy Formation through Hierarchical Clustering. Astrophys. J. 1991, 379, 52. [Google Scholar] [CrossRef]
- White, S.D.M.; Rees, M.J. Core condensation in heavy halos: A two-stage theory for galaxy formation and clustering. Mon. Not. R. Astron. Soc. 1978, 183, 341–358. [Google Scholar] [CrossRef]
- Larson, R.B. Effects of supernovae on the early evolution of galaxies. Mon. Not. R. Astron. Soc. 1974, 169, 229–246. [Google Scholar] [CrossRef]
- Dekel, A.; Silk, J. The Origin of Dwarf Galaxies, Cold Dark Matter, and Biased Galaxy Formation. Astrophys. J. 1986, 303, 39. [Google Scholar] [CrossRef]
- Blumenthal, G.R.; Faber, S.M.; Flores, R.; Primack, J.R. Contraction of Dark Matter Galactic Halos Due to Baryonic Infall. Astrophys. J. 1986, 301, 27. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Kravtsov, A.V.; Klypin, A.A.; Nagai, D. Response of Dark Matter Halos to Condensation of Baryons: Cosmological Simulations and Improved Adiabatic Contraction Model. Astrophys. J. 2004, 616, 16–26. [Google Scholar] [CrossRef]
- Abadi, M.G.; Navarro, J.F.; Fardal, M.; Babul, A.; Steinmetz, M. Galaxy-induced transformation of dark matter haloes. Mon. Not. R. Astron. Soc. 2010, 407, 435–446. [Google Scholar] [CrossRef]
- Schaller, M.; Robertson, A.; Massey, R.; Bower, R.G.; Eke, V.R. The offsets between galaxies and their dark matter in Λ cold dark matter. Mon. Not. R. Astron. Soc. 2015, 453, L58–L62. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Zhao, H. Maximum feedback and dark matter profiles of dwarf galaxies. Mon. Not. R. Astron. Soc. 2002, 333, 299–306. [Google Scholar] [CrossRef]
- Read, J.I.; Gilmore, G. Mass loss from dwarf spheroidal galaxies: The origins of shallow dark matter cores and exponential surface brightness profiles. Mon. Not. R. Astron. Soc. 2005, 356, 107–124. [Google Scholar] [CrossRef]
- Brooks, A.M.; Zolotov, A. Why Baryons Matter: The Kinematics of Dwarf Spheroidal Satellites. Astrophys. J. 2014, 786, 87. [Google Scholar] [CrossRef]
- Mashchenko, S.; Wadsley, J.; Couchman, H.M.P. Stellar Feedback in Dwarf Galaxy Formation. Science 2008, 319, 174. [Google Scholar] [CrossRef] [PubMed]
- Macciò, A.V.; Stinson, G.; Brook, C.B.; Wadsley, J.; Couchman, H.M.P.; Shen, S.; Gibson, B.K.; Quinn, T. Halo Expansion in Cosmological Hydro Simulations: Toward a Baryonic Solution of the Cusp/Core Problem in Massive Spirals. Astrophys. J. Lett. 2012, 744, L9. [Google Scholar] [CrossRef]
- Pontzen, A.; Governato, F. How supernova feedback turns dark matter cusps into cores. Mon. Not. R. Astron. Soc. 2012, 421, 3464–3471. [Google Scholar] [CrossRef]
- Pontzen, A.; Governato, F. Cold dark matter heats up. Nature 2014, 506, 171–178. [Google Scholar] [CrossRef]
- Madau, P.; Dickinson, M. Cosmic Star-Formation History. Annu. Rev. Astron. Astrophys. 2014, 52, 415–486. [Google Scholar] [CrossRef]
- Freundlich, J.; Dekel, A.; Jiang, F.; Ishai, G.; Cornuault, N.; Lapiner, S.; Dutton, A.A.; Macciò, A.V. A model for core formation in dark matter haloes and ultra-diffuse galaxies by outflow episodes. Mon. Not. R. Astron. Soc. 2020, 491, 4523–4542. [Google Scholar] [CrossRef]
- Martizzi, D.; Teyssier, R.; Moore, B. Cusp-core transformations induced by AGN feedback in the progenitors of cluster galaxies. Mon. Not. R. Astron. Soc. 2013, 432, 1947–1954. [Google Scholar] [CrossRef]
- Silk, J. Feedback by Massive Black Holes in Gas-rich Dwarf Galaxies. Astrophys. J. Lett. 2017, 839, L13. [Google Scholar] [CrossRef]
- Governato, F.; Brook, C.; Mayer, L.; Brooks, A.; Rhee, G.; Wadsley, J.; Jonsson, P.; Willman, B.; Stinson, G.; Quinn, T.; et al. Bulgeless dwarf galaxies and dark matter cores from supernova-driven outflows. Nature 2010, 463, 203–206. [Google Scholar] [CrossRef] [PubMed]
- Zolotov, A.; Brooks, A.M.; Willman, B.; Governato, F.; Pontzen, A.; Christensen, C.; Dekel, A.; Quinn, T.; Shen, S.; Wadsley, J. Baryons Matter: Why Luminous Satellite Galaxies have Reduced Central Masses. Astrophys. J. 2012, 761, 71. [Google Scholar] [CrossRef]
- Di Cintio, A.; Brook, C.B.; Dutton, A.A.; Macciò, A.V.; Stinson, G.S.; Knebe, A. A mass-dependent density profile for dark matter haloes including the influence of galaxy formation. Mon. Not. R. Astron. Soc. 2014, 441, 2986–2995. [Google Scholar] [CrossRef]
- Tollet, E.; Macciò, A.V.; Dutton, A.A.; Stinson, G.S.; Wang, L.; Penzo, C.; Gutcke, T.A.; Buck, T.; Kang, X.; Brook, C.; et al. NIHAO—IV: Core creation and destruction in dark matter density profiles across cosmic time. Mon. Not. R. Astron. Soc. 2016, 456, 3542–3552. [Google Scholar] [CrossRef]
- Hopkins, P.F.; Wetzel, A.; Kereš, D.; Faucher-Giguère, C.A.; Quataert, E.; Boylan-Kolchin, M.; Murray, N.; Hayward, C.C.; Garrison-Kimmel, S.; Hummels, C.; et al. FIRE-2 simulations: Physics versus numerics in galaxy formation. Mon. Not. R. Astron. Soc. 2018, 480, 800–863. [Google Scholar] [CrossRef]
- Chan, T.K.; Kereš, D.; Oñorbe, J.; Hopkins, P.F.; Muratov, A.L.; Faucher-Giguère, C.A.; Quataert, E. The impact of baryonic physics on the structure of dark matter haloes: The view from the FIRE cosmological simulations. Mon. Not. R. Astron. Soc. 2015, 454, 2981–3001. [Google Scholar] [CrossRef]
- Fitts, A.; Boylan-Kolchin, M.; Elbert, O.D.; Bullock, J.S.; Hopkins, P.F.; Oñorbe, J.; Wetzel, A.; Wheeler, C.; Faucher-Giguère, C.A.; Kereš, D.; et al. fire in the field: Simulating the threshold of galaxy formation. Mon. Not. R. Astron. Soc. 2017, 471, 3547–3562. [Google Scholar] [CrossRef]
- Wetzel, A.R.; Hopkins, P.F.; Kim, J.h.; Faucher-Giguère, C.A.; Kereš, D.; Quataert, E. Reconciling Dwarf Galaxies with ΛCDM Cosmology: Simulating a Realistic Population of Satellites around a Milky Way-mass Galaxy. Astrophys. J. Lett. 2016, 827, L23. [Google Scholar] [CrossRef]
- Oñorbe, J.; Boylan-Kolchin, M.; Bullock, J.S.; Hopkins, P.F.; Kereš, D.; Faucher-Giguère, C.A.; Quataert, E.; Murray, N. Forged in FIRE: Cusps, cores and baryons in low-mass dwarf galaxies. Mon. Not. R. Astron. Soc. 2015, 454, 2092–2106. [Google Scholar] [CrossRef]
- Garrison-Kimmel, S.; Wetzel, A.; Bullock, J.S.; Hopkins, P.F.; Boylan-Kolchin, M.; Faucher-Giguère, C.A.; Kereš, D.; Quataert, E.; Sanderson, R.E.; Graus, A.S.; et al. Not so lumpy after all: Modelling the depletion of dark matter subhaloes by Milky Way-like galaxies. Mon. Not. R. Astron. Soc. 2017, 471, 1709–1727. [Google Scholar] [CrossRef]
- Peirani, S.; Dubois, Y.; Volonteri, M.; Devriendt, J.; Bundy, K.; Silk, J.; Pichon, C.; Kaviraj, S.; Gavazzi, R.; Habouzit, M. Density profile of dark matter haloes and galaxies in the HORIZON-AGN simulation: The impact of AGN feedback. Mon. Not. R. Astron. Soc. 2017, 472, 2153–2169. [Google Scholar] [CrossRef]
- Schaye, J.; Dalla Vecchia, C.; Booth, C.M.; Wiersma, R.P.C.; Theuns, T.; Haas, M.R.; Bertone, S.; Duffy, A.R.; McCarthy, I.G.; van de Voort, F. The physics driving the cosmic star formation history. Mon. Not. R. Astron. Soc. 2010, 402, 1536–1560. [Google Scholar] [CrossRef]
- Duffy, A.R.; Schaye, J.; Kay, S.T.; Dalla Vecchia, C.; Battye, R.A.; Booth, C.M. Impact of baryon physics on dark matter structures: A detailed simulation study of halo density profiles. Mon. Not. R. Astron. Soc. 2010, 405, 2161–2178. [Google Scholar] [CrossRef]
- Sawala, T.; Frenk, C.S.; Fattahi, A.; Navarro, J.F.; Bower, R.G.; Crain, R.A.; Dalla Vecchia, C.; Furlong, M.; Helly, J.C.; Jenkins, A.; et al. The APOSTLE simulations: Solutions to the Local Group’s cosmic puzzles. Mon. Not. R. Astron. Soc. 2016, 457, 1931–1943. [Google Scholar] [CrossRef]
- Fattahi, A.; Navarro, J.F.; Sawala, T.; Frenk, C.S.; Oman, K.A.; Crain, R.A.; Furlong, M.; Schaller, M.; Schaye, J.; Theuns, T.; et al. The APOSTLE project: Local Group kinematic mass constraints and simulation candidate selection. Mon. Not. R. Astron. Soc. 2016, 457, 844–856. [Google Scholar] [CrossRef]
- Bose, S.; Frenk, C.S.; Jenkins, A.; Fattahi, A.; Gómez, F.A.; Grand, R.J.J.; Marinacci, F.; Navarro, J.F.; Oman, K.A.; Pakmor, R.; et al. No cores in dark matter-dominated dwarf galaxies with bursty star formation histories. Mon. Not. R. Astron. Soc. 2019, 486, 4790–4804. [Google Scholar] [CrossRef]
- Benítez-Llambay, A.; Frenk, C.S.; Ludlow, A.D.; Navarro, J.F. Baryon-induced dark matter cores in the EAGLE simulations. Mon. Not. R. Astron. Soc. 2019, 488, 2387–2404. [Google Scholar] [CrossRef]
- Power, C.; Navarro, J.F.; Jenkins, A.; Frenk, C.S.; White, S.D.M.; Springel, V.; Stadel, J.; Quinn, T. The inner structure of ΛCDM haloes—I. A numerical convergence study. Mon. Not. R. Astron. Soc. 2003, 338, 14–34. [Google Scholar] [CrossRef]
- Bullock, J.S.; Boylan-Kolchin, M. Small-Scale Challenges to the ΛCDM Paradigm. Annu. Rev. Astron. Astrophys. 2017, 55, 343–387. [Google Scholar] [CrossRef]
- Dutton, A.A.; Macciò, A.V.; Buck, T.; Dixon, K.L.; Blank, M.; Obreja, A. NIHAO XX: The impact of the star formation threshold on the cusp-core transformation of cold dark matter haloes. Mon. Not. R. Astron. Soc. 2019, 486, 655–671. [Google Scholar] [CrossRef]
- Wang, L.; Dutton, A.A.; Stinson, G.S.; Macciò, A.V.; Penzo, C.; Kang, X.; Keller, B.W.; Wadsley, J. NIHAO project—I. Reproducing the inefficiency of galaxy formation across cosmic time with a large sample of cosmological hydrodynamical simulations. Mon. Not. R. Astron. Soc. 2015, 454, 83–94. [Google Scholar] [CrossRef]
- Read, J.I.; Agertz, O.; Collins, M.L.M. Dark matter cores all the way down. Mon. Not. R. Astron. Soc. 2016, 459, 2573–2590. [Google Scholar] [CrossRef]
- Springel, V.; Pakmor, R.; Pillepich, A.; Weinberger, R.; Nelson, D.; Hernquist, L.; Vogelsberger, M.; Genel, S.; Torrey, P.; Marinacci, F.; et al. First results from the IllustrisTNG simulations: Matter and galaxy clustering. Mon. Not. R. Astron. Soc. 2018, 475, 676–698. [Google Scholar] [CrossRef]
- Oman, K.A.; Navarro, J.F.; Fattahi, A.; Frenk, C.S.; Sawala, T.; White, S.D.M.; Bower, R.; Crain, R.A.; Furlong, M.; Schaller, M.; et al. The unexpected diversity of dwarf galaxy rotation curves. Mon. Not. R. Astron. Soc. 2015, 452, 3650–3665. [Google Scholar] [CrossRef]
- Peñarrubia, J.; Pontzen, A.; Walker, M.G.; Koposov, S.E. The Coupling between the Core/Cusp and Missing Satellite Problems. Astrophys. J. Lett. 2012, 759, L42. [Google Scholar] [CrossRef]
- Revaz, Y.; Jablonka, P. Pushing back the limits: Detailed properties of dwarf galaxies in a ΛCDM universe. arXiv 2018, arXiv:1801.06222. [Google Scholar] [CrossRef]
- Garrison-Kimmel, S.; Rocha, M.; Boylan-Kolchin, M.; Bullock, J.S.; Lally, J. Can feedback solve the too-big-to-fail problem? Mon. Not. R. Astron. Soc. 2013, 433, 3539–3546. [Google Scholar] [CrossRef]
- de Blok, W.J.G. The Core-Cusp Problem. Adv. Astron. 2010, 2010, 789293. [Google Scholar] [CrossRef]
- Genina, A.; Benítez-Llambay, A.; Frenk, C.S.; Cole, S.; Fattahi, A.; Navarro, J.F.; Oman, K.A.; Sawala, T.; Theuns, T. The core-cusp problem: A matter of perspective. Mon. Not. R. Astron. Soc. 2018, 474, 1398–1411. [Google Scholar] [CrossRef]
- de Martino, I.; Chakrabarty, S.S.; Cesare, V.; Gallo, A.; Ostorero, L.; Diaferio, A. Dark Matters on the Scale of Galaxies. Universe 2020, 6, 107. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- Klypin, A.; Kravtsov, A.V.; Valenzuela, O.; Prada, F. Where Are the Missing Galactic Satellites? Astrophys. J. 1999, 522, 82–92. [Google Scholar] [CrossRef]
- Schneider, M.D.; Frenk, C.S.; Cole, S. The shapes and alignments of dark matter halos. J. Cosmol. Astropart. Phys. 2012, 2012, 030. [Google Scholar] [CrossRef]
- Boylan-Kolchin, M.; Bullock, J.S.; Kaplinghat, M. Too big to fail? The puzzling darkness of massive Milky Way subhaloes. Mon. Not. R. Astron. Soc. 2011, 415, L40–L44. [Google Scholar] [CrossRef]
- Battaglia, G.; Helmi, A.; Breddels, M. Internal kinematics and dynamical models of dwarf spheroidal galaxies around the Milky Way. New Astron. Rev. 2013, 57, 52–79. [Google Scholar] [CrossRef]
- Walker, M. Dark Matter in the Galactic Dwarf Spheroidal Satellites. In Planets, Stars and Stellar Systems; Oswalt, T.D., Gilmore, G., Eds.; Springer Science+Business Media: Dordrecht, The Netherlands, 2013; Volume 5, p. 1039. ISBN 978-94-007-5611-3. [Google Scholar] [CrossRef]
- Hammer, F.; Yang, Y.; Arenou, F.; Babusiaux, C.; Wang, J.; Puech, M.; Flores, H. Galactic Forces Rule the Dynamics of Milky Way Dwarf Galaxies. Astrophys. J. 2018, 860, 76. [Google Scholar] [CrossRef]
- Errani, R.; Peñarrubia, J.; Walker, M.G. Systematics in virial mass estimators for pressure-supported systems. Mon. Not. R. Astron. Soc. 2018, 481, 5073–5090. [Google Scholar] [CrossRef]
- Read, J.I.; Walker, M.G.; Steger, P. Dark matter heats up in dwarf galaxies. Mon. Not. R. Astron. Soc. 2019, 484, 1401–1420. [Google Scholar] [CrossRef]
- Binney, J. The radius-dependence of velocity dispersion in elliptical galaxies. Mon. Not. R. Astron. Soc. 1980, 190, 873–880. [Google Scholar] [CrossRef]
- Binney, J.; Tremaine, S. Galactic Dynamics, 2nd ed.; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Binney, J.; Mamon, G.A. M/L and velocity anisotropy from observations of spherical galaxies, of must M 87 have a massive black hole ? Mon. Not. R. Astron. Soc. 1982, 200, 361–375. [Google Scholar] [CrossRef]
- Walker, M.G.; Mateo, M.; Olszewski, E.W.; Peñarrubia, J.; Evans, N.W.; Gilmore, G. A Universal Mass Profile for Dwarf Spheroidal Galaxies? Astrophys. J. 2009, 704, 1274–1287. [Google Scholar] [CrossRef]
- Read, J.I.; Steger, P. How to break the density-anisotropy degeneracy in spherical stellar systems. Mon. Not. R. Astron. Soc. 2017, 471, 4541–4558. [Google Scholar] [CrossRef]
- Genina, A.; Read, J.I.; Frenk, C.S.; Cole, S.; Benitez-Llambay, A.; Ludlow, A.D.; Navarro, J.F.; Oman, K.A.; Robertson, A. To beta or not to beta: Can higher-order Jeans analysis break the mass-anisotropy degeneracy in simulated dwarfs? arXiv 2019, arXiv:1911.09124. [Google Scholar]
- Merrifield, M.R.; Kent, S.M. Fourth Moments and the Dynamics of Spherical Systems. Astron. J. 1990, 99, 1548. [Google Scholar] [CrossRef]
- Evans, N.W.; An, J.; Walker, M.G. Cores and cusps in the dwarf spheroidals. Mon. Not. R. Astron. Soc. 2009, 393, L50–L54. [Google Scholar] [CrossRef]
- Gilmore, G.; Wilkinson, M.I.; Wyse, R.F.G.; Kleyna, J.T.; Koch, A.; Evans, N.W.; Grebel, E.K. The Observed Properties of Dark Matter on Small Spatial Scales. Astrophys. J. 2007, 663, 948–959. [Google Scholar] [CrossRef]
- Walker, M.G.; Peñarrubia, J. A Method for Measuring (Slopes of) the Mass Profiles of Dwarf Spheroidal Galaxies. Astrophys. J. 2011, 742, 20. [Google Scholar] [CrossRef]
- Agnello, A.; Evans, N.W. A Virial Core in the Sculptor Dwarf Spheroidal Galaxy. Astrophys. J. Lett. 2012, 754, L39. [Google Scholar] [CrossRef]
- Strigari, L.E.; Frenk, C.S.; White, S.D.M. Kinematics of Milky Way satellites in a Lambda cold dark matter universe. Mon. Not. R. Astron. Soc. 2010, 408, 2364–2372. [Google Scholar] [CrossRef]
- Breddels, M.A.; Helmi, A.; van den Bosch, R.C.E.; van de Ven, G.; Battaglia, G. Orbit-based dynamical models of the Sculptor dSph galaxy. Mon. Not. R. Astron. Soc. 2013, 433, 3173–3189. [Google Scholar] [CrossRef]
- Richardson, T.; Fairbairn, M. On the dark matter profile in Sculptor: Breaking the β degeneracy with Virial shape parameters. Mon. Not. R. Astron. Soc. 2014, 441, 1584–1600. [Google Scholar] [CrossRef]
- Battaglia, G.; Helmi, A.; Tolstoy, E.; Irwin, M.; Hill, V.; Jablonka, P. The Kinematic Status and Mass Content of the Sculptor Dwarf Spheroidal Galaxy. Astrophys. J. Lett. 2008, 681, L13. [Google Scholar] [CrossRef]
- Łokas, E.L. The mass and velocity anisotropy of the Carina, Fornax, Sculptor and Sextans dwarf spheroidal galaxies. Mon. Not. R. Astron. Soc. 2009, 394, L102–L106. [Google Scholar] [CrossRef]
- Jardel, J.R.; Gebhardt, K.; Fabricius, M.H.; Drory, N.; Williams, M.J. Measuring Dark Matter Profiles Non-Parametrically in Dwarf Spheroidals: An Application to Draco. Astrophys. J. 2013, 763, 91. [Google Scholar] [CrossRef]
- Wilkinson, M.I.; Kleyna, J.; Evans, N.W.; Gilmore, G. Dark matter in dwarf spheroidals—I. Models. Mon. Not. R. Astron. Soc. 2002, 330, 778–791. [Google Scholar] [CrossRef]
- Strigari, L.E.; Bullock, J.S.; Kaplinghat, M. Determining the Nature of Dark Matter with Astrometry. Astrophys. J. Lett. 2007, 657, L1–L4. [Google Scholar] [CrossRef]
- Massari, D.; Breddels, M.A.; Helmi, A.; Posti, L.; Brown, A.G.A.; Tolstoy, E. Three-dimensional motions in the Sculptor dwarf galaxy as a glimpse of a new era. Nat. Astron. 2018, 2, 156–161. [Google Scholar] [CrossRef]
- Lazar, A.; Bullock, J.S. Accurate mass estimates from the proper motions of dispersion-supported galaxies. Mon. Not. R. Astron. Soc. 2020, 493, 5825–5837. [Google Scholar] [CrossRef]
- Hayashi, K.; Chiba, M.; Ishiyama, T. Diversity of Dark Matter Density Profiles in the Galactic Dwarf Spheroidal Satellites. Astrophys. J. 2020, 904, 45. [Google Scholar] [CrossRef]
- Irwin, M.; Hatzidimitriou, D. Structural parameters for the Galactic dwarf spheroidals. Mon. Not. R. Astron. Soc. 1995, 277, 1354–1378. [Google Scholar] [CrossRef]
- Vera-Ciro, C.A.; Sales, L.V.; Helmi, A.; Navarro, J.F. The shape of dark matter subhaloes in the Aquarius simulations. Mon. Not. R. Astron. Soc. 2014, 439, 2863–2872. [Google Scholar] [CrossRef]
- Kuhlen, M.; Diemand, J.; Madau, P. The Shapes, Orientation, and Alignment of Galactic Dark Matter Subhalos. Astrophys. J. 2007, 671, 1135–1146. [Google Scholar] [CrossRef]
- Jing, Y.P.; Suto, Y. Triaxial Modeling of Halo Density Profiles with High-Resolution N-Body Simulations. Astrophys. J. 2002, 574, 538–553. [Google Scholar] [CrossRef]
- Hayashi, K.; Chiba, M. Structural Properties of Non-spherical Dark Halos in Milky Way and Andromeda Dwarf Spheroidal Galaxies. Astrophys. J. 2015, 810, 22. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Ostriker, J.P. On the Self-consistent Response of Stellar Systems to Gravitational Shocks. Astrophys. J. 1999, 513, 626–637. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Lee, H.M.; Ostriker, J.P. Effects of Tidal Shocks on the Evolution of Globular Clusters. Astrophys. J. 1999, 522, 935–949. [Google Scholar] [CrossRef]
- Spitzer, L. Dynamical evolution of globular clusters. Annu. Rev. Astron. Astrophys. 1987, 25, 565–601. [Google Scholar]
- Hammer, F.; Yang, Y.; Wang, J.; Arenou, F.; Puech, M.; Flores, H.; Babusiaux, C. On the Absence of Dark Matter in Dwarf Galaxies Surrounding the Milky Way. Astrophys. J. 2019, 883, 171. [Google Scholar] [CrossRef]
- Hammer, F.; Yang, Y.; Arenou, F.; Wang, J.; Li, H.; Bonifacio, P.; Babusiaux, C. Orbital Evidences for Dark-matter-free Milky Way Dwarf Spheroidal Galaxies. Astrophys. J. 2020, 892, 3. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Hernquist, L.; Ostriker, J.P. Tidal Shocking by Extended Mass Distributions. Astrophys. J. 1999, 514, 109–118. [Google Scholar] [CrossRef]
- Chang, L.J.; Necib, L. Dark matter density profiles in dwarf galaxies: Linking Jeans modelling systematics and observation. Mon. Not. R. Astron. Soc. 2021, 507, 4715–4733. [Google Scholar] [CrossRef]
- Amorisco, N.C.; Evans, N.W. Dark matter cores and cusps: The case of multiple stellar populations in dwarf spheroidals. Mon. Not. R. Astron. Soc. 2012, 419, 184–196. [Google Scholar] [CrossRef]
- Adams, J.J.; Simon, J.D.; Fabricius, M.H.; van den Bosch, R.C.E.; Barentine, J.C.; Bender, R.; Gebhardt, K.; Hill, G.J.; Murphy, J.D.; Swaters, R.A.; et al. Dwarf Galaxy Dark Matter Density Profiles Inferred from Stellar and Gas Kinematics. Astrophys. J. 2014, 789, 63. [Google Scholar] [CrossRef]
- Wolf, J.; Martinez, G.D.; Bullock, J.S.; Kaplinghat, M.; Geha, M.; Muñoz, R.R.; Simon, J.D.; Avedo, F.F. Accurate masses for dispersion-supported galaxies. Mon. Not. R. Astron. Soc. 2010, 406, 1220–1237. [Google Scholar] [CrossRef]
- Macciò, A.V.; Frings, J.; Buck, T.; Penzo, C.; Dutton, A.A.; Blank, M.; Obreja, A. The edge of galaxy formation—I. Formation and evolution of MW-satellite analogues before accretion. Mon. Not. R. Astron. Soc. 2017, 472, 2356–2366. [Google Scholar] [CrossRef]
- Robles, V.H.; Bullock, J.S.; Elbert, O.D.; Fitts, A.; González-Samaniego, A.; Boylan-Kolchin, M.; Hopkins, P.F.; Faucher-Giguère, C.A.; Kereš, D.; Hayward, C.C. SIDM on FIRE: Hydrodynamical self-interacting dark matter simulations of low-mass dwarf galaxies. Mon. Not. R. Astron. Soc. 2017, 472, 2945–2954. [Google Scholar] [CrossRef] [PubMed]
- Noh, Y.; McQuinn, M. A physical understanding of how reionization suppresses accretion on to dwarf haloes. Mon. Not. R. Astron. Soc. 2014, 444, 503–514. [Google Scholar] [CrossRef]
- Bullock, J.S.; Kravtsov, A.V.; Weinberg, D.H. Reionization and the Abundance of Galactic Satellites. Astrophys. J. 2000, 539, 517–521. [Google Scholar] [CrossRef]
- Efstathiou, G. Suppressing the formation of dwarf galaxies via photoionization. Mon. Not. R. Astron. Soc. 1992, 256, 43P–47P. [Google Scholar] [CrossRef]
- Quinn, T.; Katz, N.; Efstathiou, G. Photoionization and the formation of dwarf galaxies. Mon. Not. R. Astron. Soc. 1996, 278, L49–L54. [Google Scholar] [CrossRef]
- Burger, J.D.; Zavala, J. SN-driven mechanism of cusp-core transformation: An appraisal. arXiv 2021, arXiv:2103.01231. [Google Scholar]
- Vogelsberger, M.; Zavala, J.; Simpson, C.; Jenkins, A. Dwarf galaxies in CDM and SIDM with baryons: Observational probes of the nature of dark matter. Mon. Not. R. Astron. Soc. 2014, 444, 3684–3698. [Google Scholar] [CrossRef]
- Fry, A.B.; Governato, F.; Pontzen, A.; Quinn, T.R. Self Interacting Dark Matter and Baryons. In American Astronomical Society Meeting Abstracts; American Astronomical Society: Washington, DC, USA, 2015; Volume 225, p. 402. Available online: https://ui.adsabs.harvard.edu/abs/2015AAS...22540205F/abstract (accessed on 27 October 2021).
- Burger, J.D.; Zavala, J. The nature of core formation in dark matter haloes: Adiabatic or impulsive? Mon. Not. R. Astron. Soc. 2019, 485, 1008–1028. [Google Scholar] [CrossRef]
- Ceverino, D.; Klypin, A.; Klimek, E.S.; Trujillo-Gomez, S.; Churchill, C.W.; Primack, J.; Dekel, A. Radiative feedback and the low efficiency of galaxy formation in low-mass haloes at high redshift. Mon. Not. R. Astron. Soc. 2014, 442, 1545–1559. [Google Scholar] [CrossRef]
- Wheeler, C.; Hopkins, P.F.; Pace, A.B.; Garrison-Kimmel, S.; Boylan-Kolchin, M.; Wetzel, A.; Bullock, J.S.; Kereš, D.; Faucher-Giguère, C.A.; Quataert, E. Be it therefore resolved: Cosmological simulations of dwarf galaxies with 30 solar mass resolution. Mon. Not. R. Astron. Soc. 2019, 490, 4447–4463. [Google Scholar] [CrossRef]
- Zentner, A.R.; Bullock, J.S. Halo Substructure and the Power Spectrum. Astrophys. J. 2003, 598, 49–72. [Google Scholar] [CrossRef]
- Colín, P.; Avila-Reese, V.; Valenzuela, O. Substructure and Halo Density Profiles in a Warm Dark Matter Cosmology. Astrophys. J. 2000, 542, 622–630. [Google Scholar] [CrossRef]
- Goodman, J. Repulsive dark matter. New Astron. 2000, 5, 103–107. [Google Scholar] [CrossRef]
- Hu, W.; Barkana, R.; Gruzinov, A. Fuzzy Cold Dark Matter: The Wave Properties of Ultralight Particles. Phys. Rev. Lett. 2000, 85, 1158–1161. [Google Scholar] [CrossRef]
- Kaplinghat, M.; Knox, L.; Turner, M.S. Annihilating Cold Dark Matter. Phys. Rev. Lett. 2000, 85, 3335–3338. [Google Scholar] [CrossRef]
- Peebles, P.J.E. Fluid Dark Matter. Astrophys. J. Lett. 2000, 534, L127–L129. [Google Scholar] [CrossRef]
- Sommer-Larsen, J.; Dolgov, A. Formation of Disk Galaxies: Warm Dark Matter and the Angular Momentum Problem. Astrophys. J. 2001, 551, 608–623. [Google Scholar] [CrossRef]
- Buchdahl, H.A. Non-linear Lagrangians and cosmological theory. Mon. Not. R. Astron. Soc. 1970, 150, 1. [Google Scholar] [CrossRef]
- Godani, N.; Samanta, G.C. Traversable wormholes in f(R) gravity with constant and variable redshift functions. New Astron. 2020, 80, 101399. [Google Scholar] [CrossRef]
- Bengochea, G.R.; Ferraro, R. Dark torsion as the cosmic speed-up. Phys. Rev. D 2009, 79, 124019. [Google Scholar] [CrossRef]
- Linder, E.V. Einstein’s other gravity and the acceleration of the Universe. Phys. Rev. D 2010, 81, 127301. [Google Scholar] [CrossRef]
- Dent, J.B.; Dutta, S.; Saridakis, E.N. f(T) gravity mimicking dynamical dark energy. Background and perturbation analysis. J. Cosmol. Astropart. Phys. 2011, 2011, 009. [Google Scholar] [CrossRef]
- Zheng, R.; Huang, Q.G. Growth factor in f(T) gravity. J. Cosmol. Astropart. Phys. 2011, 2011, 002. [Google Scholar] [CrossRef]
- Haghi, H.; Amiri, V. Testing modified gravity with dwarf spheroidal galaxies. Mon. Not. R. Astron. Soc. 2016, 463, 1944–1951. [Google Scholar] [CrossRef][Green Version]
- de Martino, I. Giant low-surface-brightness dwarf galaxy as a test bench for MOdified Gravity. Mon. Not. R. Astron. Soc. 2020, 493, 2373–2376. [Google Scholar] [CrossRef]
- Haghi, H.; Khodadadi, A.; Ghari, A.; Zonoozi, A.H.; Kroupa, P. Rotation curves of galaxies and the stellar mass-to-light ratio. Mon. Not. R. Astron. Soc. 2018, 477, 4187–4199. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365–370. [Google Scholar] [CrossRef]
- Famaey, B.; McGaugh, S.S. Modified Newtonian Dynamics (MOND): Observational Phenomenology and Relativistic Extensions. Living Rev. Relativ. 2012, 15, 10. [Google Scholar] [CrossRef]
- Angus, G.W. Dwarf spheroidals in MOND. Mon. Not. R. Astron. Soc. 2008, 387, 1481–1488. [Google Scholar] [CrossRef]
- Angus, G.W.; Gentile, G.; Diaferio, A.; Famaey, B.; van der Heyden, K.J. N-body simulations of the Carina dSph in MOND. Mon. Not. R. Astron. Soc. 2014, 440, 746–761. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Dynamical Friction. I. General Considerations: The Coefficient of Dynamical Friction. Astrophys. J. 1943, 97, 255. [Google Scholar] [CrossRef]
- Petts, J.A.; Read, J.I.; Gualandris, A. A semi-analytic dynamical friction model for cored galaxies. Mon. Not. R. Astron. Soc. 2016, 463, 858–869. [Google Scholar] [CrossRef][Green Version]
- El-Zant, A.; Shlosman, I.; Hoffman, Y. Dark Halos: The Flattening of the Density Cusp by Dynamical Friction. Astrophys. J. 2001, 560, 636–643. [Google Scholar] [CrossRef]
- Boldrini, P.; Mohayaee, R.; Silk, J. Flattening of Dark Matter Cusps during Mergers: Model of M31. Astrophys. J. 2021, 919, 86. [Google Scholar] [CrossRef]
- Goerdt, T.; Moore, B.; Read, J.I.; Stadel, J. Core Creation in Galaxies and Halos Via Sinking Massive Objects. Astrophys. J. 2010, 725, 1707–1716. [Google Scholar] [CrossRef][Green Version]
- Read, J.I.; Wilkinson, M.I.; Evans, N.W.; Gilmore, G.; Kleyna, J.T. The tidal stripping of satellites. Mon. Not. R. Astron. Soc. 2006, 366, 429–437. [Google Scholar] [CrossRef]
- Fitts, A.; Boylan-Kolchin, M.; Bullock, J.S.; Weisz, D.R.; El-Badry, K.; Wheeler, C.; Faucher-Giguère, C.A.; Quataert, E.; Hopkins, P.F.; Kereš, D.; et al. No assembly required: Mergers are mostly irrelevant for the growth of low-mass dwarf galaxies. Mon. Not. R. Astron. Soc. 2018, 479, 319–331. [Google Scholar] [CrossRef]
- Diemand, J.; Kuhlen, M.; Madau, P.; Zemp, M.; Moore, B.; Potter, D.; Stadel, J. Clumps and streams in the local dark matter distribution. Nature 2008, 454, 735–738. [Google Scholar] [CrossRef]
- Springel, V.; Wang, J.; Vogelsberger, M.; Ludlow, A.; Jenkins, A.; Helmi, A.; Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Aquarius Project: The subhaloes of galactic haloes. Mon. Not. R. Astron. Soc. 2008, 391, 1685–1711. [Google Scholar] [CrossRef]
- Banik, N.; Bovy, J.; Bertone, G.; Erkal, D.; de Boer, T.J.L. Evidence of a population of dark subhaloes from Gaia and Pan-STARRS observations of the GD-1 stream. Mon. Not. R. Astron. Soc. 2021, 502, 2364–2380. [Google Scholar] [CrossRef]
- Zavala, J.; Frenk, C.S. Dark Matter Haloes and Subhaloes. Galaxies 2019, 7, 81. [Google Scholar] [CrossRef]
- Boldrini, P.; Mohayaee, R.; Silk, J. Subhalo sinking and off-centre massive black holes in dwarf galaxies. Mon. Not. R. Astron. Soc. 2020, 495, L12–L16. [Google Scholar] [CrossRef]
- Wetzel, A.R. On the orbits of infalling satellite haloes. Mon. Not. R. Astron. Soc. 2011, 412, 49–58. [Google Scholar] [CrossRef]
- Orkney, M.D.A.; Read, J.I.; Rey, M.P.; Nasim, I.; Pontzen, A.; Agertz, O.; Kim, S.Y.; Delorme, M.; Dehnen, W. EDGE: Two routes to dark matter core formation in ultra-faint dwarfs. Mon. Not. R. Astron. Soc. 2021, 504, 3509–3522. [Google Scholar] [CrossRef]
- Leung, G.Y.C.; Leaman, R.; van de Ven, G.; Battaglia, G. A dwarf-dwarf merger and dark matter core as a solution to the globular cluster problems in the Fornax dSph. Mon. Not. R. Astron. Soc. 2020, 493, 320–336. [Google Scholar] [CrossRef]
- Nipoti, C.; Binney, J. Early flattening of dark matter cusps in dwarf spheroidal galaxies. Mon. Not. R. Astron. Soc. 2015, 446, 1820–1828. [Google Scholar] [CrossRef]
- Cole, D.R.; Dehnen, W.; Wilkinson, M.I. Weakening dark matter cusps by clumpy baryonic infall. Mon. Not. R. Astron. Soc. 2011, 416, 1118–1134. [Google Scholar] [CrossRef]
- Del Popolo, A.; Le Delliou, M. A unified solution to the small scale problems of the ΛCDM model II: Introducing parent-satellite interaction. J. Cosmol. Astropart. Phys. 2014, 2014, 051. [Google Scholar] [CrossRef]
- Inoue, S.; Saitoh, T.R. Cores and revived cusps of dark matter haloes in disc galaxy formation through clump clusters. Mon. Not. R. Astron. Soc. 2011, 418, 2527–2531. [Google Scholar] [CrossRef]
- Peebles, P.J.E. Dark matter and the origin of galaxies and globular star clusters. Astrophys. J. 1984, 277, 470–477. [Google Scholar] [CrossRef]
- Bromm, V.; Clarke, C.J. The Formation of the First Globular Clusters in Dwarf Galaxies before the Epoch of Reionization. Astrophys. J. Lett. 2002, 566, L1–L4. [Google Scholar] [CrossRef]
- Mashchenko, S.; Sills, A. Globular Clusters with Dark Matter Halos. II. Evolution in a Tidal Field. Astrophys. J. 2005, 619, 258–269. [Google Scholar] [CrossRef]
- Ricotti, M.; Parry, O.H.; Gnedin, N.Y. A Common Origin for Globular Clusters and Ultra-faint Dwarfs in Simulations of the First Galaxies. Astrophys. J. 2016, 831, 204. [Google Scholar] [CrossRef]
- Conroy, C.; Loeb, A.; Spergel, D.N. Evidence against Dark Matter Halos Surrounding the Globular Clusters MGC1 and NGC 2419. Astrophys. J. 2011, 741, 72. [Google Scholar] [CrossRef]
- Ibata, R.; Nipoti, C.; Sollima, A.; Bellazzini, M.; Chapman, S.C.; Dalessandro, E. Do globular clusters possess dark matter haloes? A case study in NGC 2419. Mon. Not. R. Astron. Soc. 2013, 428, 3648–3659. [Google Scholar] [CrossRef]
- Shin, J.; Kim, S.S.; Lee, Y.W. Dark Matter Content in Globular Cluster NGC 6397. J. Korean Astron. Soc. 2013, 46, 173–181. [Google Scholar] [CrossRef][Green Version]
- Moore, B. Constraints on the Global Mass-to-Light Ratios and on the Extent of Dark Matter Halos in Globular Clusters and Dwarf Spheroidals. Astrophys. J. Lett. 1996, 461, L13. [Google Scholar] [CrossRef]
- Baumgardt, H.; Côté, P.; Hilker, M.; Rejkuba, M.; Mieske, S.; Djorgovski, S.G.; Stetson, P. The velocity dispersion and mass-to-light ratio of the remote halo globular cluster NGC2419. Mon. Not. R. Astron. Soc. 2009, 396, 2051–2060. [Google Scholar] [CrossRef]
- Lane, R.R.; Kiss, L.L.; Lewis, G.F.; Ibata, R.A.; Siebert, A.; Bedding, T.R.; Székely, P.; Balog, Z.; Szabó, G.M. Halo globular clusters observed with AAOmega: Dark matter content, metallicity and tidal heating. Mon. Not. R. Astron. Soc. 2010, 406, 2732–2742. [Google Scholar] [CrossRef]
- Hurst, T.J.; Zentner, A.R.; Natarajan, A.; Badenes, C. Indirect probes of dark matter and globular cluster properties from dark matter annihilation within the coolest white dwarfs. Phys. Rev. D 2015, 91, 103514. [Google Scholar] [CrossRef]
- Peter, A.H.G.; Moody, C.E.; Kamionkowski, M. Dark-matter decays and self-gravitating halos. Phys. Rev. D 2010, 81, 103501. [Google Scholar] [CrossRef]
- Davis, A.J.; Khochfar, S.; Dalla Vecchia, C. The First Billion Years project: Dark matter haloes going from contraction to expansion and back again. Mon. Not. R. Astron. Soc. 2014, 443, 985–1001. [Google Scholar] [CrossRef]
- Saitoh, T.R.; Koda, J.; Okamoto, T.; Wada, K.; Habe, A. Tidal Disruption of Dark Matter Halos around Proto-Globular Clusters. Astrophys. J. 2006, 640, 22–30. [Google Scholar] [CrossRef]
- Bekki, K.; Yong, D. On the origin of the stellar halo and multiple stellar populations in the globular cluster NGC 1851. Mon. Not. R. Astron. Soc. 2012, 419, 2063–2076. [Google Scholar] [CrossRef]
- Pillepich, A.; Springel, V.; Nelson, D.; Genel, S.; Naiman, J.; Pakmor, R.; Hernquist, L.; Torrey, P.; Vogelsberger, M.; Weinberger, R.; et al. Simulating galaxy formation with the IllustrisTNG model. Mon. Not. R. Astron. Soc. 2018, 473, 4077–4106. [Google Scholar] [CrossRef]
- Boldrini, P.; Mohayaee, R.; Silk, J. Embedding globular clusters in dark matter minihaloes solves the cusp-core and timing problems in the Fornax dwarf galaxy. Mon. Not. R. Astron. Soc. 2020, 492, 3169–3178. [Google Scholar] [CrossRef]
- Angus, G.W.; Diaferio, A. Resolving the timing problem of the globular clusters orbiting the Fornax dwarf galaxy. Mon. Not. R. Astron. Soc. 2009, 396, 887–893. [Google Scholar] [CrossRef][Green Version]
- Sanders, J.L.; Evans, N.W.; Dehnen, W. Tidal disruption of dwarf spheroidal galaxies: The strange case of Crater II. Mon. Not. R. Astron. Soc. 2018, 478, 3879–3889. [Google Scholar] [CrossRef]
- Torrealba, G.; Belokurov, V.; Koposov, S.E.; Li, T.S.; Walker, M.G.; Sanders, J.L.; Geringer-Sameth, A.; Zucker, D.B.; Kuehn, K.; Evans, N.W.; et al. The hidden giant: Discovery of an enormous Galactic dwarf satellite in Gaia DR2. Mon. Not. R. Astron. Soc. 2019, 488, 2743–2766. [Google Scholar] [CrossRef]
- Frings, J.; Macciò, A.; Buck, T.; Penzo, C.; Dutton, A.; Blank, M.; Obreja, A. The edge of galaxy formation—II. Evolution of Milky Way satellite analogues after infall. Mon. Not. R. Astron. Soc. 2017, 472, 3378–3389. [Google Scholar] [CrossRef]
- Genina, A.; Read, J.I.; Fattahi, A.; Frenk, C.S. Can tides explain the low dark matter density in Fornax? arXiv 2020, arXiv:2011.09482. [Google Scholar] [CrossRef]
- Walker, M.G.; Mateo, M.; Olszewski, E.W.; Bernstein, R.; Wang, X.; Woodroofe, M. Internal Kinematics of the Fornax Dwarf Spheroidal Galaxy. Astron. J. 2006, 131, 2114–2139. [Google Scholar] [CrossRef]
- Wang, M.Y.; de Boer, T.; Pieres, A.; Li, T.S.; Drlica-Wagner, A.; Koposov, S.E.; Vivas, A.K.; Pace, A.B.; Santiago, B.; Walker, A.R.; et al. The Morphology and Structure of Stellar Populations in the Fornax Dwarf Spheroidal Galaxy from Dark Energy Survey Data. Astrophys. J. 2019, 881, 118. [Google Scholar] [CrossRef]
- Laporte, C.F.P.; Penarrubia, J. Under the sword of Damocles: Plausible regeneration of dark matter cusps at the smallest galactic scales. Mon. Not. R. Astron. Soc. 2015, 449, L90–L94. [Google Scholar] [CrossRef]
- Dekel, A.; Devor, J.; Hetzroni, G. Galactic halo cusp-core: Tidal compression in mergers. Mon. Not. R. Astron. Soc. 2003, 341, 326–342. [Google Scholar] [CrossRef]
- Peñarrubia, J.; Benson, A.J.; Walker, M.G.; Gilmore, G.; McConnachie, A.W.; Mayer, L. The impact of dark matter cusps and cores on the satellite galaxy population around spiral galaxies. Mon. Not. R. Astron. Soc. 2010, 406, 1290–1305. [Google Scholar] [CrossRef]
- Dutton, A.A.; Macciò, A.V. Cold dark matter haloes in the Planck era: Evolution of structural parameters for Einasto and NFW profiles. Mon. Not. R. Astron. Soc. 2014, 441, 3359–3374. [Google Scholar] [CrossRef]
- Brook, C.B.; Stinson, G.; Gibson, B.K.; Wadsley, J.; Quinn, T. MaGICC discs: Matching observed galaxy relationships over a wide stellar mass range. Mon. Not. R. Astron. Soc. 2012, 424, 1275–1283. [Google Scholar] [CrossRef][Green Version]
- Teyssier, R.; Pontzen, A.; Dubois, Y.; Read, J.I. Cusp-core transformations in dwarf galaxies: Observational predictions. Mon. Not. R. Astron. Soc. 2013, 429, 3068–3078. [Google Scholar] [CrossRef]
- Madau, P.; Shen, S.; Governato, F. Dark Matter Heating and Early Core Formation in Dwarf Galaxies. Astrophys. J. Lett. 2014, 789, L17. [Google Scholar] [CrossRef]
- McGaugh, S. Predictions and Outcomes for the Dynamics of Rotating Galaxies. Galaxies 2020, 8, 35. [Google Scholar] [CrossRef]
- Bode, P.; Ostriker, J.P.; Turok, N. Halo Formation in Warm Dark Matter Models. Astrophys. J. 2001, 556, 93–107. [Google Scholar] [CrossRef]
- Schneider, A.; Smith, R.E.; Macciò, A.V.; Moore, B. Non-linear evolution of cosmological structures in warm dark matter models. Mon. Not. R. Astron. Soc. 2012, 424, 684–698. [Google Scholar] [CrossRef]
- Macciò, A.V.; Paduroiu, S.; Anderhalden, D.; Schneider, A.; Moore, B. Cores in warm dark matter haloes: A Catch 22 problem. Mon. Not. R. Astron. Soc. 2012, 424, 1105–1112. [Google Scholar] [CrossRef]
- Shao, S.; Gao, L.; Theuns, T.; Frenk, C.S. The phase-space density of fermionic dark matter haloes. Mon. Not. R. Astron. Soc. 2013, 430, 2346–2357. [Google Scholar] [CrossRef]
- Lovell, M.R.; Frenk, C.S.; Eke, V.R.; Jenkins, A.; Gao, L.; Theuns, T. The properties of warm dark matter haloes. Mon. Not. R. Astron. Soc. 2014, 439, 300–317. [Google Scholar] [CrossRef]
- Kochanek, C.S.; White, M. A Quantitative Study of Interacting Dark Matter in Halos. Astrophys. J. 2000, 543, 514–520. [Google Scholar] [CrossRef]
- Miralda-Escudé, J. A Test of the Collisional Dark Matter Hypothesis from Cluster Lensing. Astrophys. J. 2002, 564, 60–64. [Google Scholar] [CrossRef]
- Zavala, J.; Vogelsberger, M.; Walker, M.G. Constraining self-interacting dark matter with the Milky way’s dwarf spheroidals. Mon. Not. R. Astron. Soc. 2013, 431, L20–L24. [Google Scholar] [CrossRef]
- Elbert, O.D.; Bullock, J.S.; Garrison-Kimmel, S.; Rocha, M.; Oñorbe, J.; Peter, A.H.G. Core formation in dwarf haloes with self-interacting dark matter: No fine-tuning necessary. Mon. Not. R. Astron. Soc. 2015, 453, 29–37. [Google Scholar] [CrossRef]
- Burkert, A. The Structure and Evolution of Weakly Self-interacting Cold Dark Matter Halos. Astrophys. J. Lett. 2000, 534, L143–L146. [Google Scholar] [CrossRef]
- Spergel, D.N.; Steinhardt, P.J. Observational Evidence for Self-Interacting Cold Dark Matter. Phys. Rev. Lett. 2000, 84, 3760–3763. [Google Scholar] [CrossRef]
- Hui, L.; Ostriker, J.P.; Tremaine, S.; Witten, E. Ultralight scalars as cosmological dark matter. Phys. Rev. D 2017, 95, 043541. [Google Scholar] [CrossRef]
- Hui, L.; Joyce, A.; Landry, M.J.; Li, X. Vortices and waves in light dark matter. J. Cosmol. Astropart. Phys. 2021, 2021, 011. [Google Scholar] [CrossRef]
- Mocz, P.; Fialkov, A.; Vogelsberger, M.; Becerra, F.; Amin, M.A.; Bose, S.; Boylan-Kolchin, M.; Chavanis, P.H.; Hernquist, L.; Lancaster, L.; et al. First Star-Forming Structures in Fuzzy Cosmic Filaments. Phys. Rev. Lett. 2019, 123, 141301. [Google Scholar] [CrossRef]
- Nori, M.; Murgia, R.; Iršič, V.; Baldi, M.; Viel, M. Lyman α forest and non-linear structure characterization in Fuzzy Dark Matter cosmologies. Mon. Not. R. Astron. Soc. 2019, 482, 3227–3243. [Google Scholar] [CrossRef]
- Marsh, D.J.E.; Silk, J. A model for halo formation with axion mixed dark matter. Mon. Not. R. Astron. Soc. 2014, 437, 2652–2663. [Google Scholar] [CrossRef]
- Chavanis, P.H. Derivation of a generalized Schrödinger equation for dark matter halos from the theory of scale relativity. Phys. Dark Univ. 2018, 22, 80–95. [Google Scholar] [CrossRef]
- Schive, H.Y.; Chiueh, T.; Broadhurst, T. Cosmic structure as the quantum interference of a coherent dark wave. Nat. Phys. 2014, 10, 496–499. [Google Scholar] [CrossRef]
- Boldrini, P.; Miki, Y.; Wagner, A.Y.; Mohayaee, R.; Silk, J.; Arbey, A. Cusp-to-core transition in low-mass dwarf galaxies induced by dynamical heating of cold dark matter by primordial black holes. Mon. Not. R. Astron. Soc. 2020, 492, 5218–5225. [Google Scholar] [CrossRef]
- Marsh, D.J.E. Axion cosmology. Phys. Rep. 2016, 643, 1–79. [Google Scholar] [CrossRef]
- Widrow, L.M.; Kaiser, N. Using the Schroedinger Equation to Simulate Collisionless Matter. Astrophys. J. Lett. 1993, 416, L71. [Google Scholar] [CrossRef]
- Woo, T.P.; Chiueh, T. High-Resolution Simulation on Structure Formation with Extremely Light Bosonic Dark Matter. Astrophys. J. 2009, 697, 850–861. [Google Scholar] [CrossRef]
- Böhmer, C.G.; Harko, T. Can dark matter be a Bose Einstein condensate? J. Cosmol. Astropart. Phys. 2007, 2007, 025. [Google Scholar] [CrossRef]
- Robles, V.H.; Bullock, J.S.; Boylan-Kolchin, M. Scalar field dark matter: Helping or hurting small-scale problems in cosmology? Mon. Not. R. Astron. Soc. 2019, 483, 289–298. [Google Scholar] [CrossRef]
- Lee, J.W.; Lim, S. Minimum mass of galaxies from BEC or scalar field dark matter. J. Cosmol. Astropart. Phys. 2010, 2010, 007. [Google Scholar] [CrossRef]
- El-Zant, A.A. Galaxy formation and dark matter: Small scale problems and quantum effects on astrophysical scales. J. Phys. Conf. Ser. 2019, 1253, 012007. [Google Scholar] [CrossRef]
- Veltmaat, J.; Schwabe, B.; Niemeyer, J.C. Baryon-driven growth of solitonic cores in fuzzy dark matter halos. Phys. Rev. D 2020, 101, 083518. [Google Scholar] [CrossRef]
- Safarzadeh, M.; Spergel, D.N. Ultra-light Dark Matter Is Incompatible with the Milky Way’s Dwarf Satellites. Astrophys. J. 2020, 893, 21. [Google Scholar] [CrossRef]
- Davies, E.Y.; Mocz, P. Fuzzy dark matter soliton cores around supermassive black holes. Mon. Not. R. Astron. Soc. 2020, 492, 5721–5729. [Google Scholar] [CrossRef]
- Bar, N.; Blum, K.; Lacroix, T.; Panci, P. Looking for ultralight dark matter near supermassive black holes. J. Cosmol. Astropart. Phys. 2019, 2019, 045. [Google Scholar] [CrossRef]
- Desjacques, V.; Nusser, A. Axion core-halo mass and the black hole-halo mass relation: Constraints on a few parsec scales. Mon. Not. R. Astron. Soc. 2019, 488, 4497–4503. [Google Scholar] [CrossRef]
- Davoudiasl, H.; Denton, P.B. Ultralight Boson Dark Matter and Event Horizon Telescope Observations of M 87*. Phys. Rev. Lett. 2019, 123, 021102. [Google Scholar] [CrossRef]
- Deng, H.; Hertzberg, M.P.; Namjoo, M.H.; Masoumi, A. Can light dark matter solve the core-cusp problem? Phys. Rev. D 2018, 98, 023513. [Google Scholar] [CrossRef]
- González-Morales, A.X.; Marsh, D.J.E.; Peñarrubia, J.; Ureña-López, L.A. Unbiased constraints on ultralight axion mass from dwarf spheroidal galaxies. Mon. Not. R. Astron. Soc. 2017, 472, 1346–1360. [Google Scholar] [CrossRef]
- Marsh, D.J.E.; Pop, A.R. Axion dark matter, solitons and the cusp-core problem. Mon. Not. R. Astron. Soc. 2015, 451, 2479–2492. [Google Scholar] [CrossRef]
- Schive, H.Y.; Liao, M.H.; Woo, T.P.; Wong, S.K.; Chiueh, T.; Broadhurst, T.; Hwang, W.Y.P. Understanding the Core-Halo Relation of Quantum Wave Dark Matter from 3D Simulations. Phys. Rev. Lett. 2014, 113, 261302. [Google Scholar] [CrossRef]
- De Martino, I.; Broadhurst, T.; Henry Tye, S.H.; Chiueh, T.; Schive, H.Y. Dynamical evidence of a dark solitonic core of 109M⊙ in the milky way. Phys. Dark Univ. 2020, 28, 100503. [Google Scholar] [CrossRef]
- Chen, S.R.; Schive, H.Y.; Chiueh, T. Jeans analysis for dwarf spheroidal galaxies in wave dark matter. Mon. Not. R. Astron. Soc. 2017, 468, 1338–1348. [Google Scholar] [CrossRef]
- Martin, N.F.; Ibata, R.A.; Collins, M.L.M.; Rich, R.M.; Bell, E.F.; Ferguson, A.M.N.; Laevens, B.P.M.; Rix, H.W.; Chapman, S.C.; Koch, A. Triangulum II: A Very Metal-poor and Dynamically Hot Stellar System. Astrophys. J. 2016, 818, 40. [Google Scholar] [CrossRef]
- Di Paolo, C.; Salucci, P. Fundamental properties of the dark and the luminous matter from Low Surface Brightness discs. arXiv 2020, arXiv:2005.03520. [Google Scholar]
- Salucci, P.; Burkert, A. Dark Matter Scaling Relations. Astrophys. J. Lett. 2000, 537, L9–L12. [Google Scholar] [CrossRef]
- Burkert, A. Fuzzy Dark Matter and Dark Matter Halo Cores. Astrophys. J. 2020, 904, 161. [Google Scholar] [CrossRef]
- Mina, M.; Mota, D.F.; Winther, H.A. Solitons in the dark: Non-linear structure formation with fuzzy dark matter. arXiv 2020, arXiv:2007.04119. [Google Scholar]
- Jowett Chan, H.Y.; Ferreira, E.G.M.; May, S.; Hayashi, K.; Chiba, M. The Diversity of Core Halo Structure in the Fuzzy Dark Matter Model. arXiv 2021, arXiv:2110.11882. [Google Scholar]
- Mocz, P.; Vogelsberger, M.; Robles, V.H.; Zavala, J.; Boylan-Kolchin, M.; Fialkov, A.; Hernquist, L. Galaxy formation with BECDM—I. Turbulence and relaxation of idealized haloes. Mon. Not. R. Astron. Soc. 2017, 471, 4559–4570. [Google Scholar] [CrossRef]
- Schwabe, B.; Niemeyer, J.C.; Engels, J.F. Simulations of solitonic core mergers in ultralight axion dark matter cosmologies. Phys. Rev. D 2016, 94, 043513. [Google Scholar] [CrossRef]
- Nori, M.; Baldi, M. Scaling relations of fuzzy dark matter haloes—I. Individual systems in their cosmological environment. Mon. Not. R. Astron. Soc. 2021, 501, 1539–1556. [Google Scholar] [CrossRef]
- de Laix, A.A.; Scherrer, R.J.; Schaefer, R.K. Constraints on Self-interacting Dark Matter. Astrophys. J. 1995, 452, 495. [Google Scholar] [CrossRef]
- Carlson, E.D.; Machacek, M.E.; Hall, L.J. Self-interacting Dark Matter. Astrophys. J. 1992, 398, 43. [Google Scholar] [CrossRef]
- Robertson, A.; Massey, R.; Eke, V. What does the Bullet Cluster tell us about self-interacting dark matter? Mon. Not. R. Astron. Soc. 2017, 465, 569–587. [Google Scholar] [CrossRef]
- Rocha, M.; Peter, A.H.G.; Bullock, J.S.; Kaplinghat, M.; Garrison-Kimmel, S.; Oñorbe, J.; Moustakas, L.A. Cosmological simulations with self-interacting dark matter—I. Constant-density cores and substructure. Mon. Not. R. Astron. Soc. 2013, 430, 81–104. [Google Scholar] [CrossRef]
- Peter, A.H.G.; Rocha, M.; Bullock, J.S.; Kaplinghat, M. Cosmological simulations with self-interacting dark matter—II. Halo shapes versus observations. Mon. Not. R. Astron. Soc. 2013, 430, 105–120. [Google Scholar] [CrossRef]
- Vogelsberger, M.; Zavala, J.; Loeb, A. Subhaloes in self-interacting galactic dark matter haloes. Mon. Not. R. Astron. Soc. 2012, 423, 3740–3752. [Google Scholar] [CrossRef]
- Colín, P.; Avila-Reese, V.; Valenzuela, O.; Firmani, C. Structure and Subhalo Population of Halos in a Self-interacting Dark Matter Cosmology. Astrophys. J. 2002, 581, 777–793. [Google Scholar] [CrossRef]
- Davé, R.; Spergel, D.N.; Steinhardt, P.J.; Wandelt, B.D. Halo Properties in Cosmological Simulations of Self-interacting Cold Dark Matter. Astrophys. J. 2001, 547, 574–589. [Google Scholar] [CrossRef]
- Yoshida, N.; Springel, V.; White, S.D.M.; Tormen, G. Weakly Self-interacting Dark Matter and the Structure of Dark Halos. Astrophys. J. Lett. 2000, 544, L87–L90. [Google Scholar] [CrossRef]
- Yoshida, N.; Springel, V.; White, S.D.M.; Tormen, G. Collisional Dark Matter and the Structure of Dark Halos. Astrophys. J. Lett. 2000, 535, L103–L106. [Google Scholar] [CrossRef]
- D’Onghia, E.; Burkert, A. The Failure of Self-Interacting Dark Matter to Solve the Overabundance of Dark Satellites and the Soft Core Question. Astrophys. J. 2003, 586, 12–16. [Google Scholar] [CrossRef]
- Tulin, S.; Yu, H.B. Dark matter self-interactions and small scale structure. Phys. Rep. 2018, 730, 1–57. [Google Scholar] [CrossRef]
- Kaplinghat, M.; Tulin, S.; Yu, H.B. Dark Matter Halos as Particle Colliders: Unified Solution to Small-Scale Structure Puzzles from Dwarfs to Clusters. Phys. Rev. Lett. 2016, 116, 041302. [Google Scholar] [CrossRef]
- Zavala, J.; Lovell, M.R.; Vogelsberger, M.; Burger, J.D. Diverse dark matter density at sub-kiloparsec scales in Milky Way satellites: Implications for the nature of dark matter. Phys. Rev. D 2019, 100, 063007. [Google Scholar] [CrossRef]
- Loeb, A.; Weiner, N. Cores in Dwarf Galaxies from Dark Matter with a Yukawa Potential. Phys. Rev. Lett. 2011, 106, 171302. [Google Scholar] [CrossRef]
- Feng, J.L.; Kaplinghat, M.; Yu, H.B. Halo-Shape and Relic-Density Exclusions of Sommerfeld-Enhanced Dark Matter Explanations of Cosmic Ray Excesses. Phys. Rev. Lett. 2010, 104, 151301. [Google Scholar] [CrossRef] [PubMed]
- Lynden-Bell, D.; Wood, R. The gravo-thermal catastrophe in isothermal spheres and the onset of red-giant structure for stellar systems. Mon. Not. R. Astron. Soc. 1968, 138, 495. [Google Scholar] [CrossRef]
- Balberg, S.; Shapiro, S.L.; Inagaki, S. Self-Interacting Dark Matter Halos and the Gravothermal Catastrophe. Astrophys. J. 2002, 568, 475–487. [Google Scholar] [CrossRef]
- Koda, J.; Shapiro, P.R. Gravothermal collapse of isolated self-interacting dark matter haloes: N-body simulation versus the fluid model. Mon. Not. R. Astron. Soc. 2011, 415, 1125–1137. [Google Scholar] [CrossRef]
- Pollack, J.; Spergel, D.N.; Steinhardt, P.J. Supermassive Black Holes from Ultra-strongly Self-interacting Dark Matter. Astrophys. J. 2015, 804, 131. [Google Scholar] [CrossRef]
- Nishikawa, H.; Boddy, K.K.; Kaplinghat, M. Accelerated core collapse in tidally stripped self-interacting dark matter halos. Phys. Rev. D 2020, 101, 063009. [Google Scholar] [CrossRef]
- Sameie, O.; Yu, H.B.; Sales, L.V.; Vogelsberger, M.; Zavala, J. Self-Interacting Dark Matter Subhalos in the Milky Way’s Tides. Phys. Rev. Lett. 2020, 124, 141102. [Google Scholar] [CrossRef]
- Kahlhoefer, F.; Kaplinghat, M.; Slatyer, T.R.; Wu, C.L. Diversity in density profiles of self-interacting dark matter satellite halos. J. Cosmol. Astropart. Phys. 2019, 2019, 010. [Google Scholar] [CrossRef]
- Fry, A.B.; Governato, F.; Pontzen, A.; Quinn, T.; Tremmel, M.; Anderson, L.; Menon, H.; Brooks, A.M.; Wadsley, J. All about baryons: Revisiting SIDM predictions at small halo masses. Mon. Not. R. Astron. Soc. 2015, 452, 1468–1479. [Google Scholar] [CrossRef]
- Elbert, O.D.; Bullock, J.S.; Kaplinghat, M.; Garrison-Kimmel, S.; Graus, A.S.; Rocha, M. A Testable Conspiracy: Simulating Baryonic Effects on Self-interacting Dark Matter Halos. Astrophys. J. 2018, 853, 109. [Google Scholar] [CrossRef]
- Kaplinghat, M.; Keeley, R.E.; Linden, T.; Yu, H.B. Tying Dark Matter to Baryons with Self-Interactions. Phys. Rev. Lett. 2014, 113, 021302. [Google Scholar] [CrossRef]
- Kamada, A.; Kaplinghat, M.; Pace, A.B.; Yu, H.B. Self-Interacting Dark Matter Can Explain Diverse Galactic Rotation Curves. Phys. Rev. Lett. 2017, 119, 111102. [Google Scholar] [CrossRef]
- Creasey, P.; Sameie, O.; Sales, L.V.; Yu, H.B.; Vogelsberger, M.; Zavala, J. Spreading out and staying sharp—Creating diverse rotation curves via baryonic and self-interaction effects. Mon. Not. R. Astron. Soc. 2017, 468, 2283–2295. [Google Scholar] [CrossRef]
- Ren, T.; Kwa, A.; Kaplinghat, M.; Yu, H.B. Reconciling the Diversity and Uniformity of Galactic Rotation Curves with Self-Interacting Dark Matter. Phys. Rev. X 2019, 9, 031020. [Google Scholar] [CrossRef]
- Kaplinghat, M.; Ren, T.; Yu, H.B. Dark Matter Cores and Cusps in Spiral Galaxies and their Explanations. arXiv 2019, arXiv:1911.00544. [Google Scholar] [CrossRef]
- Fitts, A.; Boylan-Kolchin, M.; Bozek, B.; Bullock, J.S.; Graus, A.; Robles, V.; Hopkins, P.F.; El-Badry, K.; Garrison-Kimmel, S.; Faucher-Giguère, C.A.; et al. Dwarf galaxies in CDM, WDM, and SIDM: Disentangling baryons and dark matter physics. Mon. Not. R. Astron. Soc. 2019, 490, 962–977. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M. SPARC: Mass Models for 175 Disk Galaxies with Spitzer Photometry and Accurate Rotation Curves. Astron. J. 2016, 152, 157. [Google Scholar] [CrossRef]
- Zel’dovich, Y.B.; Novikov, I.D. The Hypothesis of Cores Retarded during Expansion and the Hot Cosmological Model. Sov. Astron. 1967, 10, 602. [Google Scholar]
- Hawking, S. Gravitationally collapsed objects of very low mass. Mon. Not. R. Astron. Soc. 1971, 152, 75. [Google Scholar] [CrossRef]
- Khlopov, M.Y. Primordial black holes. Res. Astron. Astrophys. 2010, 10, 495–528. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. Seven hints for primordial black hole dark matter. Phys. Dark Univ. 2018, 22, 137–146. [Google Scholar] [CrossRef]
- Inomata, K.; Kawasaki, M.; Mukaida, K.; Tada, Y.; Yanagida, T.T. Inflationary primordial black holes as all dark matter. Phys. Rev. D 2017, 96, 043504. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. Massive primordial black holes from hybrid inflation as dark matter and the seeds of galaxies. Phys. Rev. D 2015, 92, 023524. [Google Scholar] [CrossRef]
- Carr, B.; Kuhnel, F. Primordial Black Holes as Dark Matter Candidates. arXiv 2021, arXiv:2110.02821. [Google Scholar]
- Carr, B.; Raidal, M.; Tenkanen, T.; Vaskonen, V.; Veermäe, H. Primordial black hole constraints for extended mass functions. Phys. Rev. D 2017, 96, 023514. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Amaro-Seoane, P.; Audley, H.; Babak, S.; Baker, J.; Barausse, E.; Bender, P.; Berti, E.; Binetruy, P.; Born, M.; Bortoluzzi, D.; et al. Laser Interferometer Space Antenna. arXiv 2017, arXiv:1702.00786. [Google Scholar]
- Spitzer, L., Jr. Equipartition and the Formation of Compact Nuclei in Spherical Stellar Systems. Astrophys. J. Lett. 1969, 158, L139. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Stochastic Problems in Physics and Astronomy. Rev. Mod. Phys. 1943, 15, 1–89. [Google Scholar] [CrossRef]
- Brandt, T.D. Constraints on MACHO Dark Matter from Compact Stellar Systems in Ultra-faint Dwarf Galaxies. Astrophys. J. Lett. 2016, 824, L31. [Google Scholar] [CrossRef]
- Koushiappas, S.M.; Loeb, A. Dynamics of Dwarf Galaxies Disfavor Stellar-Mass Black Holes as Dark Matter. Phys. Rev. Lett. 2017, 119, 041102. [Google Scholar] [CrossRef]
- Zhu, Q.; Vasiliev, E.; Li, Y.; Jing, Y. Primordial black holes as dark matter: Constraints from compact ultra-faint dwarfs. Mon. Not. R. Astron. Soc. 2018, 476, 2–11. [Google Scholar] [CrossRef]
- Inman, D.; Ali-Haïmoud, Y. Early structure formation in primordial black hole cosmologies. Phys. Rev. D 2019, 100, 083528. [Google Scholar] [CrossRef]
- Collaboration, G.; Brown, A.G.A.; Vallenari, A.; Prusti, T.; de Bruijne, J.H.J.; Babusiaux, C.; Biermann, M.; Creevey, O.L.; Evans, D.W.; Eyer, L.; et al. Gaia Early Data Release 3. Summary of the contents and survey properties. Astron. Astrophys. 2021, 649, A1. [Google Scholar] [CrossRef]
- Ebisu, T.; Ishiyama, T.; Hayashi, K. Constraining Self-Interacting Dark Matter with Dwarf Spheroidal Galaxies and High-resolution Cosmological N-body Simulations. arXiv 2021, arXiv:2107.05967. [Google Scholar]
- Burger, J.D.; Zavala, J.; Sales, L.V.; Vogelsberger, M.; Marinacci, F.; Torrey, P. Degeneracies Between Self-interacting Dark Matter and Supernova Feedback as cusp-core transformation mechanisms. arXiv 2021, arXiv:2108.07358. [Google Scholar]
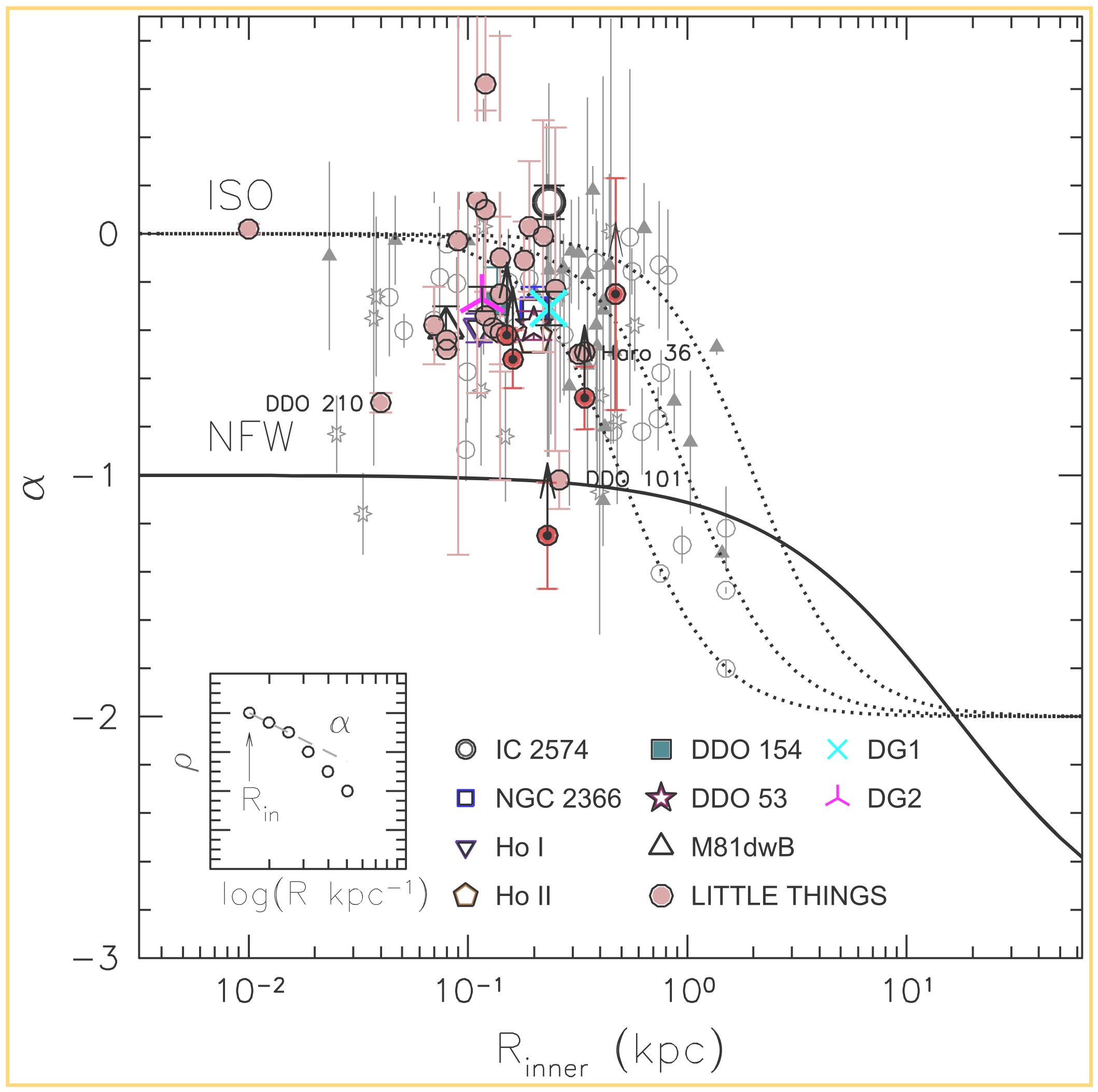
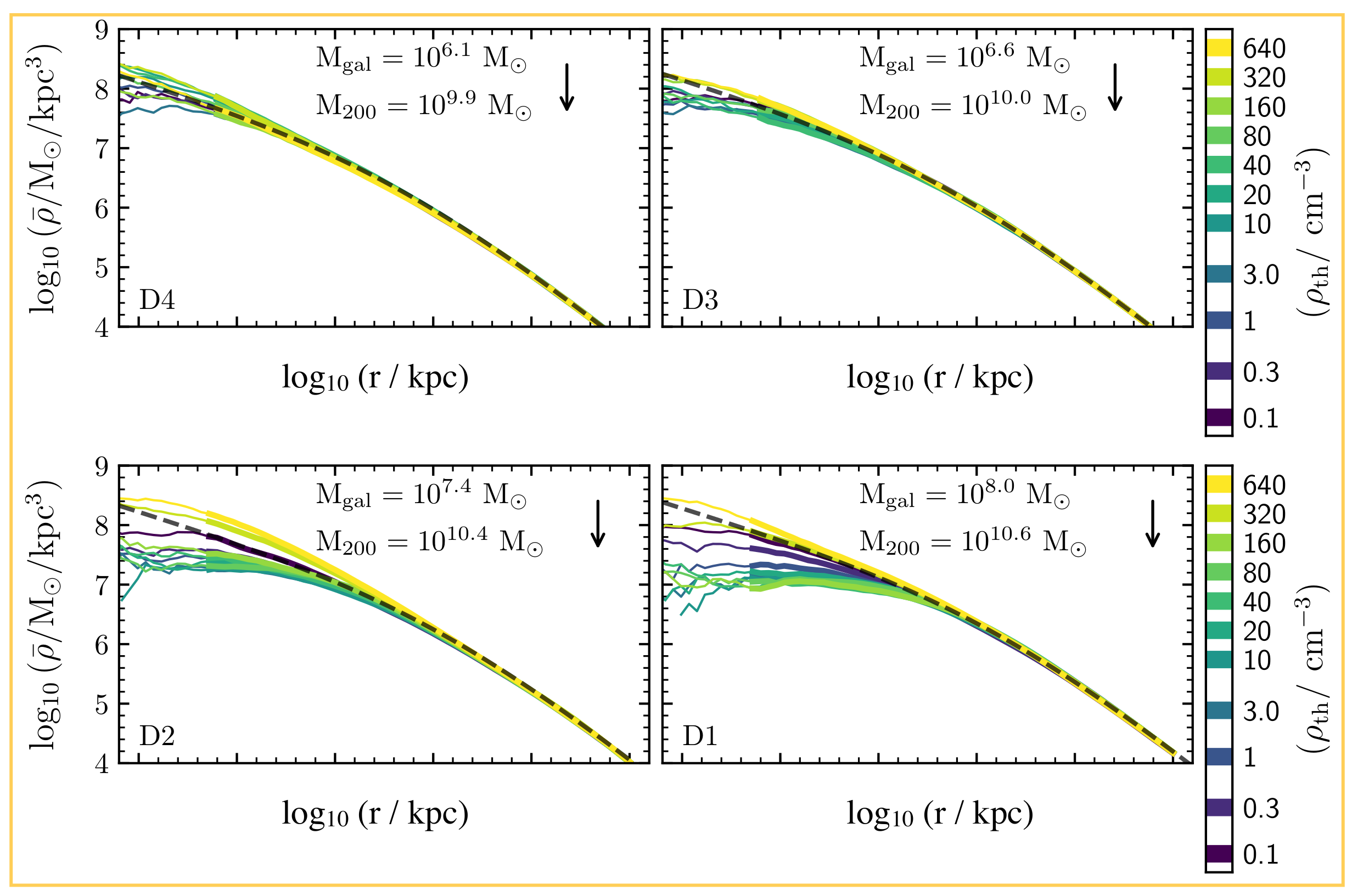


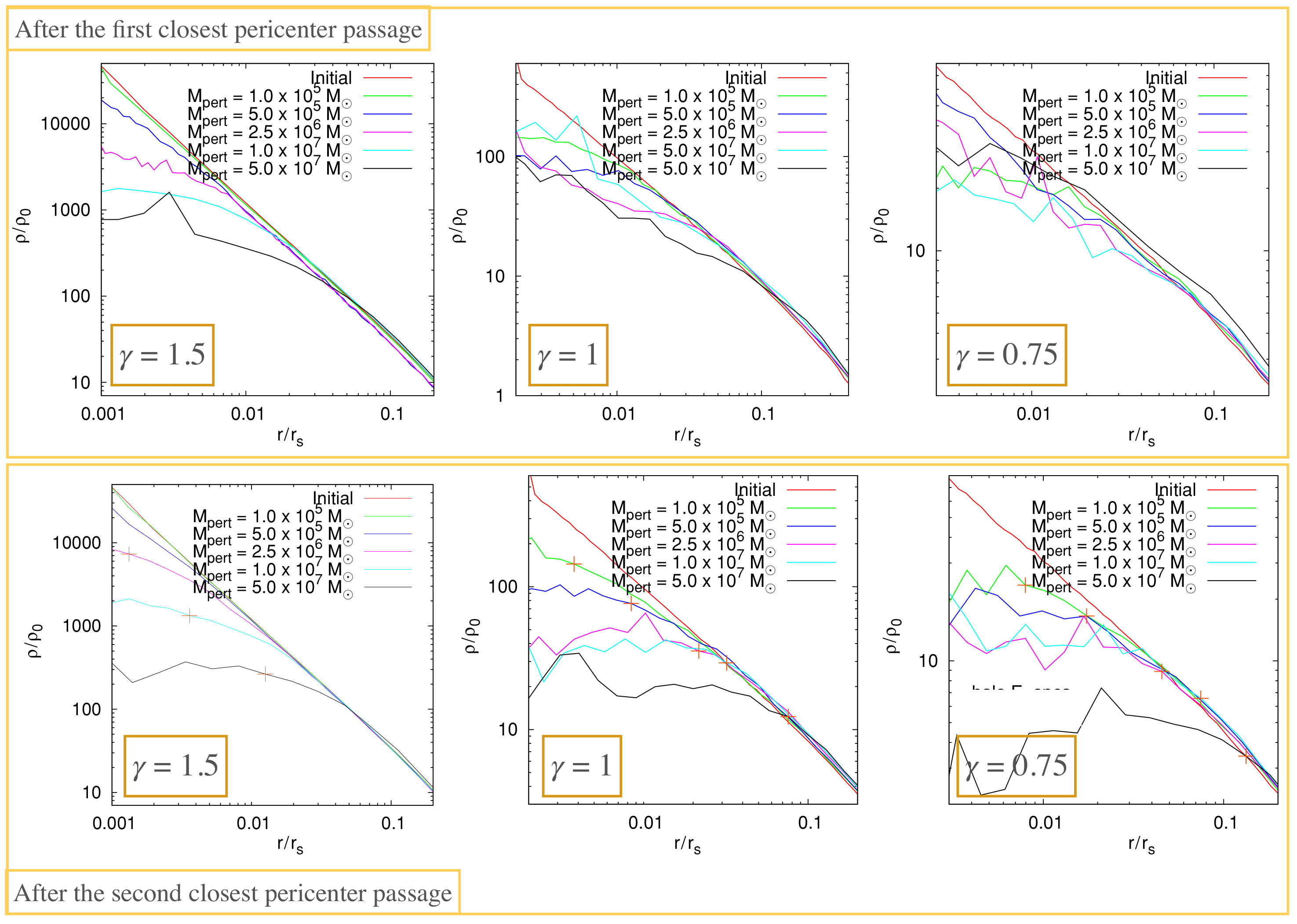
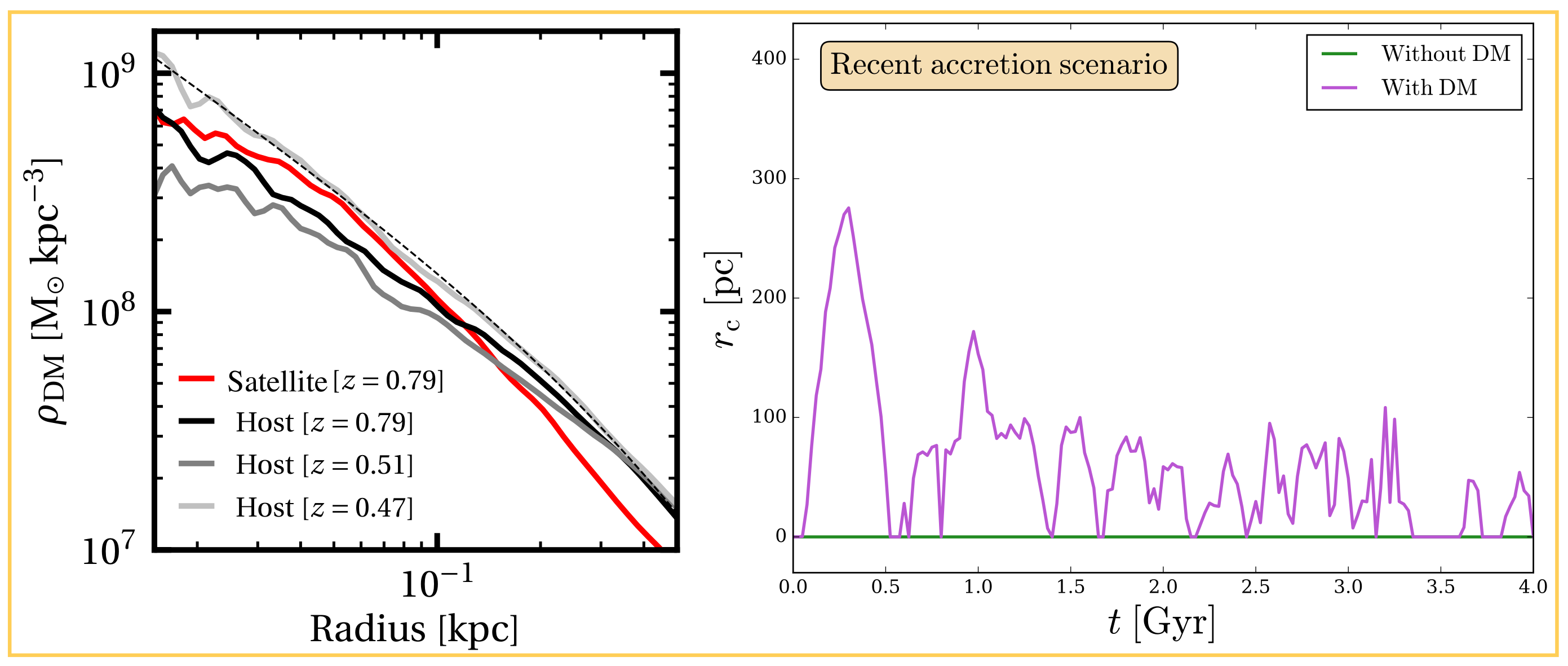


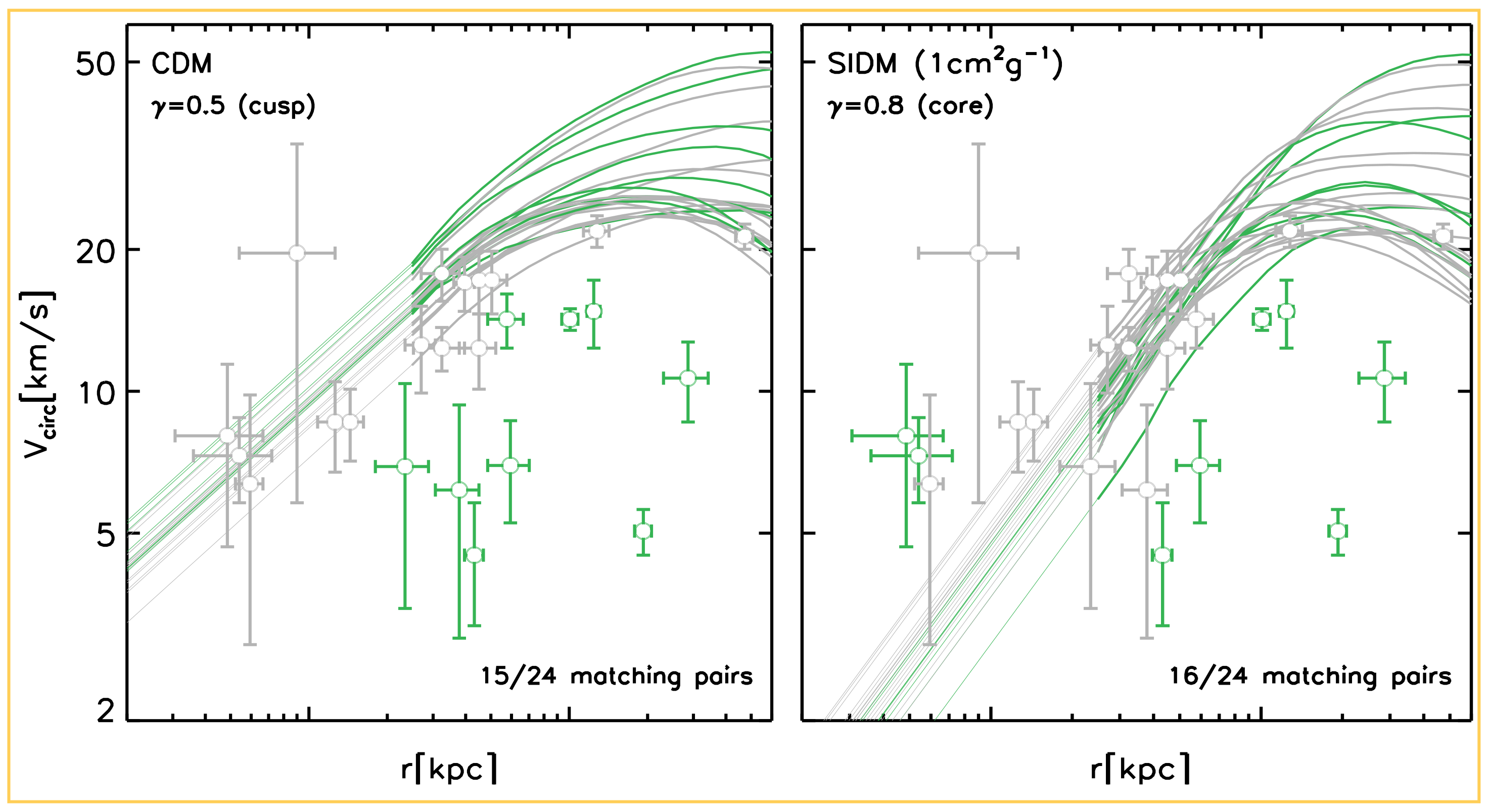
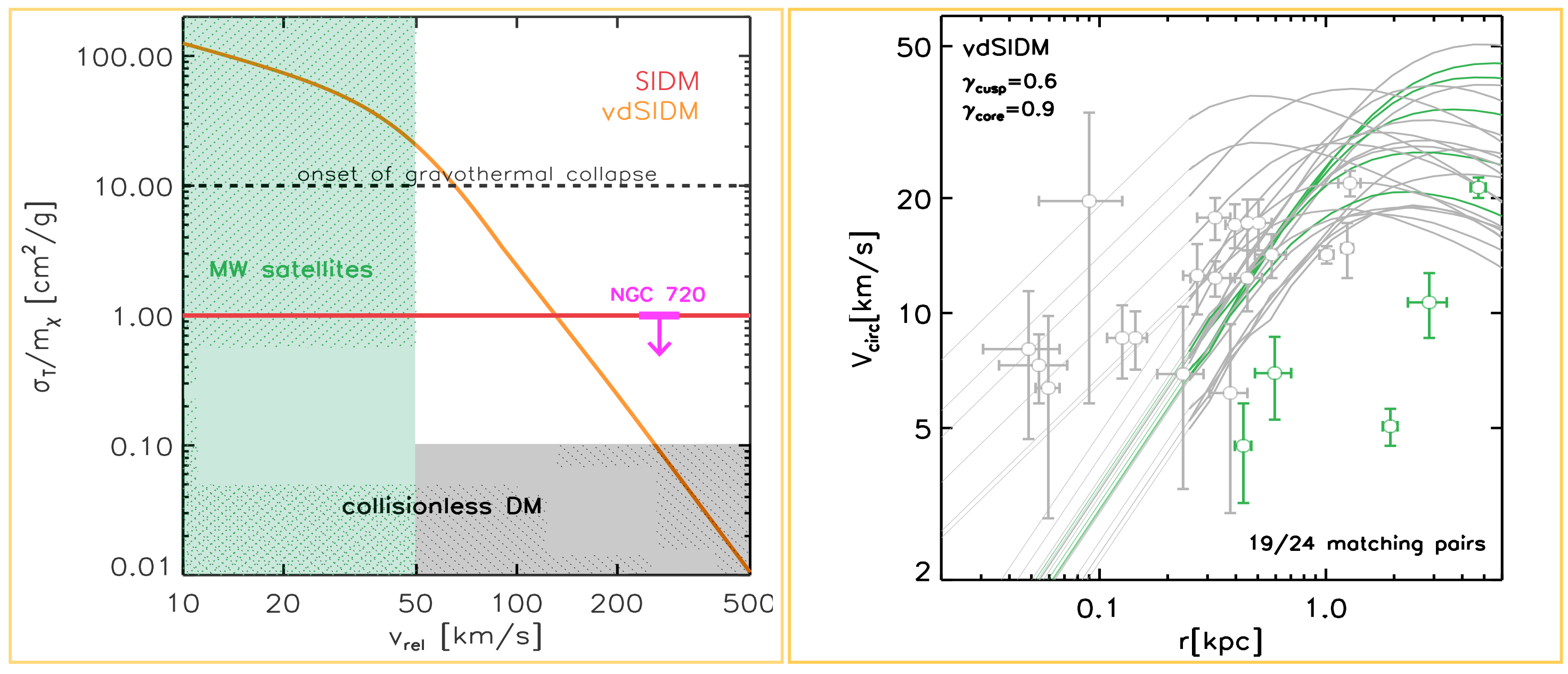

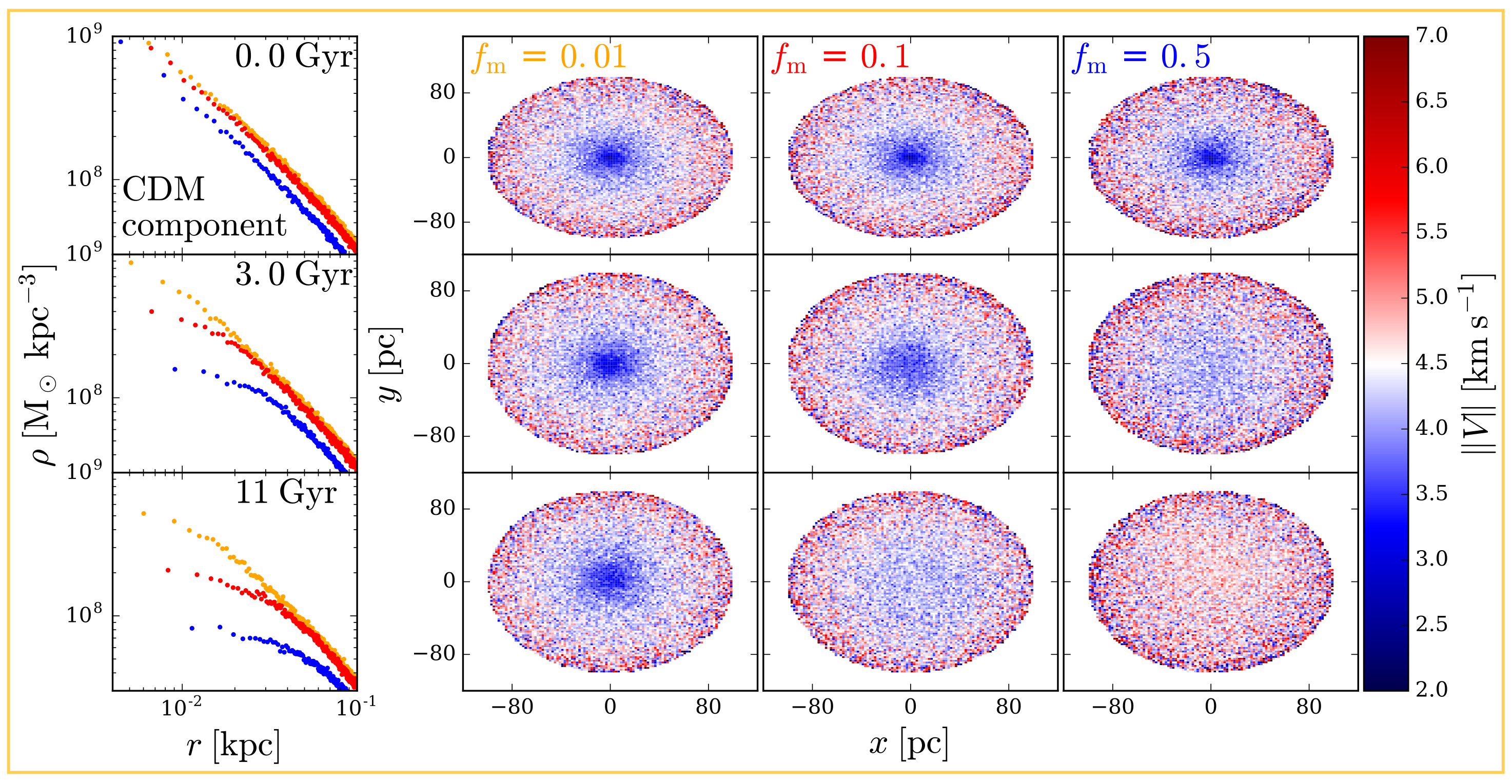
| Dwarf | M [ M] | M [ M] | M/M [] | Member Stars |
|---|---|---|---|---|
| Fornax | 14 ± 4 | 2.5 | 56 | 2573 |
| Leo I | 3.4 ± 1.1 | 25 | 1.3 | 328 |
| Sculptor | 1.4 ± 0.6 | 25 | 0.5 | 1351 |
| Leo II | 0.59 ± 0.18 | 25 | 0.23 | 186 |
| Sextans | 0.41 ± 0.19 | 0.4 | 10.25 | 417 |
| Carina | 0.24 ± 0.1 | 2.0 | 1.2 | 767 |
| Ursa Minor | 0.20 ± 0.09 | 25 | 0.07 | 430 |
| Draco | 0.27 ± 0.04 | 25 | 0.1 | 504 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boldrini, P. The Cusp–Core Problem in Gas-Poor Dwarf Spheroidal Galaxies. Galaxies 2022, 10, 5. https://doi.org/10.3390/galaxies10010005
Boldrini P. The Cusp–Core Problem in Gas-Poor Dwarf Spheroidal Galaxies. Galaxies. 2022; 10(1):5. https://doi.org/10.3390/galaxies10010005
Chicago/Turabian StyleBoldrini, Pierre. 2022. "The Cusp–Core Problem in Gas-Poor Dwarf Spheroidal Galaxies" Galaxies 10, no. 1: 5. https://doi.org/10.3390/galaxies10010005
APA StyleBoldrini, P. (2022). The Cusp–Core Problem in Gas-Poor Dwarf Spheroidal Galaxies. Galaxies, 10(1), 5. https://doi.org/10.3390/galaxies10010005





