Low-Field Magnetic Resonance Imaging: A Full-Wave Simulation of Radiofrequency Birdcage Coils for Musculoskeletal Limb Imaging
Abstract
:1. Introduction
2. Materials and Methods
2.1. FDTD Simulation
2.2. Coil Resistance and Sample-Induced Resistance Evaluation
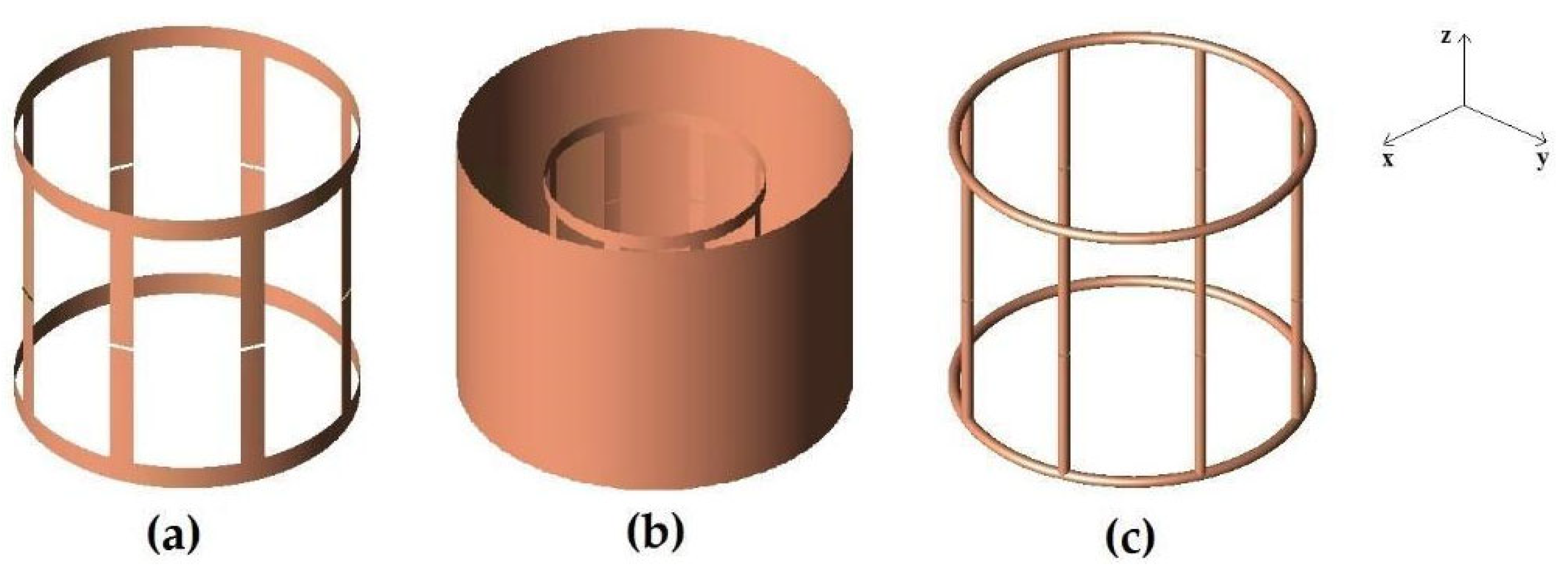
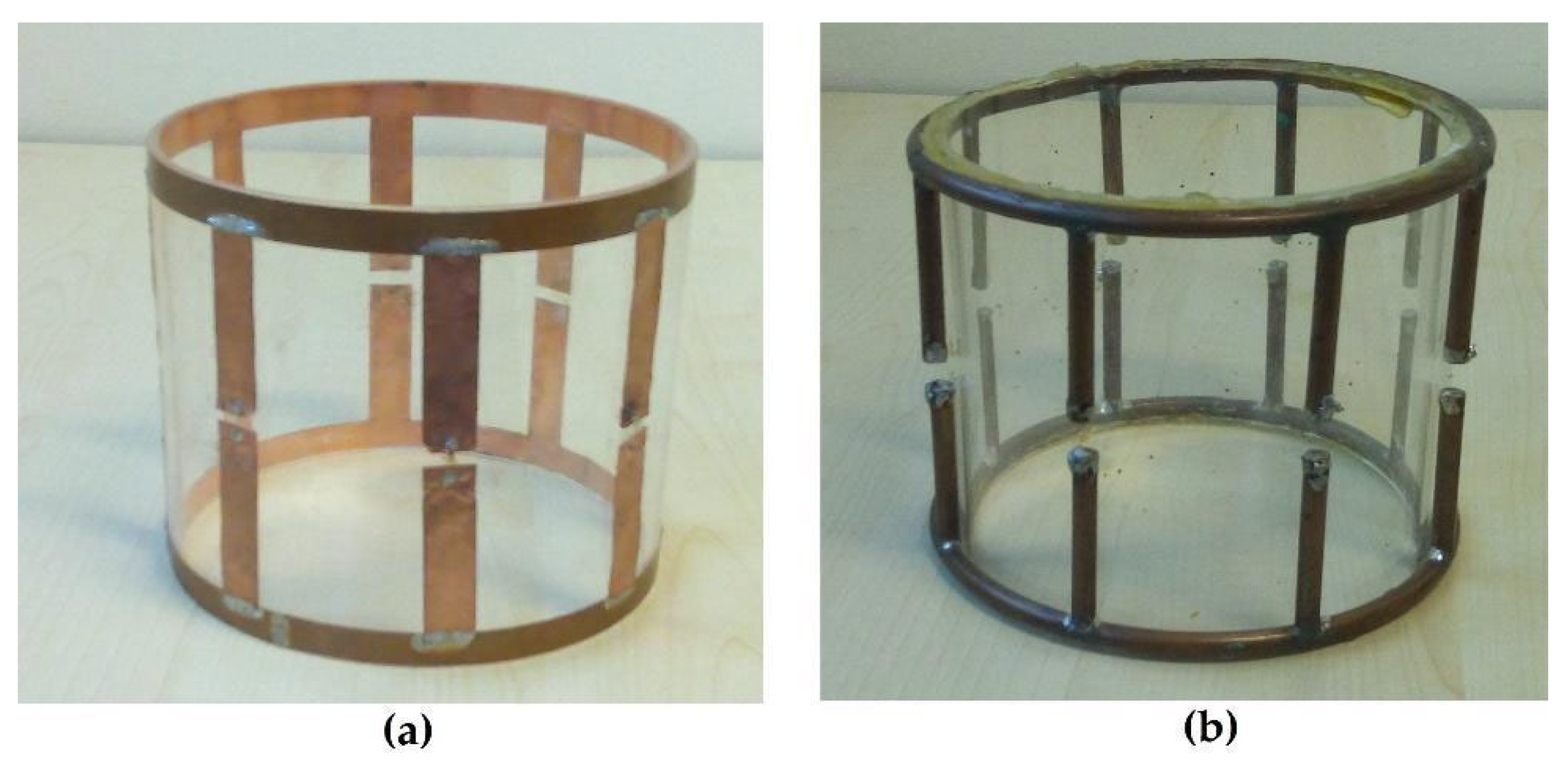
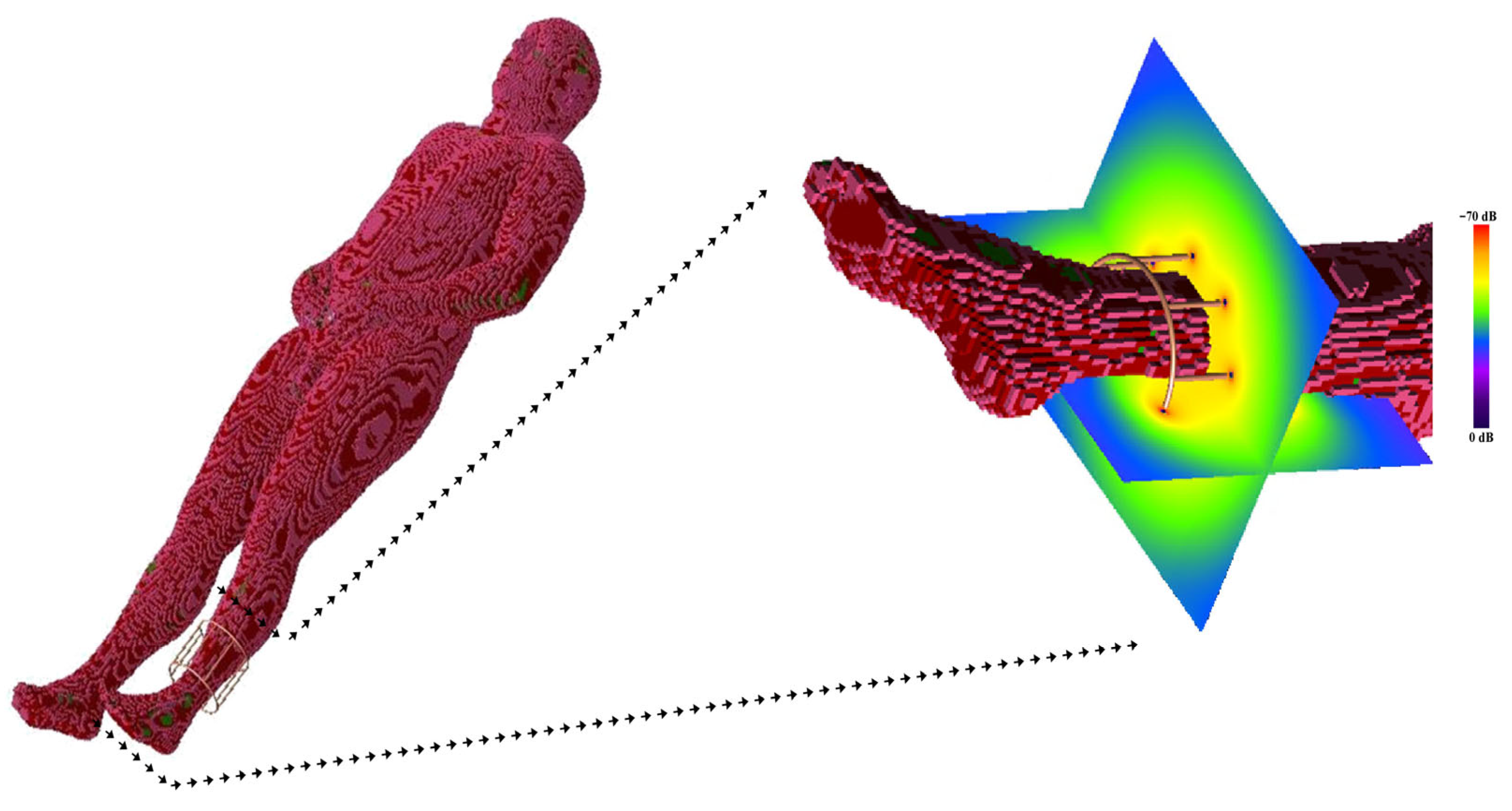
2.3. Strip Birdcage Coil
2.4. Wire Birdcage Coil
2.4.1. Linear Mode
2.4.2. Quadrature Mode
3. Results
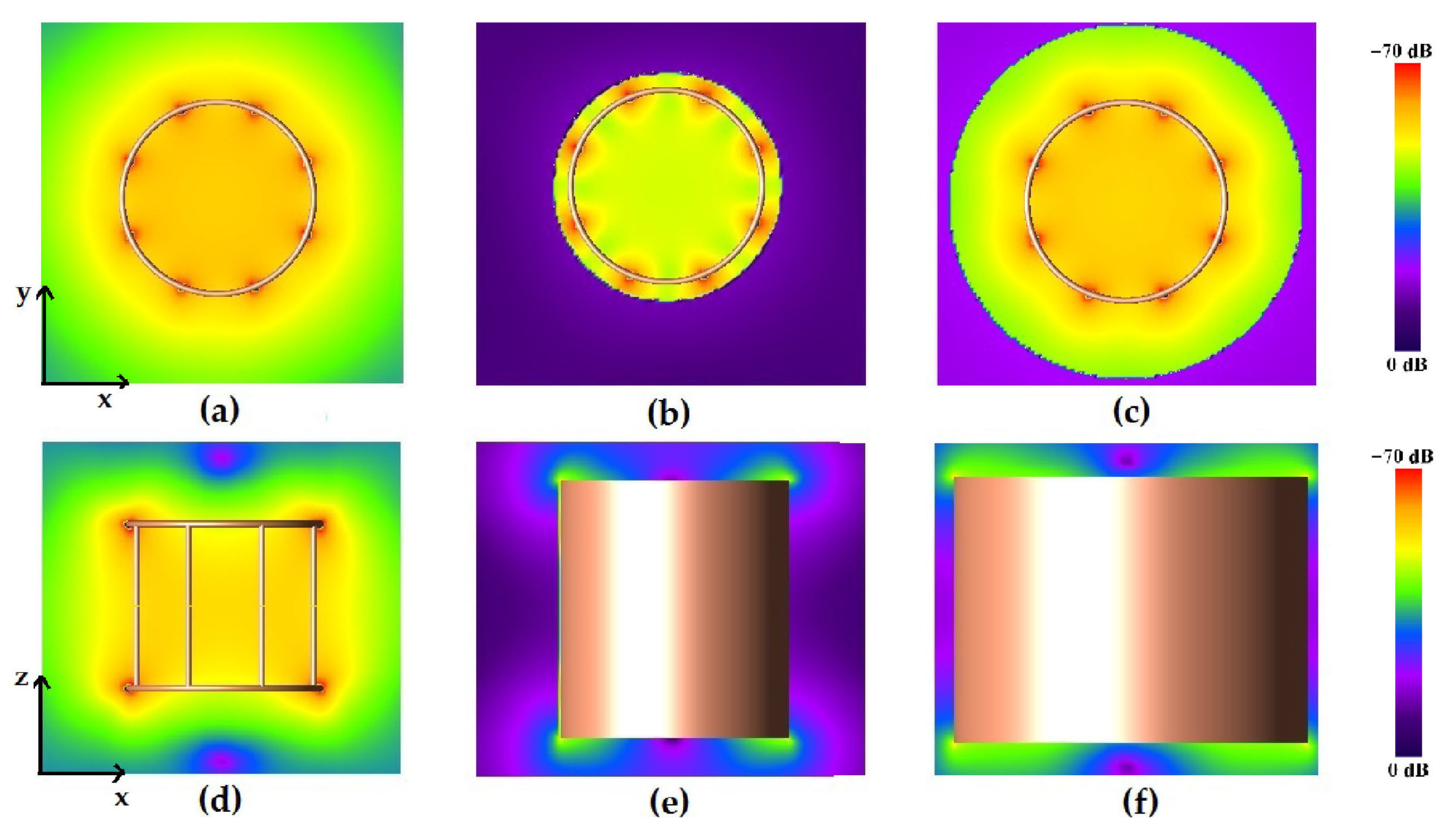
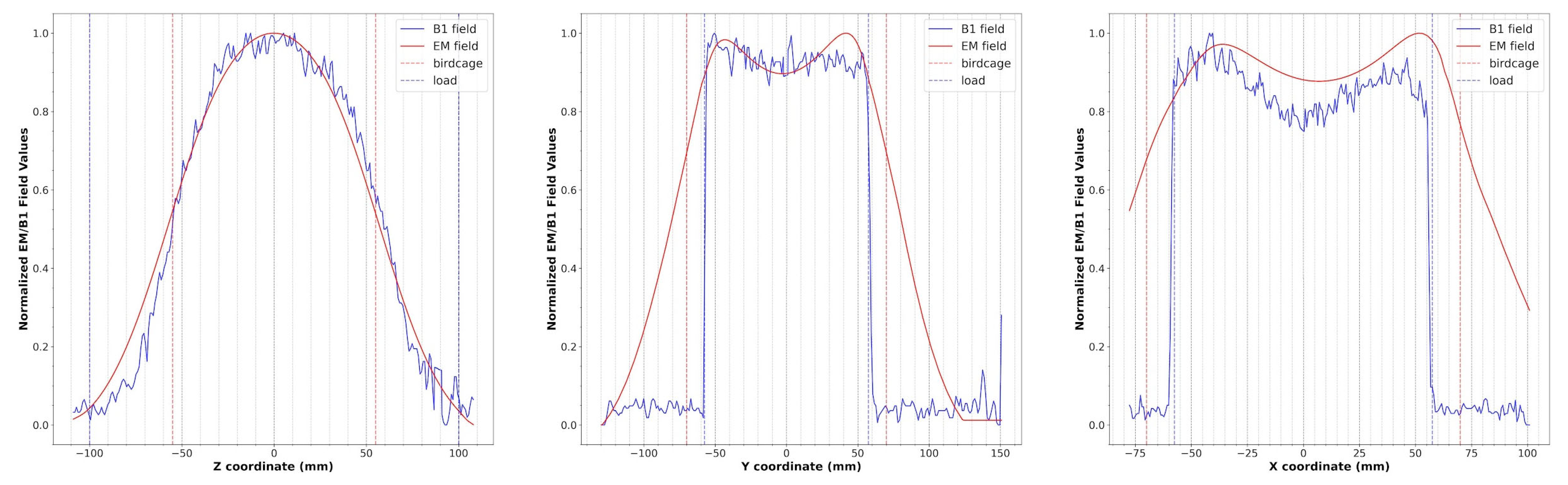
3.1. Strip Birdcage Coil
3.2. Wire Birdcage Coil
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hori, M.; Hagiwara, A.; Goto, M.; Wada, A.; Aoki, S. Low-Field Magnetic Resonance Imaging: Its History and Renaissance. Investig. Radiol. 2021, 56, 669–679. [Google Scholar] [CrossRef] [PubMed]
- Hennig, J. An Evolution of Low-Field Strength MRI. Magn. Reson. Mater. Phys. Biol. Med. 2023, 36, 335–346. [Google Scholar] [CrossRef] [PubMed]
- Arnold, T.C.; Freeman, C.W.; Litt, B.; Stein, J.M. Low-Field MRI: Clinical Promise and Challenges. J. Magn. Reson. Imaging 2022, 57, 25–44. [Google Scholar] [CrossRef] [PubMed]
- Roemer, F.W.; Guermazi, A. What Is the Role of 3 T MRI in Sports Medicine? Revisiting the Marriage after the Honeymoon. Br. J. Sports Med. 2016, 50, 894–895. [Google Scholar] [CrossRef]
- Van Dyck, P.; Kenis, C.; Vanhoenacker, F.M.; Lambrecht, V.; Wouters, K.; Gielen, J.L.; Dossche, L.; Parizel, P.M. Comparison of 1.5- and 3-T MR Imaging for Evaluating the Articular Cartilage of the Knee. Knee Surg. Sports Traumatol. Arthrosc. 2014, 22, 1376–1384. [Google Scholar] [CrossRef]
- Pogarell, T.; Heiss, R.; Janka, R.; Nagel, A.M.; Uder, M.; Roemer, F.W. Modern Low-Field MRI. Skeletal Radiol. 2024, 53, 1751–1760. [Google Scholar] [CrossRef]
- Heiss, R.; Nagel, A.M.; Laun, F.B.; Uder, M.; Bickelhaupt, S. Low-Field Magnetic Resonance Imaging: A New Generation of Breakthrough Technology in Clinical Imaging. Investig. Radiol. 2021, 56, 726–733. [Google Scholar] [CrossRef]
- Khodarahmi, I.; Keerthivasan, M.B.; Brinkmann, I.M.; Grodzki, D.; Fritz, J. Modern Low-Field MRI of the Musculoskeletal System: Practice Considerations, Opportunities, and Challenges. Investig. Radiol. 2023, 58, 76–87. [Google Scholar] [CrossRef]
- Marques, J.P.; Simonis, F.F.J.; Webb, A.G. Low-Field MRI: An MR Physics Perspective. J. Magn. Reson. Imaging 2019, 49, 1528–1542. [Google Scholar] [CrossRef]
- Samardzija, A.; Selvaganesan, K.; Zhang, H.Z.; Sun, H.; Sun, C.; Ha, Y.; Galiana, G.; Constable, R.T. Low-Field, Low-Cost, Point-of-Care Magnetic Resonance Imaging. Annu. Rev. Biomed. Eng. 2024, 26, 67–91. [Google Scholar] [CrossRef]
- Zhao, Y.; Bhosale, A.A.; Zhang, X. Multimodal Surface Coils for Low-Field MR Imaging. Magn. Reson. Imaging 2024, 112, 107–115. [Google Scholar] [CrossRef] [PubMed]
- Karasawa, T.; Saikawa, J.; Munaka, T.; Kobayashi, T. Homogeneous B0 Coil Design Method for Open-Access Ultra-Low Field Magnetic Resonance Imaging: A Simulation Study. Magn. Reson. Imaging 2024, 112, 128–135. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, G.; Frijia, F.; Flori, A. Radiofrequency Coils for Low-Field (0.18–0.55 T) Magnetic Resonance Scanners: Experience from a Research Lab–Manufacturing Companies Cooperation. Electronics 2022, 11, 4233. [Google Scholar] [CrossRef]
- Hayes, C.E.; Edelstein, W.A.; Schenck, J.F.; Mueller, O.M.; Eash, M. An Efficient, Highly Homogeneous Radiofrequency Coil for Whole-Body NMR Imaging at 1.5 T. J. Magn. Reson. (1969) 1985, 63, 622–628. [Google Scholar] [CrossRef]
- Leifer, M.C. Resonant Modes of the Birdcage Coil. J. Magn. Reson. 1997, 124, 51–60. [Google Scholar] [CrossRef]
- Giovannetti, G.; Landini, L.; Santarelli, M.F.; Positano, V. A Fast and Accurate Simulator for the Design of Birdcage Coils in MRI. Magma 2002, 15, 36–44. [Google Scholar] [CrossRef]
- Giovannetti, G.; Francesconi, R.; Landini, L.; Santarelli, M.F.; Positano, V.; Viti, V.; Benassi, A. Conductor Geometry and Capacitor Quality for Performance Optimization of Low-Frequency Birdcage Coils. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2004, 20B, 9–16. [Google Scholar] [CrossRef]
- Giovannetti, G.; Francesconi, R.; Landini, L.; Viti, V.; Santarelli, M.F.; Positano, V.; Benassi, A. A quadrature lowpass birdcage coil for a vertical low field MRI scanner. Concepts Magn. Reson. Part B Magn. Reson. 2004, 22, 1–6. [Google Scholar] [CrossRef]
- Yee, K.S. Numerical Solution of Initial Boundary Value Problems Involving Maxwell’s Equations in Isotropic Media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar]
- Duan, Y.; Ibrahim, T.; Peterson, B.; Liu, F.; Kangarlu, A. Assessment of a PML Boundary Condition for Simulating an MRI Radio Frequency Coil. Int. J. Antennas Propag. 2008, 2008, 563196. [Google Scholar] [CrossRef]
- Liu, W.; Collins, C.M.; Smith, M.B. Calculations of B1 Distribution, Specific Energy Absorption Rate, and Intrinsic Signal-to-Noise Ratio for a Body-Size Birdcage Coil Loaded with Different Human Subjects at 64 and 128 MHz. Appl. Magn. Reson. 2005, 29, 5–18. [Google Scholar] [CrossRef] [PubMed]
- Collins, C.M.; Smith, M.B. Spatial Resolution of Numerical Models of Man and Calculated Specific Absorption Rate Using the FDTD Method: A Study at 64 MHz in a Magnetic Resonance Imaging Coil. J. Magn. Reson. Imaging 2003, 18, 383–388. [Google Scholar] [CrossRef] [PubMed]
- Ibrahim, T.S.; Lee, R.; Baertlein, B.A.; Yu, Y.; Robitaille, P.-M.L. Computational Analysis of the High Pass Birdcage Resonator: Finite Difference Time Domain Simulations for High-Field MRI. Magn. Reson. Imaging 2000, 18, 835–843. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.N.; Hoult, D.I. Biomedical Magnetic Resonance Technology; Adam Hilger: Bristol, UK, 1989. [Google Scholar]
- Hurlston, S.E.; Cofer, G.P.; Johnson, G.A. Optimized Radiofrequency Coils for Increased Signal-to-Noise Ratio in Magnetic Resonance Microscopy. Int. J. Imaging Syst. Technol. 1997, 8, 277–284. [Google Scholar] [CrossRef]
- Giovannetti, G.; Viti, V.; Liu, Y.; Yu, W.; Mittra, R.; Landini, L.; Benassi, A. An Accurate Simulator for Magnetic Resonance Coil Sensitivity Estimation. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2008, 33B, 209–215. [Google Scholar] [CrossRef]
- Vullo, T.; Zipagan, R.T.; Pascone, R.; Whalen, J.P.; Cahill, P.T. Experimental Design and Fabrication of Birdcage Resonators for Magnetic Resonance Imaging. Magn. Reson. Med. 1992, 24, 243–252. [Google Scholar] [CrossRef]
- Pascone, R.J.; Garcia, B.J.; Fitzgerald, T.M.; Vullo, T.; Zipagan, R.; Cahill, P.T. Generalized Electrical Analysis of Low-Pass and High-Pass Birdcage Resonators. Magn. Reson. Imaging 1991, 9, 395–408. [Google Scholar] [CrossRef]
- Roemer, P.B.; Edelstein, W.A.; Hayes, C.E.; Souza, S.P.; Mueller, O.M. The NMR Phased Array. Magn. Reson. Med. 1990, 16, 192–225. [Google Scholar] [CrossRef]
- ASTM F 2182-09; Standard test method for measurement of radio frequency induced heating near passive implants during magnetic resonance imaging. ASTM Committee F04 on Medical and Surgical Materials and Devices, Subcommittee F04.15 on Material Test Methods; ASTM International: West Conshohocken, PA, USA, 2009.
- Giovannetti, G.; Viti, V.; Positano, V.; Santarelli, M.F.; Landini, L.; Benassi, A. Coil Sensitivity Map-Based Filter for Phased-Array Image Reconstruction in Magnetic Resonance Imaging. Int. J. Biomed. Eng. Technol. 2007, 1, 4–17. [Google Scholar] [CrossRef]
- Penny, C. Computation of fields and SAR for MRI with finite-difference, time-domain software. Microw. J. 2007, 50, 118–128. [Google Scholar]
- Chen, C.N.; Hoult, D.I.; Sank, V.J. Quadrature Detection Coils—A Further √2 Improvement in Sensitivity. J. Magn. Reson. (1969) 1983, 54, 324–327. [Google Scholar] [CrossRef]
- Bandurski, W. Simulation of Single and Coupled Transmission Lines Using Time-Domain Scattering Parameters. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2000, 47, 1224–1234. [Google Scholar] [CrossRef]
- Rogovich, A.; Monorchio, A.; Nepa, P.; Manara, G.; Giovannetti, G.; Landini, L. Design of Magnetic Resonance Imaging (MRI) RF Coils by Using the Method of Moments. IEEE Antennas Propag. Soc. Symp. 2004, 1, 950–953. [Google Scholar]
- Puig, S.; Kuruvilla, Y.C.; Ebner, L.; Endel, G. Magnetic resonance tomography of the knee joint. Skeletal Radiol. 2015, 44, 1427–1434. [Google Scholar] [CrossRef]
- Vellet, A.D.; Lee, D.H.; Munk, P.L.; Hewett, L.; Eliasziw, M.; Dunlavy, S.; Vidito, L.; Fowler, P.J.; Miniaci, A.; Amendola, A. Anterior cruciate ligament tear: Prospective evaluation of diagnostic accuracy of middle and high-field-strength MR imaging at 1.5 and 0.5 T. Radiology 1995, 197, 826–830. [Google Scholar] [CrossRef]
- Cotten, A.; Delfaut, E.; Demondion, X.; Lapègue, F.; Boukhelifa, M.; Boutry, N.; Chastanet, P.; Gougeon, F. MR imaging of the knee at 0.2 and 1.5 T: Correlation with surgery. AJR Am. J. Roentgenol. 2000, 174, 1093–1097. [Google Scholar] [CrossRef]
- Okamoto, Y.; Maehara, K.; Kanahori, T.; Hiyama, T.; Kawamura, T.; Minami, M. Incidence of elbow injuries in adolescent baseball players: Screening by a low field magnetic resonance imaging system specialized for small joints. Jpn. J. Radiol. 2016, 34, 300–306. [Google Scholar] [CrossRef]
- Dean, D.G. The role of MRI in musculoskeletal practice: A clinical perspective. J. Man. Manip. Ther. 2011, 19, 152–161. [Google Scholar] [CrossRef]
- Brydie, A.; Raby, N. Early MRI in the management of clinical scaphoid fracture. Br. J. Radiol. 2003, 76, 296–300. [Google Scholar] [CrossRef]
- Crues, J.V.; Shellock, F.G.; Dardashti, S.; James, T.W.; Troum, O.M. Identification of wrist and metacarpophalangeal joint erosions using a portable magnetic resonance imaging system compared to conventional radiographs. J. Rheumatol. 2004, 31, 676–685. [Google Scholar]
- Tavernier, T.; Cotten, A. High- versus low-field MR imaging. Radiol. Clin. N. Am. 2005, 43, 673–681. [Google Scholar] [CrossRef] [PubMed]
- Harris, C.A.; White, L.M. Metal artifact reduction in musculoskeletal magnetic resonance imaging. Orthop. Clin. N. Am. 2006, 37, 349–359. [Google Scholar] [CrossRef] [PubMed]
- Klein, H.M. Low-field magnetic resonance imaging. Rofo 2020, 192, 537–548. [Google Scholar] [CrossRef] [PubMed]
- Collins, C.M.; Li, S.; Yang, Q.X.; Smith, M.B. A Method for Accurate Calculation of B1 Fields in Three Dimensions. Effects of Shield Geometry on Field Strength and Homogeneity in the Birdcage Coil. J. Magn. Reson. 1997, 125, 233–241. [Google Scholar] [CrossRef]
- Vaughan, J.T.; Griffiths, J.R. RF Coils for MRI; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Giovannetti, G. Birdcage Coils: Equivalent Capacitance and Equivalent Inductance. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2014, 44, 32–38. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giovannetti, G.; Frijia, F.; Santarelli, M.F.; Positano, V. Low-Field Magnetic Resonance Imaging: A Full-Wave Simulation of Radiofrequency Birdcage Coils for Musculoskeletal Limb Imaging. Diagnostics 2025, 15, 713. https://doi.org/10.3390/diagnostics15060713
Giovannetti G, Frijia F, Santarelli MF, Positano V. Low-Field Magnetic Resonance Imaging: A Full-Wave Simulation of Radiofrequency Birdcage Coils for Musculoskeletal Limb Imaging. Diagnostics. 2025; 15(6):713. https://doi.org/10.3390/diagnostics15060713
Chicago/Turabian StyleGiovannetti, Giulio, Francesca Frijia, Maria Filomena Santarelli, and Vincenzo Positano. 2025. "Low-Field Magnetic Resonance Imaging: A Full-Wave Simulation of Radiofrequency Birdcage Coils for Musculoskeletal Limb Imaging" Diagnostics 15, no. 6: 713. https://doi.org/10.3390/diagnostics15060713
APA StyleGiovannetti, G., Frijia, F., Santarelli, M. F., & Positano, V. (2025). Low-Field Magnetic Resonance Imaging: A Full-Wave Simulation of Radiofrequency Birdcage Coils for Musculoskeletal Limb Imaging. Diagnostics, 15(6), 713. https://doi.org/10.3390/diagnostics15060713








