Emergence of Mechano-Sensitive Contraction Autoregulation in Cardiomyocytes
Abstract
1. Introduction
2. Model Description and Results
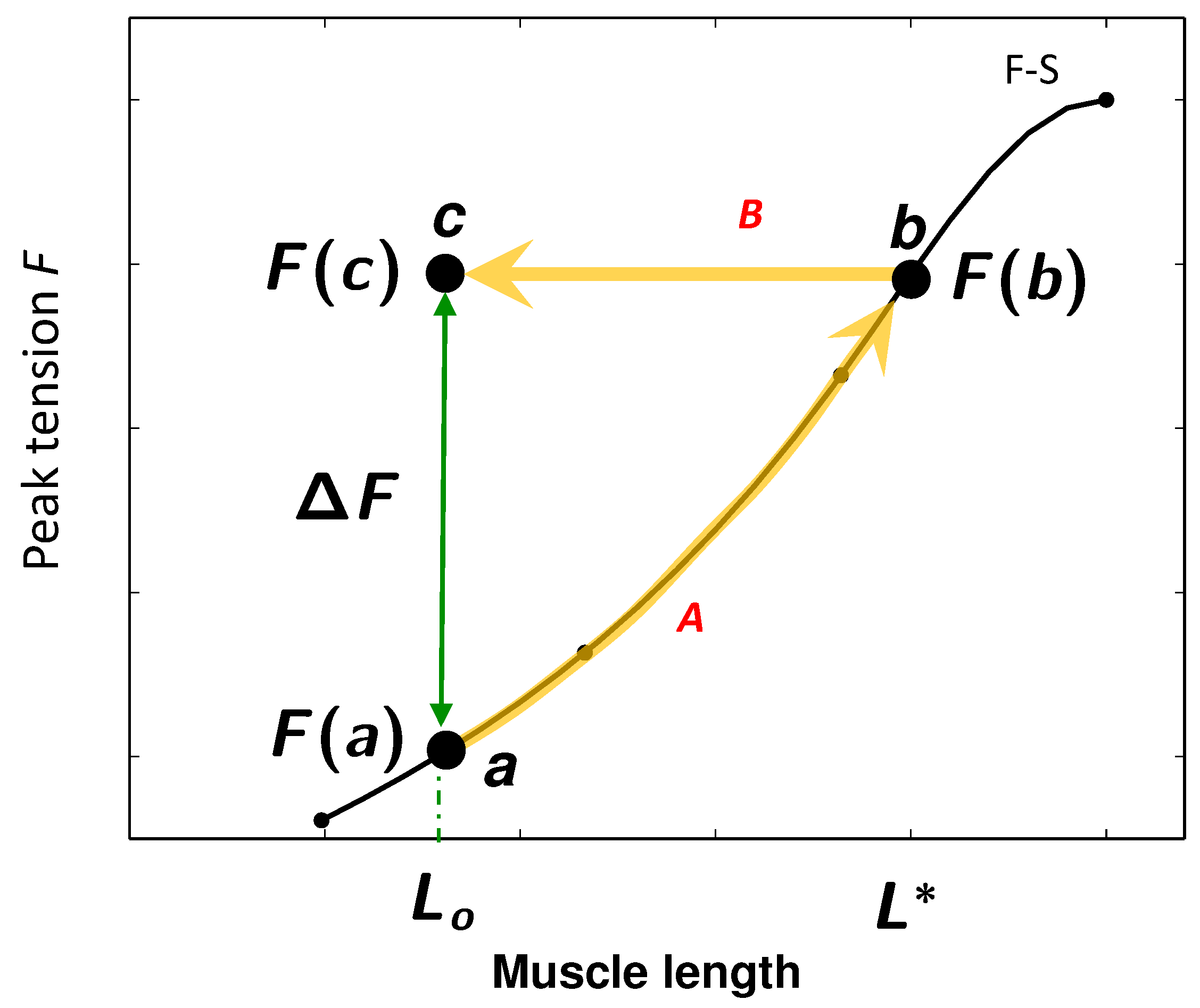
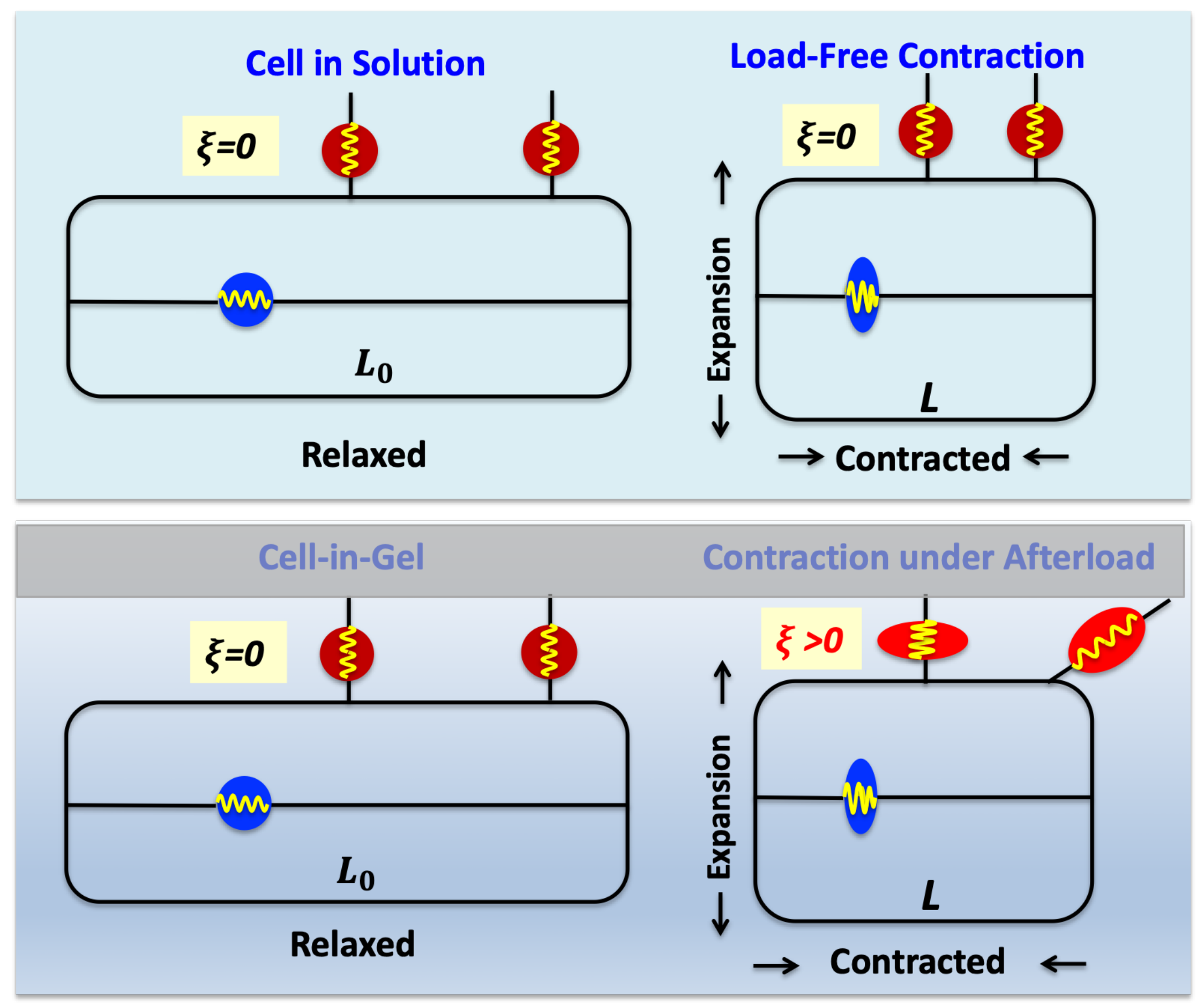
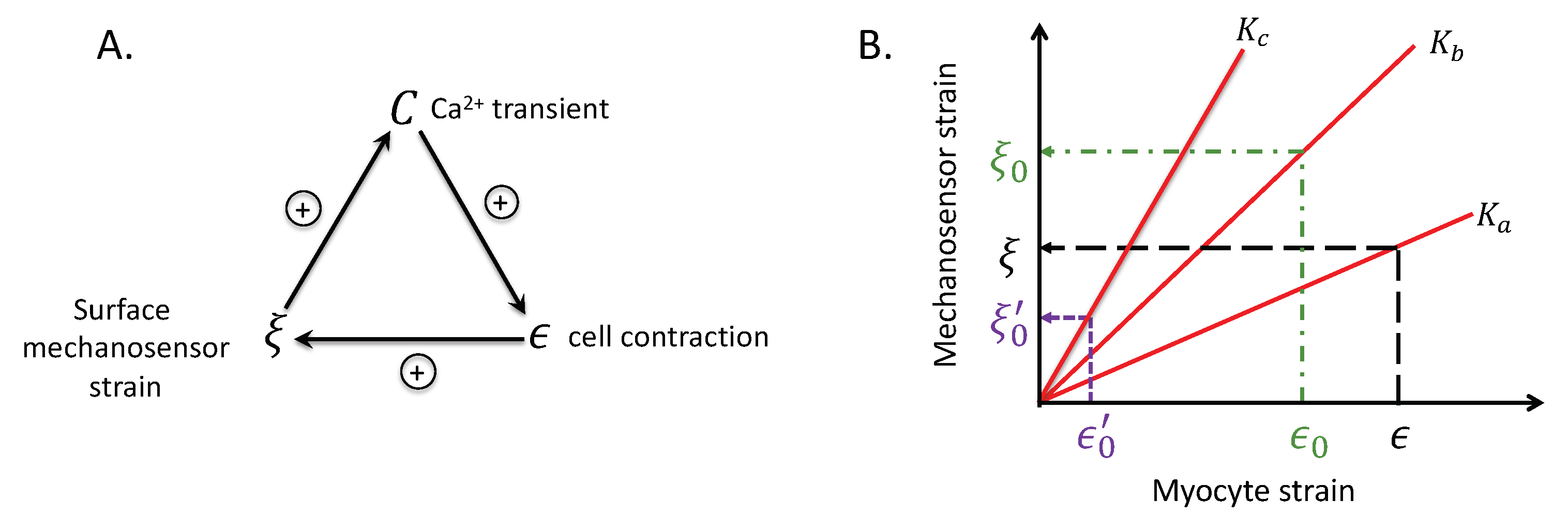
2.1. Conceptual and Mathematical Models
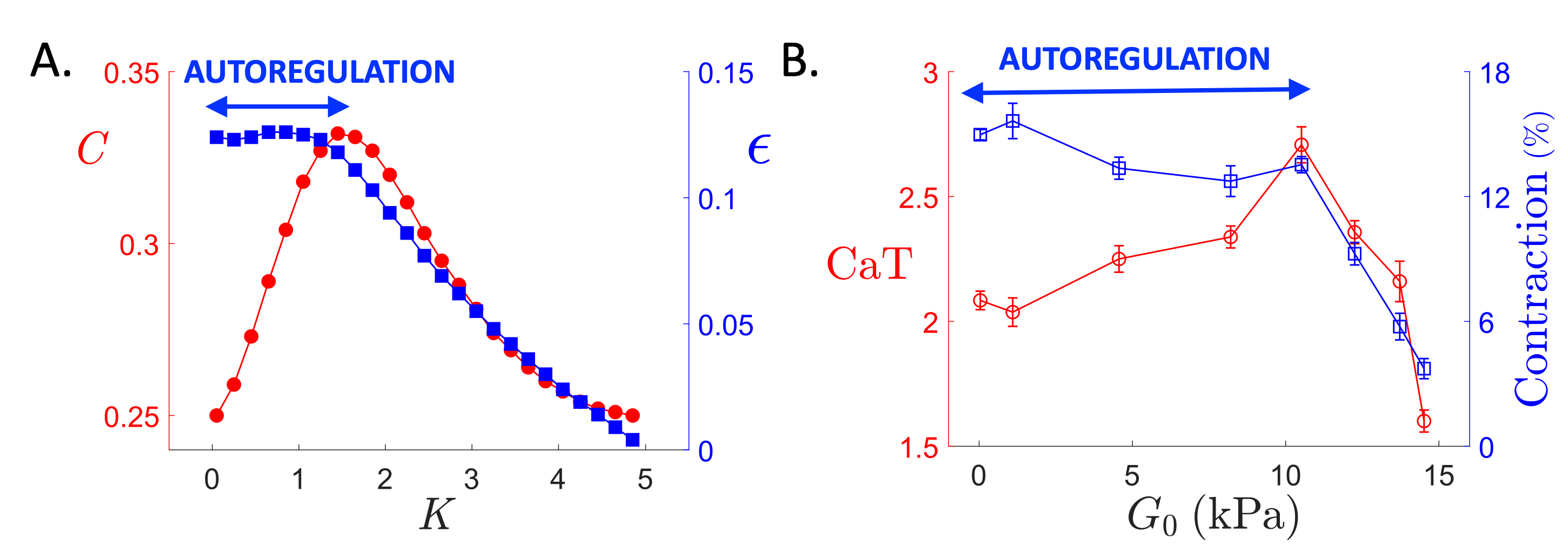
2.2. Further Insights from a Specific Example
2.3. Experimental Test of Biphasic Prediction
3. Discussion
3.1. Experimental Underpinnings for the MCT Model
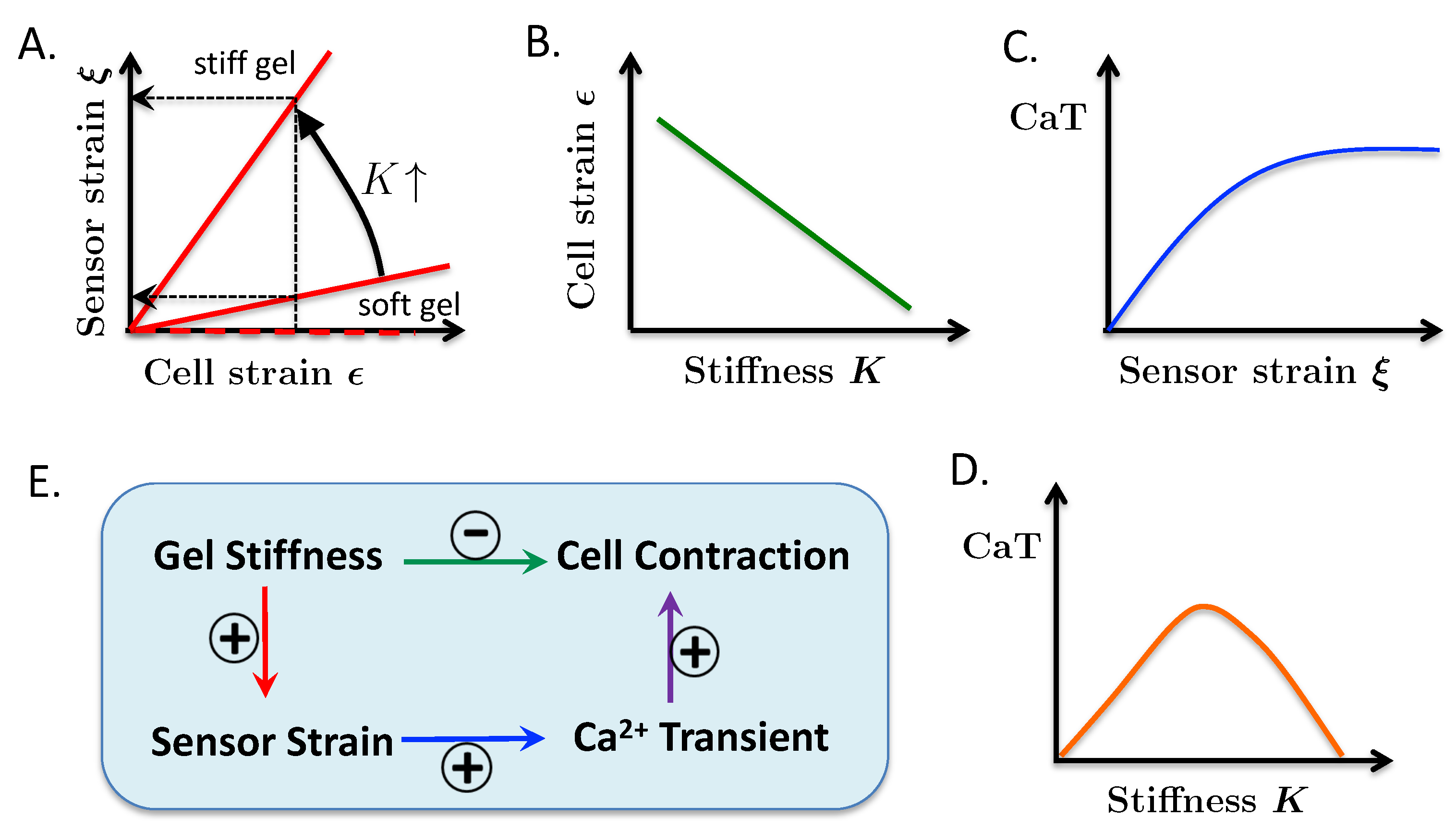
3.2. Biphasic CaT Response and Autoregulation in the MCT Model
3.3. Stability, Biphasic CaT Response, and Autoregulation Are Natural Emergent Properties of MCT
3.4. What the Theorems Tell Us about MCT
3.5. Intrinsic Inotropy
3.6. Intrinsic Inotropy and the Anrep Effect
3.7. Strength—Limitation Duality of the Model
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| CaT | transient |
| EDV | end-diastolic volume |
| F-S | Frank-Starling |
| MCT | mechano-chemo-transduction |
| L-NAME | N(ω)-nitro-l-arginine methyl ester |
| L-NPA | N(ω)-propyl-L-arginine hydrochloride |
| LV | left ventricle or left ventricular |
| NO | nitric oxide |
| NOS | nitric oxide synthase |
Appendix A
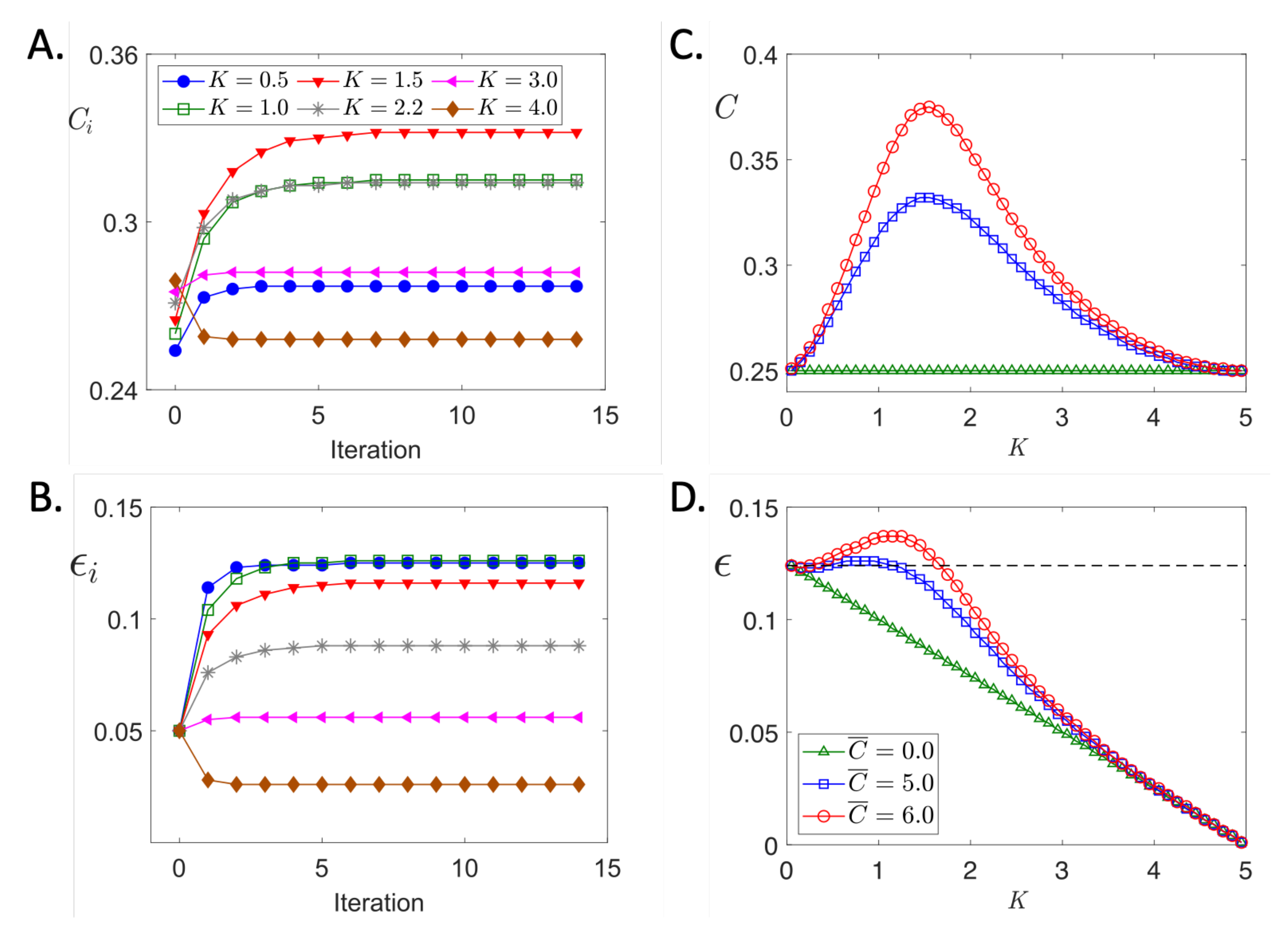
Appendix A.1. Monotonic Convergence of Equations (1)–(3)
Appendix A.2. Autoregulation Occurs Given Sufficient MCT Amplification
Appendix A.3. Biphasic C(K), ϵ(K), and ξ(K) Curves
References
- Patterson, S.W.; Piper, H.; Starling, E.H. The regulation of the heart beat. J. Physiol. Lond. 1914, 48, 465–513. [Google Scholar] [CrossRef]
- Allen, D.; Kurihara, S. The effects of muscle length on intracellular calcium transients in mammalian cardiac muscle. J. Physiol. 1982, 327, 79–94. [Google Scholar] [CrossRef]
- Ter Keurs, H. Heart failure and Starling’s law of the heart. Can. J. Cardiol. 1996, 12, 1047–1057. [Google Scholar]
- Von Anrep, G. On the part played by the suprarenals in the normal vascular reactions of the body. J. Physiol. 1912, 45, 307–317. [Google Scholar] [CrossRef] [PubMed]
- Cingolani, O.H.; Kirk, J.A.; Seo, K.; Koitabashi, N.; Lee, D.I.; Ramirez-Correa, G.; Bedja, D.; Barth, A.S.; Moens, A.L.; Kass, D.A. Thrombospondin-4 Is Required for Stretch-Mediated Contractility Augmentation in Cardiac Muscle. Novelty and Significance. Circ. Res. 2011, 109, 1410–1414. [Google Scholar] [CrossRef]
- Rosenblueth, A.; Alanís, J.; López, E.; Rubio, R. The Adaptation of Ventricular Muscle to Different Circulatory Conditions. Arch. Int. Physiol. Biochim. 1959, 67, 358–373. [Google Scholar] [CrossRef]
- Clancy, R.L.; Graham, T.P.; Ross, J.; Sonnenblick, E.H.; Braunwald, E. Influence of aortic pressure-induced homeometric autoregulation on myocardial performance. Am. J. Physiol. 1968, 214, 1186–1192. [Google Scholar] [CrossRef] [PubMed]
- Klautz, R.J.; Teitel, D.F.; Steendijk, P.; van Bel, F.; Baan, J. Interaction between afterload and contractility in the newborn heart: Evidence of homeometric autoregulation in the intact circulation. J. Am. Coll. Cardiol. 1995, 25, 1428–1435. [Google Scholar] [CrossRef]
- Sarnoff, S.J.; Mitchell, J.H.; Gilmore, J.P.; Remensnyder, J.P. Homeometric autoregulation in the heart. Circ. Res. 1960, 8, 1077–1091. [Google Scholar] [CrossRef]
- Poole-Wilson, P.A. Acidosis and contractility of heart muscle. Ciba Found. Symp. 1982, 87, 58–76. [Google Scholar] [PubMed]
- Murtagh, J.G.; Binnion, P.F.; Lal, S.; Hutchison, K.J.; Fletcher, E. Haemodynamic effects of glucagon. Br. Heart J. 1970, 32, 307–315. [Google Scholar] [CrossRef][Green Version]
- Lucchesi, B.R. Cardiac actions of glucagon. Circ. Res. 1968, 22, 777–787. [Google Scholar] [CrossRef] [PubMed]
- Ross, J.; Braunwald, E. The study of left ventricular function in man by increasing resistance to ventricular ejection with angiotensin. Circulation 1964, 29, 739–749. [Google Scholar] [CrossRef]
- Sonnenblick, E.H.; Downing, S.E. Afterload as a primary determinant of ventricular performance. Am. J. Physiol. 1963, 204, 604–610. [Google Scholar] [CrossRef] [PubMed]
- Donald, T.C.; Peterson, D.M.; Walker, A.A.; Hefner, L.L. Afterload-induced homeometric autoregulation in isolated cardiac muscle. Am. J. Physiol. 1976, 231, 545–550. [Google Scholar] [CrossRef] [PubMed]
- Jian, Z.; Han, H.; Zhang, T.; Puglisi, J.; Izu, L.T.; Shaw, J.A.; Onofiok, E.; Erickson, J.R.; Chen, Y.J.; Horvath, B.; et al. Mechanochemotransduction during cardiomyocyte contraction is mediated by localized nitric oxide signaling. Sci. Signal. 2014, 7, ra27. [Google Scholar] [CrossRef] [PubMed]
- Shimkunas, R.; Hegyi, B.; Jian, Z.; Shaw, J.A.; Kazemi-Lari, M.A.; Mitra, D.; Leach, J.K.; Li, X.; Jaradeh, M.; Balardi, N.; et al. Mechanical Load Regulates Excitation-Ca2+ Signaling-Contraction in Cardiomyocyte. Circ. Res. 2021, 128, 772–774. [Google Scholar] [CrossRef] [PubMed]
- Shaw, J.; Izu, L.; Chen-Izu, Y. Mechanical Analysis of Single Myocyte Contraction in a 3-D Elastic Matrix. PLoS ONE 2013, 8, e75492. [Google Scholar] [CrossRef]
- Chen-Izu, Y.; Izu, L.T. Mechano-chemo-transduction in cardiac myocytes. J. Physiol. Lond. 2017, 595, 3949–3958. [Google Scholar] [CrossRef]
- Niederer, S.A.; Smith, N.P. A mathematical model of the slow force response to stretch in rat ventricular myocytes. Biophys. J. 2007, 92, 4030–4044. [Google Scholar] [CrossRef]
- Bluhm, W.F.; Lew, W.Y.; Garfinkel, A.; McCulloch, A.D. Mechanisms of length history-dependent tension in an ionic model of the cardiac myocyte. Am. J. Physiol. 1998, 274, H1032–H1040. [Google Scholar] [CrossRef] [PubMed]
- Gwathmey, J.K.; Hajjar, R.J. Intracellular calcium related to force development in twitch contraction of mammalian myocardium. Cell Calcium 1990, 11, 531–538. [Google Scholar] [CrossRef]
- Backx, P.H.; Gao, W.D.; Azan-Backx, M.D.; Marban, E. The relationship between contractile force and intracellular [Ca2+] in intact rat cardiac trabeculae. J. Gen. Physiol. 1995, 105, 1–19. [Google Scholar] [CrossRef]
- Kazemi-Lari, M.A.; Shaw, J.A.; Wineman, A.S.; Shimkunas, R.; Jian, Z.; Hegyi, B.; Izu, L.; Chen-Izu, Y. A Viscoelastic Eshelby Inclusion Model and Analysis of the Cell-in-Gel System. Int. J. Eng. Sci. 2021, in press. [Google Scholar]
- White, E.; Boyett, M.R.; Orchard, C.H. The effects of mechanical loading and changes of length on single guinea-pig ventricular myocytes. J. Physiol. (Lond.) 1995, 482, 93–107. [Google Scholar] [CrossRef]
- Prosser, B.L.; Ward, C.W.; Lederer, W.J. X-ROS signaling: Rapid mechano-chemo transduction in heart. Science 2011, 333, 1440–1445. [Google Scholar] [CrossRef]
- Izu, L.T.; Kohl, P.; Boyden, P.A.; Miura, M.; Banyasz, T.; Chiamvimonvat, N.; Trayanova, N.; Bers, D.M.; Chen-Izu, Y. Mechano-electric and mechano-chemo-transduction in cardiomyocytes. J. Physiol. Lond. 2020, 598, 1285–1305. [Google Scholar] [CrossRef] [PubMed]
- Glick, G.; Parmley, W.W.; Wechsler, A.S.; Sonnenblick, E.H. Glucagon. Its enhancement of cardiac performance in the cat and dog and persistence of its inotropic action despite beta-receptor blockade with propranolol. Circ. Res. 1968, 22, 789–799. [Google Scholar] [CrossRef]
- Alvarez, B.V.; Perez, N.G.; Ennis, I.L.; Camilion de Hurtado, M.C.; Cingolani, H.E. Mechanisms underlying the increase in force and Ca(2+) transient that follow stretch of cardiac muscle: A possible explanation of the Anrep effect. Circ. Res. 1999, 85, 716–722. [Google Scholar] [CrossRef]
- Cingolani, H.E.; Perez, N.G.; Cingolani, O.H.; Ennis, I.L. The Anrep effect: 100 years later. Am. J. Physiol. Heart Circ. Physiol. 2013, 304, H175–H182. [Google Scholar] [CrossRef]
- Lewis, S.F.; Taylor, W.F.; Bastian, B.C.; Graham, R.M.; Pettinger, W.A.; Blomqvist, C.G. Haemodynamic responses to static and dynamic handgrip before and after autonomic blockade. Clin. Sci. 1983, 64, 593–599. [Google Scholar] [CrossRef] [PubMed]
- Ervasti, J.M. Costameres: The Achilles’ heel of Herculean muscle. J. Biol. Chem. 2003, 278, 13591–13594. [Google Scholar] [CrossRef] [PubMed]
- Danowski, B.A.; Imanaka-Yoshida, K.; Sanger, J.M.; Sanger, J.W. Costameres are sites of force transmission to the substratum in adult rat cardiomyocytes. J. Cell Biol. 1992, 118, 1411–1420. [Google Scholar] [CrossRef]
- Kobayashi, Y.M.; Rader, E.P.; Crawford, R.W.; Iyengar, N.K.; Thedens, D.R.; Faulkner, J.A.; Parikh, S.V.; Weiss, R.M.; Chamberlain, J.S.; Moore, S.A.; et al. Sarcolemma-localized nNOS is required to maintain activity after mild exercise. Nature 2008, 456, 511–515. [Google Scholar] [CrossRef]
- Lai, Y.; Thomas, G.D.; Yue, Y.; Yang, H.T.; Li, D.; Long, C.; Judge, L.; Bostick, B.; Chamberlain, J.S.; Terjung, R.L.; et al. Dystrophins carrying spectrin-like repeats 16 and 17 anchor nNOS to the sarcolemma and enhance exercise performance in a mouse model of muscular dystrophy. J. Clin. Investig. 2009, 119, 624–635. [Google Scholar] [CrossRef] [PubMed]
- Curran, J.; Tang, L.; Roof, S.R.; Velmurugan, S.; Millard, A.; Shonts, S.; Wang, H.; Santiago, D.; Ahmad, U.; Perryman, M.; et al. Nitric oxide-dependent activation of CaMKII increases diastolic sarcoplasmic reticulum calcium release in cardiac myocytes in response to adrenergic stimulation. PLoS ONE 2014, 9, e87495. [Google Scholar] [CrossRef]
- Burgos, J.I.; Yeves, A.M.; Barrena, J.P.; Portiansky, E.L.; Vila-Petroff, M.G.; Ennis, I.L. Nitric oxide and CaMKII: Critical steps in the cardiac contractile response To IGF-1 and swim training. J. Mol. Cell. Cardiol. 2017, 112, 16–26. [Google Scholar] [CrossRef]
- Maier, L.S.; Zhang, T.; Chen, L.; DeSantiago, J.; Brown, J.H.; Bers, D.M. Transgenic CaMKIIδC overexpression uniquely alters cardiac myocyte Ca2+ handling: Reduced SR Ca2+ load and activated SR Ca2+ release. Circ. Res. 2003, 92, 904–911. [Google Scholar] [CrossRef]
- Ai, X.; Curran, J.W.; Shannon, T.R.; Bers, D.M.; Pogwizd, S.M. Ca2+/calmodulin-dependent protein kinase modulates cardiac ryanodine receptor phosphorylation and sarcoplasmic reticulum Ca2+ leak in heart failure. Circ. Res. 2005, 97, 1314–1322. [Google Scholar] [CrossRef] [PubMed]
- Dries, E.; Santiago, D.J.; Johnson, D.M.; Gilbert, G.; Holemans, P.; Korte, S.M.; Roderick, H.L.; Sipido, K.R. Calcium/calmodulin-dependent kinase II and nitric oxide synthase 1-dependent modulation of ryanodine receptors during β-adrenergic stimulation is restricted to the dyadic cleft. J. Physiol. 2016, 594, 5923–5939. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Izu, L.; Shimkunas, R.; Jian, Z.; Hegyi, B.; Kazemi-Lari, M.; Baker, A.; Shaw, J.; Banyasz, T.; Chen-Izu, Y. Emergence of Mechano-Sensitive Contraction Autoregulation in Cardiomyocytes. Life 2021, 11, 503. https://doi.org/10.3390/life11060503
Izu L, Shimkunas R, Jian Z, Hegyi B, Kazemi-Lari M, Baker A, Shaw J, Banyasz T, Chen-Izu Y. Emergence of Mechano-Sensitive Contraction Autoregulation in Cardiomyocytes. Life. 2021; 11(6):503. https://doi.org/10.3390/life11060503
Chicago/Turabian StyleIzu, Leighton, Rafael Shimkunas, Zhong Jian, Bence Hegyi, Mohammad Kazemi-Lari, Anthony Baker, John Shaw, Tamas Banyasz, and Ye Chen-Izu. 2021. "Emergence of Mechano-Sensitive Contraction Autoregulation in Cardiomyocytes" Life 11, no. 6: 503. https://doi.org/10.3390/life11060503
APA StyleIzu, L., Shimkunas, R., Jian, Z., Hegyi, B., Kazemi-Lari, M., Baker, A., Shaw, J., Banyasz, T., & Chen-Izu, Y. (2021). Emergence of Mechano-Sensitive Contraction Autoregulation in Cardiomyocytes. Life, 11(6), 503. https://doi.org/10.3390/life11060503





