Simulation of Energy Absorption Performance of the Couplers in Urban Railway Vehicles during a Heavy Collision
Abstract
1. Introduction
2. Collision Test for Coupling Buffer System
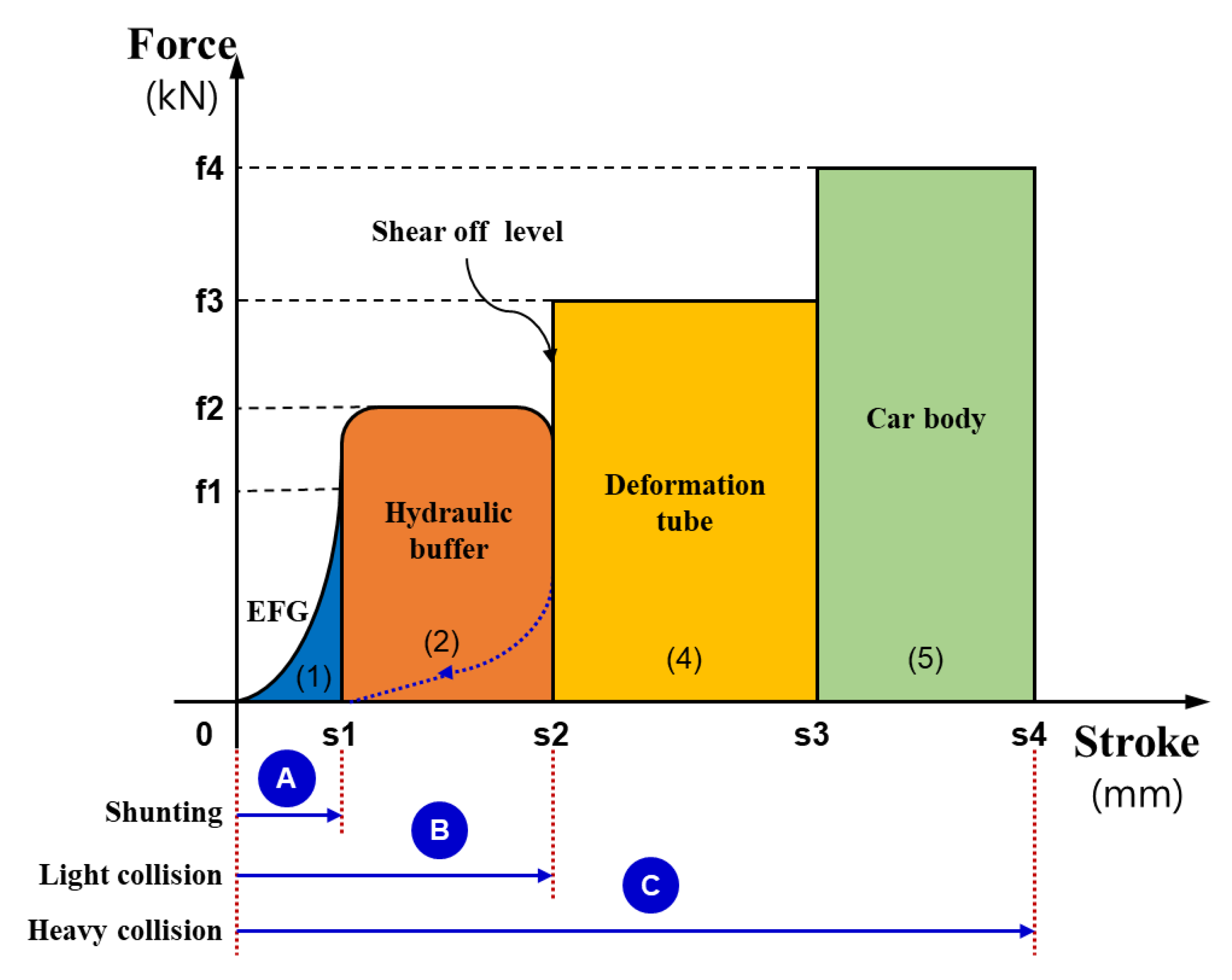
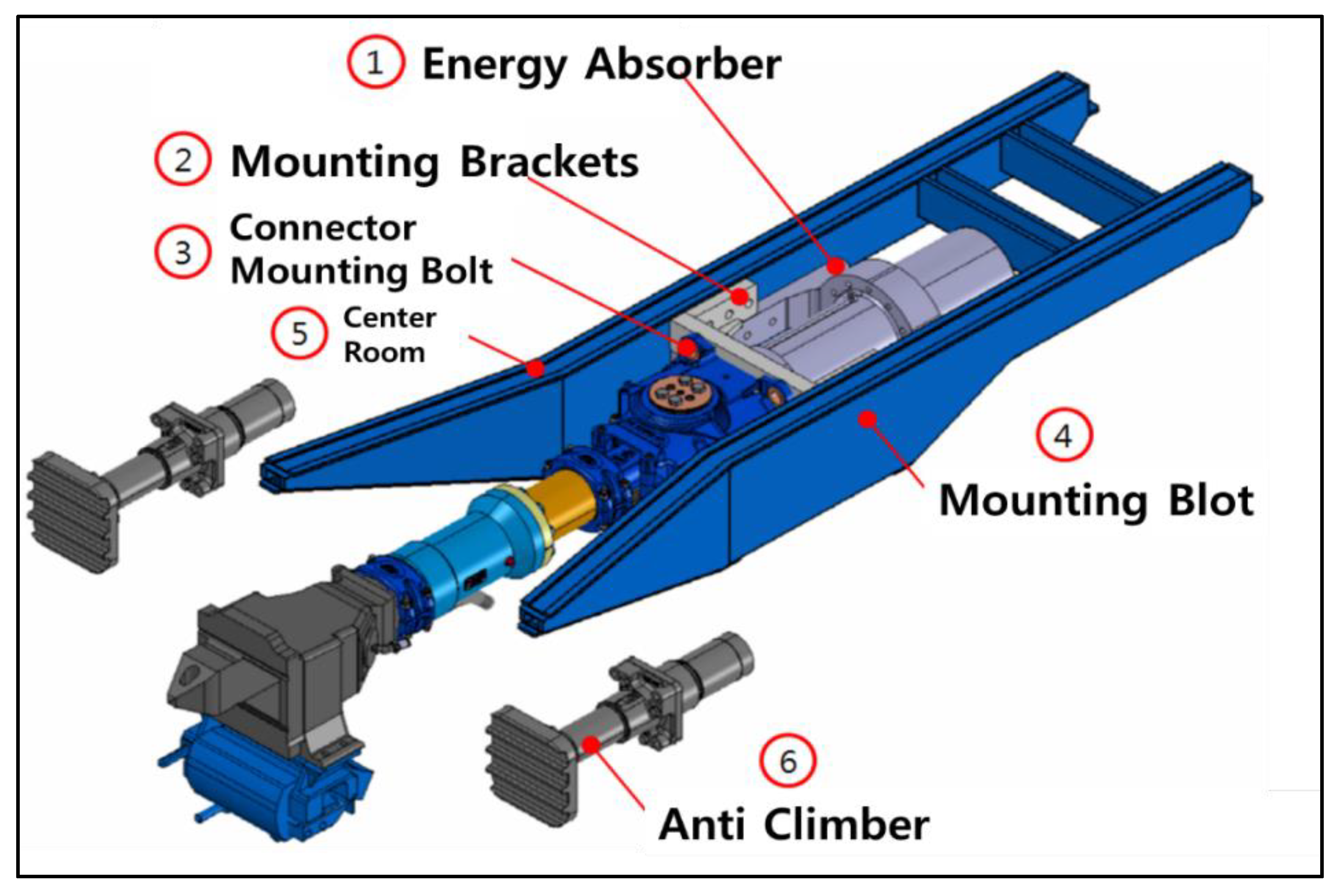
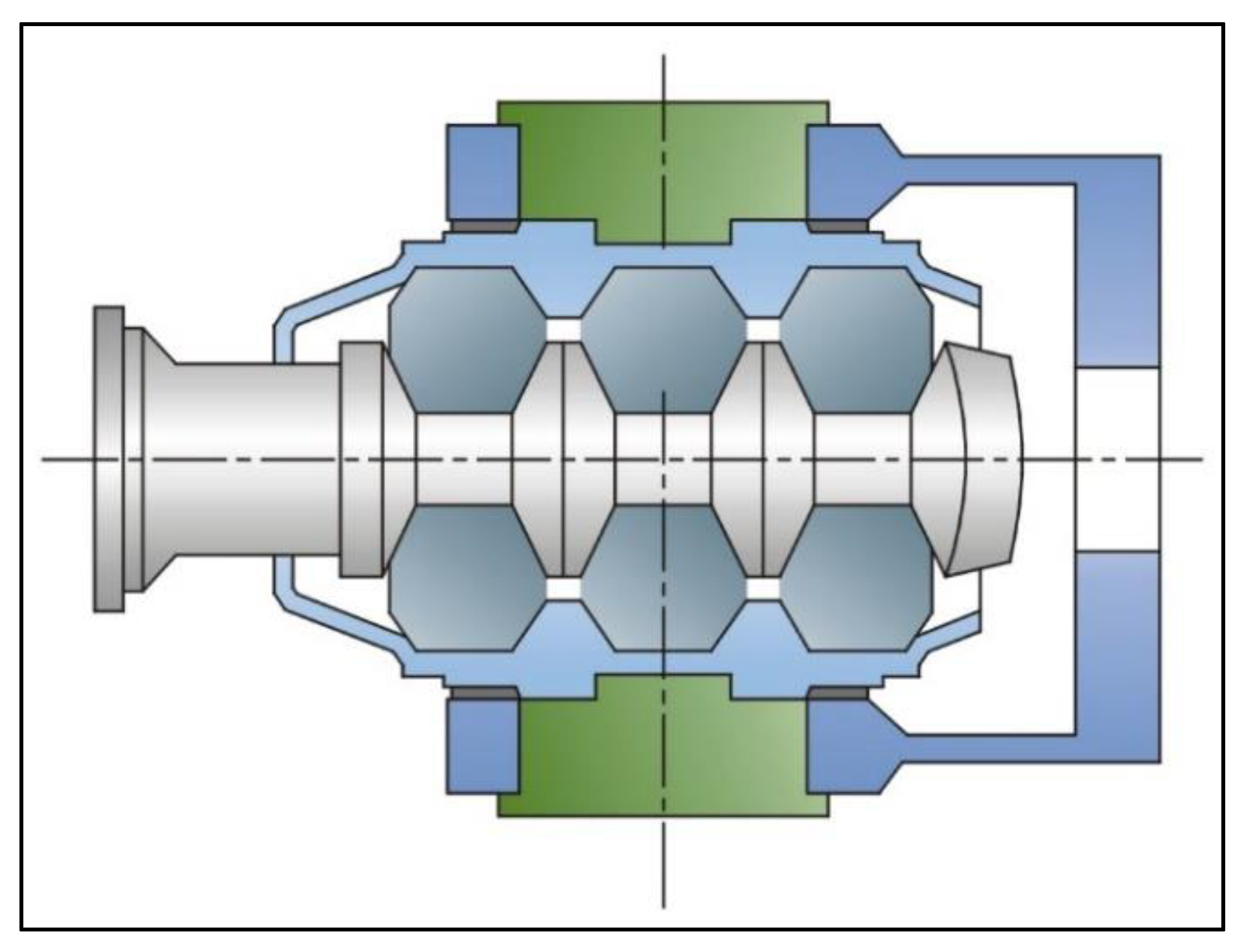
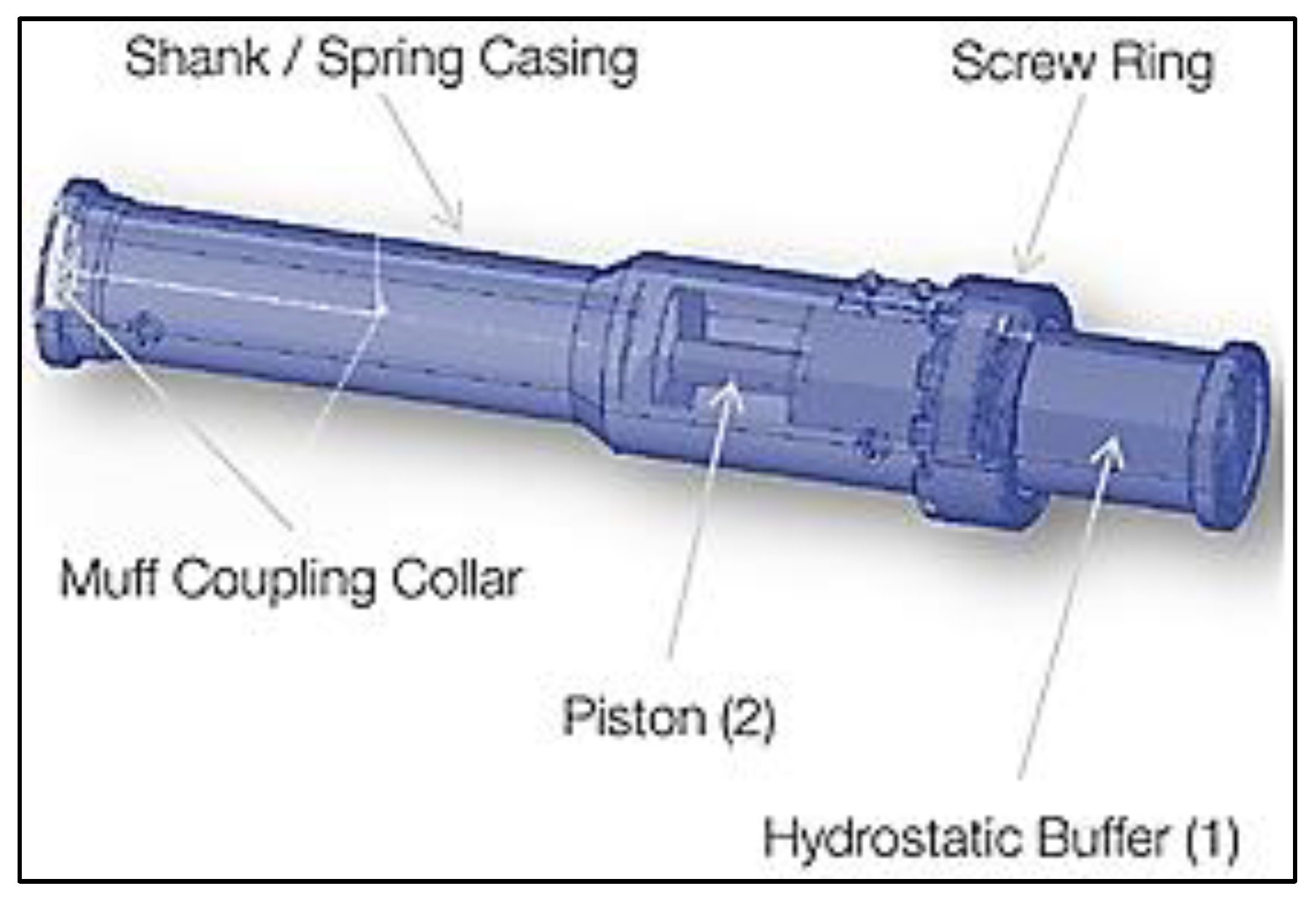
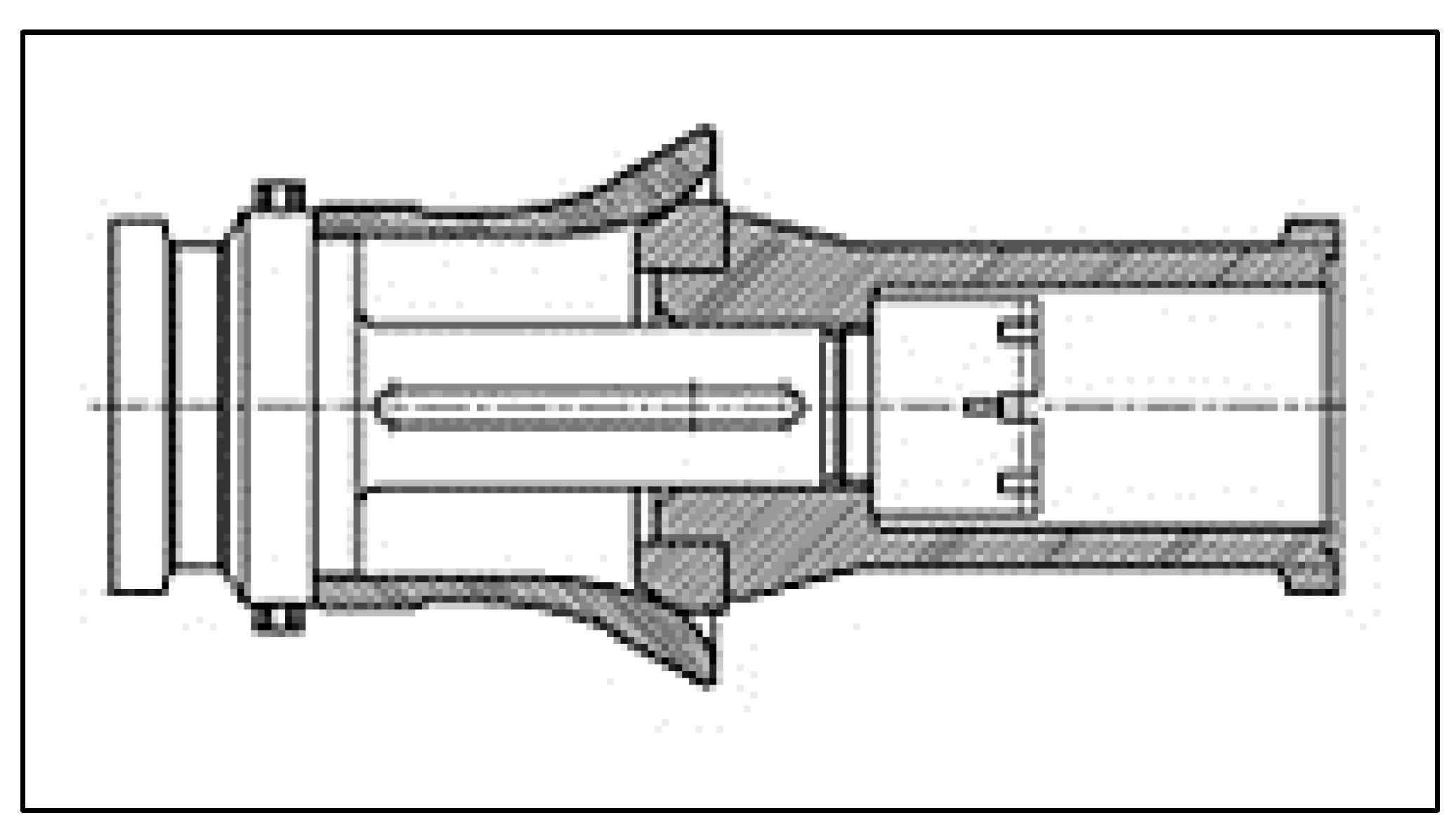
2.1. Components of Coupling Buffer System
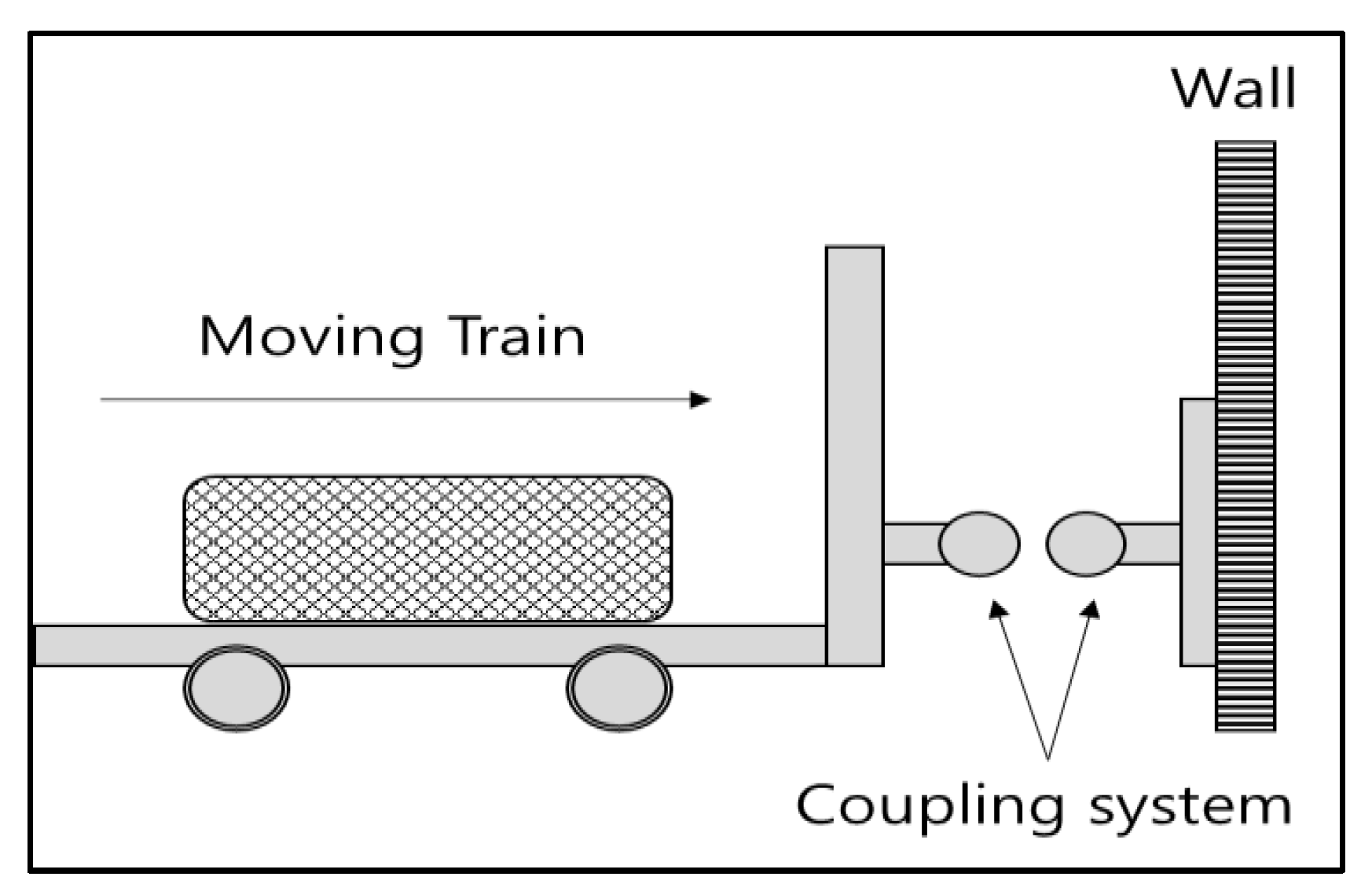
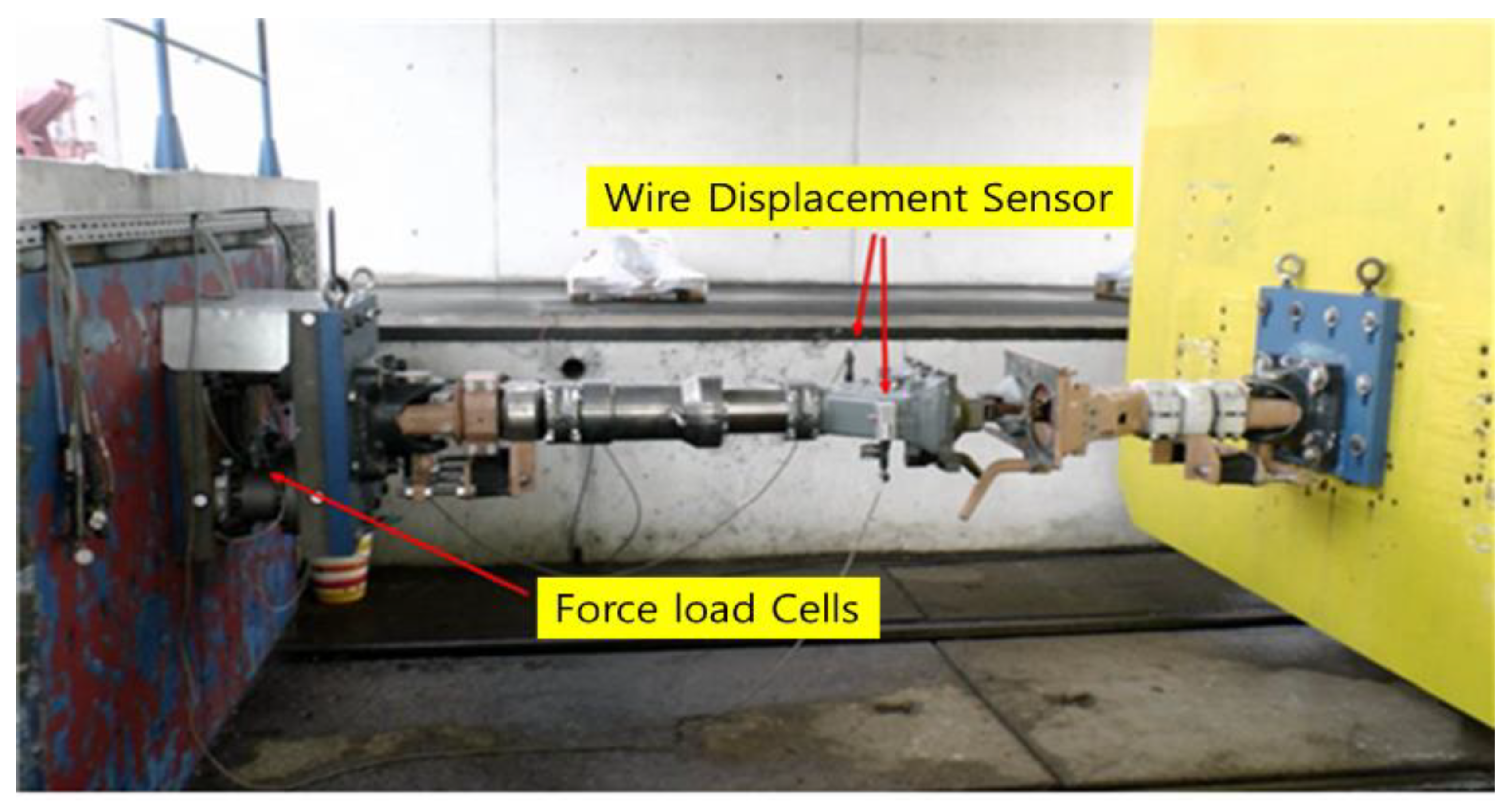
2.2. Collision Test Configuration
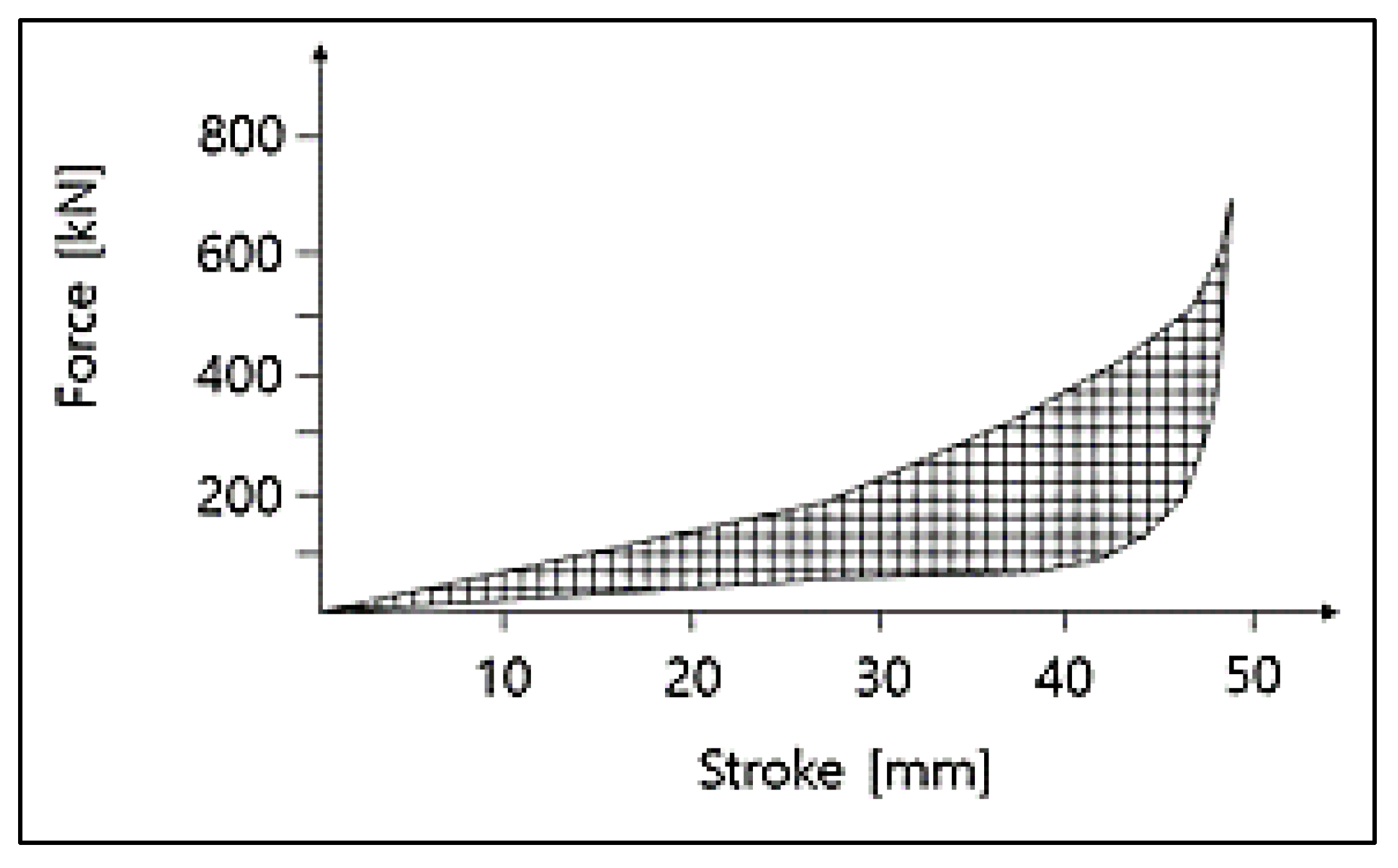
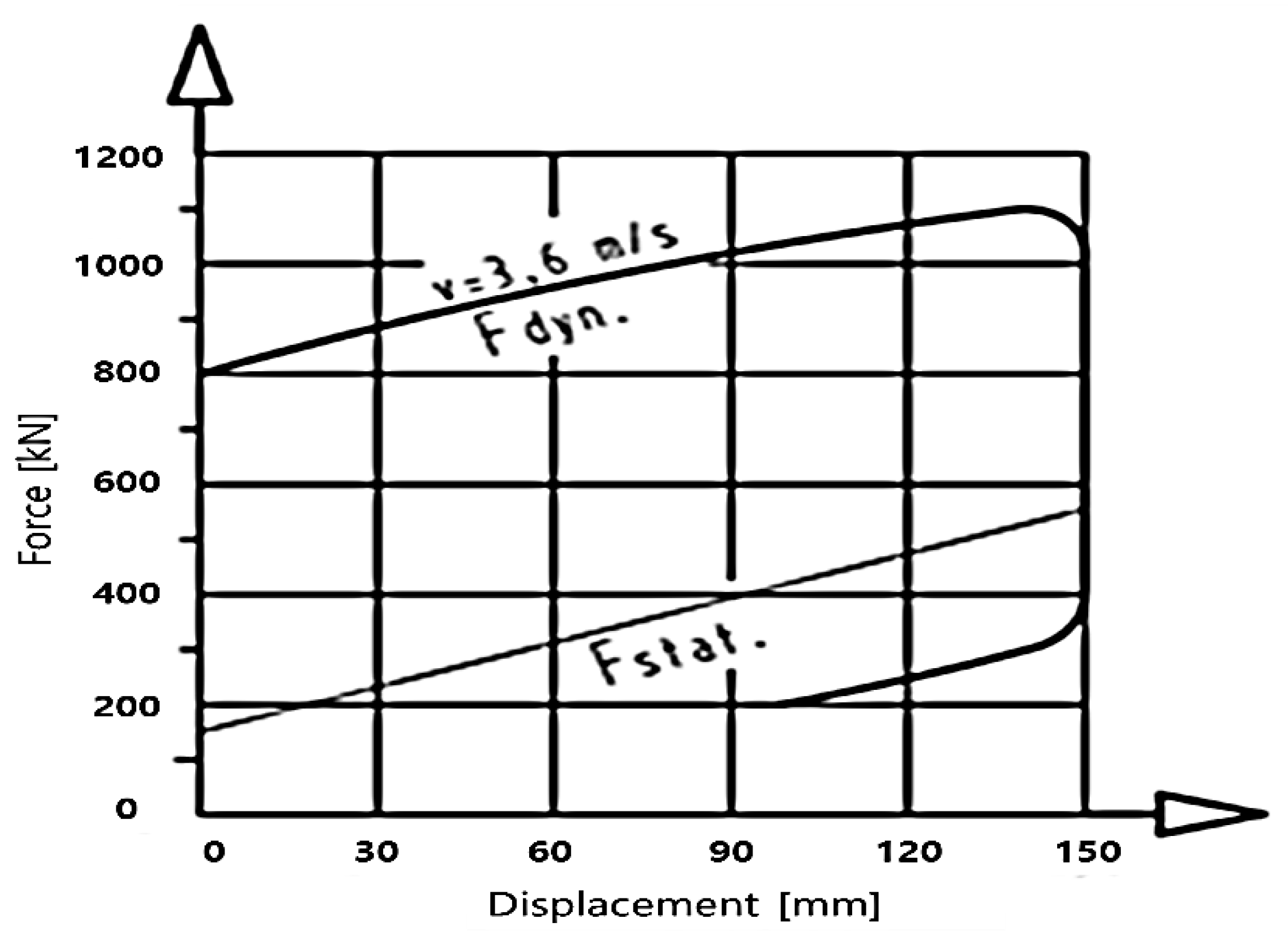
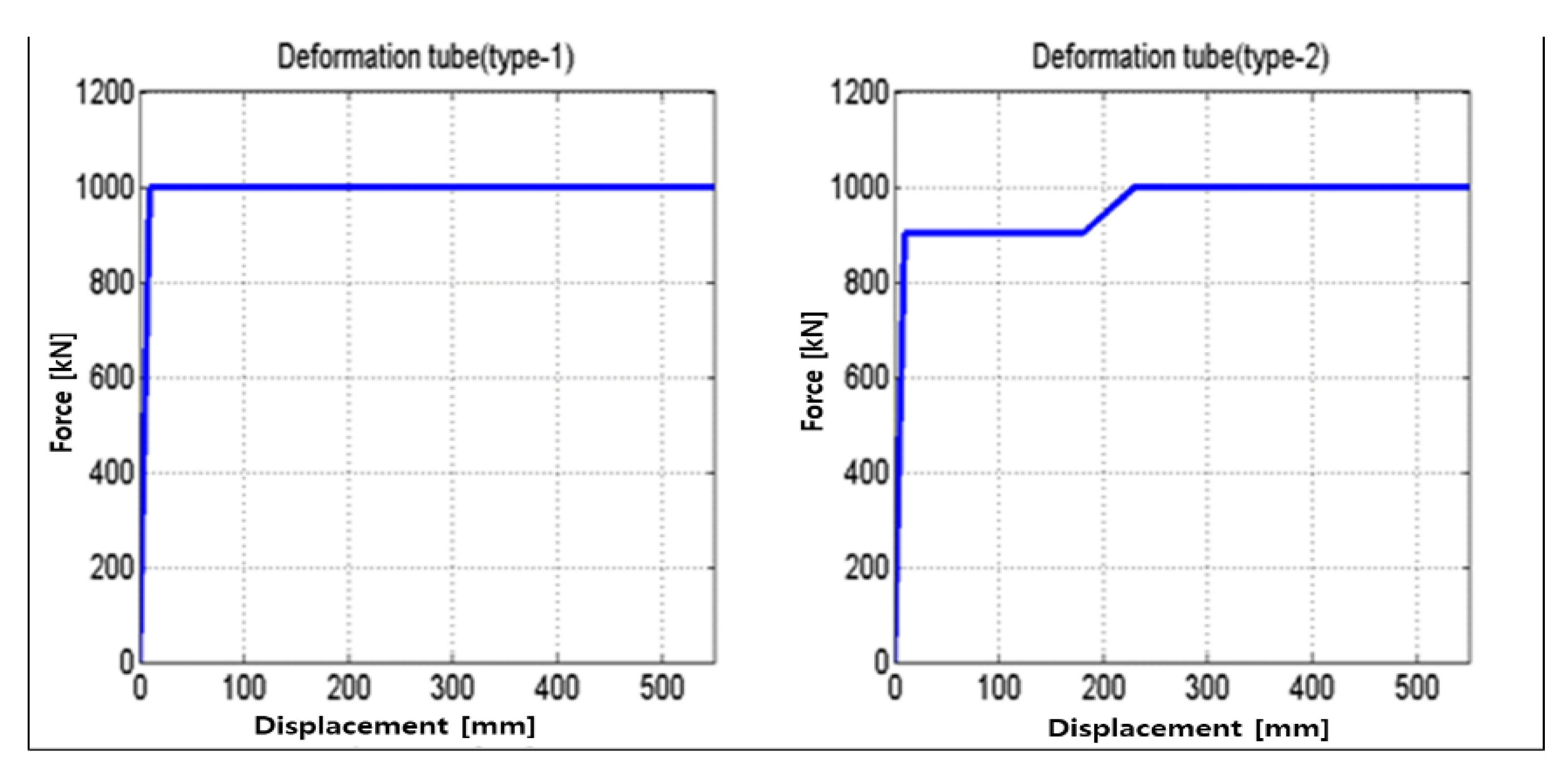
2.3. Collision Test Results
3. Collision Simulation
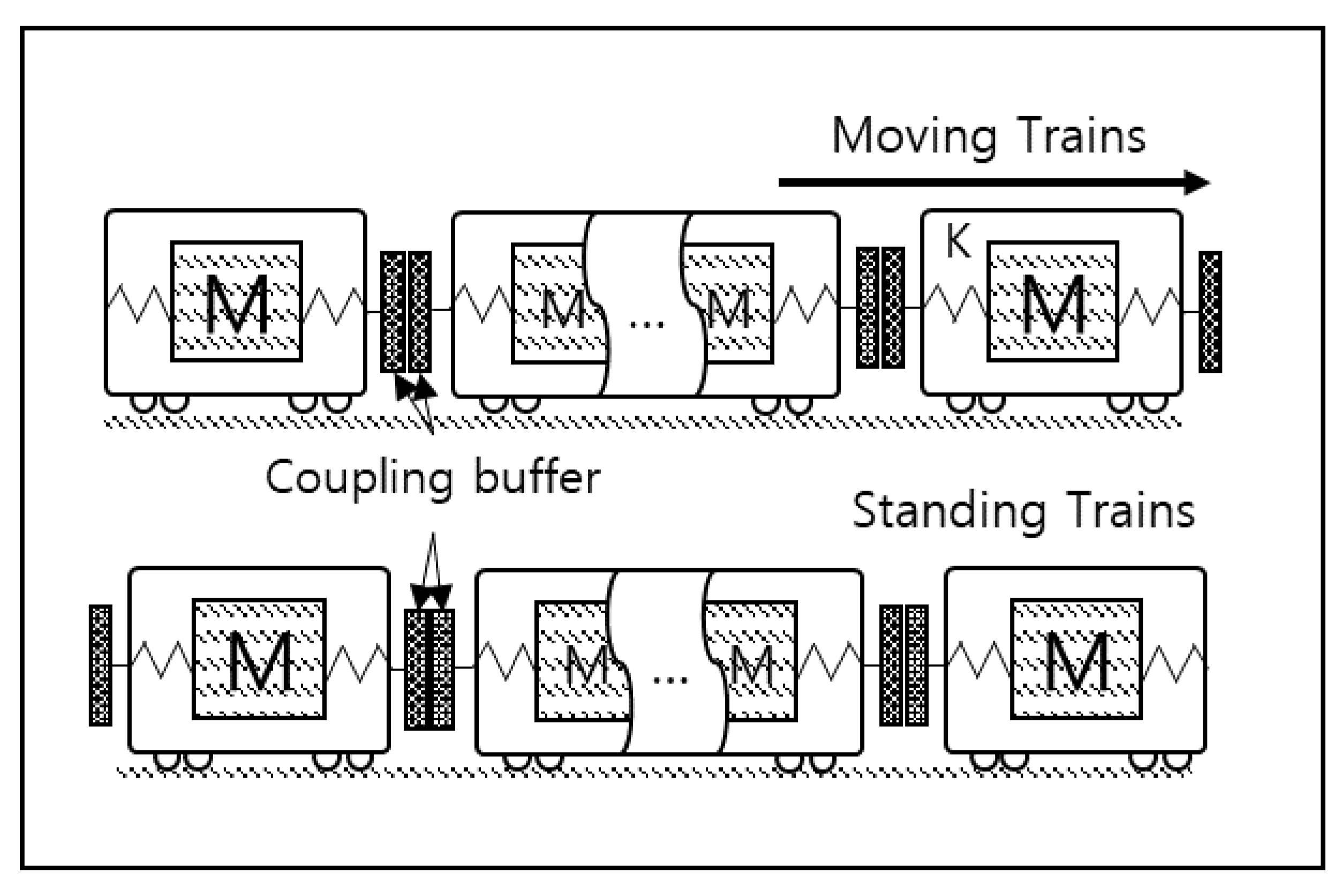
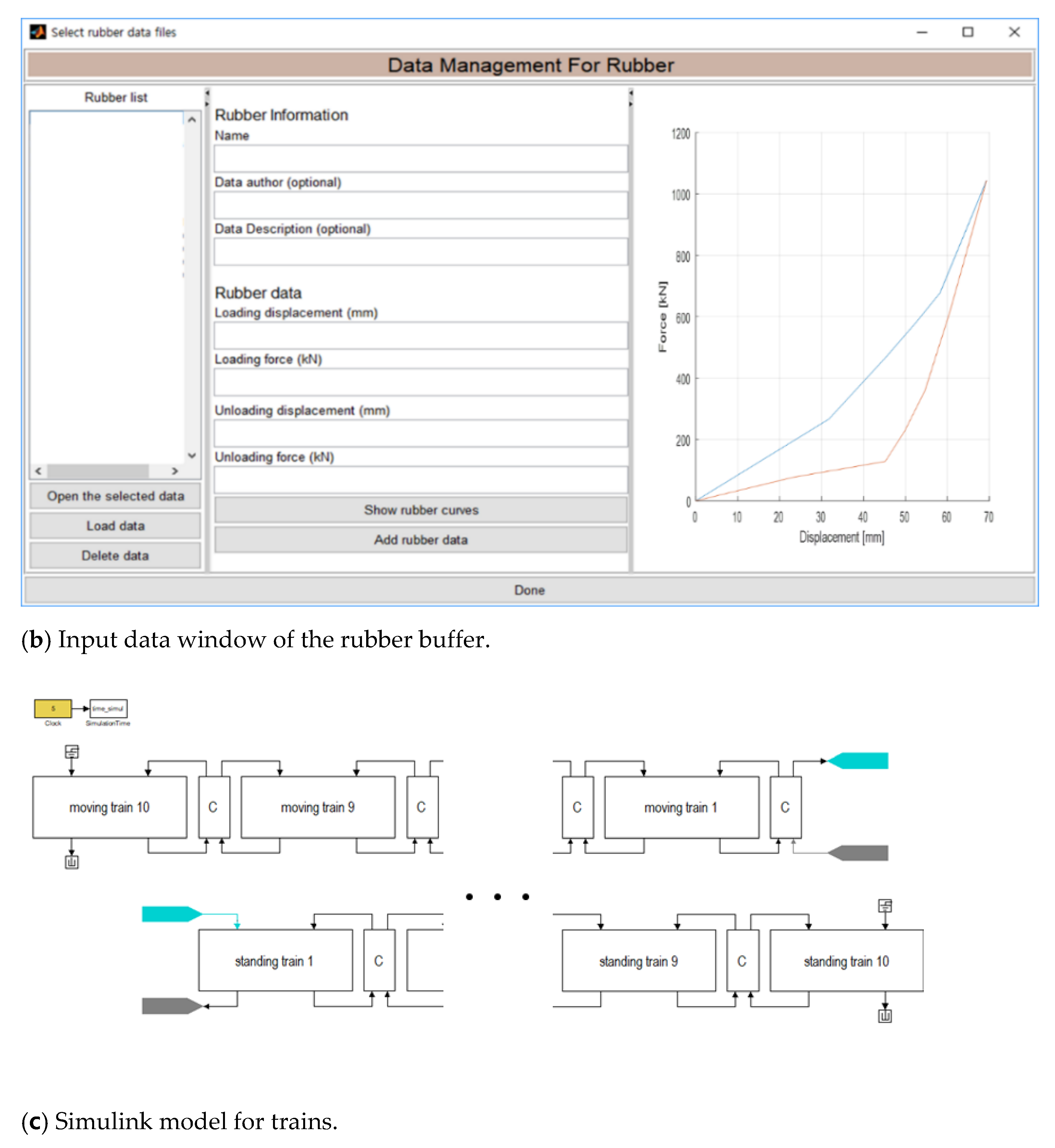
3.1. Railway Vehicle and Coupling Buffer System Model
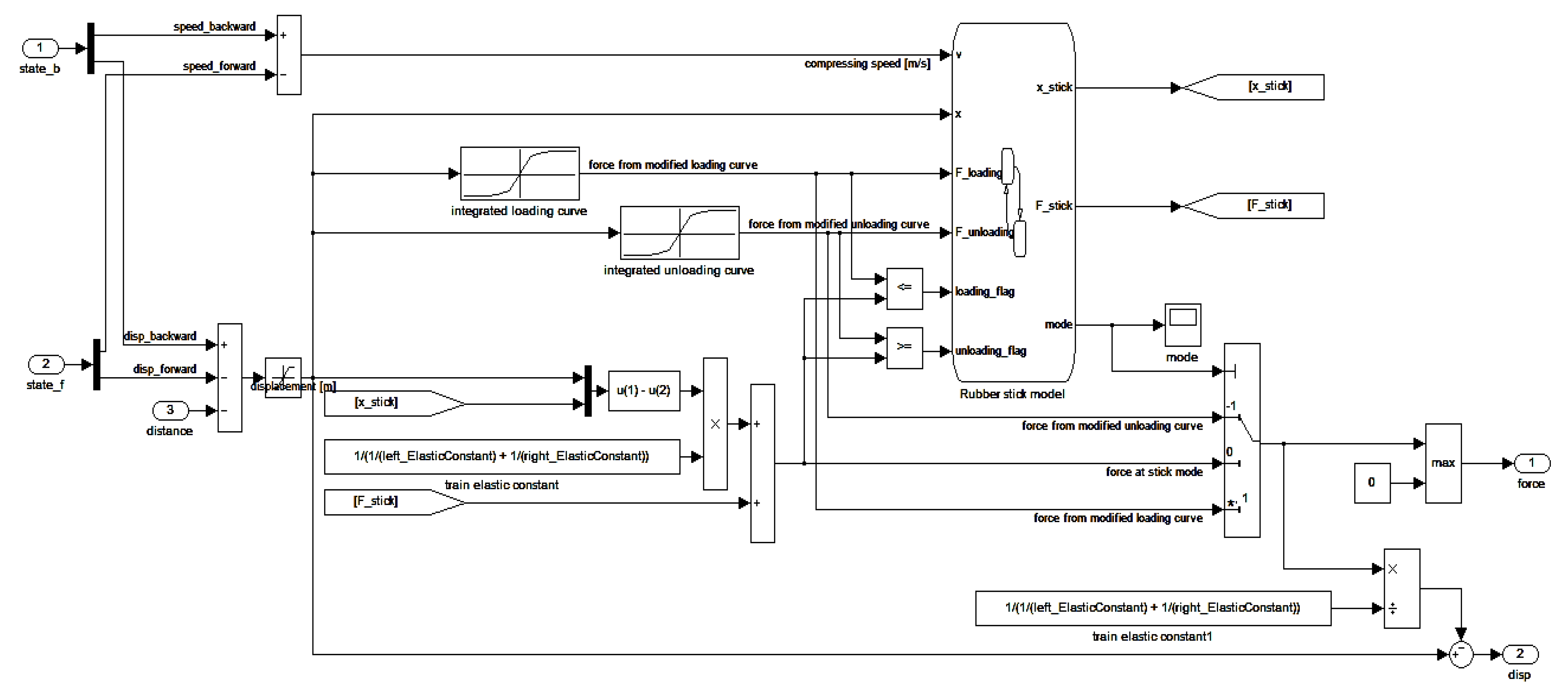
3.2. Collision Simulation Program
3.3. Simulation Condition
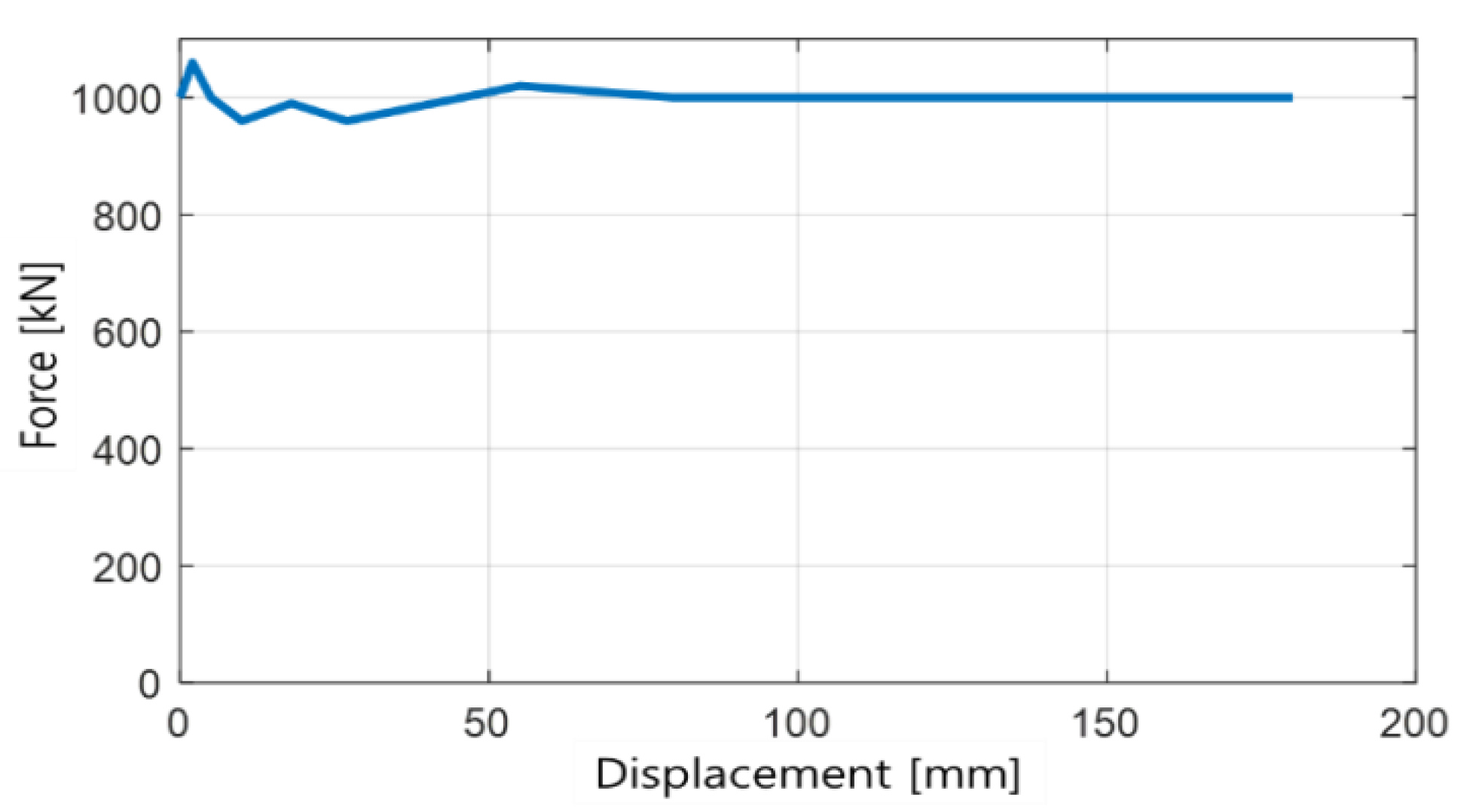
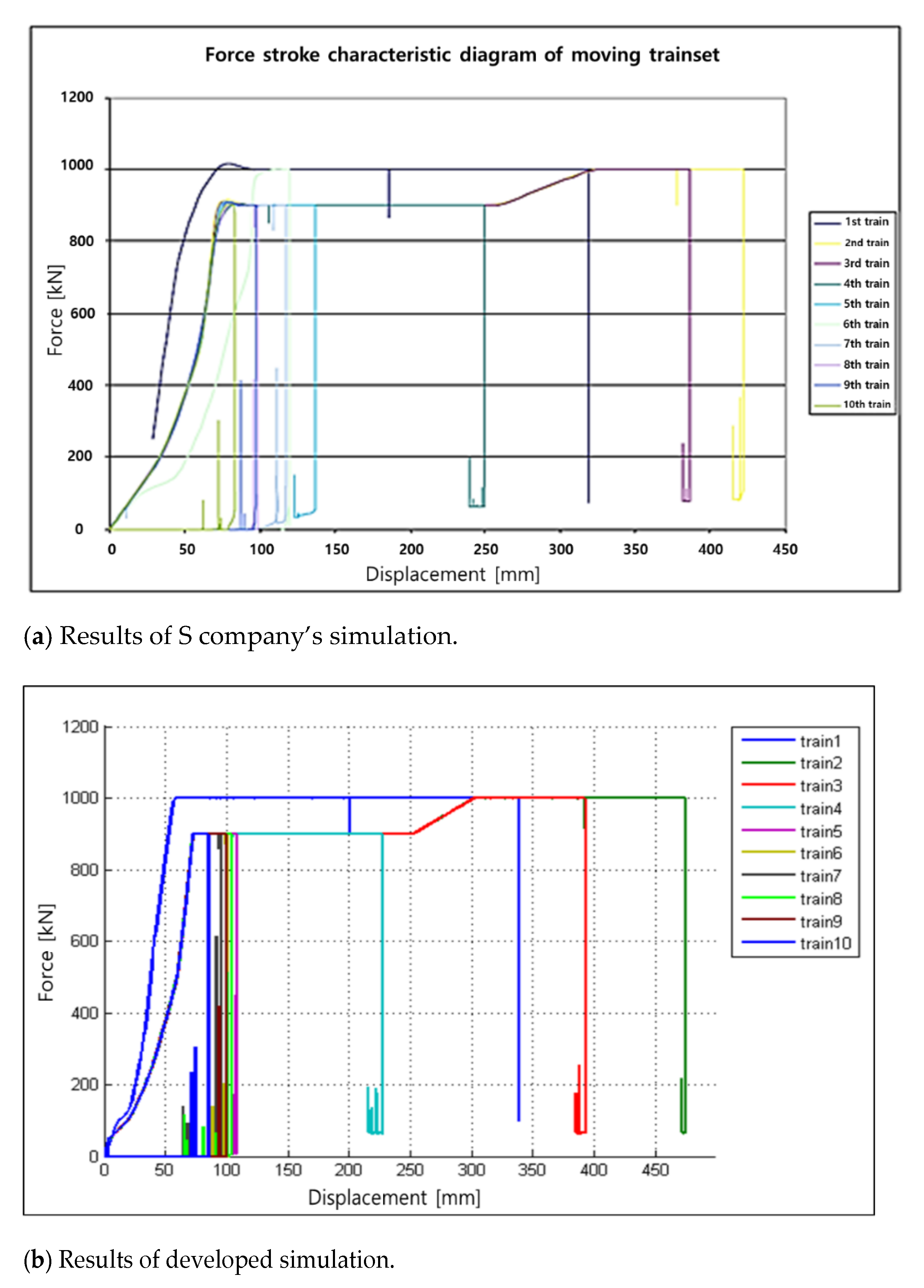
3.4. Verification for Developed Program
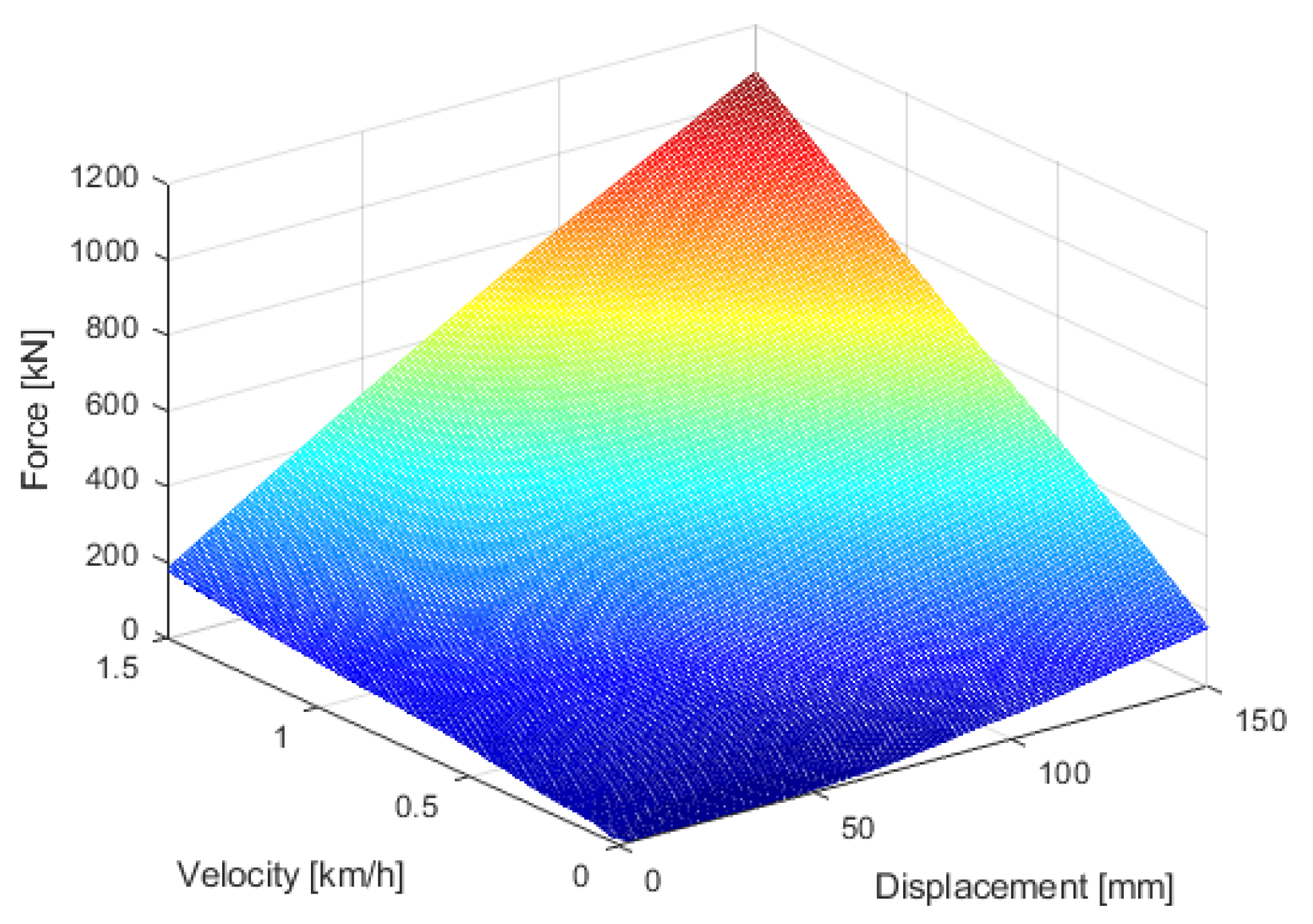
3.5. Simulation Results and Discussion
4. Conclusions
- Since it was difficult to perform a crash test on the assembled buffer, tests were performed for each component. Based on the results of these collision tests, a mathematical model was developed for each component constituting the coupling buffer system.
- A simulation program was developed to simulate a train collision situation including pre-processing and post-processing using mathematical models of vehicle and body models and buffer components. Because we compared the developed program with a program used by an actual company, the energy dissipation phenomenon was well matched, and the development program verified that the analysis process for the collision phenomenon was well implemented.
- Using the developed simulator, a situation where 10 trains collide with 10 fixed trains at a speed of 25 km/h was carried out. The results are summarized as follows.
- The rubber buffer was directly connected to the hydraulic shock absorber through solid contact, and the hydraulic buffer had little displacement due to the rapid increase of impact speed and the increase in reaction force.
- In the case of a heavy collision, the increased impact force was concentrated on the vehicle to which the collision was applied, therefore it may be appropriate to apply a deformation tube having different characteristics depending on the vehicle position.
- Immediately after a collision, it could be see that all vehicles vibrated under the influence of adjacent vehicles and eventually all vehicles came to a standstill. Due to friction, the propagation conditions of moving trains and standing trains appeared different.
- In the event of a collision, a large amount of kinetic energy was lost due to buffering and braking, and after a certain period, the connector system no longer absorbed energy and the buffer function was lost.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Urban Rail Vehicle Technology Standards [KRT-VE-Part5-2017(R1)]. Available online: https://krts.krri.re.kr/pdffile/technology/2017/%EC%B2%A0%EB%8F%84%EC%B0%A8%EB%9F%89%20%EC%95%88%EC%A0%84%ED%92%88%EB%AA%A9%EA%B2%80%EC%82%AC%EA%B8%B0%EC%A4%80.pdf (accessed on 20 April 2021).
- Kim, N.; Park, Y. Collision tests and model development of a train coupling system using a high-capacity energy absorber. J. Rail Rapid Transit. 2018, 232, 2215–2227. [Google Scholar] [CrossRef]
- Jang, H. Analysis on the Cushion Characteristics of a Rolling Stock Coupler Adapted the Hydrostatic Buffer. Ph.D. Thesis, Seoul National University of Science and Technology, Seoul, Korea, 2011. [Google Scholar]
- Gao, G.J.; Tian, H.Q. Train’s crashworthiness design and collision analysis. Int. J. Crashworthiness 2010, 12, 21–28. [Google Scholar] [CrossRef]
- Kim, N.; Park, Y. Performance Tests of a High Capacity Buffer Coupling System using a Hydraulic Device. J. Korean Soc. Saf. 2016, 31, 33–40. [Google Scholar] [CrossRef][Green Version]
- Koo, J.; Song, D. Collision analysis of the full rake TGV-K on crashworthiness. J. Korean Soc. Railw. 1998, 1, 1–9. [Google Scholar]
- Maeng, H.; Kim, J.; Park, Y. Collision Simulation for the Coupler System of Rolling Stock. J. Korean Soc. Manuf. Technol. Eng. 2013, 22, 566–572. [Google Scholar]
- Ogden, R.W. Large deformation isotropic elasticity—On the correlation of theory and experiment for incompressible rubberlike solids. Proc. R. Soc. London A Math. Phys. Sci. 1972, 326, 565–584. [Google Scholar] [CrossRef]
- Jang, H.M.; Kim, K.N.; Park, Y.I. Comparison of simulation models for train buffer couplings. Trans. KSAE 2010, 18, 107–114. [Google Scholar]
- Park, Y. Simulation Technology on the Cushion Characteristics of a Rolling Stock Coupler. Auto J. 2011, 33, 26–31. [Google Scholar]
- Jang, H.; Park, Y.; Goo, B.; Lee, J.; Park, H. Impact analysis of an electric train considering buffer characteristic. In Proceedings of the KSAE Conference, Gunsan, Korea, 14–16 October 2004; pp. 630–635. [Google Scholar]
- VOIT. Available online: https://voith.com/corp-en/connection-components-couplings/railway-couplers.html (accessed on 2 October 2020).
- Park, Y.; Jang, H.; Kim, K.; Kim, N. Analysis on the Cushion Characteristics of a Rolling Stock adapted the Dynamic model for Buffer couplers. In Proceedings of the KSAE Conference, Jeju, Korea, 19–21 May 2011; pp. 2432–2434. [Google Scholar]
- BS EN 15227. Railway Applications-Crashworthiness Requirement for Railway Vehicle Bodies. Available online: https://shop.bsigroup.com/ProductDetail/?pid=000000000030217909 (accessed on 30 April 2021).
- Kim, N.; Jang, H.M.; Kim, K.N.; Park, Y.I. Equivalent model of coupling system based on experimental results. In Proceedings of the KSAE Conference, Seoul, Korea, 22–24 April 2008; Volume 4, pp. 1061–1065. [Google Scholar]
- Craciun, C.; Mitu, A.M.; Cruceanu, C.; Sireteanu, T. Modeling the Buffers Hysteretic Behavior for Evaluation of Longitudinal Dynamic In-train Forces. In Proceedings of the SISOM 2012 and Session of the Commission of Acoustics, Bucharest, Romania, 30–31 October 2012. [Google Scholar]
- Kajita, Y.; Kitahara, T.; Nishimoto, Y.; Otsuka, H. Estimation of maximum impact force on natural rubber during collision of two steel bars. In Proceedings of the 1st European Conference on Earthquake Engineering and Seismology, Geneva, Switzerland, 3–8 September 2006; pp. 3–8. [Google Scholar]
- Guo, Z. Computational Modeling of Rubber-Like Materials under Monotonic and Cyclic Loading. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2006. [Google Scholar]
- Freidenberg, A.; Lee, C.W.; Durant, B.; Nesterenko, V.F.; Stewart, L.K.; Hegemier, G.A. Characterization of the blast simulator elastomer material using a pseudo-elastic rubber model. Int. J. Impact Eng. 2013, 60, 58–66. [Google Scholar] [CrossRef]
- Kim, Y.H.; Kim, K.N.; Jang, H.M.; Park, Y.I. Modeling of coupling device for crash analysis of an electric vehicle. In Proceedings of the KSR Conference, Singapore, 30–31 October 2004; pp. 640–645. [Google Scholar]

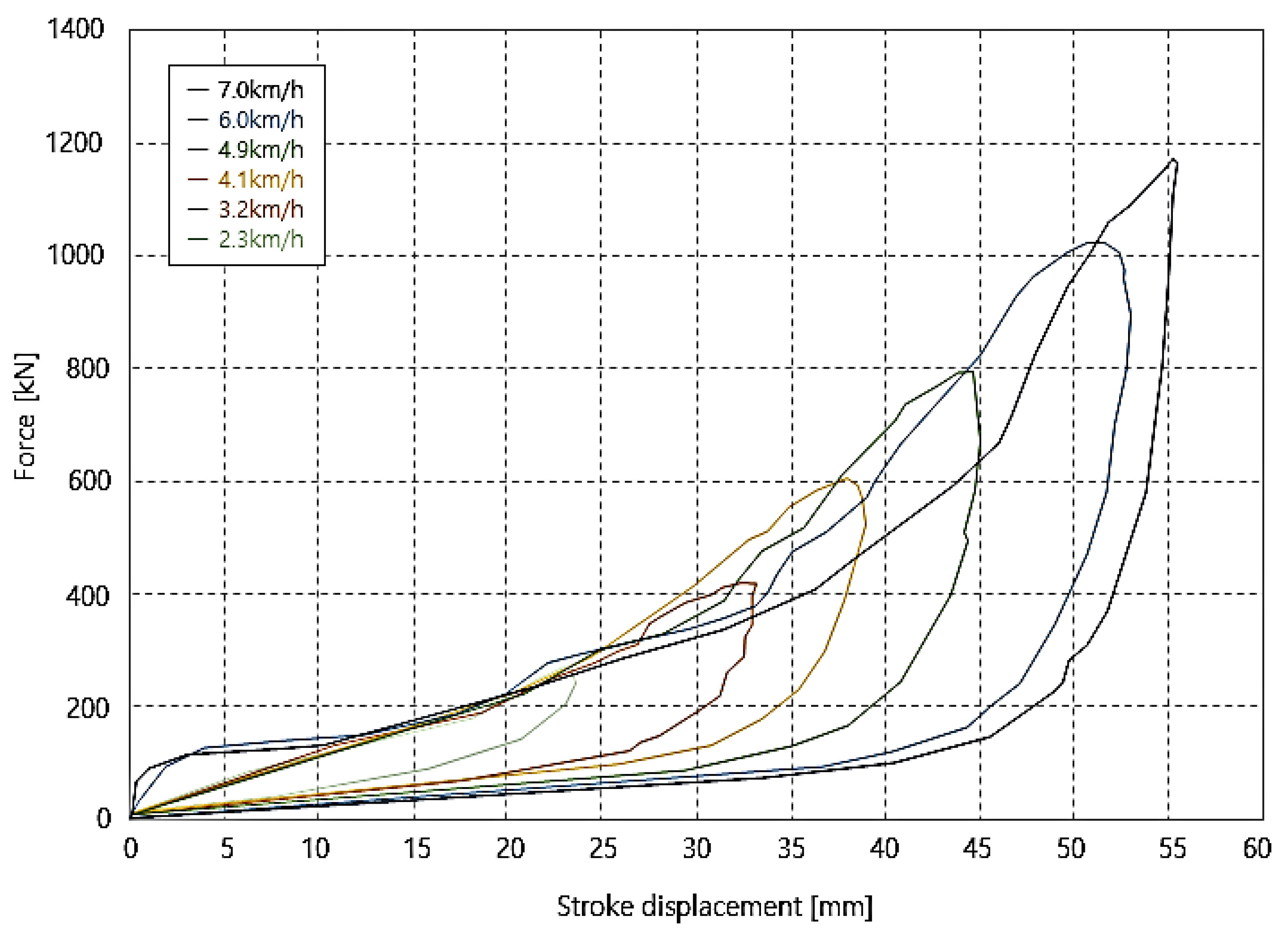
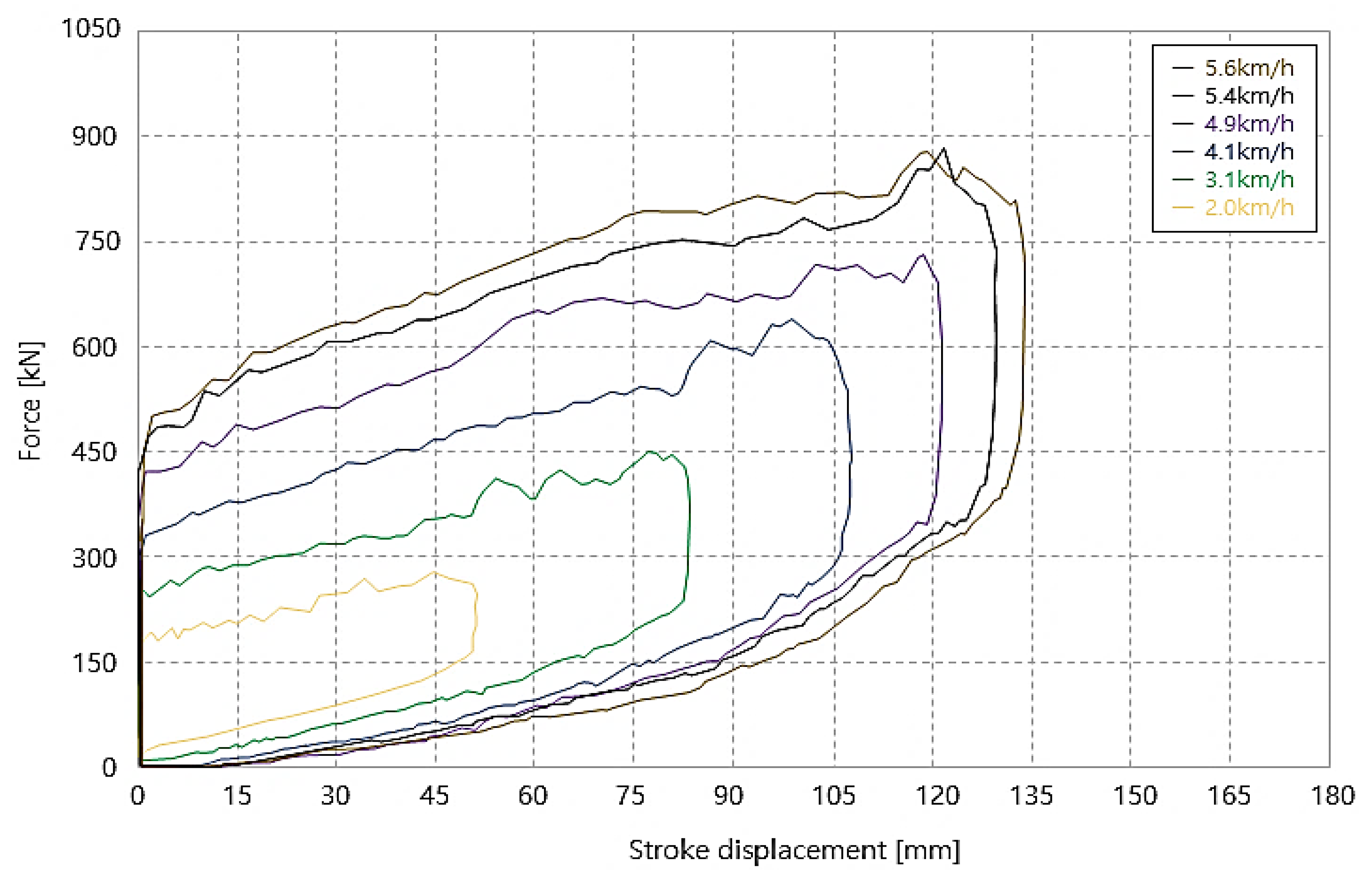







| Buffer Type | Velocity [km/h] | Wight [ton] |
|---|---|---|
| Rubber buffer | 2.3–7.0 | 20.5 |
| Hydraulic buffer | 2.0–5.6 | 81.1 |
| Middle connector | 14.7 | 20.5 |
| Energy | S Company | Developed |
|---|---|---|
| Initial energy | 4411 [kJ] | 4411.1 [kJ] |
| Final energy | 36.0 [kJ] | 4.3 [kJ] |
| Absorbed energy buffer | 2412.0 [kJ] | 2443.5 [kJ] |
| Absorbed energy buffer (car body) | 0.0 [kJ] | 18.0 [kJ] |
| Energy dissipation | 1961.0 [kJ] | 1946.0 [kJ] |
| Energy balance mistake | 0.04 [%] | 0 [%] |
| Energy | Results |
|---|---|
| Initial energy | 11,362.7 [kJ] |
| Final energy | 0.8 [kJ] |
| Absorbed energy buffer | 7742.2 [kJ] |
| Absorbed energy buffer (car body) | 3.2 [kJ] |
| Energy dissipation, brake | 3615.9 [kJ] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lim, S.; Ji, Y.-h.; Park, Y.-i. Simulation of Energy Absorption Performance of the Couplers in Urban Railway Vehicles during a Heavy Collision. Machines 2021, 9, 91. https://doi.org/10.3390/machines9050091
Lim S, Ji Y-h, Park Y-i. Simulation of Energy Absorption Performance of the Couplers in Urban Railway Vehicles during a Heavy Collision. Machines. 2021; 9(5):91. https://doi.org/10.3390/machines9050091
Chicago/Turabian StyleLim, Sunghyun, Yong-hyeon Ji, and Yeong-il Park. 2021. "Simulation of Energy Absorption Performance of the Couplers in Urban Railway Vehicles during a Heavy Collision" Machines 9, no. 5: 91. https://doi.org/10.3390/machines9050091
APA StyleLim, S., Ji, Y.-h., & Park, Y.-i. (2021). Simulation of Energy Absorption Performance of the Couplers in Urban Railway Vehicles during a Heavy Collision. Machines, 9(5), 91. https://doi.org/10.3390/machines9050091






