Design of Delivery Valve for Hydraulic Pumps
Abstract
1. Introduction
- -
- the v of the fluid, which depends on the load loss corresponding to the passage through the valve;
- -
- v′, which affects the water pressure acting against the dish-shaped valve;
- -
- and the vv of the dish-shaped valve of the considered system.
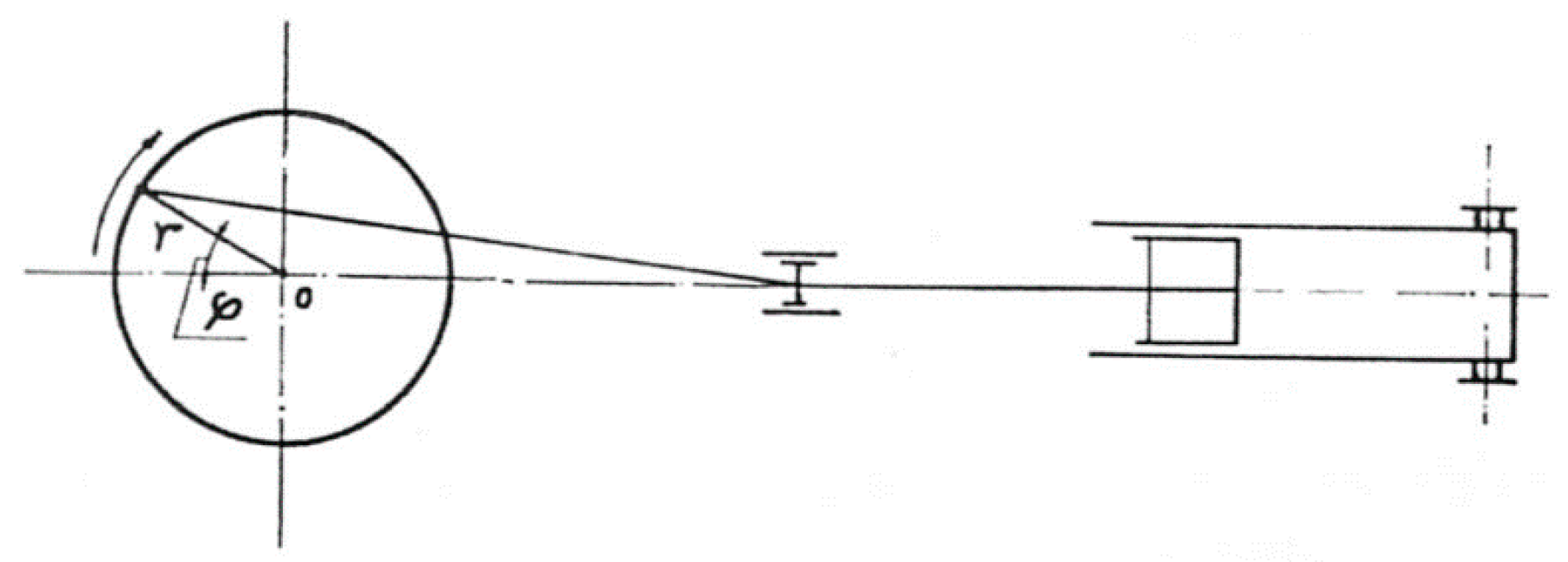
2. Preliminary Analysis
3. Additional Effects and Their Influence
4. Design Method
| -Pumps for exhaustion (small prevalence) | μ·v = 1÷2 m/s |
| -Pumps for large prevalence | μ·v = 1.5 ÷ 2.5 m/s |
| -Pumps for draining mines | μ·v = 2 ÷ 3 m/s |
| -Pumps for high pressures | μ·v = 3 ÷ 5 m/s |
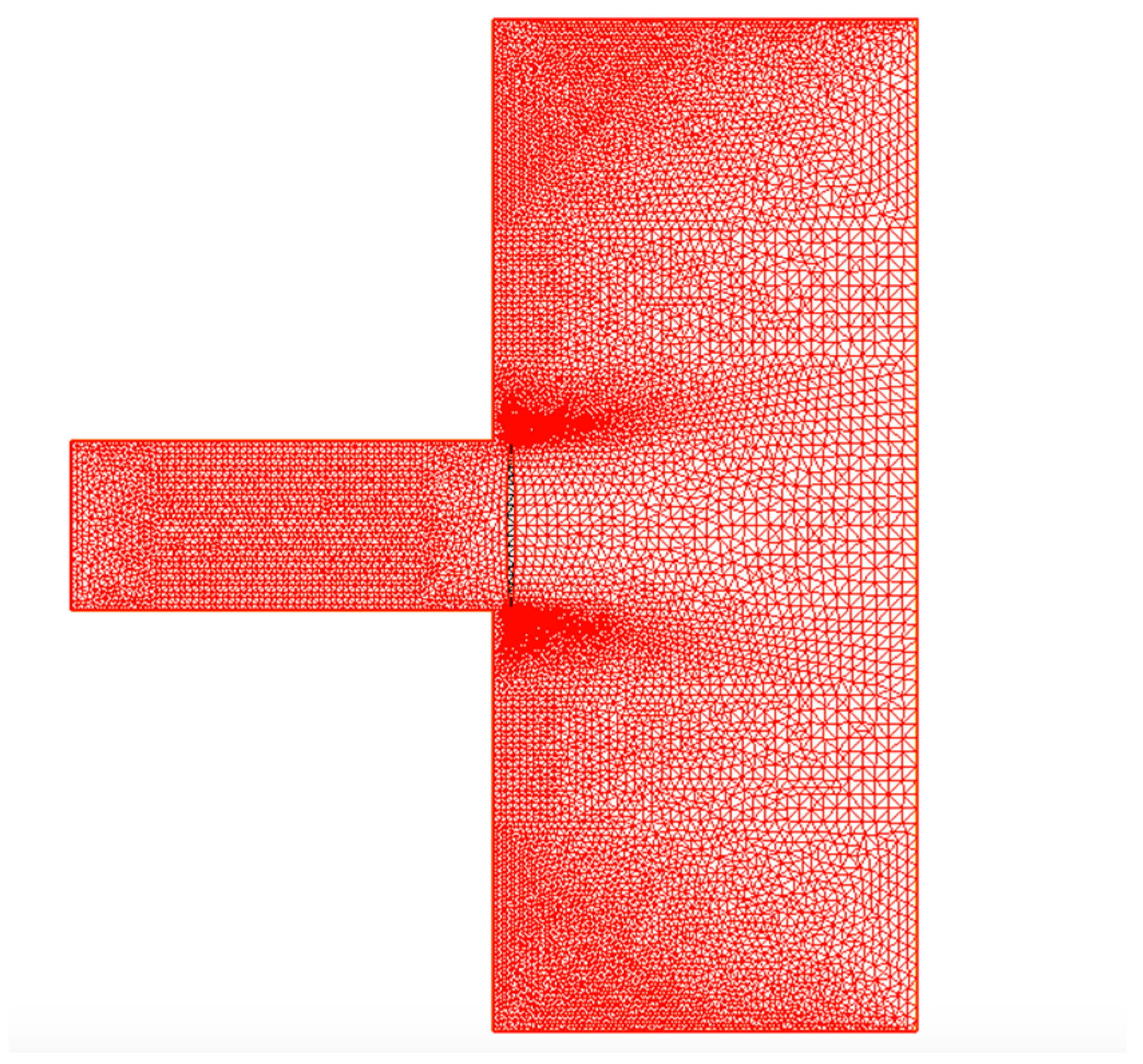
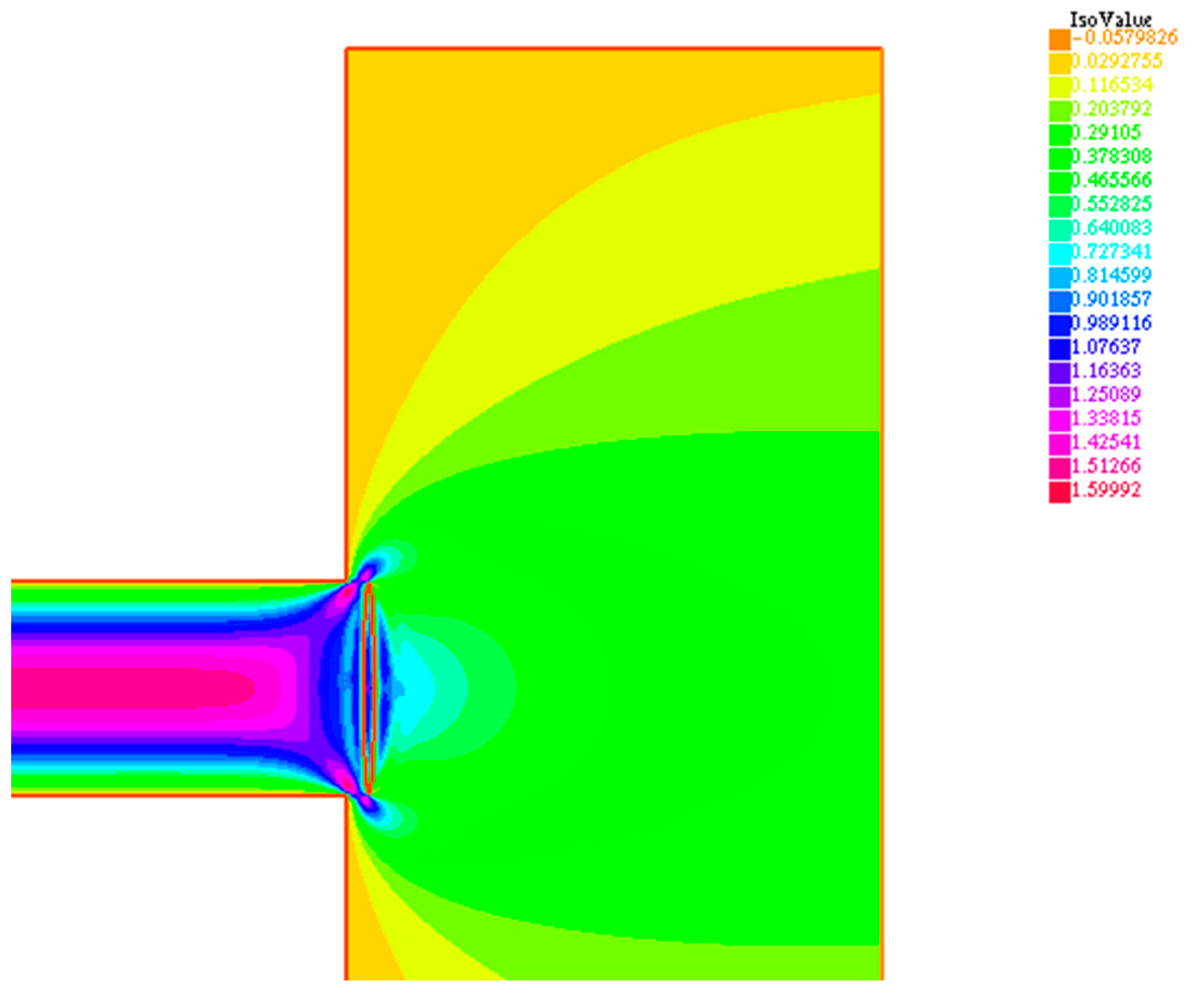
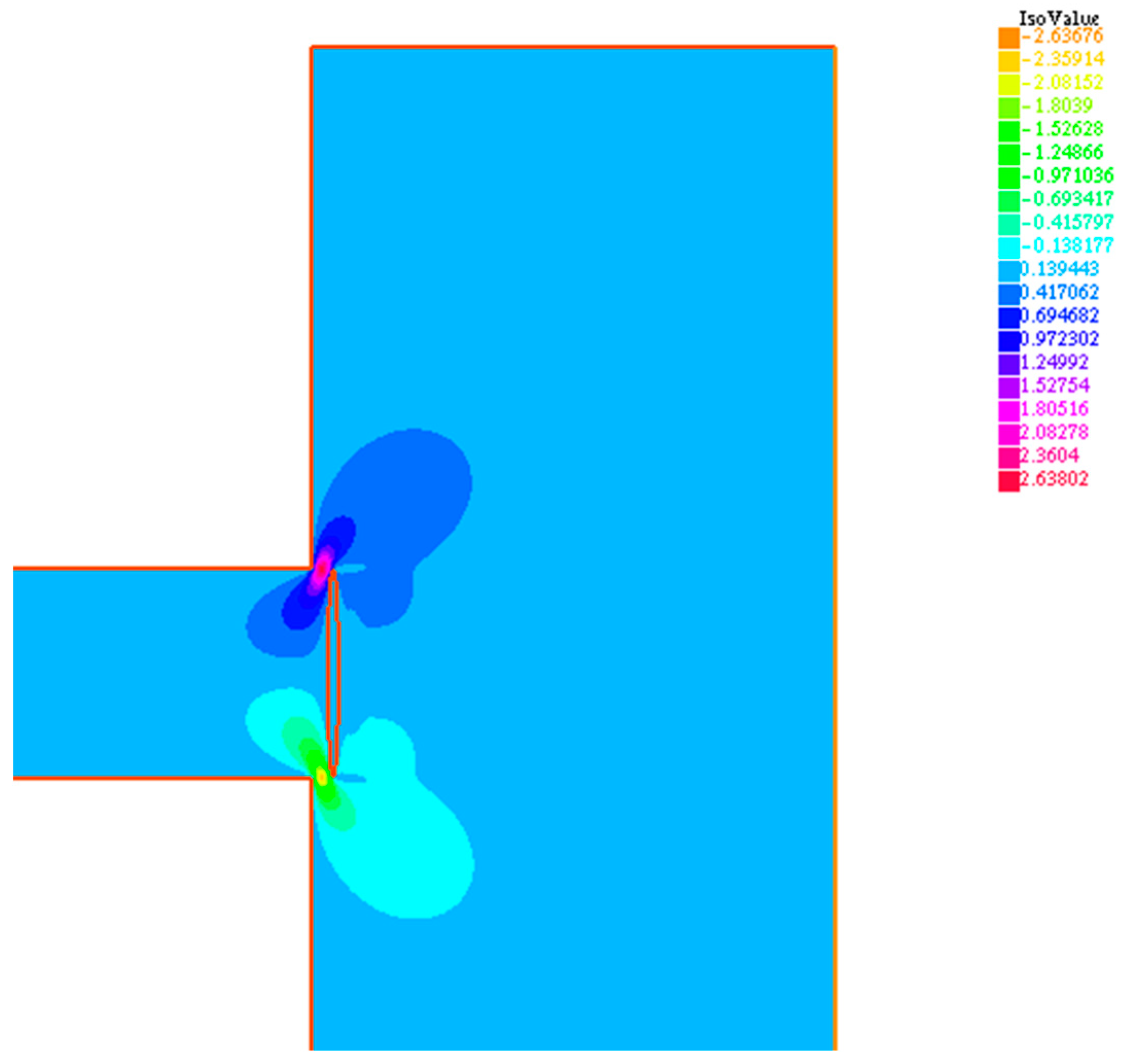
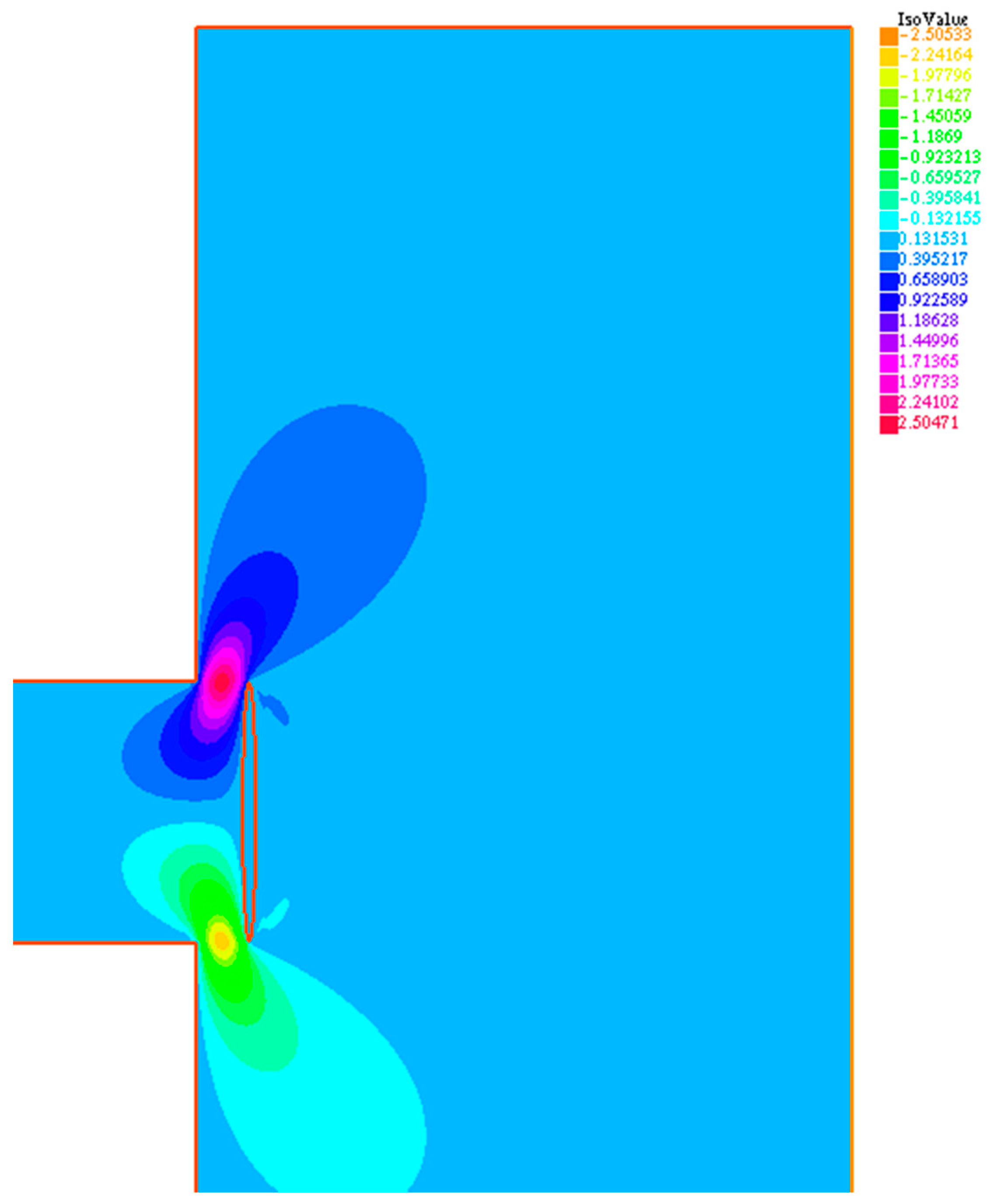
5. Numerical Simulation
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Symbol | |
| angular delay | |
| ω | angular speed of the crank |
| f1 | area of the valve section in the plane of the seat |
| v′ | component of the speed normal to the valve plate = the theoretical outflow speed |
| δ | corner of the seat |
| ξ1 | corrective coefficient that takes into account the water dragged from the plate |
| r | crank radius |
| μ | efflux coefficient |
| vc | impact velocity during closure |
| Q | instant flow |
| h | lift at time t |
| M | mass of the plate |
| hmax | maximum lift |
| dm | medium diameter |
| n | number of rounds |
| μp | particular efflux coefficient determined experimentally and dependent on the type of valve considered |
| φ | path angle |
| l | peripheral development of the port |
| vs | plunger speed |
| F | plunger surface |
| a | radial width |
| s | space traveled by the plunger |
| γ | specific weight of the material of which the plate is made |
| γ1 | specific weight of the pumped liquid |
| u | speed component along the x-axis |
| T | spring load on the valve plate |
| f | straight section of the hole = area of the outflow port |
| v | theoretical water speed through the section |
| vv | valve plate speed |
| P | water thrust on the valve plate |
| G | weight of the plate |
References
- Akers, A.; Gassman, M.; Smith, R. Hydraulic Power System Analysis; Taylor & Francis: New York, NY, USA, 2006. [Google Scholar]
- Pappalardo, C.M.; Zhang, Z.; Shabana, A.A. Use of independent volume parameters in the development of new large displacement ANCF triangular plate/shell elements. Nonlinear Dyn. 2018, 91, 2171–2202. [Google Scholar] [CrossRef]
- Cho, J.; Zhang, X.; Manring, N.D.; Nair, S.S. Dynamic Modelling and Parametric Studies of an Indexing Valve Plate Pump. Int. J. Fluid Power 2002, 3, 37–48. [Google Scholar] [CrossRef]
- Barbagallo, R.; Sequenzia, G.; Cammarata, A.; Oliveri, S.M.; Fatuzzo, G. Redesign and Multibody Simulation of a Motorcycle Rear Suspension with Eccentric Mechanism. Int. J. Interact. Des. Manuf. 2017, 12, 517–524. [Google Scholar] [CrossRef]
- Rivera, Z.B.; De Simone, M.C. Waypoint Navigation for Autonomous Mobile Robots in Ros-based Environments. Robotics 2018, submitted. [Google Scholar]
- Rahmat, M.F.; Rozali, S.M. Modeling and Controller Design of an ElectroHydraulic Actuator System. Am. J. Appl. Sci. 2010, 7, 1100–1108. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Wang, T.; Shabana, A.A. Development of ANCF tetrahedral finite elements for the nonlinear dynamics of flexible structures. Nonlinear Dyn. 2017, 89, 2905–2932. [Google Scholar] [CrossRef]
- Loukianov, A.G.; Rivera, J.; Orlov, Y.V.; Teraoka, E.Y.M. Robust trajectory tracking for an electro-hydraulic actuator. IEEE Trans. Ind. Electron. 2009, 56, 3523–3531. [Google Scholar] [CrossRef]
- De Simone, M.C.; Guida, D. Modal coupling in presence of dry friction. Machines 2018, 6, 8. [Google Scholar] [CrossRef]
- De Simone, M.C.; Rivera, Z.B.; Guida, D. Finite element analysis on squeal-noise in railway applications. FME Trans. 2018, 46, 93–100. [Google Scholar] [CrossRef]
- Battistoni, M.; Foschini, L.; Postrioti, L.; Cristiani, M. Development of an Electro-Hydraulic Camless WA System. SAE Tech. Pap. 2007. [Google Scholar] [CrossRef]
- Turner, C.; Babbitt, G.; Balton, C.; Raimao, M. Design and Control of a Two-stage Electro-hydraulic Valve Actuation System. SAE Tech. Pap. 2004, 1, 1265. [Google Scholar]
- Cammarata, A.; Sequenzia, G.; Oliveri, S.M.; Fatuzzo, G. Modified Chain Algorithm to Study Planar Compliant Mechanisms. Int. J. Interact. Des. Manuf. 2016, 10, 191–201. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Wang, T.; Shabana, A.A. On the formulation of the planar ANCF triangular finite elements. Nonlinear Dyn. 2017, 89, 1019–1045. [Google Scholar] [CrossRef]
- Rahmat, M.F.; Rozali, S.M.; Wahab, N.A.; Zulfatman, A. Application of Draw Wire Sensor in Position Tracking of Electro-Hydraulic Actuator System. Int. J. Smart Sens. Intell. Syst. 2010, 3, 736–755. [Google Scholar] [CrossRef]
- De Simone, M.C.; Guida, D. Identification and control of a Unmanned Ground Vehicle by using Arduino. UPB Sci. Bull. Ser. D Mech. Eng. 2018, 80, 141–154. [Google Scholar]
- Ghomshei, M.; Villecco, F.; Porkhial, S.; Pappalardo, M. Complexity in Energy Policy: A Fuzzy Logic Methodology. In Proceedings of the 6th International Conference on Fuzzy Systems and Knowledge Discovery (FSKD 2009), Tianjin, China, 14–16 August 2009; IEEE: Los Alamitos, CA, USA, 2009; Volume 7, pp. 128–131. [Google Scholar]
- Cammarata, A.; Sinatra, R. Condensed stiffness matrices to study vibrations in multibody systems. In Proceedings of the 8th ECCOMAS Thematic Conference on Multibody Dynamics 2017 (MBD 2017), Prague, Czech Republic, 19–22 June 2017; pp. 47–56. [Google Scholar]
- Norton, R.L. Cam Design and Manufacturing Handbook; Industrial Press Inc.: New York, NY, USA, 2002. [Google Scholar]
- Kaddissi, C.; Kenne, J.P.; Saad, M. Identification and real-time control of an electrohydraulic servo system based on nonlinear back-stepping. IEEE Trans. Mechatron. 2005, 12, 12–21. [Google Scholar] [CrossRef]
- Kalyoncu, M.; Haydim, M. Mathematical modelling and fuzzy logic based position control of an electro-hydraulic servo system with internal leakage. Mechatronics 2009, 19, 847–858. [Google Scholar] [CrossRef]
- Frosina, E.; Buono, D.; Senatore, A. A Performance Prediction Method for Pumps as Turbines (PAT) Using a Computational Fluid Dynamics (CFD) Modeling Approach. Energies 2017, 10, 103. [Google Scholar] [CrossRef]
- Rahmat, M.F.; Ling, T.G.; Husain, A.R.; Jusoff, K. Accuracy Comparison of ARX and ANFIS Model of an Electro-Hydraulic Actuator System. Int. J. Smart Sens. Intell. Syst. 2011, 4, 440–453. [Google Scholar]
- Sena, P.; Attianese, P.; Pappalardo, M.; Villecco, F. FIDELITY: Fuzzy Inferential Diagnostic Engine for on-LIne supporT to phYsicians. In Proceedings of the 4th International Conference on the Development of Biomedical Engineering in Vietnam, Ho Chi Minh City, Vietnam, 8–10 January 2012; Springer: Berlin, Germany, 2013; pp. 396–400. [Google Scholar]
- Pappalardo, C.M.; Wallin, M.; Shabana, A.A. A New Ancf/CRBF Fully Parameterized Plate Finite Element. J. Comput. Nonlinear Dyn. 2017, 12, 031008. [Google Scholar] [CrossRef]
- Fisher, A. Control Valve Handbook, 3rd ed.; Fisher Controls International Inc.: Marshalltown, IA, USA, 2001. [Google Scholar]
- Cammarata, A.; Sinatra, R. Parametric Study for the Steady-State Equilibrium of a Towfish. J. Intell. Robot. Syst. Theory Appl. 2016, 81, 231–240. [Google Scholar] [CrossRef]
- Matic, V. Design and Selection Criteria of Check Valves; Val-Matic Valve and Manufacturing Corp.: Elmhurst, IL, USA, 2011. [Google Scholar]
- Diana, S.; Iorio, B.; Giglio, V.; Police, G. The effect of Valve Lift Shape and timing on Air Motion and Mixture Formation of DISI Engines Adopting Different VVA Actuators. SAE Tech. Pap. 2001. [Google Scholar] [CrossRef]
- Casoli, P.; Bedotti, A.; Campanini, F.; Pastori, M. A Methodology Based on Cyclostationary Analysis for Fault Detection of Hydraulic Axial Piston Pumps. Energies 2018, 11, 1874. [Google Scholar] [CrossRef]
- Kulkarni, S.; Pappalardo, C.M.; Shabana, A.A. Pantograph/Catenary contact formulations. J. Vib. Acoust. Trans. ASME 2017, 139, 011010. [Google Scholar] [CrossRef]
- Zhang, J.; Tan, L. Energy Performance and Pressure Fluctuation of a Multiphase Pump with Different Gas Volume Fractions. Energies 2018, 11, 1216. [Google Scholar] [CrossRef]
- Woo, S.; Opperwall, T.; Vacca, A.; Rigosi, M. Modeling Noise Sources and Propagation in External Gear Pumps. Energies 2017, 10, 1068. [Google Scholar] [CrossRef]
- Capurso, T.; Stefanizzi, M.; Torresi, M.; Pascazio, G.; Caramia, G.; Camporeale, S.M.; Fortunato, B.; Bergamini, L. How to Improve the Performance Prediction of a Pump as Turbine by Considering the Slip Phenomenon. Proceedings 2018, 2, 683. [Google Scholar] [CrossRef]
- Iannone, V.; De Simone, M.C. Modelling of a DC Gear Motor for Feed-Forward Control Law Design for Unmanned Ground. Actuators 2018. under review. [Google Scholar]
- Formato, A.; Gallo, M.; Ianniello, D.; Montesano, D.; Naviglio, D. Supercritical Fluid Extraction of α- and β-acids from Hops Compared to Cyclically Pressurized Solid-liquid Extraction. J. Supercrit. Fluids 2013, 84, 113–120. [Google Scholar] [CrossRef]
- Azri, F.A.; Selamat, J.; Sukor, R. Electrochemical Immunosensor for the Detection of Aflatoxin B1 in Palm Kernel Cake and Feed Samples. Sensors 2017, 17, 2776. [Google Scholar] [CrossRef] [PubMed]
- Pappalardo, C.M.; Yu, Z.; Zhang, X.; Shabana, A.A. Rational ANCF Thin Plate Finite Element. J. Comput. Nonlinear Dyn. 2016, 11, 051009. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Patel, M.D.; Tinsley, B.; Shabana, A.A. Contact force control in multibody pantograph/catenary systems. Proc. Inst. Mech. Eng. Part K 2016, 230, 307–328. [Google Scholar] [CrossRef]
- Pappalardo, C.M. A natural absolute coordinate formulation for the kinematic and dynamic analysis of rigid multibody systems. Nonlinear Dyn. 2015, 81, 1841–1869. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Patel, M.; Tinsley, B.; Shabana, A.A. Pantograph/catenary contact force control. In Proceedings of the ASME Design Engineering Technical Conference, Boston, MA, USA, 2–5 August 2015; Volume 6. [Google Scholar]
- Seo, M.; Yoo, C.; Park, S.-S.; Nam, K. Development of Wheel Pressure Control Algorithm for Electronic Stability Control (ESC) System of Commercial Trucks. Sensors 2018, 18, 2317. [Google Scholar] [CrossRef] [PubMed]
- Cammarata, A. A novel method to determine position and orientation errors in clearance-affected overconstrained mechanisms. Mech. Mach. Theory 2017, 118, 247–264. [Google Scholar] [CrossRef]
- Amici, C.; Cappellini, V. Inverse Kinematics of a Serial Robot. MATEC Web Conf. 2017, 53, 01060. [Google Scholar] [CrossRef]
- De Marchis, M.; Milici, B.; Volpe, R.; Messineo, A. Energy Saving in Water Distribution Network through Pump as Turbine Generators: Economic and Environmental Analysis. Energies 2016, 9, 877. [Google Scholar] [CrossRef]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Formato, A.; Guida, D.; Ianniello, D.; Villecco, F.; Lenza, T.L.; Pellegrino, A. Design of Delivery Valve for Hydraulic Pumps. Machines 2018, 6, 44. https://doi.org/10.3390/machines6040044
Formato A, Guida D, Ianniello D, Villecco F, Lenza TL, Pellegrino A. Design of Delivery Valve for Hydraulic Pumps. Machines. 2018; 6(4):44. https://doi.org/10.3390/machines6040044
Chicago/Turabian StyleFormato, Andrea, Domenico Guida, Domenico Ianniello, Francesco Villecco, Tony Leopoldo Lenza, and Arcangelo Pellegrino. 2018. "Design of Delivery Valve for Hydraulic Pumps" Machines 6, no. 4: 44. https://doi.org/10.3390/machines6040044
APA StyleFormato, A., Guida, D., Ianniello, D., Villecco, F., Lenza, T. L., & Pellegrino, A. (2018). Design of Delivery Valve for Hydraulic Pumps. Machines, 6(4), 44. https://doi.org/10.3390/machines6040044






