Design, Analysis and Application of Control Techniques for Driving a Permanent Magnet Synchronous Motor in an Elevator System
Abstract
1. Introduction
2. PMSM in Elevator Technology
2.1. Permanent Magnet Synchronous Motor
- Efficiency. This is the main focus, as high efficiency is realized due to the magnetic field created by the magnets, so lower resistive losses and consequently lower copper losses are generated [16]. Generally, in conventional elevators we can find PMSMs with efficiencies up to 90% that are multi-pole, high speed and high torque [17]. Both the electrical and mechanical characteristics of the motor are adapted to the overall system of the elevator and the load it is required to serve. Also, PMSMs have a high-power density and a higher magnetic flux density in the gap than induction motors [18,19]. With their excellent dynamic performance, they have the capability of adjusting their speed in various operating situations. Moreover, in elevator applications, both the speed of the motor and the elevator can be varied according to the load [20].
- Energy savings. The gearless PMSMs with a transmission ratio of 1:1 present moderate mechanical losses and minimal energy and heat losses. This results in modern elevators achieving energy savings of 30–50% [21]. In addition, with the functional capabilities of the drive system, more energy can be saved by using regenerative braking. When the elevator load moves in the direction of gravity, the synchronous motors acts as a generator by returning part of the energy to the grid [22].
- Maintenance requirements. The condition monitoring of the gearbox minimizes friction, which results in heat losses and wear on the gears [23]. It is one of the main causes of breakdowns that can lead to the permanent destruction of the electric motor. In this case, maintenance costs are reduced as no additional lubricant costs are required [24].
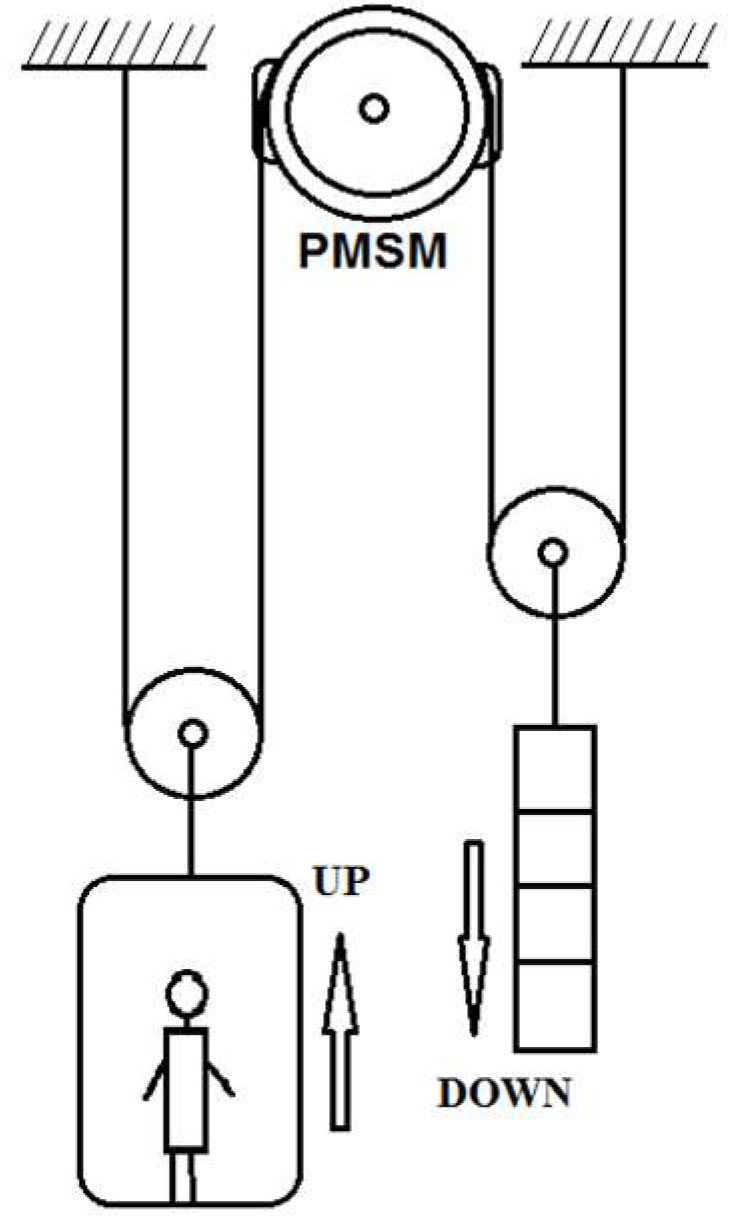
2.2. Traction Elevator System
3. Design Procedure of the Permanent Magnet Synchronous Machine
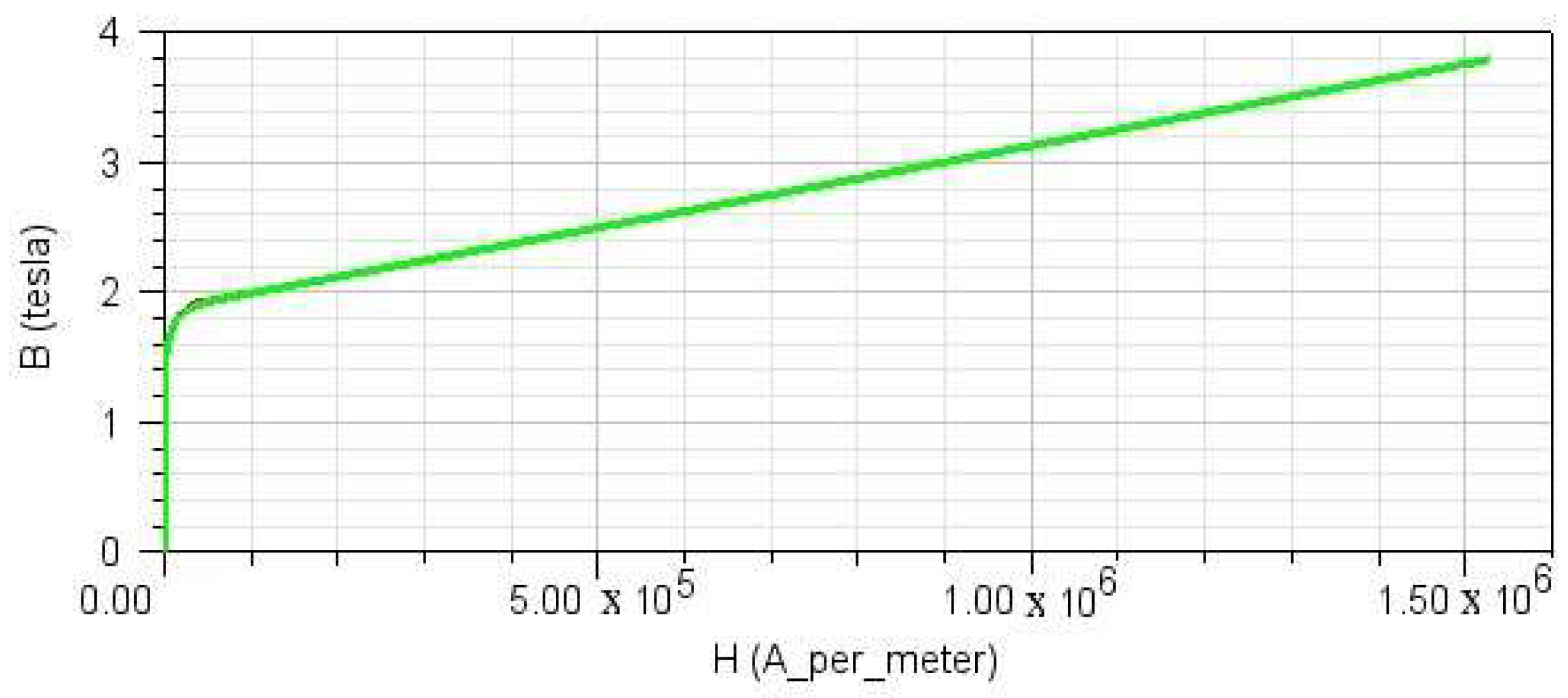
3.1. Mathematical Model Electromagnetic Analysis
3.2. Design Methodology of PMSM
4. Preliminary Design Stage of the PMSM and Experimental Validation
4.1. Description of the Experiment
4.2. Design Methodology
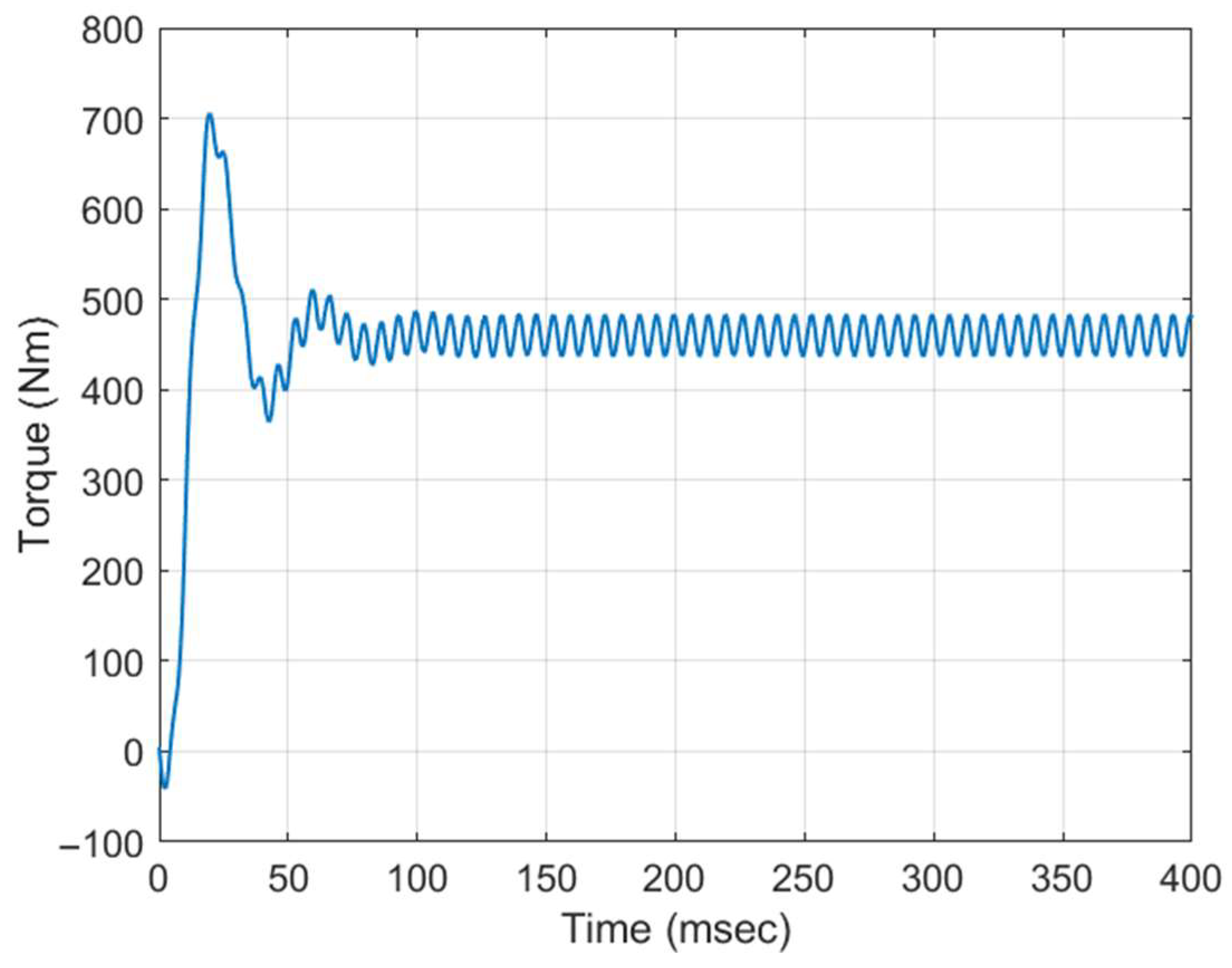
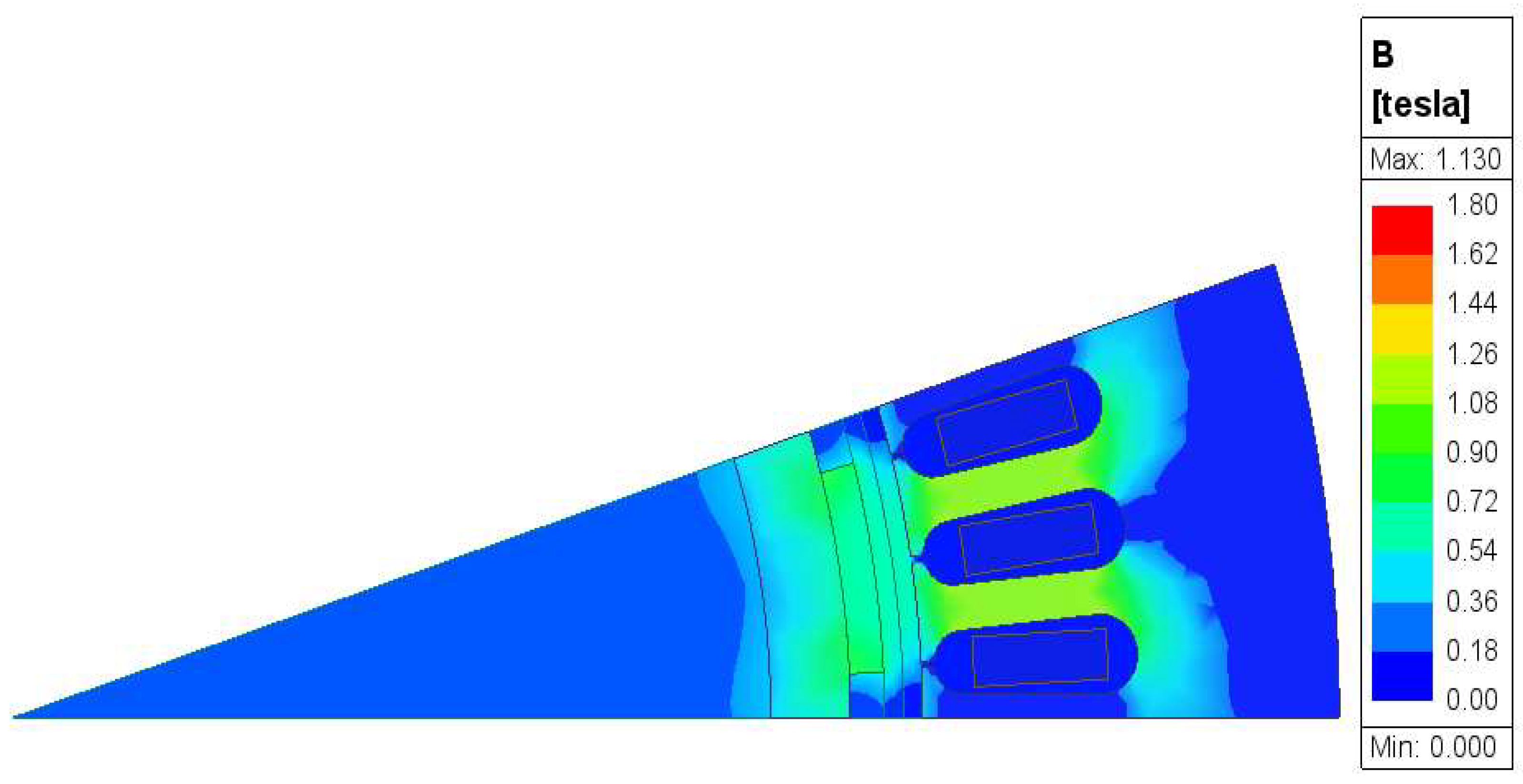
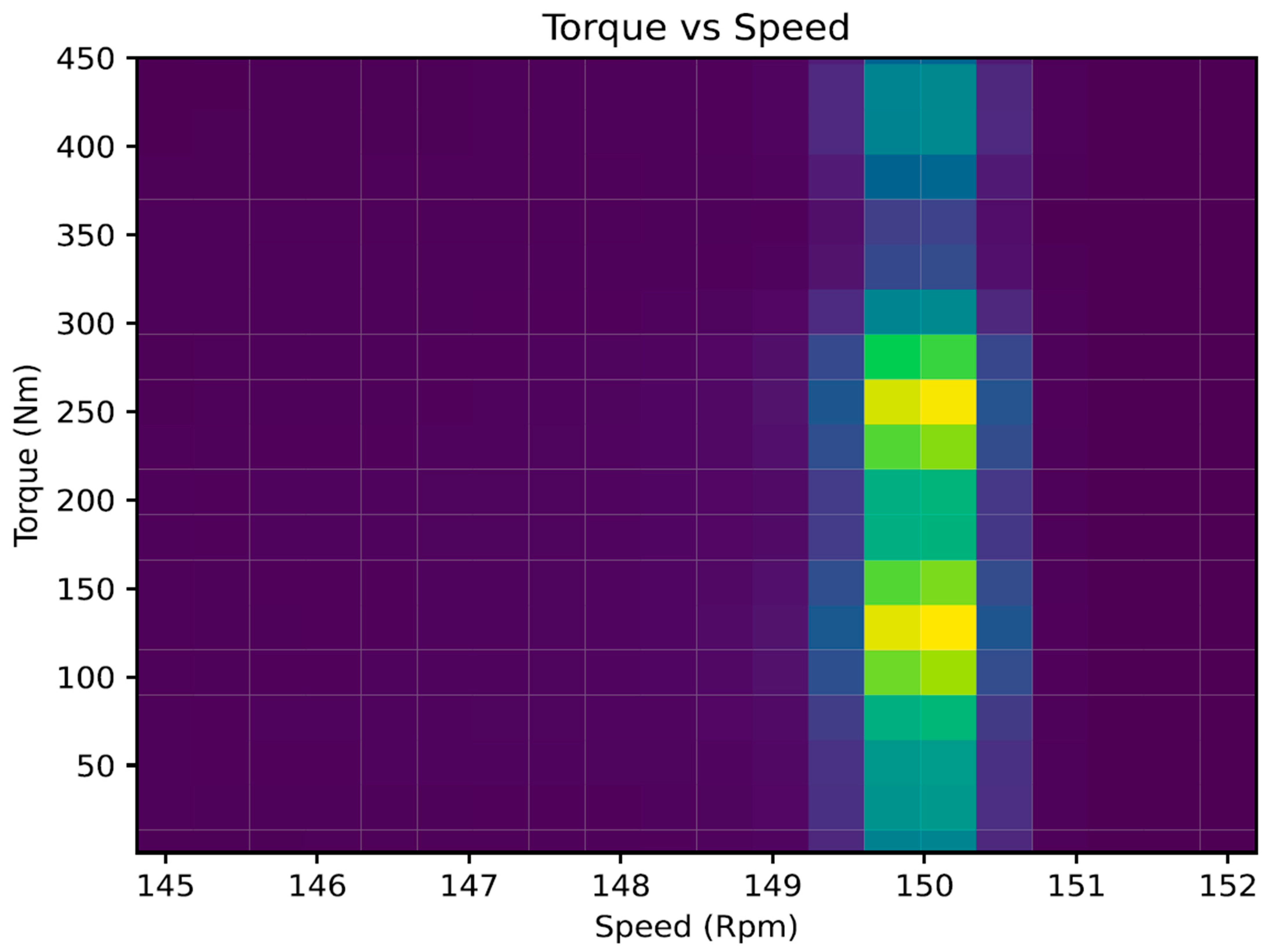
5. Two-Dimensional Finite Element Analysis
6. Application VVVF in Elevators
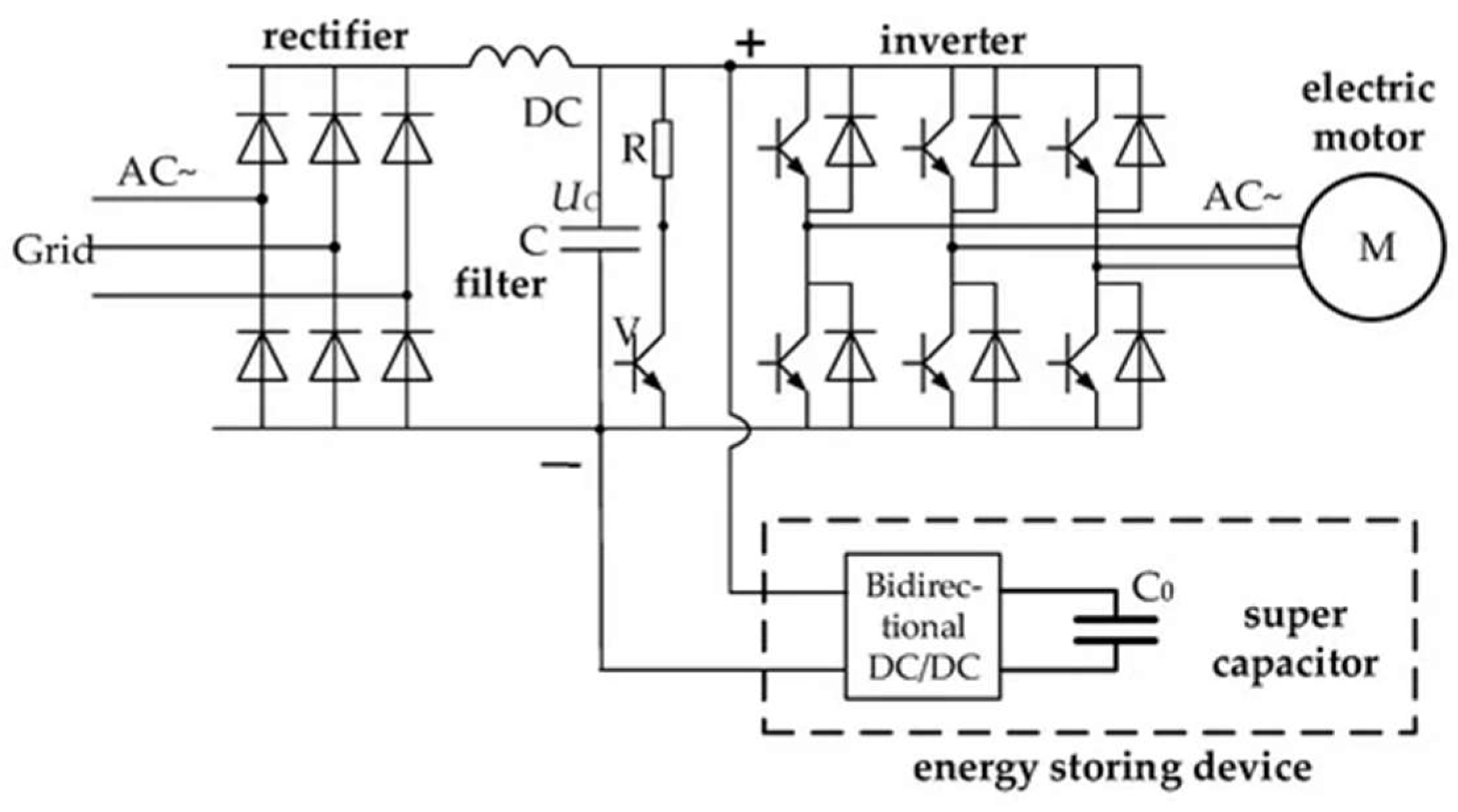
6.1. Modeling of the System
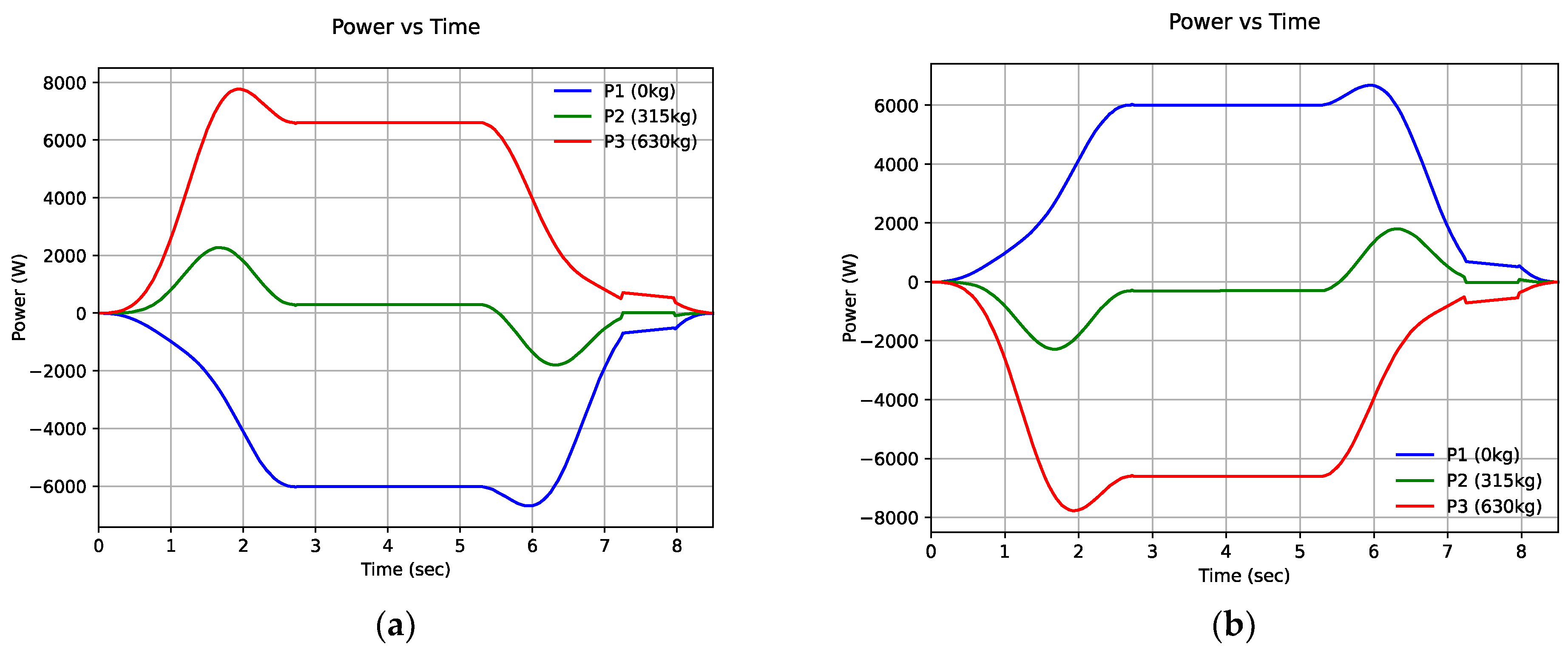
6.2. Energy Savings with the VVVF Control Technique
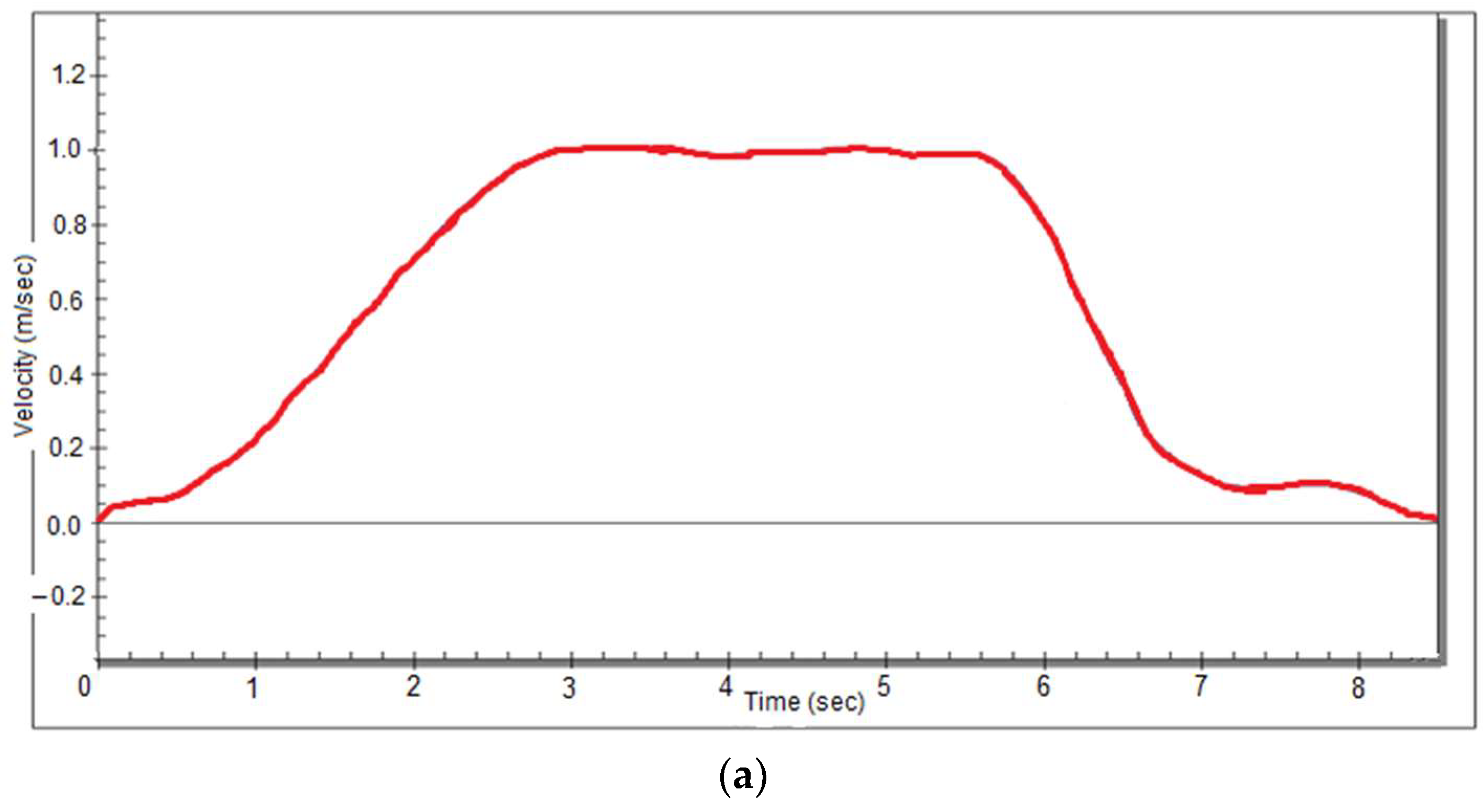
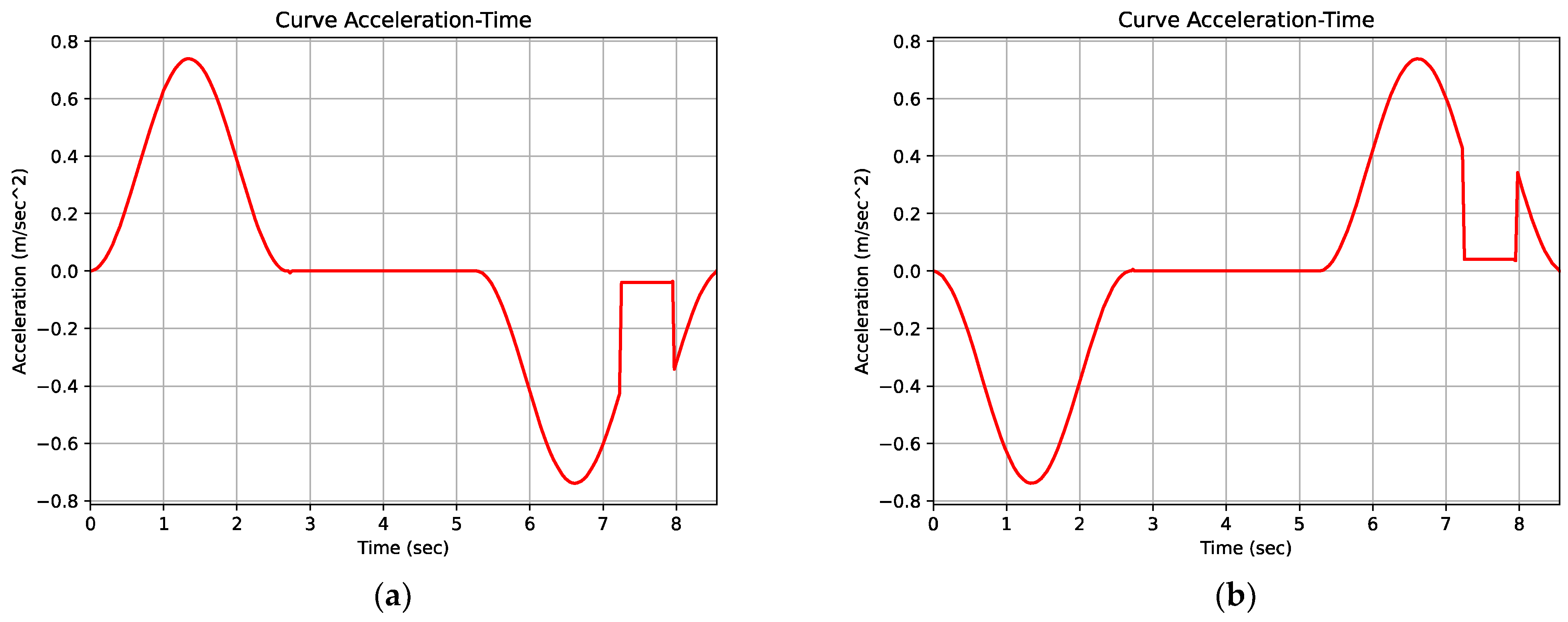
6.3. Elevator Duty Cycle Analysis
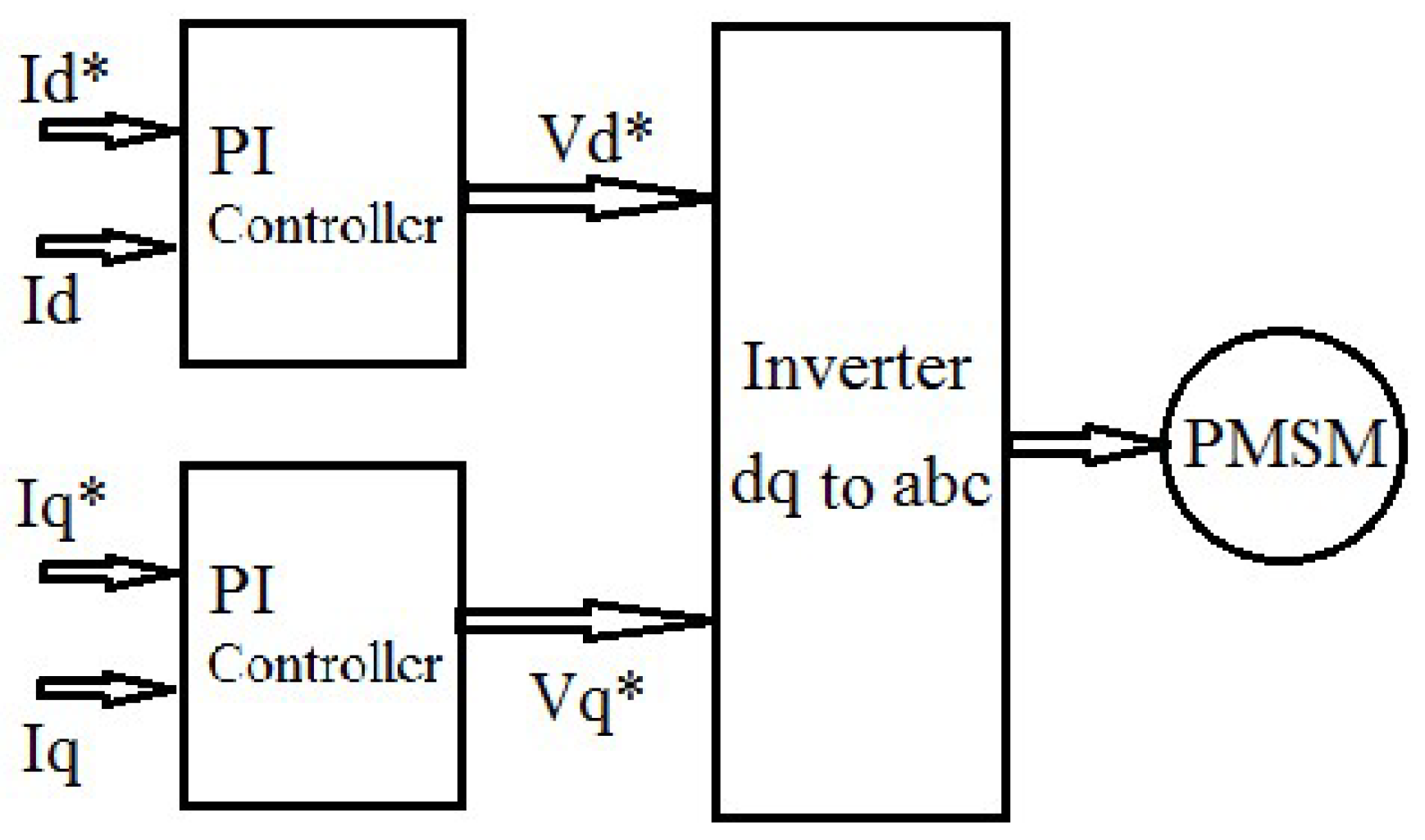
7. Control Techniques for Driving a PMSM
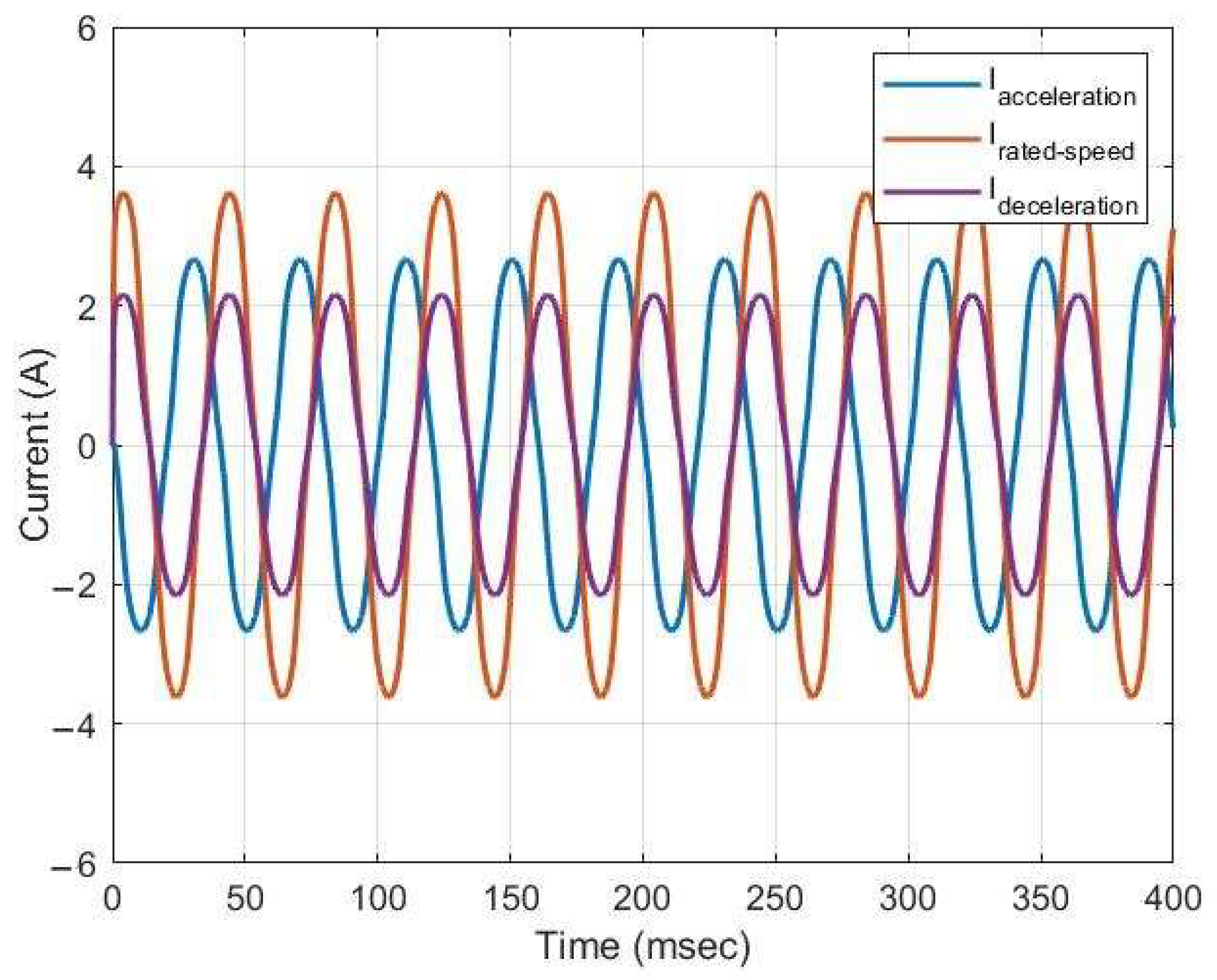
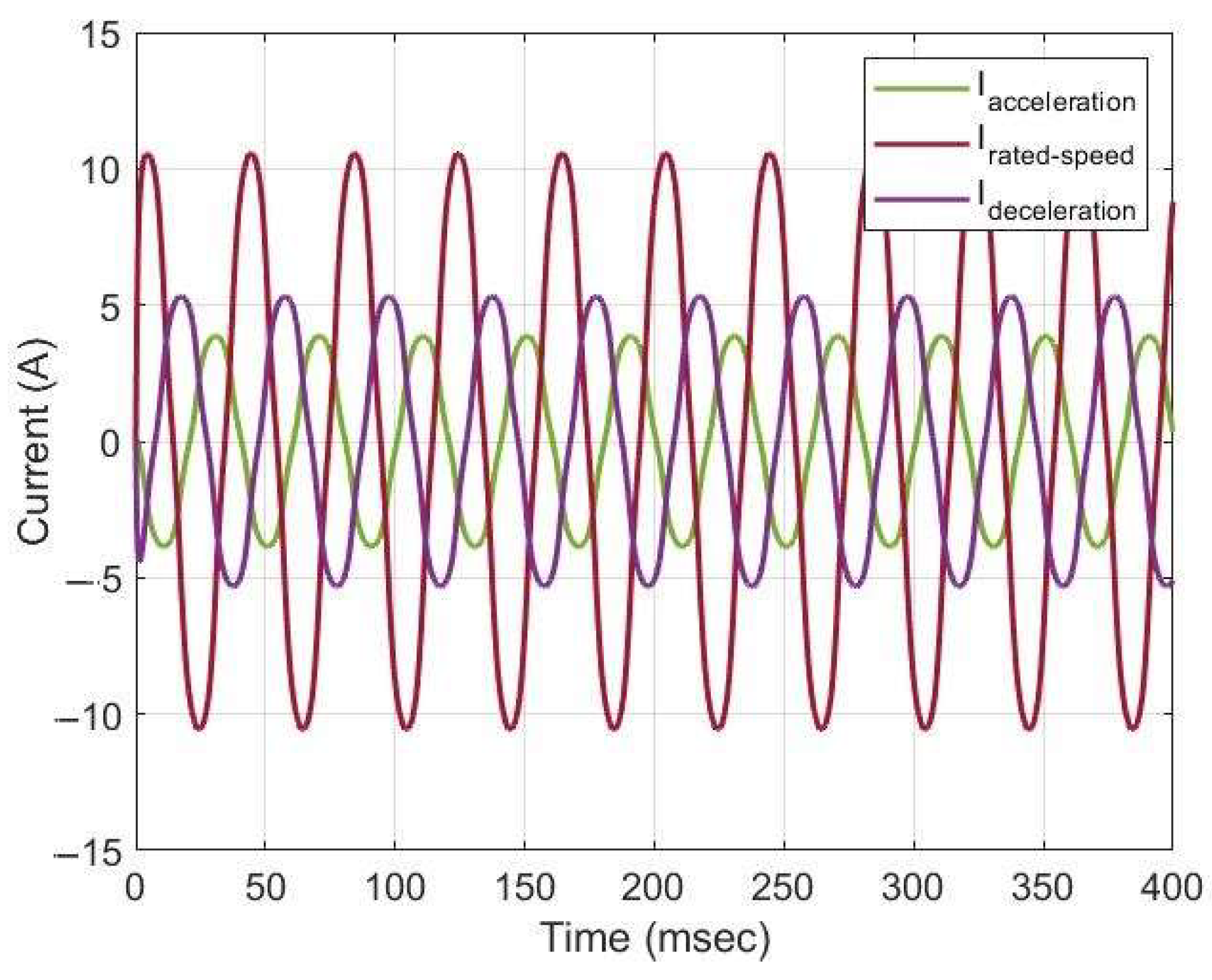
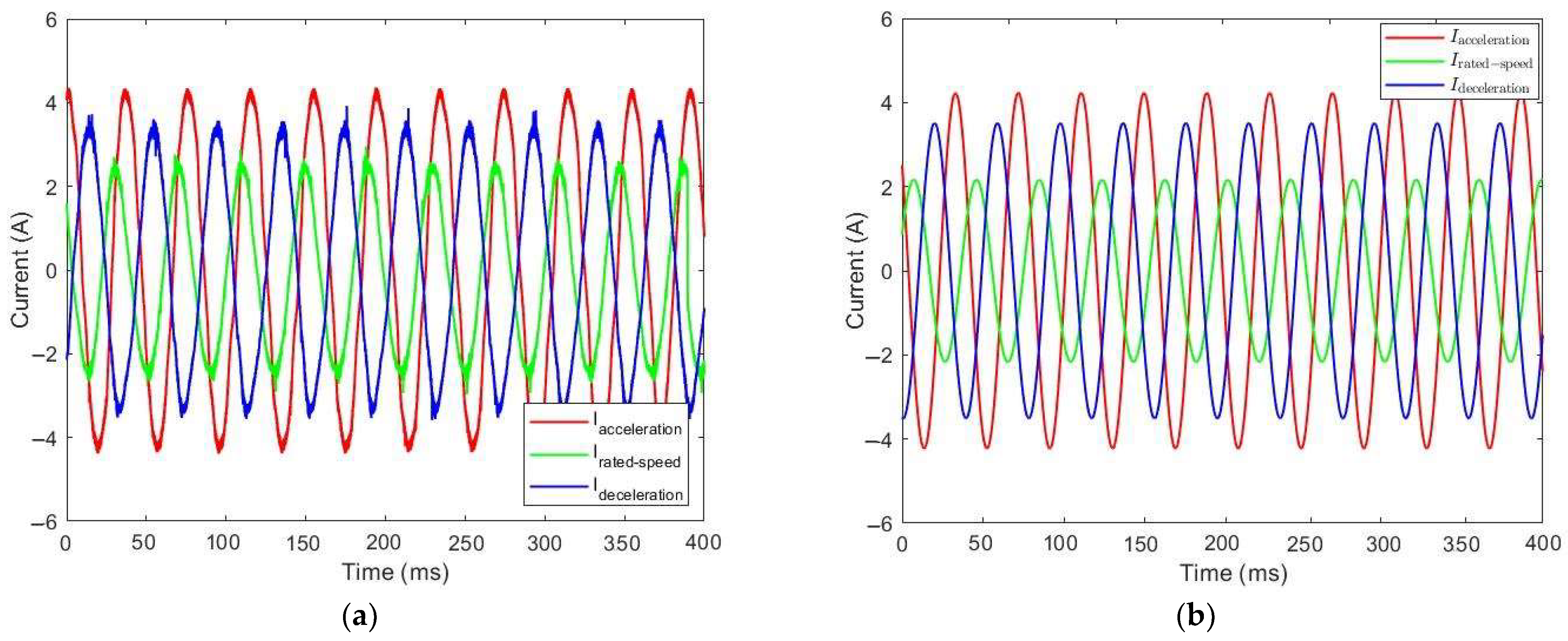
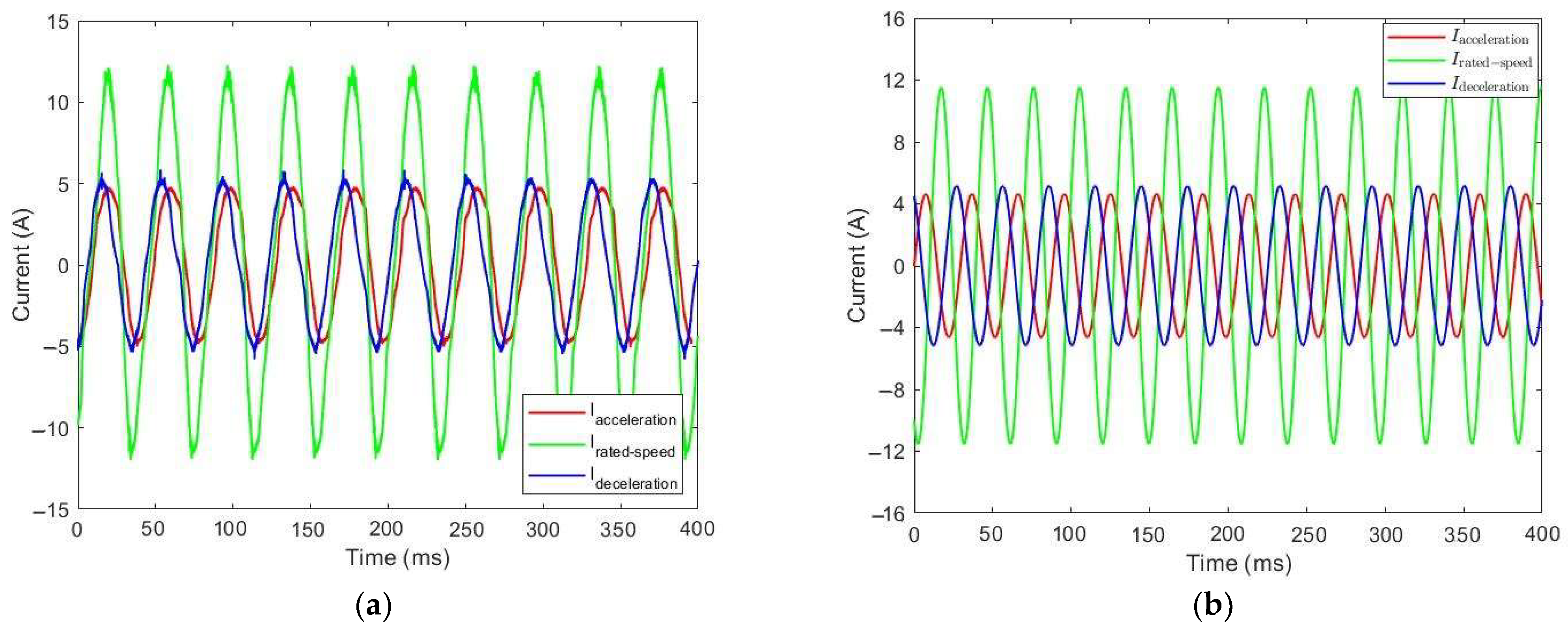
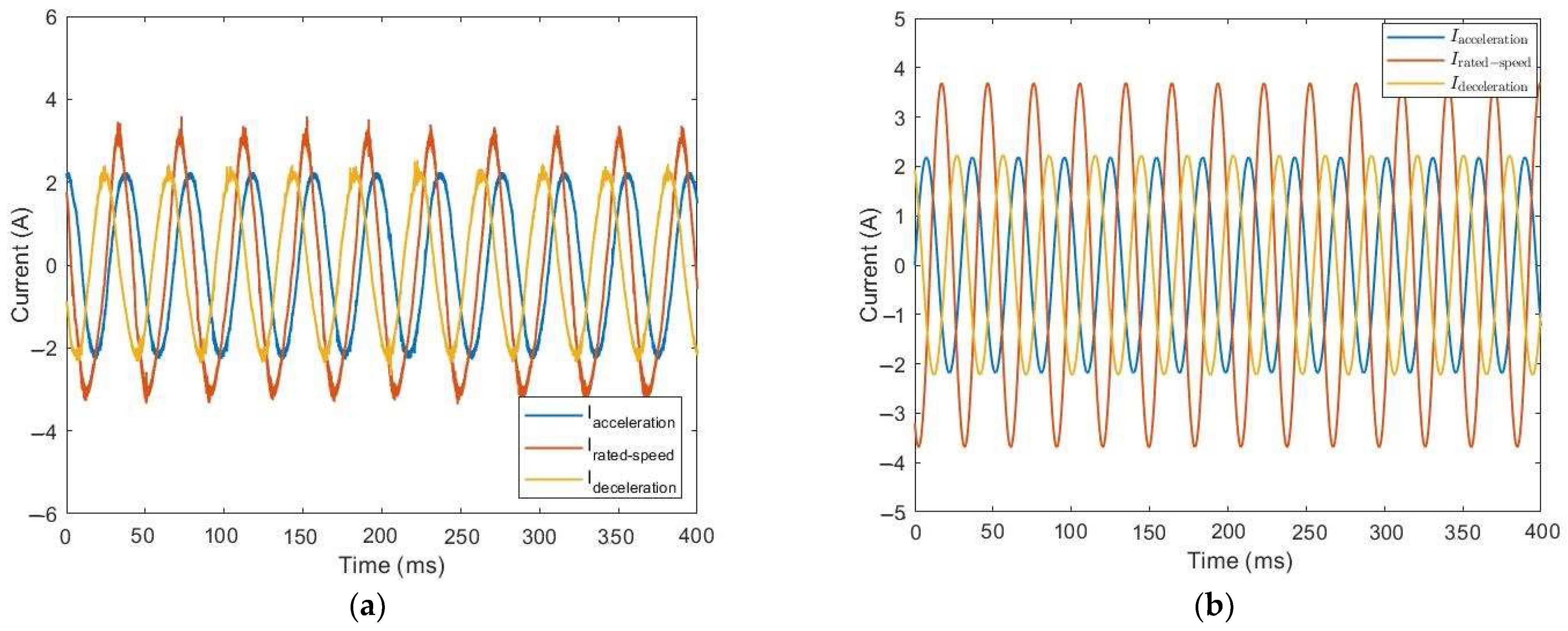
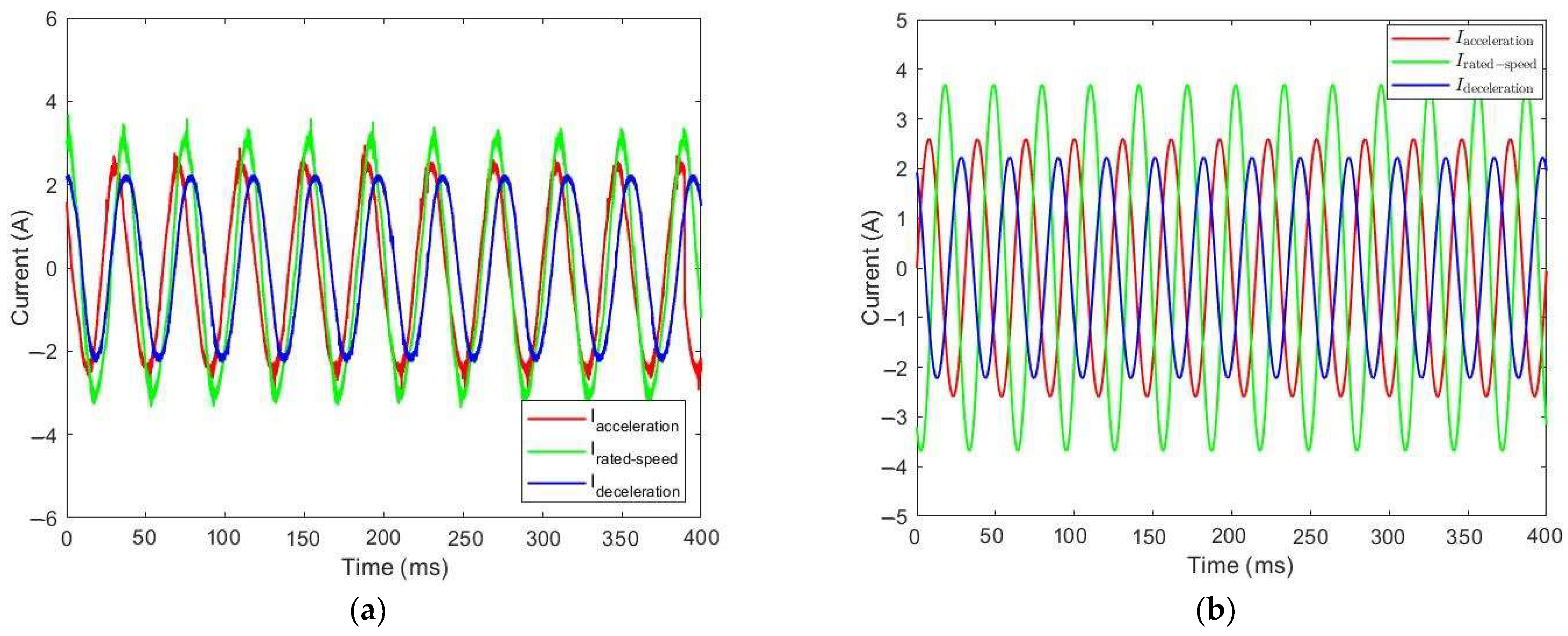
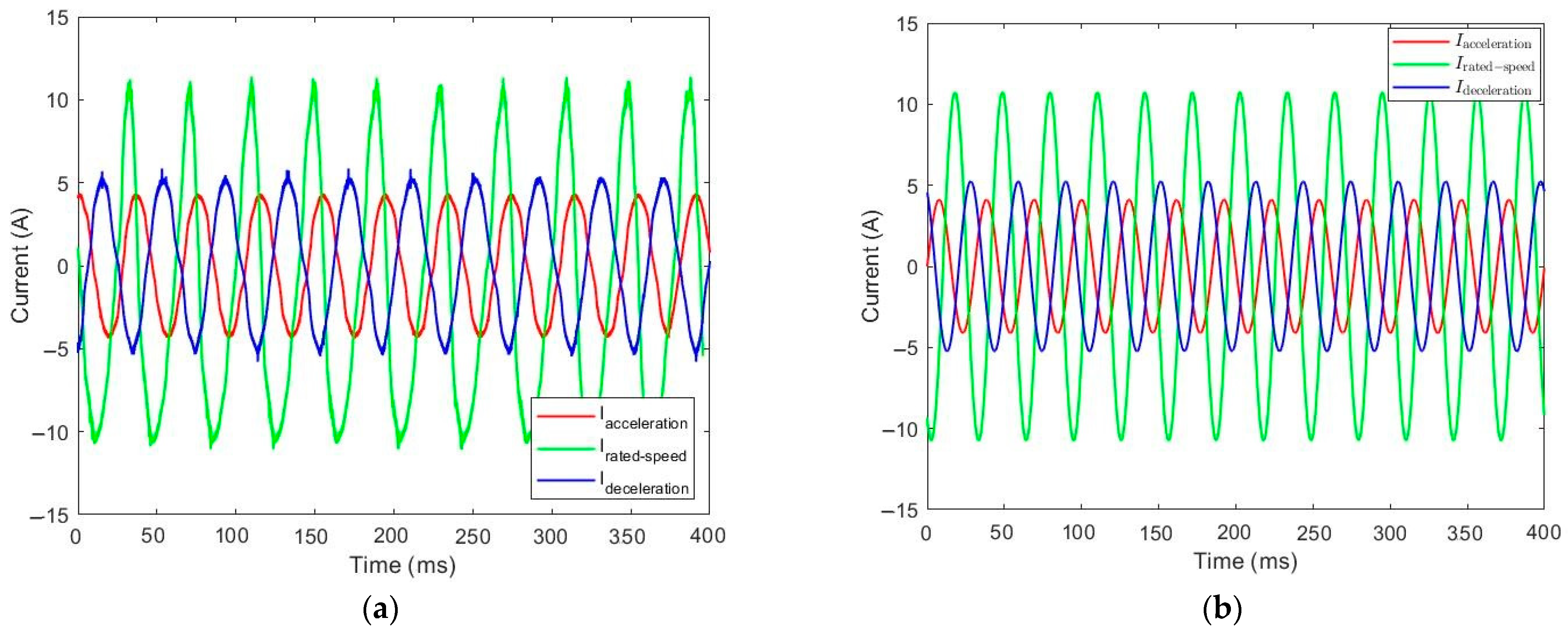
7.1. Current Consumption PMSM
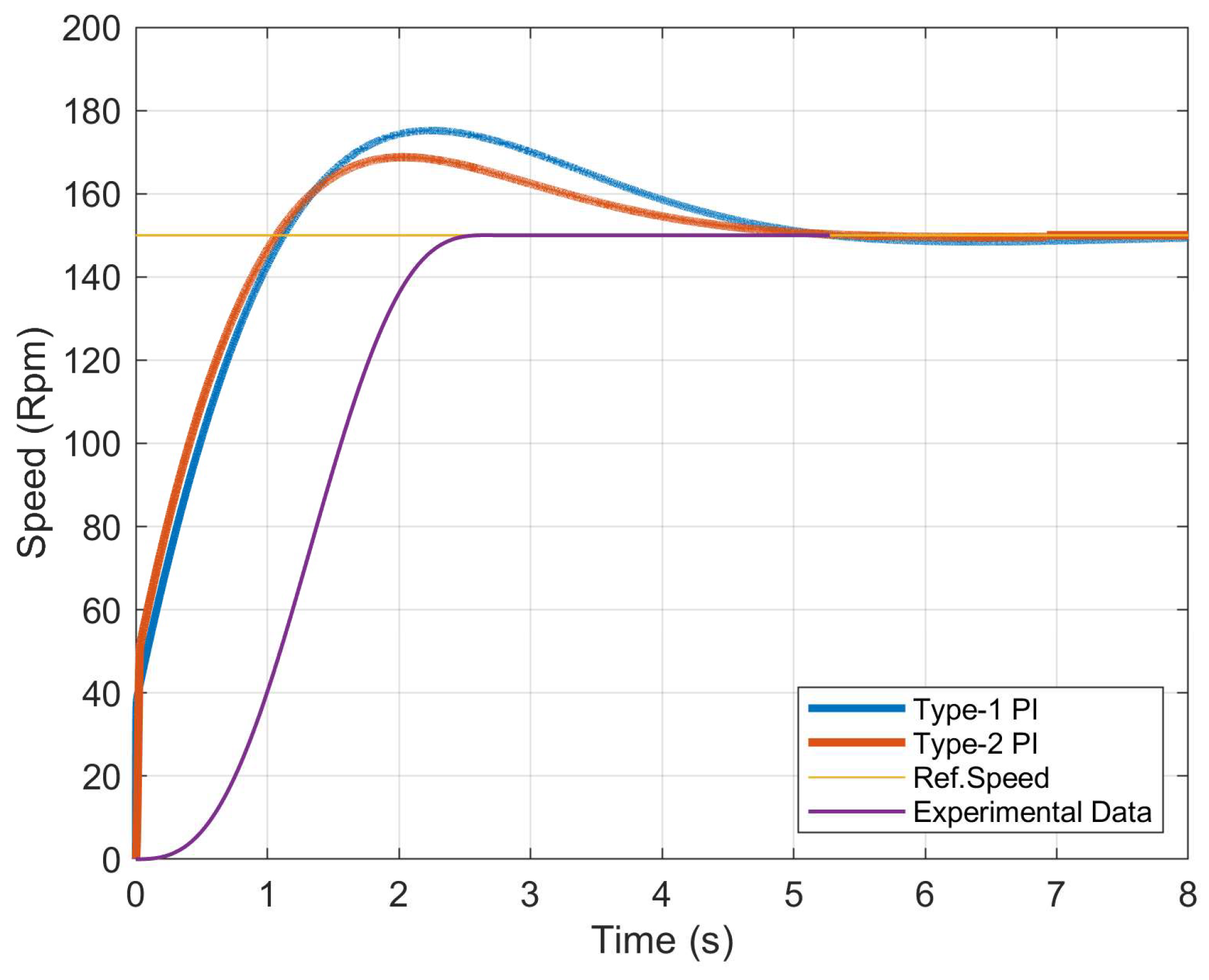
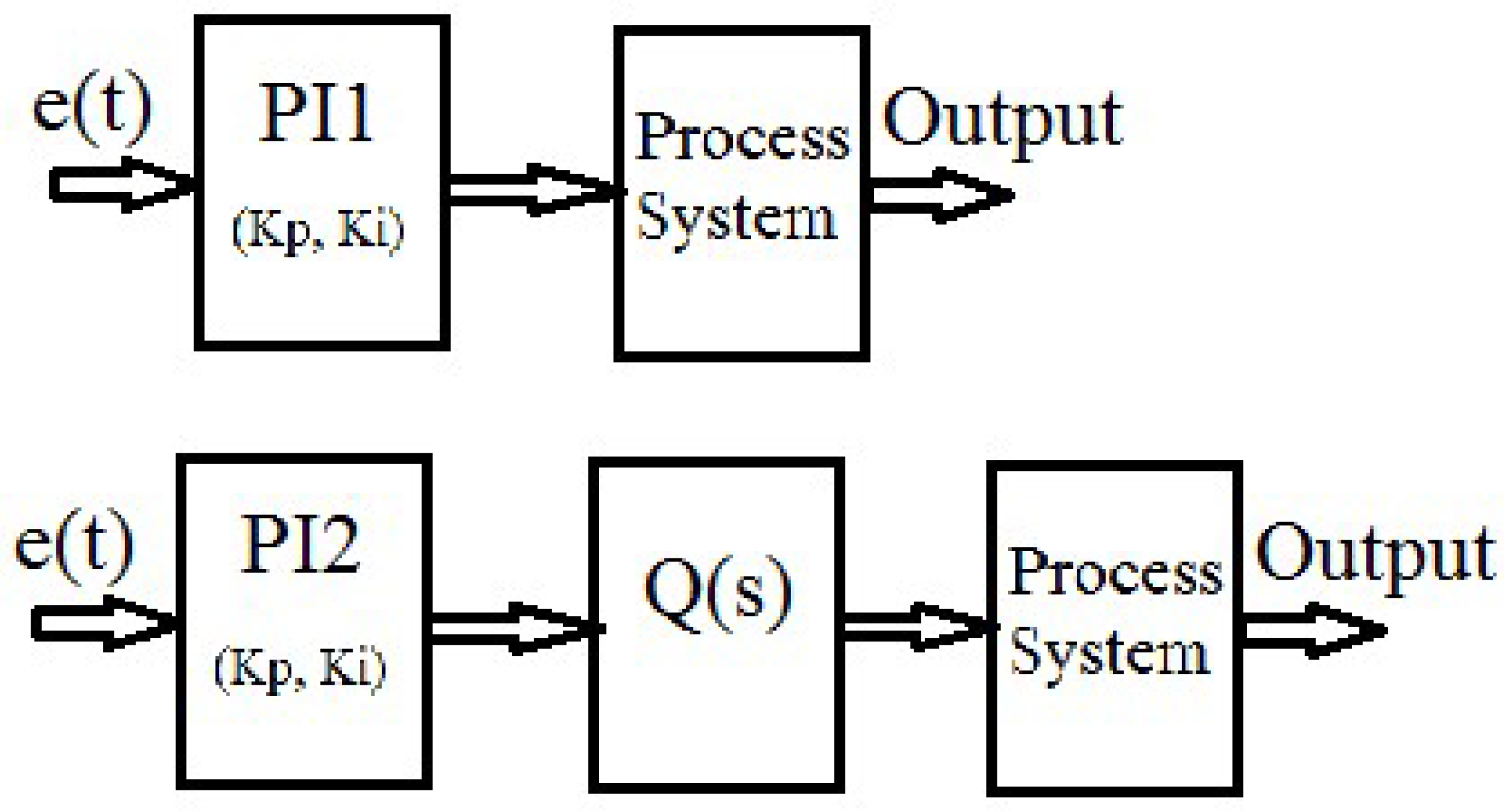
7.2. Different Speed Control Techniques for Driving PMSM
8. Discussion
- Thermal and structure analysis of the selected geometry in order to understand if there are potential thermal issues throughout the operating cycles, and especially during overloads;
- Manufacturing and geometric configuration of a multiphase motor with high efficiency fault tolerance and low losses in elevator systems;
- Use of an inverter capable of maintaining motor operation in any possible fault that may occur;
- An examination of alternative winding configurations, such as concentrated non-overlapping winding (which may reduce copper losses), and compared with the results of the current investigation;
- Comparative investigation of other control techniques in order to study speed and current control;
- Application of geometric machine optimization methods, such as using various genetic algorithms and neural networks (NN);
- Comparative energy savings study using regenerative braking, because in high-speed elevators, a back-to-back inverter voltage source is used, as an elevator driven by an electric motor requires bidirectional control of the power flow for motor operation and power generation, which occurs repeatedly during acceleration and deceleration operations;
- Application of a filter to reduce the harmonic losses of the motor resulting from the use of the inverter.
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, Y.; Ba, X.; Liu, L.; Lu, H.; Lei, G.; Yin, W.; Zhu, J. A Review of Electric Motors with Soft Magnetic Composite Cores for Electric Drives. Energies 2023, 16, 2053. [Google Scholar] [CrossRef]
- Zhang, B.; Song, Z.; Liu, S.; Huang, R.; Liu, C. Overview of Integrated Electric Motor Drives: Opportunities and Challenges. Energies 2022, 15, 8299. [Google Scholar] [CrossRef]
- Umland, N.; Winkler, K.; Inkermann, D. Multidisciplinary Design Automation of Electric Motors-Systematic Literature Review and Methodological Framework. Energies 2023, 16, 7070. [Google Scholar] [CrossRef]
- Hunt, J.D.; Nascimento, A.; Zakeri, B.; Jurasz, J.; Dabek, P.B.; Barbosa, P.S.F.; Brandao, R.; De Castro, N.J.; Filho, W.L.; Riahi, K. Lift Energy Storage Technology: A solution for decentralized urban energy storage. Energy 2022, 254, 124102. [Google Scholar] [CrossRef]
- Bakhtiarzadeh, H.; Polat, A.; Ergene, L.T. Design and analysis of a permanent magnet synchronous motor for elevator applications. In Proceedings of the International Conference on Optimization of Electrical and Electronic (OPTIM) & Intl Aegean Conference on Electrical Machines and Power Electronics (ACEMP), Brasov, Romania, 25–27 May 2017; pp. 293–298. [Google Scholar]
- Ang, J.H.; Yusup, Y.; Zaki, S.A.; Salehabadi, A.; Ahmad, M.I. Comprehensive Energy Consumption of Elevator Systems Based on Hybrid Approach of Measurements and Calculation in Low-and High-Rise Buildings of Tropical Climate towards Energy Efficiency. Sustainability 2022, 14, 4779. [Google Scholar] [CrossRef]
- Al-Sharif, L.; Tutunji, T.A.; Ragab, D.; Kayfi, R. Using Elevator system modelling and simulation for integrated learning in mechatronics engineering. In Proceedings of the International Workshop on Research and Education in Mechatronics (REM), El Gouna, Egypt, 9–11 September 2014. [Google Scholar]
- Chen, J.W.; Tran, T.N.T.; Tsai, M.F. Design on Phase Angle Control Strategy for Three-Phase-Current PMSM Elevator. In Proceedings of the 46th Annual Conference of the IEEE Industrial Electronics Society (IECON), Singapore, 18–21 October 2020; pp. 926–930. [Google Scholar]
- Wang, G.; Xu, J.; Li, T.; Zhang, G.; Zhan, H.; Ding, L.; Xu, D. Weight-Transducerlsess Starting Torque Compensation of Gearless Permanent-Magnet Traction Machine for Direct-Drive Elevators. IEEE Trans. Ind. Electr. 2014, 61, 4594–4604. [Google Scholar] [CrossRef]
- Stawinski, L.; Zakharov, V.; Kosucki, A.; Minav, T. Electromechanical Actuator-Based Solution for a Scissor Lift. Actuators 2023, 12, 394. [Google Scholar] [CrossRef]
- Ezzaldean, M.M.; Bakr, M.S. Control System for Experimental Model of Electric Elevator. J. Contr. Robot. Mechan. Syst. 2015, 1, 22–26. [Google Scholar]
- Bernard, P.; Deaconu, I.D.; Popescu, S.V.; Ghita, C.; Deaconu, A.S.; Chirila, A.I. PLC Controlled Elevator Drive System. In Proceedings of the International Symposium on Advanced Topics in Electrical Engineering (ATEE), Bucharest, Romania, 7–9 May 2015; pp. 166–169. [Google Scholar]
- Selek, M.; Terzioglu, H.; Kazan, F.A. The Design of Training Elevators for Effective Learning. In Proceedings of the International Conference on Information Science and Control Engineering, Shanghai, China, 24–26 April 2015; pp. 956–959. [Google Scholar]
- Knezevic, B.; Blabusa, B.; Marcetic, D. Design of Elevator Drive with Jerk Control. In Proceedings of the International Symposium on Power Electronics, Novi Sad, Servia, 26–28 October 2011; pp. 1–4. [Google Scholar]
- Blanusa, B.; Knezevic, B.; Erceg, B.; Lekic, O.; Mrsic, P. Elevator drives: Present trends and perspectives. In Proceedings of the International Conference on Electromechanical and Power Systems (SIELMEN), Iasi, Romania, 11–13 October 2017; pp. 001–009. [Google Scholar]
- Bakhtiarzadeh, H.; Polat, A.; Ergene, L.T. The analysis of different stator designs of a PMSM for elevator application. In Proceedings of the International Conference on Control Engineering & Information Technology (CEIT), Istanbul, Turkey, 25–27 October 2018. [Google Scholar]
- Avsar, Y.; Fenercioglu, A.; Soyaslan, M. Design Optimization of PM Synchronous Motor Used in Belt Drive Elevator Systems. In Proceedings of the International Conference on Computing, Power and Communication Technologies (GUCON), Kuala Lumpur, Malaysia, 24–26 September 2021; pp. 1–5. [Google Scholar]
- Fang, S.; Liu, H.; Wang, H.; Yang, H.; Lin, H. High Power Density Permanent Magnet Synchronous Motor With Lightweight Structure and High-Performance Soft Magnetic Alloy Core. In Proceedings of the IEEE International Conference on Applied Superconductively and Electromagnetic Devices (ASEMD), Tianjin, China, 15–18 April 2018; pp. 1–2. [Google Scholar]
- Wang, H.; Leng, J. Summary on development of permanent magnet synchronous motor. In Proceedings of the Chinese Control And Decision Conference (CCDC), Shenyang, China, 9–11 June 2018; pp. 689–693. [Google Scholar]
- Rafaq, M.S.; Jung, J.W. A Comprehensive Review of State-of-the-Art Parameter Estimation Techniques for Permanent Magnet Synchronous Motors in Wide Speed Range. IEEE Trans. Ind. Inform. 2020, 16, 4747–4758. [Google Scholar] [CrossRef]
- Avsar, Y.; Fenercioglu, A.; Soyaslan, M. Design Optimization of PM Synchronous Motor: Rail Mounted Belt Drive Elevator Systems. IEEE Trans. Ind. Appl. 2024, 60, 301–311. [Google Scholar] [CrossRef]
- Erica, D.; Godec, D.; Kutija, M.; Pravica, L.; Pavlic, I. Analysis of Regenerative Cycles and Energy Efficiency of Regenerative Elevators. In Proceedings of the International on Electrical Drives & Power Electronics (EDPE), Dubrovnik, Croatia, 22–24 September 2021; pp. 212–219. [Google Scholar]
- Sawna, J.; Seferian, V.; Khatounian, F.; Monmasson, E.; Ghosn, R. An improved anti-rollback control algorithm for gearless traction motor in elevator applications. Mechatronics 2021, 79, 102659. [Google Scholar] [CrossRef]
- Anand, R.; Mahesh, M. Analysis of elevator drives energy consumptions with permanent magnet machines. In Proceedings of the IEEE Smart Energy Grid Engineering (SEGE), Oshawa, ON, Canada, 21–24 September 2016; pp. 186–190. [Google Scholar]
- Yetis, H.; Mese, E.; Bayikli, M. Design and Comparison of Ferrite Based IPM and NdFeB Based SPM Synchronous Motors for Gearless Elevator Systems. In Proceedings of the International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 635–641. [Google Scholar]
- Vagati, A.; Boazzo, B.; Guglielmi, P.; Pellegrino, G. Design of Ferrite-Assisted Synchronous Reluctance Machines Robust toward Demagnetization. IEEE Trans. Ind. Appl. 2014, 50, 1768–1779. [Google Scholar] [CrossRef]
- Sim, J.H.; Ahn, D.G.; Kim, D.K.; Son, D.K.; Kim, S.; Hong, J.P.; Lee, T.H. Multiphysics Design of Triple 3-Phase PMSM for Ultra-High Speed Elevator Applications. In Proceedings of the International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 284–290. [Google Scholar]
- Petrov, I.; Pyrhonen, J. Performance of Low-Cost Permanent Magnet Material in PM Synchronous Machines. IEEE Trans. Ind. Electron. 2013, 60, 2131–2138. [Google Scholar] [CrossRef]
- Vlachou, V.I.; Sakkas, G.K.; Xintaropoulos, F.P.; Pechlivanidou, M.S.C.; Kefalas, T.D.; Tsili, M.A.; Kladas, A.G. Overview on Permanent Magnet Motor Trends and Developments. Energies 2024, 17, 538. [Google Scholar] [CrossRef]
- Laskaris, K.I.; Kladas, A.G. Internal Permanent Magnet Motor Design for Electric Vehicle Drive. IEEE Trans. Ind. Electron. 2009, 57, 138–145. [Google Scholar] [CrossRef]
- Mohammed, J.A.; Shuraiji, A.L. Modelling of DC Elevator Motor Drive for Mid-rise Building. Eng. Technol. J. 2013, 31, 2320–2342. [Google Scholar] [CrossRef]
- Tukia, T.; Uimonen, S.; Siikonen, M.L.; Hakala, H.; Lehtonen, M. A study for improving the energy efficiency of lifts with adjustable counterweight. Build. Serv. Eng. Res. Technol. 2017, 38, 421–435. [Google Scholar] [CrossRef]
- Karakatsanis, T.S. Modeling of elevators as Driving systems. In Proceedings of the International Association of Elevator Engineering (IAEE), Thessaloniki, Greece, 11–13 June 2008. [Google Scholar]
- Yetis, H.; Boztepeli, H.; Yasa, Y.; Mese, E. Comparative design of direct drive PM synchronous motors in gearless elevator systems. In Proceedings of the International Conference on Electric Power and Energy Conversion Systems, Istanbul, Turkey, 2–4 October 2013. [Google Scholar]
- Ford, P.J.; Amiri, E.; Mendrela, E. Electric elevator drive with position control. Electr. Eng. 2016, 98, 309–319. [Google Scholar] [CrossRef][Green Version]
- Yetis, H.; Mese, E. Design of IPM Synchronous Motor for Gearless Elevator Applications. In Proceedings of the International Conference on Engineering Technology an Innovation (ICETI), Sarajevo, Bosnia and Herzegovina, 23–26 March 2017; pp. 1–6. [Google Scholar]
- Kannojia, P.; Chinmaya, K.A. Comparative Review and Finite Element Analysis of Energy Efficient Motors. In Proceedings of the International Conference on Power Electronics and Energy (ICPEE), Bhubaneswar, India, 3–5 January 2023. [Google Scholar]
- Yan, B.; Li, X.; Wang, X.; Yang, Y.; Chen, D. An Improved 2-D Subdomain Method toward Electromagnetic-Performance Analysis of Line-Start Permanent Magnet Synchronous Motor. IEEE Trans. Transp. Electr. 2023, 57, 4339–4351. [Google Scholar] [CrossRef]
- Chadebec, O.; Bui, V.P.; Granjon, P.; Rouve, L.L.; Bihan, N.L.; Coulomb, J.L. Rotor fault detection of electrical machines by low frequency magnetic stray field analysis. In Proceedings of the International Symposium on Diagnosis for Electric Machines, Power Electronics and Drives, Vienna, Austria, 7–9 September 2005. [Google Scholar]
- Lehikoinen, A.; Arkkio, A.; Belahcen, A. Reduced Basis Finite Element Modeling of Electrical Machines with Multiconductor Windings. IEEE Trans. Ind. Appl. 2017, 53, 4252–4259. [Google Scholar] [CrossRef]
- Pechlivanidou, M.S.C.; Chasiotis, I.D.; Karnavas, Y.L. A comparative study on 2D and 3D magnetic field analysis of permanent magnet synchronous motor using FEM simulations. J. Electromagn. Waves Appl. 2019, 33, 2215–2241. [Google Scholar] [CrossRef]
- Mitolo, M.; Araneo, R. A Brief History of Maxwell’s Equations. IEEE Ind. Appl. Mag. 2019, 25, 8–13. [Google Scholar] [CrossRef]
- Bozek, P.; Krenicky, T.; Prajova, V. Digital Induction Motor Model Based on the Finite Element Method. Appl. Sci. 2023, 13, 5124. [Google Scholar] [CrossRef]
- Cui, D.; Max, L.; Bostrom, C.; Ekergard, B. Design of Spoke Type Traction Motor with Ferrite Material for EV Application. In Proceedings of the International Conference on Electrical Machines (ICEM), Valencia, Spain, 5–8 September 2022; pp. 315–320. [Google Scholar]
- Pechlivanidou, M.S.C.; Kladas, A.G. Three-Dimensional-Printed Magnetic Iron Material Modeling for High Speed Actuators. IEEE Trans. Magn. 2023, 59, 8001305. [Google Scholar] [CrossRef]
- Stoev, B.; Todorov, G.; Rizov, P.; Pagiatakis, G.; Dritsas, L. Finite element analysis of rotating electrical machines-An educational approach. In Proceedings of the Global Engineering Education Conference (EDUCON), Athens, Greece, 25–28 April 2017; pp. 262–269. [Google Scholar]
- Orlowska-Kowalska, T.; Wolkiewicz, M.; Pietrzak, P.; Skowron, M.; Ewert, P.; Tarchala, G.; Krzysztofiak, M.; Kowalski, C.T. Fault Diagnosis and Fault-Tolerant Control of PMSM Drives-State of the Art and Future Challenges. IEEE Access 2022, 10, 59979–60024. [Google Scholar] [CrossRef]
- Jung, E.; Yoo, H.; Sul, S.K.; Choi, H.S.; Choi, Y.Y. A Nine-Phase Permanent-Magnet Motor Drive System for an Ultrahigh-Speed Elevator. IEEE Trans. Ind. Appl. 2012, 48, 987–995. [Google Scholar] [CrossRef]
- Kumar, P.; Kumar, R.; Kumar, B.; Kumar, A. Design of a High Performance PMSM for Electric Vehicles. In Proceedings of the International Conference on Emerging Frontiers in Electrical and Electronic Technologies (ICEFEET), Patna, India, 24–25 June 2022. [Google Scholar]
- Chin, J.W.; Cha, K.S.; Park, M.R.; Park, S.H.; Lee, E.C.; Lim, M.S. High Efficiency PMSM with High Slot Fill Factor Coil for Heavy-Duty EV Traction Considering AC Resistance. IEEE Trans. Energy Convers. 2021, 36, 883–894. [Google Scholar] [CrossRef]
- Kakoudakis, I.D.; Karamanis, E.K.; Kikidis, I.I.; Kladas, A.G. Comparison of Permanent Magnet Motor Configurations for Electric Vehicle Applications. In Proceedings of the International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 2058–2064. [Google Scholar]
- Jin, Y.X.; Wang, A.Y.; Wang, T.; Sun, J.; Wang, M.X. Optimal Design of Loss of Permanent Magnet Synchronous Motor Based on Particle Swarm Optimization. In Proceedings of the IEEE Student Conference on Electric Machines and Systems, Huzhou, China, 14–16 September 2018. [Google Scholar]
- Al-Timimy, A.; Giangrande, P.; Degano, M.; Galea, M.; Gerada, C. Investigation of AC Copper and Iron Losses in High-Speed High-Power Density PMSM. In Proceedings of the International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 263–269. [Google Scholar]
- Jun, H.W.; Lee, J.W.; Yoon, G.H.; Lee, J. Optimal Design of the PMSM Retaining Plate with 3-D Barrier Structure and Eddy-Current Loss-Reduction Effect. IEEE Trans. Ind. Electron. 2018, 65, 1808–1818. [Google Scholar] [CrossRef]
- Liang, Y.; Zhao, F.; Xu, K.; Wang, W.; Liu, J.; Yang, P. Analysis of Copper Loss of Permanent Magnet Synchronous Motor with Formed Transportation Winding. IEEE Access 2021, 9, 101105–101114. [Google Scholar] [CrossRef]
- Wei, D.; He, H.; Li, J. A Computationally Efficiency Optimal Design for a Permanent Magnet Synchronous Motor in Hybrid Electric Vehicles. In Proceedings of the International Power Electronics and Motion Control Conference (IPEMC2020-ECCE Asia), Nanjing, China, 20 November–2 December 2020; pp. 43–47. [Google Scholar]
- Pechlivanidou, M.S.C.; Kladas, A.G. Winding Structure Impact On High Speed Permanent Magnet Motor Efficiency. In Proceedings of the International Conference on Electrical Machines (ICEM), Valencia, Spain, 5–8 September 2022; pp. 2283–2289. [Google Scholar]
- Sakkas, G.K.; Vasilopoulos, C.R.C.; Bourchas, K.G.; Kladas, A.G. Advanced Design of PWM Inverter Fed Permanent Magnet Motors. IEEE Trans. Magn. 2023, 59, 8204711. [Google Scholar] [CrossRef]
- Babayomi, O.; Balogun, A.; Osheku, C. Loss Minimizing Control of PMSM for Electric Power Steering. In Proceedings of the International Conference on Modelling and Simulation (UKSim), Cambridge, UK, 25–27 March 2015; pp. 438–443. [Google Scholar]
- Chen, Y.; Liang, S.; Li, W.; Liang, H.; Wang, C. Faults and Diagnosis Methods of Permanent Magnet Synchronous Motors: A Review. Appl. Sci. 2019, 9, 2116. [Google Scholar] [CrossRef]
- Park, S.H.; Lee, E.C.; Park, J.C.; Hwang, S.W.; Lim, M.S. Prediction of Mechanical Loss for High-Power-Density PMSM Considering Eddy Current Loss of PMs and Conductors. IEEE Trans. Magn. 2021, 57, 6300205. [Google Scholar] [CrossRef]
- De Almeida, A.; Hirzel, S.; Patrao, C.; Fong, J.; Dutschke, E. Energy-efficient elevators and escalators in Europe: An analysis of energy efficiency potentials and policy measures. Energy Build. 2012, 47, 151–158. [Google Scholar] [CrossRef]
- Thu, M.M.; Naing, T.L. α-β Reference Frame Modeling and Implementation of VVVF Three-Phase Inverter for Induction Motor Drive. In Proceedings of the 12th National Conference on Science and Engineering (NCSE), Mandalay, Myanmar, 21 June 2019; pp. 371–376. [Google Scholar]
- Ambhorkar, K.; Rana, A.K.; Tutakne, D.R. Enhancing Power Factor and Efficiency of Three Phase Induction Motor Drive using Single Main PWM Switch and Three Auxiliary Switches. In Proceedings of the International Conference on Power Electronics, Drives and Energy Systems (PEDES), Chennai, India, 18–21 December 2018. [Google Scholar]
- Vlachou, V.I.; Karakatsanis, T.S.; Kladas, A.G. Energy Savings in Elevators by Using a Particular Permanent-Magnet Motor Drive. Energies 2023, 16, 4716. [Google Scholar] [CrossRef]
- Marsong, S.; Plangklang, B. Implementation analysis of an elevator energy regenerative unit (EERU) for energy savings in a building. In Proceedings of the International Conference on Electrical Engineering/Electronics, Computer, Telecommunications and Information Technology (ECTI-CON), Chiang Mai, Thailand, 28 June–1 July 2016. [Google Scholar]
- Karlis, A.D. Energy consumption estimation on lift systems: The advantages of VVVF drives. In Proceedings of the International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 751–755. [Google Scholar]
- Wu, C.Z. Design of Electrical Control System Elevator Based on VVVF. In Proceedings of the International Conference on Computational Intelligence and Communication Networks (CICN), Jabalpur, India, 12–14 December 2015; pp. 1490–1492. [Google Scholar]
- Vlachou, V.I.; Karakatsanis, T.S.; Kladas, A.G. Energy savings in elevators by using a particular permanent magnet motor drive. In Proceedings of the Mediterranean Conference on Power Generation, Transmission, Distribution and Energy Conversion (MEDPOWER 2022), Valetta, Malta, 7–9 November 2022; pp. 273–278. [Google Scholar] [CrossRef]
- Ma, R.; Zhu, J.; Lin, Q.; Zhang, Y. Influence of Winding Distribution on Fault Tolerant Performance in a Fault-Tolerant Permanent Magnet Rim Driven Motor. IEEE Access 2019, 7, 183236–183244. [Google Scholar] [CrossRef]
- Ullah, K.; Guzinski, J.; Mirza, A.F. Critical Review on Robust Speed Control Techniques for Permanent Magnet Synchronous Motor (PMSM) Speed Regulation. Energies 2022, 15, 1235. [Google Scholar] [CrossRef]
- Rangarajan, S.; Agarwal, V. Novel Active Damping Scheme to Stabilize and Enhance the Performance of v/f Controlled Permanent Magnet Synchronous Motor Driven Passenger Elevator System. In Proceedings of the Industry Applications Society Annual Meeting (IAS), Detroit, MI, USA, 9–14 November 2022; pp. 1–7. [Google Scholar]
- Zhang, X.; Xie, X.; Yao, R. Field oriented control for permanent magnet synchronous motor based on DSP experimental platform. In Proceedings of the 27th Chinese Control and Decision Conference (2015 CCDC), Qingdao, China, 23–25 May 2015; pp. 1870–1875. [Google Scholar]


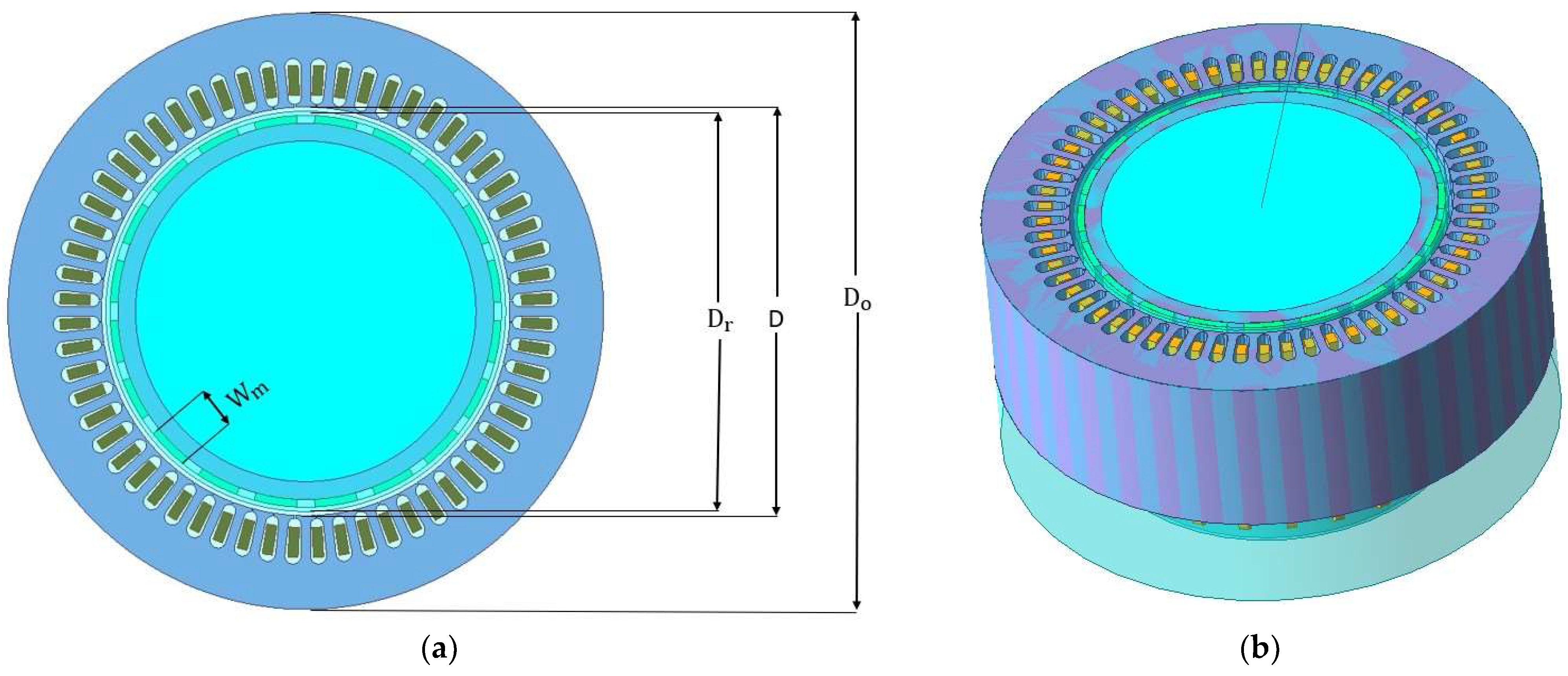

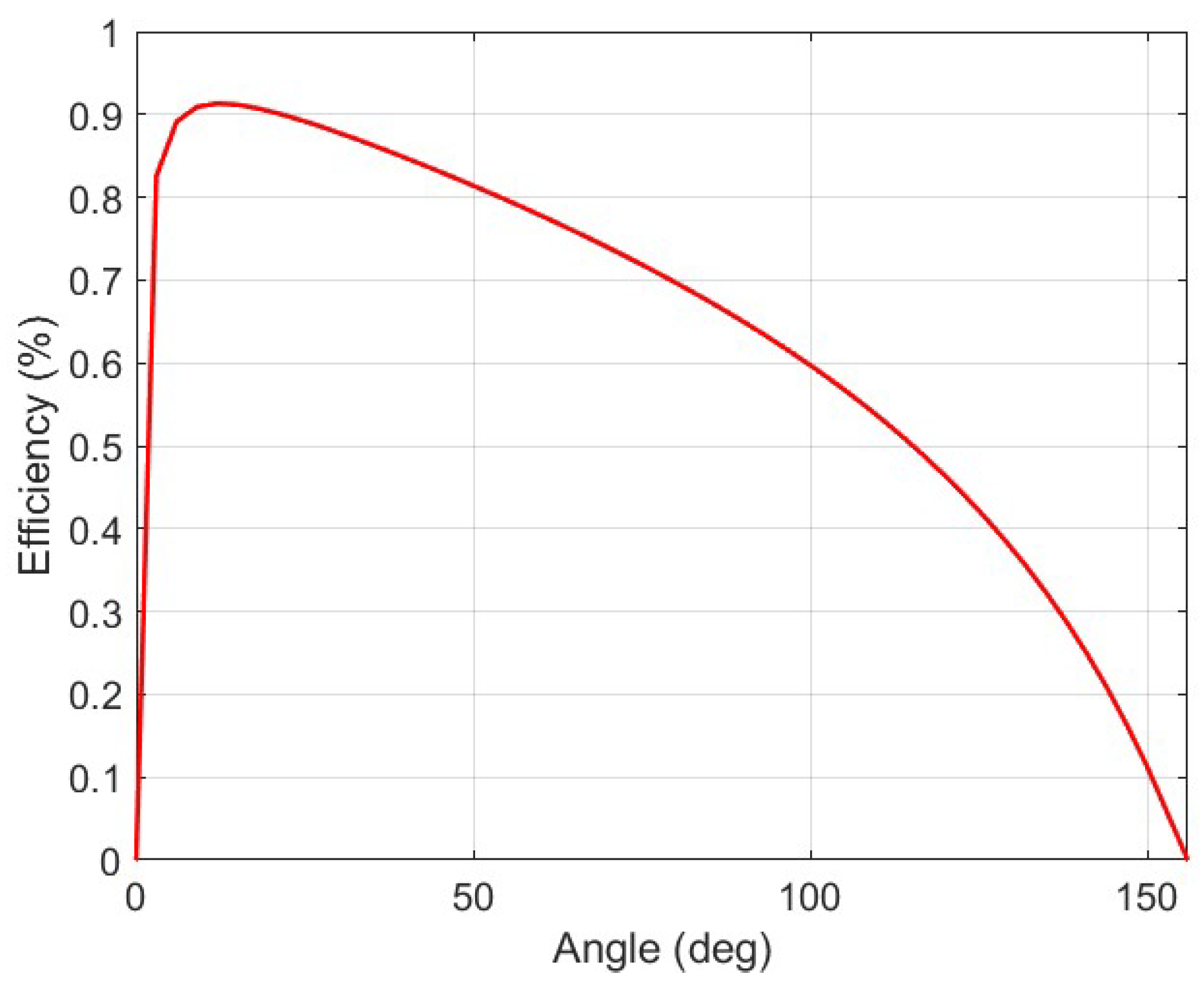
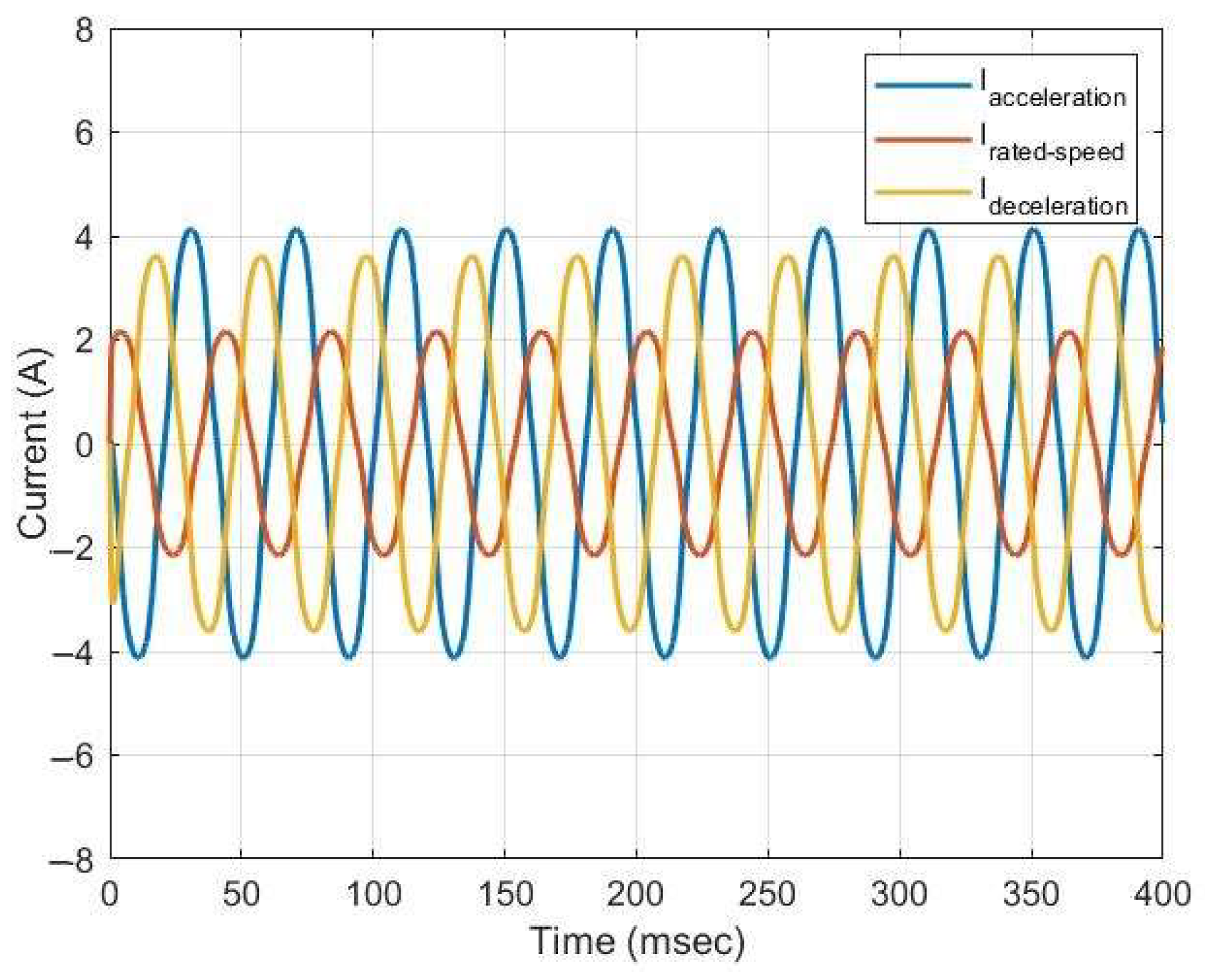
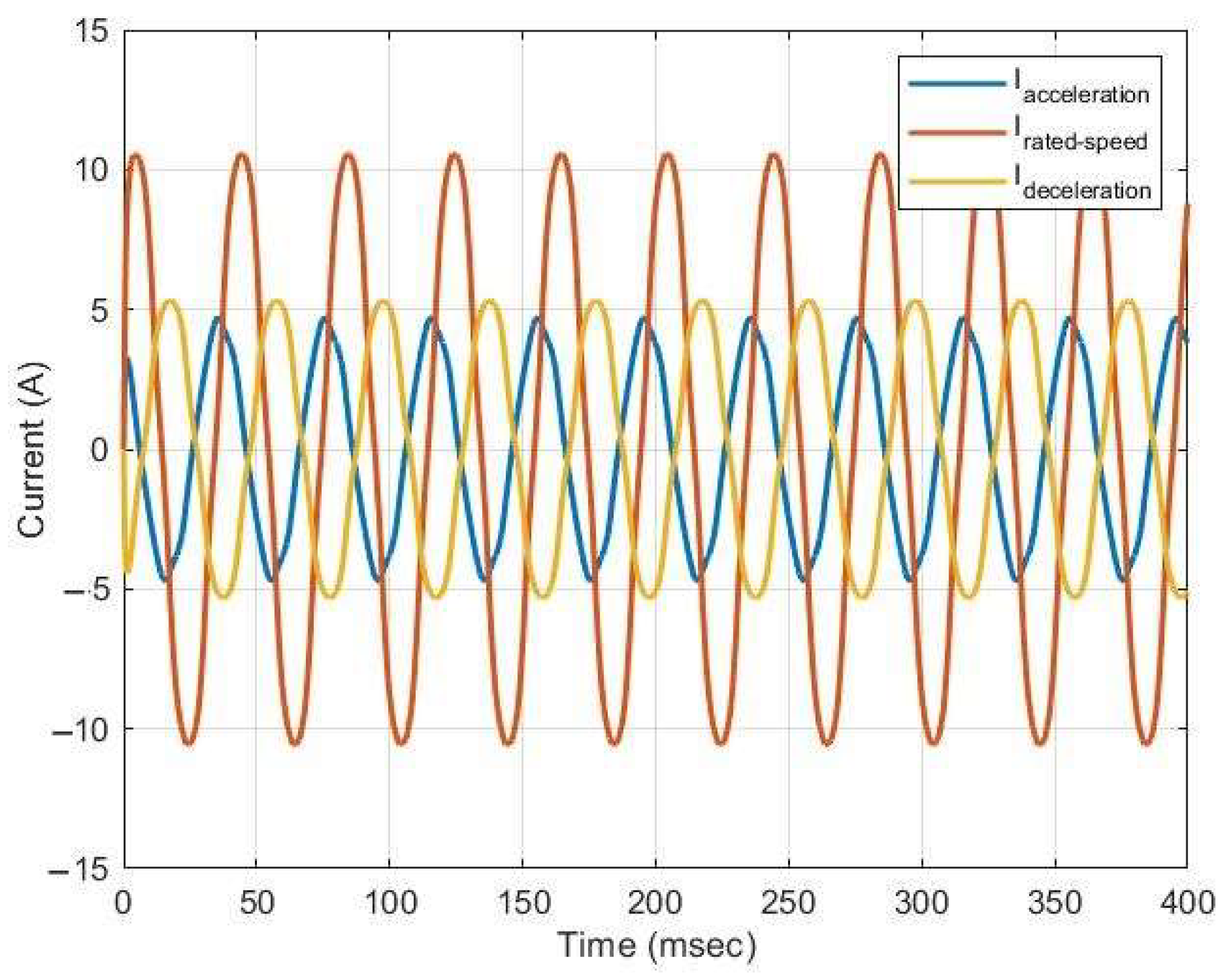
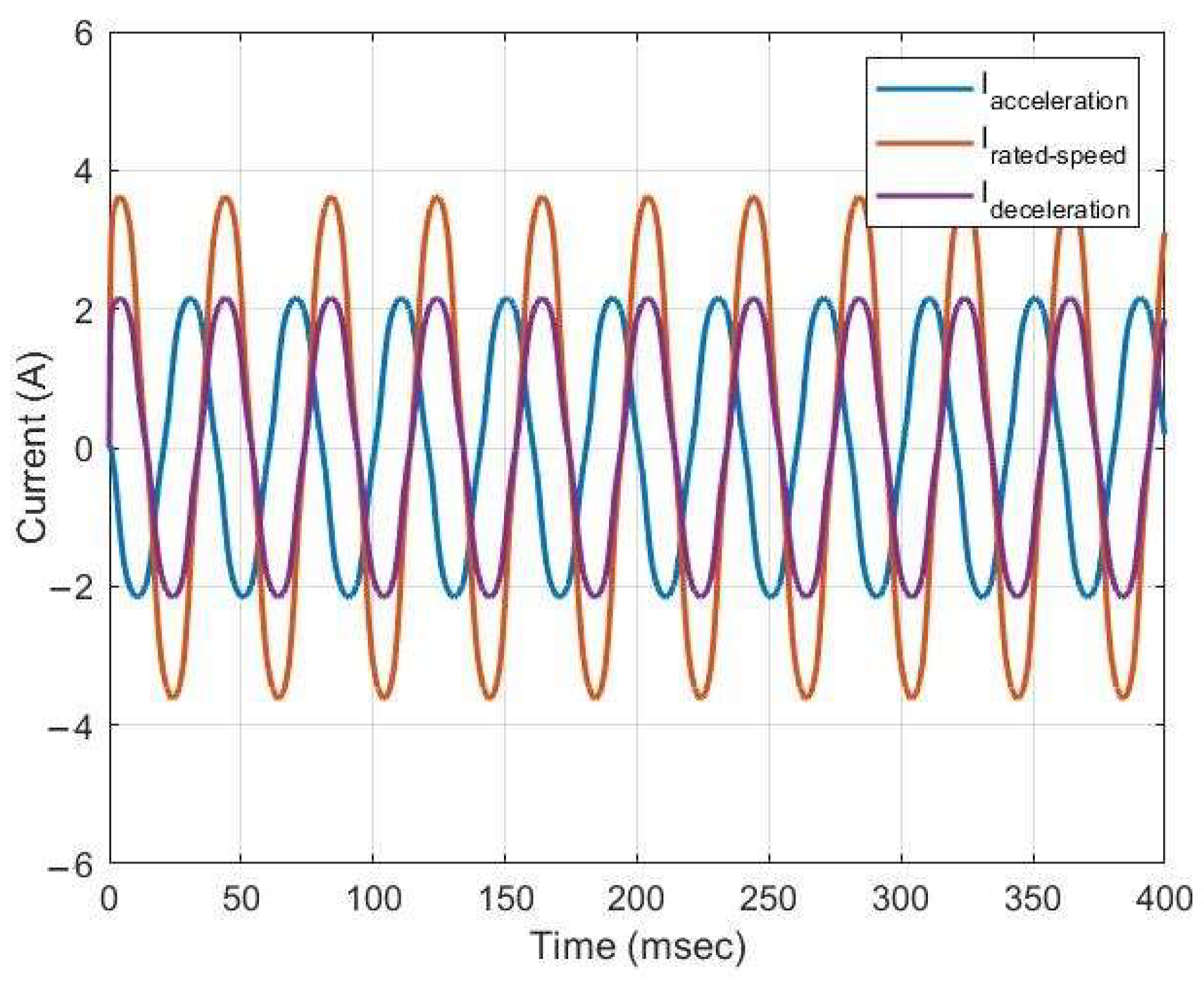






| Variable | Symbol | Value |
|---|---|---|
| Stator outer diameter | 300–350–400 mm | |
| Stator inner diameter | D | 200–240–280 mm |
| Airgap thickness | 0.8–1–1.2 mm | |
| Motor axial length | L | 180–220–260 mm |
| Rotor outer diameter | 200–230–250 mm | |
| Stator slot opening width | 0.2–0.7–1 mm | |
| Stator slot base width | 7–7.46–7.5 mm | |
| Stator slot top width | 3.8–4.2–4.6 mm | |
| Stator slot opening height | 1.5–1.7–1.9 mm | |
| Stator slot base height | 2.2–2.5–2.8 mm | |
| Stator slot top height | 18–18.3–18.5 mm | |
| Stator slot total | Q | 18–36–60 |
| Winding factor | Kw | 0.95 |
| Fill factor | ff | 50% |
| Magnet thickness | 4–4.5–4.8 mm |
| Parameters | Symbol | Values |
|---|---|---|
| Rated load | Q | 630 kg |
| Weigh Chamber | M | 750 kg |
| Counterweight | G | 1050 kg |
| Rated speed | v | 1 m/s |
| Distance | S | 6.2 m |
| Acceleration of gravity | g | 9.81 m/ |
| Friction pulley radius | 0.13 m | |
| Gear ratio | K | 1:1 |
| Number of stops | 7 | |
| Rotor mass inertia together with friction pulley | J | 0.5 kg |
| Quantity | Symbol | Value-Unit |
|---|---|---|
| Electrical motor | Gearless PMSM | - |
| Output power | 6600 W | |
| Input power | 7500 W | |
| Efficiency | η | 89% |
| Power factor | cosφ | 0.85 |
| Electrical frequency | f | 25 Hz |
| Nominal voltage | 360 V | |
| Terminal resistance | 1.32 Ω | |
| Rotor inductance d-q axis | , | 0.008 H |
| Nominal current | 12.4 A | |
| Maximum current | 19 A | |
| Number of phases | m | 3 |
| Number of poles | p | 20 |
| Nominal torque | 450 Nm | |
| Switching frequency | 3000 Hz | |
| Maximum torque | 650 Nm | |
| DC link voltage | 540.19 V | |
| Rated speed | 150 rpm | |
| Rotor inertia | J | 0.0283 kg |
| Flux linkage | Φ | 0.0742 Wb |
| PI | Type-1 | Type-2 |
|---|---|---|
| 0.9 | 0.8 | |
| 0.08 | 0.15 |
| Parameters | Type-1 PI | Type-2 PI |
|---|---|---|
| Rise time (s) | 2.25 | 2.20 |
| Settling time (s) | 4.7 | - |
| Overshoot (%) | 18.67% | 13.33% |
| PID | Type-1 | Type-2 |
|---|---|---|
| 0.8 | 1.2 | |
| 0.08 | 0.15 | |
| 0.05 | 0.1 |
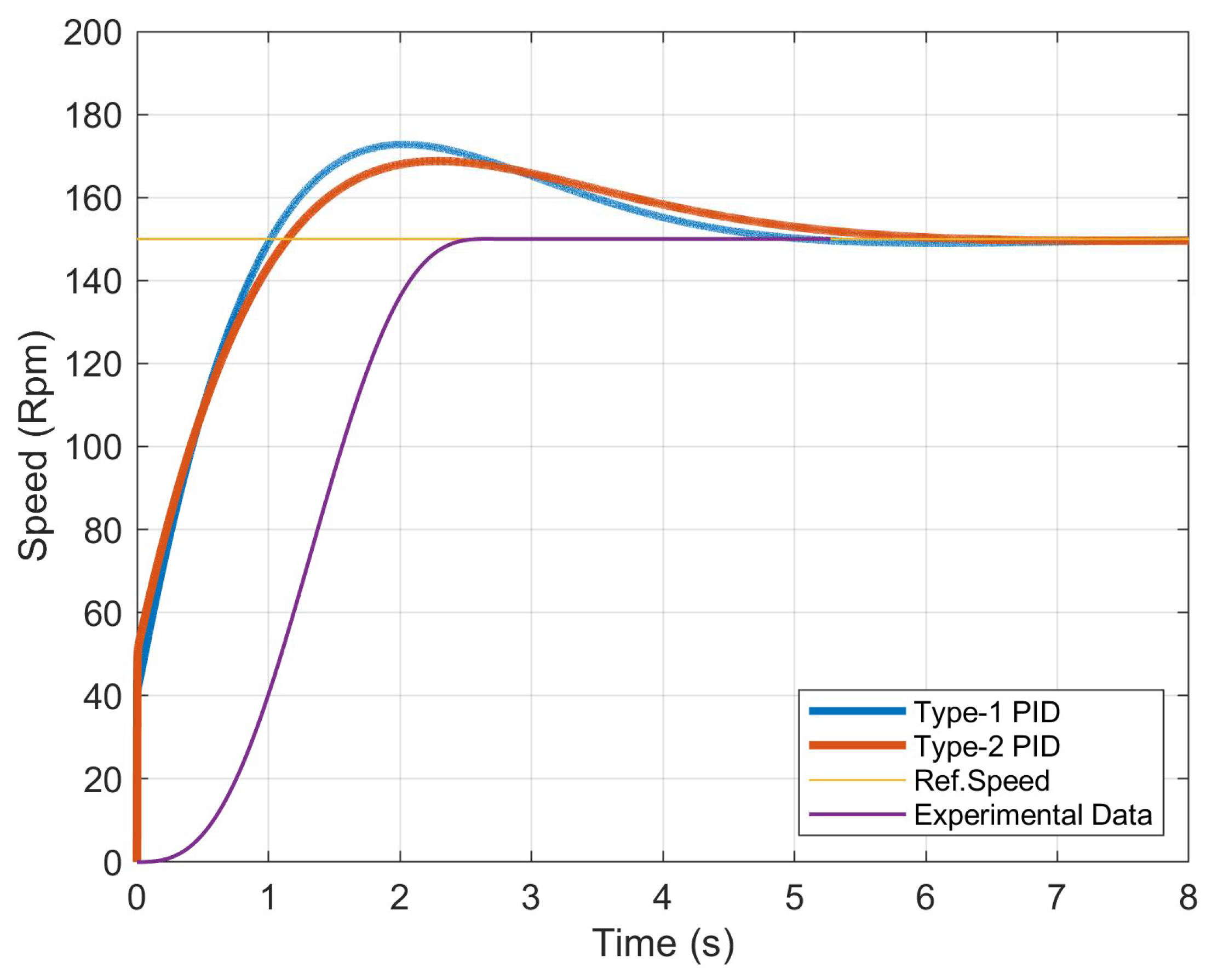
| Parameters | Type-1 PID | Type-2 PID |
|---|---|---|
| Rise time (s) | 0.8 | 1.2 |
| Settling time (s) | 4.7 | - |
| Overshoot (%) | 16.67% | 8.67% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vlachou, V.I.; Efstathiou, D.E.; Karakatsanis, T.S. Design, Analysis and Application of Control Techniques for Driving a Permanent Magnet Synchronous Motor in an Elevator System. Machines 2024, 12, 560. https://doi.org/10.3390/machines12080560
Vlachou VI, Efstathiou DE, Karakatsanis TS. Design, Analysis and Application of Control Techniques for Driving a Permanent Magnet Synchronous Motor in an Elevator System. Machines. 2024; 12(8):560. https://doi.org/10.3390/machines12080560
Chicago/Turabian StyleVlachou, Vasileios I., Dimitrios E. Efstathiou, and Theoklitos S. Karakatsanis. 2024. "Design, Analysis and Application of Control Techniques for Driving a Permanent Magnet Synchronous Motor in an Elevator System" Machines 12, no. 8: 560. https://doi.org/10.3390/machines12080560
APA StyleVlachou, V. I., Efstathiou, D. E., & Karakatsanis, T. S. (2024). Design, Analysis and Application of Control Techniques for Driving a Permanent Magnet Synchronous Motor in an Elevator System. Machines, 12(8), 560. https://doi.org/10.3390/machines12080560








