Time-Delay Estimation Improves Active Disturbance Rejection Control for Time-Delay Nonlinear Systems
Abstract
1. Introduction
1.1. Related Work
1.2. Paper Contribution
2. Mathematical Preliminaries and System Description
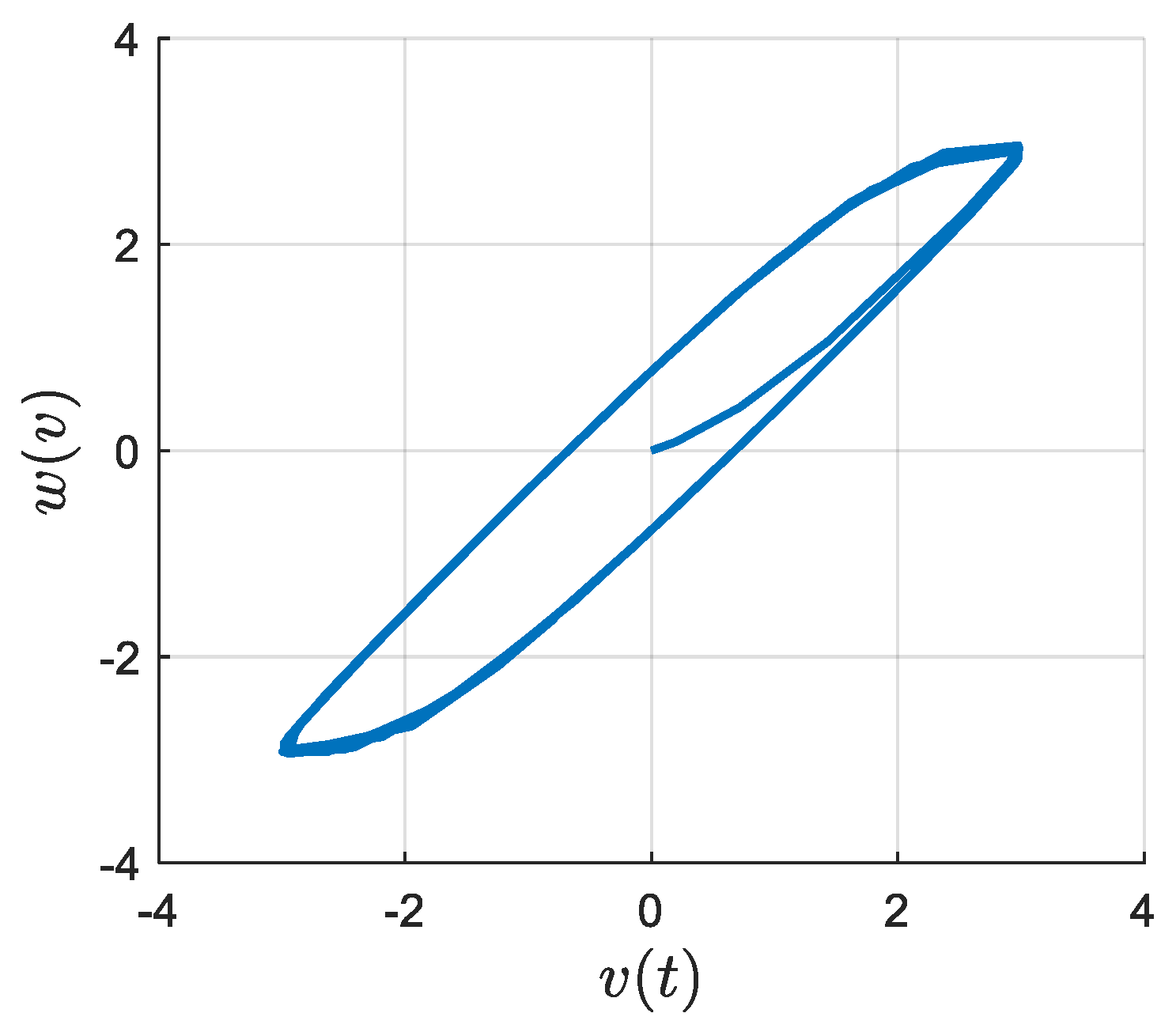
2.1. Duhem Backlash-Like Hysteresis Mathematical Model
2.2. System Description
2.3. Time-Delay Estimation Mechanism
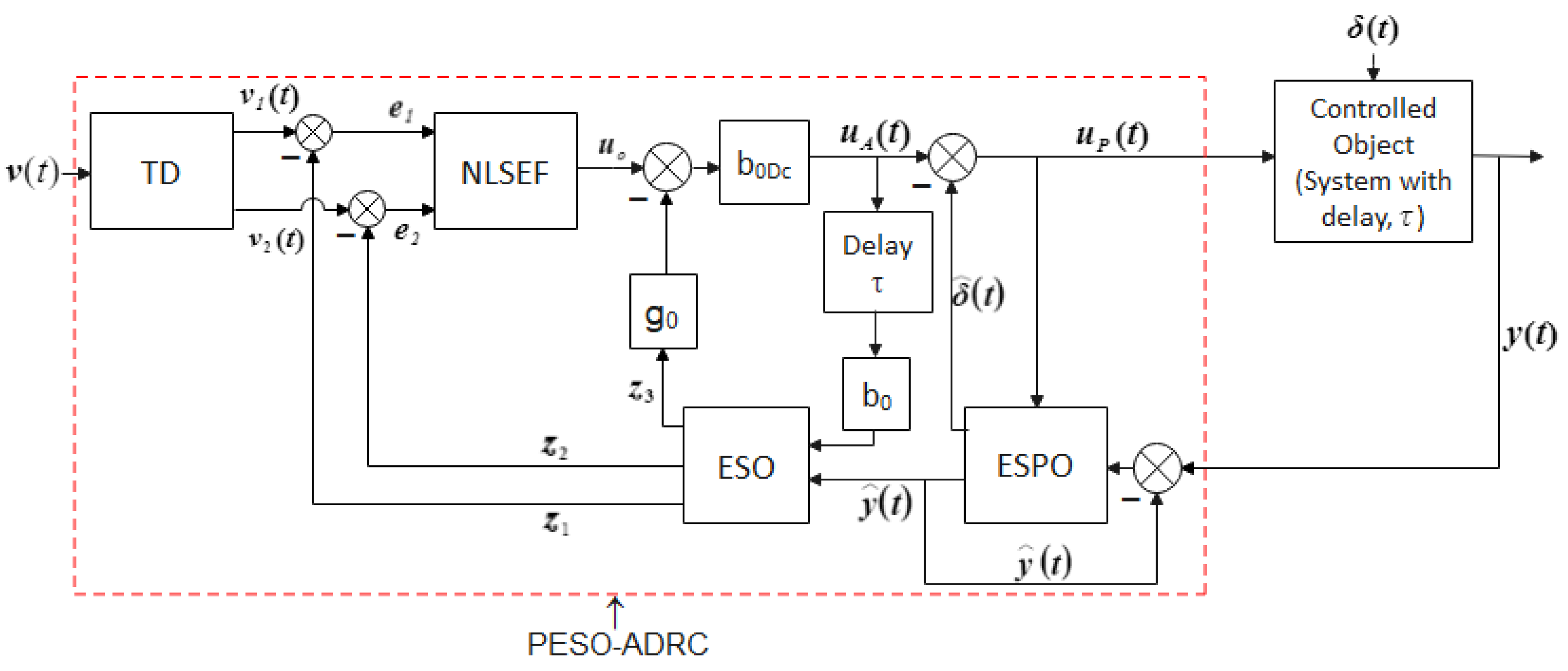
2.4. ADRC with Delayed Input System
3. Modified ADRC Structures Using the TDE Mechanism
3.1. The TDE Mechanism
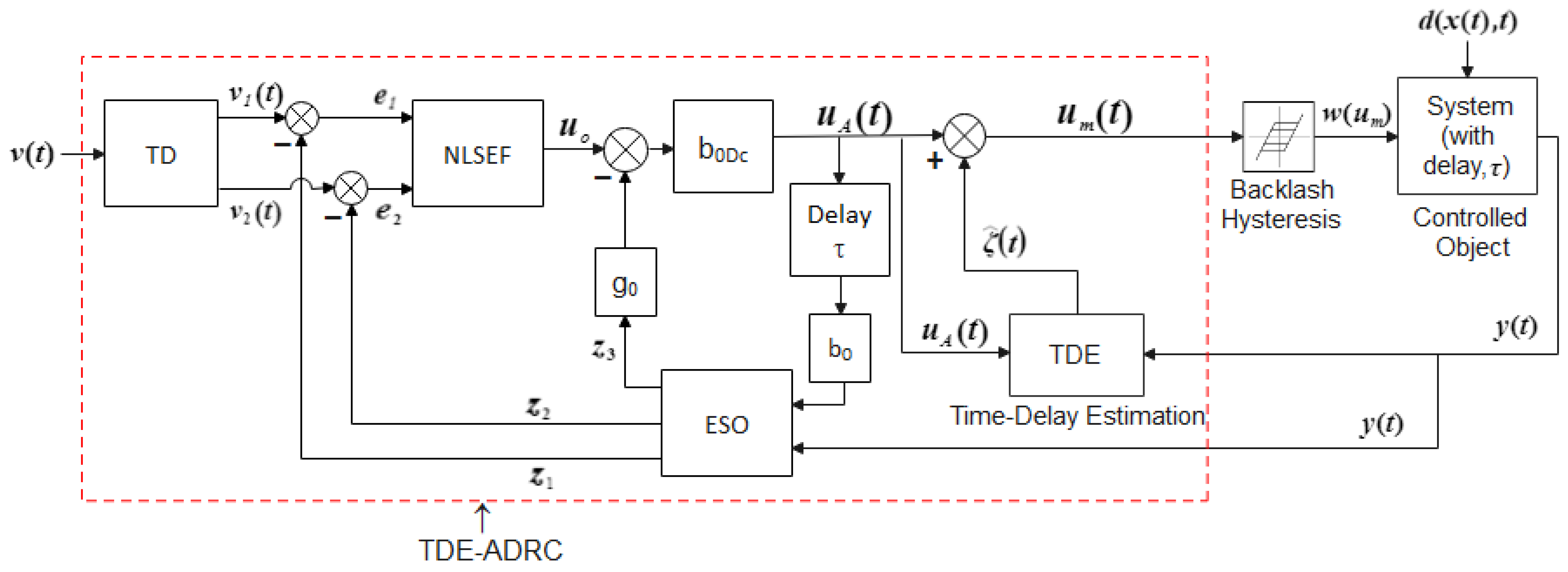
3.2. Integrate TDE in ADRC with Delayed Input
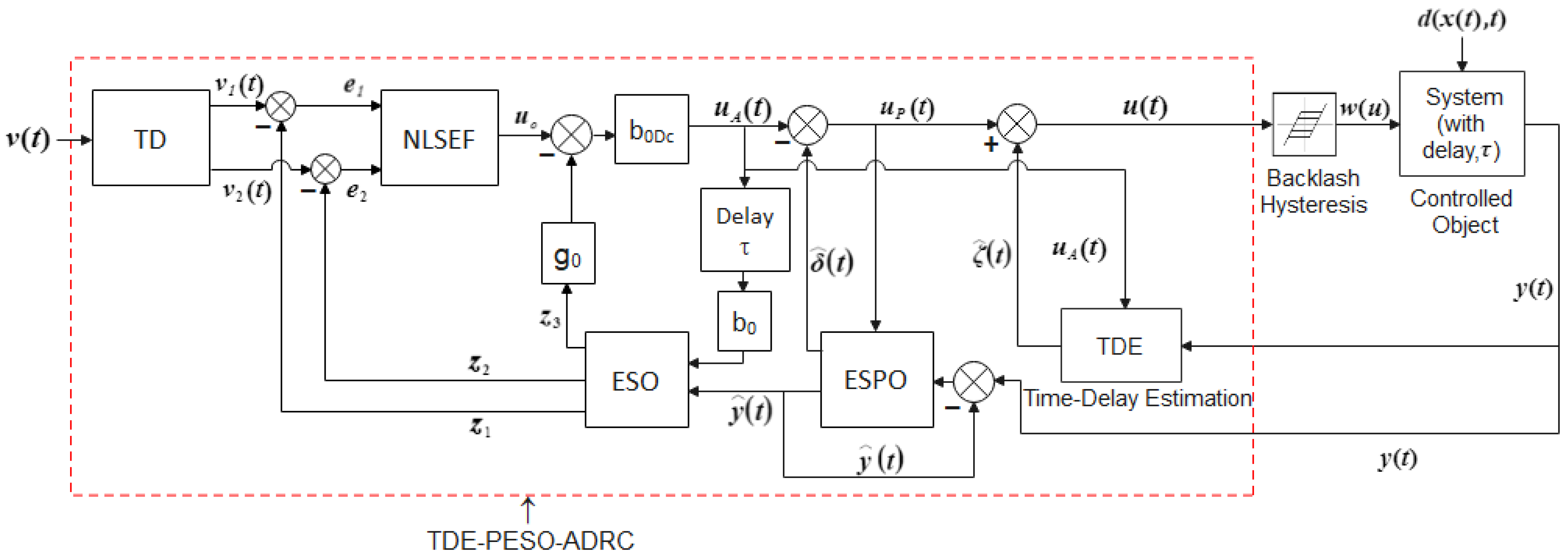
3.3. Integrate TDE into PESO-ADRC
4. Experimental Results, Analysis, and Discussions
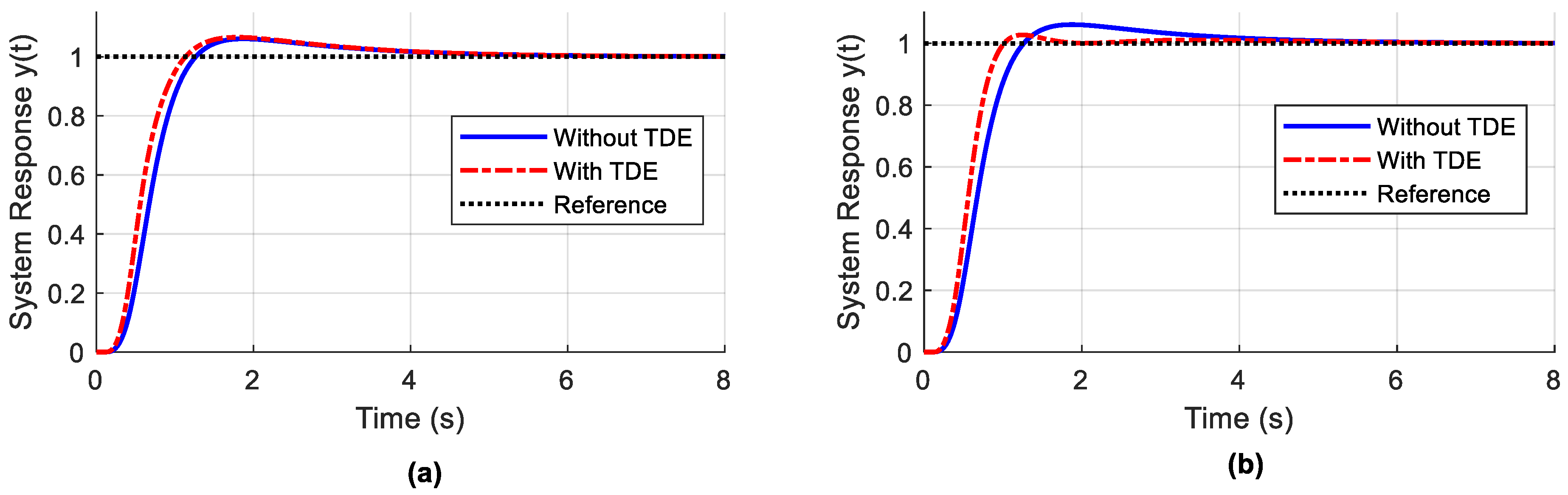
4.1. Effect of TDE on ADRC Methods without Backlash-Like Hysteresis or Disturbances
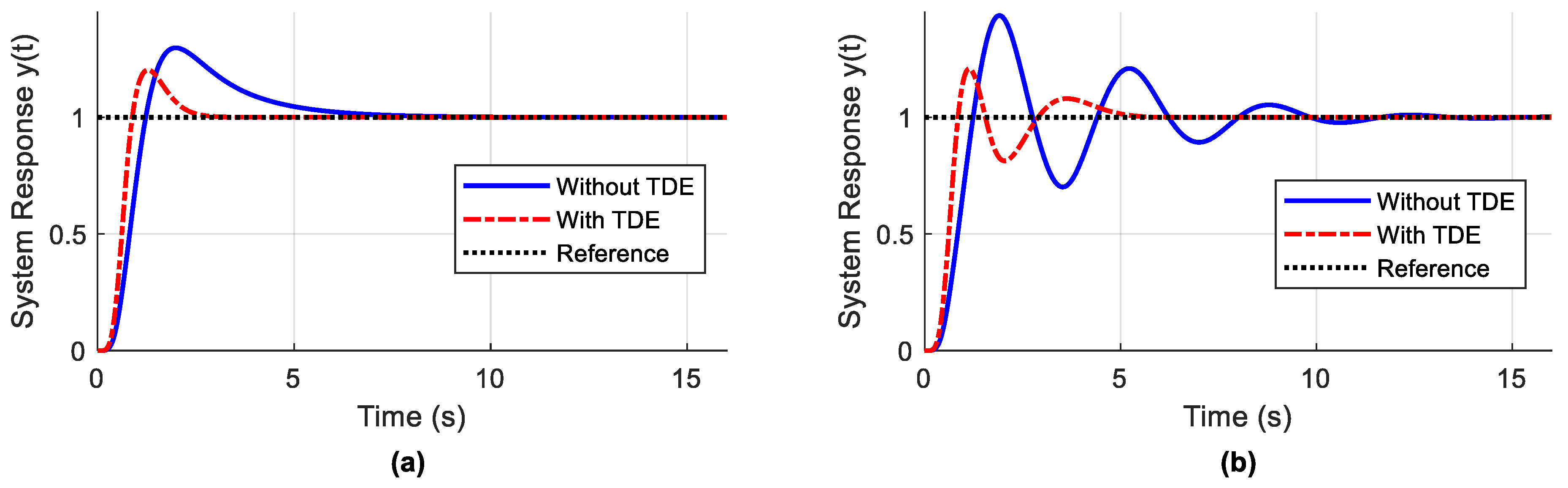
4.2. Effect of TDE on ADRC Methods under Hysteresis
4.3. Effect of TDE on ADRC Methods under Both Hysteresis and Parameter Perturbation
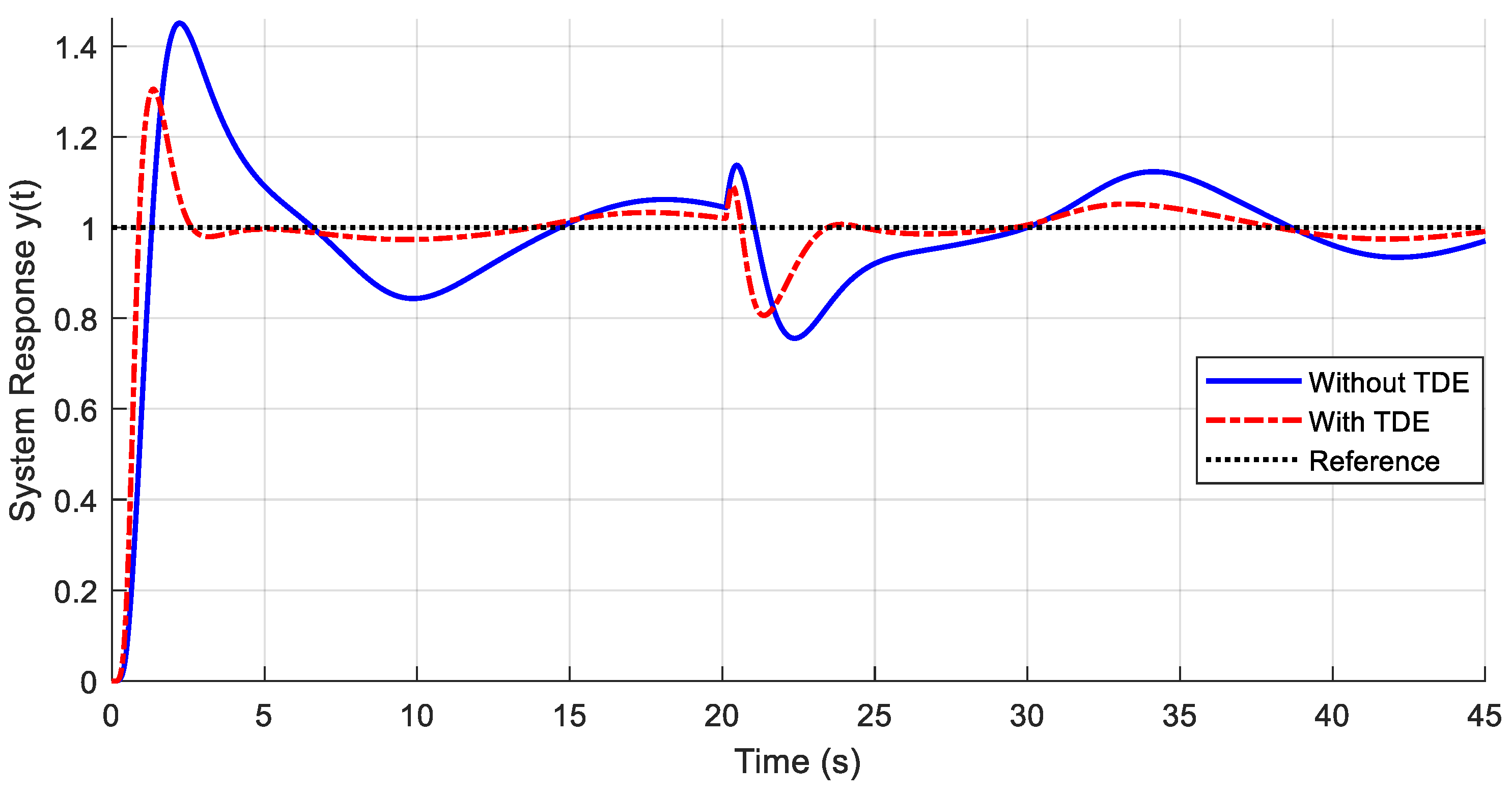
4.4. Effect of TDE on ADRC Methods under Hysteresis, Parameter Perturbation, and External Disturbance
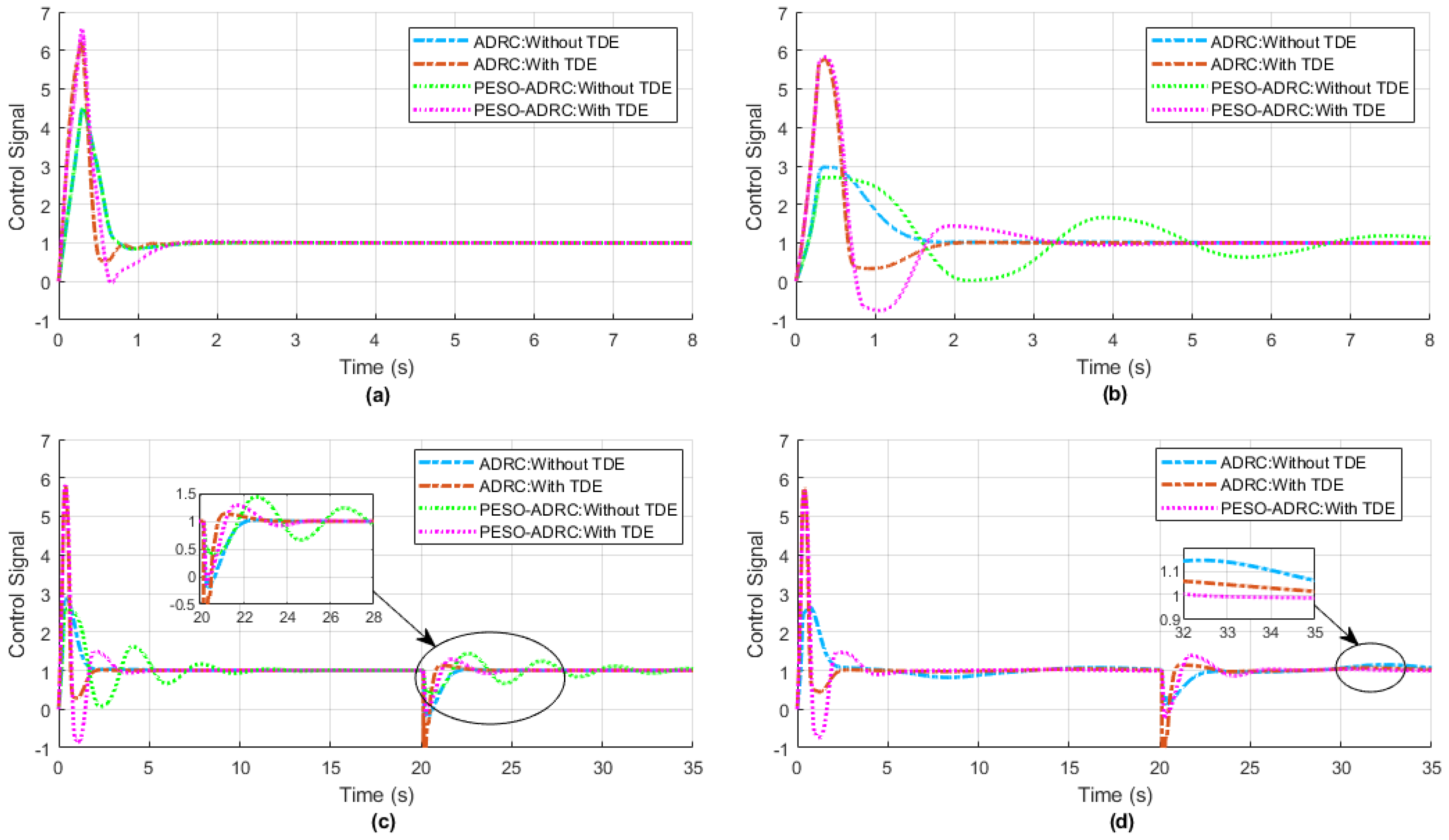
4.5. Control Signal for Different Scenarios
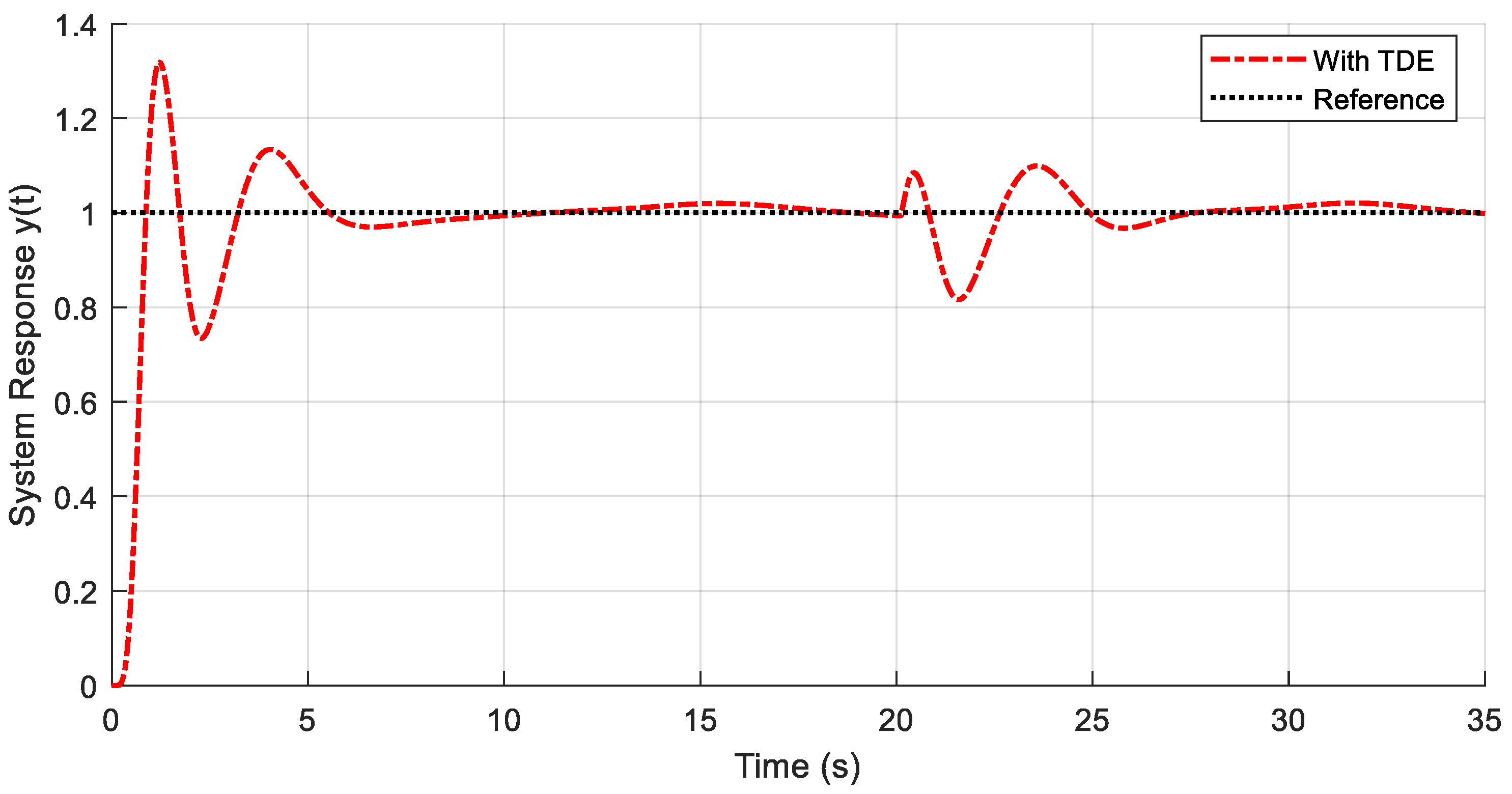
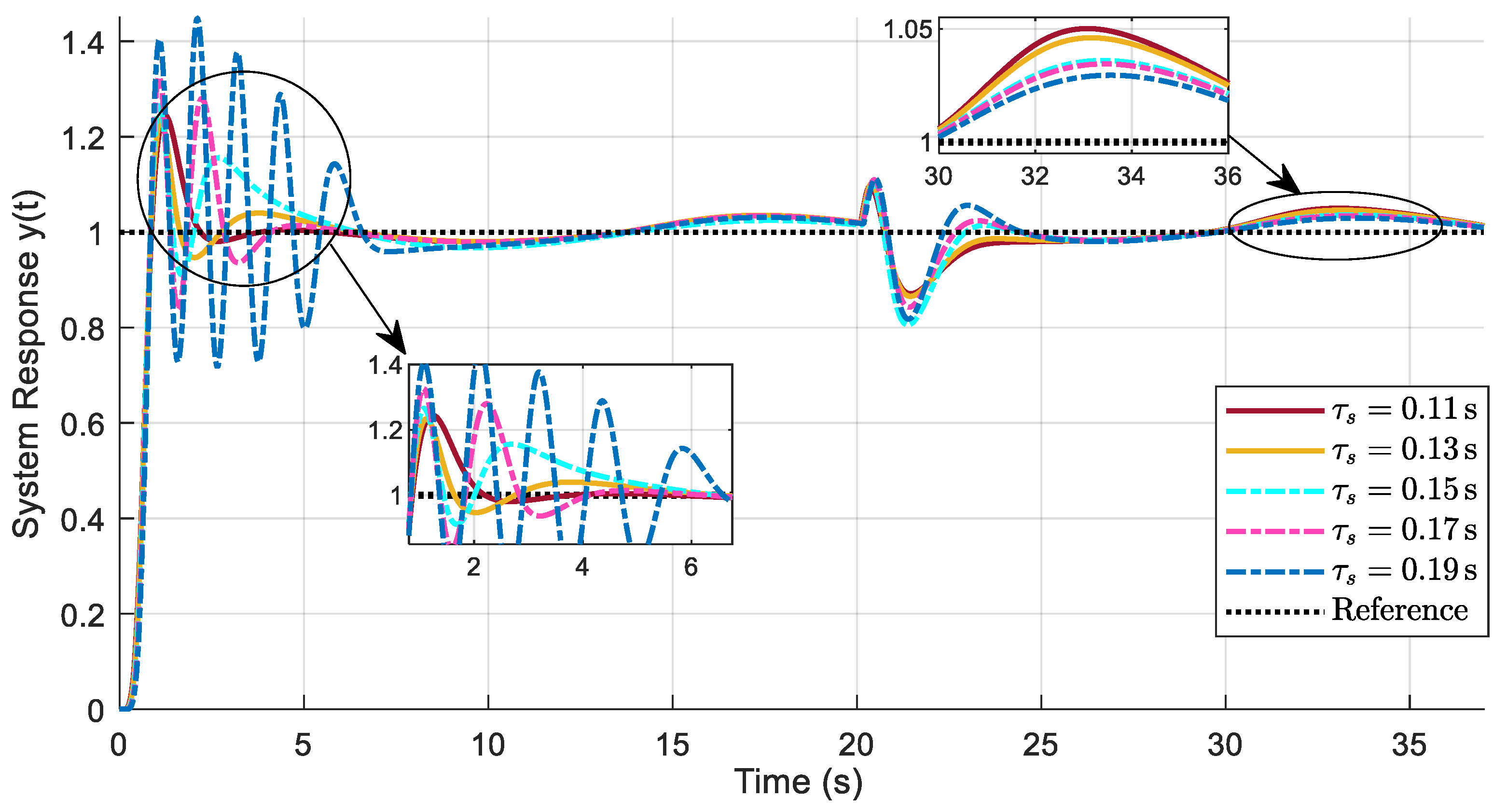
4.6. Effect of Change in System Time Delay on the Proposed TDE-ADRC Methods
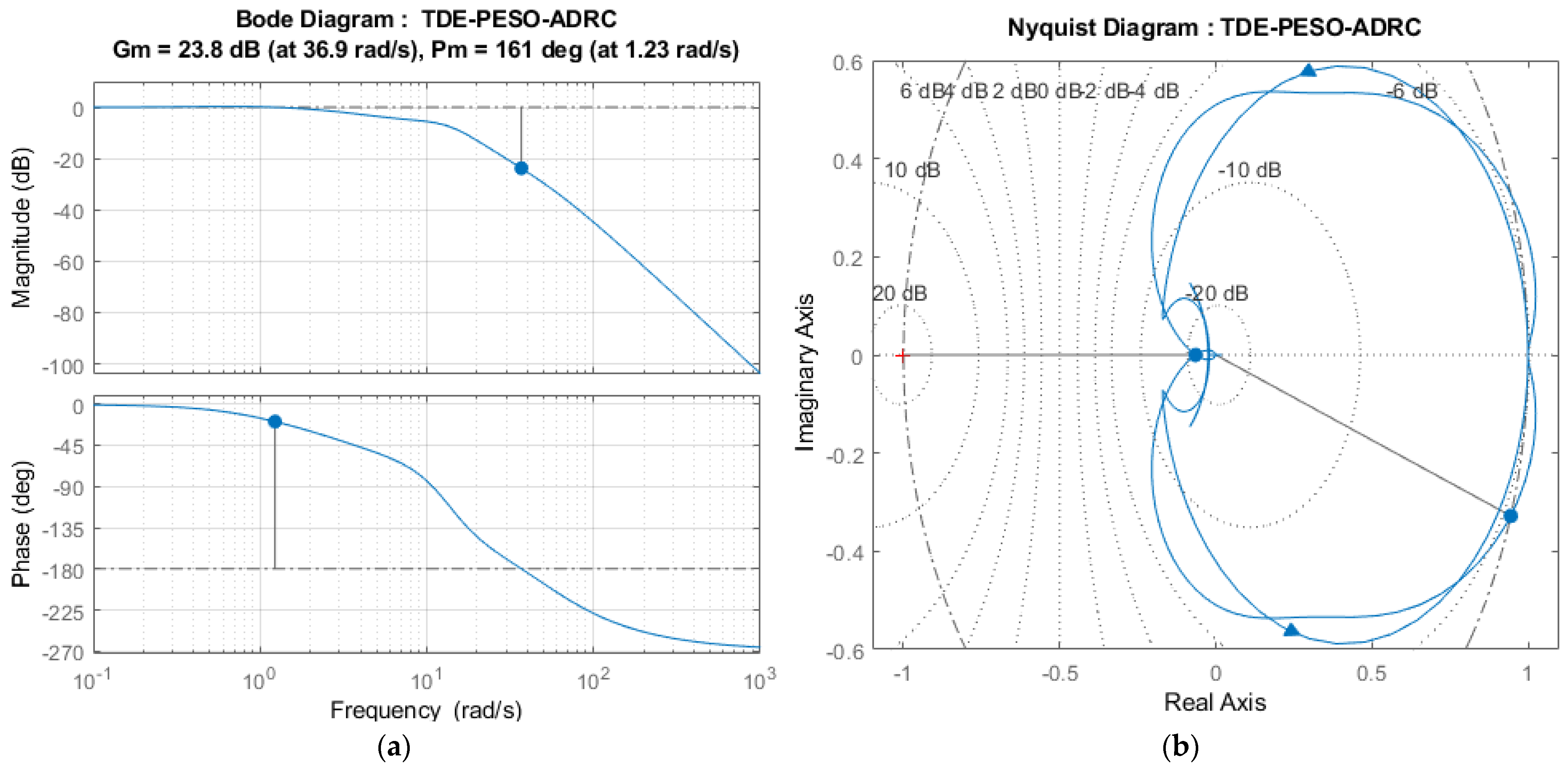
4.7. Stability Analysis of the Proposed TDE-ADRC Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Han, J. From PID to Active Disturbance Rejection Control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Han, J.Q. Auto Disturbance Rejection Controller and It’s Applications. Control Decis. 1998, 13, 19–23. [Google Scholar]
- Nahri, S.N.F.; Du, S.; van Wyk, B.J.; Nyasulu, T.D. Mitigating the Time Delay and Parameter Perturbation by a Predictive Extended State Observer-based Active Disturbance Rejection Control. In Proceedings of the Towards Autonomous Robotic Systems: 25th Annual Conference, TAROS 2024, Springer Lecture Notes in Artificial Intelligence (LNAI) Series, London, UK, 21–23 August 2024. in press. [Google Scholar]
- Shamsuzzoha, M.; Raja, G.L. Disturbance Rejection Control; IntechOpen: London, UK, 2023. [Google Scholar] [CrossRef]
- Xu, L.; Zhuo, S.; Liu, J.; Jin, S.; Huangfu, Y.; Gao, F. Advancement of active disturbance rejection control and its applications in power electronics. IEEE Trans. Ind. Appl. 2023, 60, 1680–1694. [Google Scholar] [CrossRef]
- Zheng, Q.; Gao, Z. Active disturbance rejection control: Some recent experimental and industrial case studies. Control Theory Technol. 2018, 16, 301–313. [Google Scholar] [CrossRef]
- Fareh, R.; Khadraoui, S.; Abdallah, M.Y.; Baziyad, M.; Bettayeb, M. Active disturbance rejection control for robotic systems: A review. Mechatronics 2021, 80, 102671. [Google Scholar] [CrossRef]
- Wu, Z.; Gao, Z.; Li, D.; Chen, Y.; Liu, Y. On transitioning from PID to ADRC in thermal power plants. Control Theory Technol. 2021, 19, 3–18. [Google Scholar]
- Hezzi, A.; Elbouchikhi, E.; Bouzid, A.; Ben Elghali, S.; Zerrougui, M.; Benbouzid, M. Active Disturbance Rejection Control for Distributed Energy Resources in Microgrids. Machines 2024, 12, 67. [Google Scholar] [CrossRef]
- Nahri, S.N.F.; Du, S.; van Wyk, B.J. Active Disturbance Rejection Control Design for a Haptic Machine Interface Platform. Adv. Sci. Technol. Eng. Syst. J. 2021, 6, 898–911. [Google Scholar]
- Nahri, S.N.F.; Du, S.; Van Wyk, B.J. A Review on Haptic Bilateral Teleoperation Systems. J. Intell. Robot. Syst. 2022, 104, 13. [Google Scholar] [CrossRef]
- Goforth, F.J.; Gao, Z. An active disturbance rejection control solution for hysteresis compensation. In Proceedings of the 2008 American Control Conference, Seattle, WA, USA, 11–13 June 2008; pp. 2202–2208. [Google Scholar]
- Liu, W.; Cheng, L.; Hou, Z.-G.; Tan, M. An active disturbance rejection controller with hysteresis compensation for piezoelectric actuators. In Proceedings of the 2016 12th World Congress on Intelligent Control and Automation (WCICA), Guilin, China, 12–15 June 2016; pp. 2148–2153. [Google Scholar]
- Liu, W.; Zhao, T. An active disturbance rejection control for hysteresis compensation based on neural networks adaptive control. ISA Trans. 2021, 109, 81–88. [Google Scholar] [CrossRef]
- Liu, W.; Zhao, T.; Wu, Z.; Huang, W. Linear active disturbance rejection control for hysteresis compensation based on backpropagation neural networks adaptive control. Trans. Inst. Meas. Control 2021, 43, 915–924. [Google Scholar] [CrossRef]
- Du, S.; van Wyk, B.; Nahri, N.; Tu, C. Auto Disturbance Rejection Control Based on A Neural Network Predictive State Observer. In Proceedings of the 2023 15th International Conference on Intelligent Human-Machine Systems and Cybernetics (IHMSC), Hangzhou, China, 26–27 August 2023; pp. 233–236. [Google Scholar]
- Chen, S.; Xue, W.; Zhong, S.; Huang, Y. On comparison of modified ADRCs for nonlinear uncertain systems with time delay. Sci. China Inf. Sci. 2018, 61, 70223. [Google Scholar] [CrossRef]
- Ran, M.; Wang, Q.; Dong, C.; Xie, L. Active disturbance rejection control for uncertain time-delay nonlinear systems. Automatica 2020, 112, 108692. [Google Scholar] [CrossRef]
- Xue, W.; Liu, P.; Chen, S.; Huang, Y. On extended state predictor observer based active disturbance rejection control for uncertain systems with sensor delay. In Proceedings of the 2016 16th International Conference on Control, Automation and Systems (ICCAS), Gyeongju, Republic of Korea, 16–19 October 2016; pp. 1267–1271. [Google Scholar]
- Tan, W.; Fu, C. Analysis of active disturbance rejection control for processes with time delay. In Proceedings of the 2015 American control conference (ACC), Chicago, IL, USA, 1–3 July 2015; pp. 3962–3967. [Google Scholar]
- Zhao, S.; Gao, Z. Modified active disturbance rejection control for time-delay systems. ISA Trans. 2014, 53, 882–888. [Google Scholar] [CrossRef]
- Fu, C.; Tan, W. Linear active disturbance rejection control for processes with time delays: IMC interpretation. IEEE Access 2020, 8, 16606–16617. [Google Scholar] [CrossRef]
- Nahri, S.N.F.; Du, S.; van Wyk, B.J. Predictive Extended State Observer-Based Active Disturbance Rejection Control for Systems with Time Delay. Machines 2023, 11, 144. [Google Scholar] [CrossRef]
- Youcef-Toumi, K.; Ito, O. A time delay controller for systems with unknown dynamics. In Proceedings of the 1988 American Control Conference, Atlanta, GA, USA, 15–17 June 1988. [Google Scholar] [CrossRef]
- Hsia, T.S.; Lasky, T.A.; Guo, Z. Robust independent joint controller design for industrial robot manipulators. IEEE Trans. Ind. Electron. 1991, 38, 21–25. [Google Scholar] [CrossRef]
- Lee, S.-U.; Chang, P.H. Control of a heavy-duty robotic excavator using time delay control with integral sliding surface. Control Eng. Pract. 2002, 10, 697–711. [Google Scholar] [CrossRef]
- Kim, J.; Chang, P.-H.; Jin, M. Fuzzy PID controller design using time-delay estimation. Trans. Inst. Meas. Control 2017, 39, 1329–1338. [Google Scholar] [CrossRef]
- Lee, J.; Park, S.H.; Chang, P.H.; Suh, J.; Seo, K.-H.; Jin, M. Improved adaptive PID control using time-delay estimation for robot manipulators. In Proceedings of the 2019 16th International Conference on Ubiquitous Robots (UR), Jeju, Republic of Korea, 24–27 June 2019; pp. 87–91. [Google Scholar]
- Ahmed, S.; Wang, H.; Tian, Y. Adaptive high-order terminal sliding mode control based on time delay estimation for the robotic manipulators with backlash hysteresis. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 1128–1137. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, H.; Deng, W. A New Practical Robust Adaptive Control of Cable-driven Robots. Int. J. Control Autom. Syst. 2023, 21, 2685–2697. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, K.; Yan, F.; Chen, B. Adaptive super-twisting nonsingular fast terminal sliding mode control for cable-driven manipulators using time-delay estimation. Adv. Eng. Softw. 2019, 128, 113–124. [Google Scholar] [CrossRef]
- Li, J.; Zhang, L.; Li, S.; Su, J. A time delay estimation interpretation of extended state observer-based controller with application to structural vibration suppression. IEEE Trans. Autom. Sci. Eng. 2023, 21, 1965–1973. [Google Scholar] [CrossRef]
- Feng, Y.; Rabbath, C.A.; Chai, T.; Su, C.-Y. Robust adaptive control of systems with hysteretic nonlinearities: A Duhem hysteresis modelling approach. In Proceedings of the AFRICON 2009, Nairobi, Kenya, 23–25 September 2009; pp. 1–6. [Google Scholar]
- Ikhouane, F. A survey of the hysteretic Duhem model. Arch. Comput. Methods Eng. 2018, 25, 965–1002. [Google Scholar] [CrossRef]






| Method | Performance Criteria | Without TDE | With TDE | (%) |
|---|---|---|---|---|
| ADRC with delayed input | ITAE | 0.5916 | 0.5480 | 7.3700 |
| Rise time (s) | 0.6492 | 0.5806 | 10.5669 | |
PESO-ADRC | ITAE | 0.5916 | 0.2771 | 53.1609 |
| Rise time (s) | 0.6492 | 0.4946 | 55.9300 | |
| Settling time (s) | 3.7300 | 1.4223 | 61.8686 | |
| Overshoot (%) | 5.9532 | 2.6234 | 55.9329 |
| Method | Performance Criteria | Without TDE | With TDE | (%) |
|---|---|---|---|---|
ADRC with delayed input | ITAE | 2.3790 | 0.4152 | 82.5473 |
| Rise time (s) | 0.6525 | 0.4208 | 31.7886 | |
| Settling time (s) | 6.2432 | 2.4114 | 61.3756 | |
| Overshoot (%) | 29.7770 | 20.3113 | 31.7886 | |
PESO-ADRC | ITAE | 5.3570 | 1.0130 | 81.0901 |
| Rise time (s) | 0.6715 | 0.4032 | 52.0444 | |
| Settling time (s) | 10.9744 | 4.8639 | 55.6796 | |
| Overshoot (%) | 43.3960 | 20.8108 | 52.0444 |
| Method | Performance Criteria | Without TDE | With TDE | (%) |
|---|---|---|---|---|
ADRC with delayed input | ITAE | 19.9800 | 5.7420 | 71.2613 |
| Rise time (s) | 0.6725 | 0.4132 | 24.9301 | |
| Settling time (s) | 25.8333 | 22.9382 | 11.2069 | |
| Overshoot (%) [from 0 to 3 s] | 30.3688 | 22.7978 | 24.9302 | |
| Overshoot (%) [from 20 to 22 s] | 8.5700 | 7.2900 | 14.9358 | |
PESO-ADRC | ITAE | 27.9900 | 6.7860 | 75.7556 |
| Rise time (s) | 0.6931 | 0.4010 | 46.1113 | |
| Settling time (s) | 34.4842 | 24.3004 | 29.5318 | |
| Overshoot (%) [from 0 to 3 s] | 44.2609 | 23.8516 | 46.1114 | |
| Overshoot (%) [from 20 to 22 s] | 10.7500 | 9.4600 | 12.0000 |
| Method | Performance Criteria | Without TDE | With TDE | (%) |
|---|---|---|---|---|
| ADRC with delayed input | ITAE | 70.6600 | 26.8400 | 62.0153 |
| Rise time (s) | 0.6732 | 0.4047 | 39.8841 | |
| Overshoot (%) [from 0 to 3 s] | 49.5175 | 31.6211 | 36.0000 | |
| Overshoot (%) [from 20 to 22 s] | 13.5500 | 9.1000 | 32.8413 | |
PESO-ADRC | ITAE | Unstable | 15.9200 | - |
| Rise time (s) | Unstable | 0.4017 | - | |
| Overshoot (%) [from 0 to 3 s] | Unstable | 32.0155 | - | |
| Overshoot (%) [from 20 to 22 s] | Unstable | 8.5000 | - |
| TDE-ADRC | Rise Time (s) | Response Time (s) | Overshoot (%) | ITAE |
|---|---|---|---|---|
| = 0.11 s | 0.4037 | 35.3057 | 22.8240 | 20.1500 |
| = 0.13 s | 0.3769 | 35.2035 | 21.7502 | 19.3200 |
| = 0.15 s | 0.3552 | 34.7406 | 25.1789 | 18.6900 |
| = 0.17 s | 0.3392 | 34.6135 | 31.1730 | 17.3100 |
| = 0.19 s | 0.3270 | 28.6165 | 43.4998 | 20.5000 |
| TDE-PESO-ADRC | Rise Time (s) | Response Time (s) | Overshoot (%) | ITAE |
|---|---|---|---|---|
| = 0.11 s | 0.3893 | 33.1176 | 31.4353 | 15.0600 |
| = 0.13 s | 0.3728 | 33.0609 | 36.6789 | 15.6000 |
| = 0.15 s | 0.3605 | 32.9865 | 43.7253 | 18.2300 |
| = 0.17 s | 0.3516 | 32.8235 | 58.4140 | 33.1700 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nahri, S.N.F.; Du, S.; van Wyk, B.J.; Nyasulu, T.D. Time-Delay Estimation Improves Active Disturbance Rejection Control for Time-Delay Nonlinear Systems. Machines 2024, 12, 552. https://doi.org/10.3390/machines12080552
Nahri SNF, Du S, van Wyk BJ, Nyasulu TD. Time-Delay Estimation Improves Active Disturbance Rejection Control for Time-Delay Nonlinear Systems. Machines. 2024; 12(8):552. https://doi.org/10.3390/machines12080552
Chicago/Turabian StyleNahri, Syeda Nadiah Fatima, Shengzhi Du, Barend J. van Wyk, and Tawanda Denzel Nyasulu. 2024. "Time-Delay Estimation Improves Active Disturbance Rejection Control for Time-Delay Nonlinear Systems" Machines 12, no. 8: 552. https://doi.org/10.3390/machines12080552
APA StyleNahri, S. N. F., Du, S., van Wyk, B. J., & Nyasulu, T. D. (2024). Time-Delay Estimation Improves Active Disturbance Rejection Control for Time-Delay Nonlinear Systems. Machines, 12(8), 552. https://doi.org/10.3390/machines12080552







